A Mokken Scale Analysis of the Last Series of the Standard Progressive Matrices (SPM-LS)
Abstract
1. Introduction
1.1. The SPM-LS
1.2. Mokken Scale Analysis
1.3. The Benefits of Mokken Scale Analysis
1.3.1. The Monotone Homogeneity and Double Monotonicity Models
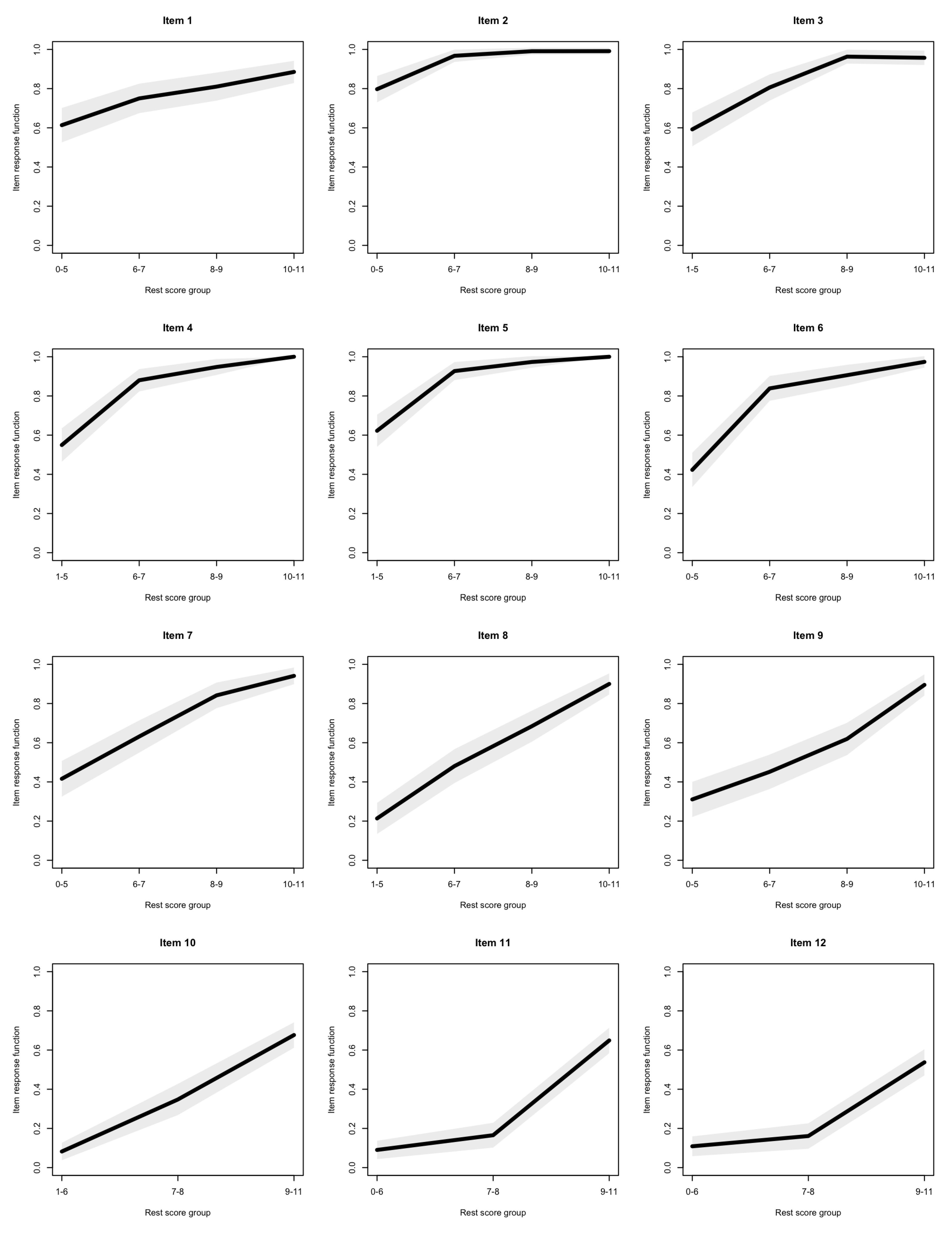
1.3.2. Monotonicity of Item Response Functions
1.3.3. Invariant Item Ordering
2. Materials and Methods
2.1. Participants
2.2. Instrument
2.3. Analysis
2.3.1. Data Preparation
2.3.2. Scalability
2.3.3. Local Independence
2.3.4. Monotonicity
2.3.5. Invariant Item Ordering
2.3.6. Reliability
3. Results
3.1. Scalability
3.2. Local Independence
3.3. Monotonicity
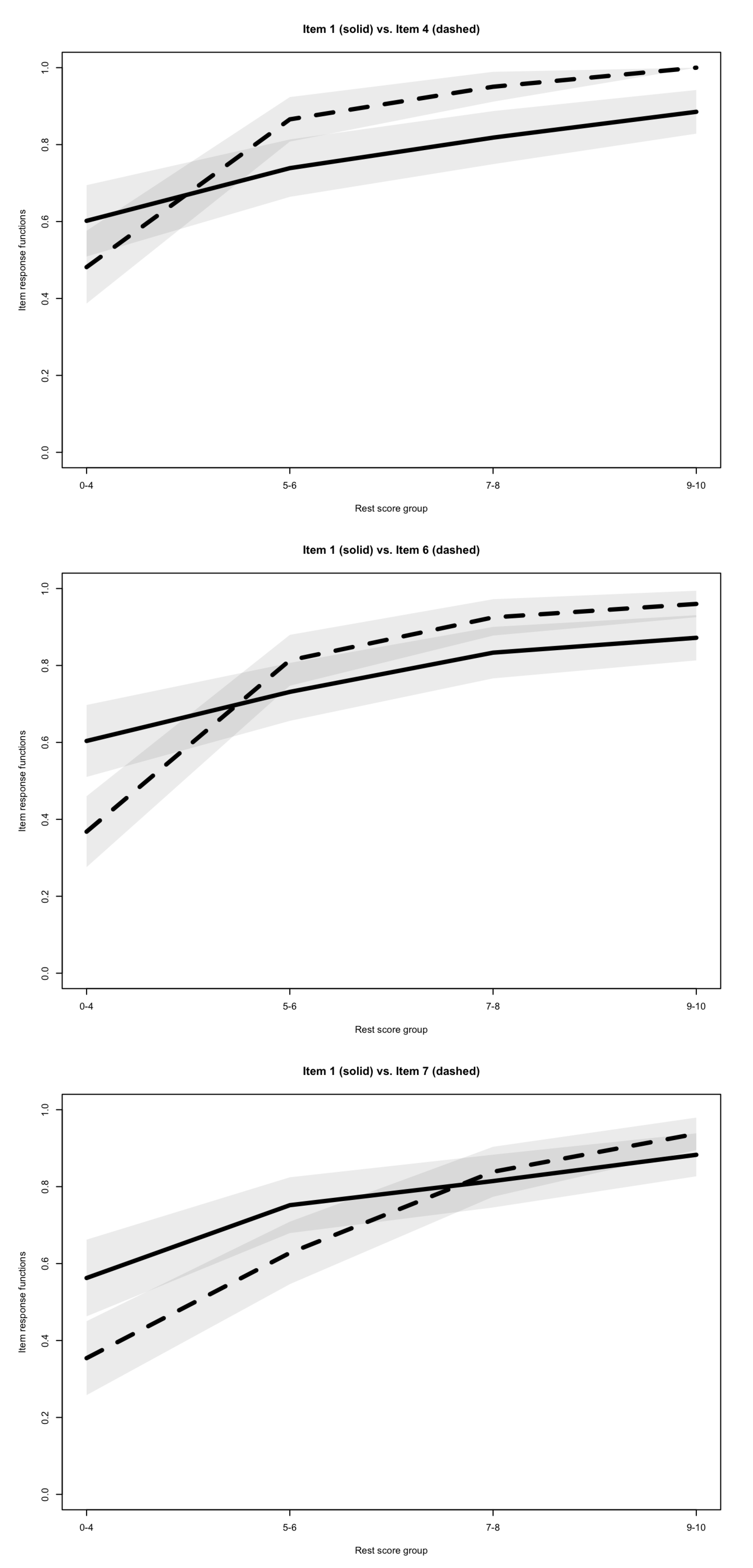
3.4. Invariant Item Ordering
3.5. Reliability
4. Discussion
4.1. Conclusions on the SPM-LS
4.2. Limitations
4.3. Future Directions
Funding
Conflicts of Interest
References
- Bock, R. Darrell. 1997. The Nominal Categories Model. In Handbook of Modern Item Response Theory. Edited by Wim J. van der Linden and Ronald K. Hambleton. New York: Springer, pp. 33–49. [Google Scholar] [CrossRef]
- Bors, Douglas A., and Tonya L. Stokes. 1998. Raven’s Advanced Progressive Matrices: Norms for First-Year University Students and the Development of a Short Form. Educational and Psychological Measurement 58: 382–98. [Google Scholar] [CrossRef]
- Bürkner, Paul-Christian. 2020. Analysing Standard Progressive Matrices (SPM-LS) with Bayesian Item Response Models. Journal of Intelligence 8: 5. [Google Scholar] [CrossRef] [PubMed]
- Carpenter, Patricia A., Marcel A. Just, and Peter Shell. 1990. What one intelligence test measures: A theoretical account of the processing in the Raven Progressive Matrices Test. Psychological Review 97: 404–31. [Google Scholar] [CrossRef] [PubMed]
- Garcia-Garzon, Eduardo, Francisco J. Abad, and Luis E. Garrido. 2019. Searching for G: A New Evaluation of SPM-LS Dimensionality. Journal of Intelligence 7: 14. [Google Scholar] [CrossRef] [PubMed]
- Gignac, Gilles E. 2015. Raven’s is not a pure measure of general intelligence: Implications for g factor theory and the brief measurement of g. Intelligence 52: 71–79. [Google Scholar] [CrossRef]
- Hamel, Ronald, and Verena D. Schmittmann. 2006. The 20-Minute Version as a Predictor of the Raven Advanced Progressive Matrices Test. Educational and Psychological Measurement 66: 1039–46. [Google Scholar] [CrossRef]
- Junker, Brian W., and Klaas Sijtsma. 2000. Latent and Manifest Monotonicity in Item Response Models. Applied Psychological Measurement 24: 65–81. [Google Scholar] [CrossRef]
- Ligtvoet, Rudy, L. Andries Van der Ark, Janneke M. Te Marvelde, and Klaas Sijtsma. 2010. Investigating an Invariant Item Ordering for Polytomously Scored Items. Educational and Psychological Measurement 70: 578–95. [Google Scholar] [CrossRef]
- Meijer, Rob R., Klaas Sijtsma, and Nico G. Smid. 1990. Theoretical and Empirical Comparison of the Mokken and the Rasch Approach to IRT. Applied Psychological Measurement 14: 283–98. [Google Scholar] [CrossRef]
- Mokkan, Robert J., and Charles Lewis. 1982. A Nonparametric Approach to the Analysis of Dichotomous Item Responses. Applied Psychological Measurement 6: 417–30. [Google Scholar] [CrossRef]
- Mokken, Robert Jan. 1971. A Theory and Procedure of Scale Analysis. The Hague and Berlin: Mouton de Gruyter. [Google Scholar]
- Mokken, Robert J. 1997. Nonparametric Models for Dichotomous Responses. In Handbook of Modern Item Response Theory. Edited by Wim J. van der Linden and Ronald K. Hambleton. New York: Springer, pp. 351–67. [Google Scholar] [CrossRef]
- Molenaar, Ivo W., and Klaas Sijtsma. 2000. User’s Manual MSP5 for Windows. Groningen: IEC ProGAMMA. [Google Scholar]
- Myszkowski, Nils, and Martin Storme. 2018. A snapshot of g? Binary and polytomous item-response theory investigations of the last series of the Standard Progressive Matrices (SPM-LS). Intelligence 68: 109–16. [Google Scholar] [CrossRef]
- Rasch, Georg. 1993. Probabilistic Models for Some Intelligence and Attainment Tests. Chicago: MESA Press. [Google Scholar]
- Raven, John C., John Raven, and John Hugh Court. 1962. Advanced Progressive Matrices. London: HK Lewis. [Google Scholar]
- Raven, John C. 1941. Standardization of Progressive Matrices, 1938. British Journal of Medical Psychology 19: 137–50. [Google Scholar] [CrossRef]
- Ree, Malcolm James, and James A. Earles. 1992. Intelligence Is the Best Predictor of Job Performance. Current Directions in Psychological Science 1: 86–89. [Google Scholar] [CrossRef]
- Rohde, Treena Eileen, and Lee Anne Thompson. 2007. Predicting academic achievement with cognitive ability. Intelligence 35: 83–92. [Google Scholar] [CrossRef]
- Salgado, Jesús F., Neil Anderson, Silvia Moscoso, Cristina Bertua, Filip De Fruyt, and Jean Pierre Rolland. 2003. A Meta-Analytic Study of General Mental Ability Validity for Different Occupations in the European Community. The Journal of Applied Psychology 88: 1068–81. [Google Scholar] [CrossRef] [PubMed]
- Sijtsma, Klaas, and Rob R. Meijer. 1992. A method for investigating the intersection of item response functions in Mokken’s nonparametric IRT model. Applied Psychological Measurement 16: 149–57. [Google Scholar] [CrossRef]
- Sijtsma, Klaas, and Ivo W. Molenaar. 1987. Reliability of test scores in nonparametric item response theory. Psychometrika 52: 79–97. [Google Scholar] [CrossRef]
- Sijtsma, Klaas, and L. Andries van der Ark. 2017. A tutorial on how to do a Mokken scale analysis on your test and questionnaire data. British Journal of Mathematical and Statistical Psychology 70: 137–58. [Google Scholar] [CrossRef]
- Sijtsma, Klaas, Rob R. Meijer, and L. Andries van der Ark. 2011. Mokken scale analysis as time goes by: An update for scaling practitioners. Personality and Individual Differences 50: 31–37. [Google Scholar] [CrossRef]
- Sijtsma, Klaas. 1998. Methodology Review: Nonparametric IRT Approaches to the Analysis of Dichotomous Item Scores. Applied Psychological Measurement 22: 3–31. [Google Scholar] [CrossRef]
- Storme, Martin, Nils Myszkowski, Simon Baron, and David Bernard. 2019. Same Test, Better Scores: Boosting the Reliability of Short Online Intelligence Recruitment Tests with Nested Logit Item Response Theory Models. Journal of Intelligence 7: 17. [Google Scholar] [CrossRef] [PubMed]
- Straat, J. Hendrik, L. Andries van der Ark, and Klaas Sijtsma. 2014. Minimum Sample Size Requirements for Mokken Scale Analysis. Educational and Psychological Measurement 74: 809–22. [Google Scholar] [CrossRef]
- Straat, J. Hendrik, L. Andries van der Ark, and Klaas Sijtsma. 2016. Using Conditional Association to Identify Locally Independent Item Sets. Methodology 12: 117–23. [Google Scholar] [CrossRef]
- Suh, Youngsuk, and Daniel M. Bolt. 2010. Nested Logit Models for Multiple-Choice Item Response Data. Psychometrika 75: 454–73. [Google Scholar] [CrossRef]
- Thissen, David, and Lynne Steinberg. 1984. A Response Model for Multiple Choice Items. Psychometrika 49: 501–19. [Google Scholar] [CrossRef]
- Tijmstra, Jesper, and Maria Bolsinova. 2019. Bayes Factors for Evaluating Latent Monotonicity in Polytomous Item Response Theory Models. Psychometrika 84: 846–69. [Google Scholar] [CrossRef]
- Tijmstra, Jesper, Herbert Hoijtink, and Klaas Sijtsma. 2015. Evaluating Manifest Monotonicity Using Bayes Factors. Psychometrika 80: 880–96. [Google Scholar] [CrossRef]
- Van der Ark, L. Andries. 2012. New Developments in Mokken Scale Analysis in R. Journal of Statistical Software 48: 1–27. [Google Scholar] [CrossRef]
- Van der Ark, L. Andries. 2007. Mokken Scale Analysis in R. Journal of Statistical Software 20: 1–19. [Google Scholar] [CrossRef]
- van der Ark, L. Andries, Daniël W. van der Palm, and Klaas Sijtsma. 2011. A Latent Class Approach to Estimating Test-Score Reliability. Applied Psychological Measurement 35: 380–92. [Google Scholar] [CrossRef]
- Winsberg, Suzanne, David Thissen, and Howard Wainer. 1984. Fitting Item Characteristic Curves with Spline Functions. ETS Research Report Series 1984. [Google Scholar] [CrossRef]
| 1. | For Figure 3, the plotting function of the mokken package was modified in order for all rest score groups on the x-axis to be consistent. In addition, it can be noted that the three plots involve item 1, but that its item response function appears slightly different in the three plots. The reason for this is that the rest score is computed in each plot using all items but the two items involved in the comparison. Since the item pair is different in each plot, the rest score group is therefore different, leading to slightly different response functions for the same item. |

| Index | Item | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | |||||||||||||
| 2 | 0.616 | ||||||||||||
| 3 | 0.331 | 0.535 | |||||||||||
| 4 | 0.248 | 0.613 | 0.286 | ||||||||||
| 5 | 0.294 | 0.493 | 0.421 | 0.772 | |||||||||
| 6 | 0.272 | 0.511 | 0.509 | 0.520 | 0.675 | ||||||||
| 7 | 0.122 | 0.544 | 0.295 | 0.471 | 0.575 | 0.362 | |||||||
| 8 | 0.263 | 0.647 | 0.442 | 0.636 | 0.701 | 0.547 | 0.429 | ||||||
| 9 | 0.095 | 0.393 | 0.460 | 0.441 | 0.481 | 0.416 | 0.427 | 0.378 | |||||
| 10 | 0.327 | 0.709 | 0.799 | 0.938 | 0.921 | 0.743 | 0.526 | 0.449 | 0.403 | ||||
| 11 | 0.399 | 0.664 | 0.569 | 0.822 | 0.774 | 0.677 | 0.595 | 0.506 | 0.522 | 0.467 | |||
| 12 | 0.267 | 0.717 | 0.253 | 0.839 | 0.847 | 0.576 | 0.613 | 0.602 | 0.462 | 0.400 | 0.449 | ||
| 0.265 | 0.568 | 0.426 | 0.545 | 0.602 | 0.499 | 0.422 | 0.476 | 0.401 | 0.529 | 0.536 | 0.500 |
| Index | Item | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 3.044 | 3.621 | 3.842 | 3.852 | 4.714 | 2.897 | 3.963 | 1.857 | 2.956 | 1.955 | 1.556 | ||
| 2 | 0.508 | 1.865 | 3.742 | 3.196 | 1.908 | 1.497 | 2.806 | 0.661 | 1.932 | 0.349 | 0.309 | ||
| 3 | 1.073 | 2.381 | 2.512 | 3.065 | 2.503 | 1.324 | 2.432 | 1.449 | 3.655 | 0.909 | 0.634 | ||
| 4 | 0.036 | 2.128 | 0.503 | 3.039 | 1.018 | 0.468 | 1.772 | 0.095 | 2.959 | 0.019 | 0.162 | ||
| 5 | 0.072 | 1.844 | 0.430 | 3.299 | 1.043 | 0.591 | 2.739 | 0.181 | 2.915 | 0.021 | 0.037 | ||
| 6 | 0.352 | 1.996 | 0.389 | 1.668 | 2.098 | 0.392 | 1.237 | 0.159 | 2.578 | 0.101 | 0.704 | ||
| 7 | 0.766 | 2.287 | 1.529 | 2.730 | 3.621 | 1.666 | 1.523 | 0.779 | 2.265 | 0.282 | 0.310 | ||
| 8 | 0.136 | 1.450 | 0.445 | 2.325 | 2.537 | 0.721 | 0.775 | 0.502 | 0.605 | 0.045 | 1.769 | ||
| 9 | 0.483 | 3.996 | 2.731 | 3.375 | 3.486 | 2.376 | 2.507 | 3.225 | 2.176 | 1.241 | 0.873 | ||
| 10 | 0.077 | 3.838 | 1.735 | 3.742 | 3.316 | 1.245 | 0.893 | 0.317 | 0.326 | 0.448 | 0.107 | ||
| 11 | 0.425 | 5.611 | 1.779 | 8.765 | 8.429 | 2.972 | 1.813 | 0.854 | 1.451 | 0.994 | 0.274 | ||
| 12 | 1.137 | 5.129 | 1.017 | 5.455 | 7.380 | 3.288 | 2.525 | 2.370 | 2.441 | 2.234 | 2.089 | ||
| 49.281 | 38.952 | 42.890 | 30.910 | 27.740 | 39.323 | 44.227 | 33.246 | 44.265 | 28.471 | 35.393 | 33.611 | ||
| 1 | |||||||||||||
| 2 | 3.116 | ||||||||||||
| 3 | 3.487 | 2.708 | |||||||||||
| 4 | 4.338 | 3.408 | 5.276 | ||||||||||
| 5 | 3.826 | 3.321 | 3.457 | 0.297 | |||||||||
| 6 | 4.534 | 5.199 | 1.561 | 2.944 | 2.022 | ||||||||
| 7 | 6.683 | 3.861 | 5.579 | 2.820 | 1.616 | 5.398 | |||||||
| 8 | 3.869 | 3.653 | 4.941 | 2.959 | 2.919 | 3.122 | 2.187 | ||||||
| 9 | 7.181 | 5.033 | 3.288 | 4.118 | 4.116 | 5.143 | 4.575 | 1.376 | |||||
| 10 | 4.405 | 3.469 | 2.120 | 0.990 | 1.626 | 2.111 | 3.628 | 3.555 | 2.584 | ||||
| 11 | 3.540 | 2.756 | 4.269 | 2.037 | 2.424 | 3.074 | 5.086 | 3.468 | 3.870 | 1.604 | |||
| 12 | 4.303 | 2.428 | 6.204 | 1.723 | 2.116 | 4.215 | 2.797 | 1.198 | 2.982 | 2.378 | 3.267 |
| Item | ||
|---|---|---|
| 1 | 825.15 | 1.44 |
| 2 | 34,666.90 | 3.49 |
| 3 | 57,682.66 | 3.89 |
| 4 | 871,824.00 | 8.26 |
| 5 | 89,668.37 | 4.20 |
| 6 | 95.22 | 1.15 |
| 7 | 9594.47 | 4.13 |
| 8 | 818,417.90 | 6.40 |
| 9 | 12.08 | 2.58 |
| 10 | 50,455.13 | 3.80 |
| 11 | 1.98 | 4.31 |
| 12 | 1.64 | 0.81 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Myszkowski, N. A Mokken Scale Analysis of the Last Series of the Standard Progressive Matrices (SPM-LS). J. Intell. 2020, 8, 22. https://doi.org/10.3390/jintelligence8020022
Myszkowski N. A Mokken Scale Analysis of the Last Series of the Standard Progressive Matrices (SPM-LS). Journal of Intelligence. 2020; 8(2):22. https://doi.org/10.3390/jintelligence8020022
Chicago/Turabian StyleMyszkowski, Nils. 2020. "A Mokken Scale Analysis of the Last Series of the Standard Progressive Matrices (SPM-LS)" Journal of Intelligence 8, no. 2: 22. https://doi.org/10.3390/jintelligence8020022
APA StyleMyszkowski, N. (2020). A Mokken Scale Analysis of the Last Series of the Standard Progressive Matrices (SPM-LS). Journal of Intelligence, 8(2), 22. https://doi.org/10.3390/jintelligence8020022





