Abstract
The objective of this present paper is to utilize an auxiliary equation method for constructing exact solutions associated with variable coefficient function forms for certain nonlinear partial differential equations (NPDEs) in the sense of the conformable derivative. Utilizing the specific fractional transformations, the conformable derivatives appearing in the original equation can be converted into integer order derivatives with respect to new variables. As for applications of the method, we particularly obtain variable coefficient exact solutions for the conformable time (2 + 1)-dimensional Kadomtsev–Petviashvili equation and the conformable space-time (2 + 1)-dimensional Boussinesq equation. As a result, the obtained exact solutions for the equations are solitary wave solutions including a soliton solitary wave solution and a bell-shaped solitary wave solution. The advantage of the used method beyond other existing methods is that it provides variable coefficient exact solutions covering constant coefficient ones. In consequence, the auxiliary equation method based on setting all coefficients of an exact solution as variable function forms can be more extensively used, straightforward and trustworthy for solving the conformable NPDEs.
1. Introduction
Over the last few decades, many researchers have thoroughly investigated many methods for finding exact solutions of nonlinear partial differential equations (NPDEs). This is because the exact solutions play a crucial role in exactly describing physical phenomena such as nonlinear wave spreads in incompressible fluid, electromagnetic field, shallow water waves, epidemic diseases and water pollutant modeled by certain NPDEs [,,,,]. Precise behaviors of a mathematical system expressed by NPDEs can be analyzed through their exact solutions. In addition, symbolic computer software packages (e.g., Mathematica and Maple) have been continuously and progressively developed for several years. According to these reasons, it brings a vital challenge for solving NPDEs to scholars’ attention. Scientists and researchers have attempted to propose new and efficient techniques for constructing exact traveling wave solutions of NPDEs such as the -expansion method [,,], the multiple Exp-function method [], the modified Jacobi elliptic expansion method [], the improved Riccati equation mapping method [] and the modified extended simple equation [].
This paper addresses the use of an auxiliary equation method used to generate variable coefficient exact solutions for a particular type of NPDEs. Hence, some significant investigations of the method and its modifications are briefly reviewed as follows. Pinar [] used appropriate Lie group transformations and the auxiliary equation method in which the Bernoulli differential equation is considered to find exact solutions of the foam-drainage equation. Mahak and Akram [] utilized the modified auxiliary equation method to construct bright soliton waves, stable bright periodic waves and symmetric waves, which were exact solutions of the perturbed nonlinear Schrödinger equation with Kerr law nonlinearity. The modified form of the auxiliary equation method was employed to determine a large family of optical solutions to the Kundu–Eckhaus equation []. Moreover, the auxiliary equation method was applied to obtain exact traveling wave solutions of (2 + 1)-dimensional Zoomeron equation with conformable time derivative and the third order modified KdV equation with conformable time derivative []. The solutions obtained by the method were expressed in terms of the exponential function. More applications of an auxiliary equation method and its improvements can be found in [,,,,,] and the references therein.
In this work, we utilized an auxiliary equation method to extract exact solutions of two nonlinear conformable partial differential equations in which the conformable partial derivatives are involved. The two equations are described as follows.
- The conformable time (2 + 1)-dimensional Kadomtsev–Petviashvili equation of order is given aswhere is the conformable partial derivative of the dependent variable with respect to the independent variable t of order and is a positive real number. If Equation (1) becomes the integer order (2 + 1)-dimensional Kadomtsev–Petviashvili equation [,,] describing the evolution of nonlinear, long waves of small amplitudes with slow dependence on the transverse coordinate. In physics, this equation often provides multi-soliton solutions.
- The conformable space-time (2 + 1)-dimensional Boussinesq equation isAll of the partial derivatives appearing in Equation (2) are the conformable partial derivatives. When Equation (2) turns out to be the integer order (2 + 1)-dimensional Boussinesq equation [,]. The equation describes the propagation of gravity waves on the surface of water, especially the head-on collision of oblique waves.
The arrangement of the rest of this article is organized as follows. In Section 2, the definition of the conformable derivative and its important properties are given. In Section 3, the algorithm of an auxiliary equation method is briefly described. The applications of the method for finding exact solutions of Equations (1) and (2) are demonstrated in Section 4. Also, graphs of the selected exact solutions for the problems are depicted in this section. In Section 5, we present some discussions and conclusions.
2. Conformable Derivative and Its Characteristics
In this section, a definition of the conformable derivative and its essential properties are reviewed as follows.
Definition 1.
Given a function . Then the conformable derivative of f of order α is defined by [,,,,,,]
for all and If the limit in Equation (3) exists, then one can say that f is α-conformable differentiable at a point .
Theorem 1
([,,,,,,]). Let , and be α-conformable differentiable at a point . Then, we have
Remark 1.
The conformable derivatives of some interesting functions are as follows [,,,,,,].
- (1)
- .
- (2)
- .
- (3)
- .
- (4)
- .
- (5)
- , provided that is differentiable.
The following chain rule may be useful for a transformation between the conformable derivative and the classical derivative.
Theorem 2
([,,,,,,]). Let be a function such that f is differentiable and α-conformable differentiable. Further assume that g is a differentiable function defined in the range of f. Then, we have
where the prime symbol represents the ordinary derivative.
3. Algorithm of the Auxiliary Equation Method
In this section, the description of the auxiliary equation method for solving nonlinear partial differential equations, where the associated partial derivatives are the conformable derivative, is provided. Consider a conformable space–time partial differential equation in the independent variables as follows
where is an unknown function and each value of the fractional orders and the others appearing in Equation (4) is in . Here, the symbol represents the conformable partial derivative of a dependent variable u with respect to an independent variable v of order . Hence, the symbols and , for example, in Equation (4) are the conformable derivatives of the variable u with respect to the variable t of order , to the variable of order and to the variable of order respectively. In addition, the notation P in the equation represents a polynomial of the unknown function u and its various partial derivatives including the conformable derivatives.
Without loss of generality, we may assume that the conformable partial derivatives emerging in Equation (4) are related to only the variables t and ; however, the other partial derivatives with respect to the remaining variables are of integer orders. Next, the main steps of the auxiliary equation method for solving Equation (4) are described as follows [].
Step 1: The conformable partial derivatives in Equation (4), which are now can be converted into integer order partial derivatives with respect to the new variables using the following two fractional complex transformations:
Using Theorem 2, one can obtain
Consequently, the conformable partial differential Equation (4) can be transformed into an integer order partial differential equation as
Step 2: Assume that the solution of Equation (7) can be written by a polynomial in as
where are unknown functions to be determined at a later step and the function satisfies some certain auxiliary equation expressed in the form
for which its solutions are already known. Moreover, the positive integer m in Equation (8) can be computed via the homogeneous balance principle, balancing the degrees between the highest order derivatives and the nonlinear terms occurring in Equation (7). The formulas for computing the degree of some particular terms for this step can be found in Equation (10) of [].
In particular, one can select the Riccati equation
where as the auxiliary equation. It is not difficult to verify that
where d is an arbitrary constant, is a solution of Equation (10). Then, we have
Specifically choosing , we obtain
Step 3: Substituting Equation (8) into Equation (7) along with the relation between and obtained by Equation (9) (or, particularly Equation (10)), and then collecting all terms with the same power of together, the left-hand side of Equation (7) becomes another polynomial in . Equating each coefficient of the resulting polynomial to zero, one gives a set of partial differential equations for
Step 4: Solving the system of the partial differential equations obtained in Step 3 with the help of Maple and using the solutions of Equation (9) (or, particularly the solution in (11)), together with the fractional complex transformations in (5), we eventually obtain exact solutions of Equation (4).
Remark 2.
The idea of setting the solution in (8) in which ξ is a general function of the independent variables including T and in (5) and satisfies the auxiliary Equation (9) is reminiscent of the direct approach called a similarity reduction [,]. This method is more general than our technique because it does not specify the auxiliary equation for and involve with group theoretical techniques. In general, the appropriate similarity reduction can reduce the partial differential equation to an ordinary differential equation.
Remark 3.
We can change the solution form (8) and the Riccati equation (10) in the above process so that the new algorithm, called the modified auxiliary equation method, is obtained. To further grasp the modified approaches, we can refer to [,,,,]. Some of the alternative auxiliary equations used in the the modified auxiliary equation methods are as follows. The first example is the Bernoulli type differential equation expressed as [,]
where and are any functions and is an integer. The solutions of (14) alter depending upon and . The second example of the auxiliary equation is [,]
where and σ are parameters to be determined and . The solutions of (15) can be expressed in terms of tangent, cotangent, hyperbolic tangent, hyperbolic cotangent, or rational functions.
4. Applications of the Method
In this section, we applied the auxiliary equation method to construct exact solutions with variable coefficient function forms for the conformable time (2 + 1)-dimensional Kadomtsev–Petviashvili equation and the conformable space–time (2 + 1)-dimensional Boussinesq equation. In addition, some selected solutions obtained using the method are graphically displayed to demonstrate their physical behaviors.
4.1. Conformable Time (2 + 1)-Dimensional Kadomtsev–Petviashvili Equation
We revisit the conformable time (2 + 1)-Kadomtsev–Petviashvili equation expressed as
where is the conformable partial derivative of the dependent variable with respect to the independent variable t of order and is a positive real number. Applying the transformations for the variable t and u as
where c is an arbitrary constant and using the chain rule in Theorem 2, we then have . Performing a small algebraic manipulation, Equation (16) is reduced into the following integer order partial differential equation
We assume that the solution of Equation (18) can be expressed by a polynomial in as
where and are undetermined functions. For convenience, we here set all coefficients to be functions of only two variables y and T. The function , which is the solution of the following auxiliary equation
where , can be expressed as
where d is an arbitrary constant. Consequently, we have
and if , then the ratio in Equation (22) becomes
Letting and then using Equation (10) of [] to balance the degree of the highest order derivative and of the nonlinear term in (18), we have and then . Thus, the solution form of Equation (18) can be written as
Substituting Equation (24) into Equation (18) with the aid of Equation (20), collecting all of the terms with the same power of together and then equating each of the resulting coefficients to zero, we get a set of partial differential equations in variables . Due to the limited space, only some parts of the obtained equations are displayed if they are too long. The system of the resulting PDEs for this stage is as follows.
Solving system (25) with the assistance of the symbolic software package such as Maple 17 and after removing trivial and redundant solutions, we obtain eight different sets of the unknown functions . Next, we can write the exact solutions with variable coefficient function forms for Equation (16) using (22) (or (23)) and (24) as follows.
Result 2:
where are arbitrary functions of T. Hence, the exact solution of Equation (16) for this case is
where and . If we choose , then in (29) can be reduced into
Result 3:
where are arbitrary functions of T. Thus, the solution of Equation (16) is
where and . When , the solution in (32) becomes
Result 4:
where are arbitrary constants. Hence, the exact solution of Equation (16) is written as
where . If , then in (35) turns out to be
Result 5:
where are arbitrary constants. Thus, the exact solution of Equation (16) is expressed as
where . When we let , then in (38) becomes
Result 6:
where is an arbitrary constant and are arbitrary functions of T. So, the exact solution of Equation (16) for this result is
where and . If , then in (41) can be converted into
Result 7:
where are arbitrary functions of T. Thus, the exact solution of Equation (16) is
where and . When , the solution in (44) becomes
Result 8:
where are arbitrary functions of T. Thus, the exact solution for Equation (16) can be expressed as
where and . If , then in (47) turns out to be
Next, we provide interesting graphical representations of some exact solutions obtained using the auxiliary equation method for the conformable time (2 + 1)-Kadomtsev–Petviashvili equation expressed in (16). The 3-D, 2-D and contour graphs for each of the selected solutions are plotted on a certain domain with different values of the fractional order . In Figure 1, the graphs of in (30) are revealed by letting and . In particular, Figure 1a–c shows the 3-D plot on , the 2-D plot with and the contour plot of when . Proceeding in a similar manner to the above plots, the 3-D, 2-D and contour graphs of when and are shown in Figure 1d–f and g–i, respectively. Moreover, Figure 2 displays the associated graphs of in (42) by setting and . Particularly, Figure 2a–c shows the 3-D graph on , the 2-D graph with and the contour graph of when . In the same manner, Figure 2d–f and g–i are portrayed using and respectively. Finally, the solution in (45) with and is drawn as the 3-D, 2-D and contour plots in Figure 3. Specifically, is used for plotting the 3-D graph on , the 2-D graph with and the contour graph of as shown in Figure 3a–c. Proceeding in the same manner, Figure 3d–f and g–i are drawn when and respectively.
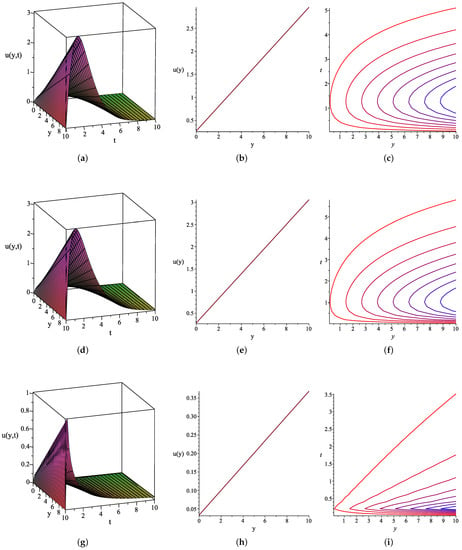
Figure 1.
Associated plots of in Equation (30) obtained using the auxiliary equation method: (a–c) 3-D plot, 2-D plot and contour plot, respectively when ; (d–f) 3-D plot, 2-D plot and contour plot, respectively when ; (g–i) 3-D plot, 2-D plot and contour plot, respectively when .
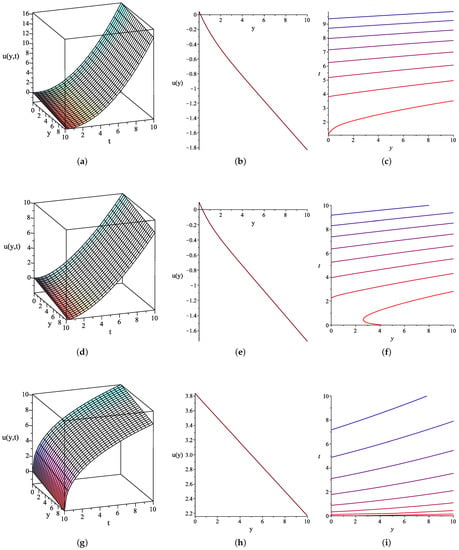
Figure 2.
Associated plots of in Equation (42) obtained using the auxiliary equation method: (a–c) 3-D plot, 2-D plot and contour plot, respectively when ; (d–f) 3-D plot, 2-D plot and contour plot, respectively when ; (g–i) 3-D plot, 2-D plot and contour plot, respectively when .
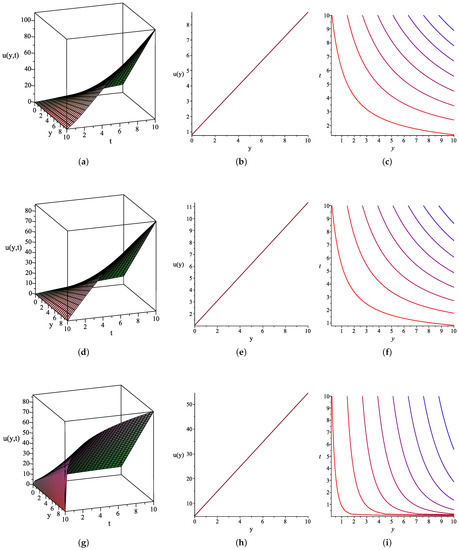
Figure 3.
Associated plots of in Equation (45) obtained using the auxiliary equation method: (a–c) 3-D plot, 2-D plot and contour plot, respectively when ; (d–f) 3-D plot, 2-D plot and contour plot, respectively when ; (g–i) 3-D plot, 2-D plot and contour plot, respectively when .
4.2. Conformable Space–Time (2 + 1)-Dimensional Boussinesq Equation
Consider the conformable space–time (2 + 1)-dimensional Boussinesq equation expressed as
All of the partial derivatives appearing in Equation (49) are the conformable partial derivatives, for instance, represents the conformable partial derivative of the unknown function with respect to the independent variable x of order . Applying the fractional complex transformations for the variables and u as
where are arbitrary constants and utilizing some properties of the conformable derivative described in Section 2, we have and . Proceeding in the manner above, the rest terms in (49) can be transformed into integer order partial derivatives and we consequently get the following PDE
By the auxiliary equation method, the exact solution form for Equation (51) can be written as
where and are some functions that are determined at a later step. Alternatively, we can set each coefficient as any function of the old and new variables but we here let be a function of only the new variables Y and T. Similarly, the function is the solution of the Riccati Equation (20). The expressions for and can be found in Equations (21) and (22) (or (23)), respectively.
Denoting and then utilizing Equation (10) of [] to balance the degree of the highest order derivative and of the nonlinear term in (51), we get and then . Hence, the solution form for Equation (51) can be determined as
Inserting Equation (53) into Equation (51) along with Equation (20), collecting all of the terms with the same power of together and then equating each of the obtained coefficients to zero, we have a set of partial differential equations in variables . Due to the minimalism, only some parts of the resulting PDEs are demonstrated if they are too long. The system of the obtained PDEs can be expressed as follows.
Solving system (54) with the help of Maple 17 and after removing trivial and redundant solutions, we obtain 14 distinct sets of the unknown functions
. In consequence, we can write the exact solutions with variable coefficient function forms for Equation (49) using (22) (or (23)) and (53) as follows.
Result 1:
where are arbitrary functions of and , respectively. Thus, the exact solution of Equation (49) is
where and .
Result 2:
where is an arbitrary constant and are arbitrary functions of and are arbitrary functions of . Thus, the exact solution for Equation (49) is shown as
where and . If we select , then in (58) becomes
Result 3:
where are arbitrary functions of and , respectively. Thus, the exact solution of Equation (49) is as follows
where and . When , then in (61) turns out to be
Result 4:
where are arbitrary constants. So, the exact solution of Equation (49) is
where . If , then in (64) is transformed into
Result 5:
where are arbitrary constants. Thus, the exact solution of Equation (49) is expressed as
where and . If we choose , then in (67) becomes
Result 6:
where is an arbitrary constant and is an arbitrary function of . Hence, the exact solution of Equation (49) for this case is
where and . If , then in (70) becomes
Result 7:
where is an arbitrary constant and is an arbitrary function of . Thus, the exact solution of Equation (49) is expressed as
where and .
If , then in (73) becomes
Result 8:
where are arbitrary constants. Thus, the exact solution of Equation (49) is read as
where and . If , then in (76) becomes
Result 9:
where are arbitrary constants and is an arbitrary function of . Therefore, the exact solution of Equation (49) is
where and . When , the solution in (79) is transformed as
Result 10:
where is an arbitrary constant and are arbitrary functions of
and , respectively. Therefore, the exact solution of Equation (49) is
where and . If , then the solution in (82) becomes
Result 11:
where are arbitrary functions of and , respectively. Thus, the exact solution of Equation (49) is expressed as
where and . If we select , then the solution in (85) turns out to be
Result 12:
where are arbitrary functions of . Thus, the exact solution for Equation (49) is as follows
where . If , then in (88) is transformed into
Result 13:
where is an arbitrary constant and are arbitrary functions of and are arbitrary functions of . Thus, the exact solution of Equation (49) is
where and . If , then in (91) becomes
Result 14:
where are arbitrary functions of and , respectively. Thus, the exact solution of Equation (49) is
where and . When we choose , then the solution in (94) turns out to be
Some selected exact solutions, which are obtained using the auxiliary equation method, of the conformable space–time (2 + 1)-dimensional Boussinesq equation expressed in (49) is graphically presented by considering some effect of distinct values of the fractional order . Their graphical representations include the 3-D, 2-D and contour plots on a particular domain. In Figure 4, the graphs of in (71) are portrayed by setting and . Specifically, Figure 4a–c shows the 3-D plot on , the 2-D plot with and the contour plot of when . In the same manner, the 3-D, 2-D and contour graphs of when and are displayed in Figure 4d–f and g–i, respectively.
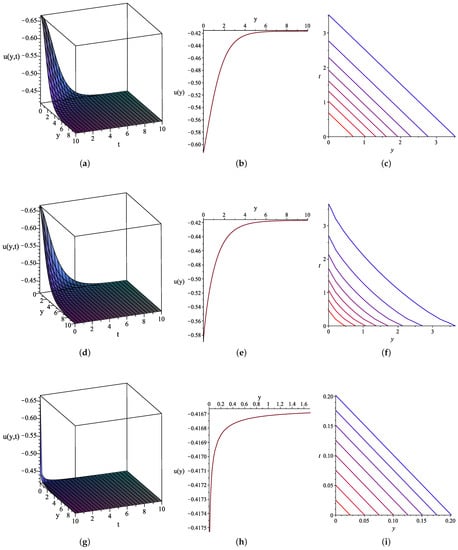
Figure 4.
Associated plots of in Equation (71) obtained using the auxiliary equation method: (a–c) 3-D plot, 2-D plot and contour plot, respectively when ; (d–f) 3-D plot, 2-D plot and contour plot, respectively when ; (g–i) 3-D plot, 2-D plot and contour plot, respectively when .
Furthermore, Figure 5 shows the associated plots for in (77) evaluated when . In particular, Figure 5a–c shows the 3-D graph on , the 2-D graph with and the contour graph of when . In the same manner, Figure 5d–f and g–i are portrayed using and respectively. The exact solution in (80) with and is drawn as the 3-D, 2-D and contour graphs in Figure 6. Specifically, is employed for plotting the 3-D graph on , the 2-D graph with and the contour graph of as demonstrated in Figure 6a–c. Proceeding in a similar manner, Figure 6d–f and g–i are portrayed when and respectively. Lastly, the 3-D, 2-D and contour plots of the solution in (89) are exhibited in Figure 7 using and . In particular, Figure 7a–c shows the 3-D plot on , the 2-D graph with and the contour plot of when . Proceeding in like manner, the 3-D, 2-D and contour graphs of when and are shown in Figure 7d–f and g–i, respectively.

Figure 5.
Associated plots of in Equation (77) obtained using the auxiliary equation method: (a–c) 3-D plot, 2-D plot and contour plot, respectively when ; (d–f) 3-D plot, 2-D plot and contour plot, respectively when ; (g–i) 3-D plot, 2-D plot and contour plot, respectively when .
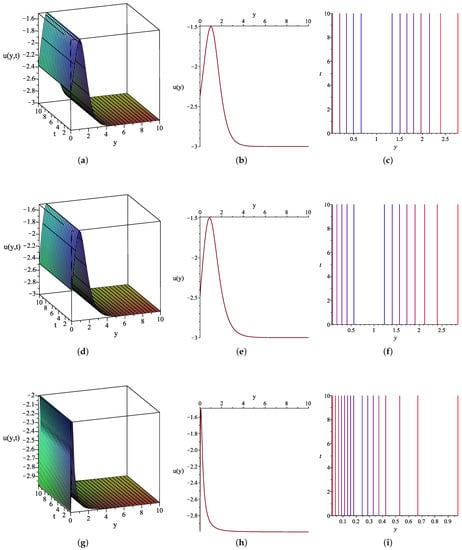
Figure 6.
Associated plots of in Equation (80) obtained using the auxiliary equation method: (a–c) 3-D plot, 2-D plot and contour plot, respectively when ; (d–f) 3-D plot, 2-D plot and contour plot, respectively when ; (g–i) 3-D plot, 2-D plot and contour plot, respectively when .
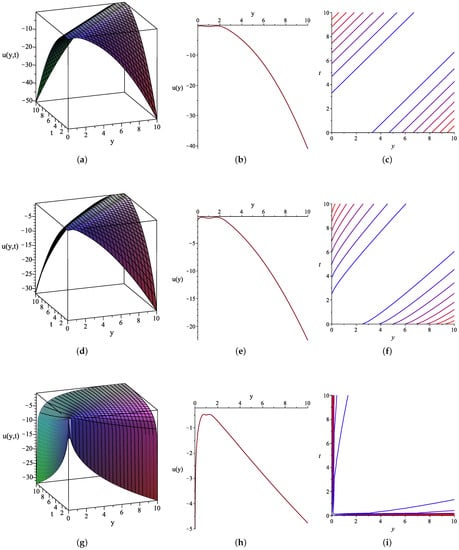
Figure 7.
Associated plots of in Equation (89) obtained using the auxiliary equation method: (a–c) 3-D plot, 2-D plot and contour plot, respectively when ; (d–f) 3-D plot, 2-D plot and contour plot, respectively when ; (g–i) 3-D plot, 2-D plot and contour plot, respectively when .
5. Conclusions and Outlook
The brief algorithm of an auxiliary equation method for solving a nonlinear conformable PDE for an exact solution, in which its coefficients are in the form of variable functions, comprises the following steps. Firstly, the conformable PDE is converted into an integer order PDE via some fractional complex transformations. Secondly, we assume that an exact solution of the transformed equation is in the form of a polynomial, which has variable function coefficients and is associated with a solution of an auxiliary equation such that the Riccati equation. The degree of such a polynomial solution can be determined using the homogeneous balance principle. Thirdly, we use symbolic software packages such as Maple 17 to solve a system of PDEs expressed in terms of the variable function coefficients. Such a system of the PDEs is derived by inserting the known solution form into the integer order PDE and then equating all coefficients of the solution of the auxiliary equation to be zero. Finally, the exact solution of the original equation can be formulated via the known variable function coefficients. According to the use of the method, we have obtained eight different exact solutions for the conformable time (2 + 1)-dimensional Kadomtsev–Petviashvili Equation (16) and fourteen exact solutions for the conformable space–time (2 + 1)-dimensional Boussinesq Equation (49). In particular, most of the obtained exact solutions for both equations can be written in terms of hyperbolic tangent functions if we set . As a result, some graphical representations of the obtained outcomes demonstrate certain physical behaviors, for example, the graphs of in (30) are characterized as an inclined bell-shaped solitary wave solution and the plots of in (77) represent a 1-soliton solitary wave solution. Since our exact solutions for the two equations have the variable coefficients including constant coefficients, then the obtained results are new and reported here for the first time. Of course, all of the exact solutions for both equations constructed using the method have been checked by substituting them back into their corresponding equations with the help of Maple 17.
In summary, an auxiliary equation method along with the use of symbolic computation packages is a reliable, efficient and convenient tool for extracting exact solutions with variable coefficient function forms for the interesting NPDEs arising in applied sciences and engineering. Such solution forms include the case of constant coefficients which has been already used for assuming an exact solution form for other existing methods. Changing function forms for variable coefficients in the assumed exact solution such as replacing with or a use of other auxiliary equations different from the Riccati equation could consequently produce more and various exact solutions for the NPDEs.
Author Contributions
Conceptualization, S.S.; data curation, T.I.; formal analysis, S.S. and T.I.; funding acquisition, S.S.; investigation, S.S., N.T. and T.I.; methodology, S.S., N.T. and T.I.; project administration, S.S.; software, T.I.; supervision, S.S. and N.T.; validation, S.S., N.T. and T.I.; visualization, S.S., N.T. and T.I.; writing—original draft, S.S. and T.I.; writing—review and editing, S.S. and N.T. All authors have read and approved to the published version of the manuscript.
Funding
The first author was financially supported by the Faculty of Applied Science, King Mongkut’s University of Technology North Bangkok under contract no. 641080.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Acknowledgments
The authors are grateful to anonymous referees for the valuable comments, which have greatly improved this article.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Ali, M.R.; Ma, W.X. New exact solutions of nonlinear (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Adv. Math. Phys. 2019, 2019, 9801638. [Google Scholar] [CrossRef]
- Gepreel, K.A. Analytical Methods for Nonlinear Evolution Equations in Mathematical Physics. Mathematics 2020, 8, 2211. [Google Scholar] [CrossRef]
- Alharbi, A.; Almatrafi, M.B. Exact and numerical solitary wave structures to the variant Boussinesq system. Symmetry 2020, 12, 1473. [Google Scholar] [CrossRef]
- Li, Y.; Teng, Z.; Hu, C.; Ge, Q. Global stability of an epidemic model with age-dependent vaccination, latent and relapse. Chaos Solitons Fractals 2017, 105, 195–207. [Google Scholar] [CrossRef]
- Pananu, K.; Sungnul, S.; Sirisubtawee, S.; Phongthanapanich, S. Convergence and Applications of the Implicit Finite Difference Method for Advection-Diffusion-Reaction Equations. IAENG Int. J. Comput. Sci. 2020, 47, 645–663. [Google Scholar]
- Miah, M.M.; Ali, H.S.; Akbar, M.A.; Seadawy, A.R. New applications of the two variable (G′/G,1/G)–expansion method for closed form traveling wave solutions of integro-differential equations. J. Ocean. Eng. Sci. 2019, 4, 132–143. [Google Scholar] [CrossRef]
- Yokus, A.; Durur, H.; Ahmad, H.; Thounthong, P.; Zhang, Y.F. Construction of exact traveling wave solutions of the Bogoyavlenskii equation by (G′/G,1/G)–expansion and (1/G′)–expansion techniques. Results Phys. 2020, 19, 103409. [Google Scholar] [CrossRef]
- Yokuş, A.; Durur, H.; Abro, K.A.; Kaya, D. Role of Gilson–Pickering equation for the different types of soliton solutions: A nonlinear analysis. Eur. Phys. J. Plus 2020, 135, 1–19. [Google Scholar] [CrossRef]
- Wan, P.; Manafian, J.; Ismael, H.F.; Mohammed, S.A. Investigating one-, two-, and triple-wave solutions via multiple exp-function method arising in engineering sciences. Adv. Math. Phys. 2020, 2020. [Google Scholar] [CrossRef]
- Hosseini, K.; Mirzazadeh, M.; Osman, M.; Al Qurashi, M.; Baleanu, D. Solitons and Jacobi elliptic function solutions to the complex Ginzburg–Landau equation. Front. Phys. 2020, 8, 225. [Google Scholar] [CrossRef]
- Tala-Tebue, E.; Rezazadeh, H.; Eslami, M.; Bekir, A. New approach to model coupled nerve fibers and exact solutions of the system. Chin. J. Phys. 2019, 62, 179–186. [Google Scholar] [CrossRef]
- Yue, C.; Lu, D.; Arshad, M.; Nasreen, N.; Qian, X. Bright-Dark and Multi Solitons Solutions of (3+1)-Dimensional Cubic-Quintic Complex Ginzburg–Landau Dynamical Equation with Applications and Stability. Entropy 2020, 22, 202. [Google Scholar] [CrossRef]
- Pinar, Z. The combination of conservation laws and auxiliary equation method. Int. J. Appl. Comput. Math. 2020, 6, 1–7. [Google Scholar] [CrossRef]
- Mahak, N.; Akram, G. The modified auxiliary equation method to investigate solutions of the perturbed nonlinear Schrödinger equation with Kerr law nonlinearity. Optik 2020, 207, 164467. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Korkmaz, A.; Eslami, M.; Mirhosseini-Alizamini, S.M. A large family of optical solutions to Kundu–Eckhaus model by a new auxiliary equation method. Opt. Quantum Electron. 2019, 51, 1–12. [Google Scholar] [CrossRef]
- Akbulut, A.; Kaplan, M. Auxiliary equation method for time-fractional differential equations with conformable derivative. Comput. Math. Appl. 2018, 75, 876–882. [Google Scholar] [CrossRef]
- Khater, M.M.; Seadawy, A.R.; Lu, D. New optical soliton solutions for nonlinear complex fractional Schrödinger equation via new auxiliary equation method and novel (G′/G,1/G)-expansion method. Pramana 2018, 90, 1–20. [Google Scholar] [CrossRef]
- Meng, F.; Feng, Q. Exact solutions with variable coefficient function forms for conformable fractional partial differential equations by an auxiliary equation method. Adv. Math. Phys. 2018, 2018. [Google Scholar] [CrossRef]
- Lu, D.; Seadawy, A.R.; Iqbal, M. Mathematical methods via construction of traveling and solitary wave solutions of three coupled system of nonlinear partial differential equations and their applications. Results Phys. 2018, 11, 1161–1171. [Google Scholar] [CrossRef]
- Pinar, Z.; Kocak, H. Exact solutions for the third-order dispersive-Fisher equations. Nonlinear Dyn. 2018, 91, 421–426. [Google Scholar] [CrossRef]
- Owyed, S.; Abdou, M.; Abdel-Aty, A.H.; Ray, S.S. New optical soliton solutions of nolinear evolution equation describing nonlinear dispersion. Commun. Theor. Phys. 2019, 71, 1063. [Google Scholar] [CrossRef]
- Durur, H.; Tasbozan, O.; Kurt, A. New analytical solutions of conformable time fractional bad and good modified Boussinesq equations. Appl. Math. Nonlinear Sci. 2020, 5, 447–454. [Google Scholar] [CrossRef]
- Xu, Z.; Chen, H.; Dai, Z. Rogue wave for the (2 + 1)-dimensional Kadomtsev–Petviashvili equation. Appl. Math. Lett. 2014, 37, 34–38. [Google Scholar] [CrossRef]
- Ghanbari, B.; Liu, J.G. Exact solitary wave solutions to the (2 + 1)-dimensional generalised Camassa–Holm–Kadomtsev–Petviashvili equation. Pramana 2020, 94, 1–11. [Google Scholar] [CrossRef]
- Zhang, Z.; Yang, X.; Li, W.; Li, B. Trajectory equation of a lump before and after collision with line, lump, and breather waves for (2 + 1)-dimensional Kadomtsev–Petviashvili equation. Chin. Phys. B 2019, 28, 110201. [Google Scholar] [CrossRef]
- Lu, D.; Seadawy, A.R.; Ahmed, I. Applications of mixed lump-solitons solutions and multi-peaks solitons for newly extended (2 + 1)-dimensional Boussinesq wave equation. Mod. Phys. Lett. 2019, 33, 1950363. [Google Scholar] [CrossRef]
- Feng, L.L.; Tian, S.F.; Zhang, T.T. Bäcklund transformations, nonlocal symmetries and soliton–cnoidal interaction solutions of the (2 + 1)-dimensional Boussinesq Equation. Bull. Malays. Math. Sci. Soc. 2020, 43, 141–155. [Google Scholar] [CrossRef]
- Khalil, R.; Horani, M.A.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Kumar, D.; Seadawy, A.R.; Joardar, A.K. Modified Kudryashov method via new exact solutions for some conformable fractional differential equations arising in mathematical biology. Chin. J. Phys. 2018, 56, 75–85. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Korkmaz, A.; Eslami, M.; Vahidi, J.; Asghari, R. Traveling wave solution of conformable fractional generalized reaction Duffing model by generalized projective Riccati equation method. Opt. Quantum Electron. 2018, 50, 1–13. [Google Scholar] [CrossRef]
- Sirisubtawee, S.; Koonprasert, S.; Sungnul, S. Some Applications of the (G′/G,1/G)–Expansion Method for Finding Exact Traveling Wave Solutions of Nonlinear Fractional Evolution Equations. Symmetry 2019, 11, 952. [Google Scholar] [CrossRef]
- Sirisubtawee, S.; Koonprasert, S.; Sungnul, S.; Leekparn, T. Exact traveling wave solutions of the space–time fractional complex Ginzburg–Landau equation and the space-time fractional Phi-4 equation using reliable methods. Adv. Differ. Equ. 2019, 2019, 1–23. [Google Scholar] [CrossRef]
- Sirisubtawee, S.; Koonprasert, S.; Sungnul, S. New Exact Solutions of the Conformable Space-Time Sharma–Tasso–Olver Equation Using Two Reliable Methods. Symmetry 2020, 12, 644. [Google Scholar] [CrossRef]
- Topsakal, M.; TaŞcan, F. Exact Travelling Wave Solutions for Space-Time Fractional Klein-Gordon Equation and (2 + 1)-Dimensional Time-Fractional Zoomeron Equation via Auxiliary Equation Method. Appl. Math. Nonlinear Sci. 2020, 5, 437–446. [Google Scholar] [CrossRef]
- Clarkson, P.A. New similarity solutions for the modified Boussinesq equation. J. Phys. Math. Gen. 1989, 22, 2355. [Google Scholar] [CrossRef]
- Clarkson, P.A.; Kruskal, M.D. New similarity reductions of the Boussinesq equation. J. Math. Phys. 1989, 30, 2201–2213. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).