Near-Field Flow Structure and Entrainment of a Round Jet at Low Exit Velocities: Implications on Microclimate Ventilation
Abstract
1. Introduction
2. Materials and Methods
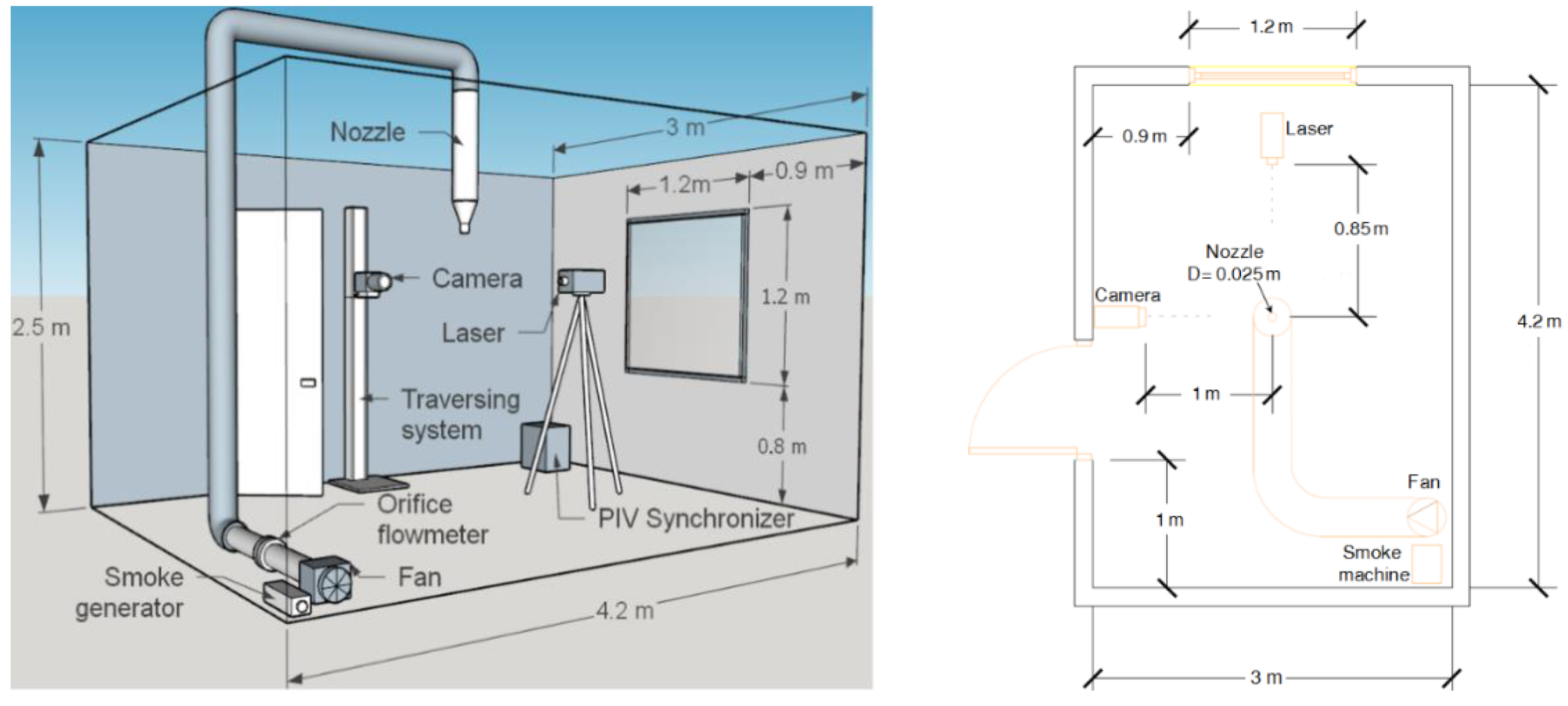
2.1. Experimental Setup and Equipment
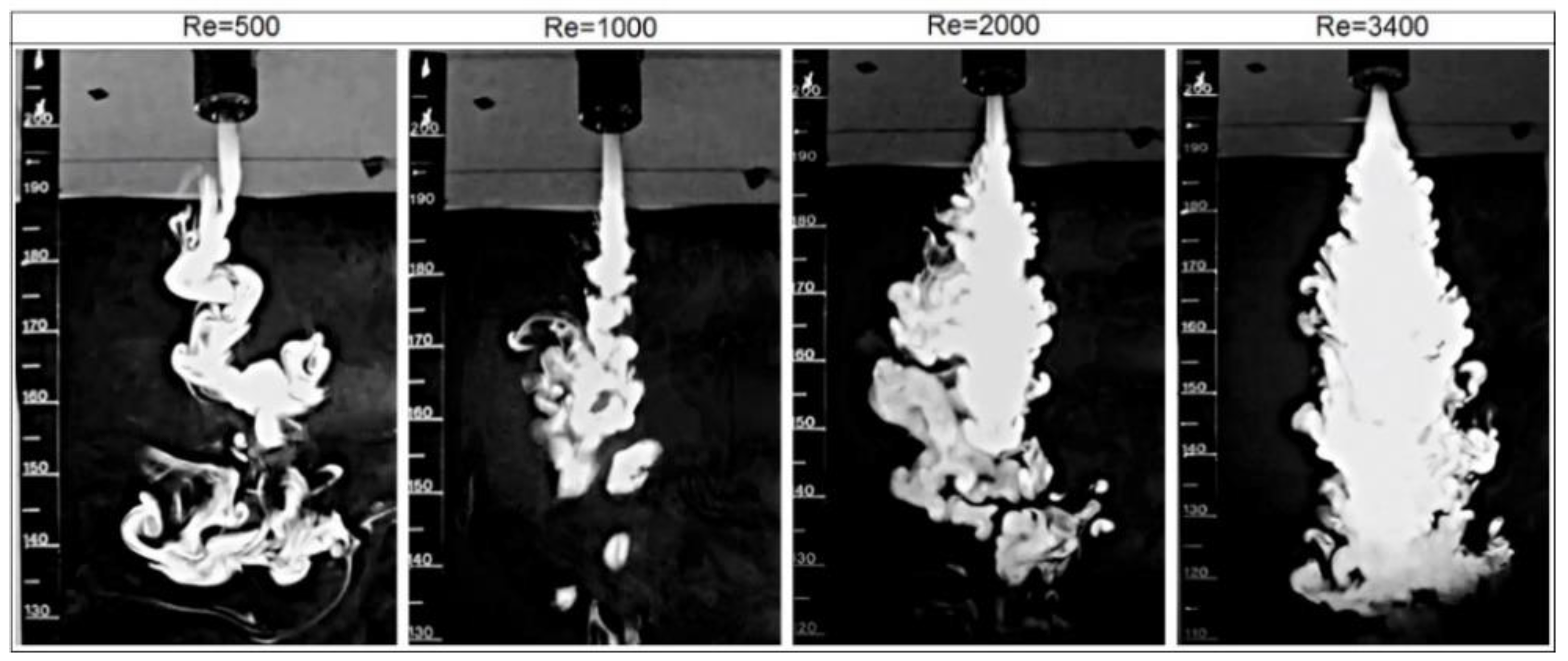
2.2. Experimental Conditions
3. Results
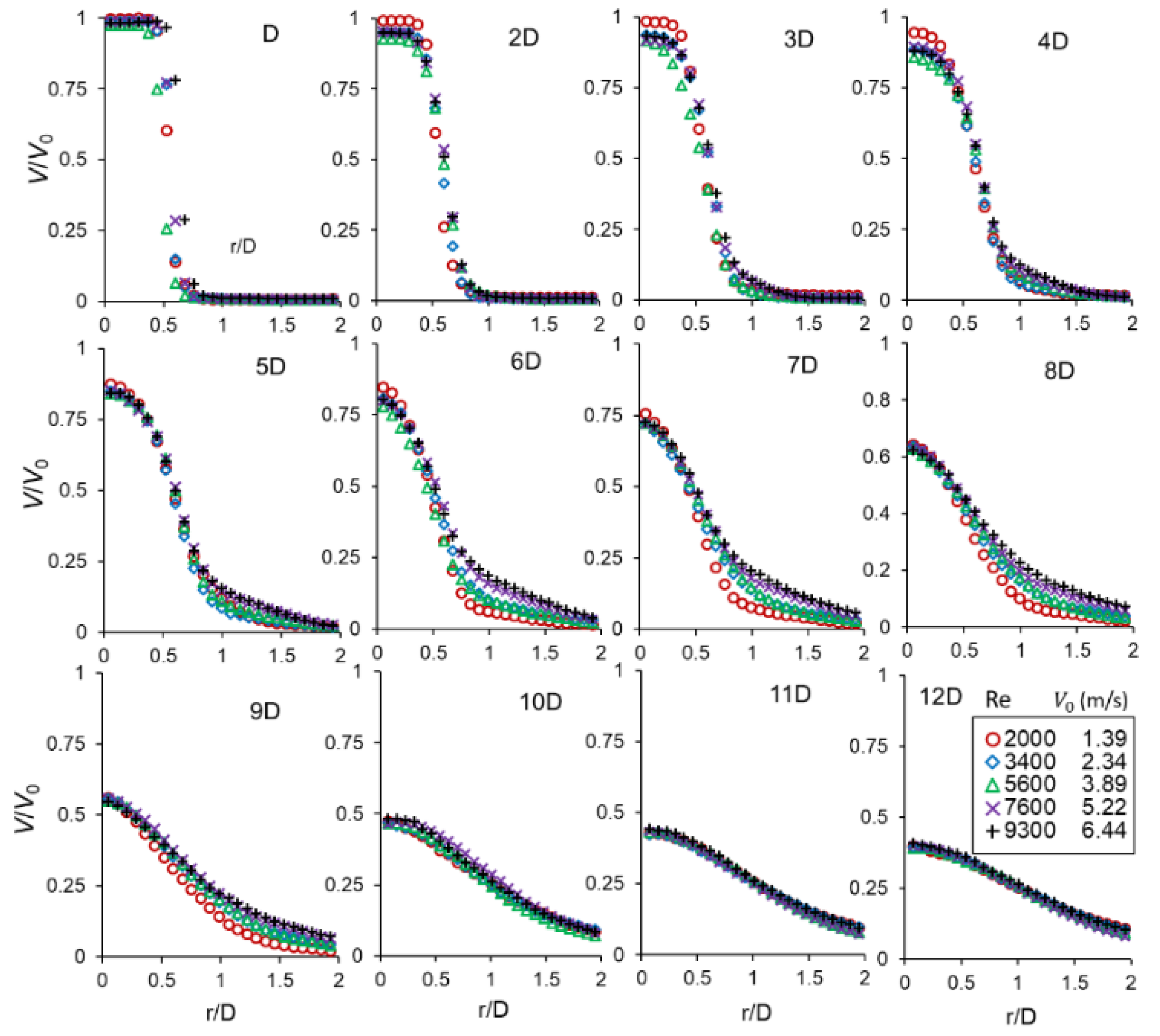
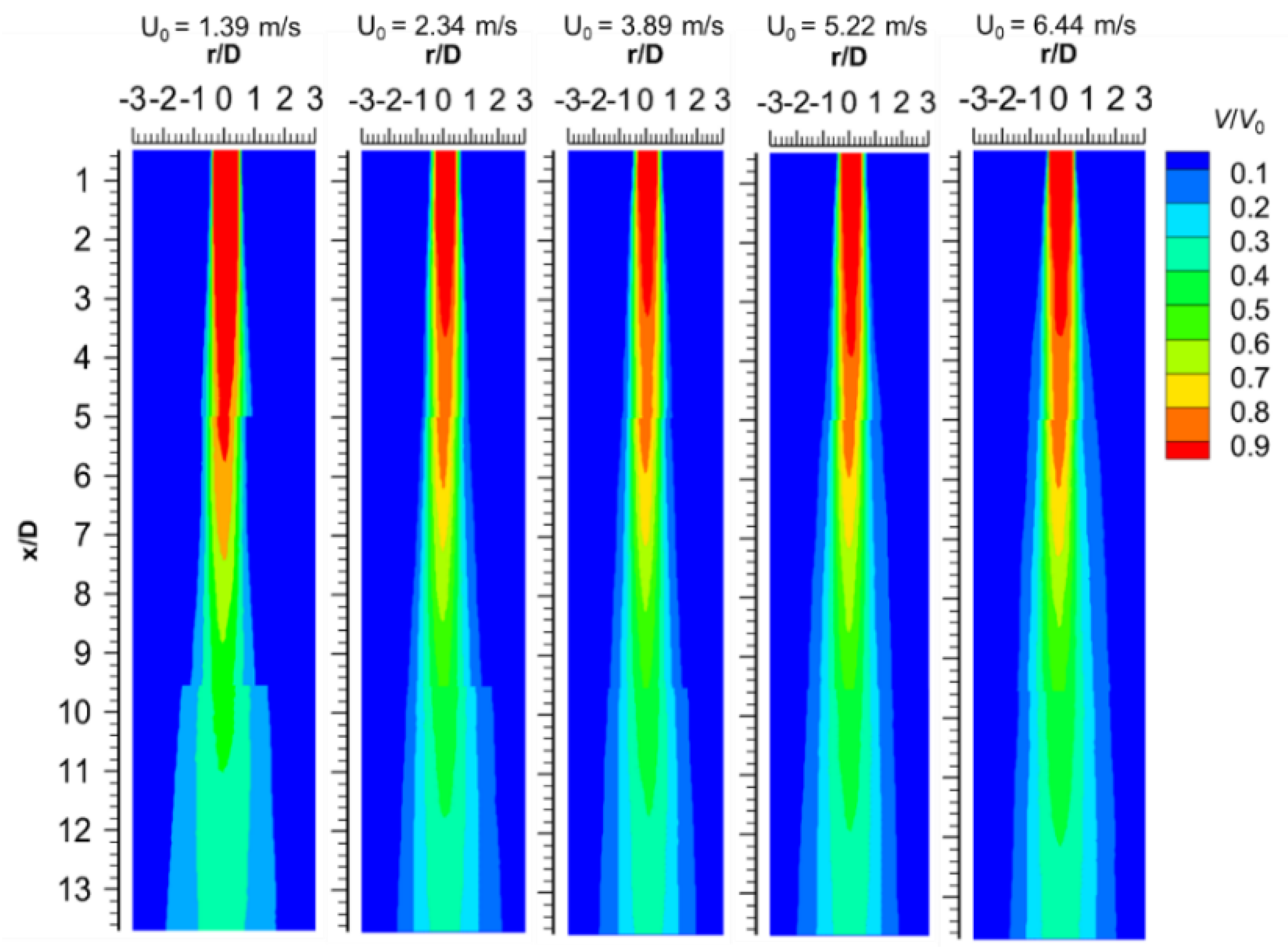
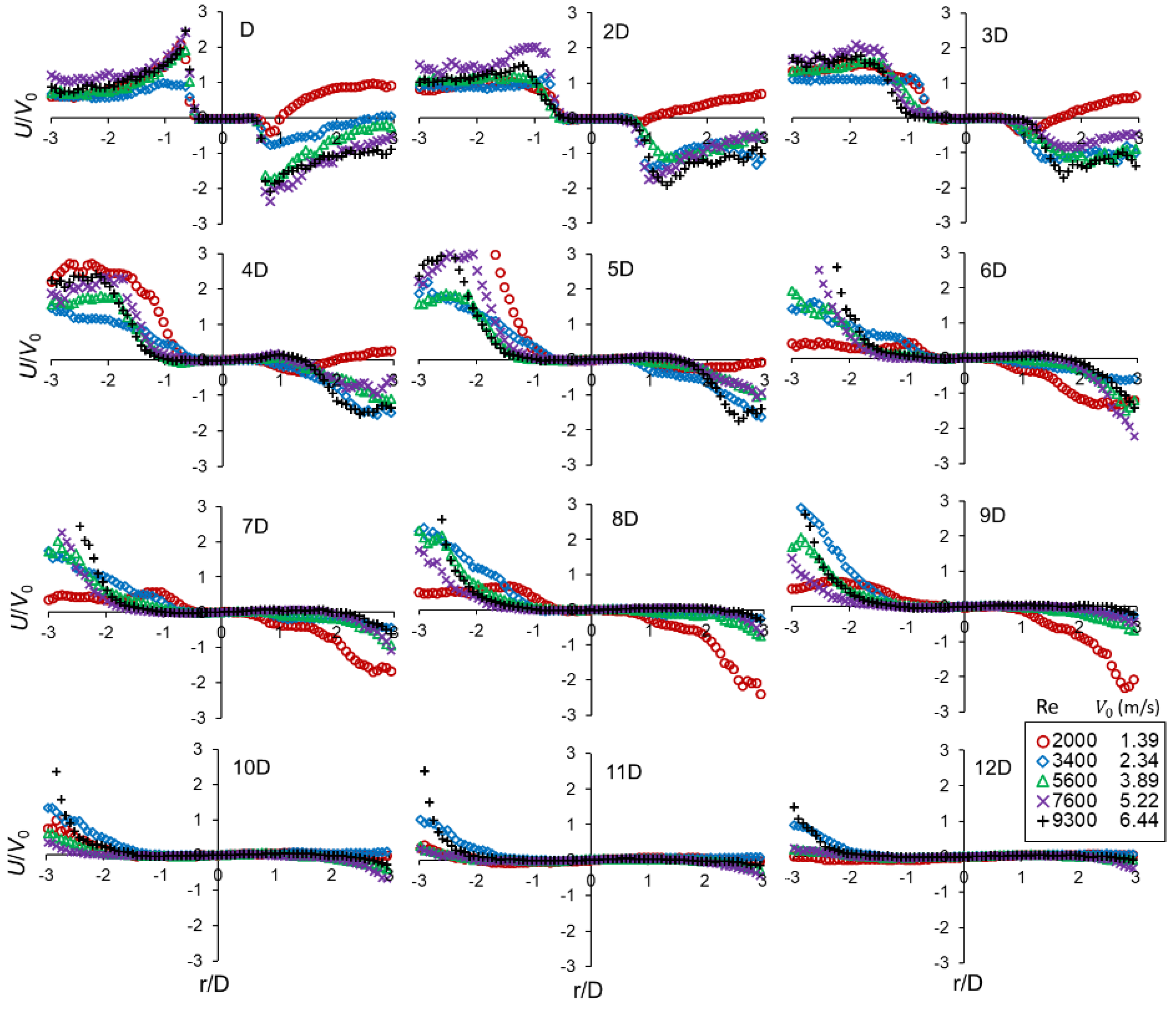
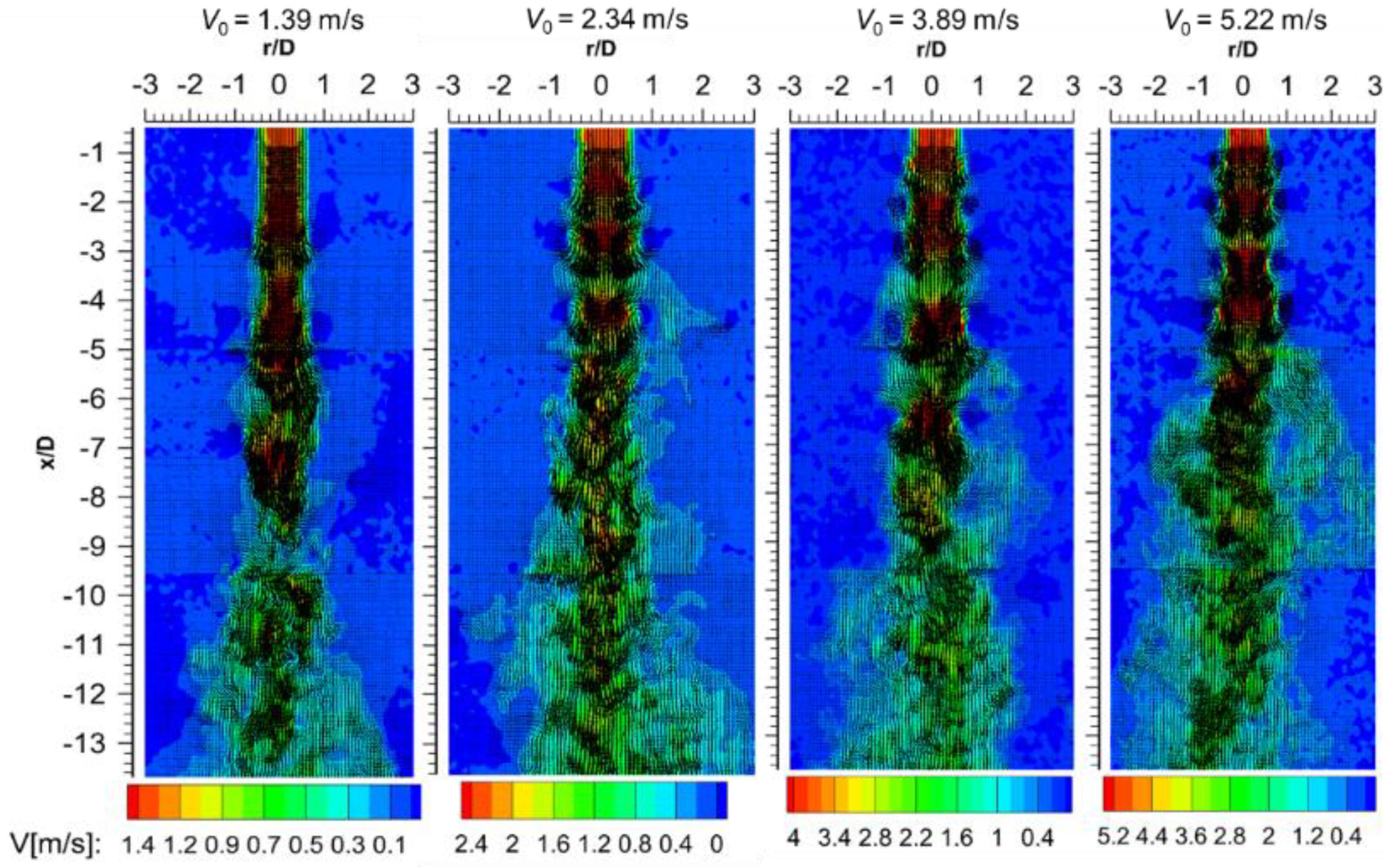
3.1. Evolution of the Mean Velocity Field
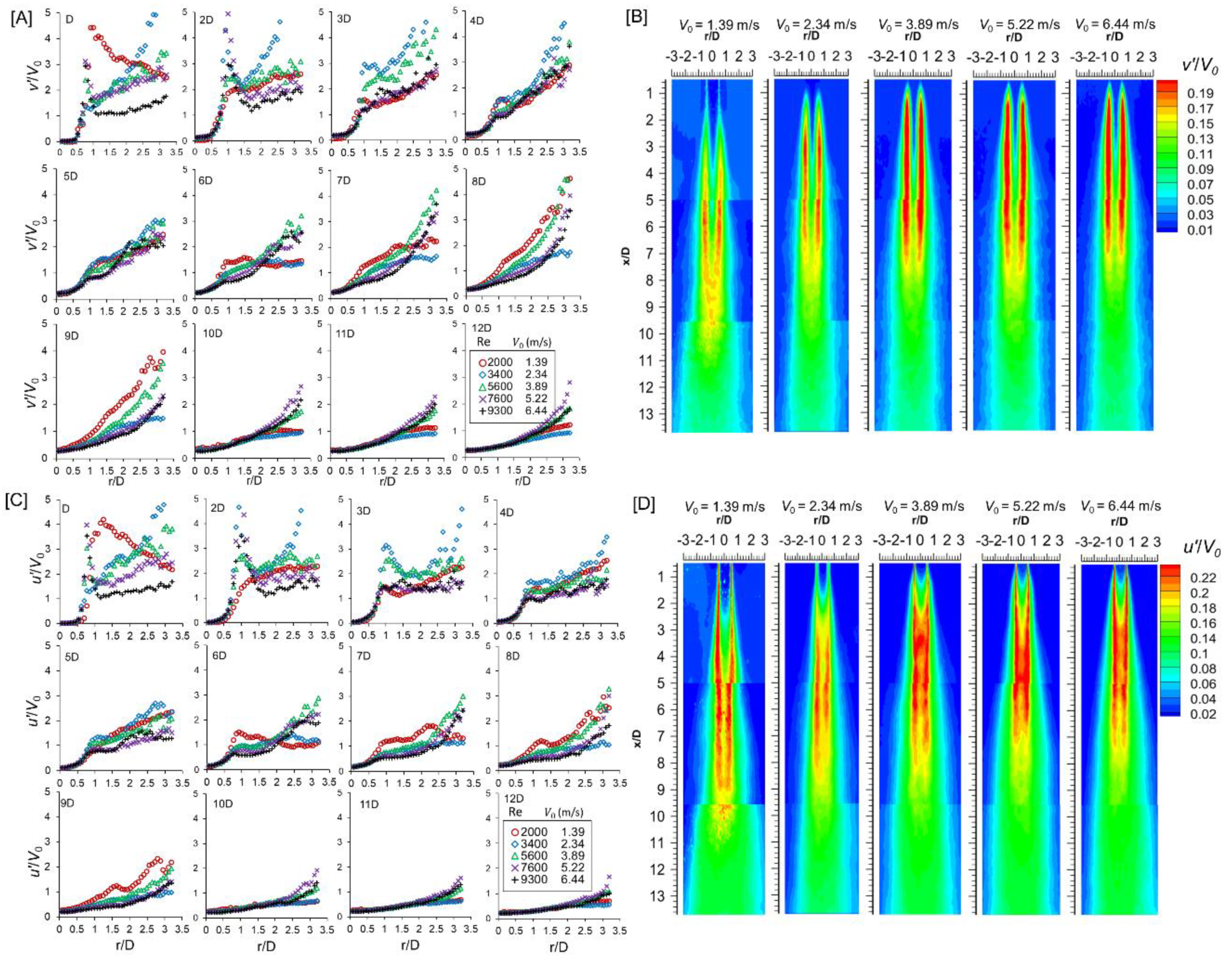
3.2. Evolution of the Turbulent Velocity Field
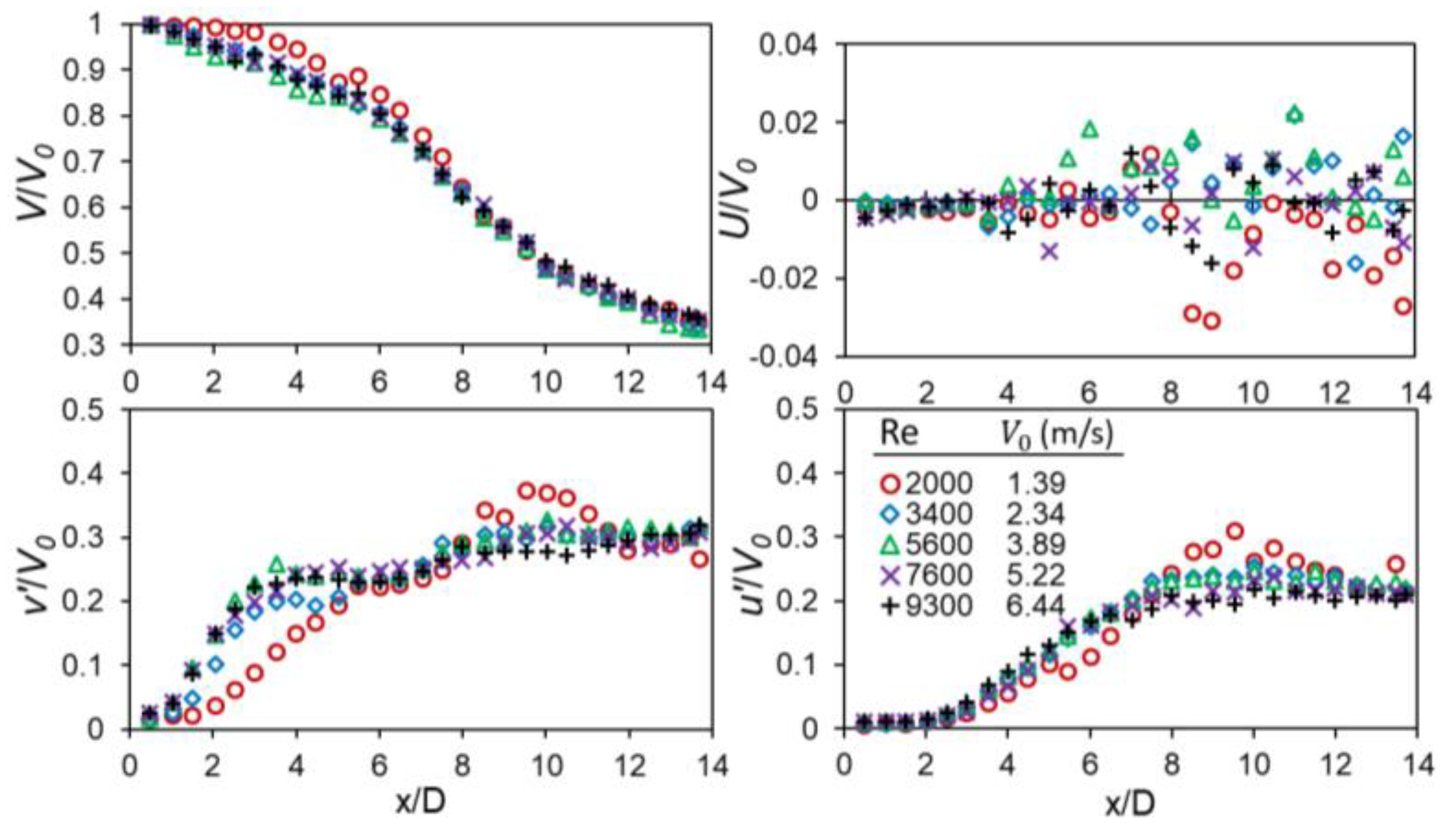
3.3. Characteristics of the Mean and Turbelent Flow on the Jet Centerline
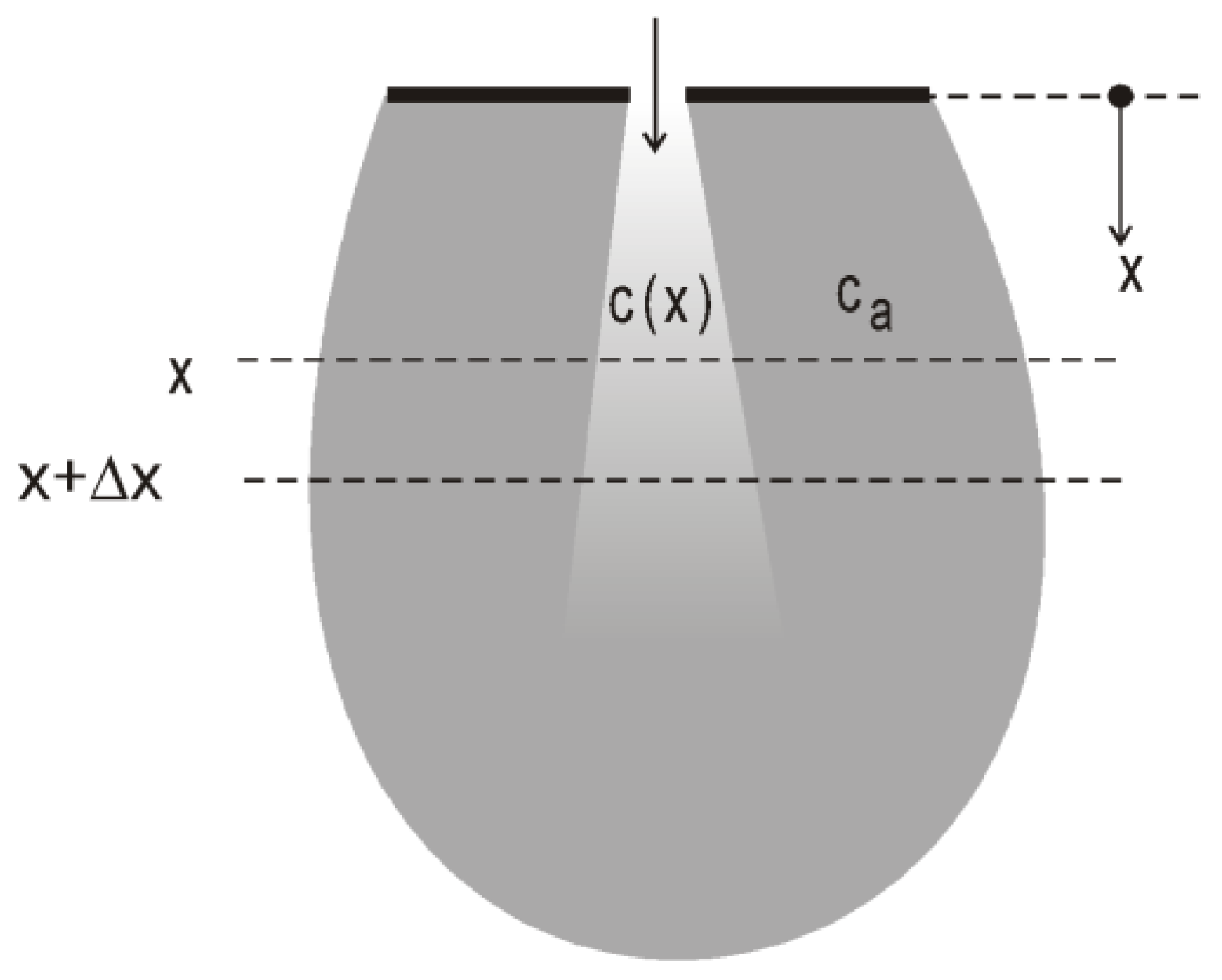
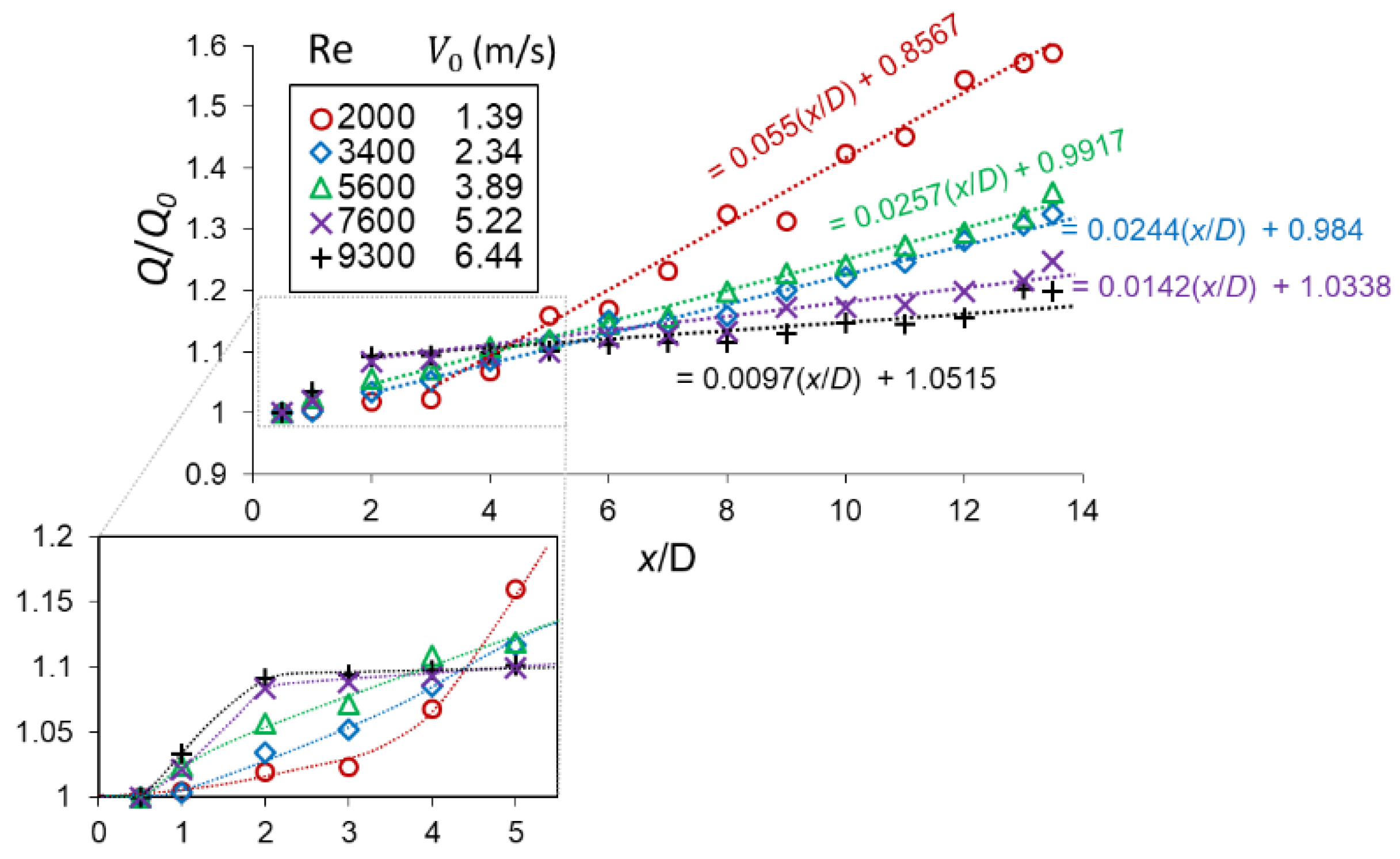
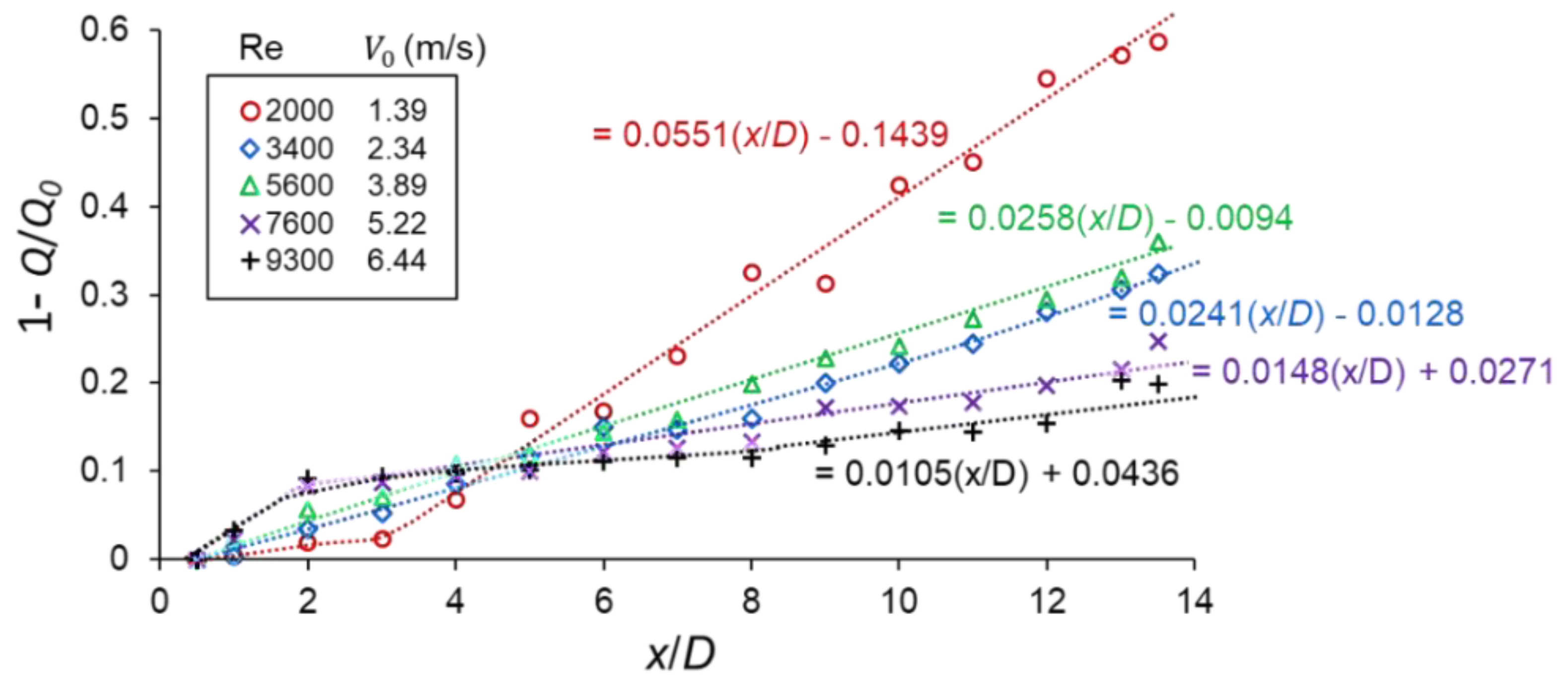
3.4. Evolution of the Mass Flowrate and Jet Entrainment
4. Discussion
Funding
Acknowledgments
Conflicts of Interest
Appendix A

References
- Ball, C.G.; Fellouah, H.; Pollard, A. The flow field in turbulent round free jets. Prog. Aerosp. Sci. 2012, 50, 1–26. [Google Scholar]
- Fiedler, H.E. Control of free turbulent shear flows. In Flowcontrol: Fundamentalsandpractices; Hak, M., Pollard, A., Bonnet, J., Eds.; Springer: Berlin, Germany, 1998; pp. 335–429. [Google Scholar]
- Townsend, A.A. Entrainment in Free Turbulent Flows. In Advances in Turbulence 2; Springer: Berlin, Germany, 1989; pp. 109–112. [Google Scholar]
- Mi, J.; Nathan, G.J.; Nobes, D.S. Influence of jet exit conditions on the passive scalar field of an axisymmetric free jet. J. Fluid Mech. 2001, 432, 91–125. [Google Scholar]
- Sreenivasan, K.R.; Antonia, R.A. The phenomenology of small-scale turbulence. Annu. Rev. Fluid Mech. 1997, 29, 435–472. [Google Scholar]
- George, W.K. The self-preservation of turbulent flows and its relation to initial conditions and coherent structures. Adv Turbul. 1989, 3973, 39–74. [Google Scholar]
- Capp, S.P. Experimental Investigation of the Turbulent Axisymmetric Jet; State Univ. of New York: Albany, NY, USA, 1984. [Google Scholar]
- Sandberg, M.; Kabanshi, A.; Wigö, H. Is building ventilation a process of diluting contaminants or delivering clean air? Indoor Built Environ. 2020, 29, 768–774. [Google Scholar] [CrossRef]
- Kabanshi, A.; Sandberg, M. Entrainment and its implications on microclimate ventilation systems: Scaling the velocity and temperature field of a round free jet. Indoor Air 2019, 29, 331–346. [Google Scholar] [CrossRef]
- Kabanshi, A.; Sattari, A.; Linden, E.; Wigö, H.; Sandberg, M. Experimental study on contaminant entrainment in air distribution systems with free jets. In Proceedings of the Healthy Buildings 2017 Europe, Lublin, Poland, 2–5 July 2017. [Google Scholar]
- Tavangar, T.; Tofighian, H.; Tarokh, A. Investigation of the Horizontal Motion of Particle-Laden Jets. Computation 2020, 8, 23. [Google Scholar]
- Haque, J.N.; Mahmud, T.; Roberts, K.J.; Rhodes, D. Modeling turbulent flows with free-surface in unbaffled agitated vessels. Ind. Eng. Chem. Res. 2006, 45, 2881–2891. [Google Scholar]
- Svensson, K.; Ghahremanian, S.; Moshfegh, B.; Tummers, M. Numerical and experimental investigation of flow behavior in a confluent jet ventilation system for industrial premises. In Proceedings of the The 10th International Conference on Industrial Ventilation, Paris, France, 17–19 September 2012. [Google Scholar]
- van Reeuwijk, M.; Craske, J. Energy-consistent entrainment relations for jets and plumes. J. Fluid Mech. 2015, 782, 333–355. [Google Scholar]
- Viggiano, B.; Dib, T.; Ali, N.; Mastin, L.G.; Cal, R.B.; Solovitz, S.A. Turbulence, entrainment and low-order description of a transitional variable-density jet. J. Fluid Mech. 2018, 836, 1009–1049. [Google Scholar]
- List, E.J.; Imberger, J. Turbulent entrainment in buoyant jets and plumes. J. Hydraul. Div. 1973, 99, 1461–1474. [Google Scholar]
- Ricou, F.P.; Spalding, D.B. Measurement of entrainment by axisymmetric turbulent. J. Fluid Mech. 1961, 11, 21–31. [Google Scholar]
- Hill, B.J. Measurement of local entrainment rate in the initial region of axisymmetric turbulent air jets. J. Fluid Mech. 1972, 51, 773–779. [Google Scholar]
- Todde, V.; Spazzini, P.G.; Sandberg, M. Experimental analysis of low-Reynolds number free jets. Exp. Fluids 2009, 47, 279–294. [Google Scholar]
- Todde, V.; Linden, E.; Sandberg, M. Indoor Low Speed Air Jet Flow: Fibre Film Probe Measurements. In Proceedings of the ROOMVENT’98, Stockholm, Sweden, 14–17 June 1998. [Google Scholar]
- Raffel, M.; Willert, C.E.; Scarano, F.; Kähler, C.J.; Wereley, S.T.; Kompenhans, J. Particle Image Velocimetry: A Practical Guide; Springer: Cham, Germany, 2018; ISBN 3319688529. [Google Scholar]
- Feng, L.; Yao, S.; Sun, H.; Jiang, N.; Liu, J. TR-PIV measurement of exhaled flow using a breathing thermal manikin. Build. Environ. 2015, 94, 683–693. [Google Scholar]
- Cao, X.; Liu, J.; Pei, J.; Zhang, Y.; Li, J.; Zhu, X. 2D-PIV measurement of aircraft cabin air distribution with a high spatial resolution. Build. Environ. 2014, 82, 9–19. [Google Scholar]
- Westerweel, J. Theoretical analysis of the measurement precision in particle image velocimetry. Exp. Fluids 2000, 29, S003–S012. [Google Scholar]
- Cao, X.; Liu, J.; Jiang, N.; Chen, Q. Particle image velocimetry measurement of indoor airflow field: A review of the technologies and applications. Energy Build. 2014, 69, 367–380. [Google Scholar]
- Du, Z.; Jin, X.; Fan, B. Evaluation of operation and control in HVAC (heating, ventilation and air conditioning) system using exergy analysis method. Energy 2015, 89, 372–381. [Google Scholar]
- Ferdman, E.; Ötugen, M.V.; Kim, S. Effect of Initial Velocity Profile on the Development of Round Jets. J. Propuls. Power 2000, 16, 676–686. [Google Scholar]
- Etheridge, D.W.; Sandberg, M. Building Ventilation: Theory and Measurement; John Wiley & Sons: Chichester, UK, 1996; ISBN 9780471960874. [Google Scholar]
- New, T.H.; Lim, T.T.; Luo, S.C. Effects of jet velocity profiles on a round jet in cross-flow. Exp. Fluids 2006, 40, 859–875. [Google Scholar]
- Falcone, A.M.; Cataldo, J.C. Entrainment Velocity in an Axisymmetric Turbulent Jet. J. Fluids Eng. 2003, 125, 620–627. [Google Scholar]
- Mih, W.C. Equations for axisymmetric and twodimensional turbulent jets. J. Hydraul. Eng. 1989, 115, 1715–1719. [Google Scholar]
- Morton, B.R.; Taylor, G.I.; Turner, J.S. Turbulent gravitational convection from maintained and instantaneous sources. Proc. R. Soc. London. Ser. A Math. Phys. Sci. 1956, 234, 1–23. [Google Scholar]
- List, E.J. Turbulent jets and plumes. Annu. Rev. Fluid Mech. 1982, 14, 189–212. [Google Scholar]
- Melikov, A.K. Personalized ventilation. Indoor Air 2004, 14, 157–167. [Google Scholar]
- Mi, J.; Kalt, P.; Nathan, G.J.; Wong, C.Y. PIV measurements of a turbulent jet issuing from round sharp-edged plate. Exp. Fluids 2007, 42, 625–637. [Google Scholar]
- Russ, S.; Strykowski, P.J. Turbulent structure and entrainment in heated jets: The effect of initial conditions. Phys. Fluids A Fluid Dyn. 1993, 5, 3216–3225. [Google Scholar]
- Philip, J.; Marusic, I. Large-scale eddies and their role in entrainment in turbulent jets and wakes. Phys. Fluids 2012, 24, 55–108. [Google Scholar]
| Re | V0 (m/s) 1 | ||
|---|---|---|---|
| 1 | 2000 | 0.00068 | 1.39 |
| 2 | 3400 | 0.00115 | 2.34 |
| 3 | 5600 | 0.00191 | 3.89 |
| 4 | 7600 | 0.00256 | 5.22 |
| 5 | 9300 | 0.00316 | 6.44 |
| Downstream Distance (x/D) 1 | |||
|---|---|---|---|
| V0 (m/s) | Graphical Method | ||
| 1 | 1.39 | 21 | 33 |
| 2 | 2.34 | 39 | 67 |
| 3 | 3.89 | 42 | 71 |
| 4 | 5.22 | 66 | 118 |
| 5 | 6.44 | 91 | 172 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kabanshi, A. Near-Field Flow Structure and Entrainment of a Round Jet at Low Exit Velocities: Implications on Microclimate Ventilation. Computation 2020, 8, 100. https://doi.org/10.3390/computation8040100
Kabanshi A. Near-Field Flow Structure and Entrainment of a Round Jet at Low Exit Velocities: Implications on Microclimate Ventilation. Computation. 2020; 8(4):100. https://doi.org/10.3390/computation8040100
Chicago/Turabian StyleKabanshi, Alan. 2020. "Near-Field Flow Structure and Entrainment of a Round Jet at Low Exit Velocities: Implications on Microclimate Ventilation" Computation 8, no. 4: 100. https://doi.org/10.3390/computation8040100
APA StyleKabanshi, A. (2020). Near-Field Flow Structure and Entrainment of a Round Jet at Low Exit Velocities: Implications on Microclimate Ventilation. Computation, 8(4), 100. https://doi.org/10.3390/computation8040100





