Spatiotemporal Dynamics of a Generalized Viral Infection Model with Distributed Delays and CTL Immune Response
Abstract
1. Introduction
- (H0)
- g(0,I) = 0, for all ; (or is a strictly monotone increasing function with respect to T when ) and , for all and .
- (H1)
- , for all and ,
- (H2)
- is a strictly monotone increasing function with respect to T (or when is a strictly monotone increasing function with respect to T), for any fixed and ,
- (H3)
- is a monotone decreasing function with respect to I and V.
2. Model Formulation and Preliminaries
3. Global Stability
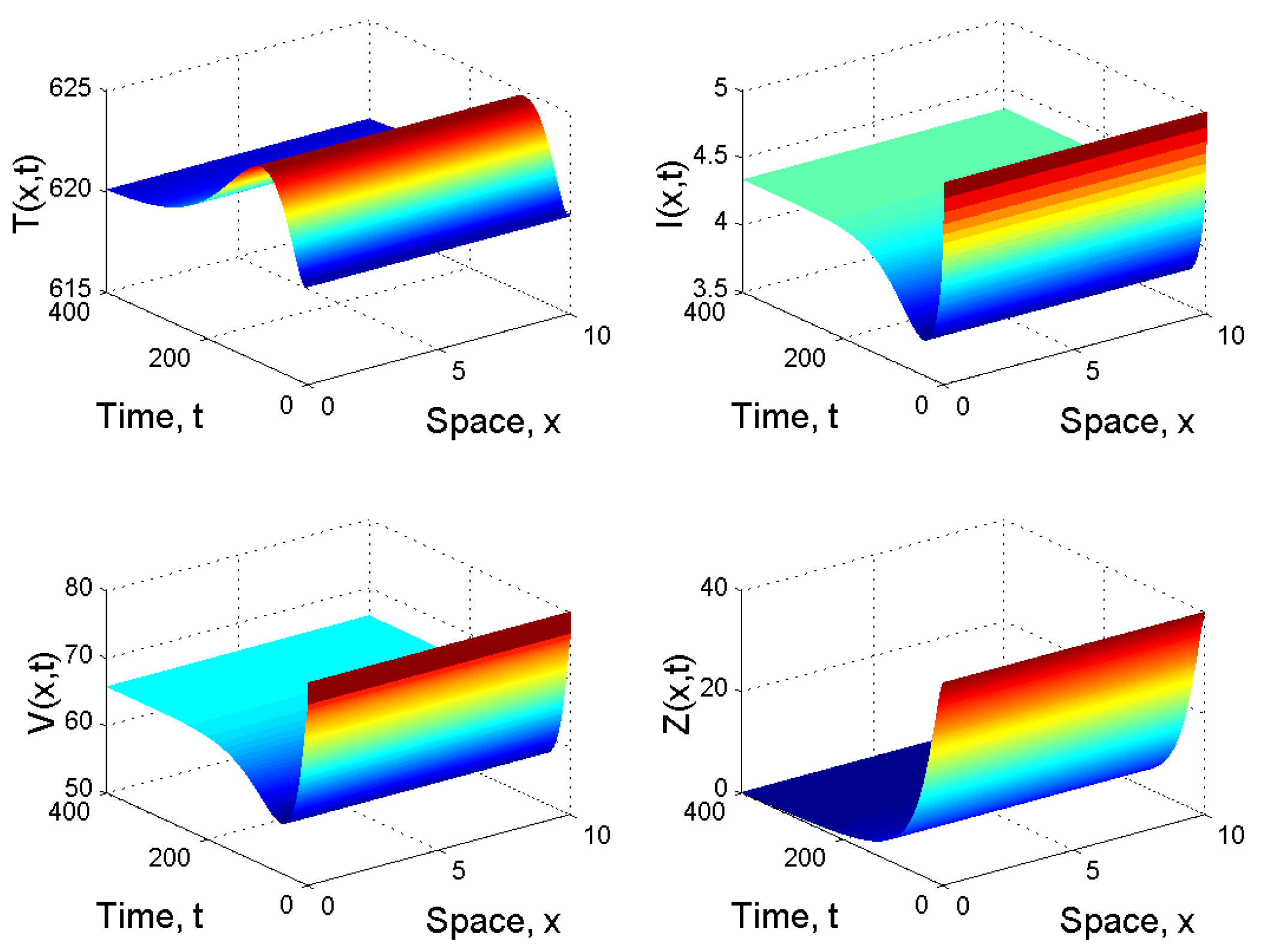
4. Application and Numerical Simulations
- If, then system (21) has two infection equilibria that are:
- (i)
- the infection equilibrium without cellular immunitythat is globally asymptotically stable if;
- (ii)
- the infection equilibrium with cellular immunitythat is globally asymptotically stable if.
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Nowak, M.A.; Bangham, C.R.M. Population dynamics of immune responses to persistent viruses. Science 1996, 272, 74–79. [Google Scholar] [CrossRef]
- Ebert, D.; Zschokke-Rohringer, C.D.; Carius, H.J. Dose effects and density-dependent regulation of two microparasites of Daphnia magna. Oecologia 2000, 122, 200–209. [Google Scholar] [CrossRef] [PubMed]
- Mclean, A.R.; Bostock, C.J. Scrapie infections initiated at varying doses: An analysis of 117 titration experiments. Philos. Trans. Roy. Soc. London Ser. B 2000, 355, 1043–1050. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Tao, Y.; Song, X. Global stability of a virus dynamics model with Beddington-DeAngelis incidence rate and CTL immune response. Nonlinear Dyn. 2011, 66, 825–830. [Google Scholar] [CrossRef]
- Hattaf, K.; Yousfi, N.; Tridane, A. Global stability analysis of a generalized virus dynamics model with the immune response. Can. Appl. Math. Q. 2012, 20, 499–518. [Google Scholar]
- Li, Y.; Xu, R.; Li, Z.; Mao, S. Global dynamics of a delayed HIV-1 infection model with CTL immune response. Discret. Dyn. Nat. Soc. 2011, 2011, 673843. [Google Scholar] [CrossRef]
- Li, X.; Fu, S. Global stability of a virus dynamics model with intracellular delay and CTL immune response. Math. Methods Appl. Sci. 2015, 38, 420–430. [Google Scholar] [CrossRef]
- Wang, J.; Guo, M.; Liu, X.; Zhao, Z. Threshold dynamics of HIV-1 virus model with cell-to-cell transmission, cell-mediated immune responses and distributed delay. Appl. Math. Comput. 2016, 291, 149–161. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Raezah, A.A.; Hattaf, K. Stability of HIV-1 infection with saturated virus-target and infected-target incidences and CTL immune response. Int. J. Biomath. 2017, 10, 1–29. [Google Scholar] [CrossRef]
- Hattaf, K.; Yousfi, N. A generalized virus dynamics model with cell-to-cell transmission and cure rate. Adv. Differ. Equ. 2016, 2016, 174. [Google Scholar] [CrossRef][Green Version]
- Hattaf, K.; Yousfi, N. Qualitative analysis of a generalized virus dynamics model with both modes of transmission and distributed delays. Int. J. Differ. Equ. 2018, 2018, 9818372. [Google Scholar] [CrossRef]
- Hattaf, K.; Yousfi, N. A numerical method for a delayed viral infection model with general incidence rate. J. King Saud Univ.-Sci. 2016, 28, 368–374. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Hattaf, K.; Huo, H.-F.; Xiang, H. Stability analysis of a delayed social epidemics model with general contact rate and its optimal control. J. Ind. Manag. Optim. 2016, 12, 1267–1285. [Google Scholar] [CrossRef]
- Strain, M.C.; Richman, D.D.; Wong, J.K.; Levine, H. Spatiotemporal dynamics of HIV propagation. J. Theor. Biol. 2002, 218, 85–96. [Google Scholar] [CrossRef]
- Funk, G.A.; Jansen, V.A.; Bonhoeffer, S.; Killingback, T. Spatial models of virus-immune dynamics. J. Theor. Biol. 2005, 233, 221–236. [Google Scholar] [CrossRef] [PubMed]
- Cantrell, R.; Cosner, C. Spatial Ecology via Reaction Diffusion Equations; Wiley: New York, NY, USA, 2003. [Google Scholar]
- Wang, S.; Feng, X.; He, Y. Global asymptotical properties for a diffused HBV infection model with CTL immune response and nonlinear incidence. Acta Math. Sci. 2011, 31, 1959–1967. [Google Scholar]
- Yang, Y.; Xu, Y. Global stability of a diffusive and delayed virus dynamics model with Beddington-DeAngelis incidence function and CTL immune response. Comput. Math. Appl. 2016, 71, 922–930. [Google Scholar] [CrossRef]
- Kang, C.; Miao, H.; Chen, X.; Xu, J.; Huang, D. Global stability of a diffusive and delayed virus dynamics model with Crowley-Martin incidence function and CTL immune response. Adv. Differ. Equ. 2017. [Google Scholar] [CrossRef]
- Protter, M.H.; Weinberger, H.F. Maximum Principles in Differential Equations; Prentice Hall: Englewood Cliffs, NJ, USA, 1967. [Google Scholar]
- Travis, C.C.; Webb, G.F. Existence and stability for partial functional differential equations. Trans. Am. Math. Soc. 1974, 200, 395–418. [Google Scholar] [CrossRef]
- Fitzgibbon, W.E. Semilinear functional differential equations in Banach space. J. Differ. Equ. 1978, 29, 1–14. [Google Scholar] [CrossRef]
- Martin, R.H.; Smith, H.L. Abstract functional differential equations and reaction-diffusion systems. Trans. Am. Math. Soc. 1990, 321, 1–44. [Google Scholar]
- Martin, R.H.; Smith, H.L. Reaction-diffusion systems with time delays: Monotonicity, invariance, comparison and convergence. J. Reine Angew. Math. 1991, 413, 1–35. [Google Scholar]
- Wu, J. Theory and Applications of Partial Functional Differential Equations; Springer: New York, NY, USA, 1996. [Google Scholar]
- Henry, D. Geometric Theory of Semilinear Parabolic Equations; Lecture Notes in Mathematics; Springer: Berlin, Germany; New York, NY, USA, 1993; Volume 840. [Google Scholar]
- Hattaf, K.; Yousfi, N. Modeling the adaptive immunity and both modes of transmission in HIV infection. Computation 2018, 6, 37. [Google Scholar] [CrossRef]
- Hattaf, K.; Yousfi, N. Global stability for reaction-diffusion equations in biology. Comput. Math. Appl. 2013, 66, 1488–1497. [Google Scholar] [CrossRef]
- Hale, J.K.; Verduyn Lunel, S.M. Introduction to Functional Differential Equations; Springer: New York, NY, USA, 1993. [Google Scholar]
- Xu, J.; Geng, Y.; Hou, J. Global dynamics of a diffusive and delayed viral infection model with cellular infection and nonlinear infection rate. Comput. Math. Appl. 2017, 73, 640–652. [Google Scholar] [CrossRef]
- Sun, H.; Wang, J. Dynamics of a diffusive virus model with general incidence function, cell-to-cell transmission and time delay. Comput. Math. Appl. 2019, 77, 284–301. [Google Scholar] [CrossRef]


| Parameter | Value | Parameter | Value |
|---|---|---|---|
| 10 | |||
| d | |||
| k | 50 | ||
| a | b | ||
| p | |||
| 3 | |||
| Varied | |||
| c | Varied |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hattaf, K. Spatiotemporal Dynamics of a Generalized Viral Infection Model with Distributed Delays and CTL Immune Response. Computation 2019, 7, 21. https://doi.org/10.3390/computation7020021
Hattaf K. Spatiotemporal Dynamics of a Generalized Viral Infection Model with Distributed Delays and CTL Immune Response. Computation. 2019; 7(2):21. https://doi.org/10.3390/computation7020021
Chicago/Turabian StyleHattaf, Khalid. 2019. "Spatiotemporal Dynamics of a Generalized Viral Infection Model with Distributed Delays and CTL Immune Response" Computation 7, no. 2: 21. https://doi.org/10.3390/computation7020021
APA StyleHattaf, K. (2019). Spatiotemporal Dynamics of a Generalized Viral Infection Model with Distributed Delays and CTL Immune Response. Computation, 7(2), 21. https://doi.org/10.3390/computation7020021





