Numerical Study on Sloshing Characteristics with Reynolds Number Variation in a Rectangular Tank
Abstract
:1. Introduction
2. Analysis Model and Methods
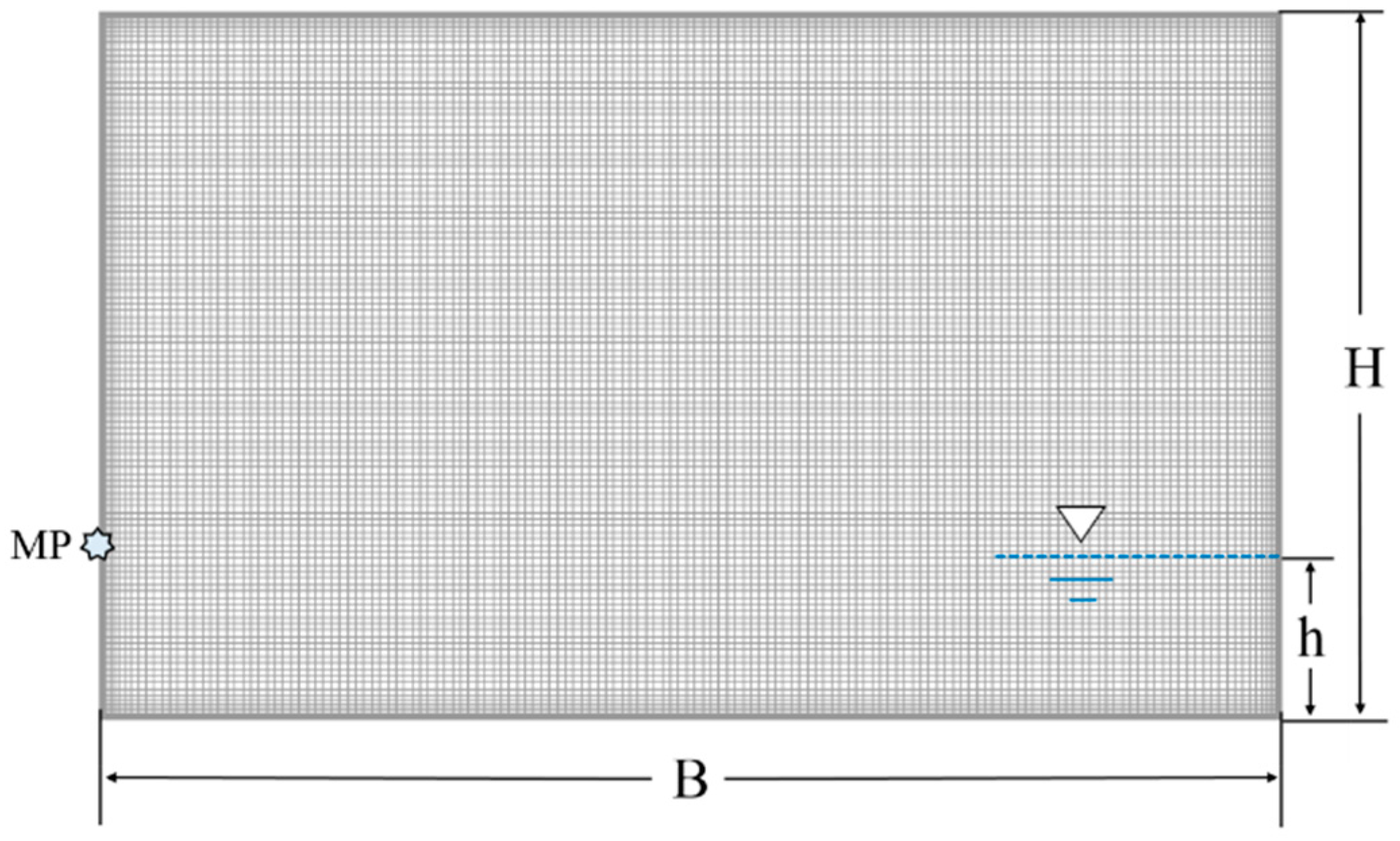
2.1. Computational Domain and Boundary Conditions
2.2. Governing Equations
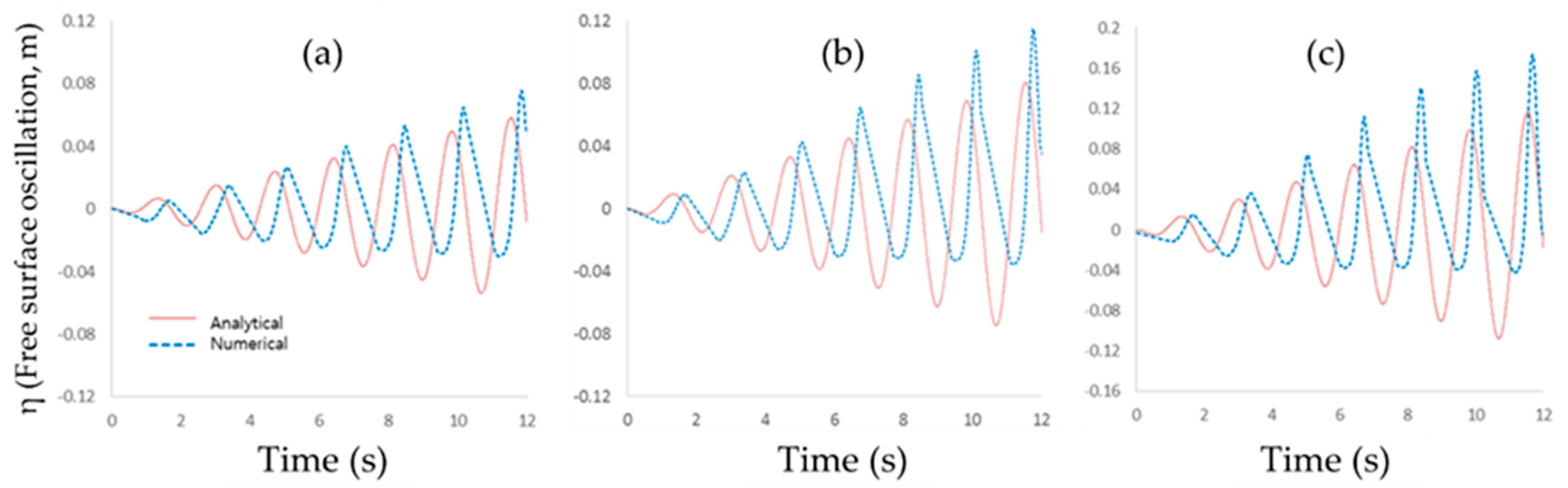
2.3. Analytical Solution for Linear Sloshing
2.4. Discussion of the Numerical Model with Inhomogeneous VOF
3. Results and Discussion
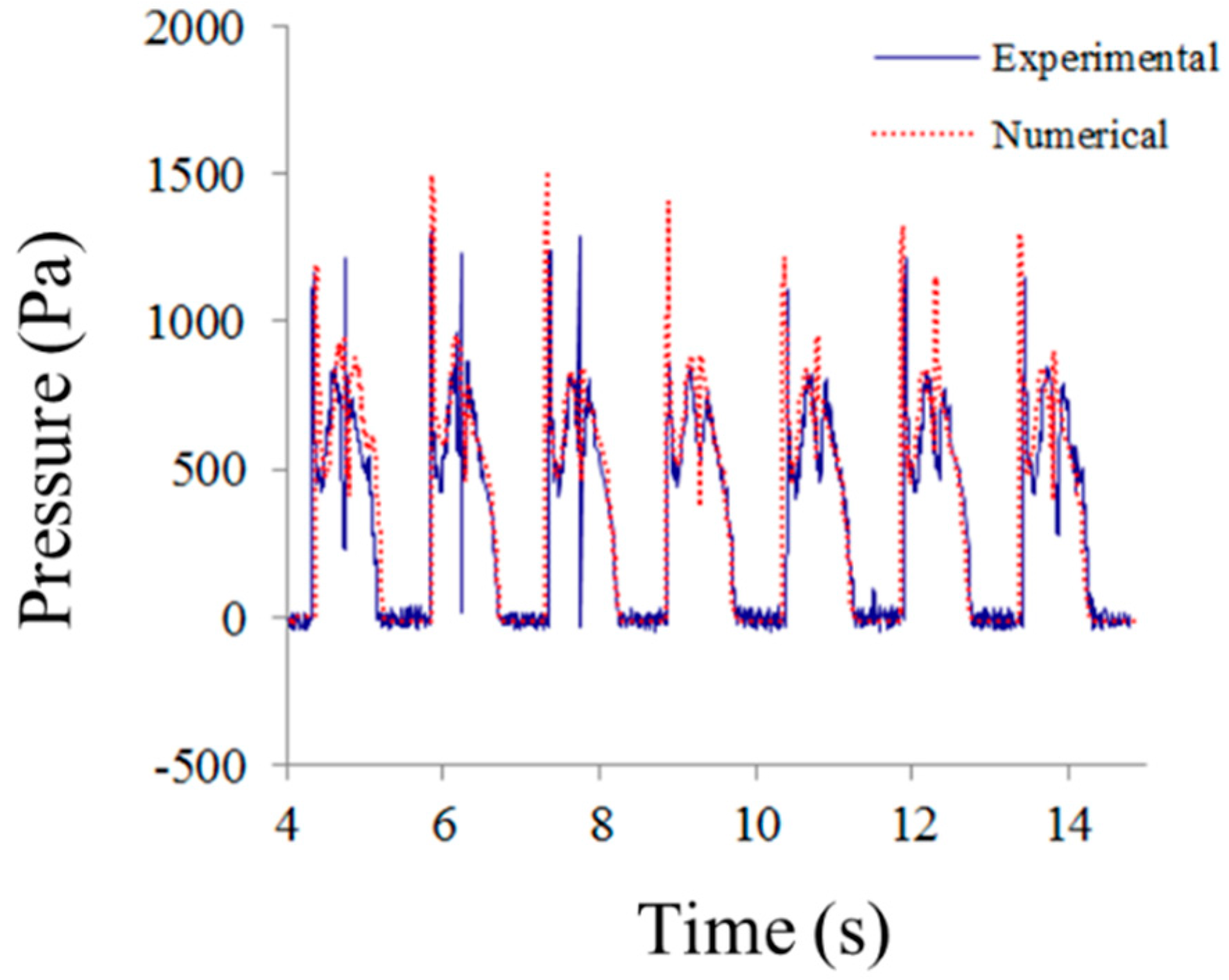
3.1. Observing Nonlinearity of Sloshing Flows
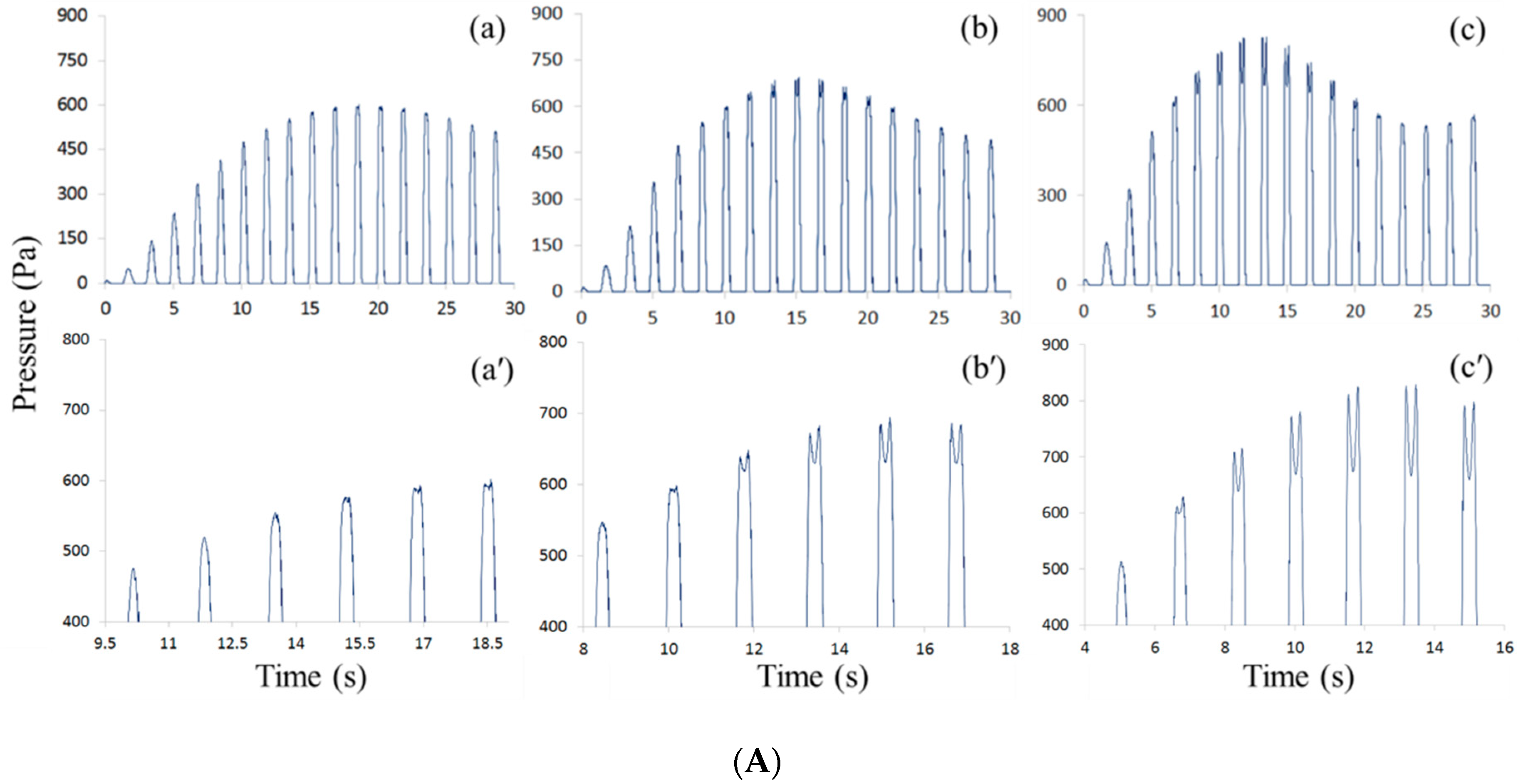
3.2. Sloshing Characteristics with Reynolds Number Variation
3.3. Comparison of Sloshing Characteristics by FFT Analysis
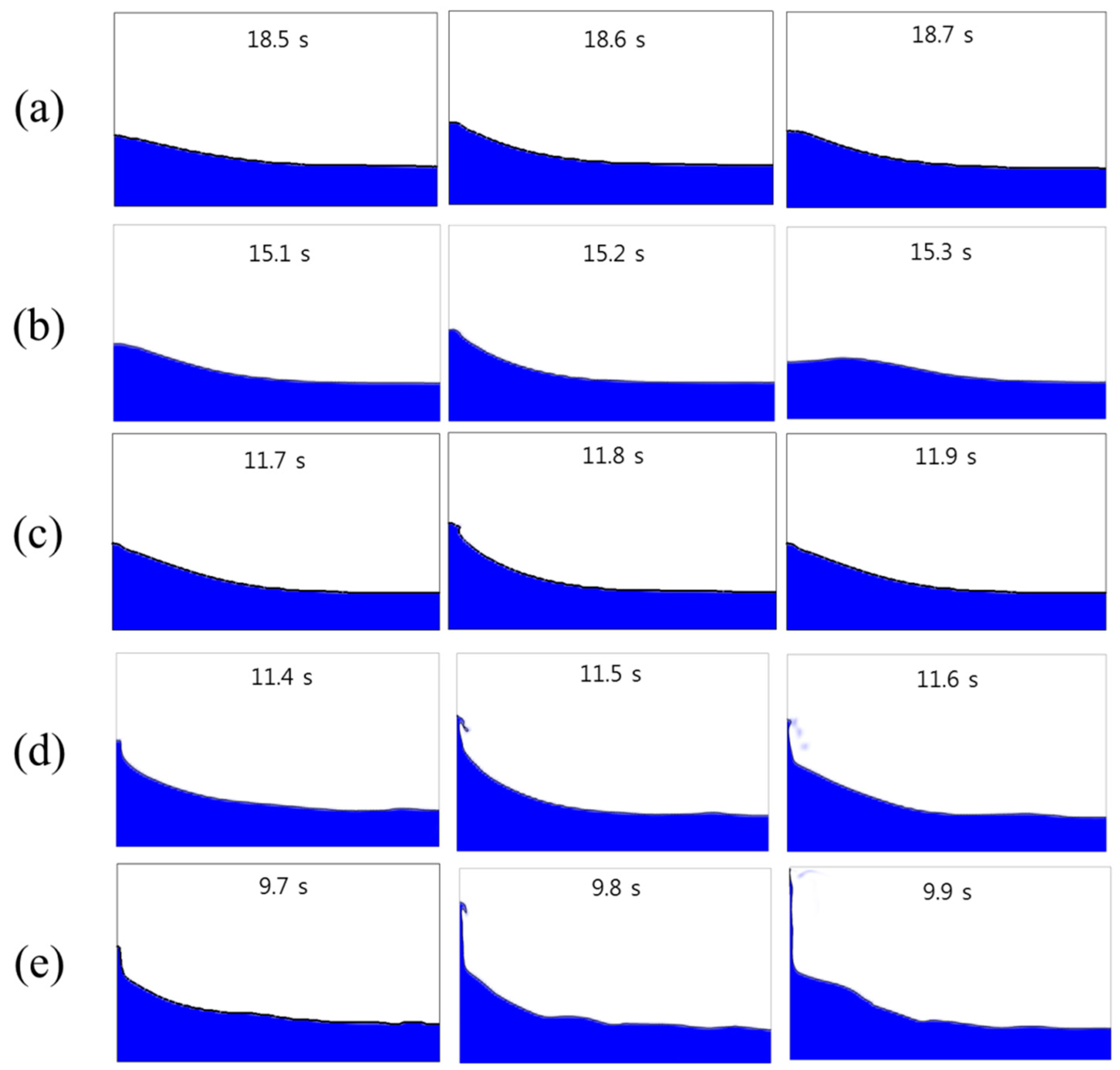
3.4. Visual Observation on the Sloshing Impact Motion
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Xue, M.-A.; Lin, P. Numerical study of ring baffle effects on reducing violent liquid sloshing. Comput. Fluids 2011, 52, 116–129. [Google Scholar] [CrossRef]
- Wang, W.; Guo, Z.; Peng, Y.; Zhang, Q. A numerical study of the effects of the T-shaped baffles on liquid sloshing in horizontal elliptical tanks. Ocean Eng. 2016, 111, 543–568. [Google Scholar] [CrossRef]
- Liu, D.; Tang, W.; Wang, J.; Xue, H.; Wang, K. Comparison of laminar model, RANS, LES and VLES for simulation of liquid sloshing. Appl. Ocean Res. 2016, 59, 638–649. [Google Scholar] [CrossRef]
- Godderidge, B. A Phenomenological Rapid Sloshing Model for Use as an Operator Guidance System on Liquefied Natural Gas Carriers. Doctoral Dissertation, University of Southampton, Southampton, UK, 2009. [Google Scholar]
- Kim, Y. Numerical simulation of sloshing flows with impact load. Appl. Ocean Res. 2001, 23, 53–62. [Google Scholar] [CrossRef]
- Chen, B.-F.; Nokes, R. Time-independent finite difference analysis of fully non-linear and viscous fluid sloshing in a rectangular tank. J. Comput. Phys. 2005, 209, 47–81. [Google Scholar] [CrossRef]
- Wu, C.-H.; Chen, B.-F.; Hung, T.-K. Hydrodynamic forces induced by transient sloshing in a 3D rectangular tank due to oblique horizontal excitation. Comput. Math. Appl. 2013, 65, 1163–1186. [Google Scholar] [CrossRef]
- Arafa, M. Finite element analysis of sloshing in rectangular liquid-filled tanks. J. Vib. Control 2007, 13, 883–903. [Google Scholar] [CrossRef]
- Mitra, S.; Upadhyay, P.P.; Sinhamahapatra, K.P. Slosh dynamics of inviscid fluids in two-dimensional tanks of various geometry using finite element method. Int. J. Numer. Methods Fluids 2008, 56, 1625–1651. [Google Scholar] [CrossRef]
- Nayak, S.K.; Biswal, K.C. Quantification of seismic response of partially filled rectangular liquid tank with submerged block. J. Earthq. Eng. 2013, 17, 1023–1062. [Google Scholar] [CrossRef]
- Chen, Y.G.; Djidjeli, K.; Price, W.G. Numerical simulation of liquid sloshing phenomena in partially filled containers. Comput. Fluids 2009, 38, 830–842. [Google Scholar] [CrossRef]
- Akyildız, H.; Ünal, N.E. Sloshing in a three-dimensional rectangular tank: Numerical simulation and experimental validation. Ocean Eng. 2006, 33, 2135–2149. [Google Scholar] [CrossRef]
- Li, J.G.; Hamamoto, Y.; Liu, Y.; Zhang, X. Sloshing impact simulation with material point method and its experimental validations. Comput. Fluids 2014, 103, 86–99. [Google Scholar] [CrossRef]
- Birknes-Berg, J.; Pedersen, G.K. The “Chain of Markers” Code Applied to Large Scale Problems; Solitary Waves, Sloshing and a Plunging Wave. Research Report in Mechanics. 2017. Available online: http://urn.nb.no/URN:NBN:no-23419 (accessed on 1 September 2017).
- Fox, D.W.; Kuttler, J.R. Sloshing frequencies, Zeitschrift für Angewandte Mathematik und Physik (ZAMP). J. Appl. Math. Phys. 1983, 34, 668–696. [Google Scholar]
- Lee, D.; Kim, M.H.; Kwon, S.H.; Kim, J.W.; Lee, Y.B. A parametric sensitivity study on LNG tank sloshing loads by numerical simulations. Ocean Eng. 2007, 34, 3–9. [Google Scholar] [CrossRef]
- Godderidge, B.; Turnock, S.; Tan, M.; Earl, C. An investigation of multiphase CFD modelling of a lateral sloshing tank. Comput. Fluids 2009, 38, 183–193. [Google Scholar] [CrossRef]
- Faltinsen, O.M. A numerical nonlinear method of sloshing in tanks with two-dimensional flow. J. Ship Res. 1978, 22, 193–202. [Google Scholar]
- Liu, D.; Lin, P. A numerical study of three-dimensional liquid sloshing in tanks. J. Comput. Phys. 2008, 227, 3921–3939. [Google Scholar] [CrossRef]
- Frandsen, J.B. Sloshing motions in excited tanks. J. Comput. Phys. 2004, 196, 53–87. [Google Scholar] [CrossRef]
- Parthasarathty, N.; Kim, H.; Choi, Y.H.; Lee, Y.W. A numerical study on sloshing impact loads in prismatic tanks under forced horizontal motion. J. Korean Soc. Mar. Eng. 2017, 41, 150–155. [Google Scholar] [CrossRef]
- Kim, H.; Choi, Y.-H.; Lee, Y.-W. Numerical analysis of sloshing impact in horizontally excited prismatic tanks. Prog. Comput. Fluid Dyn. 2017, 17, 361–367. [Google Scholar] [CrossRef]
- Kishev, Z.R.; Hu, C.; Kashiwagi, M. Numerical simulation of violent sloshing by a CIP-based method. J. Mar. Sci. Technol. 2006, 11, 111–122. [Google Scholar] [CrossRef]



| Case | Reynolds No. | Natural Frequency (ω1, s−1) | Excited Frequency (Hz) |
|---|---|---|---|
| 1 | 1.8 × 104 | 3.68 | 0.586 |
| 2 | 2.5 × 104 | ||
| 3 | 3.6 × 104 | ||
| 4 | 5.0 × 104 | ||
| 5 | 7.2 × 104 |
| Case | S |
|---|---|
| 1 | 1.111 |
| 2 | 1.567 |
| 3 | 1.759 |
| Case | Max. Pressure Fluctuation |
|---|---|
| No. | Pa |
| 1 (Re 1.8 × 104) | 15 |
| 2 (Re 2.5 × 104) | 64 |
| 3 (Re 3.6 × 104) | 162 |
| 4 (Re 5.0 × 104) | 315 |
| 5 (Re 7.2 × 104) | 789 |
| Case | PMax | Max. Amplitude Spectrum | |
|---|---|---|---|
| No. | Pa | Second | - |
| 1 (Re 1.8 × 104) | 602 | 18.5 | 1682 |
| 2 (Re 2.5 × 104) | 695 | 15.1 | 1994 |
| 3 (Re 3.6 × 104) | 829 | 11.7 | 2317 |
| 4 (Re 5.0 × 104) | 1020 | 11.4 | 2748 |
| 5 (Re 7.2 × 104) | 1520 | 9.7 | 3430 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, H.; Dey, M.K.; Oshima, N.; Lee, Y.W. Numerical Study on Sloshing Characteristics with Reynolds Number Variation in a Rectangular Tank. Computation 2018, 6, 53. https://doi.org/10.3390/computation6040053
Kim H, Dey MK, Oshima N, Lee YW. Numerical Study on Sloshing Characteristics with Reynolds Number Variation in a Rectangular Tank. Computation. 2018; 6(4):53. https://doi.org/10.3390/computation6040053
Chicago/Turabian StyleKim, Hyunjong, Mohan Kumar Dey, Nobuyuki Oshima, and Yeon Won Lee. 2018. "Numerical Study on Sloshing Characteristics with Reynolds Number Variation in a Rectangular Tank" Computation 6, no. 4: 53. https://doi.org/10.3390/computation6040053
APA StyleKim, H., Dey, M. K., Oshima, N., & Lee, Y. W. (2018). Numerical Study on Sloshing Characteristics with Reynolds Number Variation in a Rectangular Tank. Computation, 6(4), 53. https://doi.org/10.3390/computation6040053




