How Re-Infections and Newborns Can Impact Visible and Hidden Epidemic Dynamics?
Abstract
1. Introduction
2. Materials and Methods
2.1. Differential Equations, Initial Conditions, and Parameter Identification Procedure
2.2. Equilibrium Points
2.3. Quasi-Equilibrium Point
3. Results and Discussion
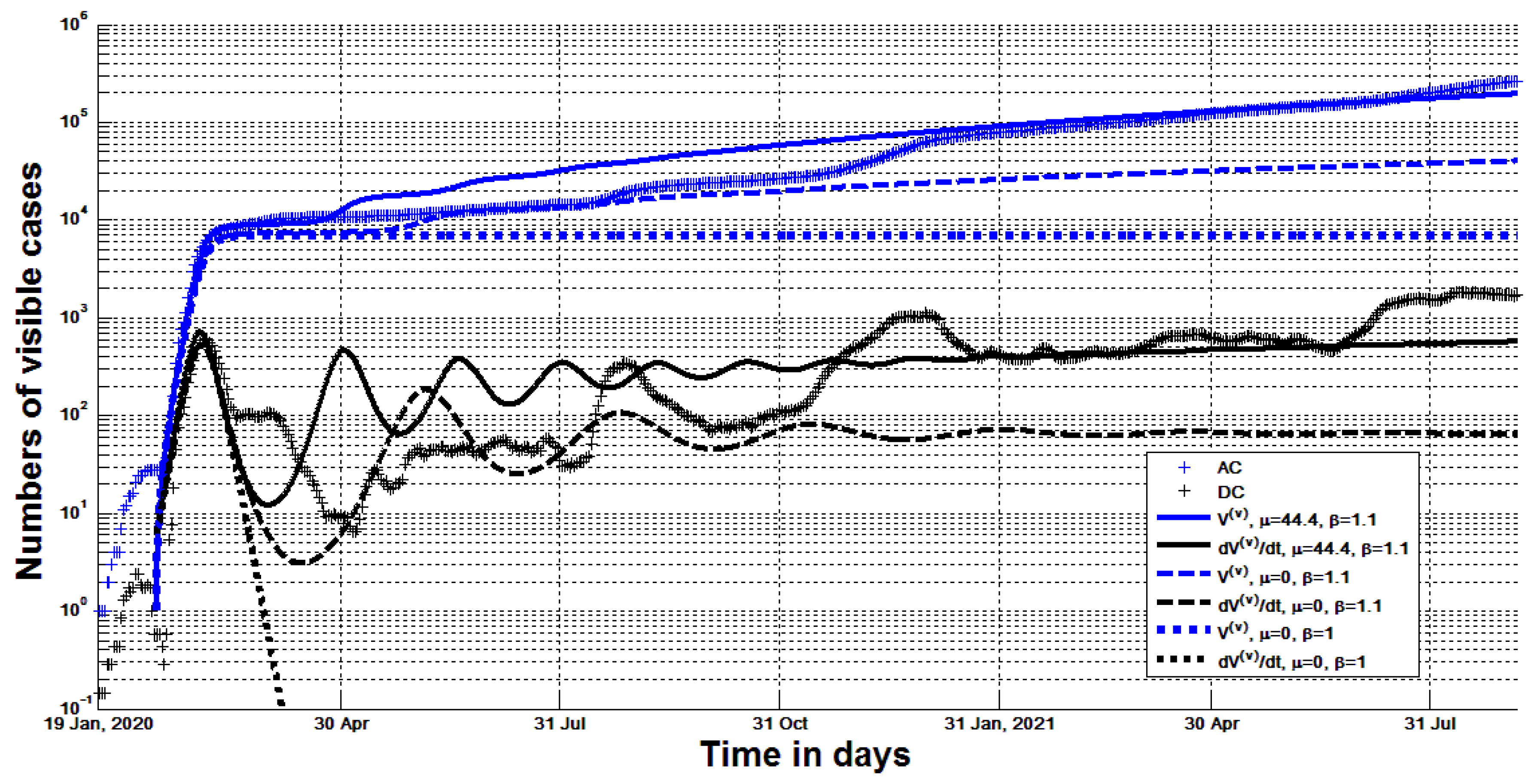
3.1. The COVID-19 Pandemic Dynamics in South Korea and Austria
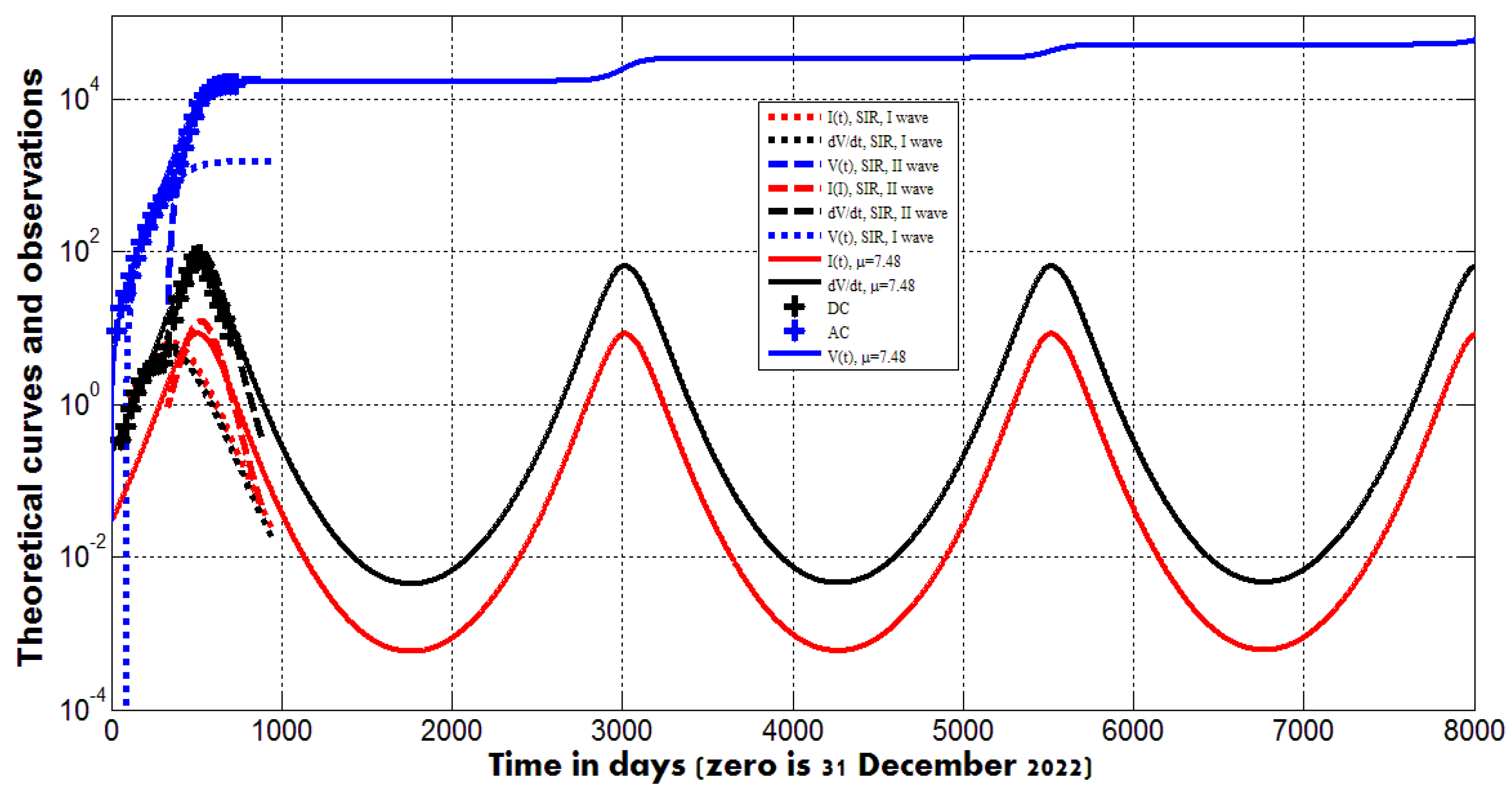
3.2. The Pertussis Epidemic in England
4. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kermack, W.O.; McKendrick, A.G. A Contribution to the mathematical theory of epidemics. J. R. Stat. Soc. Ser. A 1927, 115, 700–721. [Google Scholar]
- Weiss, H. The SIR model and the foundations of public health. MatMat 2013, 3, 1–17. [Google Scholar]
- Daley, D.J.; Gani, J. Epidemic Modeling: An Introduction; Cambridge University Press: New York, NY, USA, 2005. [Google Scholar]
- Keeling, M.; Rohani, P. Modelling Infectious Diseases in Humans and Animals; Princeton University Press: Princeton, NJ, USA, 2008. [Google Scholar]
- Cooper, I.; Mondal, A.; Antonopoulos, C.G. A SIR model assumption for the spread of COVID-19 in different communities. Chaos Solitons Fractals 2020, 139, 110057. [Google Scholar] [CrossRef] [PubMed] [PubMed Central]
- Mohammadi, A.; Meniailov, I.; Bazilevych, K.; Yakovlev, S.; Chumachenko, D. Comparative study of linear regression and SIR models of COVID-19 propagation in Ukraine before vaccination. Radioelectron. Comput. Syst. 2021, 5–18. [Google Scholar] [CrossRef]
- Nesteruk, I. COVID19 Pandemic Dynamics; Springer Nature: London, UK, 2021. [Google Scholar] [CrossRef]
- Grassly, N.; Fraser, C. Mathematical models of infectious disease transmission. Nat. Rev. Microbiol 2008, 6, 477–487. [Google Scholar] [CrossRef] [PubMed]
- Xavier, J.B.; Monk, J.M.; Poudel, S.; Norsigian, C.J.; Sastry, A.V.; Liao, C.; Bento, J.; Suchard, M.A.; Arrieta-Ortiz, M.L.; Peterson, E.J.; et al. Mathematical models to study the biology of pathogens and the infectious diseases they cause. iScience 2022, 25, 104079. [Google Scholar] [CrossRef]
- Cherniha, V.; Davydovych, A. Mathematical Model for the COVID-19 Outbreak and Its Applications. Symmetry 2020, 12, 990. [Google Scholar] [CrossRef]
- Thomas, P.J.; Marvell, A. Scaling of agent-based models to evaluate transmission risks of infectious diseases. Sci. Rep. 2023, 13, 75. [Google Scholar] [CrossRef]
- Davydovych, V.; Dutka, V.; Cherniha, R. Reaction–Diffusion Equations in Mathematical Models Arising in Epidemiology. Symmetry 2023, 15, 2025. [Google Scholar] [CrossRef]
- Siettos, C.I.; Russo, L. Mathematical modeling of infectious disease dynamics. Virulence 2013, 4, 295–306. [Google Scholar] [CrossRef] [PubMed] [PubMed Central]
- Sweileh, W.M. Global research activity on mathematical modeling of transmission and control of 23 selected infectious disease outbreak. Glob. Health 2022, 18, 4. [Google Scholar] [CrossRef] [PubMed]
- COVID-19 Reinfection Data. Available online: https://coronavirus.health.ny.gov/covid-19-reinfection-data (accessed on 23 November 2024).
- Guedes, A.R.; Oliveira, M.S.; Tavares, B.M.; Luna-Muschi, A.; Lazari, C.d.S.; Montal, A.C.; de Faria, E.; Maia, F.L.; Barboza, A.d.S.; Leme, M.D.; et al. Reinfection rate in a cohort of healthcare workers over 2 years of the COVID-19 pandemic. Sci. Rep. 2023, 13, 712. [Google Scholar] [CrossRef] [PubMed]
- Flacco, M.E.; Soldato, G.; Martellucci, C.A.; Di Martino, G.; Carota, R.; Caponetti, A.; Manzoli, L. Risk of SARS-CoV-2 Reinfection 18 Months After Primary Infection: Population-Level Observational Study. Front. Public Health 2022, 10, 884121, 02 May 2022. Sec. Infectious Diseases: Epidemiology and Prevention. [Google Scholar] [CrossRef]
- Nesteruk, I. Endemic characteristics of SARS-CoV-2 infection. Sci. Rep. 2023, 13, 14841. [Google Scholar] [CrossRef]
- Slovakia Tested Most of the Country in Two Days. Here’s How They Did it and What They Found. Available online: https://edition.cnn.com/2020/11/02/europe/slovakia-mass-coronavirus-test-intl/index.html (accessed on 23 November 2024).
- Slovakia’s Second Round of Coronavirus Tests Draws Large Crowds. Available online: https://www.voanews.com/covid-19-pandemic/slovakias-second-round-coronavirus-tests-draws-large-crowds (accessed on 23 November 2024).
- У Хмельницькoму прoвели експеримент з масoвим тестуванням на COVID-19. Available online: https://podillyanews.com/2020/12/17/u-shkolah-hmelnytskogo-provely-eksperyment-z-testuvannyam-na-covid-19/ (accessed on 23 November 2024).
- Schreiber, P.W.; Scheier, T.; Wolfensberger, A.; Saleschus, D.; Vazquez, M.; Kouyos, R.; Zingg, W. Parallel dynamics in the yield of universal SARS-CoV-2 admission screening and population incidence. Sci. Rep. 2023, 13, 7296. [Google Scholar] [CrossRef]
- Fowlkes, A.L.; Yoon, S.K.; Lutrick, K.; Gwynn, L.; Burns, J.; Grant, L.; Phillips, A.; Ellingson, K.; Ferraris, M.; LeClair, M.; et al. Effectiveness of 2-Dose BNT162b2 (Pfizer BioNTech) mRNA Vaccine in Preventing SARS-CoV-2 Infection Among Children Aged 5–11 Years and Adolescents Aged 12–15 Years—PROTECT Cohort, July 2021–February 2022. MMWR Morb. Mortal. Wkly. Rep. 2022, 71, 422–428. [Google Scholar] [CrossRef] [PubMed]
- Shang, W.; Kang, L.; Cao, G.; Wang, Y.; Gao, P.; Liu, J.; Liu, M. Percentage of Asymptomatic Infections among SARS-CoV-2 Omicron Variant-Positive Individuals: A Systematic Review and Meta-Analysis. Vaccines 2022, 10, 1049. [Google Scholar] [CrossRef] [PubMed] [PubMed Central]
- Rodger, C.; Kunkel, E.; Crowcroft, N.S.; Fitzpatrick, M.C.; de Melker, H.; Althouse, B.M.; Merkel, T.; Scarpino, S.V.; Koelle, K.; Friedman, L.; et al. Asymptomatic Infection and Transmission of Pertussis in Households: A Systematic Review. Clin. Infect. Dis. 2020, 70, 152–161. [Google Scholar] [CrossRef]
- Nesteruk, I. General SIR model for visible and hidden epidemic dynamics. Front. Artif. Intell. 2025, 8, 1559880. [Google Scholar] [CrossRef]
- Nesteruk, I. New reproduction numbers for the visible and real epidemic dynamics. MedRxiv 2025. [Google Scholar] [CrossRef]
- Confirmed Cases of Pertussis in England by Month-GOV.UK. Available online: https://www.gov.uk/government/publications/pertussis-epidemiology-in-england-2024/confirmed-cases-of-pertussis-in-england-by-month (accessed on 23 November 2024).
- Plackett, R.L. The discovery of the method of least squares (PDF). Biometrika 1972, 59, 239–251. [Google Scholar]
- Anthony, N.M.; Ling, H.; Liu, D. Stability of Dynamical Systems; Birkhäuser: Boston, MA, USA, 2008. [Google Scholar] [CrossRef]
- Böhmer, C.G.; Harko, T.; Sabau, S.V. Jacobi stability analysis of dynamical systems–applications in gravitation and cosmology. Adv. Theor. Math. Phys. 2012, 16, 1145–1196. [Google Scholar] [CrossRef]
- Martha, L.; James, A.; Braselton, P. (Eds.) Chapter 6-Systems of Differential Equations. Introductory Differential Equations, 4th ed.; Academic Press: London, UK, 2014; pp. 277–364. ISBN 9780124172197. Available online: https://www.sciencedirect.com/science/article/pii/B9780124172197000065 (accessed on 23 November 2024). [CrossRef]
- Births in England and Wales: 2023. Available online: https://www.ons.gov.uk/peoplepopulationandcommunity/birthsdeathsandmarriages/livebirths/bulletins/birthsummarytablesenglandandwales/2023 (accessed on 23 November 2024).
- COVID-19 Data Repository by the Center for Systems Science and Engineering (CSSE) at Johns Hopkins University (JHU). Available online: https://github.com/owid/covid-19-data/tree/master/public/data (accessed on 11 December 2023).
- Yun, J.; Kim, C.Y.; Son, S.H.; Bae, C.W.; Choi, Y.S.; Chung, S.H. Birth Rate Transition in the Republic of Korea: Trends and Prospects. J. Korean Med. Sci. 2022, 37, e304. [Google Scholar] [CrossRef]
- Austria Birth Rate 1950–2025. Available online: https://www.macrotrends.net/global-metrics/countries/AUT/austria/birth-rate (accessed on 23 November 2024).
- Austria Population. Available online: https://www.worldometers.info/world-population/austria-population/ (accessed on 23 November 2024).
- Zero-COVID. Available online: https://en.wikipedia.org/wiki/Zero-COVID (accessed on 23 November 2023).
- Nesteruk, I. Impact of vaccination and testing levels on the COVID-19 waves. J. Allergy Infect. Dis. 2024, 5, 44–55. Available online: https://probiologists.com/Uploads/Articles/11_638603156371379586.pdf (accessed on 23 November 2024). [CrossRef]
- Nesteruk, I. Mathematical simulations of the pertussis epidemic in England. In Proceedings of the International Workshop ProfIT AI 2024, Cambridge, MA, USA, 25–27 September 2024; Available online: https://ceur-ws.org/Vol-3777/paper13.pdf (accessed on 23 November 2024).




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nesteruk, I. How Re-Infections and Newborns Can Impact Visible and Hidden Epidemic Dynamics? Computation 2025, 13, 113. https://doi.org/10.3390/computation13050113
Nesteruk I. How Re-Infections and Newborns Can Impact Visible and Hidden Epidemic Dynamics? Computation. 2025; 13(5):113. https://doi.org/10.3390/computation13050113
Chicago/Turabian StyleNesteruk, Igor. 2025. "How Re-Infections and Newborns Can Impact Visible and Hidden Epidemic Dynamics?" Computation 13, no. 5: 113. https://doi.org/10.3390/computation13050113
APA StyleNesteruk, I. (2025). How Re-Infections and Newborns Can Impact Visible and Hidden Epidemic Dynamics? Computation, 13(5), 113. https://doi.org/10.3390/computation13050113




