Numerical Methodology for Enhancing Heat Transfer in a Channel with Arc-Vane Baffles
Abstract
1. Introduction
- The influence of various arc-vane baffle designs (r/H = of 0.125, 0.25, 0.375, and 0.5) on flow patterns and heat transfer behavior at Reynolds numbers between 6000 and 24,000.
- The potential of arc-vane baffles to generate secondary flows and vortices.
- The impact of arc-vane baffles on pressure drops, assessing the trade-offs between enhanced heat transfer and increased flow resistance.
- An evaluation of the thermal performance factor (TPF) as a comparative metric for heat transfer efficiency, benchmarked against exchangers without such inserts.
2. Geometry of Arc-Vane Baffles
3. Numerical Method
4. Data Reduction
5. Numerical Results Analysis
5.1. Verification of the Experimental Setup
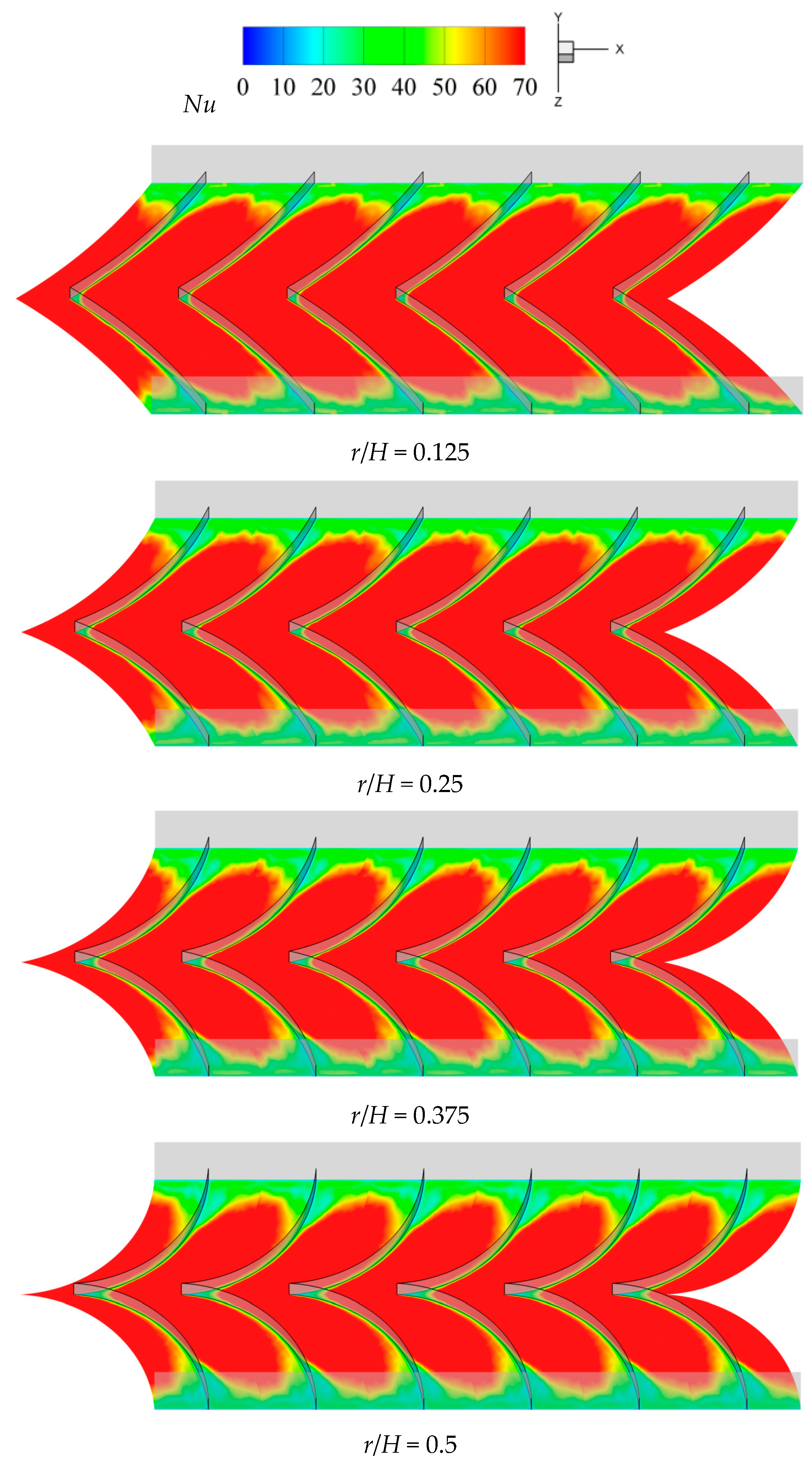
5.2. Heat Transfer and Fluid Flow Mechanisms
5.3. Overall Performance Evaluation
6. Comparison with Related Works
7. Conclusions
- An arc-vane baffle generates double vortices along the axial direction, causing flow reattachment on the heated surface and resulting in a significant enhancement in heat transfer.
- The baffles with smaller r/H ratios generate stronger flow reattachment and improve fluid contact with heat transfer surfaces, as evidenced by reduced dead zone areas. The ones with the smallest r/H yield a Nu/Nus ratio of up to 4.91 at the lowest Reynolds numbers (Re) of 6000.
- The friction factor and friction factor ratio increase as the r/H ratio rises, attributed to the greater curvature and surface area of each baffle, which creates more flow obstruction within the channel.
- The arc-vane baffles with r/H = 0.125 offer TPF greater than unity across the studied Re (6000 ≤ Re ≤ 24,000). Within the range studied, the TPF reaches a maximum of 2.28 at r/H = 0.125 and Re = 6000, reflecting the optimal balance between high heat transfer and manageable friction losses. This suggests that using the arc-vane baffles leads to overall energy savings.
- The arc-vane baffles with varying r/H ratios significantly influence heat transfer, pressure drop, and thermal performance characteristics, requiring size optimization for future development. In future work, we can attempt to modify the arc-vane baffles at r/H = 0.125 to decrease the pressure drop while enhancing the thermal performance factor by incorporating a hole in the baffle to promote enhanced fluid mixing between the fluid in the channel and the hot channel wall.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | heat transfer area, mm2 |
| Ac | cross-section area, mm2 |
| BR | blockage ratio, dimensionless |
| Dh | hydraulic diameter, mm |
| e | baffle height, mm |
| f | friction factor, dimensionless |
| fs | friction factor for the smooth channel |
| Gk | generation of turbulent kinetic energy, m2s−3 |
| H | channel height, mm |
| h | convective heat transfer coefficient, W m−2 K−1 |
| k | turbulent kinetic energy, m2 s–2 |
| L | channel length, mm |
| Nu | average Nusselt number, dimensionless |
| Nus | average Nusselt number for smooth channel, dimensionless |
| ΔP | static pressure, Pa |
| P | baffle pitch, m |
| Pc | wetted perimeter, m |
| Pr | Prandtl number, dimensionless |
| convection heat flux, W m−2 | |
| heat flux input, W m−2 | |
| r | radius of arc-vane baffle, mm |
| Re | Reynolds number, dimensionless |
| T | temperature, K |
| Tm | average air temperature, K |
| Tw | wall temperature, K |
| TPF | thermal performance factor, dimensionless |
| u | velocity, m s−1 |
| mean velocity, m s−1 | |
| friction velocity, m2 s–2 | |
| y | y–position or distance from the wall, mm |
| y+ | distance from the first near-wall cell center to the wall, dimensionless |
| Greek letters | |
| μ | dynamic viscosity, kg s−1 m−1 |
| μt | turbulent dynamic viscosity, kg s−1 m−1 |
| Γ | molecular thermal diffusivity, kg s−1 m−1 |
| Γt | turbulent thermal diffusivity, kg s−1 m−1 |
| ε | turbulent dissipation rate, m2 s–3 |
| ρ | density, kg m−3 |
| wall shear stress, N m–2 | |
| Subscripts | |
| s | without turbulator/smooth channel |
| t | turbulator component |
| a | air |
References
- Bhowmick, S.; Roy, L.R.; Xu, F.; Saha, S.C. Natural convection fluid flow and heat transfer in a valley-shaped cavity. Computation 2024, 12, 146. [Google Scholar] [CrossRef]
- Chamoli, S.; Lu, R.; Yu, P. Thermal characteristic of a turbulent flow through a circular tube fitted with perforated vortex generator inserts. Appl. Therm. Eng. 2017, 121, 1117–1134. [Google Scholar] [CrossRef]
- Reddy, G.J.; Kumar, V.G.; Kumar, M.A. The pdepe solver for analysing the flow of MHD Cu–H2O nanofluid across an oscillating vertical plate. Case Stud. Chem. Environ. Eng. 2024, 10, 100910. [Google Scholar]
- Wan, T.; Zhao, P.; Chen, Z.; Jin, Y.; Li, Y.; Peng, C. Study of the hydraulic roughness impact on turbulent heat transfer at supercritical pressure based on a fast direct numerical simulation method. Int. J. Heat. Mass. Transf. 2023, 217, 124647. [Google Scholar] [CrossRef]
- Ayoob, H.W.; Omar, I.; Ghanim, W.K.; Fares, M.N.; Fazilati, M.A.; Salahshour, S.; Esmaeili, S. The thermal-flow performance of water-Al2O3 nanofluid flow in an elliptical duct heat exchanger equipped with two rotating twisted tapes. Case Stud. Chem. Environ. Eng. 2025, 11, 101094. [Google Scholar] [CrossRef]
- Thianpong, C.; Promvonge, P.; Skullong, S.; Promthaisong, P.; Nakhchi, M.E. Numerical heat transfer study of square duct equipped with novel flapped V-baffles. Int. J. Therm. Sci. 2024, 197, 108819. [Google Scholar] [CrossRef]
- Bezbaruah, P.J.; Das, R.S.; Sarkar, B.K. Experimental and numerical analysis of solar air heater accoutered with modified conical vortex generators in a staggered fashion. Renew. Energy 2021, 180, 109–131. [Google Scholar] [CrossRef]
- Guérin, L.; Flageul, C.; Cordier, L.; Grieu, S.; Agostini, L. Preferential enhancement of convective heat transfer over drag via near-wall turbulence manipulation using spanwise wall oscillations. Int. J. Heat. Fluid. Fl. 2024, 110, 109564. [Google Scholar] [CrossRef]
- Poncet, C.; Ferrouillat, S.; Vignal, L.; Courouble, A.; Bulliard-Sauret, O.; Gondrexon, N. Ultrasonically-enhanced convective heat transfer: Evidence of a relationship with the thermal boundary layer. Appl. Therm. Eng. 2022, 216, 119069. [Google Scholar] [CrossRef]
- Patankar, S.V.; Liu, C.H.; Sparrow, E.M. Fully developed flow and heat transfer in ducts having streamwise-periodic variations of cross-sectional area. J. Heat. Transf. 1977, 99, 180–186. [Google Scholar] [CrossRef]
- Kelkar, K.M.; Patankar, S.V. Numerical prediction of flow and heat transfer in a parallel plate channel with staggered fins. J. Heat. Transf. 1987, 109, 25–30. [Google Scholar] [CrossRef]
- Webb, B.W.; Ramadhyani, S. Conjugate heat transfer in a channel with staggered ribs. Int. J. Heat. Mass. Transf. 1985, 28, 1679–1687. [Google Scholar] [CrossRef]
- Wu, H.; Yang, S.; Wang, E.; Cao, R.; Fernandes, A.C.; Yin, X.; Xiao, Q. Three-dimensional numerical analysis of a vertical axis autorotation current turbine (VAACT): Effects of lateral blockage and free surface. Appl. Ocean. Res. 2024, 150, 104138. [Google Scholar] [CrossRef]
- Salmi, M.; Menni, Y.; Chamkha, A.J.; Ameur, H.; Maouedj, R.; Youcef, A. CFD-Based simulation and analysis of hydrothermal aspects in solar channel heat exchangers with various designed vortex generators. Comput. Model. Eng. Sci. 2020, 126, 147–173. [Google Scholar]
- Ejaz, F.; Qasem, N.A.A.; Zubair, S.M. Thermal-hydraulic analysis of wetted airside plain fin flat tube heat exchangers and the role of vortex generators. Appl. Therm. Eng. 2024, 258, 124651. [Google Scholar] [CrossRef]
- Ding, J.; Zhao, Z.; Liu, X.; Li, C.; Pu, X. Buoyancy influences on local heat transfer characteristic of supercritical LNG across the pseudo-phase transition in variable cross-section horizontal channels. Appl. Therm. Eng. 2024, 245, 122823. [Google Scholar] [CrossRef]
- Ran, S.; Zhang, P.; Rao, Y. Numerical study of heat transfer and flow structure over channel surfaces featuring miniature V rib-dimples with various configurations. Int. J. Therm. Sci. 2021, 172, 107342. [Google Scholar] [CrossRef]
- Yuan, Z.X.; Tao, W.Q.; Wang, Q.W. Numerical prediction for laminar forced convection heat transfer in parallel-plate channels with streamwise-periodic rod disturbances. Int. J. Numer. Methods Fluids 1998, 28, 1371–1387. [Google Scholar] [CrossRef]
- Young, T.J.; Vafai, K. Convective cooling of a heated obstacle in a channel. Int. J. Heat. Mass. Transf. 1998, 41, 3131–3148. [Google Scholar] [CrossRef]
- Promvonge, P.; Promthaisong, P.; Skullong, S. Heat transfer augmentation in solar heat exchanger duct with louver-punched V-baffles. Sol. Energy 2022, 248, 103–120. [Google Scholar] [CrossRef]
- Mohit, M.K.; Gupta, R. Numerical investigation of the performance of rectangular micro-channel equipped with micro-pin-fin, Case. Stud. Therm. Eng. 2022, 32, 101884. [Google Scholar] [CrossRef]
- Jayranaiwachira, N.; Promvonge, P.; Promthaisong, P.; Nakhchi, M.E.; Skullong, S. Heat transfer analysis in a tube contained with louver-punched triangular baffles. Results Eng. 2024, 22, 102276. [Google Scholar] [CrossRef]
- Habet, M.A.E.; Ahmed, S.A.; Saleh, M.A. The effect of using staggered and partially tilted perforated baffles on heat transfer and flow characteristics in a rectangular channel. Int. J. Therm. Sci. 2021, 174, 107422. [Google Scholar] [CrossRef]
- Zhang, P.; Xu, C.; Rao, Y.; Liang, C. Experimental and numerical study of heat transfer and turbulent flow in a rotating channel with V rib-dimple hybrid structures. Int. J. Therm. Sci. 2023, 187, 108162. [Google Scholar] [CrossRef]
- Yadav, A.S.; Bhagoria, J.L. A CFD (computational fluid dynamics) based heat transfer and fluid flow analysis of a solar air heater provided with circular transverse wire rib roughness on the absorber plate. Energy 2013, 55, 1127–1142. [Google Scholar] [CrossRef]
- Yadav, A.S.; Bhagoria, J.L. A CFD based thermo-hydraulic performance analysis of an artificially roughened solar air heater having equilateral triangular sectioned rib roughness on the absorber plate. Int. J. Heat. Mass. Transf. 2013, 70, 1016–1039. [Google Scholar] [CrossRef]
- Zhang, P.; Xia, P.; Guo, X.; Xie, S.; Ma, W. A CFD-adjoint reverse design of transverse rib profile for enhancing thermo-hydraulic performance in the solar air heater, Renew. Energy 2022, 198, 587–601. [Google Scholar]
- Thakur, D.S.; Khan, M.K.; Pathak, M. Solar air heater with hyperbolic ribs: 3D simulation with experimental validation. Renew. Energy 2017, 113, 357–368. [Google Scholar] [CrossRef]
- Misra, R.; Singh, J.; Jain, S.K.; Faujdar, S.; Agrawal, M.; Mishra, A.; Goyal, P.K. Prediction of behavior of triangular solar air heater duct using V-down rib with multiple gaps and turbulence promoters as artificial roughness: A CFD analysis. Int. J. Heat. Mass. Transf. 2020, 162, 120376. [Google Scholar] [CrossRef]
- Jamal, I.; Barhdadi, F.Z.; Amghar, K.; Daoudi, S.; Yahiaoui, R.; Ghoumid, K. Enhancing performance in solar air channels: A numerical analysis of turbulent flow and heat transfer with novel shaped baffles. Appl. Therm. Eng. 2024, 251, 123561. [Google Scholar] [CrossRef]
- Mahanand, Y.; Senapati, J.R. Thermal enhancement study of a transverse inverted-T shaped ribbed solar air heater. Int. Commun. Heat. Mass. Transf. 2020, 119, 104922. [Google Scholar] [CrossRef]
- Li, Y.; Rao, Y.; Wang, D.; Zhang, P.; Wu, X. Heat transfer and pressure loss of turbulent flow in channels with miniature structured ribs on one wall. Int. J. Heat. Mass. Transf. 2018, 131, 584–593. [Google Scholar] [CrossRef]
- Jin, D.; Zhang, M.; Wang, P.; Xu, S. Numerical investigation of heat transfer and fluid flow in a solar air heater duct with multi V-shaped ribs on the absorber plate. Energy 2015, 89, 178–190. [Google Scholar] [CrossRef]
- Jin, D.; Quan, S.; Zuo, J.; Xu, S. Numerical investigation of heat transfer enhancement in a solar air heater roughened by multiple V-shaped ribs. Renew. Energy 2018, 134, 78–88. [Google Scholar] [CrossRef]
- Eiamsa-ard, S.; Suksangpanomrung, A.; Promthaisong, P. Enhanced heat transfer mechanism and flow topology of a channel contained with semi-circular hinged V-shaped baffles. Int. J. Therm. Sci. 2022, 177, 107577. [Google Scholar] [CrossRef]
- Menni, Y.; Chamkha, A.; Zidani, C.; Benyoucef, B. Baffle orientation and geometry effects on turbulent heat transfer of a constant property incompressible fluid flow inside a rectangular channel. Int. J. Numer. Methods Heat. Fluid. Flow. 2019, 30, 3027–3052. [Google Scholar] [CrossRef]
- Phila, A.; Keaitnukul, W.; Eiamsa-ard, S.; Naphon, P.; Maruyama, N.; Hirota, M.; Chuwattanakul, V. Influence of notched baffles on aerothermal performance behaviors in a channel. Case Stud. Therm. Eng. 2023, 47, 103070. [Google Scholar] [CrossRef]
- Phila, A.; Eiamsa-ard, S.; Thianpong, C. Thermal Performance Evaluation of a Channel Installed with Inclined-Baffle Turbulators. Arab. J. Sci. Eng. 2019, 45, 609–621. [Google Scholar] [CrossRef]
- Eiamsa-ard, S.; Pattanapipat, S.; Promvonge, P. Influence of triangular wavy baffles on heat and fluid flow characteristics in a channel, J. Mech. Sci. Technol. 2013, 27, 2199–2208. [Google Scholar] [CrossRef]
- Rashid, F.L.; Al-Gaheeshi, A.M.R.; Mohammed, H.I.; Ameen, A. Heat convection in a channel-opened cavity with two heated sources and baffle. Energies 2024, 17, 1209–1241. [Google Scholar] [CrossRef]
- Rashid, F.L.; Al-Gaheeshi, A.M.R.; Al-Obaidi, M.A.; Mohammed, H.I.; Togun, H.; Agyekum, E.B. Analysing fluid flow and heat transfer comparatively in flow passage systems: Evaluating thermal impacts and geometric configurations. Int. J. Thermofluids 2024, 24, 100894. [Google Scholar] [CrossRef]
- Rashid, F.L.; Eleiwi, M.A.; Tahseen, T.A.; Mohammed, H.I.; Tuama, S.A.; Ameen, A.; Agyekum, E.B. Influence of adiabatic semi-circular grooved in backward-facing step on thermal-hydraulic characteristics of nanofluid. Int. J. Thermofluids 2025, 26, 101052. [Google Scholar] [CrossRef]
- Matsson, J. An Introduction to ANSYS Fluent 2019; SDC Publications: Mission, KS, USA, 2019. [Google Scholar]
- Bender, E. Numerical heat transfer and fluid flow, Von S.V. Patankar, Hemisphere Publishing Corporation, Washington—New York—London McGraw Hill Book Company, New York 1980 1 Aufl, 197 S, 76 Abb, geb, DM 71,90. Chem. Ing. Tech. 1981, 53, 225. [Google Scholar] [CrossRef]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow; Hemisphere Publishing Corporation: Washington, DC, USA, 2018. [Google Scholar]
- Promvonge, P.; Skullong, S. Enhanced thermal performance in tubular heat exchanger contained with V-shaped baffles. Appl. Therm. Eng. 2021, 185, 116307. [Google Scholar] [CrossRef]
- Promvonge, P.; Skullong, S. Heat transfer in solar receiver heat exchanger with combined punched-V-ribs and chamfer-V-grooves. Int. J. Heat. Mass. Transf. 2019, 143, 118486. [Google Scholar] [CrossRef]
- Incropera, F.; Dewitt, P.D. Introduction to Heat Transfer, 5th ed.; John Wiley & Sons Inc.: New York, NY, USA, 2006. [Google Scholar]
- Eiamsa-ard, S.; Phila, A.; Pimsarn, M.; Maruyama, N.; Hirota, M. Heat transfer mechanism and thermal performance of a channel with square-wing perforated transverse baffles installed: Effect of square-wing location. J. Therm. Anal. Calorim. 2023, 148, 3835–3849. [Google Scholar] [CrossRef]
- Kumar, R.; Nadda, R.; Kumar, S.; Razak, A.; Sharifpure, M.; Aybar, H.S.; Saleel, C.A.; Afzal, A. Influence of artificial roughness parametric variation on thermal performance of solar thermal collector: An experimental study, response surface analysis and ANN modelling. Sustain. Energy Technol. Assess. 2022, 52, 102047. [Google Scholar] [CrossRef]
- El Habet, M.A.; Ahmed, S.A.; Saleh, M.A. Thermal/hydraulic characteristics of a rectangular channel with inline/staggered perforated baffles. Int. Commun. Heat. Mass. Transf. 2021, 128, 105591. [Google Scholar] [CrossRef]
- Chamoli, S.; Thakur, N.S. Correlations for solar air heater duct with V-shaped perforated baffles as roughness elements on absorber plate. Int. J. Sustain. Energy 2016, 35, 1–20. [Google Scholar] [CrossRef]
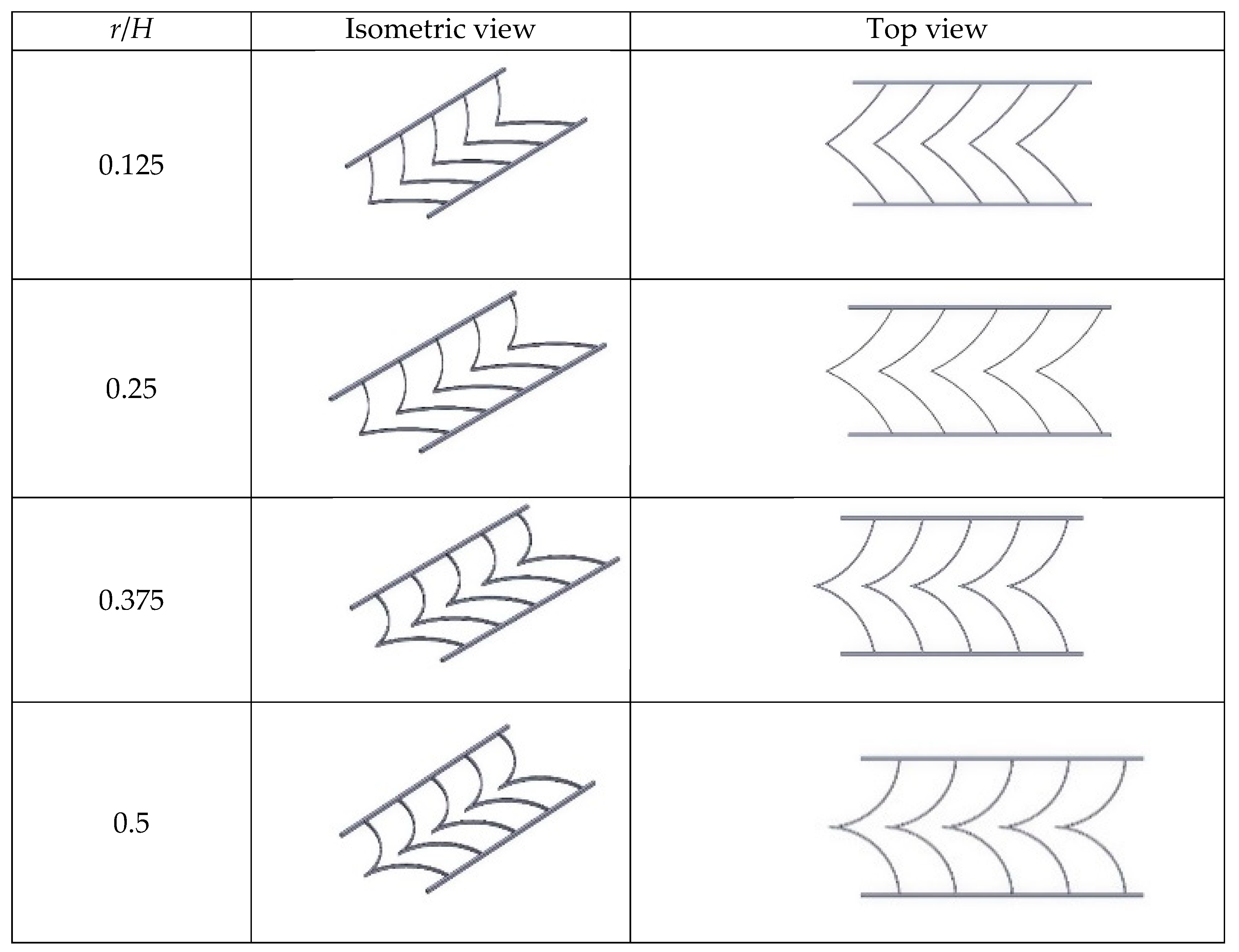
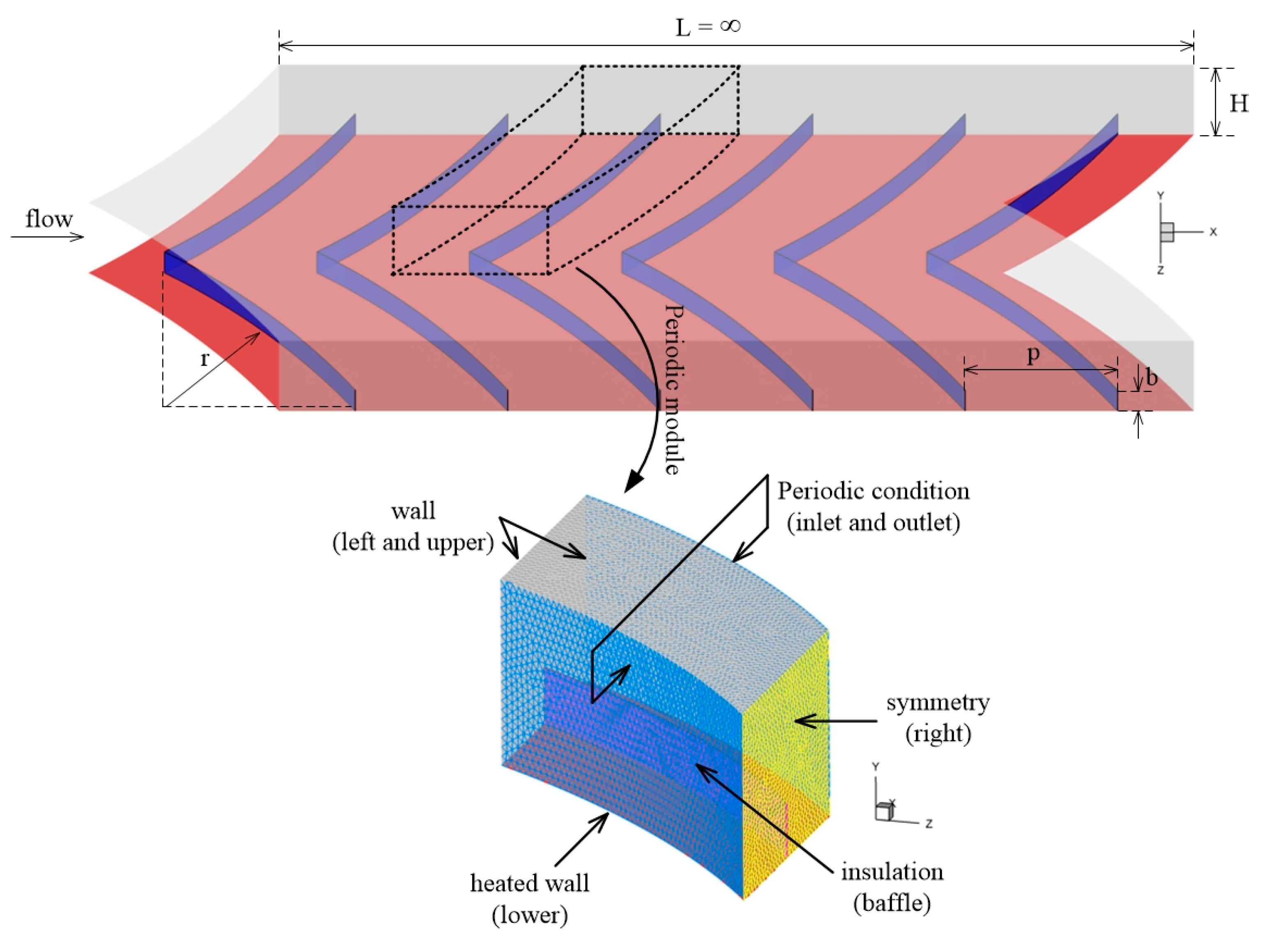









| Location | Boundary Type | Conditions |
| Inlet and outlet | Periodic [44] | Air enters at 300 K (Pr = 0.707) |
| Upper wall | Adiabatic, no-slip | No heat transfer through the wall |
| Lower wall | Constant heat flux, no-slip | Constant heat flux at 600 W/m2 and no-slip |
| Left and right walls | Symmetry | Symmetry conditions applied |
| Arc-vane baffles | Adiabatic, no-slip | Baffles are thermally insulated, no-slip |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thapmanee, P.; Phila, A.; Wongcharee, K.; Maruyama, N.; Hirota, M.; Chuwattanakul, V.; Eiamsa-ard, S. Numerical Methodology for Enhancing Heat Transfer in a Channel with Arc-Vane Baffles. Computation 2025, 13, 71. https://doi.org/10.3390/computation13030071
Thapmanee P, Phila A, Wongcharee K, Maruyama N, Hirota M, Chuwattanakul V, Eiamsa-ard S. Numerical Methodology for Enhancing Heat Transfer in a Channel with Arc-Vane Baffles. Computation. 2025; 13(3):71. https://doi.org/10.3390/computation13030071
Chicago/Turabian StyleThapmanee, Piphatpong, Arnut Phila, Khwanchit Wongcharee, Naoki Maruyama, Masafumi Hirota, Varesa Chuwattanakul, and Smith Eiamsa-ard. 2025. "Numerical Methodology for Enhancing Heat Transfer in a Channel with Arc-Vane Baffles" Computation 13, no. 3: 71. https://doi.org/10.3390/computation13030071
APA StyleThapmanee, P., Phila, A., Wongcharee, K., Maruyama, N., Hirota, M., Chuwattanakul, V., & Eiamsa-ard, S. (2025). Numerical Methodology for Enhancing Heat Transfer in a Channel with Arc-Vane Baffles. Computation, 13(3), 71. https://doi.org/10.3390/computation13030071









