Elasto-Plastic Analysis of Two-Way Reinforced Concrete Slabs Strengthened with Carbon Fiber Reinforced Polymer Laminates
Abstract
1. Introduction
2. Theoretical Background
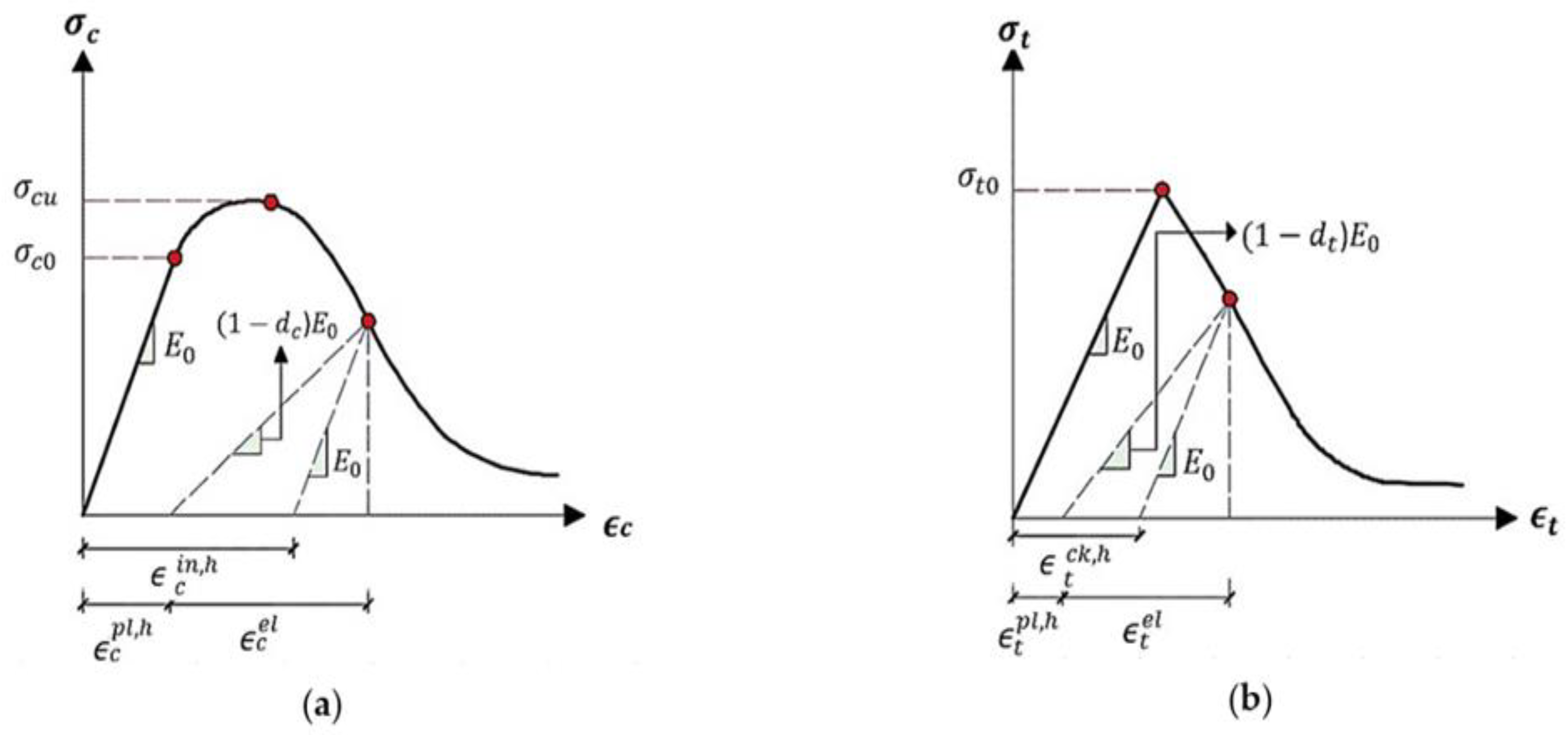
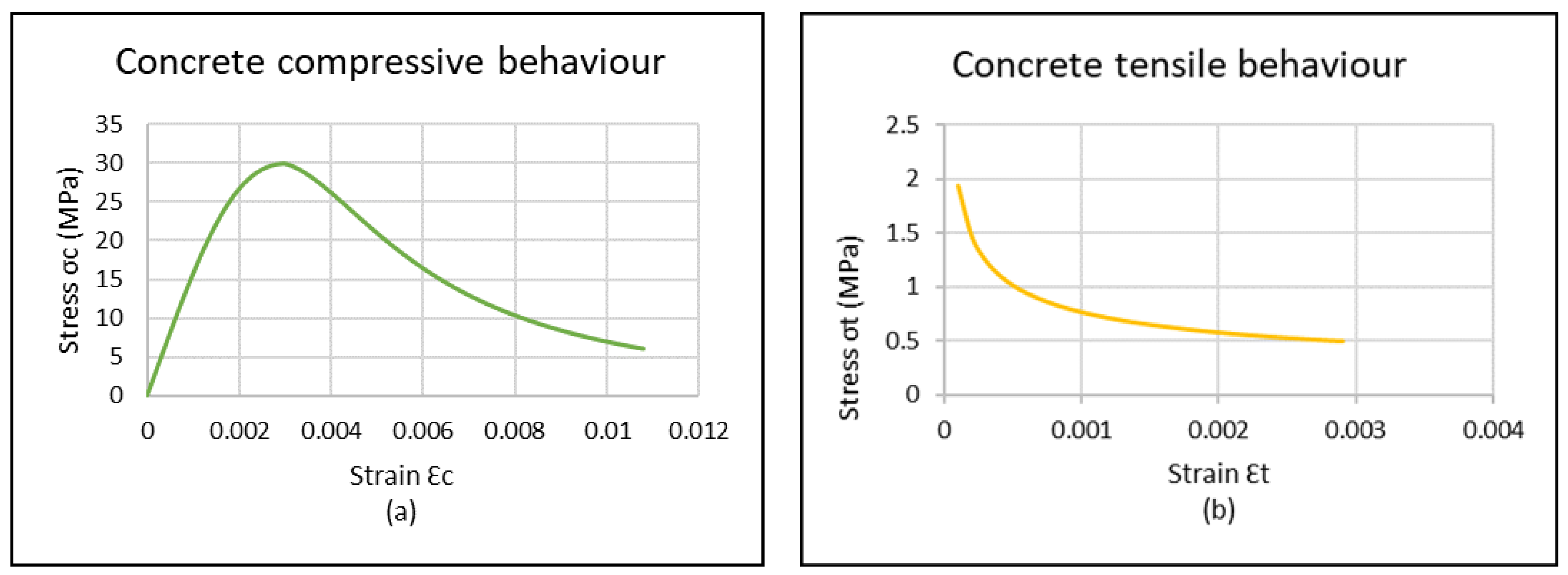
2.1. Concrete Constitutive Model
2.2. Utilizing the Static Principles of Plastic Limit Analysis
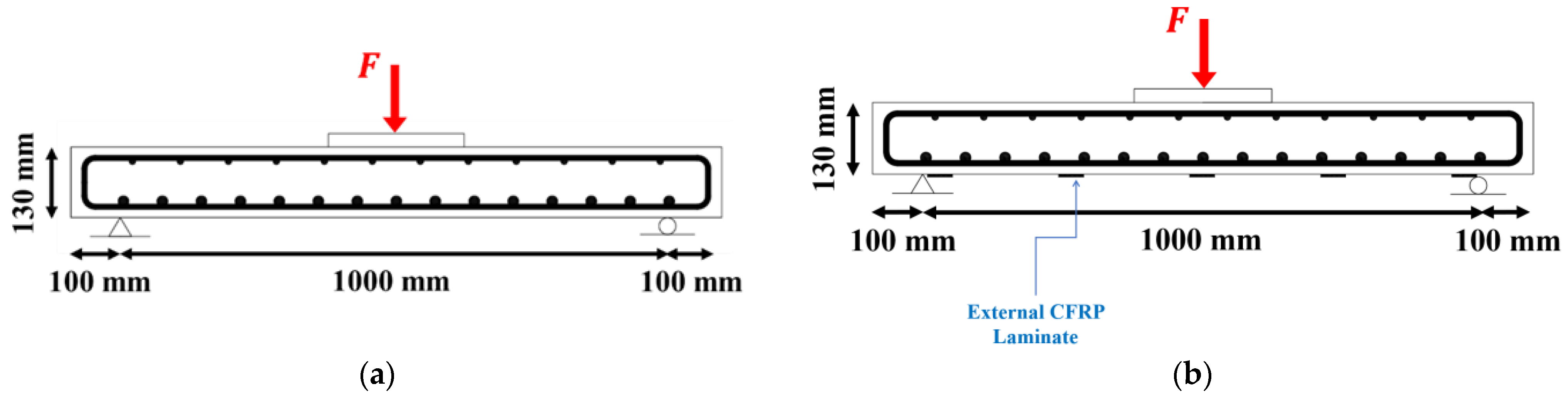
3. Experimental Work
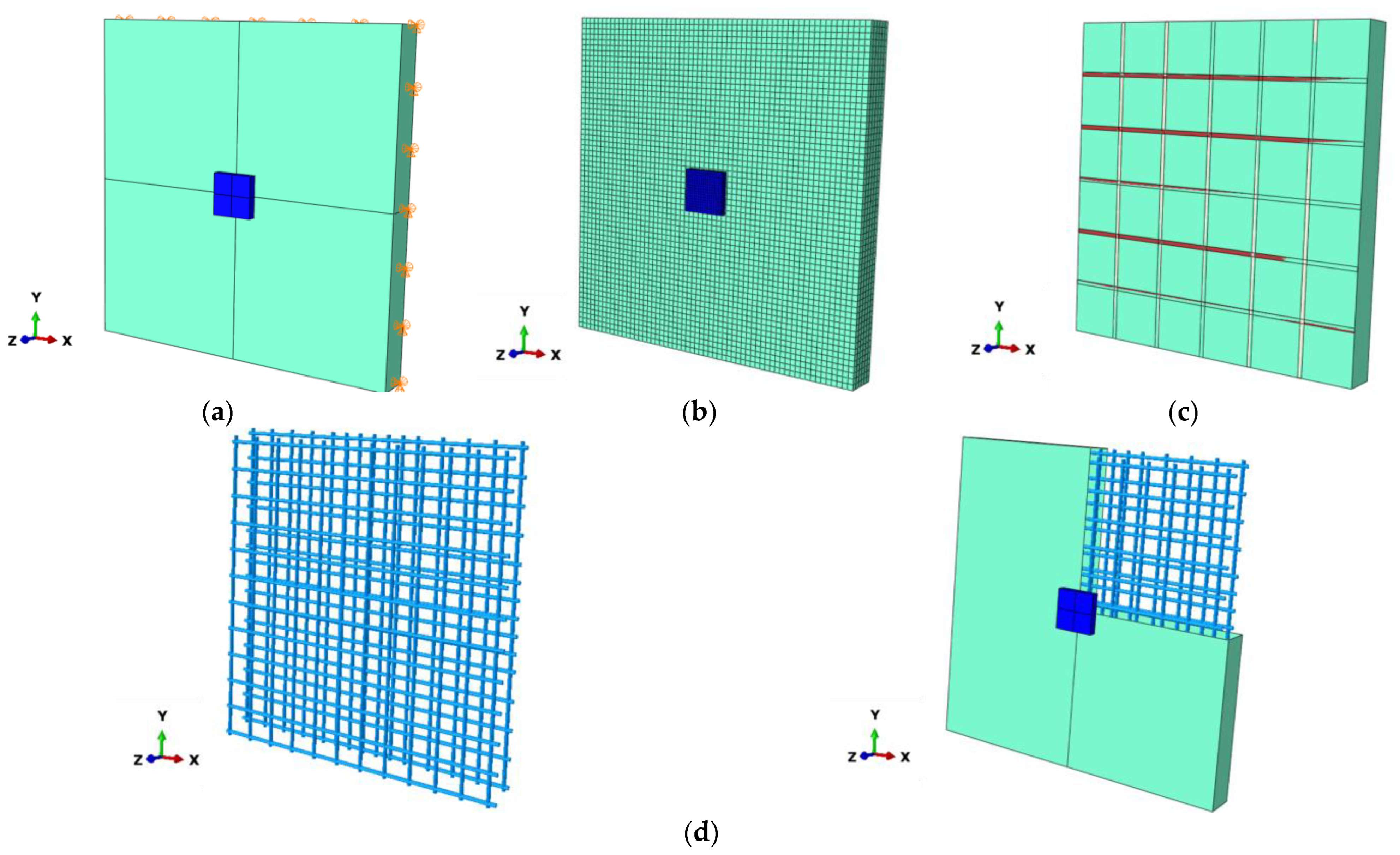
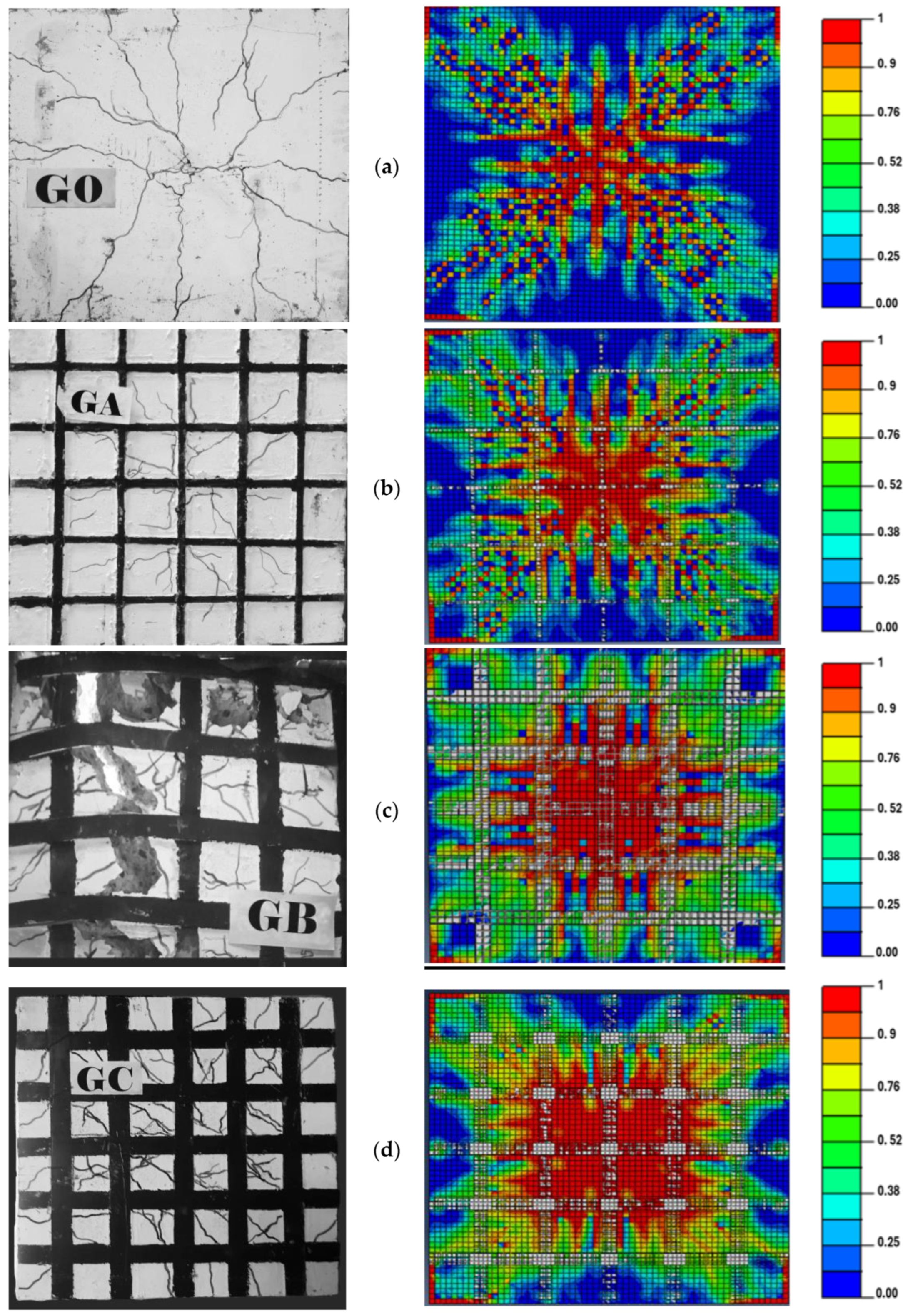
- Slab GA was reinforced with five strips of CFRP laminates in both directions with 1.2 mm thickness and 15 mm width, as shown in Figure 2b.
- Slab GB was reinforced with five strips of CFRP laminates in both directions with 1.2 mm thickness and 30 mm width.
- Slab GC was reinforced with five strips of CFRP laminates in both directions with 1.2 mm thickness and 45 mm width.
4. Test Procedure and the Response of Concrete Slabs
5. Numerical Modeling
6. Results and Conclusions
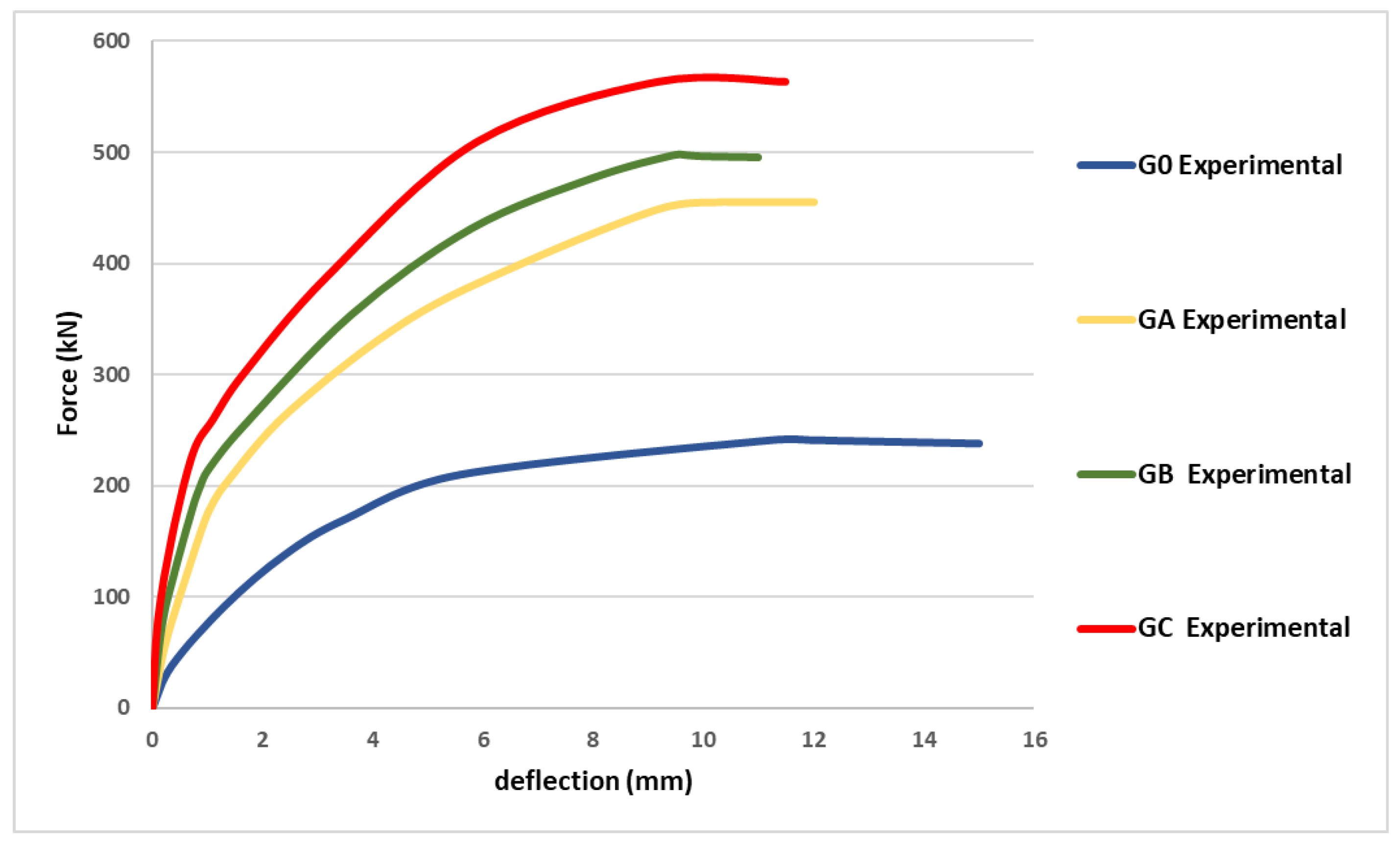
- The performance of the reinforced slabs was distinctly influenced by the strengthening ratios. It was observed that an elevation in the strengthening ratio consistently led to a higher ultimate strength.
- The enhancement of reinforcement ratios across all the three specimens led to a rise in initial cracking, alongside a simultaneous decrease in deflection.
- Strengthening through CFRP laminate exhibited superior ultimate strength, cracking load, and minimized crack width, when compared to the control slab.
- The damage pattern presented in the data reflects the percentages of concrete damage due to tension. It can be deduced that, as the applied load rises, the severity of damage also increases. This suggests that the additional load imparts additional initial stresses to both the steel and concrete components. The function of complementary strain energy is emphasized by reflecting the plastic damage and, by controlling it, the plastic damage and failure may also be managed.
- The outcomes underscored the commendable efficacy of CFRPs in enhancing the ultimate load capacity of slabs, while simultaneously decreasing crack width and central deflection under equivalent loads.
- Alternatively, based on the numerical findings, varying quantities of CFRP strips have been observed to influence the behavior of the slabs. A comparison between experimental and numerical data reveals that the presence of CFRPs results in an increase in the slabs’ ultimate load capacity, serving as additional support to withstand shear stress and confine the concrete section, thereby inducing a more brittle and abrupt failure mode.
- In general, a reduction in the number of CFRP strips tends to exacerbate the extent of red area propagation, suggesting potential damage to either the steel or concrete. Conversely, employing more CFRP strips tends to mitigate the expansion of these red areas, akin to the effect observed with lower reinforcement ratios. In such cases, structures endure elevated stresses under lighter loads, when lower strengthening ratios are applied, resulting in failure at correspondingly lower loads.
- Linear behavior was observed in all specimens up to the first cracking load, with the load deflection curve at mid-span potentially approximated by a bilinear or trilinear curve.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Binici, B. Punching Shear Strengthening of Reinforced Concrete Slabs Using Fiber Reinforced Polymers. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2003. [Google Scholar]
- Bisby, L.A.; Fitzwilliam, J. An introduction to FRP composites for construction. ISIS Educ. Modul. Manit. Can. 2006. [Google Scholar]
- Khan, A.R.; Baluch, M.H.; Al-Gadhib, A.H. Repair and Strengthening of Reinforced Concrete Structures Using CFRP Plates. In Proceedings of the International Bhurban Conference on Applied Sciences and Technology, Islamabad, Pakistan, 7–12 June 2004. [Google Scholar]
- Ombres, L.; Mazzuca, P. Residual Flexural Behavior of PBO FRCM-Strengthened Reinforced Concrete Beams after Exposure to Elevated Temperatures. J. Compos. Constr. 2024, 28, 4023063. [Google Scholar] [CrossRef]
- Limam, O.; Foret, G.; Ehrlacher, A. RC two-way slabs strengthened with CFRP strips: Experimental study and a limit analysis approach. Compos. Struct. 2003, 60, 467–471. [Google Scholar] [CrossRef]
- Mosallam, A.S.; Mosalam, K.M. Strengthening of two-way concrete slabs with FRP composite laminates. Constr. Build. Mater. 2003, 17, 43–54. [Google Scholar] [CrossRef]
- Limam, O.; Nguyen, V.T.; Foret, G. Numerical and experimental analysis of two-way slabs strengthened with CFRP strips. Eng. Struct. 2005, 27, 841–845. [Google Scholar] [CrossRef]
- Hosseini, M.M.; Dias, S.; Barros, J. Flexural strengthening of reinforced low strength concrete slabs using prestressed NSM CFRP laminates. Compos. Part B Eng. 2015, 90, 14–29. [Google Scholar] [CrossRef]
- Yılmaz T, Kıraç N, Anil Ö, Erdem RT, Sezer C. Low-velocity impact behaviour of two way RC slab strengthening with CFRP strips. Constr. Build. Mater. 2018, 186, 1046–1063. [Google Scholar] [CrossRef]
- Akhundzada, H.; Donchev, T.; Petkova, D. Strengthening of slab-column connection against punching shear failure with CFRP laminates. Compos. Struct. 2019, 208, 656–664. [Google Scholar] [CrossRef]
- Mercimek, Ö.; Ghoroubi, R.; Erbaş, Y.; Anil, Ö. Comparison of strengthening methods to improve punching behavior of two-way RC flat slabs. Structures 2022, 46, 1495–1516. [Google Scholar] [CrossRef]
- Türer, A.; Mercimek, Ö.; Anıl, Ö.; Erbaş, Y. Experimental and numerical investigation of punching behavior of two-way RC slab with different opening locations and sizes strengthened with CFRP strip. Structures 2023, 49, 918–942. [Google Scholar] [CrossRef]
- Enochsson, O.; Lundqvist, J.; Täljsten, B.; Rusinowski, P.; Olofsson, T. CFRP strengthened openings in two-way concrete slabs—Experimental and numerical study. Constr. Build. Mater. 2007, 21, 810–826. [Google Scholar] [CrossRef]
- Esfahani, M.R.; Kianoush, M.R.; Moradi, A.R. Punching shear strength of interior slab–column connections strengthened with carbon fiber reinforced polymer sheets. Eng. Struct. 2009, 31, 1535–1542. [Google Scholar] [CrossRef]
- Meisami, M.H.; Mostofinejad, D.; Nakamura, H. Punching shear strengthening of two-way flat slabs using CFRP rods. Compos. Struct. 2013, 99, 112–122. [Google Scholar] [CrossRef]
- Elsanadedy, H.M.; Almusallam, T.H.; Alsayed, S.H.; Al-salloum, Y.A. Experimental and FE study on RC one-way slabs upgraded with FRP composites. KSCE J. Civ. Eng. 2015, 19, 1024–1040. [Google Scholar] [CrossRef]
- Behzard, P.; Sharbatdar, M.K.; Kheyroddin, A. Strengthening of existing RC two-way slabs using new combined FRP fabric/rod technique. J. Rehabil. Civ. Eng. 2015, 3, 30–44. [Google Scholar]
- Mahmoud, H.S.; Hawileh, R.A.; Abdalla, J.A. Strengthening of high strength reinforced concrete thin slabs with CFRP laminates. Compos. Struct. 2021, 75, 114412. [Google Scholar] [CrossRef]
- Rad, M.M.; Ibrahim, S.K.; Lógó, J. Limit design of reinforced concrete haunched beams by the control of the residual plastic de-formation. Structures 2022, 39, 987–996. [Google Scholar] [CrossRef]
- Carol, I.; Rizzi, E.; Willam, K. On the formulation of anisotropic elastic degradation. I. Theory based on a pseudo-logarithmic damage tensor rate. Int. J. Solids Struct. 2001, 38, 491–518. [Google Scholar] [CrossRef]
- Khaleel Ibrahim, S.; Movahedi Rad, M. Limited Optimal Plastic Behavior of RC Beams Strengthened by Carbon Fiber Polymers Using Reliability-Based Design. Polymers 2023, 15, 569. [Google Scholar] [CrossRef] [PubMed]
- Rad, M.M.; Papp, F.; Ibrahim, S.K.; Szép, J.; Gosztola, D.; Harrach, D. Elasto-plastic analysis and optimal design of composite integral abutment bridge extended with limited residual plastic deformation. Sci. Rep. 2023, 13, 5461. [Google Scholar] [CrossRef] [PubMed]
- Gatuingt, F.; Pijaudier-Cabot, G. Coupled damage and plasticity modelling in transient dynamic analysis of concrete. Int. J. Numer. Anal. Methods Geomech. 2002, 26, 1–24. [Google Scholar] [CrossRef]
- Krätzig, W.B.; Pölling, R. An elasto-plastic damage model for reinforced concrete with minimum number of material parameters. Comput. Struct. 2004, 82, 1201–1215. [Google Scholar] [CrossRef]
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Imran, I.; Pantazopoulou, S.J. Plasticity model for concrete under triaxial compression. J. Eng. Mech. 2001, 127, 281–290. [Google Scholar] [CrossRef]
- Ananiev, S.; Ozbolt, J. Plastic–damage model for concrete in principal directions. In Fracture Mechanics of Concrete Structures; Li, V., Leung, C.K.Y., William, K.J., Billington, S.L., Eds.; Elsevier: Amsterdam, The Netherlands, 2004; pp. 271–278. [Google Scholar]
- Szép, J.; Habashneh, M.; Lógó, J.; Rad Movahedi, M. Reliability assessment of reinforced concrete beams under elevated temperatures: A probabilistic approach using finite element and physical models. Sustainability 2023, 15, 6077. [Google Scholar] [CrossRef]
- Habashneh, M.; Movahedi Rad, M. Plastic-Limit Probabilistic Structural Topology Optimization of Steel Beams. Appl. Math. Model. 2024, 128, 347–369. [Google Scholar] [CrossRef]
- Habashneh, M.; Cucuzza, R.; Domaneschi, M.; Movahedi Rad, M. Advanced Elasto-Plastic Topology Optimization of Steel Beams under Elevated Temperatures. Adv. Eng. Softw. 2024, 190, 103596. [Google Scholar] [CrossRef]

| Slab Designation | Average Compressive Strength (MPa) | ||
|---|---|---|---|
| f c` at 28 Days | at 28 Days | ||
| G0 | 31 | 35.5 | 0.87 |
| GA | 31.1 | 37.1 | 0.84 |
| GB | 30 | 36 | 0.83 |
| GC | 29.2 | 36 | 0.81 |
| Reinforcement | Diameter of 6 mm | Diameter of 10 mm |
|---|---|---|
| Properties | ||
| Yield Stress (MPa) | 335 | 485 |
| Ultimate Stress (MPa) | 480 | 620 |
| Modulus of Elasticity (MPa) | 193 | 194 |
| CFRP Strengthening | CFRP Laminate |
|---|---|
| Properties | |
| Tensile strength (MPa) | 2800 |
| Elongation (mm) | 1.7 |
| Thickness (mm) | 1.2 |
| Modulus of Elasticity (GPa) | 165 |
| The Epoxy used with CFRP | |
| Properties | |
| Density (kg/lt) | 1.31 |
| Mixing ratio | White/Gray component = 1:3 |
| Tensile strength (MPa) | 30 |
| Open time, min. | 30 (at +35 °C) |
| Full cure, days | 7 (at +35 °C) |
| Specimen | Pcr * (kN) | Wcr * (mm) | Δv * (mm) | Pu * (kN) | Pcr/Pu | Pcr/Pcr.Control | Pu/Pu.Control |
|---|---|---|---|---|---|---|---|
| G0 | 120 | 1.3 | 11.2 | 241 | 0.49 | 1 | 1 |
| GA | 190.6 | 0.83 | 10.2 | 455 | 0.4 | 1.58 | 1.88 |
| GB | 208.5 | 0.8 | 9.5 | 498 | 0.418 | 1.73 | 2.06 |
| GC | 237.28 | 0.78 | 9.2 | 564 | 0.42 | 1.97 | 2.34 |
| Dilation Angle | Eccentricity | Fb0/fc0 | k | Viscosity Parameter |
|---|---|---|---|---|
| 31 | 0.2 | 1.16 | 0 | 0.001 |
| Slab Specimen | Load Multiplier | CDP Model | %) | |
|---|---|---|---|---|
| GC | 1 | 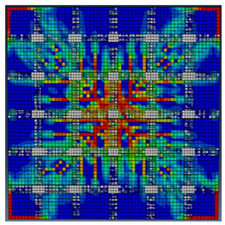 |  | 2.5% |
| 2.37 | 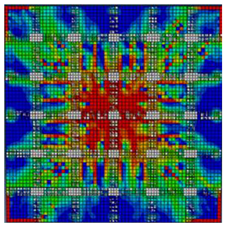 |  | 6.7% | |
| GC | 3 | 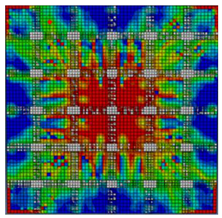 |  | 7.86% |
| 4 | 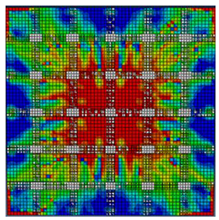 | 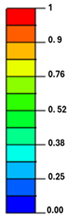 | 9.98% | |
| 5 |  | 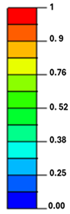 | 11% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharhan, Z.S.; Movahedi Rad, M. Elasto-Plastic Analysis of Two-Way Reinforced Concrete Slabs Strengthened with Carbon Fiber Reinforced Polymer Laminates. Computation 2024, 12, 93. https://doi.org/10.3390/computation12050093
Sharhan ZS, Movahedi Rad M. Elasto-Plastic Analysis of Two-Way Reinforced Concrete Slabs Strengthened with Carbon Fiber Reinforced Polymer Laminates. Computation. 2024; 12(5):93. https://doi.org/10.3390/computation12050093
Chicago/Turabian StyleSharhan, Zahraa Saleem, and Majid Movahedi Rad. 2024. "Elasto-Plastic Analysis of Two-Way Reinforced Concrete Slabs Strengthened with Carbon Fiber Reinforced Polymer Laminates" Computation 12, no. 5: 93. https://doi.org/10.3390/computation12050093
APA StyleSharhan, Z. S., & Movahedi Rad, M. (2024). Elasto-Plastic Analysis of Two-Way Reinforced Concrete Slabs Strengthened with Carbon Fiber Reinforced Polymer Laminates. Computation, 12(5), 93. https://doi.org/10.3390/computation12050093







