A Circuit Theory Perspective on the Modeling and Analysis of Vibration Energy Harvesting Systems: A Review
Abstract
1. Introduction
2. Modeling
2.1. Modeling Ambient Mechanical Vibrations
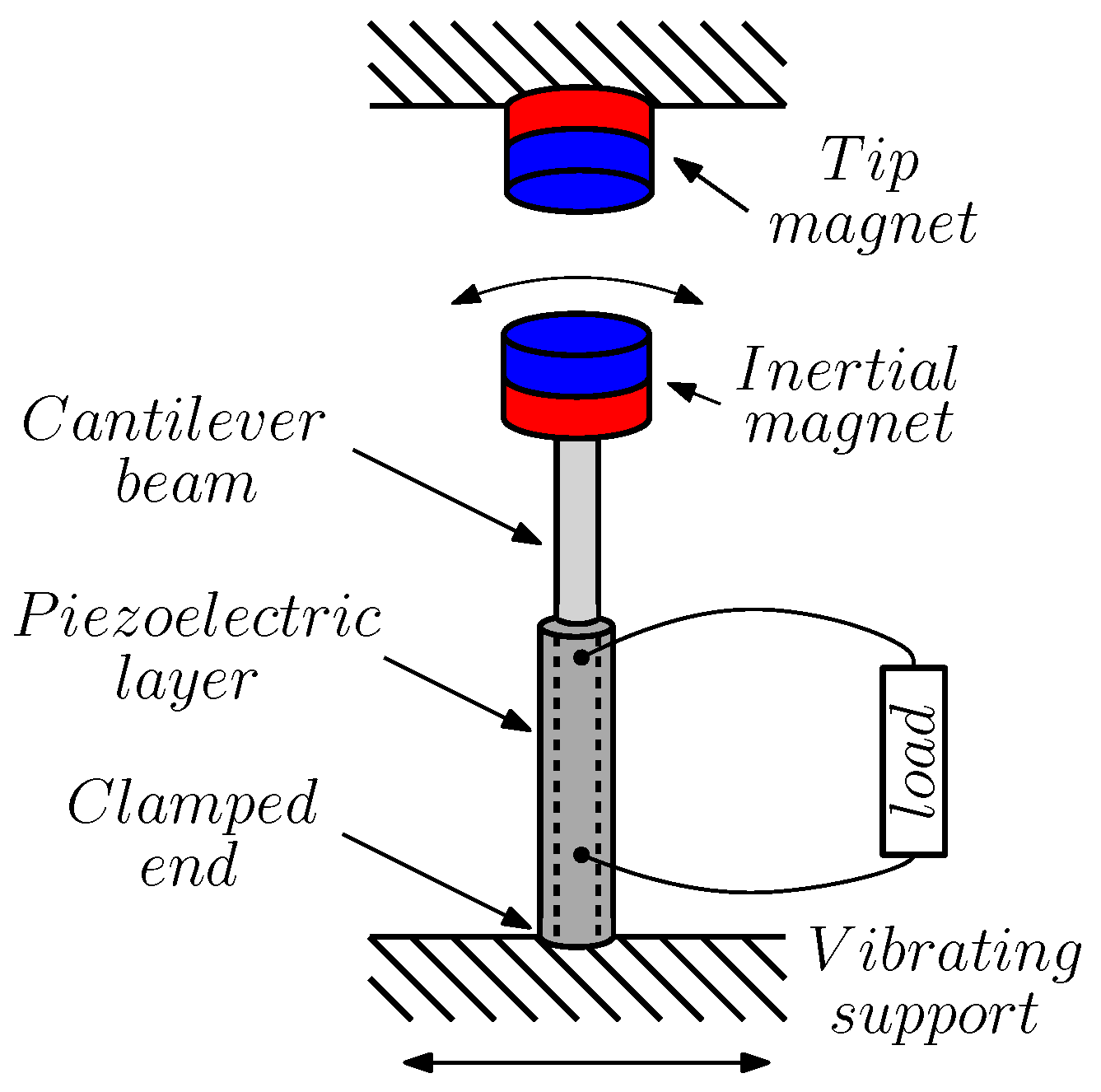
2.2. Modeling Vibration Energy Harvester Architectures
2.2.1. Piezoelectric Harvesters
- the compliance tensor , defined for a constant electric field as the strain generated per unit stress;
- the tensor d of piezoelectric charge constants
- the absolute permittivity , namely the dielectric displacement per unit electric field for constant stress [12]
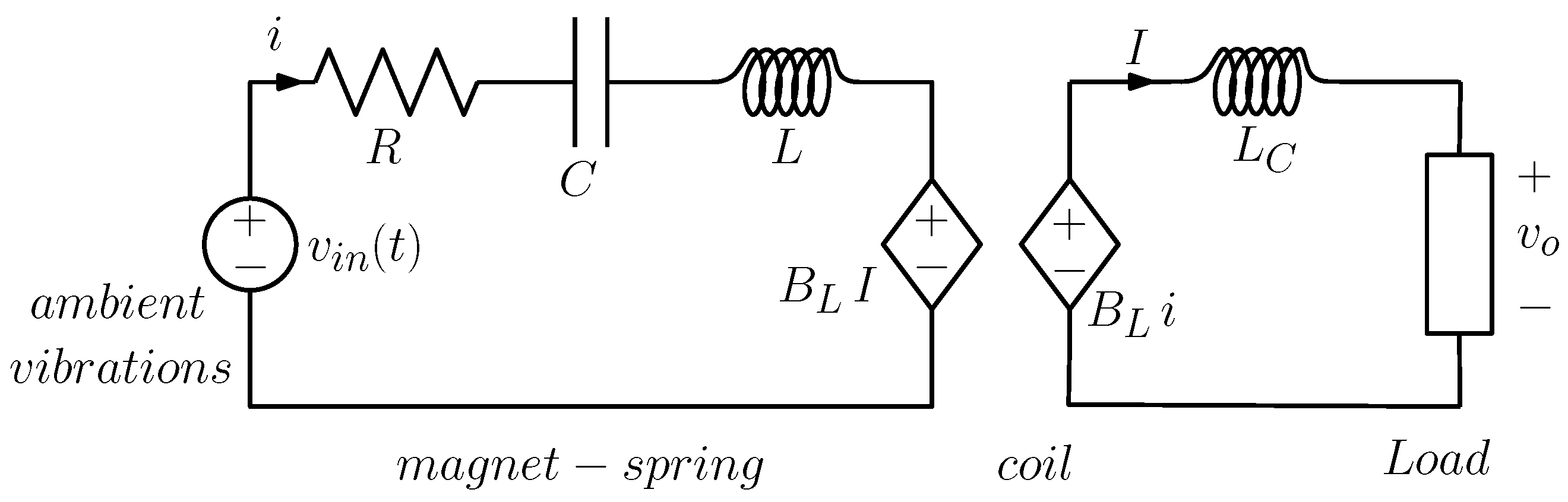
2.2.2. Electromagnetic Induction Harvesters
2.3. Equivalent Circuit Models
2.4. Load Modeling
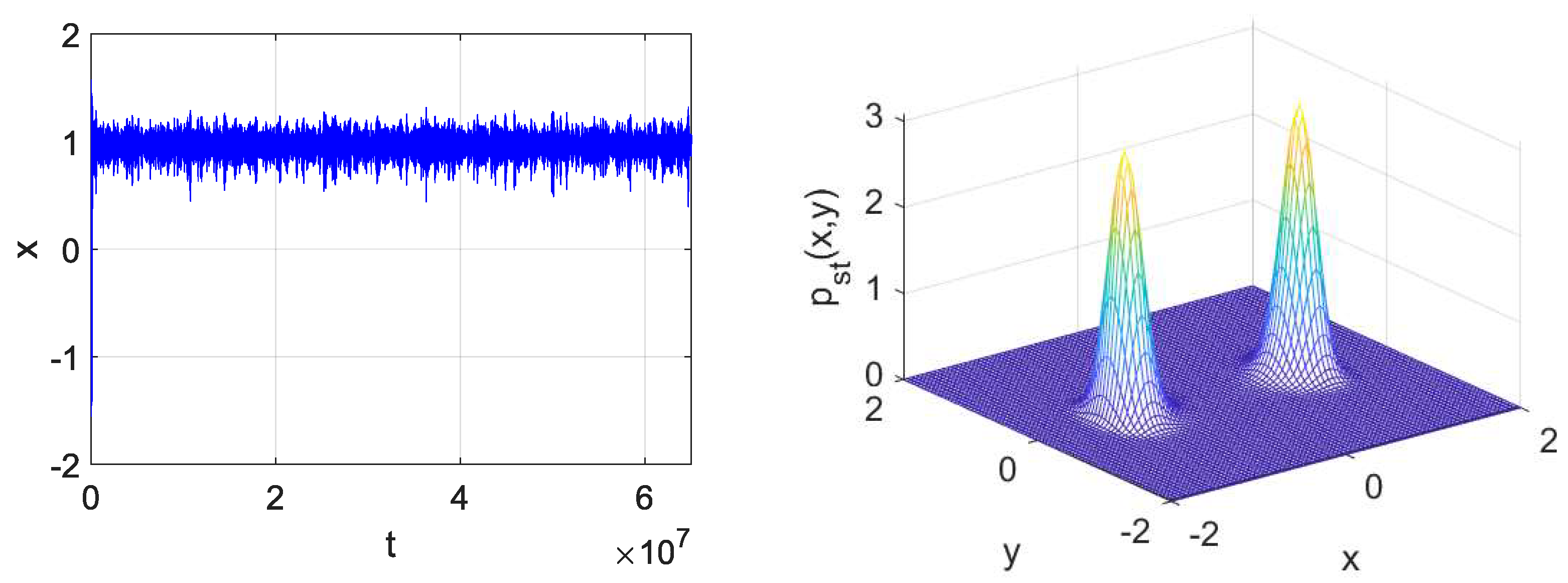
2.5. Nonlinear Harvester Modeling
3. Analysis
3.1. Frequency Domain Methods for Linear Systems
3.2. Frequency Domain Methods for Nonlinear Systems: Harmonic Balance
3.3. Stochastic Analysis: Averaging Techniques
3.3.1. Periodically Forced Nonlinear Oscillators and Averaging
3.3.2. Stochastic Averaging and Adiabatic Elimination
3.4. Numerical Analysis Methods
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Misra, S.; Mukherjee, A.; Roy, A. Introduction to IoT; University of Cambridge ESOL Examinations: Cambridge, UK, 2021. [Google Scholar]
- IoT 2022: Connected Devices Growing 18% to 14.4 Billion Globally. Available online: https://www.iotforall.com/state-of-iot-2022 (accessed on 22 February 2023).
- Munoz-Ausecha, C.; Ruiz-Rosero, J.; Ramirez-Gonzalez, G. RFID Applications and Security Review. Computation 2021, 9, 69. [Google Scholar] [CrossRef]
- Penella-López, M.T.; Gasulla-Forner, M. Powering Autonomous Sensors An Integral Approach with Focus on Solar and RF Energy Harvesting; Springer London, Limited: London, UK, 2011. [Google Scholar]
- Roundy, S.; Wright, P.K.; Rabaey, J.M. Energy Scavenging for Wireless Sensor Networks; Springer: New York, NY, USA, 2003. [Google Scholar]
- Paradiso, J.A.; Starner, T. Energy scavenging for mobile and wireless electronics. IEEE Pervasive Comput. 2005, 4, 18–27. [Google Scholar] [CrossRef]
- Beeby, S.P.; Tudor, M.J.; White, N.M. Energy harvesting vibration sources for microsystems applications. Meas. Sci. Technol. 2006, 17, R175. [Google Scholar] [CrossRef]
- Mitcheson, P.; Yeatman, E.; Rao, G.; Holmes, A.; Green, T. Energy Harvesting From Human and Machine Motion for Wireless Electronic Devices. Proc. IEEE 2008, 96, 1457–1486. [Google Scholar] [CrossRef]
- Lu, X.; Wang, P.; Niyato, D.; Kim, D.I.; Han, Z. Wireless Networks with RF Energy Harvesting: A Contemporary Survey. IEEE Commun. Surv. Tutor. 2015, 17, 757–789. [Google Scholar] [CrossRef]
- Akinaga, H. Recent advances and future prospects in energy harvesting technologies. Jpn. J. Appl. Phys. 2020, 59, 110201. [Google Scholar] [CrossRef]
- Anton, S.R.; Sodano, H.A. A review of power harvesting using piezoelectric materials (2003–2006). Smart Mater. Struct. 2007, 16, R1. [Google Scholar] [CrossRef]
- Priya, S.; Inman, D.J. Energy Harvesting Technologies; Springer: New York, NY, USA, 2009; Volume 21. [Google Scholar]
- Liu, H.; Zhong, J.; Lee, C.; Lee, S.W.; Lin, L. A comprehensive review on piezoelectric energy harvesting technology: Materials, mechanisms, and applications. Appl. Phys. Rev. 2018, 5, 041306. [Google Scholar] [CrossRef]
- Covaci, C.; Gontean, A. Piezoelectric energy harvesting solutions: A review. Sensors 2020, 20, 3512. [Google Scholar] [CrossRef]
- Tang, L.; Yang, Y.; Soh, C.K. Toward broadband vibration-based energy harvesting. J. Intell. Mater. Syst. Struct. 2010, 21, 1867–1897. [Google Scholar] [CrossRef]
- Khaligh, A.; Zeng, P.; Zheng, C. Kinetic energy harvesting using piezoelectric and electromagnetic technologies–state of the art. IEEE Trans. Ind. Electron. 2009, 57, 850–860. [Google Scholar] [CrossRef]
- Wei, C.; Jing, X. A comprehensive review on vibration energy harvesting: Modelling and realization. Renew. Sustain. Energy Rev. 2017, 74, 1–18. [Google Scholar] [CrossRef]
- Iqbal, M.; Nauman, M.M.; Khan, F.U.; Abas, P.E.; Cheok, Q.; Iqbal, A.; Aissa, B. Vibration-based piezoelectric, electromagnetic, and hybrid energy harvesters for microsystems applications: A contributed review. Int. J. Energy Res. 2021, 45, 65–102. [Google Scholar] [CrossRef]
- Gammaitoni, L.; Neri, I.; Vocca, H. The benefits of noise and nonlinearity: Extracting energy from random vibrations. Chem. Phys. 2010, 375, 435–438. [Google Scholar] [CrossRef]
- Gammaitoni, L. There’s plenty of energy at the bottom (micro and nano scale nonlinear noise harvesting). Contemp. Phys. 2012, 53, 119–135. [Google Scholar] [CrossRef]
- Daqaq, M.F.; Masana, R.; Erturk, A.; Dane Quinn, D. On the role of nonlinearities in vibratory energy harvesting: A critical review and discussion. Appl. Mech. Rev. 2014, 66. [Google Scholar] [CrossRef]
- Hannan, M.A.; Mutashar, S.; Samad, S.A.; Hussain, A. Energy harvesting for the implantable biomedical devices: Issues and challenges. Biomed. Eng. Online 2014, 13, 1–23. [Google Scholar] [CrossRef]
- Yildirim, T.; Ghayesh, M.H.; Li, W.; Alici, G. A review on performance enhancement techniques for ambient vibration energy harvesters. Renew. Sustain. Energy Rev. 2017, 71, 435–449. [Google Scholar] [CrossRef]
- Tran, N.; Ghayesh, M.H.; Arjomandi, M. Ambient vibration energy harvesters: A review on nonlinear techniques for performance enhancement. Int. J. Eng. Sci. 2018, 127, 162–185. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Kumar, P.; Leong, J. Finite Element Modelling and Simulations of Piezoelectric Actuators Responses with Uncertainty Quantification. Computation 2018, 6, 60. [Google Scholar] [CrossRef]
- Khazaee, M.; Rezania, A.; Rosendahl, L. Piezoelectric resonator design and analysis from stochastic car vibration using an experimentally validated finite element with viscous-structural damping model. Sustain. Energy Technol. Assess. 2022, 52, 102228. [Google Scholar] [CrossRef]
- Khazaee, M.; Huber, J.E.; Rosendahl, L.; Rezania, A. Four-point bending piezoelectric energy harvester with uniform surface strain toward better energy conversion performance and material usage. J. Sound Vib. 2023, 548, 117492. [Google Scholar] [CrossRef]
- Erturk, A.; Hoffmann, J.; Inman, D.J. A piezomagnetoelastic structure for broadband vibration energy harvesting. Appl. Phys. Lett. 2009, 94, 254102. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D.J. Piezoelectric Energy Harvesting; John Wiley & Sons: Chichester, UK, 2011. [Google Scholar]
- Harne, R.L.; Thota, M.; Wang, K.W. Concise and high-fidelity predictive criteria for maximizing performance and robustness of bistable energy harvesters. Appl. Phys. Lett. 2013, 102, 053903. [Google Scholar] [CrossRef]
- Bonnin, M.; Traversa, F.L.; Bonani, F. Leveraging circuit theory and nonlinear dynamics for the efficiency improvement of energy harvesting. Nonlinear Dyn. 2021, 104, 367–382. [Google Scholar] [CrossRef]
- Kuznetsov, Y.A.; Kuznetsov, I.A.; Kuznetsov, Y. Elements of Applied Bifurcation Theory; Springer: New York, NY, USA, 1998; Volume 112. [Google Scholar]
- Guckenheimer, J.; Holmes, P. Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields; Springer: New York, NY, USA, 2013; Volume 42. [Google Scholar]
- Bonnin, M. Harmonic balance, Melnikov method and nonlinear oscillators under resonant perturbation. Int. J. Circuit Theory Appl. 2008, 36, 247–274. [Google Scholar] [CrossRef]
- Bonnin, M.; Traversa, F.; Bonani, F. Efficient spectral domain technique for the frequency locking analysis of nonlinear oscillators. Eur. Phys. J. Plus 2018, 133, 1–12. [Google Scholar] [CrossRef]
- Gardiner, C.W. Handbook of Stochastic Methods; Springer: Berlin/Heidelberg, Germany, 1985; Volume 3. [Google Scholar]
- Ksendal, B. Stochastic Differential Equations, 6th ed.; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Rice, S.O. Mathematical Analysis of Random Noise. Bell Syst. Tech. J. 1944, 23, 282–332. [Google Scholar] [CrossRef]
- Patzold, M.; Killat, U.; Laue, F. A deterministic digital simulation model for Suzuki processes with application to a shadowed Rayleigh land mobile radio channel. IEEE Trans. Veh. Technol. 1996, 45, 318–331. [Google Scholar] [CrossRef]
- Le Maître, O.; Knio, O.M. Spectral Methods for Uncertainty Quantification: With Applications to Computational Fluid Dynamics; Springer: Dordrecht, The Netherlands, 2010. [Google Scholar]
- Xiu, D. Numerical Methods for Stochastic Computations; Princeton University Press: Princeton, NJ, USA, 2010. [Google Scholar]
- Smith, R.C. Uncertainty Quantification: Theory, Implementation, and Applications; SIAM: Philadelphia, PA, USA, 2013; Volume 12. [Google Scholar]
- Kaintura, A.; Dhaene, T.; Spina, D. Review of polynomial chaos-based methods for uncertainty quantification in modern integrated circuits. Electronics 2018, 7, 30. [Google Scholar] [CrossRef]
- Bonnin, M.; Traversa, F.L.; Bonani, F. Colored noise in oscillators. Phase-amplitude analysis and a method to avoid the Ito-Stratonovich dilemma. IEEE Trans. Circuits Syst. I Regul. Pap. 2019, 66, 3917–3927. [Google Scholar] [CrossRef]
- Bonnin, M.; Song, K. Frequency domain analysis of a piezoelectric energy harvester with impedance matching network. Energy Harvest. Syst. 2022, 100, 119–133. [Google Scholar] [CrossRef]
- Priya, S.; Song, H.C.; Zhou, Y.; Varghese, R.; Chopra, A.; Kim, S.G.; Kanno, I.; Wu, L.; Ha, D.S.; Ryu, J.; et al. A Review on Piezoelectric Energy Harvesting: Materials, Methods, and Circuits. Energy Harvest. Syst. 2017, 4, 3–39. [Google Scholar] [CrossRef]
- Costanzo, L.; Lo Schiavo, A.; Sarracino, A.; Vitelli, M. Stochastic thermodynamics of a piezoelectric energy harvester model. Entropy 2021, 23, 677. [Google Scholar] [CrossRef] [PubMed]
- Costanzo, L.; Lo Schiavo, A.; Sarracino, A.; Vitelli, M. Stochastic Thermodynamics of an Electromagnetic Energy Harvester. Entropy 2022, 24, 1222. [Google Scholar] [CrossRef] [PubMed]
- Costanzo, L.; Lo Schiavo, A.; Vitelli, M. Improving the Electromagnetic Vibration Energy Harvester Performance by Using a Double Coil Structure. Appl. Sci. 2022, 12, 1166. [Google Scholar] [CrossRef]
- Pertin, O.; Guha, K.; Jakšić, O. Artificial Intelligence-Based Optimization of a Bimorph-Segmented Tapered Piezoelectric MEMS Energy Harvester for Multimode Operation. Computation 2021, 9, 84. [Google Scholar] [CrossRef]
- IEEE Standard on Piezoelectricity. 1988. Available online: https://ieeexplore.ieee.org/document/26560 (accessed on 22 February 2023).
- Jones, T.B.; Nenadic, N.G. Electromechanics and MEMS; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Zhou, S.; Cao, J.; Inman, D.J.; Lin, J.; Li, D. Harmonic balance analysis of nonlinear tristable energy harvesters for performance enhancement. J. Sound Vib. 2016, 373, 223–235. [Google Scholar] [CrossRef]
- Yang, Z.; Erturk, A.; Zu, J. On the efficiency of piezoelectric energy harvesters. Extrem. Mech. Lett. 2017, 15, 26–37. [Google Scholar] [CrossRef]
- Huang, D.; Zhou, S.; Litak, G. Analytical analysis of the vibrational tristable energy harvester with a RL resonant circuit. Nonlinear Dyn. 2019, 97, 663–677. [Google Scholar] [CrossRef]
- Yu, T.; Zhou, S. Performance investigations of nonlinear piezoelectric energy harvesters with a resonant circuit under white Gaussian noises. Nonlinear Dyn. 2021, 103, 183–196. [Google Scholar] [CrossRef]
- Mann, B.P.; Sims, N.D. Energy harvesting from the nonlinear oscillations of magnetic levitation. J. Sound Vib. 2009, 319, 515–530. [Google Scholar] [CrossRef]
- Elvin, N.G.; Elvin, A.A. An experimentally validated electromagnetic energy harvester. J. Sound Vib. 2011, 330, 2314–2324. [Google Scholar] [CrossRef]
- Kwon, S.D.; Park, J.; Law, K. Electromagnetic energy harvester with repulsively stacked multilayer magnets for low frequency vibrations. Smart Mater. Struct. 2013, 22, 055007. [Google Scholar] [CrossRef]
- Kucab, K.; Górski, G.; Mizia, J. Energy harvesting in the nonlinear electromagnetic system. Eur. Phys. J. Spec. Top. 2015, 224, 2909–2918. [Google Scholar] [CrossRef]
- Firestone, F.A. A new analogy between mechanical and electrical systems. J. Acoust. Soc. Am. 1933, 4, 249–267. [Google Scholar] [CrossRef]
- Bourouina, T.; Grandchamp, J.P. Modeling micropumps with electrical equivalent networks. J. Micromech. Microeng. 1996, 6, 398. [Google Scholar] [CrossRef]
- Janschek, K. Mechatronic Systems Design: Methods, Models, Concepts; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Freeborn, T.J. A survey of fractional-order circuit models for biology and biomedicine. IEEE J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 416–424. [Google Scholar] [CrossRef]
- Civalleri, P.P.; Gilli, M.; Bonnin, M. Basic concepts of quantum systems versus classical networks. Int. J. Circuit Theory Appl. 2004, 32, 383–405. [Google Scholar] [CrossRef]
- Vool, U.; Devoret, M. Introduction to quantum electromagnetic circuits. Int. J. Circuit Theory Appl. 2017, 45, 897–934. [Google Scholar] [CrossRef]
- Bonnin, M.; Traversa, F.L.; Bonani, F. An Impedance Matching Solution to Increase the Harvested Power and Efficiency of Nonlinear Piezoelectric Energy Harvesters. Energies 2022, 15, 2764. [Google Scholar] [CrossRef]
- Roundy, S.; Zhang, Y. Toward self-tuning adaptive vibration-based microgenerators. In Proceedings of the Smart Structures, Devices, and Systems II. SPIE, Sydney, Australia, 28 February 2005; Voume 5649, pp. 373–384. [Google Scholar] [CrossRef]
- Shahruz, S. Design of mechanical band-pass filters for energy scavenging. J. Sound Vib. 2006, 292, 987–998. [Google Scholar] [CrossRef]
- Challa, V.R.; Prasad, M.; Shi, Y.; Fisher, F.T. A vibration energy harvesting device with bidirectional resonance frequency tunability. Smart Mater. Struct. 2008, 17, 015035. [Google Scholar] [CrossRef]
- Shin, Y.H.; Choi, J.; Kim, S.J.; Kim, S.; Maurya, D.; Sung, T.H.; Priya, S.; Kang, C.Y.; Song, H.C. Automatic resonance tuning mechanism for ultra-wide bandwidth mechanical energy harvesting. Nano Energy 2020, 77, 104986. [Google Scholar] [CrossRef]
- Wang, Z.; Du, Y.; Li, T.; Yan, Z.; Tan, T. A flute-inspired broadband piezoelectric vibration energy harvesting device with mechanical intelligent design. Appl. Energy 2021, 303, 117577. [Google Scholar] [CrossRef]
- Triplett, A.; Quinn, D.D. The effect of non-linear piezoelectric coupling on vibration-based energy harvesting. J. Intell. Mater. Syst. Struct. 2009, 20, 1959–1967. [Google Scholar] [CrossRef]
- Aliasghary, M.; Azizi, S.; Madinei, H.; Khodaparast, H.H. On the Efficiency Enhancement of an Actively Tunable MEMS Energy Harvesting Device. Vibration 2022, 5, 603–612. [Google Scholar] [CrossRef]
- Wang, X.; Wu, H.; Yang, B. Nonlinear multi-modal energy harvester and vibration absorber using magnetic softening spring. J. Sound Vib. 2020, 476, 115332. [Google Scholar] [CrossRef]
- Nguyen, S.D.; Halvorsen, E. Nonlinear springs for bandwidth-tolerant vibration energy harvesting. J. Microelectromech. Syst. 2011, 20, 1225–1227. [Google Scholar] [CrossRef]
- Zhou, S.; Cao, J.; Inman, D.J.; Lin, J.; Liu, S.; Wang, Z. Broadband tristable energy harvester: Modeling and experiment verification. Appl. Energy 2014, 133, 33–39. [Google Scholar] [CrossRef]
- Zhang, Y.; Duan, X.; Shi, Y.; Yue, X. Response Analysis of the Tristable Energy Harvester with an Uncertain Parameter. Appl. Sci. 2021, 11, 9979. [Google Scholar] [CrossRef]
- Wang, G.; Zheng, Y.; Zhu, Q.; Liu, Z.; Zhou, S. Asymmetric tristable energy harvester with a compressible and rotatable magnet-spring oscillating system for energy harvesting enhancement. J. Sound Vib. 2023, 543, 117384. [Google Scholar] [CrossRef]
- Zhang, Q.; Yan, Y.; Han, J.; Hao, S.; Wang, W. Dynamic Design of a Quad-Stable Piezoelectric Energy Harvester via Bifurcation Theory. Sensors 2022, 22, 8453. [Google Scholar] [CrossRef] [PubMed]
- Zhou, S.; Lallart, M.; Erturk, A. Multistable vibration energy harvesters: Principle, progress, and perspectives. J. Sound Vib. 2022, 528, 116886. [Google Scholar] [CrossRef]
- Wang, T.; Lou, H.; Zhu, S. Bandwidth enhancement of a gimbaled-pendulum vibration energy harvester using spatial multi-stable mechanism. Appl. Energy 2022, 326, 120047. [Google Scholar] [CrossRef]
- Wang, T.; Zhu, S. Analysis and Experiments of a Pendulum Vibration Energy Harvester with a Magnetic Multi-Stable Mechanism. IEEE Trans. Magn. 2022, 58, 1–7. [Google Scholar] [CrossRef]
- Yang, X.; Lai, S.K.; Wang, C.; Wang, J.M.; Ding, H. On a spring-assisted multi-stable hybrid-integrated vibration energy harvester for ultra-low-frequency excitations. Energy 2022, 252, 124028. [Google Scholar] [CrossRef]
- Chen, Z.Y.; Jiang, W.A.; Chen, L.Q.; Bi, Q.S. Bursting analysis of multi-stable nonlinear mechanical oscillator and its application in energy harvesting. Eur. Phys. J. Spec. Top. 2022, 231, 2223–2236. [Google Scholar] [CrossRef]
- Kundert, K.S.; Sangiovanni-Vincentelli, A.L.; White, J.K. Steady-State Methods for Simulating Analog and Microwave Circuits; Springer: New York, NY, USA, 2010. [Google Scholar]
- Bonani, F.; Cappelluti, F.; Guerrieri, S.D.; Traversa, F.L. Harmonic Balance Simulation and Analysis. In Wiley Encyclopedia of Electrical and Electronics Engineering; Webster, J., Ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2014; pp. 1–16. [Google Scholar] [CrossRef]
- Traversa, F.L.; Bonani, F.; Donati Guerrieri, S. A frequency-domain approach to the analysis of stability and bifurcations in nonlinear systems described by differential-algebraic equations. Int. J. Circuit Theory Appl. 2008, 36, 421–439. [Google Scholar] [CrossRef]
- Traversa, F.L.; Bonani, F. Improved harmonic balance implementation of Floquet analysis for nonlinear circuit simulation. AEU-Int. J. Electron. Commun. 2012, 66, 357–363. [Google Scholar] [CrossRef]
- Traversa, F.; Bonani, F. Frequency-domain evaluation of the adjoint Floquet eigenvectors for oscillator noise characterisation. IET Circuits Devices Syst. 2011, 5, 46. [Google Scholar] [CrossRef]
- Xu, M.; Jin, X.; Wang, Y.; Huang, Z. Stochastic averaging for nonlinear vibration energy harvesting system. Nonlinear Dyn. 2014, 78, 1451–1459. [Google Scholar] [CrossRef]
- Bonnin, M.; Traversa, F.L.; Bonani, F. Analysis of influence of nonlinearities and noise correlation time in a single-DOF energy-harvesting system via power balance description. Nonlinear Dyn. 2020, 100, 119–133. [Google Scholar] [CrossRef]
- Khasminskii, R. On the Principle of Averaging the Ito’s Stochastic Differential Equations. Kibernetika 1968, 4, 260–279. [Google Scholar]
- Zhu, W. Recent developments and applications of the stochastic averaging method in random vibration. Appl. Mech. Rev. 1996, 149, 72–80. [Google Scholar] [CrossRef]
- Zhu, W.; Huang, Z.; Yang, Y. Stochastic averaging of quasi–integrable Hamiltonian systems. J. Appl. Mech. 1997, 64, 975–984. [Google Scholar] [CrossRef]
- Zhu, W.Q.; Yang, Y.Q. Stochastic averaging of quasi-nonintegrable-Hamiltonian systems. J. Appl. Mech. 1997, 64, 157–164. [Google Scholar] [CrossRef]
- Givon, D.; Kupferman, R.; Stuart, A. Extracting macroscopic dynamics: Model problems and algorithms. Nonlinearity 2004, 17, R55. [Google Scholar] [CrossRef]
- Stinis, P. A comparative study of two stochastic mode reduction methods. Phys. D Nonlinear Phenom. 2006, 213, 197–213. [Google Scholar] [CrossRef]
- Chorin, A.; Stinis, P. Problem reduction, renormalization, and memory. Commun. Appl. Math. Comput. Sci. 2007, 1, 1–27. [Google Scholar] [CrossRef]
- Gillespie, D.T. Exact numerical simulation of the Ornstein-Uhlenbeck process and its integral. Phys. Rev. E 1996, 54, 2084. [Google Scholar] [CrossRef] [PubMed]
- Higham, D.J. An algorithmic introduction to numerical simulation of stochastic differential equations. SIAM Rev. 2001, 43, 525–546. [Google Scholar] [CrossRef]
- Higham, D.J. Stochastic ordinary differential equations in applied and computational mathematics. IMA J. Appl. Math. 2011, 76, 449–474. [Google Scholar] [CrossRef]
- Kloeden, P.E.; Platen, E. Stochastic differential equations. In Numerical Solution of Stochastic Differential Equations; Springer: Berlin/Heidelberg, Germany, 1992; pp. 103–160. [Google Scholar]
- Milstein, G.N. Numerical Integration of Stochastic Differential Equations; Springer: Dordrecht, The Netherlands, 1994; Volume 313. [Google Scholar]
- Rößler, A. Runge–Kutta methods for the strong approximation of solutions of stochastic differential equations. SIAM J. Numer. Anal. 2010, 48, 922–952. [Google Scholar] [CrossRef]
- Särkkä, S.; Solin, A. Applied Stochastic Differential Equations; Cambridge University Press: Cambridge, UK, 2019; Volume 10. [Google Scholar]
- Burrage, K.; Burrage, P.; Higham, D.J.; Kloeden, P.E.; Platen, E. Comment on “Numerical methods for stochastic differential equations”. Phys. Rev. E 2006, 74, 068701. [Google Scholar] [CrossRef]











| Mechanical | Electrical |
|---|---|
| Force, f | Voltage, v |
| Displacement, x | Charge, q |
| Momentum | Flux linkage, |
| Mass, m | Inductance L |
| Compliance, | Capacity, C |
| Damping, | Resistance, R |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bonnin, M.; Song, K.; Traversa, F.L.; Bonani, F. A Circuit Theory Perspective on the Modeling and Analysis of Vibration Energy Harvesting Systems: A Review. Computation 2023, 11, 45. https://doi.org/10.3390/computation11030045
Bonnin M, Song K, Traversa FL, Bonani F. A Circuit Theory Perspective on the Modeling and Analysis of Vibration Energy Harvesting Systems: A Review. Computation. 2023; 11(3):45. https://doi.org/10.3390/computation11030045
Chicago/Turabian StyleBonnin, Michele, Kailing Song, Fabio L. Traversa, and Fabrizio Bonani. 2023. "A Circuit Theory Perspective on the Modeling and Analysis of Vibration Energy Harvesting Systems: A Review" Computation 11, no. 3: 45. https://doi.org/10.3390/computation11030045
APA StyleBonnin, M., Song, K., Traversa, F. L., & Bonani, F. (2023). A Circuit Theory Perspective on the Modeling and Analysis of Vibration Energy Harvesting Systems: A Review. Computation, 11(3), 45. https://doi.org/10.3390/computation11030045






