A Stochastic Model for Determining Static Stability Margins in Electric Power Systems
Abstract
:1. Introduction
- The stability margin is determined relying on the calculation of the limiting mode probability, which makes it possible to assess the risk of the planned measures to be taken to change the mode.
- The essential difference between the approach proposed below and the known methods for determining limiting modes is that the corresponding Jacobian matrix is nondegenerate at the solution point, which ensures reliable convergence of computational processes when calculating stability margins [3].
- Reliable result is provided and the iterative process does not converge to the point of a trivial solution corresponding to the initial steady state.
2. Problem Statement
3. Stochastic Approach to Determining the Stability Margin
- The trivial solution corresponds to zero values of the vectors
- The desired solution exists if one or several components of the vectors and are not equal to zero; at the same time, the equations correspond to the condition
| Components of Vector DY | ||
| 0 | ||
| 0 | ||
| 0 |
| Components of Vector DY | ||
| 0 | ||
| 0 |
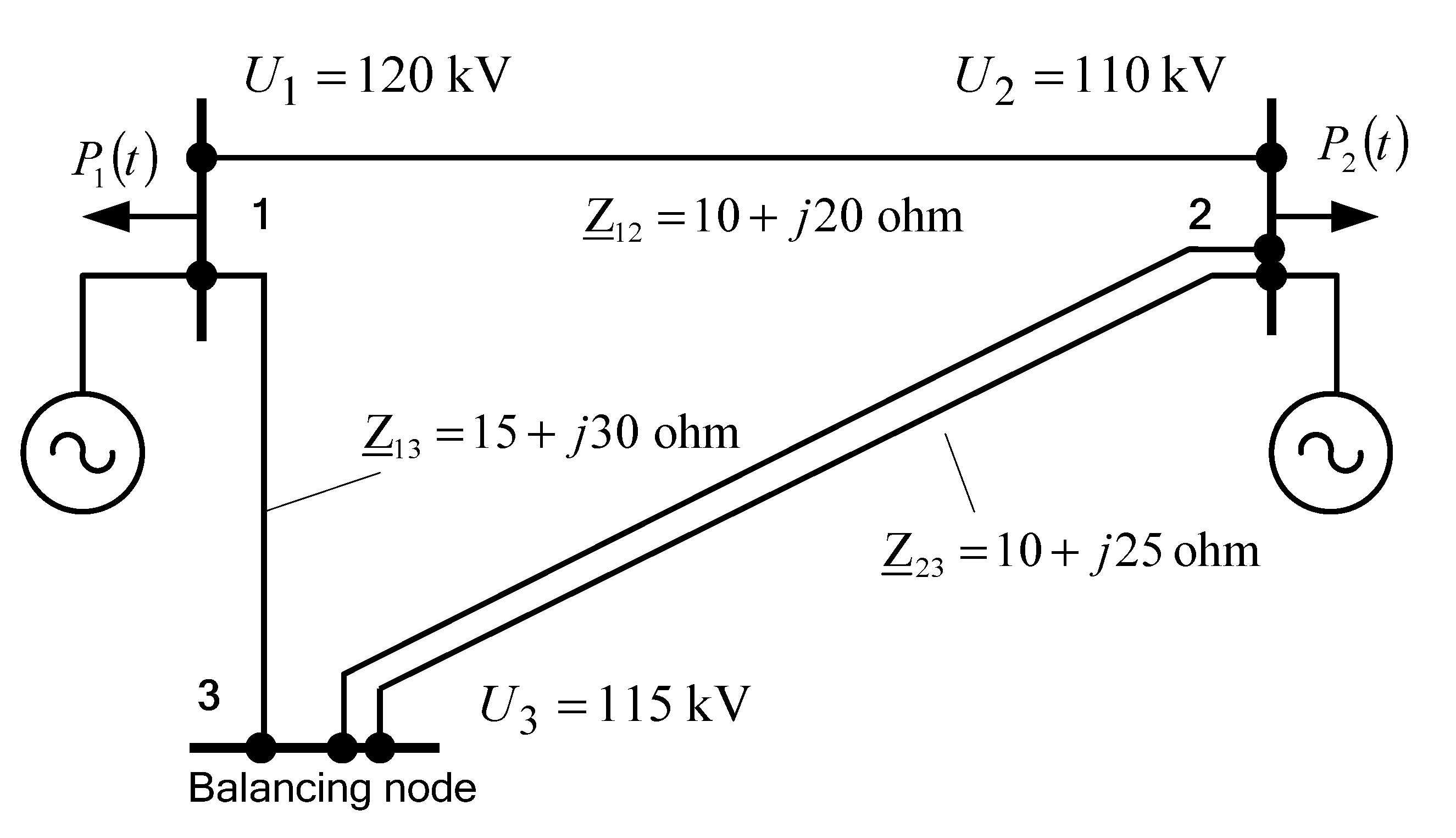
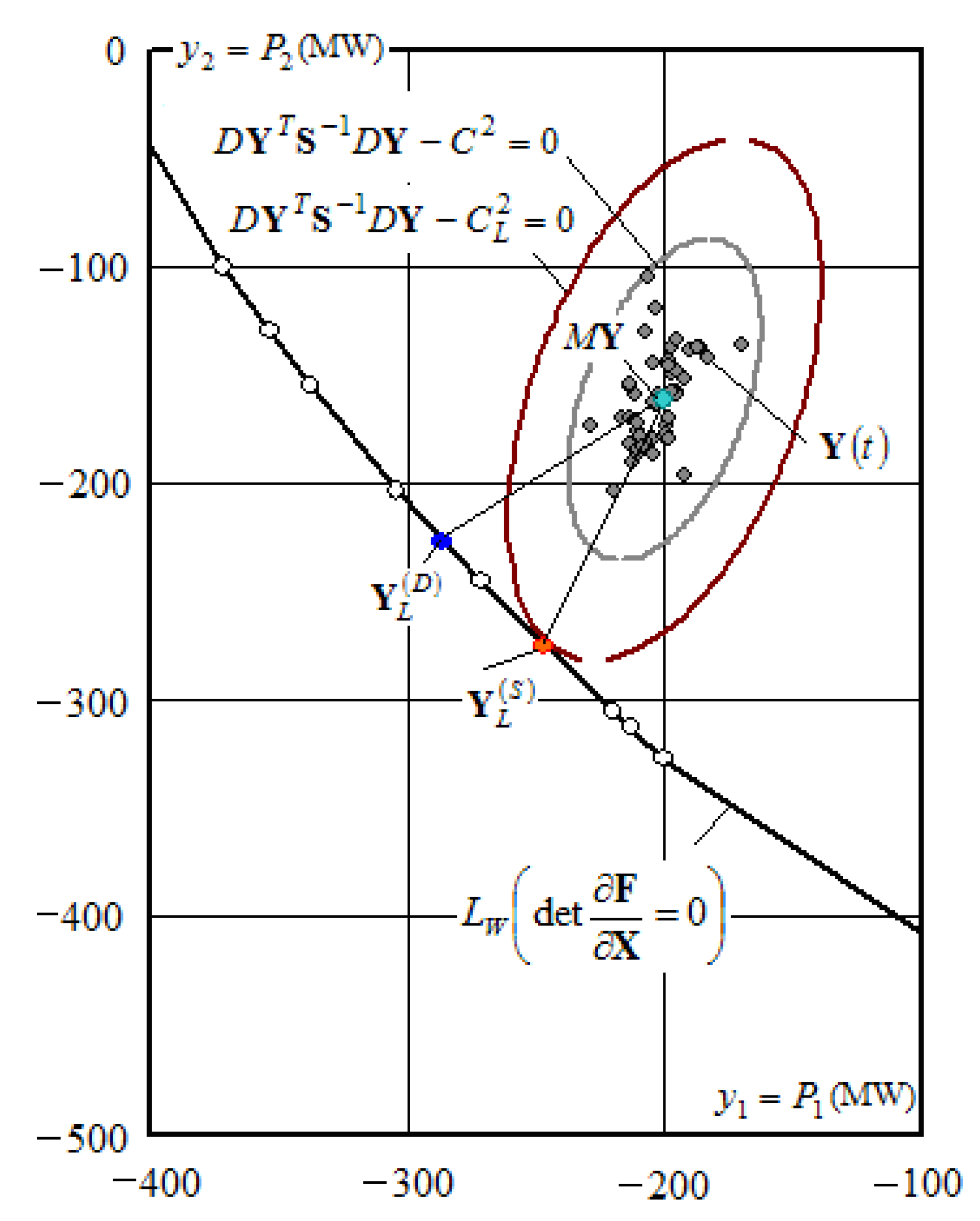
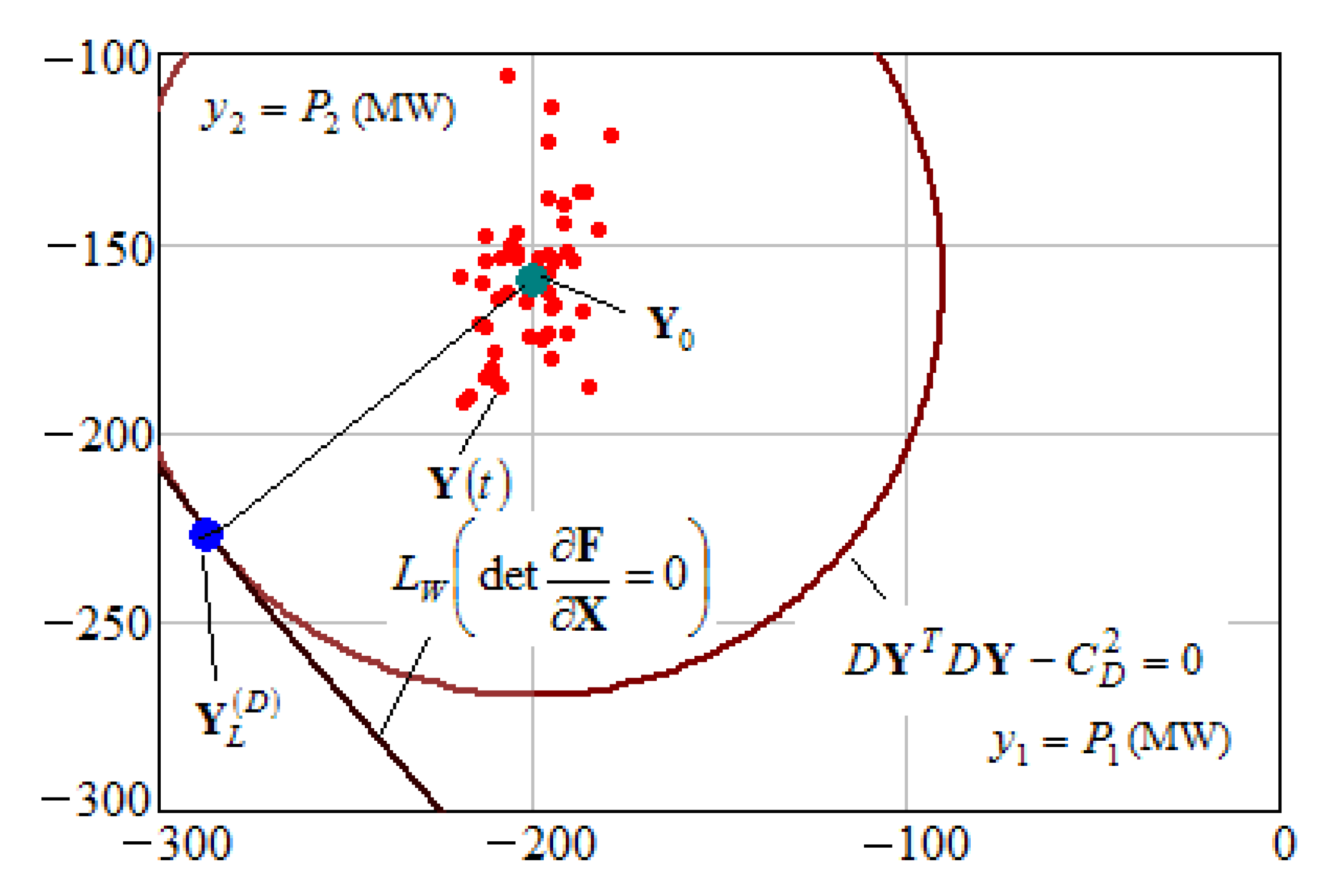
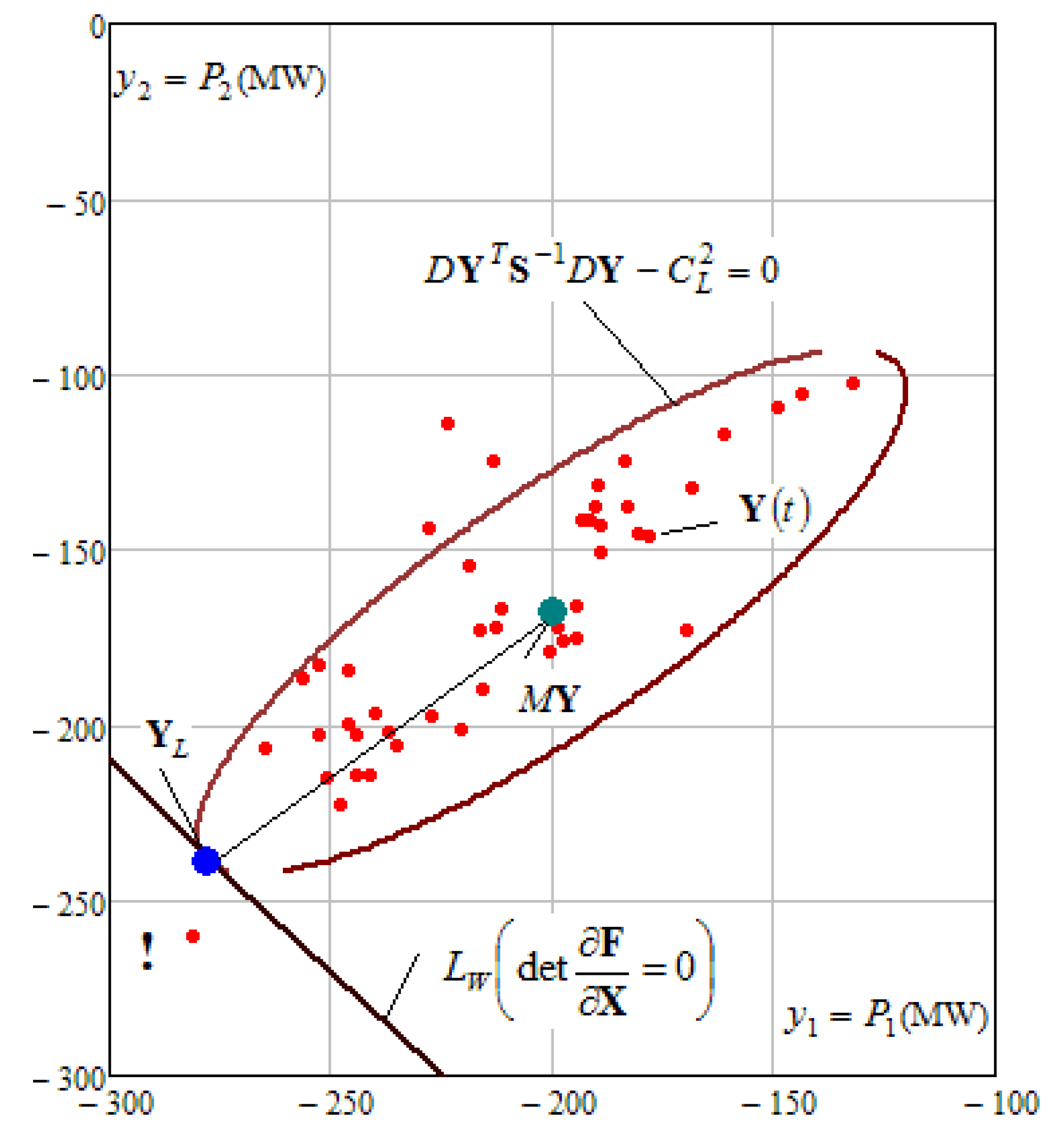
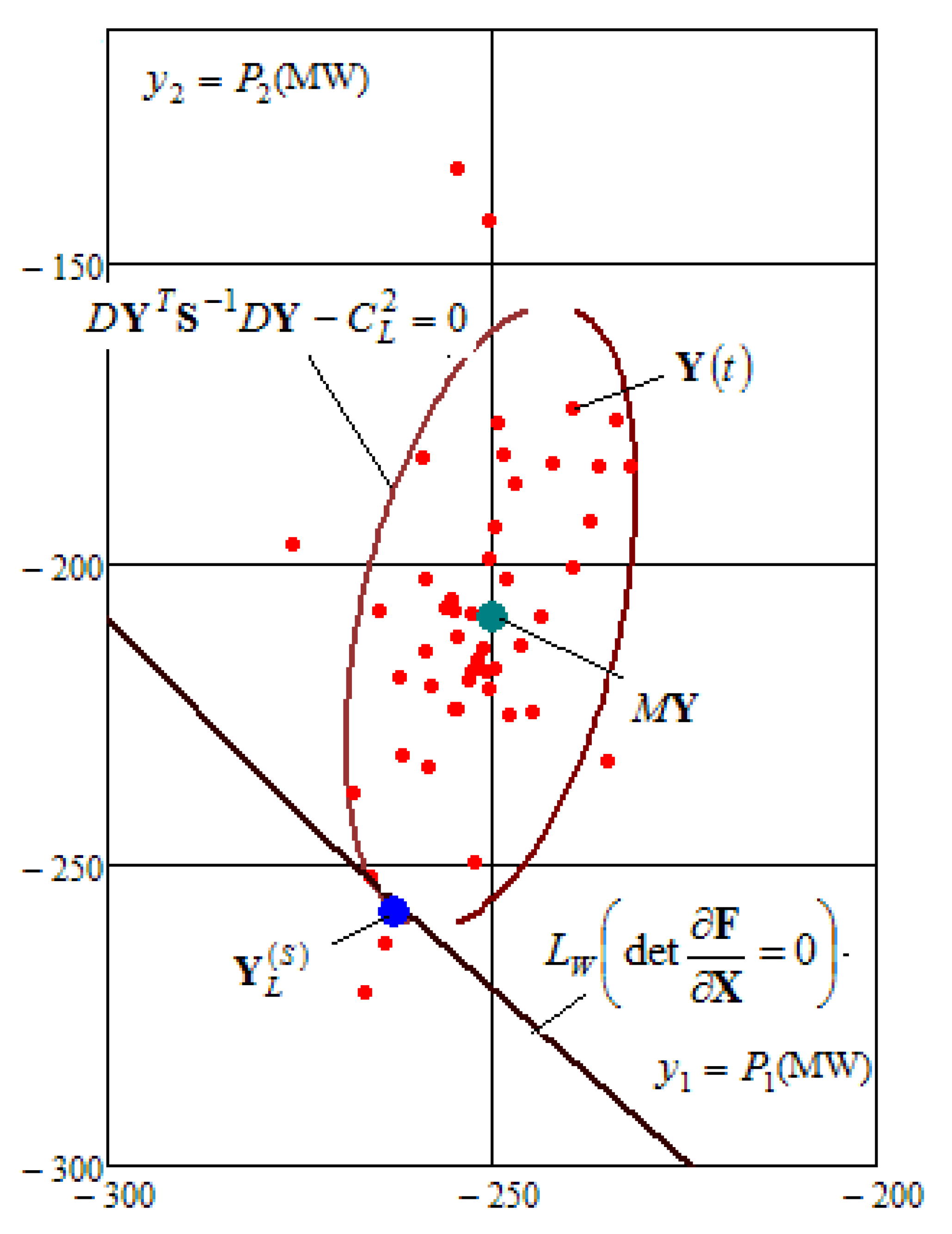
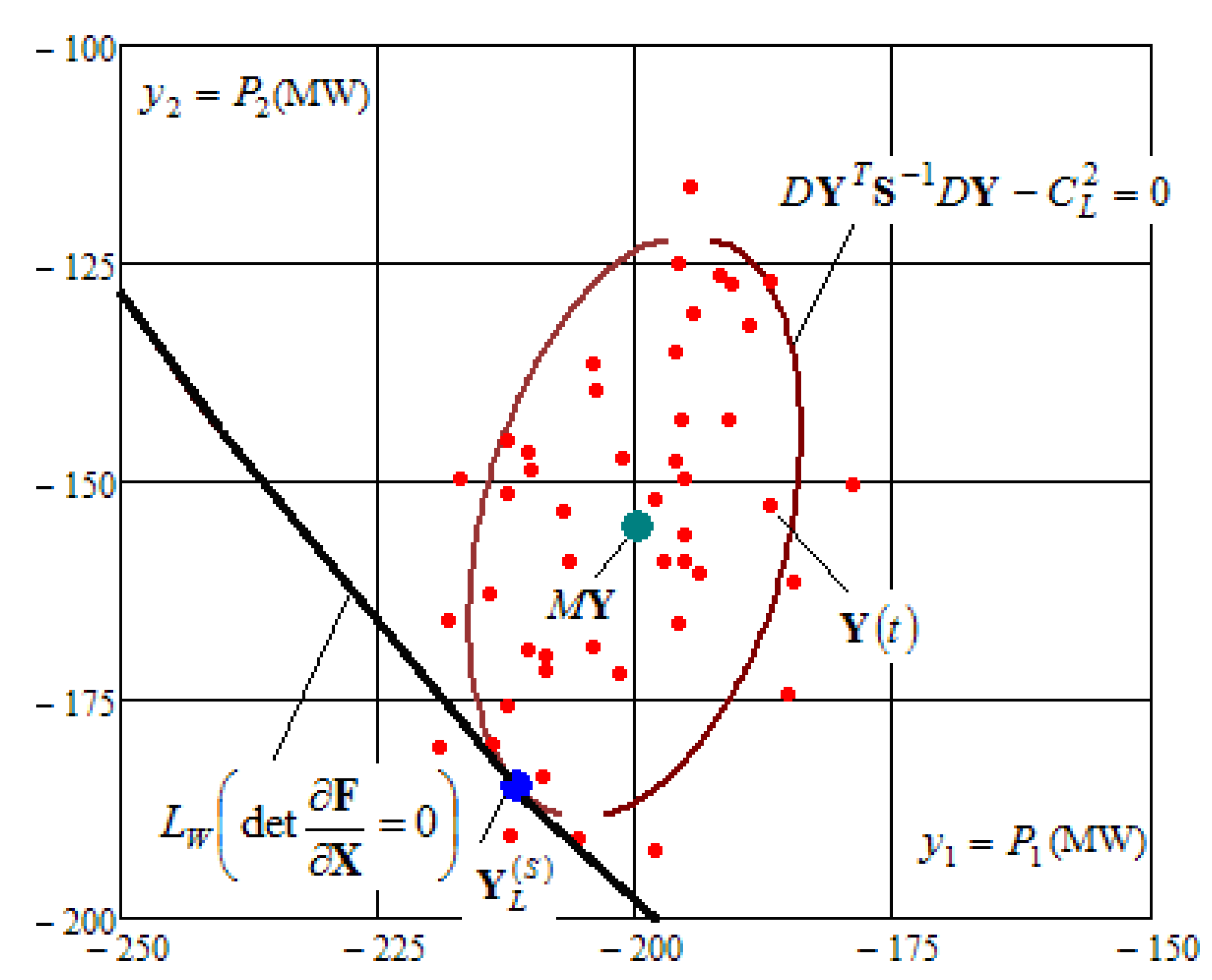
4. Simulation Results
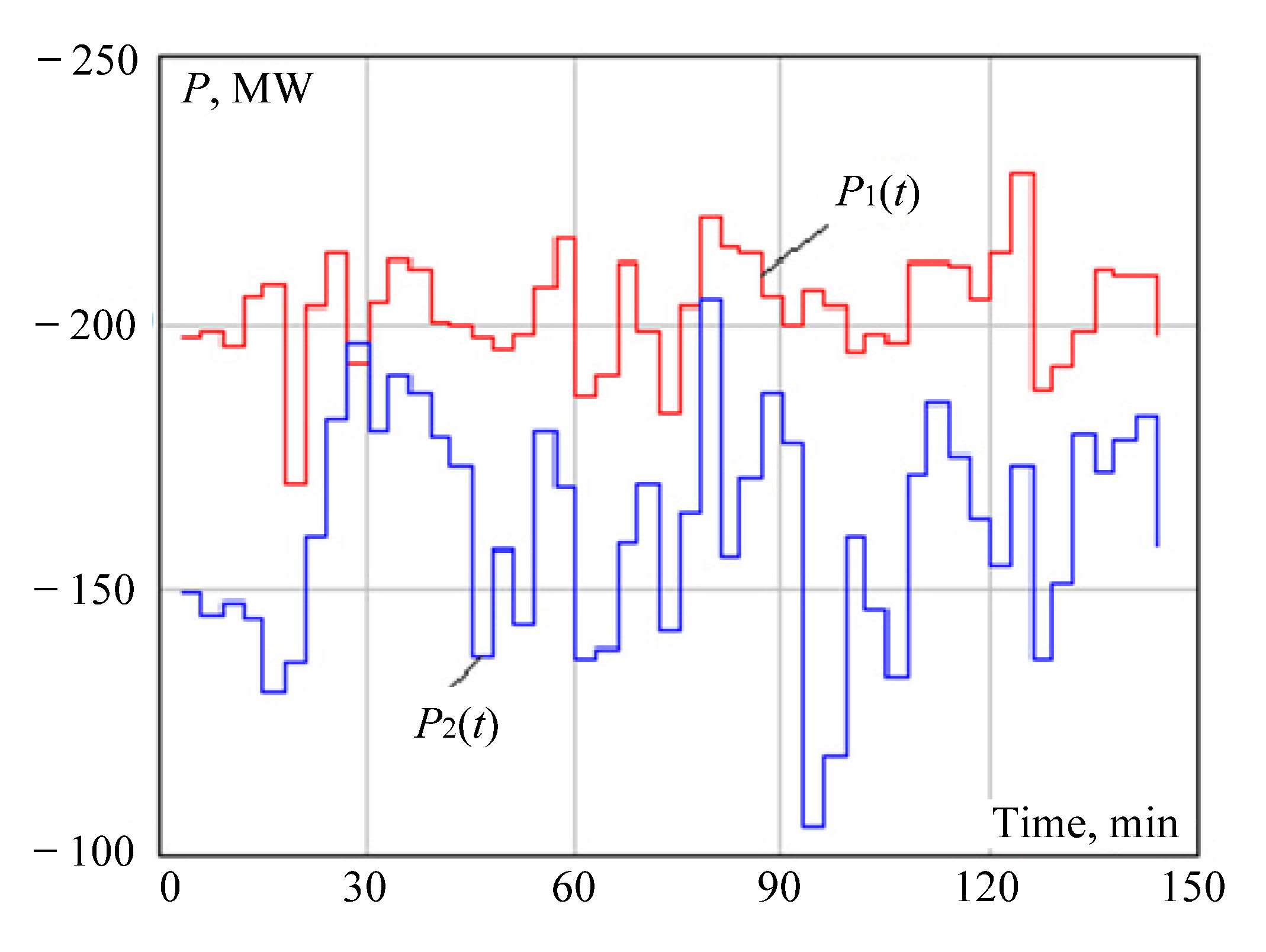
- a stochastic approach was used for consumer loads; at the same time, their capacities changed according to the graphs shown in Figure 2;
- deterministic models traditionally used in practice were employed for the powers of the generator; it should be noted that the algorithm described above can also be used without modifications in the case of a stochastic nature of changes in the capacities of generators.
5. Conclusions
- The modified equations that do not allow the iterative process to converge to the trivial solution ensure high reliability of results when determining the stability margins in a stochastic statement; a technique based on the introduction of an additional variable can be used to improve the convergence of computational processes in determining the stability margins in a deterministic statement.
- The parameters of the limiting modes obtained in the deterministic and stochastic formulations may significantly differ.
- With an increase in the variance of load graphs, the risk of stability violation increases significantly; at the same time, the amount of the margin determined on the basis of the Euclidean norm remains overly optimistic.
- In the illustrative example, a significant increase in the risk of stability violation occurs during planned and emergency shutdowns of the EPS components.
- At present, the concept of cyber-physical systems is being actively developed. It provides the foundation for building modern, efficient, and reliable EPSs. This concept presupposes the use of not only physical objects, but also deep integration of digital components that process a large amount of information coming from sensors, which is then used to simulate and control physical objects. The proposed method for determining the stability margins in a stochastic statement can be effectively used to solve the problems of analyzing SAS in cyber-physical EPS equipped with distributed generation units.
- Further research could be aimed at factoring in the asymmetry of loads when determining the stability margins in a stochastic formulation based on the use of phase coordinates to write the equations of limiting modes [28].
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ayuev, B.I.; Davydov, V.V.; Erokhin, P.M. Models of Closest Marginal States of Power Systems in p-Norms. IEEE Trans. Power Syst. 2018, 33, 1195–1208. [Google Scholar] [CrossRef]
- Ayuev, B.I.; Davydov, V.V.; Erokhin, P.M. Fast and reliable method of searching power system marginal states. IEEE Trans. Power Syst. 2016, 31, 4525–4533. [Google Scholar] [CrossRef]
- Kontorovich, A.M.; Kryukov, A.V. The Use of Equations of Limiting Modes in Problems of Power Systems Control; News of the Academy of Sciences of the USSR Power Engineering and Transportation: Moscow, Russia, 1987; pp. 25–33. [Google Scholar]
- Bulatov, Y.N.; Kryukov, A.V. Prevention of Outages in Power Systems with Distributed Generation Plants. Energy Syst. Res. 2019, 2, 68–83. [Google Scholar]
- Makarov, Y.V.; Ma, J.; Dong, Z.Y. Determining Static Stability Boundaries Using A Non-Iterative Method. In Proceedings of the IEEE Power Engineering Society General Meeting, Tampa, FL, USA, 24–28 June 2007; pp. 1–9. [Google Scholar] [CrossRef]
- Vasin, V.P.; Kondakova, V.G. Investigation of the regions of existence of the regime of electric power systems using power series. Izv. RAS Energy 1995, 1, 47–57. [Google Scholar]
- Kirshtein, B.K.; Litvinov, G.L. Analysis of steady-state modes of electric power systems and tropical geometry of the equations of power balances over complex multipoles. Autom. Telemech. 2014, 10, 110–124. [Google Scholar]
- Misrikhanov, M.S.; Ryabchenko, V.N. Methods for the Analysis of Robust Static Stability of Electric Power Systems. In Modern Control Methods for Dynamic Systems; Energoatomizdat: Moscow, Russia, 2003; pp. 483–498. [Google Scholar]
- Bushuev, V.V.; Polyak, A.D.; Pustovitov, V.I. The use of dominant roots to assess static stability margins. Izv. SB AS USSR Ser. Eng. Sci. 1973, 6, 98–104. [Google Scholar]
- Venikov, V.A.; Stroyev, V.A.; Idelchik, V.I.; Vinogradov, A.A. Calculation of the static stability margin of the electrical system. Izv. AS USSR Energy Transp. 1984, 3, 56–65. [Google Scholar]
- Sizhuo, L.; Chao, Z.; Xinfu, S.; Pan, L. Study on the Influence of VSC-HVDC on Receiving Power Grid Voltage Stability Margin and Improvement Measures. In Proceedings of the International Conference on Power System Technology (POWERCON), Guangzhou, China, 6–9 November 2018; pp. 2503–2508. [Google Scholar] [CrossRef]
- Bharati, A.K.; Ajjarapu, V. Investigation of Relevant Distribution System Representation with DG for Voltage Stability Margin Assessment. in IEEE Trans. Power Syst. 2020, 35, 2072–2081. [Google Scholar] [CrossRef]
- Chen, Y.; Mazhari, S.M.; Chung, C.Y.; Faried, S.O.; Pal, B.C. Rotor Angle Stability Prediction of Power Systems with High Wind Power Penetration Using a Stability Index Vector. IEEE Trans. Power Syst. 2020, 35, 4632–4643. [Google Scholar] [CrossRef]
- Saleh, M.S.; Althaibani, A.; Esa, Y.; Mhandi, Y.; Mohamed, A.A. Impact of clustering microgrids on their stability and resilience during blackouts. In Proceedings of the International Conference on Smart Grid and Clean Energy Technologies (ICSGCE), Offenburg, Germany, 20–23 October 2015; pp. 195–200. [Google Scholar]
- Mohsen, F.N.; Amin, M.S.; Hashim, H. Application of smart power grid in developing countries. In Proceedings of the IEEE 7th International Power Engineering and Optimization Conference (PEOCO), Langkawi, Malaysia, 3–4 June 2013. [Google Scholar] [CrossRef]
- Wang, J.; Huang, A.Q.; Sung, W.; Liu, Y.; Baliga, B.J. Smart Grid Technologies. IEEE Ind. Electron. Mag. 2009, 3, 16–23. [Google Scholar] [CrossRef]
- Olivares, D.E.; Mehrizi-Sani, A.; Etemadi, A.H.; Cañizares, C.A.; Iravani, R.; Hajimiragha, A.H.; Gomis-Bellmunt, O.; Saeedifard, M.; Palma-Behnke, R.; Jiménez-Estévez, G.A.; et al. Trends in Microgrid Control. IEEE Trans. Smart Grid 2014, 5, 1905–1919. [Google Scholar] [CrossRef]
- Bulatov, Y.; Kryukov, A.; Suslov, K.; Shamarova, N. Ensuring postemergency modes stability in power supply systems equipped with distributed generation plants. In Proceedings of the 10th International Scientific Symposium on Electrical Power Engineering «Elektroenergetika 2019», Stara Lesna, Slovakia, 16–18 September 2019; pp. 38–42. [Google Scholar]
- Kumar, R.; Singh, R.; Ashfaq, H. Stability enhancement of multi-machine power systems using Ant colony optimization-based static Synchronous Compensator. Comput. Electr. Eng. 2020, 83, 106589. [Google Scholar] [CrossRef]
- Kumar, R.; Diwania, S.; Singh, R.; Ashfaq, H.; Khetrapal, P.; Singh, S. An intelligent Hybrid Wind-PV farm as a static compensator for overall stability and control of multi-machine power system. ISA Trans. 2021, 123, 286–302. [Google Scholar] [CrossRef] [PubMed]
- Sarathkumar, D.; Kavithamani, V.; Velmurugan, S.; Santhakumar, C.; Srinivasan, M.; Semiannual, R. Power system stability enhancement in two machine system by using fuel cell as STATCOM (static synchronous compensator). Mater. Today Proc. 2021, 45, 2130–2138. [Google Scholar] [CrossRef]
- Yang, F.; Ling, Z.; Wei, M.; Mi, T.; Yang, H.; Qiu, R.C. Real-time static voltage stability assessment in large-scale power systems based on spectrum estimation of phasor measurement unit data. Int. J. Electr. Power Energy Syst. 2021, 124, 106196. [Google Scholar] [CrossRef]
- Lombardi, P.; Sokolnikova, T.; Suslov, K.; Voropai, N.; Styczynski, Z. Isolated power system in Russia: A chance for renewable energies? Renew. Energy 2016, 90, 523–541. [Google Scholar] [CrossRef]
- Park, K.J.; Zheng, R.; Liu, X. Cyber-physical Systems: Milestones and Research Challenges. Editor. Comput. Commun. 2012, 36, 1–7. [Google Scholar] [CrossRef]
- Khaitan, S.K.; McCalley, J.D. Design techniques and applications of cyberphysical systems: A survey. IEEE Syst. J. 2014, 9, 350–365. [Google Scholar] [CrossRef]
- Gamm, A.Z. Statistical Methods for Assessing the State of Electric Power Systems; Nauka: Moscow, Russia, 1976. [Google Scholar]
- Pichugin, Y.A.; Malafeev, O.A. On the assessment of the bankruptcy risk of the company. In Proceedings of the International Conference on Dynamical Systems: Stability, Management, Optimization, Minsk, Belarus, 1–5 October 2013. [Google Scholar]
- Zakaryukin, V.P.; Kryukov, A.V. Complicated Asymmetrical Modes of Electrical Systems; Irkutsk State Transport University: Irkutsk, Russia, 2005; p. 273. [Google Scholar]
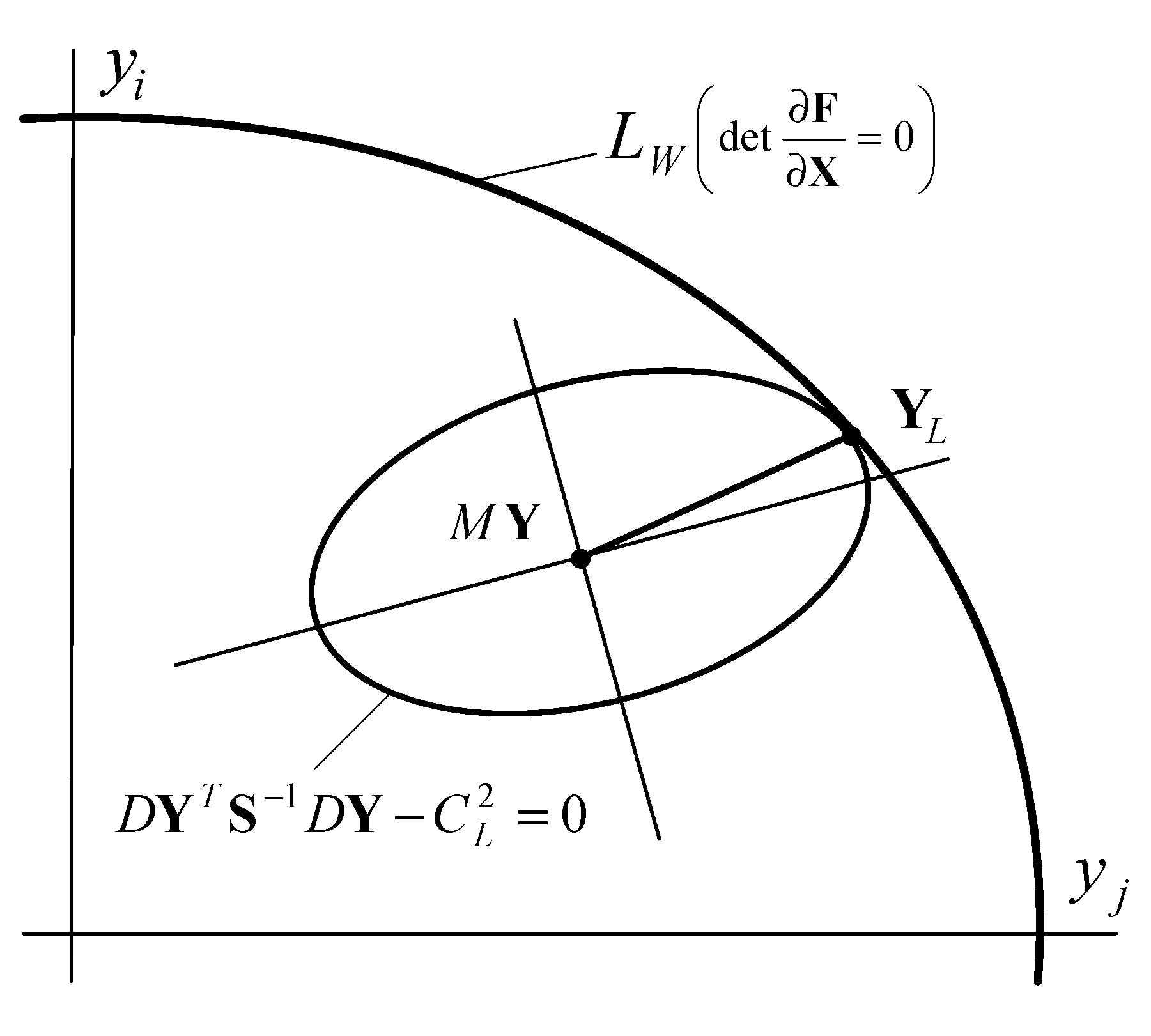


| Z, % | |||||
|---|---|---|---|---|---|
| 10 | 6.4 | 1.28·10–7 | –248.00 | –275 | 74.76 |
| 20 | 4.03 | 0.03 | –273.00 | –245 | 63.67 |
| 30 | 2.5 | 4.4 | –276.00 | –241 | 62.55 |
| 40 | 1.9 | 16.4 | –278.00 | –239 | 62.19 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bulatov, Y.; Kryukov, A.; Senko, V.; Suslov, K.; Sidorov, D. A Stochastic Model for Determining Static Stability Margins in Electric Power Systems. Computation 2022, 10, 67. https://doi.org/10.3390/computation10050067
Bulatov Y, Kryukov A, Senko V, Suslov K, Sidorov D. A Stochastic Model for Determining Static Stability Margins in Electric Power Systems. Computation. 2022; 10(5):67. https://doi.org/10.3390/computation10050067
Chicago/Turabian StyleBulatov, Yuri, Andrey Kryukov, Vladislav Senko, Konstantin Suslov, and Denis Sidorov. 2022. "A Stochastic Model for Determining Static Stability Margins in Electric Power Systems" Computation 10, no. 5: 67. https://doi.org/10.3390/computation10050067
APA StyleBulatov, Y., Kryukov, A., Senko, V., Suslov, K., & Sidorov, D. (2022). A Stochastic Model for Determining Static Stability Margins in Electric Power Systems. Computation, 10(5), 67. https://doi.org/10.3390/computation10050067








