Fundamentals of Natural Representation
Abstract
1. Introduction and Definitions
2. The Basis of Natural Representation
2.1. Causal Correlation, Coherence Relation, and Representation
2.2. Non-Uniqueness of State and Transmission of Correlation
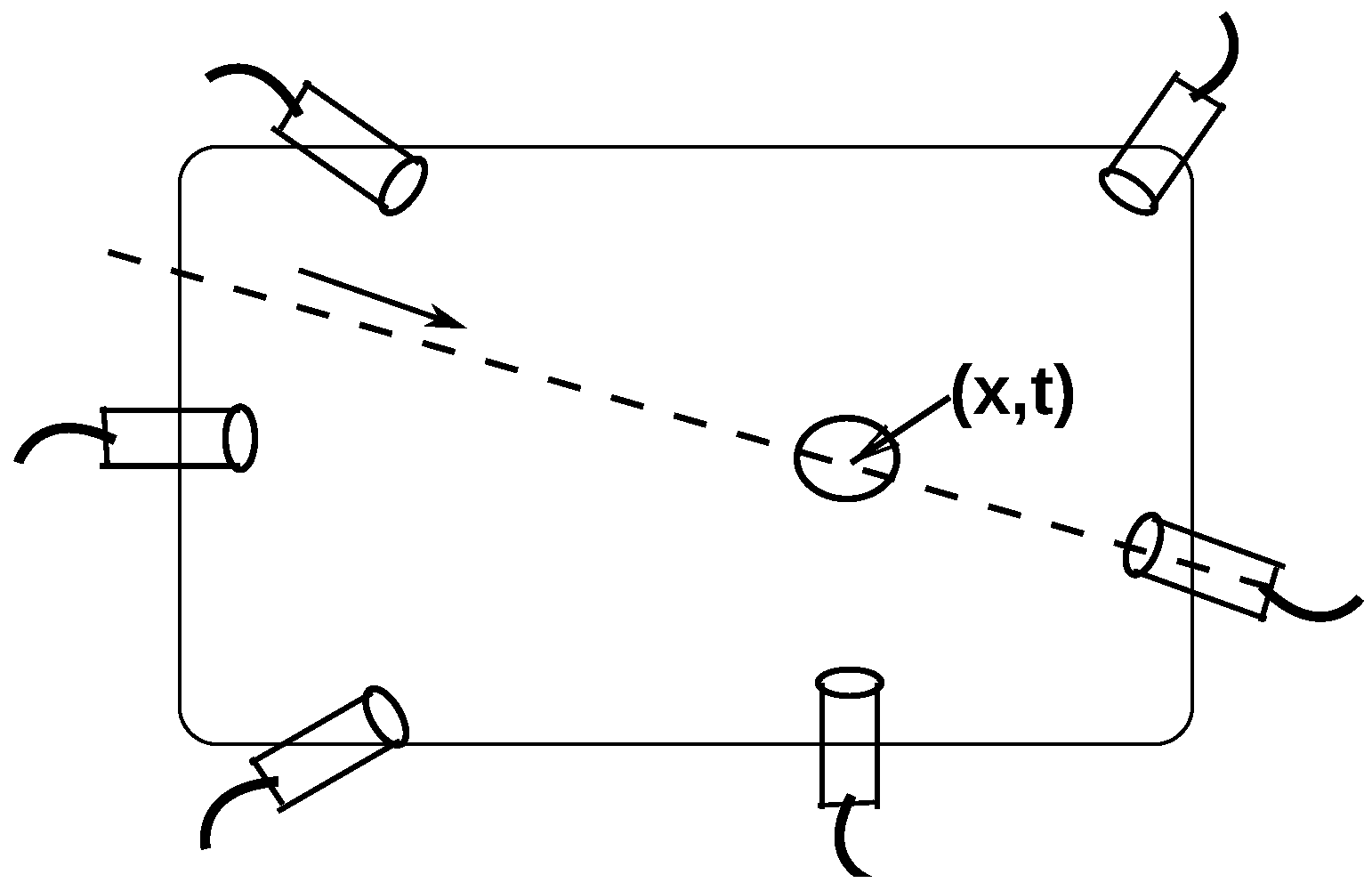
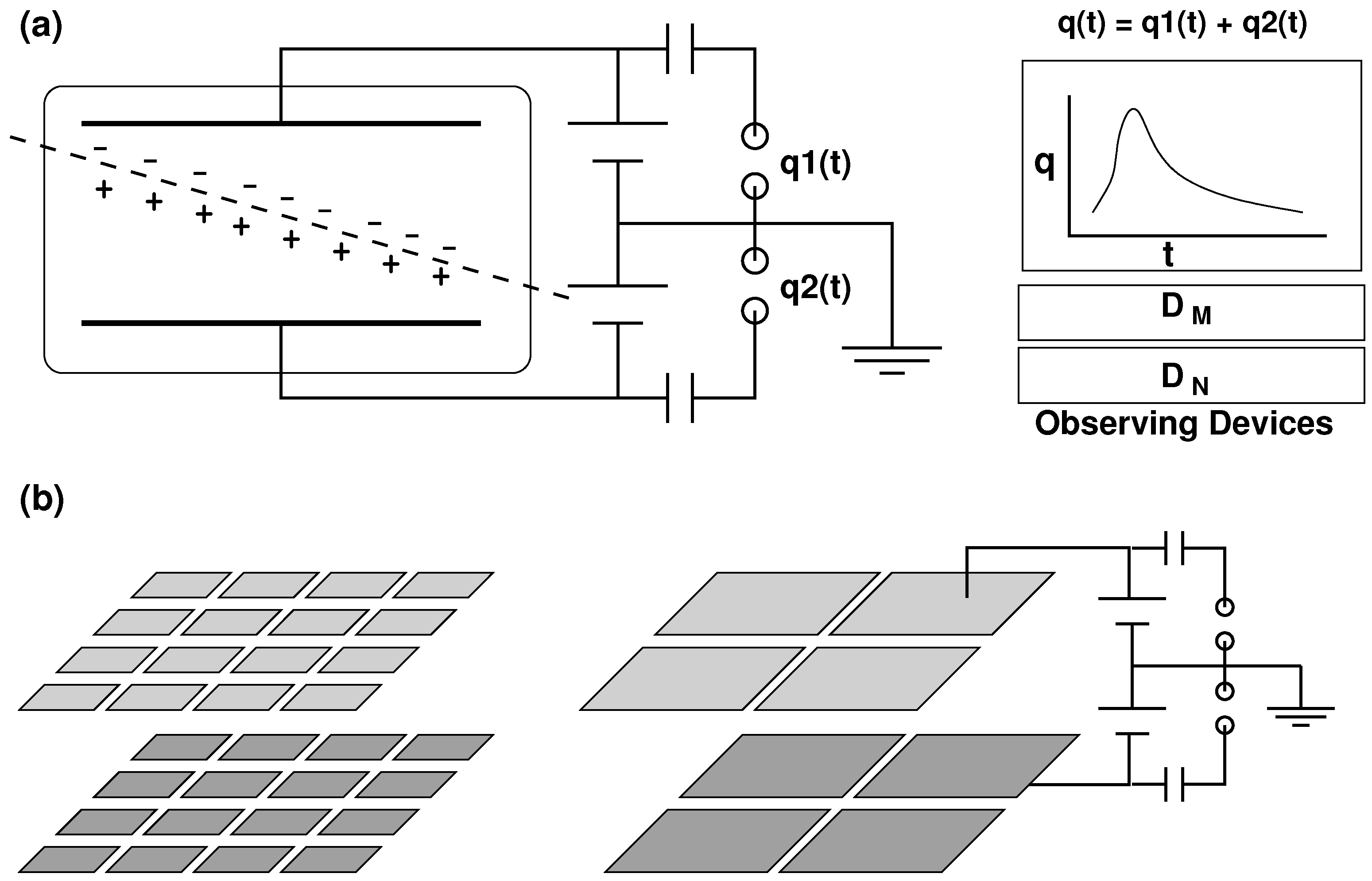
- While the existential state of the anode at the moment of measurement must correlate with the conjunction of specific correlations of states of M electrons which, in reality, converge on the anode, the state of the observing device, , even intrinsically, can not distinguish between the accumulation of N electrons or more on the observed anode.
- The device, , functions as a filter responding to certain state description of the anode that is neither comprehensive nor absolute. Similarly, the observed state of the anode depends on the electron’s state of availability within spatio-temporal limits; the energy-momentum component remains irrelevant to this state description. All PSRs, microscopic or macroscopic, function as filters, selecting only certain relative measures. Therefore, with respect to the correlation with information, a default comprehensive description of state of a PSR is immaterial and irrelevant—it is the observed state that remains relevant.
- As per the natural limits of causation, the active state of also must correlate with what the threshold current at the anode correlates with. The transmitted value of the semantics of correlation from one physical entity to another is dependent on the limit of state of one, as observed by another—the greater the specificity of the state observed, the more specific its transmitted semantics of correlation is.
- The state description of the anode as observed by can be satisfied by all combinations of N or more electrons accumulating during the period of measurement. Each combination is a conjunction of specific set of electrons. Therefore, the active state of correlates with terms in disjunction, where each term evaluates a conjunction of relevant states of subsets of greater than or equal to N electrons. The disjunctive relation is not equivalent to the conjunction of M electrons; therefore, the semantics of correlation of the observed state is not equivalent to that of the comprehensive existential state of the anode.
- Each combination of N or more electrons together must correlate with the conjunction of correlations of their specific states relevant for their convergence. The disjunction of such conjunctions makes the semantics independent of individually specific correlations of electrons and of their conjunctions, where each conjunction correlates with the heavy ion event. Therefore, the state of is said to represent the generic semantics of the ‘heavy ion event’ but without the particular correlations of electrons and their conjunctions. Here, the usage shows a distinction between the terms ‘correlation’ and ‘representation’, while the state is said to correlate with each conjunction, but it represents the value of disjunction of all correlations. This forms a mechanism to transmit the semantics of relation among the states and their respective correlations but without the specifics of states. Correlation is a more general term than representation, since correlation may also refer to the value represented by a state.
- A ‘heavy ion event’ is a generic, but constant, discrete semantic value which does not describe a real unique physical heavy ion or a real physical process, because the ions with a range of states and events with different specifics are all be referred to by the same value. Yet, even in scientific parlance, it is usually taken to be an objective description of physical reality. In fact, it describes an object (a process) corresponding to a common noun semantic value, an equivalence class. While the primary aim of the experiment is to detect the ‘heavy ion events’, that happens to be a class description, not an element of physical reality.
- The interaction of a heavy ion with the atoms, and of the electrons with the anode, causes the creation of a relation among the interacting entities, the PSRs. Every physical entity (PSR) plays the role of an observer, specific interaction as the mode of observation, and causally accountable descriptions of interacting entities as objects observed. Under a quantum mechanical consideration, an interaction is complete when decoherence occurs; therefore, the separation of the observer and the observed has no issues here. A PSR acquires a relative identity due to differential causal accountability. One may refer to Chris Fields [19] for a discussion on other notions of what constitutes an observer.
- Let us consider two possible instances for the threshold activated device—one, which turns active when N or more electrons accumulate within the specified time, , and another, when M or more electrons accumulate in the same period of time, . In the case considered, the anode receives M or higher number of electrons activating both the devices. Descriptions of specific configurations of physical states or processes that could not cause sufficiently greater than N electrons to be deposited in the given time duration to activate , then the class of such states, C, would negatively correlate with the state of the anode, which indeed receives electrons, and with the active state of . Class C may include specific combinations of high energy ions in conjunction with specific lower densities of the argon gas. However, the instances of the same class are positively correlated with the state of the device that activates with N electrons only. If the purpose of the experiment is merely to count the events, then detects more events than , i.e, the active state of is more specific than that of . Moreover, the instances that negatively correlate with correlate positively with for the same experiment. Furthermore, a conjunction of these two correlates must conform to the one with greater specificity, whereas a disjunction of the two must correlate with the one that encapsulates both, since it is not guaranteed that the more specific correlation is the one that caused the result of the disjunction; the less specific correlation always encapsulates the more stringent limits. Therefore, while a conjunction of the two yields a negative correlation with class C, the disjunction yields a positive correlation.
2.3. State as a Non-Discrete Value and Its Semantics of Correlation
2.4. Relative Measures Form Elements of State Description
3. Symbolic Interpretation of the Semantics of Correlation
- A given set of arguments merely covers one possible instance within the limits of all possible correlations. In addition, the state description of the PSR under consideration may itself be one of the parameters in the list expressing the correlation of the state with other values in the list.
- In order to have all objects represented in complete generality, the parametric space and its respective argument values may come from all realities and all expressible semantics.
- It is possible though that a state may bear a positive correlation with a contrast relation among semantic values; this is not to be confused with the positive and negative correlations with the contrasting values. For example, active states of some retinal ganglion cells may positively correlate with either on-center or off-center receptive fields [21,22] that bear contrast relation between the center and the surround in the field of view. Yet, this is not the same as the positive correlation with the center and the negative correlation with the surround or vice versa. However, it may be true that a positive correlation with an on-center contrast relation may imply a negative correlation with the off-center relation. This is equivalent to the statement that if a state correlates positively with , then it bears negative correlation with .
3.1. Conjunction and Disjunction as Semantic Quantifiers
- The s are not necessarily discrete, making it more general and potent to represent entire object space with a finite number of elements.
- The expression permits overlaps among the profiles of s, again enhancing the generality and the scope for achieving arbitrary precision.
- The list of s may not necessarily cover the entire space to form a class, even though a partial class allows for representation of abstract objects within the range of observation at the cost of completeness—a good method for observing systems to build abstractions that do not have access to the entire space or when entirety of space is undefined.
4. Transience of Information and Emergence of Models
4.1. Emergence of Quantitative Models, Units, and Scale
5. Implications of Representation on Physical Sciences
5.1. Preservation of Correlation on Extreme Scales
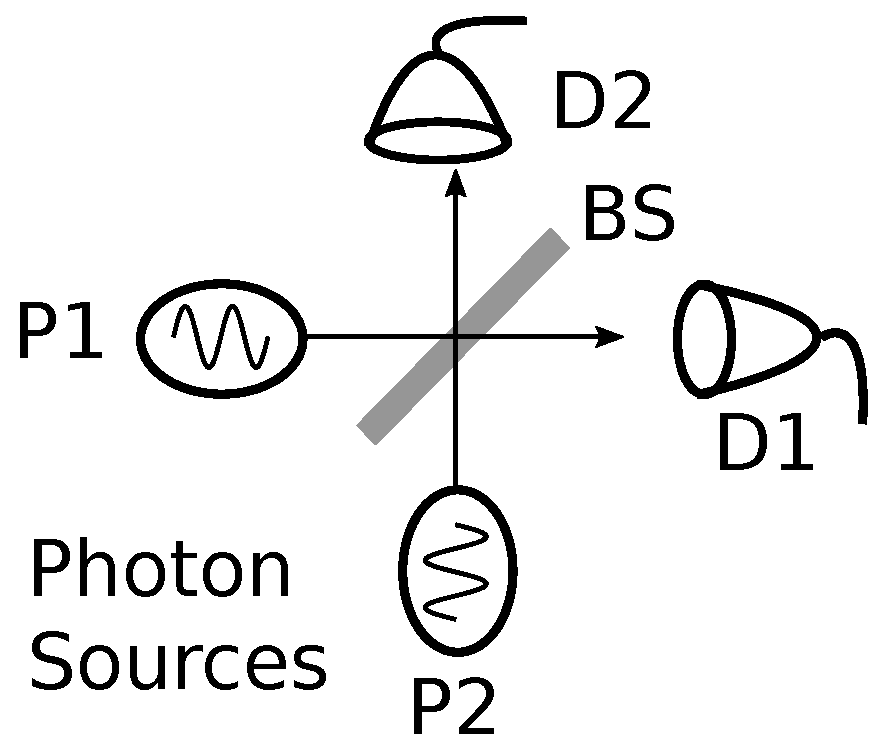
- Interaction-free evolution of states over large distances and long times preserves objective causal correlation.
- Interaction-free evolution of coherent systems preserve mutual coherence, and therefore preserve the semcoherence.
- Interactions of correlated microscopic states with macroscopic systems that do not differentially probe the correlated states preserve the mutual correlation, as is evident from radio waves interacting with the interstellar magnetic field, and coherent photons encountering a lens, or beam splitters that modify their state.
- Non-local spatio-temporal causations, if any, remain transparent to the natural correlations.
- Every interaction results in the exchange of information among interacting entities. Observer-observed relation must hold among the entities. Therefore, each resultant state must bear the semantics of the correlation of the limits of states observed, irrespective of their micro or macro physical limits. That is, even the resultant states of macroscopic entities must correlate with exchanged semantics of correlation of microscopic entity (the implications are to be discussed elsewhere).
5.2. Physical Sciences in the Light of Natural Representation
6. The Laws of Natural Representation
- The genesis of an accountable physical entity and its associated state is based exclusively on the limits of constancy (uniform regularity) of cause and effect relation in the nature of change.
- The perspective of the semantic value of representation is based on the observable state of a physical entity that serves as a reference state of a reference physical entity.
- Post-interaction, the observable resultant state, S, of a physical entity, P, represents a definite semantic value, C, that is derived from all causally equivalent configurations of reality, describable in terms of states of interacting entities, that result in the state S of entity P. The semantic value C is constituted of the following components.
- (a)
- A value that is equivalent to disjunction of conjunctions of values of respective states in each configuration.
- (b)
- A value that is equivalent to disjunction of conjunctions of semantic values of correlation of respective states in each configuration.
7. A Simulation of Information Processing
Specifics of the Method
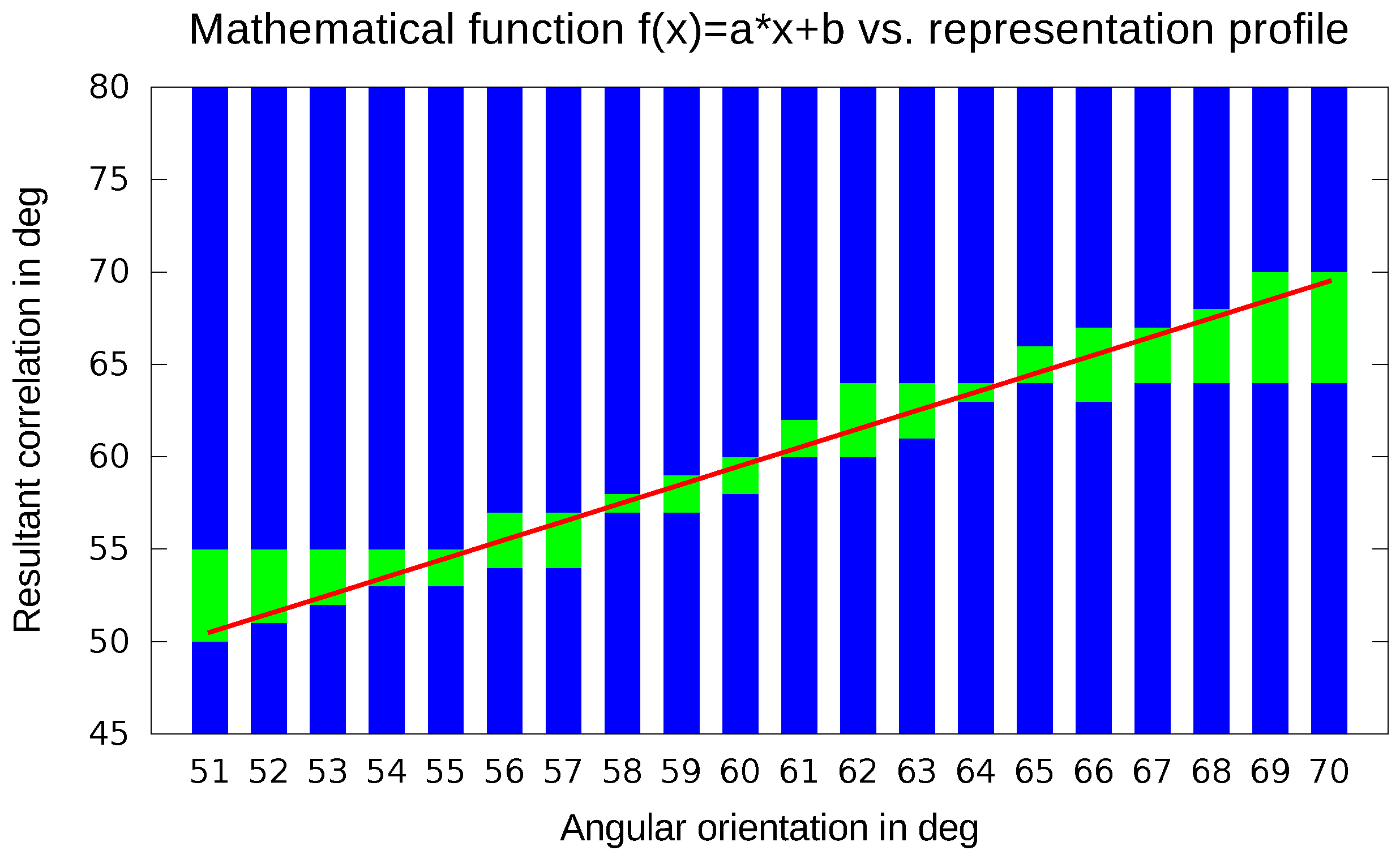
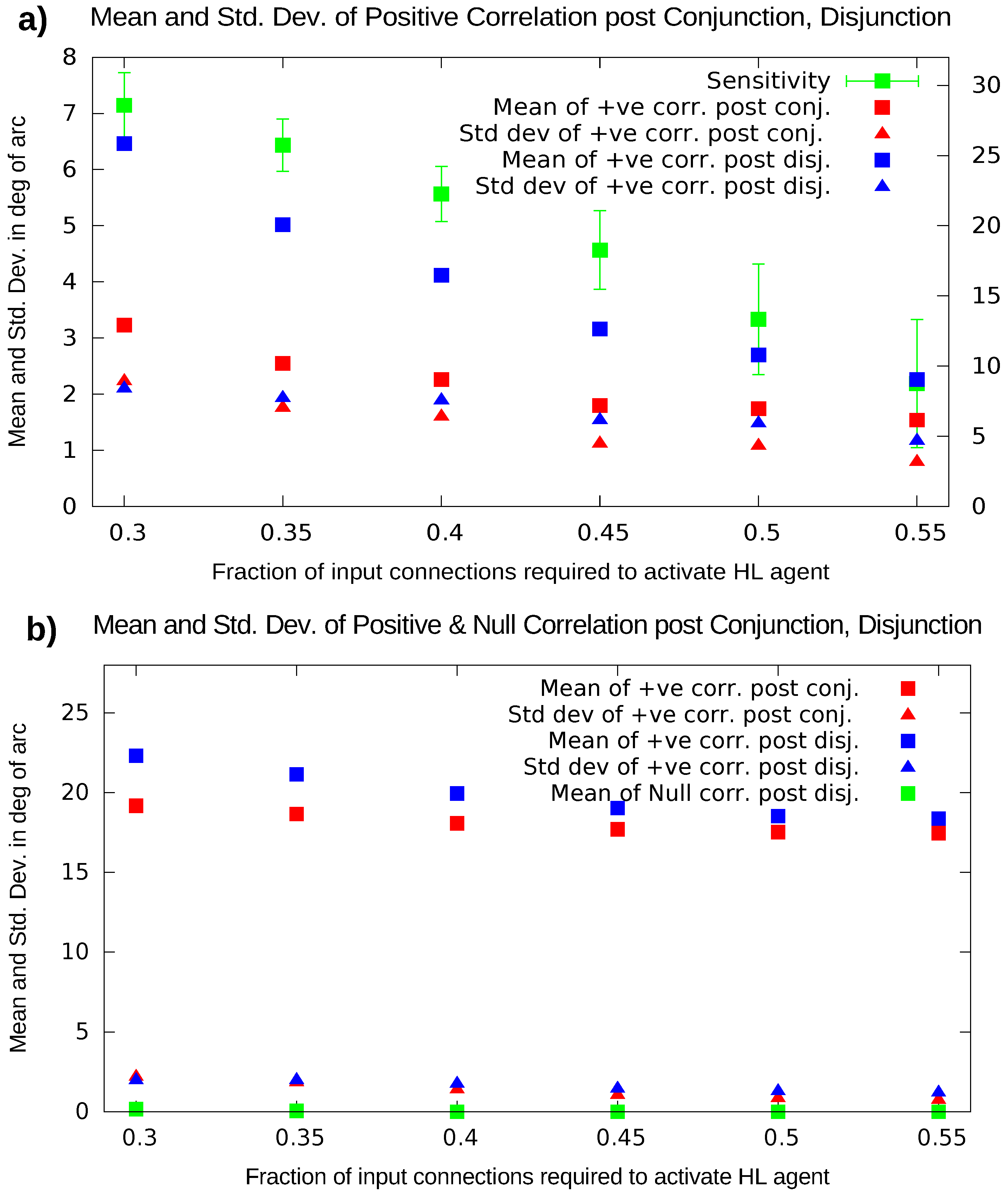
- It is evident from the results of the simulation that the specificity of the correlation profile of an HL agent, when it turns active, is far greater than what is originally assigned to LL or HL agents, yet it remains consistent with the presented orientation. The enhancement in specificity is a result of diverse negative correlation range of LL agents.
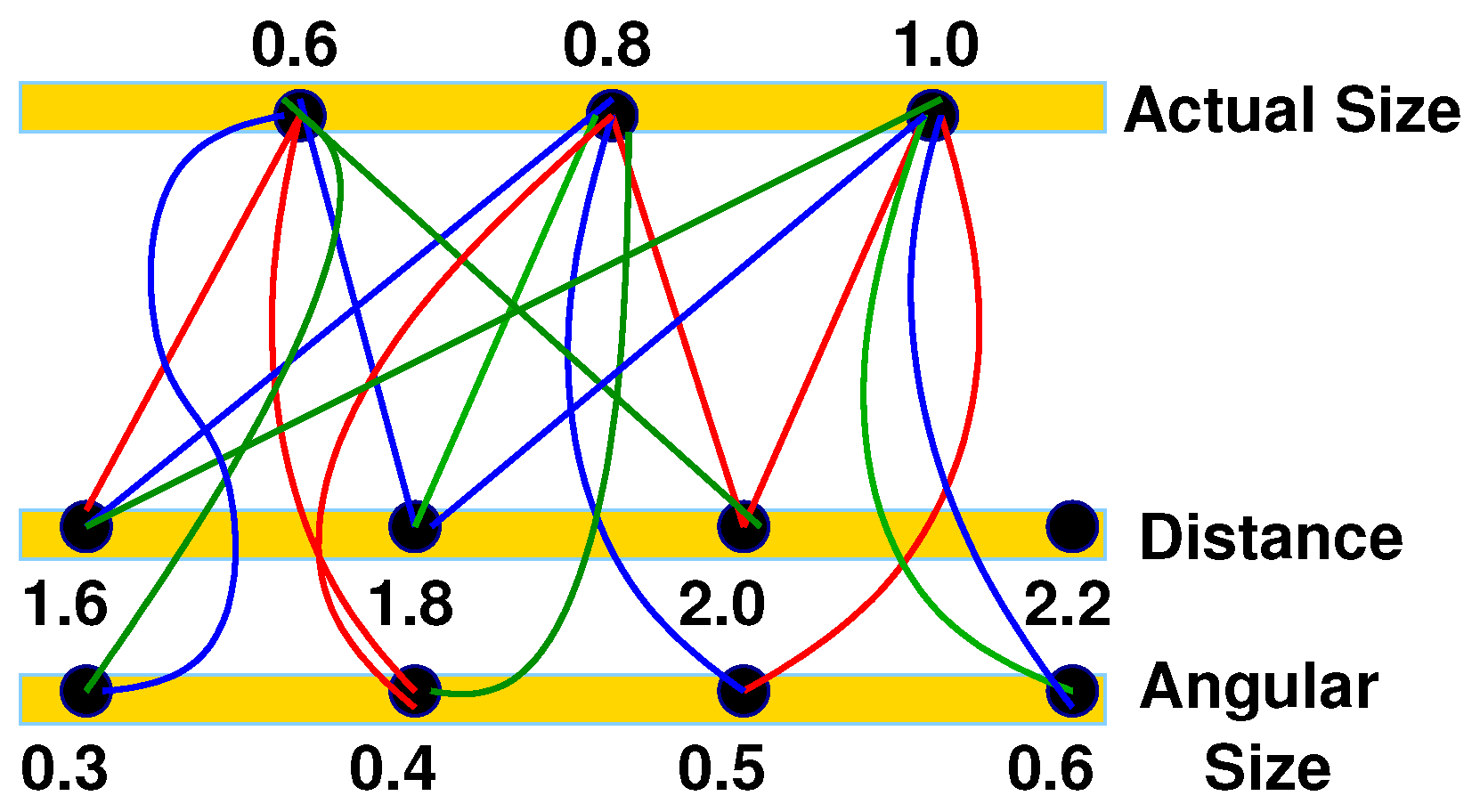
- This method of processing forms a good example of coarse coding, yet it achieves high specificity in its results. When the method is conjugated with the relation mapping scheme, as shown in Figure 5, the potential in generic information processing becomes apparent. Mapping represents relations among arbitrary objects, and coarse coding achieves greater specificity.
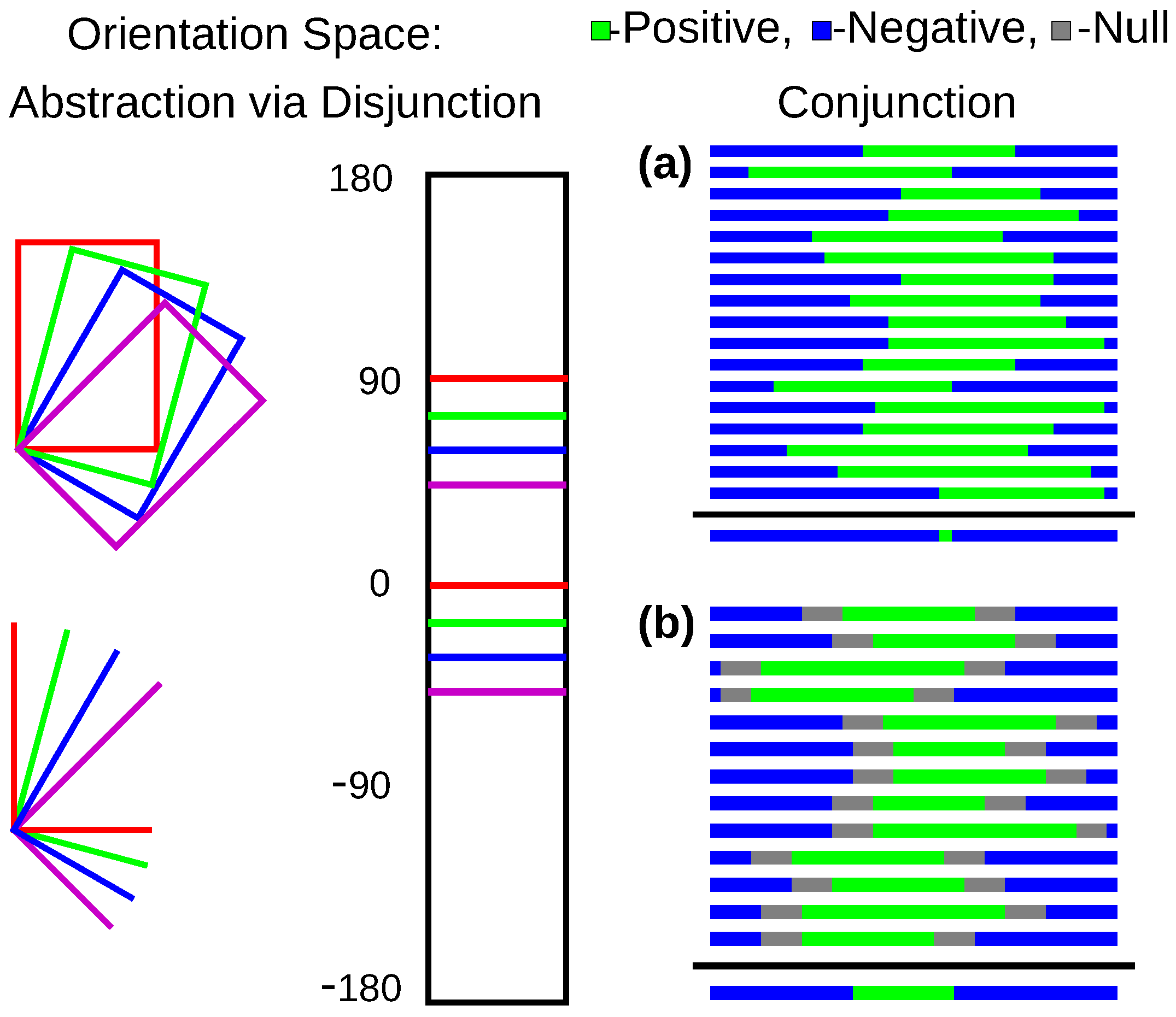
- Comparing the results of conjunction and disjunction, as shown in Figure 10a,b, with that of Equation (1), we note that the specificity achieved in either case is high. While the former is based on first principles of processing that occur at each interaction, the later requires an interpretation based on a model of probabilities.
- The results of the simulation suggest that for a re-entrant system of processing, when the new set of active states are fed back in a controlled loop within the same level of processing as well as to the lower level, the evolving profile of positive correlation could get more and more specific closer to the maximum resolution of reality presented. For this reason, it did not matter much if the range of null correlation was wide to begin with, since, initially, the positive range of correlation would grow wider, but the null correlation would decay out quickly, and then, the positive correlation would also begin to gain specificity.
- Considering how the conjunction of correlation profiles limits the range of positive correlation sharply around the specifics of reality present in the observed system, it is an advantage to a processing system to capture or sample as much of a diversity in the correlation profiles as possible with the agents [24,48,49] within the sampling domains. Even the feedback profiles could initially bear greater diversity. This may appear as noise to an external system that makes measurements on the signals and correlation profiles of the agents [50,51].
8. Discussion, Interpretation, Implication, and Conclusions
8.1. Realism of Natural Representation
8.2. Function of Natural Representation
8.3. Role of Natural Representation in the Universe
8.4. Symbolism as a Powerful Tool of Language and Mathematics
8.5. Limitations of Processing in the Physical Domain
8.6. State vs. Specificity of Representation
8.7. Sparseness of Objects With Greater Specificity
8.8. A New Perspective of Physical Universe
8.9. Reality vs. Measurability
8.10. Method of Information Processing
Funding
Acknowledgments
Conflicts of Interest
References
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423, 623–656. [Google Scholar] [CrossRef]
- Hintikka, J. On Semantic Information. In Physics, Logic, and History; Yourgrau, W., Breck, A.D., Eds.; Springer: Boston, MA, USA, 1970; pp. 147–172. [Google Scholar]
- Dietrich, E. Semantics and the computational paradigm in cognitive psychology. Synthese 1989, 79, 119–141. [Google Scholar] [CrossRef]
- Ralph, M.A.L.; Jefferies, E.; Patterson, K.; Rogers, T.T. The neural and computational bases of semantic cognition. Nat. Rev. Neurosci. 2017, 18, 42–55. [Google Scholar] [CrossRef] [PubMed]
- Binder, J.R.; Desai, R.H. The neurobiology of semantic memory. Trends Cogn. Sci. 2011, 15, 527–536. [Google Scholar] [CrossRef] [PubMed]
- Kiefer, M.; Pulvermüller, F. Conceptual representations in mind and brain: Theoretical developments, current evidence and future directions. Cortex 2012, 48, 805–825. [Google Scholar] [CrossRef] [PubMed]
- McClelland, J.L.; Rogers, T.T. The parallel distributed processing approach to semantic cognition. Nat. Rev. Neurosci. 2003, 4, 310–322. [Google Scholar] [CrossRef] [PubMed]
- Landauer, R. Information is a physical entity. Physics A 1999, 263, 63–67. [Google Scholar] [CrossRef]
- Vedral, V. Information and physics. Information 2012, 3, 219–223. [Google Scholar] [CrossRef]
- Karnani, M.; Pääkkönen, K.; Annila, A. The physical character of information. Proc. R. Soc. A 2009, 465, 2155–2175. [Google Scholar] [CrossRef]
- Davies, P. Information and the nature of reality: From physics to metaphysics. In Universe from Bit; Davies, P., Gregersen, N.H., Eds.; Cambridge University Press: Cambridge, UK, 2010; pp. 65–91. [Google Scholar]
- Dretske, F.I. Naturaliozing the Mind; MIT Press: Cambridge, MA, USA, 1997. [Google Scholar]
- Barwise, J.; Seligman, J. Information Flow: The Logic of Distributed Systems; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Roederer, J.G. On the Concept of Information and Its Role in Nature. Entropy 2003, 5, 3–33. [Google Scholar] [CrossRef]
- Bais, F.A.; Farmer, J.D. The Physics of Information. arXiv, 2007; arXiv:0708.2837v2. [Google Scholar]
- Goyal, P. Information Physics-Towards a New Conception of Physical Reality. Information 2012, 3, 567–594. [Google Scholar] [CrossRef]
- Knoll, G.F. Radiation Detection and Measurement, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2000. [Google Scholar]
- Gell-Mann, M.; Hartle, J.B. Decoherent histories quantum mechanics with one real fine-grained history. Phys. Rev. A 2012, 85, 062120. [Google Scholar] [CrossRef]
- Fields, C. If Physics Is an Information Science, What Is an Observer? Information 2012, 3, 92–123. [Google Scholar] [CrossRef]
- Einstein, A.; Podolsky, B.; Rosen, N. Can Quantum-Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 1935, 47, 777–780. [Google Scholar] [CrossRef]
- Hirsch, J.A. Synaptic Physiology and Receptive Field Structure in the Early Visual Pathway of the Cat. Cereb. Cortex 2003, 13, 63–69. [Google Scholar] [CrossRef] [PubMed]
- Lee, B.B. Receptive field structure in the primate retina. Vis. Res. 1996, 36, 631–644. [Google Scholar] [CrossRef]
- Khrennikov, A. Quantum-like model of processing of information in the brain based on classical electromagnetic field. Biosystems 2011, 105, 250–262. [Google Scholar] [CrossRef] [PubMed]
- Basole, A.; White, L.E.; Fitzpatrick, D. Mapping multiple features in the population response of visual cortex. Nature 2003, 423, 986–990. [Google Scholar] [CrossRef] [PubMed]
- Albright, T.D. Direction and orientation selectivity of neurons in visual area MT of the macaque. J. Neurophys. 1984, 52, 1106–1130. [Google Scholar] [CrossRef] [PubMed]
- Treisman, A. The binding problem. Curr. Opin. Neurobiol. 1996, 6, 171–178. [Google Scholar] [CrossRef]
- Roskies, A.L. The Binding Problem. Neuron 1999, 24, 7–9. [Google Scholar] [CrossRef]
- Singer, W. Consciousness and the Binding Problem. Ann. N. Y. Acad. Sci. 2001, 929, 123–146. [Google Scholar] [CrossRef] [PubMed]
- McNorgan, C.; Reid, J.; McRae, K. Integrating conceptual knowledge within and across representational modalities. Cognition 2011, 118, 211–233. [Google Scholar] [CrossRef] [PubMed]
- Yuval-Greenberg, S.; Deouell, L.Y. What You See Is Not (Always) What You Hear: Induced Gamma Band Responses Reflect Cross-Modal Interactions in Familiar Object Recognition. J. Neurosci. 2007, 27, 1090–1096. [Google Scholar] [CrossRef] [PubMed]
- Harris, K.D.; Shepherd, G.M.G. The neocortical circuit: Themes and variations. Nat. Neurosci. 2015, 18, 170–181. [Google Scholar] [CrossRef] [PubMed]
- Harris, K.D. Cortical computation in mammals and birds. Proc. Natl. Acad. Sci. USA 2015, 112, 3184–3185. [Google Scholar] [CrossRef] [PubMed]
- Calabrese, A.; Woolley, S.M.N. Coding principles of the canonical cortical microcircuit in the avian brain. Proc. Natl. Acad. Sci. USA 2015, 112, 3517–3522. [Google Scholar] [CrossRef] [PubMed]
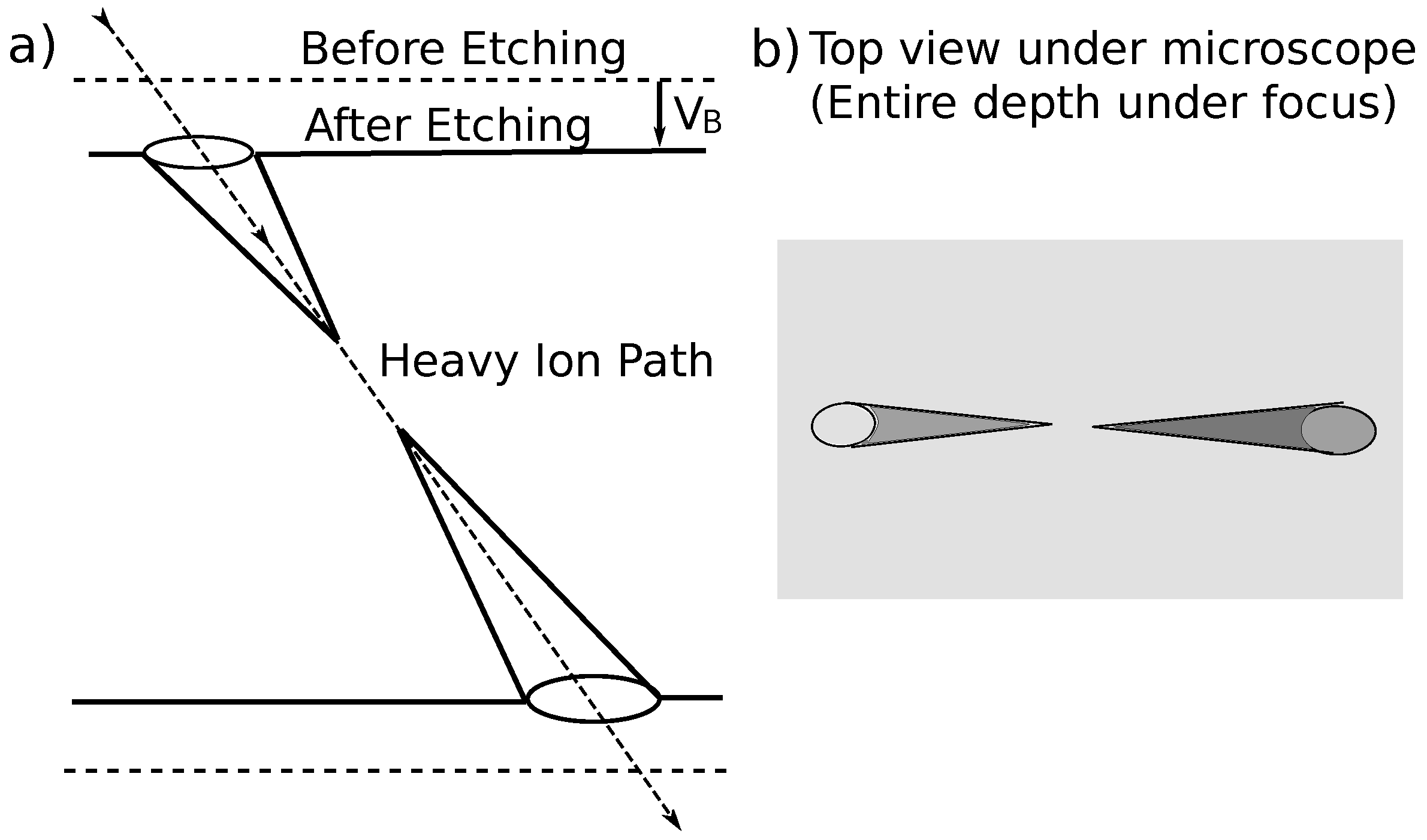
- Durrani, S.A.; Bull, R.K. Solid State Nuclear Track Detection: Principles, Methods, and Applications; International Series of Monographs in Natural Philosophy; Pergramon Press: Oxford, UK, 1987. [Google Scholar]
- Fleischer, R.L.; Price, P.B.; Walker, R.M. Nuclear Tracks in Solids: Principles and Applications; University of California Press: Berkeley, CA, USA, 1975. [Google Scholar]
- Yadav, J.S.; Singh, R.K. Error analysis for particle identification in CR-39 track detectors. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms. 1990, 51, 69–75. [Google Scholar] [CrossRef]
- Henke, R.P.; Benton, E.V. On geometry of tracks in dielectric nuclear track detectors. Nucl. Instrum. Methods 1971, 97, 483–489. [Google Scholar] [CrossRef]
- Yadav, J.S.; Singh, R.K. Space Lab-3 Anuradha detector response and the expected charge resolution. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms. 1990, 51, 63–68. [Google Scholar] [CrossRef]
- Benton, E.V. On latent track formation in organic nuclear charged particle track detectors. Radiat. Eff. 1970, 2, 273–280. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics, 6th ed.; Pergoman Press: Oxford, UK, 1980; Chapter X. [Google Scholar]
- Thompson, A.; Moran, J.; Swenson, G., Jr. Interferometry and Synthesis in Radio Astronomy, 2nd ed.; Wiley-VCH: Weinheim, Germany, 2001. [Google Scholar]
- Bhatnagar, S.; Cornwell, T.; Uson, J. Correcting direction-dependent gains in the deconvolution of radio interferometric images. Astron. Astrophys. 2008, 487, 419–429. [Google Scholar] [CrossRef]
- Intema, H.; Van der Tol, S.; Cotton, W. Ionospheric calibration of low frequency radio interferometric observations using the peeling scheme-I.Method description and first results. Astron. Astrophys. 2009, 501, 1185–1205. [Google Scholar] [CrossRef]
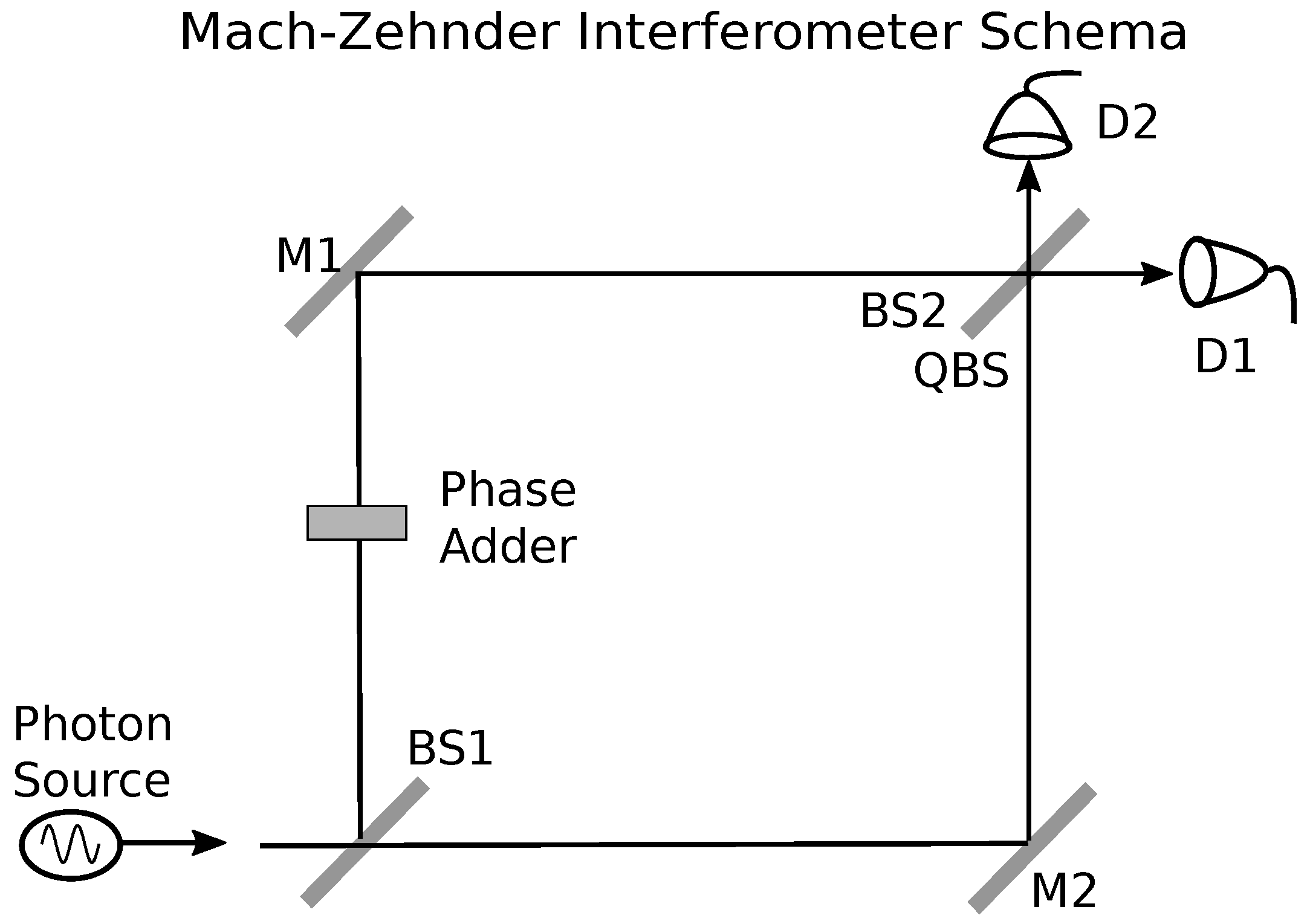
- Jacques, V.; Wu, E.; Grosshans, F.; Treussart, F.; Grangier, P.; Aspect, A.; Roch, J.F. Experimental Realization of Wheeler’s Delayed-Choice Gedanken Experiment. Science 2007, 315, 966–968. [Google Scholar] [CrossRef] [PubMed]
- Kaiser, F.; Coudreau, T.; Milman, P.; Ostrowsky, D.B.; Tanzilli, S. Entanglement-Enabled Delayed-Choice Experiment. Science 2012, 338, 637–640. [Google Scholar] [CrossRef] [PubMed]
- Peruzzo, A.; Shadbolt, P.; Brunner, N.; Popescu, S.; O’Brien, J.L. A Quantum Delayed-Choice Experiment. Science 2012, 338, 634–637. [Google Scholar] [CrossRef] [PubMed]
- Marquis de Laplace, P.S. A Philosophical Essay on Probablities; John Wiley and Sons: Hoboken, NJ, USA, 1902. [Google Scholar]
- Binzegger, T.; Douglas, R.J.; Martin, K.A.C. Stereotypical Bouton Clustering of Individual Neurons in Cat Primary Visual Cortex. J. Neurosci. 2007, 27, 12242–12254. [Google Scholar] [CrossRef] [PubMed]
- Potjans, T.C.; Diesmann, M. The Cell-Type Specific Cortical Microcircuit: Relating Structure and Activity in a Full-Scale Spiking Network Model. Cereb. Cortex 2014, 24, 785–806. [Google Scholar] [CrossRef] [PubMed]
- Tabareau, N.; Slotine, J.J.; Pham, Q.C. How Synchronization Protects from Noise. PLoS Comput. Biol. 2010, 6, e1000637. [Google Scholar] [CrossRef] [PubMed]
- Stein, R.B.; Gossen, E.R.; Jones, K.E. Neuronal variability: Noise or part of the signal? Nat. Rev. Neurosci. 2005, 6, 389–397. [Google Scholar] [CrossRef] [PubMed]
- Wheeler, J.A. Information, Physics, Quantum: The Search for Links. In Proceedings of the 3rd International Symposium on Foundations of Quantum Mechanics, Tokyo, Japan, 28–31 August 1989; pp. 354–358. [Google Scholar]
- Tegmark, M. The Mathematical Universe. Found. Phys. 2008, 38, 101–150. [Google Scholar] [CrossRef]
- Kako, E.; Wagner, L. The semantics of syntactic structures. Trends Cogn. Sci. 2001, 5, 102–108. [Google Scholar] [CrossRef]
- Hutten, E.H. On Semantics and Physics. Proc. Aristot. Soc. 1948, 49, 115–132. [Google Scholar] [CrossRef]
- Penrose, R. Shadows of the Mind: A Search for the Missing Science of Consciousness; Oxford University Press: Oxford, UK, 1994. [Google Scholar]
- Hut, P.; Alford, M.; Tegmark, M. On Math, Matter and Mind. Found. Phys. 2006, 36, 765–794. [Google Scholar] [CrossRef]
- Hameroff, S.; Penrose, R. Consciousness in the universe: A review of the ‘Orch OR’ theory. Phys. Life Rev. 2014, 11, 39–78. [Google Scholar] [CrossRef] [PubMed]


| Corr1 | Corr2 | Conjunction | Disjunction |
|---|---|---|---|
| 01 (Pos) | 01 (Pos) | 01 (Pos) | 01 (Pos) |
| 01 (Pos) | 00 (Neg) | 00 (Neg) | 01 (Pos) |
| 01 (Pos) | 11 (Nul) | 01 (Pos) | 11 (Nul) |
| 00 (Neg) | 00 (Neg) | 00 (Neg) | 00 (Neg) |
| 00 (Neg) | 11 (Nul) | 00 (Neg) | 11 (Nul) |
| 11 (Nul) | 11 (Nul) | 11 (Nul) | 11 (Nul) |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Singh, R.K. Fundamentals of Natural Representation. Information 2018, 9, 168. https://doi.org/10.3390/info9070168
Singh RK. Fundamentals of Natural Representation. Information. 2018; 9(7):168. https://doi.org/10.3390/info9070168
Chicago/Turabian StyleSingh, Rajiv K. 2018. "Fundamentals of Natural Representation" Information 9, no. 7: 168. https://doi.org/10.3390/info9070168
APA StyleSingh, R. K. (2018). Fundamentals of Natural Representation. Information, 9(7), 168. https://doi.org/10.3390/info9070168





