Lateral Cross Localization Algorithm Using Orientation Angle for Improved Target Estimation in Near-Field Environments
Abstract
:1. Introduction
2. Problem Formulation
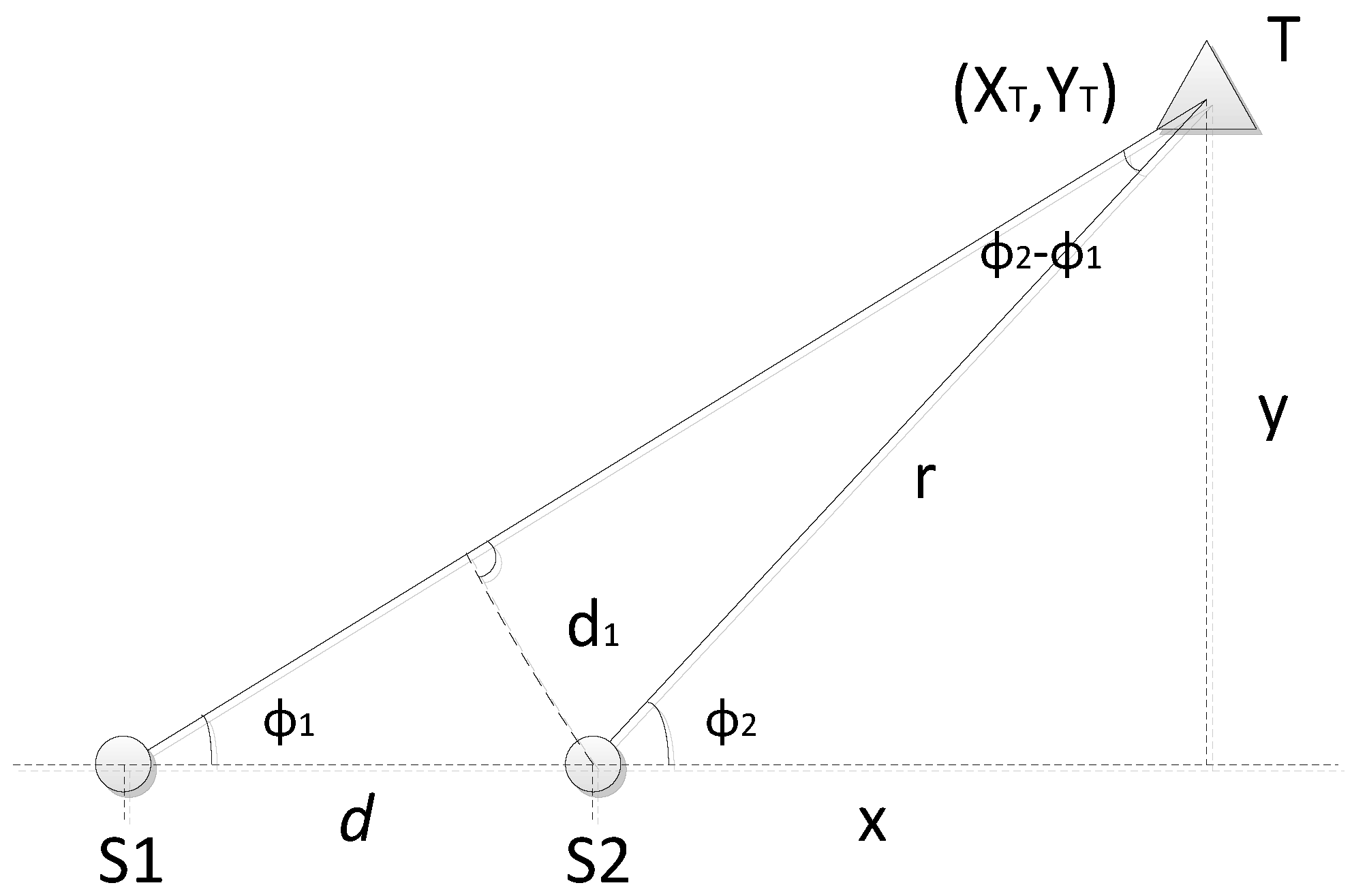
3. Lateral Cross Positioning System Model
3.1. Description of Lateral Cross Positioning Method
3.2. Targeting Model
4. Proposed Lateral Cross Localization Algorithm Using Orientation Angle
4.1. Description of the Proposed Algorithm
4.2. Iterative Process Control
4.3. Analysis of Estimation Error
5. Performance Analysis
5.1. Algorithm Effectiveness
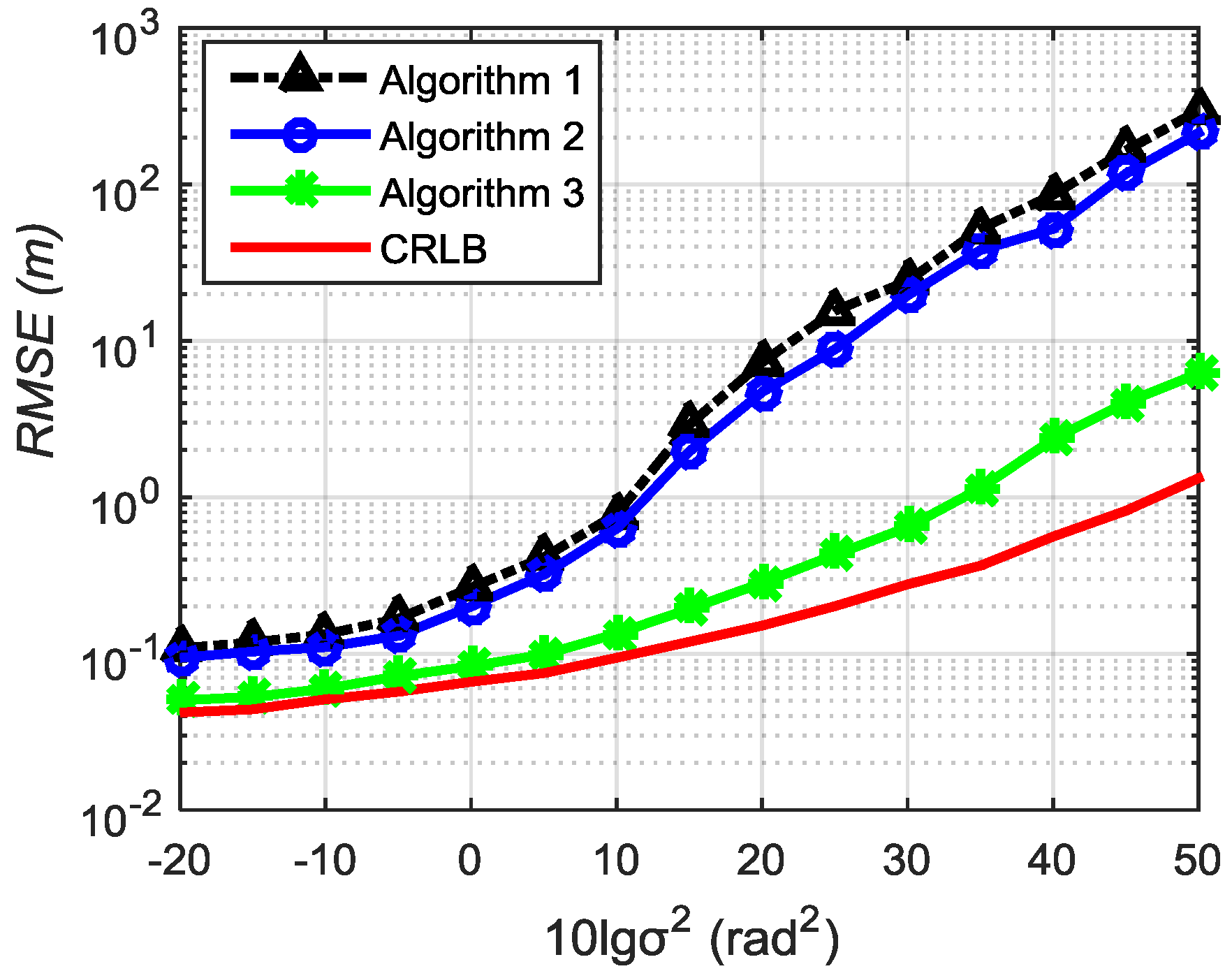
5.2. Algorithm Performance in Different Orientation Angle Measurement Error
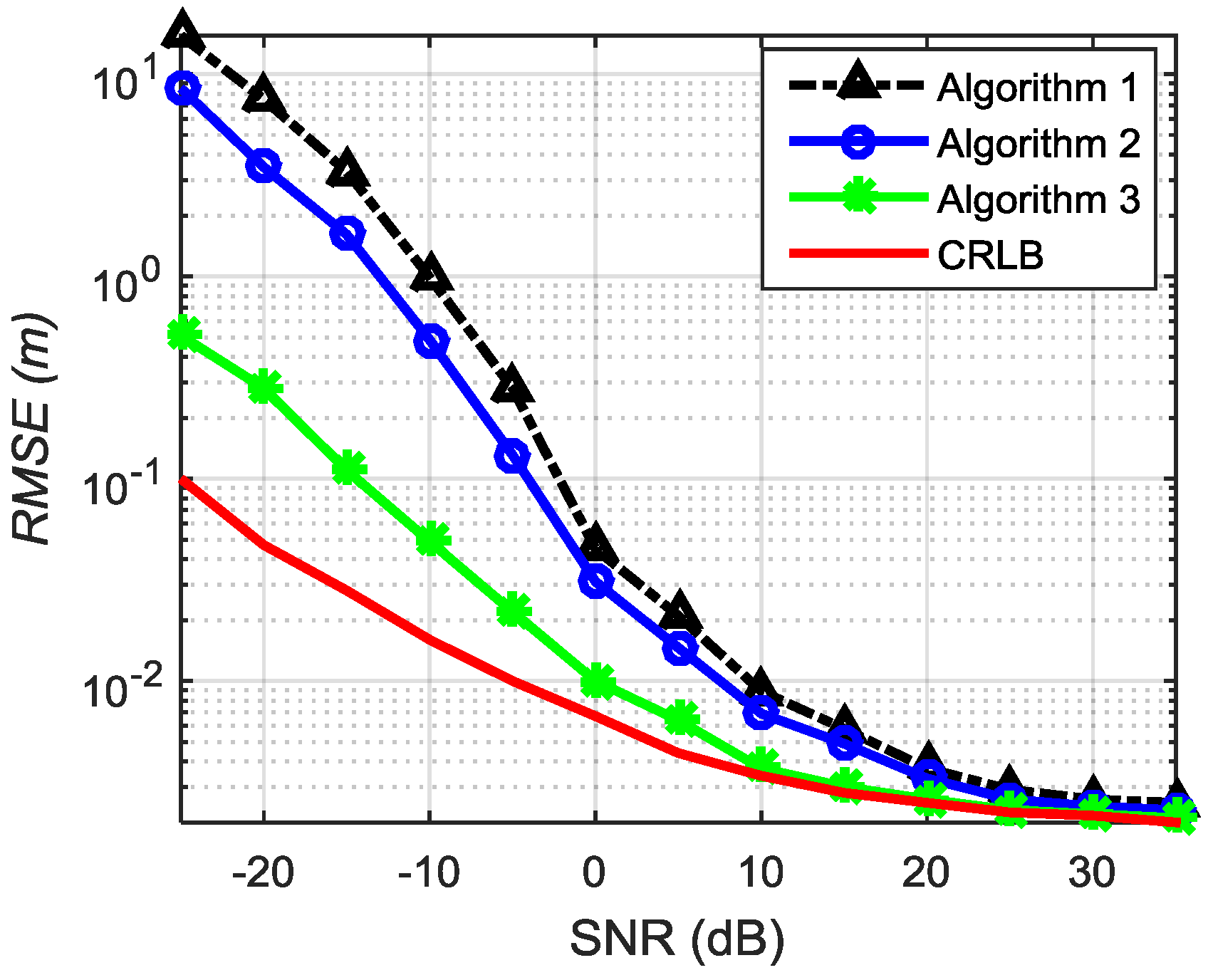
5.3. Performance for Varying SNR
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Zhang, L.; Gan, J.Q.; Wang, H. Localization of neural efficiency of the mathematically gifted brain through a feature subset selection method. Cogn. Neurodyn. 2015, 9, 495–508. [Google Scholar] [CrossRef] [PubMed]
- Otero-Garcia, M.; Agustin-Pavon, C.; Lanuza, E.; Martínez-García, E. Distribution of oxytocin and co-localization with arginine vasopressin in the brain of mice. Brain Struct. Funct. 2015, 20. [Google Scholar] [CrossRef] [PubMed]
- Bulychev, Y.G.; Bulychev, V.Y.; Ivakina, S.S.; Nasenkov, I.G. Complex Matching–Identification Method as Applied to Multiposition Angle-Measuring Systems of Passive Location Based on Invariants. J. Commun. Technol. Electron. 2015, 60, 762–772. [Google Scholar] [CrossRef]
- Perrine-Walker, F.M.; Jublanc, E. The localization of auxin transporters PIN3 and LAX3 during lateral root development in Arabidopsis thaliana. Biol. Plant. 2014, 58, 778–782. [Google Scholar] [CrossRef]
- Lobkova, L.M.; Golovin, V.V.; Troitsky, A.V. The Impact of Angle-of-Arrival Fluctuations of Electromagnetic Wave on the Direction of Maximum Radiation of Aperture Antennas. Radioelectron. Commun. Syst. 2008, 51, 150–155. [Google Scholar] [CrossRef]
- Fu, Y.; Nistor, A.I. Constant Angle Property and Canonical Principal Directions for Surfaces in M2(c) × R1. Mediterr. J. Math. 2013, 10, 1035–1049. [Google Scholar] [CrossRef]
- Zsedrovits, T.; Zarandy, A.; Vanek, B.; Peni, T.; Bokor, J.; Roska, T. Estimation of Relative Direction Angle of Distant, Approaching Airplane in Sense-and-Avoid. J. Intell. Robot Syst. 2013, 69, 407–415. [Google Scholar] [CrossRef]
- Guillian, E.H. Far Field Monitoring of Rogue Nuclear Activity with an Array of Large Anti-neutrino Detectors. Earth Moon Planets 2006, 99, 309–330. [Google Scholar] [CrossRef]
- Bulychev, Y.G.; Vernygora, V.N.; Mozol, A.A. The Direction-Finding Powered Method for Definition of Distance to Target, Using Two Measurements of Autonomous Angle-Measurement System. Radioelectron. Commun. Syst. 2009, 52, 606–612. [Google Scholar] [CrossRef]
- Luo, Q.; Meng, Z.; Han, C. Solution algorithm of a quasi-Lambert’s problem with fixed flight-direction angle constraint. Celest. Mech. Dyn. Astr. 2011, 109, 409–427. [Google Scholar] [CrossRef]
- Weiss, A.J. Direct position determination of narrowband radio frequency transmitters. IEEE Signal Process. Lett. 2004, 11, 513–516. [Google Scholar] [CrossRef]
- Chen, J.C.; Hudson, R.E.; Yao, K. Maximum-likelihood source localization and unknown sensor location estimation for wideband signals in the near-field. IEEE Trans. Signal Process. 2002, 50, 1843–1854. [Google Scholar] [CrossRef]
- Weiss, A.J.; Amar, A. Direct position determination of multiple radio signals. EURASIP J. Adv. Signal Process. 2005. [Google Scholar] [CrossRef]
- Bakhti, S.; Destouches, N.; Tishchenko, A.V. Singular Representation of Plasmon Resonance Modes to Optimize the Near and Far Field Properties of Metal Nanoparticles. Plasmonics 2015, 10, 1391–1399. [Google Scholar] [CrossRef]
- Dunn, R.J.K.; Zigic, S.; Shiell, G.R. Modelling the dispersion of treated wastewater in a shallow coastal wind-driven environment, Geographe Bay, Western Australia: implications for environmental management. Environ. Monit. Assess. 2014, 186, 6107–6125. [Google Scholar] [CrossRef] [PubMed]
- Yan, K.; Liu, L.; Yao, N.; Liu, K.; Du, W.; Zhang, W.; Yan, W.; Wang, C.; Luo, X. Far-Field Super-Resolution Imaging of Nano-transparent Objects by Hyperlens with Plasmonic Resonant Cavity. Plasmonics 2016, 11, 475–481. [Google Scholar] [CrossRef]
- Tounsi, R.; Markiewicz, E.; Haugou, G.; Chaari, F.; Zouari, B. Combined effects of the in-plane orientation angle and the loading angle on the dynamic enhancement of honeycombs under mixed shear-compression loading. Eur. Phys. J. Spec. Top. 2016, 225, 243–252. [Google Scholar] [CrossRef]
- Girod, L.; Lukac, M.; Trifa, V.; Estrin, D. The design and implementation of a self-calibrating distributed acoustic sensing platform. In Proceedings of the 4th International Conference on Embedded Networked Sensor Systems, Boulder, CO, USA, 1–3 November 2006; pp. 71–84.
- Yip, L.; Comanor, K.; Chen, J.; Hudson, R.E.; Yao, K.; Vandenberghe, L. Array processing for target DOA, localization, and classification based on AML and SVM algorithms in sensor networks. In Information Processing in Sensor Networks, Proceedings of the Second International Workshop IPSN 2003, Palo Alto, CA, USA, 22–23 April 2003; Springer-Verlag: Berlin/Heidelberg, Germany, 2003; pp. 269–284. [Google Scholar]
- Yao, K.; Chen, J.C.; Hudson, R.E. Maximum-likelihood acoustic source localization: experimental results. In Proceedings of the IEEE International Conference on Acoustics, Speech, and Signal Processing (ICASSP), Orlando, FL, USA, 13–17 May 2002; Volume 3, pp. 2949–2952.
- Allen, M.; Girod, L.; Newton, R.; Madden, S.; Blumstein, D.T.; Estrin, D. Voxnet: An interactive, rapidly-deployable acoustic monitoring platform. In Proceedings of International Conference on Information Processing in Sensor Networks (IPSN), St. Louis, MO, USA, 22–24 April 2008; pp. 371–382.
- Kaplan, L.M.; Le, Q.; Molnar, N. Maximum likelihood methods for bearings-only target localization. In Proceedings of the IEEE International Conference on Acoustics, Speech, and Signal Processing (ICASSP), Salt Lake City, UT, USA, 7–11 May 2001; Volume 5, pp. 3001–3004.
- Kaplan, L.M.; Le, Q. On exploiting propagation delays for passive target localization using bearings-only measurements. J. Frankl. Inst. 2005, 342, 193–211. [Google Scholar] [CrossRef]
- Stansfield, R.G. Statistical theory of DF fixing. Electr. Eng.-Part IIIA J. Inst. Radio Commun. 1947, 94, 762–770. [Google Scholar]
- Dogancay, K. Bearings-only target localization using total least squares. Signal Process. 2005, 85, 1695–1710. [Google Scholar] [CrossRef]
- Chan, Y.T.; Rudnicki, S.W. Bearings-only and Doppler-bearing tracking using instrumental variables. IEEE Trans. Aerosp. Electr. Syst. 1992, 28, 1076–1083. [Google Scholar] [CrossRef]
- Dogancay, K. Passive emitter localization using weighted instrumental variables. Signal Process. 2004, 84, 487–97. [Google Scholar] [CrossRef]
- Zhang, Y.J.; Xu, G.Z. Bearings-only target motion analysis via instrumental variable estimation. IEEE Trans. Signal Process. 2010, 58, 5523–5533. [Google Scholar] [CrossRef]
- Ho, K.C.; Chan, Y.T. An asymptotically unbiased estimator for bearings-only and Doppler-bearing target motion analysis. IEEE Trans. Signal Process. 2006, 54, 809–822. [Google Scholar] [CrossRef]
- Gu, G. A novel power-bearing approach and asymptotically optimum estimator for target motion analysis. IEEE Trans. Signal Process. 2011, 59, 912–922. [Google Scholar] [CrossRef]
- Vaghefi, R.M.; Gholami, M.R.; Strom, E.G. Bearing-only target localization with uncertainties in observer position. In Proceedings of International Symposium on Personal, Indoor and Mobile Radio Communications Workshops, Instanbul, Turkey, 26–30 September 2010; pp. 238–242.
- Le Cadre, J.P.; Jaetffret, C. On the convergence of iterative methods for bearings-only tracking. IEEE Trans. Aerosp. Electron. Syst. 1999, 35, 801–818. [Google Scholar] [CrossRef]
- Gavish, M.; Weiss, A.J. Performance analysis of bearing-only target location algorithms. IEEE Trans. Aerosp. Electron. Syst. 1992, 28, 817–828. [Google Scholar] [CrossRef]
- Bishop, A.N.; Anderson, B.D.O.; Fidan, B.; Pathirana, P.N.; Mao, G. Bearing-only localization using geometrically constrained optimization. IEEE Trans. Aerosp. Electron. Syst. 2009, 45, 308–320. [Google Scholar] [CrossRef]
- Serov, A.V.; Mamonov, I.A.; Kol’tsov, A.V. Angular Distributions of Reflected and Refracted Relativistic Electron Beams Crossing a Thin Planar Target at a Small Angle to Its Surface. J. Exp. Theor. Phys. 2015, 121, 572–577. [Google Scholar] [CrossRef]
- Wolbrecht, E.; Gill, B.; Borth, R.; Canning, J.; Anderson, M.; Edwards, D. Hybrid Baseline Localization for Autonomous Underwater Vehicles. J. Intell. Robot. Syst. 2015, 78, 593–611. [Google Scholar] [CrossRef]


| Algorithm | Absolute Deviation of Estimated Position (m) | ||
|---|---|---|---|
| x | y | z | |
| Algorithm 1 | 1.9 | 2.1 | 0.8 |
| Algorithm 2 | 1.4 | 1.5 | 0.7 |
| Algorithm 3 | 0.6 | 0.8 | 0.2 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, P.; Yan, B. Lateral Cross Localization Algorithm Using Orientation Angle for Improved Target Estimation in Near-Field Environments. Information 2016, 7, 40. https://doi.org/10.3390/info7030040
Xu P, Yan B. Lateral Cross Localization Algorithm Using Orientation Angle for Improved Target Estimation in Near-Field Environments. Information. 2016; 7(3):40. https://doi.org/10.3390/info7030040
Chicago/Turabian StyleXu, Penghao, and Bing Yan. 2016. "Lateral Cross Localization Algorithm Using Orientation Angle for Improved Target Estimation in Near-Field Environments" Information 7, no. 3: 40. https://doi.org/10.3390/info7030040
APA StyleXu, P., & Yan, B. (2016). Lateral Cross Localization Algorithm Using Orientation Angle for Improved Target Estimation in Near-Field Environments. Information, 7(3), 40. https://doi.org/10.3390/info7030040





