Philosophy and Meanings of the Information Entropy Analysis of Road Safety: Case Study of Russian Cities
Abstract
1. Introduction
1.1. General Provisions
- Minimizing of the number of road traffic accidents (RTA) and their consequences;
- Minimizing of the number of RTA victims;
- Minimizing of the number of RTA deaths;
- Minimizing of the socio-economic damage caused by road traffic accidents rate.
1.2. An Overview of the Field Significance, Purpose and Objectives of the Study, Important Contributions
1.3. Outline of the Article
1.4. Study’s Limitations
2. Analysis of Previous Works
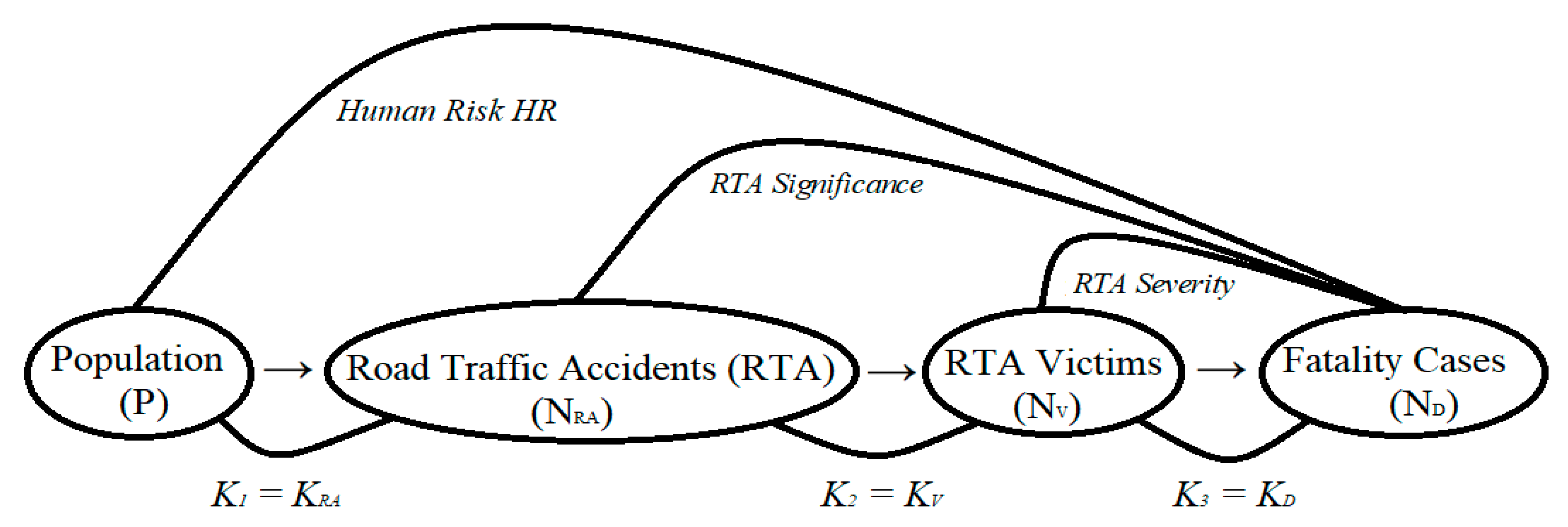
3. Method of Quantitative Assessment of the Relative Entropy Hn RS, the Most Important Indicator of the Orderliness of Urban Road Safety Systems
3.1. Main Idea of the Method
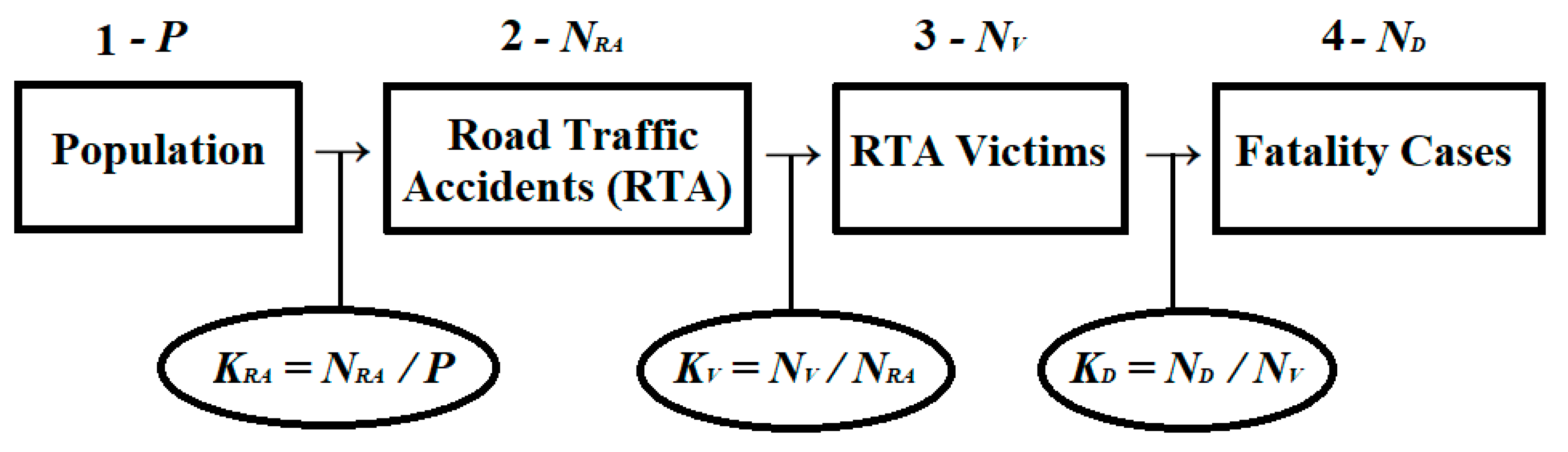
3.2. Method of Quantitative Assessment of the Relative Entropy Hn RS of Road Safety Systems
- n—the number of links in the causal chain of the road traffic accident rate formation;
- —positive weight coefficients of chain links that meet the normalization condition .
- n—the number of links in the causal chain of the road traffic accident rate formation;
- n = 3 for the case of estimating the entropy of urban road safety management systems.
4. Data
- The compared groups of cities are not neighbors within the classification (there is a 3–10-fold difference in population).
- The style, organization and quality of life of citizens in such cities are radically different.
- The structural complexity of the management systems of the most important subsystems of the urban economy in such cities also varies significantly.
5. Results of the Study of Information Entropy Specifics of the Road Traffic Accident Rate in Russia’s Cities
5.1. Quantification of the Relative Entropy Hn-3 RS of Urban Road Safety Systems
5.2. Specifics of the Processes of the Road Traffic Accident Rate Formation in Urban Road Safety Systems with Different Levels of the Relative Entropy Hn-3 RS
5.3. Structural Interpretation of the Assessment Results of the Relative Entropy Hn-3 RS
6. Specific Comparative Example of the Semantic Interpretation of Various Results of Assessing the Relative Entropy Hn-3 RS
6.1. Specifics of Traffic Management on the Roads of Cities with High (Tyumen) and Low (Novosibirsk) Levels of the Relative Entropy Hn-3 RS
- According to Russian Traffic rules [63], the permitted speed in cities is 60 km/h; the non-penalized threshold for exceeding this limit is 20 km/h. The actual speed of most vehicles in Russian cities under favorable conditions (summer, dry road surface, no traffic jams) is 75–80 km/h. On city roads of the first and second categories (for example, on the belt road around Tyumen), traffic is allowed at a speed of 90 km/h; the actual speed reaches 105–110 km/h. This is a fairly high speed for vehicles, which does not fully ensure road safety.
- In Tyumen, 96% of all traffic light units in the city are characterized by the obligatory dedicated pedestrian phase in the traffic light cycle. In Novosibirsk, no more than 25% of the city’s traffic lights have a dedicated pedestrian phase. This clear visual difference between the traffic management systems in the two compared cities is the main impression for the observer.
- A distinctive feature of traffic management in Tyumen is not only a dedicated pedestrian phase in the traffic light cycle but also more complex multi-phase cycles of traffic light regulation in general. The purpose of such a management decision is to differentiate vehicle and pedestrian flows in time and to minimize the number of conflict points at intersections. In Novosibirsk, traffic managers pay much less attention to the differentiation of traffic flows over time.
6.2. Specifics of the Behavior of Road Users on the Roads of Cities with High (Tyumen) and Low (Novosibirsk) Levels of the Relative Entropy Hn-3 RS
- Tyumen drivers and pedestrians are generally characterized by relatively greater carelessness and a lower level of concentration. This is facilitated by the common and expected absence of conflict at the intersections of the city’s road network [63]. Car and pedestrian flows are separated in time by using multi-phase traffic control schemes. In Novosibirsk, the situation is reversed: drivers and pedestrians are alert constantly. The reason for this is the presence of conflict points at intersections [64,65,66,67,68,69]. As a result, there is less likelihood of dangerous traffic situations due to the greater attention of road users to the general situation.
- At the same time, we can state the fact of a greater intensity of drivers’ operator actions in Tyumen and traffic on the stretches of the city’s road network at relatively higher speeds than in Novosibirsk. Analyzing this observation, the author came to the conclusion that Tyumen drivers try to use the high dynamics (intense acceleration, high speed) as a compensation for the loss of time during numerous and rather long downtimes while waiting for a permitting traffic signal at regulated intersections [70,71]. In Novosibirsk, these actions of drivers are expressed to a lesser extent than in Tyumen. The reason is the lesser loss of time for Novosibirsk traffic participants while waiting for the permitting traffic signal.
- The specifics of urban demographics, in particular the median age of the population, have a certain influence on the behavior of road users. In Novosibirsk, the median age of the population is 38.9 years; in Tyumen—35.8 years [72]. The level of acceptable risk homeostasis [73,74] in Tyumen is higher than in Novosibirsk. The reason is the peculiarities of age demographics.
- An attempt to complicate the traffic management system in order to increase road safety reduces the severity of accidents, but increases the probability of their occurrence (more on this in Section 6.1);
- The first conclusion leads to the second conclusion about changing the structure of the accident rate, but not eliminating or reducing it when trying to complicate the road safety management system.
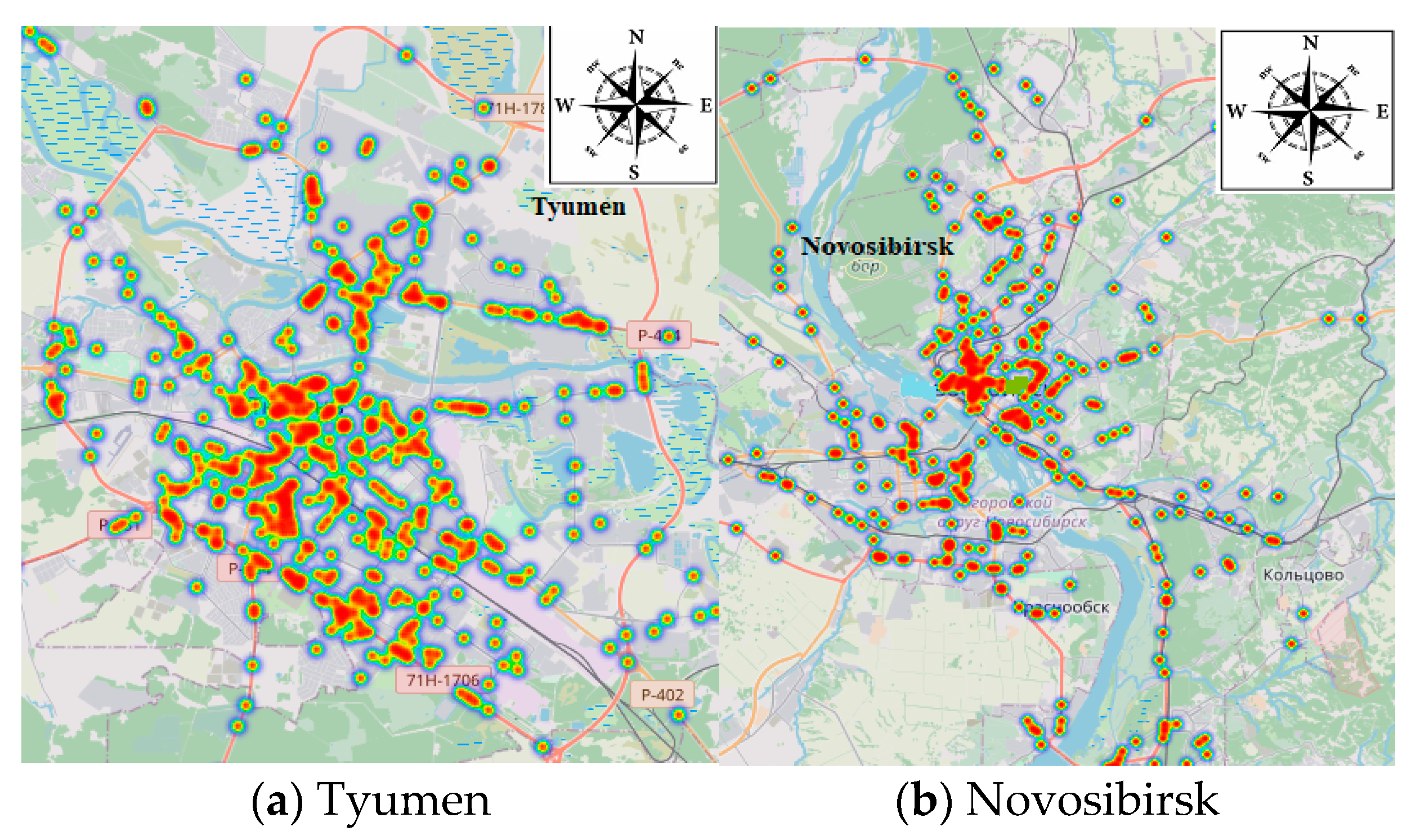
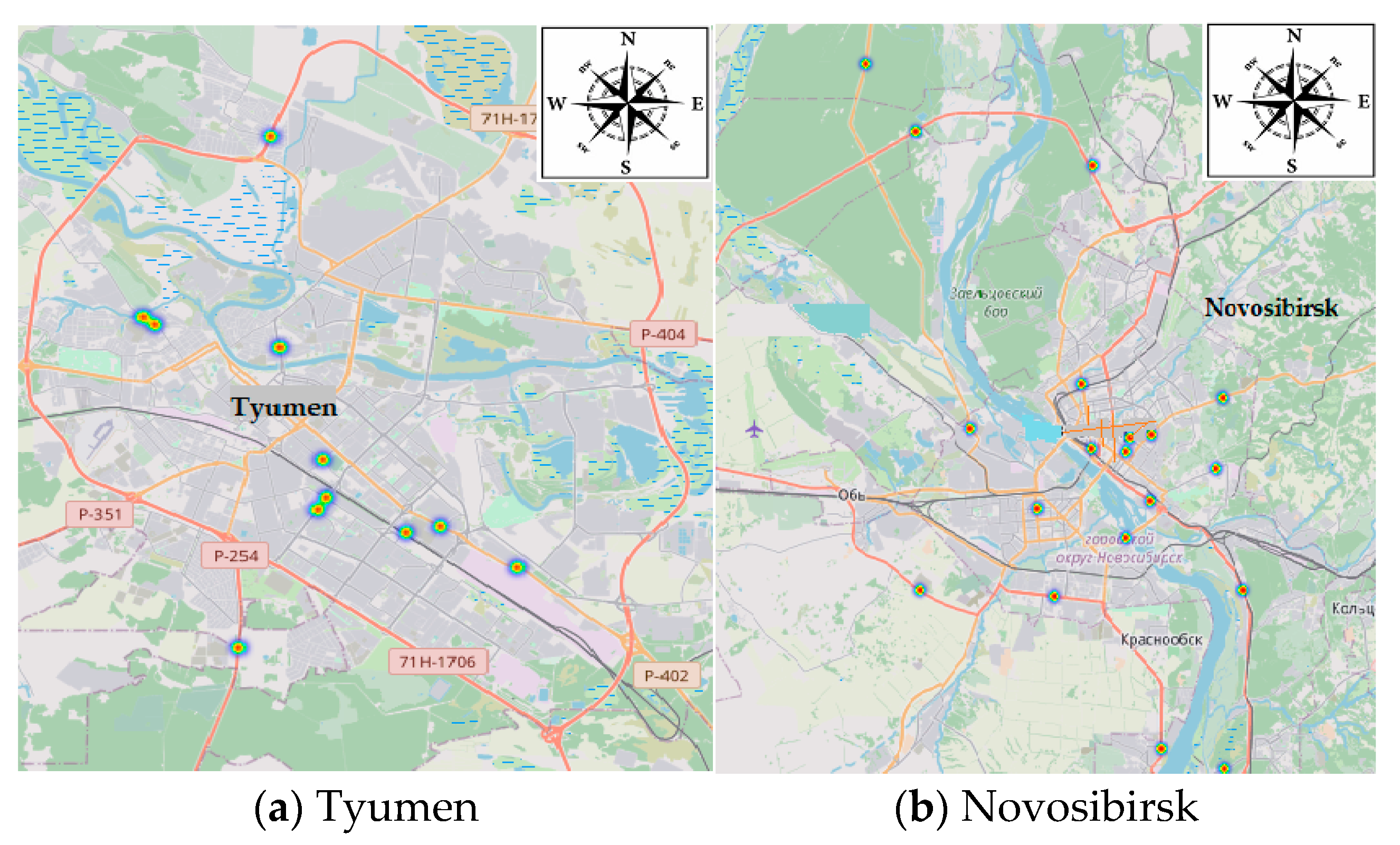
6.3. Specifics of the Localization of Places and the Probability of an Accident on the Roads of Cities with a High and Low Level of the Relative Entropy Hn-3 RS
6.4. Specifics of the Rolling Stock Used by the Population of Cities with High (Tyumen) and Low (Novosibirsk) Levels of the Relative Entropy Hn-3 RS
7. Discussion of the Results
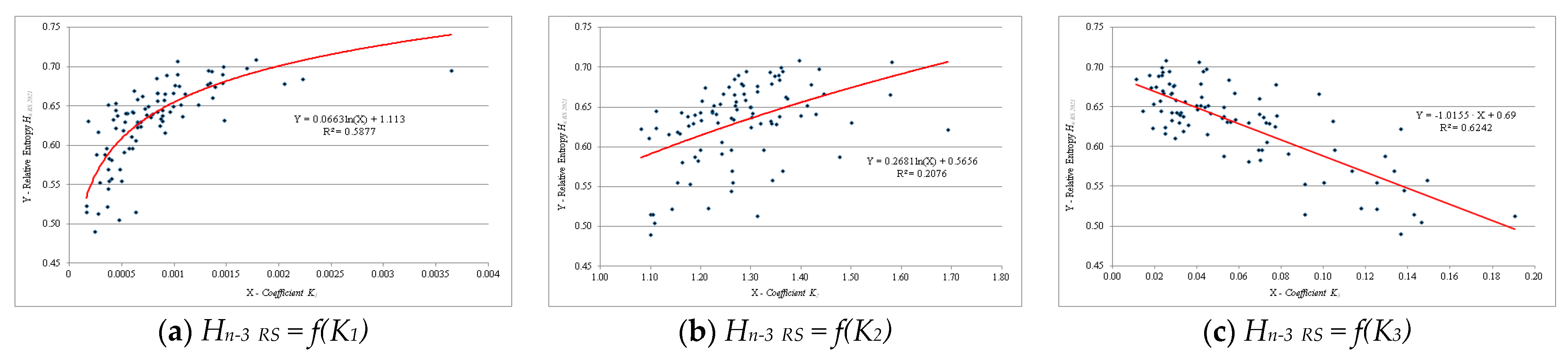
7.1. Models of Statistical Relation of Information Transfer Coefficients Ki with the Relative Entropy Hn-3 RS of Urban Road Safety Systems
- T—total time T = (T1 + T2 + 0.5∙T3) required to ensure efficient braking with the maximum possible deceleration, s;
- T1—reaction time of the driver to the danger, s;
- T2—brake actuation time, s;
- T3—time to reach maximum car deceleration, s;
- Vv—original speed of the car, m/s;
- j—deceleration of the car in full braking mode (breaking slip).
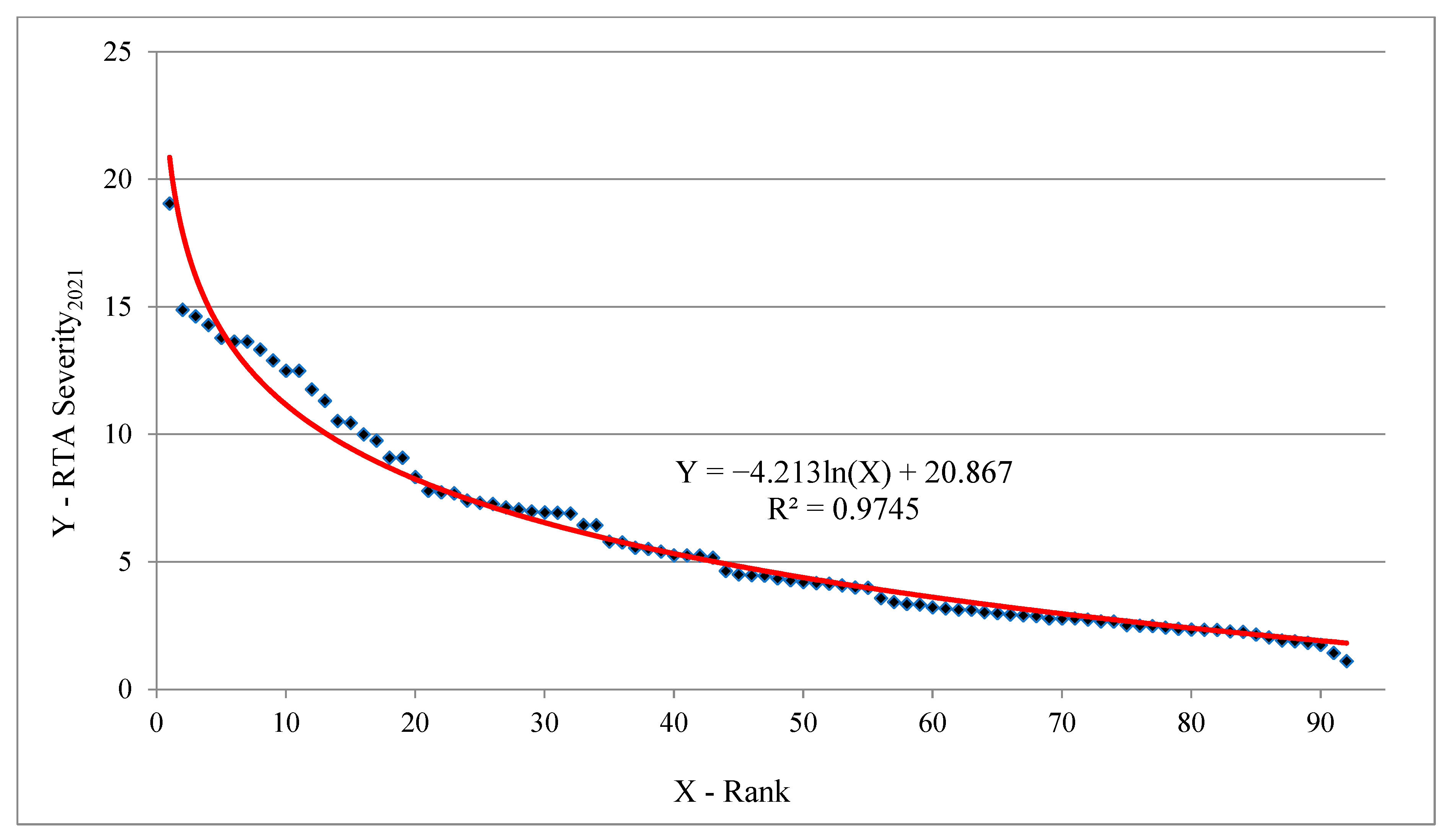
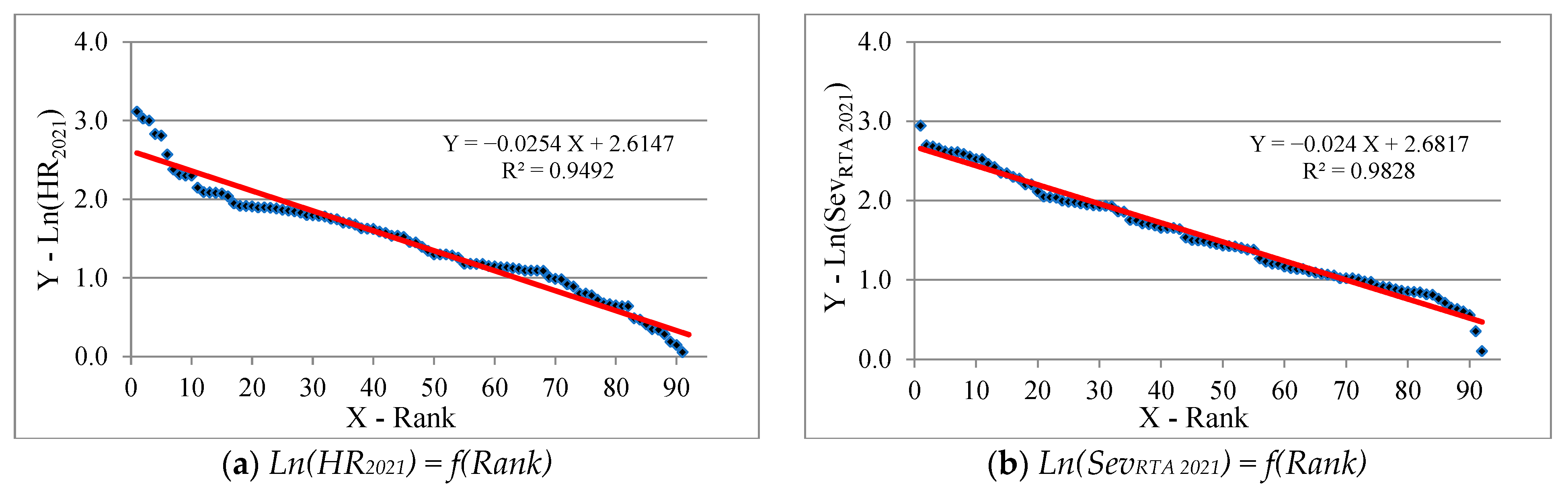
7.2. Logarithmic Law in the Description of the Ranking Distribution of Road Safety Systems in Russian Cities in Terms of Human Risk HR, RTA Severity and Hn RS
- -
- About the fundamentally different states of road safety systems in Russian cities (obviously, corresponding to different road safety paradigms [78]);
- -
- About the incorrectness of applying the same type of road safety management methods in cities characterized by such different levels of the two important indicators (HR, RTA Severity).
7.3. Philosophical and Semantic Aspects of Information Entropy Analysis of the Road Traffic Accident Rate
- -
- The probability of becoming a participant in a road accident in Feodosia is higher than in Minusinsk;
- -
- The specific number of victims in road accidents in Feodosia is higher than in Minusinsk.
7.4. Comparing the Obtained Results with Other Studies
7.5. Prospects for Further Research
8. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| NoNo | City | City Population, People | Road Traffic Accidents (RTA), Unit | RTA Victims, People | RTA Fatality Cases, Ptople | Human Risk HR, Deaths in RTA/ 100 Thous. People | RTA Severity, % | Hn-3 RS |
|---|---|---|---|---|---|---|---|---|
| 1 | Moscow | 13,010,112 | 8516 | 10,008 | 358 | 2.75 | 3.58 | 0.627 |
| 2 | St. Petersburg | 5,601,911 | 5006 | 5878 | 197 | 3.52 | 3.35 | 0.638 |
| 3 | Novosibirsk | 1,633,595 | 980 | 1175 | 83 | 5.08 | 7.06 | 0.596 |
| 4 | Ekaterinburg | 1,544,376 | 1164 | 1513 | 66 | 4.27 | 4.36 | 0.650 |
| NoNo | City | City Population, People | Road Traffic Accidents (RTA), Unit | RTA Victims, People | RTA Fatality Cases, Ptople | Human Risk HR, Deaths in RTA/ 100 Thous. People | RTA Severity, % | Hn-3 RS |
|---|---|---|---|---|---|---|---|---|
| 1 | Krasnodar | 948,827 | 939 | 1219 | 64 | 6.75 | 5.25 | 0.650 |
| 2 | Saratov | 830,155 | 1103 | 1503 | 40 | 4.82 | 2.66 | 0.695 |
| 3 | Tyumen | 816,800 | 1452 | 2027 | 51 | 6.24 | 2.52 | 0.709 |
| 4 | Togliatti | 693,072 | 633 | 802 | 22 | 3.17 | 2.74 | 0.666 |
| 5 | Izhevsk | 646,468 | 544 | 652 | 21 | 3.25 | 3.22 | 0.643 |
| 6 | Barnaul | 631,127 | 830 | 1066 | 31 | 4.91 | 2.91 | 0.677 |
| 7 | Ulyanovsk | 625,462 | 634 | 832 | 24 | 3.84 | 2.88 | 0.676 |
| 8 | Irkutsk | 617,315 | 861 | 1041 | 19 | 3.08 | 1.83 | 0.674 |
| 9 | Khabarovsk | 610,305 | 647 | 821 | 37 | 6.06 | 4.51 | 0.652 |
| 10 | Makhachkala | 604,266 | 333 | 476 | 33 | 5.46 | 6.93 | 0.641 |
| 11 | Yaroslavl | 601,403 | 598 | 744 | 18 | 2.99 | 2.42 | 0.667 |
| 12 | Vladivostok | 600,871 | 737 | 903 | 36 | 5.99 | 3.99 | 0.652 |
| 13 | Orenburg | 572,819 | 482 | 612 | 21 | 3.67 | 3.43 | 0.657 |
| 14 | Tomsk | 568,508 | 227 | 264 | 17 | 2.99 | 6.44 | 0.581 |
| 15 | Kemerovo | 552,546 | 568 | 770 | 18 | 3.26 | 2.34 | 0.690 |
| 16 | Novokuznetsk | 544,583 | 382 | 523 | 22 | 4.04 | 4.21 | 0.663 |
| 17 | Ryazan | 534,801 | 730 | 977 | 23 | 4.30 | 2.35 | 0.694 |
| 18 | Naberezhnye Chelny | 532,074 | 478 | 575 | 13 | 2.44 | 2.26 | 0.658 |
| 19 | Astrakhan | 524,371 | 702 | 899 | 24 | 4.58 | 2.67 | 0.679 |
| 20 | Kirov | 521,091 | 762 | 971 | 17 | 3.26 | 1.75 | 0.690 |
| 21 | Penza | 516,450 | 759 | 1032 | 24 | 4.65 | 2.33 | 0.700 |
| 22 | Sevastopol | 509,992 | There is no reliable data | |||||
| 23 | Balashikha | 507,307 | 139 | 161 | 4 | 0.79 | 2.48 | 0.617 |
| 24 | Lipetsk | 503,213 | 436 | 552 | 32 | 6.36 | 5.80 | 0.634 |
| NoNo | City | City Population, People | Road Traffic Accidents (RTA), Unit | RTA Victims, People | RTA Fatality Cases, Ptople | Human Risk HR, Deaths in RTA/ 100 Thous. People | RTA Severity, % | Hn-3 RS |
|---|---|---|---|---|---|---|---|---|
| 1 | Novokuibyshevsk | 99,469 | 38 | 48 | 6 | 6.03 | 12.50 | 0.555 |
| 2 | Tobolsk | 98,772 | 144 | 193 | 8 | 8.10 | 4.15 | 0.679 |
| 3 | Votkinsk | 96,861 | 52 | 64 | 2 | 2.06 | 3.13 | 0.641 |
| 4 | Sarov | 96,479 | 93 | 108 | 3 | 3.11 | 2.78 | 0.643 |
| 5 | Mezhdurechensk | 96,088 | 28 | 33 | 3 | 3.12 | 9.09 | 0.553 |
| 6 | Serov | 95,141 | 36 | 43 | 3 | 3.15 | 6.98 | 0.583 |
| 7 | Sarapul | 94,554 | 42 | 52 | 1 | 1.06 | 1.92 | 0.654 |
| 8 | Anapa | 94,340 | 344 | 444 | 19 | 20.14 | 4.28 | 0.695 |
| 9 | Leninsk-Kuznetsky | 93,288 | 80 | 104 | 8 | 8.58 | 7.69 | 0.625 |
| 10 | Ukhta | 92,479 | 156 | 224 | 10 | 10.81 | 4.46 | 0.698 |
| 11 | Glazov | 91,921 | 34 | 48 | 2 | 2.18 | 4.17 | 0.652 |
| 12 | Magadan | 91,797 | 126 | 173 | 12 | 13.07 | 6.94 | 0.661 |
| 13 | Michurinsk | 89,876 | 54 | 66 | 2 | 2.23 | 3.03 | 0.643 |
| 14 | Lobnya | 89,522 | 14 | 17 | 2 | 2.23 | 11.76 | 0.523 |
| 15 | Kansk | 88,362 | 42 | 53 | 6 | 6.79 | 11.32 | 0.570 |
| 16 | Kamensk-Shakhtinsky | 87,113 | 35 | 47 | 7 | 8.04 | 14.89 | 0.558 |
| 17 | Buzuluk | 86,042 | 71 | 90 | 1 | 1.16 | 1.11 | 0.685 |
| 18 | Kiselevsk | 85,339 | 72 | 104 | 6 | 7.03 | 5.77 | 0.667 |
| 19 | Ivanteevka | 82,779 | 20 | 22 | 3 | 3.62 | 13.64 | 0.490 |
| 20 | Novotroitsk | 82,463 | 36 | 44 | 1 | 1.21 | 2.27 | 0.645 |
| 21 | Zheleznogorsk | 81,619 | 59 | 75 | 3 | 3.68 | 4.00 | 0.647 |
| 22 | Kuznetsk | 79,800 | 86 | 112 | 8 | 10.03 | 7.14 | 0.637 |
| 23 | Ust-Ilimsk | 79,746 | 52 | 67 | 2 | 2.51 | 2.99 | 0.659 |
| 24 | Yalta | 78,171 | 174 | 236 | 13 | 16.63 | 5.51 | 0.684 |
| 25 | Ozersk | 78,128 | 37 | 41 | 6 | 7.68 | 14.63 | 0.505 |
| 26 | Gelendzhik | 75,504 | 155 | 220 | 17 | 22.52 | 7.73 | 0.678 |
| 27 | Chernogorsk | 75,348 | 43 | 57 | 6 | 7.96 | 10.53 | 0.596 |
| 28 | Usolye-Sibirskoe | 75,062 | 49 | 53 | 1 | 1.33 | 1.89 | 0.623 |
| 29 | Novoaltaysk | 74,586 | 78 | 98 | 2 | 2.68 | 2.04 | 0.675 |
| 30 | Shadrinsk | 74,400 | 69 | 93 | 2 | 2.69 | 2.15 | 0.689 |
| 31 | Verkhnyaya Pyshma | 74,262 | 55 | 77 | 6 | 8.08 | 7.79 | 0.639 |
| 32 | Troitsk | 72,723 | 47 | 62 | 4 | 5.50 | 6.45 | 0.630 |
| 33 | Chapaevsk | 70,944 | 30 | 36 | 1 | 1.41 | 2.78 | 0.633 |
| 34 | Belovo | 70,611 | 78 | 123 | 12 | 16.99 | 9.76 | 0.666 |
| 35 | Birobidzhan | 70,433 | 63 | 70 | 1 | 1.42 | 1.43 | 0.645 |
| 36 | Feodosia | 67,750 | 100 | 134 | 14 | 20.66 | 10.45 | 0.632 |
| 37 | Minusinsk | 67,234 | 60 | 74 | 4 | 5.95 | 5.41 | 0.631 |
| 38 | Sosnovy Bor | 67,054 | 53 | 63 | 2 | 2.98 | 3.17 | 0.640 |
| 39 | Anzhero-Sudzhensk | 66,933 | 56 | 72 | 2 | 2.99 | 2.78 | 0.667 |
| 40 | Buynaksk | 66,422 | 12 | 18 | 1 | 1.51 | 5.56 | 0.631 |
| 41 | Zarechny | 65,493 | 23 | 29 | 2 | 3.05 | 6.90 | 0.596 |
| 42 | Gorno-Altaysk | 64,504 | 59 | 67 | 3 | 4.65 | 4.48 | 0.616 |
| 43 | Gukovo | 62,658 | 10 | 11 | 1 | 1.60 | 9.09 | 0.515 |
| 44 | Asbest | 62,285 | 21 | 31 | 4 | 6.42 | 12.90 | 0.588 |
| 45 | Zelenogorsk | 61,821 | 23 | 29 | 4 | 6.47 | 13.79 | 0.545 |
| 46 | Donskoy | 61,477 | 28 | 38 | 2 | 3.25 | 5.26 | 0.638 |
| 47 | Izberbash | 61,296 | 16 | 19 | 1 | 1.63 | 5.26 | 0.588 |
| 48 | Revda | 60,761 | 40 | 54 | 4 | 6.58 | 7.41 | 0.629 |
| 49 | Berezovsky | 60,334 | 62 | 98 | 4 | 6.63 | 4.08 | 0.707 |
| 50 | Sibai | 60,017 | 38 | 42 | 6 | 10.00 | 14.29 | 0.515 |
| 51 | Polevskoy | 60,012 | 41 | 55 | 4 | 6.67 | 7.27 | 0.630 |
| 52 | Lytkarino | 59,937 | 16 | 21 | 4 | 6.67 | 19.05 | 0.513 |
| 53 | Kumertau | 59,129 | 26 | 44 | 6 | 10.15 | 13.64 | 0.622 |
| 54 | Fryazino | 58,938 | 21 | 24 | 3 | 5.09 | 12.50 | 0.522 |
| 55 | Prokhladny | 58,747 | 22 | 30 | 4 | 6.81 | 13.33 | 0.570 |
| 56 | Nyagan | 58,733 | 46 | 58 | 3 | 5.11 | 5.17 | 0.636 |
| 57 | Krasnoturinsk | 55,910 | 29 | 36 | 3 | 5.37 | 8.33 | 0.591 |
| 58 | Severomorsk | 54,473 | 33 | 43 | 2 | 3.67 | 4.65 | 0.642 |
| 59 | Volzhsk | 52,703 | 36 | 40 | 1 | 1.90 | 2.50 | 0.624 |
| 60 | Vorkuta | 52,292 | 33 | 41 | 3 | 5.74 | 7.32 | 0.606 |
| 61 | Goose-Crystal | 51,998 | 32 | 42 | 1 | 1.92 | 2.38 | 0.670 |
| 62 | Snezhinsk | 51,921 | 26 | 30 | 3 | 5.78 | 10.00 | 0.555 |
| 63 | Arsenyev | 52,723 | 31 | 34 | 1 | 1.90 | 2.94 | 0.611 |
| 64 | Salekhard | 51,186 | 26 | 30 | 1 | 1.95 | 3.33 | 0.619 |
| 65 | Kotelniki | 50,723 | 27 | 32 | 1 | 1.97 | 3.13 | 0.630 |
| NoNo | Cities | Values (2021) | ||||
|---|---|---|---|---|---|---|
| HRS | Hn RS | K1 = KRA | K2 = KV | K3 = KD | ||
| Largest cities (1500 thous. people < Population < 13,000 thous. people) | ||||||
| 1 | Moscow | 0.689 | 0.627 | 0.00065 | 1.1752 | 0.0358 |
| 2 | St. Petersburg | 0.700 | 0.638 | 0.00089 | 1.1742 | 0.0335 |
| 3 | Novosibirsk | 0.655 | 0.596 | 0.00060 | 1.1990 | 0.0706 |
| 4 | Ekaterinburg | 0.715 | 0.650 | 0.00075 | 1.2998 | 0.0436 |
| Large cities (500 thous. people < Population < 1000 thous. people) | ||||||
| 1 | Krasnodar | 0.714 | 0.650 | 0.00099 | 1.2982 | 0.0525 |
| 2 | Saratov | 0.763 | 0.695 | 0.00133 | 1.3626 | 0.0266 |
| 3 | Tyumen | 0.779 | 0.709 | 0.00178 | 1.3960 | 0.0252 |
| 4 | Togliatti | 0.732 | 0.666 | 0.00091 | 1.2670 | 0.0274 |
| 5 | Izhevsk | 0.707 | 0.643 | 0.00084 | 1.1985 | 0.0322 |
| 6 | Barnaul | 0.744 | 0.677 | 0.00132 | 1.2843 | 0.0291 |
| 7 | Ulyanovsk | 0.743 | 0.676 | 0.00101 | 1.3123 | 0.0288 |
| 8 | Irkutsk | 0.740 | 0.674 | 0.00139 | 1.2091 | 0.0183 |
| 9 | Khabarovsk | 0.717 | 0.652 | 0.00106 | 1.2689 | 0.0451 |
| 10 | Makhachkala | 0.704 | 0.641 | 0.00055 | 1.4294 | 0.0693 |
| 11 | Yaroslavl | 0.733 | 0.667 | 0.00099 | 1.2441 | 0.0242 |
| 12 | Vladivostok | 0.716 | 0.652 | 0.00123 | 1.2252 | 0.0399 |
| 13 | Orenburg | 0.722 | 0.657 | 0.00084 | 1.2697 | 0.0343 |
| 14 | Tomsk | 0.639 | 0.581 | 0.00040 | 1.1630 | 0.0644 |
| 15 | Kemerovo | 0.758 | 0.690 | 0.00103 | 1.3556 | 0.0234 |
| 16 | Novokuznetsk | 0.728 | 0.663 | 0.00070 | 1.3691 | 0.0421 |
| 17 | Ryazan | 0.762 | 0.694 | 0.00136 | 1.3384 | 0.0235 |
| 18 | Naberezhnye Chelny | 0.722 | 0.658 | 0.00090 | 1.2029 | 0.0226 |
| 19 | Astrakhan | 0.746 | 0.679 | 0.00134 | 1.2806 | 0.0267 |
| 20 | Kirov | 0.758 | 0.690 | 0.00146 | 1.2743 | 0.0175 |
| 21 | Penza | 0.769 | 0.700 | 0.00147 | 1.3597 | 0.0233 |
| 22 | Sevastopol | 0.678 | 0.617 | 0.00027 | 1.1583 | 0.0248 |
| 23 | Balashikha | 0.697 | 0.634 | 0.00087 | 1.2661 | 0.0580 |
| Small cities (50 thous. people < Population < 100 thous. people) | ||||||
| 1 | Novokuibyshevsk | 0.610 | 0.555 | 0.00038 | 1.2632 | 0.1250 |
| 2 | Tobolsk | 0.746 | 0.679 | 0.00146 | 1.3403 | 0.0415 |
| 3 | Votkinsk | 0.704 | 0.641 | 0.00054 | 1.2308 | 0.0313 |
| 4 | Sarov | 0.706 | 0.643 | 0.00096 | 1.1613 | 0.0278 |
| 5 | Mezhdurechensk | 0.607 | 0.553 | 0.00029 | 1.1786 | 0.0909 |
| 6 | Serov | 0.640 | 0.583 | 0.00038 | 1.1944 | 0.0698 |
| 7 | Sarapul | 0.719 | 0.654 | 0.00044 | 1.2381 | 0.0192 |
| 8 | Anapa | 0.763 | 0.695 | 0.00365 | 1.2907 | 0.0428 |
| 9 | Leninsk-Kuznetsky | 0.687 | 0.625 | 0.00086 | 1.3000 | 0.0769 |
| 10 | Ukhta | 0.767 | 0.698 | 0.00169 | 1.4359 | 0.0446 |
| 11 | Glazov | 0.717 | 0.652 | 0.00037 | 1.4118 | 0.0417 |
| 12 | Magadan | 0.726 | 0.661 | 0.00137 | 1.3730 | 0.0694 |
| 13 | Michurinsk | 0.706 | 0.643 | 0.00060 | 1.2222 | 0.0303 |
| 14 | Lobnya | 0.575 | 0.523 | 0.00016 | 1.2143 | 0.1176 |
| 15 | Kansk | 0.627 | 0.570 | 0.00048 | 1.2619 | 0.1132 |
| 16 | Kamensk-Shakhtinsky | 0.613 | 0.558 | 0.00040 | 1.3429 | 0.1489 |
| 17 | Buzuluk | 0.753 | 0.685 | 0.00083 | 1.2676 | 0.0111 |
| 18 | Kiselevsk | 0.732 | 0.667 | 0.00084 | 1.4444 | 0.0577 |
| 19 | Ivanteevka | 0.538 | 0.490 | 0.00024 | 1.1000 | 0.1364 |
| 20 | Novotroitsk | 0.709 | 0.645 | 0.00044 | 1.2222 | 0.0227 |
| 21 | Zheleznogorsk | 0.711 | 0.647 | 0.00072 | 1.2712 | 0.0400 |
| 22 | Kuznetsk | 0.700 | 0.637 | 0.00108 | 1.3023 | 0.0714 |
| 23 | Ust-Ilimsk | 0.724 | 0.659 | 0.00065 | 1.2885 | 0.0299 |
| 24 | Yalta | 0.752 | 0.684 | 0.00223 | 1.3563 | 0.0551 |
| 25 | Ozersk | 0.555 | 0.505 | 0.00047 | 1.1081 | 0.1463 |
| 26 | Gelendzhik | 0.744 | 0.678 | 0.00205 | 1.4194 | 0.0773 |
| 27 | Chernogorsk | 0.654 | 0.596 | 0.00057 | 1.3256 | 0.1053 |
| 28 | Usolye-Sibirskoe | 0.685 | 0.623 | 0.00065 | 1.0816 | 0.0189 |
| 29 | Novoaltaysk | 0.742 | 0.675 | 0.00105 | 1.2564 | 0.0204 |
| 30 | Shadrinsk | 0.756 | 0.689 | 0.00093 | 1.3478 | 0.0215 |
| 31 | Verkhnyaya Pyshma | 0.702 | 0.639 | 0.00074 | 1.4000 | 0.0779 |
| 32 | Troitsk | 0.693 | 0.630 | 0.00065 | 1.3191 | 0.0645 |
| 33 | Chapaevsk | 0.695 | 0.633 | 0.00042 | 1.2000 | 0.0278 |
| 34 | Belovo | 0.732 | 0.666 | 0.00110 | 1.5769 | 0.0976 |
| 35 | Birobidzhan | 0.709 | 0.645 | 0.00089 | 1.1111 | 0.0143 |
| 36 | Feodosia | 0.694 | 0.632 | 0.00148 | 1.3400 | 0.1045 |
| 37 | Minusinsk | 0.693 | 0.631 | 0.00089 | 1.2333 | 0.0541 |
| 38 | Sosnovy Bor | 0.703 | 0.640 | 0.00079 | 1.1887 | 0.0317 |
| 39 | Anzhero-Sudzhensk | 0.733 | 0.667 | 0.00084 | 1.2857 | 0.0278 |
| 40 | Buynaksk | 0.693 | 0.631 | 0.00018 | 1.5000 | 0.0556 |
| 41 | Zarechny | 0.655 | 0.596 | 0.00035 | 1.2609 | 0.0690 |
| 42 | Gorno-Altaysk | 0.676 | 0.616 | 0.00091 | 1.1356 | 0.0448 |
| 43 | Gukovo | 0.565 | 0.515 | 0.00016 | 1.1000 | 0.0909 |
| 44 | Asbest | 0.646 | 0.588 | 0.00034 | 1.4762 | 0.1290 |
| 45 | Zelenogorsk | 0.599 | 0.545 | 0.00037 | 1.2609 | 0.1379 |
| 46 | Donskoy | 0.701 | 0.638 | 0.00046 | 1.3571 | 0.0526 |
| 47 | Izberbash | 0.646 | 0.588 | 0.00026 | 1.1875 | 0.0526 |
| 48 | Revda | 0.691 | 0.629 | 0.00066 | 1.3500 | 0.0741 |
| 49 | Berezovsky | 0.776 | 0.707 | 0.00103 | 1.5806 | 0.0408 |
| 50 | Sibai | 0.566 | 0.515 | 0.00063 | 1.1053 | 0.1429 |
| 51 | Polevskoy | 0.692 | 0.630 | 0.00068 | 1.3415 | 0.0727 |
| 52 | Lytkarino | 0.563 | 0.513 | 0.00027 | 1.3125 | 0.1905 |
| 53 | Kumertau | 0.684 | 0.622 | 0.00044 | 1.6923 | 0.1364 |
| 54 | Fryazino | 0.574 | 0.522 | 0.00036 | 1.1429 | 0.1250 |
| 55 | Prokhladny | 0.626 | 0.570 | 0.00037 | 1.3636 | 0.1333 |
| 56 | Nyagan | 0.698 | 0.636 | 0.00078 | 1.2609 | 0.0517 |
| 57 | Krasnoturinsk | 0.650 | 0.591 | 0.00052 | 1.2414 | 0.0833 |
| 58 | Severomorsk | 0.705 | 0.642 | 0.00061 | 1.3030 | 0.0465 |
| 59 | Volzhsk | 0.686 | 0.624 | 0.00068 | 1.1111 | 0.0250 |
| 60 | Vorkuta | 0.666 | 0.606 | 0.00063 | 1.2424 | 0.0732 |
| 61 | Goose-Crystal | 0.736 | 0.670 | 0.00062 | 1.3125 | 0.0238 |
| 62 | Snezhinsk | 0.609 | 0.555 | 0.00050 | 1.1538 | 0.1000 |
| 63 | Arsenyev | 0.671 | 0.611 | 0.00059 | 1.0968 | 0.0294 |
| 64 | Salekhard | 0.680 | 0.619 | 0.00051 | 1.1538 | 0.0333 |
| 65 | Kotelniki | 0.692 | 0.630 | 0.00053 | 1.1852 | 0.0313 |
References
- Blincoe, L.J.; Miller, T.R.; Zaloshnja, E.; Lawrence, B.A. The Economic and Societal Impact of Motor Vehicle Crashes, 2010 (Revised); Report No. DOT HS 812 013; National Highway Traffic Safety Administration: Washington, DC, USA, 2015. Available online: https://crashstats.nhtsa.dot.gov/Api/Public/ViewPublication/812013 (accessed on 8 February 2023).
- Blincoe, L.; Miller, T.; Wang, J.-S.; Swedler, D.; Coughlin, T.; Lawrence, B.; Guo, F.; Klauer, S.; Dingus, T. The Economic and Societal Impact of Motor Vehicle Crashes, 2019 (Revised); Report No. DOT HS 813 403; National Highway Traffic Safety Administration: Washington, DC, USA, 2023; Available online: https://www.researchgate.net/publication/367460305_The_Economic_and_Societal_Impact_of_Motor_Vehicle_Crashes_2019_Revised/link/63eea3fb2958d64a5cd5f9d0/download (accessed on 8 February 2023).
- IRTAD Road Safety Annual Report 2022; OECD Publishing: Paris, France, 2022. Available online: https://www.itf-oecd.org/sites/default/files/docs/irtad-road-safety-annual-report-2022.pdf (accessed on 8 February 2023).
- Petrov, A.I.; Kolesov, V.I. Road traffic accident rate in Russia: Main socio-economic factors of its formation and spatio-temporal features. Econ. Soc. Chang. Facts Trends Forecast. 2021, 14, 199–220. [Google Scholar] [CrossRef]
- Smeed, R.J. Some Statistical Aspects of Road Safety Research. J. R. Stat. Soc. Ser. A 1949, 112, 1–34. [Google Scholar] [CrossRef]
- Global Status Report on Road Safety 2018. World Health Organization, Geneva, 2018. Available online: https://www.who.int/publications/i/item/9789241565684 (accessed on 11 February 2023).
- Doyle, P. Marketing Management and Strategy; Prentice Hall Europe: London, UK; New York, NY, USA, 1998; p. 465. [Google Scholar]
- Provost, F.; Fawcett, T. Data Science for Business; O’Reilly Media, Inc.: Beijing, China; Cambridge, UK; Tokyo, Japan, 2013; p. 409. Available online: https://www.booksfree.org/wp-content/uploads/2022/06/Data-Science-for-Business-by-Foster-Provost-and-Tom-Fawcett-pdf-free-download-booksfree.org_.pdf (accessed on 13 February 2023).
- Derriks, H.M.; Mak, P.M. Underreporting of Road Traffic Casualties: IRTAD Special Report. 2007, p. 39. Available online: https://www.itf-oecd.org/sites/default/files/docs/repndl2007.pdf (accessed on 15 February 2023).
- Pyankova, A.I.; Fattakhov, T.A.; Kozlov, V.A. The association between beverage-specific alcohol consumption and mortality among road users in Russia, 1965–2019. Accid. Anal. Prev. 2022, 178, 106859. [Google Scholar] [CrossRef] [PubMed]
- Fattahov, T.A.; Piankova, A.I. The Years of Life Lost as a Result of Road Traffic Accidents in Russia in 2000–2012. Probl. Sotsialnoi Gig Zdravookhranenniia Istor. Med. 2018, 26, 271–275. [Google Scholar] [CrossRef]
- Elvik, R. Which is the more important for road safety—Road design or driver behavioural adaptation? Traffic Saf. Res. 2022, 2, 000009. [Google Scholar] [CrossRef]
- Halkos, G.; Kitsos, C. Uncertainty in environmental economics: The problem of entropy and model choice. Econ. Anal. Policy 2018, 60, 127–140. [Google Scholar] [CrossRef]
- Webster, M.; Sell, J. Why Do Experiments? In Laboratory Experiments in the Social Sciences; Academic Press: Cambridge, MA, USA, 2014; Chapter 1; pp. 5–21. [Google Scholar] [CrossRef]
- Serra, D. The Experimental method in economics: Old issues and new challenges. Rev. Philos. Économique 2012, 1, 3–19. [Google Scholar] [CrossRef]
- Georgescu-Roegen, N. The Entropy Law and the Economic Process; Harvard University Press: Cambridge, MA, USA, 1971; p. 457. [Google Scholar] [CrossRef]
- Georgescu-Roegen, N. The Entropy Law and the Economic Process in Retrospect. East. Econ. J. 1986, 12, 3–25. [Google Scholar]
- Islamutdinov, V.F.; Kurikov, V.M. On the application of the entropy approach in economic research. Sib. Financ. Sch. 2022, 2, 168–178. (In Russian) [Google Scholar] [CrossRef]
- Avery, J.S. CADMUS. A papers series of the South-East European Division of the World Academy of Art and Science (SEED-WAAS). Entropy Econ. 2012, 1, 166–179. [Google Scholar]
- Halkos, G.; Kitsos, C. Relative risk and innovation activities: The case of Greece. Innov. Manag. Policy Pract. 2012, 14, 156–159. [Google Scholar] [CrossRef]
- Devine, S. An Economy Viewed as a Far–from–Equilibrium System from the Perspective of Algorithmic Information Theory. Entropy 2018, 20, 228. [Google Scholar] [CrossRef] [PubMed]
- Bailey, K.D. Social Entropy Theory; SUNY Press: Albany, NY, USA, 1990; Available online: https://books.google.ru/books?hl=ru&lr=&id=gSX-tdH0Z0kC&oi=fnd&pg=PR13&ots=xzeA4KCxe8&sig=DCEhhYxoprF8eOHiViiW0AdHsSw&redir_esc=y#v=onepage&q&f=false (accessed on 27 February 2023).
- Mayer, A.L.; Donovan, R.P.; Pawlowski, C.W. Information and entropy theory for the sustainability of coupled human and natural systems. Ecol. Soc. 2014, 19, 11. [Google Scholar] [CrossRef]
- Tolstykh, T.; Shmeleva, N.; Vertakova, Y.; Plotnikov, V. The Entropy Model for Sustainability Assessment in Industrial Ecosystems. Inventions 2020, 5, 54. [Google Scholar] [CrossRef]
- Saslow, W.M. An economic analogy to thermodynamics. Am. J. Phys. 1999, 67, 1239–1247. [Google Scholar] [CrossRef]
- Vozna, L.Y. The Notion of Entropy in Economic Analysis: The Classical Examples and New Perspectives. J. Heterodox Econ. 2016, 3, 1–16. [Google Scholar] [CrossRef]
- Antoniou, I.; Ivanov, V.V.; Kryanev, A.V.; Matokhin, V.V.; Shapovalov, M.V. On the efficient resources distribution in economics based on entropy. Phys. A Stat. Mech. Its Appl. 2004, 336, 549–562. [Google Scholar] [CrossRef]
- Zheng, F.; Song, Q. Entropy Maximization Based Capability Allocation of Clothing Production Enterprises in Sharing Economy. IFAC-PapersOnLine 2019, 52, 211–216. [Google Scholar] [CrossRef]
- Prigogine, I. The rediscovery of value and the opening of economics. Chapter 2. In The Evolutionary Foundation of Economics; Cambridge University Press: Cambridge, UK, 2005; pp. 61–69. [Google Scholar]
- Ho, M.W. What is (Schrödinger’s) Negentropy? Mod. Trends Bio Kinet. 1994, 3, 50–61. Available online: https://www.researchgate.net/publication/255612623_What_is_Schrodinger's_Negentropy (accessed on 2 March 2023).
- Jaynes, E.T. How Should We Use Entropy in Economics? (Some Half-Baked Ideas in Need of Criticism); St. John’s College: Cambridge, UK, 1991. Available online: https://bayes.wustl.edu/etj/articles/entropy.in.economics.pdf (accessed on 2 March 2023).
- Raine, A.; Foster, J.; Potts, J. The New Entropy Law and the Economic Process. Ecol. Complex. 2006, 3, 354–360. Available online: https://www.researchgate.net/publication/223632694_The_new_entropy_law_and_the_economic_process (accessed on 2 March 2023). [CrossRef]
- Chen, J. An Entropy Theory of Value. SSRN Electron. J. 2002, 1–22. [Google Scholar] [CrossRef]
- Yang, J. Information theoretic approaches in economics. J. Econ. Surv. 2018, 32, 940–960. [Google Scholar] [CrossRef]
- Rodríguez, R.A.; Cáceres-Hernández, J.J. Information, entropy, value, and price formation: An econophysical perspective. Phys. A: Stat. Mech. Its Appl. 2018, 512, 74–85. [Google Scholar] [CrossRef]
- Anttila, J.; Annila, A. Natural Game. Phys. Lett. A 2011, 375, 3755–3761. Available online: https://arxiv.org/abs/1103.1656v1 (accessed on 3 March 2023). [CrossRef]
- Caticha, A.; Golan, A. An entropic framework for modeling economies. Phys. A Stat. Mech. Its Appl. 2014, 408, 149–163. [Google Scholar] [CrossRef]
- Cabrales, A.; Gossner, O.; Serrano, R. Entropy and the value of information for investors. Am. Econ. Rev. 2013, 103, 360–377. [Google Scholar] [CrossRef]
- Xu, B.; Zhang, H.; Wang, Z.; Zhang, J. Test the principle of maximum entropy in constant sum 2 × 2 game: Evidence in experimental economics. Phys. Lett. A 2012, 376, 1318–1322. [Google Scholar] [CrossRef]
- Smith, J. Maximum Entropy and Information Theory Approaches to Economics; The Boeing Company: Arlington, VA, USA, 2018; p. 22. Available online: https://doi.org/10.2139/ssrn.3094757 (accessed on 5 March 2023).
- Foley, D.K. Information Theory and Behavior; Department of Economics, The New School for Social Research: New York, NY, USA, 2017; p. 21. Available online: https://www.researchgate.net/publication/322024633_Information_theory_and_behavior (accessed on 6 March 2023). [CrossRef]
- Scharfenaker, E.; Foley, D.K. A Maximum Entropy Solution to Quantal Response Equilibria in Economic Models. 2016, p. 27. Available online: https://www.researchgate.net/publication/312153408_A_Maximum_Entropy_Solution_to_Quantal_Response_Equilibria_in_Economic_Models (accessed on 6 March 2023). [CrossRef]
- Judge, G. Entropy maximization as a basis for information recovery in dynamic economic behavioral systems. Econometrics 2015, 3, 91–100. [Google Scholar] [CrossRef]
- Judge, G. Micro-Macro Connected Stochastic Dynamic Economic Behavior Systems. Econometrics 2018, 6, 46. [Google Scholar] [CrossRef]
- Duan, Y.; Mu, H.; Li, N.; Li, L.; Xue, Z. Research on comprehensive evaluation of low carbon economy development level based on AHP-Entropy method: A case study of Dalian. Energy Procedia 2016, 104, 468–474. [Google Scholar] [CrossRef]
- Jakimowicz, A. The Role of Entropy in the Development of Economics. Entropy 2020, 22, 452. [Google Scholar] [CrossRef]
- Mazumder, S.K.; Das, N.C. Maximum Entropy and Utility in a Transportation System. Yugosl. J. Oper. Res. 1999, 9, 27–34. Available online: http://elib.mi.sanu.ac.rs/files/journals/yjor/17/yujorn17p27-34.pdf (accessed on 9 March 2023).
- Zhou, H.; Bouyekhf, R.; Moudni, A.E.L. Concept of Transportation Entropy and Its Application in Traffic Signal Control. IFAC Proc. Vol. 2013, 46, 37–42. [Google Scholar] [CrossRef]
- Yu, S.; Liu, Y.; Liang, X. Evolution of Regional Transport Structure Information Entropy and Its Dynamic Analysis. J. Transp. Syst. Eng. Inf. Technol. 2011, 11, 91–96. [Google Scholar] [CrossRef]
- Bazaluk, O.; Kotenko, S.; Nitsenko, V. Entropy as an Objective Function of Optimization Multimodal Transportations. Entropy 2021, 23, 946. [Google Scholar] [CrossRef] [PubMed]
- Petrov, A.I. Entropy Method of Road Safety Management: Case Study of the Russian Federation. Entropy 2022, 24, 177. [Google Scholar] [CrossRef]
- Petrov, A.I. Information and Entropy Aspects of the Specifics of Regional Road Traffic Accident Rate in Russia. Information 2023, 14, 138. [Google Scholar] [CrossRef]
- Bernton, E.; Ghosal, P.; Nutz, M. Entropic Optimal Transport: Geometry and Large Deviations. Duke Math. J. 2022, 171, 3363–3400. Available online: https://arxiv.org/pdf/2102.04397.pdf (accessed on 11 March 2023). [CrossRef]
- Nutz, M. Introduction to Entropic Optimal Transport. 2022. Available online: https://www.math.columbia.edu/~mnutz/docs/EOT_lecture_notes.pdf (accessed on 12 March 2023).
- Liero, M.; Mielke, A.; Savare, G. Optimal Entropy-Transport Problems and a New Hellinger-Kantorovich Distance between Positive Measures. 2016. Available online: https://arxiv.org/pdf/1508.07941.pdf (accessed on 13 March 2023).
- Bogdanov, A.A. Tectology. Universal Organizational Science; Academic Project: Moscow, Russia, 2020; p. 712. Available online: https://royallib.com/book/bogdanov_aleksandr/tektologiya_vseobshchaya_organizatsionnaya_nauka.html?ysclid=lf5k6g3cj6149182741 (accessed on 15 March 2023). (In Russian)
- Haken, H. Synergetics: An Introduction: Nonequilibrium Phase Transitions and Self-Organization in Physics, Chemistry, and Biology; Springer: Berlin, Germany; New York, NY, USA, 1978; p. 355. [Google Scholar]
- Shannon, C.E. Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Shannon, C.E. Communication Theory of Secrecy Systems. Bell Syst. Tech. J. 1949, 28, 656–715. [Google Scholar] [CrossRef]
- Kolesov, V.; Petrov, A. Cybernetic Modeling in Tasks of Traffic Safety Management. Transp. Res. Procedia 2017, 20, 305–310. [Google Scholar] [CrossRef]
- Official Website of the Federal State Statistics Service of the Russian Federation. Available online: https://rosstat.gov.ru/vpn_popul (accessed on 16 March 2023).
- The Official Website of the State Traffic Inspectorate of the Russian Federation. Indicators of the State of Road Safety. Available online: http://stat.gibdd.ru/ (accessed on 1 March 2023).
- Traffic Regulations 2023. Official Text with Illustrations; Piter: Moscow, Russia, 2023; p. 96. [Google Scholar]
- Moody, S.; Melia, S. Shared space—Research, policy and problems. Proc. Inst. Civ. Eng. Transp. 2014, 167, 384–392 . [Google Scholar] [CrossRef]
- Monderman, H. An exploration of a more fundamental view on traffic structure. In Proceedings of the Third International Conference on Safety and Environment in the 21st Century: Lessons from the Past, Shaping the Future, Tel Aviv, Israel, 7–10 November 1994; pp. 390–396. [Google Scholar]
- Monderman, H.; Clarke, E.; Baillie, B.H. Shared space—The alternative approach to calming traffic. Traffic Eng. Control. 2006, 47, 290–292. [Google Scholar]
- Methorst, R.; Gerlach, J.; Boenke, D.; Leven, J. Shared Space: Safe or Dangerous? A Contribution to Objectification of a Popular Design Philosophy. 2007. Available online: https://wwbpa.org/wp-content/uploads/2010/12/SharedSpace-Safe-or-Dangerous-Walk21.pdf (accessed on 19 March 2023).
- Monderman, H.; Clarke, E. What’s next for shared space? We look at the UK experience. Traffic Eng. Control. 2008, 49, 205–207. [Google Scholar]
- Li, Y.; Cheng, H.; Zeng, Z.; Liu, H.; Sester, M. Autonomous Vehicles Drive into Shared Spaces: eHMI Design Concept Focusing on Vulnerable Road Users. Available online: https://arxiv.org/pdf/2104.06916v1.pdf (accessed on 20 March 2023).
- Bucchi, A.; Sangiorgi, C.; Vignali, V. Traffic Psychology and Driver Behavior. Procedia Soc. Behav. Sci. 2012, 53, 973–980. [Google Scholar] [CrossRef]
- Zhang, X.; Chang, R.; Sui, X.; Li, Y. Influences of Emotion on Driving Decisions at Different Risk Levels: An Eye Movement Study. Front. Psychol. 2022, 13, 788712. [Google Scholar] [CrossRef]
- Russian Demographic Data Sheet. 2019. Available online: http://populationrussia.ru/data/RussianDemographicDataSheet2019_web.pdf (accessed on 21 March 2023).
- Wilde, G.J.S. The Theory of Risk Homeostasis: Implications for Safety and Health. Risk Anal. 1982, 2, 209–225. [Google Scholar] [CrossRef]
- Wilde, G.J.S.; Target Risk 3. Risk Homeostasis in Everyday Life (Electronic Copy). 2014, p. 235. Available online: https://safetyrisk.net/wp-content/uploads/2016/09/Target-Risk-3-Free-Download.pdf (accessed on 21 March 2023).
- ODM 218.6.015-2015 Recommendations on Accounting and Analysis of Road Accidents on the Highways of the Russian Federation. Available online: https://docs.cntd.ru/document/1200120721?ysclid=lek8tio876301734531 (accessed on 22 March 2023).
- Bagnenko, S.F.; Miroshnichenko, A.G.; Alimov, R.R.; Shlyafer, S.I. Assessment of emergency medical care delivered in different conditions in the Russian Federation. Anesteziol. Reanimatol. 2021, 2, 124–130. [Google Scholar] [CrossRef]
- Russian Healthcare System Overview. Available online: file:///C:/Users/Artur/Downloads/f68e677a2e9b501660f093c5c11b62a3.pdf (accessed on 29 March 2023).
- Blinkin, M.Y.; Reshetova, E.M. Road Safety: The History of the Issue, International Experience, Basic Institutions; Publishing House of the Higher School of Economics: Moscow, Russia, 2013; 240p. (In Russian) [Google Scholar]
- Hegel, G.W.F. Lectures on the Philosophy of World History; Oxford University Press: Oxford, UK, 1975; p. 252. [Google Scholar]
- Tingting, G.; Wuhong, W.; Zhongyi, X. Intrinsic Safety Evaluation for Urban Road Traffic Based on Information Entropy and Triangular Fuzzy Number. MATEC Web Conf. 2016, 81, 681020. [Google Scholar] [CrossRef]
- Rui, Z.; Zhenhong, Y. Research on fuzzy comprehensive evaluation system of tailings based on entropy technology. J. Sci. Technol. 2011, 7, 107–111. [Google Scholar]
- Wei, Z.; Xiaojing, L. A comprehensive evaluation method based on information entropy. Sci. Technol. Eng. 2010, 10, 5839–5843. [Google Scholar]
- Qin, C.; Chen, X.; Yang, Y.; Gui, W. Research on safety Three-level evaluation of tailings reservoir based on FAHP-IE method. Control. Eng. China 2014, 21, 995–1000. [Google Scholar]
- Liu, H.H.; Wang, N.; Xie, J.C.; Jiang, R.G. Fuzzy assessment of city ecosystem health based on entropy weight method. Eng. J. Wuhan Univ. 2014, 47, 755–759. [Google Scholar]
- Damjanović, M.; Stević, Ž.; Stanimirović, D.; Tanackov, I.; Marinković, D. Impact of the number of vehicles on traffic safety: Multiphase modeling. Facta Univ. Ser. Mech. Eng. 2022, 20, 177–197. [Google Scholar] [CrossRef]
- Stević, Ž.; Subotić, M.; Softić, E.; Božić, B. Multi-Criteria Decision-Making Model for Evaluating Safety of Road Sections. J. Intell. Manag. Decis. 2022, 1, 78–87. [Google Scholar] [CrossRef]
- Li, M.; Zhou, X.Y.; Liu, J.X.; Ma, W.K.; Li, X.W. Topological Modeling and Analysis of Urban Rail Transit Safety Risk Relationship. J. Intell. Manag. Decis. 2022, 1, 108–117. [Google Scholar] [CrossRef]
- Tsvetkov, V.Y. Complexity Index. Eur. J. Technol. Des. 2013, 1, 64–69. [Google Scholar]
- Tsvetkov, V.Y. Information Constructions. Eur. J. Technol. Des. 2014, 5, 147–152. [Google Scholar]
- Tsvetkov, V.Y. Cognitive information models. Life Sci. J. 2014, 11, 468–471. [Google Scholar]
- Hung, S.Y. Expert versus novice use of the executive support systems: An empirical study. Inf. Manag. 2003, 40, 177–189. [Google Scholar] [CrossRef]
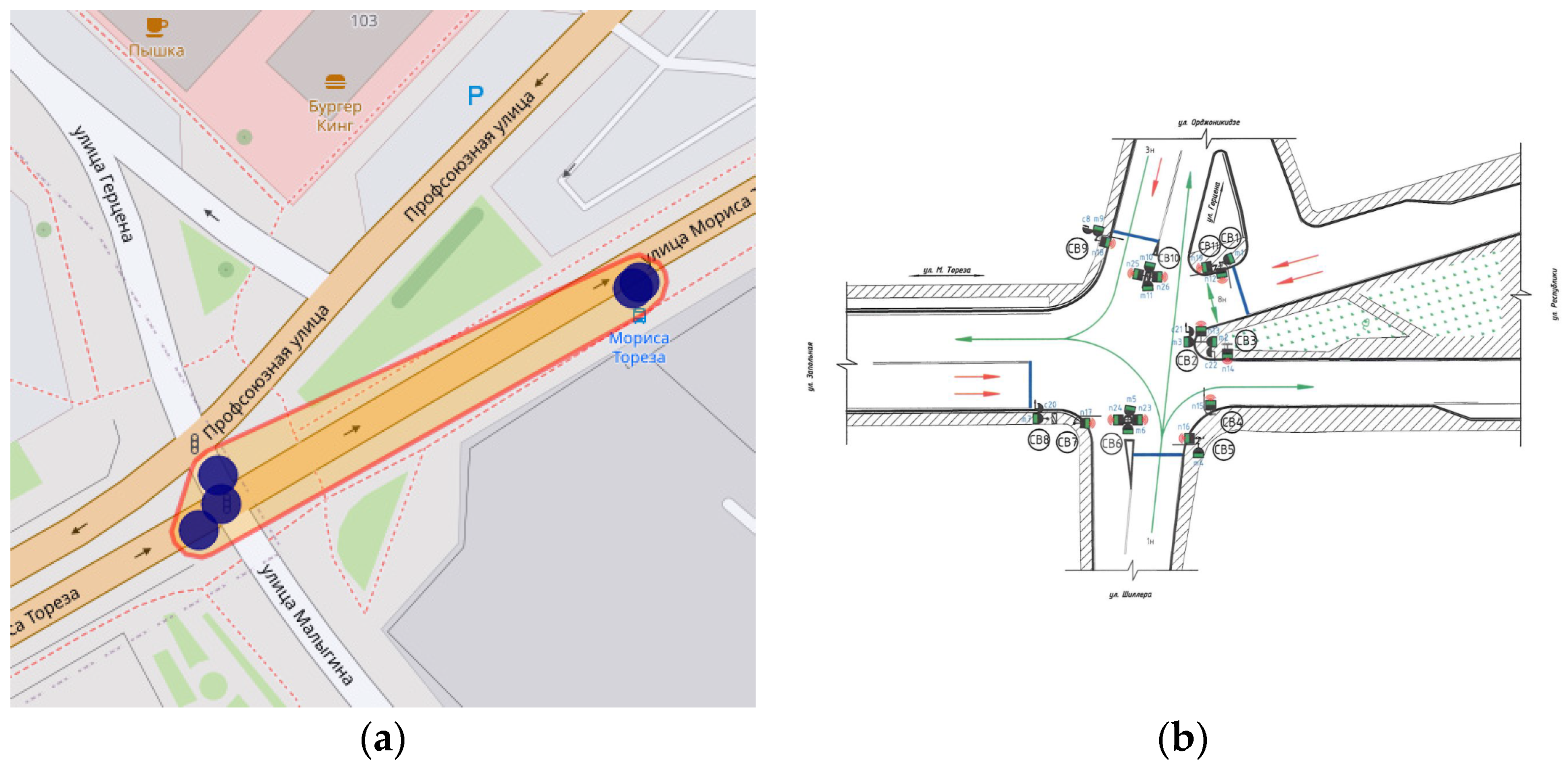
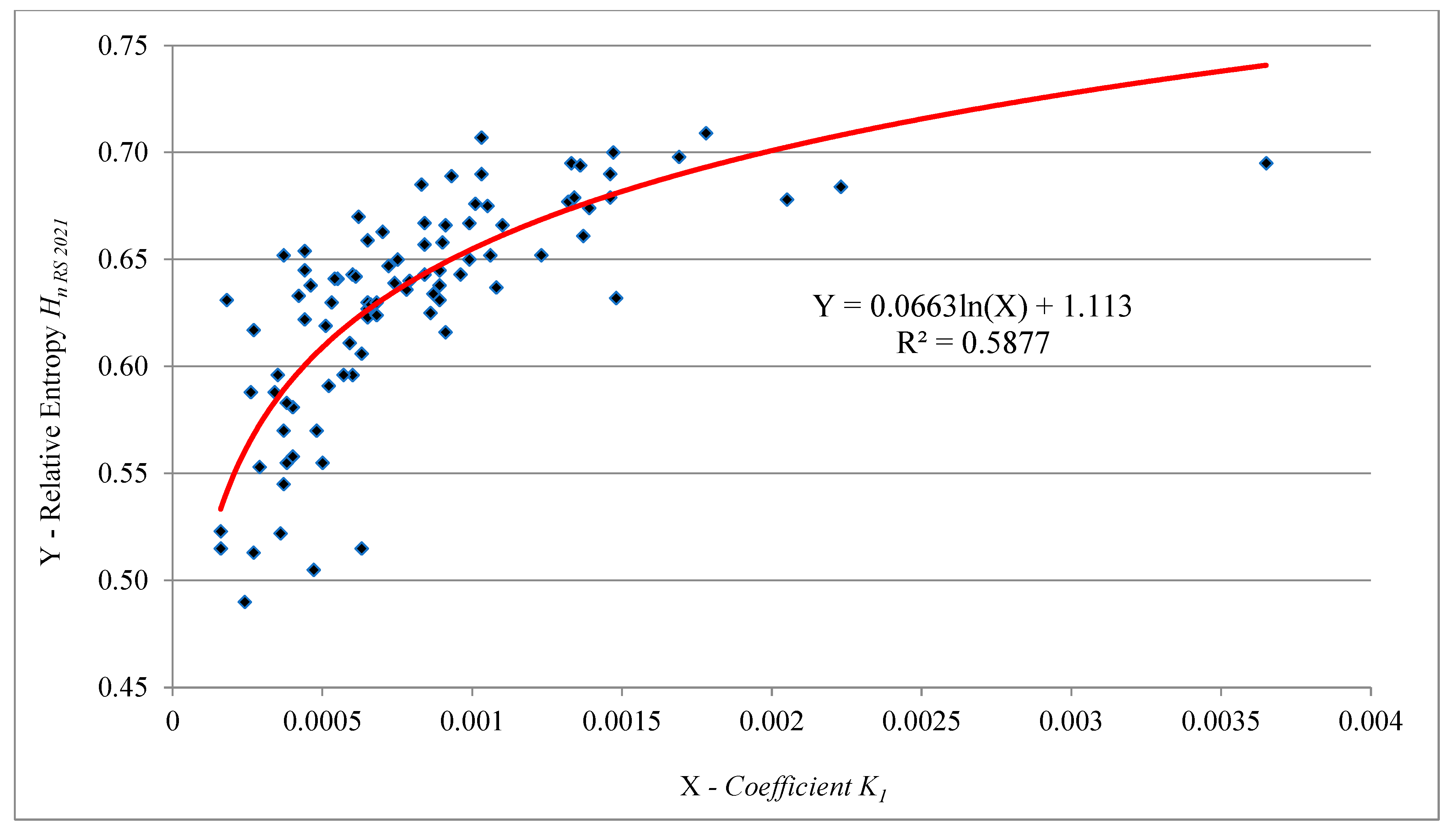
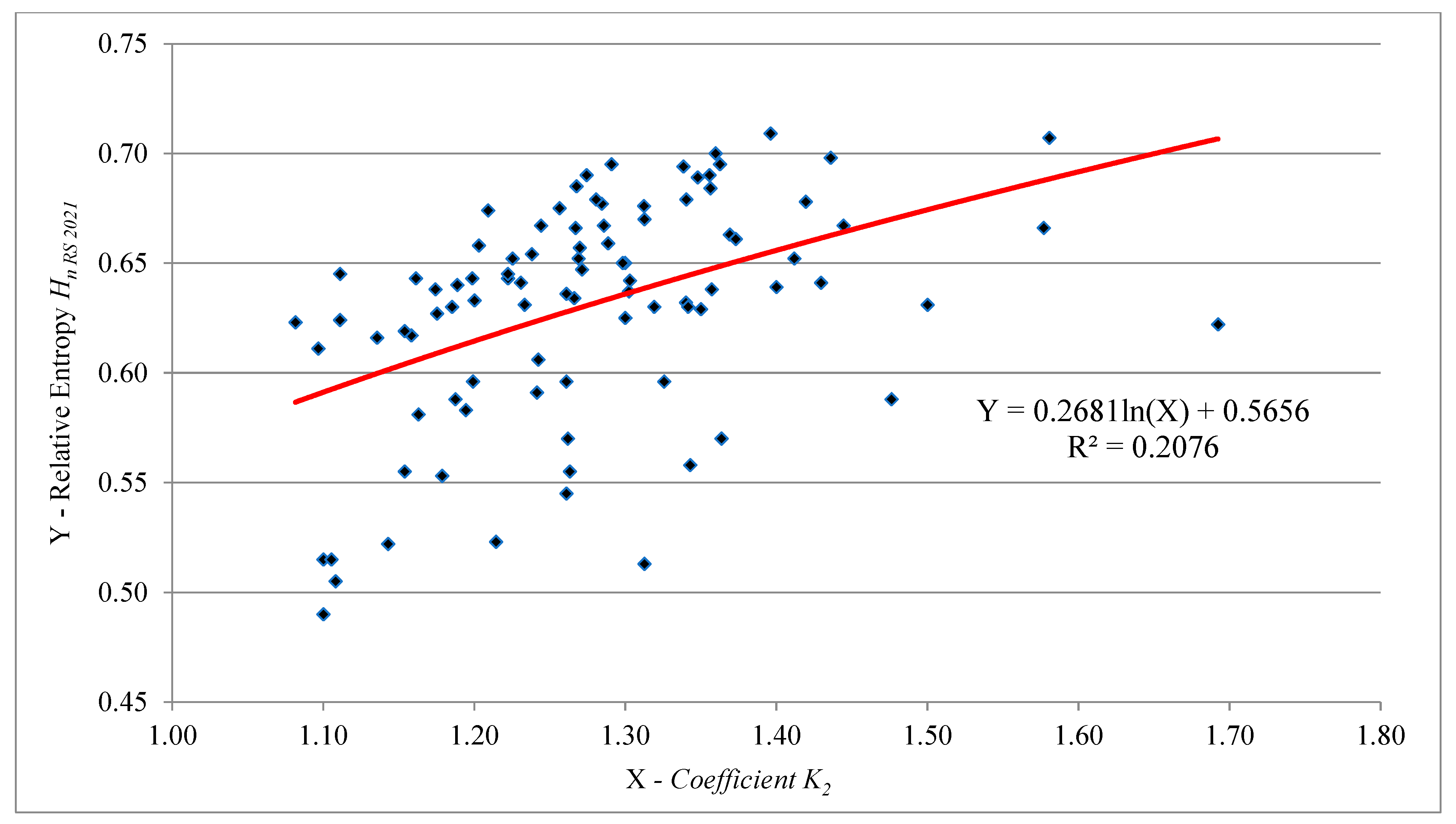
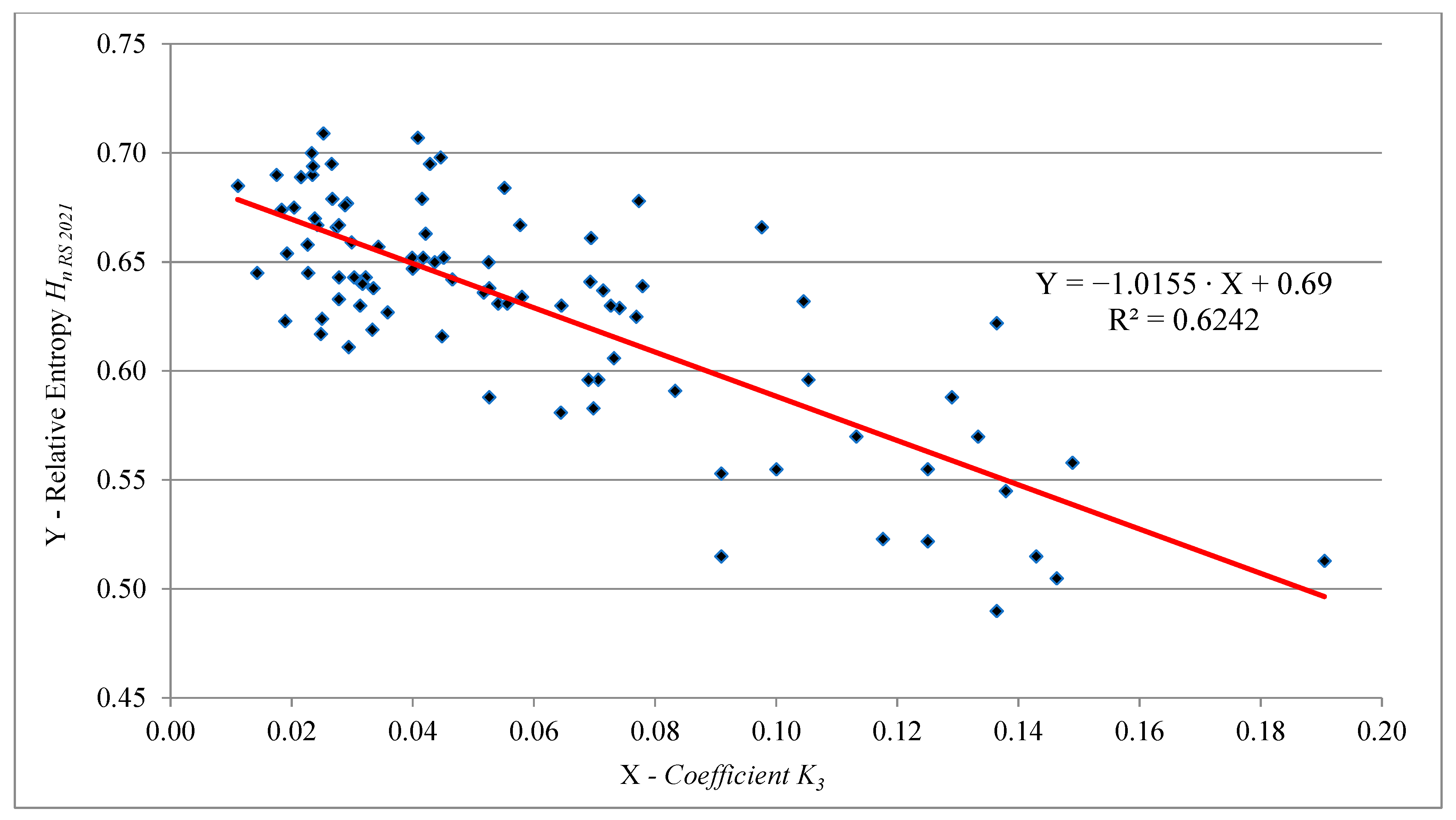


| Direction | Application of Entropy | The Authors Are Representatives of the Direction | Keywords |
|---|---|---|---|
| Analysis of ways of sustainable development | Search for ways of sustainable development based on the entropy approach | Avery J.S. [19] Halkos G., Kitsos Ch. [20] Devine S. [21] Bailey K.D. [22] Mayer, A.L. et al. [23] Tolstykh T. et al. [24] | Entropy sustainability |
| Socio-economic thermodynamics | Finding the state of economic equilibrium at the point of maximum thermodynamic entropy | Saslow W.M. [25] Vozna L.Y. [26] Antoniou I. et al. [27] Zheng F., Song Q. [28] | Entropy equilibrium |
| Socio-economic synergetics | Consideration of mechanisms and modes of entropy reduction in socio-economic systems | Prigogine I. [29] Ho M.W. [30] Jaynes E.T. [31] Raine A. et al. [32] | Entropy synergy |
| Information-entropy theory of value | The use of information entropy to justify the economic category of value and price formation | Chen J. [33] Yang J. [34] Roríguez R.A. et al. [35] | Entropy information; value or price |
| Entropy of social behavior | The use of information entropy to model the behavior of agents | Anttila J., Annila A. [36] Caticha A., Golan A. [37] Cabrales A. et al. [38] Xu B. et al. [39] Smith J. [40] Foley D.K. [41] Scharfenaker E. et al. [42] | Entropy behavior |
| Entropy in econometrics | The use of entropy to improve the quality of econometric methods | Judge G. [43,44] Duan Y. et al. [45] Jakimowicz A. [46] | Entropy econometrics |
| Links in the Cause-Effect Chain of Road Traffic Accident Rate Formation | ||
|---|---|---|
| Link “Population P— Road Traffic Accidents NRA” | Link “Road Traffic Accidents NRA— RTA Victims NV” | Link “RTA Victims NV— Fatality Cases ND” |
| Information transfer coefficients Ki | ||
| K1 = KRA | K2 = KV | K3 = KD |
| Indicator | Population, Thous. of People | ||
|---|---|---|---|
| 1500…13000 | 500…1000 | 50…100 | |
| Classification category of the city | Category A | Category B | Category C |
| Number of cities in the study Ncities, unit | 4 | 23 | 65 |
| Human Risk HR, deaths in RTA/100 thous. people | [2.75–5.08] | [0.79–6.75] | [1.06–22.52] |
| RTA Severity SevRTA, % of the dead from the number of victims | [3.35–7.06] | [1.75–6.93] | [1.11–19.05] |
| Summary data is presented in Appendix A | Table A1 | Table A2 | Table A3 |
| Range of Values of the Relative Entropy Hn-3 RS | ||||||
|---|---|---|---|---|---|---|
| ≤0.600 | 0.601…0.620 | 0.621…0.640 | 0.641…0.660 | 0.661…0.680 | 0.681…0.700 | ≥0.701 |
| Distribution of the number of urban RS systems by value ranges Hn-3 RS, unit; % | ||||||
| 1 | 1 | 1 | 7 | 7 | 5 | 1 |
| 4.3% | 4.3% | 4.3% | 30.4% | 30.4% | 21.7% | 4.3% |
| Range of Values of the Relative Entropy Hn-3 RS | ||||||
|---|---|---|---|---|---|---|
| ≤0.600 | 0.601…0.620 | 0.621…0.640 | 0.641…0.660 | 0.661…0.680 | 0.681…0.700 | ≥0.701 |
| Distribution of the number of urban RS systems by value ranges Hn-3 RS, unit; % | ||||||
| 20 | 4 | 16 | 10 | 9 | 5 | 1 |
| 30.8% | 6.2% | 24.6% | 15.4% | 13.8% | 7.7% | 1.5% |
| No of Rank in the Group | City | Human Risk HR, Deaths in RTA/ 100 Thous. People | RTA Severity, % | Hn-3 RS | Values (2021) of Information Transmission Coefficients Ki | ||
|---|---|---|---|---|---|---|---|
| K1 = KRA | K2 = KV | K3 = KD | |||||
| Largest city (1500 thous. people < Population < 13,000 thous. people) | |||||||
| 1—leader | Ekaterinburg | 4.27 | 4.36 | 0.650 | 0.00075 | 1.2998 | 0.0436 |
| 4—outsider | Novosibirsk | 5.08 | 7.06 | 0.596 | 0.00060 | 1.1990 | 0.0706 |
| Large city (500 thous. people < Population < 1000 thous. people) | |||||||
| 1—leader | Tyumen | 6.24 | 2.52 | 0.709 | 0.00178 | 1.3960 | 0.0252 |
| 23—outsider | Tomsk | 2.99 | 6.44 | 0.581 | 0.00040 | 1.1630 | 0.0644 |
| Small city (50 thous. people < Population < 100 thous. people) | |||||||
| 1—leader | Berezovsky | 6.63 | 4.08 | 0.707 | 0.00103 | 1.5806 | 0.0408 |
| 65—outsider | Ivanteevka | 3.62 | 13.64 | 0.490 | 0.00024 | 1.1000 | 0.1364 |
| Cities | Ki leader/Ki outsider for | ||
|---|---|---|---|
| K1 = KRA | K2 = KV | K3 = KD | |
| Category A | 1.25 | 1.08 | 0.61 |
| Category B | 4.45 | 1.20 | 0.39 |
| Category C | 4.29 | 1.44 | 0.30 |
| NoNo | City | Population, (P) | Road Traffic Accidents (NRA) | RTA Victims (NV) | Fataluty Cases (ND) | Values (2021) | |||
|---|---|---|---|---|---|---|---|---|---|
| K1 = KRA | K2 = KV | K3 = KD | Hn-3 RS | ||||||
| 1 | Novosibirsk | 1,633,595 | 980 | 1175 | 83 | 0.00060 | 1.1990 | 0.0706 | 0.596 |
| 2 | Tyumen | 816,800 | 1452 | 2027 | 51 | 0.00178 | 1.3960 | 0.0252 | 0.709 |
| Comparison for Ki Tyumen/Ki Novosibirsk | 2.97 | 1.16 | 0.36 | 1.19 | |||||
| NoNo | City | Population (P), People | Area of City, km2 | Road Traffic Accidents or RTA (NRA), Units | RTA Victims (NV), Units | Fataluty Cases (ND), Units | Values (2021) | ||
|---|---|---|---|---|---|---|---|---|---|
| Number of RTA/ 1000 People;/ Number of RTA/km2 | Number of Victims in RTA/1000 People;/ The Number of Victims in a RTA/km2. | Number of Deaths in RTA/1000 People;/ Number of Deaths in a RTA/km2. | |||||||
| 1 | Novosibirsk | 1,633,595 | 502.7 | 980 | 1175 | 83 | 0.600 | 0.719 | 0.051 |
| 1.95 | 2.34 | 0.17 | |||||||
| 2 | Tyumen | 816,800 | 490.8 | 1452 | 2027 | 51 | 1.778 | 2.482 | 0.062 |
| 2.96 | 4.13 | 0.10 | |||||||
| City | Symptoms of Comparison of City Car Parks | ||
|---|---|---|---|
| Share of Right-Hand Drive Vehicles, % | Share of Cars of 4,5,6 Ecological Classes, % | Share of Cars More Than 10 Years Old, % | |
| Novosibirsk | 14.8 | 52.0 | 21.8 |
| Tyumen | 1.2 | 59.5 | 10.6 |
| Entropy Hn RS Level, Characteristic of the RS Assurance System | Perception of the Traffic Situation by Road Users/Driving Speed, km/h or m/s | Parameters of the Time Required for Braking in Danger Mode | Stopping Way in Full Braking Mode Sstopping way, m | |||
|---|---|---|---|---|---|---|
| Reaction Time of the Driver to the Danger T1, s | Brake Actuation Time T2, s; | Time to Reach Maximum Car Deceleration T3, s; | The Total Time T = (T1 + T2 + 0.5∙T3), s; | |||
| High (0.68–0.72)/ Representative City—Tyumen | Lack of anxiety about waiting for danger/ 72 km/h or 20 m/s | 1.0–1.2 | 0.1–0.2 | 0.1–0.2 | 1.2–1.6 (≈1.4) | 20.0 ∙ 1.4 + 20.02 m/s/[2 ∙ 6.5 m/s2] ≈ 58.7 |
| Low (0.56–0.60)/ Representative City—Novosibirsk | High anxiety about waiting for danger/ 50 km/h or 13.9 m/s | 0.6–0.8 | 0.1–0.2 | 0.1–0.2 | 0.8–1.2 (≈1.0) | 13.9 ∙ 1.0 + 13.92 m/s/[2 ∙ 6.5 m/s2] ≈ 28.8 |
| It is assumed in calculations that the deceleration j of a serviceable vehicle in full braking mode under dry pavement conditions (φ ≈ 0.65...0.70) is approximately equal to j = 6.5 m/s2. | ||||||
| NoNo Table A3 Appendix A | City | Population, (P) | Road Traffic Accidents (NRA) | RTA Victims (Nv) | Fataluty Cases (ND) | Human Risk HR, Deaths in RTA/100 Thous. People | RTA Severity SevRTA, % of the Dead from the Number of Victims | Hn-3 RS |
|---|---|---|---|---|---|---|---|---|
| 36 | Feodosia | 67,750 | 100 | 134 | 14 | 20.66 | 10.45 | 0.632 |
| 37 | Minusinsk | 67,234 | 60 | 74 | 4 | 5.95 | 5.41 | 0.631 |
| NoNo Table A4 Appendix A | City | Values (2021) | ||||
|---|---|---|---|---|---|---|
| HRS | Hn RS | K1 = KRA | K2 = KV | K3 = KD | ||
| 36 | Feodosia | 0.694 | 0.632 | 0.00148 | 1.3400 | 0.1045 |
| 37 | Minusinsk | 0.693 | 0.631 | 0.00089 | 1.2333 | 0.0541 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petrov, A.I. Philosophy and Meanings of the Information Entropy Analysis of Road Safety: Case Study of Russian Cities. Information 2023, 14, 302. https://doi.org/10.3390/info14060302
Petrov AI. Philosophy and Meanings of the Information Entropy Analysis of Road Safety: Case Study of Russian Cities. Information. 2023; 14(6):302. https://doi.org/10.3390/info14060302
Chicago/Turabian StylePetrov, Artur I. 2023. "Philosophy and Meanings of the Information Entropy Analysis of Road Safety: Case Study of Russian Cities" Information 14, no. 6: 302. https://doi.org/10.3390/info14060302
APA StylePetrov, A. I. (2023). Philosophy and Meanings of the Information Entropy Analysis of Road Safety: Case Study of Russian Cities. Information, 14(6), 302. https://doi.org/10.3390/info14060302






