Generalized Frame for Orthopair Fuzzy Sets: (m,n)-Fuzzy Sets and Their Applications to Multi-Criteria Decision-Making Methods
Abstract
1. Introduction
- (1)
- In Section 2, we survey orthopairs in the light of fuzzy computing with an illustrative example.
- (2)
- Section 3 introduces a novel class of generalized IFSs called -Fuzzy sets and displays a set of operations between -Fuzzy sets.
- (3)
- Section 4 initiates the weighted aggregated operators via -Fuzzy sets and scrutinizes their master features.
- (4)
- Section 5 is dedicated to investigate an MCDM method with respect to these operators. The followed technique to implement this method is demonstrated through an example.
- (5)
- Finally, we point out novel facets of the current paradigm and summarize some possible future contributions in Section 6.
2. Preliminaries
3. (m, n)-Fuzzy Sets
- IFS if.
- PFS if.
- FFS if.
- q-ROFS if.
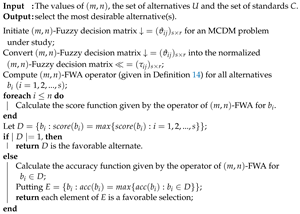
- -FS if and .
- Every IFS is an -FS.
- If and , then a PFS is an -FS.
- If and , then a FFS is an -FS.
- If and , then a q-ROFS is an -FS.
- If and , then a -FS is an -FS.
- .
- .
- .
- .
- .
- and.
- .
- .
- .
- .
- .
- Similar to 1 above.
- 1.
- .
- 2.
- .
- . And,. Then,Thus, and . Hence, .
- . And,. Then,Thus, and . Hence, .
- 1.
- .
- 2.
- .
- For the -FSs and , according to Definition 6, we obtain.
- Similar to 1.
- 1.
- .
- 2.
- .
- (i)
- If, then.
- (ii)
- If, then.
- (iii)
- If, then.
- (i)
- If, then.
- (ii)
- If, then.
- (iii)
- If, then
- 1.
- If, then.
- 2.
- If, then.
- 3.
- If, then.
4. Aggregation of -Fuzzy Sets with Applications
4.1. Some Operations on -FSs
- 1.
- .
- 2.
- .
- 3.
- .
- 4.
- .
- 1.
- .
- 2.
- .
- 3.
- .
- 4.
- .
- 1.
- .
- 2.
- .
- .
- .
- 1.
- for.
- 2.
- for.
- 3.
- for.
- 4.
- for.
- .And .
- .
- .
- .
- 1.
- .
- 2.
- .
- .And.
- Similar to 1.
- 1.
- .
- 2.
- .
- 3.
- .
- 4.
- .
- .
- .
- .
- .
- 1.
- .
- 2.
- .
- 3.
- .
- 4.
- .
- .And.Hence, .
- Similar to 1.
- .And.Hence, .
- Similar to 3.
- 1.
- .
- 2.
- .
- .
- Similar to 1.
4.2. Aggregation of -Fuzzy Sets
- 1.
- an-Fuzzy weighted average (-FWA) operator is given by-.
- 2.
- an-Fuzzy weighted geometric (-FWG) operator is given by-.
- 3.
- an-Fuzzy weighted power average (-FWPA) operator is given by-.
- 4.
- an-Fuzzy weighted power geometric (-FWPG) operator is given by-.
- 1.
- -.
- 2.
- -.
- 3.
- -.
- 4.
- -.
- 1.
- --.
- 2.
- --.
- 3.
- --.
- 4.
- --.
- 1.
- --.
- 2.
- --.
- 3.
- --.
- 4.
- --.
- 1.
- --.
- 2.
- --.
- 3.
- --.
- 4.
- --.
- 1.
- --.
- 2.
- --.
- 3.
- --.
- 4.
- --.
5. Decision-Making Approach Using -FSs and Their Aggregations
5.1. Representation and Model of MCDM Problems via the Frame of -FSs
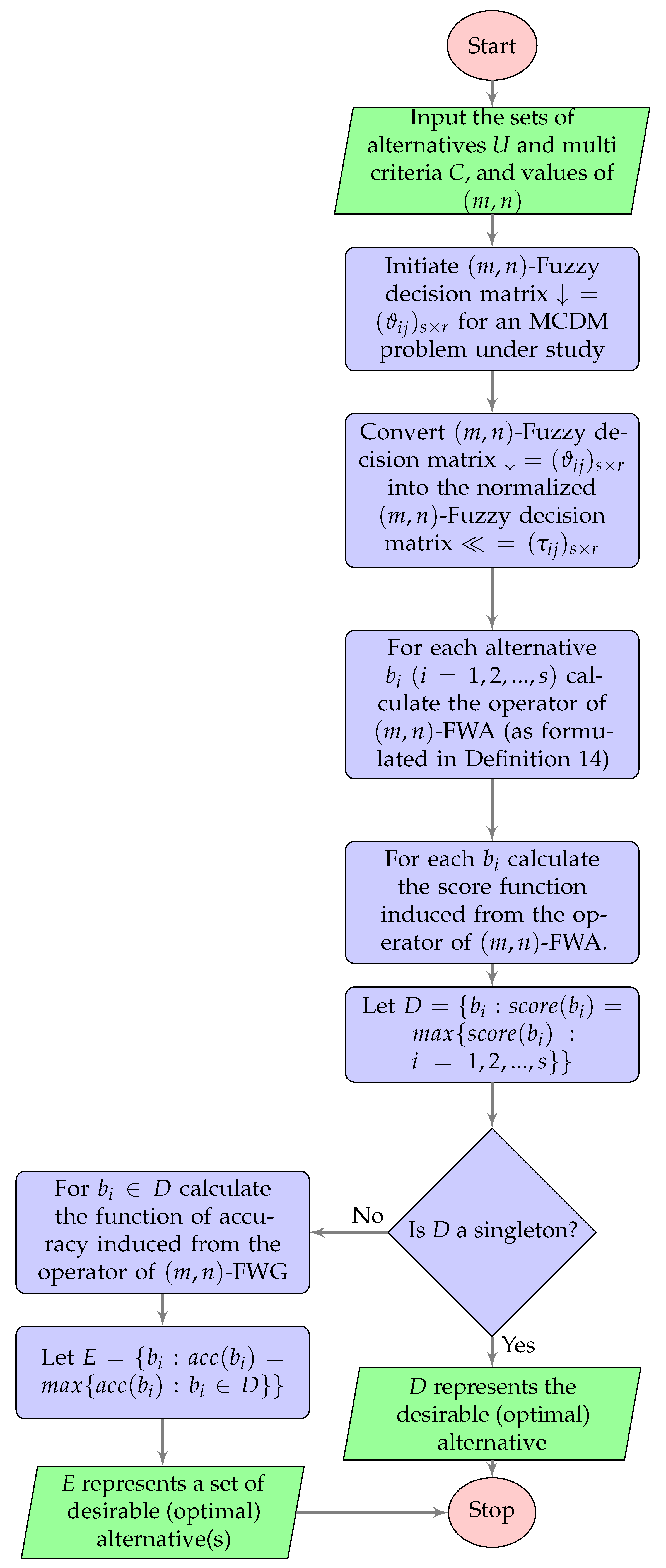
- Step 1:
- Determine the convenient values of m and n applied to handle this MCDM.
- Step 2:
- Initiate the -Fuzzy decision matrix for an MCDM problem under study.
- Step 3:
- Convert -Fuzzy decision matrix into the normalized -Fuzzy decision matrix . In this step, if there are different kinds of criteria, namely benefit X and cost Y then the rating values of X and Y can be transformed using the below normalization formula:
- Step 4:
- Evaluate the alternatives’ aggregations based on the normalized -Fuzzy decision matrix. In other words, compute all types of -Fuzzy weighted operators given in Definition 14 (i.e., -FWA, -FWG, -FWPA and -FWPG operators) for each alternative .
- Step 5:
- Calculate the functions of score and accuracy for each -FNs provided in Step 3. According Remark 2, the ordered values obtained from these operators need not be an -FS; however, we extend the formulas of scores and accuracy functions given in Definition 11 for those ordered values.
- Step 6:
- Compare the given alternatives based on the scores and accuracy.
- Step 7:
- If there are some alternatives that have the same scores, then compare them with respect to their accuracy.
- Step 8:
- By Definition 11, rank the alternatives and determine the favorable one(s).
| Algorithm 1: The optimal alternative(s) induced from the operator of -FWA. |
 |
5.2. Illustrative Examples
- (i)
- It cannot be handled the ordered pairs of data given in the illustrative example using IFSs because the sum of the degrees of non-membership and membership is greater than one.
- (ii)
- The company management proposed different importances for the degrees of membership and non-membership, which cannot be treated using the extensions of IFSs known in the published manuscripts.
6. Conclusions and Future Works
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Yager, R.R. Pythagorean membership grades in multicriteria decision making. IEEE Trans. Fuzzy Syst. 2013, 22, 958–965. [Google Scholar] [CrossRef]
- Senapati, T.; Yager, R.R. Fermatean fuzzy weighted averaging/geometric operators and its application in multi-criteria decision-making methods. Eng. Appl. Artif. Intell. 2019, 85, 112–121. [Google Scholar] [CrossRef]
- Kirişci, M.; Demir, I.; Simsek, N. Fermatean fuzzy ELECTRE multi-criteria group decision-making and most suitable biomedical material selection. Artif. Intell. Med. 2022, 127, 102278. [Google Scholar] [CrossRef] [PubMed]
- Yager, R.R. Generalized orthopair fuzzy sets. IEEE Trans. Fuzzy Syst. 2017, 25, 1222–1230. [Google Scholar] [CrossRef]
- Ibrahim, H.Z.; Al-shami, T.M.; Elbarbary, O.G. (3,2)-Fuzzy sets and their applications to topology and optimal choices. Comput. Intell. Neurosci. 2021, 2021, 1272266. [Google Scholar] [CrossRef]
- Al-shami, T.M. (2,1)-Fuzzy sets: Properties, weighted aggregated operators and their applications to multi-criteria decision-making methods. Complex Intell. Syst. 2022, 1–19. [Google Scholar] [CrossRef]
- Al-shami, T.M.; Ibrahim, H.Z.; Azzam, A.A.; L-Maghrabi, A.I.E. SR-fuzzy sets and their applications to weighted aggregated operators in decision-making. J. Funct. Spaces 2022, 2022, 3653225. [Google Scholar] [CrossRef]
- Gao, S.; Zhang, X. Linear orthopair fuzzy sets. Int. J. Fuzzy Syst. 2022, 24, 1814–1838. [Google Scholar] [CrossRef]
- Xu, Z. Intuitionistic fuzzy aggregation operators. IEEE Trans. Fuzzy Syst. 2007, 15, 1179–1187. [Google Scholar]
- Xu, Z.; Yager, R.R. Some geometric aggregation operators based on intuitionistic fuzzy sets. Int. J. Gen. Syst. 2006, 35, 417–433. [Google Scholar] [CrossRef]
- Khan, A.A.; Ashraf, S.; Abdullah, S.; Qiyas, M.; Luo, J.; Khan, S.U. Pythagorean fuzzy dombi aggregation operators and their application in decision support system. Symmetry 2019, 11, 383. [Google Scholar] [CrossRef]
- Peng, X.; Yuan, H. Fundamental properties of Pythagorean fuzzy aggregation operators. Fundam. Informaticae 2016, 147, 415–446. [Google Scholar] [CrossRef]
- Jana, C.; Garg, H.; Pal, M. Multi-attribute decision making for power Dombi operators under Pythagorean fuzzy information with MABAC method. J. Ambient. Intell. Human Comput. 2022, 1–8. [Google Scholar] [CrossRef]
- Peng, X.; Yang, Y.; Song, J.; Jiang, Y. Pythagorean fuzzy soft set and its application. Comput. Eng. 2015, 41, 224–229. [Google Scholar]
- Rahman, K.; Abdullah, S.; Jamil, M.; Khan, M.Y. Some generalized intuitionistic fuzzy Einstein hybrid aggregation operators and their application to multiple attribute group decision making. Int. J. Fuzzy Syst. 2018, 20, 1567–1575. [Google Scholar] [CrossRef]
- Shahzadi, G.; Akram, M.; Al-Kenani, A. Decision-making approach under pythagorean fuzzy yager weighted operators. Mathematics 2020, 8, 70. [Google Scholar] [CrossRef]
- Yager, R.R.; Abbasov, A.M. Pythagorean membership grades, complex numbers and decision making. Int. J. Intell. Syst. 2013, 28, 436–452. [Google Scholar] [CrossRef]
- Jana, C.; Pal, M.; Liu, P. Multiple attribute dynamic decision making method based on some complex aggregation functions in CQROF setting. Comp. Appl. Math. 2022, 41, 103. [Google Scholar] [CrossRef]
- Maji, P.K.; Biswas, R.; Roy, A.R. Fuzzy soft sets. J. Fuzzy Math. 2001, 9, 589–602. [Google Scholar]
- Ahmad, B.; Kharal, A. On Fuzzy Soft Sets. Adv. Fuzzy Syst. 2009, 2009, 586507. [Google Scholar] [CrossRef]
- Alcantud, J.C.R.; Varela, G.; Santos-Buitrago, B.; Santos-García, G.; Jiménez, M.F. Analysis of survival for lung cancer resections cases with fuzzy and soft set theory in surgical decision making. PLoS ONE 2019, 14, e0218283. [Google Scholar] [CrossRef] [PubMed]
- Cağman, N.; Enginoxgxlu, S.; Çitak, F. Fuzzy soft set theory and its application. Iran. J. Fuzzy Syst. 2011, 8, 137–147. [Google Scholar]
- Xu, Y.-J.; Sun, Y.-K.; Li, D.-F. Intuitionistic Fuzzy Soft Set. In Proceedings of the 2010 2nd International Workshop on Intelligent Systems and Applications, Wuhan, China, 22–23 May 2010; pp. 1–4. [Google Scholar] [CrossRef]
- Sivadas, A.; John, S.J. Fermatean Fuzzy Soft Sets and Its Applications. In Computational Sciences—Modelling, Computing and Soft Computing; CSMCS 2020. Communications in Computer and Information Science; Awasthi, A., John, S.J., Panda, S., Eds.; Springer: Singapore, 2021; Volume 1345. [Google Scholar]
- Hamida, M.T.; Riaz, M.; Afzal, D. Novel MCGDM with q-rung orthopair fuzzy soft sets and TOPSIS approach under q-Rung orthopair fuzzy soft topology. J. Intell. Fuzzy Syst. 2020, 39, 3853–3871. [Google Scholar] [CrossRef]
- Al-shami, T.M.; Alcantud, J.C.R.; Mhemdi, A. New generalization of fuzzy soft sets: (a,b)-Fuzzy soft sets. AIMS Math. 2023, 8, 2995–3025. [Google Scholar] [CrossRef]
- Atef, M.; Ali, M.I.; Al-shami, T.M. Fuzzy soft covering-based multi-granulation fuzzy rough sets and their applications. Comput. Appl. Math. 2021, 40, 115. [Google Scholar] [CrossRef]
- Jan, N.; Mahmood, T.; Zedam, L.; Ali, Z. Multi-valued picture fuzzy soft sets and their applications in group decision-making problems. Soft Comput. 2020, 24, 18857–18879. [Google Scholar] [CrossRef]
- Kirişci, M.; Simsek, N. Decision making method related to pythagorean fuzzy soft sets with infectious diseases application. J. King Saud-Univ.-Comput. Inf. Sci. 2022, 34, 5968–5978. [Google Scholar] [CrossRef]
- Yang, B. Fuzzy covering-based rough set on two different universes and its application. Artif. Intell. Rev. 2022, 55, 4717–4753. [Google Scholar] [CrossRef]
- Yang, B.; Hu, B.Q. Communication between fuzzy information systems using fuzzy covering-based rough sets. Int. J. Approx. Reason. 2018, 103, 414–436. [Google Scholar] [CrossRef]
- Ameen, Z.A.; Al-shami, T.M.; Azzam, A.A.; Mhemdi, A. A novel fuzzy structure: Infra-fuzzy topological spaces. J. Funct. Spaces 2022, 2022, 9778069. [Google Scholar] [CrossRef]
- Gulzar, M.; Alghazzawi, D.; Mateen, M.H.; Kausar, N. A Certain Class of t-Intuitionistic Fuzzy Subgroups. IEEE Access 2020, 8, 163260–163268. [Google Scholar] [CrossRef]
- Saleh, S.; Abu-Gdairi, R.; Al-shami, T.M.; Abdo, M.S. On categorical property of fuzzy soft topological spaces. Appl. Math. Inf. Sci. 2022, 16, 635–641. [Google Scholar]
- Senapati, T.; Yager, R.R. Fermatean fuzzy sets. J. Ambient. Intell. Humaniz. Comput. 2020, 11, 663–674. [Google Scholar] [CrossRef]
| Companies | ||||
|---|---|---|---|---|
| food company | (0.8, 0.3) | (0.4, 0.4) | (0.5, 0.7) | (0.2, 0.6) |
| clothes company | (0.9, 0.4) | (0.4, 0.8) | (0.6, 0.7) | (0.4, 0.7) |
| computer company | (0.6, 0.5) | (0.5, 0.7) | (0.5, 0.7) | (0.50193, 0.6) |
| car company | (0.7, 0.6) | (0.2, 0.9) | (0.4, 0.6) | (0.9, 0.1) |
| Food Company | Clothes Company | Computer Company | Car Company | |
|---|---|---|---|---|
| - | (0.49, 0.49) | (0.56, 0.68) | (0.520193, 0.65) | (0.43, 0.67) |
| 0.372351 | 0.245568 | 0.245568 | 0.129237 | |
| 0.607649 | 0.874432 | 0.794818 | 0.730763 | |
| - | (0.458391, 0.465150) | (0.531280, 0.660218) | (0.518768, 0.644433) | (0.367683, 0.589892) |
| 0.357749 | 0.243499 | 0.251139 | 0.162417 | |
| - | (0.49, 0.1555) | (0.56, 0.3548) | (0.520193, 0.2867) | (0.43, 0.3997) |
| 0.48624 | 0.515337 | 0.496627 | 0.366144 | |
| - | (0.591436, 0.550289) | (0.628727, 0.720414) | (0.478361, 0.663311) | (0.489898, 0.780151) |
| 0.424799 | 0.254834 | 0.186516 | −0.015070 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-shami, T.M.; Mhemdi, A. Generalized Frame for Orthopair Fuzzy Sets: (m,n)-Fuzzy Sets and Their Applications to Multi-Criteria Decision-Making Methods. Information 2023, 14, 56. https://doi.org/10.3390/info14010056
Al-shami TM, Mhemdi A. Generalized Frame for Orthopair Fuzzy Sets: (m,n)-Fuzzy Sets and Their Applications to Multi-Criteria Decision-Making Methods. Information. 2023; 14(1):56. https://doi.org/10.3390/info14010056
Chicago/Turabian StyleAl-shami, Tareq M., and Abdelwaheb Mhemdi. 2023. "Generalized Frame for Orthopair Fuzzy Sets: (m,n)-Fuzzy Sets and Their Applications to Multi-Criteria Decision-Making Methods" Information 14, no. 1: 56. https://doi.org/10.3390/info14010056
APA StyleAl-shami, T. M., & Mhemdi, A. (2023). Generalized Frame for Orthopair Fuzzy Sets: (m,n)-Fuzzy Sets and Their Applications to Multi-Criteria Decision-Making Methods. Information, 14(1), 56. https://doi.org/10.3390/info14010056








