1. Introduction
Games predate human culture [
1]. With the emergence of games, human culture gradually began to take shape. On one level, games reflect different aspects of human cultures. Humans create games and, at the same time, learn from games. Many research efforts have been directed towards investigating reasons for the game’s excitement, from rules alteration to game setting. With the development of digital game technology, various games gradually formed, which are getting more sophisticated in either the types or rules, attracting more players, and initiating new research interests. Most games tend to go towards the sophistication direction that harmoniously address fairness, entertainment, and challenge [
2]. Various types of fun games and serious games have been explored from different aspects, which bring us closer to the nature of real entertainment perceived in mind. Nevertheless, it is still unclear how the engagement of a game would affect the multi-player game in terms of the number of players, playing hands, and cooperation mode.
Confrontational games are focusing on a single specialized player (Go, Chess, Mahjong, Olympic track and field events), and also cooperation games that need teamwork (Bridge, Diablo 3, Olympic games except for track and field events). It has been known that creating confrontation is the fundamental means of a game, whether it is between players or between players and rules, where the goal is to give users timely feedback on wins and losses [
3]. Game theory explains how and why cooperation emerged [
4]. An essential condition for cooperation is that both sides will reciprocate, where cooperation can happen when both are equally profitable. However, how cooperation would affect a game is not well understand. Games could simulate reality. Hence, it is interesting to observe cooperation in games when taking game benefits, such as engagement, into consideration.
Card games have a long history of their form being easy to simulate, and the rules are relatively simple, while it is capable of explaining the sophistication of the game structure. Moreover, card games are popular with all ages. In this study, we consider using a shedding-type card game, called DouDiZhu, in which the primary purpose is to empty one’s hand of all cards before all other players (
https://en.wikipedia.org/wiki/List_of_shedding-type_games). DouDiZhu is used as the benchmark to explore how settings changing in multi-player games would affect the engagement of players. Among all the settings, we further studied the importance of cooperation in the gameplay.
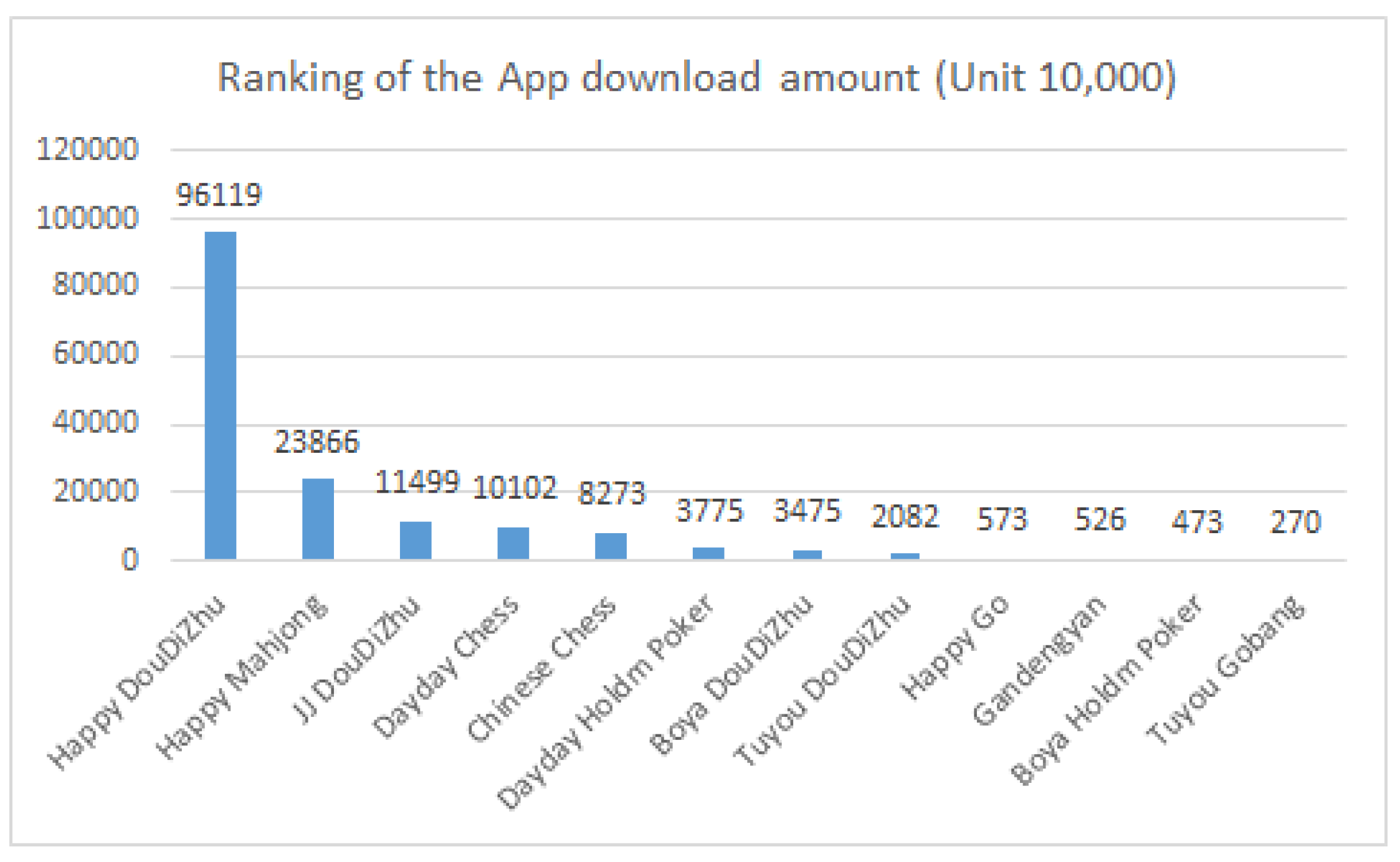
DouDiZhu [
5], one of the most popular game in China, also known as ‘Fighting Landlord’, ‘2 against 1’. It has a massive amount of users and generally regarded as the most sought-after card game in China (
Figure 1). The DouDiZhu’s mobile application was downloaded 1.13 billion times in 2017 alone (
http://youxiputao.com/articles/13003/). The classic DouDiZhu game involves three players, two of whom, called “the peasants”, need to cooperate against one another, called “the landlord”. The game is short, usually lasted around one to three minutes. This situation allowed people to play the game anytime and anywhere. The peasants win if any of their hands are played first; otherwise, the landlord wins. The profits or losses in the game are shared between the peasants while the landlord carries himself alone, which means the game is a zero-sum game satisfying the Nash Equilibrium. As in most card games, the starting hand of DouDiZhu can primarily affect the outcome of the game. The rules of DouDiZhu are not complicated; however, the two essential aspects of winning the game required strategies and skills.
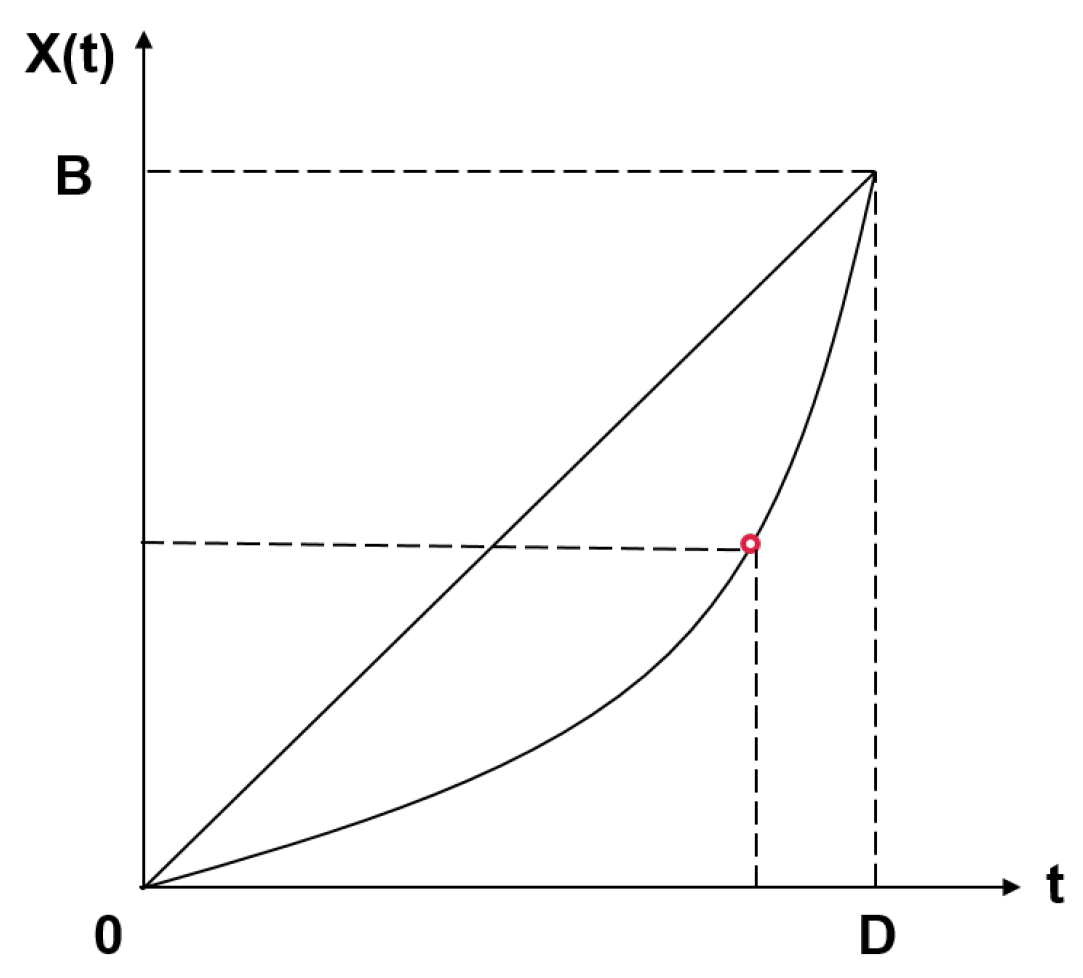
The conventional wisdom is that a good game should have both strategic challenges and player skills, but there should also be an element of chance (or rather, uncertainty). This study attempts to explore the optimal parameter setting of DouDiZhu, and analyze the game from the perspective of game progression through the game refinement theory [
6].
Analysis of the rules of DouDiZhu is conducted where its respective game length and branching factor is found. Then, a simulation experiment is conducted by changing the setting of the game in several variants of the game. The bidding session to be a landlord is also skipped for simplicity. After that, the optimal setting of DouDiZhu is adopted as the benchmark to consider game cooperation issues. Mainly, the investigation of this study is concerned with the following questions:
Can the DouDiZhu game be considered a good game? As an incomplete information dynamic card game, how well sophisticated is the game for different level players?
What would happen if the settings of DouDiZhu changes? Does the game have the most enjoyable settings already? How does cooperation affect engagement among these types of card games?
There are many card games similar to DouDiZhu with different hand settings. Moreover, there are also other well-refined card and board games in China. Cooperation is their most distinct missing feature. Is cooperation the essential factor that makes DouDiZhu game becoming so popular and exciting with only about 30 years’ development?
4. Simulation and Data Collection
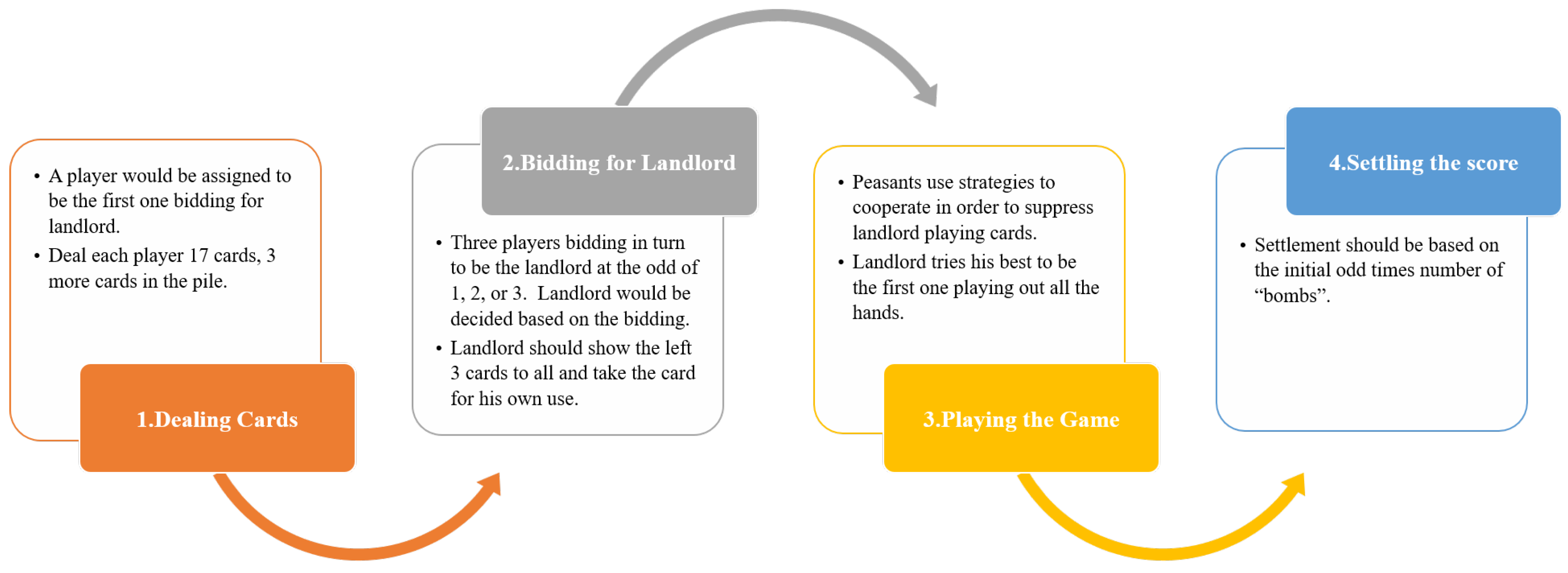
Usually, DouDiZhu is played in four stages, as shown in
Figure 3. The simulation experiment is conducted by utilizing a simulation program (namely as DouDiZhu AI) with a fixed strategy. Such a strategy is similar to the strategy used by the best human best player, which involves calculating the numerical setting of each card category [
29]. The parameter settings used in this study are the number of players (two types of players: landlord and peasants, where only one landlord is present, and peasant can be more than one) and their card distribution. The simulation was run 10,000 times for each of the game settings. The average possible options (
B) and the average game length (
D) were calculated, which then analyzed using the
measure.
The experiments were conducted as follows. Firstly, the simulation is first set up to accommodate different settings, annotations of the experiment, and strategy adoption, which considering three different DouDiZhu AI levels (weak, fair, and strong) to simulate the presence of cooperation among the players. Secondly, the simulation experiment is conducted on Classical DouDiZhu, which then compared with different DouDiZhu AI levels. Thirdly, another simulation experiment is conducted on known variants of the DouDiZhu in China to highlight the impact of the cooperation in the Classical DouDiZhu game.
4.1. Simulation Setups
In this study, no bidding phase is conducted where the landlord assignment is random. Fifty-four cards are split randomly among three players in the classical DouDiZhu, where the cards number is (20, 17, 17), where the one with 20 cards corresponds to the landlord, which is denoted as (L, , ). From one side, the landlord (L) is fighting on his/her own. On the other side, peasant 1 () and peasant 2 () cooperate against L. The game is won by the side that first plays out all the card hands.
There are two categories of gameplay, initiative play or passive play. After dealing with the cards, the landlord will play the first card or combination (initiative play). Alternatively, , can play a bigger-force card to follow the last player’s cards one by one (passive play). If they do not have bigger cards or decided to skip, it is called a pass. When pass is chosen by two players consecutively, the round will end. Then, the third player can initiate play with any card and begin the next round. For instance, play card in turns as , , , , , , , , which then will start a new round and initiate the card plays. The game ends when any one of the three players has played all the cards.
In this study, the game length (D) of DouDiZhu is the number of the total moves of the three players. Assuming the number of game players as n, number of landlord as l, number of the peasants as p, hands allocation will be shown as , a game setting will be denoted as . In the case of classical DouDiZhu, a setting denoted as . The average number of possible moves at each hand (B) is counted as follows:
When a player begins a game phase, the number of possible conventional combinations on the player’s hand is the estimation for the number of options. For example, ’s deck is “2221K999888633”, can play a card with the possible options of 3, 6, 8, 9, k, 1, 2. Thus, a total of seven cards; possible options can play two cards is 33 88 99 22, a total of four cards; and so on until the possible option can play ten cards is “8889992233”, this is just one card. Adding all the options together, , the total for .
When a player plays a phase passively, the number of choices is limited to the last played card of the same type. For example, plays “66633”, L’s remaining card is “22”, so L’s option is only to “pass”, which is just a single option; deck is “21kkkjj8876544”, can play “kkk44”, “kk88”, “jjj88”, “JJJKK”, and “pass”; thus, totaling to 7.
Notation: peasants need to cooperate, sometimes they will choose “pass” even when they have cards to play. Sometimes they do not want to split bigger-force combinations; thus, they may also “pass” anytime when they passively play cards.
The implementation of the DouDiZhu AI program involves dealing cards and playing cards during which cooperation between peasants should work as intelligent as possible. For functional convenience, different card categories are provided with a different numeric value according to the game rules (see further in [
30]).
The adopted optimal strategy for the simulation experiments is according to the strategy mentioned by the practical technique books of DouDiZhu [
19], which corresponds to the strong DouDiZhu AI level. For initiative play, the DouDiZhu AI will prioritize playing a sequence of planes, wings, lines, triples, pairs, and singletons. However, smaller cards are used when playing against an opponent or of the same class. Passive play is one of the alternative strategies that use smaller cards. The landlord is unscrupulous. At all costs, the peasant plays a bigger card than the landlord. Finally, when the card force is less than the lower bound, the first peasant plays more cards than the second peasant to ensure the success of both. The algorithm 1 describes the optimal core strategy.
| Algorithm 1 Optimal simulation strategy for DouDiZhu. |
- 1:
Initialize player size and card size - 2:
Initialize the team for each player , - 3:
Initialize initial card number for each player , and - 4:
Initialize each player ’s cards with card number - 5:
Initialize each player ’s policy - 6:
while TRUE do - 7:
for do - 8:
if player in passive state then - 9:
player play valid cards C from or play pass according to policy - 10:
end if - 11:
if player not in passive state then - 12:
player play valid cards C from according to policy - 13:
end if - 14:
if is empty then - 15:
Team win. End game. - 16:
end if - 17:
end for - 18:
end while
|
The simulation uses three levels of DouDiZhu AI: strong, fair and weak. The strong DouDiZhu AI always follows the above strategy. For the fair DouDiZhu AI, peasants cooperate, and peasants always choose for any card, while landlords can play as long as they have a larger card. For the weak DouDiZhu AI, all three players played bigger cards or passed at random.
4.2. Experiment on The Classical DouDiZhu
The classic Doudizhu is the most popular version in China, played by three players. Valid cards include a single card, pair card, straight card, triple card, double-wing card, plane card, bomb card, rocket card, and 300 cards, which can also be played by kickers (see
Table 2).
DouDiZhu game has a unique score system. The basic score is set as 3 points in this study, where the score is doubled when the player played a bomb or rocket (
https://en.wikipedia.org/wiki/Dou_dizhu). For example, the landlord obtains a total of 24 points while the peasant loses 12 points if there are three bombs, and the landlord wins the game. The conducted simulation considers a fair level of DouDiZhu AI, which corresponds to an average level of a human player (
Table 3).
In a real gameplay situation, however, emotion tends to overtake human players where changes in decision and strategies are expected in corresponds to their experiences and preferences; thus, producing an unexpected outcome. Hence, simulating such a situation is crucial through the consideration of the computer peasants’ cooperation. Additionally, DouDiZhu has millions of players which composed of novice to master level of players. In this simulation experiment, weak DouDiZhu AI represents a novice with little skills and intentions to cooperate, while fair AI represents an average human level with a weak cooperation strategy. A strong DouDiZhu AI stands for a specific kind of professional players with tournament-level performance.
Since cooperation and competition are important aspects of the game, the expected fairness of the game setting can be determined based on the average score for each set.
Table 4 provide different settings of the landlord and peasants with their respective possible scores. The scores are collected using DouDiZhu AI with the optimal strategy that prioritizes the best sense of cooperation. As a zero-sum game, the peasants’ score and the landlord’s score should be the same from the perspective of fairness.
The result indicates that setting is relatively fair based on their respective winning rate. However, observing the score setting of , it is perceived to be less fair for the peasants’ side, which implies that it is hard for the landlord to win equivalent scores. Interestingly, for and , while also perceived to be less fair to the peasant side, actually implies the opposite where one of the peasants tend to win at the cost of another. This setting implies that one of the peasants, while co-operating with another, played selfishly; thus, promoting less cooperation.
The simulation results performed with different settings through different DouDiZhu AI levels are given in
Table 5 which suggests that both weak DouDiZhu AI with little cooperation and fair DouDiZhu AI with lower-level cooperation show almost the same performance quality. However, when it comes to the strong DouDiZhu AI with optimal cooperation strategy,
value dropped radically.
Numbers of hands also affect the experience of a card game. The setting of is found to be the most sophisticated one for novice and average players, while the setting of is ideally exciting and challenging for professional players. It might reveal that for a typical case, we should play as the setting of ; however, in the tournament, it should adjust the hands to the more significant disparity.
Different levels of the players also affect the possible outcome and attractiveness of the game [
31]. By analyzing the performance of the classic game setting
, a possible score of different DouDiZhu AI levels was collected and summarized in
Table 6. While achieving a fairest winning ratio, weak DouDiZhu AI has no cooperation which implies they fought among themselves. Comparing the strong DouDiZhu AI with fair DouDiZhu AI, it could be observed that strong DouDiZhu AI with a high level of cooperation can also maintain balanced benefits of two sides (landlord versus peasants).
In multiplayer cooperative games, cooperative strategies can keep DouDiZhu relatively fair, which may have a significant impact on game complexity. When the cooperative strategy is prioritized, the
measurement drops, meaning that the game is challenging for most players. In other words, when considering a strong cooperative strategy, the game is less fun for the novice. Summarizing the results from
Table 5 and
Table 6, it can be conjectured that DouDiZhu is profoundly refined with both luck and skill under such mode of cooperation.
4.3. Comparison with the Variants of DouDiZhu Game in China
The rules of DouDiZhu has not changed much, although its development is about 30 years. Still, its popularity showed that if the game changes, it will do so for the better.
Table 7 provides the variants of the DouDiZhu game analyzed in this section.
When two players, initial hands will be 17–25 cards for both players such as (20,20); when three players, initial hands will be (18,18,18); when four players, get rid of two jokers, initial hands will be (13,13,13,13); more people will deal two decks of card in this way.
For two-player DouDiZhu, while the rules are similar to the classic DouDiZhu, one player plays as the peasant while the other plays as the landlord. At first, each player is randomly dealt with 17 hand cards. After the bidding, the landlord will have three additional cards. As such, a total of 17 cards will be unknown during the game. On the other hand, the rules for the four-player DouDiZhu are similar to the classic DouDiZhu but utilize two decks of cards. Instead of two versus one, DouDiZhu of four players are three versus one (a single player is the landlord while the remaining players are peasants). At first, each player is dealt with 25 hand cards. After the bidding, the landlord will have eight more cards.
The simulation experiment in this section utilizes similar rules as the classical DouDiZhu to simulate other versions of the DouDiZhu game considered for this study. The first simulations were conducted for the versions of two-players and four-players setting with fair level DouDiZhu AI. The simulation result is given in
Table 8.
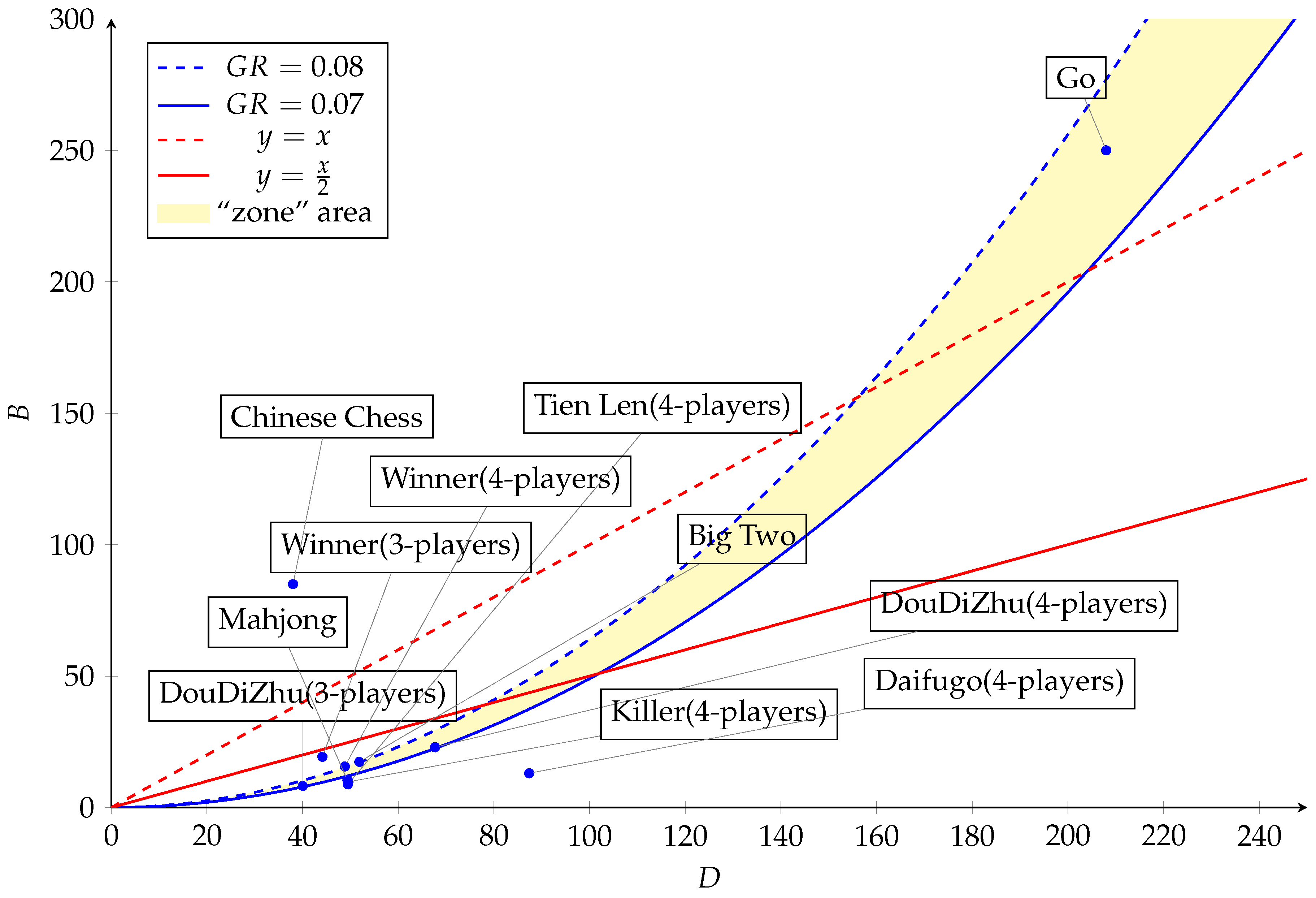
According to the
theory, a sophisticated game is a game that harmoniously balances challenge and skill as they changed over time [
6]. Since the sophisticated zone of game refinement value for most popular games has been verified to be
, it was found that the
measure is different in the traditional settings. The data might imply that the two-player DouDiZhu game (
= 0.142) is more likely based on chance, while the four-player DouDiZhu (
= 0.0649) with too many cards to play is complicated, more likely boring.
The two-player DouDiZhu is too easy to finish, making its value higher than the sophistication zone. The four-player DouDiZhu, on the other hand, is not equivalently fair for every player, and too challenging for the landlord to compete and for peasants to cooperate; thus, the value is lower than the GR zone. This distinction of the player number probably offered a numerical interpretation of the popularity of the classic DouDiZhu game in China (three-player setting).
Table 9 provides the possible scores of landlord and peasants in different settings. The scores are collected using DouDiZhu AI with the optimal strategy that prioritizes the best sense of cooperation. As a zero-sum game, the peasants’ score and the landlord’s score should be the same from the perspective of fairness.
Table 9 indicates that the settings
is relatively fair based on their respective winning rate. However, it can be observed from the score that the settings
and
are perceived to be less fair for the landlord side, which implies that it is hard for the landlord to achieve equivalent scores. These findings further justify that the four-players setting sacrifices both the expected enjoyment and fairness of the game.
The simulation results performed with different settings through different DouDiZhu AI levels are given in
Table 10. Similar to the experiment for the classical DouDiZhu,
Table 10 implies that both weak DouDiZhu AI with little cooperation had almost similar performance quality to the fair DouDiZhu AI with lower-level cooperation (except for strong DouDiZhu AI). Additional insights also can be observed from the distribution of the cards between the landlord and the peasants. Increases in the number of cards the landlord have, the lower the
value. This situation implies that the game is more challenging since the landlord has more hands at the start of the game. With more cards on hand, the landlord has to deal with more combinations and strategies (high branching factors). Peasants also have to deal with higher risks that the landlord might have a higher chance of getting high-value card categories. Such a situation demands the landlord to play out all the cards skillfully. Thus, more cards are nonequivalent to being advantageous in the DouDiZhu game.
The most popular activity in this game is the “pass”. Peasants can choose “pass” even when they have bigger cards to play to let their teammates win. Hence, based on the simulation strategy, the frequency of “pass” in a game could be taken as a parameter to estimate the cooperation between peasants.
Table 11 shows that peasants with weak DouDiZhu AI represent human novice, with no intention of cooperating, passes less than a fair DouDiZhu AI representing average players with a weak level of cooperation skills. In other words, after the novices get some sense of cooperation, they begin to reserve cards and choose to deliberately “pass” to let more cards to be played by their teammate. It can be inferred that the existence of cooperation increases the engagement of the game. By having an awareness of cooperation in playing this game, they become more cautious at every step.