Quadratic Frequency Modulation Signals Parameter Estimation Based on Product High Order Ambiguity Function-Modified Integrated Cubic Phase Function
Abstract
1. Introduction
2. Brief Review of HAF-ICPF
2.1. Quadratic Frequency Modulation Signal Model
2.2. The Principle of HAF-ICPF
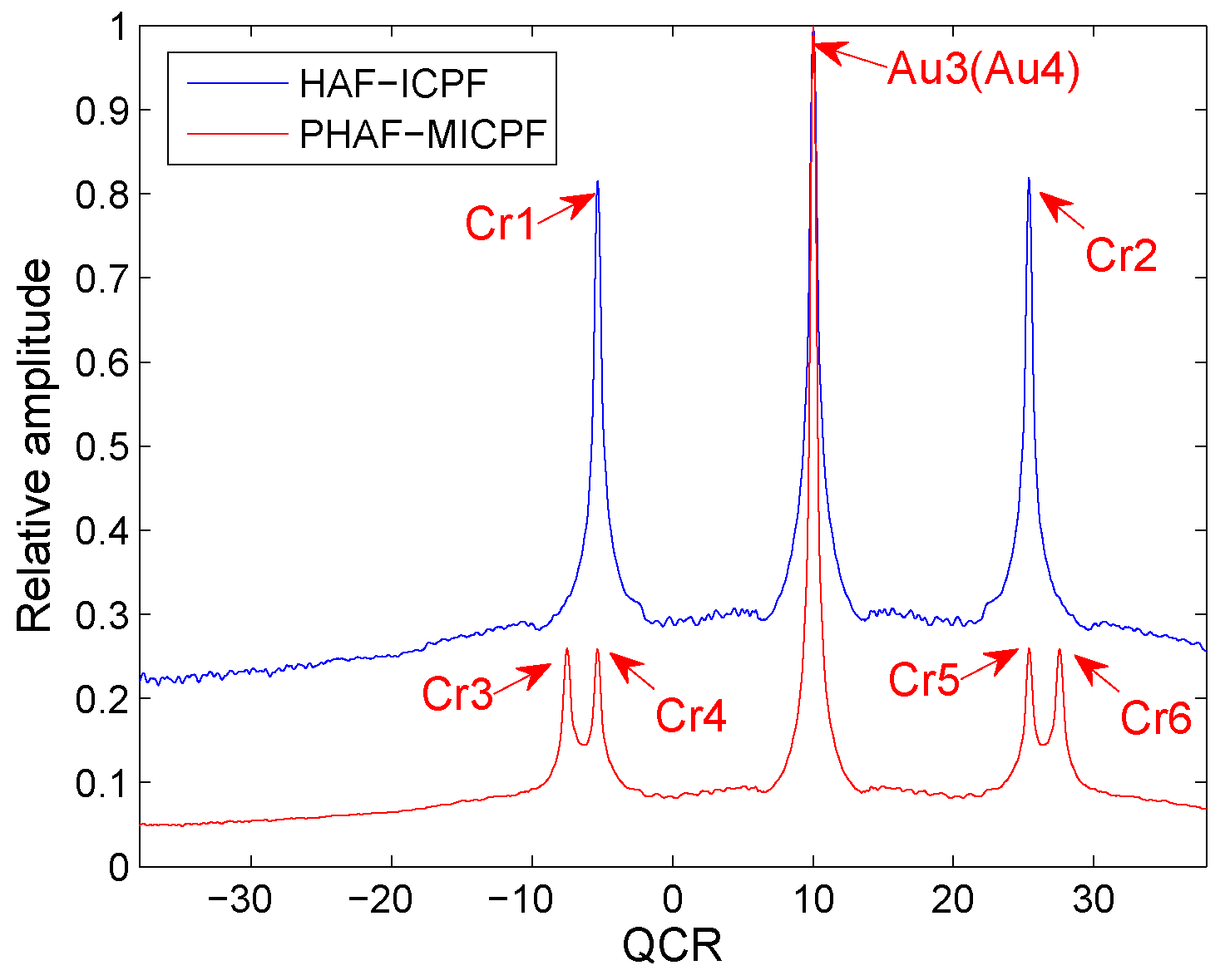
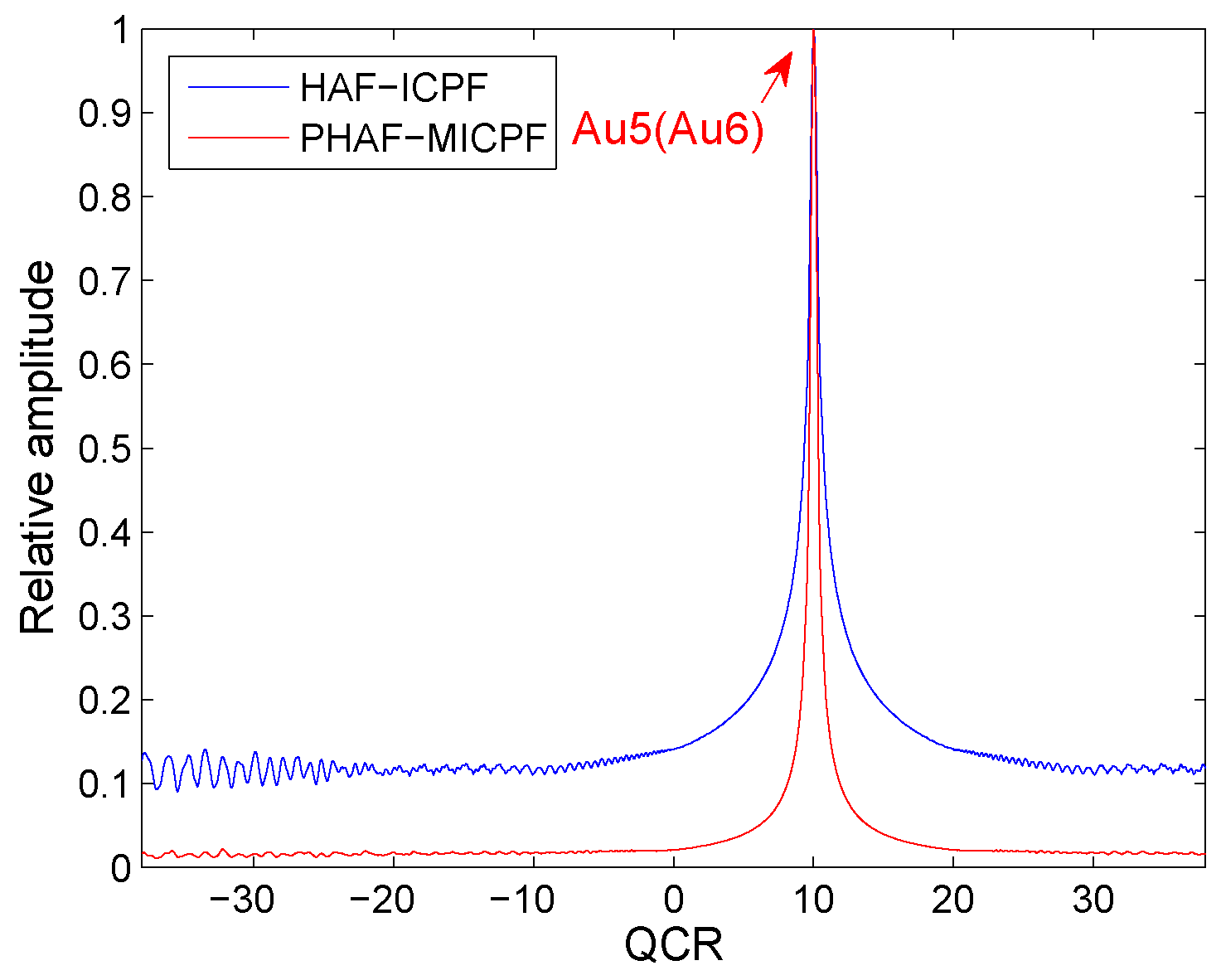
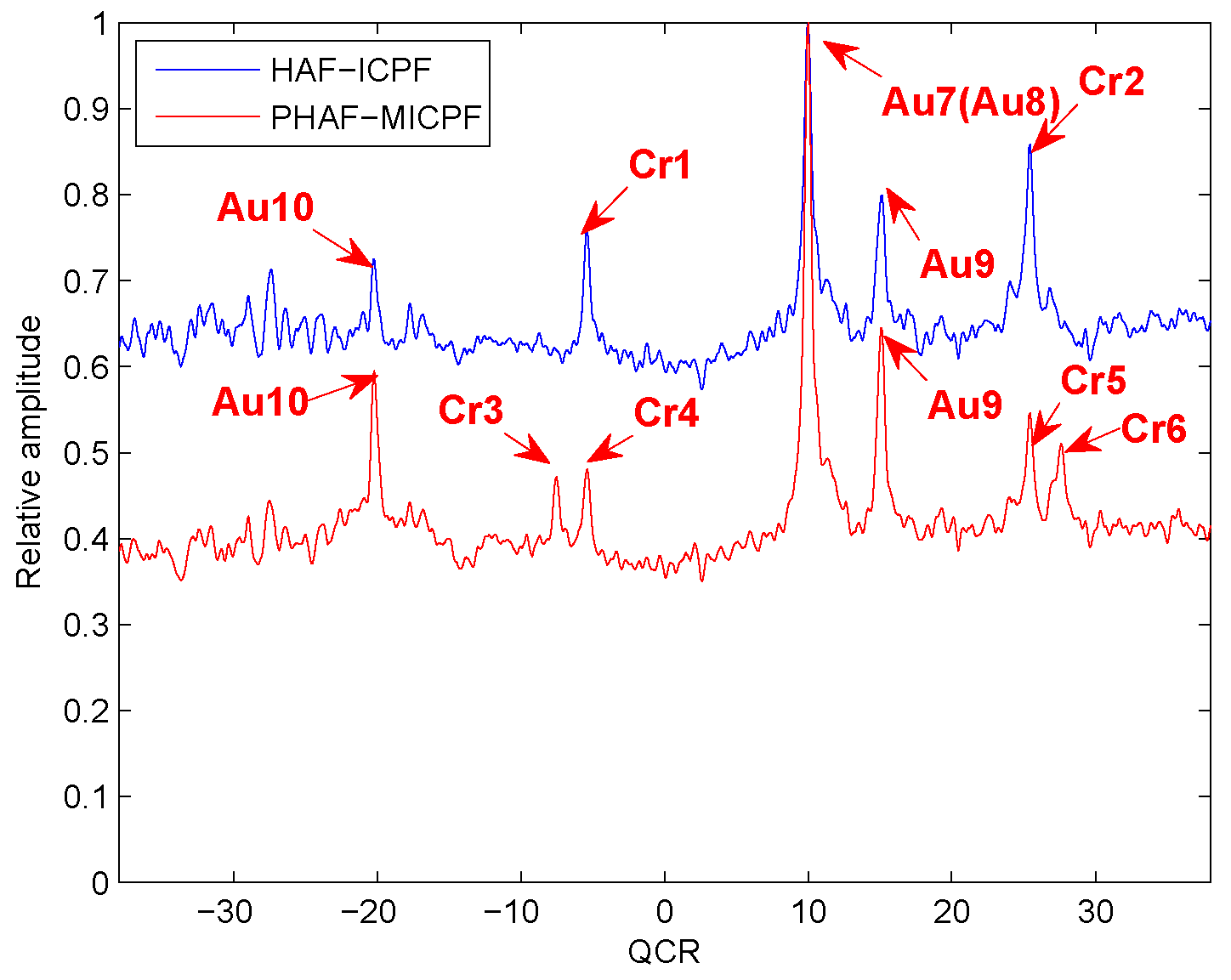
3. Cross-Term Suppression Performance Analysis of HAF-ICPF
- , , .
- , , .
- , , .
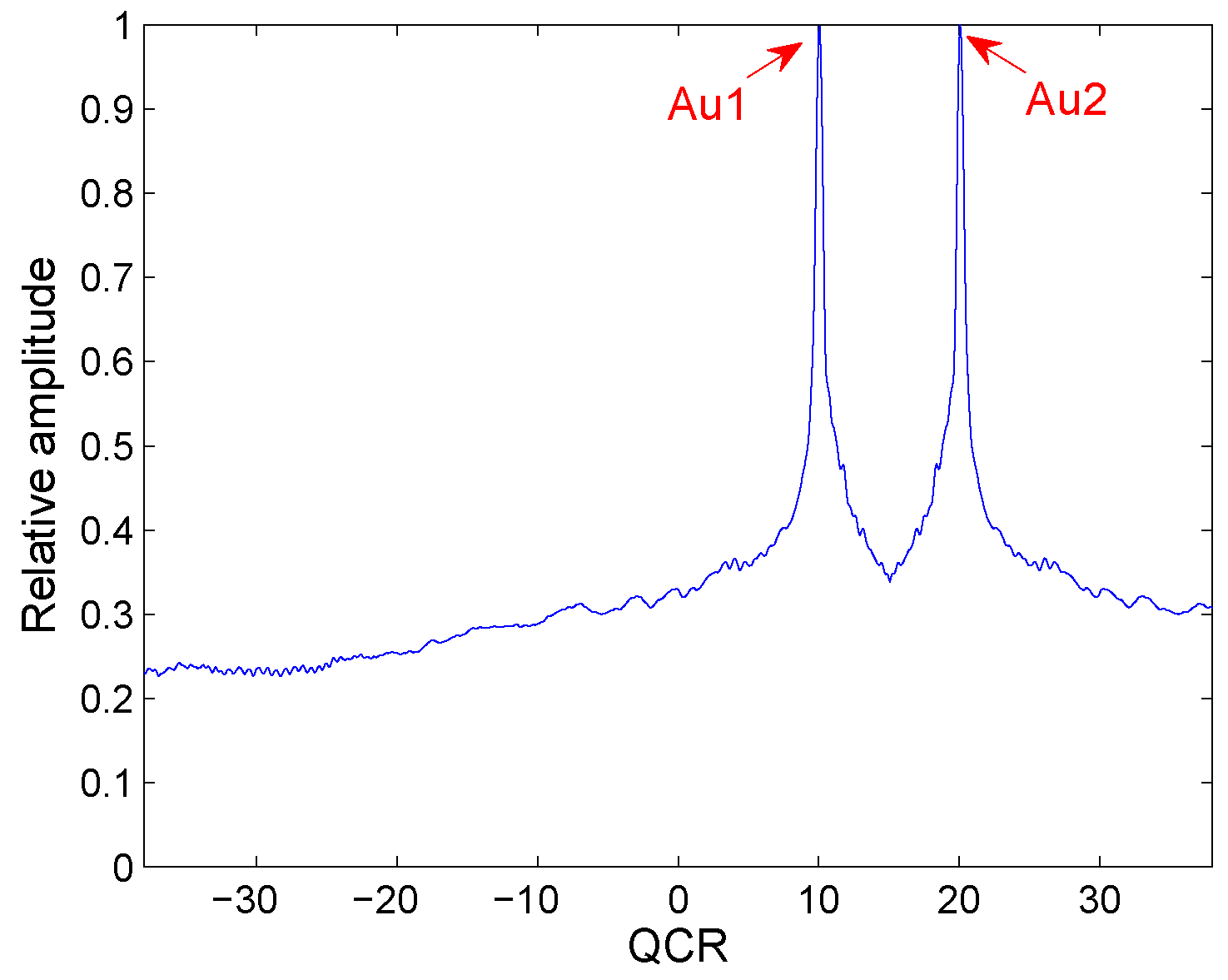
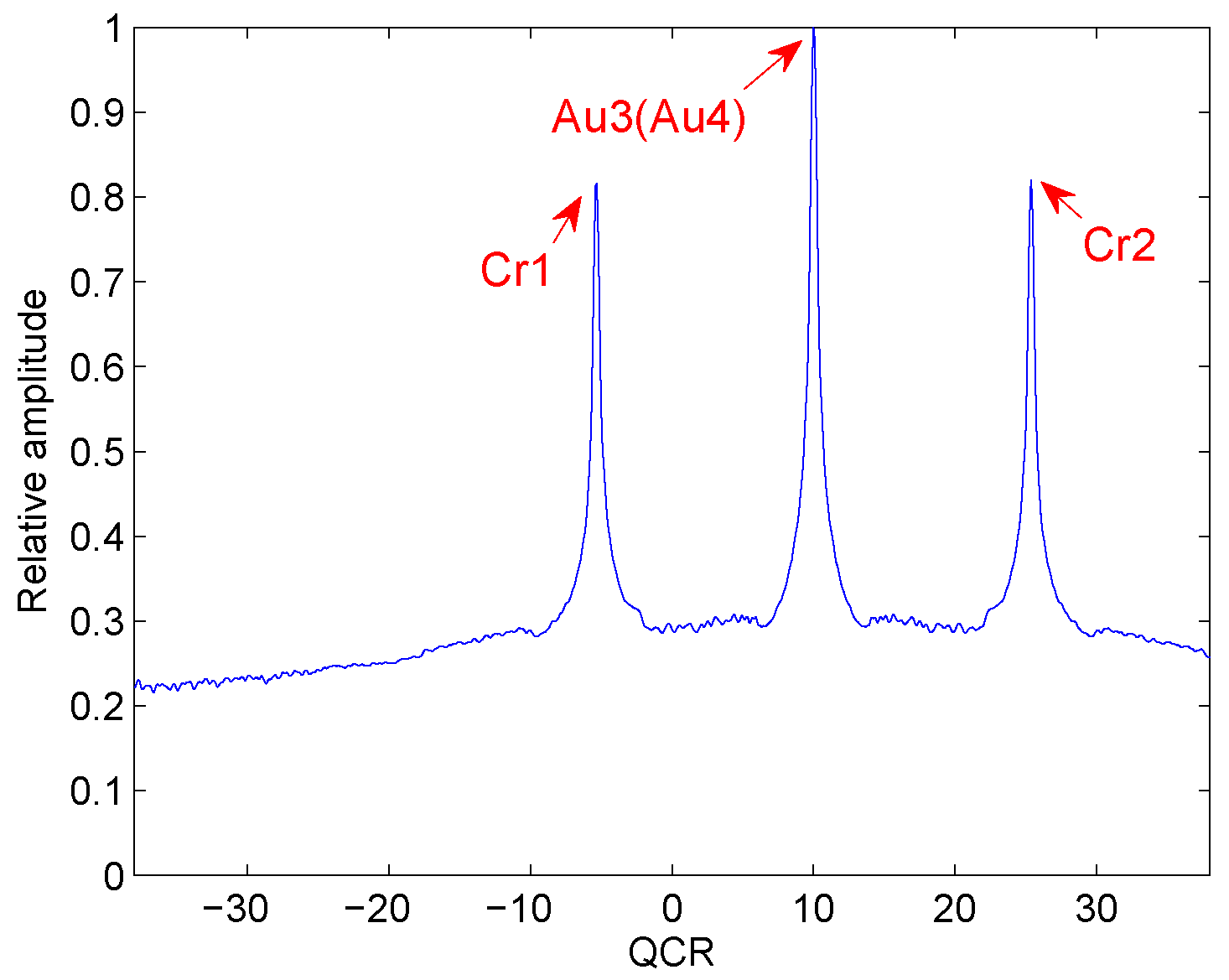
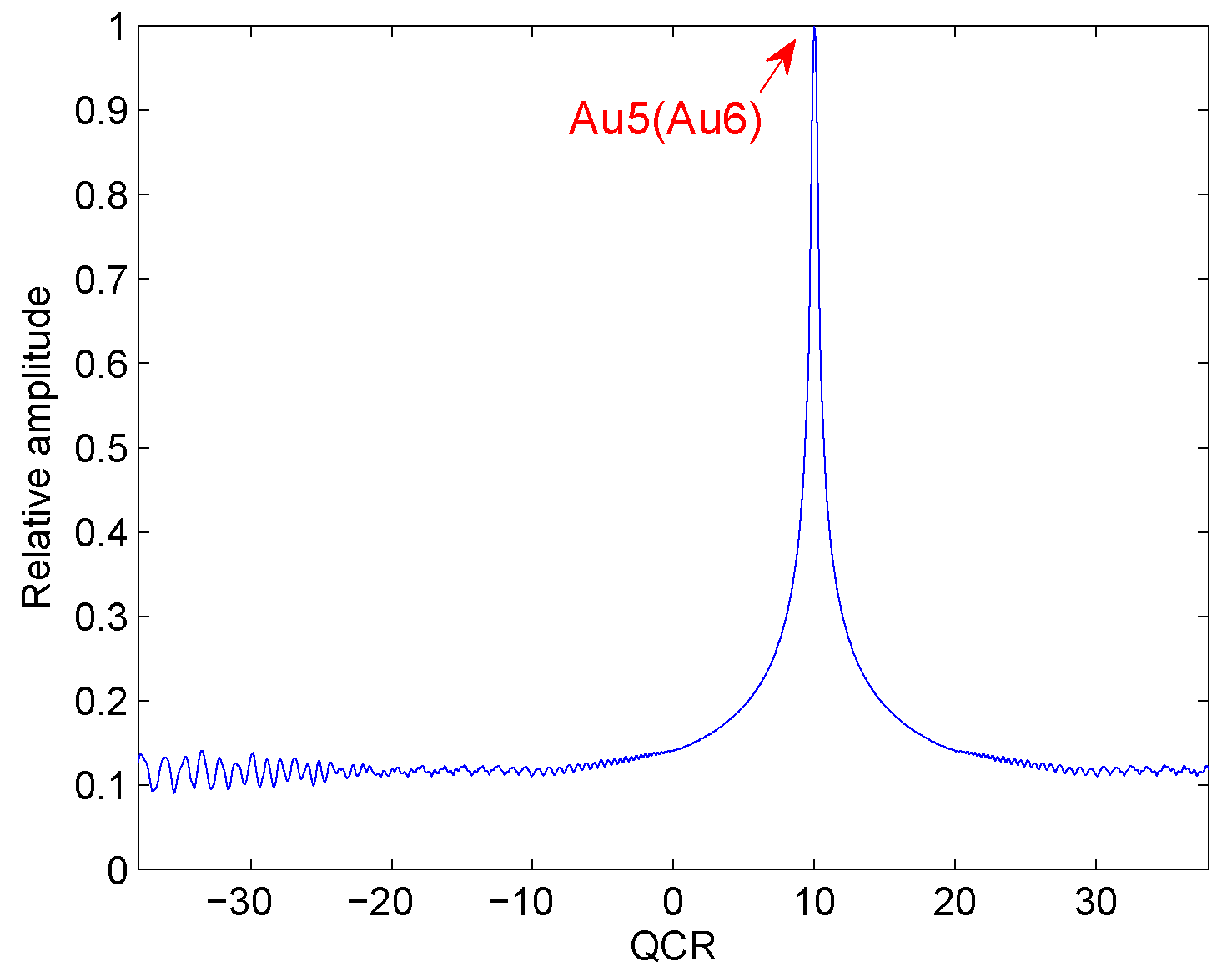
3.1. Case 1
3.2. Case 2
3.3. Case 3
4. The PHAF-MICPF Method
4.1. The Principle of PHAF-MICPF
4.2. Selection of Scale Factors
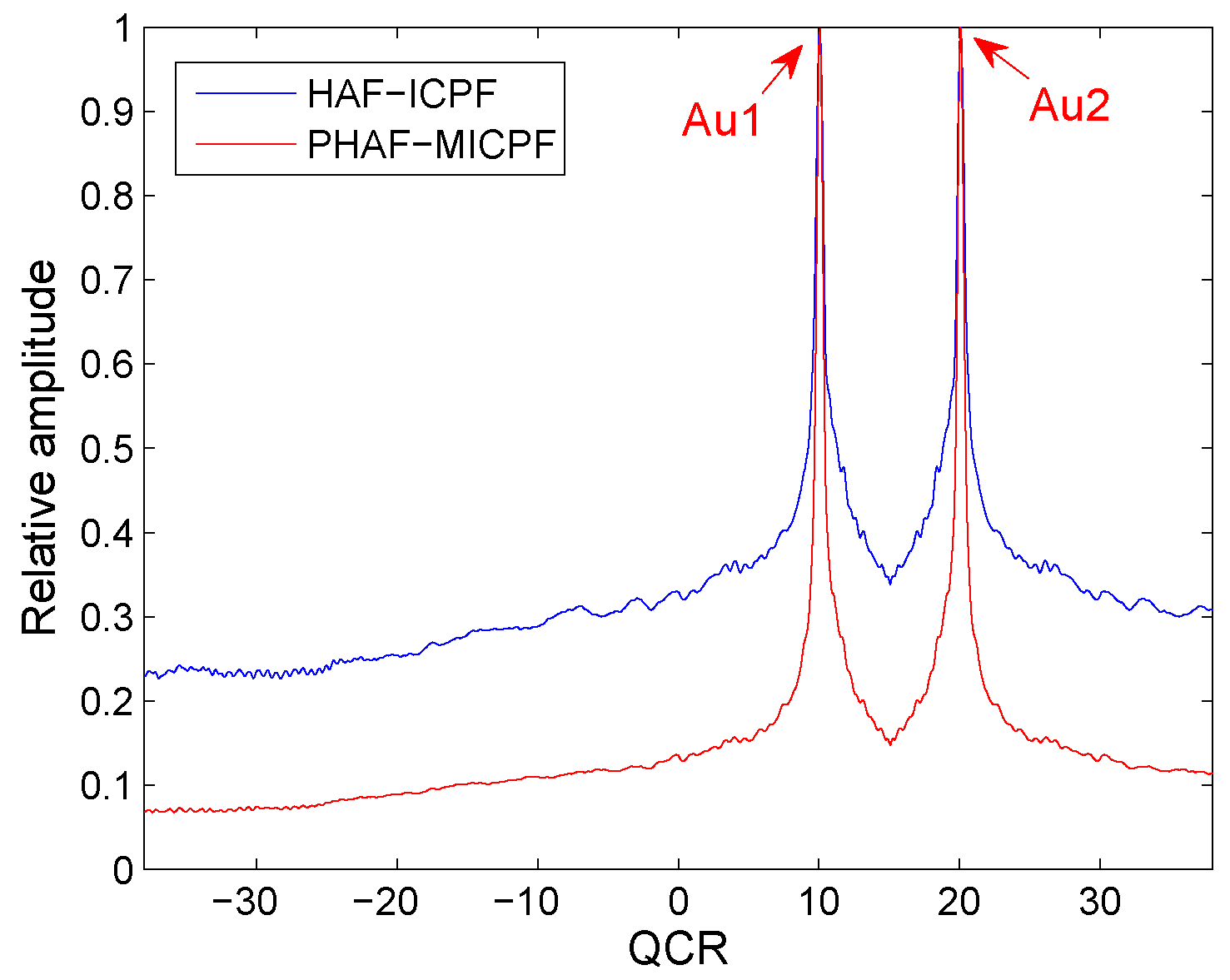
4.3. Cross-Term Suppression Ability Analysis
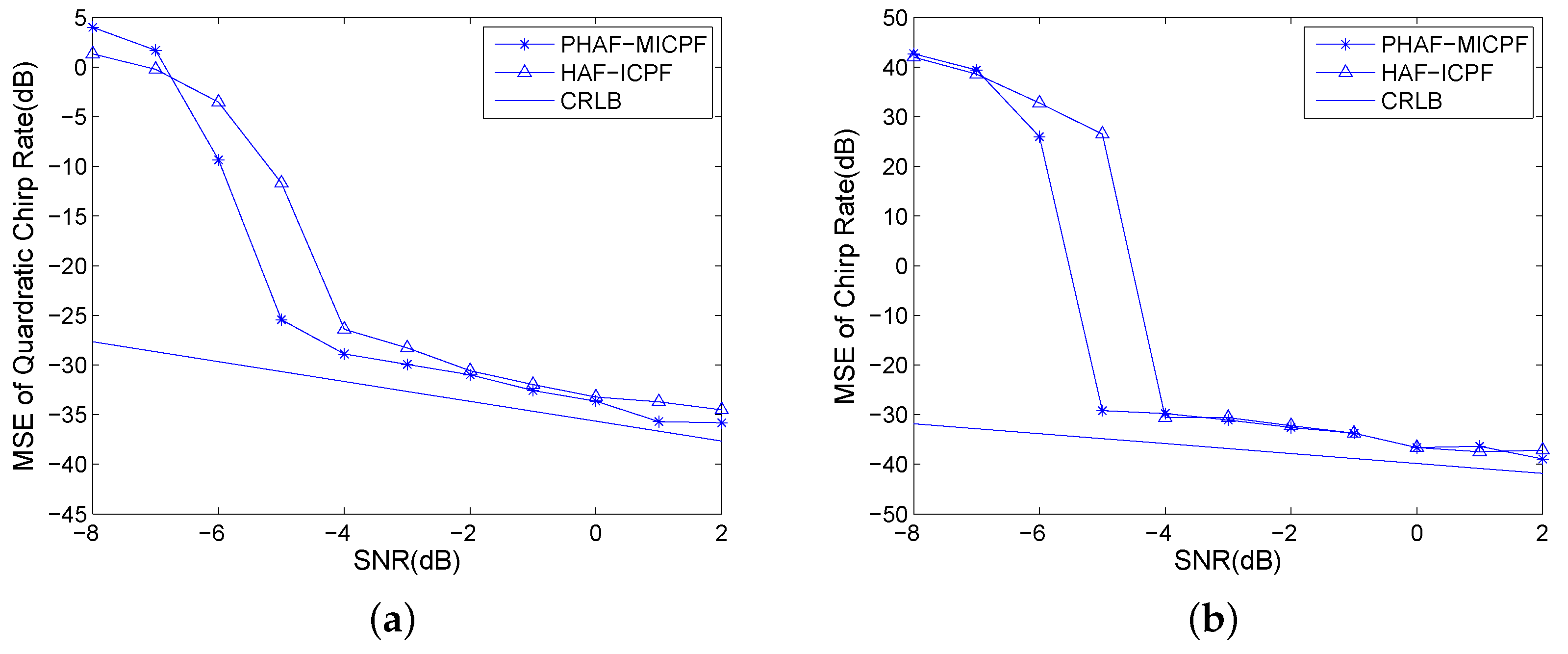
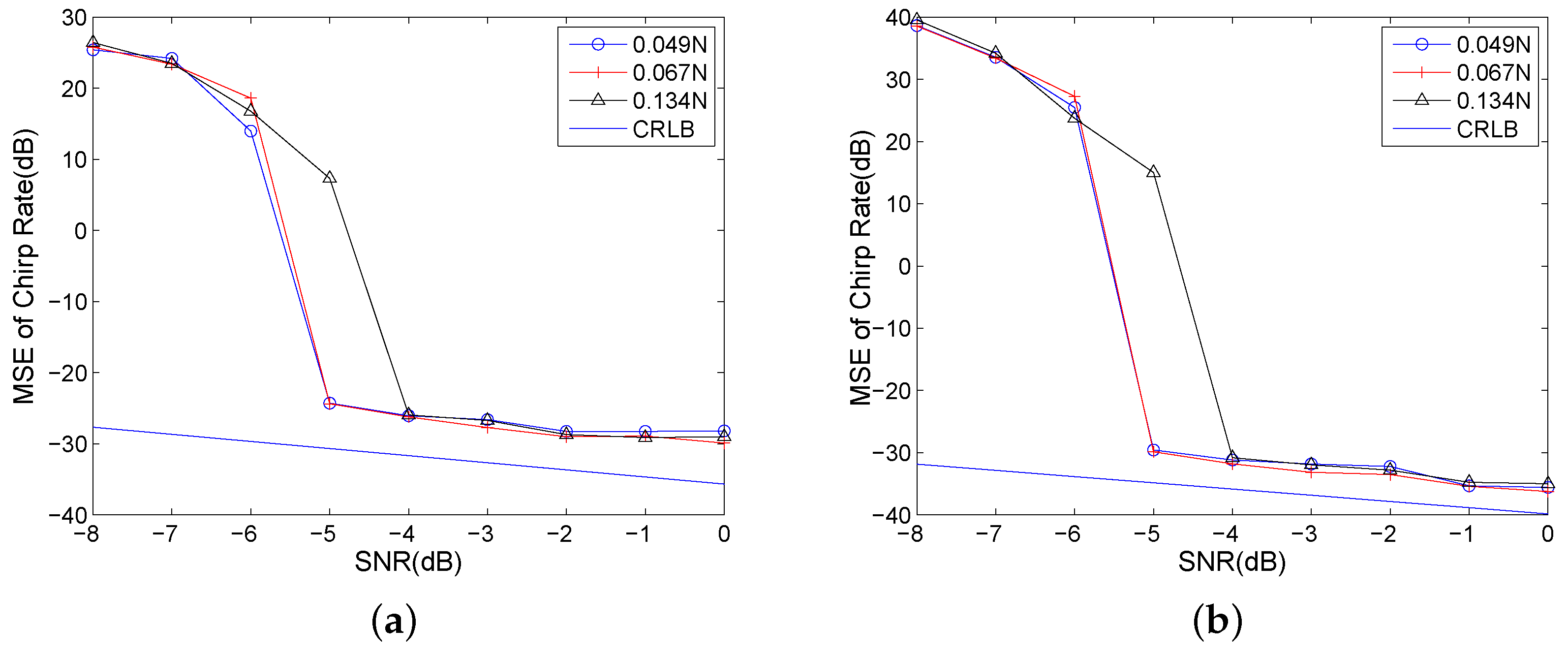
4.4. Anti-Noise Performance Analysis
5. Conclusions
Funding
Conflicts of Interest
References
- Verhaelen, K.; Bauer, A.; Guenther, F.; Mueller, B.; Nist, M.; Celik, B.U.; Weidner, C.; Kuechenhoff, H.; Wallner, P. Anticipation of food safety and fraud issues: Isar-A new screening tool to monitor food prices and commodity flows. Food Control 2018, 94, 93–101. [Google Scholar] [CrossRef]
- Lulu, A.; Mobasseri, B.G. Phase matching of coincident pulses for range-doppler estimation of multiple targets. IEEE Signal Process. Lett. 2019, 26, 199–203. [Google Scholar] [CrossRef]
- Chen, X.L.; Guan, J.; Liu, N.B.; He, Y. Maneuvering target detection via radon-fractional fourier transform-based long-time coherent integration. IEEE Trans. Signal Process. 2014, 62, 939–953. [Google Scholar] [CrossRef]
- Zhao, H.R.; Qiao, L.Y.; Zhang, J.C.; Fu, N. Generalized random demodulator associated with fractional fourier transform. Circuits Syst. Signal Process. 2018, 37, 5161–5173. [Google Scholar] [CrossRef]
- Shinde, S.; Gadre, V.M. An uncertainty principle for real signals in the fractional fourier transform domain. IEEE Trans. Signal Process. 2001, 49, 2545–2548. [Google Scholar] [CrossRef]
- Hlawatsch, F.; Boudreaux-Bartels, G.F. Linear and quadratic time-frequency signal representations. IEEE Signal Process. Mag. 1992, 9, 21–67. [Google Scholar] [CrossRef]
- Xing, M.; Wu, R.; Li, Y.; Bao, Z. New isar imaging algorithm based on modified wigner-ville distribution. IET Radar Sonar Navig. 2009, 3, 70–80. [Google Scholar]
- Su, J.; Tao, H.; Rao, X.; Xie, J.; Guo, X. Coherently integrated cubic phase function for multiple LFM signals analysis. Electron. Lett. 2015, 51, 411–413. [Google Scholar] [CrossRef]
- Abatzoglou, T. Fast maximum likelihood joint estimation of frequency and frequency rate. In Proceedings of the IEEE International Conference on Acoustics, Speech, and Signal Processing (ICASSP ’86), Tokyo, Japan, 7–11 April 1986; pp. 1409–1412. [Google Scholar]
- Zheng, J.B.; Su, T.; Liu, Q.H.; Zhang, L.; Zhu, W.T. Fast parameter estimation algorithm for cubic phase signal based on quantifying effects of doppler frequency shift. Prog. Electromagn. Res. 2013, 142, 57–74. [Google Scholar] [CrossRef]
- Shea, P.O. A new technique for instantaneous frequency rate estimation. IEEE Signal Process. Lett. 2002, 9, 251–252. [Google Scholar] [CrossRef]
- Shea, P.O. A fast algorithm for estimating the parameters of a quadratic fm signal. IEEE Trans. Signal Process. 2004, 52, 385–393. [Google Scholar] [CrossRef]
- Wang, P.; Yang, J.Y. Multicomponent chirp signals analysis using product cubic phase function. Digital Signal Process. 2006, 16, 654–669. [Google Scholar] [CrossRef]
- Wang, Y.; Jiang, Y.C. Inverse synthetic aperture radar imaging of maneuvering target based on the product generalized cubic phase function. IEEE Geosci. Remote Sens. Lett. 2011, 8, 958–962. [Google Scholar] [CrossRef]
- Djurovic, I.; Simeunovic, M.; Djukanovic, S.; Wang, P. A hybrid cpf-haf estimation of polynomial-phase signals: Detailed statistical analysis. IEEE Trans. Signal Process. 2012, 60, 5010–5023. [Google Scholar] [CrossRef]
- Li, X.L.; Kong, L.J.; Cui, G.L.; Yi, W.; Yang, Y.C. Isar imaging of maneuvering target with complex motions based on ACCF-LVD. Digit. Signal Process. 2015, 46, 191–200. [Google Scholar] [CrossRef]
- Xing, M.; Wu, R.; Lan, J.; Bao, Z. Migration through resolution cell compensation in isar imaging. IEEE Geosci. Remote Sens. Lett. 2004, 1, 141–144. [Google Scholar] [CrossRef]
- Li, X.; Liu, G.; Jinlin, N. Autofocusing of isar images based on entropy minimization. IEEE Trans. Aerosp. Electron. Syst. 1999, 35, 1240–1252. [Google Scholar] [CrossRef]
- Wahl, D.E.; Eichel, P.H.; Ghiglia, D.C.; Jakowatz, C.V. Phase gradient autofocus-a robust tool for high resolution sar phase correction. IEEE Trans. Aerosp. Electron. Syst. 1994, 30, 827–835. [Google Scholar] [CrossRef]
- Bai, X.; Tao, R.; Wang, Z.; Wang, Y. Isar imaging of a ship target based on parameter estimation of multicomponent quadratic frequency-modulated signals. IEEE Trans. Geosci. Remote Sens. 2014, 52, 1418–1429. [Google Scholar] [CrossRef]
- Wu, L.; Wei, X.; Yang, D.; Wang, H.; Li, X. Isar imaging of targets with complex motion based on discrete chirp fourier transform for cubic chirps. IEEE Trans. Geosci. Remote Sens. 2012, 50, 4201–4212. [Google Scholar] [CrossRef]
- Barbarossa, S.; Scaglione, A.; Giannakis, G.B. Product high-order ambiguity function for multicomponent polynomial-phase signal modeling. IEEE Trans. Signal Process. 1998, 46, 691–708. [Google Scholar] [CrossRef]
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, L. Quadratic Frequency Modulation Signals Parameter Estimation Based on Product High Order Ambiguity Function-Modified Integrated Cubic Phase Function. Information 2019, 10, 140. https://doi.org/10.3390/info10040140
Zhu L. Quadratic Frequency Modulation Signals Parameter Estimation Based on Product High Order Ambiguity Function-Modified Integrated Cubic Phase Function. Information. 2019; 10(4):140. https://doi.org/10.3390/info10040140
Chicago/Turabian StyleZhu, Lei. 2019. "Quadratic Frequency Modulation Signals Parameter Estimation Based on Product High Order Ambiguity Function-Modified Integrated Cubic Phase Function" Information 10, no. 4: 140. https://doi.org/10.3390/info10040140
APA StyleZhu, L. (2019). Quadratic Frequency Modulation Signals Parameter Estimation Based on Product High Order Ambiguity Function-Modified Integrated Cubic Phase Function. Information, 10(4), 140. https://doi.org/10.3390/info10040140



