On the Habitability of Aquaplanets
Abstract
:1. Introduction
| SPH | Vegetation Type | Kind of Planet |
|---|---|---|
| >0.8–1.0 | Dense vegetation | Amazonian |
| >0.6–0.8 | Mixed vegetation | Serengetian |
| >0.4–0.6 | Shrublands | Mediterranean |
| >0.2–0.4 | Grasslands | Pampian |
| >0.0–0.2 | Sparsely vegetated | Saharan |
| = 0.0 | None | Dead |
2. Materials and Methods
- (a)
- Mineral/Rock aspect: Chemical elements are needed to form stable structures (biogenic elements; for instance, on Earth all studied species contain C, H, O, N, P and S). Other elements (or its compounds) can be harmful for life; representing mineral toxicity (for instance, increased salinity near coastal areas). This is to be represented as fM in a habitability index.
- (b)
- Mixing (or solvent) aspect: Biogenic chemical elements need the (intermediate) mobility of a liquid medium to combine and form complex biomolecules. This liquid is called the solvent (on Earth it is water, but it could be hydrocarbons in colder worlds). This is to be represented as fS in a habitability index.
- (c)
- Energetic aspect: In the above mentioned (liquid) medium, an energy source is needed to overcome potential barriers between reactants (biogenic elements) and products (biomolecules). On Earth, for primary producers, the most common energy source is photosynthetically active radiation (PAR) coming mostly from the Sun. In hydrothermal vents some bacteria use geothermal PAR from hot water, or chemical energy from simple redox reactions. Both energetic aspects would reflect, in broad terms, the external and internal geodynamic activity of the planet or moon. This is to be represented as fE in a habitability index.
2.1. An Aquatic Primary Habitability Index
2.1.1. The Function f(L)
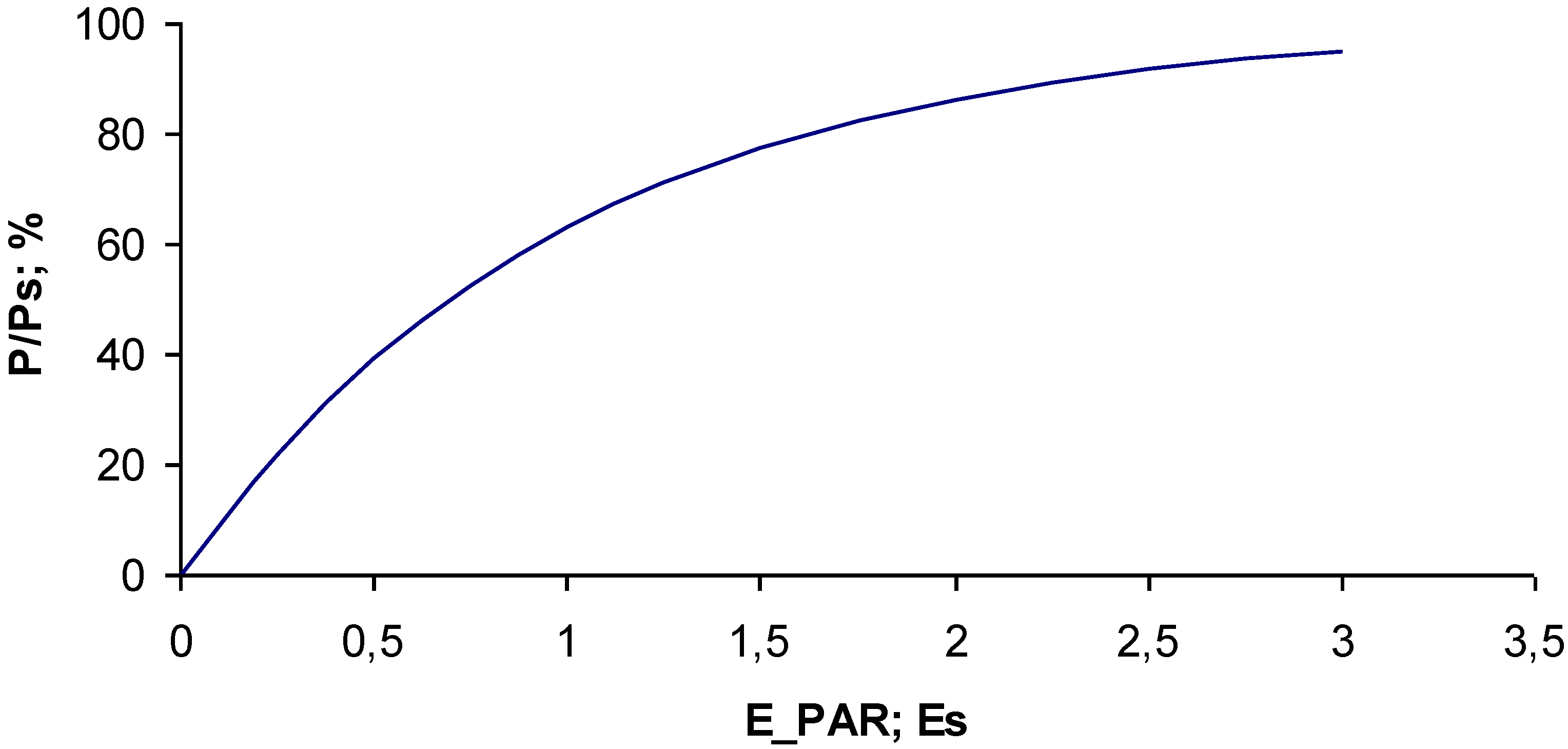
2.1.2. The Function f(T)
3. Results and Discussion
3.1. Calibration of the Aquatic Primary Habitability Index

3.2. Case Studies
3.2.1. Earth after a Galactic Gamma Ray Burst (from Core-Collapse Supernova)
3.2.2. Earth Ocean after Chicxulub Asteroid Impact
3.2.3. Current Climate Change
4. Conclusions
Author Contributions
Conflict of Interest
References
- Quantitative Habitability Theory. Available online: http://phl.upr.edu/projects/planetary-habitability (accessed on 8 May 2014).
- Méndez, A. Evolution of the Global Terrestrial Habitability during the Last Century. In Proceeedings of Sixth Astrobiology Science Conference, Houston, TX, USA, 26–29 April 2010.
- Hadjibiros, K. Ecology and Applied Environmental Science; CRC Press, Taylor and Francis Group: Boca Raton, Florida, USA, 2013; pp. 49–51. [Google Scholar]
- Kirk, J. Light and Photosynthesis in Aquatic Ecosystems, 3rd ed.; Cambridge University Press: New York, NY, USA, 2011; p. 453. [Google Scholar]
- Fennel, W.; Neumann, T. Introduction to the Modelling Marine Ecosystems; Elsevier B.V.: Amsterdam, The Netherlands, 2004; p. 133. [Google Scholar]
- Fritz, J.; Neale, P.; Davis, R.; Pelloquin, J. Response of Antarctic phytoplankton to solar UVR exposure: Inhibition and recovery of photosynthesis in coastal and pelagic assemblages. Mar. Ecol. Prog. Ser. 2008, 365, 1–16. [Google Scholar] [CrossRef]
- Rodriguez, L.; Cardenas, R.; Avila-Alonso, D. On the photosynthetic potential in open oceans. Rev. Cub. Fis. 2014, 31, 15–17. [Google Scholar]
- Jerlov, N. Marine Optics, 2nd ed.; Elsevier Scientific Publishing Company: Amsterdam, The Netherlands, 1976; pp. 127–137. [Google Scholar]
- Martin, O.; Galante, D.; Cardenas, R.; Horvath, J. Short-term effects of gamma ray bursts on Earth. Astrophys. Space. Sci. 2009, 321, 161–167. [Google Scholar] [CrossRef]
- Martin, O.; Cardenas, R.; Guimarais, M.; Peñate, L.; Horvath, J.; Galante, D. Effects of gamma ray bursts in Earth’s biosphere. Astrophys. Space Sci. 2010, 326, 61–67. [Google Scholar] [CrossRef]
- Martin, O.; Cardenas, R.; Horvath, J.; Peñate, L. Effects of galactic gamma rays bursts on planetary atmospheres. Int. J. Mod. Phys. E-Nucl. Phys. 2011, 20, 67–70. [Google Scholar]
- Peñate, L.; Martin, O.; Cardenas, R.; Agusti, S. Short-term effects of gamma ray bursts on oceanic photosynthesis. Astrophys. Space Sci. 2010, 330, 211–217. [Google Scholar]
- Cardenas, R.; Martin, O.; Peñate, L.; Horvath, J. Effects of galactic gamma rays bursts on planetary biospheres. Int. J. Mod. Phys. E-Nucl. Phys. 2011, 20, 132–135. [Google Scholar]
- Thomas, B.; Melott, A.; Jackman, C.; Laird, C.; Medvedev, M.; Stolarski, R.; Gehrels, N.; Cannizzo, J.; Hogan, D.; Ejzak, L. Gamma-ray bursts and the Earth: Exploration of atmospheric, biological, climatic and biogeochemical effects. Astrophys. J. 2005, 634, 509–533. [Google Scholar] [CrossRef]
- Tropospheric Ultraviolet and Visible Radiation Model. Available online: http://cprm.acd.ucar.edu/Models/TUV/ (accessed on 21 August 2014).
- Ribeiro, S.; Berge, T.; Lundholm, N.; Andersen, T.; Abrantes, F.; Ellegaard, M. Phytoplankton growth after a century of dormancy illuminates past resilience to catastrophic darkness. Nat. Commun. 2011, 2, 1–7. [Google Scholar] [CrossRef]
- Perez, N.; Martin, O.; Cardenas, R. Photobiological damage associated to the Chicxulub asteroid impact. Rev. Cub. Fis. 2014. submitted. [Google Scholar]
- Hader, D.; Kumar, H.; Smith, R.; Worrest, R. Effects of solar UV radiation on aquatic ecosystems and interactions with climate change. Photochem. Photobiol. Sci. 2007, 6, 267–285. [Google Scholar] [CrossRef] [PubMed]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Cardenas, R.; Perez, N.; Martinez-Frias, J.; Martin, O. On the Habitability of Aquaplanets. Challenges 2014, 5, 284-293. https://doi.org/10.3390/challe5020284
Cardenas R, Perez N, Martinez-Frias J, Martin O. On the Habitability of Aquaplanets. Challenges. 2014; 5(2):284-293. https://doi.org/10.3390/challe5020284
Chicago/Turabian StyleCardenas, Rolando, Noel Perez, Jesus Martinez-Frias, and Osmel Martin. 2014. "On the Habitability of Aquaplanets" Challenges 5, no. 2: 284-293. https://doi.org/10.3390/challe5020284
APA StyleCardenas, R., Perez, N., Martinez-Frias, J., & Martin, O. (2014). On the Habitability of Aquaplanets. Challenges, 5(2), 284-293. https://doi.org/10.3390/challe5020284





