Performance Assessment of a Planing Hull Using the Smoothed Particle Hydrodynamics Method
Abstract
1. Introduction
- Fu et al., (2014) [13] showed the results from a collaborative research effort involving two different CFD codes: CFDShip-Iowa and Numerical Flow Analysis – NFA. The results were presented and discussed examining the hydrodynamic forces, moments, hull pressures, accelerations, motions, and the multi-phase free-surface flow field generated by a prismatic planing craft at high speed in calm water and waves. The comparison between numerical and experimental data for still water conditions indicated that at high Froude Number (), the dynamic trim was generally under-predicted and the resistance over-predicted.
- Kandasamy et al., (2011) [14] exposed a Verification and Validation (V&V) analysis in full scale with the Unsteady Reynolds-Averaged Navier–Stokes (URANS) code CFDShip-Iowa for two high-speed semi-planing foil-assisted catamarans. Comparing the experimental data against the full-scale simulation results, the resistance comparison error was in the range of 9.6% to 15.5% and the dynamic trim angle comparison error was in the range of −44.1% to 0.8%.
- Yousefy et al., (2013) [15] conducted a comprehensive study on the existing numerical techniques for planing craft and they used several different commercially available CFD software programs (ANSYS-FLUENT, ANSYS-CFX, CFD Ship-Iowa, ShipFlow, Tdyn, CD-Adapco Star-CCM+) to determine the flow field around a planing hull.
- De Luca et al., (2016) [18] showed the results of a comprehensive V&V campaign of simulations of resistance test in still water condition using the hulls of the warped planing hulls of the Naples Systematic Series. The analysis depicts, for a wide range of speed and different hull shapes, the simulation uncertainty and the comparison errors. All the simulations in this study were carried out using the CFD software Star-CCM+.
2. DualSPHysics Code
2.1. SPH Method
2.2. Fluid-Solid Interaction
2.3. Dynamic Boundary Conditions
2.4. Open Boundary Conditions
2.5. Coupling with Project Chrono
3. Benchmark Experimental Data
3.1. Experimental Data
3.2. Testing Facility
4. Numerical Setup
5. Results
5.1. Hydrostatic Test
5.2. Total Resistance, Dynamic Trim Angle, and Sinkage
5.3. Whisker Spray
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BEM | Boundary Element Method |
| CCP | Cone Complementary Problem |
| CFD | Computational Fluid Dynamics |
| CFL | Courant-Friedrich-Lewy Number |
| DBC | Dynamic Boundary Condition |
| DII | Department of Industrial Engineering |
| DVI | Differential Variational Inequality |
| Fr | Froude Number |
| FVM | Finite Volume Method |
| GPU | Graphics Processing Unit |
| HSMV | High-Speed Marine Vehicle |
| ITTC | International Towing Tank Conference |
| LCB | Longitudinal position of the Center of Buoyancy |
| NFA | Numerical Flow Analysis |
| NS | Navier–Stokes |
| NSS | Naples Systematic Series |
| Re | Reynolds Number |
| (U)RANS | (Unsteady) Reynolds-Averaged Navier–Stokes |
| SPH | Smoothed Particle Hydrodynamics |
| V&V | Verification & Validation |
| VOF | Volume of Fluid |
| WCSPH | Weakly Compressible Smoothed Particle Hydrodynamics |
References
- ITTC. Model Tests of High Speed Marine Vehicles Specialist Committee. Final Report and Recommendations of the 22nd ITTC Committee. 1999. Available online: https://ittc.info/media/1510/specialist-committee-on-safety-of-high-speed-marine-vehicles.pdf (accessed on 18 February 2021).
- Savitsky, D. Hydrodynamic Design of Planing Hulls. Mar. Technol. SNAME News 1964, 1, 71–95. [Google Scholar] [CrossRef]
- Blount, D.L. Performance by Design: Hydrodynamics for High-Speed Vessels, 1st ed.; Blount, D.L., Ed.; Donald L. Blount and Associates, Inc.: Chesapeake, VA, USA, 2014. [Google Scholar]
- Di Caterino, F.; Bilandi, R.N.; Mancini, S.; Dashtimanesh, A.; De Carlini, M. A Numerical Way for a Stepped Planing Hull Design and Optimization. In Proceedings of the NAV 2018, 19th International Conference on Ship and Maritime Research, Trieste, Italy, 20–22 June 2018. [Google Scholar] [CrossRef]
- Thornhill, E.; Oldford, D.; Bose, N.; Veitch, B.; Liu, P. Planing hull performance from model tests. Int. Shipbuild. Prog. 2003, 50, 5–18. [Google Scholar]
- Begovic, E.; Bertorello, C. Resistance assessment of warped hullform. Ocean Eng. 2012, 56, 28–42. [Google Scholar] [CrossRef]
- Matveev, K.I. Hydrodynamic modeling of planing hulls with twist and negative deadrise. Ocean Eng. 2014, 82, 14–19. [Google Scholar] [CrossRef]
- Sukas, O.F.; Kinaci, O.K.; Cakici, F.; Gokce, M.K. Hydrodynamic assessment of planing hulls using overset grids. Appl. Ocean Res. 2017, 65, 35–46. [Google Scholar] [CrossRef]
- Jiang, Y.; Sun, H.; Zou, J.; Hu, A.; Yang, J. Analysis of tunnel hydrodynamic characteristics for planing trimaran by model tests and numerical simulations. Ocean Eng. 2016, 113, 101–110. [Google Scholar] [CrossRef]
- De Marco, A.; Mancini, S.; Miranda, S.; Scognamiglio, R.; Vitiello, L. Experimental and numerical hydrodynamic analysis of a stepped planing hull. Appl. Ocean Res. 2017, 64, 135–154. [Google Scholar] [CrossRef]
- Bilandi, R.; Mancini, S.; Vitiello, L.; Miranda, S.; De Carlini, M. A Validation of Symmetric 2D + T Model Based on Single-Stepped Planing Hull Towing Tank Tests. J. Mar. Sci. Eng. 2018, 6, 136. [Google Scholar] [CrossRef]
- Tavakoli, S.; Niazmand Bilandi, R.; Mancini, S.; De Luca, F.; Dashtimanesh, A. Dynamic of a planing hull in regular waves: Comparison of experimental, numerical and mathematical methods. Ocean Eng. 2020, 217, 107959. [Google Scholar] [CrossRef]
- Fu, T.; Brucker, K.; Mousaviraad, M.; Ikeda-Gilbert, C.; Lee, E.; O’Shea, T.; Wang, Z.; Stern, F.; Judge, C. An Assessment of Computational Fluid Dynamics Predictions of the Hydrodynamics of High-Speed Planing Craft in Calm Water and Waves. In Proceedings of the 30th Symposium on Naval Hydrodynamics Hobart, Tasmania, Hobart, Australia, 2–7 November 2014. [Google Scholar]
- Kandasamy, M.; Ooi, S.K.; Carrica, P.; Stern, F.; Campana, E.; Peri, D.; Osborne, P.; Cote, J.; Macdonald, N.; de Waal, N. CFD validation studies for a high-speed foil-assisted semi-planing catamaran. J. Mar. Sci. Technol. 2011, 16, 157–167. [Google Scholar] [CrossRef]
- Yousefi, R.; Shafaghat, R.; Shakeri, M. Hydrodynamic analysis techniques for high-speed planing hulls. Appl. Ocean Res. 2013, 42, 105–113. [Google Scholar] [CrossRef]
- Mousaviraad, S.M.; Wang, Z.; Stern, F. URANS studies of hydrodynamic performance and slamming loads on high-speed planing hulls in calm water and waves for deep and shallow conditions. Appl. Ocean Res. 2015, 51, 222–240. [Google Scholar] [CrossRef]
- Fridsma, G. A Systematic Study of the Rough-Water Performance of Planing Boats; Technical Report, Davidson Laboratory Report 1275; Stevens Institute of Technology Davidson Laboratory, Castle Point Station: Hoboken, NY, USA, 1969. [Google Scholar]
- De Luca, F.; Mancini, S.; Miranda, S.; Pensa, C. An Extended Verification and Validation Study of CFD Simulations for Planing Hulls. J. Ship Res. 2016, 60, 101–118. [Google Scholar] [CrossRef]
- Kocaman, S.; Güzel, H.; Evangelista, S.; Ozmen-Cagatay, H.; Viccione, G. Experimental and Numerical Analysis of a Dam-Break Flow through Different Contraction Geometries of the Channel. Water 2020, 12, 1124. [Google Scholar] [CrossRef]
- Volpi, S.; Diez, M.; Sadat-Hosseini, H.; Kim, D.H.; Stern, F.; Thodal, R.; Grenestedt, J. Composite bottom panel slamming of a fast planing hull via tightly coupled fluid-structure interaction simulations and sea trials. Ocean Eng. 2017, 143, 240–258. [Google Scholar] [CrossRef]
- Rosén, A.; Garme, K.; Razola, M.; Begovic, E. Numerical modelling of structure responses for high-speed planing craft in waves. Ocean Eng. 2020, 217, 107897. [Google Scholar] [CrossRef]
- Violeau, D.; Rogers, B. Smoothed particle hydrodynamics (SPH) for free-surface flows: Past, present and future. J. Hydraul. Res. 2016, 54, 1–26. [Google Scholar] [CrossRef]
- Gotoh, H.; Khayyer, A. On the state-of-the-art of particle methods for coastal and ocean engineering. Coast. Eng. J. 2018, 60, 1–25. [Google Scholar] [CrossRef]
- Manenti, S.; Wang, D.; Domínguez, J.; Li, S.; Amicarelli, A.; Albano, R. SPH Modeling of Water-Related Natural Hazards. Water 2019, 11, 1875. [Google Scholar] [CrossRef]
- Landrini, M.; Colagrossi, A.; Greco, M.; Tulin, M. The fluid mechanics of splashing bow waves on ships: A hybrid BEM–SPH analysis. Ocean Eng. 2012, 53, 111–127. [Google Scholar] [CrossRef]
- Marrone, S.; Bouscasse, B.; Colagrossi, A.; Antuono, M. Study of ship wave breaking patterns using 3D parallel SPH simulations. Comput. Fluids 2012, 69, 54–66. [Google Scholar] [CrossRef]
- Dashtimanesh, A.; Ghadimi, P. A three-dimensional SPH model for detailed study of free surface deformation, just behind a rectangular planing hull. J. Braz. Soc. Mech. Sci. Eng. 2013, 35, 369–380. [Google Scholar] [CrossRef]
- Tafuni, A.; Sahin, I.; Hyman, M. Numerical investigation of wave elevation and bottom pressure generated by a planing hull in finite-depth water. Appl. Ocean Res. 2016, 58, 281–291. [Google Scholar] [CrossRef]
- Brizzolara, S.; Viviani, M.; Savio, L. Comparison of SPH and RANSE methods for the evaluation of impact problems in the marine field. In Proceedings of the 8th World Congress on Computational Mechanics (WCCM8), Venice, Italy, 30 June–4 July 2008. [Google Scholar]
- Campbell, J.; Patel, M. Modelling fluid–structure impact with the coupled FE-SPH approach. In Proceedings of the William Froude Conference on Advances in Theoretical and Applied Hydrodynamic, Portsmouth, UK, 24–25 November 2010; pp. 131–137. [Google Scholar]
- Fragassa, C. Engineering Design Driven by Models and Measures: The Case of a Rigid Inflatable Boat. J. Mar. Sci. Eng. 2019, 7, 6. [Google Scholar] [CrossRef]
- Altomare, C.; Viccione, G.; Tagliafierro, B.; Bovolin, V.; Domínguez, J.; Crespo, A. Free-Surface Flow Simulations with Smoothed Particle Hydrodynamics Method using High-Performance Computing. In Computational Fluid Dynamics-Basic Instruments and Applications in Science; Adela Ionescu, IntechOpen: London, UK, 2018; pp. 73–100. [Google Scholar] [CrossRef][Green Version]
- Crespo, A.; Domínguez, J.; Rogers, B.; Gómez-Gesteira, M.; Longshaw, S.; Canelas, R.; Vacondio, R.; Barreiro, A.; García-Feal, O. DualSPHysics: Open-source parallel CFD solver based on Smoothed Particle Hydrodynamics (SPH). Comput. Phys. Commun. 2015, 187, 204–216. [Google Scholar] [CrossRef]
- NVIDIA; Vingelmann, P.; Fitzek, F.H. CUDA, Release: 10.2.89 [Internet]. 2020. Available online: https://developer.nvidia.com/cuda-toolkit (accessed on 18 February 2021).
- Tasora, A.; Serban, R.; Mazhar, H.; Pazouki, A.; Melanz, D.; Fleischmann, J.; Taylor, M.; Sugiyama, H.; Negrut, D. Chrono: An Open Source Multi-physics Dynamics Engine. In International Conference on High Performance Computing in Science and Engineering; Springer: Cham, Switzerland, 2016; pp. 19–49. [Google Scholar] [CrossRef]
- Brito, M.; Canelas, R.; García-Feal, O.; Domínguez, J.; Crespo, A.; Ferreira, R.; Neves, M.; Teixeira, L. A numerical tool for modelling oscillating wave surge converter with nonlinear mechanical constraints. Renew. Energy 2020, 146, 2024–2043. [Google Scholar] [CrossRef]
- Ropero-Giralda, P.; Crespo, A.J.; Tagliafierro, B.; Altomare, C.; Domínguez, J.M.; Gómez-Gesteira, M.; Viccione, G. Efficiency and survivability analysis of a point-absorber wave energy converter using DualSPHysics. Renew. Energy 2020, 162, 1763–1776. [Google Scholar] [CrossRef]
- Tagliafierro, B.; Montuori, R.; Vayas, I.; Ropero-Giralda, P.; Crespo, A.; Domìnguez, J.; Altomare, C.; Viccione, G.; Gòmez-Gesteira, M. A new open source solver for modelling fluid-structure interaction: Case study of a point-absorber wave energy converter with a power take-off unit. In Proceedings of the 11th International Conference on Structural Dynamics, Athens, Greece, 22–24 June 2020. [Google Scholar] [CrossRef]
- Ropero-Giralda, P.; Crespo, A.J.C.; Coe, R.G.; Tagliafierro, B.; Domínguez, J.M.; Bacelli, G.; Gómez-Gesteira, M. Modelling a Heaving Point-Absorber with a Closed-Loop Control System Using the DualSPHysics Code. Energies 2021, 14, 760. [Google Scholar] [CrossRef]
- Mogan, S.C.; Chen, D.; Hartwig, J.; Sahin, I.; Tafuni, A. Hydrodynamic analysis and optimization of the Titan submarine via the SPH and Finite–Volume methods. Comput. Fluids 2018, 174, 271–282. [Google Scholar] [CrossRef]
- Viccione, G.; Bovolin, V.; Carratelli, E.P. Defining and optimizing algorithms for neighbouring particle identification in SPH fluid simulations. Int. J. Numer. Methods Fluids 2008, 58, 625–638. [Google Scholar] [CrossRef]
- Domínguez, J.M.; Crespo, A.J.C.; Gómez-Gesteira, M.; Marongiu, J.C. Neighbour lists in smoothed particle hydrodynamics. Int. J. Numer. Methods Fluids 2011, 67, 2026–2042. [Google Scholar] [CrossRef]
- Colagrossi, A.; Landrini, M. Numerical Simulation of Interfacial Flows by Smoothed Particle Hydrodynamics. J. Comput. Phys. 2003, 191, 448–475. [Google Scholar] [CrossRef]
- Antuono, M.; Colagrossi, A.; Marrone, S.; Molteni, D. Free-surface flows solved by means of SPH schemes with numerical diffusive terms. Comput. Phys. Commun. 2010, 181, 532–549. [Google Scholar] [CrossRef]
- Pugliese Carratelli, E.; Viccione, G.; Bovolin, V. Free surface flow impact on a vertical wall: A numerical assessment. Theor. Comput. Fluid Dyn. 2016, 30, 403–414. [Google Scholar] [CrossRef]
- Khayyer, A.; Gotoh, H.; Falahaty, H.; Shimizu, Y. An enhanced ISPH–SPH coupled method for simulation of incompressible fluid–elastic structure interactions. Comput. Phys. Commun. 2018, 232, 139–164. [Google Scholar] [CrossRef]
- Domínguez, J.; Crespo, A.; Hall, M.; Altomare, C.; Wu, M.; Stratigaki, V.; Troch, P.; Cappietti, L.; Gómez-Gesteira, M. SPH simulation of floating structures with moorings. Coast. Eng. 2019, 153, 103560. [Google Scholar] [CrossRef]
- De Padova, D.; Meftah, M.; De Serio, F.; Mossa, M.; Sibilla, S. Characteristics of breaking vorticity in spilling and plunging waves investigated numerically by SPH. Environ. Fluid Mech. 2019, 1–28. [Google Scholar] [CrossRef]
- Amicarelli, A.; Manenti, S.; Albano, R.; Agate, G.; Paggi, M.; Longoni, L.; Mirauda, D.; Ziane, L.; Viccione, G.; Todeschini, S.; et al. SPHERA v.9.0.0: A Computational Fluid Dynamics research code, based on the Smoothed Particle Hydrodynamics mesh-less method. Comput. Phys. Commun. 2020, 250, 107157. [Google Scholar] [CrossRef]
- Monaghan, J.J. Smoothed particle hydrodynamics. Rep. Prog. Phys. 2005, 68, 1703–1759. [Google Scholar] [CrossRef]
- Monaghan, J.J. Smoothed Particle Hydrodynamics. Annu. Rev. Astron. Astrophys. 1992, 30, 543–574. [Google Scholar] [CrossRef]
- Wendland, H. Piecewise polynomial, positive definite and compactly supported radial basis functions of minimal degree. Adv. Comput. Math. 1995, 4, 389–396. [Google Scholar] [CrossRef]
- Molteni, D.; Colagrossi, A. A simple procedure to improve the pressure evaluation in hydrodynamic context using the SPH. Comput. Phys. Commun. 2009, 180, 861–872. [Google Scholar] [CrossRef]
- Antuono, M.; Colagrossi, A.; Marrone, S. Numerical diffusive terms in weakly-compressible SPH schemes. Comput. Phys. Commun. 2012, 183, 2570–2580. [Google Scholar] [CrossRef]
- Fourtakas, G.; Dominguez, J.M.; Vacondio, R.; Rogers, B.D. Local uniform stencil (LUST) boundary condition for arbitrary 3-D boundaries in parallel smoothed particle hydrodynamics (SPH) models. Comput. Fluids 2019, 190, 346–361. [Google Scholar] [CrossRef]
- Leimkuhler, B.; Reich, S.; Zentrum, K.; Str, H.; Skeel, R. Integration Methods for Molecular Dynamics. In Mathematical Approaches to Biomolecular Structure and Dynamics; Springer: New York, NY, USA, 1995; Volume 82. [Google Scholar] [CrossRef]
- Monaghan, J.J.; Cas, R.A.F.; Kos, A.M.; Hallworth, M. Gravity currents descending a ramp in a stratified tank. J. Fluid Mech. 1999, 379, 39–69. [Google Scholar] [CrossRef]
- Monaghan, J.; Kos, A.; Issa, N. Fluid Motion Generated by Impact. J. Waterw. Port Coast. Ocean Eng. 2003, 129, 250–259. [Google Scholar] [CrossRef]
- Canelas, R.B.; Domínguez, J.M.; Crespo, A.J.; Gómez-Gesteira, M.; Ferreira, R.M. A Smooth Particle Hydrodynamics discretization for the modelling of free surface flows and rigid body dynamics. Int. J. Numer. Methods Fluids 2015, 78, 581–593. [Google Scholar] [CrossRef]
- Crespo, A.; Gómez-Gesteira, M.; Dalrymple, R. Boundary conditions generated by dynamic particles in SPH methods. Comput. Mater. Contin. 2007, 5, 173–184. [Google Scholar]
- Liu, M.; Liu, G. Restoring particle consistency in smoothed particle hydrodynamics. Appl. Numer. Math. 2006, 56, 19–36. [Google Scholar] [CrossRef]
- Tafuni, A.; Domínguez, J.; Vacondio, R.; Crespo, A. A versatile algorithm for the treatment of open boundary conditions in Smoothed particle hydrodynamics GPU models. Comput. Methods Appl. Mech. Eng. 2018, 342. [Google Scholar] [CrossRef]
- Novak, G.; Tafuni, A.; Domínguez, J.; Četina, M.; Žagar, D. A Numerical Study of Fluid Flow in a Vertical Slot Fishway with the Smoothed Particle Hydrodynamics Method. Water 2019, 11, 1928. [Google Scholar] [CrossRef]
- Team, P.D. Chrono: An Open Source Framework for the Physics-Based Simulation of Dynamic Systems. Available online: https://github.com/projectchrono/chrono (accessed on 7 May 2020).
- Canelas, R.; Brito, M.; Feal, O.; Domínguez, J.; Crespo, A. Extending DualSPHysics with a Differential Variational Inequality: Modeling fluid-mechanism interaction. Appl. Ocean Res. 2018, 76, 88–97. [Google Scholar] [CrossRef]
- De Luca, F.; Pensa, C. The Naples warped hard chine hulls systematic series. Ocean Eng. 2017, 139, 205–236. [Google Scholar] [CrossRef]
- Gomez-Gesteira, M.; Rogers, B.; Crespo, A.; Dalrymple, R.; Narayanaswamy, M.; Dominguez, J. SPHysics – development of a free-surface fluid solver – Part 1: Theory and formulations. Comput. Geosci. 2012, 48, 289–299. [Google Scholar] [CrossRef]
- Duarte, H.; Droguett, E.; Ramos Martins, M.; Lützhöft, M.; Pereira, P.; Lloyd, J. Review of practical aspects of shallow water and bank effects. Int. J. Marit. Eng. 2016, 158, 177–186. [Google Scholar] [CrossRef]
- Rota Roselli, R.A.; Vernengo, G.; Altomare, C.; Brizzolara, S.; Bonfiglio, L.; Guercio, R. Ensuring numerical stability of wave propagation by tuning model parameters using genetic algorithms and response surface methods. Environ. Model. Softw. 2018, 103, 62–73. [Google Scholar] [CrossRef]
- Savitsky, D.; Morabito, M. Origin and Characteristics of the Spray Patterns Generated by Planing Hulls. J. Ship Prod. Des. 2011, 27, 63–83. [Google Scholar] [CrossRef]
- Savitsky, D.; DeLorme, M.; Datla, R. Inclusion of Whisker Spray Drag in Performance Prediction Method for High-Speed Planing Hulls. Mar. Technol. 2007, 44, 35–56. [Google Scholar]
- Verbrugghe, T.; Domínguez, J.; Altomare, C.; Tafuni, A.; Vacondio, R.; Troch, P.; Kortenhaus, A. Non-linear wave generation and absorption using open boundaries within DualSPHysics. Comput. Phys. Commun. 2019, 240, 46–59. [Google Scholar] [CrossRef]
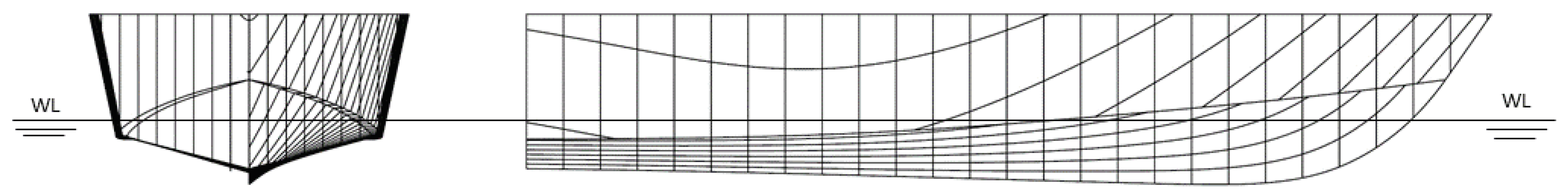

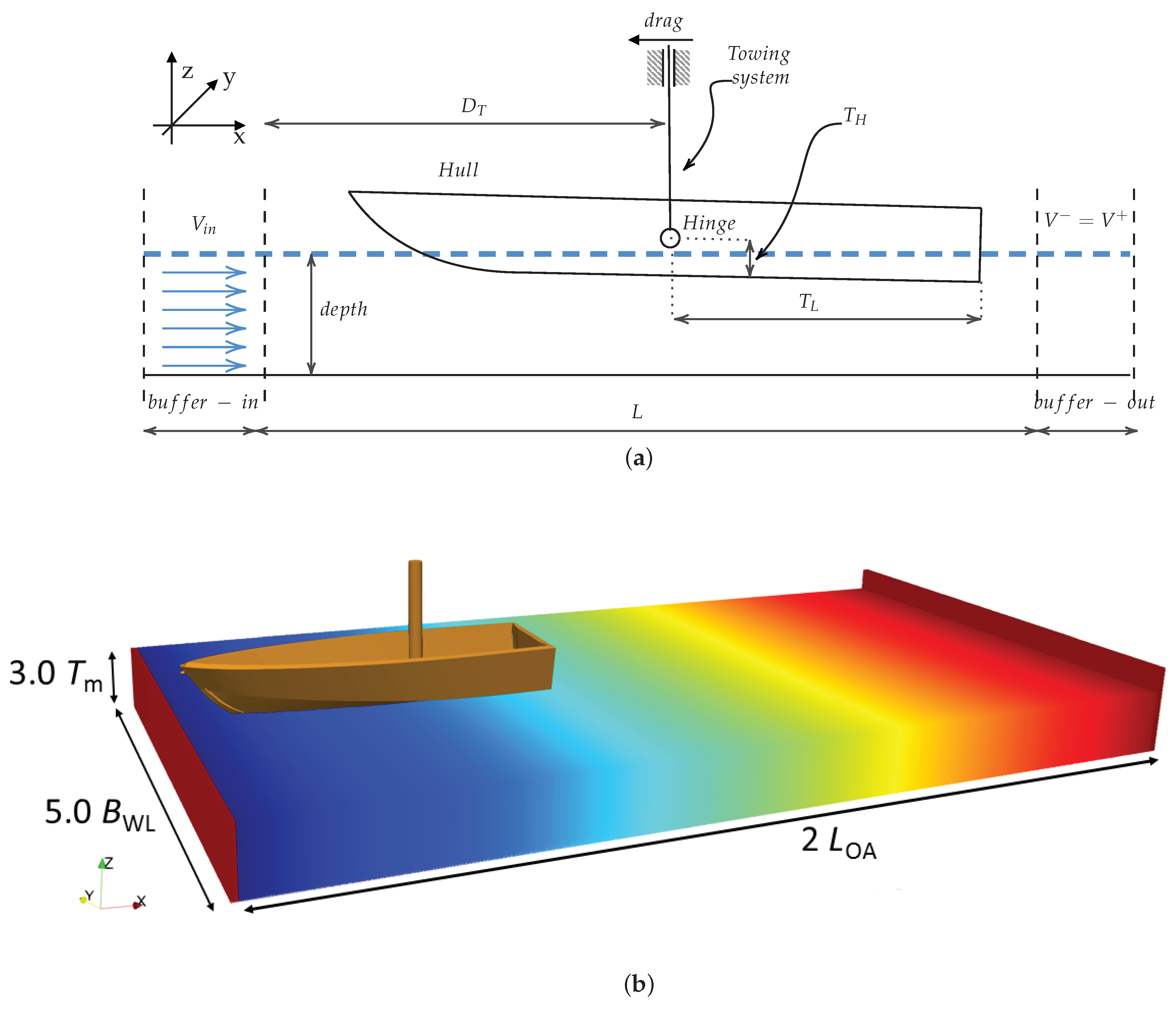
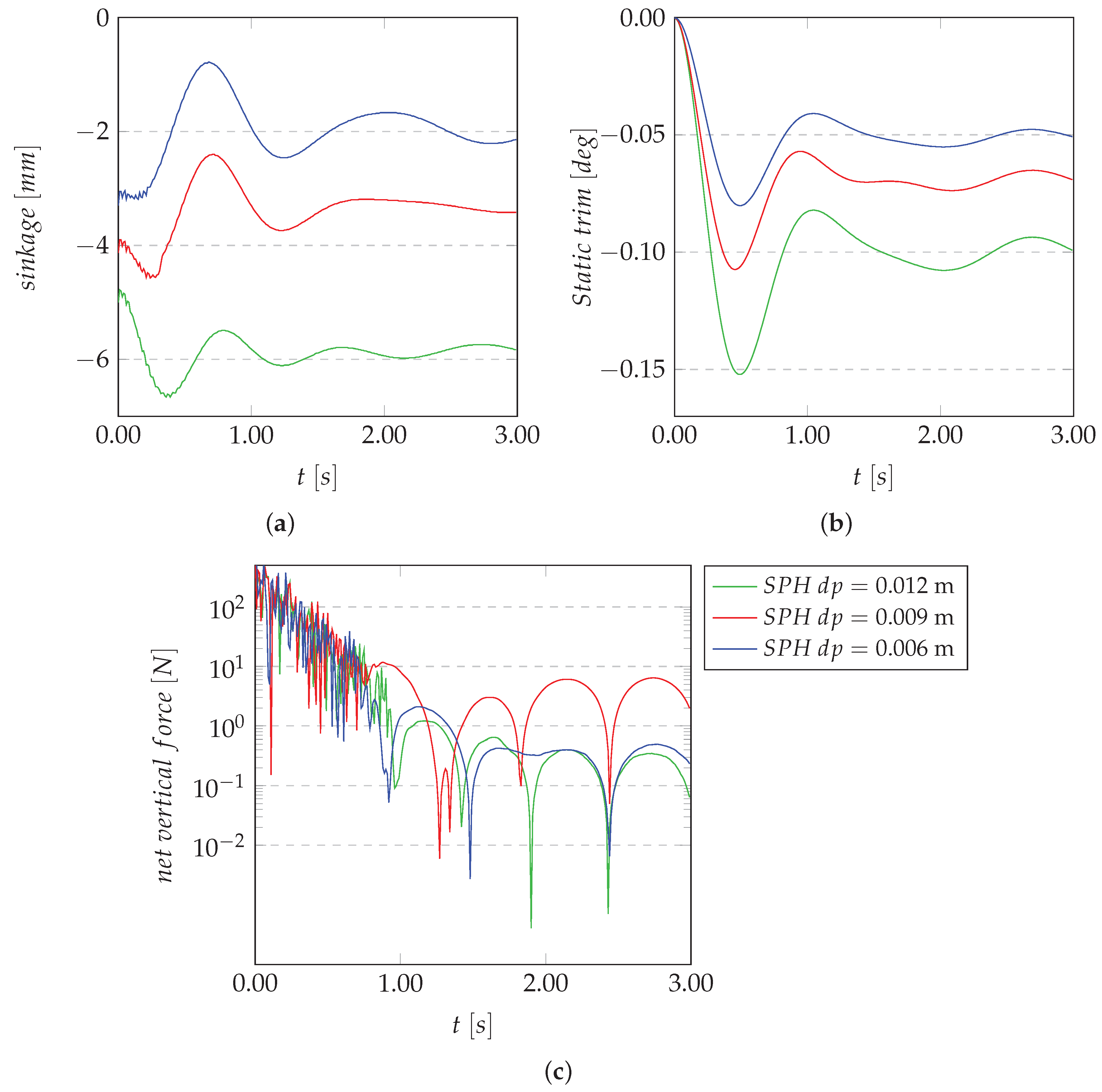
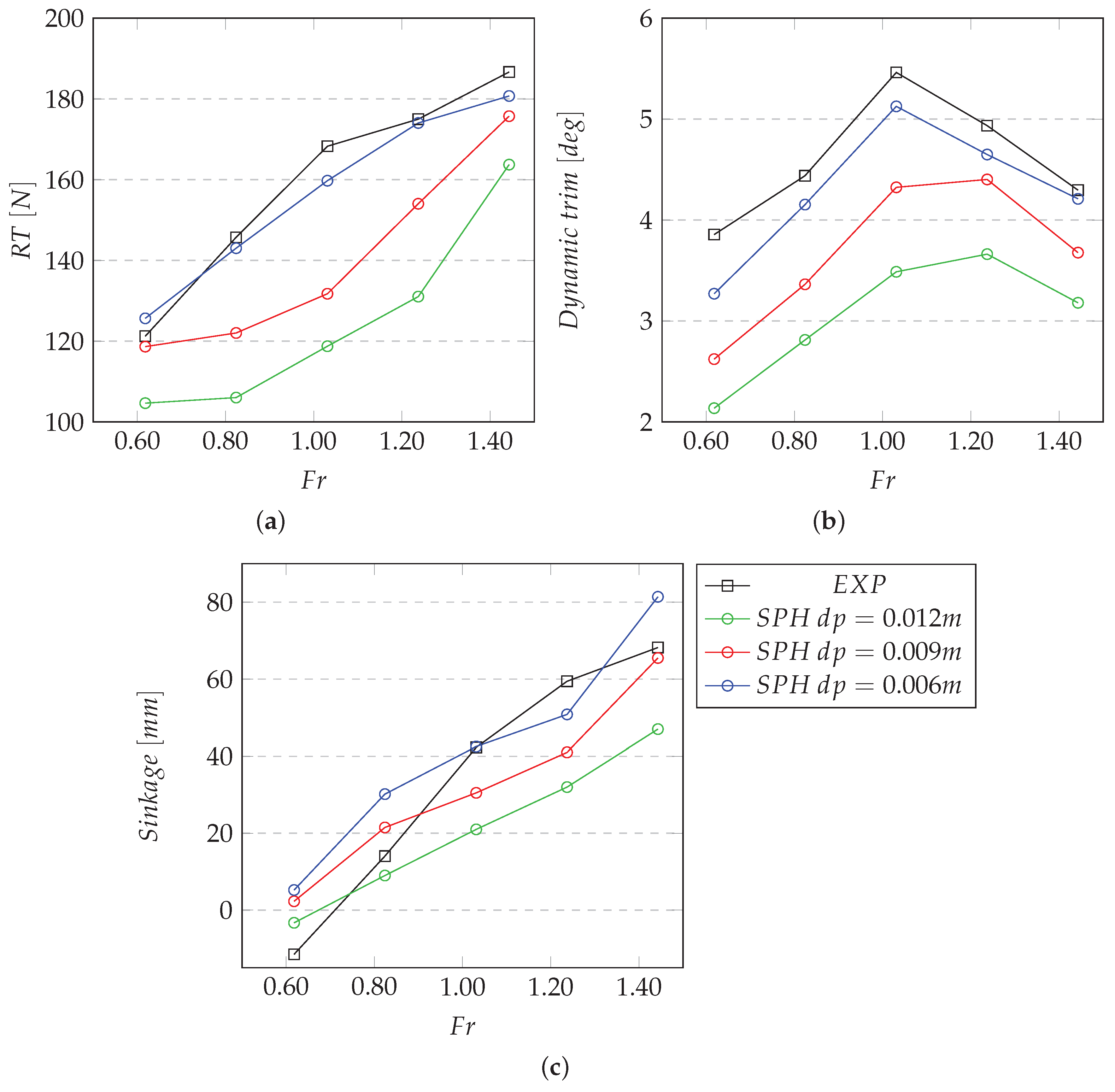
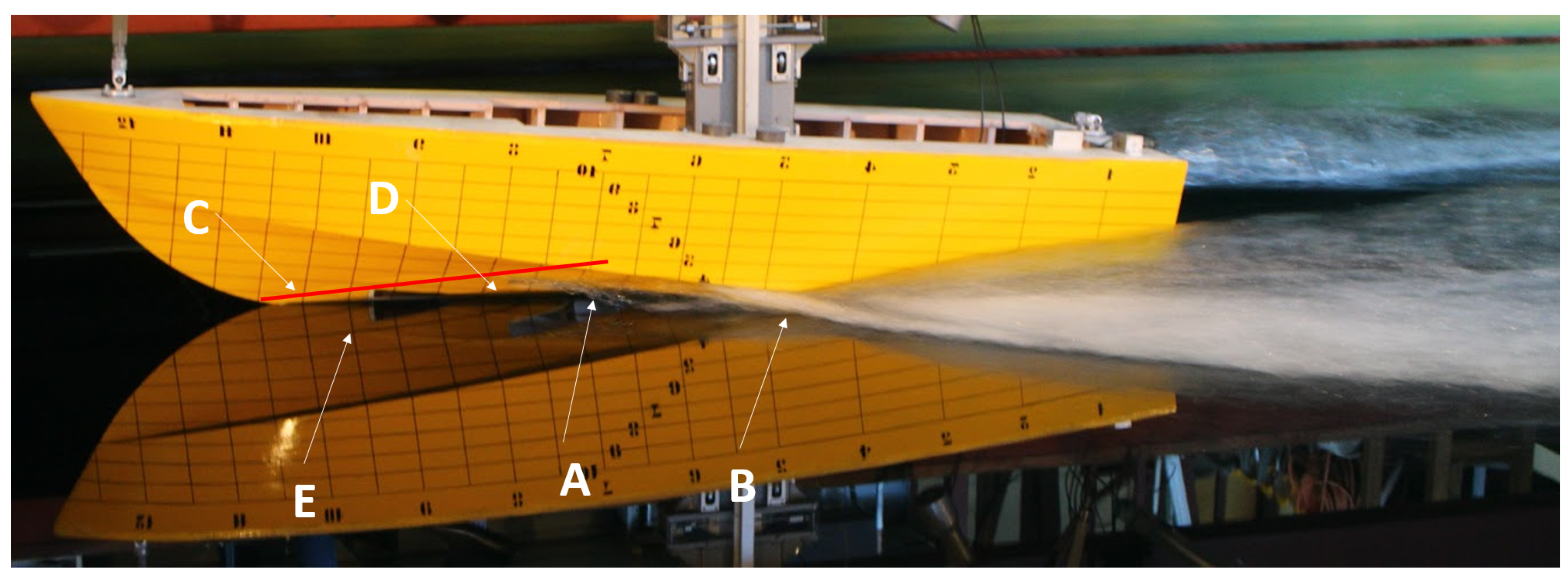
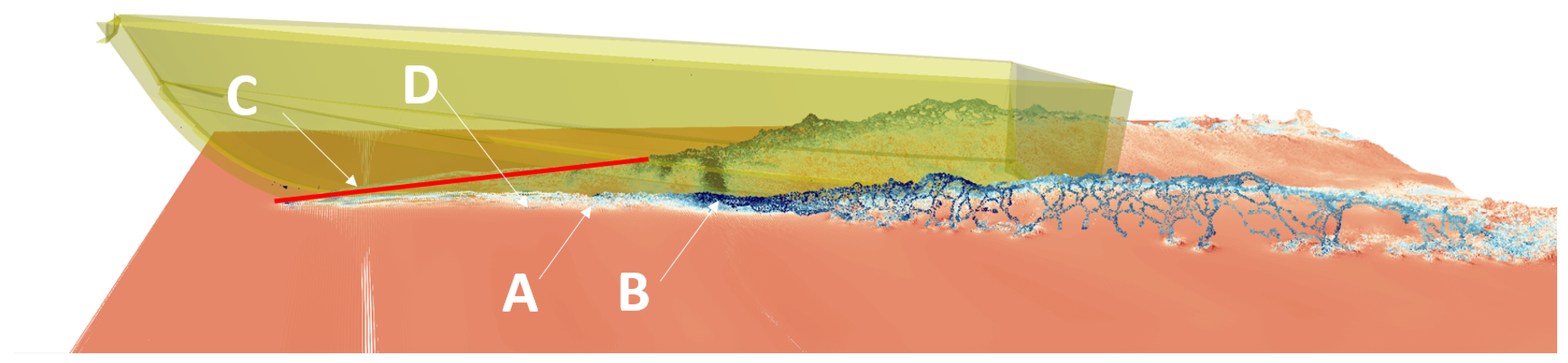
| Authors | Year | Type of Study | Type of High-Speed Craft | Test Conditions | V&V * |
|---|---|---|---|---|---|
| Thornhill et al. [5] | 2003 | Experimental | Prismatic planing hull | Still water | no |
| Begovic and Bertorello [6] | 2012 | Experimental | Prismatic and warped planing hull | Still water | no |
| Matveev [7] | 2014 | Analytical | Warped planing hull | Still water | no |
| Sukas et al. [8] | 2015 | Experimental and Numerical | Prismatic and Warped planing hull | Still water | yes |
| Jiang et al. [9] | 2016 | Experimental and Numerical | Planing trimaran hull | Still water | yes |
| De Marco et al. [10] | 2017 | Experimental and Numerical | Stepped hull | Still water | yes |
| Niazmand Bilandi et al. [11] | 2018 | Analytical and Experimental | Stepped hull | Still water | no |
| Tavakoli et al. [12] | 2020 | Experimental, Numerical, and Analytical | Warped planing hull | Still water, Regular waves | yes |
| Hull Dimensions | Unit | C1 Hull | |
|---|---|---|---|
| Length overall | [m] | 2.611 | |
| Length waterline | [m] | 2.400 | |
| Beam waterline | [m] | 0.743 | |
| Hull draft max | [m] | 0.167 | |
| Displacement | [kg] | 106.07 | |
| Wetted Surface | [m2] | 1.70 | |
| Static trim | [deg] | 0.0 | |
| Length to beam ratio | 3.45 | ||
| Length to volume ratio | 5.11 | ||
| Total Resistance | Dynamic Trim Angle | Dynamic Sinkage | |
|---|---|---|---|
| 0.618 | 3.52% | −17.92% | 319.73% |
| 0.824 | −1.89% | −6.89% | 53.41% |
| 1.031 | −5.34% | −6.55% | 0.53% |
| 1.237 | −0.55% | −6.15% | −16.95% |
| 1.443 | −3.29% | −2.04% | 16.20% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tagliafierro, B.; Mancini, S.; Ropero-Giralda, P.; Domínguez, J.M.; Crespo, A.J.C.; Viccione, G. Performance Assessment of a Planing Hull Using the Smoothed Particle Hydrodynamics Method. J. Mar. Sci. Eng. 2021, 9, 244. https://doi.org/10.3390/jmse9030244
Tagliafierro B, Mancini S, Ropero-Giralda P, Domínguez JM, Crespo AJC, Viccione G. Performance Assessment of a Planing Hull Using the Smoothed Particle Hydrodynamics Method. Journal of Marine Science and Engineering. 2021; 9(3):244. https://doi.org/10.3390/jmse9030244
Chicago/Turabian StyleTagliafierro, Bonaventura, Simone Mancini, Pablo Ropero-Giralda, José M. Domínguez, Alejandro J. C. Crespo, and Giacomo Viccione. 2021. "Performance Assessment of a Planing Hull Using the Smoothed Particle Hydrodynamics Method" Journal of Marine Science and Engineering 9, no. 3: 244. https://doi.org/10.3390/jmse9030244
APA StyleTagliafierro, B., Mancini, S., Ropero-Giralda, P., Domínguez, J. M., Crespo, A. J. C., & Viccione, G. (2021). Performance Assessment of a Planing Hull Using the Smoothed Particle Hydrodynamics Method. Journal of Marine Science and Engineering, 9(3), 244. https://doi.org/10.3390/jmse9030244










