Effect of Dissipation on the Moonpool-Javelin Wave Energy Converter
Abstract
:1. Introduction
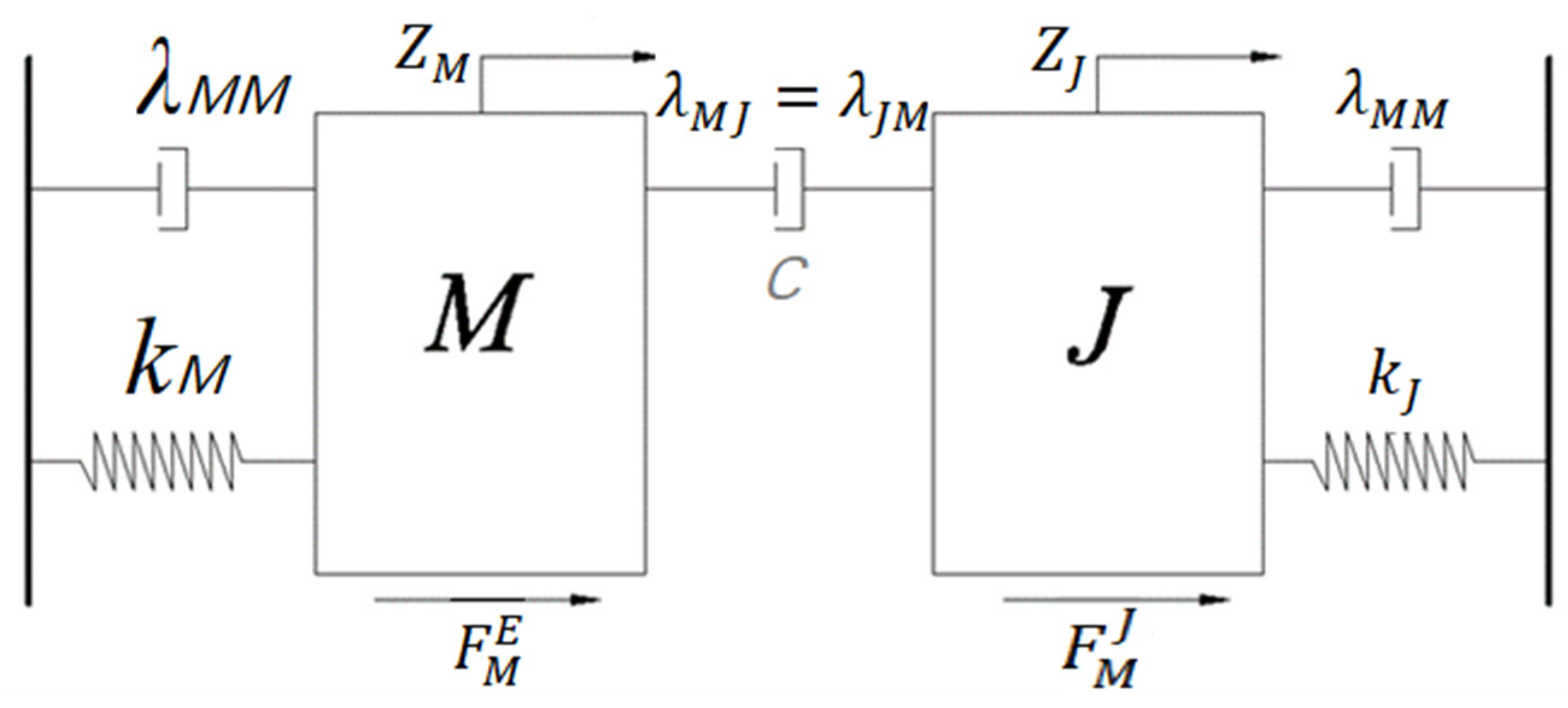
2. Mathematical and Numerical Model
2.1. Semianalytical Solution in Frequency Domain
2.1.1. Diffraction Problems
2.1.2. Radiation Problems
2.1.3. Motion Equation and Capture Width Ratio
2.2. Time-Domain Solutions
2.3. Introduce Dissipation in Potential Flow
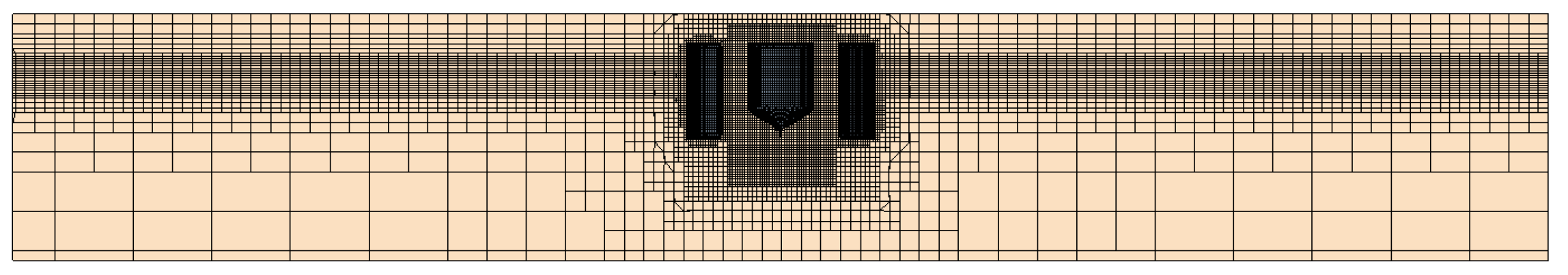
2.4. Computational Fluid Dynamics (CFD) Method
2.4.1. Reynolds Averaged Navier–Stokes (RANS) Equations
2.4.2. Computational Domain and Boundary Conditions
2.4.3. Mesh Generation
3. Numerical Results and Discussion
3.1. Dynamic Characteristic Analyses in the Frequency Domain
3.1.1. The Hydrodynamic Characteristic
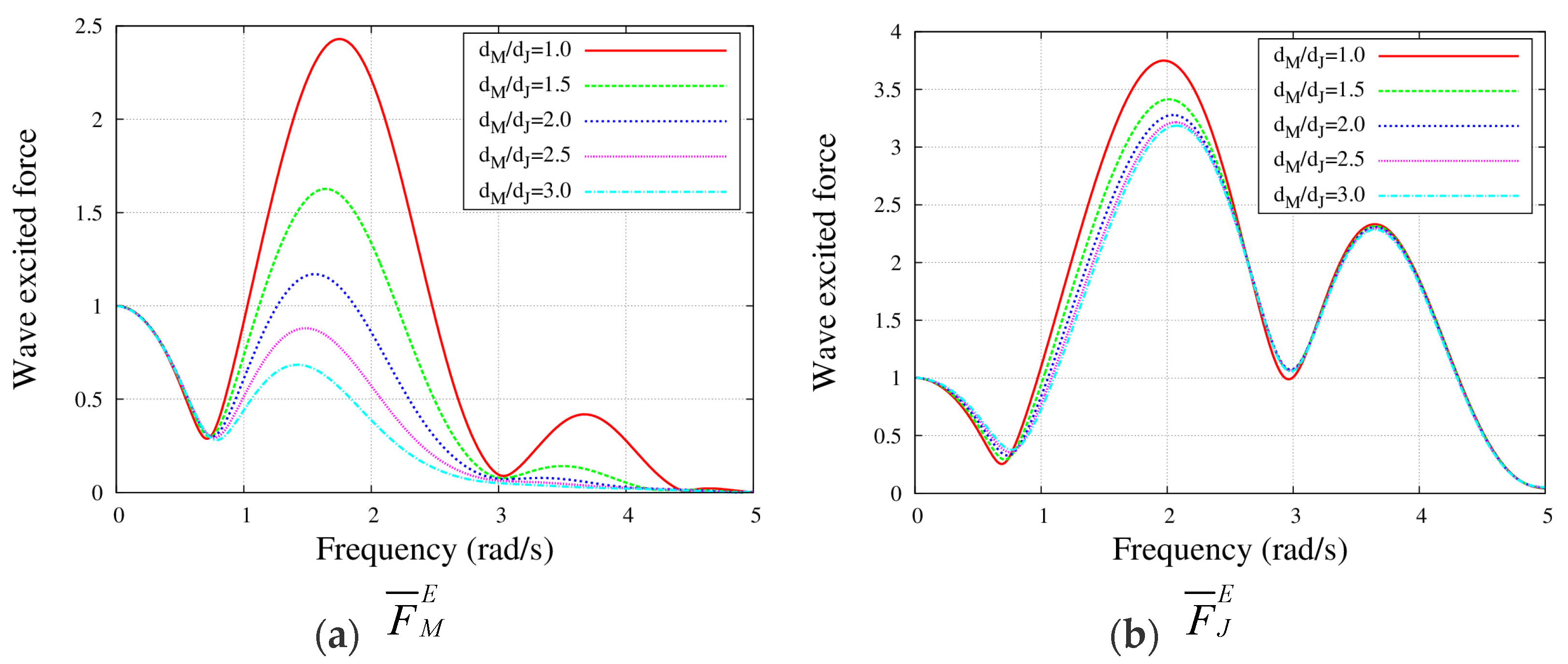
The Influence of ’s Change on the Force of the Devices
The Influence of ’s Change on the Force of the Devices
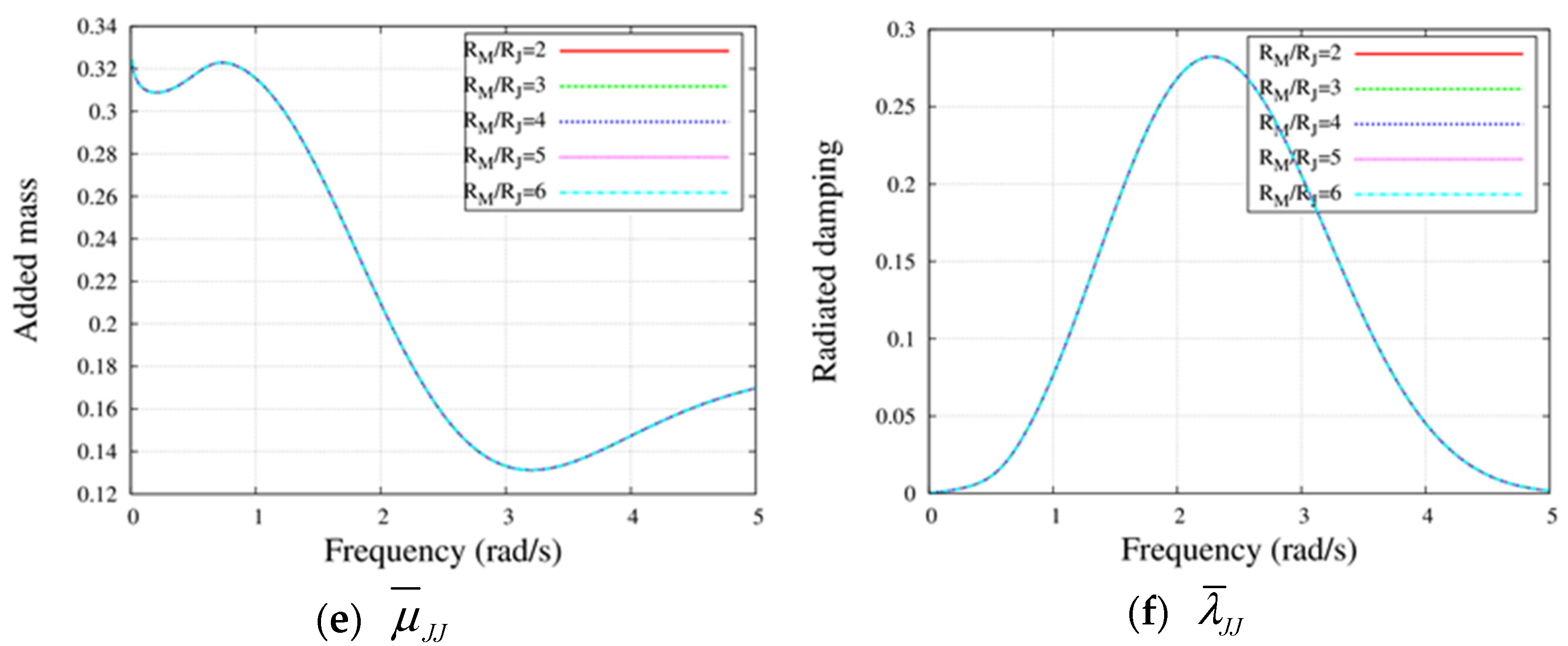
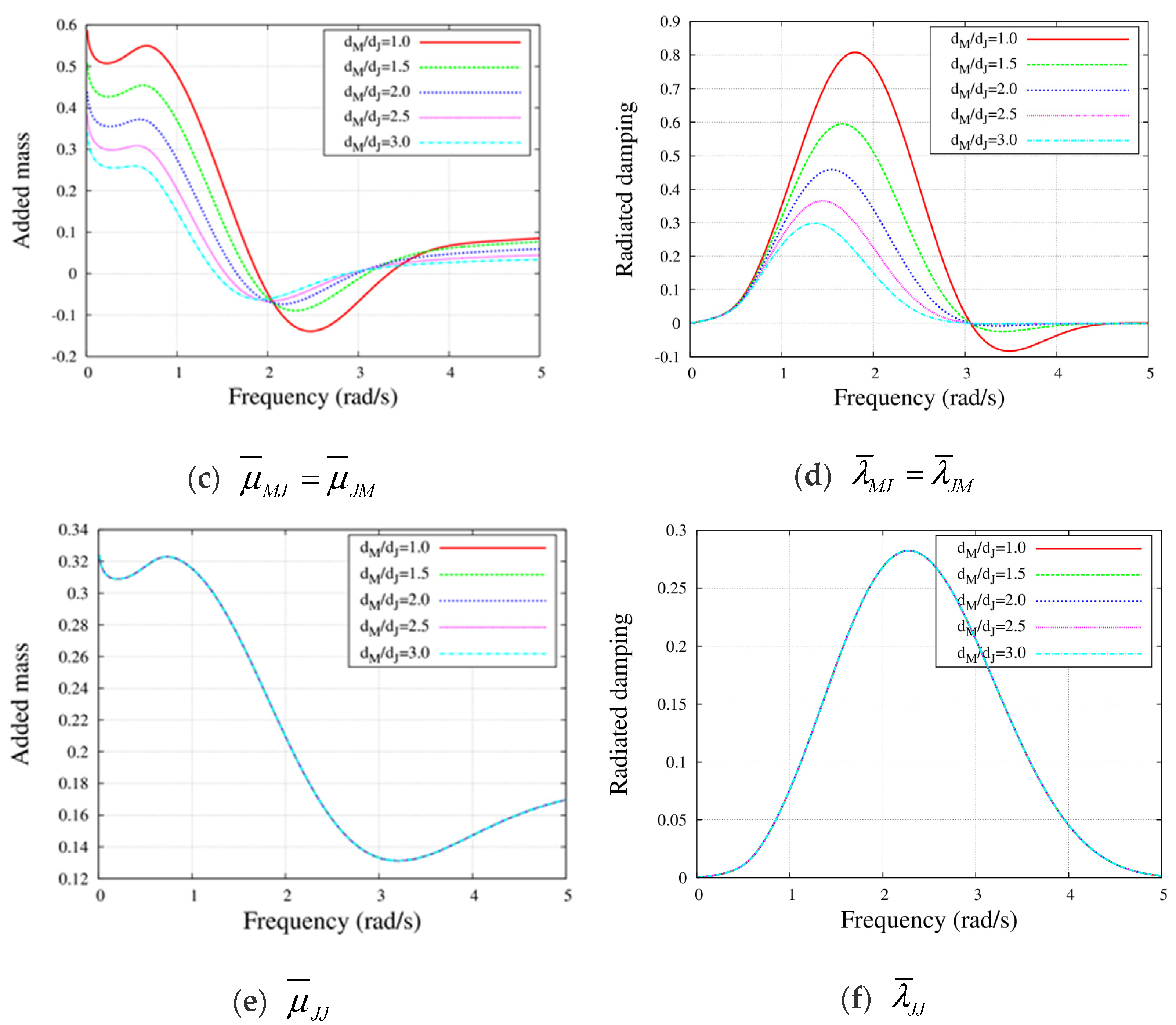
The Influence of ’s Change on Hydrodynamic Coefficients of the Devices
The Influence of ’s Change on Hydrodynamic Coefficients of the Devices
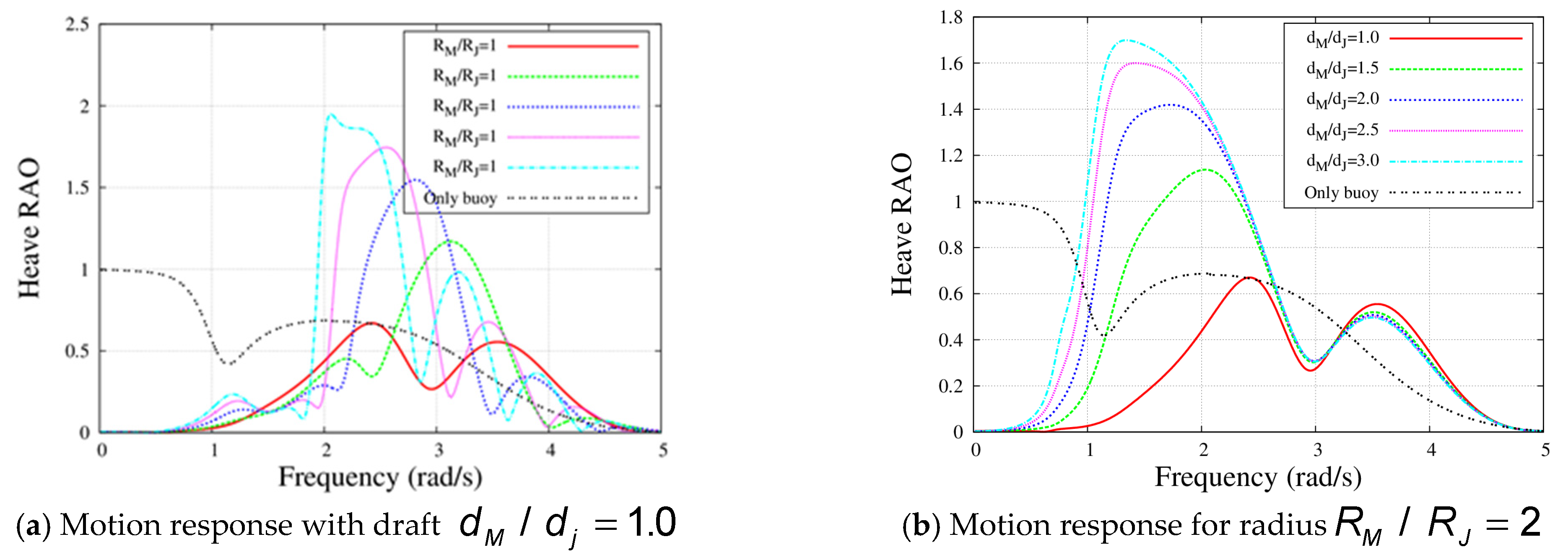
3.1.2. The Motion Response
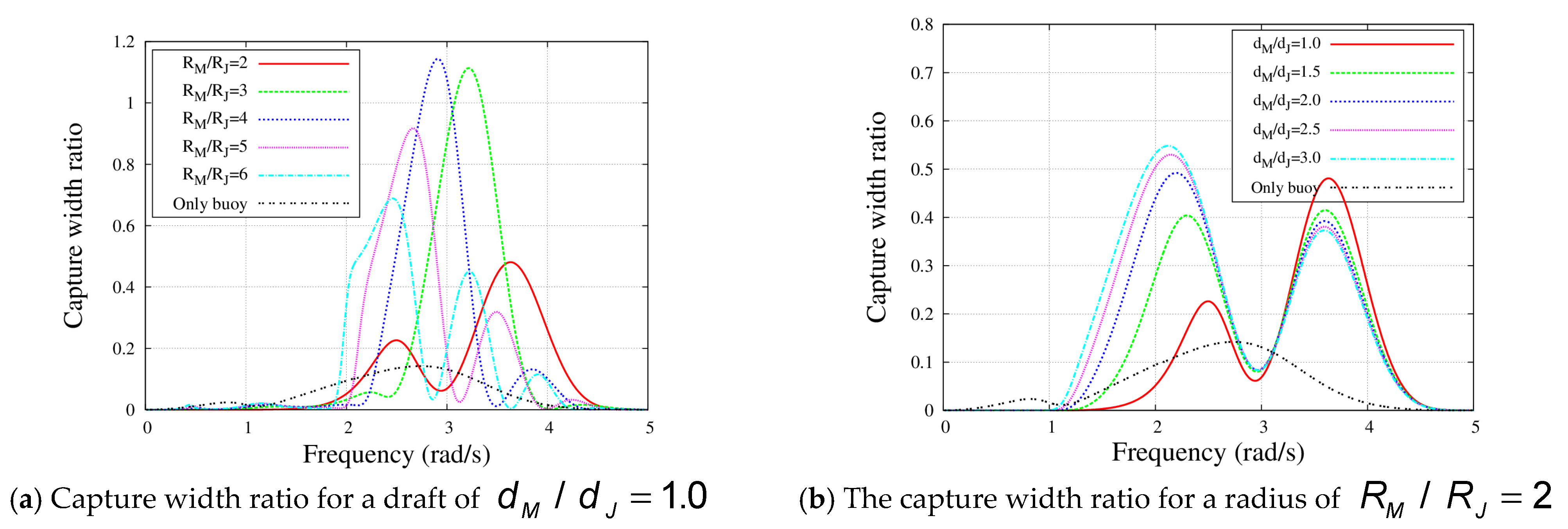
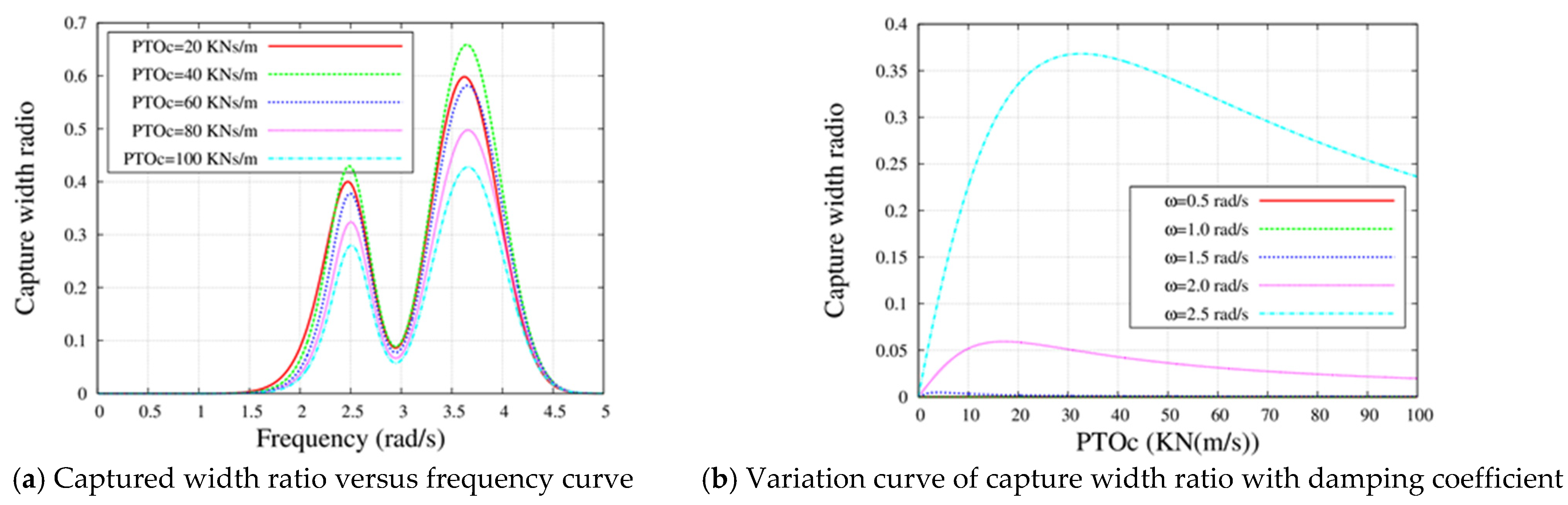
3.1.3. The Capture Width Ratio
3.2. Dynamic Characteristic Analyses in the Time Domain
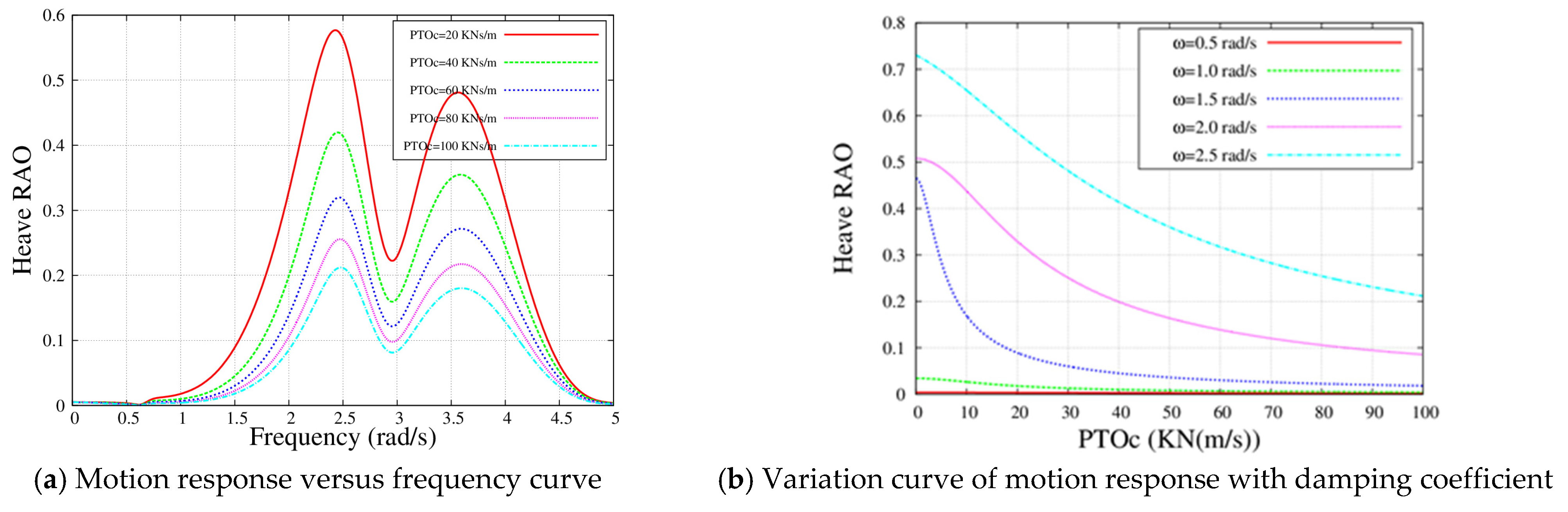
3.2.1. The Motion Response
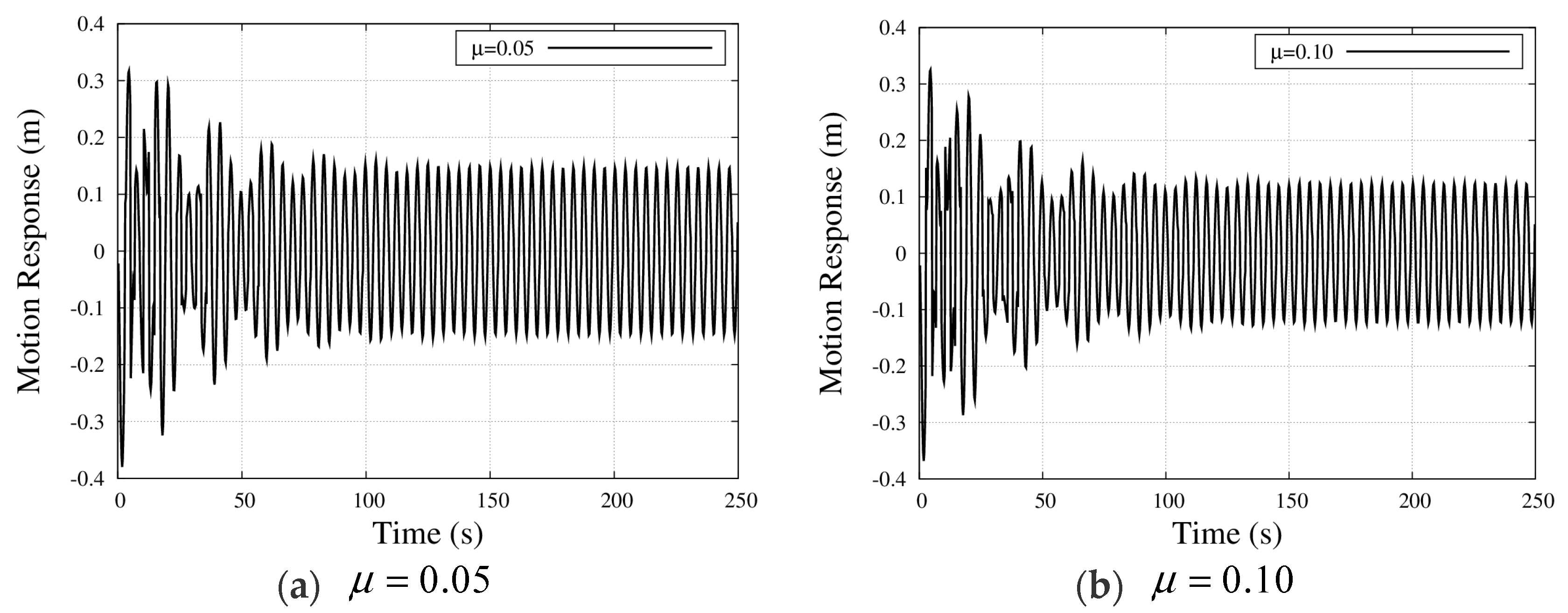
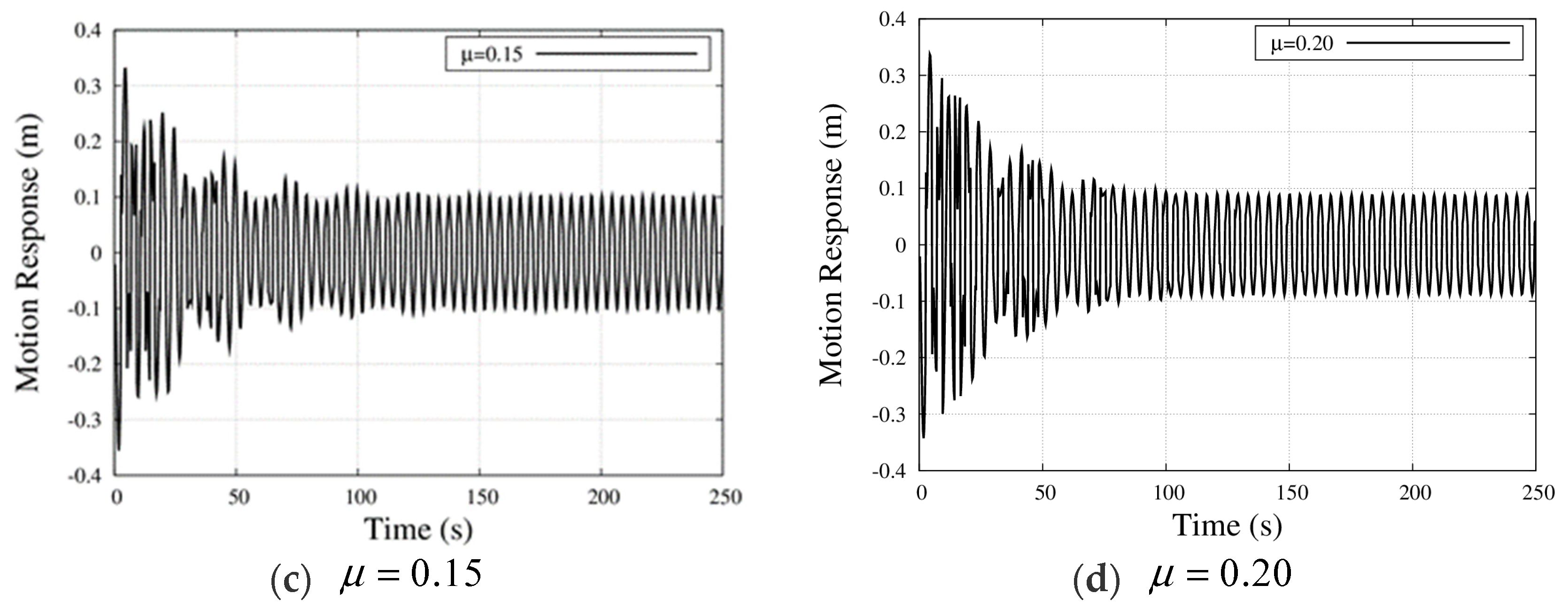
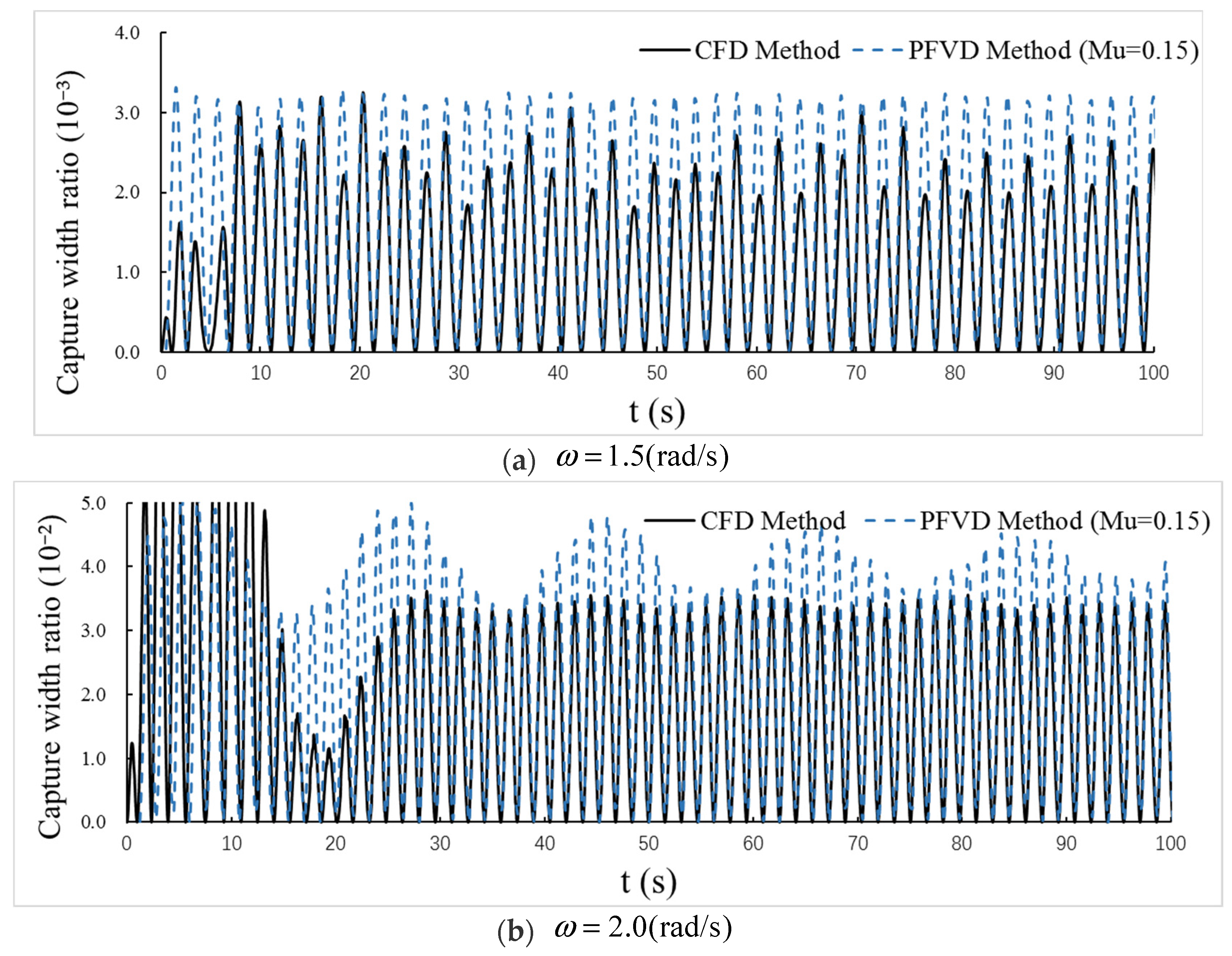
3.2.2. The Capture Width Ratio
4. Experimental Process and Results
4.1. Experimental Facility
4.2. Wave Parameter
4.3. Experimental Results
5. Conclusions
- (1)
- A comparison of axisymmetric buoys with and without a moonpool platform showed that the moonpool had an effect on the hydrodynamic coefficient of the central buoy. For the frequency-domain dynamic characteristics of the MJWEC under potential flow, when the wave frequency was , the motion and capture width ratio of the wave energy device with the moonpool platform were significantly better than those of the single javelin float.
- (2)
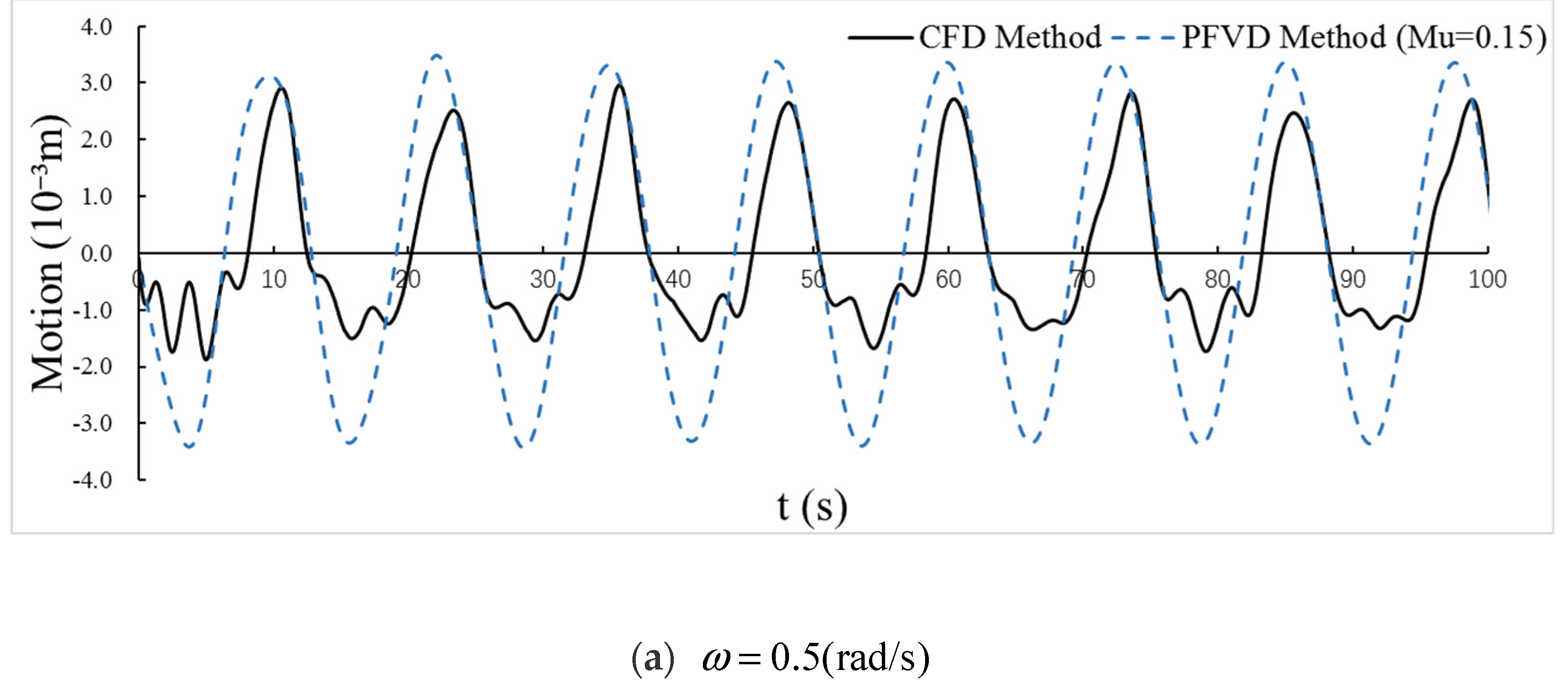
- For CFD analysis, the platform device of the moonpool did not change the wave period inside the platform of the moonpool, but it did change the wave height inside the platform, improving the motion amplitude of the float. Therefore, the platform device of the moonpool significantly improved the energy conversion quality of the whole wave energy device. The CFD calculation method and viscous dissipation method based on potential-flow theory were very close to each other in terms of the amplitude of the motion response. Comparatively speaking, the CFD calculation result was higher than the potential-flow analysis algorithm for viscous dissipation.
- (3)
- According to the analysis of the test results of the model test, according to the linear wave theory, the influence of the wave height on the motion response and power of the MJWEC was positively linear. The wave period’s effects on different devices were not the same; a single javelin float peaked at 1.6 s, while the MJWEC had two peaks at 2.0 s and 2.4 s. Contrastingly, two experiments found that for certain wave periods from 1.8 s to 2.4 s, the javelin float performed better than the MJWEC in terms of displacement and power. Considering the peak values in particular, which were two times higher for the moonpool, the moonpool platform, when applied to the engineering practice of wave energy devices, could effectively improve the efficiency of their energy conversion. By comparing the results of the viscous dissipation and the pool test, the optimal viscous dissipation coefficient was found to be suitable.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rademakers, L.W.; Van Schie, R.G.; Schuitema, R.; Vriesema, B.; Gardner, F. Physical Model Testing for Characterising the AWS; Netherlands Energy Research Foundation ECN: Petten, The Netherlands, 1998. [Google Scholar]
- Penesis, I.; Manasseh, R.; Nader, J.R.; de Chowdhury, S.; Fleming, A.; Macfarlane, G.; Hasan, M.K. Performance of ocean wave-energy arrays in Australia. In Proceedings of the 3rd AsianWave and Tidal Energy Conference (AWTEC 2016), Singapore, 24–28 October 2016; Volume 1, pp. 246–253. [Google Scholar]
- Cleason, L.; Forsberg, J.; Rylander, A. Contribution to the theory and experience of energy production and transmission from the buoy-concept. In Proceedings of the 2nd International Symposium on Wave Energy Utilization, Trondheim, Norway, 22–24 June 1982; pp. 345–370. [Google Scholar]
- Muliawan, M.J.; Gao, Z.; Moan, T. Analysis of a two-body floating wave energy converter with particular focus on the effects of power take-off and mooring systems on energy capture. J. Offshore Mech. Arct. Eng. 2013, 135, 317–328. [Google Scholar] [CrossRef]
- Ramadan, A.; Mohamed, M.H.; Abdien, S.M.; Marzouk, S.Y.; El Feky, A.; El Baz, A.R. Analytical investigation and experimental validation of an inverted cup float used for wave energy conversion. Energy 2014, 70, 539–546. [Google Scholar] [CrossRef]
- Mavrakos, S.A.; Katsaounis, G.M. Effects of floaters’ hydrodynamics on the performance of tightly moored wave energy converters. IET Renew. Power Gener. 2009, 4, 531–544. [Google Scholar] [CrossRef]
- Zang, Z.; Zhang, Q.; Qi, Y.; Fu, X. Hydrodynamic responses and efficiency analyses of a heaving-buoy wave energy converter with PTO damping in regular and irregular waves. Renew. Energy 2018, 116, 527–542. [Google Scholar] [CrossRef]
- Duan, W.Y.; Liu, H.X.; Li, R.P.; Chen, X.B. Wave loading on a group of cylinders in fairly perfect fluid[C] 10th International Conference on Hydrodynamics. Petersburg 2012, 10, 178–183. [Google Scholar]
- Chen, X.B.; Liu, H.X.; Duan, W.Y. Semi-analytical solutions to wave diffraction of cylindrical structures with a moonpool with a restricted entrance. J. Eng. Math. 2015, 90, 51–66. [Google Scholar] [CrossRef]
- Liu, H.X.; Chen, H.L.; Zhang, L.; Zhang, W.C.; Liu, M. Quadratic Dissipation Effect on the Moonpool Resonance. China Ocean Eng. 2017, 31, 665–673. [Google Scholar] [CrossRef]
- Lo, D.C.; Hsu, T.; Yang, C. Hydrodynamic Performances of Wave Pass Two Buoys-Type Wave Energy Converter. In Proceedings of the 27th International Ocean and Polar Engineering Conference, San Francisco, CA, USA, 25–30 June 2016; pp. 25–30. [Google Scholar]
- Jin, S.; Patton, R.J.; Guo, B. Viscosity effect on a point absorber wave energy converter hydrodynamics validated by simulation and experiment. Renew. Energy 2018, 129, 500–512. [Google Scholar] [CrossRef]
- Ren, N.; Ma, Z.; Fan, T.; Zhai, G.; Ou, J. Experimental and numerical study of hydrodynamic responses of a new combined monopile wind turbine and a heave-type wave energy converter under typical operational conditions. Ocean Eng. 2018, 159, 1–8. [Google Scholar] [CrossRef]
- Gao, Z.; Moan, T.; Wan, L.; Michailides, C. Comparative numerical and experimental study of two combined wind and wave energy concepts. J. Ocean Eng. Sci. 2015, 1, 36–51. [Google Scholar] [CrossRef] [Green Version]
- Wan, L.; Greco, M.; Lugni, C.; Gao, Z.; Moan, T. A combined wind and wave energy-converter concept in survival mode: Numerical and experimental study in regular waves with a focus on water entry and exit. Appl. Ocean Res. 2017, 63, 200–216. [Google Scholar] [CrossRef]
- Wan, L.; Gao, Z.; Moan, T. Experimental and numerical study of hydrodynamic responses of a combined wind and wave energy converter concept in survival modes. Coast. Eng. 2015, 104, 151–169. [Google Scholar] [CrossRef] [Green Version]
- Wan, L.; Gao, Z.; Moan, T.; Lugni, C. Experimental and numerical comparisons of hydrodynamic responses for a combined wind and wave energy converter concept under operational conditions. Renew Energy 2016, 93, 87–100. [Google Scholar] [CrossRef]
- Madhi, F.; Sinclair, M.E.; Yeung, R.W. The “Berkeley Wedge”: An asymmetrical energy-capturing floating breakwater of high performance. Mar. Syst. Ocean Technol. 2014, 9, 5–16. [Google Scholar] [CrossRef]
- Chen, X.B.; Dias, F. Visco-potential flow and time-harmonic ship waves. In Proceedings of the 25th International Workshop on Water Waves and Floating Bodies, Harbin, China, 9–12 May 2020. CNKI:SUN:HEBD.0.2010-01-011. [Google Scholar]











| Number | Height (m) | Period (s) | Number | Height (m) | Period (s) |
|---|---|---|---|---|---|
| 1 | 0.12 | 1.2 | 8 | 0.12 | 2.3 |
| 2 | 0.12 | 1.4 | 9 | 0.12 | 2.4 |
| 3 | 0.12 | 1.6 | 10 | 0.12 | 2.5 |
| 4 | 0.12 | 1.8 | 11 | 0.12 | 2.6 |
| 5 | 0.12 | 2.0 | 12 | 0.12 | 2.7 |
| 6 | 0.12 | 2.1 | 13 | 0.12 | 2.8 |
| 7 | 0.12 | 2.2 | 14 | 0.12 | 3.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, D.; Wang, K.; Jin, Y.; Kong, F.; Chen, H.; Yang, C. Effect of Dissipation on the Moonpool-Javelin Wave Energy Converter. J. Mar. Sci. Eng. 2021, 9, 1444. https://doi.org/10.3390/jmse9121444
Yu D, Wang K, Jin Y, Kong F, Chen H, Yang C. Effect of Dissipation on the Moonpool-Javelin Wave Energy Converter. Journal of Marine Science and Engineering. 2021; 9(12):1444. https://doi.org/10.3390/jmse9121444
Chicago/Turabian StyleYu, Dan, Keyi Wang, Yeqing Jin, Fankai Kong, Hailong Chen, and Can Yang. 2021. "Effect of Dissipation on the Moonpool-Javelin Wave Energy Converter" Journal of Marine Science and Engineering 9, no. 12: 1444. https://doi.org/10.3390/jmse9121444
APA StyleYu, D., Wang, K., Jin, Y., Kong, F., Chen, H., & Yang, C. (2021). Effect of Dissipation on the Moonpool-Javelin Wave Energy Converter. Journal of Marine Science and Engineering, 9(12), 1444. https://doi.org/10.3390/jmse9121444






