Study on Different Parameters of the Self-Excited Oscillation Nozzle for Cavitation Effect under Multiphase Mixed Transport Conditions
Abstract
:1. Introduction
2. Simulation and Experiment
2.1. Model Selection
2.2. Parameter Determination
2.3. Criteria
2.3.1. Simulation Criteria
2.3.2. Experiment Criteria
2.4. Experimental Materials and Devices
3. Results and Discussion
3.1. Simulation Results of Self-Excited Oscillating Cavitation Jet Nozzle
3.2. Correlation Analysis of Structural Parameters
3.3. Partial Correlation Analysis of Solid Particles
3.4. Construction of Mathematical Model under Multiphase Transport
3.5. Model Validation
4. Conclusions
- (1)
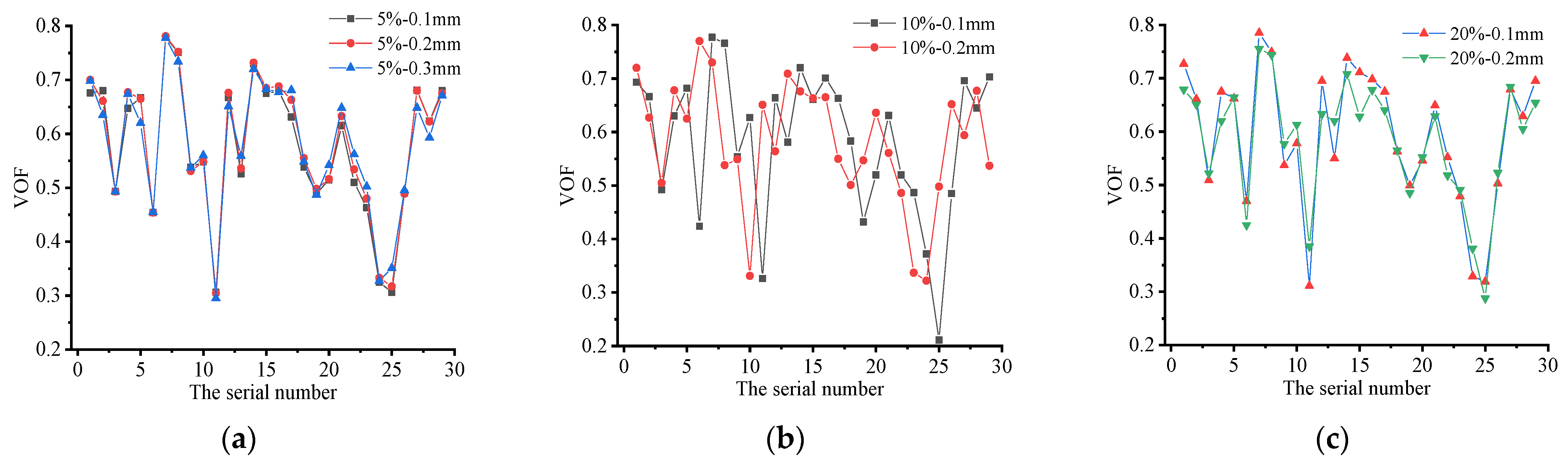
- When the content of solid particles was 5% and 10%, VOF increases with the increase in the particle diameter. When the content of solid particles was 20%, VOF decreases first and then increases with the increase in the particle diameter;
- (2)
- Cavitation performance of self-excited oscillating cavitation jet nozzle is affected by design parameters. Under the identical particle diameter, the larger the content of solid particles, the less the effect of design parameters will exert on VOF;
- (3)
- The correlation analysis of the respective design parameter revealed that its influence on vapor volume fraction was CLD > d1 > Cd21 > CDd2 > Pin;
- (4)
- The effect of Cd21 on VOF is obvious with particle diameter, while the influence of other design parameters on VOF is insignificant with particle diameter;
- (5)
- When the solid particle content was 10% and the particle diameter was 0.2 mm, an independent operating point would be generated. At this point, VOF was slightly correlated with VOF in other media;
- (6)
- A novel VOFnew with different medium parameters is determined, which could express the variation of vapor volume fraction under different medium parameters and imply the contribution rate of the respective parameter to cavitation performance. It has been verified experimentally that the slope of test fitting is basically identical to the corresponding coefficient of parameters in the model, and the model could be reasonable.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| PCA | Principal component analysis |
| CLD | Ratio of the self-excited oscillation cavitating jet nozzle cavity length L and the cavity diameter D |
| d1 | Inlet diameter |
| Cd21 | Ratio of outlet diameter to inlet diameter |
| CDd2 | Ratio of cavity diameter D and the self-excited oscillation cavitating jet nozzle outlet diameter d2 |
| Pin | Inlet pressure |
| VOF | Volume fraction of cavitation vapor |
| d2 | Outlet diameter |
| Drift speed in the kth term | |
| Density of the mixture | |
| Velocity of the mixture | |
| Volume force | |
| D | Diameter of the cavity |
| L | Length of the cavity |
| α | Impact angle of the cavity |
| w | Mass loss rate of cavitation specimen |
| m0 | Mass of specimen before cavitation erosion |
| m | Mass of specimen after cavitation erosion |
| r(X,VOF) | Partial correlation coefficient between parameter X and vapor volume fraction |
| VOFi | Vapor volume fraction under different media conditions |
| VOFnew | A new mathematical model of vapor volume fraction |
References
- Peng, Z.; Liu, X.J.; Zhang, W.; Zeng, Z.T.; Liu, Z.F.; Zhang, C.; Liu, Y.; Shao, B.B.; Liang, Q.H.; Tang, W.W.; et al. Advances in the application, toxicity and degradation of carbon nanomaterials in environment: A review. Environ. Int. 2020, 134, 105298. [Google Scholar] [CrossRef]
- Zhang, X. Study on treatment of phenolic wastewater by microbial degradation. Fresenius Environ. Bull. 2020, 29, 129–1133. [Google Scholar]
- An, H.X.; Li, X.M.; Yang, Q.; Wang, D.B.; Xie, T.; Zhao, J.W.; Xu, Q.X.; Chen, F.; Zhong, Y.; Yuan, Y.J.; et al. The behavior of melamine in biological wastewater treatment system. J. Hazard. Mater. 2017, 322, 445–453. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Liu, S.; Ji, H. Mechanism of rock breaking by pick assisted with water jet of different modes. J. Mech. Sci. Technol. 2015, 29, 5359–5368. [Google Scholar] [CrossRef]
- Huang, L.; Kinnell, P.; Shipway, P.H. Removal of heat-formed coating from a titanium alloy using high pressure waterjet: Influence of machining parameters on surface texture and residual stress. J. Mater. Process. Technol. 2015, 223, 129–138. [Google Scholar] [CrossRef] [Green Version]
- Careddu, N.; Akkoyun, O. An investigation on the efficiency of water-jet technology for graffiti cleaning. J. Cult. Herit. 2016, 19, 426–434. [Google Scholar] [CrossRef]
- Jiang, H.; Du, C.; Dong, J. Investigation of rock cutting dust formation and suppression using water jets during mining. Powder Technol. 2017, 307, 99–108. [Google Scholar] [CrossRef]
- Den Dunnen, S.; Dankelman, J.; Kerkhoffs, G.M.; Tuijthof, G. Colliding jets provide depth control for water jetting in bone tissue. J. Mech. Behav. Biomed. Mater. 2017, 72, 219–228. [Google Scholar] [CrossRef] [PubMed]
- Shen, Z.; Li, G.; Wang, Z.M.; Hou, F.S. New jet theory and prospects of application in drilling engineering. In Proceedings of the 13th World Petroleum Congress, Buenos Aires, Argentina, 20–25 October 2021; pp. 397–405. [Google Scholar]
- Johnson, V.E.J.; Chahine, G.L.; Lindenmuth, W.T.; Conn, A.F.; Frederick, G.S.; Giacchino, G.J. The development of structured cavitating jet for deep hole bits. SPE 1982, 11060, 1–8. [Google Scholar]
- Peng, C.; Tian, S.C.; Li, G.S.; Wei, M.H. Enhancement of cavitation intensity and erosion ability of submerged cavitation jet by adding micro-particles. Ocean Eng. 2020, 209, 107516. [Google Scholar] [CrossRef]
- Xu, W.L.; Zhang, Y.L.; Luo, J.; Arong; Zhang, Q.; Zhai, Y.W. The impact of particles on the collapse characteristics of cavitation bubbles. Ocean Eng. 2017, 131, 15–24. [Google Scholar] [CrossRef]
- Gensheng, L.; Zhonghou, S. Experimental study on pressure pulsation of self-resonating cavitating water jet. J. Univ. Pet. 1996, 5, 35–39. [Google Scholar]
- Li, G.S.; Shen, H.Z.; Zhou, C.S.; Zhang, D.B.; Yi, C. An experimental study on impact pressure characteristics of self-resonant cavitating jets. J. Hydrodyn. 2003, 18, 570–575. [Google Scholar]
- Li, D.; Kang, Y.; Wang, X.; Ding, X.L.; Fang, Z.L. Effects of nozzle inner surface roughness on the cavitation erosion characteristics of high speed submerged jets. Exp. Therm. Fluid Sci. 2016, 74, 444–452. [Google Scholar] [CrossRef]
- Li, D.; Kang, Y.; Ding, X.; Ding, X.L.; Wang, X.C.; Liu, W.C. Effects of feeding pipe diameter on the performance of a jet-driven Helmholtz oscillator generating pulsed waterjets. J. Mech. Sci. Technol. 2017, 31, 1203–1212. [Google Scholar] [CrossRef]
- Li, D.; Wang, Z.; Yuan, M.; Fan, Q.; Wang, X.C. Effects of nozzle exit angle on the pressure characteristics of SRWJs used for deep-hole drilling. Appl. Sci. 2019, 9, 155. [Google Scholar] [CrossRef] [Green Version]
- Qi, M.; Wang, L.F.; Chen, Q.G.; Zhao, J.L.; Ju, Y.H.; Fu, Q.Q. Numerical simulation of cavitating jet in dual chamber self-oscillation pulse nozzle. J. Drain. Irrig. Mach. Eng. 2020, 38, 457–461. [Google Scholar]
- Yang, L.; Li, X.H.; Wang, J.S.; Lu, Y.Y. The natural frequency of self-oscillating pulsed jet with structural parameters effect of characteristics. Fluid Mach. 2001, 2, 26–28. [Google Scholar]
- Tang, C.L.; Hu, D.; Pei, J.H. Experimental research on dynamic characteristics of the self-excited oscillation pulsed water jet. Water Conserv. Hydropower Technol. 2006, 12, 71–74. [Google Scholar]
- Gao, C.C.; Xie, K.Y.; Liu, X.Y.; Hu, Y.Z.; Ma, W.L. Numerical simulation and experiment on flow fields in underwater self-excited pulse jet device. J. Drain. Irrig. Mach. Eng. 2016, 34, 220–226. [Google Scholar]
- Gao, C.C.; Hu, Y.Z.; Liu, X.Y.; Xie, K.Y.; Ma, W.L.; Wang, M.F. Experimental study on suction performance of underwater self-excited pulse jet self-excited oscillation cavitation jet nozzle. South North Water Transf. Water Conserv. Technol. 2016, 1, 109–113. [Google Scholar]
- Gao, C.C.; Hu, Y.W.; Li, H.; Ma, W.L. Statistical analysis of effects of structural parameters on characteristics of underwater self-excited pulse jet. J. Drain. Irrig. Mach. Eng. 2018, 36, 327–333. [Google Scholar]
- Li, W.; Zhang, W.Q.; Shi, W.D.; Yang, Y.F.; Cao, W.D. Experimental study on cavitation cloud evolution of self-excited oscillating cavitation jet nozzle. J. Drain. Irrig. Mach. Eng. 2020, 38, 547–552. [Google Scholar]
- Lu, Y.Y.; Zhang, S.; Liu, Y.; Lu, Z.H.; Jiang, L.Y. Analysis on stress wave effect during the process of rock breaking by pulsed jet. J. Chongqing Univ. 2012, 35, 117–124. [Google Scholar]
- Lu, Y.Y.; Ge, Z.L.; Li, X.H.; Shen, D.F.; Kang, Y.; Chen, J.F. Numerical analysis of cavitation development and collapse process. J. China Univ. Min. Technol. 2009, 4, 582–585. [Google Scholar]
- Li, X.H.; Zhao, Y.; Lu, Y.Y.; Kang, Y. Mechanism of self-excited oscillation jet to improve permeability of soft coal seam. J. Liaoning Tech. Univ. 2009, S1, 202–205. [Google Scholar]
- Li, D.; Kang, Y.; Ding, X.L.; Wang, X.C.; Fang, Z.L. Effects of Nozzle Inner Surface Roughness on the Performance of Self-resonating Cavitating Waterjets under Different Ambient Pressures. J. Mech. Eng. 2017, 63, 92–102. [Google Scholar] [CrossRef]
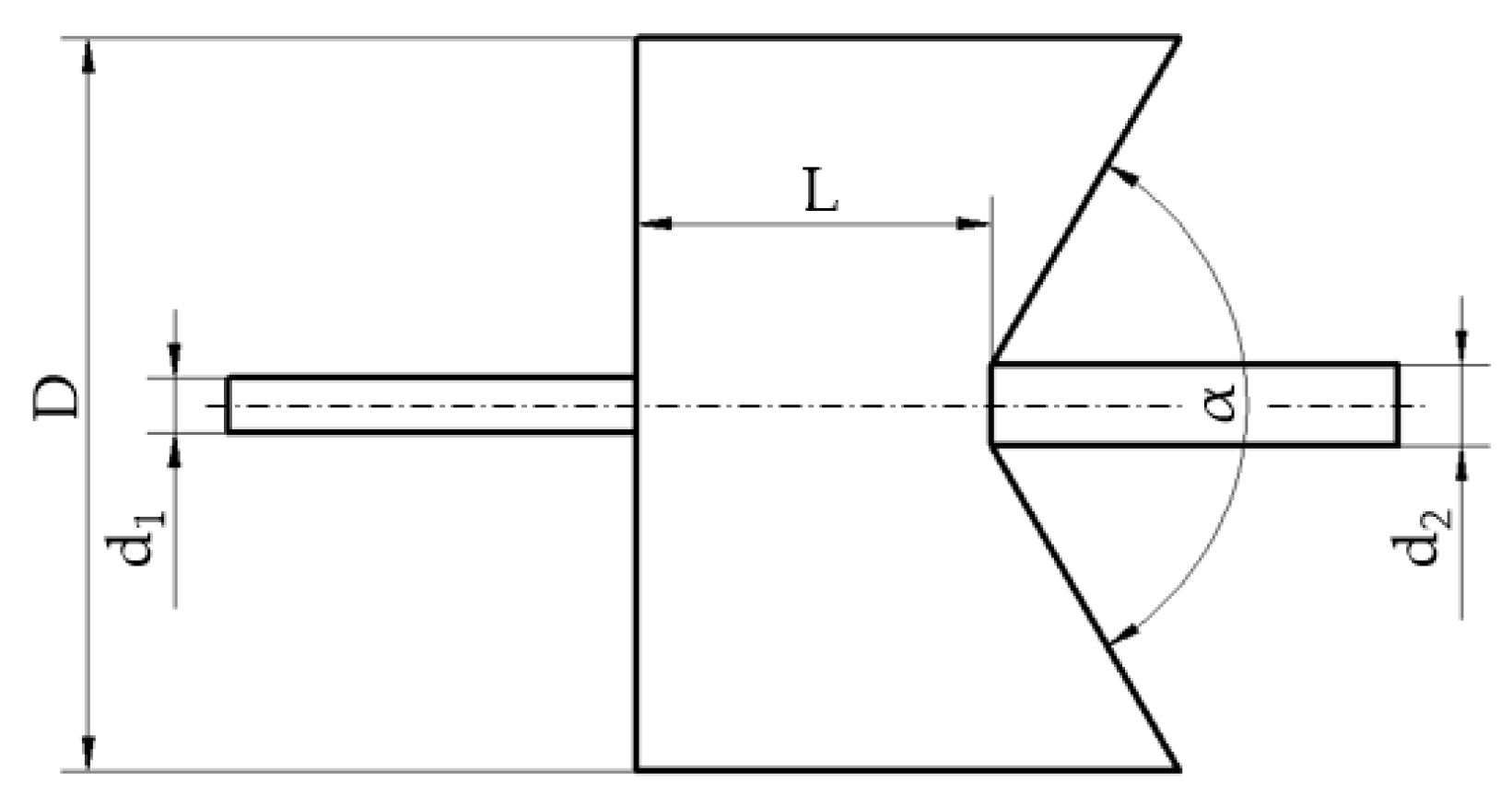
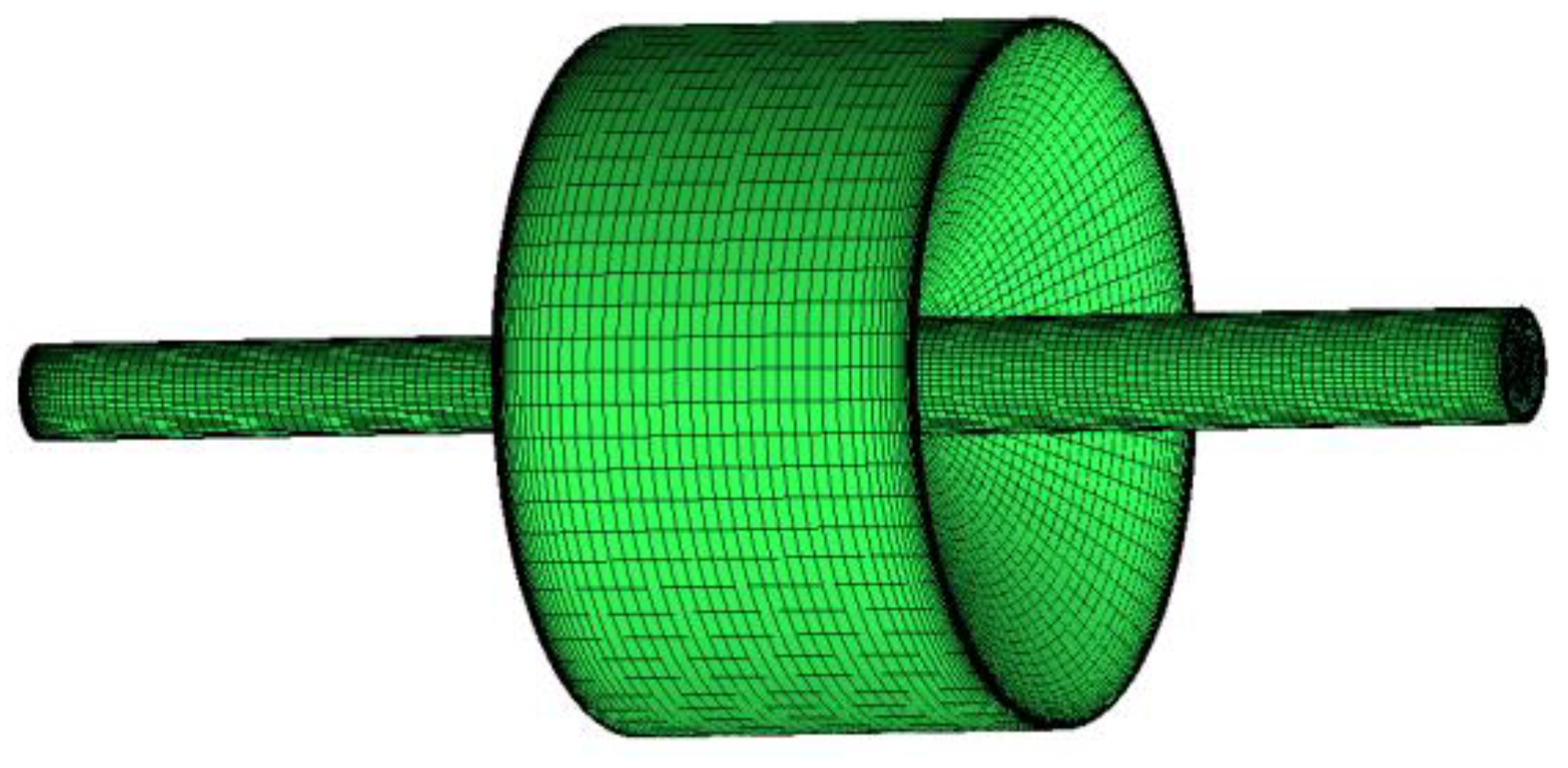
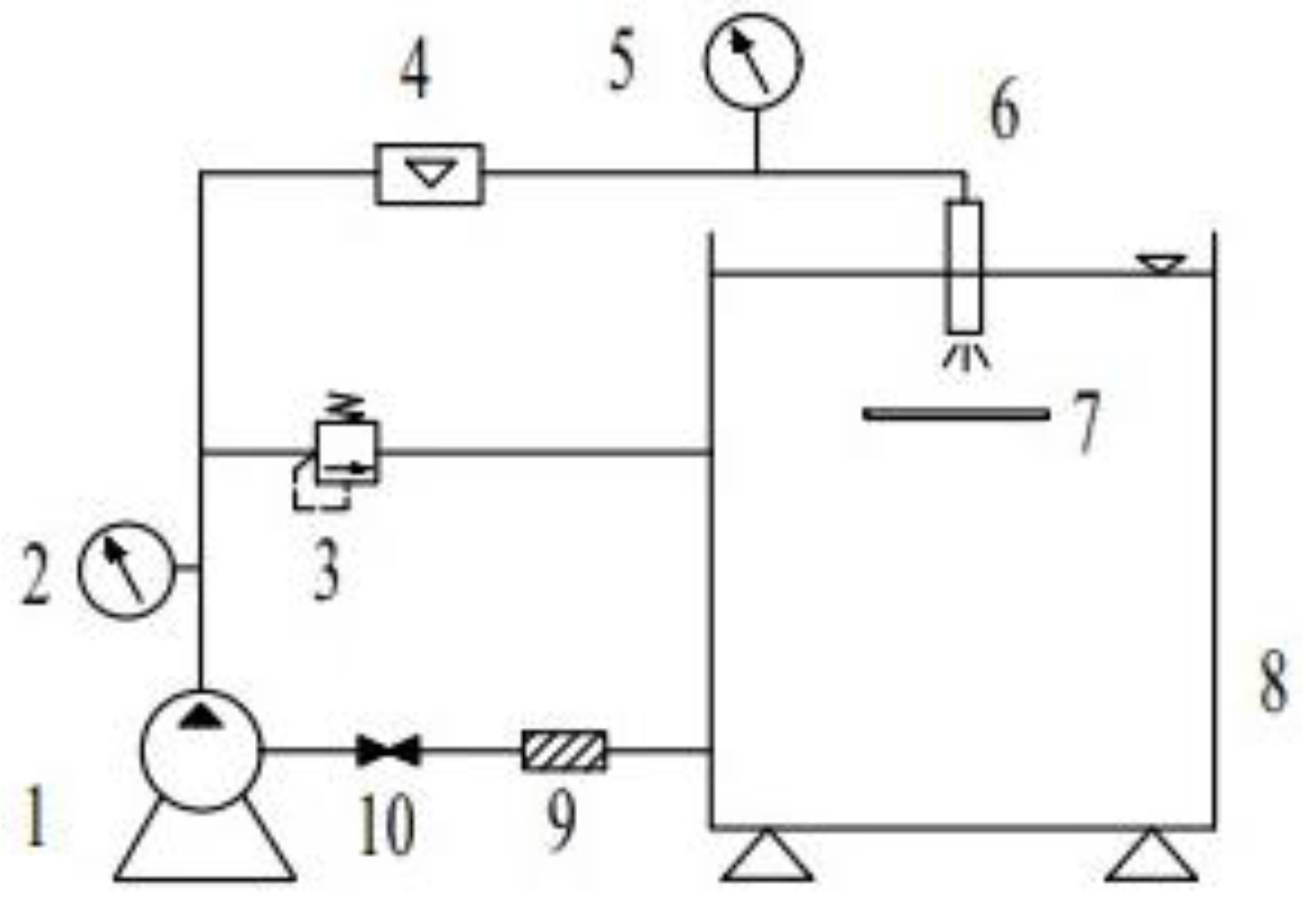

| Design Variables | Reference Value | Value Range | Output Variable (%) |
|---|---|---|---|
| d1 (mm) | 4 | (4, 5) | Vapor volume fraction(VOF) |
| Cd21 | 2 | (1.84, 2.67) | |
| CLD | 0.68 | (0.53, 0.84) | |
| CDd2 | 5.94 | (5, 6.9) | |
| Pin (MPa) | 4 | (0, 5) |
| Cd21 | CLD | CDd2 | d1 (mm) | Pin (MPa) | |
|---|---|---|---|---|---|
| 1 | 2.6092 | 5.252 | 0.621 | 4.7899 | 1.5378 |
| 2 | 1.9059 | 5.874 | 0.5235 | 4.6218 | 3.9916 |
| 3 | 2.1176 | 6.714 | 0.5874 | 4.7227 | 1.2353 |
| 4 | 1.9664 | 6.143 | 0.6681 | 4.958 | 2.0420 |
| 5 | 2.6319 | 5.42 | 0.7992 | 4.9328 | 4.1933 |
| 6 | 2.1328 | 5.891 | 0.6714 | 4.0336 | 3.8236 |
| 7 | 2.6546 | 5.437 | 0.7118 | 4.3445 | 1.6387 |
| 8 | 1.8832 | 5.555 | 0.7521 | 4.5798 | 1.1345 |
| 9 | 2.1782 | 5.588 | 0.5269 | 4.4538 | 2.6134 |
| 10 | 2.2311 | 5.773 | 0.7017 | 4.6303 | 2.1765 |
| 11 | 2.5034 | 6.076 | 0.5639 | 4.084 | 4.4958 |
| 12 | 2.0496 | 5.958 | 0.8227 | 4.2185 | 4.8319 |
| 13 | 2.0874 | 6.479 | 0.6546 | 4.437 | 2.9496 |
| 14 | 2.337 | 5.605 | 0.8664 | 4.7059 | 3.1513 |
| 15 | 1.9134 | 6.21 | 0.8496 | 4.8403 | 2.7143 |
| 16 | 2.4807 | 5.101 | 0.7891 | 4.5042 | 4.4622 |
| 17 | 1.8227 | 6.261 | 0.7723 | 4.1933 | 3.2857 |
| 18 | 2.4277 | 6.782 | 0.7824 | 4.7311 | 4.6639 |
| 19 | 2.5714 | 6.16 | 0.658 | 4.6134 | 1.2689 |
| 20 | 2.0042 | 5.168 | 0.6815 | 4.8992 | 1.8739 |
| 21 | 2.1706 | 6.395 | 0.8966 | 4.563 | 3.9244 |
| 22 | 2.4655 | 5.118 | 0.6479 | 4.5294 | 3.0168 |
| 23 | 2.5941 | 5.134 | 0.7689 | 4.0924 | 3.5882 |
| 24 | 1.9891 | 6.849 | 0.863 | 4.3782 | 2.5462 |
| 25 | 1.9361 | 6.244 | 0.8395 | 4.1176 | 1.5042 |
| 26 | 2.6924 | 5.807 | 0.7857 | 4.4202 | 3.3529 |
| 27 | 2.5487 | 5.992 | 0.6916 | 4.6807 | 4.7311 |
| 28 | 1.8303 | 5.79 | 0.6849 | 4.5966 | 2.9160 |
| 29 | 2.6697 | 5.739 | 0.6445 | 4.0252 | 2.8824 |
| 30 | 2.3824 | 5.151 | 0.7387 | 4.9496 | 2.6807 |
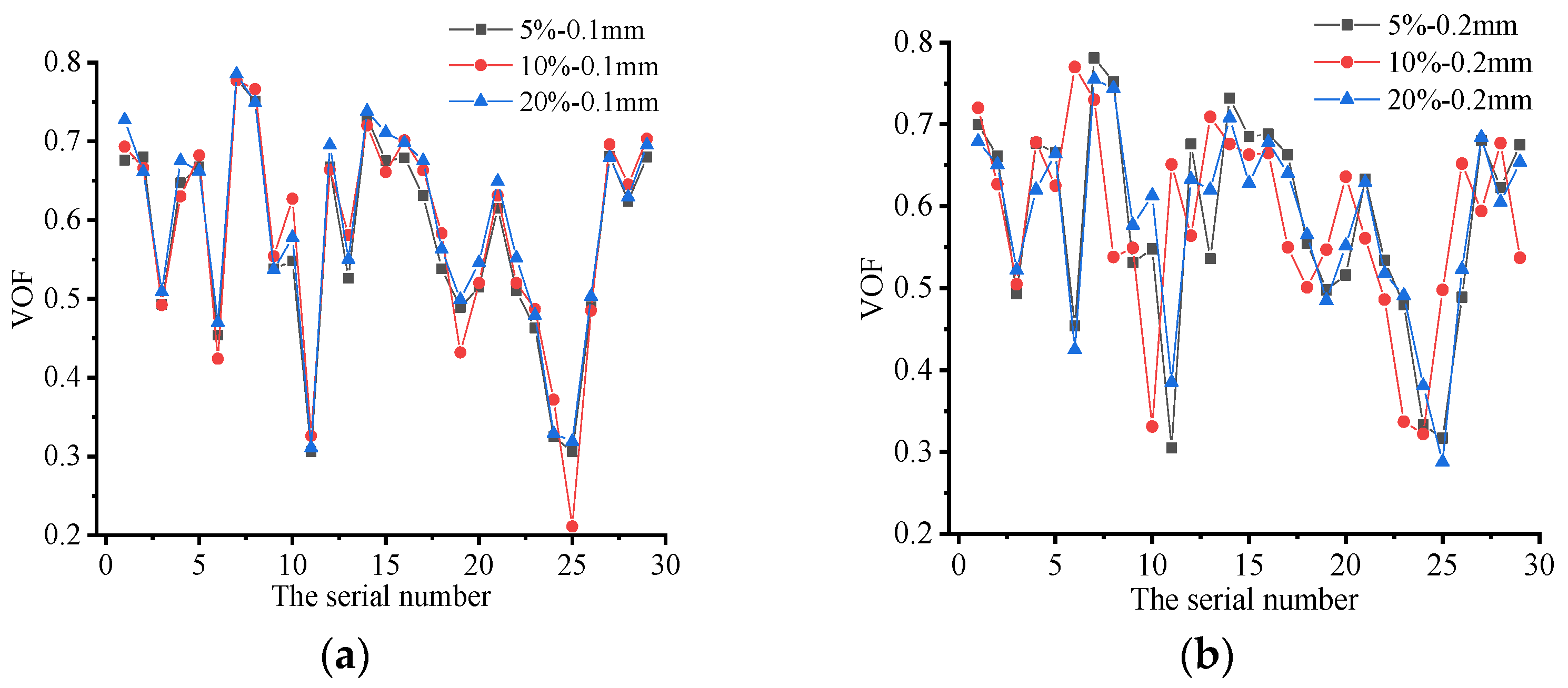
| VOF 5%-0.1 mm | VOF 5%-0.2 mm | VOF 5%-0.3 mm | VOF 10%-0.1 mm | VOF 10%-0.2 mm | VOF 20%-0.1 mm | VOF 20%-0.2 mm |
|---|---|---|---|---|---|---|
| 0.676 | 0.700 | 0.698 | 0.693 | 0.720 | 0.727 | 0.679 |
| 0.680 | 0.661 | 0.635 | 0.666 | 0.627 | 0.661 | 0.651 |
| 0.493 | 0.493 | 0.493 | 0.492 | 0.505 | 0.509 | 0.522 |
| 0.647 | 0.677 | 0.674 | 0.630 | 0.678 | 0.675 | 0.620 |
| 0.667 | 0.665 | 0.620 | 0.682 | 0.625 | 0.662 | 0.664 |
| 0.454 | 0.454 | 0.455 | 0.424 | 0.770 | 0.470 | 0.425 |
| 0.779 | 0.781 | 0.778 | 0.777 | 0.730 | 0.785 | 0.755 |
| 0.751 | 0.752 | 0.734 | 0.766 | 0.538 | 0.749 | 0.744 |
| 0.538 | 0.531 | 0.537 | 0.554 | 0.549 | 0.537 | 0.577 |
| 0.548 | 0.548 | 0.560 | 0.627 | 0.331 | 0.578 | 0.613 |
| 0.306 | 0.305 | 0.295 | 0.326 | 0.651 | 0.311 | 0.385 |
| 0.667 | 0.676 | 0.651 | 0.664 | 0.564 | 0.695 | 0.633 |
| 0.526 | 0.536 | 0.559 | 0.581 | 0.709 | 0.550 | 0.620 |
| 0.730 | 0.732 | 0.720 | 0.720 | 0.676 | 0.738 | 0.708 |
| 0.675 | 0.685 | 0.683 | 0.661 | 0.663 | 0.711 | 0.628 |
| 0.679 | 0.688 | 0.677 | 0.701 | 0.665 | 0.698 | 0.678 |
| 0.631 | 0.663 | 0.681 | 0.663 | 0.550 | 0.675 | 0.640 |
| 0.538 | 0.555 | 0.548 | 0.583 | 0.501 | 0.563 | 0.565 |
| 0.489 | 0.498 | 0.487 | 0.432 | 0.547 | 0.499 | 0.485 |
| 0.515 | 0.516 | 0.542 | 0.520 | 0.636 | 0.546 | 0.552 |
| 0.615 | 0.633 | 0.648 | 0.631 | 0.561 | 0.649 | 0.629 |
| 0.510 | 0.534 | 0.562 | 0.520 | 0.486 | 0.552 | 0.518 |
| 0.463 | 0.480 | 0.502 | 0.487 | 0.337 | 0.479 | 0.491 |
| 0.325 | 0.333 | 0.327 | 0.372 | 0.322 | 0.329 | 0.381 |
| 0.306 | 0.317 | 0.351 | 0.211 | 0.498 | 0.319 | 0.288 |
| 0.490 | 0.489 | 0.495 | 0.485 | 0.652 | 0.503 | 0.523 |
| 0.681 | 0.680 | 0.648 | 0.696 | 0.594 | 0.679 | 0.684 |
| 0.624 | 0.623 | 0.593 | 0.645 | 0.677 | 0.629 | 0.605 |
| 0.680 | 0.675 | 0.671 | 0.703 | 0.537 | 0.695 | 0.654 |
| Cd21 | CLD | CDd2 | d1 | Pin | |
|---|---|---|---|---|---|
| Cd21 | 1 | −0.3875 | −0.0791 | −0.0636 | 0.196 |
| CLD | −0.3875 | 1 | 0.1403 | −0.1085 | 0.0336 |
| CDd2 | −0.0791 | 0.1403 | 1 | 0.0129 | 0.1713 |
| d1 | −0.0636 | −0.1085 | 0.0129 | 1 | −0.2073 |
| Pin | 0.196 | 0.0336 | 0.1713 | −0.2073 | 1 |
| VOF | 0.1107 | −0.7438 | −0.0869 | −0.2467 | 0.0844 |
| VOF (5%) | VOF (10%) | VOF (20%) | |||||
|---|---|---|---|---|---|---|---|
| 0.1 mm | 0.2 mm | 0.3 mm | 0.1 mm | 0.2 mm | 0.1 mm | 0.2 mm | |
| r(Cd21, VOF) | −0.701 | −0.586 | −0.328 | −0.599 | −0.329 | −0.492 | −0.576 |
| r(CLD, VOF) | −0.920 | −0.916 | −0.894 | −0.851 | −0.878 | −0.882 | −0.868 |
| r(CDd2, VOF) | −0.222 | −0.215 | −0.185 | −0.036 | −0.163 | −0.247 | |
| r(d1, VOF) | −0.738 | −0.712 | −0.626 | −0.622 | −0.606 | −0.663 | −0.558 |
| r(Pin, VOF) | 0.645 | 0.668 | 0.620 | 0.531 | 0.601 | 0.592 | 0.466 |
| VOF (5%) | VOF (10%) | VOF (20%) | |||||
|---|---|---|---|---|---|---|---|
| 0.1 mm | 0.2 mm | 0.3 mm | 0.1 mm | 0.2 mm | 0.1 mm | 0.2 mm | |
| Coefficient of determination | 0.9127 | 0.9030 | 0.8647 | 0.8470 | 0.8511 | 0.8724 | 0.8358 |
| Residual path coefficient | 0.2954 | 0.3113 | 0.3678 | 0.3911 | 0.3858 | 0.3572 | 0.4052 |
| Correlation Coefficients | VOF (5%, 0.1) | VOF (5%, 0.1) | VOF (5%, 0.3) | VOF (10%, 0.1) | VOF (10%, 0.2) | VOF (20%, 0.1) |
|---|---|---|---|---|---|---|
| VOF (5%, 0.2) | 0.9916 | |||||
| VOF (5%, 0.3) | 0.9734 | 0.9903 | ||||
| VOF (10%, 0.1) | 0.9911 | 0.9822 | 0.9628 | |||
| VOF (10%, 0.2) | 0.6505 | 0.6839 | 0.7078 | 0.5947 | ||
| VOF (20%, 0.1) | 0.983 | 0.9962 | 0.9924 | 0.976 | 0.7026 | |
| VOF (20%, 0.2) | 0.9749 | 0.9645 | 0.9503 | 0.9935 | 0.5219 | 0.9587 |
| Principal Component | Eigenvalue | Percentage (%) | Cumulative Percentage (%) |
|---|---|---|---|
| 1 | 6.3582 | 90.8313 | 90.8313 |
| 2 | 0.5816 | 8.3087 | 99.1399 |
| 3 | 0.0364 | 0.5205 | 99.6604 |
| 4 | 0.0155 | 0.2218 | 99.8823 |
| 5 | 0.0066 | 0.0943 | 99.9766 |
| 6 | 0.0015 | 0.0215 | 99.9981 |
| 7 | 0.0001 | 0.0019 | 100 |
| Feature Vector | Factor 1 | Factor 2 | Factor 3 | Factor 4 | Factor 5 | Factor 6 | Factor 7 |
|---|---|---|---|---|---|---|---|
| 1 | 0.3932 | −0.1067 | 0.304 | −0.6078 | 0.4281 | −0.4305 | 0.0607 |
| 2 | 0.3953 | −0.044 | −0.2134 | −0.4077 | −0.1417 | 0.7038 | 0.3386 |
| 3 | 0.393 | 0.0126 | −0.6417 | 0.273 | 0.5195 | −0.0082 | −0.2986 |
| 4 | 0.3901 | −0.206 | 0.4381 | 0.1089 | −0.2052 | 0.26 | −0.7013 |
| 5 | 0.2825 | 0.9182 | 0.2328 | 0.1371 | 0.0137 | 0.0233 | 0.0588 |
| 6 | 0.395 | −0.0073 | −0.3391 | −0.0437 | −0.6908 | −0.4993 | 0.0203 |
| 7 | 0.3829 | −0.3178 | 0.2985 | 0.5977 | 0.0851 | −0.0431 | 0.5448 |
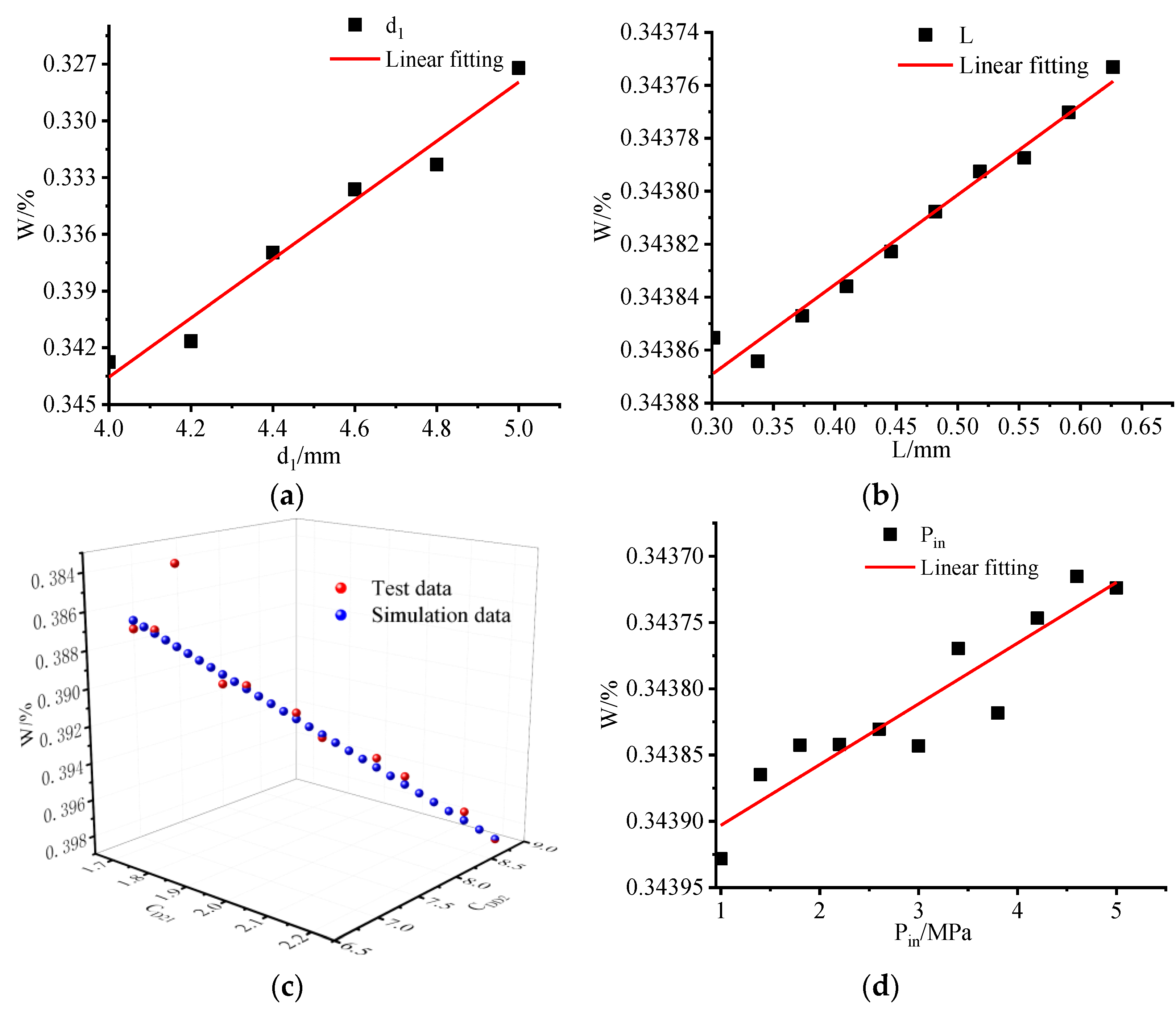
| Geometric Parameters | Slope of Test Curve | Fitting the Correlation Coefficient | the Parameters of Model Correspond to Coefficients |
|---|---|---|---|
| d1 | 0.01559 | 0.97335 | 0.0155616 |
| CLD | 3.39019 × 10−4 | 0.97422 | 3.635 × 10−4 |
| Pin | 4.58096 × 10−5 | 0.86608 | 5.36 × 10−5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, F.; Wang, X.; Xu, W.; Zhao, Y.; Zhao, G.; Zhu, H. Study on Different Parameters of the Self-Excited Oscillation Nozzle for Cavitation Effect under Multiphase Mixed Transport Conditions. J. Mar. Sci. Eng. 2021, 9, 1159. https://doi.org/10.3390/jmse9111159
Zhao F, Wang X, Xu W, Zhao Y, Zhao G, Zhu H. Study on Different Parameters of the Self-Excited Oscillation Nozzle for Cavitation Effect under Multiphase Mixed Transport Conditions. Journal of Marine Science and Engineering. 2021; 9(11):1159. https://doi.org/10.3390/jmse9111159
Chicago/Turabian StyleZhao, Fujian, Xiuli Wang, Wei Xu, Yuanyuan Zhao, Guohui Zhao, and Han Zhu. 2021. "Study on Different Parameters of the Self-Excited Oscillation Nozzle for Cavitation Effect under Multiphase Mixed Transport Conditions" Journal of Marine Science and Engineering 9, no. 11: 1159. https://doi.org/10.3390/jmse9111159
APA StyleZhao, F., Wang, X., Xu, W., Zhao, Y., Zhao, G., & Zhu, H. (2021). Study on Different Parameters of the Self-Excited Oscillation Nozzle for Cavitation Effect under Multiphase Mixed Transport Conditions. Journal of Marine Science and Engineering, 9(11), 1159. https://doi.org/10.3390/jmse9111159






