Analysis of the In Situ Steering Motion Characteristics and Sensitivity of Disc-Type Underwater Gliders
Abstract
1. Introduction
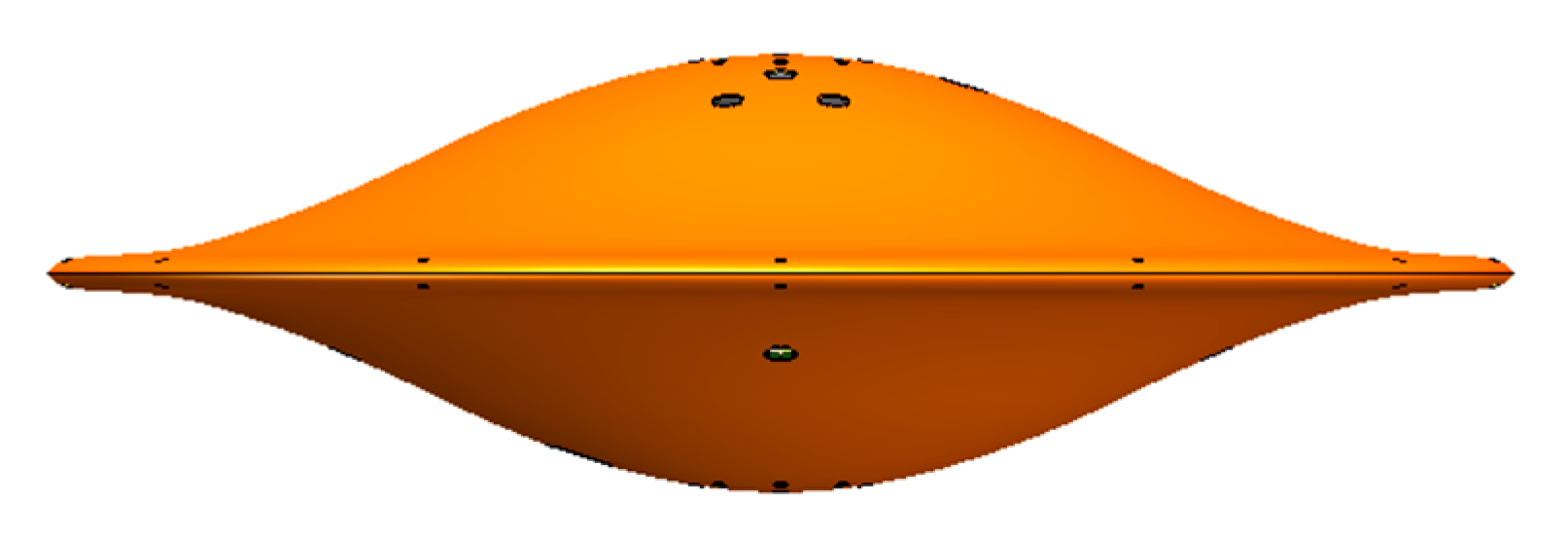
2. Modeling of the DTUG
2.1. Frames of Reference
2.2. Motion Control Equations
2.3. Calculation Method of Hydrodynamic Coefficients
3. Simulation on the Motion of DTUG
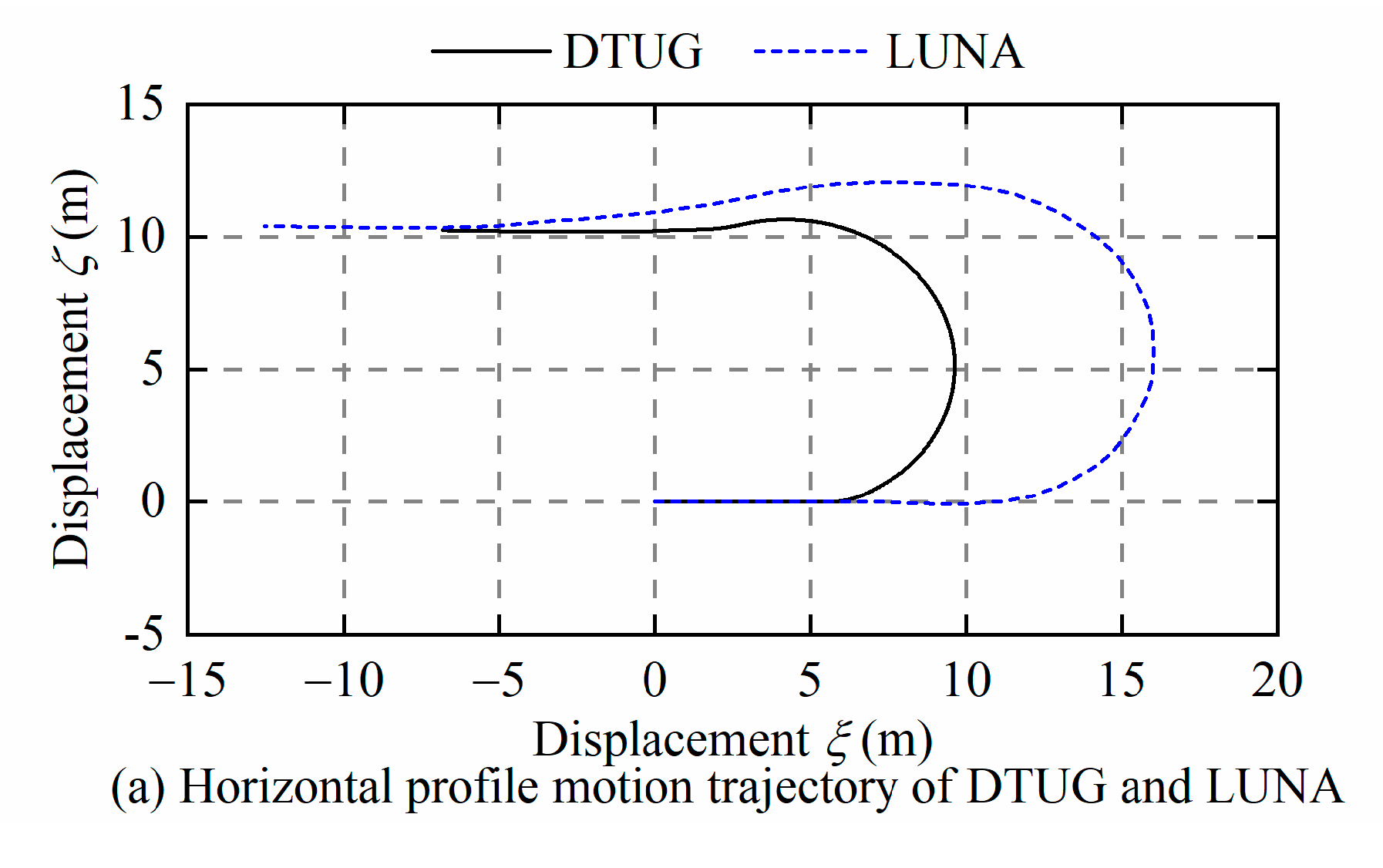
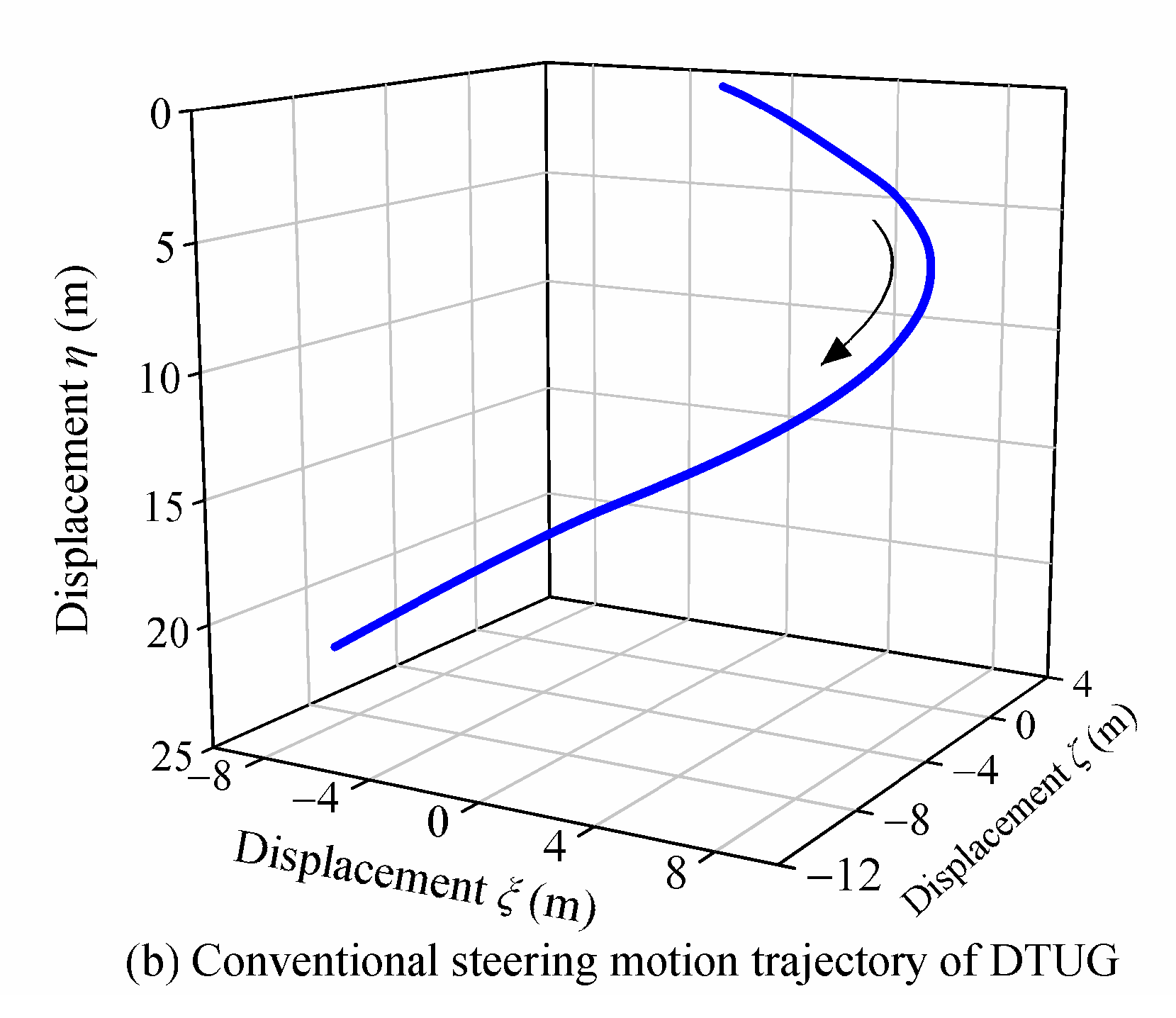
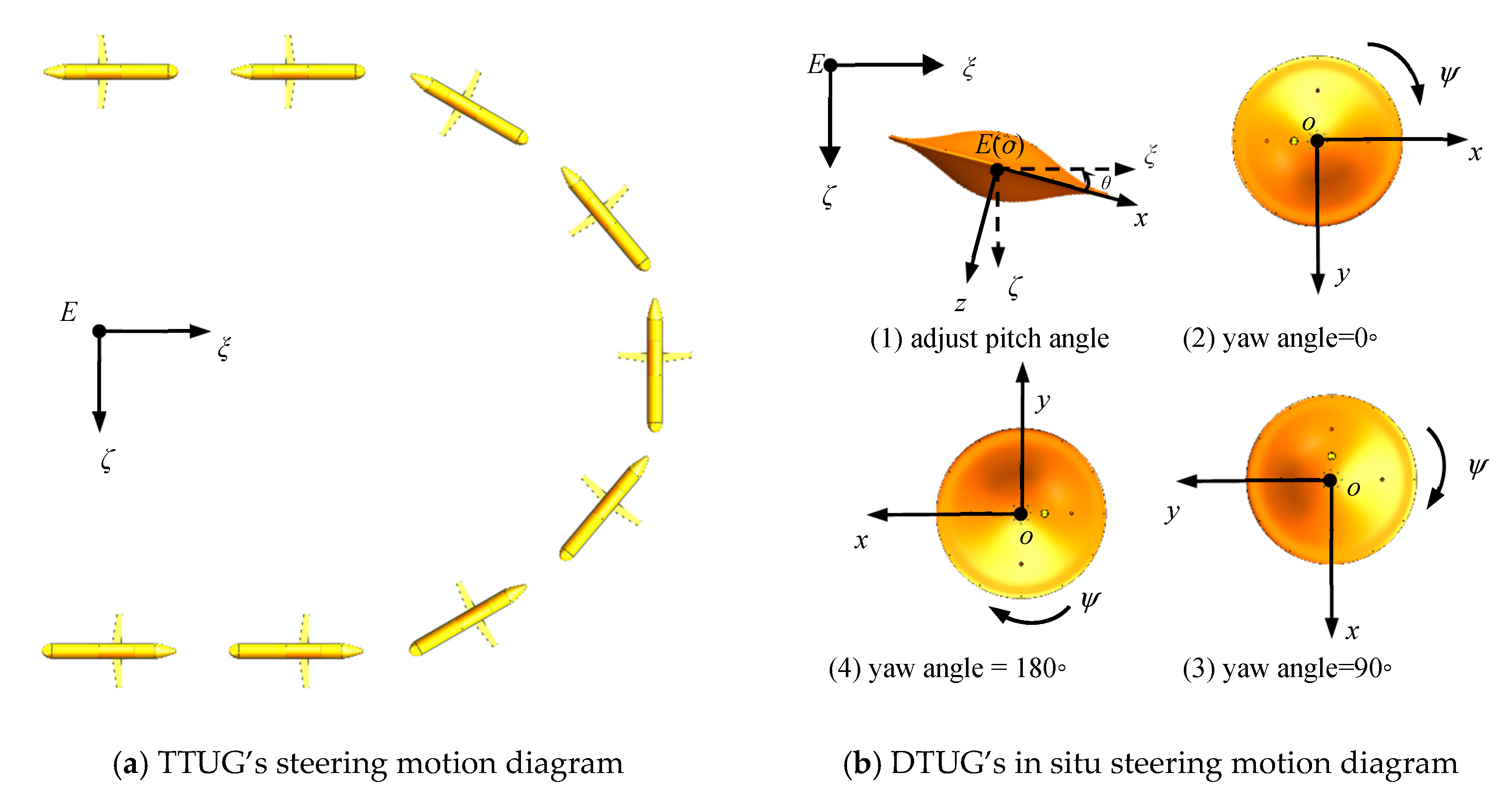
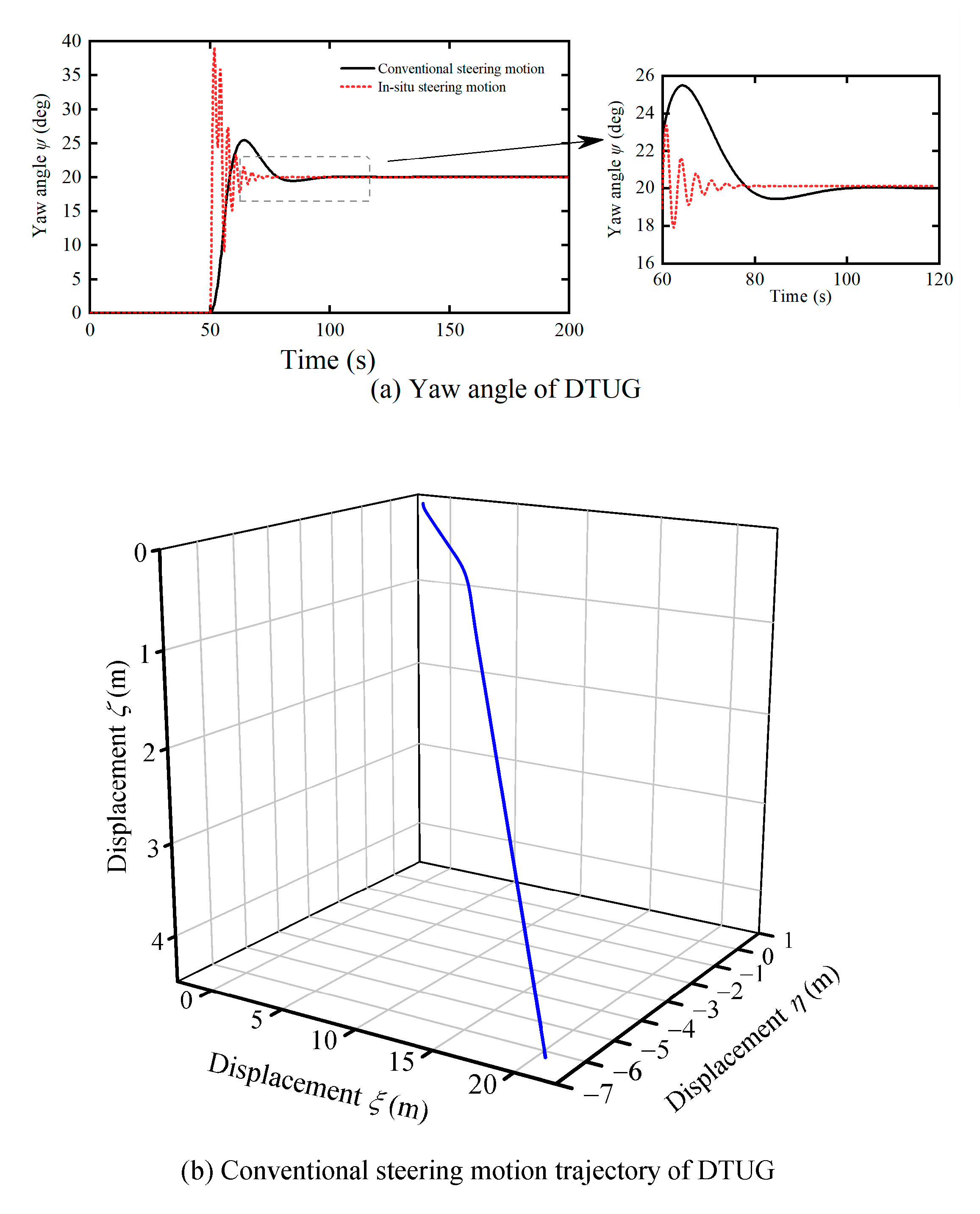
3.1. Conventional Steering Motion
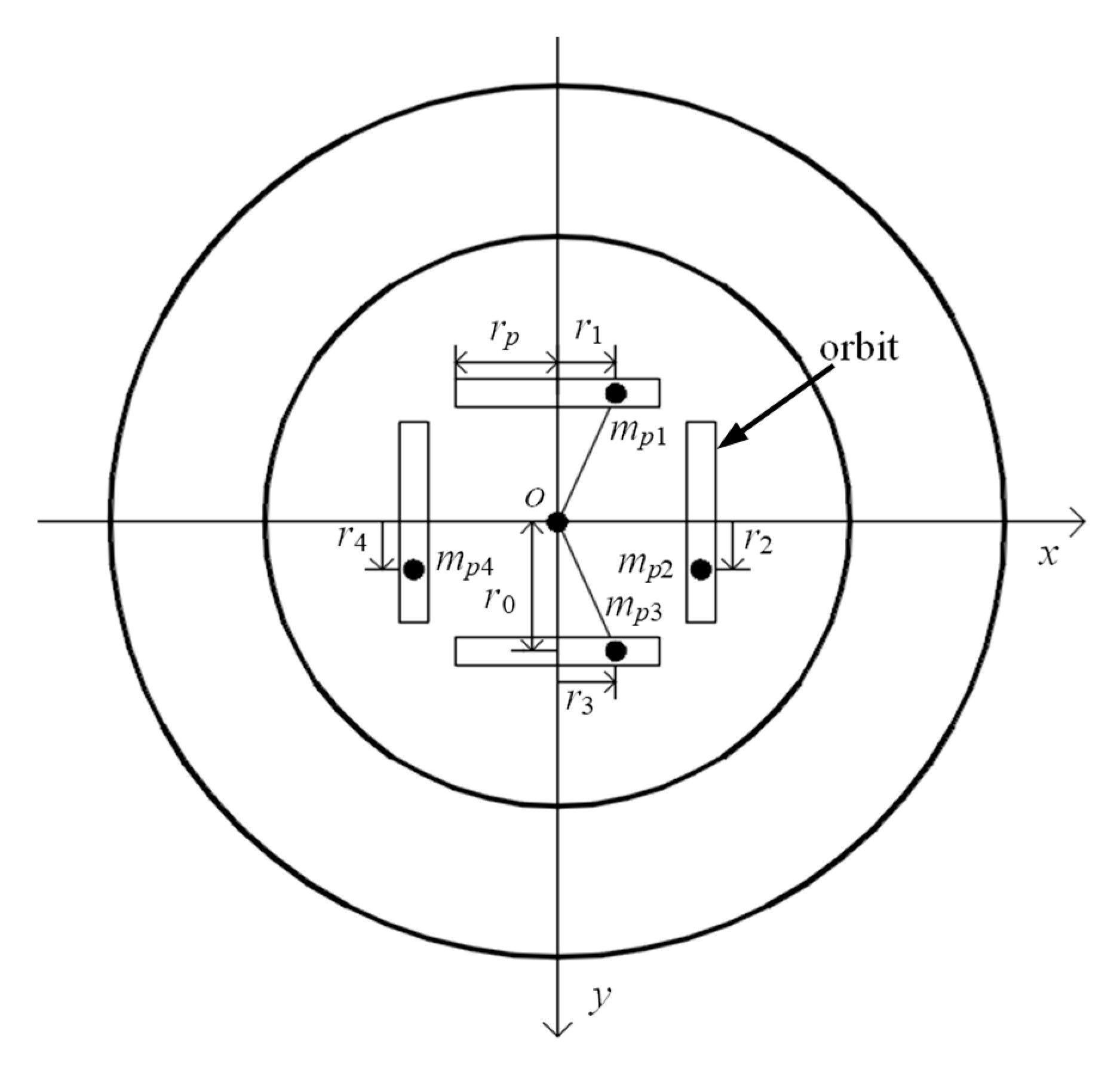
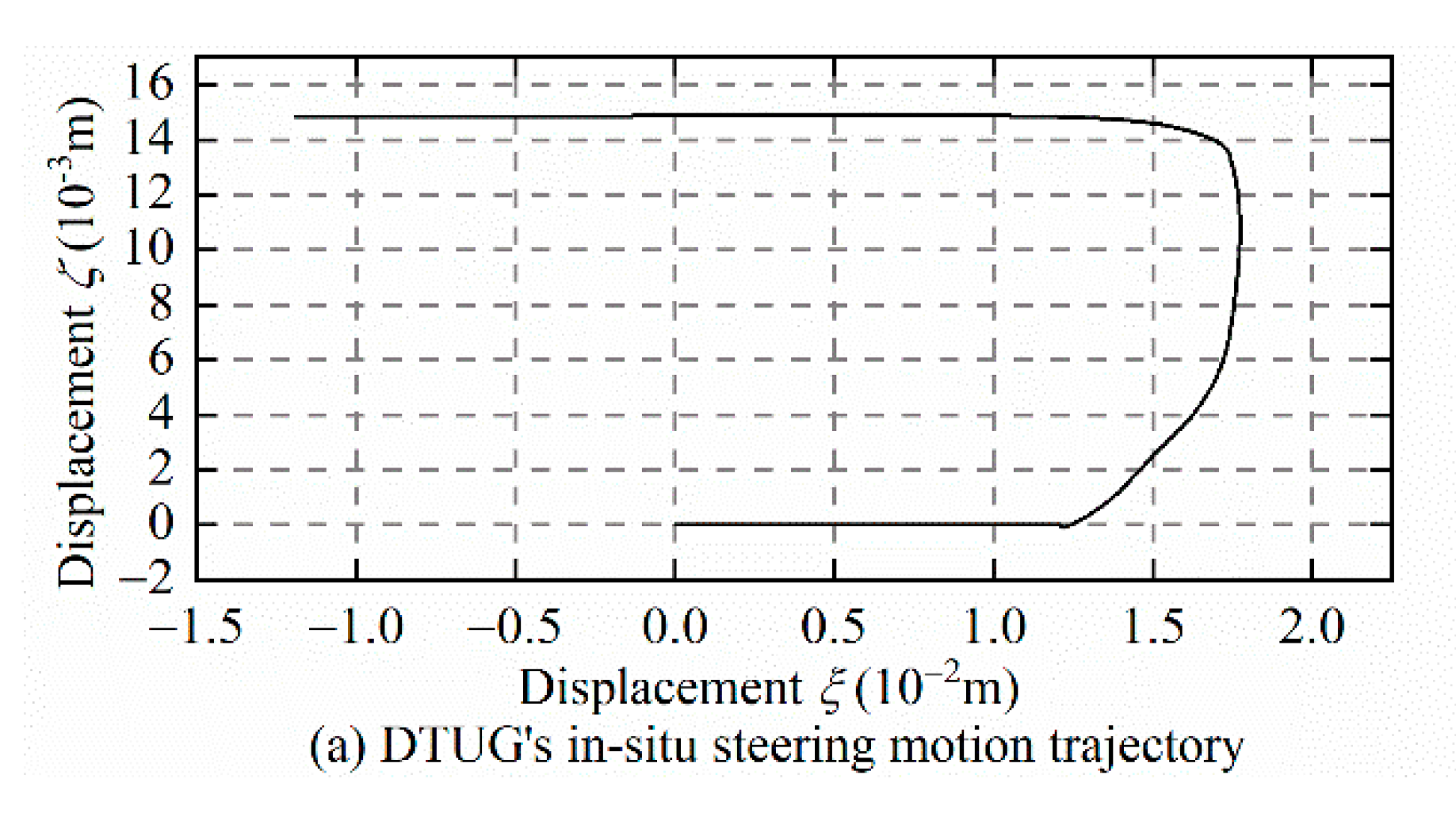
3.2. In Situ Steering Motion
3.2.1. Motion Description
3.2.2. Simulation on the Motion
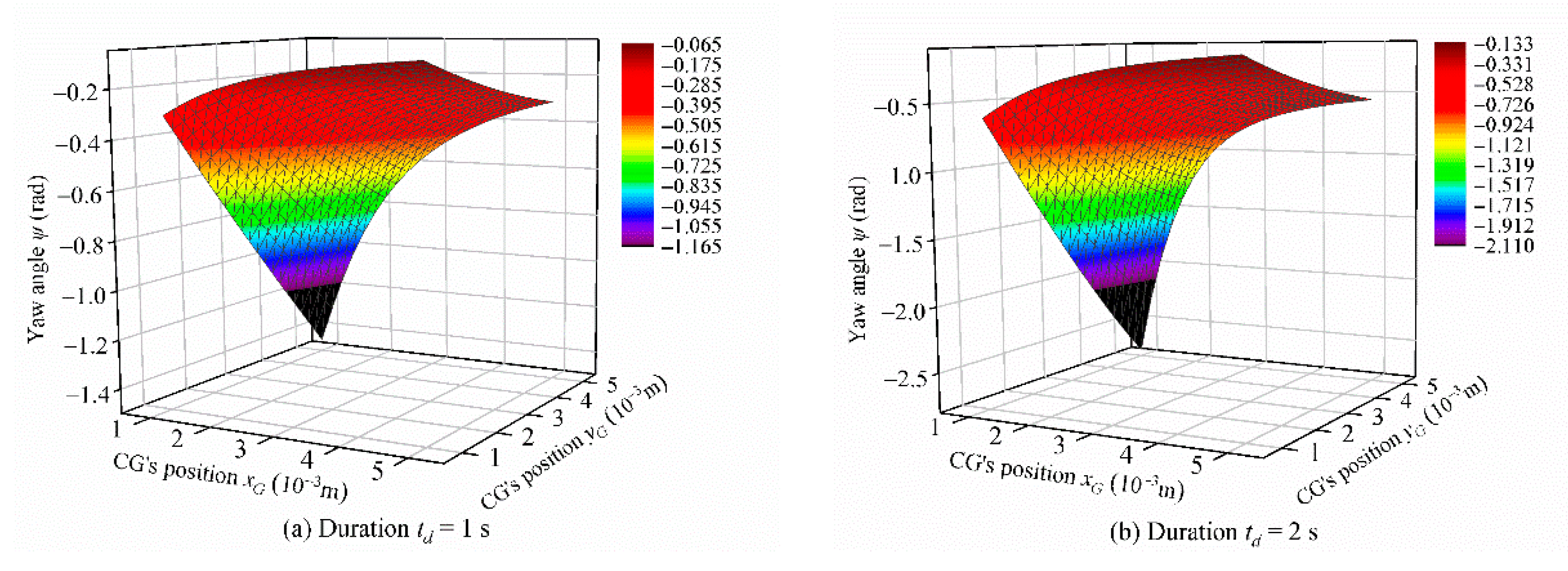
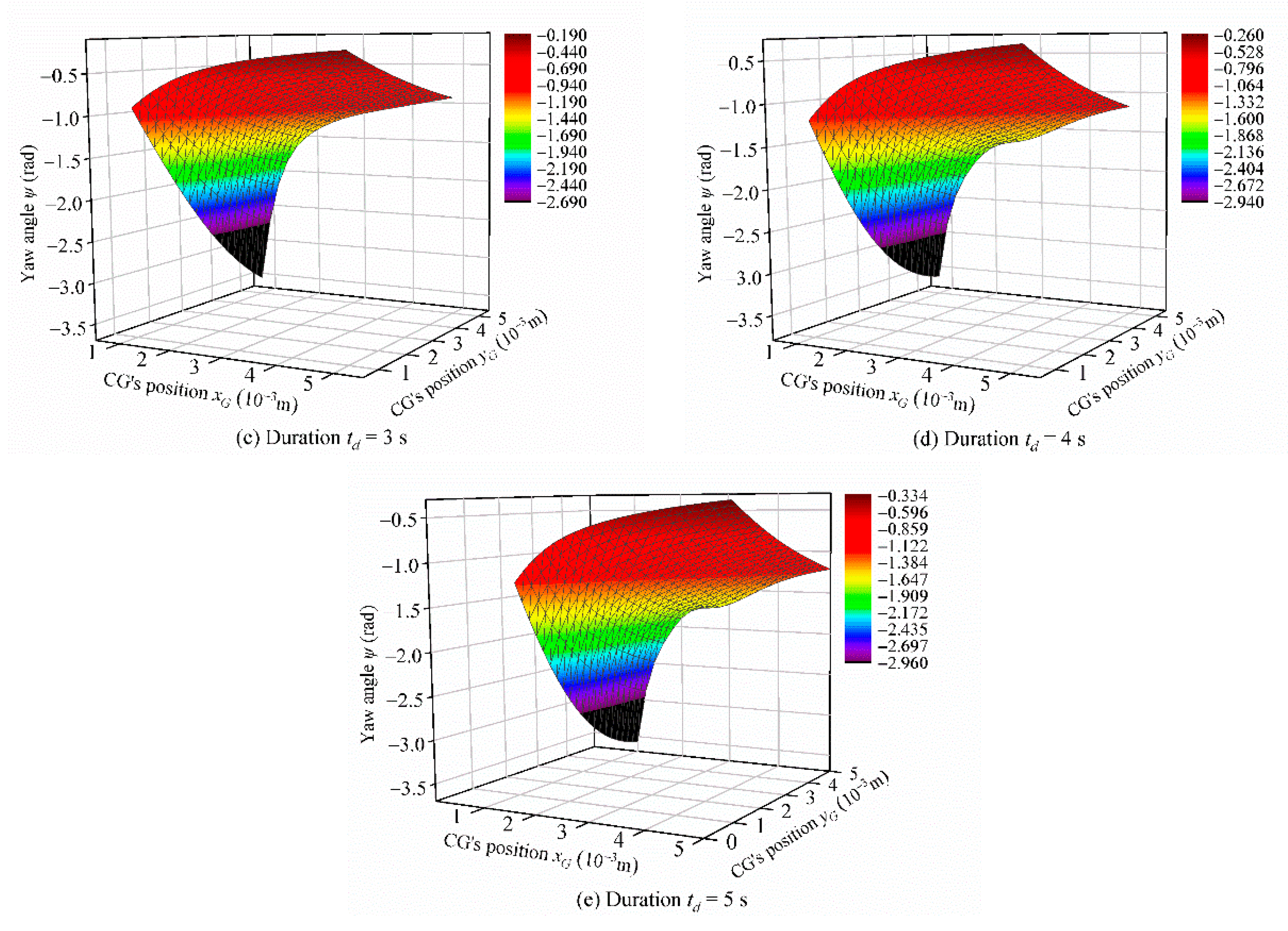
3.3. Sensitivity Analysis
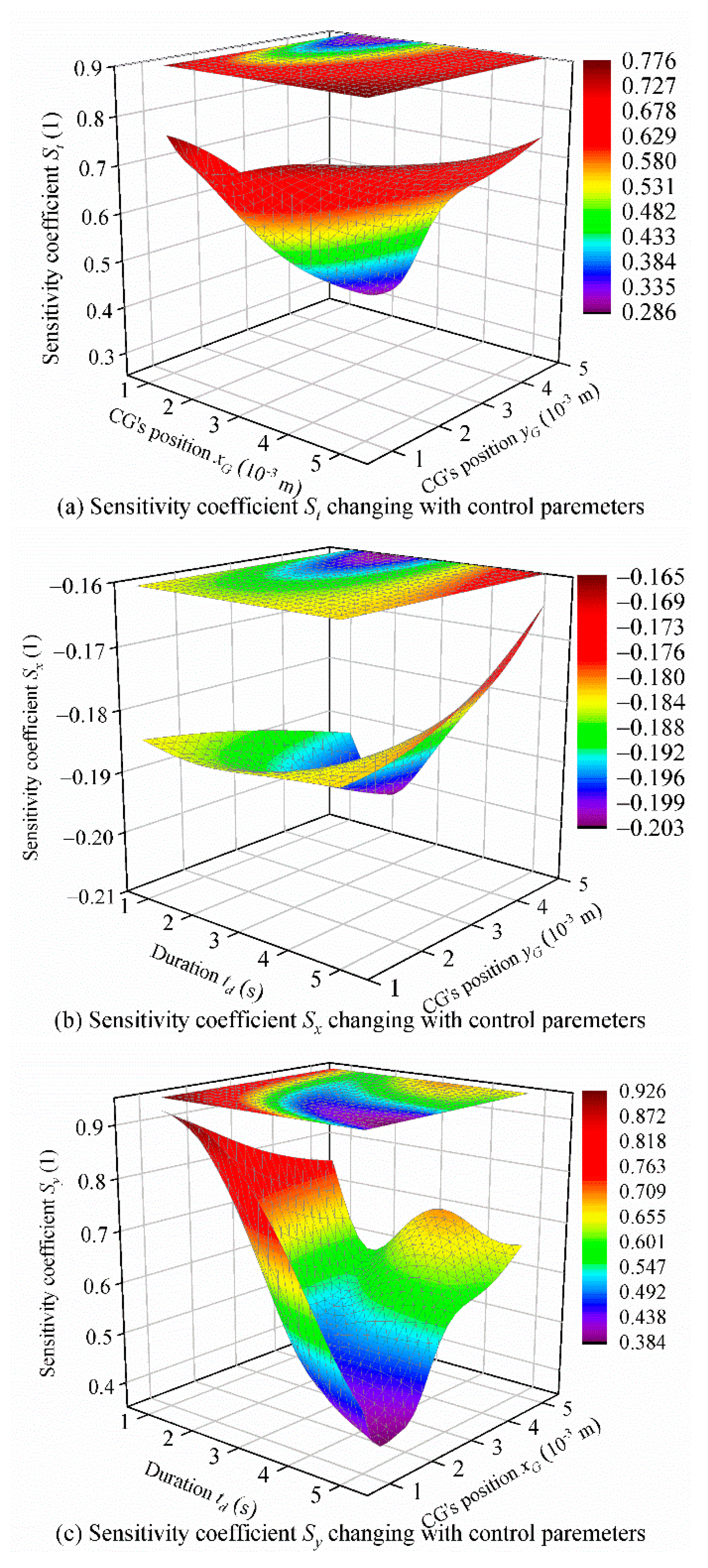
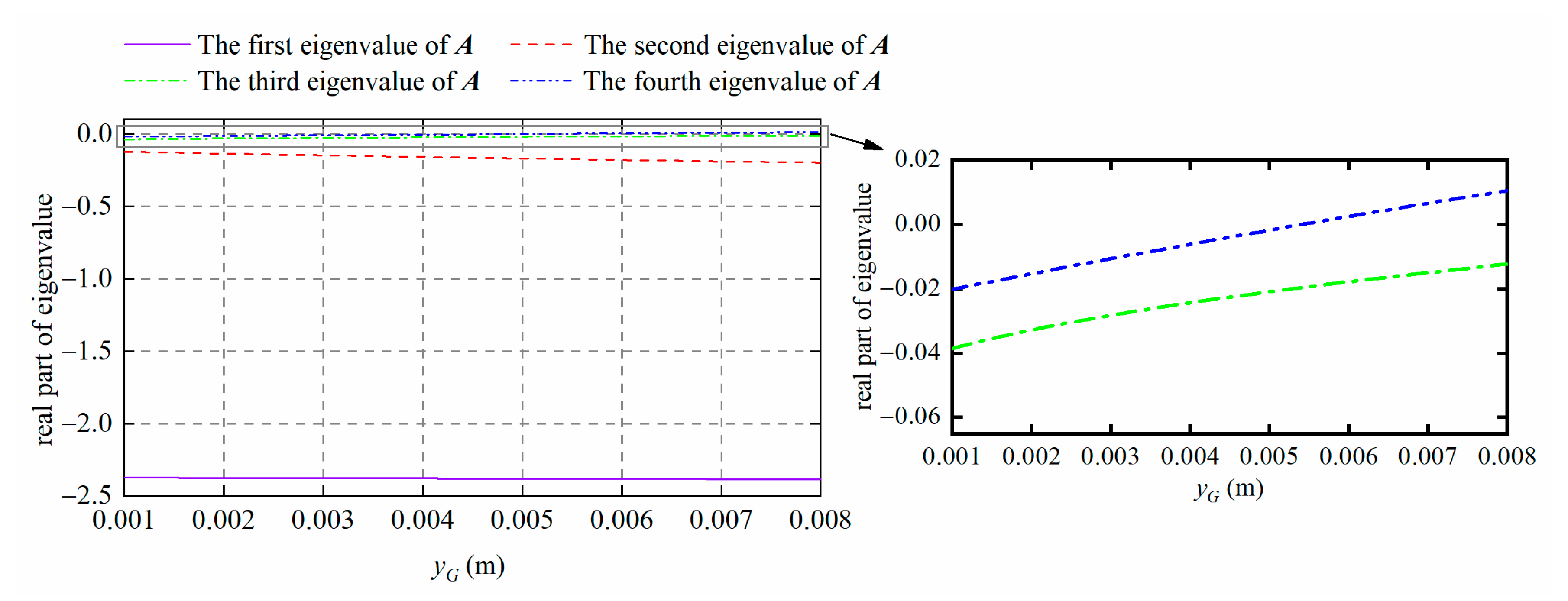
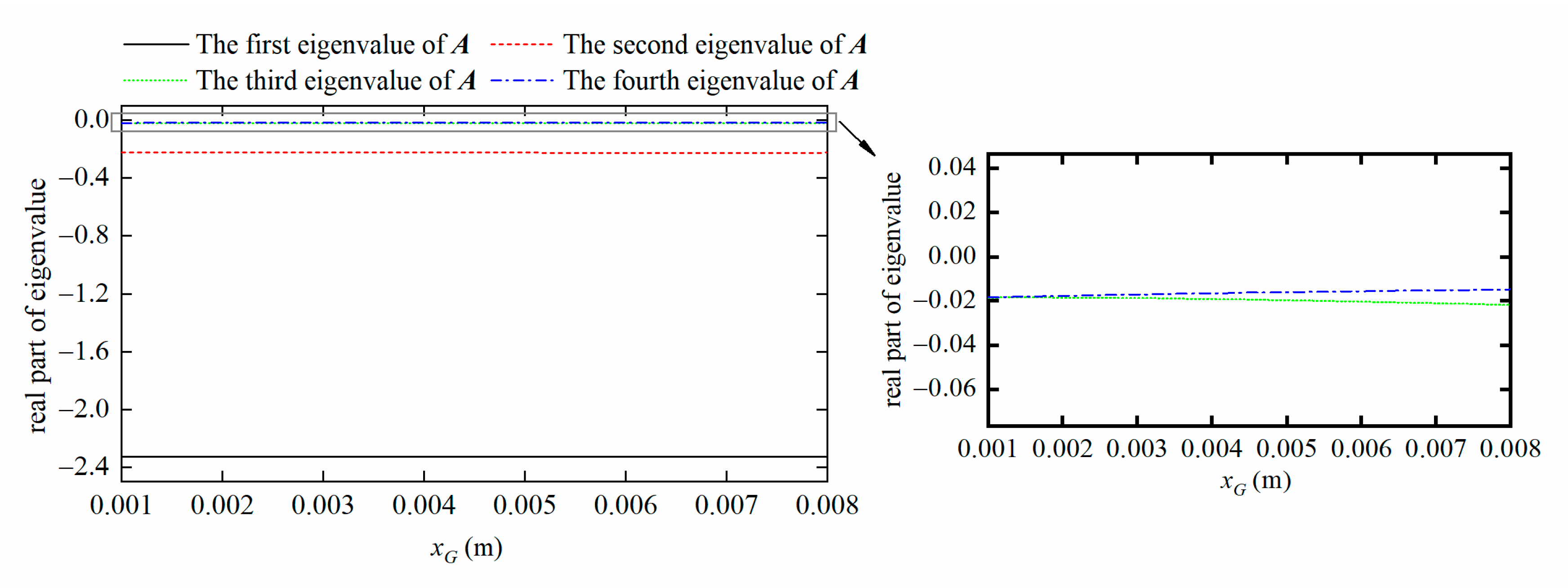
3.3.1. Local Sensitivity Analysis
3.3.2. Global Sensitivity Analysis
3.4. Stability Analysis
3.4.1. Dynamic Model Linearization
3.4.2. Lyapunov Stability Analysis
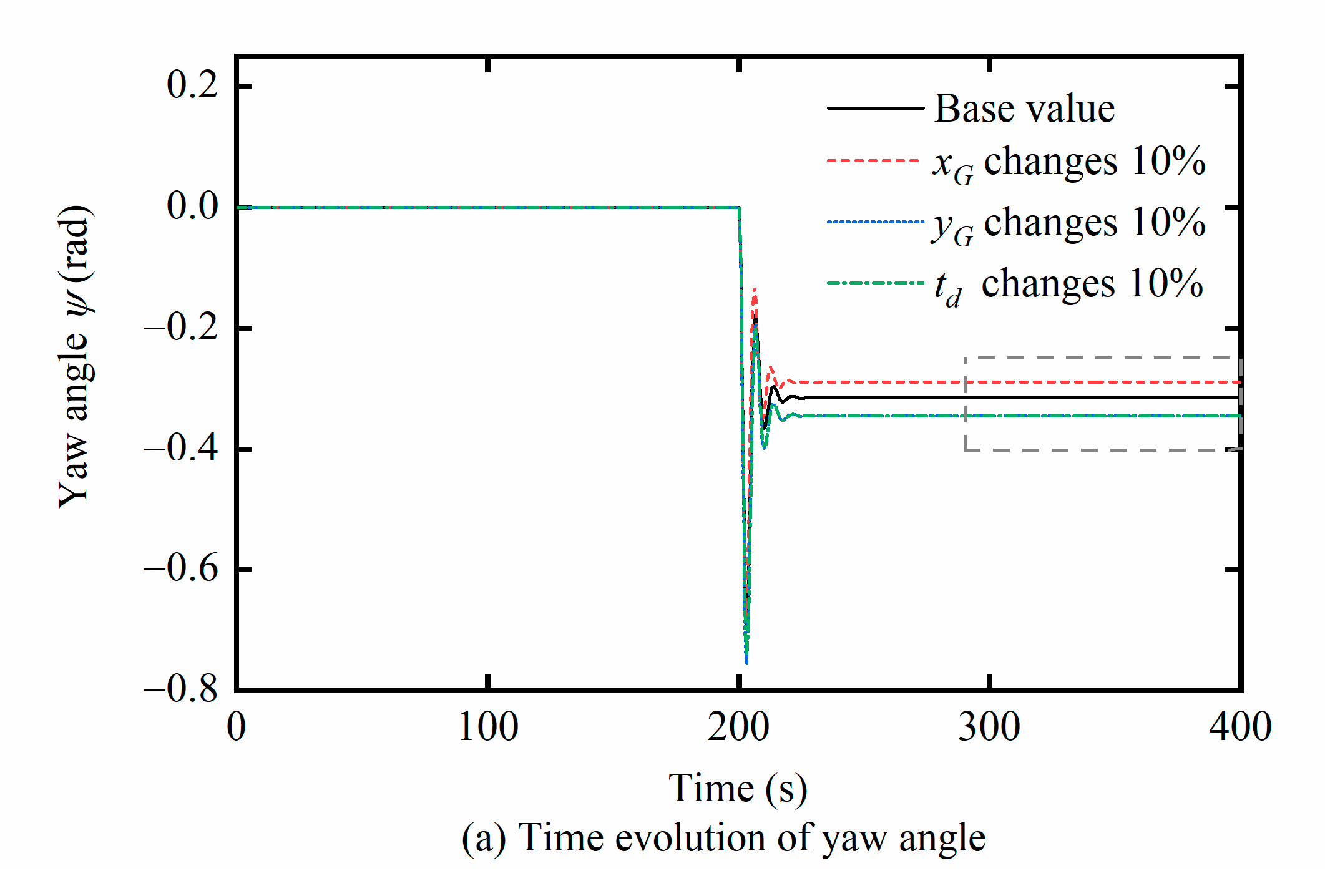
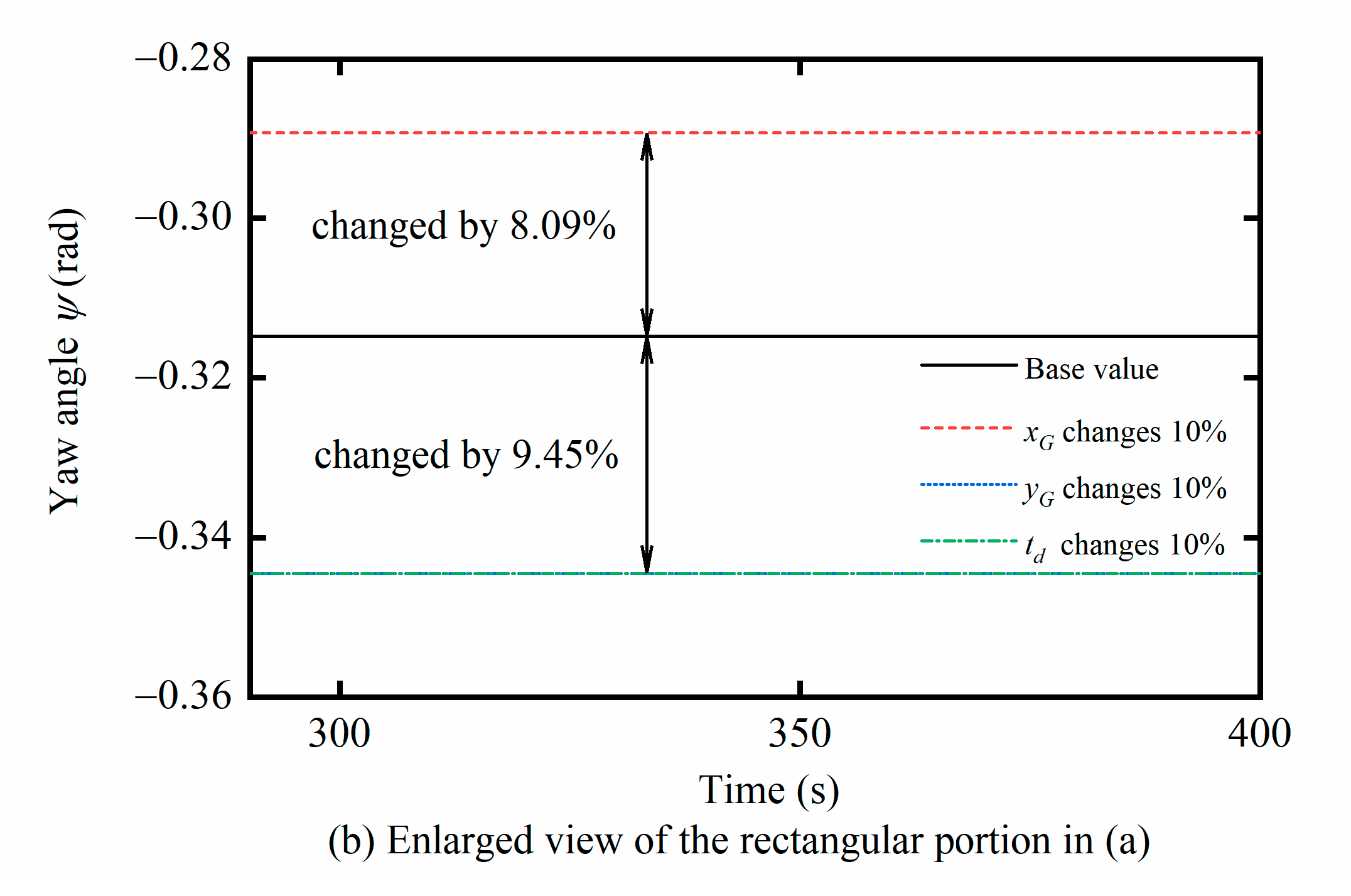
3.4.3. Simulation on In Situ Steering Motion after Disturbance
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Leonard, N.E.; Paley, D.A.; Lekien, F.; Sepulchre, R.; Fratantoni, D.M.; Davis, R.E. Collective motion, sensor networks, and ocean sampling. Proc. IEEE 2007, 95, 48–74. [Google Scholar] [CrossRef]
- Stommel, H. The slocum mission. Oceanography 1989, 2, 22–25. [Google Scholar] [CrossRef]
- Webb, D.C.; Simonetti, P.J.; Jones, C.P. SLOCUM: An underwater glider propelled by environmental energy. IEEE J. Ocean. Eng. 2001, 26, 447–452. [Google Scholar] [CrossRef]
- Eriksen, C.C.; Osse, T.J.; Light, R.D.; Wen, T.; Lehman, T.W.; Sabin, P.L.; Ballard, J.W.; Chiodi, A.M. Seaglider: A long-range autonomous underwater vehicle for oceanographic research. IEEE J. Ocean. Eng. 2001, 26, 424–436. [Google Scholar] [CrossRef]
- Sherman, J.; Davis, R.E.; Owens, W.; Valdes, J. The autonomous underwater glider “Spray”. IEEE J. Ocean. Eng. 2001, 26, 437–446. [Google Scholar] [CrossRef]
- Rudnick, D.L.; Davis, R.E.; Eriksen, C.C.; Fratantoni, D.M.; Perry, M.J. Underwater gliders for ocean research. Mar. Technol. Soc. J. 2004, 38, 73–84. [Google Scholar] [CrossRef]
- D’Spain, G.L.; Jenkins, S.A.; Zimmerman, R.; Luby, J.C.; Thode, A.M. Underwater acoustic measurements with the Liberdade/X-Ray flying wing glider. J. Acoust. Soc. Am. 2005, 117, 2624. [Google Scholar] [CrossRef]
- Buisson, N. BRIDGES project: DXP and UXP gliders bring together research and industry for the development of glider environment services. In Proceedings of the OCEANS 2019-Marseille, Marseille, France, 17–20 June 2019; IEEE: Marseille, France, 2019. [Google Scholar]
- Osse, T.J.; Eriksen, C.C. The Deepglider: A full ocean depth glider for oceanographic research. In Proceedings of the OCEANS 2007, Vancouver, BC, Canada, 29 September–4 October 2007; IEEE: Vancouver, BC, Canada, 2008. [Google Scholar]
- Claustre, H.; Beguery, L. SeaExplorer glider breaks two world records. Sea Technol. 2014, 55, 19–21. [Google Scholar]
- Nakamura, M.; Hyodo, T.; Koterayama, W. “LUNA” testbed vehicle for virtual mooring. In Proceedings of the Seventeenth International Offshore and Polar Engineering, Lisbon, Portugal, 1–6 July 2007; International Society of Offshore and Polar Engineers: Lisbon, Portugal, 2007. [Google Scholar]
- Nakamura, M.; Koterayama, W.; Inada, M.; Marubayashi, K.; Hyodo, T.; Yoshimura, H.; Morii, Y. Disk-type underwater glider for virtual mooring and field experiment. Int. J. Offshore Polar Eng. 2009, 19, 66–70. [Google Scholar]
- Nakamura, M.; Koterayama, W.; Ito, Y.; Matsuno, A.; Noda, J.; Morii, Y.; Yamawaki, N.; Shimizu, K. Oceanographic observation by disk type underwater glider for virtual mooring. In Proceedings of the 27th International Ocean and Polar Engineering Conference, San Francisco, CA, USA, 25–30 June 2017; International Society of Offshore and Polar Engineers: San Francisco, CA, USA, 2017. [Google Scholar]
- Nakamura, M.; Noda, J.; Kajiwara, H.; Shinkai, Y. Study of influence of vertical tail wing of disk-type underwater glider on motion control. In Proceedings of the 28th International Ocean and Polar Engineering Conference, Sapporo, Japan, 10–15 June 2018; International Society of Offshore and Polar Engineers: Sapporo, Japan, 2018. [Google Scholar]
- Yu, P.Y.; Wang, T.L.; Zhou, H.; Shen, C. Dynamic modeling and three-dimensional motion simulation of a disk type underwater glider. Int. J. Nav. Archit. Ocean Eng. 2018, 10, 318–328. [Google Scholar] [CrossRef]
- Zhu, Y.D. Analysis of Motion and Bottom Sitting Performance of the Dish Shaped Underwater Glider. Master’s Thesis, Dalian Maritime University, Dalian, China, 2017. [Google Scholar]
- Zhou, H.; Wang, T.L.; Sun, L.; Lan, W. Study on the vertical motion characteristics of disc-type underwater gliders with zero pitch angle. J. Mar. Sci. Technol. 2019, 25, 828–841. [Google Scholar] [CrossRef]
- Zhang, C. The Design and Motion Control of Round Dish-Shaped Underwater Vehicle. Master’s Thesis, Harbin Engineering University, Harbin, China, 2013. [Google Scholar]
- Wang, T.; Ye, X.F.; Wang, L.; Li, H. Grid search optimized SVM method for dish-like underwater robot attitude prediction. In Proceedings of the 2012 Fifth International Joint Conference on Computational Sciences and Optimization, Harbin, China, 23–26 June 2012; IEEE: Harbin, China, 2012. [Google Scholar]
- Yu, P.Y.; Shen, C.; Wang, T.L.; Zhang, B.R. Research on shape design of disk type of underwater glider. Shipbuild. China 2018, 59, 42–50. [Google Scholar]
- Zhao, B.Q. Study of modeling and simulation of disc-underwater glider dynamic stability. Ship. Sci. Technol. 2016, 38, 82–86. [Google Scholar]
- Wang, H.L.; Wang, D.; Wang, T.L.; Zhang, Y.B.; Gu, N. Adaptive cooperative diving of saucer-type underwater gliders subject to model uncertainties and input constraints. IEEE Access 2019, 7, 60042–60054. [Google Scholar] [CrossRef]
- Jin, X. Research on Formation Change and Path Planning of the Multi-Underwater-Glider System. Master’s Thesis, Dalian Maritime University, Dalian, China, 2018. [Google Scholar]
- Zhou, H.; Wang, T.L.; Yu, P.Y. Simulation of the motion of disc type underwater glider. J. Harbin Eng. Univ. 2019, 40, 696–702. [Google Scholar]
- Bhatta, P.; Naomi, E.L. Nonlinear gliding stability and control for vehicles with hydrodynamic forcing. Automatica 2008, 44, 1240–1250. [Google Scholar] [CrossRef]
- Kan, L.; Zhang, Y.W.; Fan, H.; Yang, W.G.; Chen, Z.K. MATLAB-based simulation of buoyancy-driven underwater glider motion. J. Ocean. Univ. China 2008, 8, 117–122. [Google Scholar] [CrossRef]
- Yang, C.J.; Peng, S.L.; Fan, S.S. Performance and stability analysis for ZJU glider. Mar. Technol. Soc. J. 2014, 48, 88–103. [Google Scholar] [CrossRef]
- Zhang, S.W.; Yu, J.C.; Zhang, A.Q.; Zhang, F.M. Spiraling motion of underwater gliders: Modeling, analysis, and experimental results. Ocean. Eng. 2013, 60, 1–13. [Google Scholar] [CrossRef]
- Cao, J.J.; Cao, J.L.; Yao, B.H.; Lian, L. Three dimensional model, hydrodynamics analysis and motion simulation of an underwater glider. In Proceedings of the IEEE OCEANS 2015, Genova, Italy, 18–21 May 2015; IEEE: Genova, Italy, 2015. [Google Scholar]
- Liu, F.; Wang, Y.H.; Wu, Z.L.; Wang, S.X. Motion analysis and trials of the deep sea hybrid underwater glider Petrel-II. China Ocean. Eng. 2017, 31, 55–62. [Google Scholar] [CrossRef]
- Fan, S.S.; Woolsey, C.A. Dynamics of underwater gliders in currents. Ocean. Eng. 2014, 84, 249–258. [Google Scholar] [CrossRef]
- Gu, J.N.; Li, Q.J.; Gao, L.; Zhang, Z.H. Modeling and simulation of the motion performance of underwater glider. J. Huazhong Univ. Sci. Tech. 2016, 44, 76–80. [Google Scholar]
- Wang, J.Q.; Wang, C.; Wei, Y.J.; Zhang, C.J. Hydrodynamic characteristics and motion simulation of flying-wing dish-shaped autonomous underwater glider. J. Harbin Inst. Technol. 2018, 50, 131–137. [Google Scholar]
- Yu, P.Y.; Shen, C.; Zhen, C.B.; Wang, T.L. Analysis of the steady spiral motion of an underwater glider on temporal and spatial scales. Ship. Sci. Technol. 2019, 41, 71–75. [Google Scholar]
- Zhou, H.; Wang, T.L.; Yu, P.Y. Study on motion characteristics of disc type underwater glider. J. Huazhong Univ. Sci. Tech. (Nat. Sci. Ed.) 2019, 46, 112–118. [Google Scholar]
- Eichhorn, M. Optimal routing strategies for autonomous underwater vehicles in time-varying environment. Rob. Auton. Syst. 2013, 67, 33–43. [Google Scholar] [CrossRef]
- Fossen, T.I. Handbook of Marine Craft Hydrodynamics and Motion Control; John Wiley & Sons: New York, NY, USA, 2011. [Google Scholar]
- Zhou, H. Research on the Motion Performance of the Disk Type Underwater Glider. Master’s Thesis, Dalian Maritime University, Dalian, China, 2017. [Google Scholar]
- Wang, T. Reaearches on a Novel Disk-like Underwater Robot and Attitude Predictive Control. Ph.D. Thesis, Harbin Engineering University, Harbin, China, 2013. [Google Scholar]
- Asakawa, K.; Nakamura, M.; Hyakudome, T.; Maeda, Y.; Ishihara, Y. Spiral gliding experiments of the underwater glider for long-term observation. In Proceedings of the OCEANS 2018 MTS/IEEE Charleston, Charleston, SC, USA, 22–25 October 2018; IEEE: Charleston, SC, USA, 2018. [Google Scholar]
- Wang, S.X.; Song, Y.; Wang, Y.H.; Niu, W.D.; Lei, Z. Sensitivity analysis of energy consumption parameters for petrel-II underwater glider based on sobol’ method. J. Tianjin Univ. (Sci. Technol.) 2017, 50, 113–119. [Google Scholar]
- McKay, M.D.; Beckman, R.J.; Conover, W.J. A comparison of three methods for selecting values of input variables in the analysis of output from a computer code. Technometrics 2000, 42, 55–61. [Google Scholar] [CrossRef]
- Helton, J.C.; Davis, F.J. Latin hypercube sampling and the propagation of uncertainty in analyses of complex systems. Reliab. Eng. Syst. Saf. 2003, 81, 23–69. [Google Scholar] [CrossRef]
- Bhatta, P.; Leonard, N.E. A Lyapunov function for vehicles with lift and drag: Stability of gliding. In Proceedings of the 2004 43rd IEEE Conference on Decision and Control (CDC) (IEEE Cat. No. 04CH37601), Nassau, Bahamas, 14–17 December 2004. [Google Scholar]
- Wang, Y.H. Dynamical Behavior and Robust Control Strategies of the Underwater Gliders. Ph.D. Thesis, Tianjin University, Tianjin, China, 2007. [Google Scholar]
- Zhao, B.Q.; Wang, X.H.; Yao, B.H.; Lian, L. Lyapunov stability of the underwater glider. J. Harbin Eng. Univ. 2015, 36, 83–87. [Google Scholar]
- Graver, J.G. Underwater Gliders: Dynamics, Control and Design. Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 2005. [Google Scholar]
- Asakawa, K.; Nakamura, M.; Kobayashi, T.; Watanabe, Y.; Kojima, J. Design concept of Tsukuyomi Underwater glider prototype for virtual mooring. In Proceedings of the Oceans 2011, Santander, Spain, 6–9 June 2011; IEEE: Santander, Spain, 2011. [Google Scholar]
- Hussain, N.A.A.; Chung, T.M.; Arshad, M.R.; Mokhtar, R.M.; Abdullah, M.Z. Design of an underwater glider platform for shallow-water applications. Int. J. Intell. Def. Support Syst. 2010, 3, 186. [Google Scholar] [CrossRef]
- Niu, W.D.; Wang, S.X.; Wang, Y.H.; Song, Y.; Zhu, Y.Q. Stability analysis of hybrid-driven underwater glider. China Ocean. Eng. 2017, 31, 528–538. [Google Scholar] [CrossRef]
- Chen, Z.; Yu, J.C.; Zhang, A.Q.; Zhang, F.M. Design and analysis of folding propulsion mechanism for hybrid-driven underwater gliders. Ocean. Eng. 2016, 119, 125–134. [Google Scholar] [CrossRef]
- Ge, Z.; Ji, G.R.; Yan, P.; Qin, F.L.; Li, G.Q. Research on movement performance of a vector propulsion autonomous underwater vehicle. In Proceedings of the Oceans 2019, Marseille, France, 17–20 June 2019; IEEE: Marseille, France, 2019. [Google Scholar]







| Parameter | Meaning | Parameter | Meaning |
|---|---|---|---|
| m | total stationary mass of DTUG | rpi (i = 1, 2, 3, 4) | position vector of mpi |
| mh | fixed mass | rb | position vector of mb |
| mw | fixed-point mass | u | linear velocity (x-direction) |
| mp | the sum of four movable point masses | v | linear velocity (y-direction) |
| mpi (i = 1, 2, 3, 4) | movable point mass | w | linear velocity (z-direction) |
| mb | variable ballast point mass | p | angular velocity (x-direction) |
| mv | mass of the fluid displaced by DTUG | q | angular velocity (y-direction) |
| m0 | net buoyancy | r | angular velocity (z-direction) |
| b | position vector of DTUG | REB | rotation matrix |
| rw | position vector of mw | RΛB | transformation matrix |
| Variable in the Inertial Frame | Meaning | Variable in the Body Frame | Meaning |
|---|---|---|---|
| U | linear velocity | P | static force |
| Ω | angular velocity | TP | static moment |
| RG | Position of the CG | rCG | position vector of the CG |
| JG | inertial matrix | rCB | position vector of the CB |
| FS | external force | (xG, yG, zG) | projection of the rCG on the coordinate axes of the body frame |
| TS | external moment | (xB, yB, zB) | projection of the rCB on the coordinate axes of the body frame |
| PS | static force | (xrb, yrb, zrb) | coordinates of the DTUG’s CG without moveable masses |
| static moment | F | external force | |
| hydrodynamic force | T | external moment | |
| hydrodynamic moment | Fx, Fy, Fz | expansion of F | |
| GS | gravity | Mx, My, Mz | expansion of T |
| BS | buoyancy | PH, TH | hydrodynamic force and moment |
| Coefficient | Unit | Value | Coefficient | Unit | Value |
|---|---|---|---|---|---|
| A11 | 1 | 0.039 | Yv|v| | 1 | −0.011 |
| A22 | 1 | 0.039 | Zw | 1 | −1.17 |
| A33 | 1 | 0.65 | Kp | 1 | −0.11 |
| A44 | 1 | 0.036 | Kp|p| | 1 | −0.14 |
| A55 | 1 | 0.036 | Mw | 1 | 0.28 |
| A66 | 1 | 2.8 × 10−5 | Mq | 1 | −0.11 |
| Xuu | 1 | −0.034 | Mq|q| | 1 | −0.14 |
| Xww | 1 | 0.45 | Nr | 1 | −1.9 × 10−3 |
| Yv | 1 | −0.017 | Nr|r| | 1 | −5.5 × 10−5 |
| Parameter | Unit | Value |
|---|---|---|
| mh | kg | 135 |
| mp1 | kg | 2 |
| mp2 | kg | 2 |
| mp3 | kg | 2 |
| mp4 | kg | 2 |
| mb | kg | 1.3 |
| D | m | 1.38 |
| rp | m | 0.215 |
| Parameter | First-Order Sensitivity Coefficient | Global Sensitivity Coefficient |
|---|---|---|
| xG | 0.046 | 1.006 |
| yG | 0.071 | 1.051 |
| td | 0.067 | 1.050 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, H.; Yu, P.; Jin, X.; Wang, T. Analysis of the In Situ Steering Motion Characteristics and Sensitivity of Disc-Type Underwater Gliders. J. Mar. Sci. Eng. 2020, 8, 663. https://doi.org/10.3390/jmse8090663
Zhou H, Yu P, Jin X, Wang T. Analysis of the In Situ Steering Motion Characteristics and Sensitivity of Disc-Type Underwater Gliders. Journal of Marine Science and Engineering. 2020; 8(9):663. https://doi.org/10.3390/jmse8090663
Chicago/Turabian StyleZhou, Han, Pengyao Yu, Xiang Jin, and Tianlin Wang. 2020. "Analysis of the In Situ Steering Motion Characteristics and Sensitivity of Disc-Type Underwater Gliders" Journal of Marine Science and Engineering 8, no. 9: 663. https://doi.org/10.3390/jmse8090663
APA StyleZhou, H., Yu, P., Jin, X., & Wang, T. (2020). Analysis of the In Situ Steering Motion Characteristics and Sensitivity of Disc-Type Underwater Gliders. Journal of Marine Science and Engineering, 8(9), 663. https://doi.org/10.3390/jmse8090663






