3D Numerical Simulation of the Interaction between Waves and a T-Head Groin Structure
Abstract
1. Introduction
2. Governing Equations
3. The Numerical Scheme
3.1. Boundary Conditions
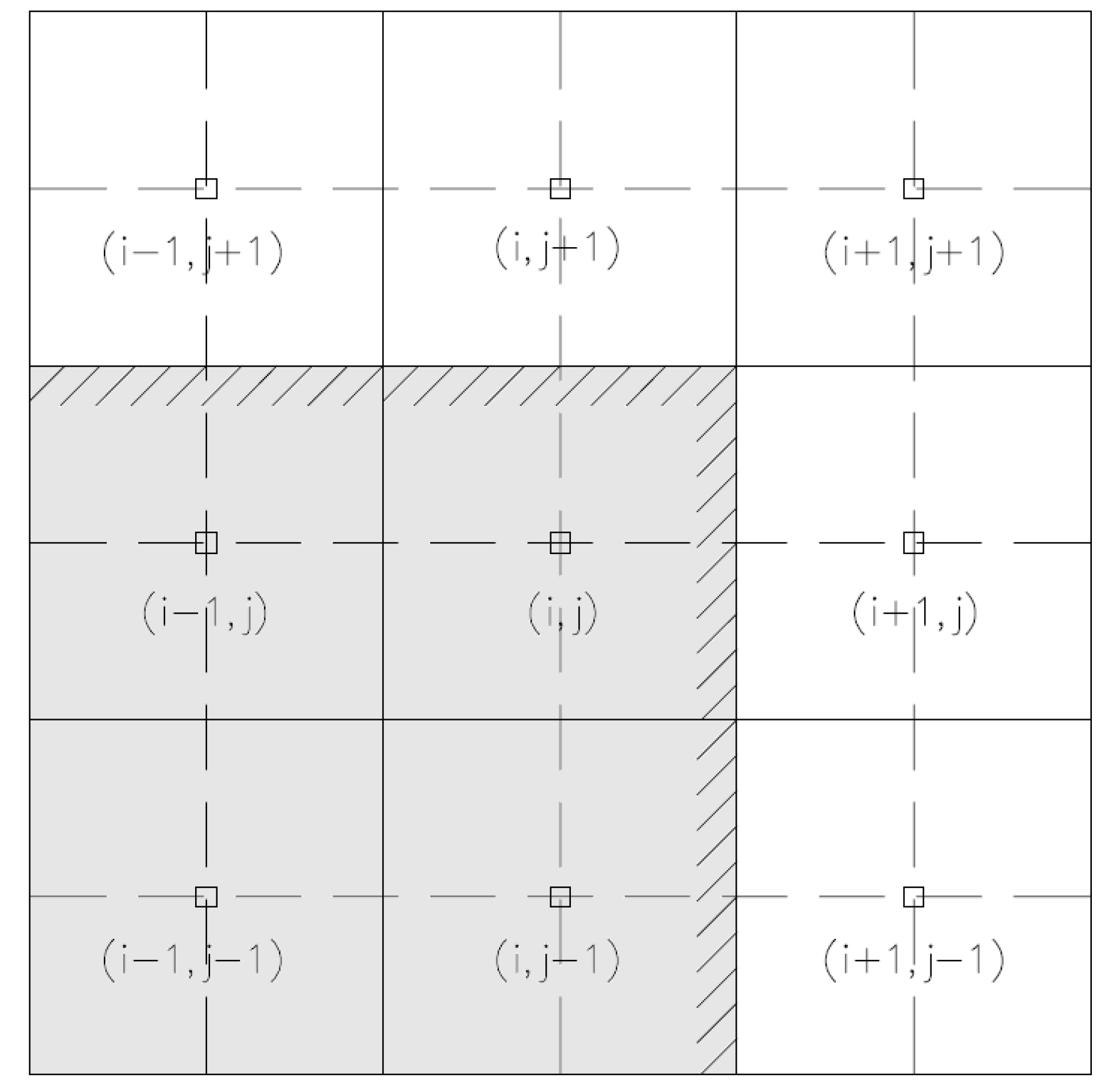
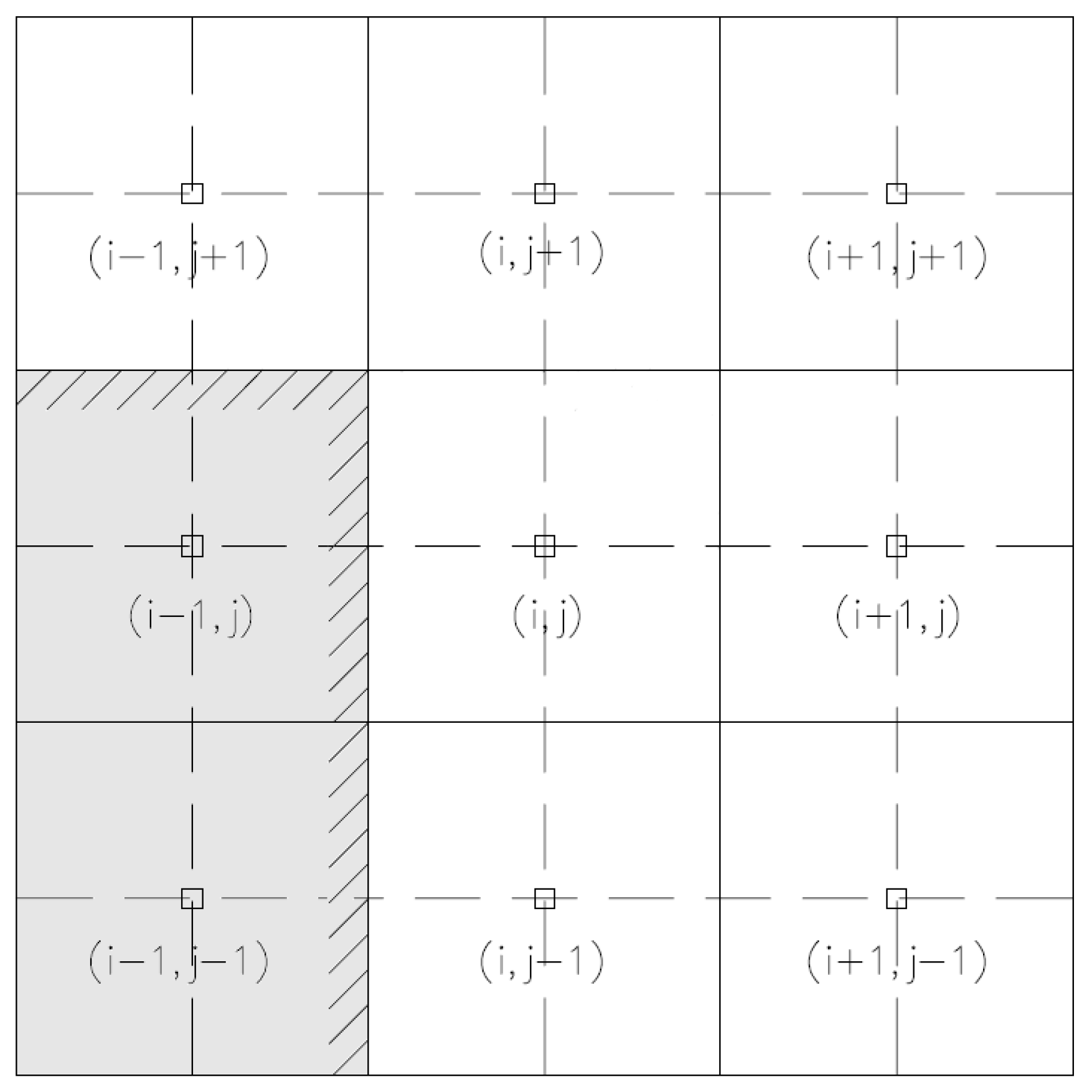
3.1.1. Internal Boundary Conditions
3.1.2. Free-Surface Boundary Conditions
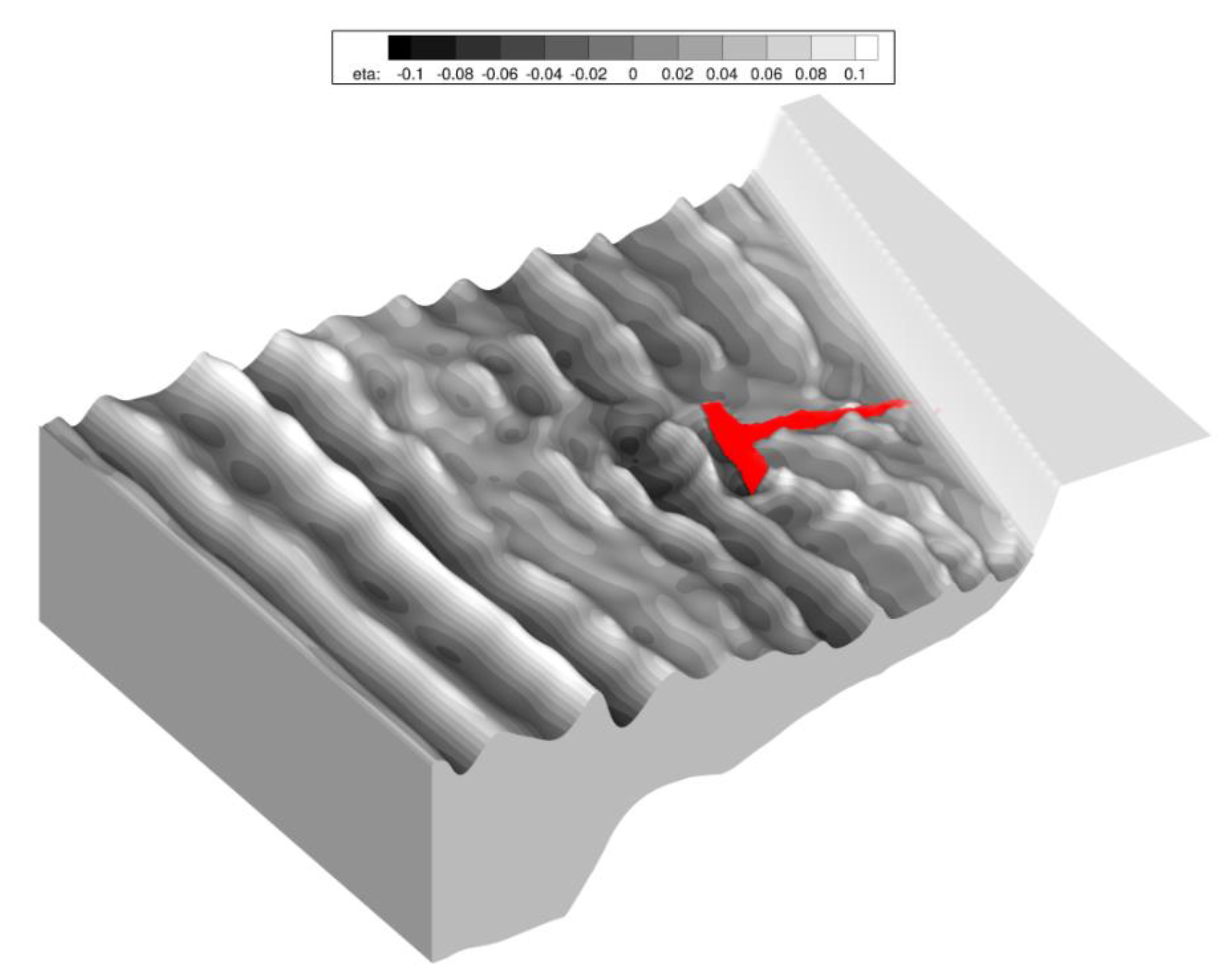
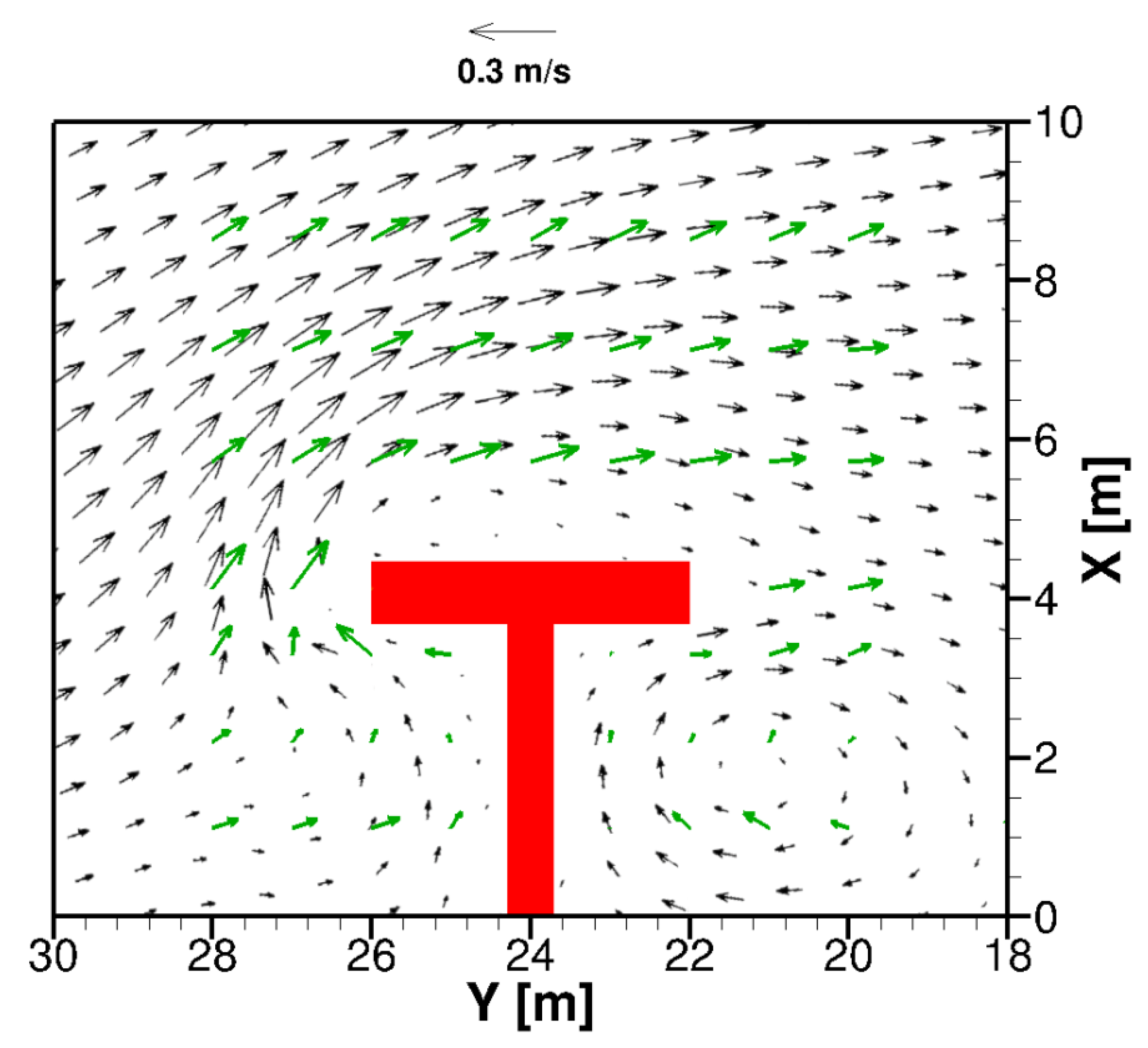
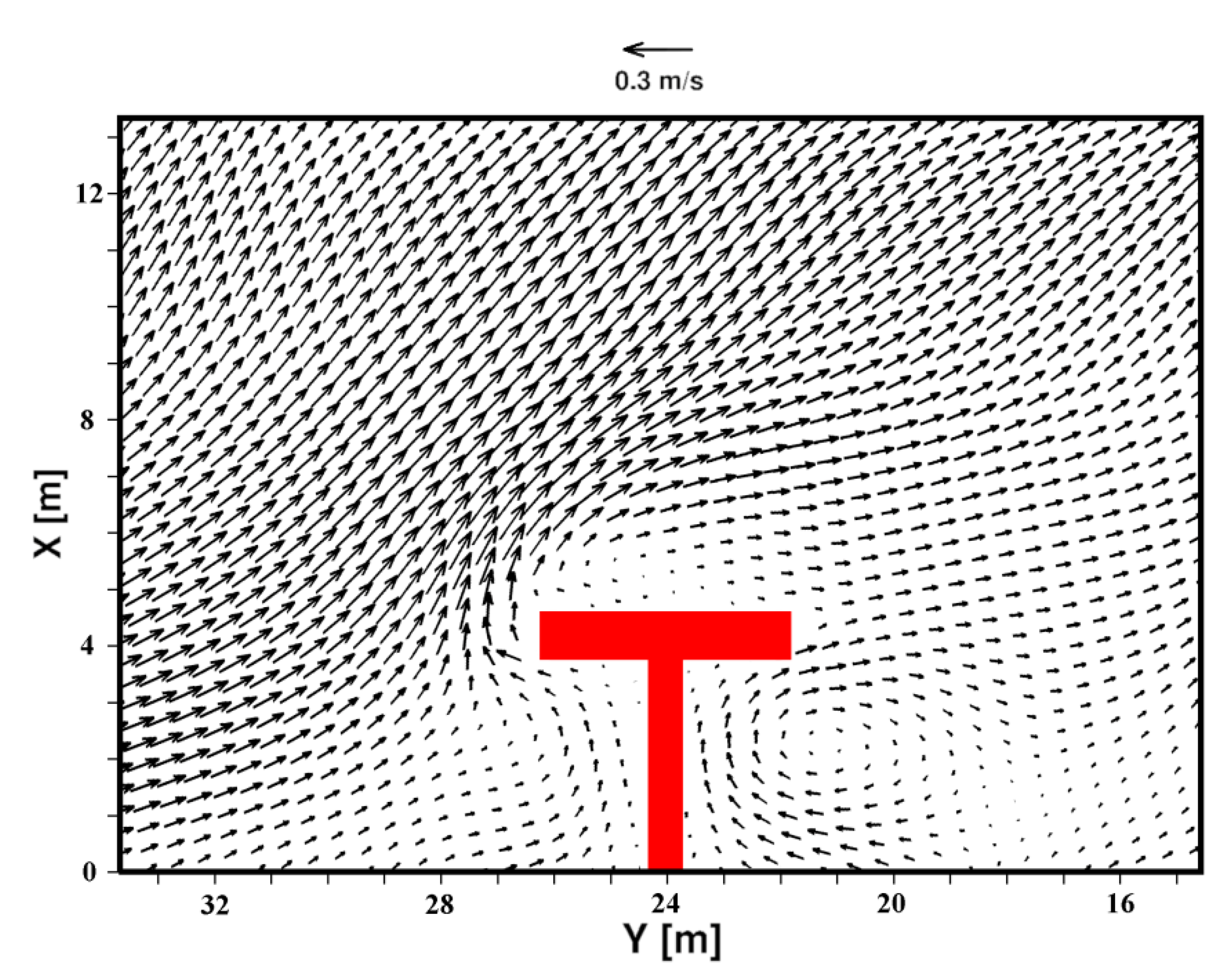
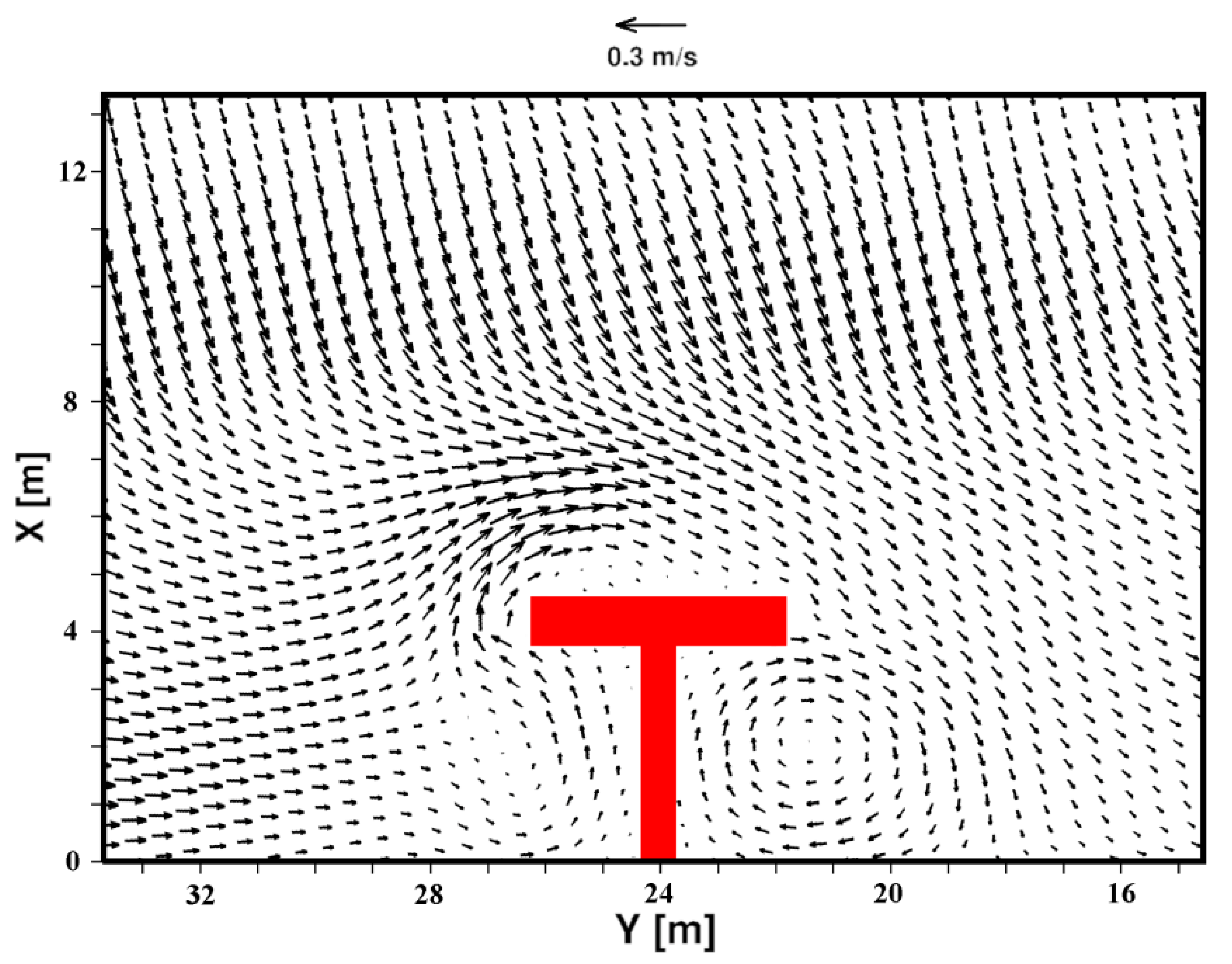
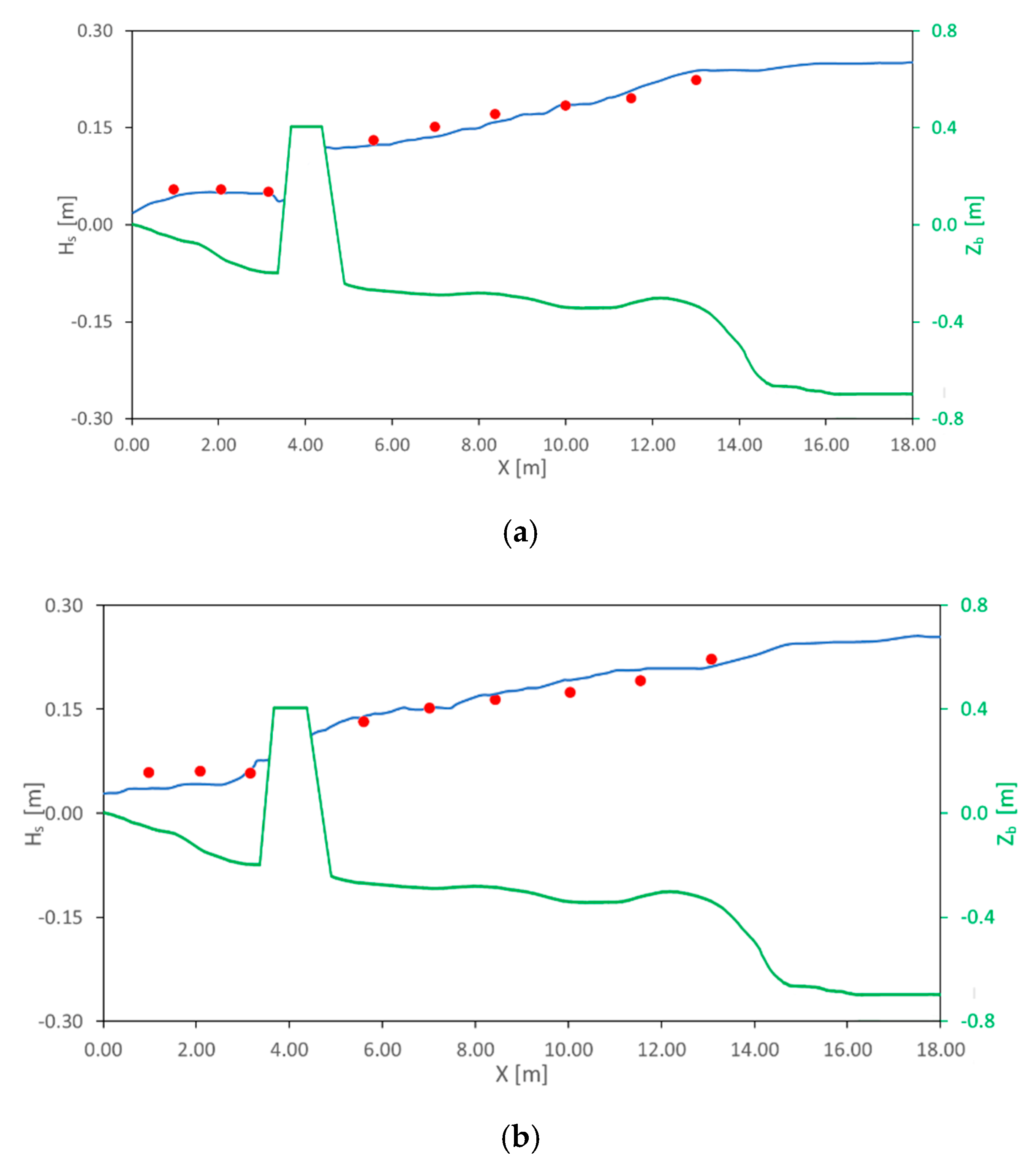
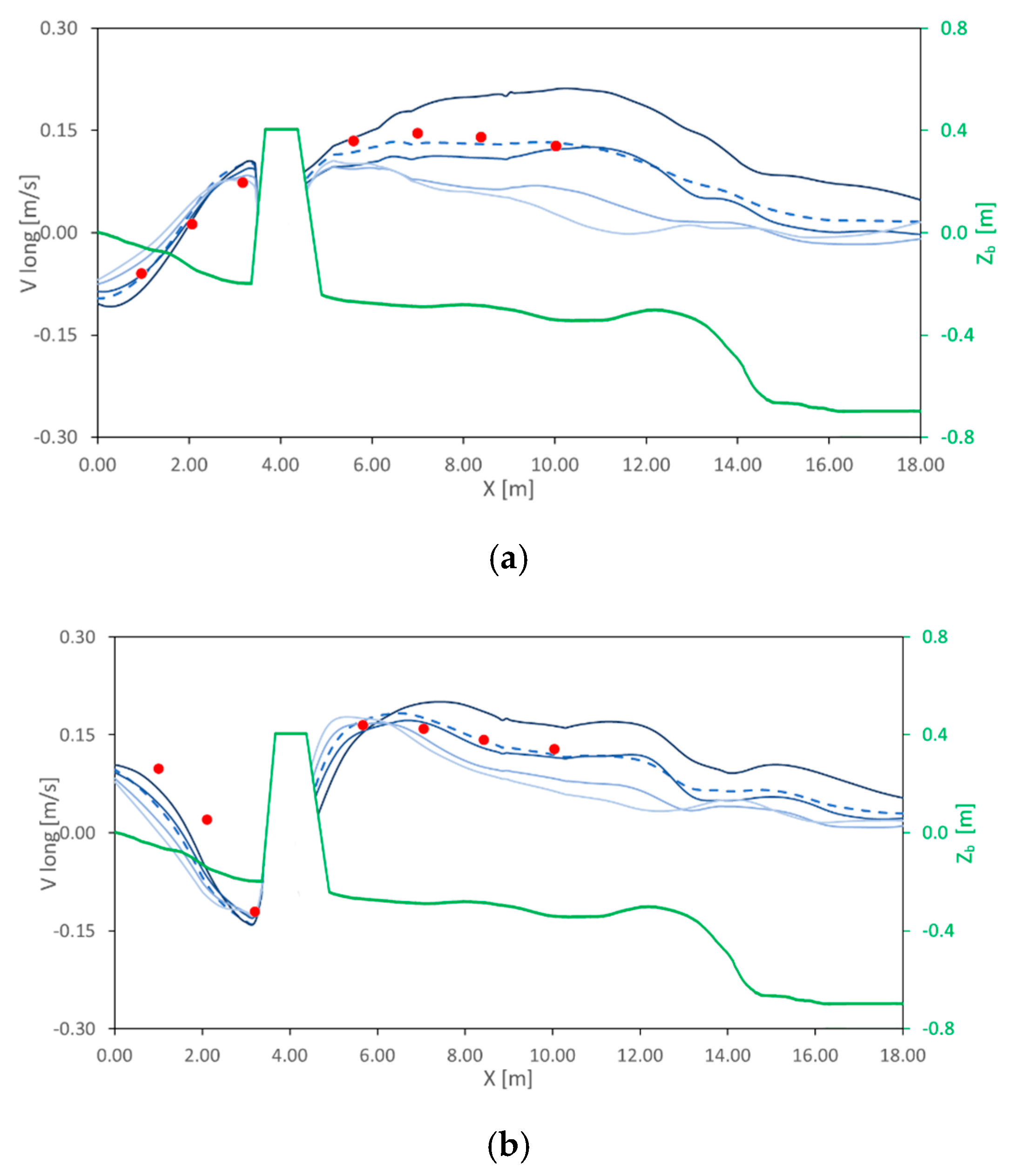
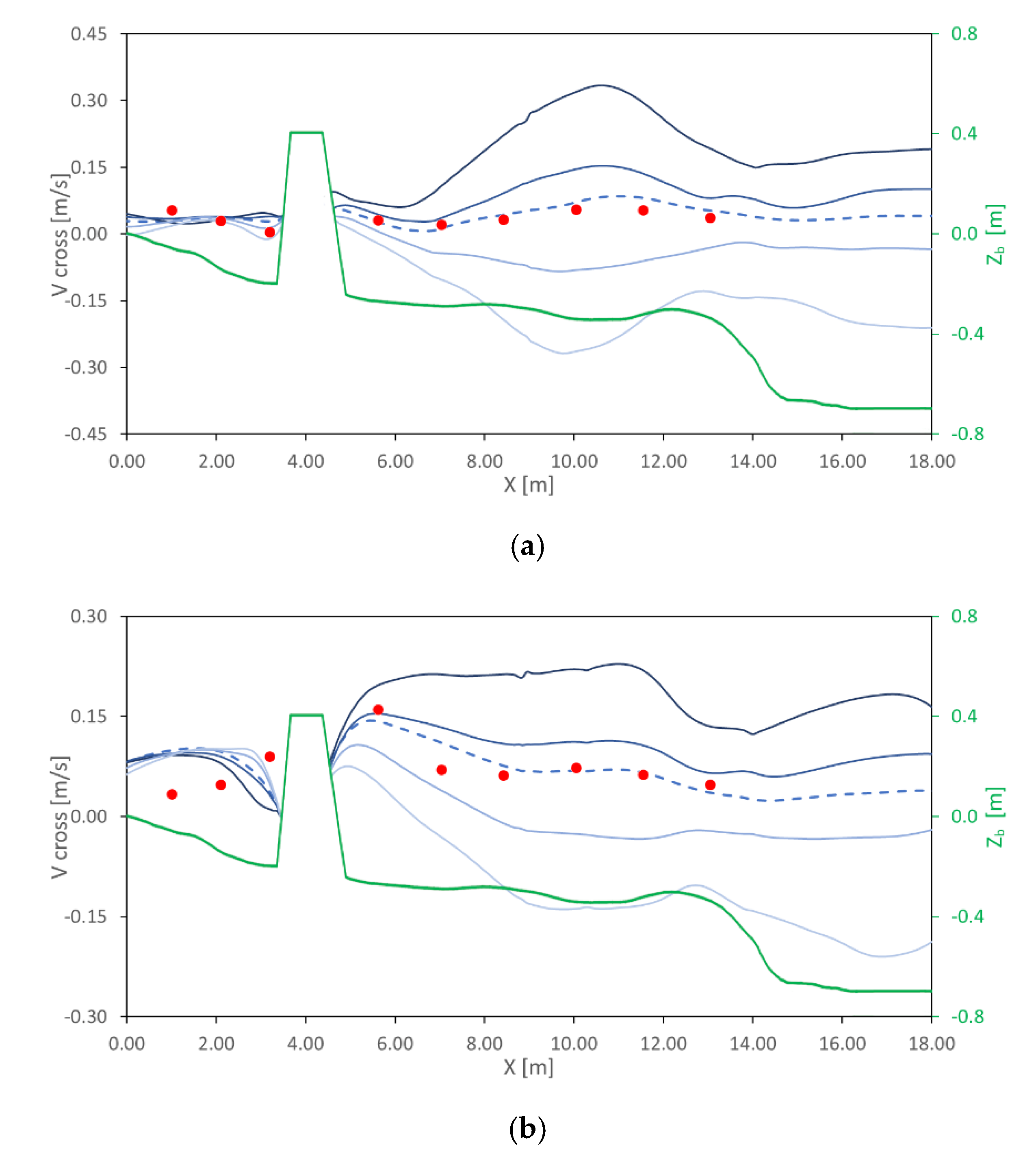
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Moore, B.D.; Humiston, K.K. Composite T-Head Groins for Erosion Control. In Proceedings of the Coastal Structures 2003, Portland, OR, USA, 26–30 August 2003. [Google Scholar]
- Özölçer, İ.H.; Kömürcü, M.İ.; Birben, A.R.; Yüksek, Ö.; Karasu, S. Effects of T-Shape Groin Parameters on Beach Accretion. Ocean Eng. 2006, 33, 382–403. [Google Scholar] [CrossRef]
- Li, B.; Yu, X.; Yu, Y. Nonlinear dynamics of nearshore irregular waves: A threedimensional numerical model and its validation. Coast. Eng. J. 2007, 49, 103–126. [Google Scholar] [CrossRef]
- Gallerano, F.; Cannata, G.; De Gaudenzi, O.; Scarpone, S. Modeling Bed Evolution Using Weakly Coupled Phase-Resolving Wave Model and Wave-Averaged Sediment Transport Model. Coast. Eng. J. 2016, 58, 1650011. [Google Scholar] [CrossRef]
- Ma, G.; Chou, Y.J.; Shi, F. A wave-resolving model for nearshore suspended sediment transport. Ocean Model. 2014, 77, 33–49. [Google Scholar] [CrossRef]
- Nam, P.T.; Oumeraci, H.; Larson, M.; Hanson, H. Modeling undertow due to random waves. Ocean Dyn. 2014, 64, 1209–1219. [Google Scholar] [CrossRef]
- Hu, K.; Mingham, C.G.; Causon, D.M. Numerical simulation of wave overtopping of coastal structures using the non-linear shallow water equations. Coast. Eng. 2000, 41, 433–465. [Google Scholar] [CrossRef]
- Cannata, G.; Petrelli, C.; Barsi, L.; Fratello, F.; Gallerano, F. A dam-break flood simulation model in curvilinear coordinates. WSEAS Trans. Fluid Mech. 2018, 13, 60–70. [Google Scholar]
- Tonelli, M.; Petti, M. Shock-capturing Boussinesq model for irregular wave propagation. Coast. Eng. 2012, 61, 8–19. [Google Scholar] [CrossRef]
- Cannata, G.; Barsi, L.; Petrelli, C.; Gallerano, F. Numerical investigation of wave fields and currents in a coastal engineering case study. WSEAS Trans. Fluid Mech. 2018, 13, 87–94. [Google Scholar]
- Guerrini, M.; Bellotti, G.; Fan, Y.; Franco, L. Numerical modelling of long waves amplification at Marina di Carrara Harbour. Appl. Ocean Res. 2014, 48, 322–330. [Google Scholar] [CrossRef]
- Lynett, P.J. Nearshore wave modeling with high-order Boussinesq-type equations. J. Waterw. Port Coast. Ocean Eng. 2006, 132, 348–357. [Google Scholar] [CrossRef]
- Chen, X. A fully hydrodynamic model for three-dimensional free-surface flows. Int. J. Numer. Meth. Fluids 2003, 42, 929–952. [Google Scholar] [CrossRef]
- Casulli, V.; Cheng, R.T. Semi-implicit finite-difference methods for three-dimensional shallow flow. Int. J. Numer. Meth. Fluids 1992, 15, 1045–1068. [Google Scholar] [CrossRef]
- De Marchis, M.; Napoli, E. Effects of irregular two-dimensional and three-dimensional surface roughness in turbulent channel flows. Int. J. Numer. Meth. Fluids 2012, 36, 7–17. [Google Scholar] [CrossRef]
- Bradford, S.F. Numerical Simulation of Surf Zone Dynamics. J. Waterw. Port Coast. Ocean Eng. 2000, 126, 1–13. [Google Scholar] [CrossRef]
- Berberović, E.; van Hinsberg, N.P.; Jakirlić, S.; Roisman, I.V.; Tropea, C. Drop impact onto a liquid layer of finite thickness: Dynamics of the cavity evolution. Phys. Rev. E 2009, 79, 036306. [Google Scholar] [CrossRef]
- Higuera, P.; Lara, J.L.; Losada, I.J. Simulating coastal engineering processes with OpenFOAM®. Coast. Eng. 2013, 71, 119–134. [Google Scholar] [CrossRef]
- Montagna, F.; Bellotti, G.; Di Risio, M. 3D numerical modeling of landslide-generated tsunamis around a conical island. Nat. Hazards 2011, 58, 591–608. [Google Scholar] [CrossRef]
- Van der Meer, J.W.; Petit, H.A.H.; van den Bosch, P.; Klopman, G.; Broekens, R.D. Numerical Simulation of Wave Motion on and in Coastal Structures. In Proceedings of the 23rd International Conference on Coastal Engineering, Venice, Italy, 4–9 October 1992. [Google Scholar]
- Romano, A.; Lara, J.L.; Barajas, G.; Di Paolo, B.; Bellotti, G.; Di Risio, M.; Losada, I.; De Girolamo, P. Numerical Modelling of Landslide-Generated Tsunamis with OpenFOAM®: A New Approach. In Proceedings of the Coastal Structures Conference 2019, Hannover, Germany, 30 September–2 October 2019. [Google Scholar]
- Lin, P.; Li, C.W. A σ-coordinate three-dimensional numerical model for surface wave propagation. Int. J. Numer. Meth. Fluids 2002, 38, 1045–1068. [Google Scholar] [CrossRef]
- Lin, P. A multiple-layer σ-coordinate model for simulation of wave–structure interaction. Comput. Fluids 2006, 35, 147–167. [Google Scholar] [CrossRef]
- Ma, G.; Shi, F.; Kirby, J.T. Shock-capturing non-hydrostatic model for fully dispersive surface wave processes. Ocean Model. 2012, 43–44, 22–35. [Google Scholar] [CrossRef]
- Cannata, G.; Gallerano, F.; Palleschi, F.; Petrelli, C.; Barsi, L. Three-dimensional numerical simulation of the velocity fields induced by submerged breakwaters. Int. J. Mech. 2019, 13, 1–14. [Google Scholar]
- Napoli, E.; De Marchis, M.; Vitanza, E. PANORMUS-SPH. A new Smoothed Particle Hydrodynamics solver for incompressible flows. Comput. Fluids 2015, 106, 185–196. [Google Scholar] [CrossRef]
- Monaghan, J. Simulating free surface flows with SPH. J. Comput. Phys. V 1994, 110, 399–406. [Google Scholar] [CrossRef]
- Cannata, G.; Petrelli, C.; Barsi, L.; Gallerano, F. Numerical integration of the contravariant integral form of the Navier–Stokes equations in time-dependent curvilinear coordinate systems for three-dimensional free surface flows. Continuum Mech. Therm. 2019, 31, 491–519. [Google Scholar] [CrossRef]
- Palleschi, F.; Iele, B.; Gallerano, F. Integral contravariant form of the Navier-Stokes equations. WSEAS Trans. Fluid Mech. 2019, 14, 101–113. [Google Scholar]
- Fadlun, E.A.; Verzicco, R.; Orlandi, P.; Mohd-Yusof, J. Combined immersed-boundary finite-difference methods for three-dimensional complex flow simulations. J. Comput. Phys. 2000, 161, 35–60. [Google Scholar] [CrossRef]
- Ma, G.; Farahani, A.A.; Kirby, J.T.; Shi, F. Modeling wave-structure interactions by an immersed boundary method in a σ-coordinate model. Ocean Eng. 2016, 125, 238–247. [Google Scholar] [CrossRef]
- Roman, F.; Napoli, E.; Milici, B.; Armenio, V. An improved immersed boundary method for curvilinear grids. Comput. Fluids 2009, 38, 1510–1527. [Google Scholar] [CrossRef]
- Gravens, M.B.; Wang, P. Data Report: LSTF Experiments—Transport by Waves and Currents & Tombolo Development Behind Headland Structures, Technical Report ERDC/CHL TR-04-9; Coastal and Hydraulics Laboratory, US Army Engineer Research and Development Center: Vicksburg, MS, USA, 2007. [Google Scholar]
- Spiteri, R.J.; Ruuth, S.J. A new class of optimal high-order strong-stability preserving time discretization methods. SIAM J. Numer. Anal. 2002, 40, 469–491. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Pearson Education: London, UK, 2007. [Google Scholar]
- Nam, P.T.; Larson, M. A model of wave and current fields around coastal structures. Proc. Coast. Dyn. 2009, 1–14. [Google Scholar] [CrossRef]
- Tamburrino, M.; Cannata, G. Simulation of wave run-up by means of the exact solution of the wet/dry Riemann problem. WSEAS Trans. Fluid Mech. 2019, 14, 114–123. [Google Scholar]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cannata, G.; Tamburrino, M.; Gallerano, F. 3D Numerical Simulation of the Interaction between Waves and a T-Head Groin Structure. J. Mar. Sci. Eng. 2020, 8, 227. https://doi.org/10.3390/jmse8030227
Cannata G, Tamburrino M, Gallerano F. 3D Numerical Simulation of the Interaction between Waves and a T-Head Groin Structure. Journal of Marine Science and Engineering. 2020; 8(3):227. https://doi.org/10.3390/jmse8030227
Chicago/Turabian StyleCannata, Giovanni, Marco Tamburrino, and Francesco Gallerano. 2020. "3D Numerical Simulation of the Interaction between Waves and a T-Head Groin Structure" Journal of Marine Science and Engineering 8, no. 3: 227. https://doi.org/10.3390/jmse8030227
APA StyleCannata, G., Tamburrino, M., & Gallerano, F. (2020). 3D Numerical Simulation of the Interaction between Waves and a T-Head Groin Structure. Journal of Marine Science and Engineering, 8(3), 227. https://doi.org/10.3390/jmse8030227






