Statistical Correlations of Ship High-Run with Broaching-to and Capsize
Abstract
1. Introduction
2. Review of Methods of Identification of Ship High-Run Incidents in Irregular Seas
2.1. Method Based on the Up-Crossing of the Instantaneous Wave Celerity
2.2. Clustering Method
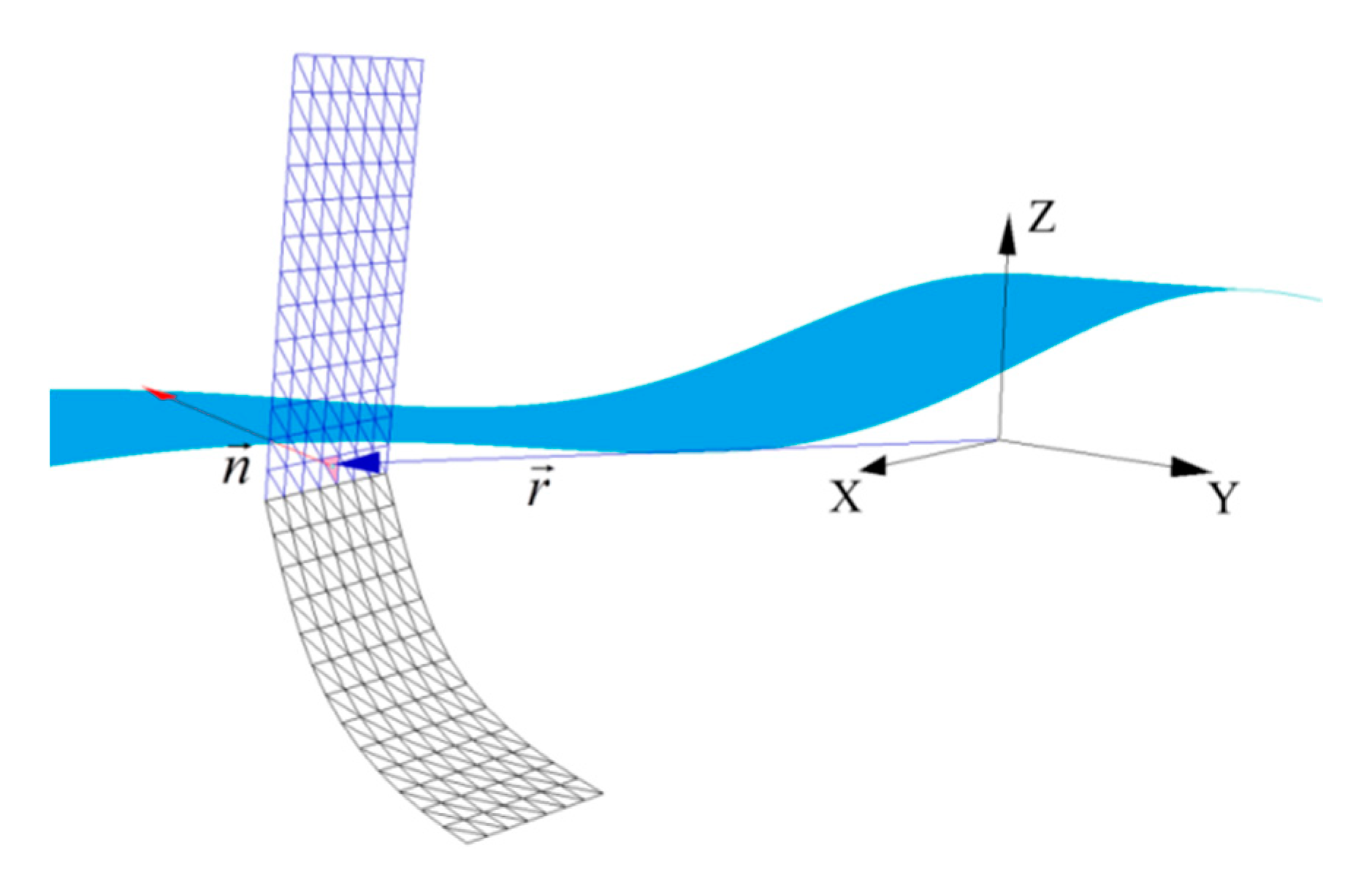
3. Mathematical Model
| Surge | |
| Sway | |
| Roll | |
| Yaw |
4. Statistics of Surf-Riding, Broaching-To and Capsize
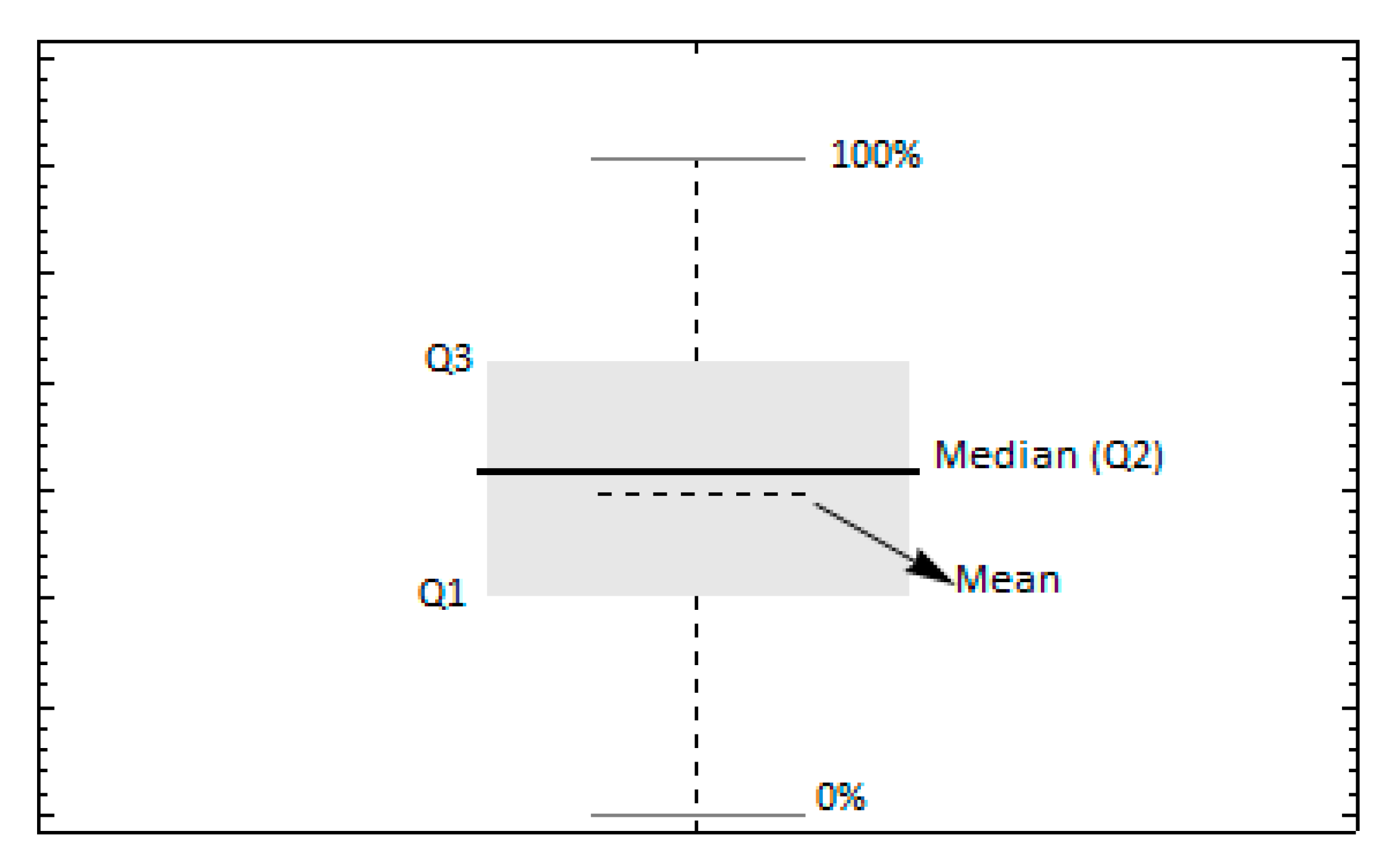
4.1. Key Points of Calculation and Presentation
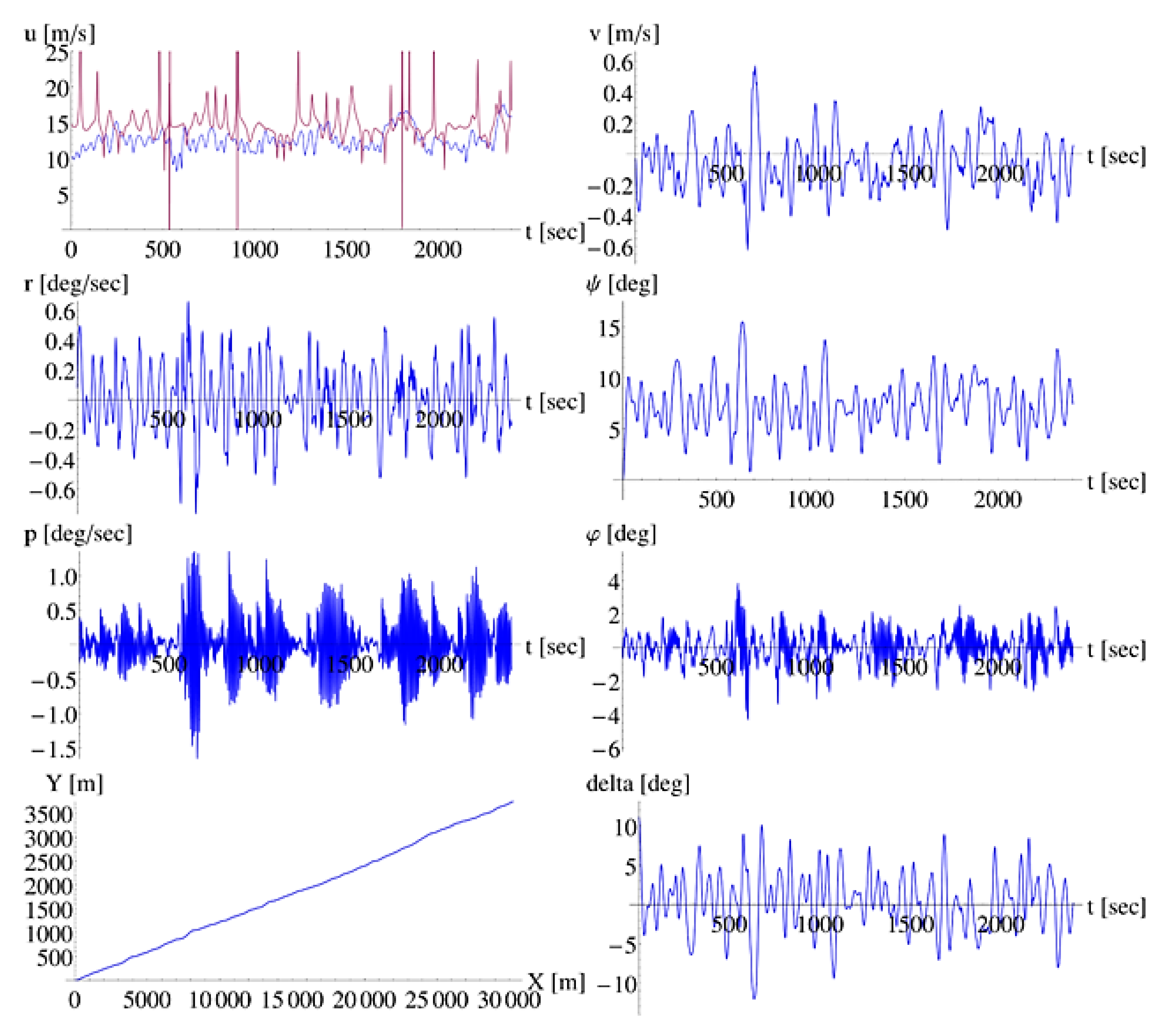
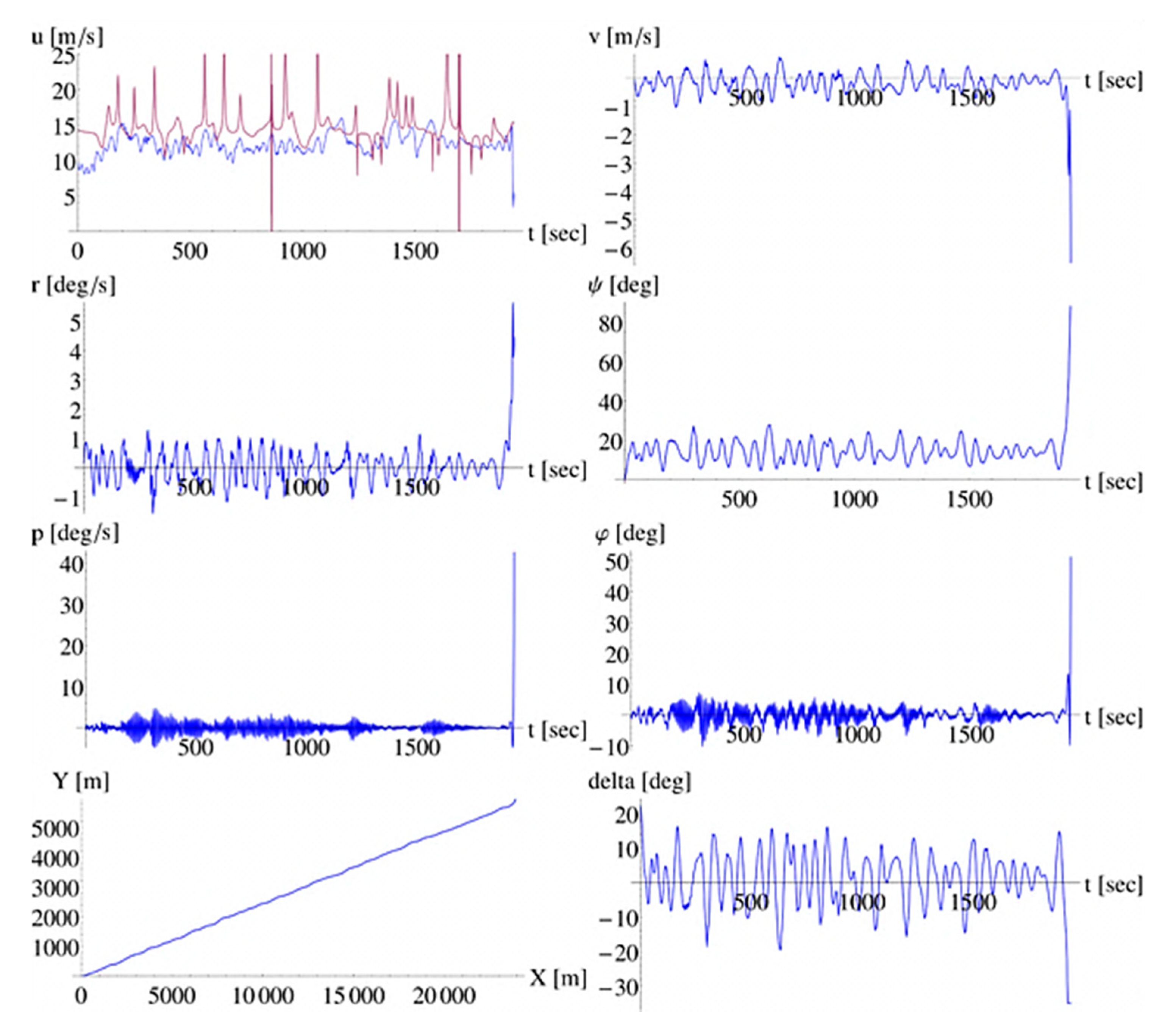
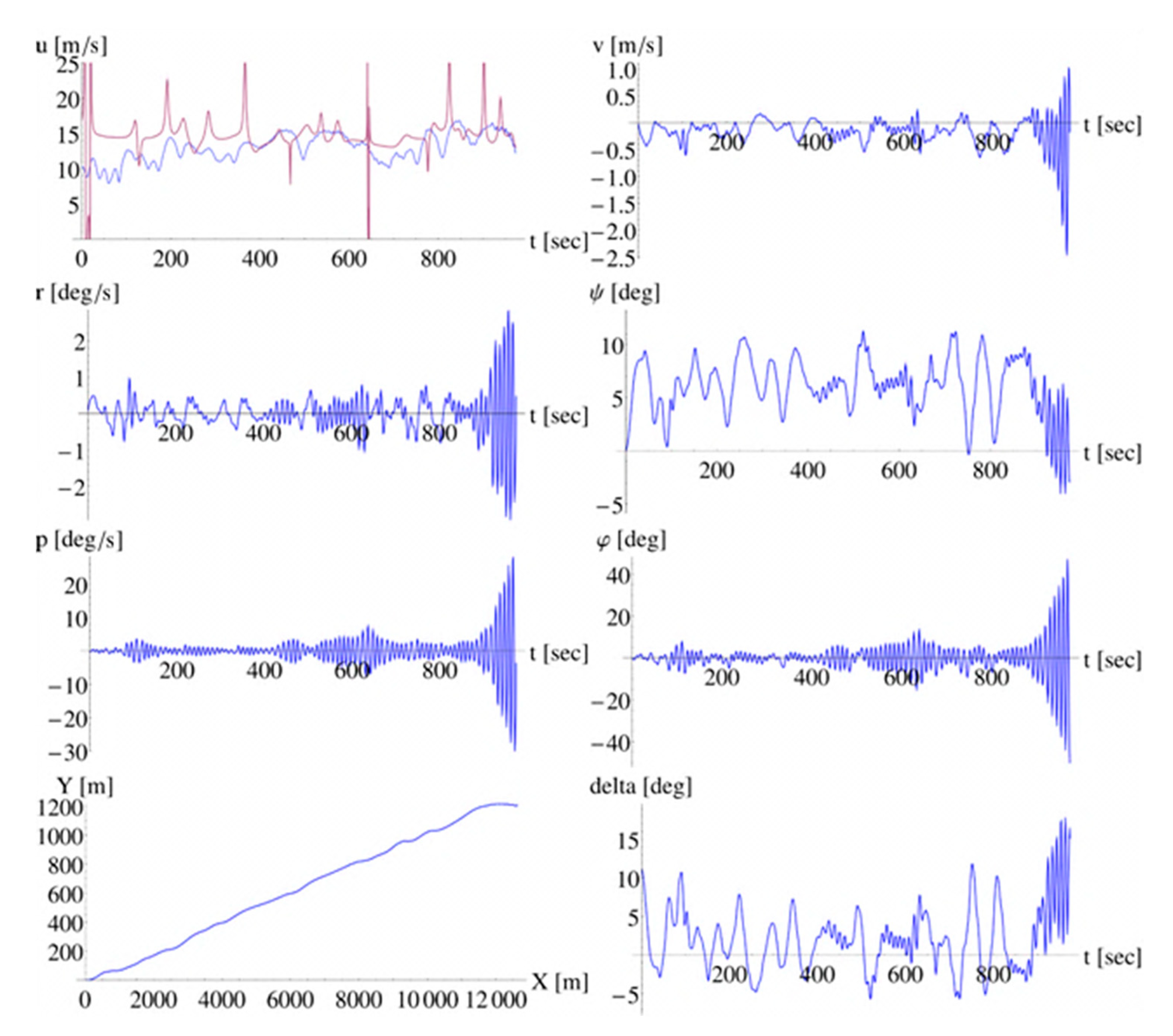
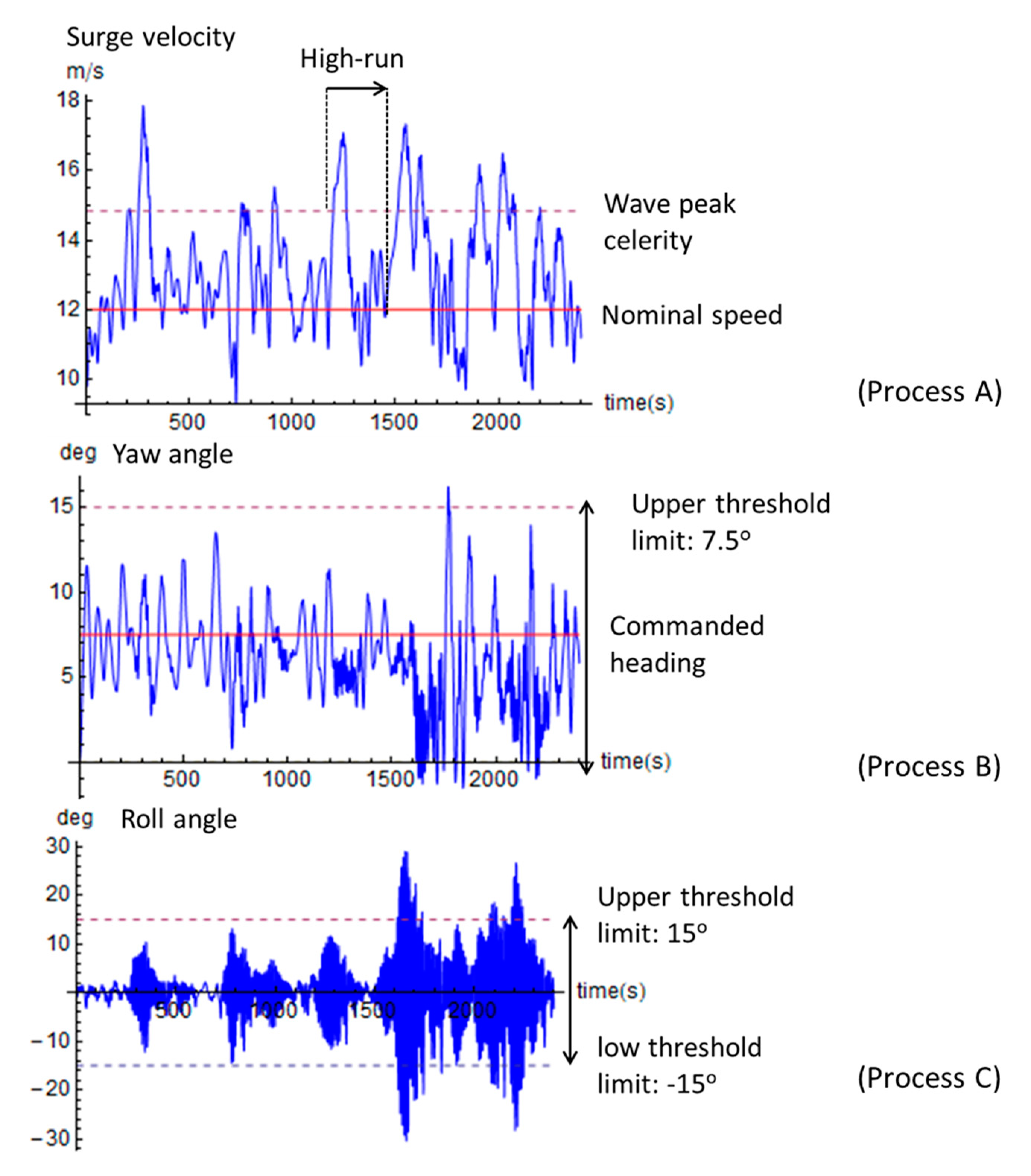
4.1.1. Process A: Surge with High-Runs
4.1.2. Process B: Yaw with Broaching-To
4.1.3. Process C: Roll with Critical Exceedances (“Capsizes”)
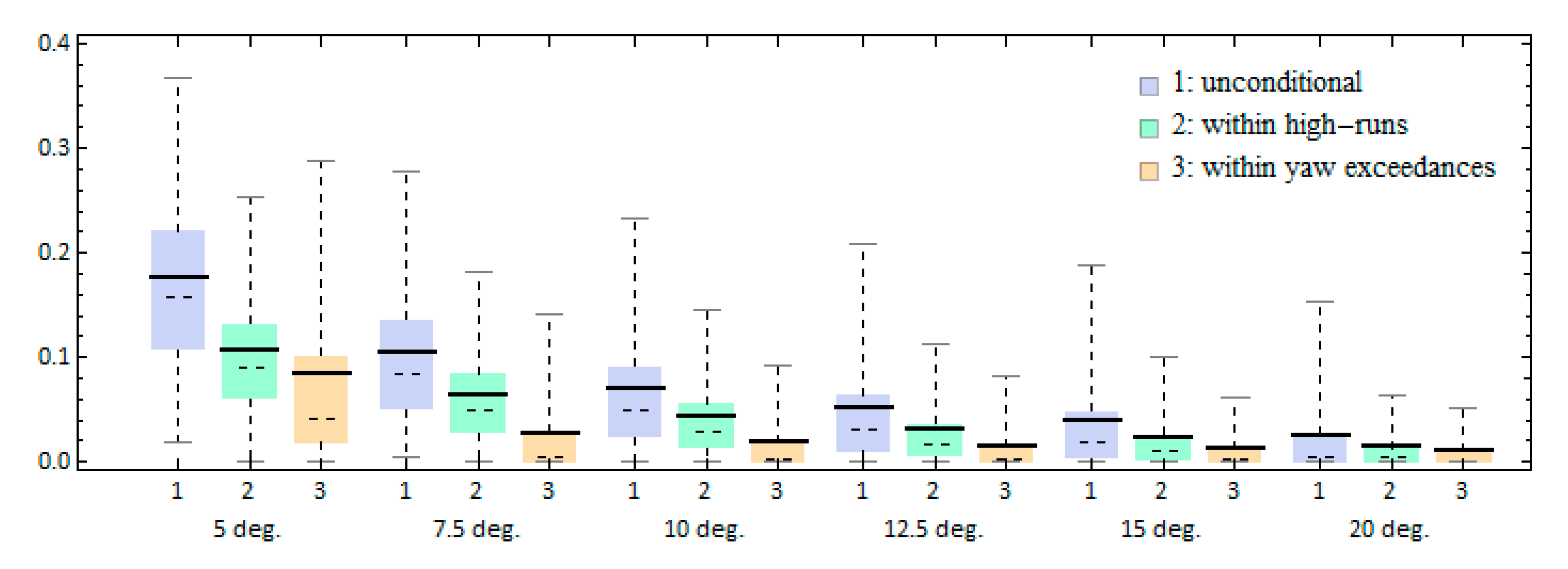
- Mean duration of high-run events.
- The ratio of the aggregate time of high-run to the total simulation time of each realization.
- The largest observed yaw angle during a full run.
- The number of exceedances of the yaw angle threshold.
- The mean duration of the yaw exceedance events.
- The time-ratio of yaw angle exceedance, which is derived by the summation of the times of exceedance to the total simulation time of each realization.
- The largest observed roll angle during a full run.
- The time-ratio of roll angle exceedance, similarly to the calculation scheme of yaw angle exceedance.
- Unconditional occurrences, where any type of roll angle exceedance is taken into account.
- Occurrences conditioned upon a high-run incident.
- Occurrences conditioned upon a yaw angle exceedance.
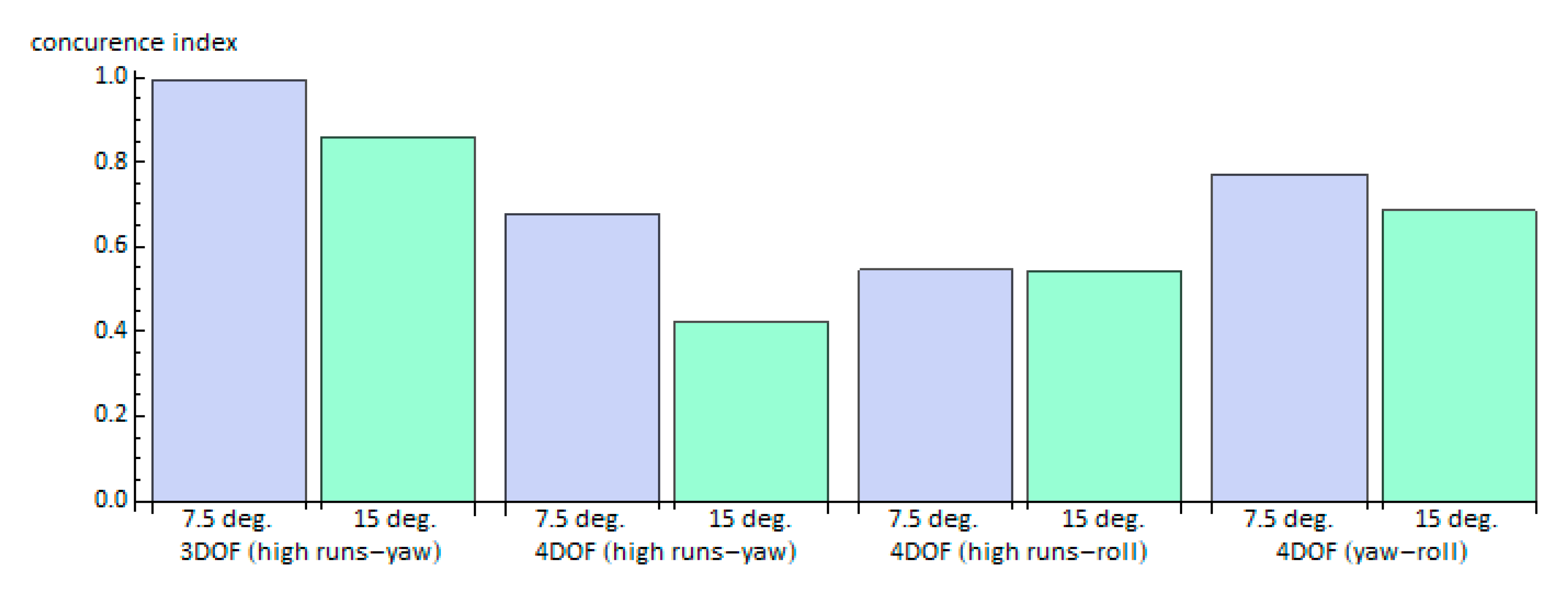
- Index of time concurrence of yaw exceedance and high-run.
- Index of time concurrence of roll exceedance and high-run.
- Index of time concurrence of roll exceedance and yaw exceedance.
4.2. Key Points of Calculation and Presentation
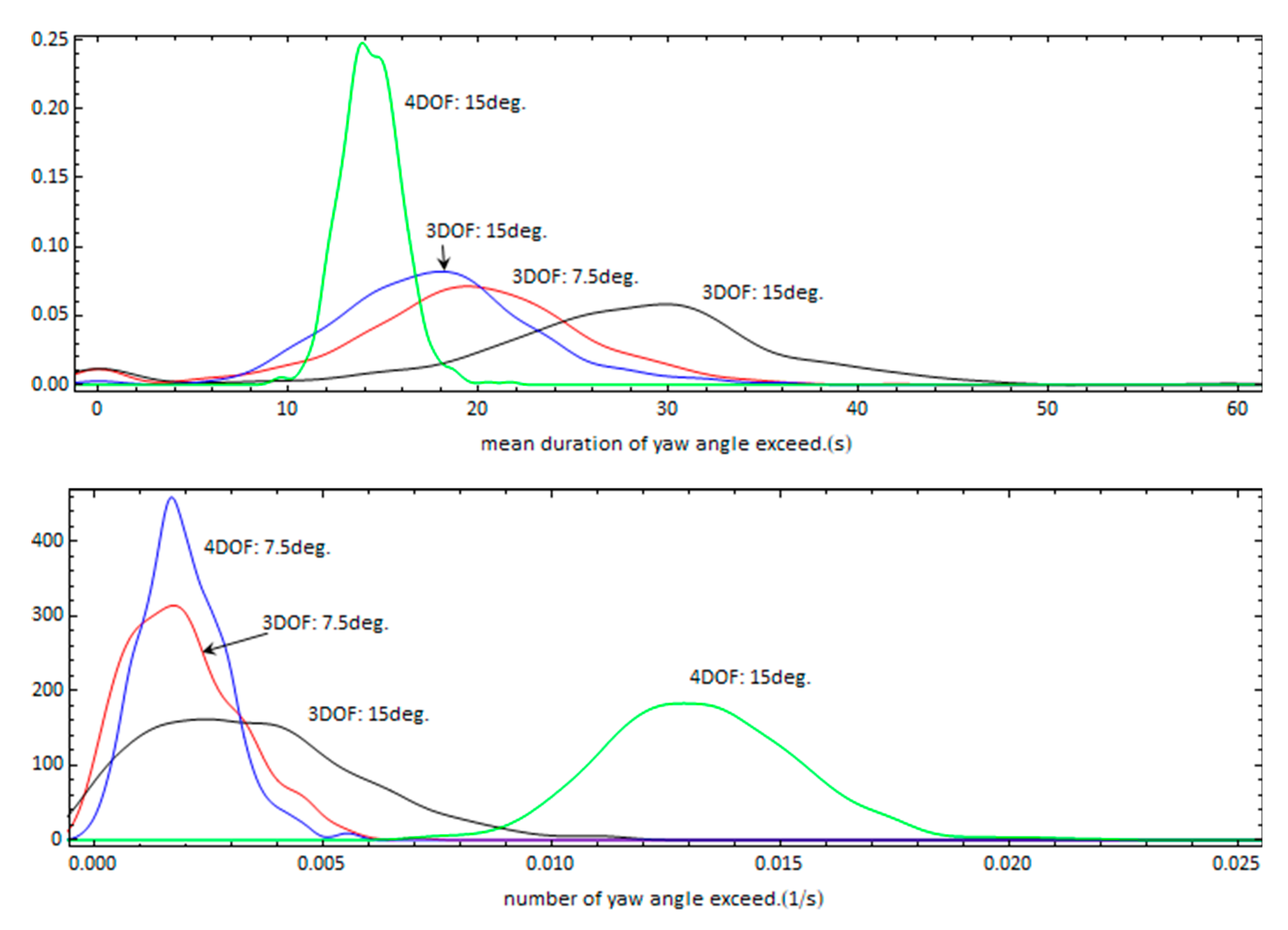
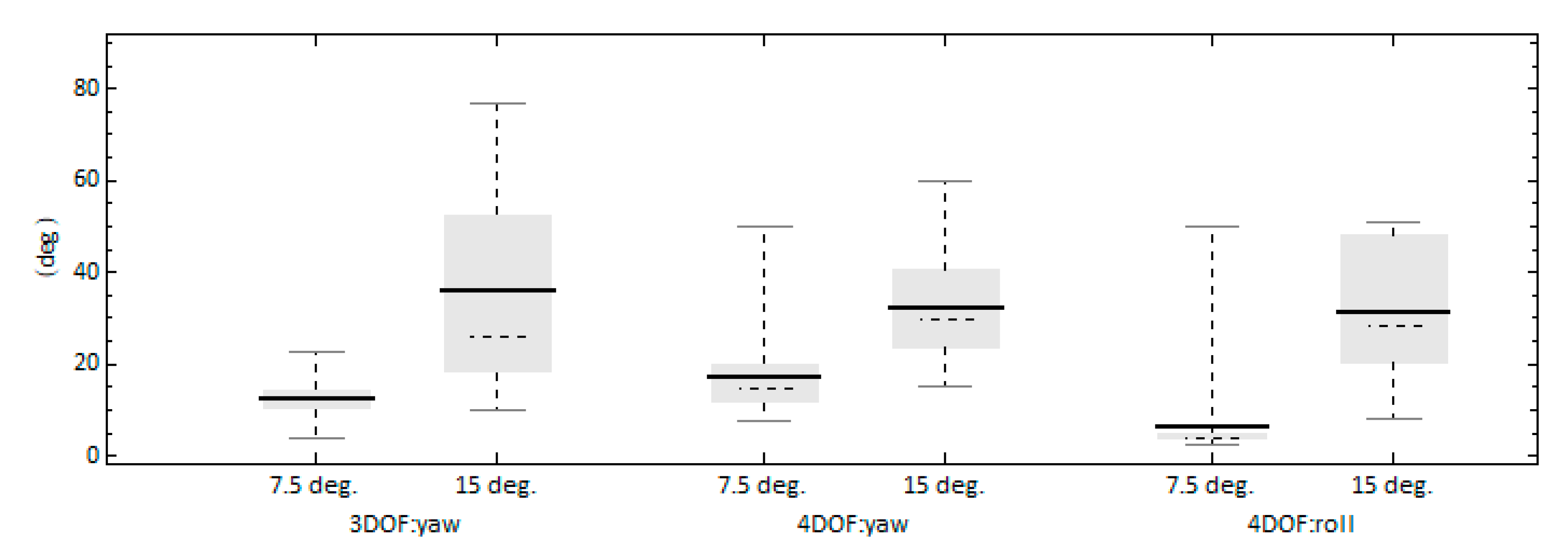
4.2.1. Effect of Commanded Heading
4.2.2. Effect of the Set Thresholds
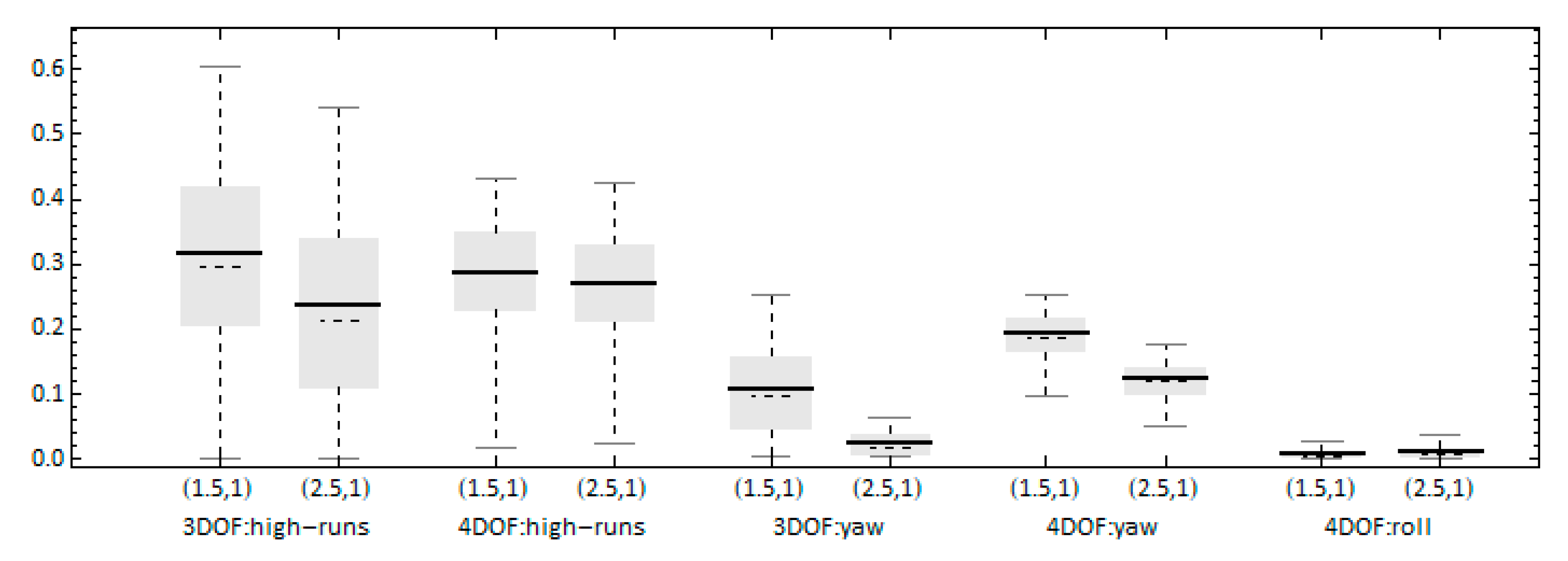
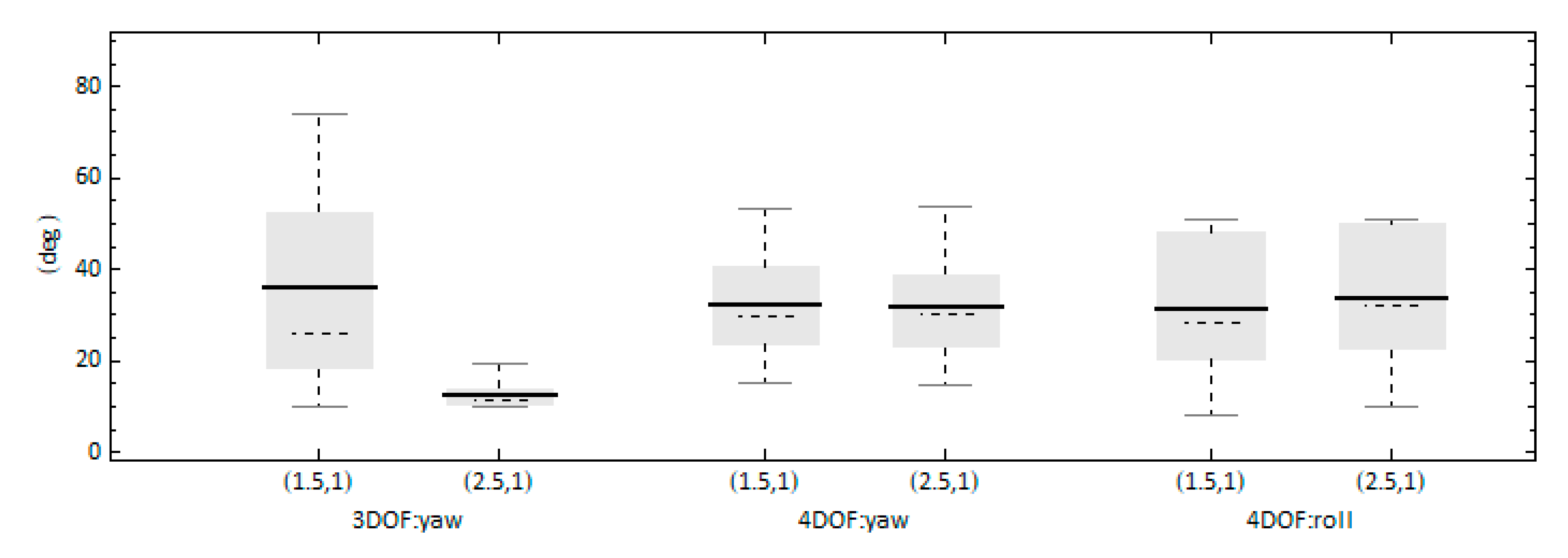
4.2.3. Effect of the PD Control Parameters
4.2.4. Effect of the KG
5. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| amplitude of wave component | ship wetted surface | ||
| Froude-Krylov loads on mode | surge velocity | ||
| hydrostatic loads on mode | sway velocity | ||
| gravity acceleration | longitudinal center of gravity | ||
| GM | metacentric height | surge added mass | |
| roll moment of inertia | acceleration coefficient for sway due to sway acceleration | ||
| yaw moment of inertia | acceleration coefficient for sway due to yaw acceleration | ||
| wave number of wave component | velocity coefficient for sway due to sway velocity | ||
| added roll moment of inertia | velocity coefficient for sway due to yaw velocity | ||
| roll damping | rudder hydrodynamic reaction in sway | ||
| rudder induced roll moment | vertical center of gravity (m) | ||
| ship mass | vertical moment arm of hull hydrodynamic force | ||
| normal vector of panel | vertical moment arm of rudder hydrodynamic force | ||
| propeller revolutions | rudder angle | ||
| acceleration coefficient for yaw due to sway acceleration | random phase shift of wave component j | ||
| acceleration coefficient for yaw due to yaw acceleration | local water elevation | ||
| velocity coefficient for yaw due to sway velocity | ordinate of ship’s position in earth fixed frame | ||
| velocity coefficient for yaw due to yaw velocity | wave direction | ||
| rudder induced yaw moment | abscissa of ship’s position in earth fixed frame | ||
| roll angular velocity | water density | ||
| yaw angular velocity | roll angle | ||
| vector pointing from origin of body fixed system to centroid of panel | yaw angle | ||
| ship resistance | commanded heading | ||
| propeller thrust | wave frequency corresponding to wave component | ||
| time |
References
- Belenky, V.L.; Spyrou, K.J.; van Walree, F.; Neves, M.A.S.; Umeda, N. (Eds.) Contemporary Ideas on Ship Stability: Risk of Capsizing; Springer: Dordrecht, The Netherlands, 2019; ISBN 978-3-030-00514-6. [Google Scholar]
- Manderbacka, T.; Themelis, N.; Bačkalov, I.; Boulougouris, E.; Eliopoulou, E.; Hashimoto, H.; Konovessis, D.; Leguen, J.-F.; González, M.M.; Rodríguez, C.A.; et al. An overview of the current research on stability of ships and ocean vehicles: The STAB2018 perspective. Ocean Eng. 2019, 186, 1–16. [Google Scholar] [CrossRef]
- de Jong, P.; Renilson, M.R.; van Walree, F. The effect of ship speed, heading angle and wave steepness on the likelihood of broaching-to in astern quartering seas. In Proceedings of the 12th International Conference on the Stability of Ships and Ocean Vehicles (STAB2015), Glasgow, UK, 14–19 June 2015; pp. 1103–1114. [Google Scholar]
- Belenky, V.; Spyrou, K.J.; Weems, K. On the probabilistic properties of surf-riding and broaching-to in irregular waves. In Proceedings of the 31st Symposium on Naval Hydrodynamics, Monterey, CA, USA, 11–16 September 2016. [Google Scholar]
- Umeda, N.; Usada, S.; Mizumoto, K.; Matsuda, A. Broaching probability for a ship in irregular stern-quartering waves: Theoretical prediction and experimental validation. J. Mar. Sci. Technol. 2016, 21, 23–37. [Google Scholar] [CrossRef]
- IMO. Finalization of Second Generation Intact Stability Criteria, Report of the Correspondence Group (Part 1), SDC7/5; International Maritime Organization (IMO): London, UK, 2019. [Google Scholar]
- Feng, P.; Fan, S.; Liu, X. Study on the second generation intact stability criteria of broaching failure mode. In Proceedings of the 12th International Conference on the Stability of Ships and Ocean Vehicles (STAB2015), Glasgow, UK, 14–19 June 2015; pp. 195–202. [Google Scholar]
- Bonci, M.; Renilson, M.; De Jong, P.; Van Walree, F.; Van’t Veer, R. On the direct assessment of broaching-to vulnerability of a high speed craft. In Proceedings of the 13th International Conference on the Stability of Ships and Ocean Vehicles (STAB2018), Kobe, Japan, 16–21 September 2018; pp. 119–129. [Google Scholar]
- Terada, D.; Amano, R.; Katayama, T. A motion estimation method of high speed craft in irregular sea by using onboard monitoring motion time series data for motion control. In Proceedings of the 16th International Ship Stability Workshop (ISSW2017), Belgrade, Serbia, 5–7 June 2017; pp. 233–238. [Google Scholar]
- Hashimoto, H.; Taniguchi, Y.; Fujii, M. A case study on operational limitations by means of navigation simulation. In Proceedings of the 16th International Ship Stability Workshop (ISSW2017), Belgrade, Serbia, 5–7 June 2017; pp. 41–48. [Google Scholar]
- Motora, S.; Fujino, M.; Koyonagi, M.; Ishida, S.; Shimada, K.; Maki, T. A consideration on the mechanism of occurrence of broaching-to phenomena. J. Soc. Nav. Archit. Jpn. 1981, 150, 211–222. [Google Scholar] [CrossRef]
- Spyrou, K.J. Dynamic instability in quartering seas: The behavior of a ship during broaching. J. Ship Res. 1996, 40, 46–59. [Google Scholar]
- Themelis, N.; Spyrou, K.J.; Belenky, V. High runs of a ship in multi-chromatic seas. Ocean Eng. 2016, 120, 230–237. [Google Scholar] [CrossRef]
- Kontolefas, I.; Spyrou, K.J. Predicting the probability of ship high-runs from phase space data. J. Ship Res. 2020, 64, 81–97. [Google Scholar] [CrossRef]
- Spyrou, K.J.; Themelis, N.; Kontolefas, I. Numerical statistical estimates of ship broaching-to. J. Ship Res. 2016, 60, 219–238. [Google Scholar] [CrossRef]
- Spyrou, K.J.; Belenky, V.; Themelis, N.; Weems, K. Detection of surf-riding behaviour of ships in irregular seas. Nonlinear Dyn. 2014, 78, 649–667. [Google Scholar] [CrossRef]
- Kan, M. Surging of large amplitude and surf-riding of ships in following seas. In Selected papers in Naval Architecture and Ocean Engineering; The Society of Naval Architects of Japan: Tokyo, Japan, 1990; pp. 152–162. [Google Scholar]
- Feldman, M. Hilbert Transform Applications in Mechanical Vibration; John Wiley & Sons, Ltd.: West Sussex, UK, 2011; ISBN 978-0-470-97827-6. [Google Scholar]
- Spyrou, K.J.; Belenky, V.; Reed, A.; Weems, K.; Themelis, N.; Kontolefas, I. Split-Time method for pure loss of stability and broaching-to. In Proceedings of the 30th Symposium on Naval Hydrodynamics, Tasmania, Australia, 2–7 November 2014. [Google Scholar]
- Lewandowski, E.M. The Dynamics of Marine Craft: Maneuvering and Seakeeping; World Scientific: Singapore, 2004; ISBN 978-9-810-24756-0. [Google Scholar]
- Lewis, E.V. (Ed.) Principles of Naval Architecture, Vol 3: Motion in Waves and Controllability; The Society of Naval Architects and Marine Engineers: Jersey City, NJ, USA, 1989; ISBN 0-939773-02-3. [Google Scholar]
- Mikelis, N.E. A procedure for the prediction of ship manoeuvring response for initial design. In Proceedings of the 5th Conference on Computer Applications in the Automation of Shipyard Operation and Ship Design, Trieste, Italy, 16–20 September 1985. [Google Scholar]
- Belenky, V.L.; Sevastianov, N.B. Stability and Safety of Ships: Risk of Capsizing; The Society of Naval Architects and Marine Engineers: Jersey City, NJ, USA, 2007; ISBN 0-939773-61-9. [Google Scholar]
- Newman, J. Marine Hydrodynamics; MIT Press: Cambridge, UK, 1977; ISBN 9-780-26228-061-7. [Google Scholar] [CrossRef]
- Bishop, B.; Belknap, W.; Turner, C.; Simon, B.; Kim, J. Parametric Investigation on the Influence of GM, Roll Damping and above Water Form, on the Roll Response of Model 5613. Report NSWCCD-50-TR-2005/027; Naval Surface Warfare Center/Carderock Division: West Bethesda, MD, USA, 2005. [Google Scholar]





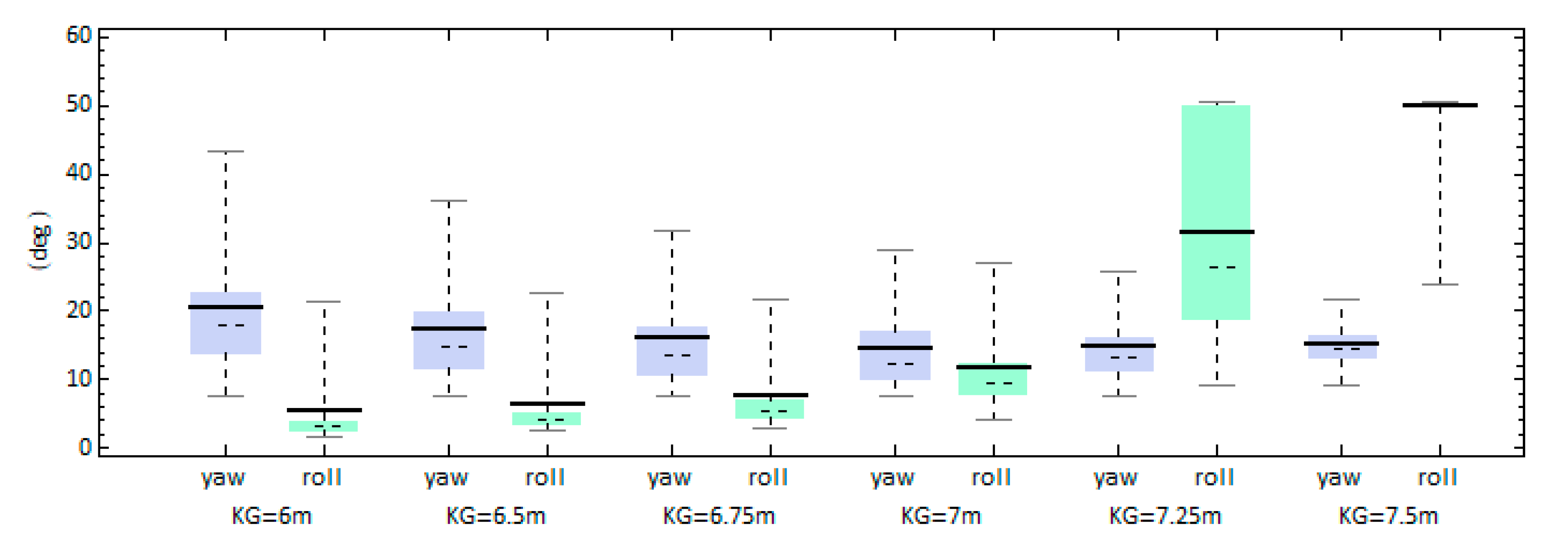
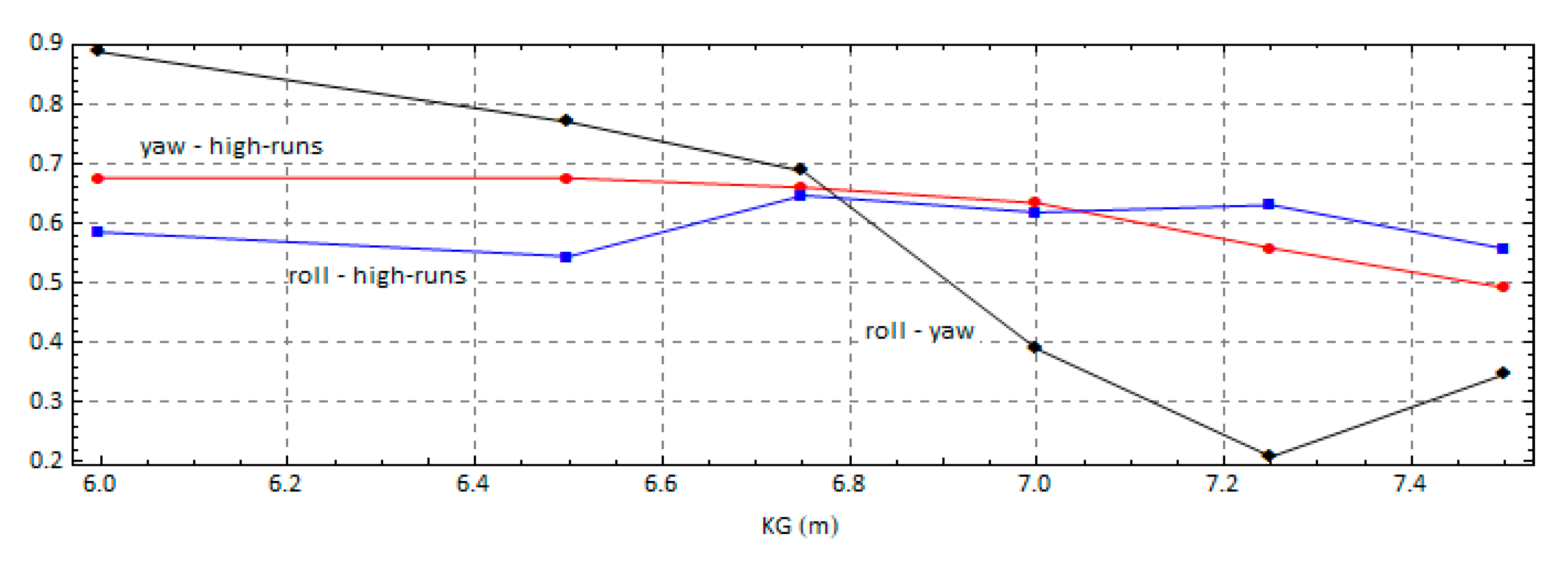
| Parameter | Value |
|---|---|
| Nominal ship speed | 12 |
| Initial ship speed | 10 |
| Number of wave realizations | 500 |
| Significant wave height (m) | 6 |
| Wave peak period (s) | 9.5 |
| Frequency range dω (rad/s) | |
| Total simulation time (s) | 2400 |
| Commanded heading (deg) | 7.5 |
| Proportional and differential gain , | (1.5, 1) |
| Exceedance thresholds (deg) | Yaw angle: 7.5 Roll angle: 15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Themelis, N.; Angelou, M.; Spyrou, K.J. Statistical Correlations of Ship High-Run with Broaching-to and Capsize. J. Mar. Sci. Eng. 2020, 8, 846. https://doi.org/10.3390/jmse8110846
Themelis N, Angelou M, Spyrou KJ. Statistical Correlations of Ship High-Run with Broaching-to and Capsize. Journal of Marine Science and Engineering. 2020; 8(11):846. https://doi.org/10.3390/jmse8110846
Chicago/Turabian StyleThemelis, Nikos, Manolis Angelou, and Kostas J. Spyrou. 2020. "Statistical Correlations of Ship High-Run with Broaching-to and Capsize" Journal of Marine Science and Engineering 8, no. 11: 846. https://doi.org/10.3390/jmse8110846
APA StyleThemelis, N., Angelou, M., & Spyrou, K. J. (2020). Statistical Correlations of Ship High-Run with Broaching-to and Capsize. Journal of Marine Science and Engineering, 8(11), 846. https://doi.org/10.3390/jmse8110846







