Numerical Investigation of Wave Generation Characteristics of Bottom-Tilting Flume Wavemaker
Abstract
:1. Introduction
2. Model Development
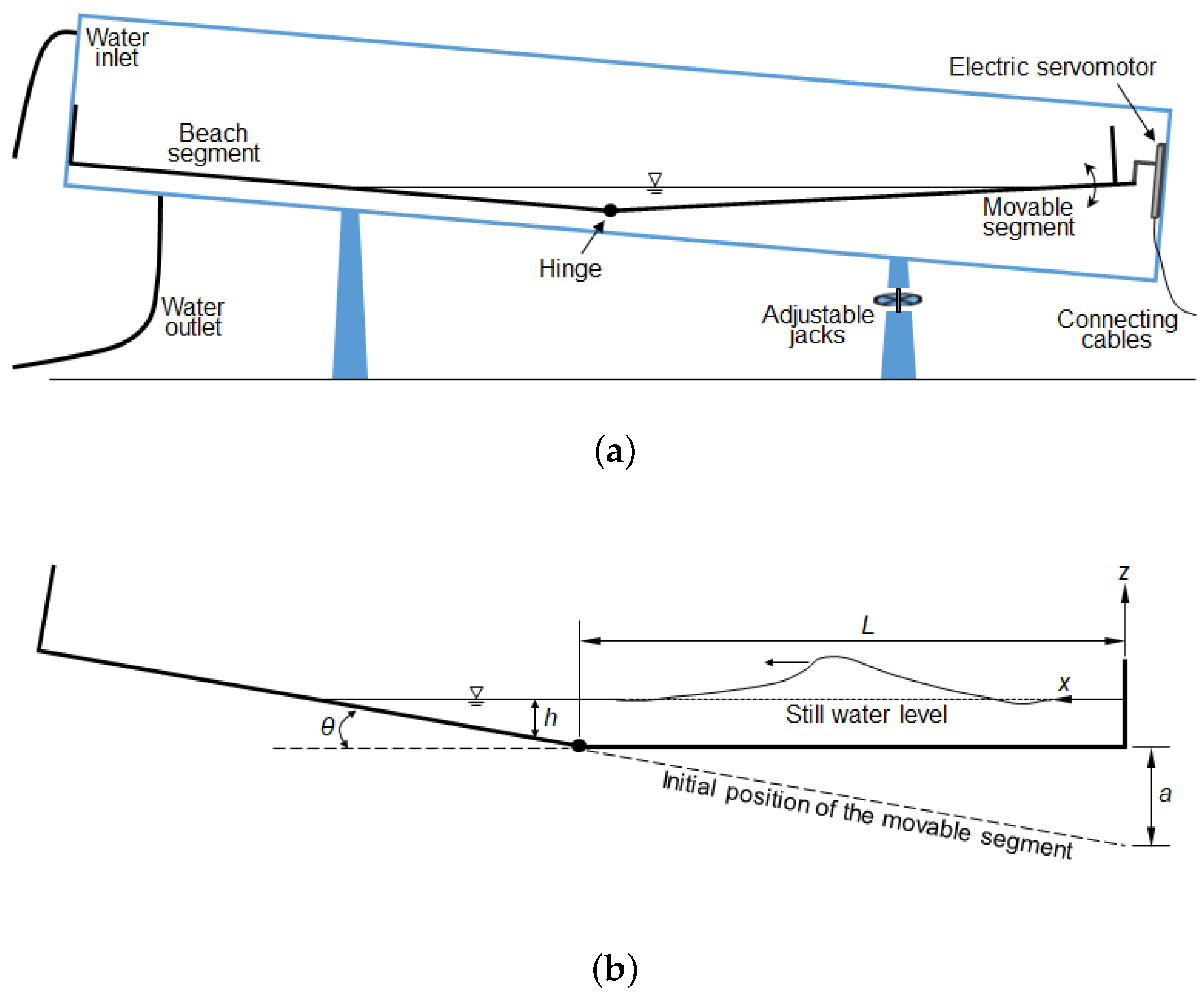
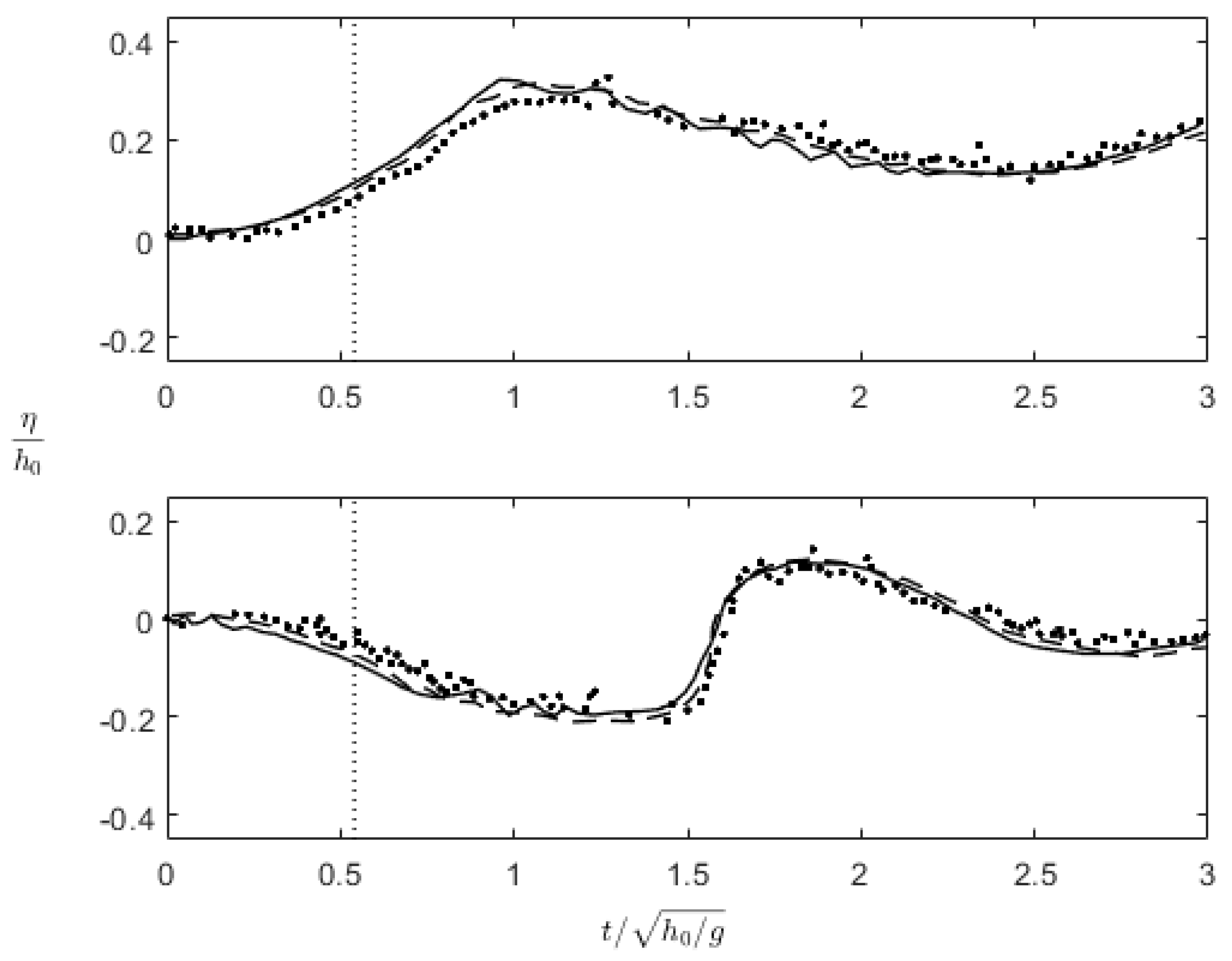
2.1. Physical Model by Lu and Colleagues
2.2. Numerical Model for Bottom-Tilting Wavemaker
3. Results
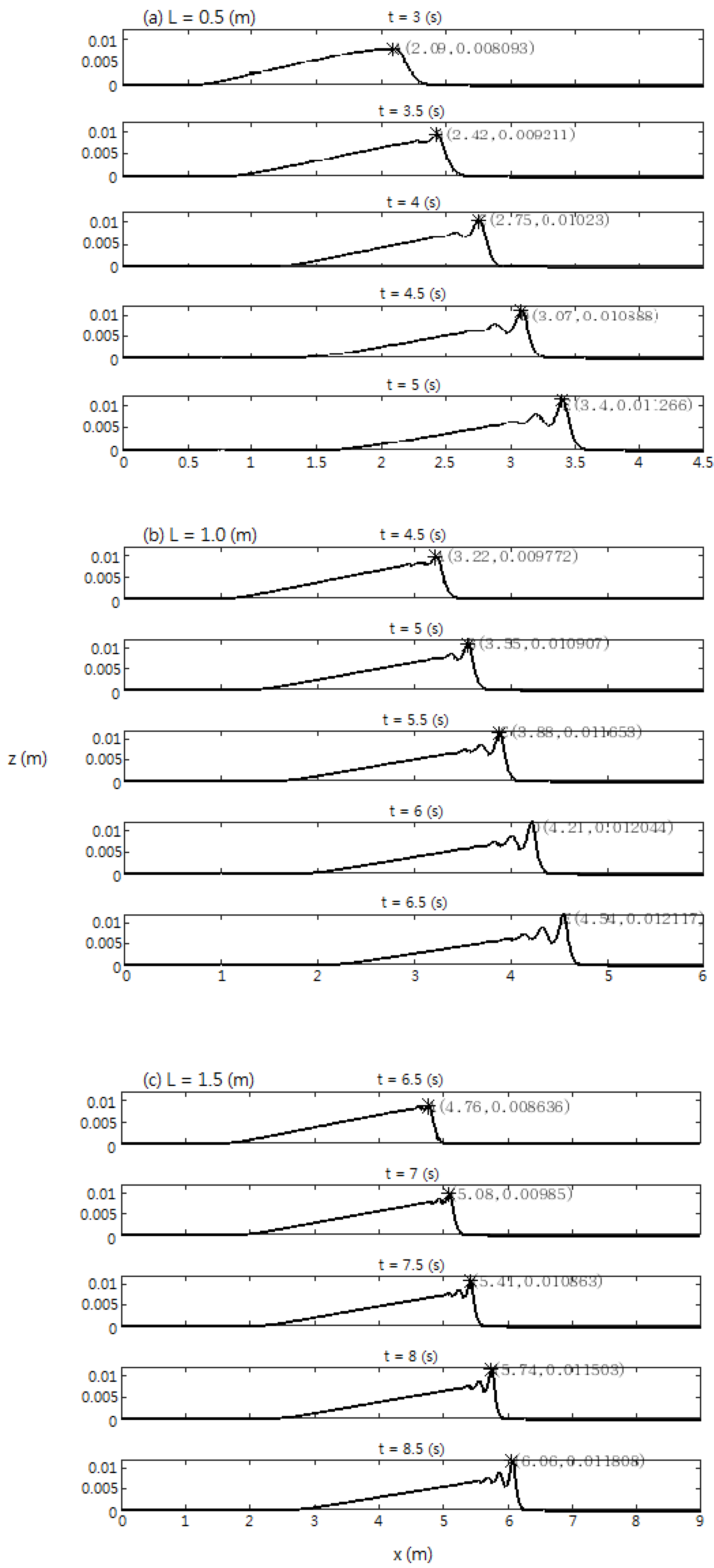
3.1. Effects of Moving Bottom Length, L
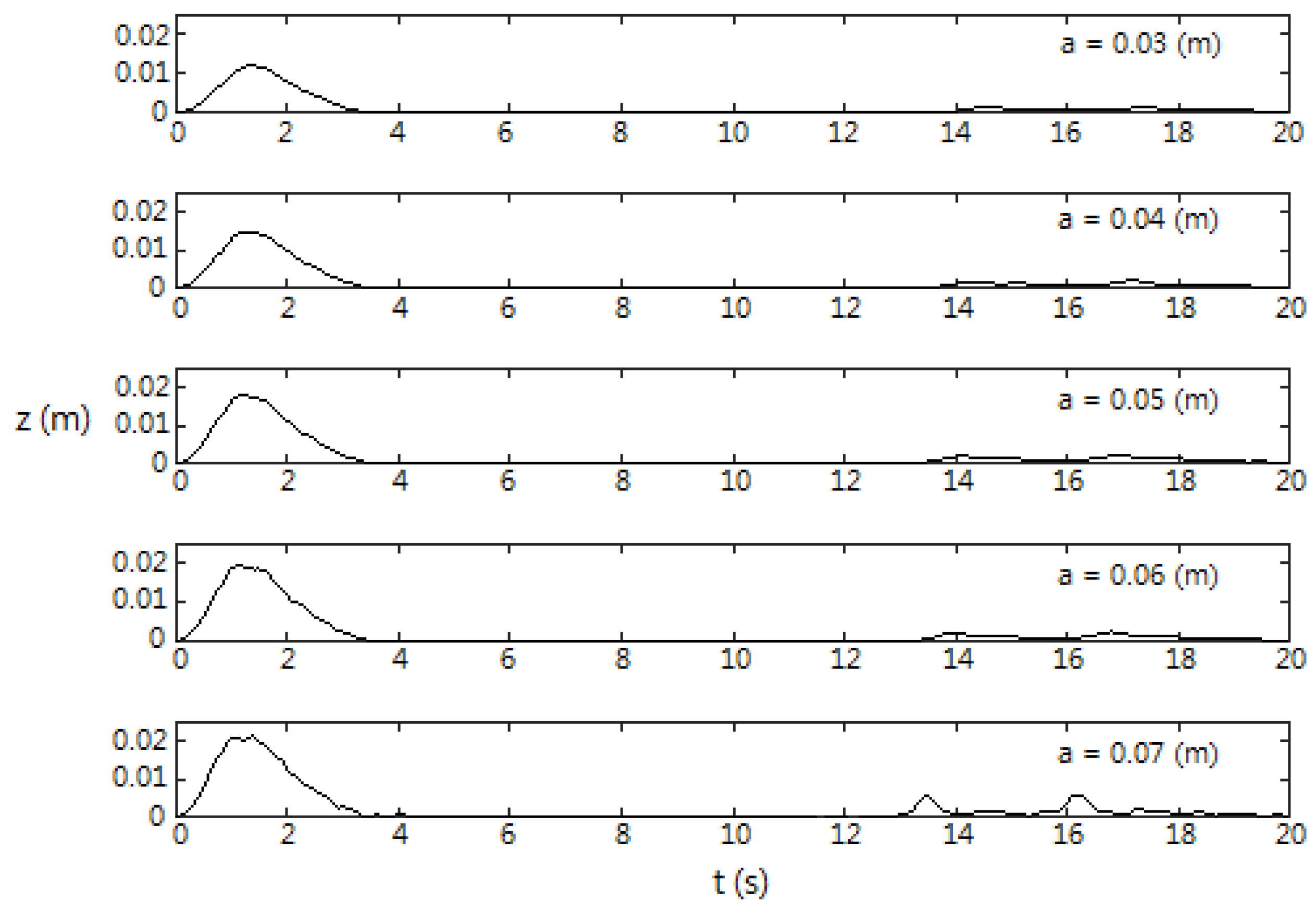
3.2. Effects of Magnitude of Vertical Displacement, a
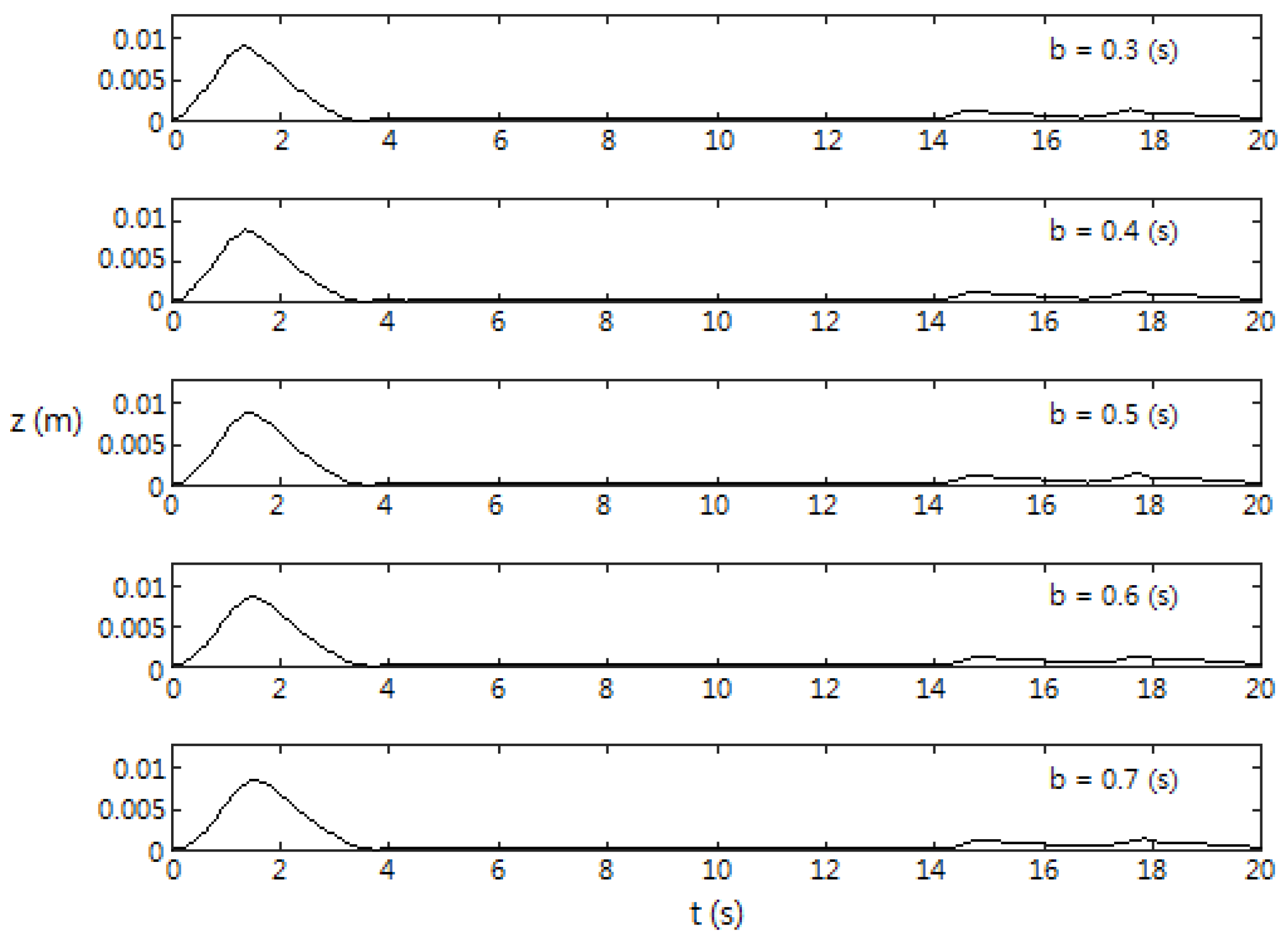
3.3. Effects of Motion Duration, b
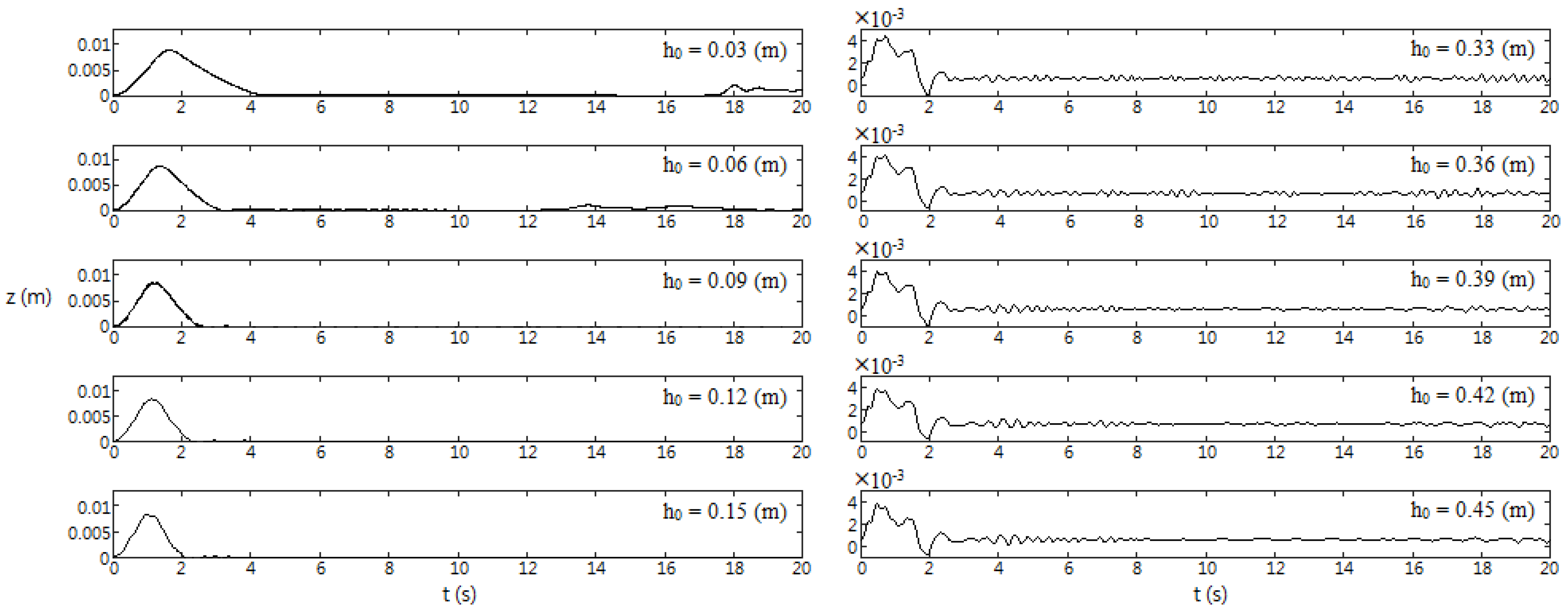
3.4. Effects of Water Depth,
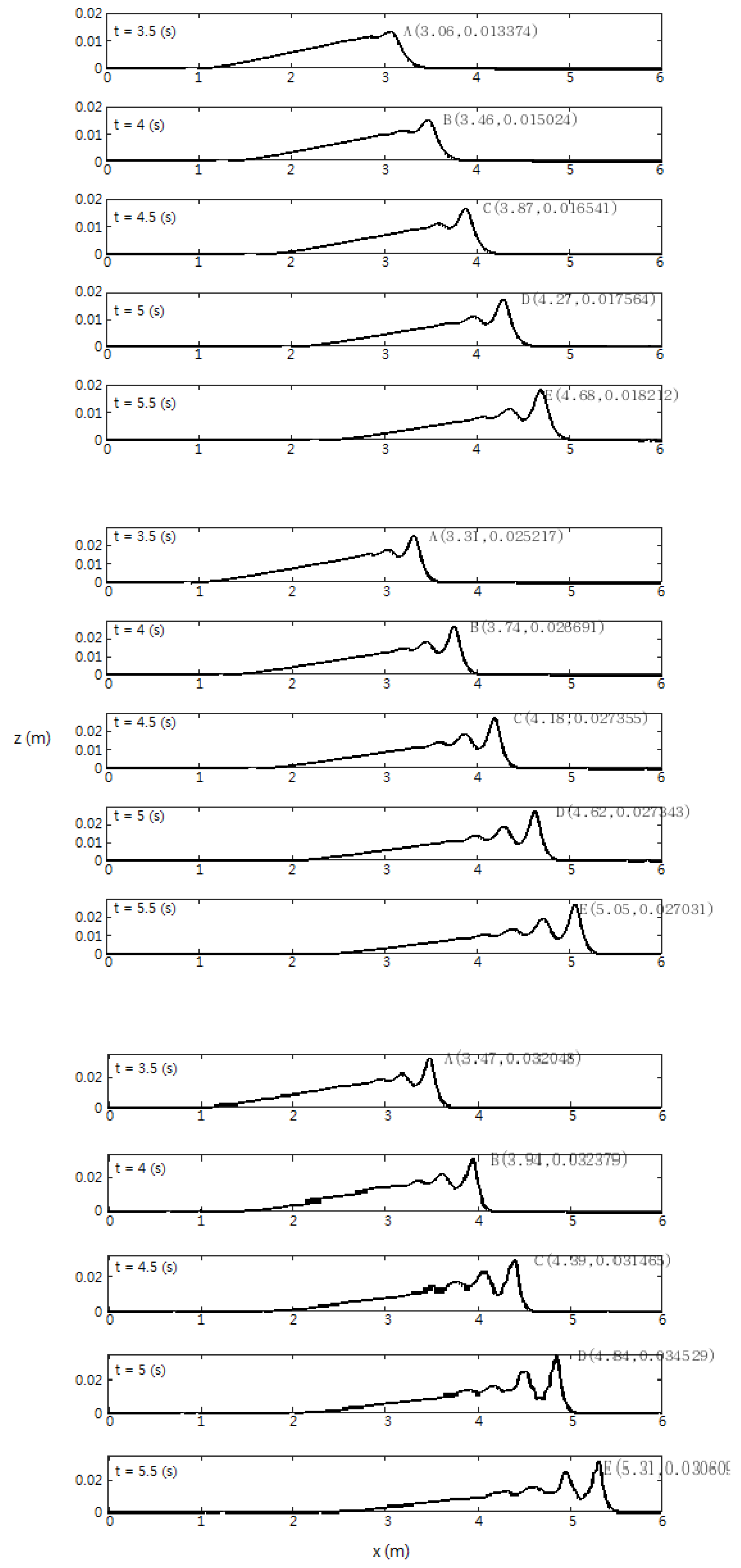
3.5. Wavelength and Wave Amplitude
4. Concluding Remarks
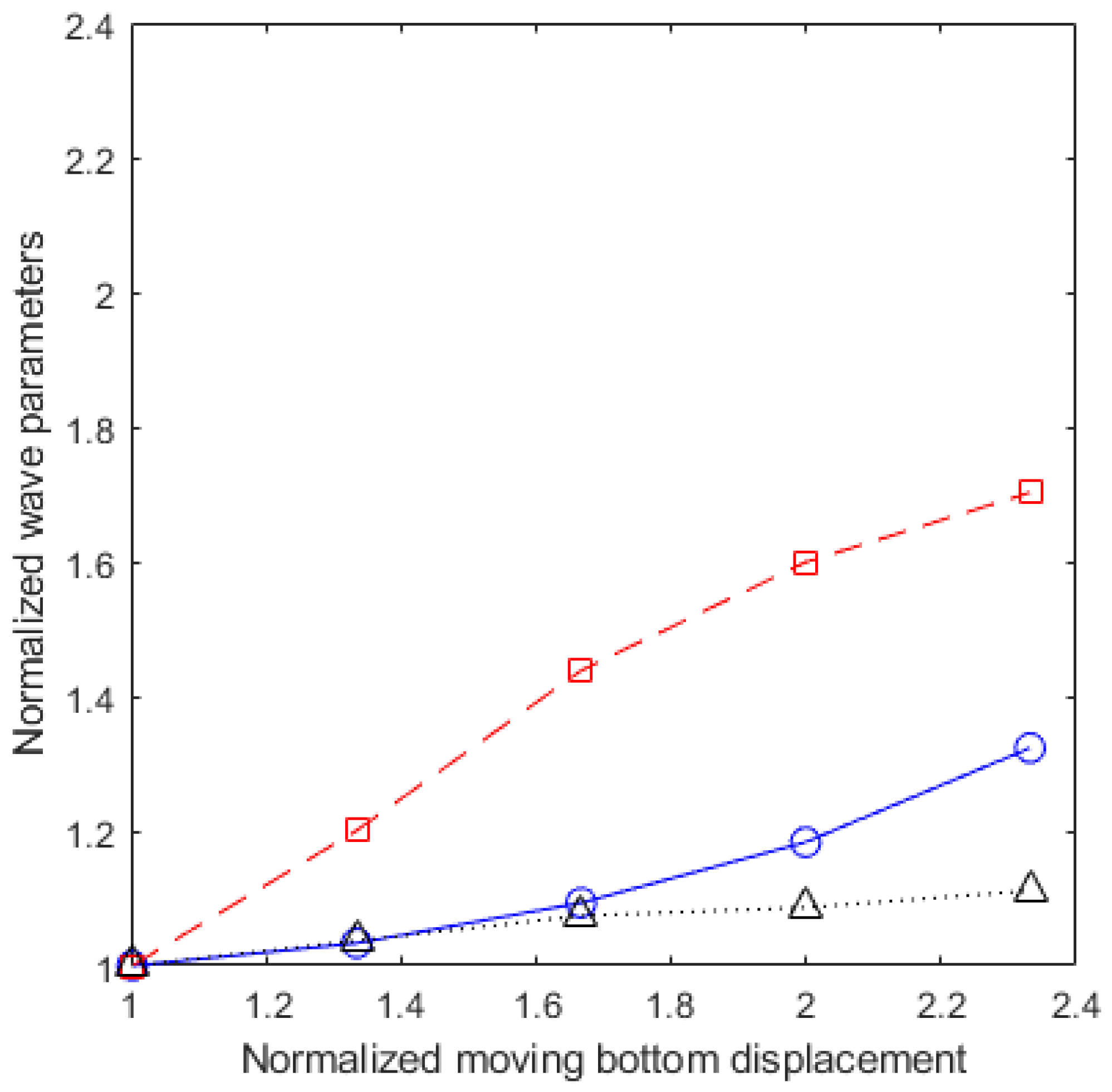
- Effects of wavemaker length, L (Section 3.1): grows rapidly as L increases while both and are not too sensitive to the change of L.
- Effects of bottom vertical displacement, a (Section 3.2): , , and all increase with the increasing of a. However, a has the most dominate effect on .
- Effects of motion duration, b (Section 3.3): Within the range of b values that we have discussed, b has little control over , , and .
- Effects of water depth, (Section 3.4): For shallower depth, decreases as increases. However, does not affect for relative deeper water. In both depth regimes, is unaffected by the change of .
- Wavelength, , and wave amplitude, (Section 3.5): depends linearly on L, . is a function of and the fitted Equation (3) is applicable for a wide range of value.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Okal, E.A. The quest for wisdom: Lessons from 17 tsunamis, 2004–2014. Philos. Trans. R. Soc. A 2015, 373, 20140370. [Google Scholar] [CrossRef] [PubMed]
- Imamura, F.; Boreta, S.P.; Suppasri, A.; Muhari, A. Recent occurrences of serious tsunami damage and the future challenges of tsunami disaster risk reduction. Prog. Disast. Sci. 2019, 1, 100009. [Google Scholar] [CrossRef]
- Widiyanto, W.; Santoso, P.B.; Hsiao, S.-C.; Imananta, R.T. Post-event field survey of 28 September 2018 Sulawesi earthquake and tsunami. Nat. Hazards Earth Syst. Sci. 2015, 19, 2781–2794. [Google Scholar] [CrossRef] [Green Version]
- Wallemacq, P.; Below, R.; McLean, D. UNISDR and CRED Report: Economic Losses, Poverty & Disasters (1998–2017); United Nations Office for Disaster Risk Reduction: Geneva, Switzerland, 2018. [Google Scholar]
- Synolakis, C.E.; Bernard, E.N. Tsunami science before and beyond Boxing Day, 2004. Philos. Trans. R. Soc. A 2006, 364, 2231–2265. [Google Scholar] [CrossRef] [PubMed]
- Satake, K. Advances in earthquake and tsunami sciences and disaster risk reduction since the 2004 Indian ocean tsunami. Geosci. Lett. 2014, 1, 15. [Google Scholar] [CrossRef] [Green Version]
- Rabinovich, A.B.; Geist, E.L.; Fritz, H.M.; Borrero, J.C. Introduction to “Tsunami Science: Ten Years after the 2004 Indian Ocean Tsunami. Volume I”. Pure Appl. Geophys. 2015, 172, 615–619. [Google Scholar] [CrossRef] [Green Version]
- Heidarzadeh, M.; Muhari, A.; Wijanarto, A.B. Insights on the source of the 28 September 2018 Sulawesi tsunami, Indonesia based on spectral analyses and numerical simulations. Pure Appl. Geophys. 2019, 176, 25–43. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Liu, P.L.-F. Numerical simulations of the 2004 Indian Ocean tsunamis—Coastal effects. J. Earthq. Tsunami 2007, 1, 273–297. [Google Scholar] [CrossRef]
- Fritz, H.M.; Mohammed, F.; Yoo, J. Lituya Bay landslide impact generated mega-tsunami 50th anniversary. Pure Appl. Geophys. 2009, 166, 153–175. [Google Scholar] [CrossRef]
- Liu, P.L.-F.; Higuera, P.; Husrin, S.; Prasetya, G.S.; Prihantono, J.; Diastomo, H.; Pryambodo, D.G.; Susmoro, H. Coastal landslides in Palu Bay during 2018 Sulawesi earthquake and tsunami. Landslides 2020, 17, 2085–2098. [Google Scholar] [CrossRef]
- Carrier, G.F.; Greenspan, H.P. Water waves of finite amplitude on a sloping beach. J. Fluid Mech. 1958, 4, 97–109. [Google Scholar] [CrossRef]
- Madsen, P.A.; Schäffer, H.A. Analytical solutions for tsunami runup on a plane beach: Single waves, N-Waves Transient Waves. J. Fluid Mech. 2010, 645, 25–57. [Google Scholar] [CrossRef]
- Liu, P.L.-F.; Cho, Y.-S.; Yoon, S.B.; Seo, S.N. Numerical simulations of the 1960 Chilean tsunami propagation and inundation at Hilo, Hawaii. In Recent Developments in Tsunami Research; El-Sabh, M.I., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1994; pp. 99–115. [Google Scholar]
- Titov, V.; Kânoğlu, U.; Synolakis, C. Development of MOST for real-time tsunami forecasting. J. Waterw. Port. Coast. 2016, 142, 03116004. [Google Scholar] [CrossRef]
- Synolakis, C.E. The runup of solitary waves. J. Fluid Mech. 1987, 185, 523–545. [Google Scholar] [CrossRef]
- Liu, P.L.-F.; Wu, T.-R.; Raichlen, F.; Synolakis, C.E.; Borrero, J.C. Runup and rundown generated by three-dimensional sliding masses. J. Fluid Mech. 2005, 536, 107–144. [Google Scholar] [CrossRef] [Green Version]
- Madsen, O.S. On the generation of long waves. J. Geophys. Res. 1971, 76, 8672–8683. [Google Scholar] [CrossRef]
- Synolakis, C.E. Generation of long waves in laboratory. J. Waterw. Port. Coast. 1990, 116, 252–266. [Google Scholar] [CrossRef]
- Kaplan, K. Generalized Laboratory Study of Tsunami Run-Up; Tech. Memo 60; Beach Erosion Board, U.S. Army Corps of Engineers: Vicksburg, MS, USA, 1995. [Google Scholar]
- Li, Y. Tsunamis: Non-Breaking and Breaking Solitary Wave Run-Up. Ph.D. Thesis, California Institute of Technology, Pasadena, CA, USA, 2000. [Google Scholar]
- Synolakis, C.E.; Deb, M.K.; Skjelbreia, J.E. The anomalous behavior of the run-up of cnoidal waves. Phys. Fluids 1998, 31, 3–5. [Google Scholar] [CrossRef]
- Lima, V.V.; Avilez-Valente, P.; Baptista, M.A.V.; Miranda, J.M. Generation of N-waves in laboratory. Coast. Eng. 2019, 148, 1–18. [Google Scholar] [CrossRef]
- Lu, H. Generation of Very Long Waves in Laboratory for Tsunamis Research. Ph.D. Thesis, University of Dundee, Dundee, UK, 2017. [Google Scholar]
- Madsen, P.A.; Fuhrman, D.R.; Schäffer, H.A. On the solitary wave paradigm for tsunamis. J. Geophys. Res. 2008, 113, C12012. [Google Scholar] [CrossRef]
- Van Gent, M.R.A. The new delta flume for large-scale testing. In Proceedings of the 36th IAHR World Congress, The Hague, The Netherlands, 28 June–3 July 2015. [Google Scholar]
- Zhang, H.; Geng, B. Introduction of the world largest wave flume constructed by TIWTE. In Proceedings of the APAC 2015, Chennai, India, 7–10 September 2015. [Google Scholar]
- Schimmels, S.; Sriram, V.; Didenkulova, I. Tsunami generation in a large scale experimental facility. Coast. Eng. 2016, 110, 32–41. [Google Scholar] [CrossRef]
- Allsop, W.; Robinson, D.; Charvet, I.; Rossetto, T.; Abernethy, R. A unique tsunami generator for physical modelling of violent flows and their impact. In Proceedings of the 14th World Conference of Earthquake Engineering, Beijing, China, 12–17 October 2008. [Google Scholar]
- Rossetto, T.; Allsop, W.; Charvet, I.; Robinson, D. Physical modelling of tsunami using a new pneumatic wave generator. Coast. Eng. 2011, 58, 517–527. [Google Scholar] [CrossRef]
- Charvet, I. Experimental Modelling of Long Elevated and Depressed Waves Using a New Pneumatic Wave Generator. Ph.D. Thesis, University College London, London, UK, 2011. [Google Scholar]
- Charvet, I.; Eames, I.; Rossetto, T. New tsunami runup relationships based on long wave experiments. Ocean Model. 2013, 69, 79–92. [Google Scholar] [CrossRef] [Green Version]
- Goseberg, N.; Wurpts, A.; Schlurmann, T. Laboratory-scale generation of tsunami and long waves. Coast. Eng. 2013, 79, 57–74. [Google Scholar] [CrossRef]
- Bremm, G.C.; Goseberg, N.; Schlurmann, T.; Nistor, I. Long wave flow interaction with a single square Structure on a sloping beach. J. Mar. Sci. Eng. 2015, 3, 821–844. [Google Scholar] [CrossRef] [Green Version]
- Drähne, U.; Goseberg, N.; Vater, S.; Beisiegel, N.; Behrens, J. An experimental and numerical study of long wave run-up on a plane beach. J. Mar. Sci. Eng. 2016, 4, 821. [Google Scholar] [CrossRef] [Green Version]
- Lu, H.; Park, Y.S.; Cho, Y.-S. Investigation of long waves generated by bottom-tilting wave maker. Coast. Eng. J. 2017, 59, 1750018. [Google Scholar] [CrossRef]
- Lu, H.; Park, Y.S.; Cho, Y.-S. Modelling of long waves generated by bottom-tilting wave maker. Coast. Eng. 2017, 122, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Lu, H.; Park, Y.S.; Cho, Y.-S. Run-up of long waves generated by bottom-tilting wave maker. J. Hydraul. Res. 2020, 58, 47–59. [Google Scholar] [CrossRef]
- Goseberg, N. The run-up of long waves—Laboratory-scaled geophysical reproduction and onshore interaction with macro-roughness elements. Ph.D. Thesis, Leibniz Universität Hannover, Hannover, Germany, 2011. [Google Scholar]
- Higuera, P. Application of Computational Fluid Dynamics to Wave Action on Structures. Ph.D. Thesis, University of Cantabria, Santander, Spain, 2015. [Google Scholar]
- Ma, G.; Shi, F.; Kirby, J.T. Shock-capturing non-hydrostatic model for fully dispersive surface wave processes. Ocean Model. 2012, 43, 22–35. [Google Scholar] [CrossRef]
- Derakhti, M.; Kirby, J.T.; Shi, F.; Ma, G. NHWAVE: Consistent boundary conditions and turbulence modelling. Ocean Model. 2016, 106, 121–130. [Google Scholar] [CrossRef]
- Gallerano, F.; Cannata, G.; Barsi, L.; Palleschi, F.; Iele, B. Simulation of wave motion and wave breaking induced energy dissipation. WSEAS Trans. Fluid Mech. 2019, 14, 62–69. [Google Scholar]
- Gallerano, F.; Cannata, G.; Barsi, L.; Palleschi, F. Hydrodynamic effects produced by submerged breakwaters in a coastal area with a curvilinear shoreline. J. Mar. Sci. Eng. 2019, 7, 337. [Google Scholar] [CrossRef] [Green Version]
- Higuera, P.; Losada, I.J.; Lara, J.L. Three-dimensional numerical wave generation with moving boundaries. Coast. Eng. 2015, 101, 35–47. [Google Scholar] [CrossRef]
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Higuera, P.; Liu, P.L.-F.; Lin, C.; Wong, W.-Y. Laboratory-scale swash flows generated by a non-breaking solitary wave on a steep slope. J. Fluid Mech. 2018, 847, 186–227. [Google Scholar] [CrossRef]
- Chan, I.-C.; Liu, P.L.-F. On the runup of long waves on a plane beach. J. Geophys. Res. 2012, 117, C08006. [Google Scholar] [CrossRef]
- Hammack, J.L.; Segur, H. The Korteweg–de Vries equation and water waves. Part 2. Comparison with experiments. J. Fluid Mech. 1974, 65, 289–314. [Google Scholar] [CrossRef] [Green Version]



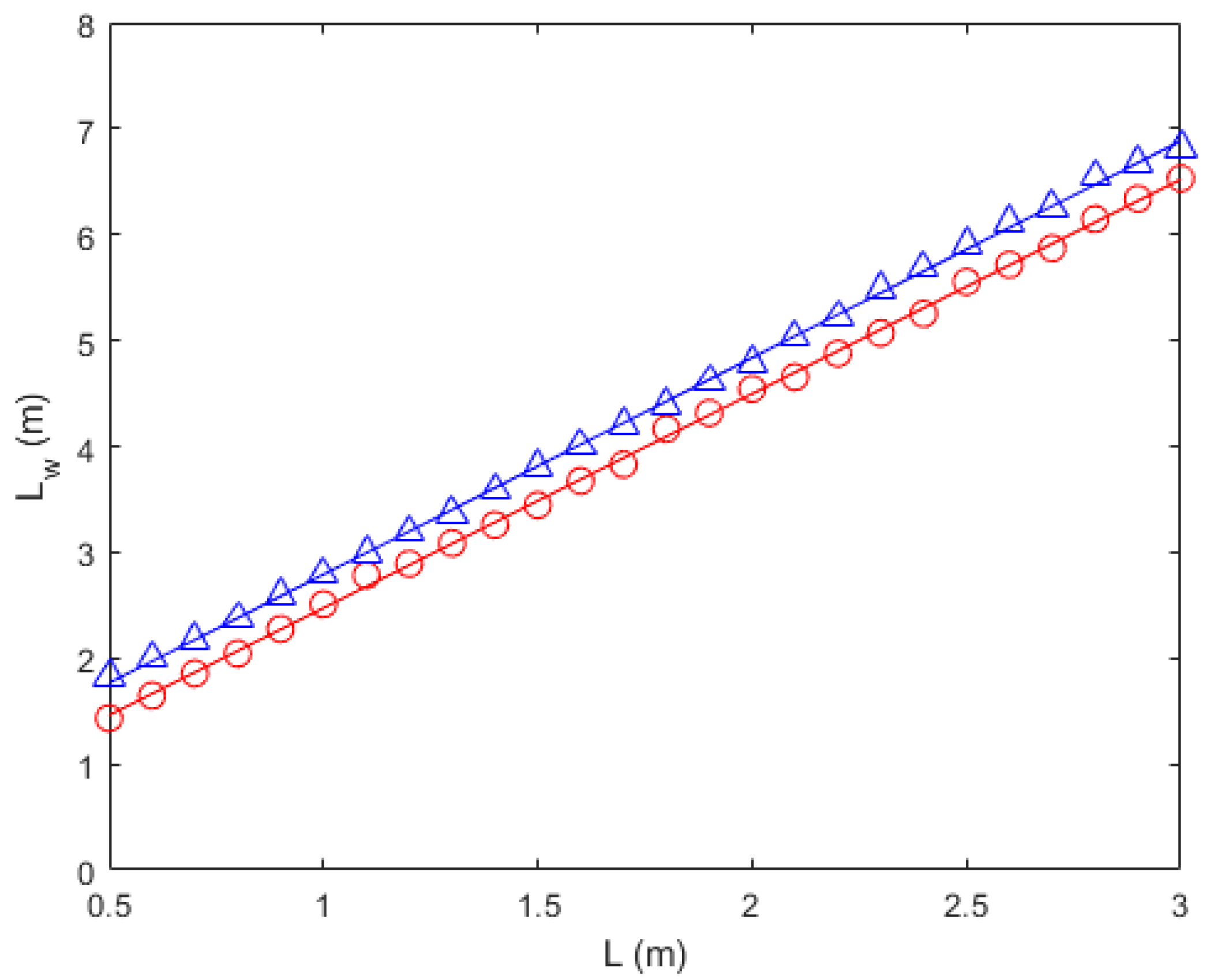
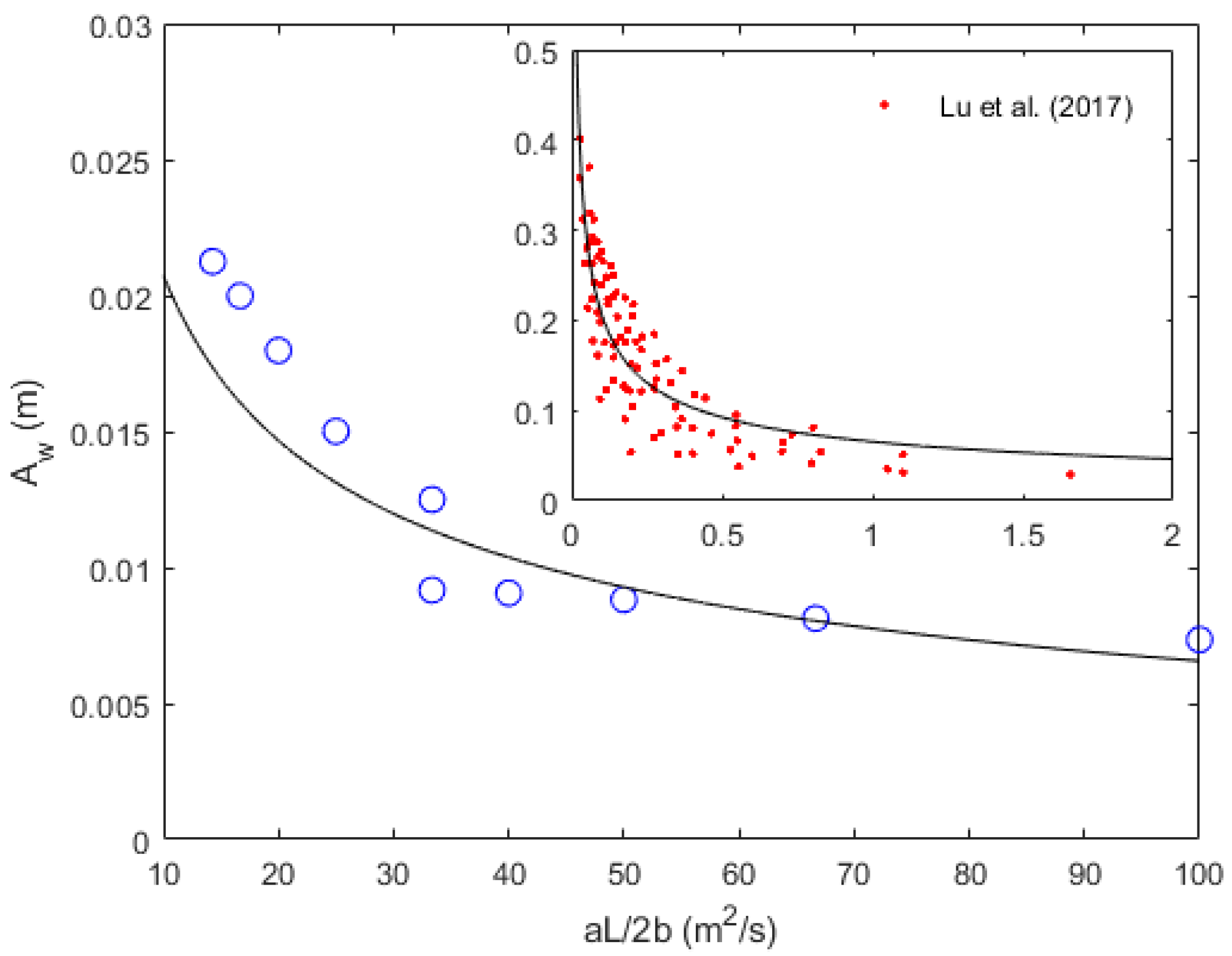
| Wavemaker Length | Wavelength | Wave Amplitude | Phase Speed |
|---|---|---|---|
| L (m) | (m) | (mm) | (m/s) |
| 0.50 | 1.80 | 7.35 | 0.660 |
| 0.75 | 2.44 | 8.13 | 0.655 |
| 1.00 | 4.40 | 8.82 | 0.660 |
| 1.25 | 6.70 | 9.06 | 0.655 |
| 1.50 | 8.68 | 9.17 | 0.650 |
| Wavemaker Displacement | Wavelength | Wave Amplitude | Phase Speed |
|---|---|---|---|
| a (m) | (m) | (mm) | (m/s) |
| 0.03 | 3.00 | 12.50 | 0.81 |
| 0.04 | 3.10 | 15.01 | 0.84 |
| 0.05 | 3.28 | 17.98 | 0.87 |
| 0.06 | 3.55 | 19.99 | 0.88 |
| 0.07 | 3.97 | 21.25 | 0.90 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.-E.; Chan, I.-C. Numerical Investigation of Wave Generation Characteristics of Bottom-Tilting Flume Wavemaker. J. Mar. Sci. Eng. 2020, 8, 769. https://doi.org/10.3390/jmse8100769
Wang H-E, Chan I-C. Numerical Investigation of Wave Generation Characteristics of Bottom-Tilting Flume Wavemaker. Journal of Marine Science and Engineering. 2020; 8(10):769. https://doi.org/10.3390/jmse8100769
Chicago/Turabian StyleWang, Hsin-Erh, and I-Chi Chan. 2020. "Numerical Investigation of Wave Generation Characteristics of Bottom-Tilting Flume Wavemaker" Journal of Marine Science and Engineering 8, no. 10: 769. https://doi.org/10.3390/jmse8100769
APA StyleWang, H.-E., & Chan, I.-C. (2020). Numerical Investigation of Wave Generation Characteristics of Bottom-Tilting Flume Wavemaker. Journal of Marine Science and Engineering, 8(10), 769. https://doi.org/10.3390/jmse8100769





