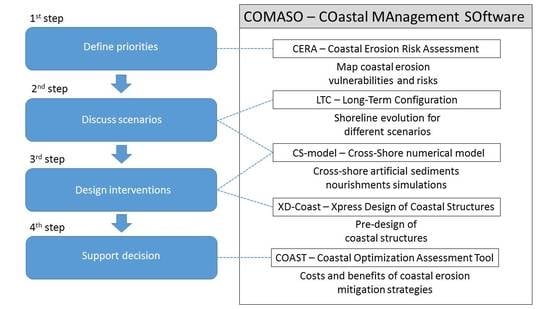
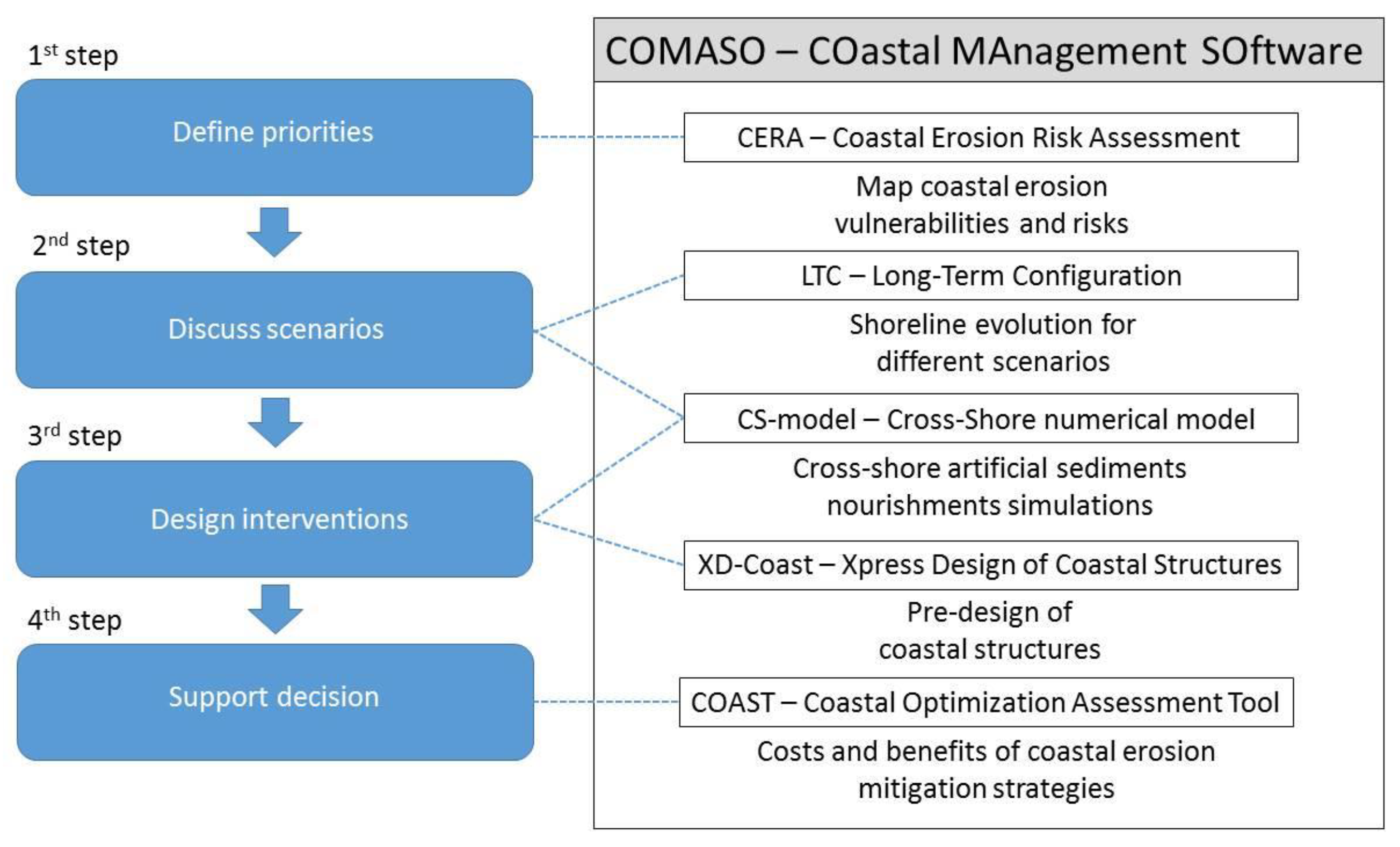
Coastal Management Software to Support the Decision-Makers to Mitigate Coastal Erosion
Abstract
:1. Introduction
2. Mapping Vulnerability and Risk
2.1. Coastal Risk Assessment Methods
2.2. Coastal Erosion Risk Assessment: CERA
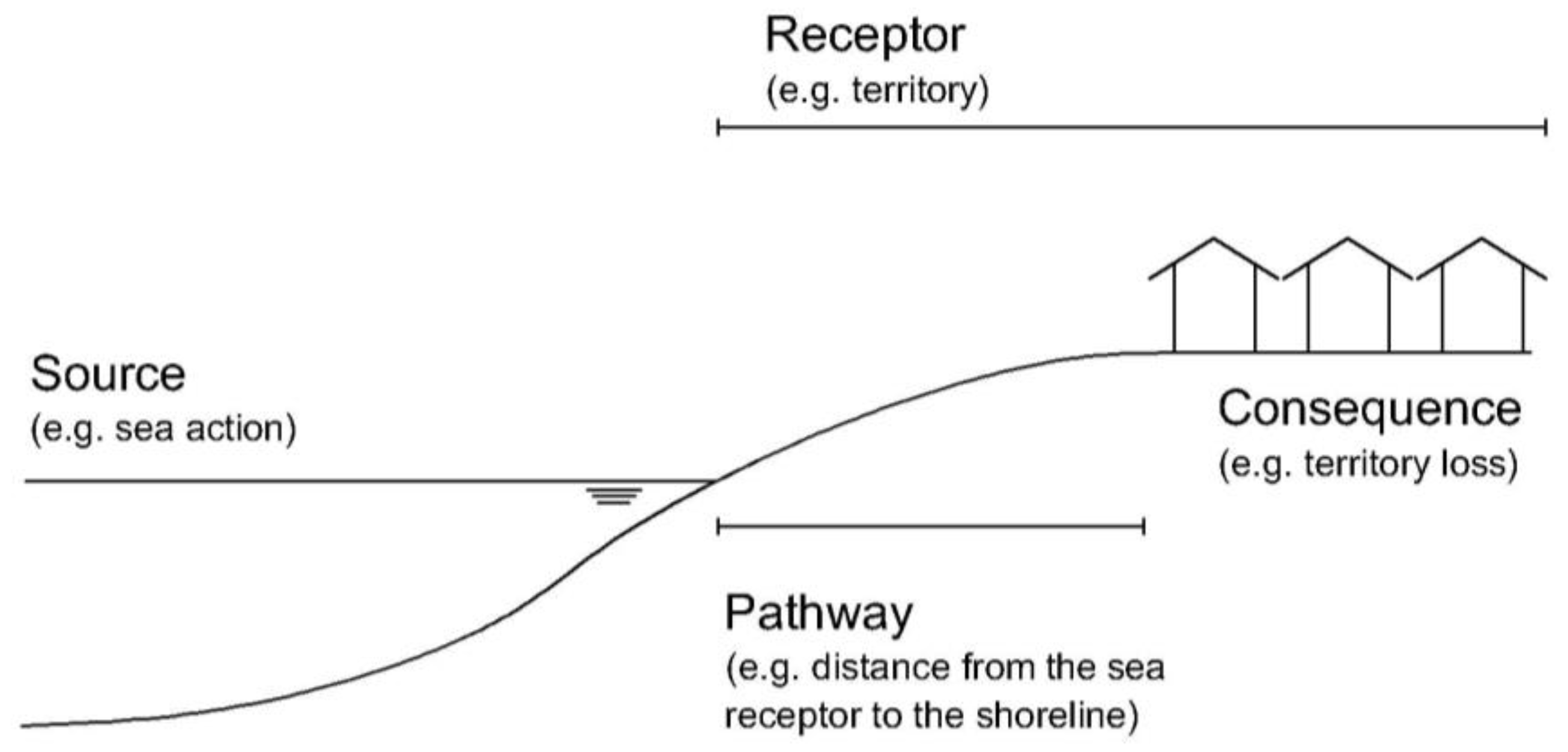
- Hazard (source)—intensity and/or likelihood of coastal erosion and/or driving sources of coastal erosion (waves, sea-level rise, extreme events);
- Exposure (receptors)—quantification of the receptors that are within range of potential land loss. The exposure assessment can be executed into levels of exposure, classifying receptors from highly exposed to low exposition;
- Susceptibility (pathway)—intrinsic characteristics of the land that makes it predisposed to be eroded by action of wave climate. The susceptibility is independent of the wave conditions at the study area;
- Value (consequence)—valorization of the territory, depending on economic, social, patrimonial and/or environmental factors;
- Vulnerability—the amount of potential damage caused by coastal erosion. Therefore, vulnerability is dependent of the soil predisposition to erode (susceptibility) and the value attributed to that same area. Vulnerability is independent of coastal erosion conditions and exposure level affecting the study area;
- Consequences—potential harm if coastal erosion affects the study area, combining exposure and vulnerability. Contrary to vulnerability, which is independent of exposure, the consequences include exposure to estimate the amount of area that is going to be affected by coastal erosion;
- Risk—a combination between the potential damage that erosion can cause and the likelihood/intensity that coastal erosion affects the study area.
3. Forecasting Shoreline and Profile Evolution
3.1. Shoreline Long-Term Configuration: LTC
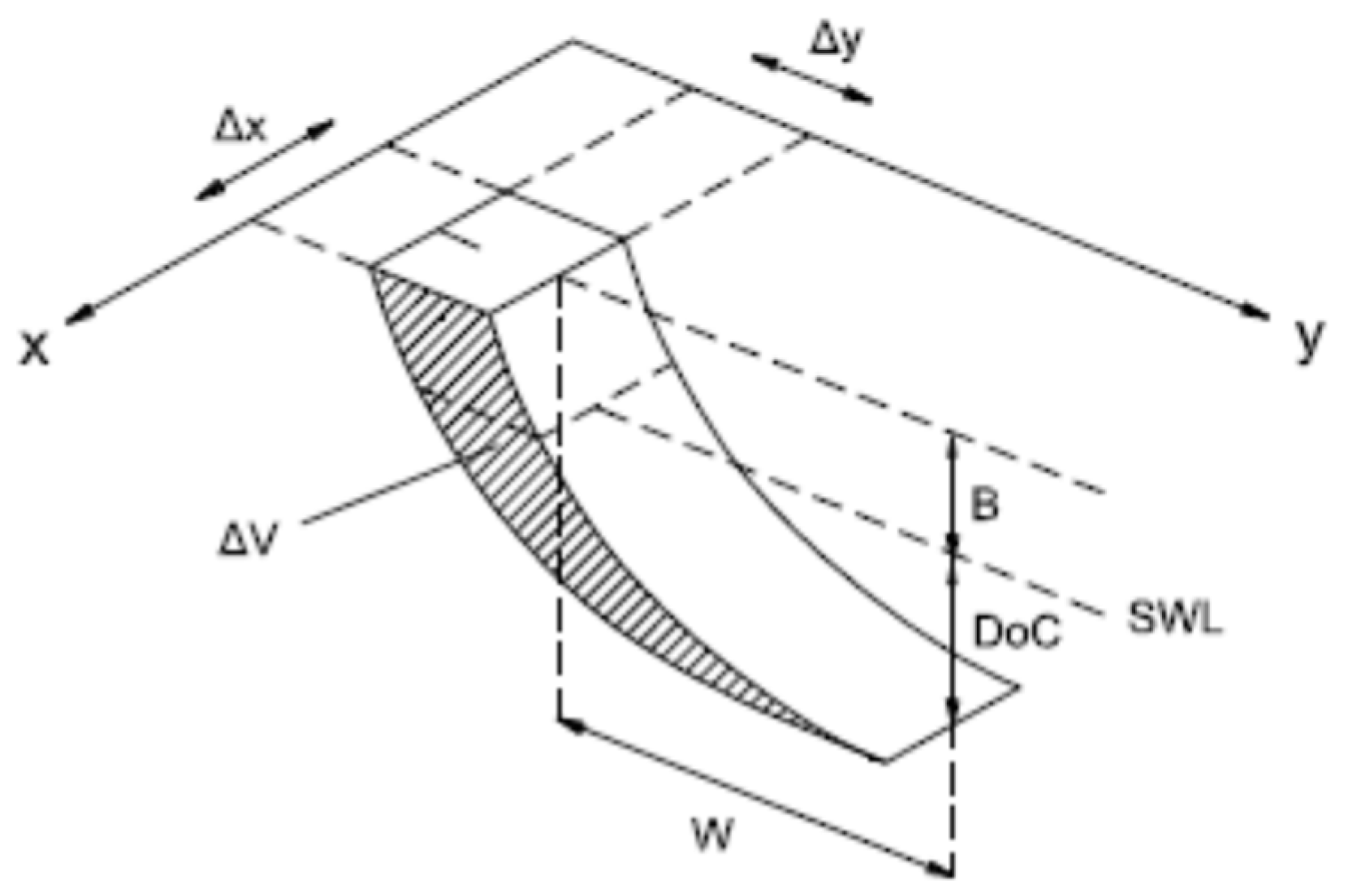
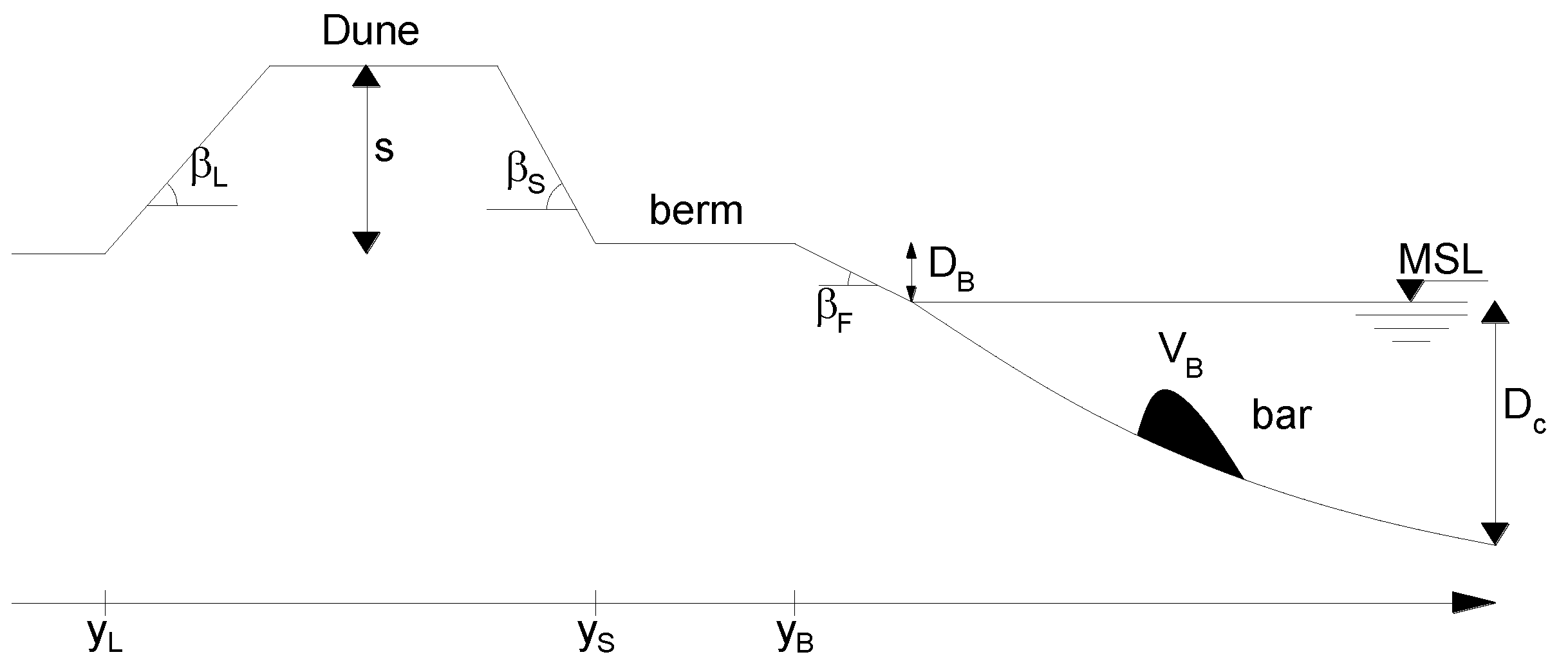
3.2. Cross-Shore Modelling: CS-Model
4. Design of Interventions
4.1. Artifitial Nourishments
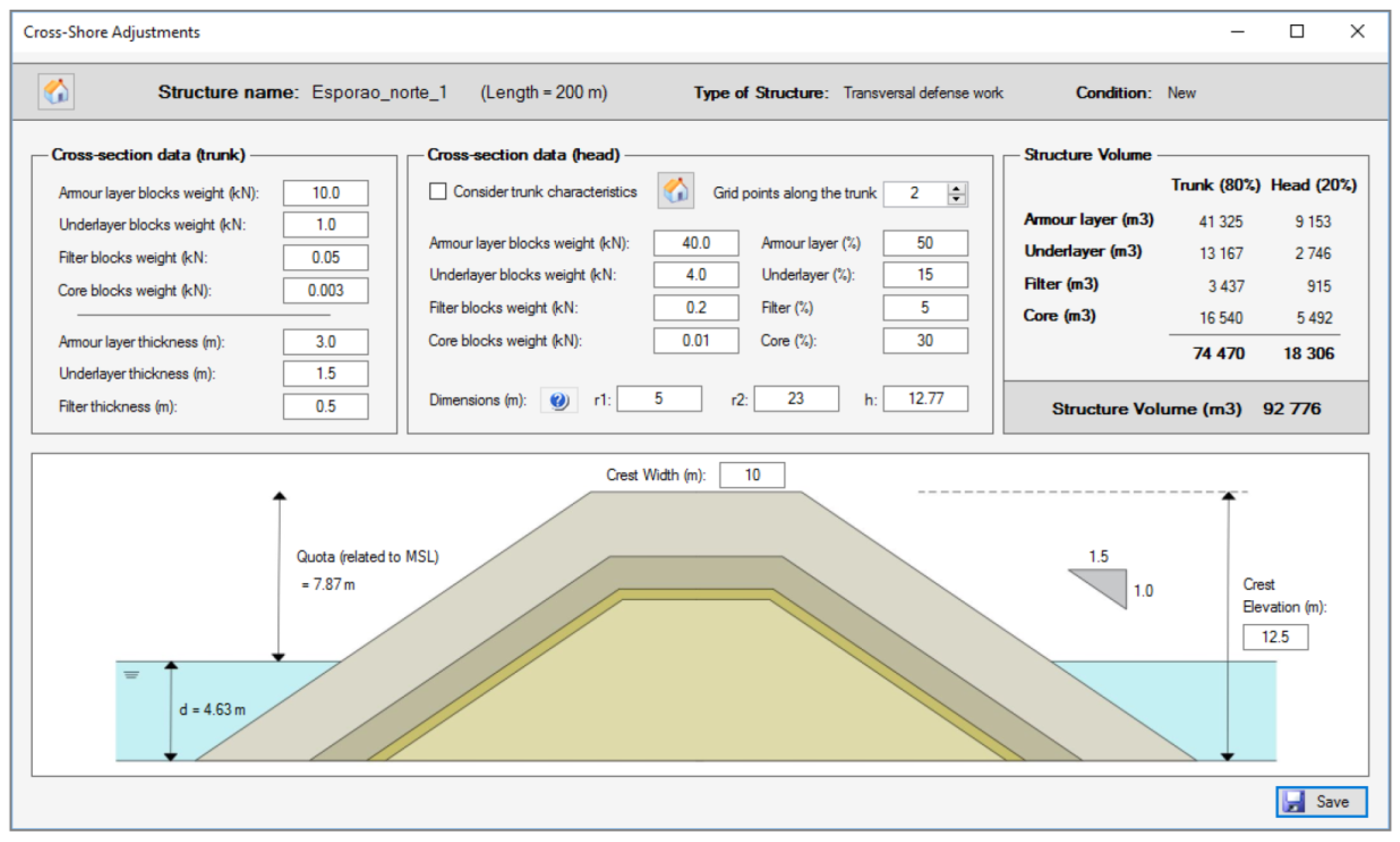
4.2. Design Coastal Structures: XD-Coast
- Shore Protection Manual [73]. It was the first publication of the United States Army Corps of Engineers, in 1974, being updated in 1984. This document contains the fundamental principles of coastal engineering;
- Coastal Engineering Manual (CEM). Following the Shore Protection Manual, CEM presents six parts, divided by chapters, being the Chapter 5 of part VI (Fundamentals of Design, [74]) direct to the pre-design of coastal structures;
- Maritime Structures—Part 7: Guide to design and construction of breakwaters, of British Standard Institution [78]. This document is a practical guide of coastal structures design, being similar to a “Eurocode for Coastal Engineering”.
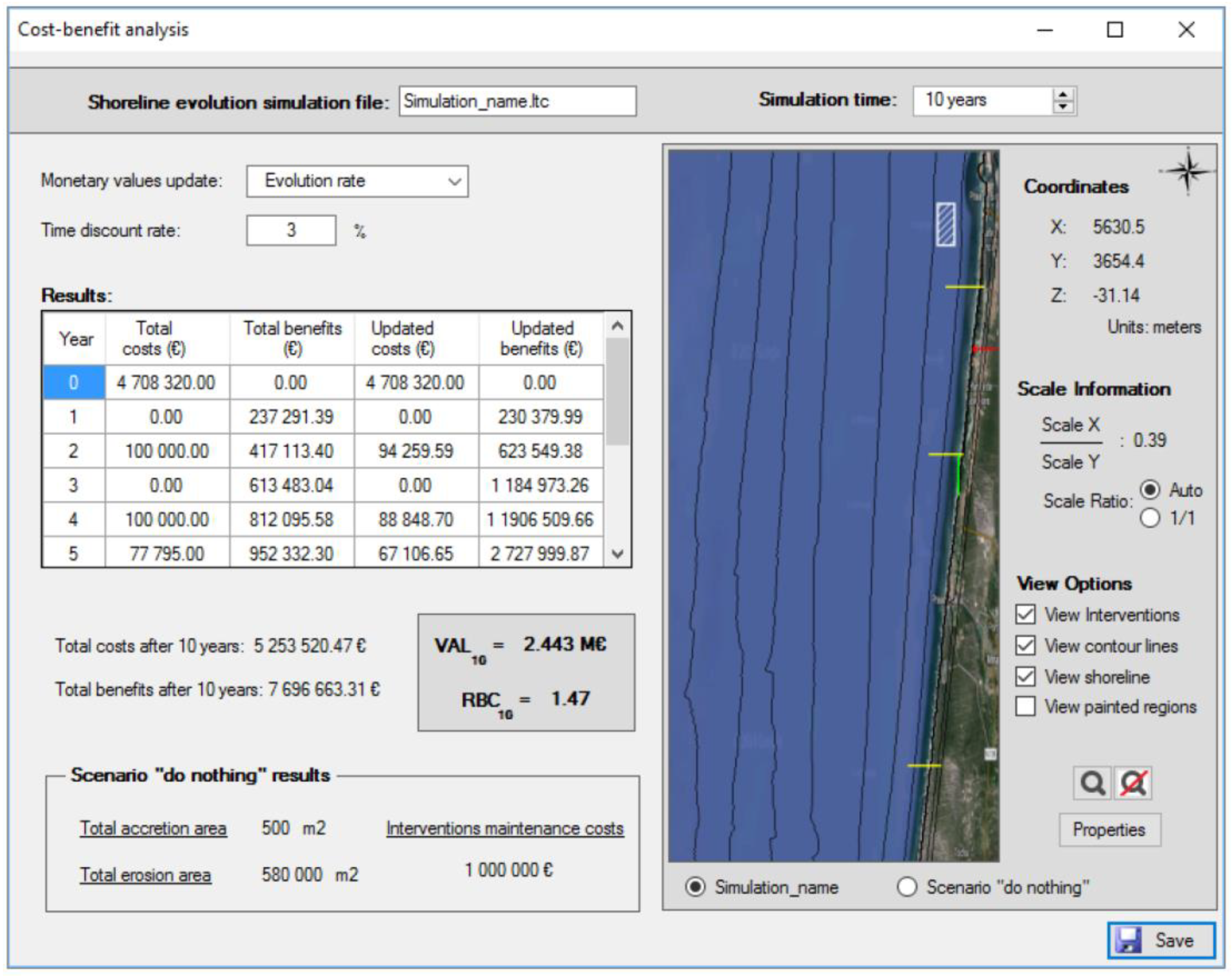
5. Costs and Benefits
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Huang, W.; Hsu, I.; Chen, C.; Ye, C. The Study of the Coastal Management Criteria Based on Risk Assessmeant: A Case Study on Yunlin Coast, Taiwan. Water 2018, 10, 988. [Google Scholar] [CrossRef] [Green Version]
- Williams, A.T.; Rangel-Buitrago, N.; Pranzini, E.; Anfuso, G. The management of coastal erosion. Ocean Coast. Manag. 2018, 156, 4–20. [Google Scholar] [CrossRef]
- Hanson, H.; Brampton, A.; Capobianco, M.; Dette, H.H.; Hamm, L.; Laustrup, C.; Lechuga, A.; Spanhoff, R. Beach nourishment projects, practices, and objectives—A European overview. Coast. Eng. J. 2002, 47, 81–111. [Google Scholar] [CrossRef]
- McFadden, L.; Penning-Roswell, E.; Tapsell, S. Strategic coastal flood-risk management in practice: Actor’s perspectives on the integration of flood risk management in London and the Thames Estuary. Ocean Coast. Manag. 2009, 52, 636–645. [Google Scholar] [CrossRef]
- EC. Participation and integration are key to coastal management. Science for Environment Policy, DG Environment News Alert Service. Eur. Comm. Coast. Manag. 2010, 8.
- Palalane, J. Processes of Long-Term Coastal Evolution and Their Mathematical Modelling—Application to the Mozambican Coast. Ph.D. Thesis, Faculty of Engineering, Lund University, Lund, Sweden, 2016; p. 54. [Google Scholar]
- Marinho, B. Artificial Nourishments as a Coastal Defense Solution: Monitoring and Modelling Approaches. Ph.D. Thesis, University of Aveiro, Aveiro, Portugal, 2018; p. 215. [Google Scholar]
- Marinho, B.; Coelho, C.; Hanson, H.; Tussupova, K. Coastal Management in Portugal: Practices for Reflection and Learn. Ocean Coast. Manag. 2019, 181, 104874. [Google Scholar] [CrossRef]
- Kerguillec, R.; Audère, M.; Baltzer, A.; Debaine, F.; Fattal, P.; Juigner, M.; Launeau, P.; Le Mauff, B.; Luquet, F.; Maanan, M.; et al. Monitoring and management of coastal hazards: Creation of a regional observatory of coastal erosion and storm surges in the pays de la Loire region (Atlantic coast, France). Ocean Coast. Manag. 2019, 181, 104904. [Google Scholar] [CrossRef]
- Booij, N.; Ris, R.C.; Holthuijsen, L.H. A third-generation wave model for coastal regions, Part I, Model description and validation. J. Geophys. Res. 1999, 104, 7649–7666. [Google Scholar] [CrossRef] [Green Version]
- Zijlema, M.; Stelling, G.; Smit, P. SWASH: An operational public domain code for simulating wave fields and rapidly varied flows in coastal waters. Coast. Eng. 2011, 58, 992–1012. [Google Scholar] [CrossRef]
- DHI. Danish Hydraulic Institute. MIKE 21 & MIKE 3 Flow Model FM, Hydrodynamic and Transport, Scientific Documentation; Danish Hydraulic Institute: Hørsholm, Denmark, 2016. [Google Scholar]
- DHI. Danish Hydraulic Institute. MIKE 21 Spectral Wave Module, Scientific Documentation; Danish Hydraulic Institute: Hørsholm, Denmark, 2016. [Google Scholar]
- Mark, B.; Gravens, N.C.; Krauss, T.; Hanson, H. GENESIS: Generalized Model for Simulating Shoreline Change; Technical Report CERC; Department of The Army: Vicksburg, MS, USA, 1991. [Google Scholar]
- Karunarathna, H.; Horrillo-Caraballo, J.; Kuriyama, Y.; Mase, H.; Ranasinghe, R.; Reeve, D. Linkages between sediment composition, wave climate and beach profile variability at multiple timescales. Mar. Geol. 2016, 381, 194–208. [Google Scholar] [CrossRef] [Green Version]
- Roelvink, D.; Reniers, A.; van Dongeren, A.; Van Thiel de Vries, J.; McCall, R.; Lescinski, J. Modelling storm impacts on beaches, dunes and barrier islands. Coast. Eng. 2009, 56, 1133–1152. [Google Scholar] [CrossRef]
- McCall, R.T.; Van Thiel de Vries, J.S.M.; Plant, N.G.; Van Dongeren, A.R.; Roelvink, J.A.; Thompson, D.M.; Reniers, A.J.H.M. Two-dimensional time dependent hurricane overwash and erosion modeling at Santa Rosa Island. Coast. Eng. 2010, 57, 668–683. [Google Scholar] [CrossRef]
- Roelvink, D.; McCall, R.; Seyedabolhossein, M.; Neferhoff, N.; Dastgheib, A. Improving predictions of swash dynamics in XBeach: The role of groupiness and incident-band run-up. Coast. Eng. 2018, 134, 103–123. [Google Scholar] [CrossRef]
- Pender, D.; Karunarathna, H. A statistical-process based approach for modelling beach profile variability. Coast. Eng. 2013, 81, 19–29. [Google Scholar] [CrossRef] [Green Version]
- Pelnard-Considere, R. Essai de Theorie de l’Evolution des Formes de Ravage en Plages de Sables et de Galets; IV’eme Journee de L’Hydraulique Question III, Rapport 1; Societe Hydrotechnique de France: Paris, France, 1956; pp. 74–110. (In French) [Google Scholar]
- Hanson, H.; Kraus, N.C. GENESIS: Generalised Model for Simulating Shoreline Change; Technical Report CERC-89-19; Coastal Engineering Research Station, US Army Corps of Engineers: Vicksburg, MS, USA, 1989; p. 185. [Google Scholar]
- Dabees, M.A.; Kamphuis, J.W. ONELINE, a numerical model for shoreline change. In Proceedings of the ASCE 27th International Conference on Coastal Engineering, ASCE, Vicksburg, MS, USA, 3 August 1998; pp. 2668–2681. [Google Scholar]
- Hanson, H.; Aarninkhof, S.; Capobianco, M.; Jimenez, J.A.; Larson, M.; Nicholls, R.; Plant, N.; Southgate, H.N.; Steetzel, H.J.; Stive, M.J.F.; et al. Modelling coastal evolution on early to decadal time scales. J. Coast. Res. 2003, 19, 790–811. [Google Scholar]
- Baptista, P.; Coelho, C.; Pereira, C.; Bernardes, C.; Veloso-Gomes, F. Beach morphology and shoreline evolution: Monitoring and modelling medium-term responses (Portuguese NW coast study site). Coast. Eng. 2013, 84, 23–37. [Google Scholar] [CrossRef]
- Coelho, C.; Lima, M.; Veloso-Gomes, F. Relationship between Cross-Shore Active Profile and One-Line Shoreline Evolution Models Performance. J. Coast. Res. 2013, 165, 2107–2112. [Google Scholar] [CrossRef]
- Stive, M.J.F.; De Vriend, H.J. Modelling shoreface profile evolution. Mar. Geol. 1995, 126, 235–248. [Google Scholar] [CrossRef] [Green Version]
- Niedoroda, A.W.; Reed, C.W.; Stive, M.; Cowell, P. Numerical simulations for coastal morphodynamic. Coast. Dyn. 2001, 403–412. [Google Scholar]
- Larson, M.; Palalane, J.; Fredriksson, C.; Hanson, H. Simulating cross-shore material exchange at decadal scale. Theory and model component validation. Coast. Eng. J. 2016, 116, 57–66. [Google Scholar] [CrossRef]
- Reeve, D.; Karunarathana, H.; Pan, S.; Horrillo-Caraballo, J.; Rózynski, G.; Ranasinghe, R. Data-Driven and hybrid coastal morphological prediction methods for mesoscale forecasting. Geomorphology 2016, 256, 49–67. [Google Scholar] [CrossRef] [Green Version]
- Narra, P. CERA: GIS-Based Assessment of Coastal Erosion Risk. Ph.D. Thesis, Civil Engineering Department, University of Aveiro, Aveiro, Portugal, 2018; p. 262. [Google Scholar]
- Aven, T. The risk concept-historical and recent development trends. Reliab. Eng. Syst. Saf. 2012, 99, 33–44. [Google Scholar] [CrossRef]
- Faber, M. Statistics and Probability Theory. In Pursuit of Engineering Decision Support, Topics in Safety, Risk, Reliability and Quality; Springer: Dordrecht, The Netherlands, 2012. [Google Scholar]
- ISO. 31000:2009, Risk Management—Principles and Guidelines; International Organization for Standardization: Geneva, Switzerland, 2009. [Google Scholar]
- United Nations Office for Disaster Risk Reduction (ISDR). UNISDR Terminology on Disaster Risk Reduction. In United Nations International Strategy for Disaster Risk Reduction; ISDR: Geneva, Switzerland, 2009. [Google Scholar]
- Smith, K.; Petley, D. Environmental Hazards: Assessing Risk and Reducing Disaster, 5th ed.; Routledge: New York, NY, USA, 2008. [Google Scholar]
- IPCC CZMS. A common methodology for assessing vulnerability to sea level rise. 2nd revision. In Global Climate Change and the Rising Challenge of the Sea; Report of the Coastal Zone Management Subgroup, Response Strategies Working Group of the Intergovernmental Panel on Climate Change; Ministry of Transport, Public Works and Water Management: Hague, The Netherlands, 1992; p. 27. [Google Scholar]
- Leatherman, S.; Nicholls, R.; Denis, K. Aerial Videotape-Assisted Vulnerability Analysis: A cost-effective approach to assess sea-level rise impacts. J. Coast. Res. 1995, 14, 14–25. [Google Scholar]
- Thieler, E.; Hammar-Klose, E. National Assessment of Coastal Vulnerability to Sea-Level Rise, U.S. Atlantic Coast; U.S. Geological Survey Open-File Report; USGS: Lawrence, KS, USA, 1999; pp. 99–593.
- Coelho, C. Riscos de Exposição de Frentes Urbanas para Diferentes Intervenções de Defesa Costeira. Ph.D. Thesis, University of Aveiro, Aveiro, Portugal, 2005; p. 404. (In Portuguese). [Google Scholar]
- Lins-de-Barros, F.; Muehe, D. The smartline approach to coastal vulnerability and social risk assessment applied to a segment of the east coast of Rio de Janeiro State, Brazil. J. Coast. Conserv. 2013, 17, 211–223. [Google Scholar] [CrossRef]
- Mokrech, M.; Hanson, S.; Nicholls, R.; Wolf, J.; Walkden, M.; Fontaine, C.; Nicholson-Cole, S.; Jude, S.; Leake, J.; Stansby, P.; et al. The Tyndall coastal simulator. J. Coast. Conserv. 2011, 15, 325–335. [Google Scholar] [CrossRef]
- Hinkel, J.; Klein, R. Integrating knowledge to assess coastal vulnerability to sea-level rise: The development of the DIVA tool. Glob. Environ. Chang. 2009, 19, 384–395. [Google Scholar] [CrossRef]
- Torresan, S.; Critto, A.; Rizzi, J.; Zabeo, A.; Furlan, E.; Marcomini, A. DESYCO: A decision support system for the regional risk assessment of climate change impacts in coastal zones. Ocean Coast. Manag. 2016, 120, 49–63. [Google Scholar] [CrossRef]
- Warrick, R. Using SimCLIM for modelling the impacts of climate extremes in a changing climate: A preliminary case study of household water harvesting in Southeast Queensland. In Proceedings of the 18th World IMACS Congress and MODSIM09 International Congress on Modelling and Simulation, Cairns, Australia, 13–17 July 2009; pp. 2583–2589. [Google Scholar]
- Zanuttigh, B.; Simcic, D.; Bagli, S.; Bozzeda, F.; Pietrantoni, L.; Zagonari, F.; Hoggart, S.; Nicholls, R.J. THESEUS decision support system for coastal risk management. Coast. Eng. 2014, 87, 218–239. [Google Scholar] [CrossRef]
- Appelquist, L.; Balstrøm, T.; Halsnæs, K. Managing climate change hazards in coastal areas: The Coastal Hazard Wheel decision-support system. U. N. Environ. Programm. 2016, 192. [Google Scholar]
- Viavattene, C.; Jiménez, J.; Ferreira, O.; Priest, S.; Owen, D.; McCall, R. Selecting coastal hotspots to storm impacts at the regional scale: A Coastal Risk Assessment Framework. Coast. Eng. 2018, 134, 33–47. [Google Scholar] [CrossRef]
- European Centre for Disease Prevention and Control (ECDC). Methods for assessing coastal vulnerability to climate change. European Centre for Disease Prevention and Control. In Operational Guidance on Rapid Risk Assessment Methodology; ECDC: Stockholm, Sweden, 2011. [Google Scholar]
- Satta, A.; Snoussi, M.; Puddu, M.; Flayou, L.; Hoult, R. An index-based method to assess risks of climate-related hazards in coastal zones: The case of Tetouan. Estuar. Coast. Shelf Sci. 2016, 175, 93–105. [Google Scholar] [CrossRef]
- Barbaro, G. Master Plan of solutions to mitigate the risk of coastal erosion in Calabria (Italy), a case study. Ocean Coast. Manag. 2016, 132, 24–35. [Google Scholar] [CrossRef]
- Pantusa, D.; D’Alessandro, F.; Riefolo, L.; Principato, F.; Tomasicchio, G. Application of a coastal vulnerability index. A case study along the Apulian Coastline, Italy. Water 2018, 10, 1218. [Google Scholar] [CrossRef] [Green Version]
- Nelson, K.; Burnside, N.G. Identification of marine management priority areas using a GIS-based multi-criteria approach. Ocean Coast. Manag. 2019, 172, 82–92. [Google Scholar] [CrossRef]
- Sarewitz, D.; Pielke, R.; Keykhah, M. Vulnerability and Risk: Some Thoughts from a Political and Policy Perspective. Risk Anal. 2003, 23, 805–810. [Google Scholar] [CrossRef] [Green Version]
- Samuels, P.; Gouldby, B. FLOODsite Language of Risk, 2nd ed.; HR Wallingford: Wallingford, UK, 2009. [Google Scholar]
- Narayan, S.; Nicholls, R.; Clarke, D.; Hanson, S.; Reeve, D.; Horrillo-Caraballo, J.; le Cozannet, G.; Hissel, F.; Kowalska, B.; Parda, R.; et al. The SPR systems model as a conceptual foundation for rapid integrated risk appraisals: Lessons from Europe. Coast. Eng. 2014, 87, 15–31. [Google Scholar] [CrossRef] [Green Version]
- Narra, P.; Coelho, C.; Sancho, F. Multicriteria GIS-based estimation of coastal erosion risk: Implementation to Aveiro sandy coast, Portugal. Ocean Coast. Manag. 2019, 178, 104845. [Google Scholar] [CrossRef]
- Papale, A. Erosion Vulnerability and Risk Assessment Along the Coasts of Veneto Region, Italy. Master’s Thesis, Universita’ di Padova, Padua, Italy, 2018. [Google Scholar]
- Hanson, H. GENESIS—A Generalized Shoreline Change Numerical Model. J. Coast. Res. 1998, 5, 1–27. [Google Scholar]
- Hoan, L.X. Long-Term Simulation of Coastal Evolution; Lund University: Lund, Sweden, 2010; p. 173. [Google Scholar]
- Rosati, J.; Walton, T.; Bodge, K. Longshore Sediment Transport. In Coastal Enginnering Manual, Part III, Coastal Sediment Processes, Chapter III-2, Engineer Manual 1110-2-1100; Walton, T., Ed.; U.S. Army Corps of Engineers: Washington, DC, USA, 2002; p. 119. [Google Scholar]
- Gravens, M.B.; Kraus, N.C.; Hanson, H. GENESIS: Generalized Model for Simulating Shoreline Change; Report 2, Workbook and System User’s Manual; USACE: Washington, DC, USA, 2001; p. 431. [Google Scholar]
- Lima, M.; Coelho, C. LTC shoreline evolution model: Assumptions, evolution, validation and application. J. Integr. Coast. Zone Manag. 2017, 17, 5–17. [Google Scholar]
- Larson, M.; Kraus, N. SBEACH: Numerical Model for Simulating Storm-Induced Beach Change, Report 1: Empirical Foundation and Model Development; Technical Report CERC-89-9; U.S. Army Engineer Waterways Experiment Station, Coastal Engineering Research Center: Vicksburg, MS, USA, 1989. [Google Scholar]
- Oliveira, F. A Dimensão Longilitoral na Resiliência Dunar Durante Tempestades Marítimas; VIII Congresso sobre Planeamento e Gestão das Zonas Costeiras dos Países de Expressão Portuguesa: Aveiro, Portugal, 2015; p. 11. (In Portuguese) [Google Scholar]
- Smith, E.; D’Alessandro, F.; Tomasicchio, G.; Cailani, J. Nearshore placement of a sand dredged mound. Coast. Eng. J. 2017, 126, 1–10. [Google Scholar] [CrossRef]
- Larson, M.; Hanson, H.; Palalane, J. Simulating cross-shore material exchange in long-term coastal evolution models. In Proceedings of the Coastal Dynamics’13, Arcachon, France, 24–28 June 2013; pp. 1037–1048. [Google Scholar]
- Marinho, B.; Larson, M.; Coelho, C.; Hanson, H. Long-term Coastal Evolution Modelling of Longshore Bars. In Proceedings of the 36th International Conference on Coastal Engineering ICCE’18, Baltimore, MD, USA, 30 July–3 August 2018. [Google Scholar]
- Larson, M.; Kraus, N. Mathematical modeling of the fate of beach fill. Coast. Eng. J. 1991, 16, 83–114. [Google Scholar] [CrossRef]
- Karunarathna, H.; Horrillo-Caraballo, J.; Ranasinghe, R.; Short, A.; Reeve, D. An analysis of the cross-shore beach morphodynamics of a sandy and a composite gravel beach. Mar. Geol. 2012, 299–302, 33–42. [Google Scholar] [CrossRef]
- Dubarbier, B.; Castelle, B.; Marieu, V.; Ruessink, G. Process-based modeling of cross-shore sandbar behavior. Coast. Eng. J. 2015, 95, 35–50. [Google Scholar] [CrossRef]
- Banyard, L.S. Breakwaters, Coastal Structures and Coastlines; Paper 15: Design of rock groynes and sand beach replenishment under strong tidal flows; N.W.H., ICE Publishing: New York, NY, USA, 2002; pp. 191–202. [Google Scholar]
- Lima, M. Ferramenta Numérica de Análise do Impacto de Intervenções de Defesa Costeira na Evolução da Linha de Costa: Custos e Benefícios. Ph.D. Thesis, University of Aveiro, Aveiro, Portugal, 2018; p. 294. (In Portuguese). [Google Scholar]
- Shore Protection Manual, 4th ed.; Volume 2, U.S. Army Engineer Waterways Experiment Station, U.S. Government Printing Office: Washington, DC, USA, 1984.
- Burcharth, H.F.; Hughes, S.A. Fundamentals of Design. Coastal Engineering Manual; Part VI: Design of Coastal Project Elements, Chapter 5; U.S. Army Corps of Engineers: Washington, DC, USA, 2006; p. 312.
- CIRIA. The Rock Manual. In The Use of Rock in Hydraulic Engineering, 2nd ed.; C683; CIRIA: London, UK, 2007. [Google Scholar]
- CIRIA. The Rock Manual. In The Use of Rock in Hydraulic Engineering, 2nd ed.; Chapter V—Physical Processes and Design Tools, C683; CIRIA: London, UK, 2007; pp. 487–756. [Google Scholar]
- CIRIA. The Rock Manual. In The Use of Rock in Hydraulic Engineering, 2nd ed.; Chapter VI—Design of Marine Structures, C683; CIRIA: London, UK, 2007; pp. 773–908. [Google Scholar]
- British Standard Institution. Maritime Structures—Part 7: Guide to the Design and Construction of Breakwaters; BS 6349-7:1991; British Standard Institution: London, UK, 1999; p. 52. [Google Scholar]
- Pilarczyk, K.W. Coastal Structures in International Perspective. In Coastal and Ocean Engineering Practice; Young, C.K., Ed.; Series on Coastal and Ocean Engineering Practice—Volume 1; World Scientific: Singapore, 2014; pp. 39–94. [Google Scholar]
- Fleming, C.A.; Allsop, N.W.H.; Leeming, M.B.; Sharp, B.N.; Slater, D.; Williams, A.J.; Titman, P. Concrete in Coastal Structures; Allen, R.T.L., Ed.; Thomas Telford: London, UK, 1998; p. 301. [Google Scholar]
- Herbich, J.B. Handbook of Coastal Engineering; Chapter 8: Coastal Protection Methods; McGraw-Hill: New York, NY, USA, 2008; p. 66. [Google Scholar]
- French, P.W. Coastal Defences: Processes, Problems and Solutions; Routledge: London, UK, 2001; p. 366. [Google Scholar]
- Reeve, D.; Chadwick, A.; Fleming, C. Coastal Engineering. In Processes, Theory and Design Practice; Spon Press: New York, NY, USA, 2004; p. 461. [Google Scholar]
- Deltares. Enabling Delta Life. Breakwat Software. Last. Available online: https://www.deltares.nl/en/software/breakwat/ (accessed on 25 May 2016).
- National Academic Research and Collaborations Information System. Last. Available online: http://www.narcis.nl/research/RecordID/OND1307179 (accessed on 25 May 2016).
- Coastal and River Engineering Support System. Available online: http://www.cress.nl/About.aspx (accessed on 25 May 2016).
- Concrete Layer Innovations. Available online: http://www.concretelayer.com/fr/calculateur (accessed on 26 May 2017).
- Hudson, R.Y. Concrete Armor Units for Protection Against Wave Attack; Miscellaneous, Paper H-74-2; U.S. Army Engineer Waterways Experiment Station: Vicksburg, MS, USA, 1974. [Google Scholar]
- Lima, M. Programação de Métodos de Pré-Dimensionamento de Obras Costeiras. Master’s Thesis, University of Aveiro, Aveiro, Portugal, 2011; p. 131. [Google Scholar]
- Lima, M.; Coelho, C.; Cachim, P. Programming Methods for Pre-Design of Coastal Structures. In Proceedings of the V International Conference on Computational Methods in Marine Engineering, Marine 2013, Hamburg, Germany, 29–31 May 2013; pp. 868–879. [Google Scholar]
- van der Meer, J.W. Stability of Cubes, Tetrapods and Accropode. In Proceedings of the Breakwaters ’88 Conference, Design of Breakwaters, Institution of Civil Engineers, Thomas Telford, London, UK, 19–20 March 1988; pp. 71–80. [Google Scholar]
- van der Meer, J.W. Rock Slopes and Gravel Beaches under Wave Attack. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1988; p. 214. [Google Scholar]
- De Jong, T.J. Stability of Tetrapods at Front Crest and Rear of a Low-Crested Breakwater; Delft Hydraulics Publication No. 453; Delft Hydraulics Laboratory: Delft, The Netherlands, 1996. [Google Scholar]
- van der Meer, J.W. Stability and Transmission at Low-Crested Structures; Delft Hydraulics Publication No. 453; Delft Hydraulics Laboratory: Delft, The Netherlands, 1991. [Google Scholar]
- Breil, M.; Catenacci, M.; Travisi, C. Impatti del Cambiamento Climatico Sulle Zone Costiere: Quantificazione Economica di Impatti e di Misure di Adattamento-Sintesi di Risultati e Indicazioni Metodologiche per la Ricerca Futura; Report prepared for the APAT Workshop; APAT Workshop: Palermo, Italy, 27–28 June 2007. [Google Scholar]
- Costa, L.; Tekken, V.; Kropp, J. Threat of sea level rise: Costs and benefits of adaptation in European union coastal countries. J. Coast. Res. 2009, 56, 223–227. [Google Scholar]
- Darwin, R.F.; Tol, R.S.J. Estimates of the Economic Effects of Sea Level Rise. Environ. Resour. Econ. 2011, 19, 113–129. [Google Scholar] [CrossRef] [Green Version]
- Bosello, F.; Roson, R.; Tol, R.S.J. Economy-wide Estimates of the Implications of Climate Change: Sea Level Rise. Environ. Resour. Econ. 2007, 37, 549–571. [Google Scholar] [CrossRef] [Green Version]
- Martinez, M.L.; Intralawan, A.; Vazquez, G.; Perez-Maqueo, O.; Sutton, P.; Landgrave, R. The coasts of our world: Ecological, economic and social importance. Ecol. Econ. 2007, 63, 254–272. [Google Scholar] [CrossRef]
- Turner, R.K.; Burgess, D.; Hadley, D.; Coombes, E.; Jackson, N. A cost-benefit appraisal of coastal managed realignment policy. Glob. Environ. Chang. 2007, 17, 397–407. [Google Scholar] [CrossRef]
- Neumann, J.; Hudgens, D.; Herter, J.; Martinich, J. The economics of adaptation along developed coastlines. Wiley Interdisciplinary Reviews: Clim. Chang. 2011, 2, 89–98. [Google Scholar] [CrossRef]
- Roebeling, P.; Coelho, C.; Reis, E. Coastal erosion and coastal defense interventions: A cost-benefit analysis. J. Coast. Res. 2011, 64, 1415–1419. [Google Scholar]
- Roebeling, P.; Rocha, J.; Coelho, C.; Alves, F.; Vizinho, A. Cost-Benefit Analysis of Coastal Erosion Adaptation Pathways Along the Barra-Vagueira Coastal Stretch. In Project BASE—Bottom-Up Climate Adaptation Strategies for Europe; Universidade de Aveiro: Aveiro, Portugal, 2015. [Google Scholar]
- Alexandrakis, G.; Manasakis, C.; Kampanis, N.A. Valuating the effects of beach erosion to tourism revenue. A management perspective. Ocean Coast. Manag. 2015, 111, 1–11. [Google Scholar] [CrossRef]
- Martino, S.; Amos, C.L. Valuation of the ecosystem services of beach nourishment in decision-making: The case study of Tarquinia Lido, Italy. Ocean Coast. Manag. 2015, 111, 82–91. [Google Scholar] [CrossRef]
- Nicholls, R.J.; Tol, R.S.J. Impacts and responses to sea-level rise: A global analysis of the SRES scenarios over the twenty-first century. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2006, 364, 1073–1095. [Google Scholar] [CrossRef] [PubMed]
- Macintosh, A. Coastal climate hazards and urban planning: How planning responses can lead to maladaptation. Mitig. Adapt. Strateg. Glob. Chang. 2013, 18, 1035–1055. [Google Scholar] [CrossRef]
- Wise, R.M.; Fazey, I.; Stafford Smith, M.; Park, S.; Eakin, H.C.; Archer Van Garderen, E.R.M.; Campbell, B. Reconceptualising adaptation to climate change as part of pathways of change and response. Glob. Environ. Chang. 2014, 28, 325–336. [Google Scholar] [CrossRef] [Green Version]
- Ellison, J.; Strickland, P. Establishing relative sea level trends where a coast lacks a long term tide gauge. Mitig. Adapt. Strateg. Glob. Chang. 2015, 20, 1211–1227. [Google Scholar] [CrossRef]
- Roebeling, P.; d’Elia, E.; Coelho, C.; Alves, T. Efficiency in the design of coastal erosion adaptation strategies: An environmental-economic modelling approach. Ocean Coast. Manag. 2018, 160, 175–184. [Google Scholar] [CrossRef]
- Costanza, R.; d’Arge, R.; de Groot, R.; Farber, S.; Grasso, M.; Hannon, B.; Limburg, K.; Naeem, S.; O’Neill, R.V.; Paruelo, J.; et al. The value of the world’s ecosystem services and natural capital. In Nature; Nature Publishing Group: London, UK, 1997; Volume 387, p. 253. [Google Scholar]
- Bossard, M.; Feranec, J.; Otahel, J. CORINE Land Cover Technical Guide—Addendum 2000; 284 EEA Technical Report No. 40/2000; European Environment Agency: Copenhagen, Denmark, 2000; p. 105.
- Zerbe, R.O.; Dively, D.D. Benefit-Cost Analysis in Theory and Practice; Harper Collins College Publishers: New York, NY, USA, 1994; p. 557. [Google Scholar]
- Hinkel, J. DIVA: An iterative method for building modular integrated models. J. Adv. Geosci. 2005, 4, 45–50. [Google Scholar] [CrossRef] [Green Version]
- Klein, R.J.T.; Hinkel, J. Global Assessment of Coastal Vulnerability to Sea-Level Rise: Experience from DINAS-COAST. Chapter 5. In Assessing Vulnerability to Global Environmental Change; Routledge: London, UK, 2009; p. 18. [Google Scholar]
- Lima, M.; Coelho, C. Assessing Costs and Benefits of Coastal Structures to Mitigate Erosion. In Proceedings of the Coastal Structures 2019, Hannover, Germany, 30 September–2 October 2019; pp. 864–874. [Google Scholar]
- Lima, M.; Coelho, C.; Veloso-Gomes, F.; Roebeling, P. An integrated physical and cost-benefit approach to assess groins as a coastal erosion mitigation strategy. Coast. Eng. 2020, 156, 103614. [Google Scholar] [CrossRef]

| Name | Year | Reference | Objective |
|---|---|---|---|
| IPCC Common Methodology (CM) | 1991 | [36] | Assessment of potential coastal impacts of sea-level rise and adaptation measures |
| Aerial Videotape-Assisted Vulnerability Analysis (AVVA) | 1992 | [37] | Assessment of coastal vulnerability to sea-level rise |
| Coastal Vulnerability Index (CVI) | 1999 | [38] | Assessment of relative vulnerability of the coast to changes due to sea-level rise |
| Coastal Vulnerability and Risk Assessment (CVRA) | 2005 | [39] | Assessment of coastal vulnerability and risk to coastal erosion |
| Smartline (SL) | 2006 | [40] | Physical and social vulnerability assessment to coastal erosion and flooding and resulting coastal risk |
| Tyndall Coastal Simulator (TCS) | 2000 | [41] | Determining geomorphological response to climate change and its effects on coastline evolution |
| Dynamic and Interactive Vulnerability Assessment (DIVA) | 2004 | [42] | Assessment of coastal vulnerability for user-selected climatic, and socio-economic scenarios and adaptation strategies |
| Decision Support System for Coastal Climate Change Impact (DESYCO) | 2005 | [43] | Assessment of climate change impacts on coastal areas and related ecosystems |
| SimCLIM (SC) | 2005 | [44] | Simulation of bio-physical impacts and socio-economic effects of climatic variations |
| THESEUS DSS | 2009 | [45] | Assessment of vulnerability, impacts and risks of coastal areas and identification and evaluation of coastal adaptation options |
| Coastal Hazard Wheel (CHW) | 2012 | [46] | Assessment of coastal hazard level for multi-hazard scenarios |
| RISC-KIT Coastal Risk Assessment Framework 1 (CRAF1) | 2013 | [47] | Assessment of hazard, exposure and risk of coastal zones for identification of high-risk segments |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Coelho, C.; Narra, P.; Marinho, B.; Lima, M. Coastal Management Software to Support the Decision-Makers to Mitigate Coastal Erosion. J. Mar. Sci. Eng. 2020, 8, 37. https://doi.org/10.3390/jmse8010037
Coelho C, Narra P, Marinho B, Lima M. Coastal Management Software to Support the Decision-Makers to Mitigate Coastal Erosion. Journal of Marine Science and Engineering. 2020; 8(1):37. https://doi.org/10.3390/jmse8010037
Chicago/Turabian StyleCoelho, Carlos, Pedro Narra, Bárbara Marinho, and Márcia Lima. 2020. "Coastal Management Software to Support the Decision-Makers to Mitigate Coastal Erosion" Journal of Marine Science and Engineering 8, no. 1: 37. https://doi.org/10.3390/jmse8010037
APA StyleCoelho, C., Narra, P., Marinho, B., & Lima, M. (2020). Coastal Management Software to Support the Decision-Makers to Mitigate Coastal Erosion. Journal of Marine Science and Engineering, 8(1), 37. https://doi.org/10.3390/jmse8010037