Sliding Mode Control in Backstepping Framework for a Class of Nonlinear Systems
Abstract
:1. Introduction
2. SBC of SISO Systems
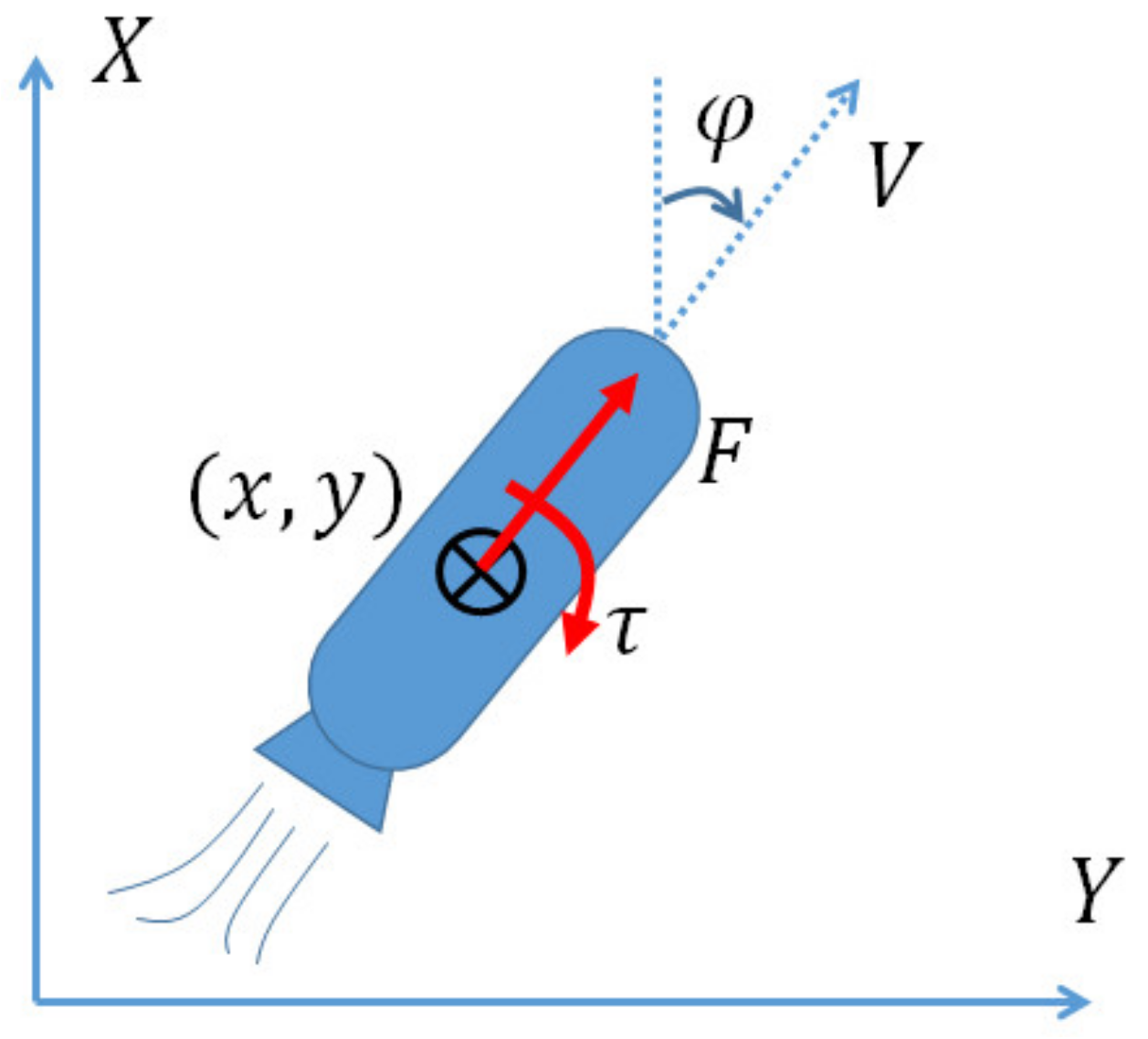
2.1. Problem Formulation
2.2. SBC Design
2.3. Integral SBC Control
2.4. Chattering Effects
3. SBC of MIMO Systems
4. Compare SBC to SMC
4.1. Recall SMC Design
4.2. Sliding Surface and Chatter
4.3. Useful Nonlinear Dynamics
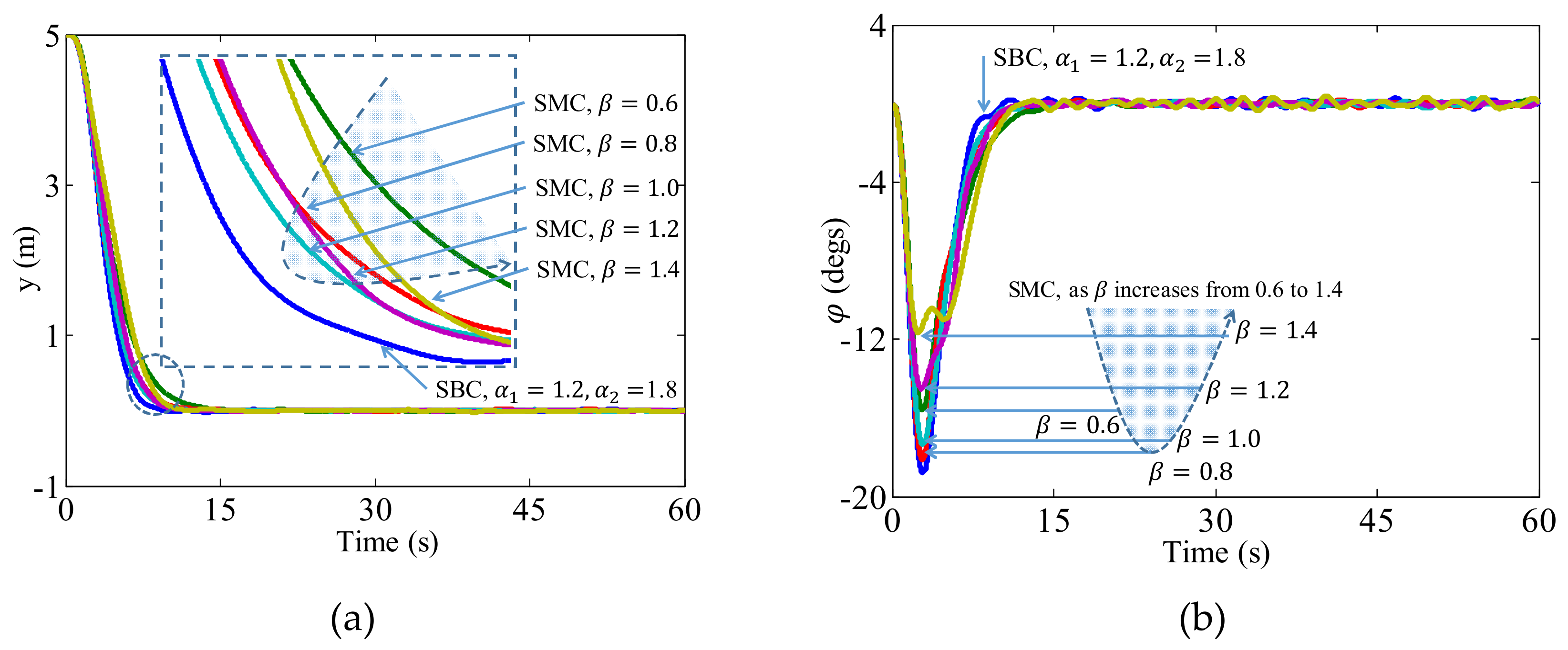
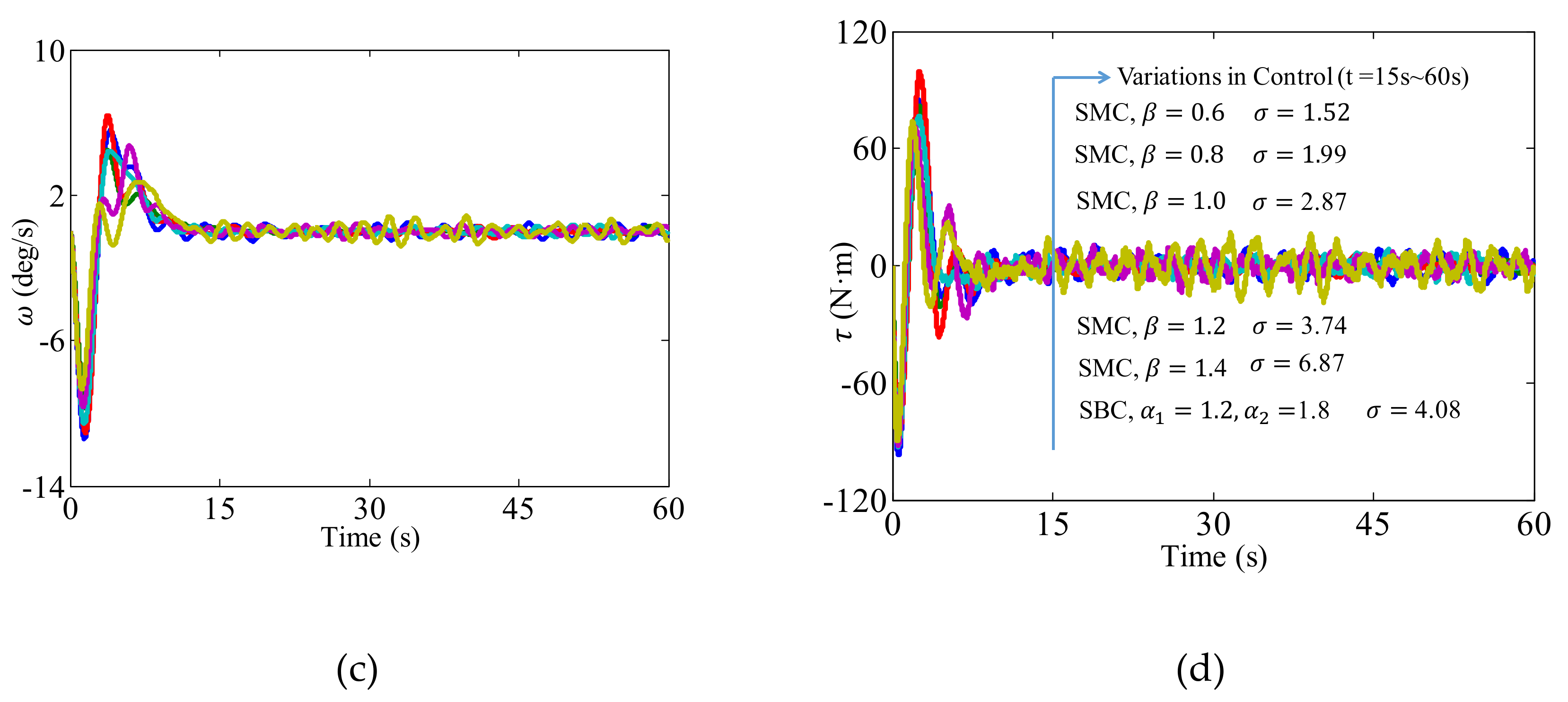
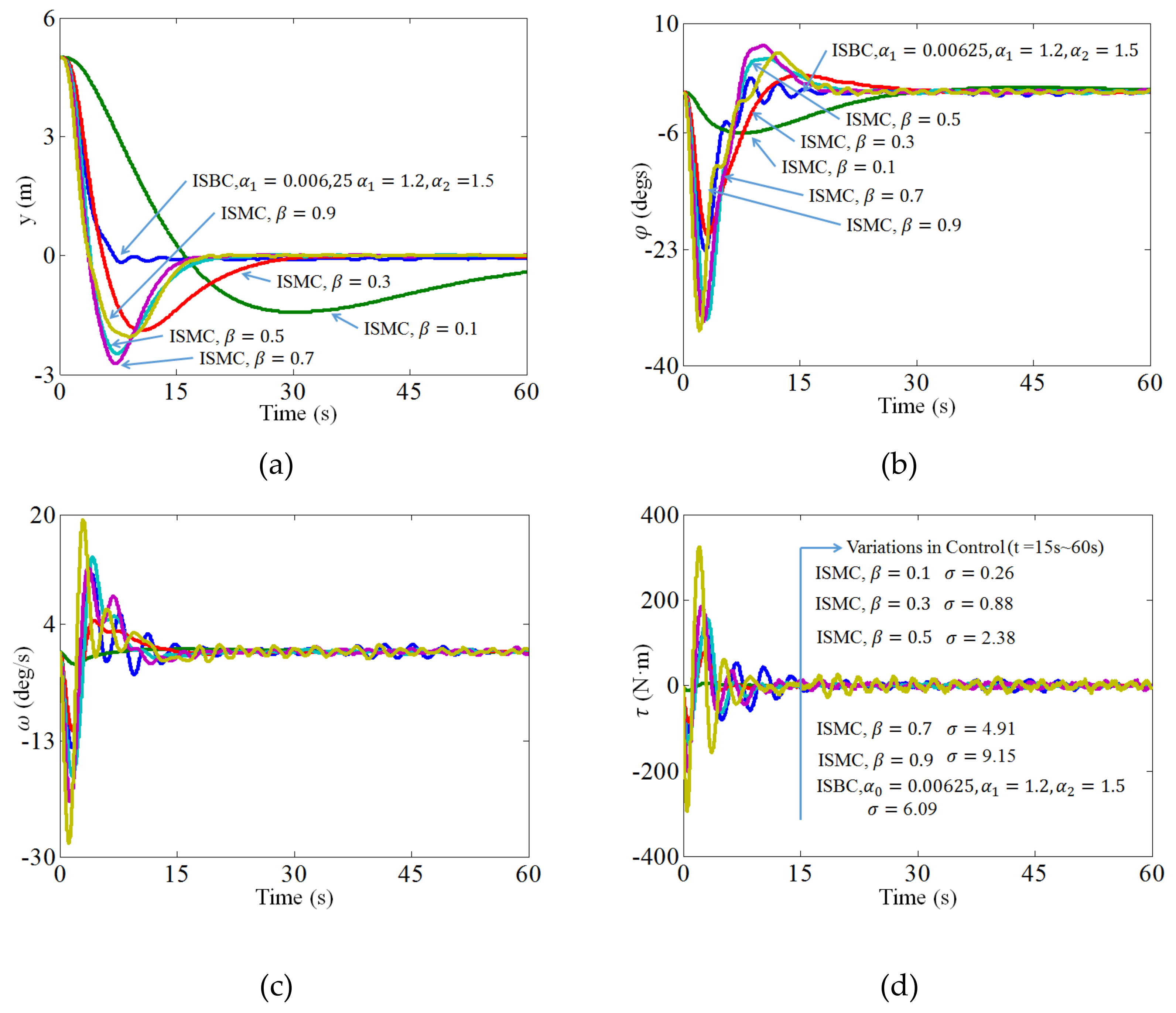
5. Simulation Results
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Khalil, H. Nonlinear Systems, 3rd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2001. [Google Scholar]
- Chen, M.; Ge, S.S.; Ren, B. Robust attitude control of helicopters with actuator dynamics using neural networks. IET Control Theory Appl. 2010, 4, 2837–2854. [Google Scholar] [CrossRef] [Green Version]
- Ki-Seok, K.; Youdan, K. Robust backstepping control for slew maneuver using nonlinear tracking function. IEEE Trans. Control Syst. Technol. 2003, 11, 822–829. [Google Scholar] [CrossRef] [Green Version]
- Mou, C.; Bin, J. Robust bounded control for uncertain flight dynamics using disturbance observer. J. Syst. Eng. Electron. 2014, 25, 640–647. [Google Scholar]
- Azinheira, J.; Moutinho, A. Hover Control of an UAV with Backstepping Design Including Input Saturations. IEEE Trans. Control Syst. Technol. 2008, 16, 517–526. [Google Scholar] [CrossRef]
- Chen, M.; Ge, S.; How, B.V.; Choo, Y.S. Robust adaptive position mooring control for marine vessels. IEEE Trans. Control Syst. Technol. 2013, 21, 395–409. [Google Scholar] [CrossRef]
- Fossen, T.; Grovlen, A. Nonlinear output feedback control of dynamically positioned ships using vectorial observer backstepping. IEEE Trans. Control Syst. Technol. 1998, 6, 121–128. [Google Scholar] [CrossRef]
- Ghommam, J.; Mnif, F.; Benali, A.; Derbel, N. Asymptotic Backstepping Stabilization of an Underactuated Surface Vessel. IEEE Trans. Control Syst. Technol. 2006, 14, 1150–1157. [Google Scholar] [CrossRef]
- Kwan, C.; Lewis, F. Robust backstepping control of induction motors using neural networks. IEEE Trans. Neural Netw. 2000, 11, 1178–1187. [Google Scholar] [CrossRef]
- Drid, S.; Naït-Saïd, M.-S.; Tadjine, M. Robust backstepping vector control for the doubly fed induction motor. IET Control Theory Appl. 2007, 1, 861–868. [Google Scholar] [CrossRef]
- Lin, F.-J.; Lee, C.-C. Adaptive backstepping control for linear induction motor drive to track periodic references. IEE Proc. Electr. Power Appl. 2000, 147, 449. [Google Scholar] [CrossRef]
- Zhao, D.; Gao, F.; Li, S.; Zhu, Q. Robust finite-time control approach for robotic manipulators. IET Control Theory Appl. 2010, 4, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Yoo, S.J.; Park, J.B.; Choi, Y.H. Adaptive Output Feedback Control of Flexible-Joint Robots Using Neural Networks: Dynamic Surface Design Approach. IEEE Trans. Neural Netw. 2008, 19, 1712–1726. [Google Scholar]
- Shojaei, K.; Shahri, A. Adaptive robust time-varying control of uncertain non-holonomic robotic systems. IET Control Theory Appl. 2012, 6, 90. [Google Scholar] [CrossRef]
- Zhou, H.; Liu, Z. Vehicle Yaw Stability-Control System Design Based on Sliding Mode and Backstepping Control Approach. IEEE Trans. Veh. Technol. 2010, 59, 3674–3678. [Google Scholar] [CrossRef]
- Shen, P.-H.; Lin, F.-J. Intelligent backstepping sliding-mode control using RBFN for two-axis motion control system. IEE Proc. Electr. Power Appl. 2005, 152, 1321. [Google Scholar] [CrossRef]
- Liu, Z.; Jia, X. Novel backstepping design for blended aero and reaction-jet missile autopilot. J. Syst. Eng. Electron. 2008, 19, 148–153. [Google Scholar]
- Hua, C.; Liu, P.X.; Guan, X.; Liu, P.; Liu, P. Backstepping Control for Nonlinear Systems With Time Delays and Applications to Chemical Reactor Systems. IEEE Trans. Ind. Electron. 2009, 56, 3723–3732. [Google Scholar]
- Mazenc, F.; Bliman, P.-A. Backstepping Design for Time-Delay Nonlinear Systems. IEEE Trans. Autom. Control 2006, 51, 149–154. [Google Scholar] [CrossRef] [Green Version]
- Shiromoto, H.S.; Andrieu, V.; Prieur, C. Relaxed and Hybridized Backstepping. IEEE Trans. Autom. Control 2013, 58, 3236–3241. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Fidan, B.; Ioannou, P.A. Backstepping control of linear time-varying systems with known and unknown parameters. IEEE Trans. Autom. Control 2003, 48, 1908–1925. [Google Scholar] [CrossRef]
- Cheng, C.-C.; Su, G.-L.; Chien, C.-W. Block backstepping controllers design for a class of perturbed non-linear systems with m blocks. IET Control Theory Appl. 2012, 6, 2021–2030. [Google Scholar] [CrossRef]
- Zhou, J.; Zhang, C.; Wen, C. Robust Adaptive Output Control of Uncertain Nonlinear Plants with Unknown Backlash Nonlinearity. IEEE Trans. Autom. Control 2007, 52, 503–509. [Google Scholar] [CrossRef]
- Wang, Z.; Ge, S.; Lee, T. Robust adaptive neural network control of uncertain nonholonomic systems with strong nonlinear drifts. IEEE Trans. Syst. Man Cybern. Part B Cybern. 2004, 34, 2048–2059. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Hu, J. Robust adaptive neural control for a class of uncertain non-linear time-delay systems with unknown dead-zone non-linearity. IET Control Theory Appl. 2011, 5, 1782–1795. [Google Scholar] [CrossRef]
- Mazenc, F.; Ito, H. Lyapunov technique and backstepping for nonlinear neutral systems. IEEE Trans. Autom. Control 2013, 58, 512–517. [Google Scholar] [CrossRef]
- Hyeongcheol, L. Robust adaptive fuzzy control by backstepping for a class of MIMO nonlinear systems. IEEE Trans. Fuzzy Syst. 2011, 19, 265–275. [Google Scholar]
- Wang, H.; Chen, B.; Liu, X.; Liu, K.; Lin, C. Robust Adaptive Fuzzy Tracking Control for Pure-Feedback Stochastic Nonlinear Systems With Input Constraints. IEEE Trans. Cybern. 2013, 43, 2093–2104. [Google Scholar] [CrossRef]
- Tong, S.-C.; He, X.-L.; Zhang, H.-G. A Combined Backstepping and Small-Gain Approach to Robust Adaptive Fuzzy Output Feedback Control. IEEE Trans. Fuzzy Syst. 2009, 17, 1059–1069. [Google Scholar] [CrossRef]
- Wai, R.; Lee, J. Robust levitation control for linear maglev rail system using fuzzy neural network. IEEE Trans. Control Syst. Technol. 2009, 17, 4–14. [Google Scholar]
- Shafiei, S.E.; Soltanpour, M.R. Robust neural network control of electrically driven robot manipulator using backstepping approach. Int. J. Adv. Robot. Syst. 2009, 6, 285–292. [Google Scholar] [CrossRef]
- Kwan, C.; Lewis, F. Robust backstepping control of nonlinear systems using neural networks. IEEE Trans. Syst. Man Cybern. Part A Syst. Hum. 2000, 30, 753–766. [Google Scholar] [CrossRef]
- Chen, M.; Ge, S.S.; How, B. Robust Adaptive Neural Network Control for a Class of Uncertain MIMO Nonlinear Systems with Input Nonlinearities. IEEE Trans. Neural Netw. 2010, 21, 796–812. [Google Scholar] [CrossRef] [PubMed]
- Kuljaca, O.; Swamy, N.; Lewis, F.; Kwan, C. Design and implementation of industrial neural network controller using backstepping. IEEE Trans. Ind. Electron. 2003, 50, 193–201. [Google Scholar] [CrossRef]
- Hsu, C.-F.; Lin, C.-M.; Lee, T.-T. Wavelet Adaptive Backstepping Control for a Class of Nonlinear Systems. IEEE Trans. Neural Netw. 2006, 17, 1175–1183. [Google Scholar]
- Wai, R.-J.; Chang, H.-H. Backstepping Wavelet Neural Network Control for Indirect Field-Oriented Induction Motor Drive. IEEE Trans. Neural Netw. 2004, 15, 367–382. [Google Scholar] [CrossRef]
- Hua, C.; Guan, X.; Shi, P. Robust backstepping control for a class of time delayed systems. IEEE Trans. Autom. Control 2005, 50, 894–899. [Google Scholar]
- Ezal, K.; Pan, Z.; Kokotovic, P. Locally optimal and robust backstepping design. IEEE Trans. Autom. Control 2000, 45, 260–271. [Google Scholar] [CrossRef]
- French, M. An analytical comparison between the nonsingular quadratic performance of robust and adaptive backstepping designs. IEEE Trans. Autom. Control 2002, 47, 670–675. [Google Scholar] [CrossRef]
- Sabanovic, A. Variable structure systems with sliding modes in motion vontrol-a survey. IEEE Trans. Ind. Inform. 2011, 7, 212–223. [Google Scholar] [CrossRef]
- Young, K.; Utkin, V.; Ozguner, U. A control engineer’s guide to sliding mode control. IEEE Trans. Control Syst. Technol. 1999, 7, 328–342. [Google Scholar] [CrossRef] [Green Version]
- Slotine, J.J.; Li, W. Applied Nonlinear Control; Prentice-Hall International: Upper Saddle River, NJ, USA, 1991. [Google Scholar]
- Kachroo, P.; Tomizuka, M. Chattering reduction and error convergence in the sliding-mode control of a class of nonlinear systems. IEEE Trans. Autom. Control 1996, 41, 1063–1068. [Google Scholar] [CrossRef] [Green Version]
- Fallaha, C.J.; Saad, M.; Kanaan, H.Y.; Al-Haddad, K. Sliding-mode robot control with exponential reaching law. IEEE Trans. Ind. Electron. 2011, 58, 600–610. [Google Scholar] [CrossRef]
- Acary, V.; Brogliato, B.; Orlov, Y.V. Chattering-rree digital sliding-mode control with state observer and disturbance rejection. IEEE Trans. Autom. Control 2012, 57, 1087–1101. [Google Scholar] [CrossRef] [Green Version]
- Lin, C.-K.; Liu, T.-H.; Wei, M.-Y.; Fu, L.-C.; Hsiao, C.-F. Design and implementation of a chattering-free non-linear sliding-mode controller for interior permanent magnet synchronous drive systems. IET Electr. Power Appl. 2012, 6, 332. [Google Scholar] [CrossRef]
- Lin, F.-J.; Shen, P.-H.; Hsu, S.-P. Adaptive backstepping sliding mode control for linear induction motor drive. IEE Proc. Electr. Power Appl. 2002, 149, 184. [Google Scholar] [CrossRef]
- Skjetne, R.; Fossen, T. On integral control in backstepping: Analysis of different techniques. In Proceedings of the 2004 American Control Conference, Boston, MA, USA, 30 June–2 July 2004; Volume 2, pp. 1899–1904. [Google Scholar]
- Xiao, L.; Zhu, Y. Passivity-based integral sliding mode active suspension control. In Proceedings of the 19th World Congress of the International Federation of Automatic Control, Cape Town, South Africa, 24–29 August 2014. [Google Scholar]
- Bartolini, G.; Ferrara, A.; Usai, E. Chattering avoidance by second-order sliding mode control. IEEE Trans. Autom. Control 1998, 43, 241–246. [Google Scholar] [CrossRef]
- Kurode, S.; Spurgeon, S.K.; Bandyopadhyay, B.; Gandhi, P.S. Sliding mode control for slosh-free motion using a nonlinear sliding surface. IEEE/ASME Trans. Mechatron. 2013, 18, 714–724. [Google Scholar] [CrossRef] [Green Version]
- Leung, F.; Wong, L.; Tam, P. Algorithm for eliminating chattering in sliding mode control. Electron. Lett. 1996, 32, 599. [Google Scholar] [CrossRef]
- Kachroo, P. Existence of solutions to a class of nonlinear convergent chattering-free sliding mode control systems. IEEE Trans. Autom. Control 1999, 44, 1620–1624. [Google Scholar] [CrossRef] [Green Version]
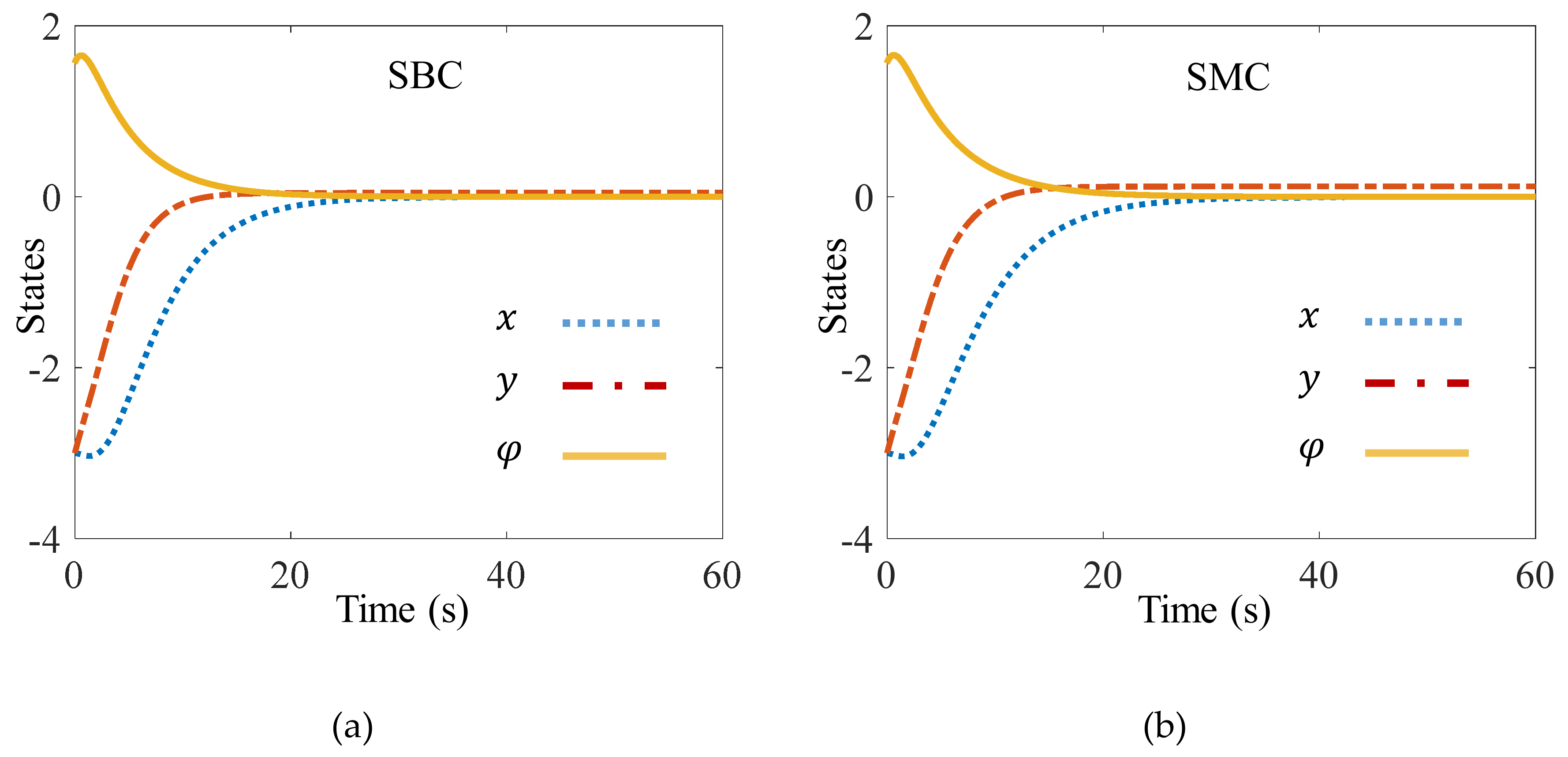
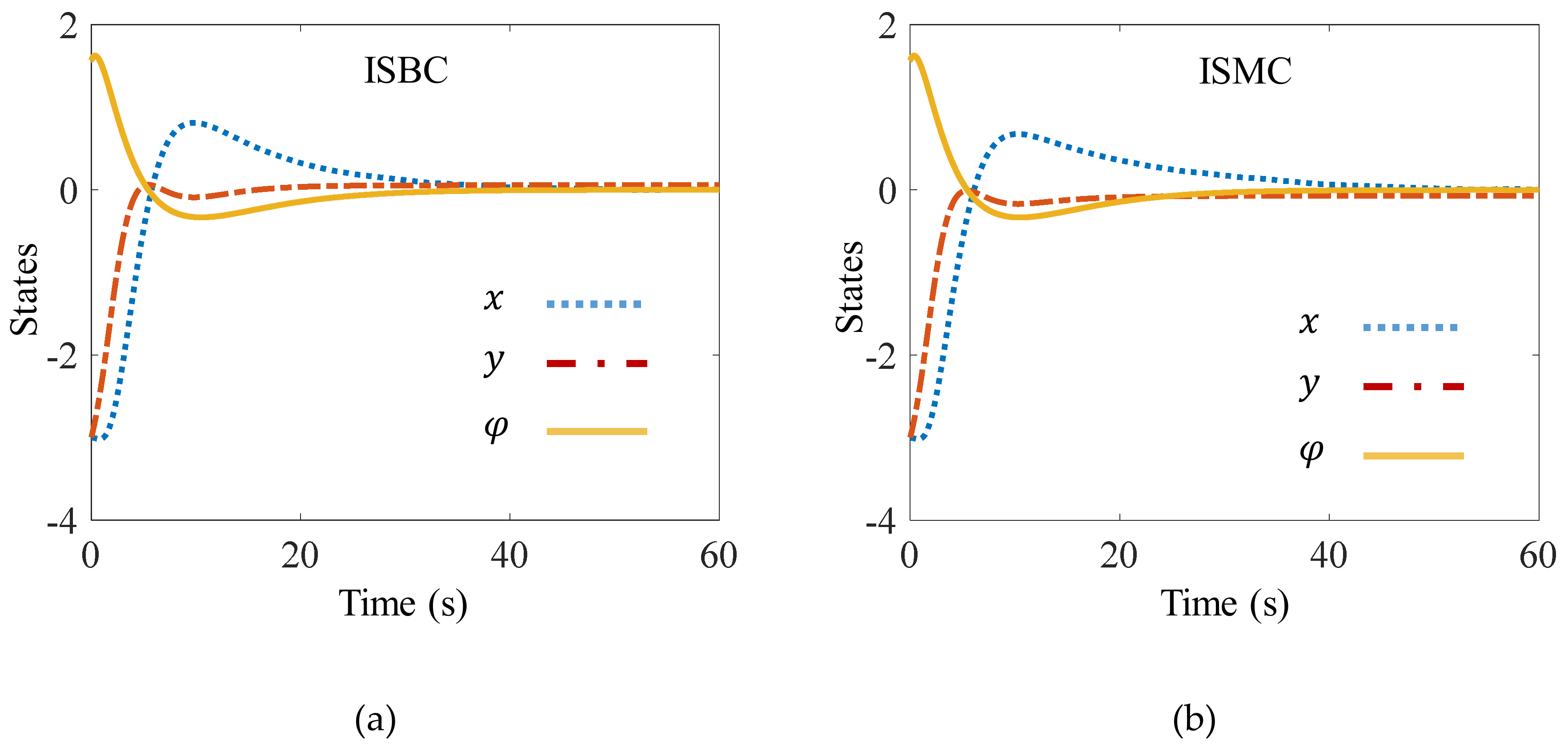
| Rise Time | C0 | C1 | C2 | C3 | C4 | C5 |
|---|---|---|---|---|---|---|
| 6.73 s | 9.61 s | 8.10 s | 7.66 s | 7.96 s | 8.65 s |
| System Response | C0 | C1 | C2 | C3 | C4 | C5 |
|---|---|---|---|---|---|---|
| tr | 6.28 s | 15.14 s | 5.32 s | 3.83 s | 3.52 s | 3.52 s |
| ts | 8.90 s | > 60 s | 30.53 s | 21.29 s | 17.01 s | 18.25 s |
| ts | 8.01 s | 30.21 s | 10.64 s | 7.50 s | 7.15 s | 8.97 s |
| σ% | 3.5% | 28.6% | 37.8% | 49.5% | 54.6% | 40.9% |
| Control Method | Parameters |
|---|---|
| SMC | |
| SBC | |
| ISMC | |
| ISBC |
| Rise Time | SMC | SBC |
|---|---|---|
| 13.01, 7.73, 11.21 (s) | 11.71, 7.18, 10.25 (s) |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, H.; Iorio, J.; Li, N. Sliding Mode Control in Backstepping Framework for a Class of Nonlinear Systems. J. Mar. Sci. Eng. 2019, 7, 452. https://doi.org/10.3390/jmse7120452
Shen H, Iorio J, Li N. Sliding Mode Control in Backstepping Framework for a Class of Nonlinear Systems. Journal of Marine Science and Engineering. 2019; 7(12):452. https://doi.org/10.3390/jmse7120452
Chicago/Turabian StyleShen, He, Joseph Iorio, and Ni Li. 2019. "Sliding Mode Control in Backstepping Framework for a Class of Nonlinear Systems" Journal of Marine Science and Engineering 7, no. 12: 452. https://doi.org/10.3390/jmse7120452
APA StyleShen, H., Iorio, J., & Li, N. (2019). Sliding Mode Control in Backstepping Framework for a Class of Nonlinear Systems. Journal of Marine Science and Engineering, 7(12), 452. https://doi.org/10.3390/jmse7120452





