Ocean Energy Systems Wave Energy Modelling Task: Modelling, Verification and Validation of Wave Energy Converters
Abstract
1. Introduction
1.1. The Background of the OES Wave Energy Conversion Modelling Verification and Validation Task
1.2. Paper Contribution
2. Description of the Numerical Tools
2.1. Linear Models
2.2. Weakly Nonlinear Models
2.3. Fully Nonlinear Models
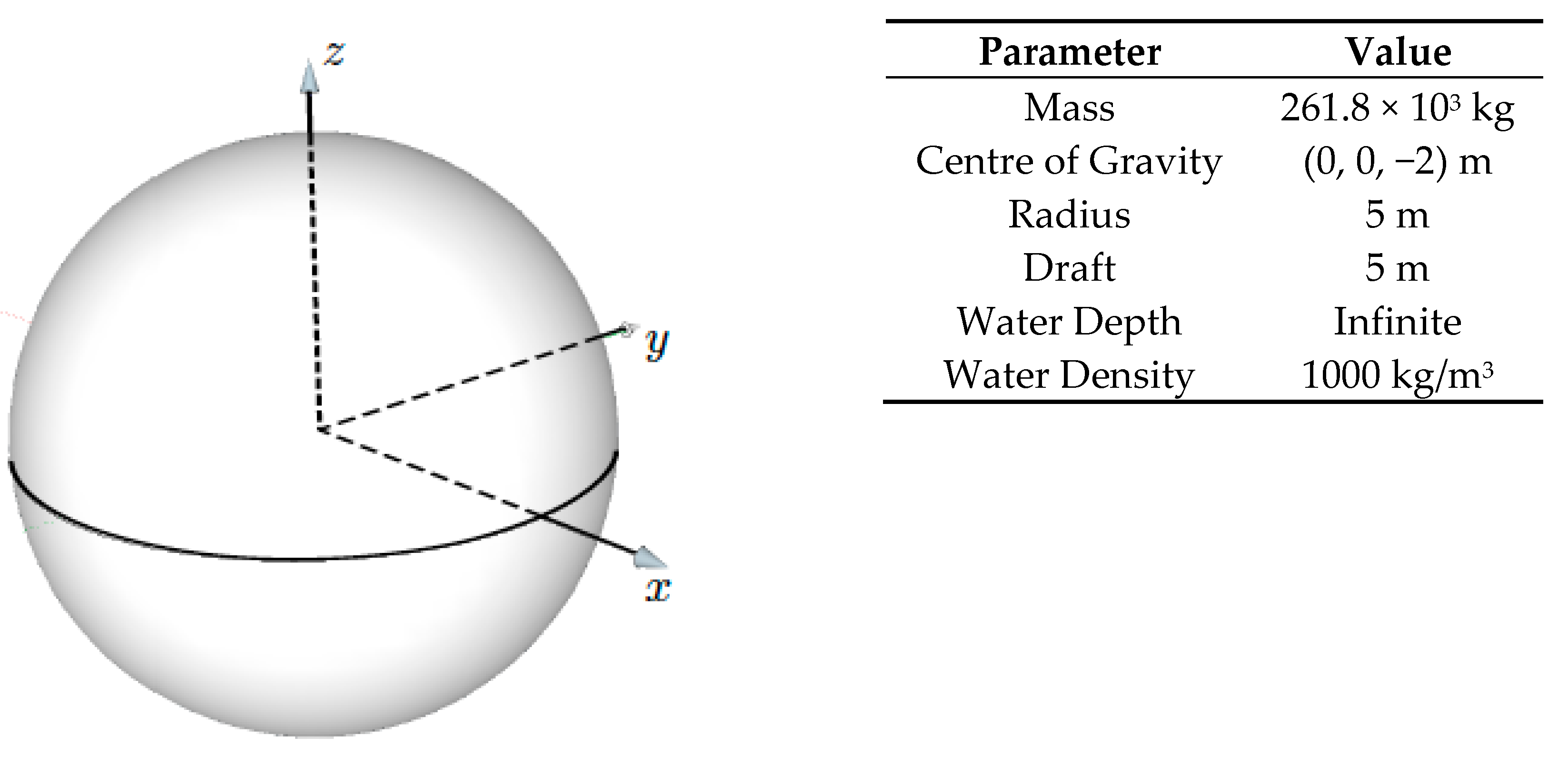
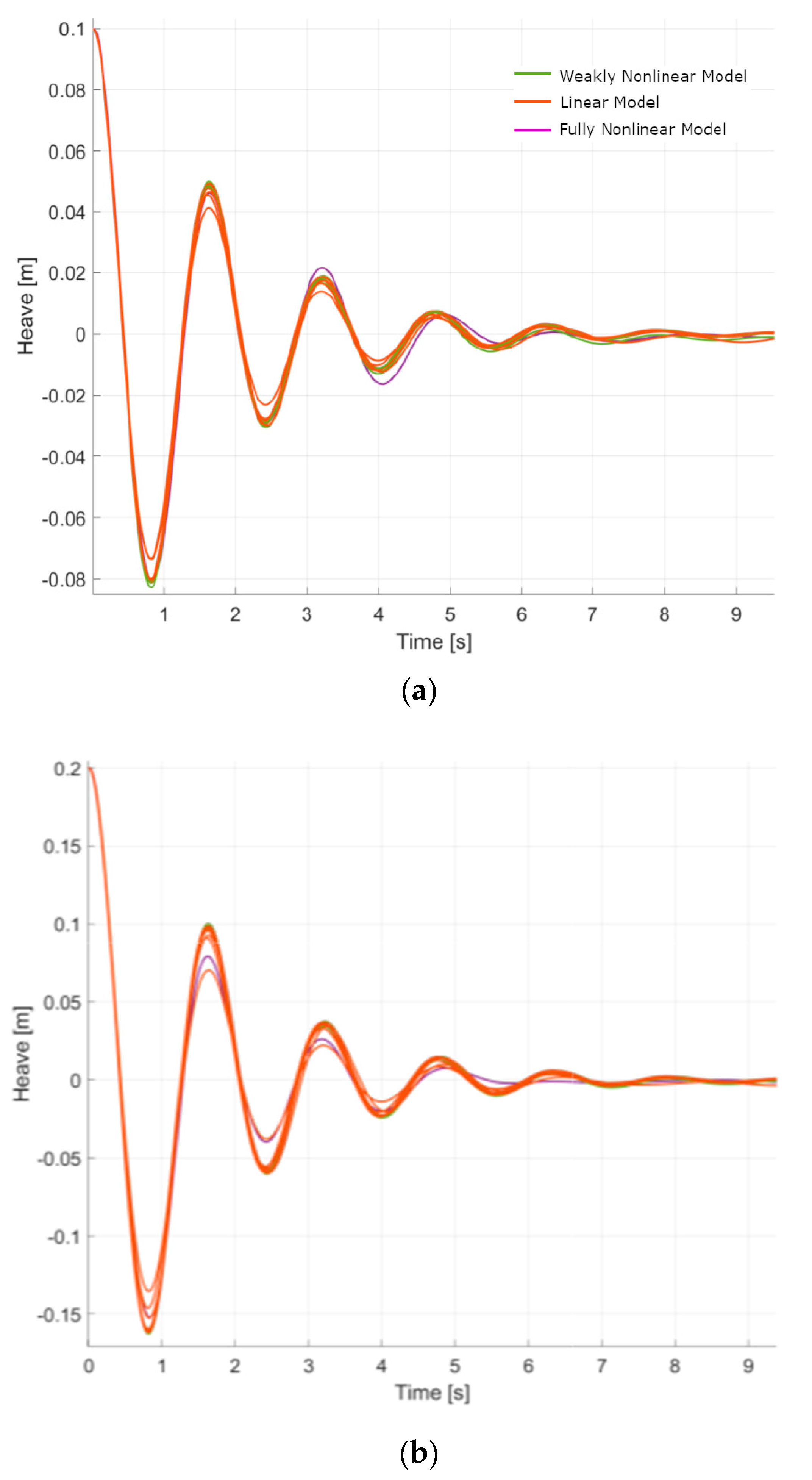
3. Code-to-Code Comparison of a Heaving Semi-Submerged Sphere
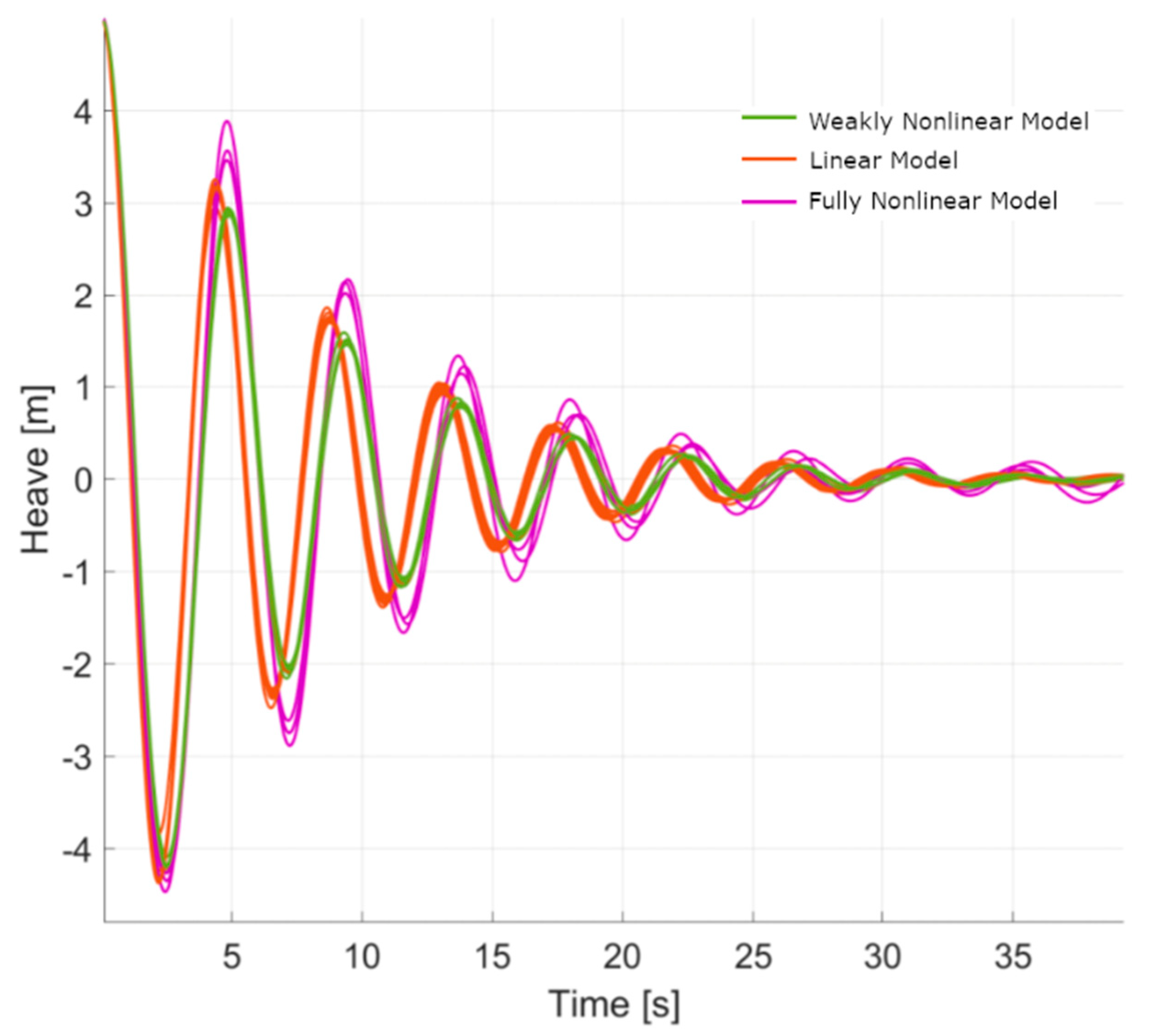
3.1. Decay Tests
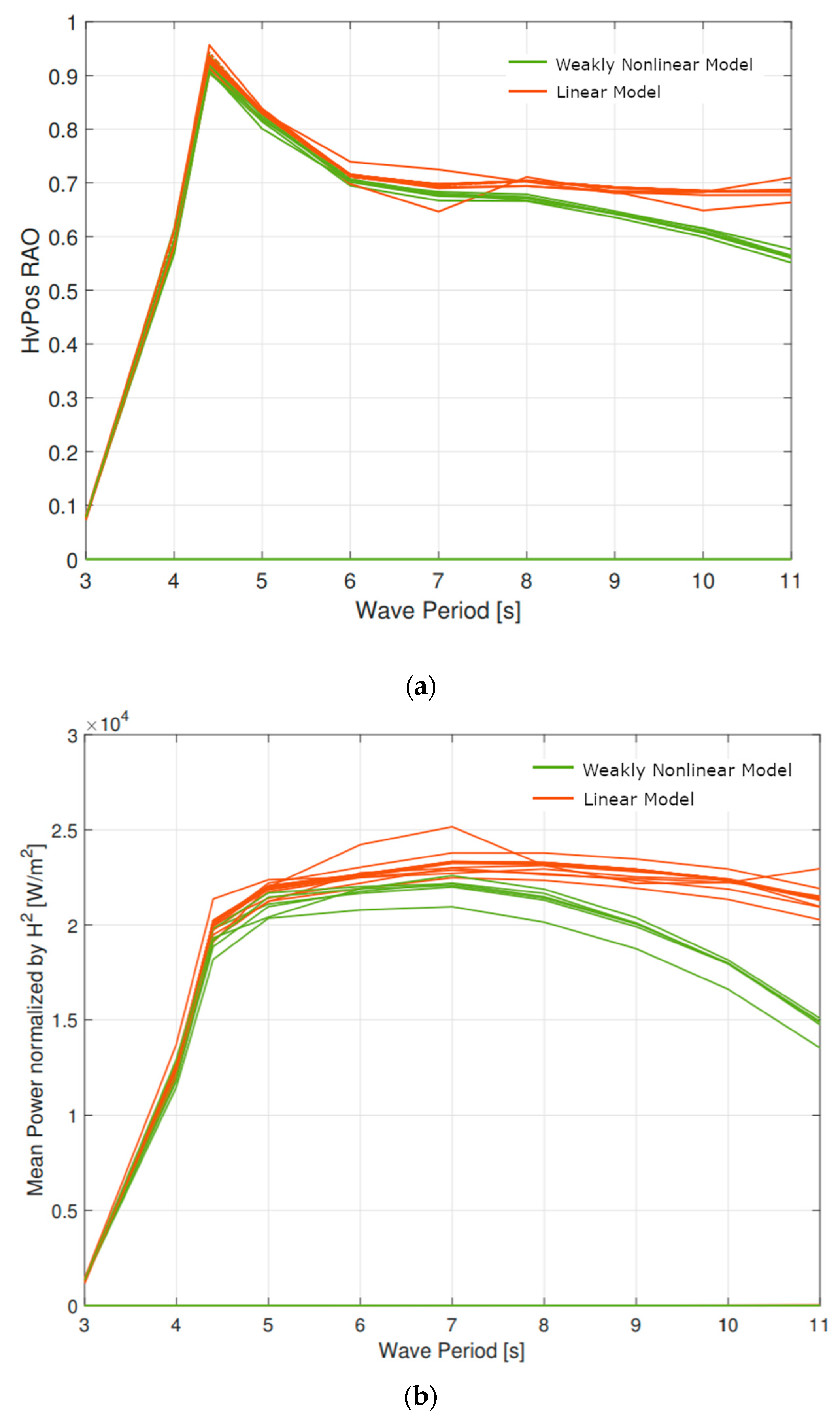
3.2. Regular Waves
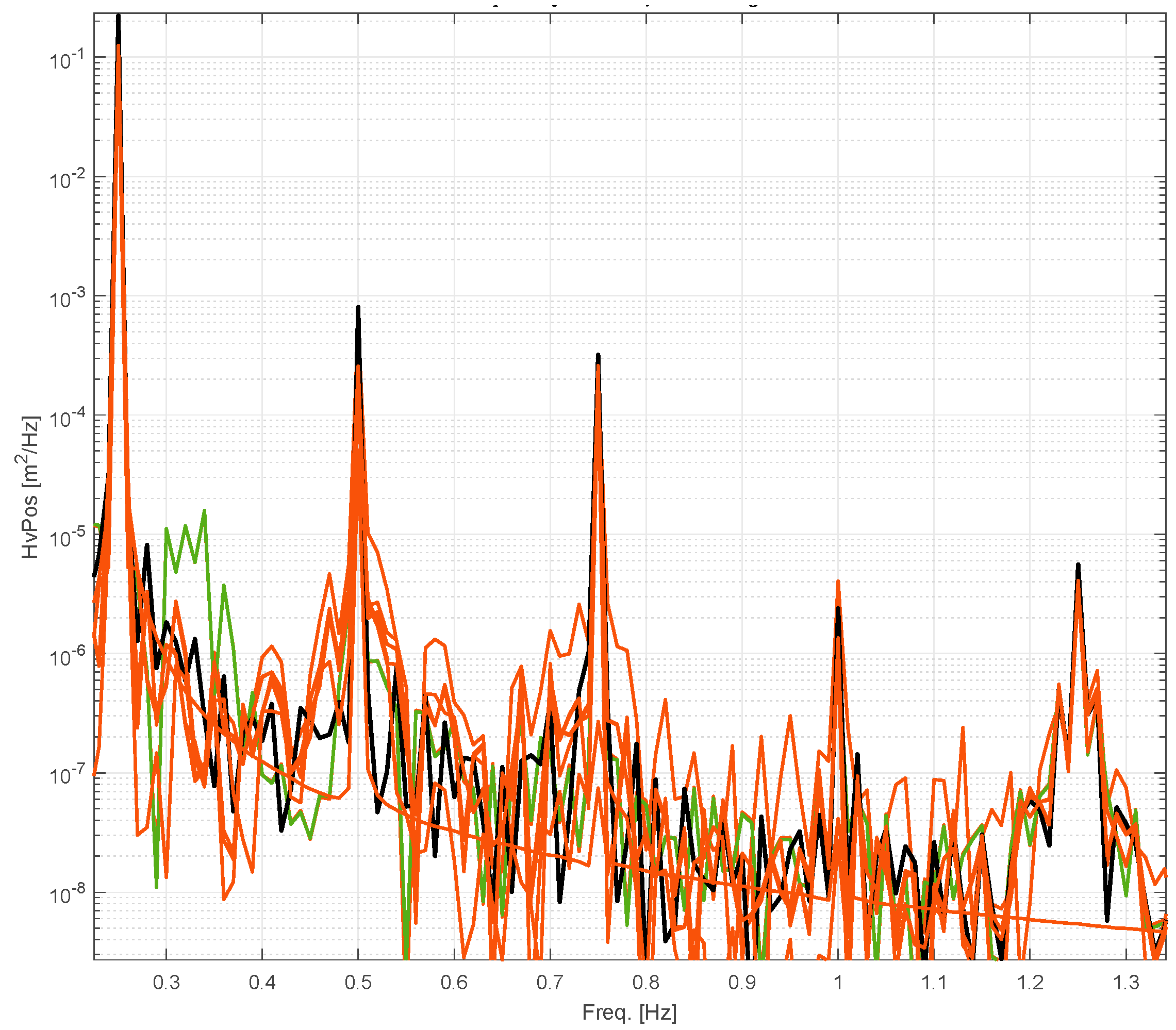
3.3. Irregular Waves
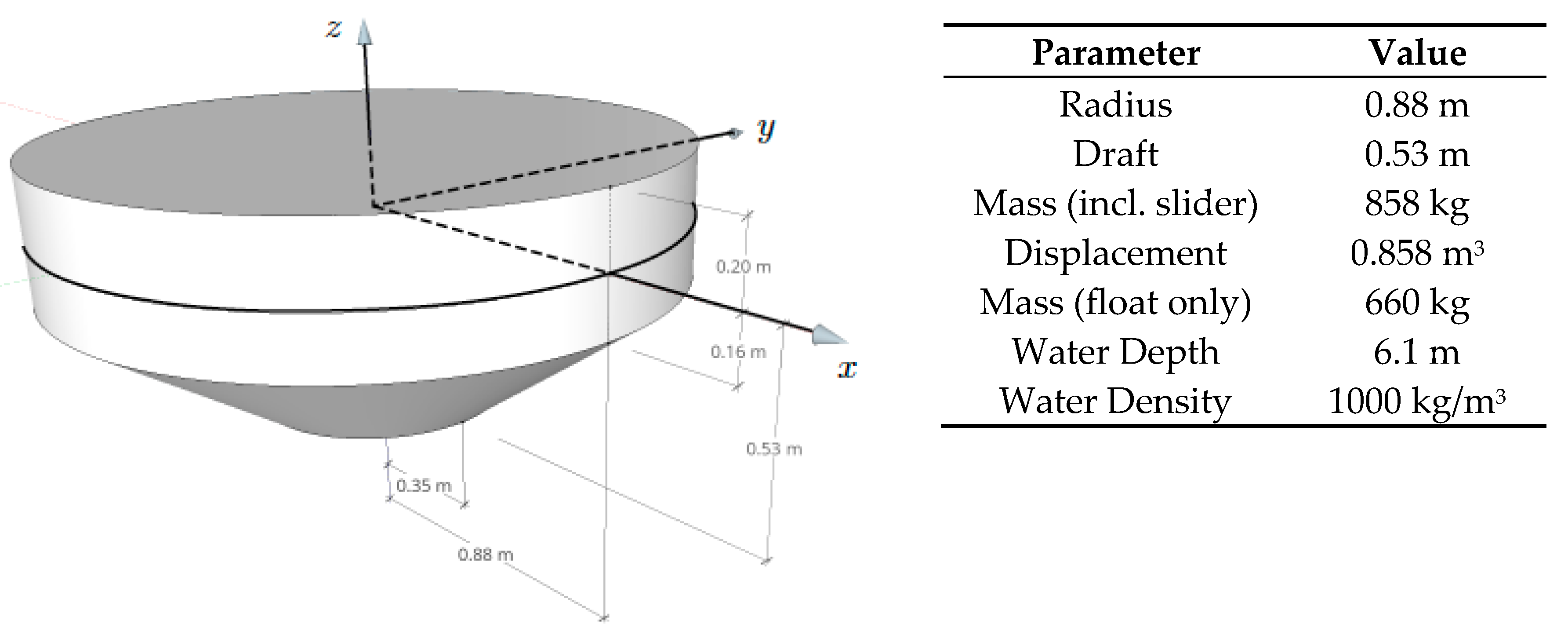
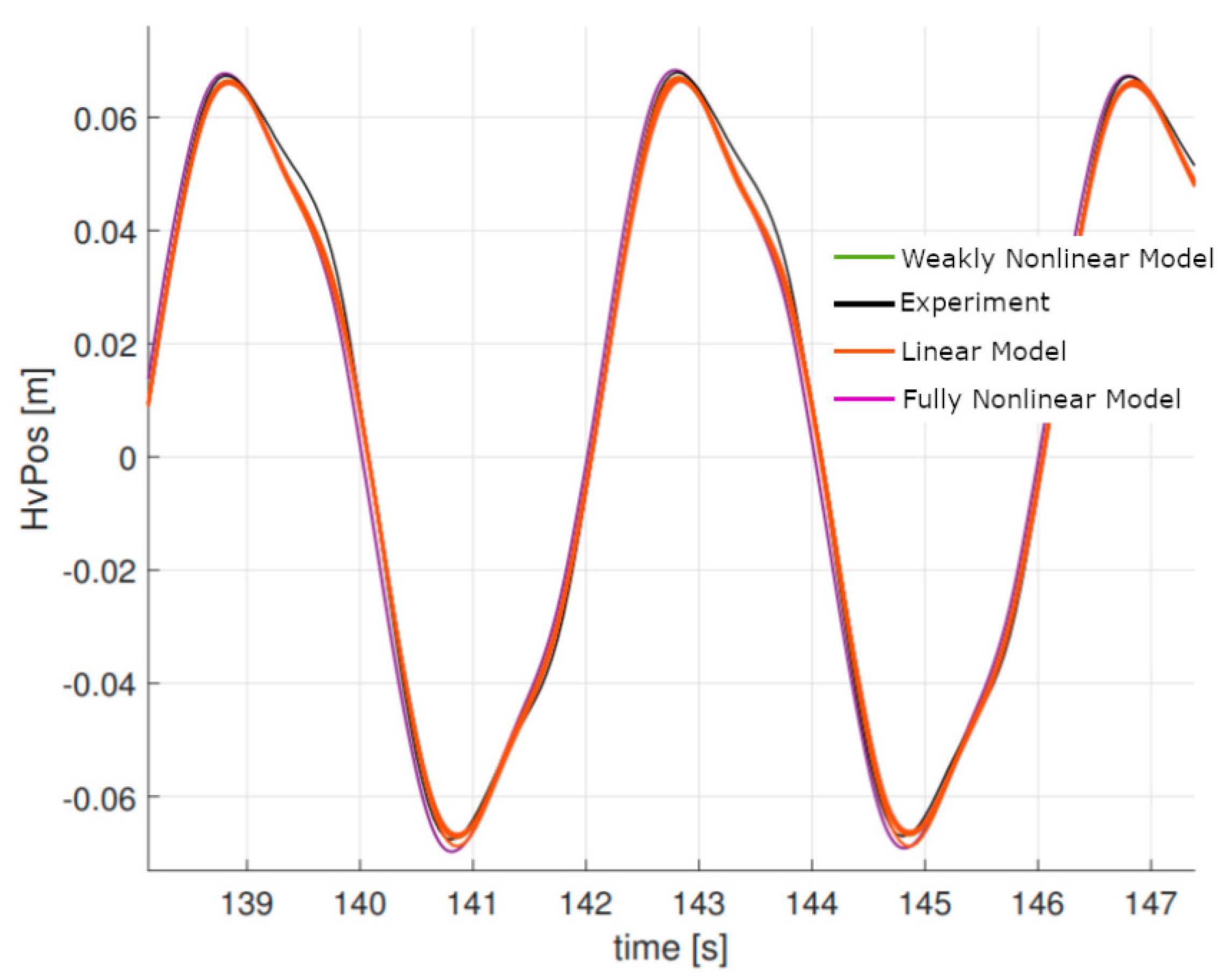
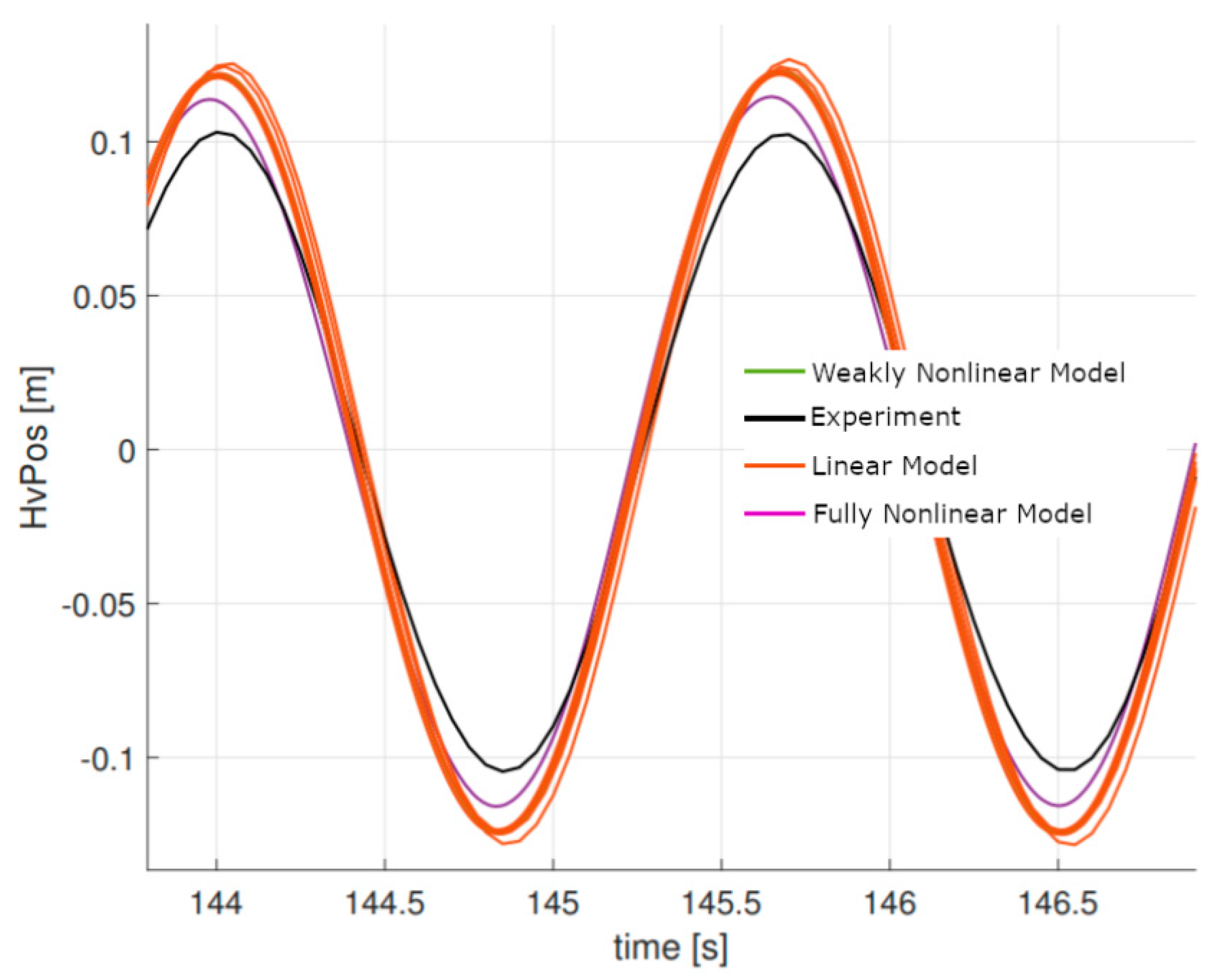
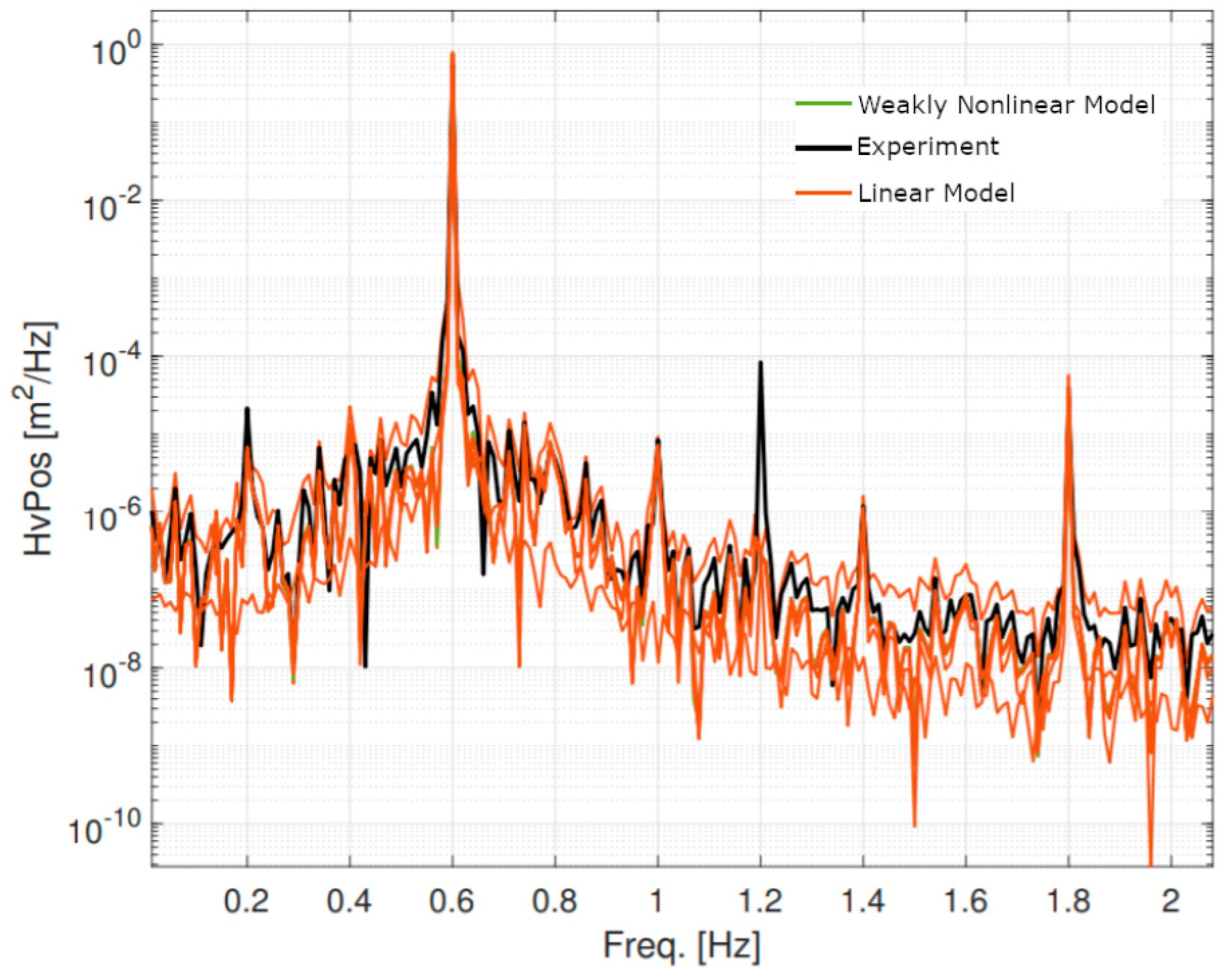
4. Validation Using Existing Experimental Data of a Heaving Float
4.1. Decay Tests
4.2. Radiation Tests
4.3. Diffraction Tests
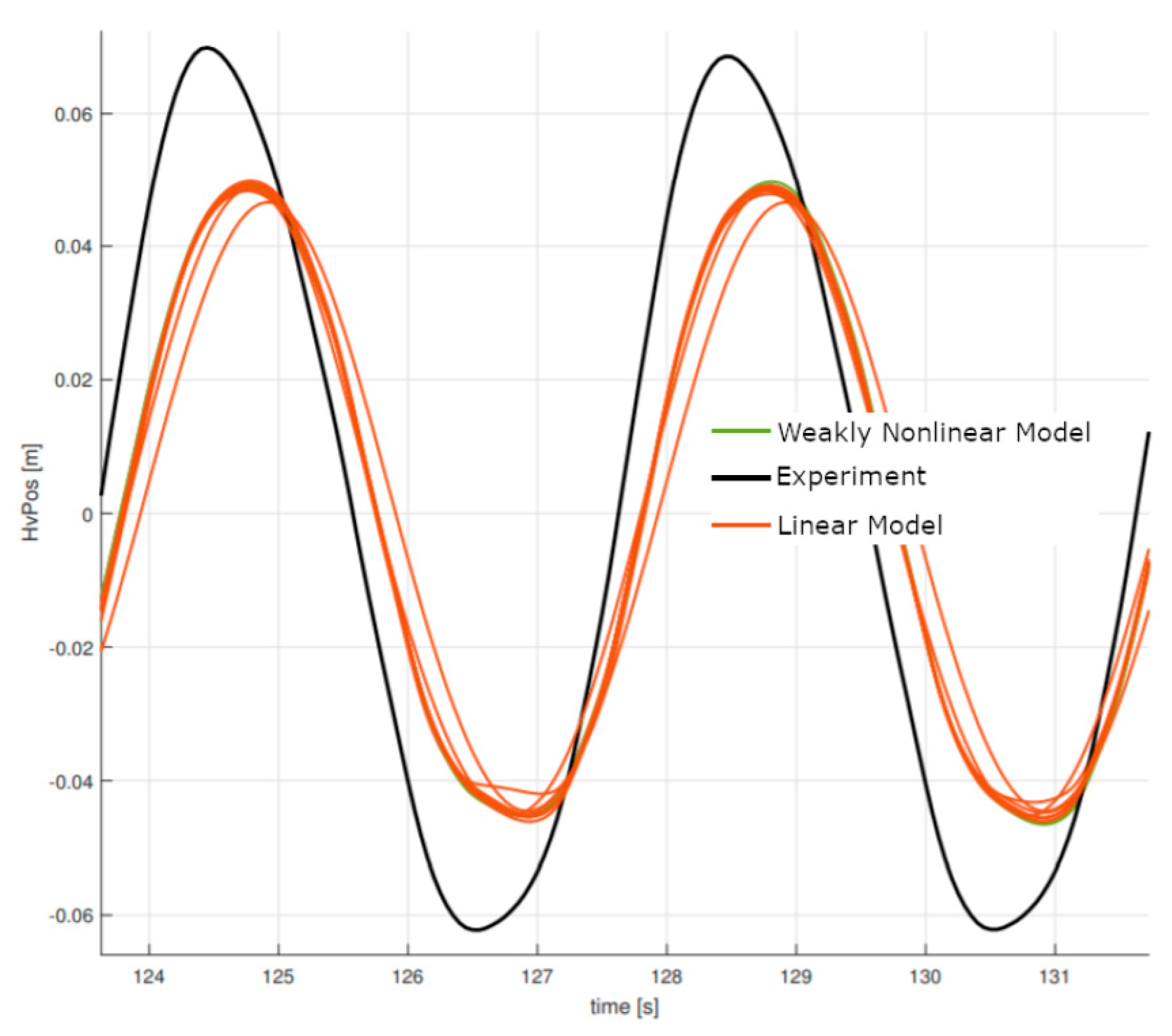
4.4. Regular Waves
5. Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| BEM | boundary element method |
| CFD | computational fluid dynamics |
| DFS | direct finite element simulation |
| FEM | finite element method |
| FK | Froude–Krylov |
| FNPF | fully nonlinear potential flow |
| FVM | finite volume method |
| IEA | International Energy Agency |
| iLES | implicit large eddy simulation |
| IRF | impulse response function |
| LCOE | levelized cost of energy |
| LS | level set |
| OES | Ocean Energy Systems |
| PSD | power spectral density |
| PTO | power take off |
| RAO | response amplitude operator |
| RK4 | 4th-order Runge–Kutta |
| SS | state space |
| SWL | still water level |
| URANS | unsteady Reynolds-averaged Navier–Stokes |
| VOF | volume of fluid |
| WE | wave elevation |
| WEC | wave energy converter |
| WEMT | Wave Energy Modelling Task |
| WS | wave spectrum |
References
- Weber, J. WEC technology readiness and performance matrix-finding the best research technology development trajectory. In Proceedings of the 4th International Conference on Ocean Energy, Dublin, Ireland, 17–19 October 2012. [Google Scholar]
- Cummins, W. The impulse response function and ship motions. Schiffstechnik 1962, 9, 101–109. [Google Scholar]
- OrcaFlex. Available online: https://www.orcina.com/SoftwareProducts/OrcaFlex/ (accessed on 2 May 2019).
- Deep Water Coupled Floater Motion Analysis—DeepC. Available online: https://www.dnvgl.com/services/deep-water-coupled-floater-motion-analysis-deepc-89424 (accessed on 2 May 2019).
- ANSYS Aqwa. Available online: https://www.ansys.com/products/structures/ansys-aqwa (accessed on 2 May 2019).
- Yu, Y.; Lawson, M.; Ruehl, K.; Michelen, C. Development and Demonstration of the WEC-Sim Wave Energy Converter Simulation Tool. In Proceedings of the 2nd Marine Energy Technology Symposium, Washington, DC, USA, 15–17 April 2014. [Google Scholar]
- Combourieu, A.; Philippe, M.; Rongère, F.; Babarit, A. InWave: A new flexible design tool dedicated to wave energy converters. In Proceedings of the 33rd International Conference on Ocean, Offshore and Arctic Engineering, San Francisco, CA, USA, 8–13 June 2014. [Google Scholar]
- Giorgi, G.; Ringwood, J.V. Nonlinear Froude-Krylov and viscous drag representations for wave energy converters in the computation/fidelity continuum. Ocean Eng. 2017, 141, 164–175. [Google Scholar] [CrossRef]
- Fitzgerald, C. Nonlinear Potential Flow Models. In Numerical Modelling of Wave Energy Converters: State-of-the Art Techniques for Single WEC and Converter Arrays; Folley, M., Ed.; Academic Press: Cambridge, MA, USA, 2016; pp. 83–104. [Google Scholar]
- Windt, C.; Davidson, J.; Ringwood, J.V. High-fidelity numerical modelling of ocean wave energy systems: A review of computational fluid dynamics-based numerical wave tanks. Renew. Sustain. Energy Rev. 2018, 93, 610–630. [Google Scholar] [CrossRef]
- Gilloteaux, J.C.; Ducrozet, G.; Babarit, A.; Clément, A. Non-linear model to simulate large amplitude motions: Application to wave energy conversion. In Proceedings of the 22nd International Workshop on Water Waves and Floating Bodies, Plitvice Lakes, Croatia, 15–18 April 2007. [Google Scholar]
- Penalba, M.; Ringwood, J. A review of wave-to-wire models for wave energy converters. Energies 2016, 9, 506. [Google Scholar] [CrossRef]
- Hall, M.; Buckham, B.; Crawford, C. Evaluating the importance of mooring line model fidelity in floating offshore wind turbine simulations. Wind Energy 2014, 17, 1835–1853. [Google Scholar] [CrossRef]
- Jonkman, J.; Musial, W. Offshore Code Comparison Collaboration (OC3) for IEA Wind Task 23 Offshore Wind Technology and Deployment; Technical Report; National Renewable Energy Lab. (NREL): Golden, CO, USA, 2010.
- Popko, W.; Vorpahl, F.; Zuga, A.; Kohlmeier, M.; Jonkman, J.; Robertson, A.; Larsen, T.J.; Yde, A.; Sætertrø, K.; Okstad, K.M.; et al. Offshore Code Comparison Collaboration Continuation (OC4), Phase 1-Results of Coupled Simulations of an Offshore Wind Turbine with Jacket Support Structure. In Proceedings of the Twenty-Second International Offshore and Polar Engineering Conference. International Society of Offshore and Polar Engineers, Rhodes, Greece, 17–22 June 2012. [Google Scholar]
- Robertson, A.N.; Wendt, F.F.; Jonkman, J.M.; Popko, W.; Vorpahl, F.; Stansberg, C.T.; Bachynski, E.E.; Bayati, I.; Beyer, F.; de Vaal, J.B.; et al. OC5 project phase I: Validation of hydrodynamic loading on a fixed cylinder. In Proceedings of the Twenty-Fifth International Ocean and Polar Engineering Conference. International Society of Offshore and Polar Engineers, Kona, HI, USA, 21–26 June 2015. [Google Scholar]
- Combourieu, A.; Lawson, M.; Babarit, A.; Ruehl, K.; Roy, A.; Costello, R.; Weywada, P.L.; Bailey, H. WEC3: Wave energy converter code comparison project. In Proceedings of the 11th European Wave and Tidal Energy Conference, Nantes, France, 6–11 September 2015. [Google Scholar]
- Qian, L.; Mingham, C.; Causon, D.; Ingram, D.; Folley, M.; Whittaker, T. Numerical simulation of wave power devices using a two-fluid free surface solver. Mod. Phys. Lett. B 2005, 19, 1479–1482. [Google Scholar] [CrossRef]
- Agamloh, E.B.; Wallace, A.K.; von Jouanne, A. Application of fluid-structure interaction simulation of an ocean wave energy extraction device. Renew. Energy 2008, 33, 748–757. [Google Scholar] [CrossRef]
- Nam, B.W.; Shin, S.H.; Hong, K.Y.; Hong, S.W. Numerical simulation of wave flow over the spiral reef overtopping device. In Proceedings of the 8th ISOPE Pacific/Asia Offshore Mechanics Symposium, Jeju, Korea, 14–17 October 2008. [Google Scholar]
- Yu, Y.H.; Li, Y. Reynolds-averaged Navier–Stokes simulation of the heave performance of a two-body floating-point absorber wave energy system. Comput. Fluids 2013, 73, 104–114. [Google Scholar] [CrossRef]
- Ransley, E.; Yan, S.; Brown, S.; Mai, T.; Graham, D.; Ma, Q.; Musiedlak, P.; Engsig-Karup, A.; Eskilsson, C.; Li, Q.; et al. A blind comparative study of focused wave interactions with a fixed FPSO-like structure (CCP-WSI Blind Test Series 1). Int. J. Offshore Polar Eng. 2019, 29, 113–127. [Google Scholar] [CrossRef]
- Wendt, F.; Yu, Y.H.; Nielsen, K.; Ruehl, K.; Bunnik, T.; Touzon, I.; Nam, B.; Kim, J.; Kim, K.H.; Janson, C.; et al. International Energy Agency Ocean Energy Systems Task 10 Wave Energy Converter Modeling Verification and Validation. In Proceedings of the 12th European Wave and Tidal Energy Conference, Cork, Ireland, 27 August–1 September 2017. [Google Scholar]
- Nielsen, K.; Wendt, F.; Yu, Y.; Ruehl, K.; Touzon, I.; Nam, B.; Kim, J.; Kim, K.; Crowley, S.; Sheng, W.; et al. OES Task 10 WEC heaving sphere performance modelling verification. In Proceedings of the 3rd International Conference on Renewable Energies Offshore (RENEW 2018), Lisbon, Portugal, 8–10 October 2018. [Google Scholar]
- WAMIT. User Manual, Version 7.2; WAMIT, Inc.: Chestnut Hill, MA, USA, 2016. [Google Scholar]
- Babarit, A.; Delhommeau, G. Theoretical and numerical aspects of the open source BEM solver NEMOH. In Proceedings of the 11th European Wave and Tidal Energy Conference (EWTEC 2015), Nantes, France, 6–11 September 2015. [Google Scholar]
- Falnes, J. Ocean Waves and Oscillating Systems; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Nielsen, K.; Pontes, T. Annex II Task 1.1 Generic and Site-Related Wave Energy Data; Technical Report T02-1.1; IEA: Paris, France, 2010. [Google Scholar]
- Coe, R.G.; Bacelli, G.; Patterson, D.; Wilson, D.G. Advanced WEC Dynamics & Controls, FY16 Testing Report; Technical Report SAND2016-10094; Sandia National Laboratories: Albuquerque, NM, USA, 2016.
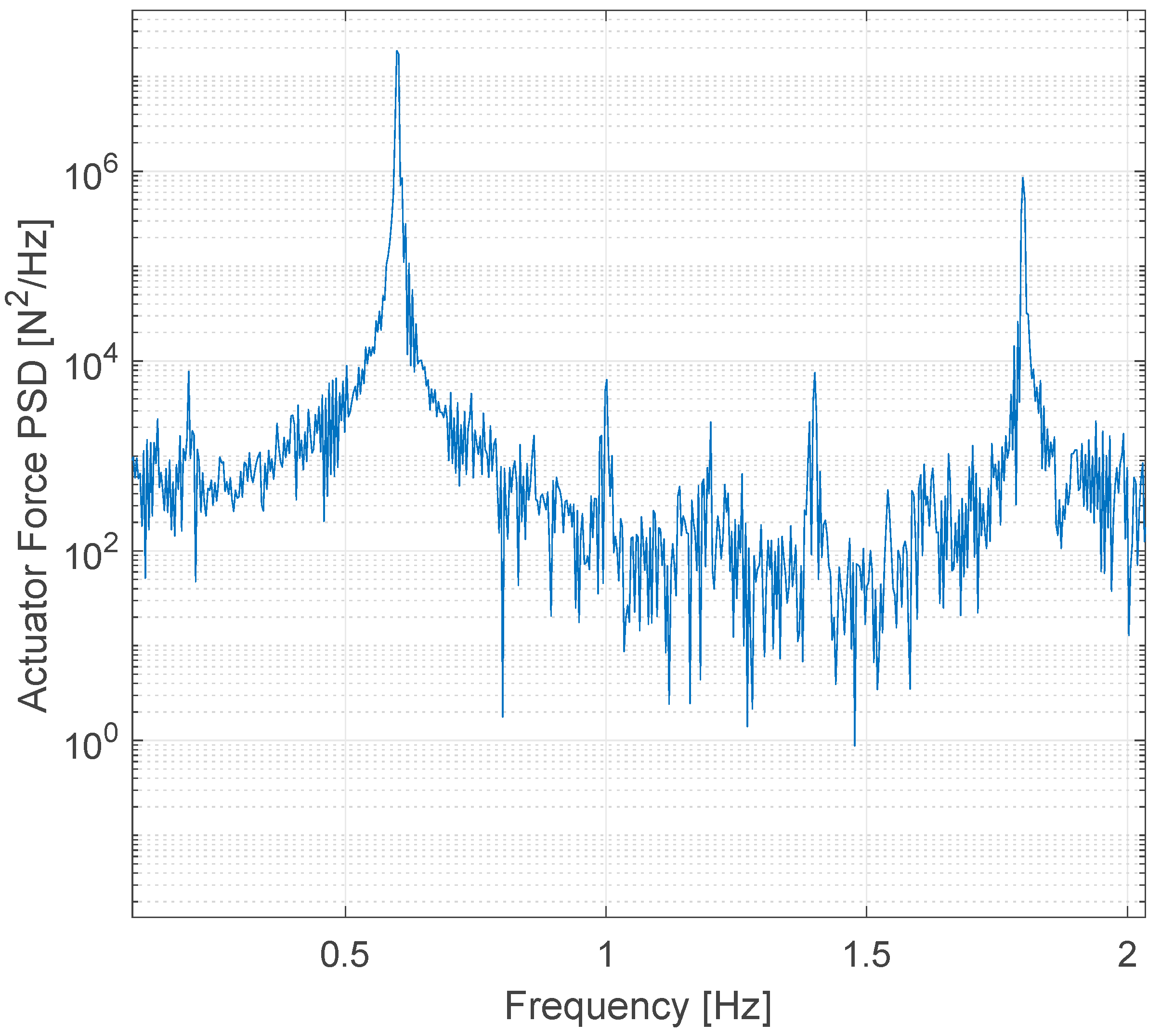
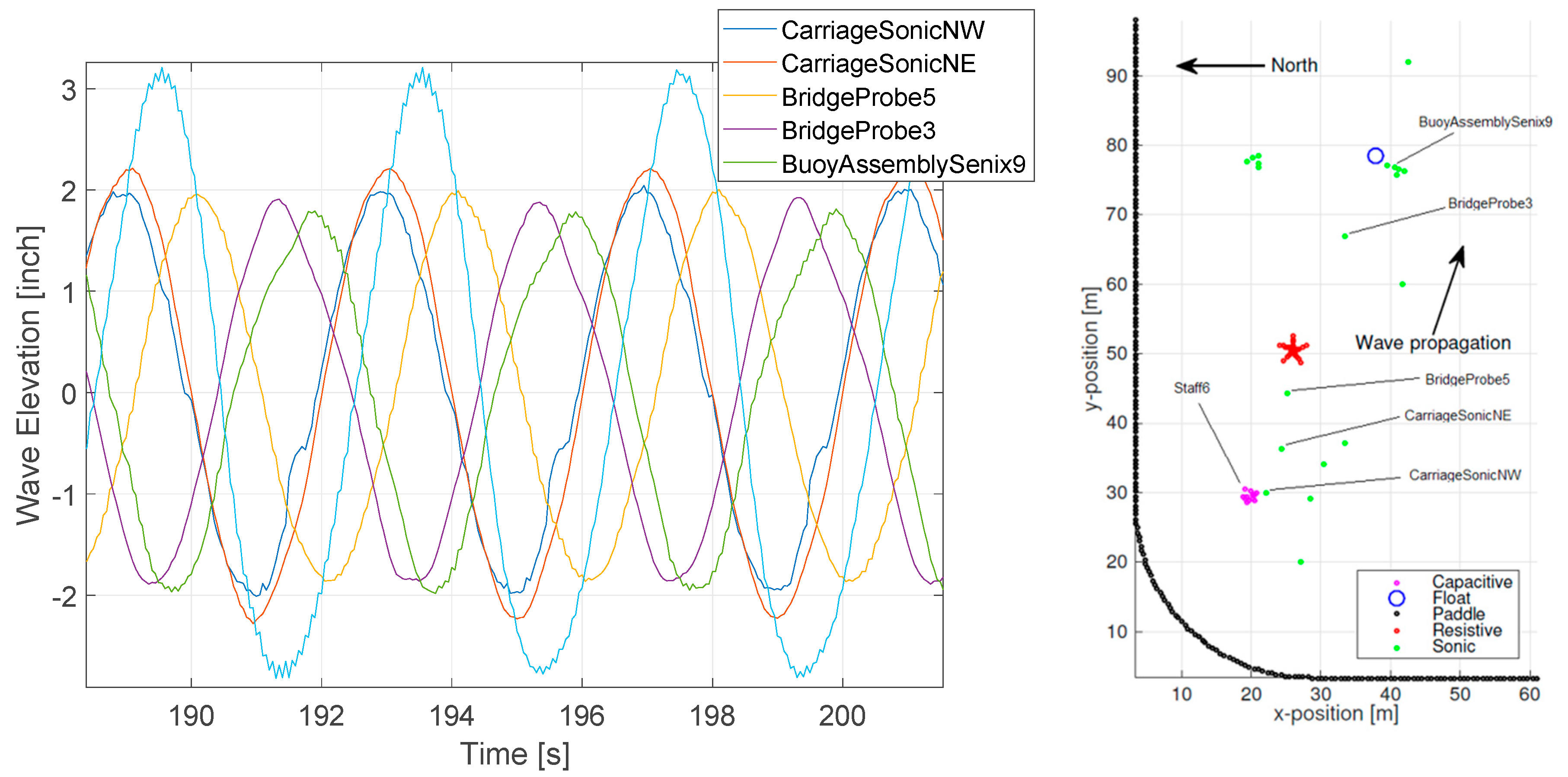
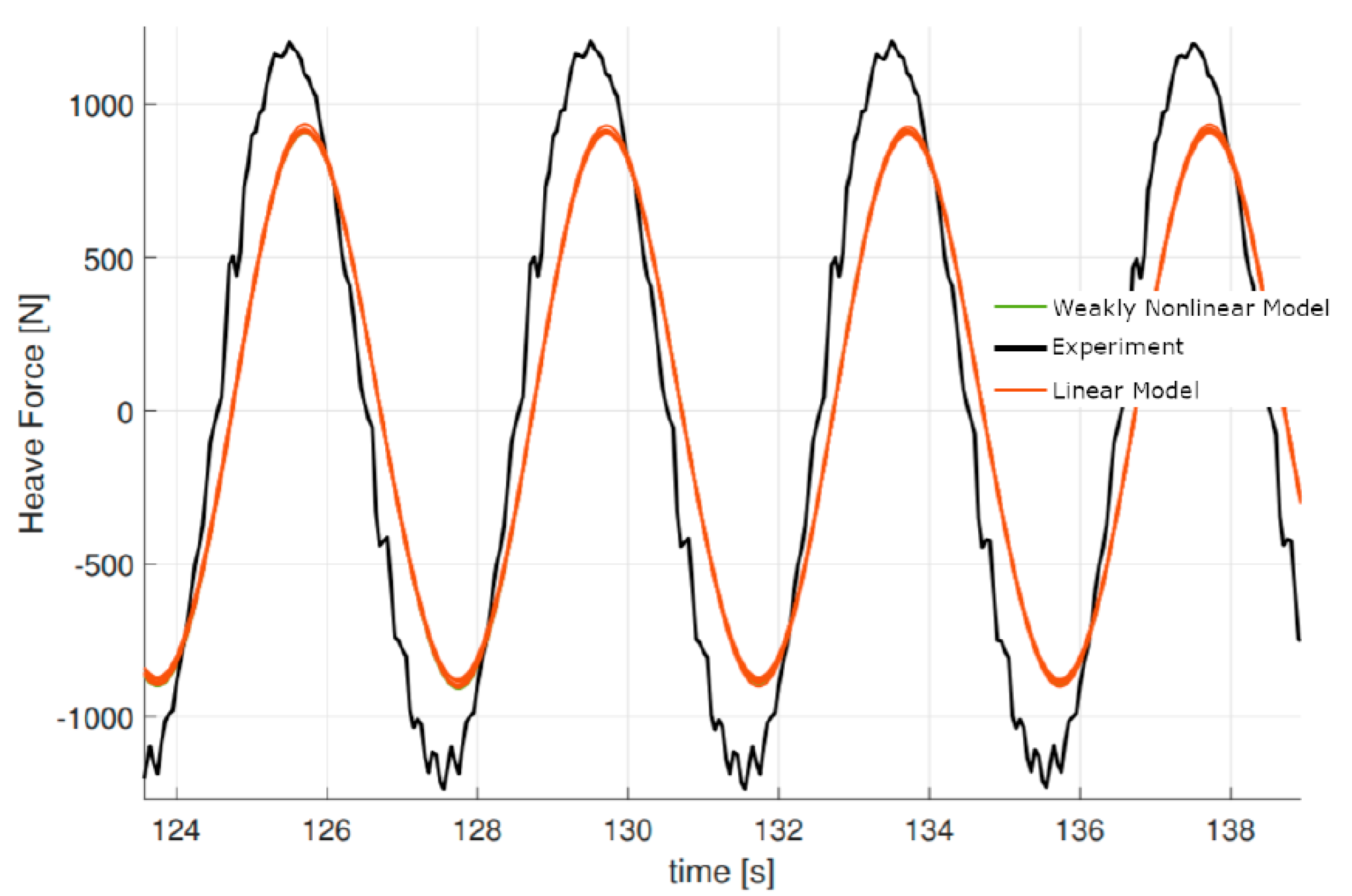
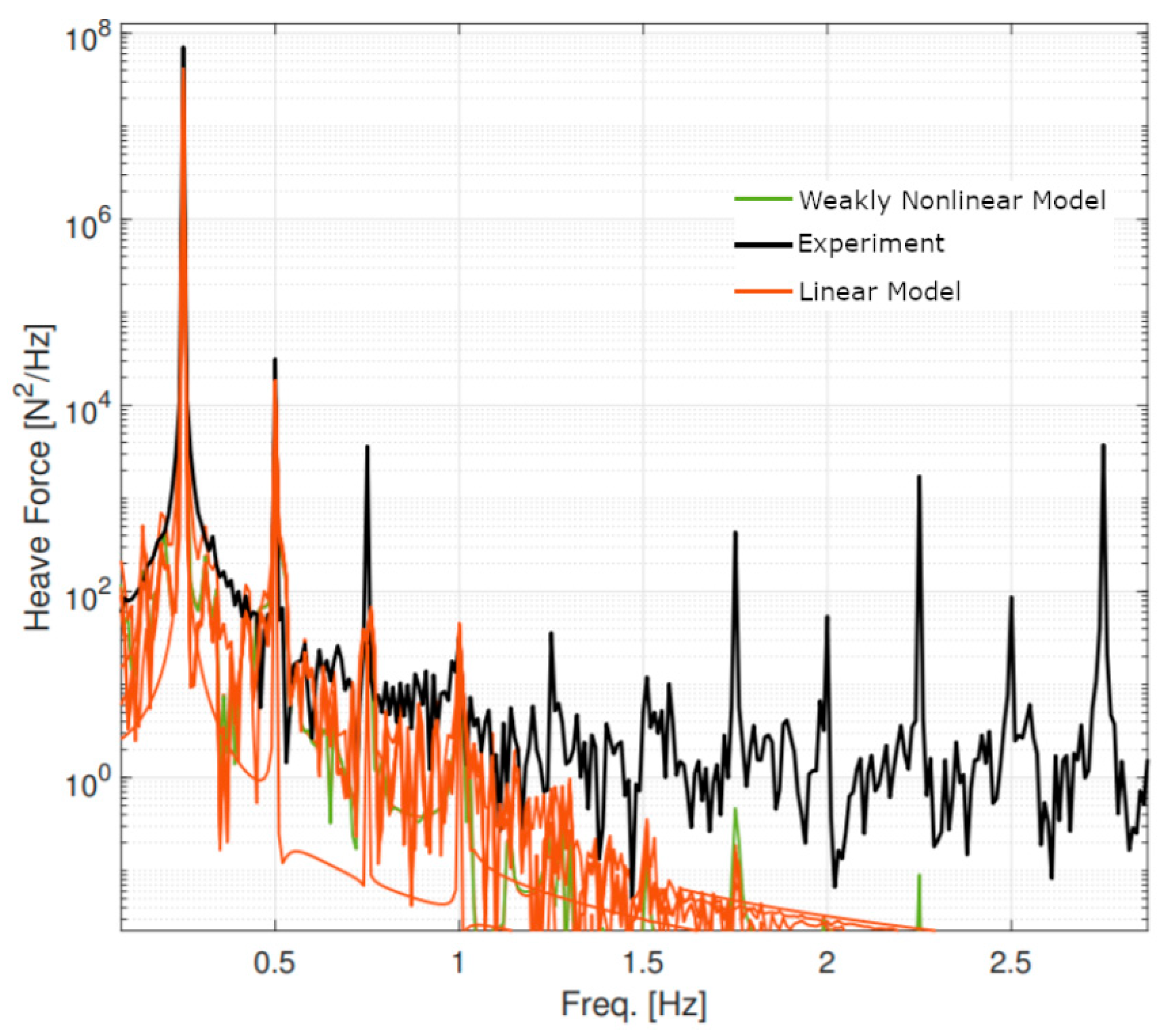
| Participant | Model Name | Frad | Fexc | BEM | Time Integration | Comments |
|---|---|---|---|---|---|---|
| AAU | - | IRF | WE | WAMIT | RK4-0.01 s (fixed) | IRFs are precalculated, with a 0.01 rad/s frequency resolution, and only values for t < 20 s are used |
| DSA | ProteusDS | IRF | WS | NEMOH | RK4-0.01 s (fixed) | - |
| DTU | DTUMotion-Simulator | IRF | WS | WAMIT | RK4-0.01 s (fixed) | Radiation IRFs are computed from the Fast Cosine Transform of the damping coefficients, then interpolated to the incident wave time-step size. |
| ECN | - | SS | WE | NEMOH | RK4 (adaptive) | - |
| EDRMedeso | ANSYS-Aqwa | IRF | WE | ANSYS-Aqwa | Semi-implicit predictor–corrector scheme, dt = 0.01 s | The term ANSYS Aqwa covers both model name and BEM. |
| FPP | FPP-Lin | SS | WE | WAMIT | RK4 (adaptive) | Radiation transfer functions are fitted in the frequency domain to the WAMIT data using the Matlab function invfreq. |
| Glosten | Python Time-Domain Simulator | IRF | WE | WAMIT | RK4-0.01 s (fixed) | - |
| HNEI | WEC-Sim | IRF | WS | WAMIT | RK4-0.01 s (fixed) | - |
| INNOSEA | InWave | IRF | WS | Adams–Moulton adaptive time-step solver, dt = 0.01 s | - | |
| KRISO | KIMAPS | IRF | AdFLOW | RK4-0.01 s (fixed) | - | |
| MARIN | aNySIM | IRF | DIFFRAC | RK4-0.01 s (fixed) | The excitation force was calculated from DIFFRAC wave force RAOs multiplied with wave amplitudes and phase shifted with wave phase for irregular waves. | |
| Navatek | Aegir | - | WE | RK4-0.01 s (fixed) | The radiation force was calculated from a purely time-domain approach using a high-order BEM. | |
| NREL and Sandia | WEC-Sim | IRF | WS | WAMIT | RK4-0.01 s (fixed) | IRF values for t < 60 s are used |
| SAGA | WEC-Sim | IRF | WE | NEMOH | RK4-0.01 s (fixed) | IRF values for t < 19 s are used |
| Tecnalia | - | IRF | WS | WAMIT | RK4-0.01 s (fixed) | - |
| UCC | SIM-UCC | IRF | WS | WAMIT | RK4-0.01 s (fixed) | Includes nonlinear restoring forces. |
| WavEC | WavEC2Wire | SS | WE | WAMIT | RK4 (adaptive) | - |
| Wave Venture | WV Daemon | IRF | WS | WAMIT | 0.01 s | - |
| Participant | Wave Theory | Nonlinear Force Calculation |
|---|---|---|
| COER | Airy + Wheeler | Linear radiation and diffraction + algebraic (mesh-less and computationally efficient) nonlinear FK, computed with respect to the instantaneous wetted surface |
| DSA | Airy + Wheeler | Standard |
| DTU | Airy + Wheeler | Standard |
| ECN | Fenton–Rienecker | BEM with linear triangular elements + fluid structure interaction computed through acceleration potential |
| ERMedeso | Airy + Wheeler | Standard |
| HNEI | Airy + Wheeler | Standard |
| INNOSEA | Standard | |
| MARIN | Airy + Wheeler | Standard |
| Navatek | Airy + Wheeler | Standard |
| NREL and Sandia | Airy + Wheeler | Standard (with 1000 triangular panels) |
| WavEC | Airy + Wheeler | Standard |
| Wave Venture | Airy + Wheeler | Standard |
| Participant | Wave Theory | Model Type | Turbulence Mmodel | Time Step | Numbers of Elements |
|---|---|---|---|---|---|
| CTH | SHIPFLOW-Motions | FNPF/BEM | inviscid | 0.01 s | 1600 on body, 2000 on free surface |
| EDRMedeso | ANSYS Fluent | URANS/FVM/VOF | laminar | dt = 0.02 s (adaptive) | 8.6 × 106 nodes, symmetry condition |
| KTH-BCAM | Unicorn-FEniCS-HPC | DFES/FEM/LS | iLES | 0.8 × 106 cells | |
| NREL and SNL | StarCCM + | URANS/FVM/VOF | k-ω SST | 0.01–0.015 s | - |
| RISE | OpenFOAM-v1712 | URANS/FVM/VOF | k-ω SST | CFL = 0.5 | ~1 × 106 cells |
| SSPA | LEMMA-ANANAS | URANS/FVM/LS | Spalart-Allmaras | - | 16 × 106 cells, symmetry condition |
| UoP | OpenFOAM-4.1 | URANS/FVM/VOF | laminar | CFL = 0.5 | ~8 × 106 cells, symmetry condition |
| T (s) | 3.0 | 4.0 | 5.0 | 6.0 | 7.0 | 8.0 | 9.0 | 10.0 | 11.0 |
|---|---|---|---|---|---|---|---|---|---|
| H (m) | 0.833 | 1.570 | 1.899 | 2.453 | 3.532 | 4.807 | 7.946 | 9.810 | 11.870 |
| S (-) | PTO Damping (Ns/m) | ||
|---|---|---|---|
| 6.2 | 1.0 | 0.0026 | 398,736.034 |
| 4.4 | 0.5 | 0.0026 | 118,149.758 |
| 15.4 | 11.0 | 0.0047 | 90,080.857 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wendt, F.; Nielsen, K.; Yu, Y.-H.; Bingham, H.; Eskilsson, C.; Kramer, M.; Babarit, A.; Bunnik, T.; Costello, R.; Crowley, S.; et al. Ocean Energy Systems Wave Energy Modelling Task: Modelling, Verification and Validation of Wave Energy Converters. J. Mar. Sci. Eng. 2019, 7, 379. https://doi.org/10.3390/jmse7110379
Wendt F, Nielsen K, Yu Y-H, Bingham H, Eskilsson C, Kramer M, Babarit A, Bunnik T, Costello R, Crowley S, et al. Ocean Energy Systems Wave Energy Modelling Task: Modelling, Verification and Validation of Wave Energy Converters. Journal of Marine Science and Engineering. 2019; 7(11):379. https://doi.org/10.3390/jmse7110379
Chicago/Turabian StyleWendt, Fabian, Kim Nielsen, Yi-Hsiang Yu, Harry Bingham, Claes Eskilsson, Morten Kramer, Aurélien Babarit, Tim Bunnik, Ronan Costello, Sarah Crowley, and et al. 2019. "Ocean Energy Systems Wave Energy Modelling Task: Modelling, Verification and Validation of Wave Energy Converters" Journal of Marine Science and Engineering 7, no. 11: 379. https://doi.org/10.3390/jmse7110379
APA StyleWendt, F., Nielsen, K., Yu, Y.-H., Bingham, H., Eskilsson, C., Kramer, M., Babarit, A., Bunnik, T., Costello, R., Crowley, S., Gendron, B., Giorgi, G., Giorgi, S., Girardin, S., Greaves, D., Heras, P., Hoffman, J., Islam, H., Jakobsen, K.-R., ... Yasutaka, I. (2019). Ocean Energy Systems Wave Energy Modelling Task: Modelling, Verification and Validation of Wave Energy Converters. Journal of Marine Science and Engineering, 7(11), 379. https://doi.org/10.3390/jmse7110379













