Study on the Suppression of Tip Leakage Vortex in Axial Flow Pumps Based on Circumferential Grooving in the Rotor Chamber
Abstract
1. Introduction
2. Materials and Methods
2.1. Geometric Model
2.2. Turbulence Model
2.3. Grid Generation and Numerical Settings
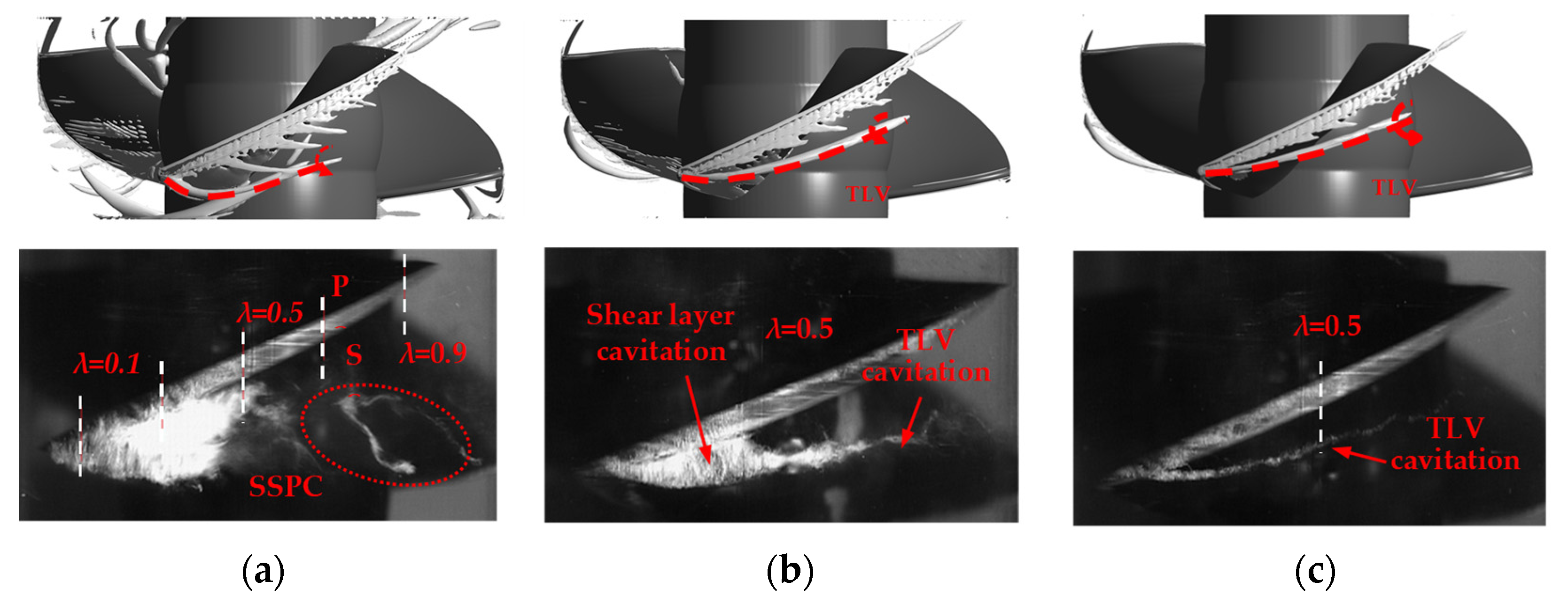
2.4. Comparison Between Numerical and Experimental Results
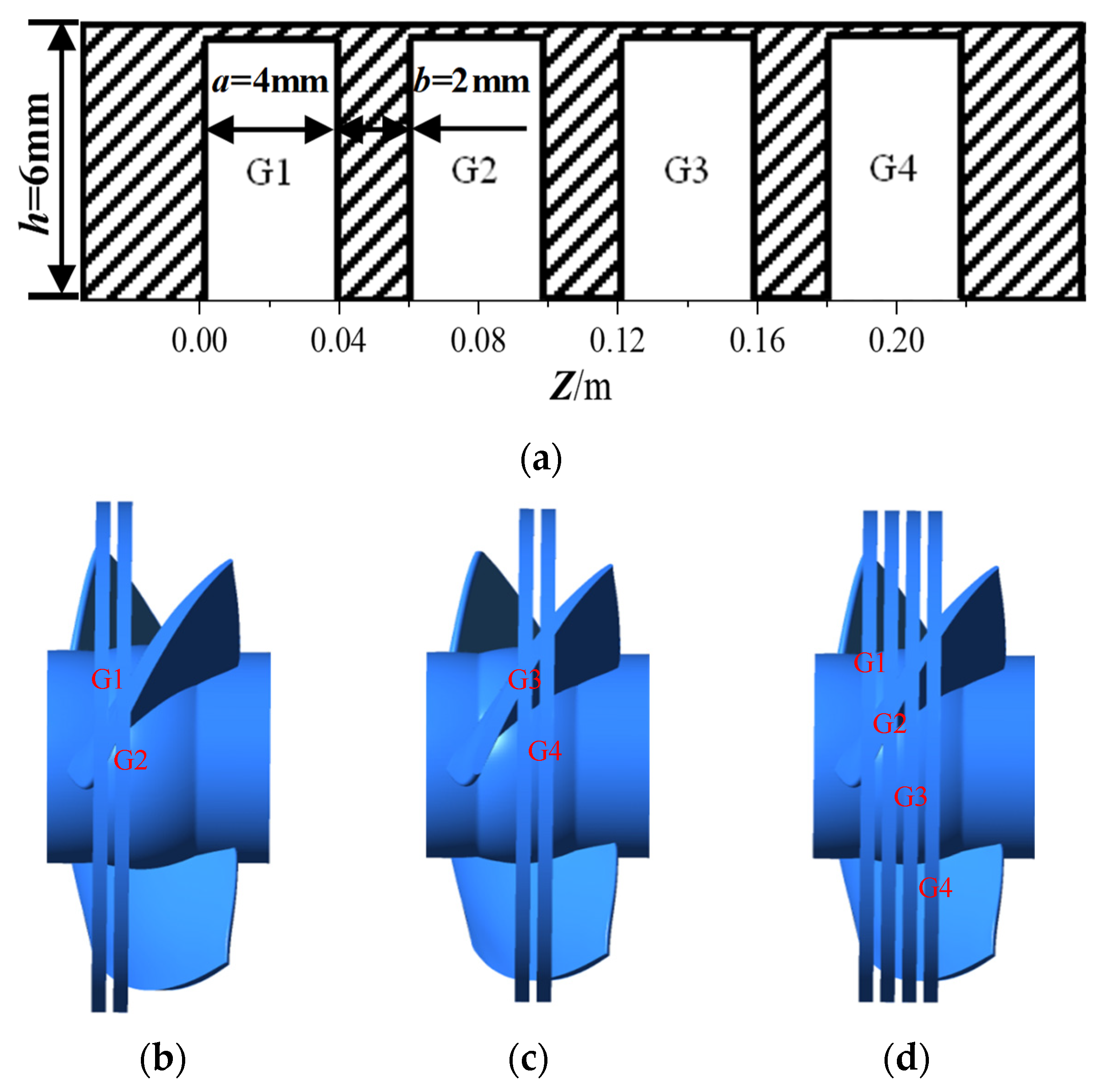
2.5. Grooving Case for the Rotor Chamber of an Axial Flow Pump
3. Results and Discussion
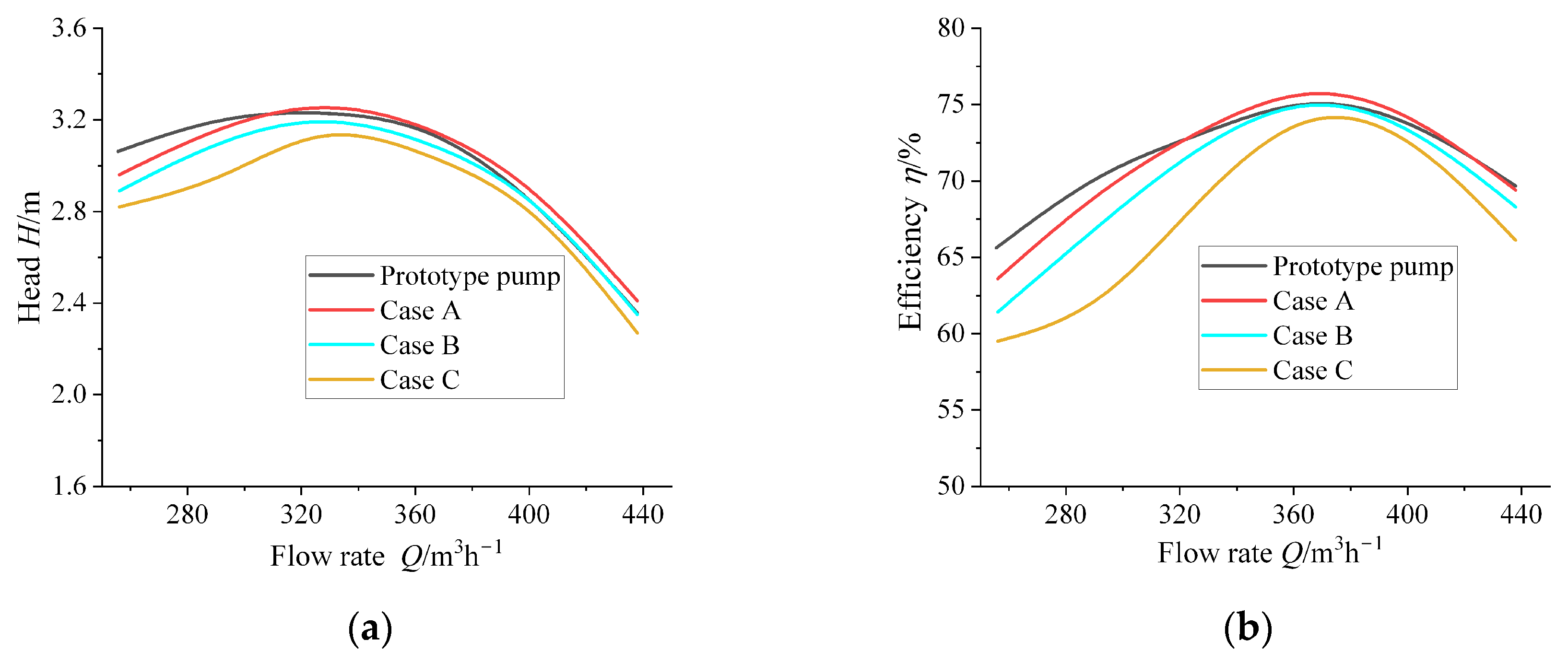
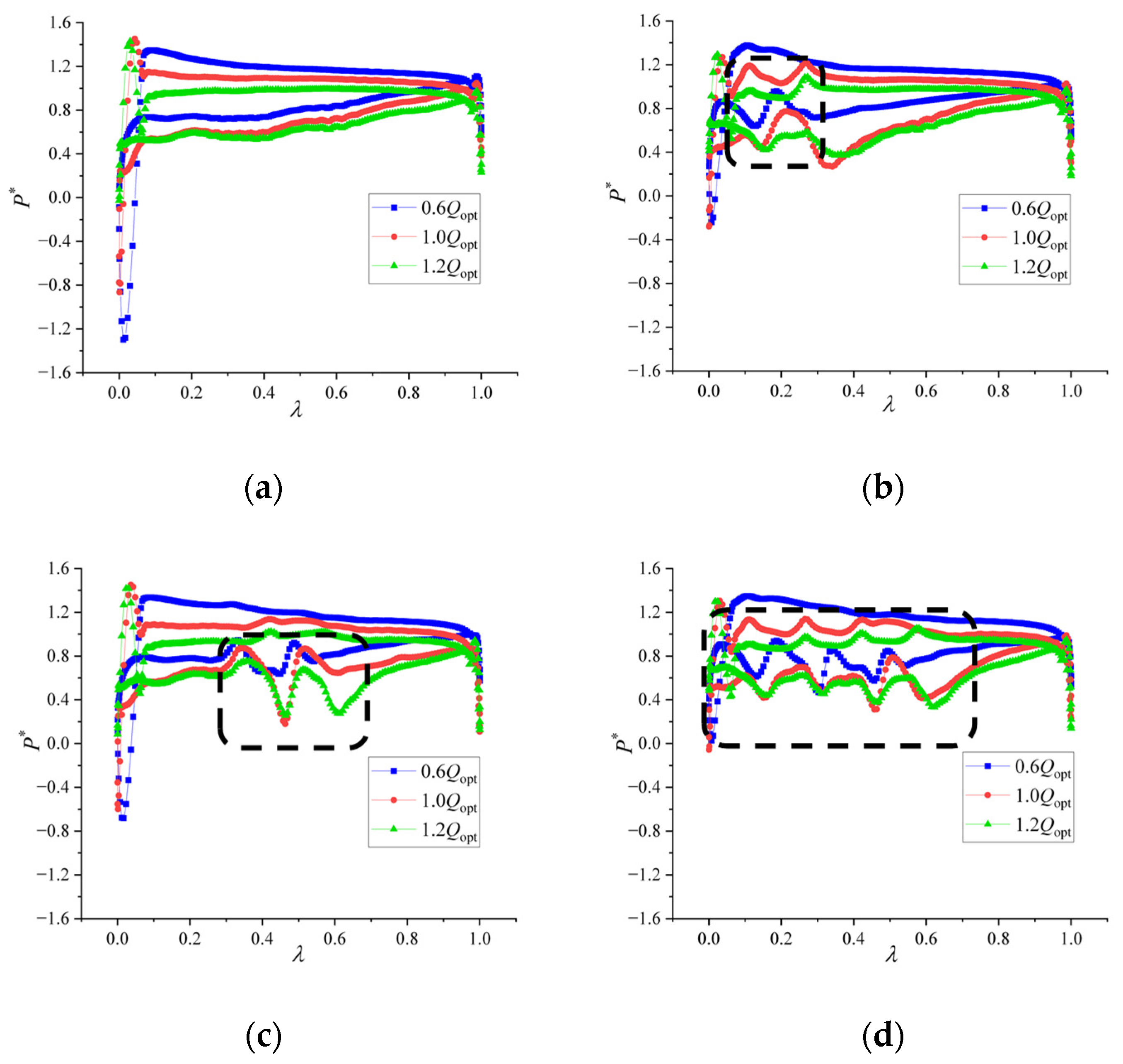
3.1. Energy Characteristics of Different Grooving Cases
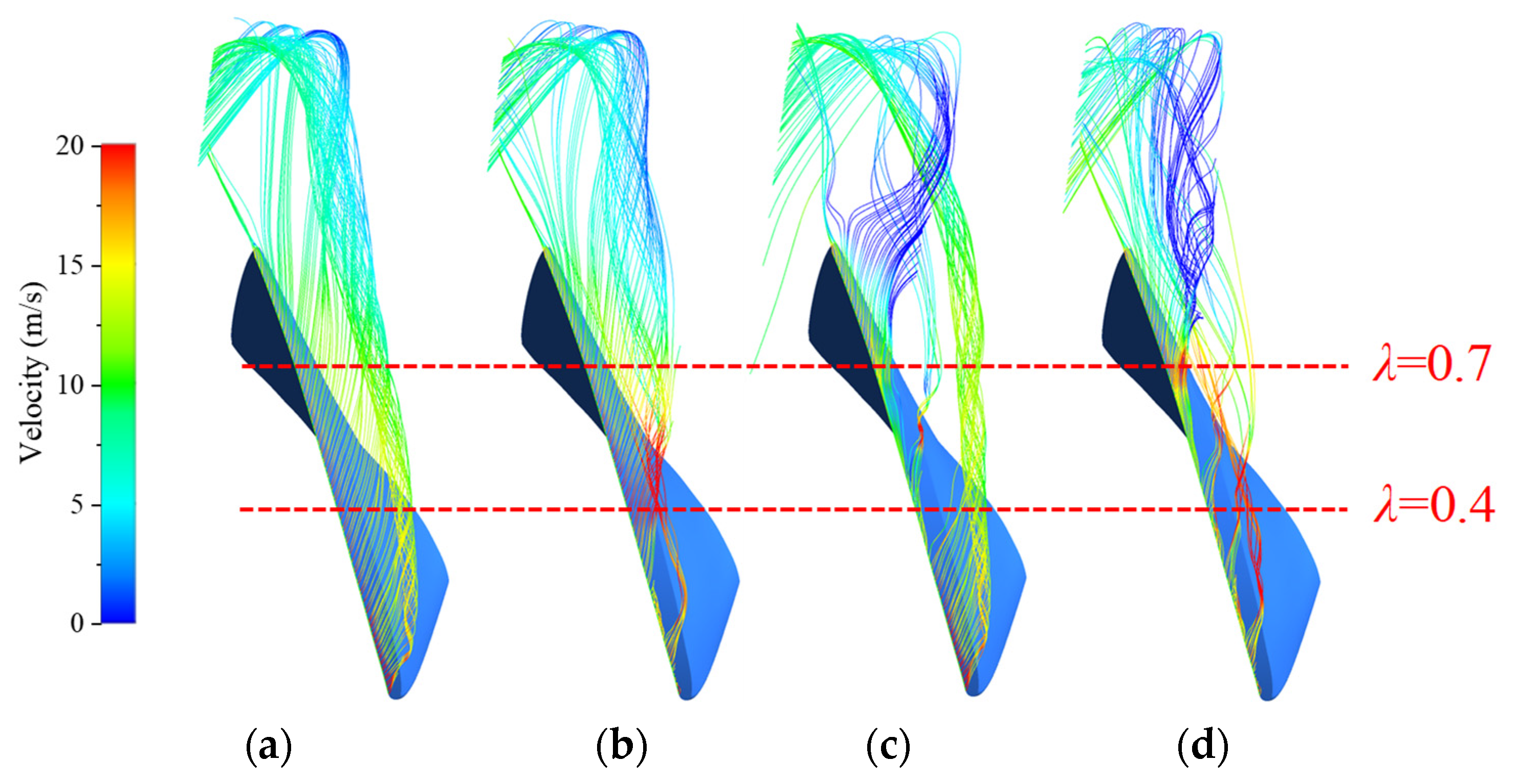
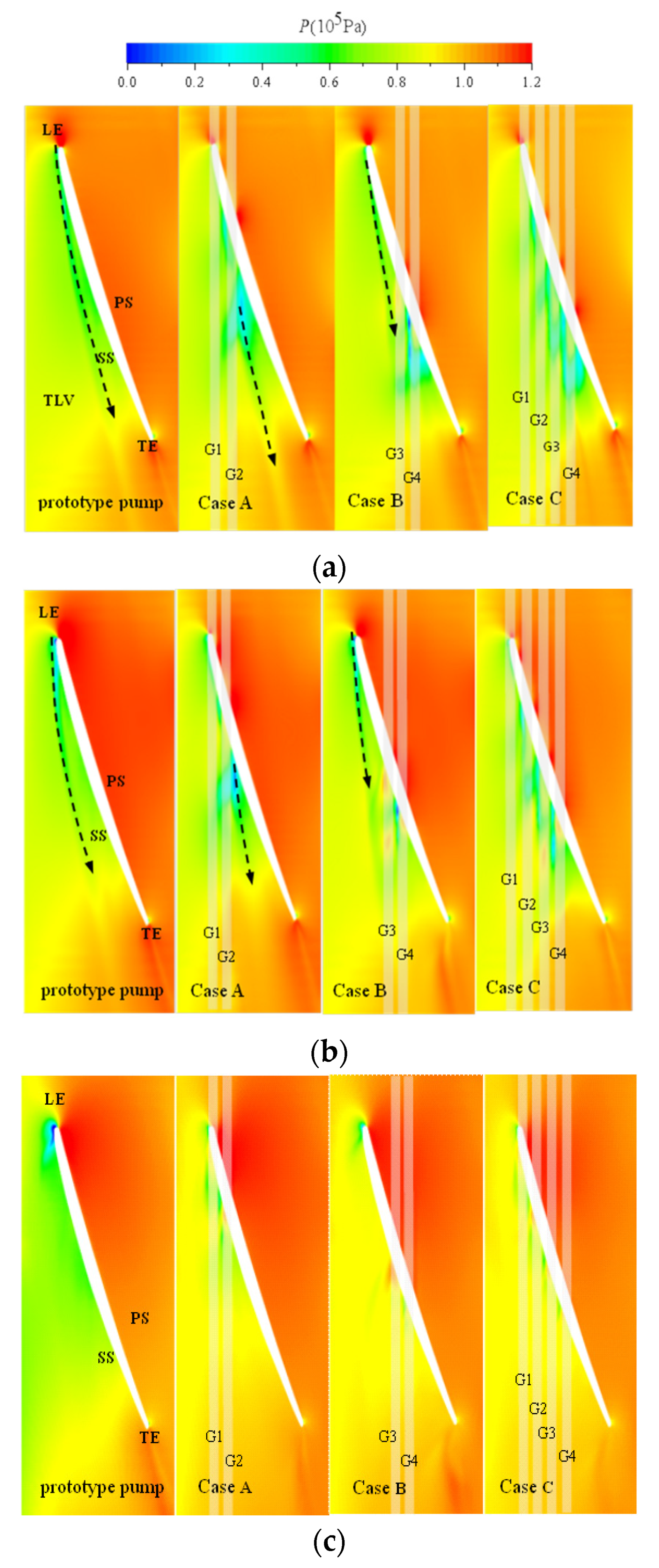
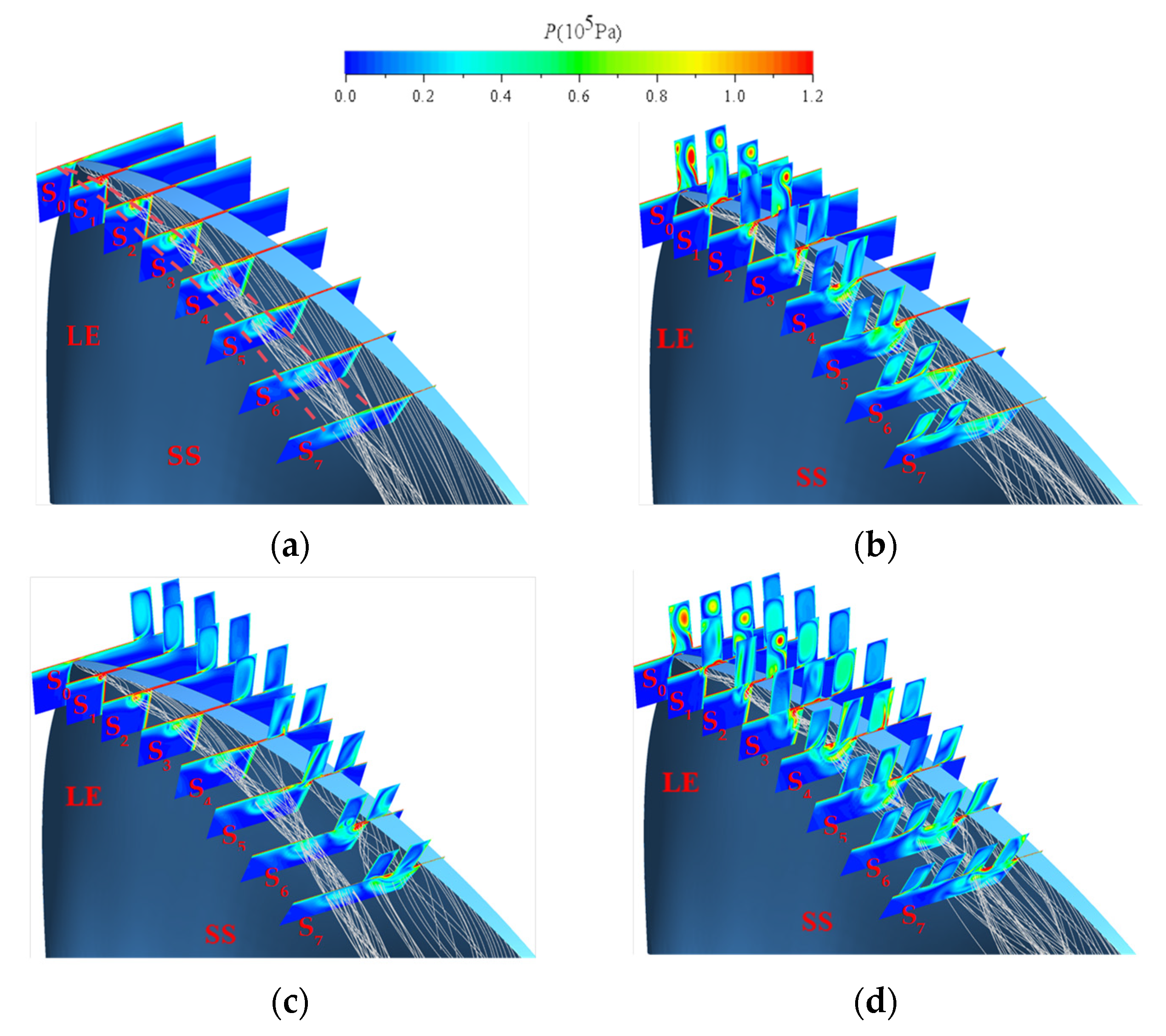
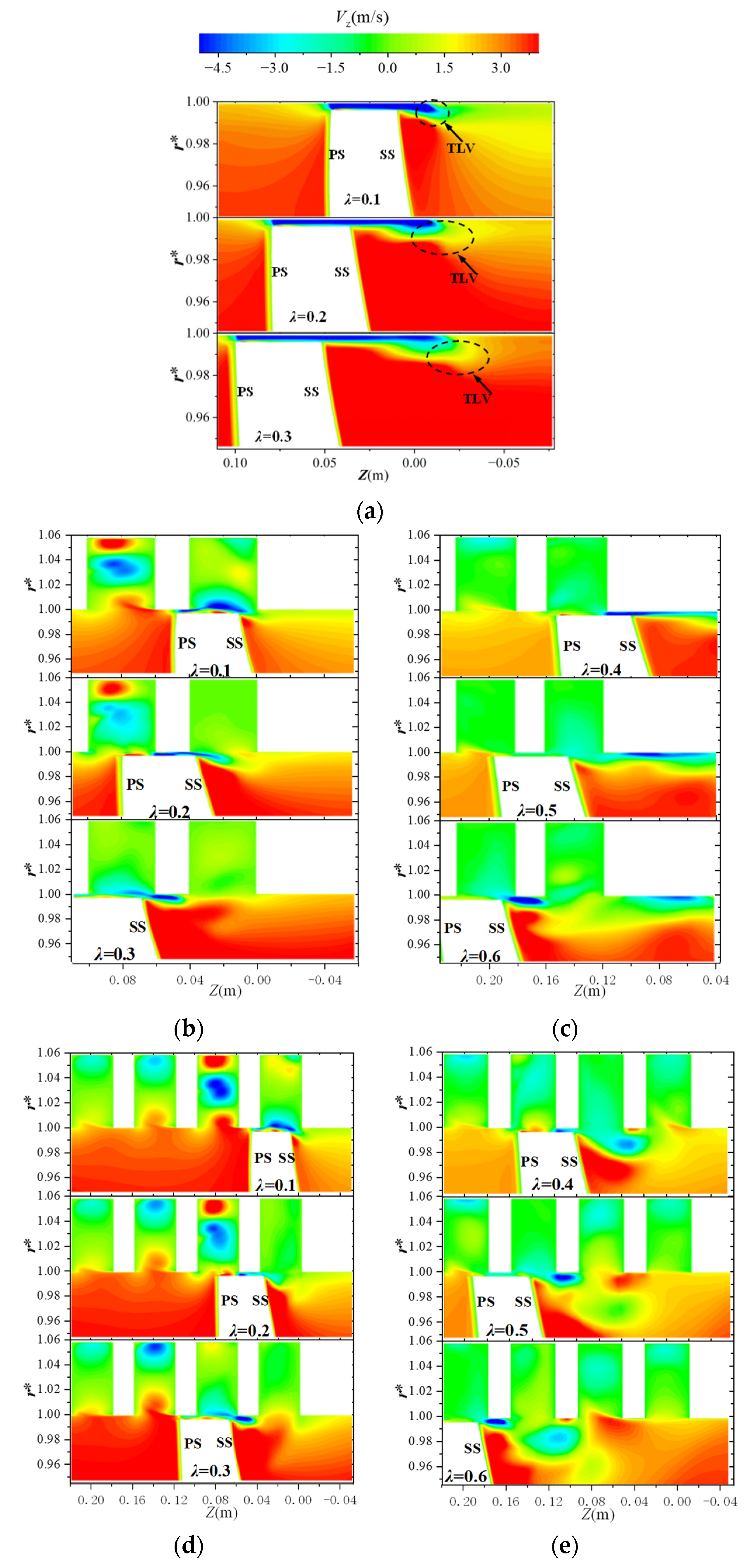
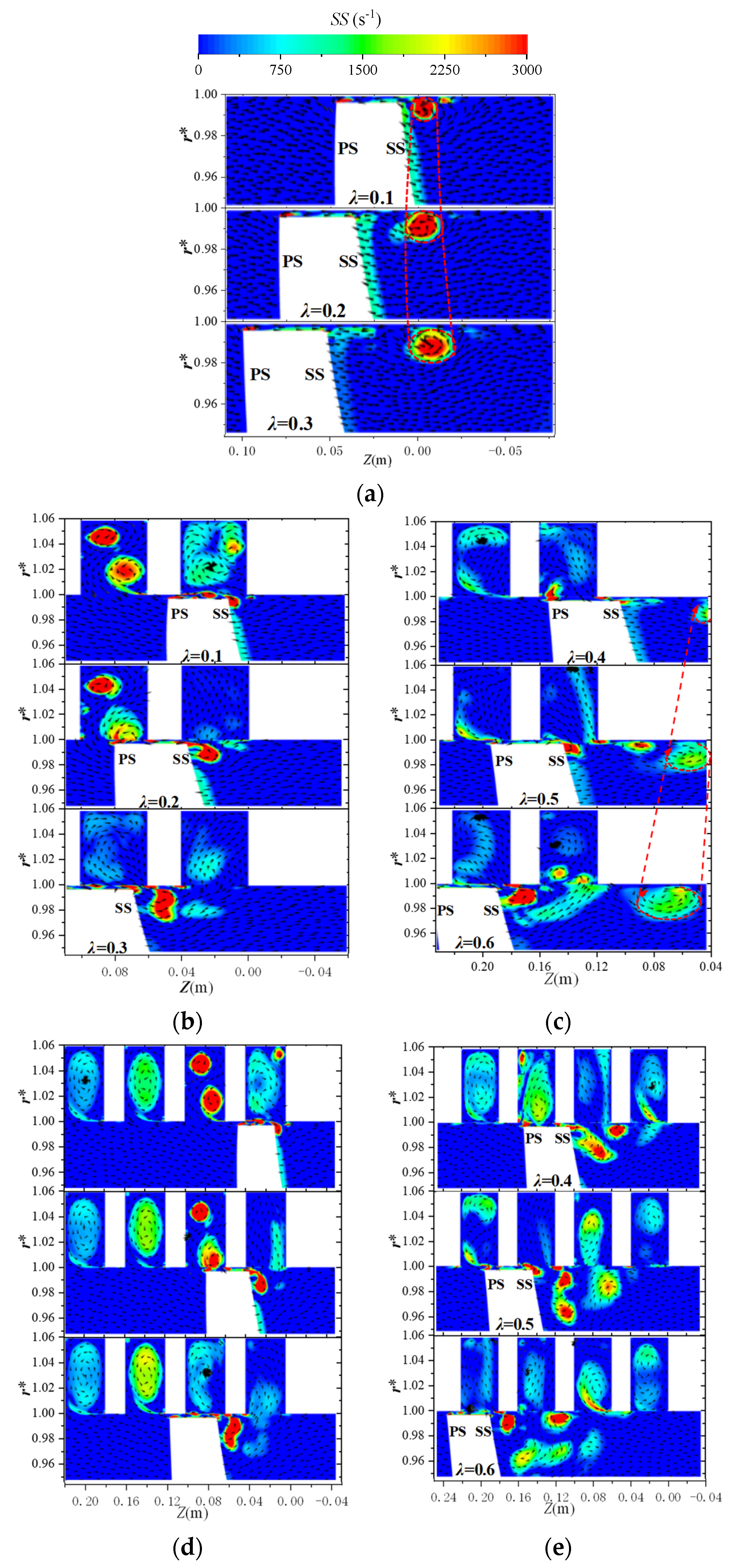
3.2. Flow Field Analysis at the Blade Tip for Various Groove Configurations
4. Conclusions
- (1)
- The numerical simulations exhibited a strong correlation between the TLV trajectories and the images captured by high-speed photography. At the design operating points, Case A enhanced the pump’s efficacy at elevated flow rates, with individual case heads akin to those of the prototype unit. Particularly, Case C exhibits significantly lower head and efficiency compared to the other cases.
- (2)
- In Case A, the G1 and G2 primarily influenced the blade tip leading edge region (λ = 0.0–0.4), altering the initial position of the TLV. In Case B, G3 and G4 mainly interfered with the secondary leakage flow in the mid-to-rear portion of the blade tip (λ = 0.3–0.7), without affecting the TLV inception at the leading edge. In Case C, G1–G4 collectively modified the tip leakage flow (λ = 0.0–0.7). Although tip leakage flow still occurred subsequently, its velocity was significantly reduced, resulting in weaker TLV formation that rapidly dissipated within the flow passage.
- (3)
- An in-depth look at the flow dynamics at the blade tip across diverse situations shows that the grooves situated near the leading edge of the blade tip within the runner cavity play a key role in affecting both the tip leakage flow and the main stream’s entrainment process. The formation of the TLV is effectively quelled, thanks to the close proximity of the vortex and the low-pressure zone to the blade’s edge. This setup minimizes the vortex’s interference with the flow through the passage. The presence of grooving in the middle-to-rear portion of the blade tip introduces a degree of interference with the tip leakage flow, simultaneously contributing to flow instability within the tip region.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbols | |
| D1 | Diameter of inlet pipe (m) |
| Z | Number of impeller blades |
| D2 | Diameter of impeller (m) |
| Zd | Number of diffuser blades |
| n | Design rotating speed |
| Qopt | Design flow rate (m3/s) |
| H | Delivery head (m) |
| τ | Tip clearance (m) |
| vz | Axial velocity (m/s) |
| r | Radius (m) |
| y+ | Non-dimensional distance from the wall |
| Abbreviations | |
| TLV | Tip leakage vortex |
| SSPCV | Suction-side-perpendicular cavitating vortices |
References
- Luo, X.; Ji, B.; Tsujimoto, Y. A review of cavitation in hydraulic machinery. J. Hydrodyn. 2016, 28, 335–358. [Google Scholar] [CrossRef]
- Zhang, R.; Chen, H. Numerical analysis of cavitation within slanted axial-flow pump. J. Hydrodyn. 2013, 25, 663–672. [Google Scholar] [CrossRef]
- Fei, Z.; Zhang, R.; Xu, H.; Feng, J.; Mu, T.; Chen, Y. Energy performance and flow characteristics of a slanted axial-flow pump under cavitation conditions. Phys. Fluids 2022, 34, 035121. [Google Scholar] [CrossRef]
- Wu, H.; Tan, D.; Miorini, R.L. Three-dimensional flow structures and associated turbulence in the tip region of a water jet pump rotor blade. Exp. Fluids 2011, 51, 1721–1737. [Google Scholar] [CrossRef]
- Miorini, R.L.; Tan, D.; Wu, H. Three-Dimensional Structure and Turbulence Within the Tip Leakage Vortex of an Axial Water jet Pump. In Proceedings of the ASME-JSME-KSME Joint Fluids Engineering Conference, Hamamatsu, Japan, 24–29 July 2011; pp. 271–281. [Google Scholar]
- Liu, Y.; Tan, L. Spatial-Temporal Evolution of Tip Leakage Vortex in a Mixed-Flow Pump with Tip Clearance. J. Fluids Eng. 2019, 141, 081–302. [Google Scholar] [CrossRef]
- Choi, M. Effects of Circumferential Casing Grooves on the Performance of a Transonic Axial Compressor. Int. J. Turbo Jet-Engines 2015, 32, 361. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, D.; Li, Y. Numerical investigations of the vortex suppression in the tip leakage flow of a hydrofoil via bionic geometries. In Proceedings of the Thirty-First National Symposium Hydrodynamics, Xiamen, China, 31 October–2 November 2020; p. 6. [Google Scholar]
- Wang, L.; Luo, X.; Feng, J. Method of bionic wavy tip on vortex and cavitation suppression of a hydrofoil in tidal energy. Ocean. Eng. 2023, 278, 114–499. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, D.; Wang, F. Vortex suppression of the tip leakage flow over a NACA0009 hydrofoil via a passive jet induced by the double-control-hole. Ocean. Eng. 2021, 237, 109–647. [Google Scholar] [CrossRef]
- Wang, G.; Chu, W.; Zhang, H.; Guo, Z. Parametric study on active flow control of a transonic axial compressor rotor using endwall synthetic jet. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2022, 236, 2031–2043. [Google Scholar] [CrossRef]
- Taghavi, Z.; Behbahani, M. Improvement of aerodynamic performance of a low speed axial compressor rotor blade row through air injection. Aerosp. Sci. Technol. 2018, 72, 409–417. [Google Scholar] [CrossRef]
- Geng, S.; Zhang, H.; Chen, J.; Huang, W. Numerical study on the response of tip leakage flow unsteadiness to micro tip injection in a low- speed isolated compressor rotor. In Proceedings of the ASME Turbo Expo 2007: Power for Land, Sea, and Air, ASME 2007-GT-27729, Montreal, QC, Canada, 4–7 June 2007. [Google Scholar]
- Wilke, I.; Kau, H.-P. A numerical investigation of the influence of casing treatments on the tip leakage flow in a HPC front stage. In Proceedings of the ASME Turbo Expo: Power for Land, Sea, and Air, Amsterdam, The Netherlands, 3–6 June 2002; pp. 1155–1165. [Google Scholar]
- Khaleghi, H. Effect of discrete endwall recirculation on the stability of a high-speed compressor rotor. Aerosp. Sci. Technol. 2014, 37, 130–137. [Google Scholar] [CrossRef]
- Hwang, Y.; Kang, S. Numerical study on the effects of casing treatment on unsteadiness of tip leakage flow in an axial compressor. In Proceedings of the ASME Turbo Expo 2012: Turbine Technical Conference and Exposition, Copenhagen, Denmark, 11–15 June 2012; American Society of Mechanical Engineers: New York, NY, USA, 2012; Volume 44748, pp. 2501–2511. [Google Scholar]
- Kurokawa, J. J-groove technique for suppressing various anomalous flow phenomena in turbomachines. Int. J. Fluid Mach. Syst. 2011, 4, 1–13. [Google Scholar] [CrossRef]
- Goltz, I.; Kosyna, G.; Stark, U.; Saathoff, H.; Bross, S. Stall inception phenomena in a single-stage axial-flow pump. J. Power Energy 2003, 217, 471–479. [Google Scholar] [CrossRef]
- Goltz, I.; Kosyna, G.; Delgado, A. Eliminating the head instability of an axial-flow pump using axial grooves. Proc. Inst. Mech. Eng. Part A J. Power Energy 2012, 227, 206–215. [Google Scholar] [CrossRef]
- Zhou, X.; Zhao, Q.; Cui, W.; Xu, J. Investigation on axial effect of slot casing treatment in a transonic compressor. Appl. Therm. Eng. 2017, 126, 53–69. [Google Scholar] [CrossRef]
- Taghavi-Zenouz, R.; Abbasi, S. Alleviation of spike stall in axial compressors utilizing grooved casing treatment. Chin. J. Aeronaut. 2015, 123, 649–658. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. J. Sci. Comput. 1986, 1, 3–51. [Google Scholar] [CrossRef]
- Qian, Z.; Wu, P.; Guo, Z.; Huai, W. Numerical simulation of air entrainment and suppression in pump sump. Sci. China Technol. Sci. 2016, 59, 1847–1855. [Google Scholar] [CrossRef]
- Shen, X.; Zhang, D.; Xu, B.; Jin, Y.; Shi, W. Experimental investigation of the transient patterns and pressure evolution of tip leakage vortex and induced-vortices cavitation in an axial flow pump. J. Fluids Eng. 2020, 142, 101–206. [Google Scholar]
- Kan, K.; Li, H.; Chen, H.; Gong, Y.; Li, T.; Shen, L. Effects of Clearance and Operating Conditions on Tip Leakage Vortex-Induced Energy Loss in an Axial-Flow Pump Using Entropy Production Method. Fluids Eng. 2023, 145, 031201. [Google Scholar] [CrossRef]
- Muller, M.W.; Schiffer, H.-P.; Hah, C. Effect of Circumferential Grooves on the Aerodynamic Performance of an Axial Single-Stage Transonic Compressor. In Proceedings of the ASME Turbo Expo: Power for Land, Sea, & Air, ASME GT2007-27365, Montreal, QC, Canada, 14–17 May 2007. [Google Scholar]
- Houghton, T.; Day, I. Stability enhancement by casing grooves: The importance of stall inception mechanism and solidity. J. Turbomach. 2012, 134, 021003. [Google Scholar] [CrossRef]
- Straka, W.A.; Farrell, K.J. The effect of spatial wandering on experimental laser velocimeter measurements of the end-wall vortices in an axial-flow pump. Exp. Fluids 1992, 13, 163–170. [Google Scholar] [CrossRef]
- Zhang, H.; Zang, J.; Zhang, D.; Shi, W.; Shen, J. Analysis of the formation mechanism of secondary tip leakage vortex (S-TLV) in an axial flow pump. Machines 2022, 10, 41. [Google Scholar] [CrossRef]
- Shen, X.; Zhang, D.; Xu, B.; Wu, H.; Wang, P.; Shi, W. Comparative study of tip leakage vortex trajectory and cavitation in an axial flow pump with various tip clearances. J. Mech. Sci. Technol. 2022, 36, 1289–1302. [Google Scholar] [CrossRef]
- Zhang, D.; Shi, L.; Shi, W.; Zhao, R.; Wang, H.; van Esch, B.P.M. Numerical analysis of unsteady tip leakage vortex cavitation cloud and unstable suction-side-perpendicular cavitating vortices in an axial flow pump. Int. J. Multiph. Flow 2015, 77, 244–259. [Google Scholar] [CrossRef]
- Shen, X.; Zhao, X.; Xu, B.; Zhang, D.; Yang, G.; Shi, W.; van Esch, B.P.M. Unsteady characteristics of tip leakage vortex structure and dynamics in an axial flow pump. Ocean. Eng. 2022, 266, 112–850. [Google Scholar] [CrossRef]
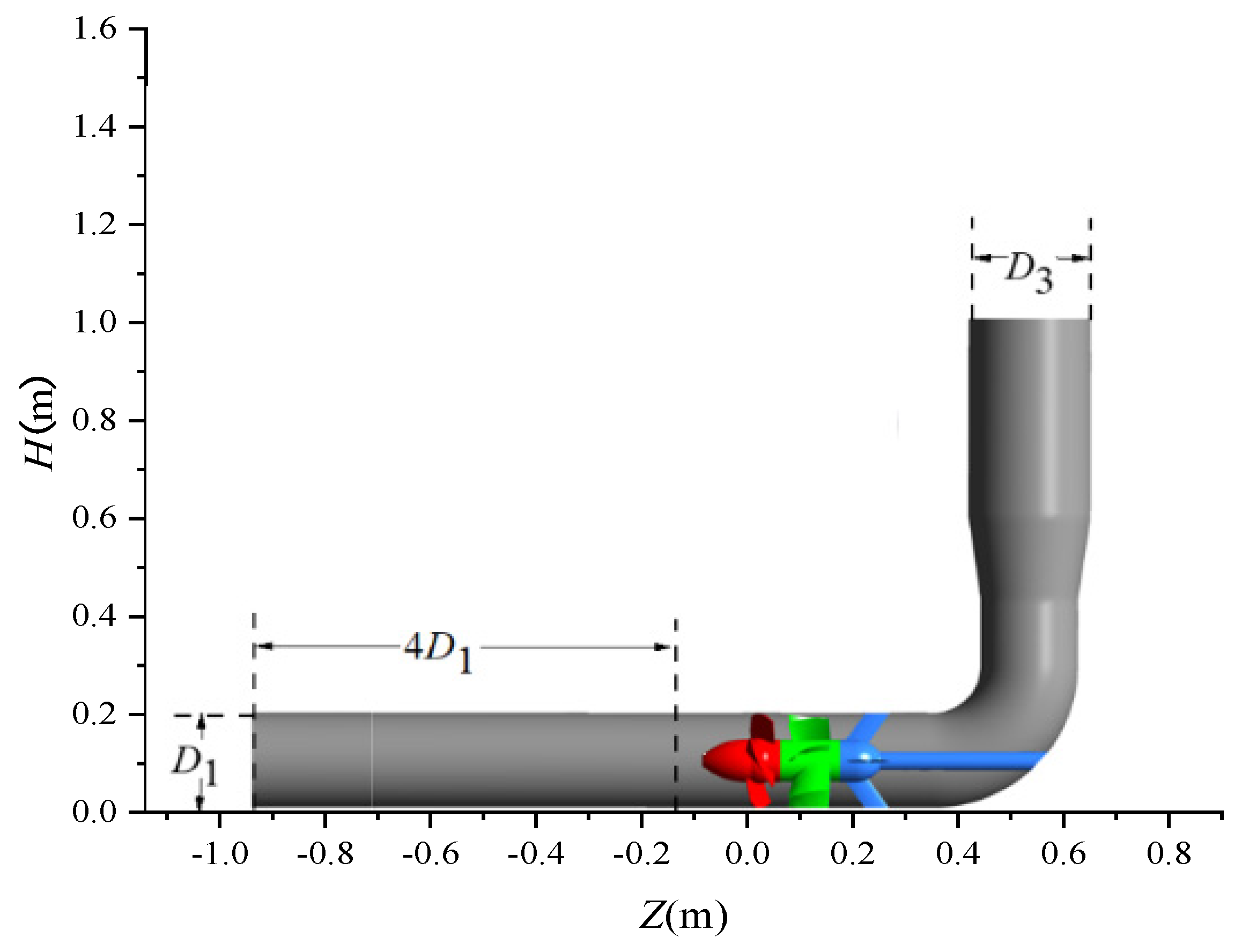



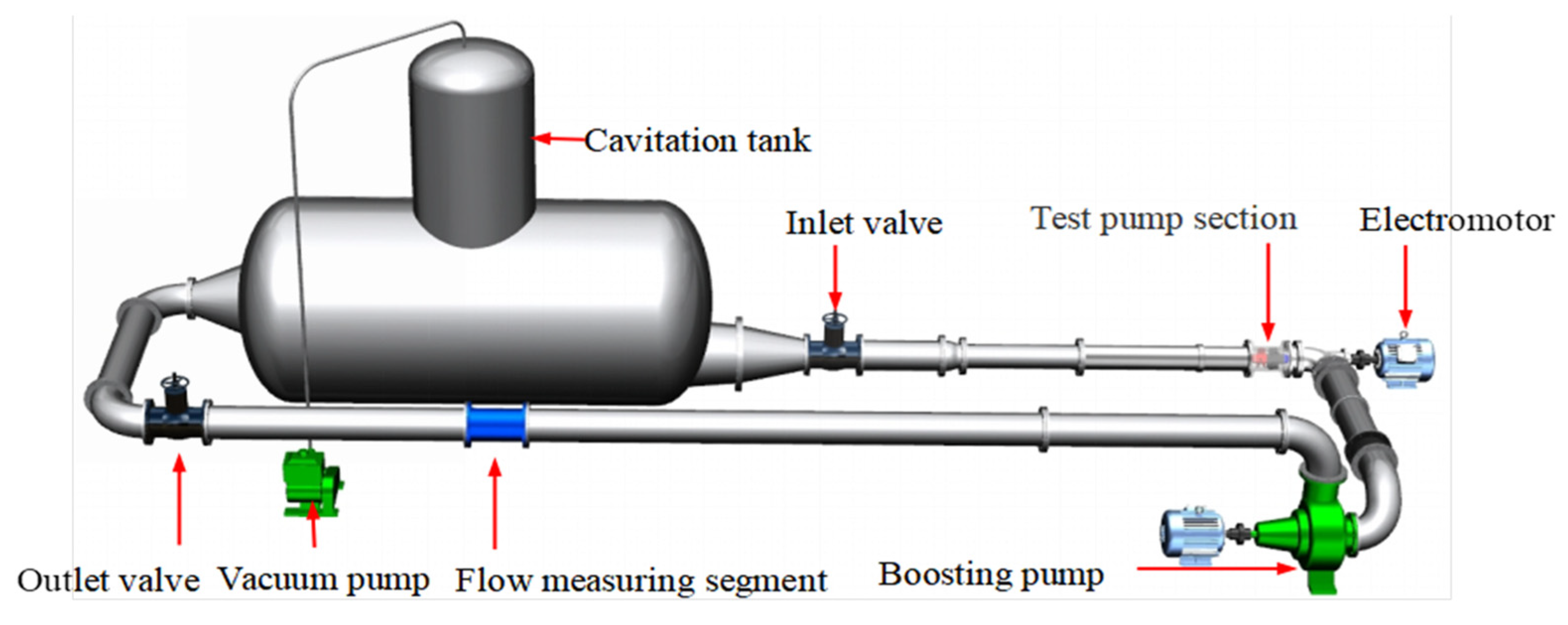
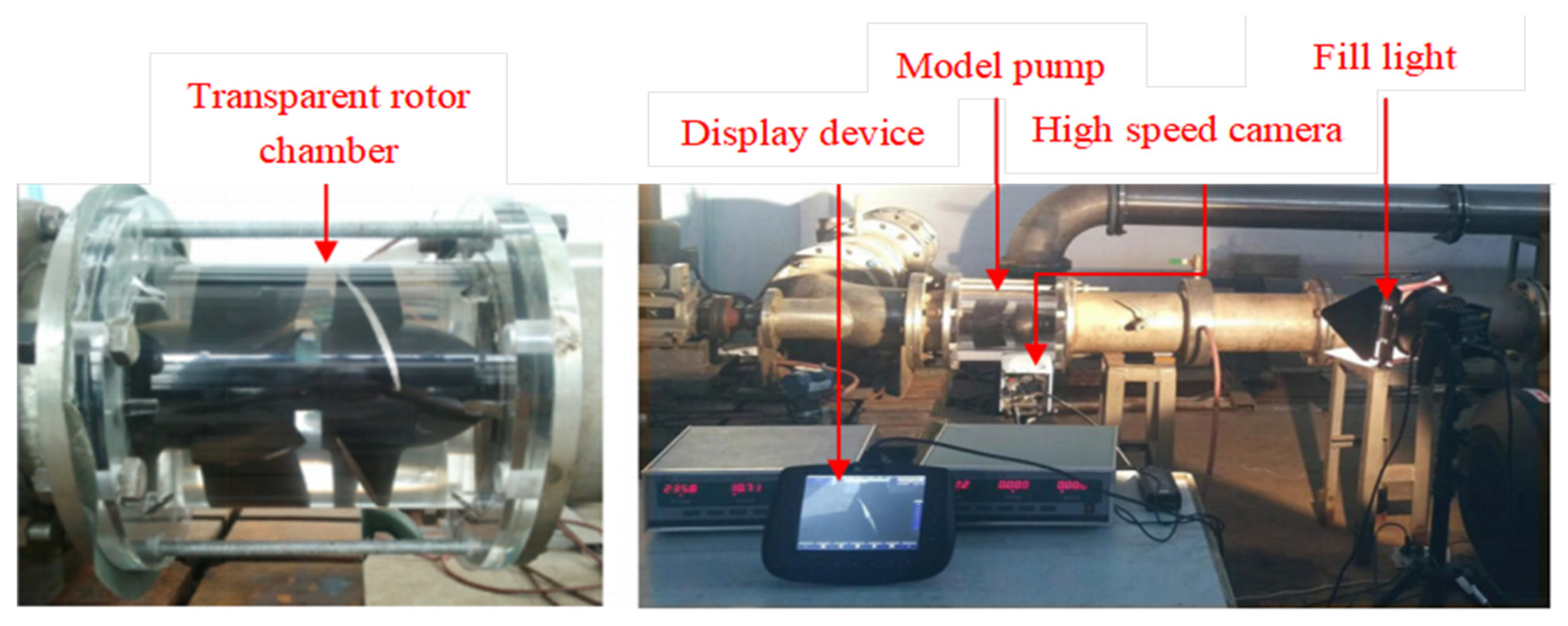
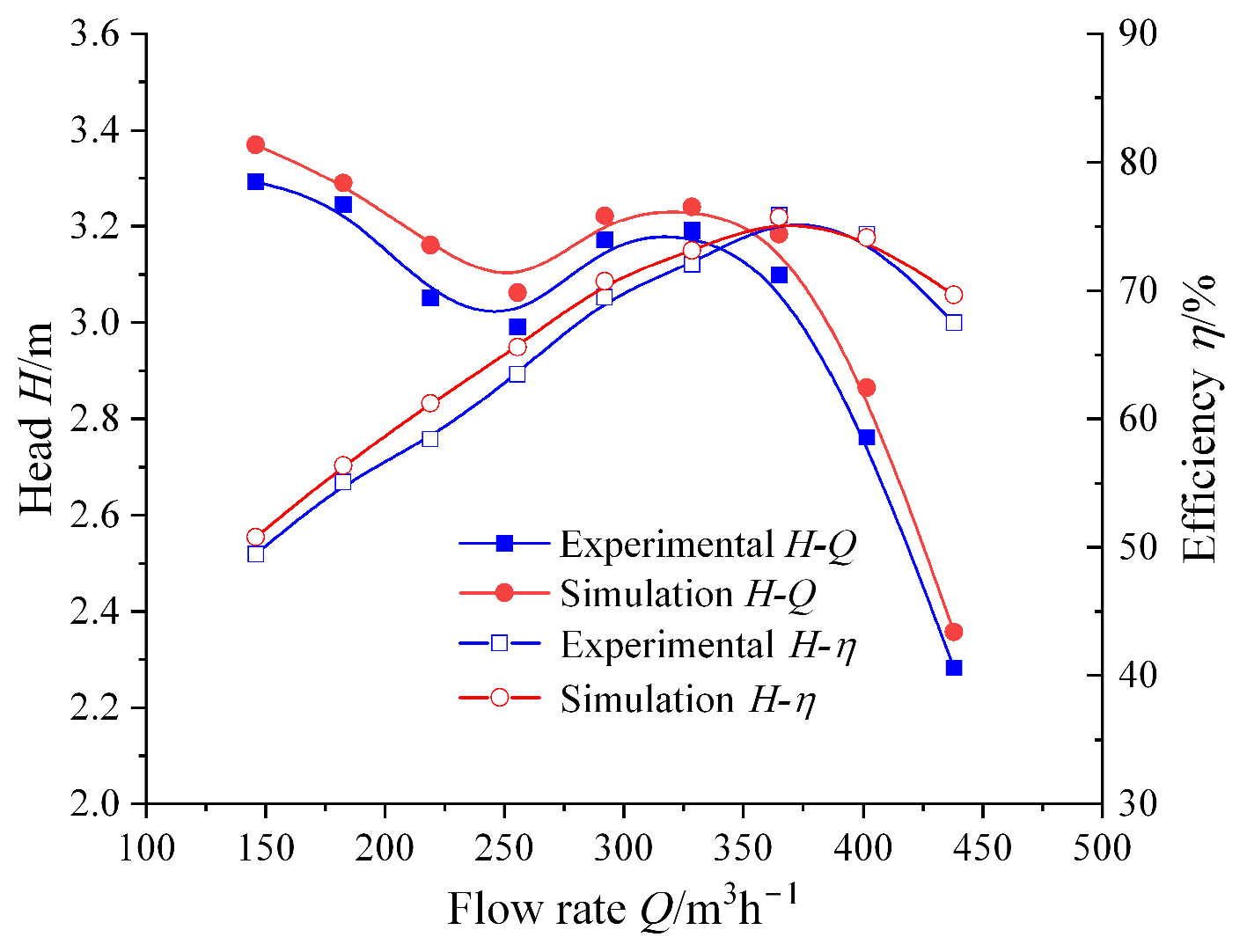

| Main Parameters | Values |
|---|---|
| Inlet diameter D1 | 201 mm |
| Number of impeller blades Z | 3 |
| Impeller diameter D2 | 200 mm |
| Number of diffuser blades Zd | 7 |
| Design rotating speed n | 1450 r/min |
| Design flow rate Qopt | 365 m3/h |
| Design head H | 3.02 m |
| Tip clearance τ | 0.5 mm |
| Blade height h | 54.5 mm |
| Parameters | Values |
|---|---|
| β * | 0.09 |
| a1 | 0.31 |
| α1 | 5/9 |
| α2 | 0.44 |
| β1 | 3/40 |
| β2 | 0.0828 |
| σ1 | 0.85 |
| σω1 | 0.85 |
| σk2 | 1 |
| σω2 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, H.; Zhang, D.; Shen, X.; Ni, C.; Yang, G. Study on the Suppression of Tip Leakage Vortex in Axial Flow Pumps Based on Circumferential Grooving in the Rotor Chamber. J. Mar. Sci. Eng. 2025, 13, 972. https://doi.org/10.3390/jmse13050972
Wu H, Zhang D, Shen X, Ni C, Yang G. Study on the Suppression of Tip Leakage Vortex in Axial Flow Pumps Based on Circumferential Grooving in the Rotor Chamber. Journal of Marine Science and Engineering. 2025; 13(5):972. https://doi.org/10.3390/jmse13050972
Chicago/Turabian StyleWu, Haoran, Desheng Zhang, Xi Shen, Chen Ni, and Gang Yang. 2025. "Study on the Suppression of Tip Leakage Vortex in Axial Flow Pumps Based on Circumferential Grooving in the Rotor Chamber" Journal of Marine Science and Engineering 13, no. 5: 972. https://doi.org/10.3390/jmse13050972
APA StyleWu, H., Zhang, D., Shen, X., Ni, C., & Yang, G. (2025). Study on the Suppression of Tip Leakage Vortex in Axial Flow Pumps Based on Circumferential Grooving in the Rotor Chamber. Journal of Marine Science and Engineering, 13(5), 972. https://doi.org/10.3390/jmse13050972








