Investigation of Directionality Effect for 10 MW Monopile Offshore Wind Turbine Excited by Wind, Wave, and Earthquakes
Abstract
1. Introduction
2. Physical Model and Environmental Condition
2.1. 10 MW MOWT
2.2. Geotechnical Condition
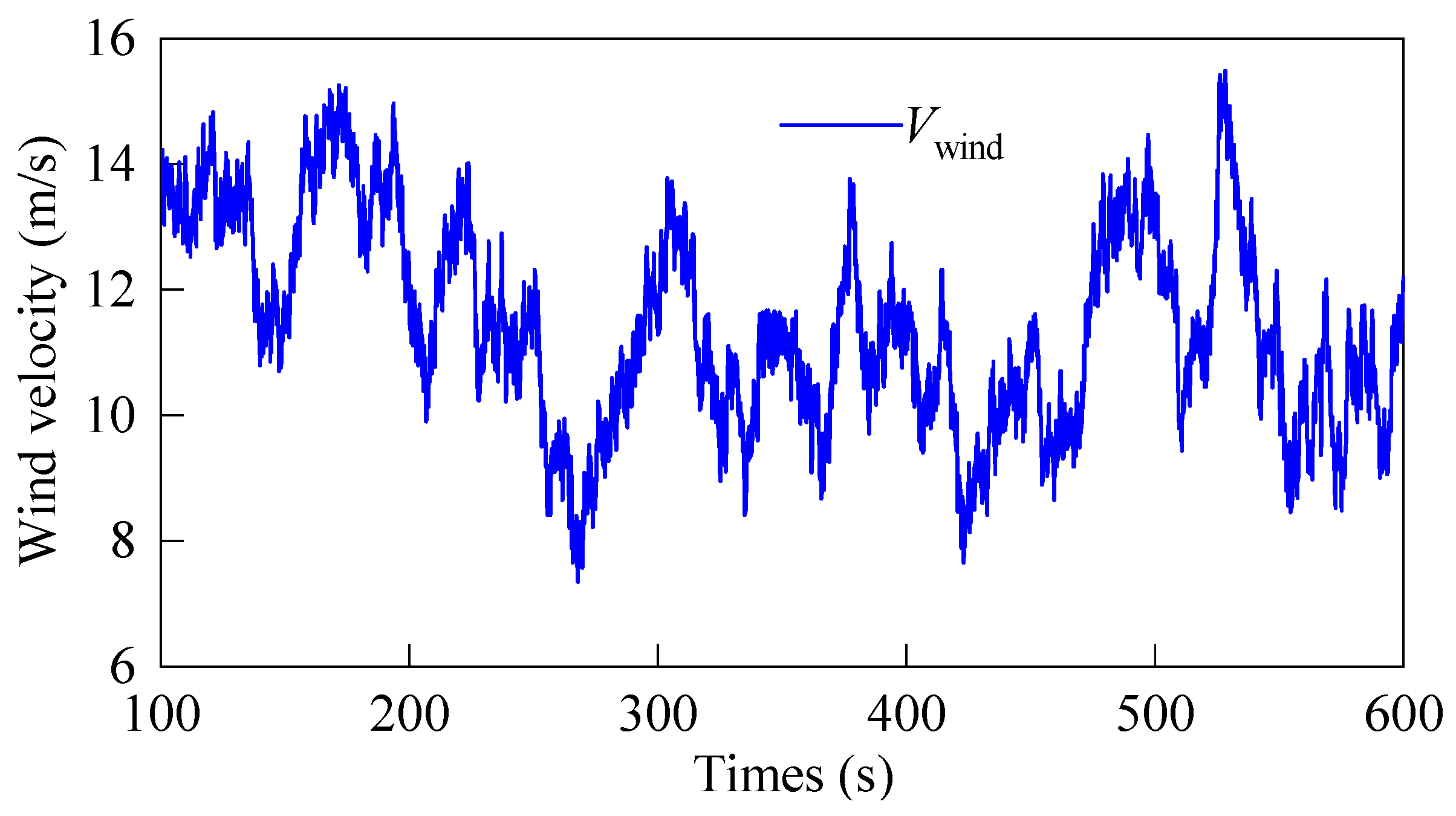
2.3. Metocean Conditions
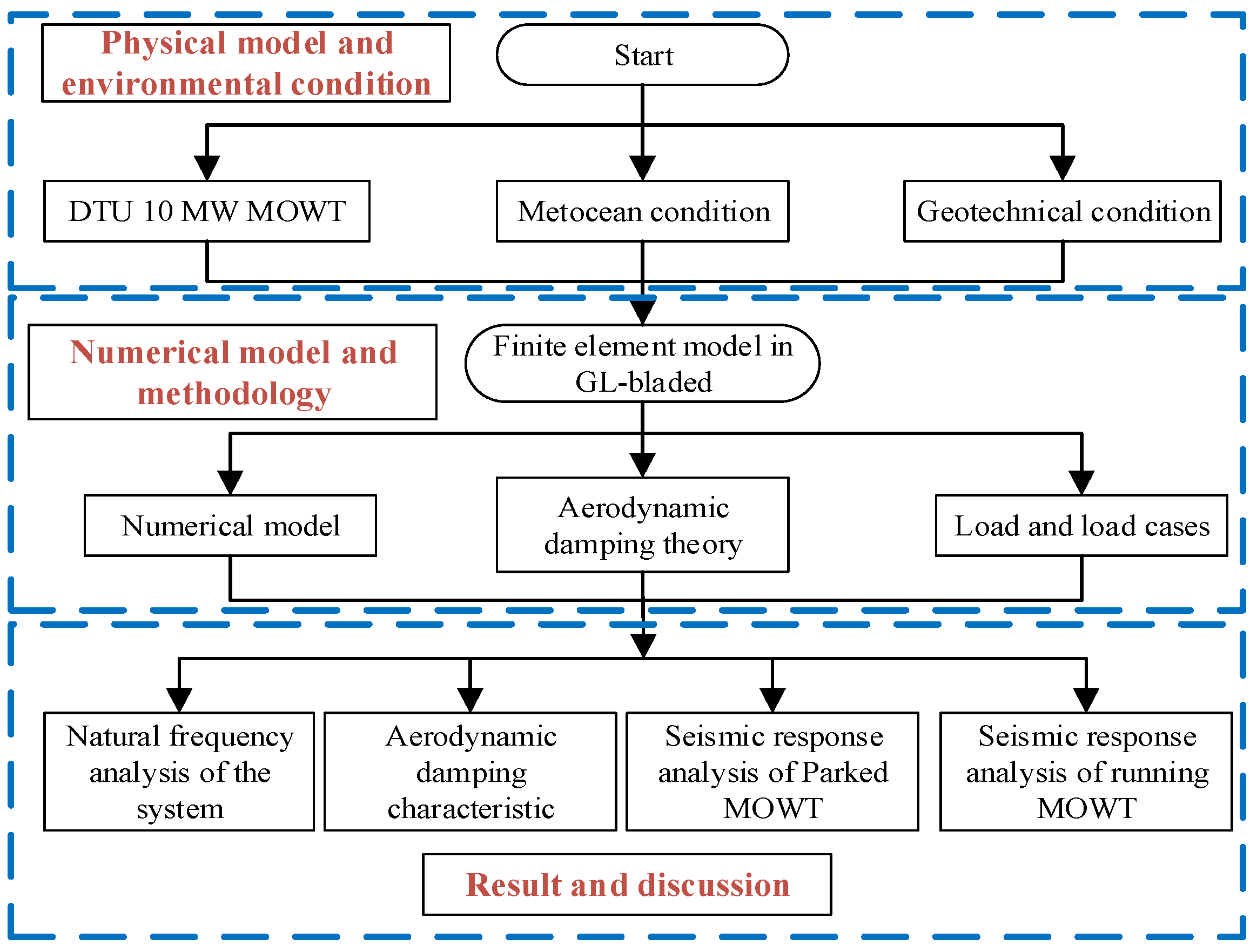
3. Numerical Model and Methodology
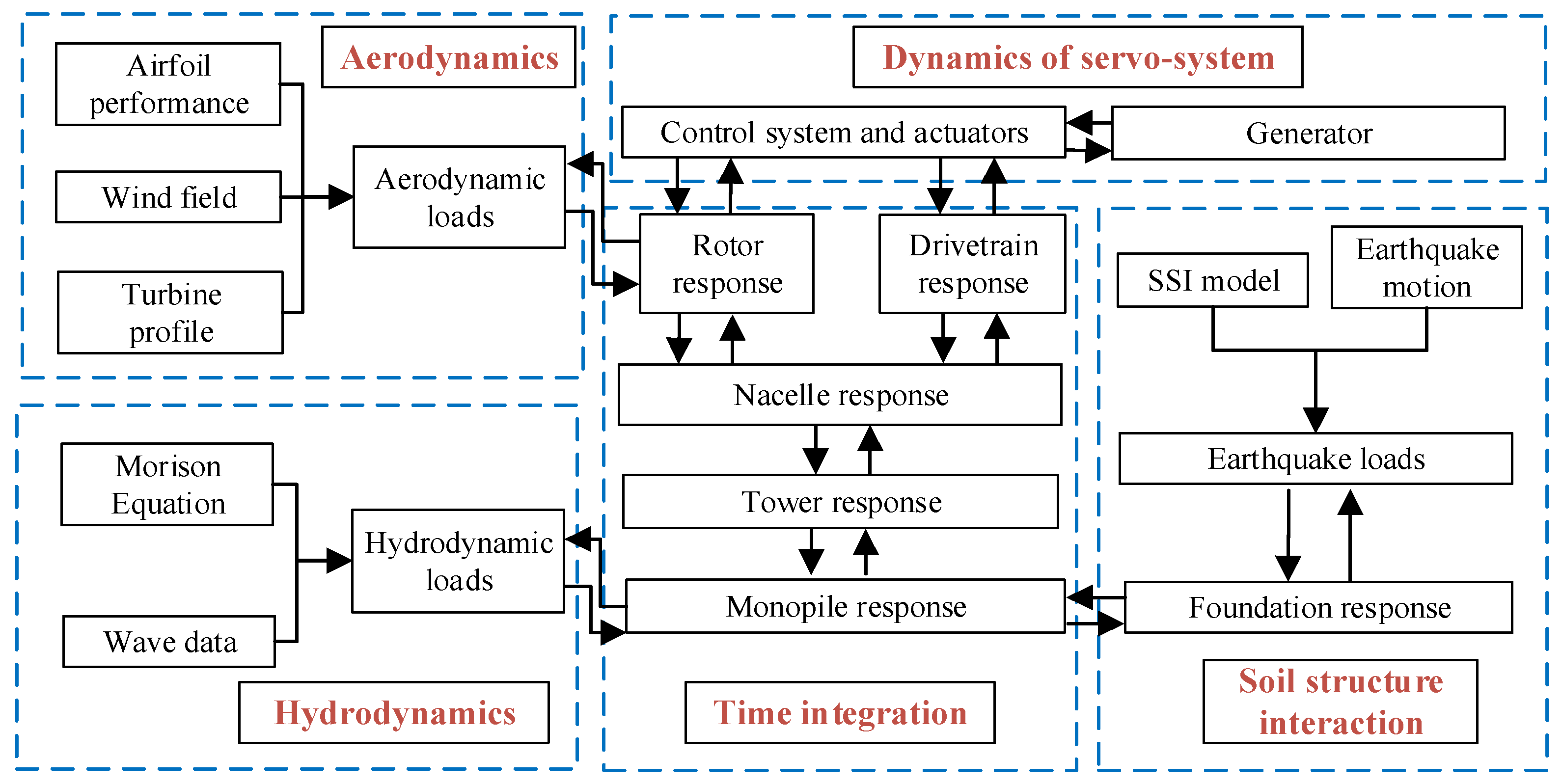
3.1. Numerical Model
3.2. Aerodynamic Damping Theory
3.3. Load and Load Cases
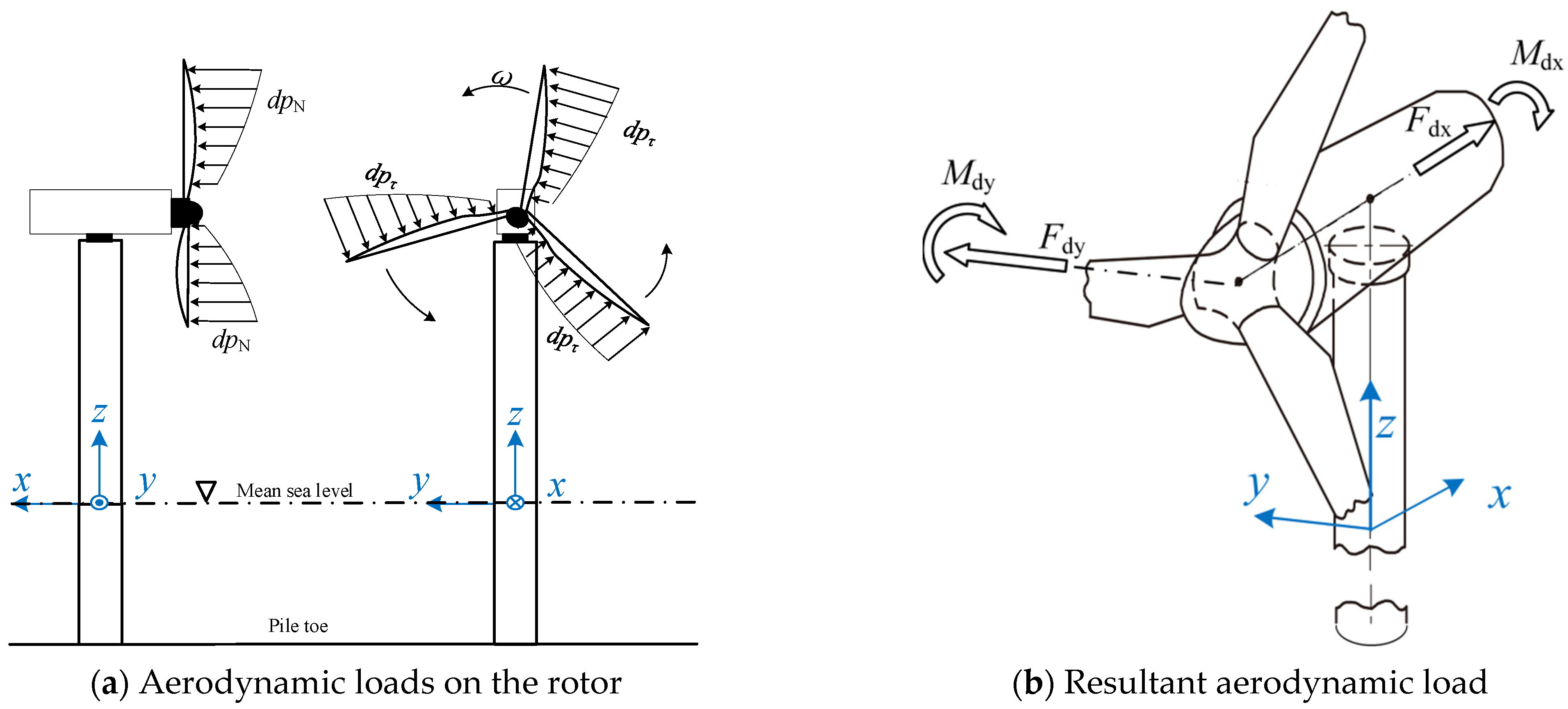
3.3.1. Aerodynamic Load
3.3.2. Hydrodynamic Load
3.3.3. Input Earthquake
4. Results and Discussion
4.1. Natural Frequency of the System
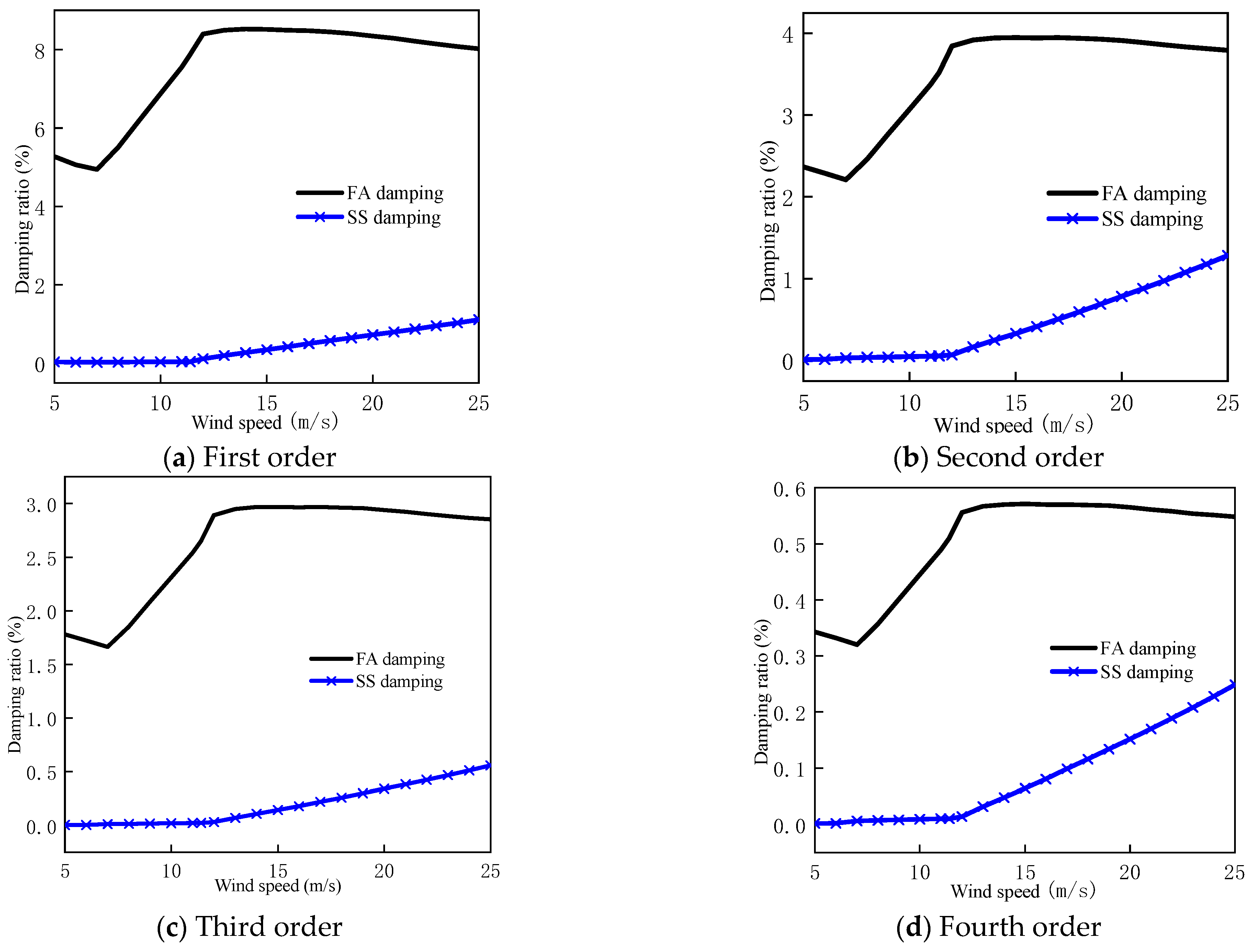
4.2. Aerodynamic Damping of 10 MW MOWT
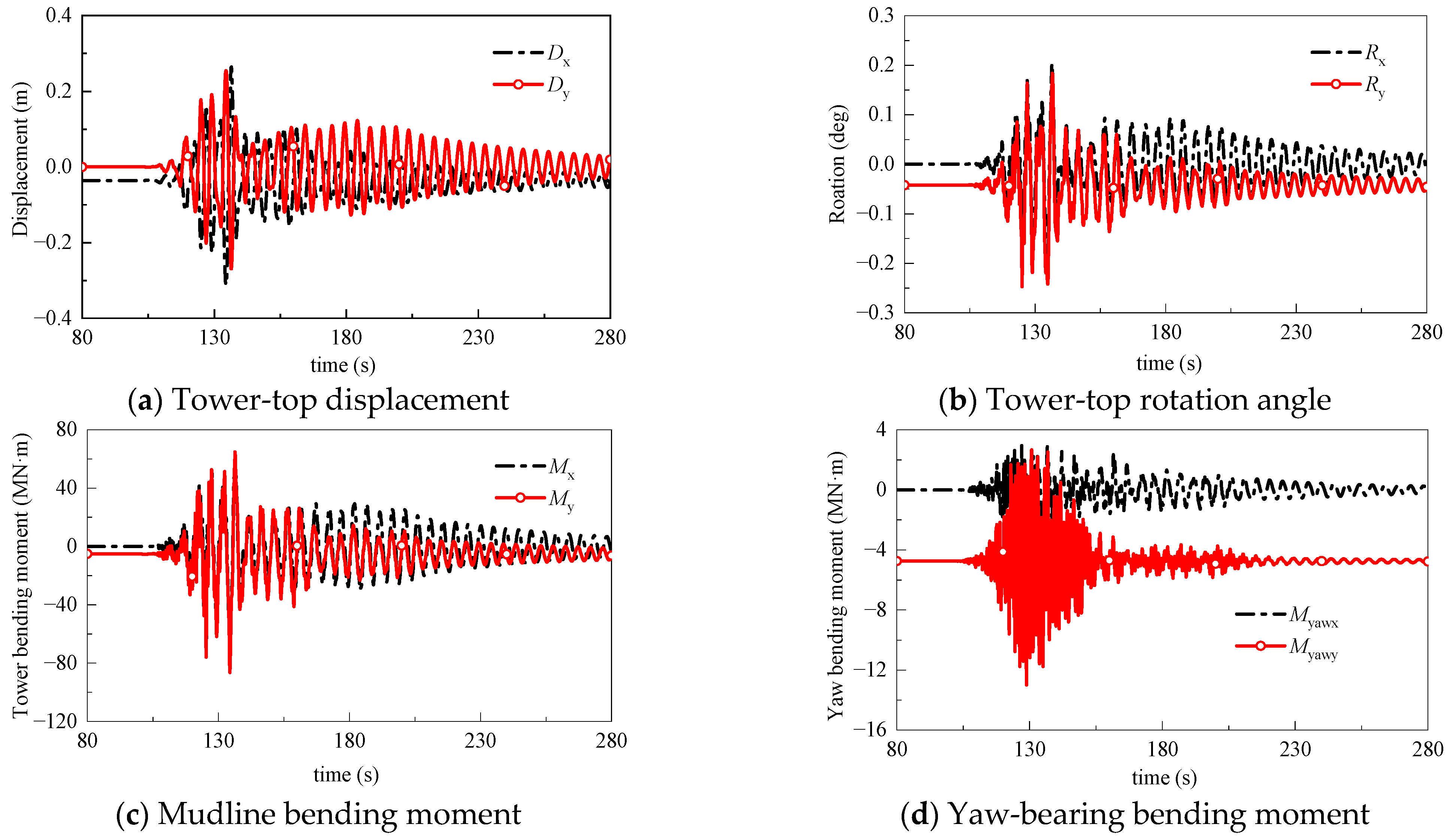
4.3. Seismic Response of 10 MW MOWT in the Parked Condition
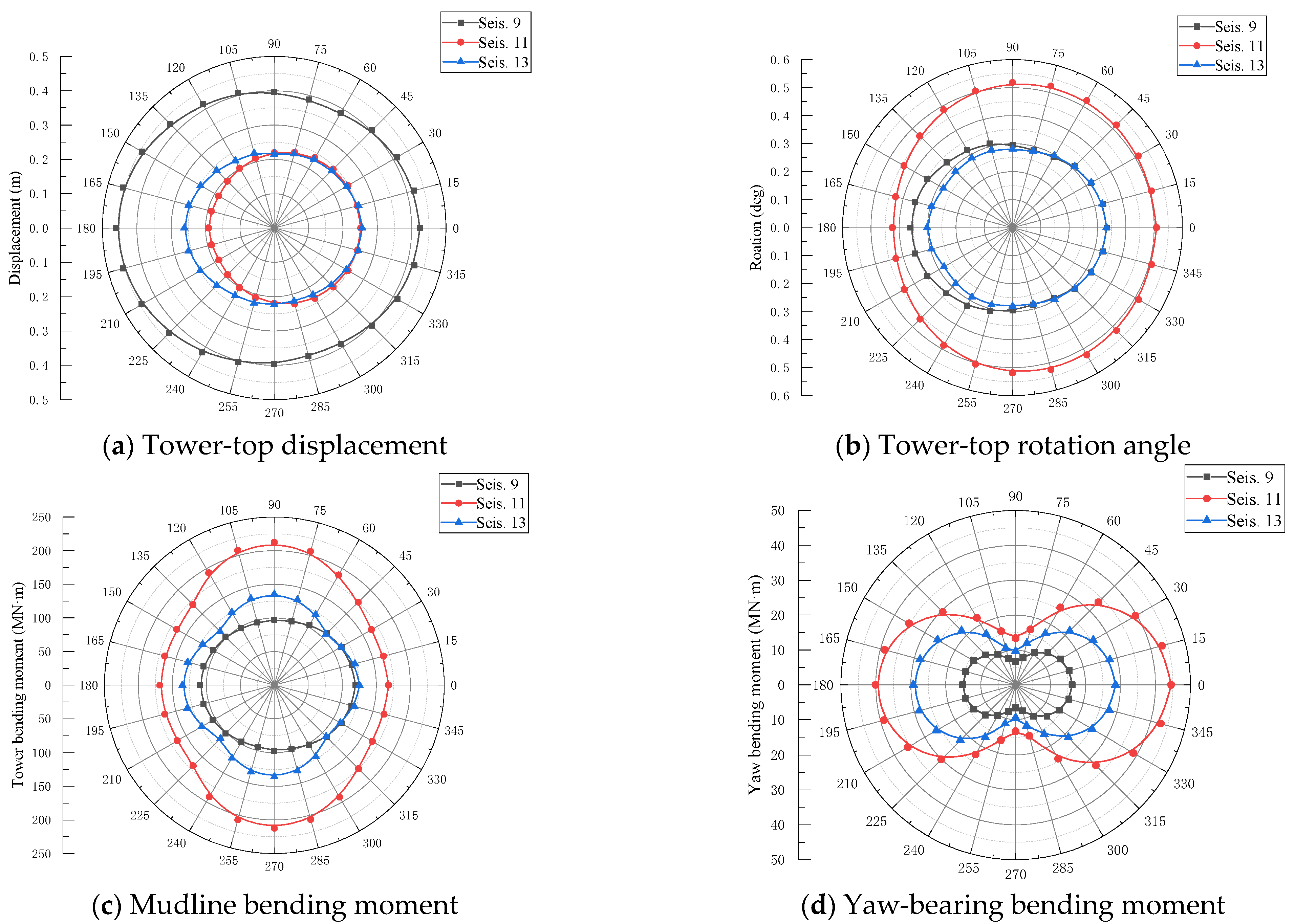
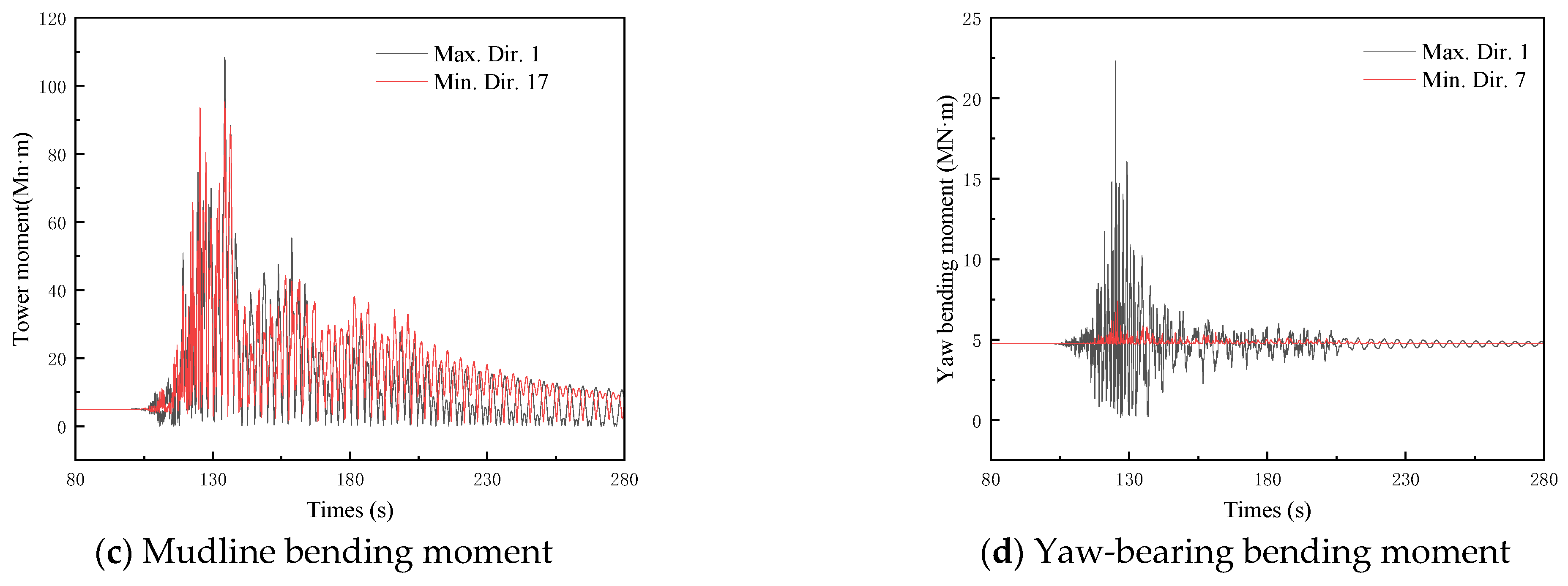
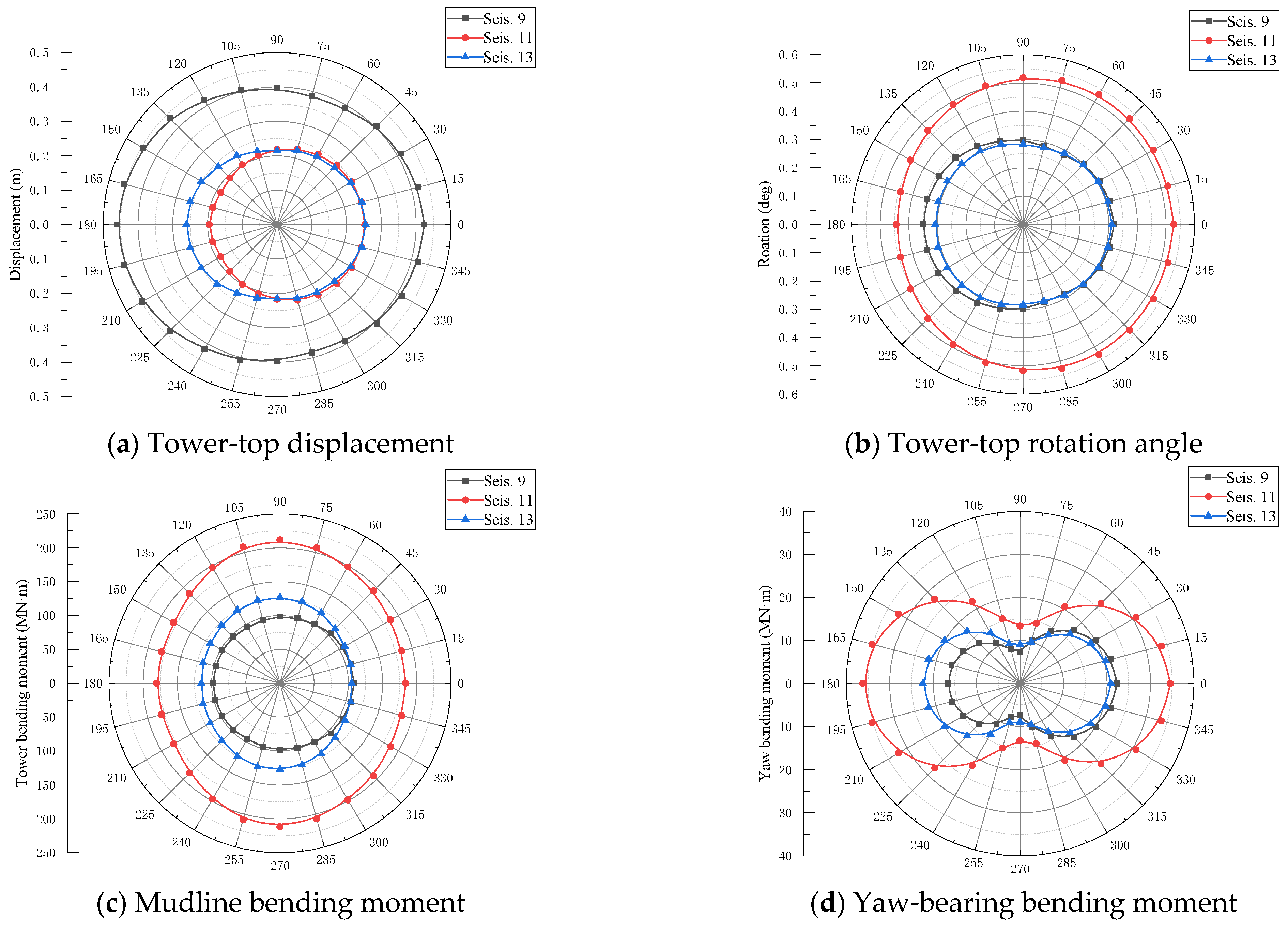
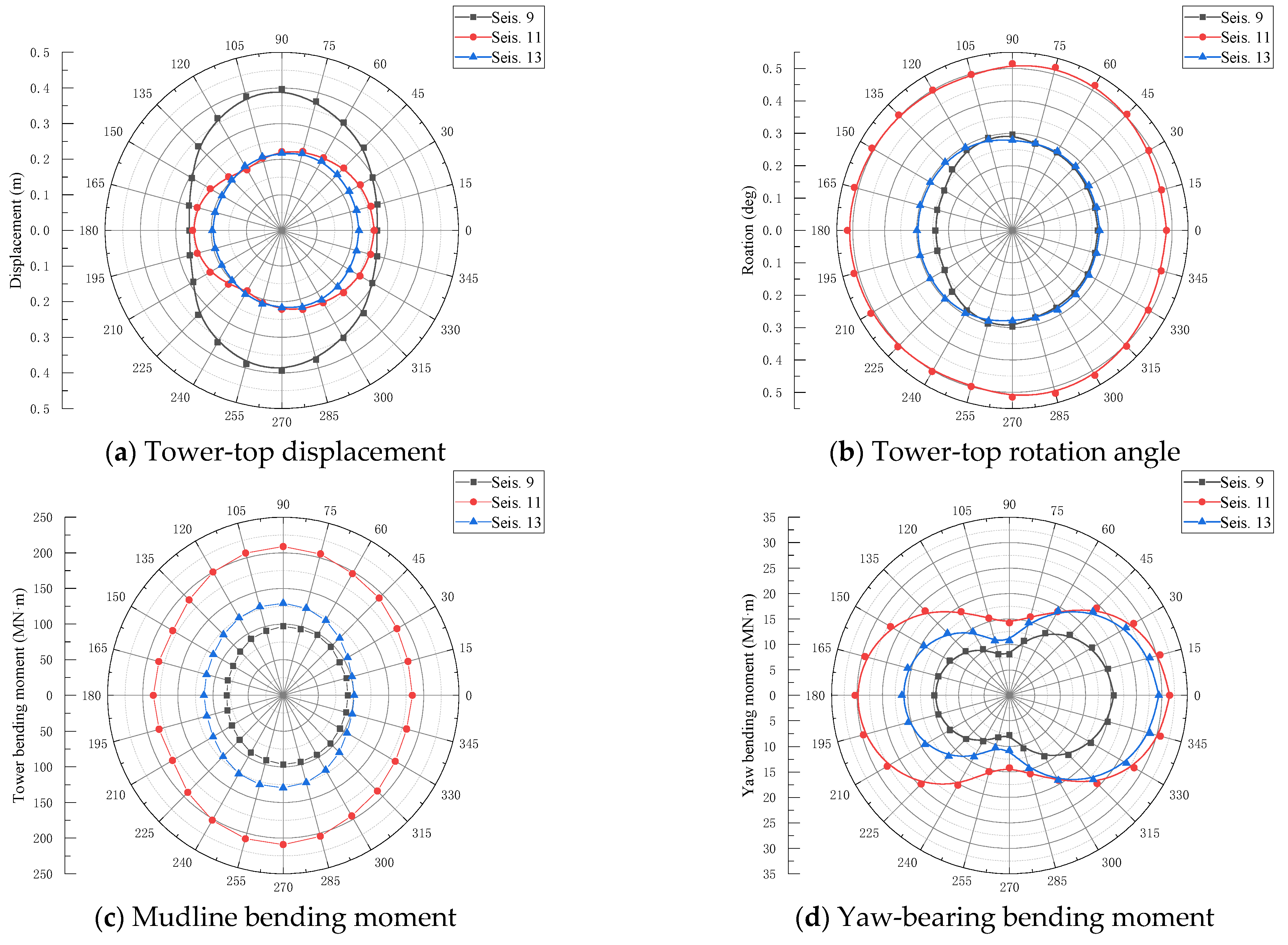
4.3.1. Directional Effect of Seismic Response for the Parked MOWT
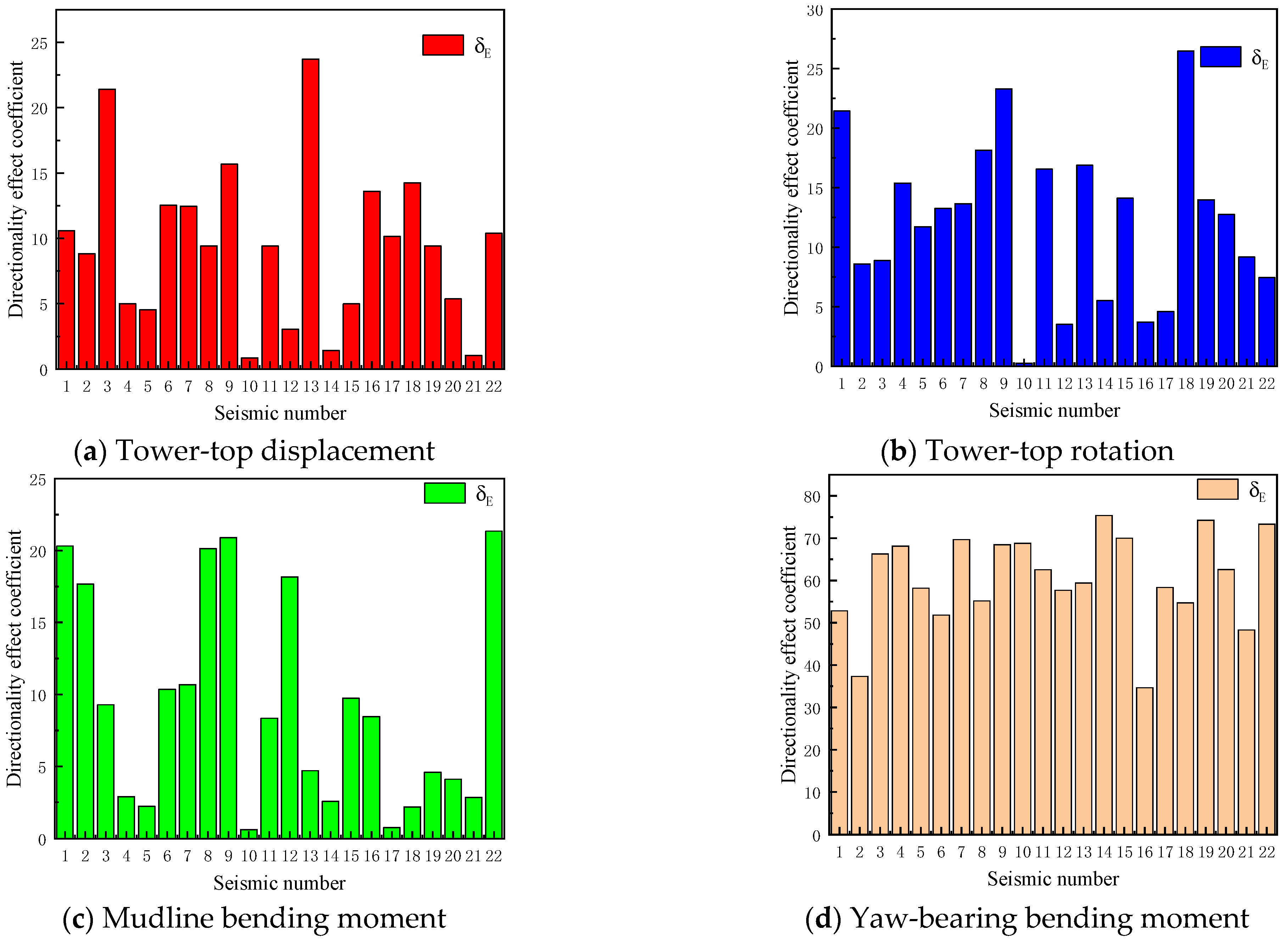
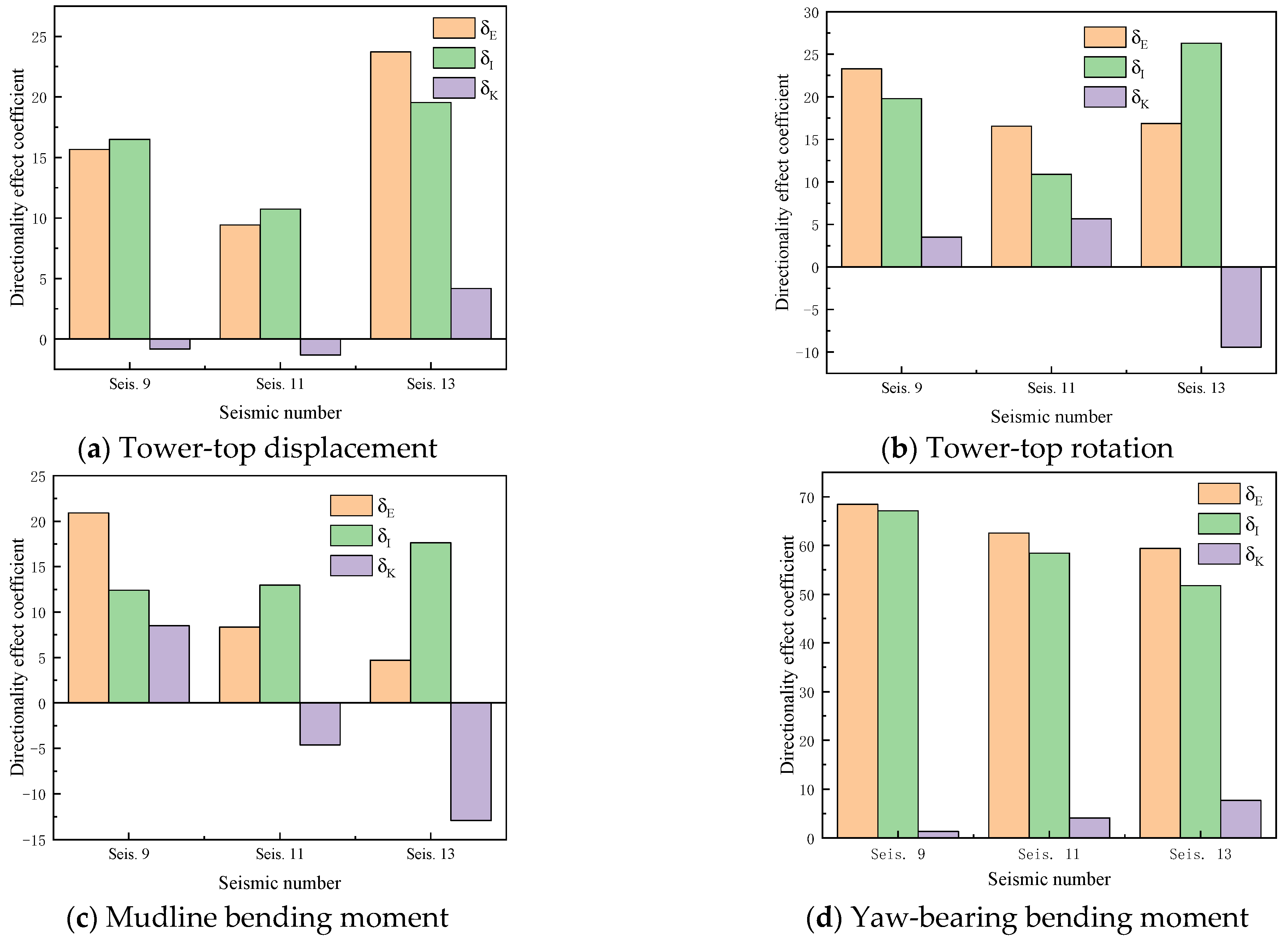
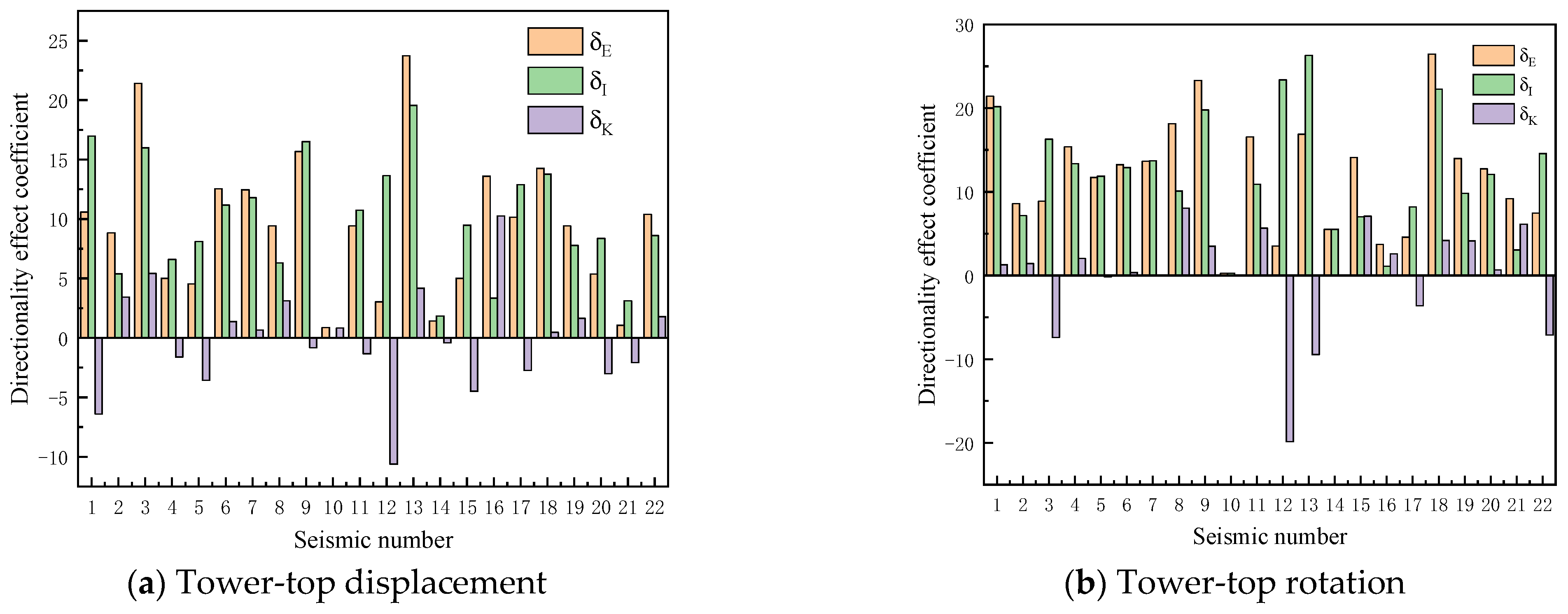
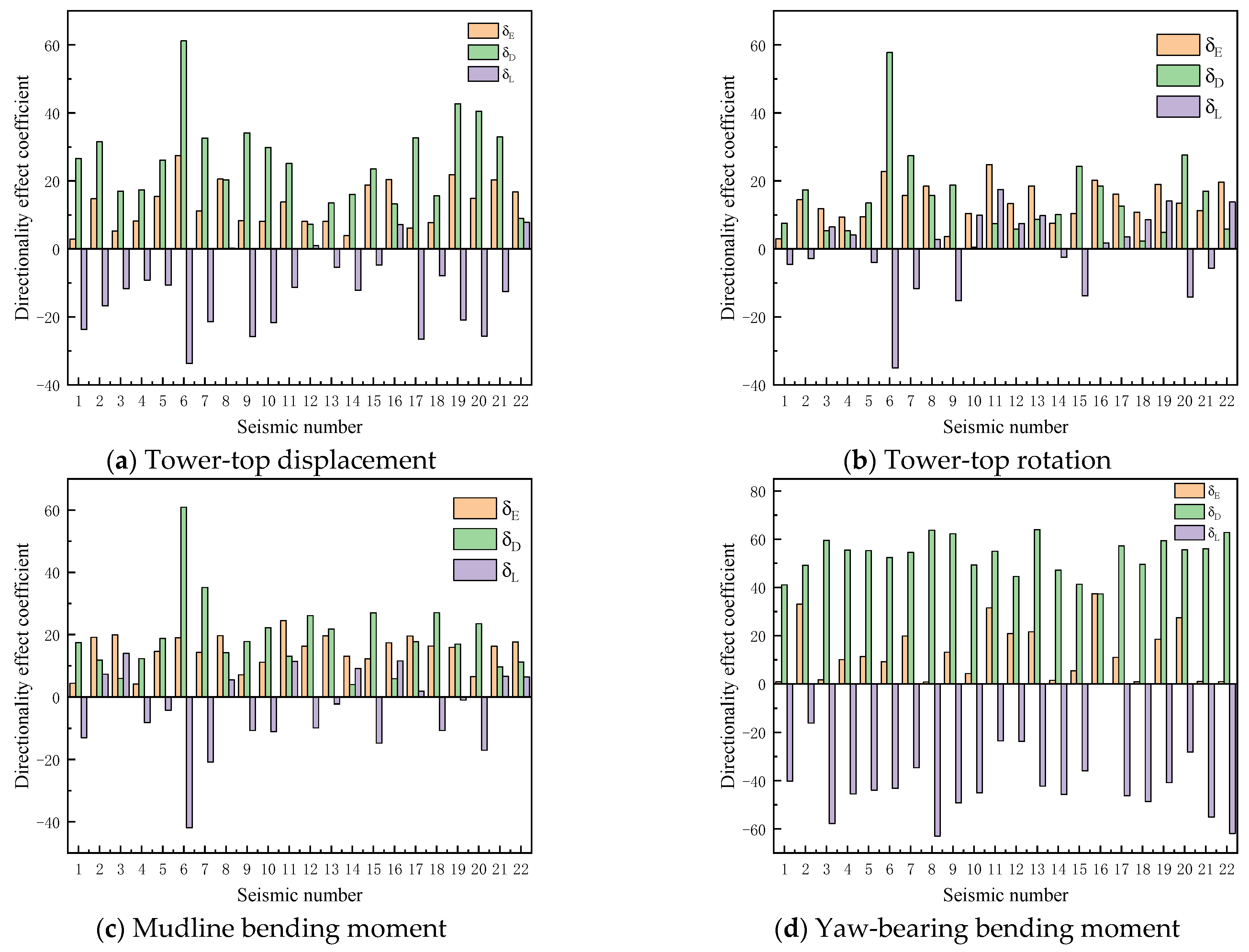
4.3.2. Parametric Study on Seismic Response Directionality for Parked MOWT
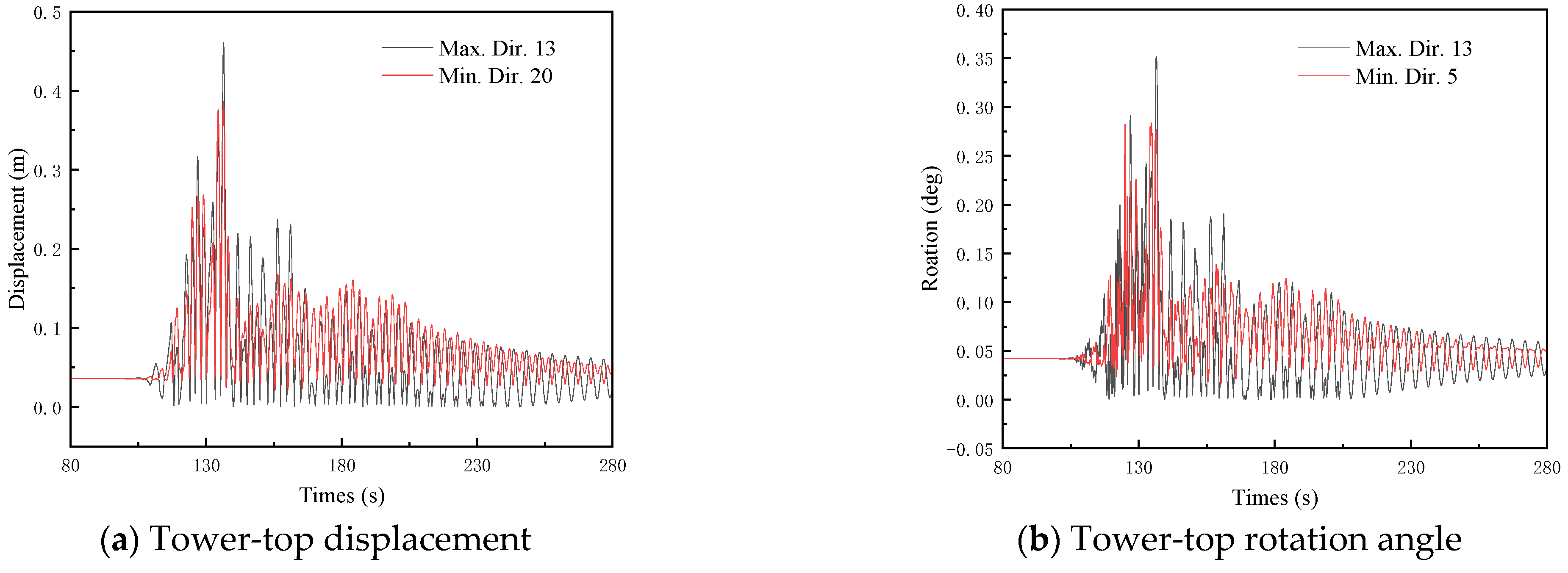
4.4. Seismic Response of 10 MW MOWT in the Running Condition
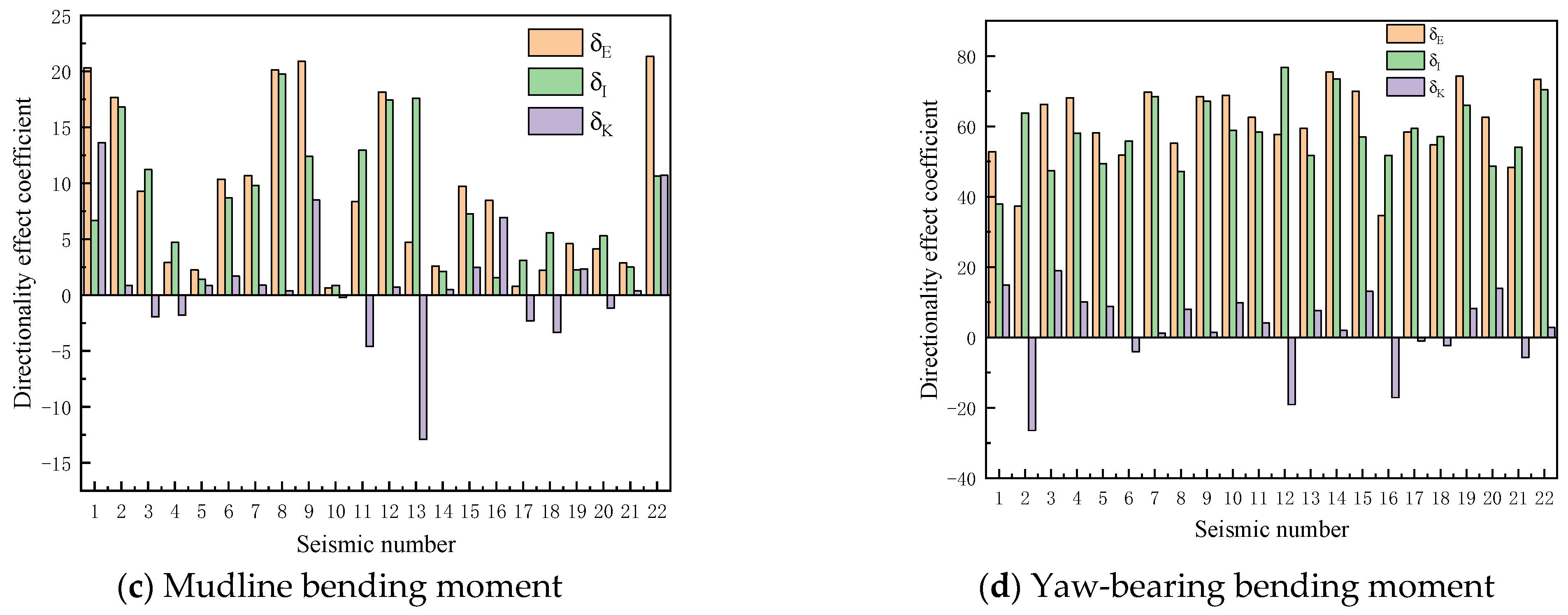
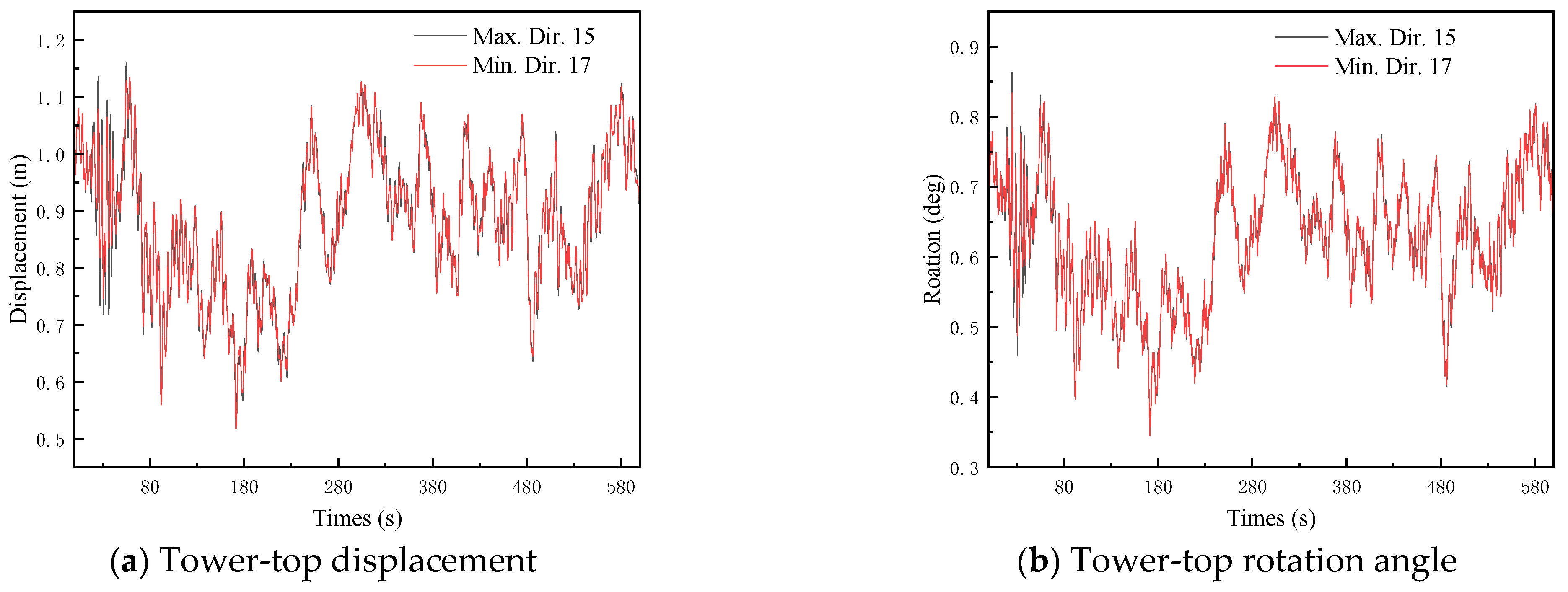
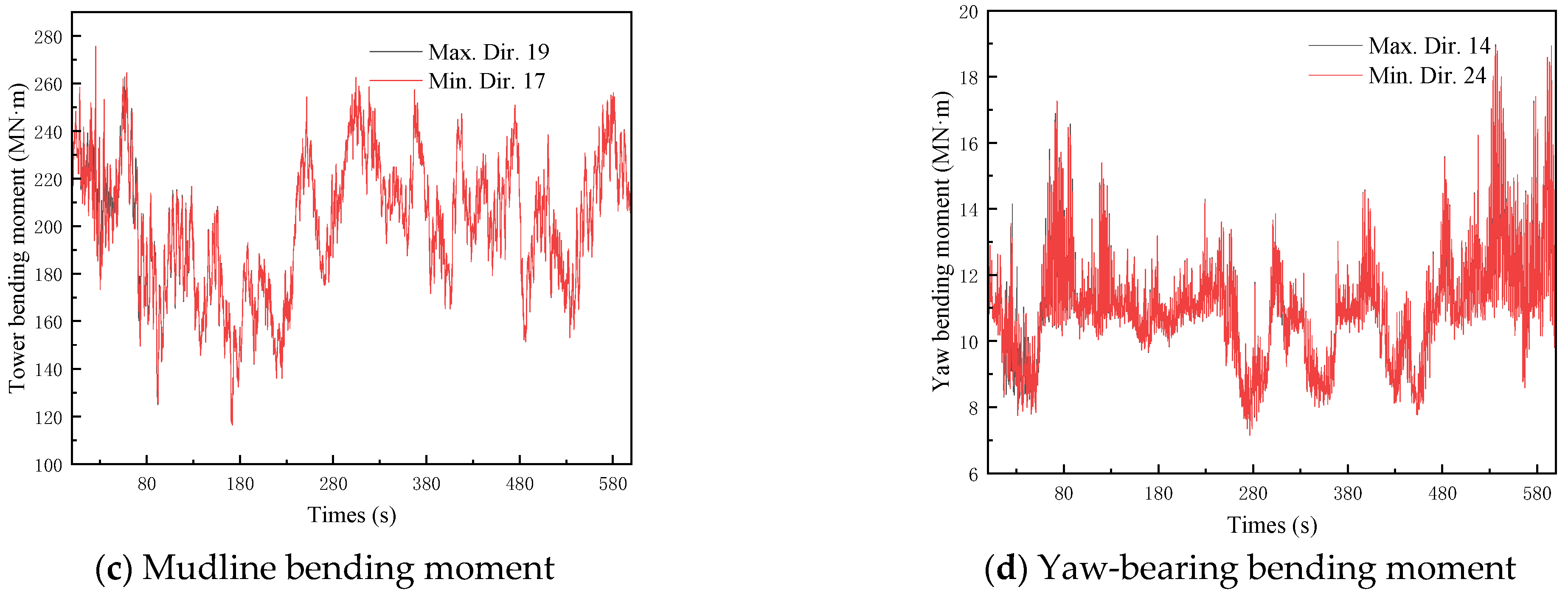
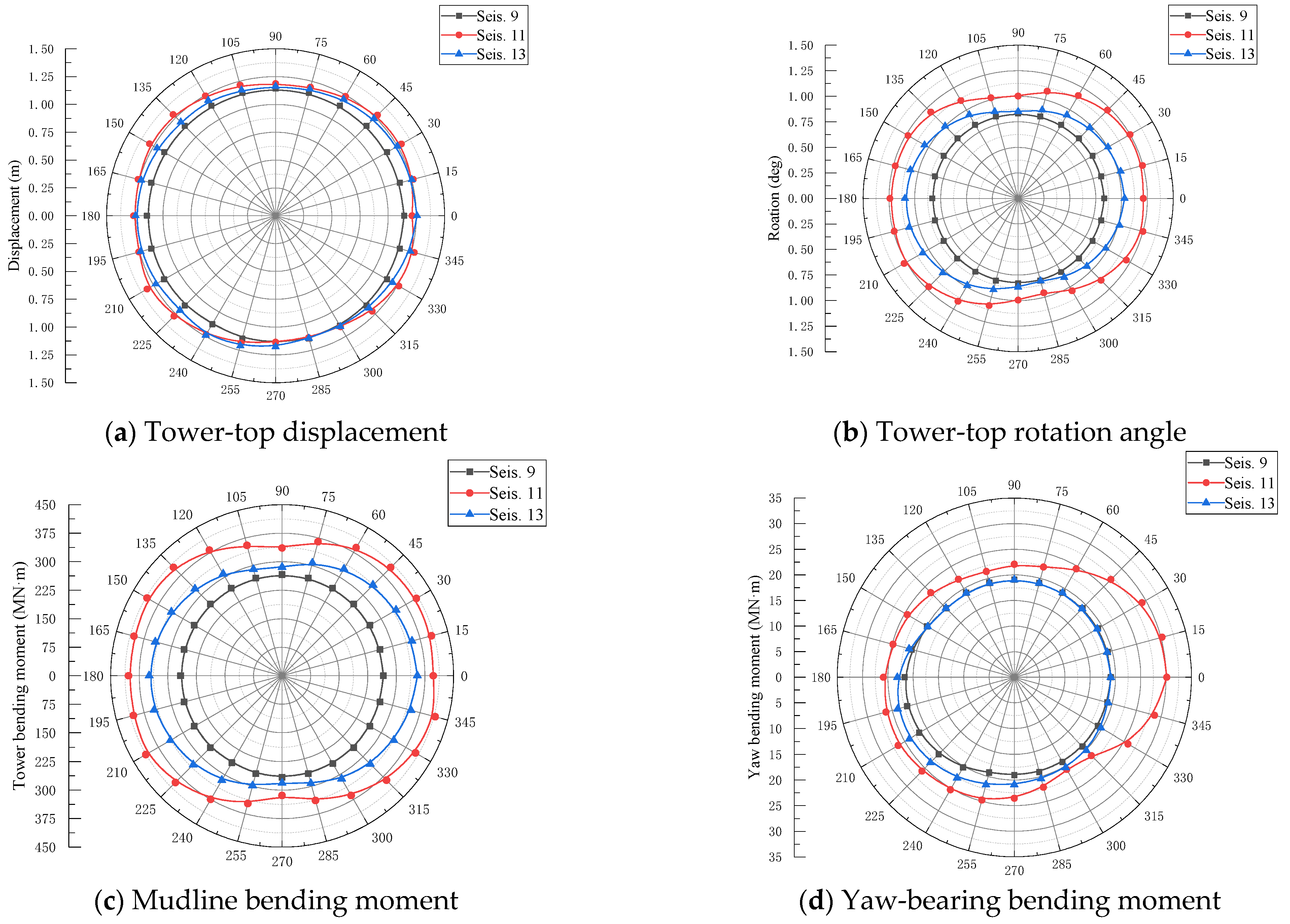
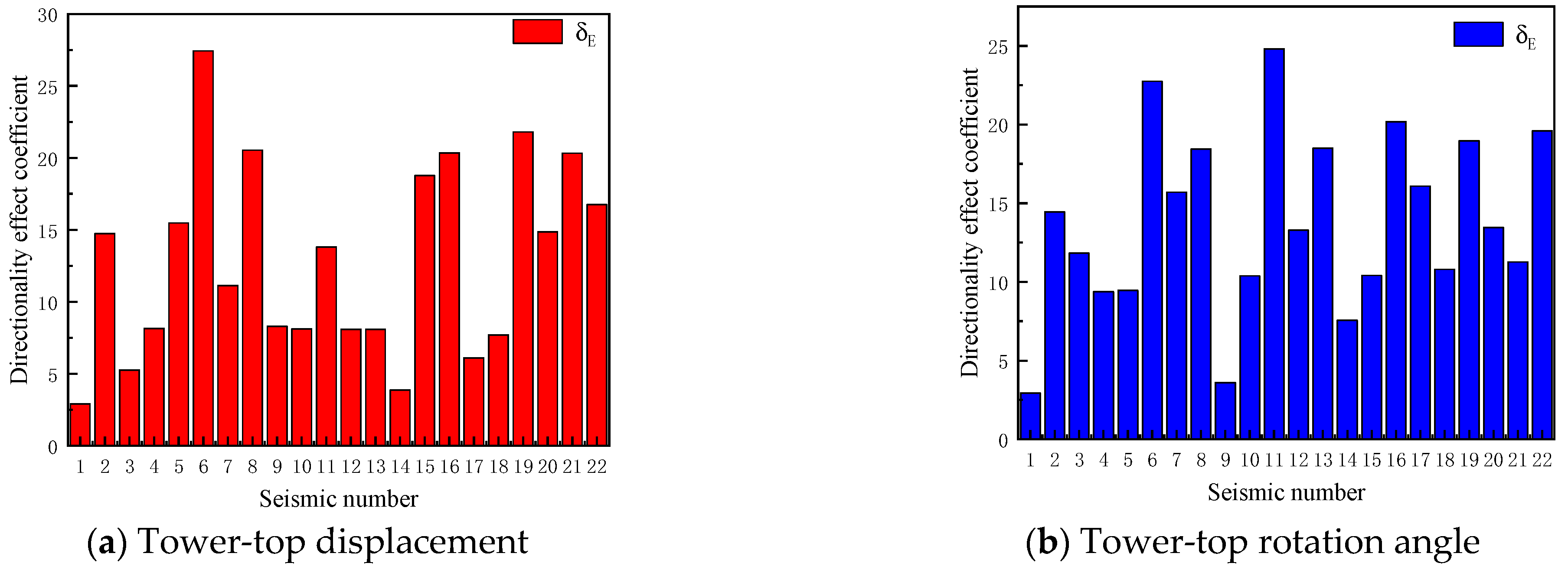
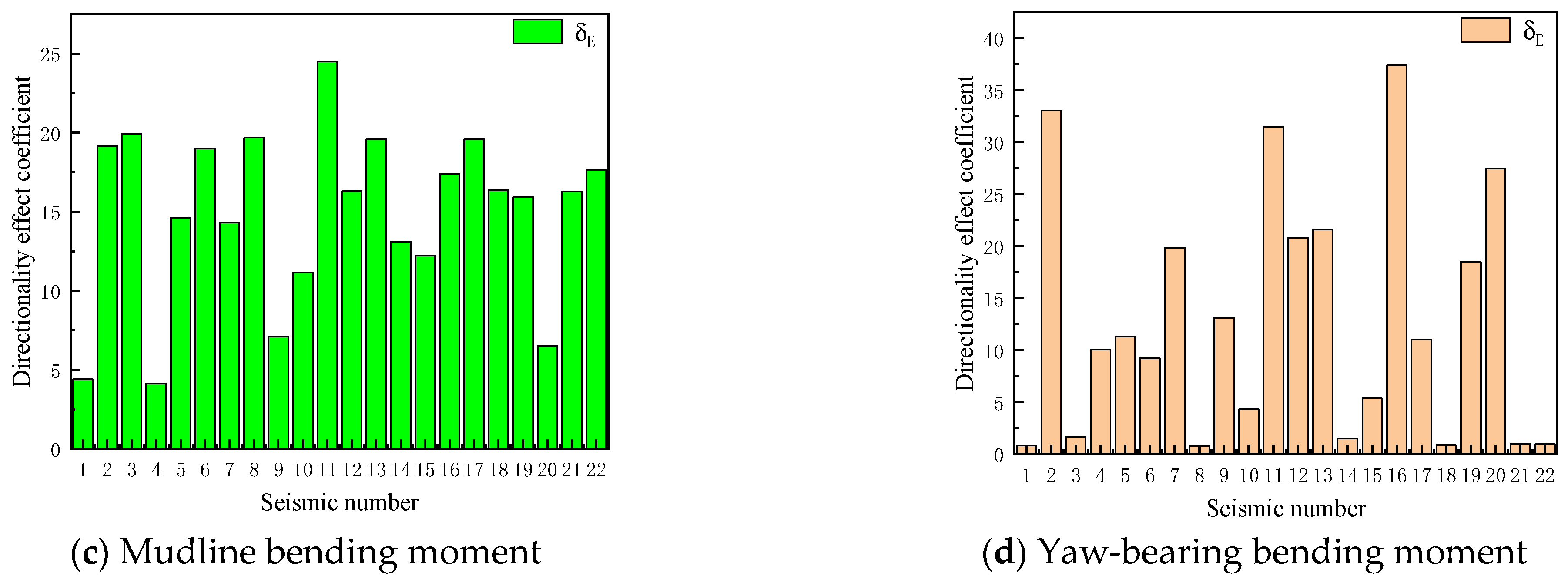
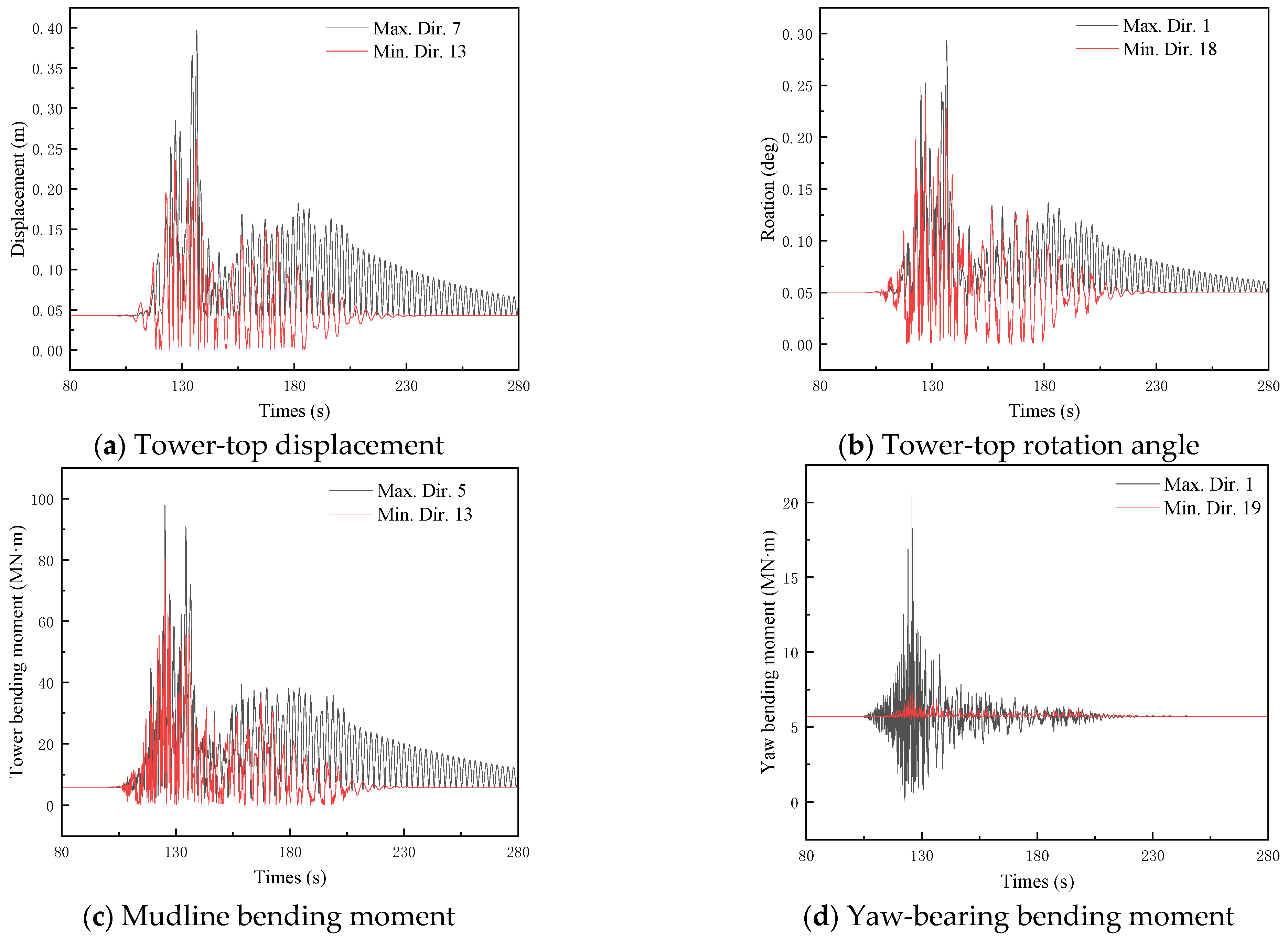
4.4.1. Directional Effect of Seismic Response for the Running MOWT
4.4.2. Parametric Study on Seismic Response Directionality for Running MOWT
5. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- BP. Energy Outlook [EB]; BP, 2023. Available online: https://www.bp.com/en/global/corporate/energy-economics/energy-outlook.html (accessed on 1 April 2025).
- Global Wind Energy Council GWEC. Global Wind Report Annual Market Update 2024; Global Wind Energy Council GWEC, 2024. Available online: https://www.gwec.net/reports/globalwindreport (accessed on 1 April 2025).
- Bhattacharya, S.; Biswal, S.; Aleem, M.; Amani, S.; Prabhakaran, A.; Prakhya, G.; Lombardi, D.; Mistry, H.K. Seismic Design of Offshore Wind Turbines: Good, Bad and Unknowns. Energy 2021, 14, 3496. [Google Scholar] [CrossRef]
- DNV RP 0585. Seismic Design of Wind Power Plants. DET NORSKE VERITAS, 2021. Available online: https://www.dnv.com/energy/standards-guidelines/dnv-rp-0585-seismic-design-of-wind-power-plants/ (accessed on 1 April 2025).
- Zheng, X.Y.; Li, H.; Rong, W.; Li, W. Joint Earthquake and Wave Action on the Monopile Wind Turbine Foundation: An Experimental Study. Mar. Struct. 2015, 44, 125–141. [Google Scholar] [CrossRef]
- Santangelo, F.; Failla, G.; Arena, F.; Ruzzo, C. On Time-Domain Uncoupled Analyses for Offshore Wind Turbines under Seismic Loads. Bull. Earthq. Eng. 2018, 16, 1007–1040. [Google Scholar] [CrossRef]
- Bhattacharya, S.; De Risi, R.; Lombardi, D.; Ali, A.; Demirci, H.E.; Haldar, S. On the Seismic Analysis and Design of Offshore Wind Turbines. Soil. Dyn. Earthq. Eng. 2021, 145, 106692. [Google Scholar] [CrossRef]
- Hacıefendioğlu, K.; Bayraktar, A. Effect of Ice Sheet on Stochastic Response of Offshore Wind Turbine–Seawater–Soil Interaction Systems Subjected to Random Seismic Excitation. J. Cold Reg. Eng. 2011, 25, 115–132. [Google Scholar] [CrossRef]
- Anastasopoulos, I.; Theofilou, M. Hybrid Foundation for Offshore Wind Turbines: Environmental and Seismic Loading. Soil Dyn. Earthq. Eng. 2016, 80, 192–209. [Google Scholar] [CrossRef]
- Bargi, K.; Dezvareh, R.; Mousavi, S.A. Contribution of Tuned Liquid Column Gas Dampers to the Performance of Offshore Wind Turbines under Wind, Wave, and Seismic Excitations. Earthq. Eng. Eng. Vib. 2016, 15, 551–561. [Google Scholar] [CrossRef]
- Wang, W.; Gao, Z.; Li, X.; Moan, T. Model Test and Numerical Analysis of a Multi-Pile Offshore Wind Turbine Under Seismic, Wind, Wave, and Current Loads. J. Offshore Mech. Arct. Eng. 2017, 139, 031901. [Google Scholar] [CrossRef]
- Huang, Y.; Zhao, M.; Wang, P.; Cheng, X.; Du, X. Analytical Solution of Dynamic Responses of Offshore Wind Turbine Supported by Monopile under Combined Earthquake, Wave and Wind. Ocean Eng. 2023, 267, 113319. [Google Scholar] [CrossRef]
- Bozyigit, B.; Bozyigit, I.; Prendergast, L.J. Analytical Approach for Seismic Analysis of Onshore Wind Turbines Considering Soil-Structure Interaction. Structures 2023, 51, 226–241. [Google Scholar] [CrossRef]
- Kourkoulis, R.S.; Lekkakis, P.C.; Gelagoti, F.M.; Kaynia, A.M. Suction Caisson Foundations for Offshore Wind Turbines Subjected to Wave and Earthquake Loading: Effect of Soil–Foundation Interface. Géotechnique 2014, 64, 171–185. [Google Scholar] [CrossRef]
- Ding, H.; Xiong, K.; Zhang, P. Seismic Response of Offshore Wind Structure Supported by Bucket Foundation. Trans. Tianjin Univ. 2016, 22, 294–301. [Google Scholar] [CrossRef]
- Zayed, M.; Kim, K.; Prabhakaran, A.; Elgamal, A. Shake Table Testing and Computational Framework for Seismic Response of Utility-Scale Bucket Foundation Offshore Wind Turbines. Soil Dyn. Earthq. Eng. 2023, 171, 107939. [Google Scholar] [CrossRef]
- Xu, Y.; Shen, T.; Zuo, J.; Bhattacharya, S.; Han, Q. Shaking Table Tests and Numerical Analysis of Monopile-Supported Offshore Wind Turbines Under Combined Wind, Wave and Seismic Loads. Soil Dyn. Earthq. Eng. 2024, 183, 108793. [Google Scholar] [CrossRef]
- Sahraeian, S.M.S.; Masoumi, M.A.; Najafgholipour, M.A.; Shafiee, A.; Pandey, B. Seismic Response of Monopile Foundation of Offshore Wind Turbines Under Near-Field and Far-Field Ground Motions. Soil Dyn. Earthq. Eng. 2023, 174, 108166. [Google Scholar] [CrossRef]
- Ngo, D.-V.; Kim, D.-H. Seismic Responses of Different Types of Offshore Wind Turbine Support Structures. Ocean Eng. 2024, 297, 117108. [Google Scholar] [CrossRef]
- Kim, D.H.; Lee, S.G.; Lee, I.K. Seismic Fragility Analysis of 5 MW Offshore Wind Turbine. Renew. Energy 2014, 65, 250–256. [Google Scholar] [CrossRef]
- Mardfekri, M.; Gardoni, P. Probabilistic Demand Models and Fragility Estimates for Offshore Wind Turbine Support Structures. Eng. Struct. 2013, 52, 478–487. [Google Scholar] [CrossRef]
- Mardfekri, M.; Gardoni, P. Multi-hazard Reliability Assessment of Offshore Wind Turbines. Wind Energy 2015, 18, 1433–1450. [Google Scholar] [CrossRef]
- Yan, Y.; Li, C.; Li, Z. Buckling Analysis of a 10 MW Offshore Wind Turbine Subjected to Wind-Wave-Earthquake Loadings. Ocean Eng. 2021, 236, 109452. [Google Scholar] [CrossRef]
- Jiang, W.; Lin, C. Lateral Responses of Monopile-Supported Offshore Wind Turbines in Sands Under Combined Effects of Scour and Earthquakes. Soil Dyn. Earthq. Eng. 2022, 155, 107193. [Google Scholar] [CrossRef]
- Zhang, T.; Wang, W.; Li, X.; Wang, B. Vibration Mitigation in Offshore Wind Turbine Under Combined Wind-Wave-Earthquake Loads Using the Tuned Mass Damper Inerter. Renew. Energy 2023, 216, 119050. [Google Scholar] [CrossRef]
- Wang, P.-G.; Bai, Y.; Wang, M.; Zhao, M.; Du, X.-L. Seismic Vulnerability and Performance-Based Assessment of Monopile Offshore Wind Turbine Considering Turbine Blades. Soil Dyn. Earthq. Eng. 2024, 186, 108918. [Google Scholar] [CrossRef]
- Huang, H.S. Simulations of 10MW Wind Turbine under Seismic Loadings. Compos. Struct. 2022, 279, 114686. [Google Scholar] [CrossRef]
- Lu, Y.; Xie, W.; Wang, Y.; Liang, H.; He, Y.; Chen, X.; Yuan, J.; Zhang, Z. Dynamic Analysis of MTMD Vibration Reduction for Offshore Wind Turbine Under Combined Wind-Wave-Seismic Loads. Structures 2025, 72, 108207. [Google Scholar] [CrossRef]
- Penzien, J.; Watabe, M. Characteristics of 3-Dimensional Earthquake Ground Motions. Earthq. Eng. Struct. Dyn. 1974, 3, 365–373. [Google Scholar] [CrossRef]
- Rigato, A.B.; Medina, R.A. Influence of Angle of Incidence on Seismic Demands for Inelastic Single-Storey Structures Subjected to Bi-Directional Ground Motions. Eng. Struct. 2007, 29, 2593–2601. [Google Scholar] [CrossRef]
- Soltanieh, S.; Memarpour, M.M.; Kilanehei, F. Performance Assessment of Bridge-Soil-Foundation System with Irregular Configuration Considering Ground Motion Directionality Effects. Soil Dyn. Earthq. Eng. 2019, 118, 19–34. [Google Scholar] [CrossRef]
- Arany, L.; Bhattacharya, S.; Adhikari, S.; Hogan, S.J.; Macdonald, J.H.G. An Analytical Model to Predict the Natural Frequency of Offshore Wind Turbines on Three-Spring Flexible Foundations Using Two Different Beam Models. Soil Dyn. Earthq. Eng. 2015, 74, 40–45. [Google Scholar] [CrossRef]
- Koukoura, C.; Natarajan, A.; Vesth, A. Identification of Support Structure Damping of a Full Scale Offshore Wind Turbine in Normal Operation. Renew. Energy 2015, 81, 882–895. [Google Scholar] [CrossRef]
- Alati, N.; Failla, G.; Arena, F. Seismic Analysis of Offshore Wind Turbines on Bottom-Fixed Support Structures. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2015, 373, 20140086. [Google Scholar] [CrossRef]
- Wang, L.; Dong, X.T. Influence of Earthquake Directions on Wind Turbine Tower under Seismic Action. Adv. Mater. Res. 2011, 243–249, 3883–3888. [Google Scholar] [CrossRef]
- Dai Kaoshan, M.Z.; Zhi, Z. Shaking Table Test Study on Seismic Responses of a Wind Turbine under Ground Motions with Different Spectral Characteristics. Adv. Eng. Sci. 2018, 50, 125–133. [Google Scholar]
- XI, R.; XU, C.; DU, X.; XU, K. Effects of Operating Conditions on the Seismic Response of Wind Turbines. Eng. Mech. 2019, 36, 80–88. [Google Scholar]
- Yang, Y.; Ye, K.; Li, C.; Michailides, C.; Zhang, W. Dynamic Behavior of Wind Turbines Influenced by Aerodynamic Damping and Earthquake Intensity. Wind Energy 2018, 21, 303–319. [Google Scholar] [CrossRef]
- Mo, R.; Cao, R.; Liu, M.; Li, M. Effect of Ground Motion Directionality on Seismic Dynamic Responses of Monopile Offshore Wind Turbines. Renew. Energy 2021, 175, 179–199. [Google Scholar] [CrossRef]
- Romero-Sánchez, C.; Padrón, L.A. Influence of Wind and Seismic Ground Motion Directionality on the Dynamic Response of Four-Legged Jacket-Supported Offshore Wind Turbines. Eng. Struct. 2024, 300, 117191. [Google Scholar] [CrossRef]
- Xi, R.; Du, X.; Xu, C.; Xu, K. Effects of Ground Motion Input Direction on Dynamic Response of Offshore Wind Turbine. J. Vib. Shock 2021, 40, 193–201. [Google Scholar]
- Bak, C.; Zahle, F.; Bitsche, R.; Kim, T.; Yde, A.; Henriksen, L.C.; Hansen, M.H.; Blasques, J.P.A.A.; Gaunaa, M.; Natarajan, A. The DTU 10-MW Reference Wind Turbine. Proc. Dan. Wind Power Res. 2013, 2013, 1–21. [Google Scholar]
- Xi, R.; Du, X.; Wang, P.; Xu, C.; Zhai, E.; Wang, S. Dynamic Analysis of 10 MW Monopile Supported Offshore Wind Turbine Based on Fully Coupled Model. Ocean Eng. 2021, 234, 109346. [Google Scholar] [CrossRef]
- Sun, Y.; Xu, C.; Du, X.; Du, X.; Xi, R. A Modified P-y Curve Model of Large-Monopiles of Offshore Wind Power Plants. Eng. Mech. 2021, 38, 44–53. [Google Scholar] [CrossRef]
- API. Recommended Practice for Planning, Design and Constructing Fixed Offshore Platforms-Working Stress Design. In API Recommended Practice 2A-WSD, 21st ed.; American Petroleum Institute: Washington, DC, USA, 2007. [Google Scholar]
- IEC 61400-3 Wind Turbines Part 3: Design Requirements for Offshore Wind Turbines; International Electrotechnical Commission: Geneva, Switzerland, 2009.
- IEC 61400-1 Wind Turbines Part 1: Design Requirements; International Electrotechnical Commission: Geneva, Switzerland, 2014.
- Hasselmann, K.; Barnett, T.P.; Bouws, E.; Carlson, H.; Cartwright, D.E.; Enke, K.; Ewing, J.A.; Gienapp, A.; Hasselmann, D.E.; Kruseman, P. Measurements of Wind-Wave Growth and Swell Decay during the Joint North Sea Wave Project (JONSWAP). Ergaenzungsheft Dtsch. Hydrogr. Z. Reihe A 1973. Available online: https://repository.tudelft.nl/record/uuid:f204e188-13b9-49d8-a6dc-4fb7c20562fc (accessed on 1 April 2025).
- Beer, F.P.; Johnston, E.R., Jr.; DeWolf, J.T.; Mazurek, D.F. Mecânica Dos Materiais; McGraw Hill Brasil: New York, NY, USA, 2011. [Google Scholar]
- Xi, R.; Wang, P.; Du, X.; Xu, K.; Xu, C.; Jia, J. A Semi-Analytical Model of Aerodynamic Damping for Horizontal Axis Wind Turbines and Its Applications. Ocean Eng. 2020, 214, 107861. [Google Scholar] [CrossRef]
- Øye, S. Tjæreborg Wind Turbine: 4th Dynamic Inflow Measurement; Technical report VK-204; Technical University of Denmark: Lyngby, Denmark, 1991. [Google Scholar]
- Hansen, M.H.; Gaunaa, M.; Madsen, H.A. A Beddoes-Leishman Type Dynamic Stall Model in State-Space and Indicial Formulations; Forskningscenter Riso: Roskilde, Denmark, 2004; ISBN 8755030904. [Google Scholar]
- Hansen, M. Aerodynamics of Wind Turbines; Routledge: London, UK, 2015; ISBN 1315769980. [Google Scholar]
- Li, Q.; Yang, W. An Improved Method of Hydrodynamic Pressure Calculation for Circular Hollow Piers in Deep Water under Earthquake. Ocean Eng. 2013, 72, 241–256. [Google Scholar] [CrossRef]
- Lai, Y.; Li, W.; He, B.; Xiong, G.; Xi, R.; Wang, P. Influence of Blade Flexibility on the Dynamic Behaviors of Monopile-Supported Offshore Wind Turbines. J. Mar. Sci. Eng. 2023, 11, 2041. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, Q.; Fan, J. The Maximum Structural Response of Structures under Bi-Directional Esrthquake Ground Motions. Eng. Mech. 2012, 29, 129–136. [Google Scholar]
- Athanatopoulou, A.M. Critical Orientation of Three Correlated Seismic Components. Eng. Struct. 2005, 27, 301–312. [Google Scholar] [CrossRef]








| Property, Unit | Value |
|---|---|
| Rated power, MW | 10 |
| Rotor orientation, number of blades | Downwind, 3 |
| Rotor diameter, m | 178.3 |
| Hub hiameter, m | 5.6 |
| Hub height, m | 119 |
| Rated wind speed, m/s | 11.4 |
| Rotor mass (including hub and blades), kg | 227,962 |
| Nacelle mass, kg | 446,036 |
| Tower mass, kg | 628,442 |
| Direction | Moment of Inertia (kg·m2) |
|---|---|
| ζζ | 165,423,200 |
| ηη | 103,705,202 |
| Vhub (m/s) | 2 | 5 | 7 | 9 | 11 | 13 | 15 | 18 | 21 | 24 |
| HS (m) | 1.0 | 1.1 | 1.2 | 1.4 | 1.5 | 1.8 | 2.0 | 2.4 | 2.9 | 3.4 |
| TP (s) | 6.0 | 5.8 | 5.7 | 5.7 | 5.8 | 5.9 | 6.2 | 6.7 | 7.0 | 7.8 |
| ID. | Event, Year | Station/Component | ID. | Event, Year | Station/Component |
|---|---|---|---|---|---|
| 1 | Duzce, 1999 | Bolu/000 | 12 | Landers, 1992 | Yermo Fire Station/270 |
| 2 | Loma Prieta, 1989 | Capitola/000 | 13 | Mexico, 2010 | Cerro Prieto Geothermal, geo000 |
| 3 | Chi-Chi, 1999 | CHY101/E | 14 | Darfield, 2010 | Christchurch Hospital, hcs89w |
| 4 | Imperial Valley, 1979 | Delta/262 | 15 | Duzce, 1999 | Duzce/180-pulse |
| 5 | Kocaeli, 1999 | Duzce/180 | 16 | Erzican, 1992 | Erzincan/s |
| 6 | Loma Prieta, 1989 | Gilroy Array-3/090 | 17 | Superstition Hills-02, 1987 | Parachute Test Site/225 |
| 7 | Northridge, 1994 | Canyon Country-WLC/000 | 18 | Northridge-01, 1994 | Rinaldi Receiving Sta/228 |
| 8 | San Fernando, 1971 | LA-Hollywood Stor./090 | 19 | Chi-Chi, 1999 | TCU065/E |
| 9 | Northridge, 1994 | Beverly Hills-Mulhol/009 | 20 | Imperial Valley-06, 1979 | Chihuahua/282 |
| 10 | Cape Mendocino, 1992 | Rio Dell Overpass/270 | 21 | Northridge-01, 1994 | Northridge-Saticoy/090 |
| 11 | Kobe, 1995 | Shin-Osaka/000 | 22 | Kocaeli, 1999 | Yarimca/330 |
| Load Cases | Operational Condition | Load Model | Blade Model |
|---|---|---|---|
| 1 | Parked | Seismic only | Original |
| 2 | Parked | Seismic only | Eliminate stiffness disparity |
| 3 | Running | Wind, wave, seismic | Original |
| 4 | Running | Seismic, additional aerodynamic damping | Original |
| Mode | This Study (Hz) | Benchmark [55] (Hz) | Error% |
|---|---|---|---|
| 1st Tower Fore–Aft | 0.2172 | 0.2138 | 1.5903% |
| 2nd Tower Fore–Aft | 1.4297 | 1.3817 | 3.4740% |
| 3rd Tower Fore–Aft | 2.258 | - | - |
| 4th Tower Fore–Aft | 5.684 | - | - |
| 1st Tower Side-to-Side | 0.2161 | 0.2152 | 0.4182% |
| 2nd Tower Side-to-Side | 1.2439 | 1.2496 | −0.4561% |
| 3rd Tower Side-to-Side | 2.258 | - | - |
| 4th Tower Side-to-Side | 5.684 | - | - |
| Mode | This Study (Hz) | Benchmark [42] (Hz) | Error% |
|---|---|---|---|
| 1st Blade Flap-wise | 0.597 | 0.61 | 2.18% |
| 2st Blade Flap-wise | 1.693 | 1.74 | 2.77% |
| 1st Blade Edge-wise | 0.906 | 0.93 | 2.64% |
| 2st Blade Edge-wise | 2.707 | 2.76 | 1.91% |
| Vwind (m/s) | 5 | 8 | 11.4 | 14 | 17 | 25 |
| Tower top fore–aft viscous damping coefficient (N·(ms−1)−1) | 108,867 | 118,676 | 169,595 | 181,354 | 180,160 | 169,281 |
| Tower top side–side viscous damping coefficient (N·(ms−1)−1) | 479 | 395 | 571 | 4725 | 8174 | 16,442 |
| Damping coefficient of the rotational damper about the x-axis (N·(ms−1)−1) | 858,494 | 2,401,654 | 3,407,045 | 15,725,672 | 32,187,204 | 81,256,801 |
| Damping coefficient of the rotational damper about the y-axis (N·(ms−1)−1) | 160,969,251 | 174,395,265 | 249,262,290 | 276,907,019 | 276,576,002 | 265,802,816 |
| Direction | Input Angle (deg) | Direction | Input Angle (deg) | Direction | Input Angle (deg) | Direction | Input Angle (deg) |
|---|---|---|---|---|---|---|---|
| 1 | 0 | 7 | 90 | 13 | 180 | 19 | 270 |
| 2 | 15 | 8 | 105 | 14 | 195 | 20 | 285 |
| 3 | 30 | 9 | 120 | 15 | 210 | 21 | 300 |
| 4 | 45 | 10 | 135 | 16 | 225 | 22 | 315 |
| 5 | 60 | 11 | 150 | 17 | 240 | 23 | 330 |
| 6 | 75 | 12 | 165 | 18 | 255 | 24 | 345 |
| Seismic Number | TTD () (%) | Most Critical Direction (deg) | TTR () (%) | Most Critical Direction (deg) | MBM () (%) | Most Critical Direction (deg) | YBM () (%) | Most Critical Direction (deg) |
|---|---|---|---|---|---|---|---|---|
| 9 | 15.7 | 180 | 23.3 | 180 | 20.9 | 0 | 68.5 | 0 |
| 11 | 9.4 | 0 | 16.6 | 300 | 8.4 | 90 | 62.5 | 0 |
| 13 | 23.7 | 180 | 16.8 | 0 | 4.7 | 270 | 59.4 | 180 |
| Seismic Number | TTD () (%) | Most Critical Direction (deg) | TTR () (%) | Most Critical Direction (deg) | MBM () (%) | Most Critical Direction (deg) | YBM () (%) | Most Critical Direction (deg) |
|---|---|---|---|---|---|---|---|---|
| 9 | 16.5 | 180 | 19.8 | 180 | 12.4 | 0 | 67.1 | 0 |
| 11 | 10.7 | 0 | 10.9 | 300 | 12.9 | 90 | 58.4 | 180 |
| 13 | 19.5 | 180 | 26.3 | 0 | 17.6 | 270 | 51.7 | 180 |
| Seismic Number | TTD () (%) | Most Critical Direction (deg) | TTR () (%) | Most Critical Direction (deg) | MBM () (%) | Most Critical Direction (deg) | YBM () (%) | Most Critical Direction (deg) |
|---|---|---|---|---|---|---|---|---|
| 9 | 1.5 | 210 | 0.6 | 225 | 1.1 | 270 | 13.1 | 195 |
| 11 | 13.8 | 210 | 24.8 | 210 | 24.5 | 345 | 31.5 | 15 |
| 13 | 9.1 | 0 | 23.7 | 180 | 20.6 | 0 | 21.6 | 210 |
| Seismic Number | TTD () (%) | Most Critical Direction (deg) | TTR () (%) | Most Critical Direction (deg) | MBM () (%) | Most Critical Direction (deg) | YBM () (%) | Most Critical Direction (deg) |
|---|---|---|---|---|---|---|---|---|
| 9 | 34.1 | 90 | 18.8 | 255 | 17.8 | 75 | 62.3 | 0 |
| 11 | 25.1 | 0 | 7.4 | 75 | 13.1 | 270 | 55.0 | 0 |
| 13 | 13.5 | 285 | 8.7 | 195 | 21.8 | 90 | 63.9 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xi, R.; Zhou, Q.; Lai, Y.; Yu, W. Investigation of Directionality Effect for 10 MW Monopile Offshore Wind Turbine Excited by Wind, Wave, and Earthquakes. J. Mar. Sci. Eng. 2025, 13, 727. https://doi.org/10.3390/jmse13040727
Xi R, Zhou Q, Lai Y, Yu W. Investigation of Directionality Effect for 10 MW Monopile Offshore Wind Turbine Excited by Wind, Wave, and Earthquakes. Journal of Marine Science and Engineering. 2025; 13(4):727. https://doi.org/10.3390/jmse13040727
Chicago/Turabian StyleXi, Renqiang, Qingxuan Zhou, Yongqing Lai, and Wanli Yu. 2025. "Investigation of Directionality Effect for 10 MW Monopile Offshore Wind Turbine Excited by Wind, Wave, and Earthquakes" Journal of Marine Science and Engineering 13, no. 4: 727. https://doi.org/10.3390/jmse13040727
APA StyleXi, R., Zhou, Q., Lai, Y., & Yu, W. (2025). Investigation of Directionality Effect for 10 MW Monopile Offshore Wind Turbine Excited by Wind, Wave, and Earthquakes. Journal of Marine Science and Engineering, 13(4), 727. https://doi.org/10.3390/jmse13040727





