An Experimental Study on the Seismic Response of Vertical and Batter Pile Foundations at Coral Sand Sites

Abstract

Liquefaction and earthquake damage to coral sand sites can cause engineering structure failure. Both testing and analyzing the seismic response characteristics of pile groups on coral sand sites are highly important for the seismic design of engineering structures. To address the lack of research on the seismic dynamic response of group pile foundations in coral sand sites, this study analyzes the characteristics of the seismic dynamic response of vertical and batter pile foundations for bridges in coral sand liquefaction foundations via the shaking table model test and investigates the variation patterns of acceleration, excess pore water pressure (EPWP), and the bending moment and displacement of foundations, soil, and superstructures under different vibration intensities. Results show that the excitation wave type significantly affects liquefaction: at 0.1 g of peak acceleration, only high-frequency sine wave tests liquefied, with small EPWP ratios, while at 0.2 g, all tests liquefied. Vertical pile foundations had lower soil acceleration than batter piles due to differences in bearing mechanisms. Before liquefaction, batter piles had smaller EPWP ratios but experienced greater bending moments under the same horizontal force. Overall, batter piles showed higher dynamic stability and anti-tilt capabilities but endured larger bending moments compared to vertical piles in coral sand foundations. In conclusion, batter pile foundations demonstrate superior seismic performance in coral sand sites, offering enhanced stability and resistance to liquefaction-induced failures.

1. Introduction

Coral sand, primarily composed of coral and shell fragments, is a natural sediment widely distributed between 30°N and 30°S latitude [1]. Coral sand ground, as special geological environments for the construction of oceanic islands and reefs worldwide, is widely attracting the attention of the engineering and scientific communities [2,3,4,5]. Moreover, the construction area of China’s islands and reefs is located at the intersection of the Eurasian, Pacific, and Indian plates and the surrounding areas, which is a high-risk area for strong earthquakes [6]. Therefore, liquefaction and seismic damage to coral sand sites have become the focus of current attention.

To explore the unique liquefaction characteristics of coral sand, many scholars have studied the strength, deformation, and pore pressure development of saturated coral sand under dynamic loading via methods such as dynamic triaxial tests [7,8,9,10], which have shown that coral sand has liquefaction characteristics and that the liquefaction process of coral sand is more complicated and difficult than that of terrestrial sand. However, these studies using unit tests have focused mainly on the liquefaction characteristics of the coral sand itself, neglecting their performance in practical marine engineering applications. As an important basic material, coral sand is widely used in marine engineering structures such as buildings, breakwaters, port docks, and airport runways. These structures are inevitably subjected to waves, earthquakes, and other loads during actual use, which may trigger coral sand liquefaction.

Pile foundations, owing to their high load-bearing capacity, low settlement, and strong seismic resistance, are widely applicable to various geological conditions, especially in areas with soft soil layers, unstable soil masses, or regions prone to earthquakes. However, soil–pile–structure interactions in liquefied foundations are extremely complex and involve multiple aspects, such as inertial interactions between the structure and the pile foundation, dynamic interactions between the pile and the soil, pore pressures induced by earthquakes, and nonlinear soil responses. Shaking table tests are important methods for studying the dynamic response of various structures and foundations at liquefiable sites under the effects of earthquakes [11]. Scholars have carried out a series of experimental studies on the dynamic responses of pile foundations in quartz sands, soft soils, and clays under the effects of earthquakes [12,13,14,15]. However, coral sand has unique physical properties, including irregular particle shapes, high susceptibility to crushing, and significant porosity, which distinguish it from typical engineering materials in terms of strength, deformation behavior, and permeability characteristics. These distinctive features give rise to distinct liquefaction mechanisms in coral sand deposits [16,17]. Wu et al. [17,18,19] conducted shaking table model tests to analyze the dynamic response of pile groups in coral sand foundations under different ground vibration intensities and showed that under the same relative density and similar particle size distribution, quartz sand is more prone to liquefaction than coral sand, and its shear transfer ability is almost completely lost after liquefaction. However, shaking table tests on coral sand pile foundations are not common, especially for batter pile foundations in coral sand.

The batter pile foundation has excellent horizontal bearing performance [20], but few studies have reported the dynamic response of batter pile foundations in coral sand liquefaction foundations. The influence of pile–soil interactions on the overall seismic performance of the foundation system in liquefied coral sand remains insufficiently explored, requiring further qualitative investigation. This study conducted a series of shaking table model tests to investigate the dynamic response characteristics of coral sand foundations and bridge pile foundations. The research focused on analyzing the dynamic response patterns of vertical and batter pile foundations under varying ground vibration intensities. Additionally, this study compared the differences in dynamic properties, such as acceleration and dynamic bending moments, between the two types of pile group foundations. This study aims to deepen the understanding of pile–soil interactions in liquefied coral sand and contribute to the development of more resilient foundation design strategies for marine engineering applications.

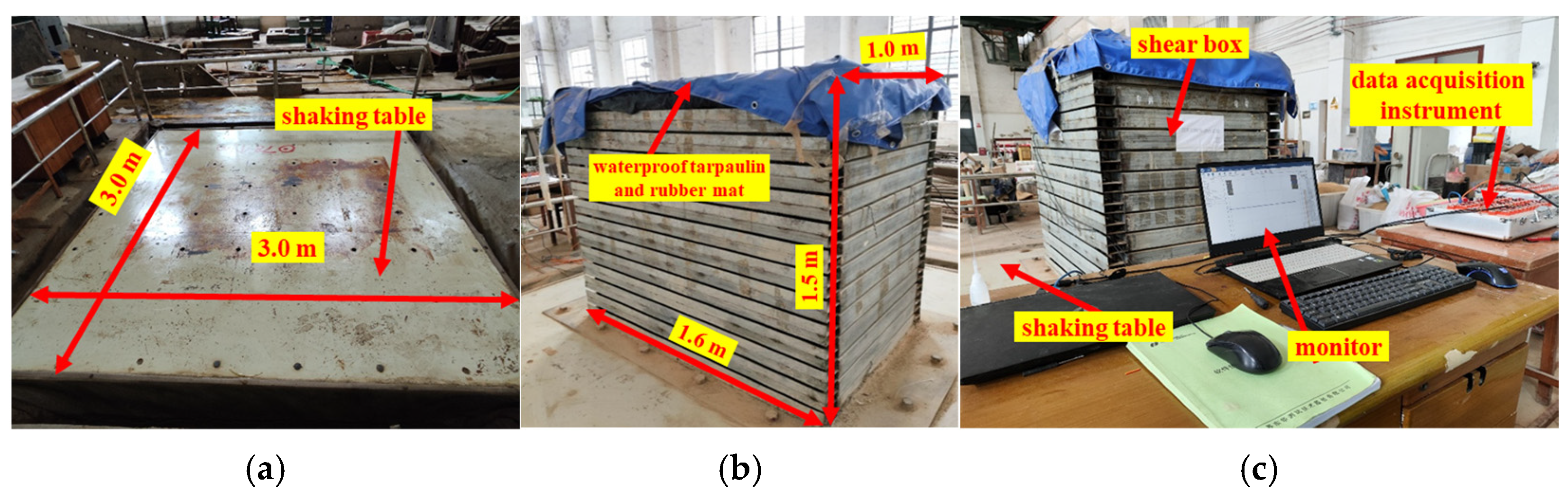
2. Experimental Methods

2.1. Experimental Design

The shaking table tests is carried out in the Seismic Laboratory of Guangxi University. The shaking table, as well as the laminar shear box adopted, is shown in Figure 1. The size of the table is 3 × 3 m; the table can carry a maximum of 10 tons; the maximum loading acceleration is ±2.0 g in a single horizontal direction, and the operating frequency is 0–40 Hz. The length, width, and height of the laminar shear box (Figure 1b) are 1.6 m, 1.0 m, and 1.5 m, respectively. A 10 cm foam board is installed on the inner walls of the model box to mitigate the reflection of seismic waves by the layered steel shear box, thereby reducing the impact on the test results.

This test is based on an actual continuous beam bridge as a prototype, which has a pile diameter of 1.0 m and a pile length of 20.0 m; a bearing platform with a length of 8.0 m, a width of 5.5 m, and a height of 2.8 m; an abutment with a height of 10.0 m; and a bridge deck with a length of 31.0 m and a width of 8.0 m. The bridge structure is made of reinforced concrete with a density of 2550 kg/m³ and an elastic modulus of 30 GPa.

In accordance with the Buckingham π theorem and related research [21,22], the experiment selects the geometric dimension L, elastic modulus E, and equivalent mass density ρ as the basic physical quantities. On the basis of the carrying capacity of the shaking table and the size of the shearing box, the geometric similarity ratio (S_L) used in the experiment is 1:50. Owing to the small size of the model structure, it is difficult to cast the model with concrete, and to simplify the test, plexiglass, which has the advantage of isotropy, is used as the material for making the scaled-down model of the bridge structure, with a density of 1100 kg/m³. This simplified method has also been adopted in many scholars’ shaking table tests with satisfactory results [23]. The elastic modulus of the plexiglass is 2.1 GPa.

Under the condition of an acceleration similarity ratio of S_a = 1.0, the equivalent mass density similarity ratio S_ρ is calculated using Equation (1), where the elastic modulus similarity ratio S_E is the ratio of the elastic modulus of the model material to that of the concrete material used in the prototype structure. The similarity relationships and ratios for the other physical quantities are shown in

Table 1. Similarity ratios for the shaking table model tests.

Physical Quantity	Physical Symbol	Similarity Relationship	Similarity Coefficient
Length	L	$S_L$	1:50
Elastic Modulus	E	$S_E$	1:15
Equivalent Density	ρ	$S_{\rho }$	10:3
Acceleration	A	$S_a=S_E{S_L}^{-1}{S_{\rho }}^{-1}$	1
Time	t	$S_t={S_E}^{-{\displaystyle \frac{1}{2}}}{S}_L{S_{\rho }}^{{\displaystyle \frac{1}{2}}}$	0.141
Frequency	f	$S_f={S_t}^{-1}$	7.09
Stress	σ	$S_{\sigma }=S_E$	1:15

.

$$S_a={\displaystyle \frac{S_E}{S_{\mathsf{\rho }}{S}_L}}$$

(1)

In the experiment, the model employed the method of added mass to satisfy the density similarity relationship, compensating for the deficiencies in inertial and gravitational effects. According to the mass similarity constant and Formula (1), it is known that

$$S_m=S_E{S_L}^2$$

(2)

The required additional mass for counterweighting can be derived as follows:

$$m_a=S_E{S_L}^2{m}_p-m_m$$

(3)

where $m_a$ is the added mass of the model, $m_p$ is the mass of the prototype, and $m_m$ is the mass of the model itself.

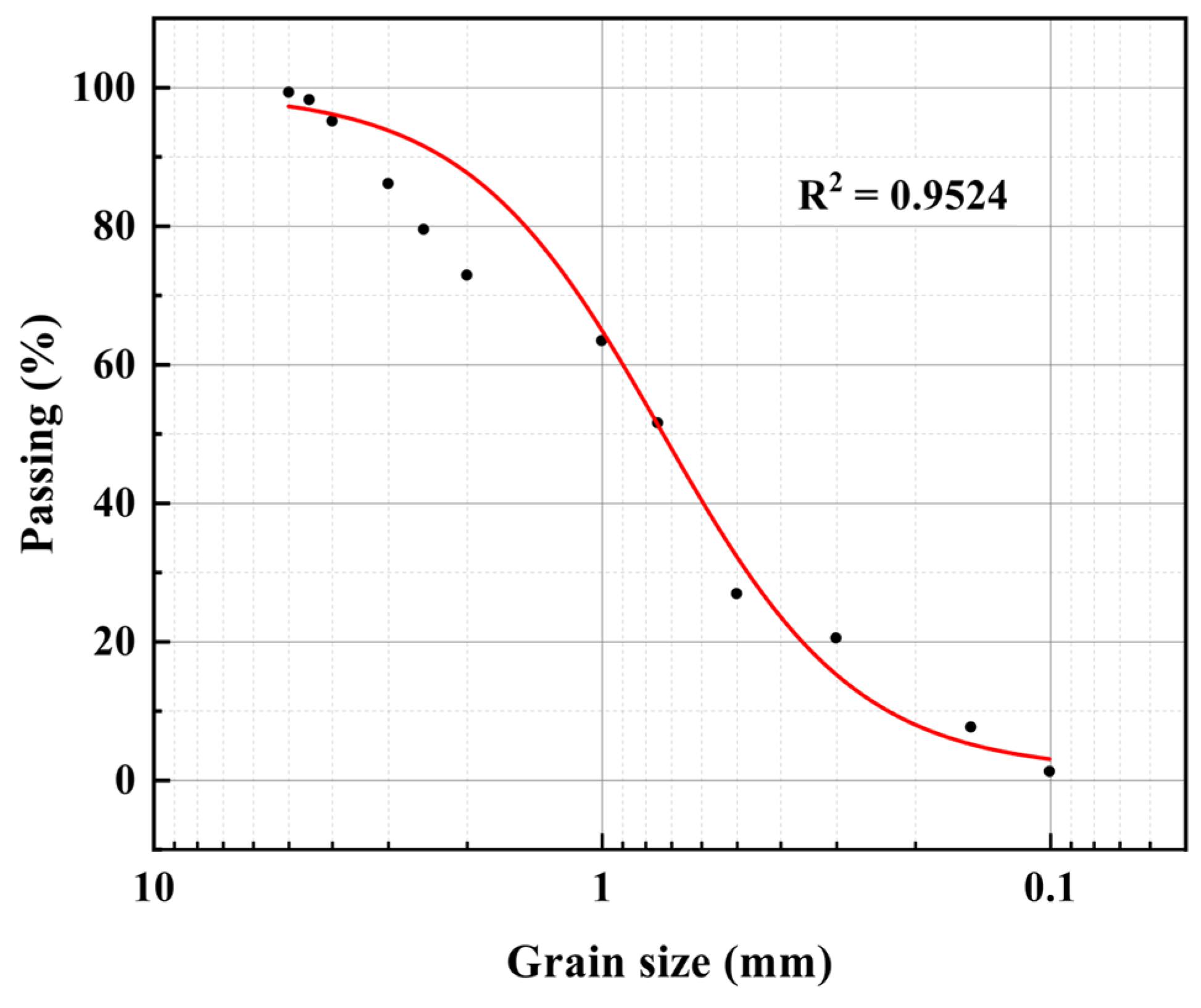
2.2. Model Foundation Materials

There are practical difficulties in the similarity ratio design of soil bodies, and the purpose of this test is to reveal the seismic liquefaction characteristics of coral sand ground. Therefore, this study does not consider the similarity ratio design of the soil body but uses prototype coral sand [24]. The coral sand used in the test was taken from an island in the South China Sea. The average particle diameter D₅₀ was 0.75 mm; the nonuniform coefficient C_u was 5.33; the relative particle density Gs was 2.79; the maximum dry density ${\rho }_{d,max}$ was 1.72 g/cm³, and the minimum dry density ${\rho }_{d,\min }$ was 1.25 g/cm³. The grain-size distribution curve of the coral sand is shown in Figure 2. The coral sand used in the experiment has a particle diameter of more than 50% of the total mass with a diameter greater than or equal to 0.25 mm, classifying it as medium-coarse coral sand.

The model foundation was constructed using the sand immersion method, ensuring uniform layering. During the filling process, the water level was consistently maintained approximately 10 cm above the sand surface, and the sand was slowly sprinkled into the water. Once the foundation filling was complete, calibrated aluminum boxes were used for sampling and analysis to ensure that the relative density of the sand layers, D_r, reached 40%. After completing the model’s fabrication, the model foundation was placed in a fully saturated state for 24 h, allowing it to be considered fully consolidated and saturated [25].

2.3. Bridge Model

Numerous studies have indicated that batter pile foundations can enhance the seismic performance of foundations [20,26]. Therefore, two types of pile group foundations, vertical piles (VPs) and batter piles (BPs), were constructed, as shown in Figure 3, and their performances in coral sand ground under seismic action were compared through experiments. The diameter of the bridge piles is d = 24 mm, and the inclination angle of the batter piles is 10°. To better reflect the seismic interaction relationship of the bridge structure, the two piers and the bridge deck are set up as an articulated system (as shown in Figure 3), thereby achieving relative motion between the piers and the bridge deck to a certain extent. Specifically, a spherical articulated structure is employed to realize a separable connection between the abutments and the bridge deck, and this concave–convex fit of the spherical nodes allows for rotations and slight displacements in multiple directions, thus more realistically simulating the relative displacements and rotations of the structure under seismic action.

Despite the constraints of the 1:50 scaling ratio and simplified models, this study offers qualitative insights into the pile behavior during liquefaction but fails to consider critical factors like pile interactions and soil–structure interface complexities.

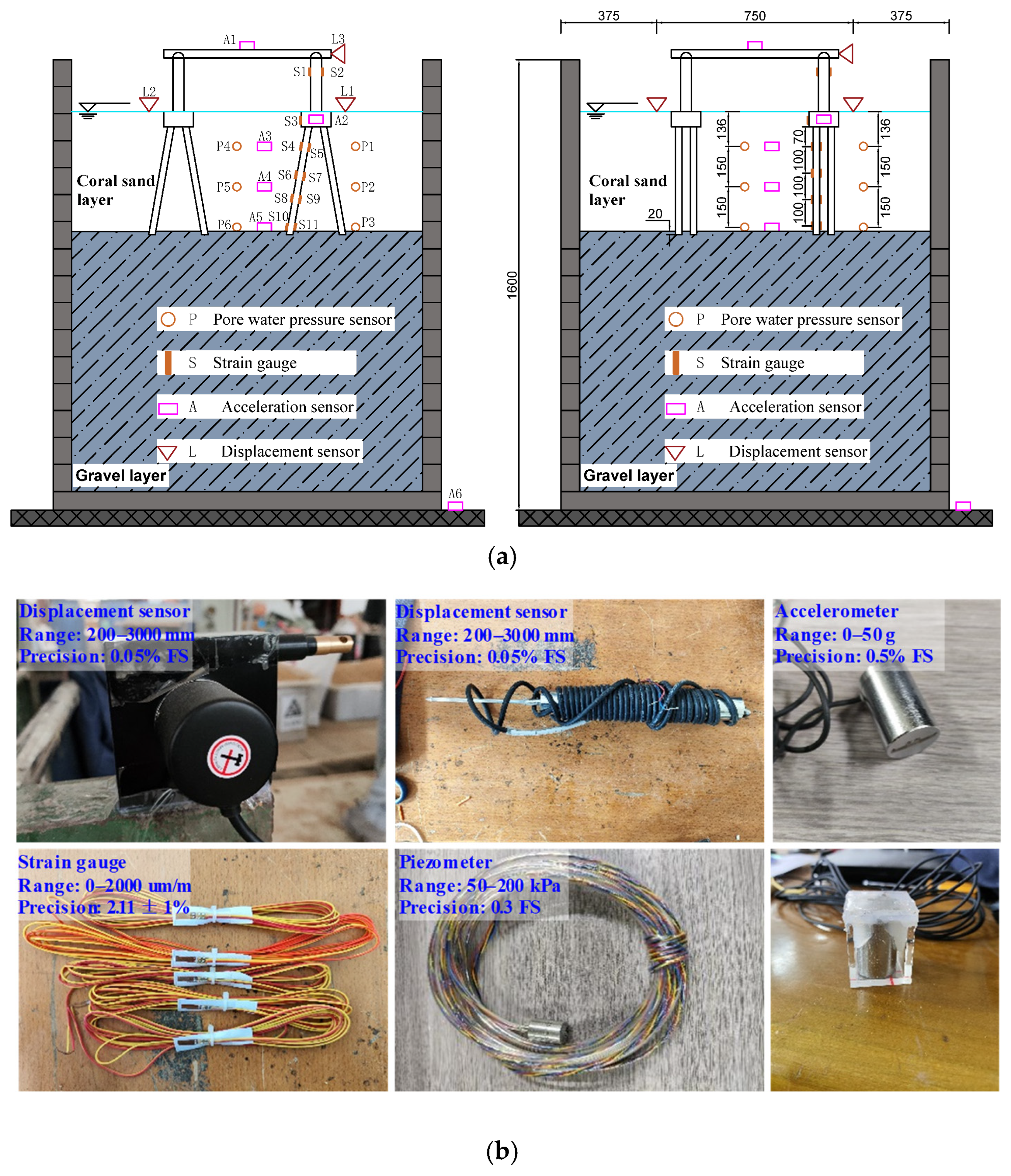
2.4. Data Collection

The main data, such as the pore water pressure, the horizontal acceleration of the superstructure and pier cap, and the strain of the piles, are monitored during the test, and the sensor arrangement and accuracy are shown in Figure 4. The two pile foundation models adopt the same sensor arrangement. Owing to the saturated state of the foundation soil, the sensors within the soil layer are prone to damage, and the density of the accelerometers is relatively high compared with that of the sandy soil, which can lead to coupled vibrations, thereby affecting the accuracy of the experimental data. To address this issue, the accelerometers are fixed inside small acrylic boxes, and strict waterproof measures are taken for the outer surface of the acrylic boxes.

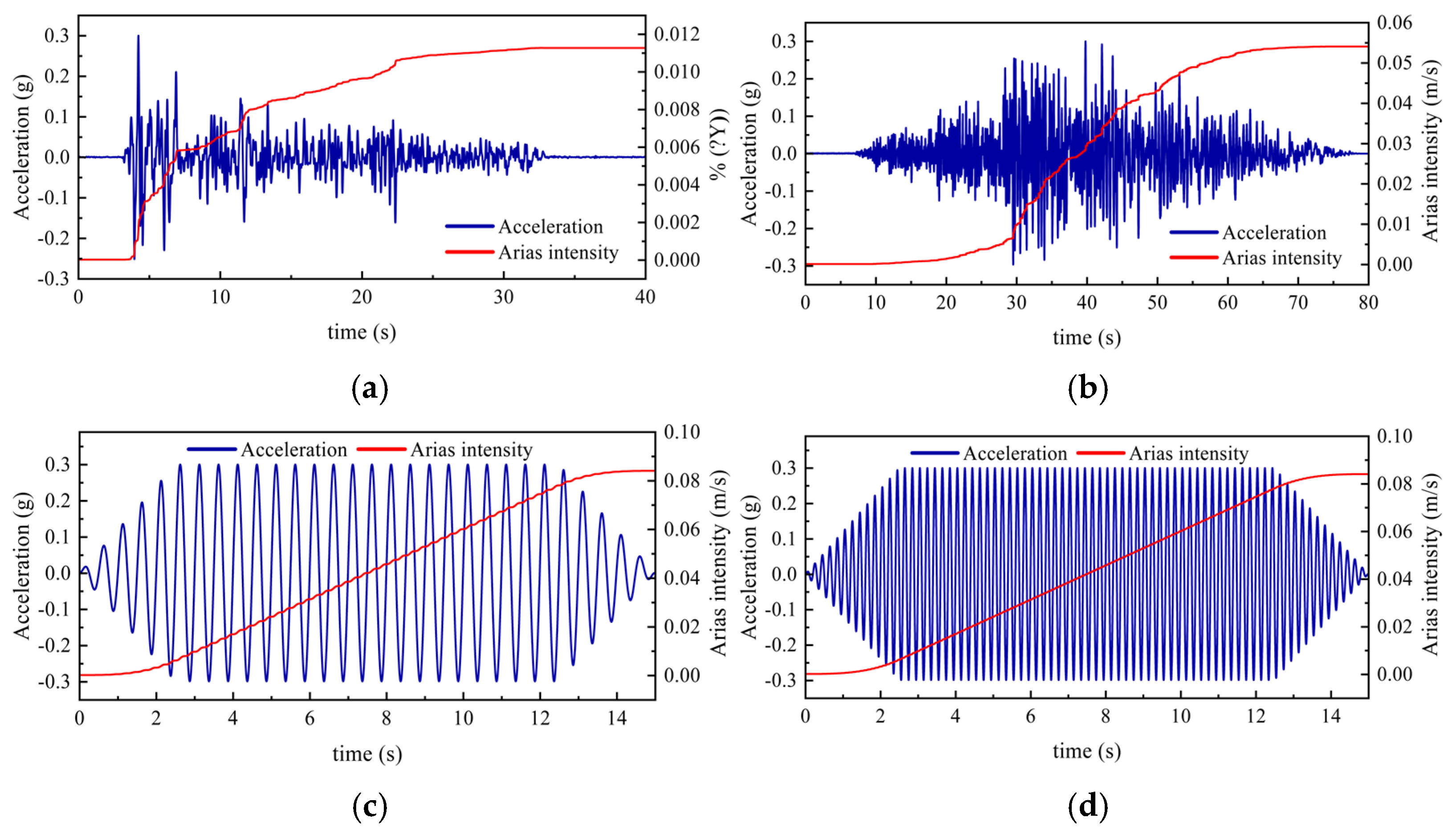
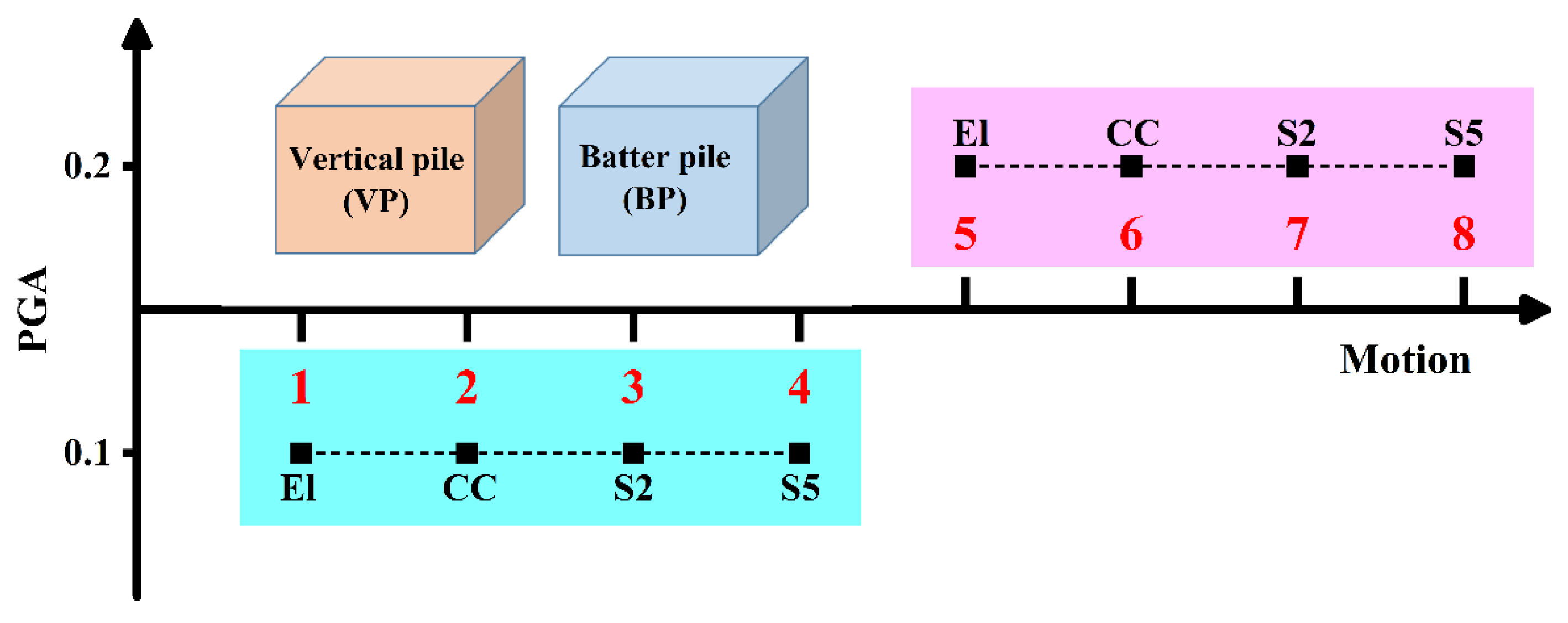
2.5. Exciting Wave Selection

The characteristics of the input excitation waves, including their frequency spectrum and magnitude, significantly affect the outcomes of the shaking table tests. In this study, four different excitation waves are selected as vibration loads: the El Centro wave (El), Chi-Chi wave (CC), and two artificial sine waves: the Sin_2 wave (S2) and Sin_5 wave (S5), with main frequencies of 1.89 Hz, 3.53 Hz, 2 Hz, and 5 Hz, respectively. Figure 5 shows the time–history curves of the excitation wave used for the test, and the cumulative Arias intensity profile for each excitation wave is marked with a red line in Figure 5. Figure 6 shows the spectral profile obtained by applying the FFT transform to the normalized excitation wave time profile. El Centro waves are widely used in structural testing and seismic response analysis, providing a robust basis for experimental practice [27]. The Chi-Chi wave, which occurred in Taiwan, was a significant land earthquake with characteristics relevant to sand liquefaction studies. It offers valuable insights into near-fault seismic effects and ground responses in island regions [28]. Compared with seismic waves, sine waves have a simpler pattern and stronger destructive power at the same peak value. The use of sine waves facilitates the analysis of the dynamic response of the overall system of the foundation–pile–superstructure of coral sand. Especially under liquefaction conditions, many scholars use sine waves for seismic excitation due to their ability to simulate consistent and controllable dynamic loading conditions [29,30,31].

Considering the limitations of the test site conditions, model conditions, costs, labor, and other factors, foundation soils were not replaced in tests of the same foundation type. In this study, the p–y curve method is used to analyze the interaction force between the foundation soil and the pile. The p–y curve method is based on Winkler’s elastic foundation beam theory and can effectively reflect the nonlinearity and complexity of the pile-soil interaction. The testing process is characterized by cumulative damage, which results in a gradual increase in the structural response, necessitating a gradual increase in the structural response. Initially, seismic signals with a peak ground acceleration (PGA) of 0.1 g are applied to both VPs and BPs, following the loading sequence of El, CC, S2, and S5. After loading at 0.1 g, the same pile foundations are subsequently subjected to the same seismic signals with PGA = 0.2 g. Figure 7 shows the experimental sequence. After each loading, a 0.05 g white noise signal lasting 20 s was used to check the model’s dynamic characteristics between tests. The 0.05 g peak was chosen to avoid influencing the structure while still being the minimum value needed for reliable excitation.

3. Results and Analysis

3.1. Experimental Phenomena

Figure 8a shows the condition of the site before the tests began. In the test with PGA = 0.1 g, the superstructure shook slightly with the input of vibration excitation, and a small amount of water was discharged from the surface of the model foundation, a phenomenon that was similar in both the BP and VP tests, and the condition of the site at the end of the test is shown in Figure 8b. The condition of the site after the test with PGA = 0.2 g is shown in Figure 8c, where a significant amount of water accumulated on the surface of the model foundation.

During the application of the 0.2 g loading test, the experimental phenomena of the superstructure were significantly different when the excitation waves were seismic and sine waves. Immediately following the application of seismic waves, the superstructure underwent a transient yet vigorous shaking episode, which subsequently tapered off over time. In contrast, when subjected to sine waves, the shaking intensity of the building gradually increased, peaking at approximately 10 s into the loading phase, and then progressively decreased in magnitude thereafter. Upon the completion of the model tests, varying degrees of settlement and tilting were observed in the models, as shown in Figure 9a,b. Notably, the tilting phenomenon was pronounced in the case of the VP, with a tilt angle reaching 10° (Figure 9c), whereas the BP exhibited a tilt angle of only 2°.

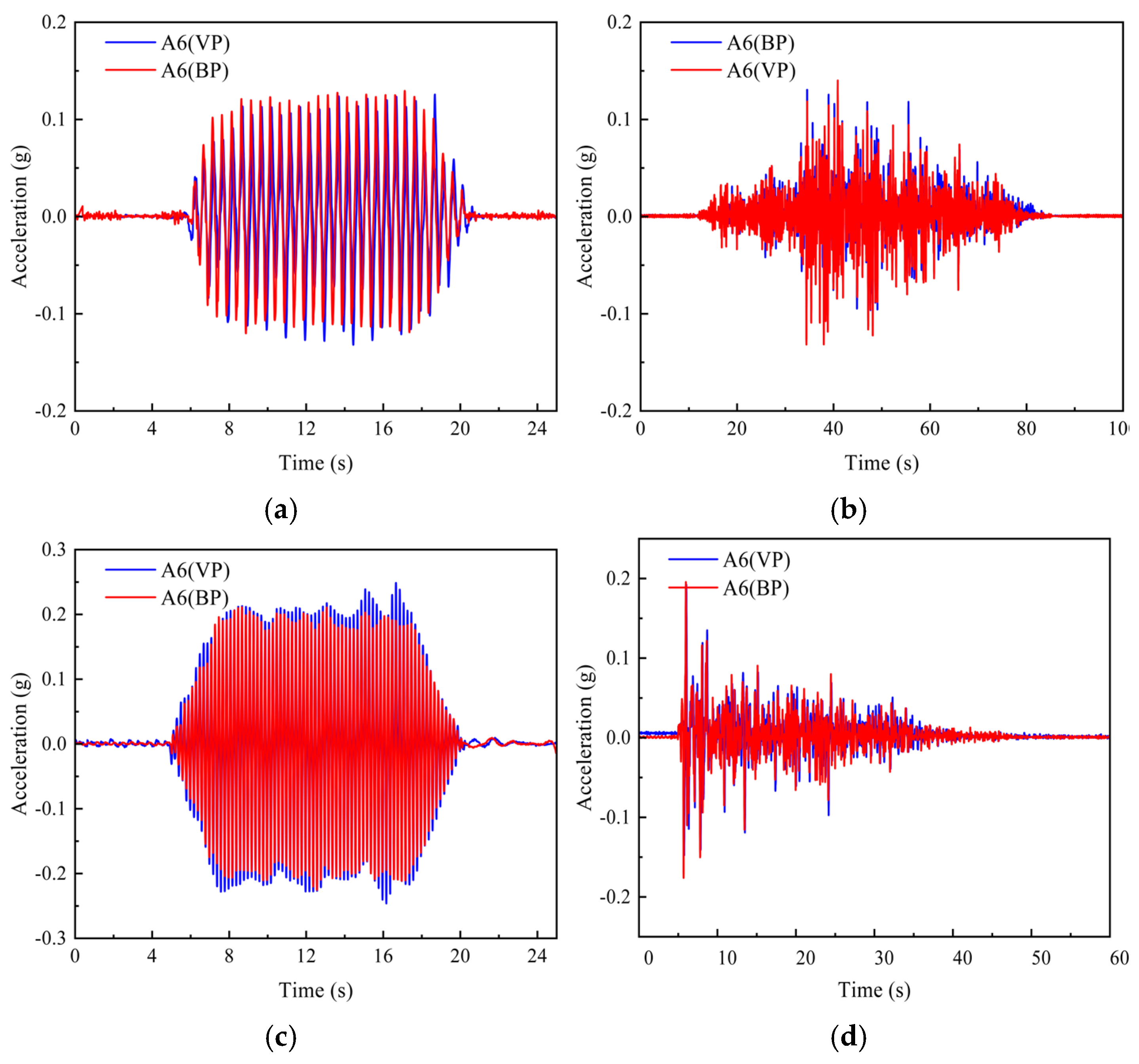
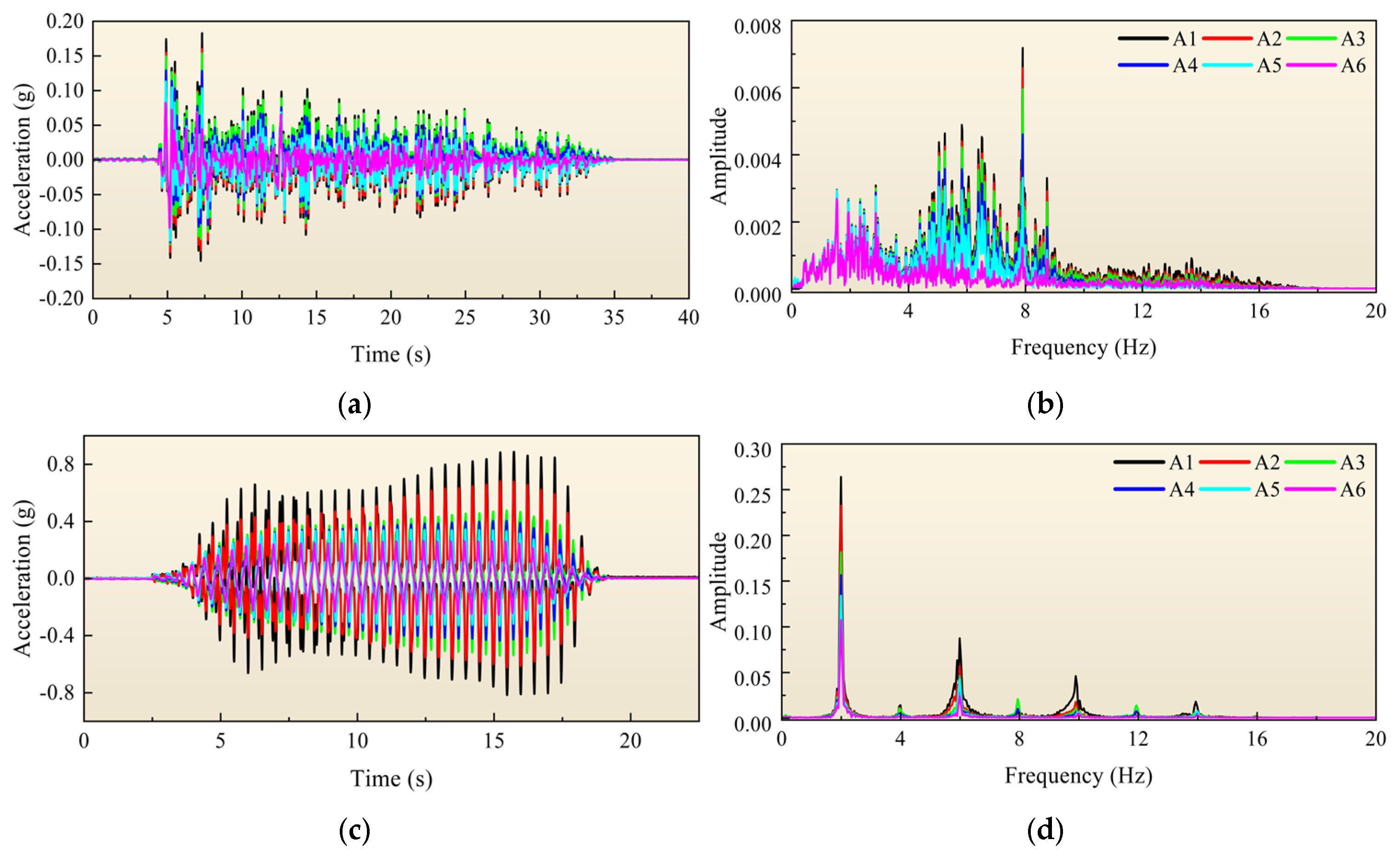
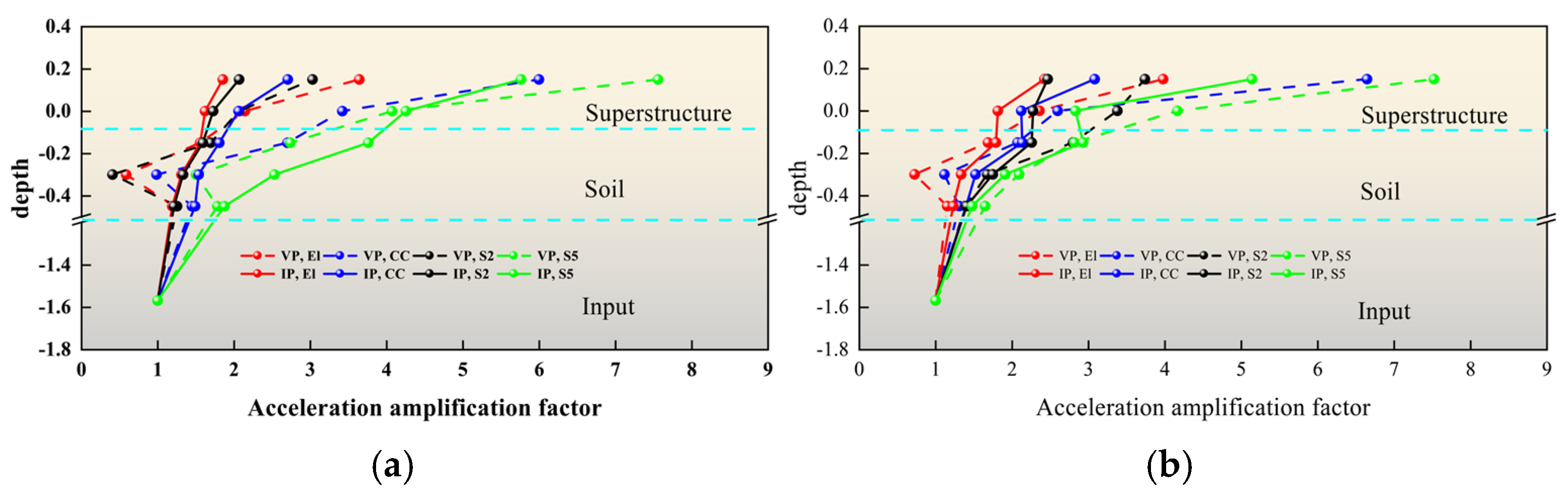
3.2. Acceleration Response

The eight representative vibration waves (A6) that were input to the shaking table surface during the loading test are depicted in Figure 10, and the corresponding response spectra (with 5% damping) are shown in Figure 11. The excitation waves are close to the raw waves but not identical. When the waveform and corresponding peak acceleration of the waves loaded in the test are the same, the acceleration amplitude, waveform, and dominant frequency components of the input motion by the shaking table dynamic system are consistent, thus providing a good comparative basis for the experiments with VPs and BPs.

Acceleration serves as one of the fundamental parameters that not only reflects the dynamic response of a structure but also constitutes a basic indicator for quantifying the dynamic response characteristics of both the structure and its foundation. Figure 12 shows two representative acceleration time–history curves and their corresponding amplitude–frequency curves. As vibration waves propagate upwards, the acceleration of the foundation soil gradually increases from the bottom to the top. Additionally, the vibration waveform undergoes some degree of distortion as it propagates upwards owing to the inhomogeneity and damping within the medium.

The acceleration amplification factor, denoted as f_amp, is defined as the ratio of the peak response acceleration to the peak input acceleration, and a bar chart of the acceleration amplification factor at different depths has been plotted, as shown in Figure 13. As the distance from the measuring point to the bottom of the container increases, the peak acceleration shows an increasing trend. The shear modulus of the soil decreases with the reduction in confining pressure; that is, after the soil reaches a liquefied state with the increase in EPWP, the shear stiffness of the soil is significantly weakened. Earthquake waves find it more difficult to continue propagating upwards through the liquefied soil, and the peak acceleration no longer increases linearly, with its growth rate slowing down in sandy soil.

Additionally, the superstructure f_amp increases with height. This is because although the nonlinearity of the soil body has reached a certain level of development, the superstructure has not been damaged, and the superstructure is still in an elastic state.

The magnitude of f_amp is related to the type of excitation wave. For shaking table tests, f_amp is greater with high-frequency, high-amplitude excitation waves than with low-frequency, low-amplitude excitation waves. In the superstructure, f_amp reaches the maximum value when the S5 wave loading test is conducted. Under the condition of a PGA of 0.1 g, the maximum f_amp reaches 7.56 for VPs and 5.76 for BPs, which is a difference of 1.31 times; similarly, under the condition of PGA = 0.2 g, the maximum f_amp for VPs reaches 8.33, and for BPs, it is 5.14, which is a difference of 1.62 times. When the foundation is batter piles, the f_amp of the foundation soil is greater than that of the vertical pile foundation, but in the superstructure, the f_amp of BPs is lower than that of VPs, which indicates that batter piles have an enhanced effect on the stability of the superstructure.

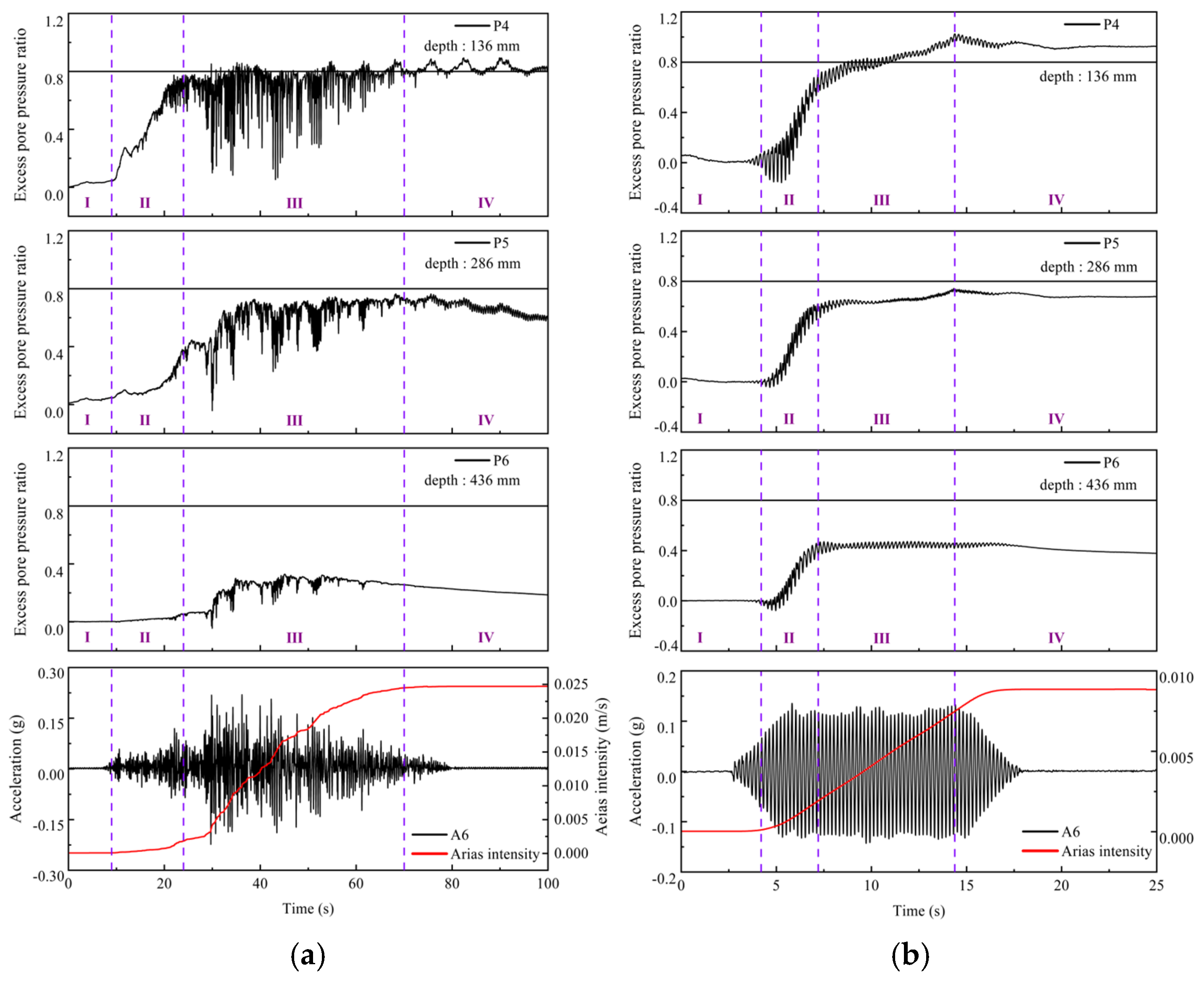
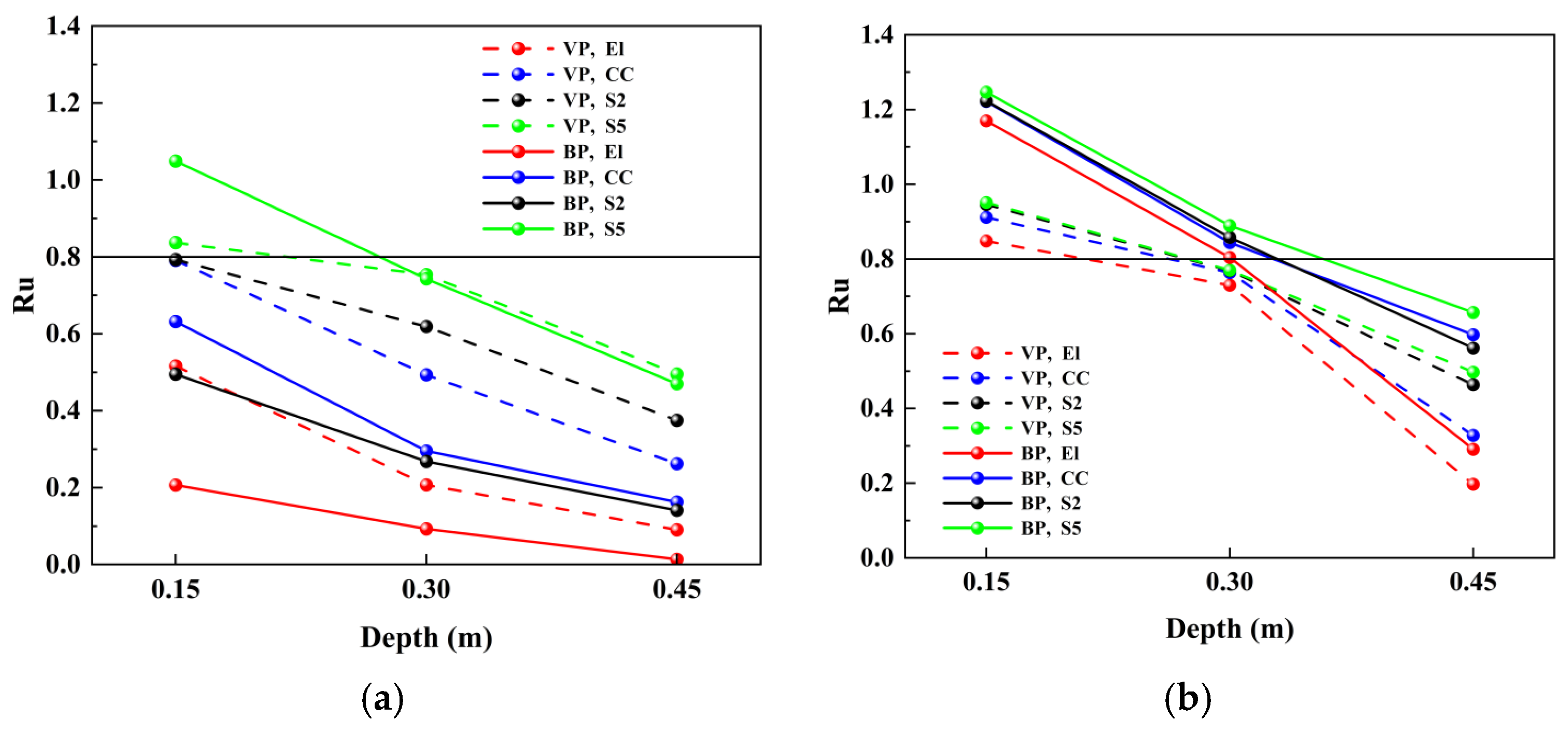
3.3. Pore Water Pressure Response

To reflect the trigger of the liquefaction of sandy soil, the excess pore water pressure (EPWP) ratio R_u is defined as the ratio of EPWP to the vertical effective stress: R_u = Δ_u/σ₀, where Δ_u is the measured EPWP and σ₀ is the vertical effective stress.

The traditional view holds that sand liquefaction occurs when R_u reaches 1.0 [32,33]. However, the literature [34,35] also indicates that cyclic mobility and large strains can emerge in sands when 0.8 < R_u < 1.0. Therefore, R_u = 0.8 is selected as the criterion for soil liquefaction.

Figure 14 shows the development time–history curve of R_u. The development curve of R_u is closely related to the Arias strength curve. As the cumulative Arias intensity increases, the pore water pressure also rises rapidly to the liquefaction level. Deep layers (P6) exhibit different patterns of pore pressure development than shallow layers (P4), with the rate of development of R_u in the shallow coral sand foundation being significantly greater than that in the deep layers. This difference gradually decreases as the depth of burial increases. Deeper soils did not reach liquefaction in the tests, and their R_u started to decrease after reaching the peak value, whereas the R_u of shallow foundations increased slowly or remained stable with time after reaching the peak value until the end of vibration. With decreasing foundation depth, the peak value of R_u increases, indicating that the sand layers at shallower depths are more susceptible to liquefaction and maintain this state for a longer period, which aligns with the general patterns of foundation liquefaction.

The irregular shape of the coral sand particles, unlike the quartz sand particles, enlarges the contact surface between the particles, enhances the interlocking effect among the particles [36,37], and thus increases the shear strength of the coral sand. This structure is not easily destroyed, which slows the trend of volume reduction in coral sand ground, leading to a scenario where the pore water pressure is not prone to increase to a level that renders the effective stress zero [38]. EPWP in the deep layers of the model foundation migrates upwards through the already damaged sand layers, replenishing EPWP in the shallow layers. This results in the replenishment rate of EPWP in the shallow layers being greater than or equal to the dissipation rate, leading to a continuous increase or stable maintenance of EPWP.

As can also be observed in Figure 14, the evolution process of R_u can be divided into four stages: initial stage I, acceleration stage II, stable or slow growth stage III, and decay stage IV. In stage I, the coral sand exhibited elastic characteristics, with R_u developing slowly, almost in a gradual accumulation phase from its initial state. In stage II, as the amplitude of the excitation wave increases and the Arias intensity of ground motion accumulates, R_u exhibits a sharp growth trend, indicating that the pore water pressure within the soil rapidly responds to the external dynamic forces. In stage III, as R_u gradually reaches its maximum value, its growth rate gradually slows until it stabilizes or maintains a slow growth level. This stage marks the approach of R_u to its maximum region. In stage IV, as the amplitude of seismic acceleration decays and the vibration intensity weakens, R_u within the soil begins to decrease. The pore water redistributes and tends towards a stable state, and the accumulated pore water pressure in the saturated sand layer starts to dissipate. However, this process is relatively lengthy, with experimental records showing a maximum dissipation time of up to 28 min.

Figure 15 depicts the peak values of R_u. Foundation liquefaction is related mainly to the type of excitation wave, soil depth, pile, etc. The type of excitation wave includes the frequency and peak value of acceleration. The measurement results of EPWP indicate that the liquefaction of the foundation occurs only when the vibration energy is sufficiently strong. Among the excitation waves used in the experiment, S5 has the highest frequency, and its peak EPWP ratio is significantly greater than that of the other excitation waves. In contrast, the El wave, which has the lowest frequency and simultaneously the lowest Arias intensity, has an EPWP ratio that is notably lower than those of the other waves.

When the PGA = 0.1 g, the peak EPWP ratio curves are not distinctly differentiated between various pile types; instead, they are interlaced with each other as the type of excitation wave is transformed: when the same excitation wave is applied, R_u for vertical piles is greater than that for batter piles. Furthermore, the higher the frequency and the greater the Arias intensity of the excitation wave, the greater the EPWP ratio. At PGA = 0.2 g, the peak EPWP ratio curves distinctly separate into two groups that correspond to the vertical and batter piles, with the peak value for the batter piles notably surpassing that for the vertical piles.

As evident in Figure 15, the vibration intensity serves as a pivotal factor in triggering soil liquefaction. The root cause of sandy soil liquefaction stems from the rearrangement of sand particles under seismic loading, causing them to densify and reduce interparticle pore volume, thereby increasing pore water pressure. Consequently, when the effective stress diminishes to zero, the liquefaction of the soil ensues. Nevertheless, if the vibration intensity is inadequate to overcome the initial interparticle interactions, even with prolonged exposure to vibration excitation (e.g., PGA = 0.1 g), the pore water pressure may not increase sufficiently to nullify the effective stress.

EPWP is closely related to the depth of the soil layer. At PGA = 0.1 g, the deep soil layers (P6) did not experience liquefaction, and only some of the shallow soil layers (P4) in the experiments experienced liquefaction. At PGA = 0.2 g, R_u in all experiments for the shallow layers exceeded 0.8, and when the foundation consisted of batter piles, nearly all the battle piles in the middle soil layers (P5) also liquefied, but the deep soil layers still did not liquefy at this time.

Additionally, the analysis of acceleration and the ratio of EPWP indicates that VPs and BPs have different mechanisms for restraining sandy soil layers, leading to different reinforcement effects on the soil. The reinforcement effect of vertical pile groups on the soil between piles (among pile groups) and the soil surrounding the pile groups within a small range is stronger, while that of batter pile groups is weaker but covers a broader area. When the foundation is composed of vertical piles, there is a significant interdependence between the piles and the sandy soil, resulting in smaller peak acceleration values of the soil surrounding the piles, while the peak acceleration response of the superstructure is larger. Compared with batter piles, the peak Ru value of the coral sand foundation before liquefaction is smaller for vertical piles, but the peak Ru value after liquefaction is larger, indicating that the constraint of vertical piles on the soil is weaker before liquefaction but stronger after liquefaction, more effectively limiting the soil movement. Therefore, in practical applications, the type of pile should be chosen by comprehensively considering the soil conditions, the characteristics of seismic loads, and the specific requirements of the foundation structure.

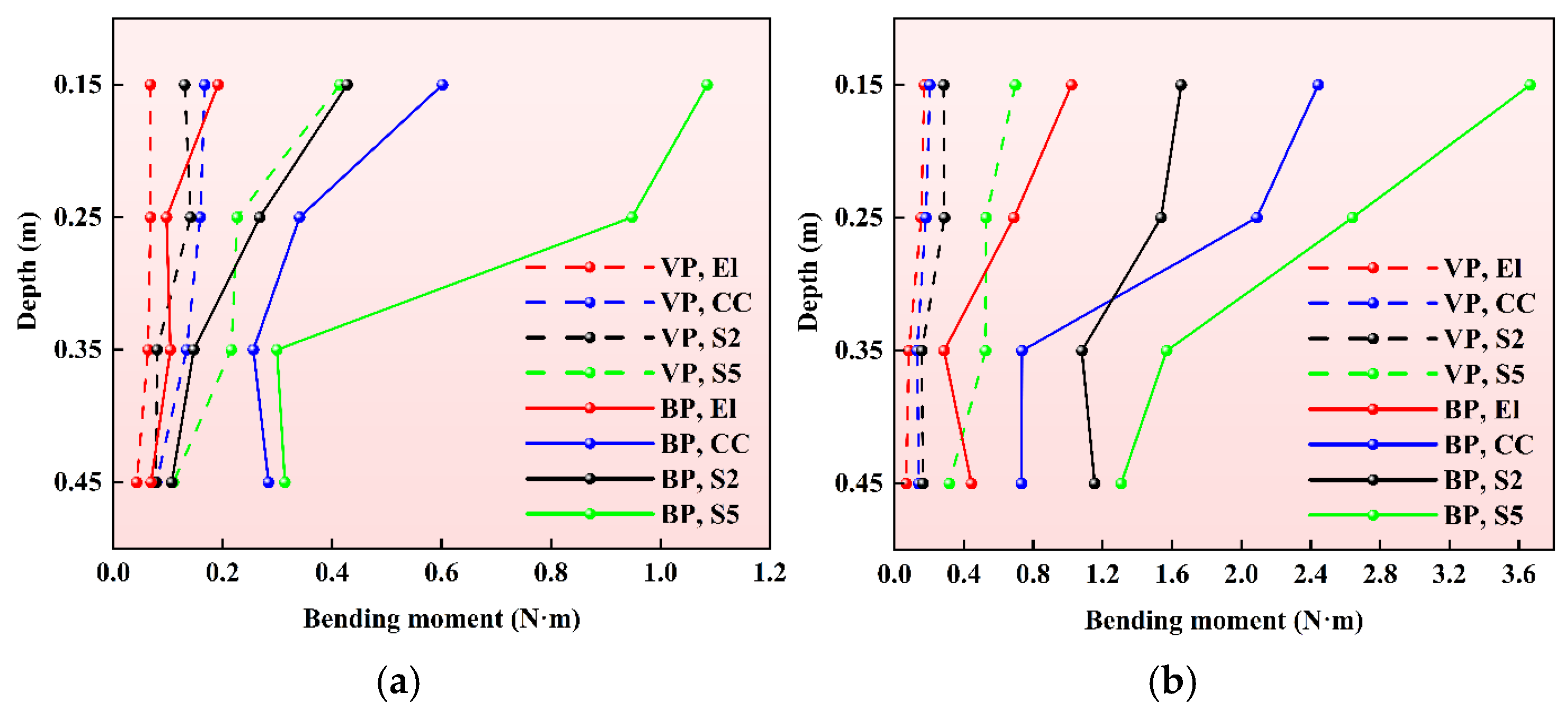
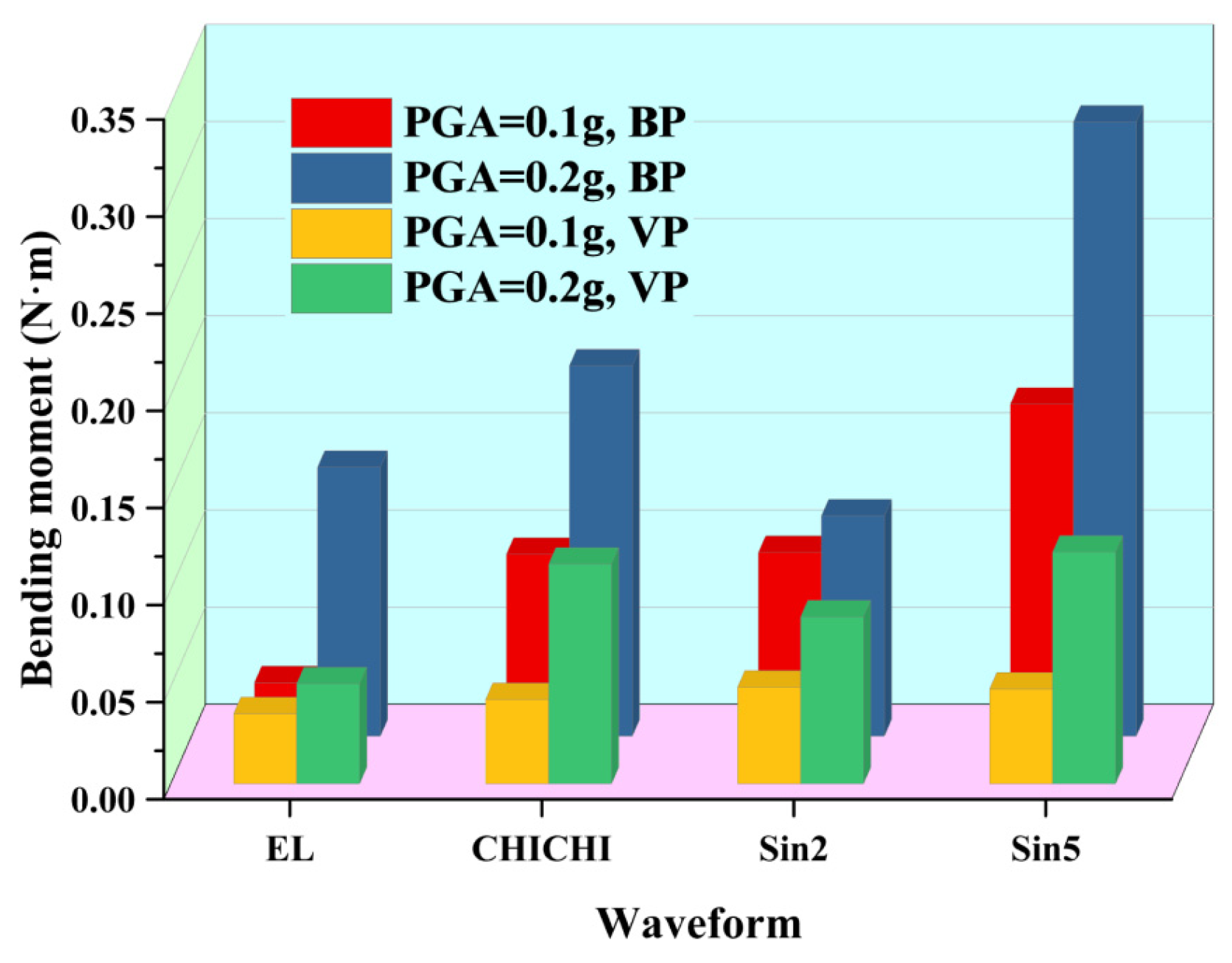
3.4. Dynamic Bending Moment Response

The bending moment is an important indicator for evaluating the internal stress and deformation state of a pile after being subjected to horizontal forces. The lateral dynamic response of pile foundations is influenced by the pile–soil–superstructure interaction: Owing to the significant difference in stiffness between the pile foundation and the surrounding soil during earthquakes, the lateral vibration of the soil around the piles imposes kinematic loads on the piles [39,40], while the vibration of the superstructure supported on the foundation will generate inertial loads on the pile [41]. The bending moment response of the pile is affected by the combined effect of kinematic and inertial loads.

According to the Euler–Bernoulli beam theory, the bending moment M of a pile foundation can be calculated via Equation (4):

$$M={\displaystyle \frac{EI({\epsilon }_{\mathrm{t}}-{\epsilon }_{\mathrm{c}})}{d}}$$

(4)

where ε_t and ε_c are the tensile and compressive strains of the pile, respectively, EI is the flexural rigidity of the pile section, and d is the diameter of the pile. A positive bending moment indicates that the outer side of the pile (perpendicular to the vibration direction) is in tension, and a negative bending moment indicates that the inner side of the pile (perpendicular to the vibration direction) is in tension.

In the tests, eight strain gauges were used to measure the strain of the pile. Figure 16 shows two representative time-history curves of pile bending moments. Specifically, M1 was derived from strain gauges S4 and S5, M2 from S6 and S7, M3 from S8 and S9, and M4 from S10 and S11. These bending moment curves specifically illustrate the bending moment behavior of the pile under S2 wave loading and CC wave loading. The shapes of these bending moment curves are almost identical to the time–history curves of the excitation waves.

Figure 17a shows the maximum value and distribution of the dynamic bending moments at different measuring points under the condition of PGA = 0.1 g. Under the influence of external excitation waves, as the depth at various positions along the pile increases, the dynamic bending moment tends to decrease. The dynamic bending moment of the pile is directly related to the type of excitation wave and generally follows the following order: El < S2 < CC < S5. This discrepancy is due primarily to the bending moment being more susceptible to the influence of the excitation wave frequency, followed by the magnitude of the excitation wave energy. When high-frequency sinusoidal waves are used for excitation, the bending moment experienced by the pile foundation reaches its maximum value. The maximum values of the dynamic bending moments of batter piles are generally greater than those of vertical piles under the same type of excitation wave.

Figure 17b shows the distribution and variation in the maximum dynamic bending moments at various measurement points when the PGA = 0.2 g, exhibiting a similar distribution pattern to that observed at PGA = 0.1 g. As the intensity of the input ground motion increases, the pile bending moment significantly increases. The maximum dynamic bending moment sustained by BP is markedly greater than that sustained by VP. The distribution pattern of the maximum dynamic bending moments is broadly categorized into two groups, corresponding to VPs and BPs. Even when the El wave, which exerts the least destructive force, is applied to BPs, it induces a greater bending moment than the S5 wave, which exerts the most destructive force when applied to VPs. Therefore, when the intensity of the excitation wave is greater, the influence of the load frequency on the bending moment of the pile is more pronounced than when the pile is subjected to an excitation wave of lesser intensity.

Batter piles can transform part of the horizontal forces into vertical forces, which are then transferred to the foundation, thereby more effectively resisting horizontal loads. However, experimental data indicate that the dynamic bending moment sustained by batter piles is actually greater than that sustained by vertical piles. According to the analysis in Section 3.2, the BP provides better stabilization to the superstructure, implying that it experiences relatively smaller inertial forces from the superstructure. However, the analysis in Section 3.3 indicates that as the peak acceleration increases, the BP is more prone to liquefaction than that of vertical piles. The dynamic loads imposed by the coral sand layer on the piles contribute more significantly to the bending moment of the pile body. Thus, when the coral sand layer experiences a lower degree of liquefaction, the bending moment of the pile is generated mainly by the dynamic loads resulting from the lateral vibrations of the coral sand layer. After liquefaction, the shear strength of the soil decreases; the lateral stiffness of the foundation decreases, and the restraining effect on the pile foundation diminishes. The bending moment of the pile increases because of the lateral flow deformation of the sand. At this stage, the bending moment is produced by the combined action of the inertial loads from the superstructure and the dynamic loads resulting from the lateral displacement of the soil.

When the input vibration wave is S5, at PGA = 0.1 g, the maximum bending moment of the batter pile is 1.09 N·m, and that of the vertical pile is 0.41 N·m, with a difference of 2.62 times; at PGA = 0.2 g, the maximum bending moment of the batter pile is 3.66 N·m, and that of the vertical pile is 0.70 N·m, with a difference of 5.25 times. When pile foundations are subjected to significant bending moments, the pile structure may fail. Under seismic loading, batter piles experience notably larger bending moments compared to vertical piles. Therefore, special attention must be paid to this aspect during the design process.

Figure 18 presents a comparison of the bending moments of the bridge piers. Similar to the distribution pattern of the maximum dynamic bending moments of the pile foundation, the peak dynamic bending moments of the bridge pier columns increase with increasing frequency of the vibration wave, the intensity of Arias, and PGA. When the El wave is applied with PGA = 0.1 g, the resulting bending moment is the smallest, whereas the bending moment reaches its maximum when the S5 wave is applied with PGA = 0.2 g. The bending moment values of the batter piles are generally greater than those of the vertical piles, and the difference is more pronounced at 0.2 g. When loaded with El waves, the bridge pier bending moments of the oblique and vertical piles are 0.14 N·m and 0.05 N·m, respectively. When loaded with S5 waves, the values are 0.32 N·m and 0.12 N·m, respectively, showing a difference of approximately 2.66 times.

It should be emphasized that this investigation specifically addresses the qualitative comparison of vertical and batter pile dynamic responses in coral sand liquefaction sites under seismic loading. No flexural failure was detected during testing, attributable to the inherent high elasticity of the plexiglass material employed.

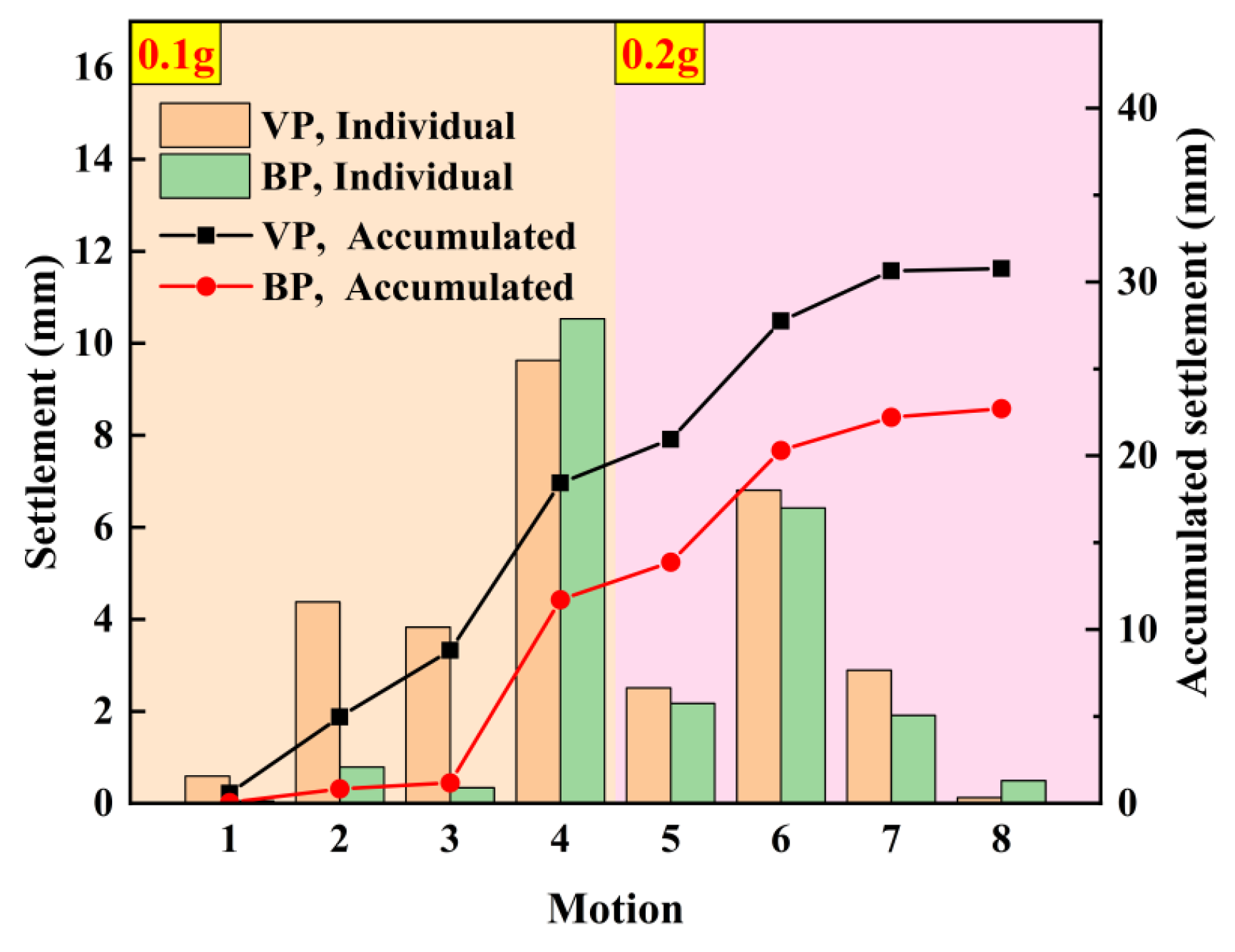
3.5. Soil Settlement

Soil settlement is a phenomenon caused by increased pore water pressure, leading to soil liquefaction and reduced shear strength. The settlement behavior of sandy soil foundations in the experiment is shown in Figure 19.

VPs and BPs exhibit both similarities and differences in settlement characteristics. Under 0.1 g load conditions, initial soil compression did not reach saturation, and pile settlement gradually increased with vibration. By the fourth loading cycle, the sandy soil became denser, resulting in accelerated settlement. At this stage, VPs and BPs showed similar settlement trends, but BPs exhibited slightly lower settlement compared to VPs. Under 0.2 g loading, further soil compression triggered liquefaction due to rising pore water pressure, significantly reducing shear strength. However, the settlement growth rate slowed because high-frequency sinusoidal loading had partially disrupted the soil void structure. It should be noted that although the sandy soil foundation became denser after being subjected to high-frequency sinusoidal waves, which affected subsequent experiments, it did not impact the comparison between VPs and BPs under the same conditions.

The accumulated settlement of VPs (30.77 mm) consistently exceeded that of BPs (22.72 mm), demonstrating that the inclined design of BPs disperses loads and optimizes the soil structure, thereby enhancing liquefaction resistance. Adjusting the inclination angle and stiffness of BPs can further improve their anti-liquefaction performance.

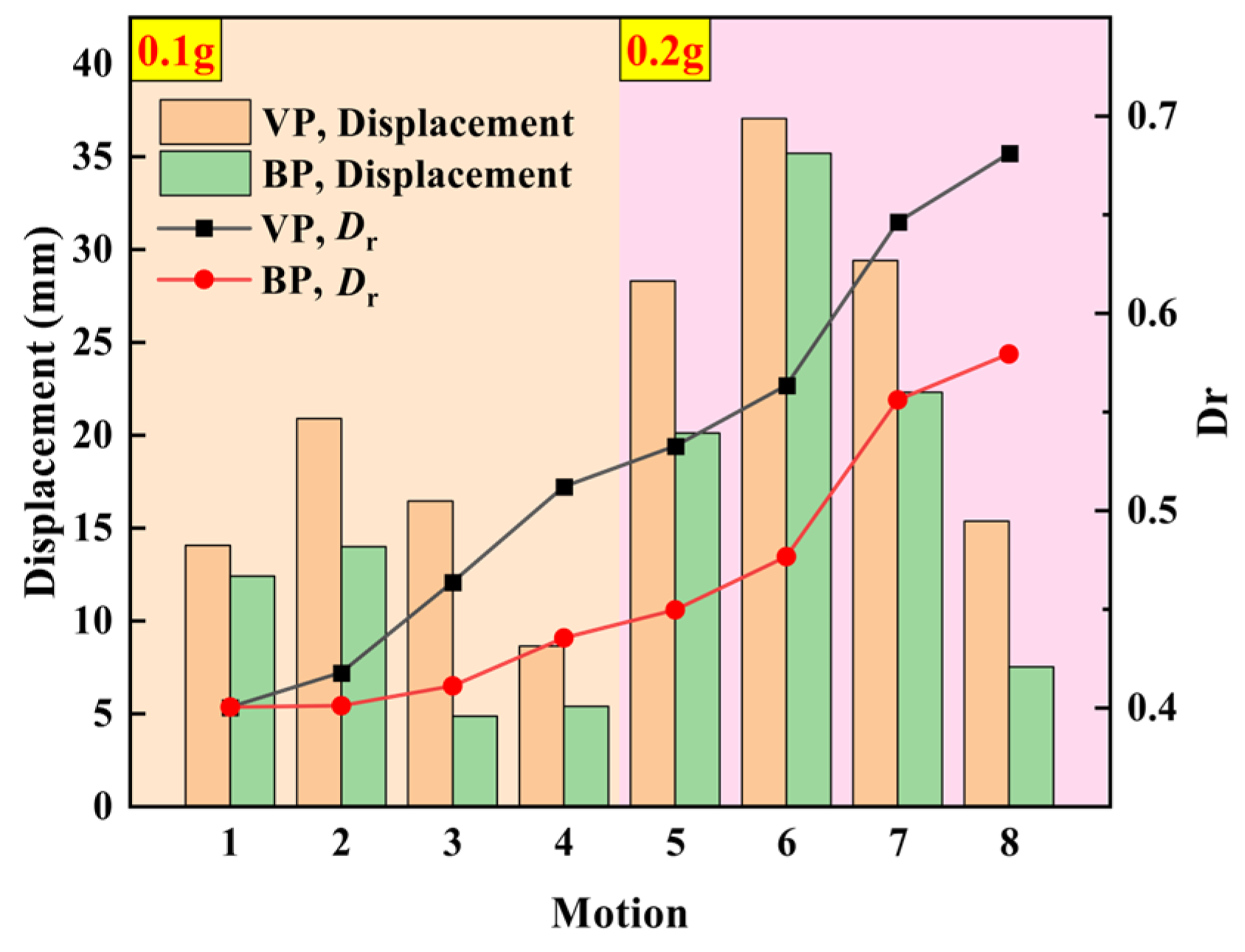
3.6. Displacement of the Superstructure

In the experiment, displacement sensor L3 was used to monitor the horizontal displacement of the superstructure. The horizontal displacement refers to the maximum displacement the structure can undergo when subjected to a vibrational load, as illustrated in Figure 20. Since changes in the relative density of soil (D_r) have a significant impact on the displacements of both the pile foundation and the superstructure, the variation of D_r is also plotted in Figure 20.

In the experiment, two displacement sensors, L1 and L2, were employed to monitor the vertical settlement of the surface of the saturated sand. Since the soil foundation was not reinstalled between each test of the same base, the soil density increased to varying extents after each loading cycle. By measuring the settlement of the saturated sand, the volume changes in the soil model were calculated, thereby determining the variations in the relative density of the soil model, as illustrated by the polyline in Figure 20.

The increase in soil compaction significantly restricted the displacement of the foundation. In the tests, the increase in soil compaction led to a reduction in the horizontal displacement of the superstructure. Following the liquefaction of the coral sand foundation, the shear stiffness of the shallow soil layer decreased, approaching zero, which temporarily weakened the soil’s constraint on the pile. However, after conducting a series of experiments, the soil, through settlement consolidation, reorganized its structure, thereby enhancing the constraint on the pile base and consequently reducing the displacement of the superstructure.

The horizontal displacement of the superstructure supported by VPs consistently exceeded that of BPs. By the final stage of the experiment, the horizontal displacement of the superstructure on the batter piles was 51.21% smaller than that on the vertical piles. The inclined design of the batter piles demonstrates superior structural stability under nonlinear loading conditions. This enhanced performance stems from the inclination angle of the batter piles, which promotes more uniform load distribution, mitigates vibration-induced differential settlement, and thereby improves the overall stability of the superstructure.

4. Conclusions

This study is based on shaking table model tests of two types of pile foundations, vertical and batter piles, and the dynamic response of the overall system of pile–soil superstructures in the coral sand sites under different types of excitation waves and vibration intensities is investigated via physical quantities such as the pore water pressure, acceleration, dynamic bending moment, soil consolidation settlement, and horizontal displacement of superstructures as reference indices. The following conclusions are obtained:

(1) The experimental results show that foundation liquefaction is strongly influenced by both PGA and the loading characteristics. At PGA = 0.1 g, liquefaction occurred exclusively in the shallow layer of the foundation and was solely triggered by high-frequency sinusoidal loading. In contrast, at PGA = 0.2 g, all tested cases exhibited complete liquefaction of the shallow foundation layer, accompanied by progressive extension of liquefaction zones into deeper soil strata. Notably, batter piles exhibited lower EPWP ratios than vertical piles under PGA = 0.1 g, attributable to improved load redistribution through their inclined geometry. However, at PGA = 0.2 g, the EPWP ratio of vertical piles is lower than that of batter piles.

(2) In earthquake-prone regions, batter pile foundations demonstrate superior effectiveness compared to vertical pile foundations. The soil–pile interaction in batter piles induces higher peak soil accelerations while maintaining lower superstructural accelerations. Although vertical piles exhibit lower peak EPWP ratios prior to liquefaction, their performance degrades post-liquefaction due to reduced shear stiffness in coral sand. Consequently, BPs display enhanced overall performance in preserving soil–structure integrity during major seismic events, and the stability of the superstructure is better.

(3) Under identical lateral loads, BPs and piers exhibit greater dynamic bending moments than VPs. When the liquefaction degree of coral sand is low, the bending moments in the piles are primarily generated by lateral vibration. After liquefaction, the influence of lateral displacement becomes more significant.

(4) The soil settlement of batter pile foundations is notably smaller than that of vertical pile foundations. Specifically, the final settlement of vertical pile foundations reaches approximately 1.35 times that of batter pile foundations. Moreover, the lateral displacement of the superstructure in batter piles demonstrates a 51.21% reduction compared to vertical pile foundations.