Mohr–Coulomb-Model-Based Study on Gas Hydrate-Bearing Sediments and Associated Variance-Based Global Sensitivity Analysis

Abstract

Different gas hydrate types, such as methane hydrate and carbon dioxide hydrate, exhibit distinct geomechanical responses and hydrate morphologies in gas-hydrate-bearing sediments (GHBSs). However, most constitutive models for GHBSs focus on methane-hydrate-bearing sediments (MHBSs), while largely overlooking carbon-dioxide-hydrate-bearing sediments (CHBSs). This paper proposes a modified Mohr–Coulomb (M-C) model for GHBSs that incorporates the geomechanical effects of both MHBSs and CHBSs. The model integrates diverse hydrate morphologies—cementing, load-bearing, and pore-filling—into hydrate saturation and incorporates an effective confining pressure. Its validity was demonstrated through simulations of reported triaxial compression tests for both MHBSs and CHBSs. Moreover, a variance-based sensitivity analysis using Sobol’s method evaluated the effects of hydrate-related soil properties on the geomechanical behavior of GHBSs. The results indicate that the shear modulus influences the yield axial strain of the CHBSs and could be up to 1.15 times more than that of the MHBSs. Similarly, the bulk modulus showed an approximate 5% increase in its impact on the yield volumetric strain of the CHBSs compared with the MHBSs. These findings provide a unified framework for modeling GHBSs and have implications for CO₂-injection-induced methane production from deep sediments, advancing the understanding and simulation of GHBS geomechanical behavior.

1. Introduction

Gas-hydrate-bearing sediments (GHBSs) consist of solid gas hydrates in the pore space of host sediments, which have attracted global research interest recently [1,2,3,4,5]. The prediction and assessment of geomechanical stability in hydrate reservoirs represent one of the primary challenges faced by GHBSs. One promising method for gas hydrate extraction is carbon dioxide (CO₂) injection, which not only facilitates the extraction of methane hydrates but also enables the storage of CO₂, offering environmental advantages [6,7,8]. However, this method involves two distinct types of hydrates—methane hydrates and carbon dioxide hydrates—making the assessment of reservoir stability more complex. Geological records show that the dissociation of methane hydrates can trigger geological disasters [9,10]. On the other hand, the formation of CO₂ hydrates can induce changes in pore pressure, leading to the destabilization of surrounding sediments [11,12]. Therefore, further studies are required on the geomechanical behaviors of MHBSs and CHBSs.

There were laboratory studies on GHBSs regarding the general and respective geomechanical behaviors of MHBSs and CHBSs. Figure 1 and Figure 2 summarize the triaxial compression tests of gas-hydrate-bearing soil samples under different hydrate saturations and confining pressures [13,14,15,16,17,18,19,20,21,22,23]. The tests in Figure 1 and Figure 2 involve artificially synthesized specimens with Toyoura sand as the host sediment, as well as natural samples collected from the Nankai Trough. From the laboratory results, it was found that the strength, stiffness, and dilatancy of both MHBSs and CHBSs generally increased with hydrate saturation. Additionally, it was also observed that MHBSs and CHBSs of the same hydrate saturation had different values of geomechanical properties [15,20,24]. Miyazaki et al. (2016) [20] and Luo et al. (2020) [24] attributed the different geomechanical behaviors of MHBSs and CHBSs to the different hydrate morphologies, such as the crystal growth or cavity occupancy in the pore spaces [25,26,27].

Constitutive model studies were developed for simulating geomechanical behavior of gas-hydrate-bearing sediment, including the modified Duncan–Chang model, modified Mohr–Coulomb model, critical state model, and statistical damage model. The modified Duncan–Chang model considers the strain-hardening behavior of MHBSs with the effects of hydrate saturation and effective confining pressure [28,29]. The modified Mohr–Coulomb model simulates the stress–strain behavior and volumetric dilation of MHBSs [30,31]. The modified critical state model [32,33,34,35,36,37] uses the state parameters to reproduce the geomechanical response. The statistical damage model [38,39] incorporates statistical damage theory into a constitutive model to predict the change trend of the MHBS stress–strain relationship. However, these constitutive models are mostly based on MHBSs and lack studies on CHBSs. Despite the significance of different hydrate morphologies on the geomechanical properties of GHBSs being widely recognized, few constitutive models explicitly account for the microstructural features associated with different hydrates.

This study adopted the Mohr–Coulomb model and modified the model by introducing the concepts of hydrate morphology and an effective confining pressure. In order to demonstrate the applicability of the proposed model, drained triaxial compression tests on both MHBSs and CHBSs were simulated. Furthermore, the effects of hydrate-related geomechanical properties on sediment geomechanical behavior were quantitatively compared for MHBSs and CHBSs via the variance-based sensitivity analysis (Sobol’s method), providing a method for evaluating the parameter sensitivity under different sediment conditions. These findings mark a significant step forward in improving the prediction of geomechanical behavior across different types of GHBSs, offering a more comprehensive framework for constitutive modeling and the geomechanical analysis of GHBSs.

In the following section, the mechanical behaviors of MHBSs and CHBSs are first introduced, providing background information on their key characteristics and an overview of previous studies on Mohr–Coulomb constitutive models for GHBSs. The main components of the modified model are presented afterward, followed by the model’s validation. Then, a global sensitivity analysis was conducted to evaluate the influence of the parameters on the mechanical behavior. Finally, the key conclusions of this study are summarized.

2. Previous Mechanical Study on GHBSs

2.1. Laboratory Experiments

Figure 1 and Figure 2 illustrate the drained triaxial compression tests conducted on methane-hydrate-bearing sediments (MHBSs) and carbon-dioxide-hydrate-bearing sediments (CHBSs). It is evident that most laboratory experiments utilize artificially synthesized specimens. Although Toyoura sand is an idealized system and does not include clay minerals, it is widely used as the host sediment in laboratory studies of GHBSs due to its grain size distribution, composition, and similarity to natural sandy sediments [14,15,17,18,19,20,21,22]. Compared with in situ soil samples, synthetic specimens are easier to prepare and store. For synthetic gas-hydrate-bearing soil samples, the partially water-saturated (PWS) method is a common sample preparation method that is adopted to form cementation between the surface of soil particles and hydrate particles [13,14,15,16,17,18,19,20,21,22,23]. This method starts with wetting host sand with a determined initial moisture content by a wet compaction approach. Sample freezing follows. Then, the gas is injected under certain temperature and pressure conditions for around 24 h to form the GHBS specimen. The preparation processes of MHBSs and CHBSs with the PWS method are shown in Figure 3. After obtaining the hydrate-bearing sand sample, the back pressure and temperature are adjusted to the desired testing condition. Isotropic consolidation is carried out until the required effective stress is reached, after which triaxial compression tests are conducted. Most of the studies show that the critical strengths of the samples increase with increasing hydrate saturation and effective confining pressure. Meanwhile, the volume deformation demonstrates a higher trend of dilation as the saturation increases, while a high effective confining pressure inhibits this phenomenon [40]. In laboratory comparisons, MHBSs generally showed larger stiffnesses, shear strengths, and volumetric dilatancies compared with CHBSs under similar hydrate saturation and effective confining pressure conditions.

This study adopted the tests by both Hyodo et al. (2013, 2014) [14,15] and Miyazaki et al. (2010, 2016) [18,20] for numerical modeling in Section 3, both of which conducted triaxial compression tests on CHBS samples and MHBS samples of Toyoura sand under similar hydrate saturation and confining pressure conditions.

2.2. Mohr–Coulomb Model on Gas-Hydrate-Bearing Sediments

Based on laboratory testing, constitutive model studies were conducted to investigate the geomechanical behavior of GHBSs. Because of its applicability and simplicity, the Mohr–Coulomb (M-C) model has been long applied for simulating the geomechanical behavior of soil in the engineering field. The stiffness, strength, and dilatancy parameters in the M-C model have well-defined physical meanings and can be extended to incorporate additional hydrate effects.

Table 1. List of representative Mohr–Coulomb (M-C) model applications for GHBSs.

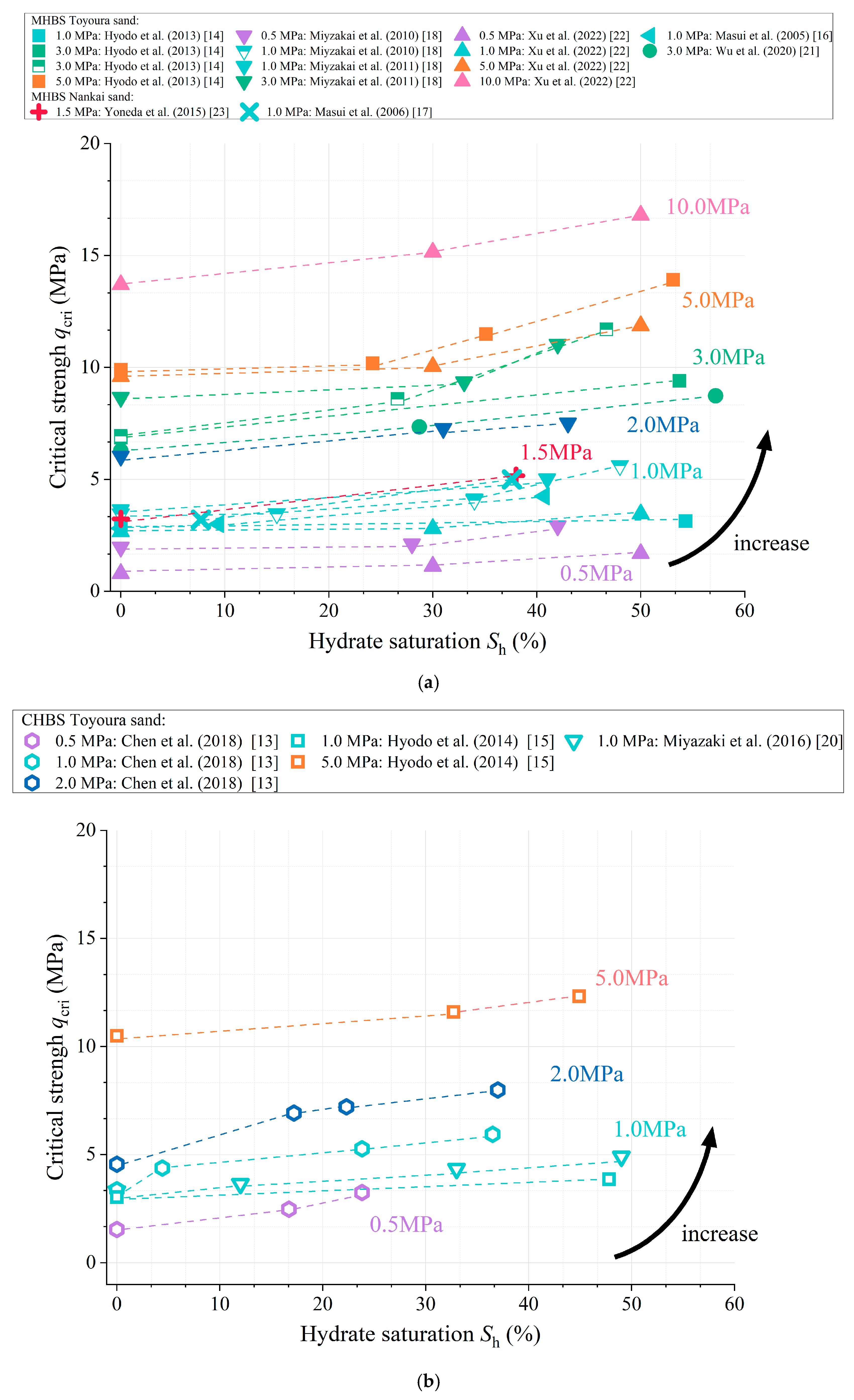
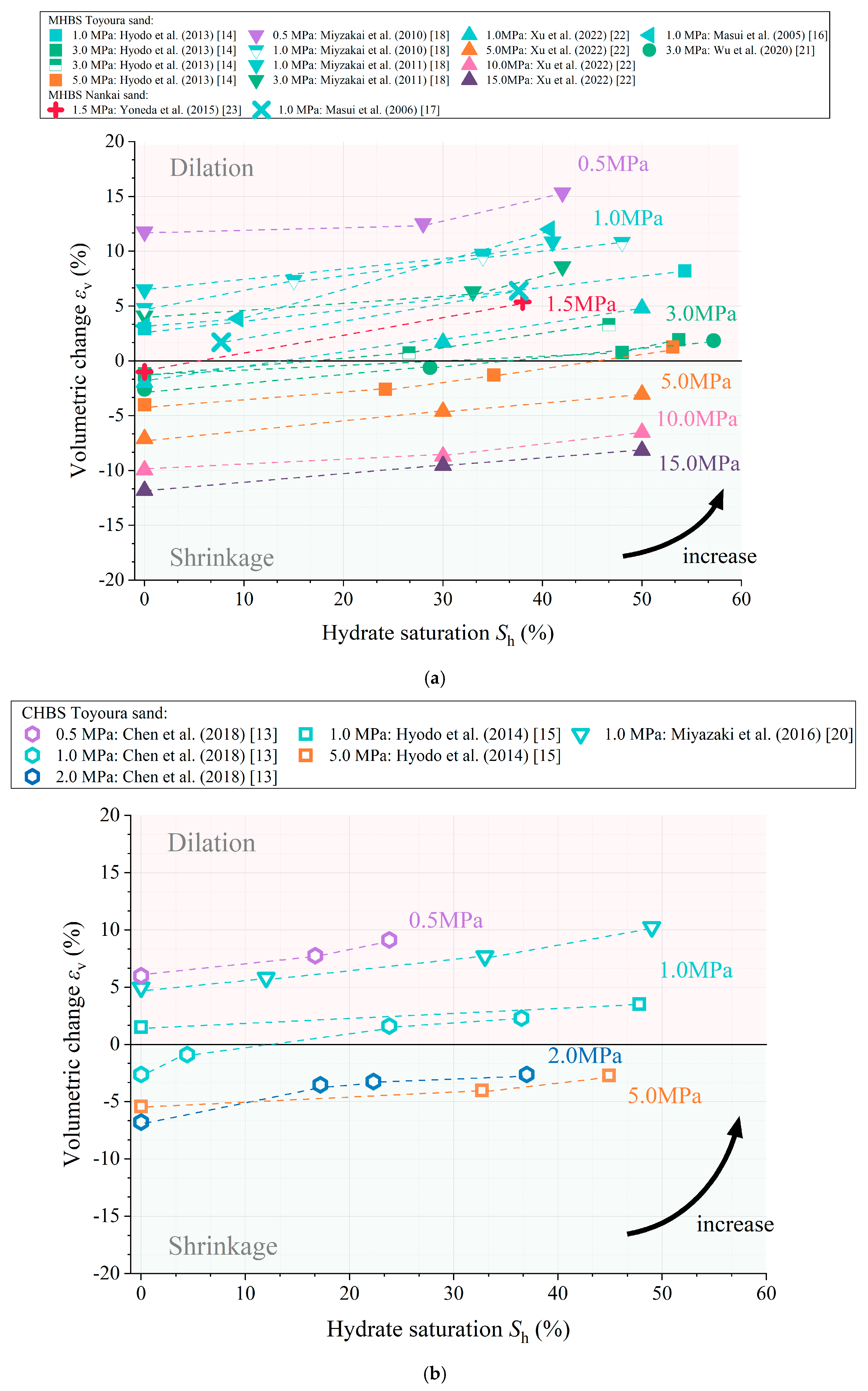
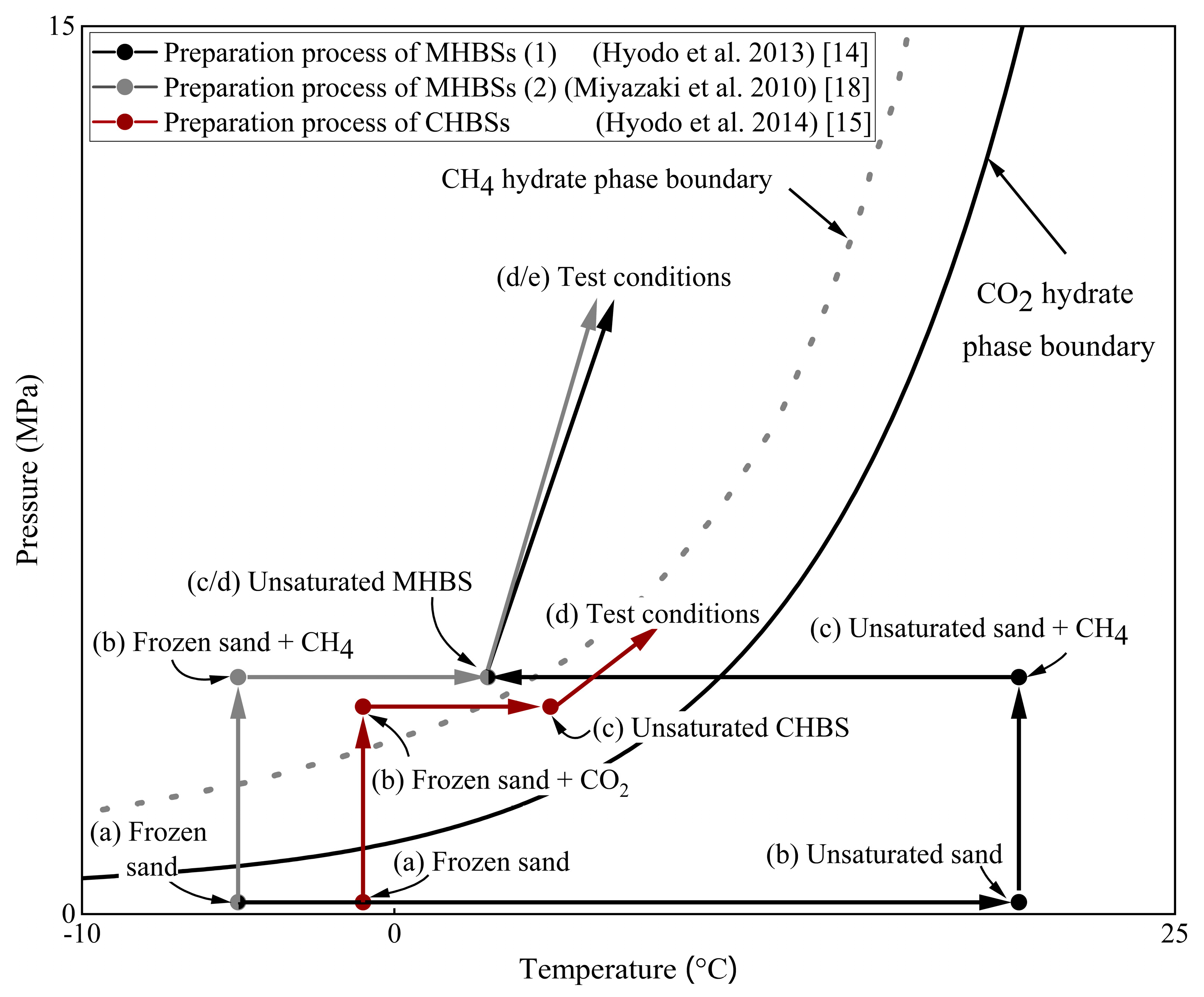
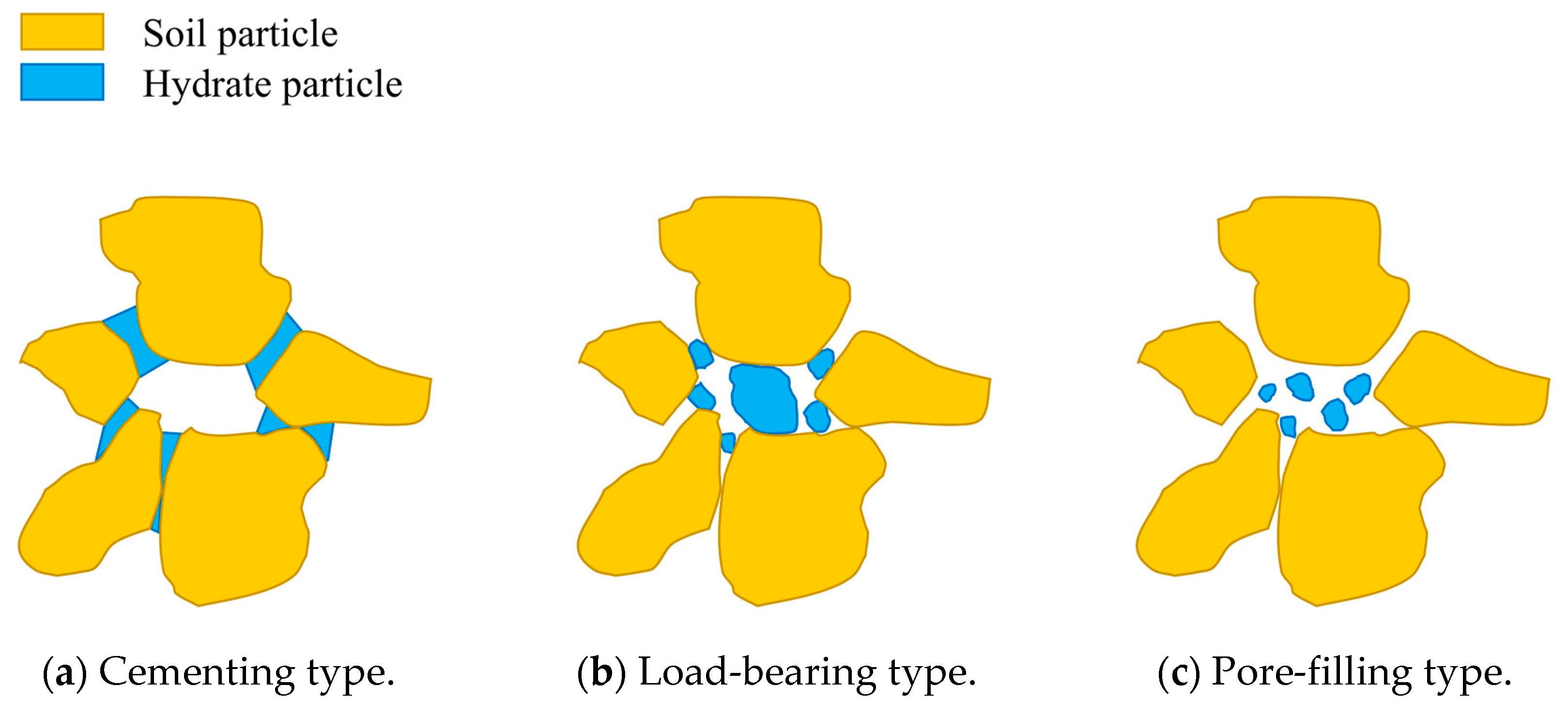
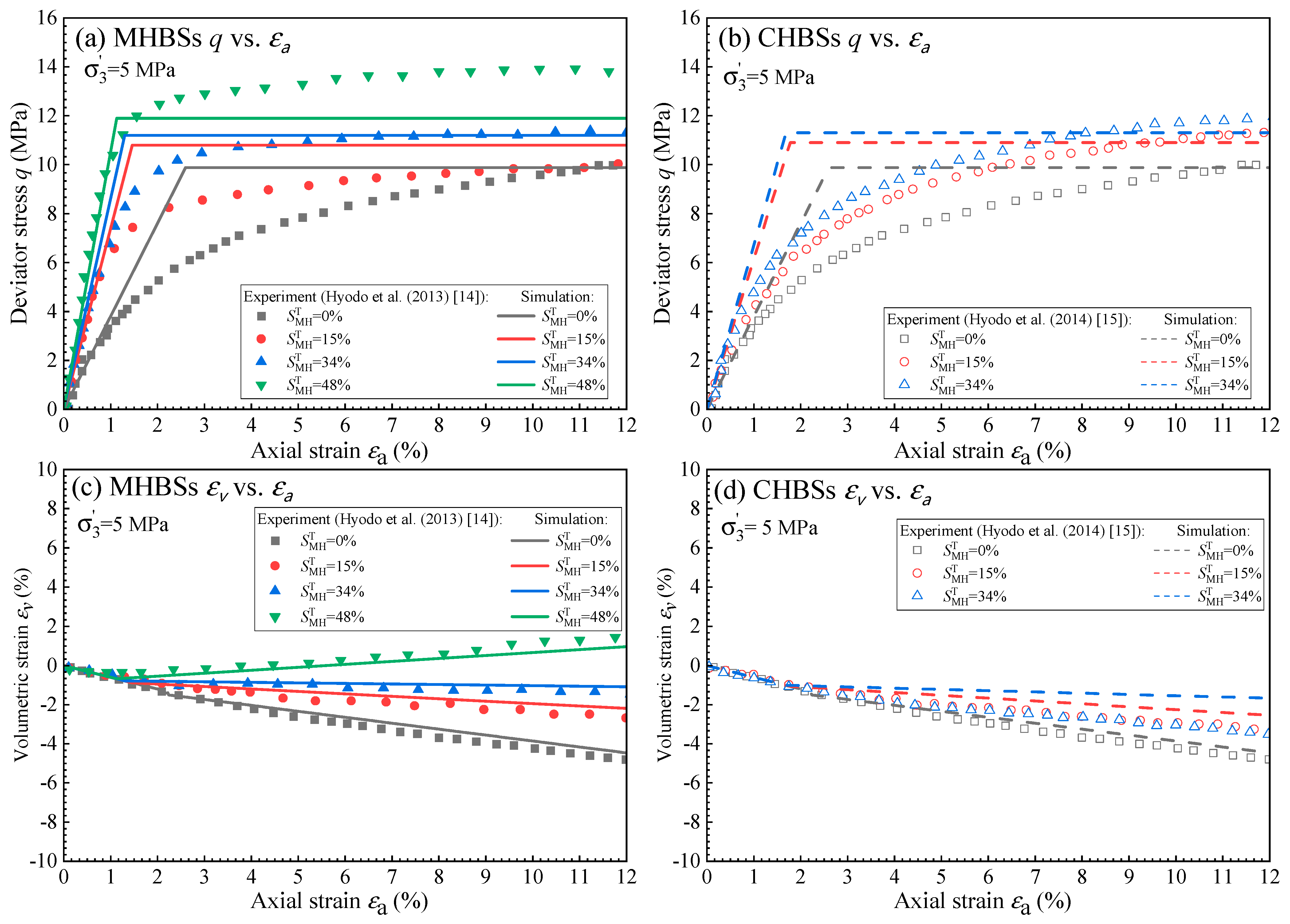
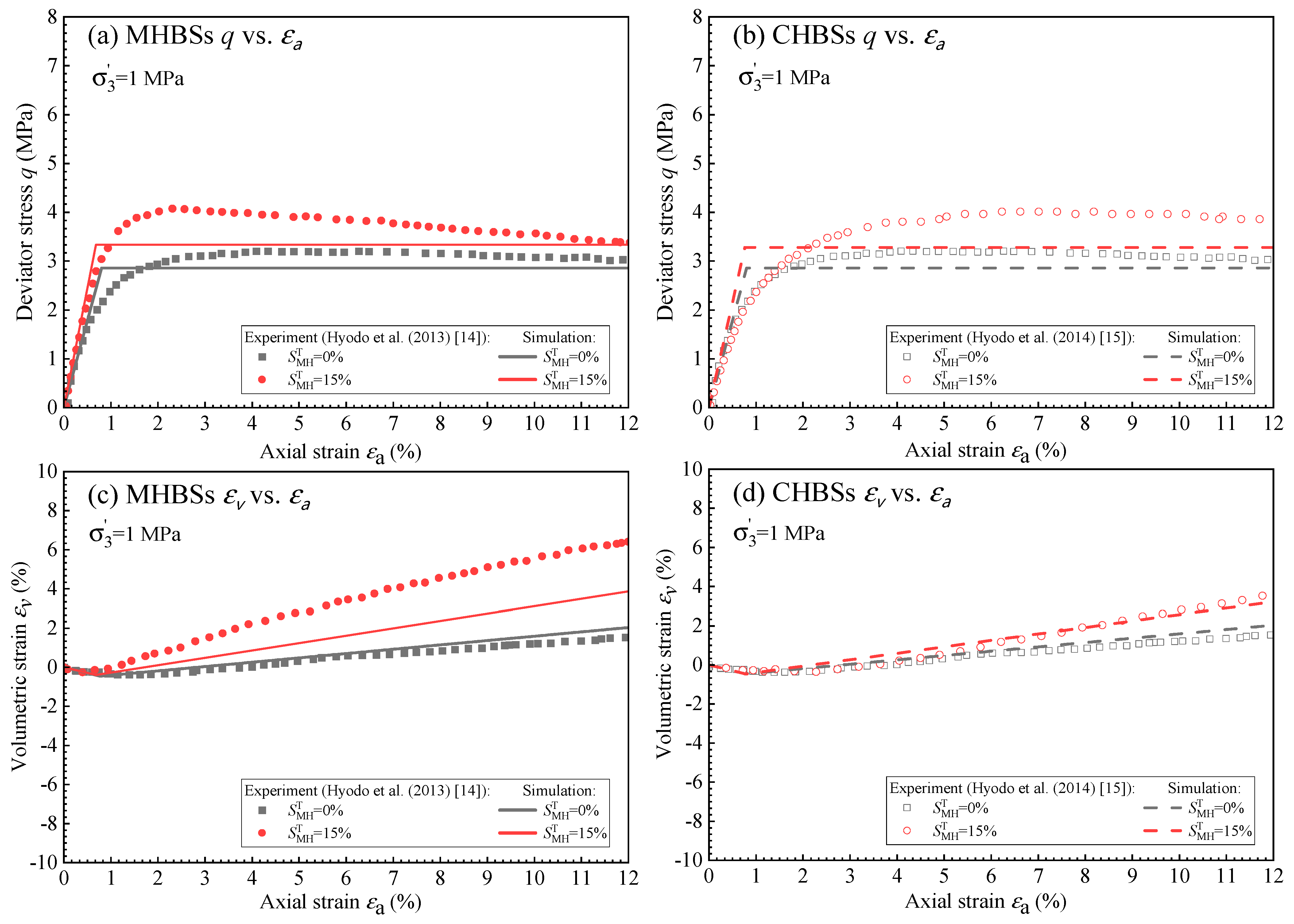
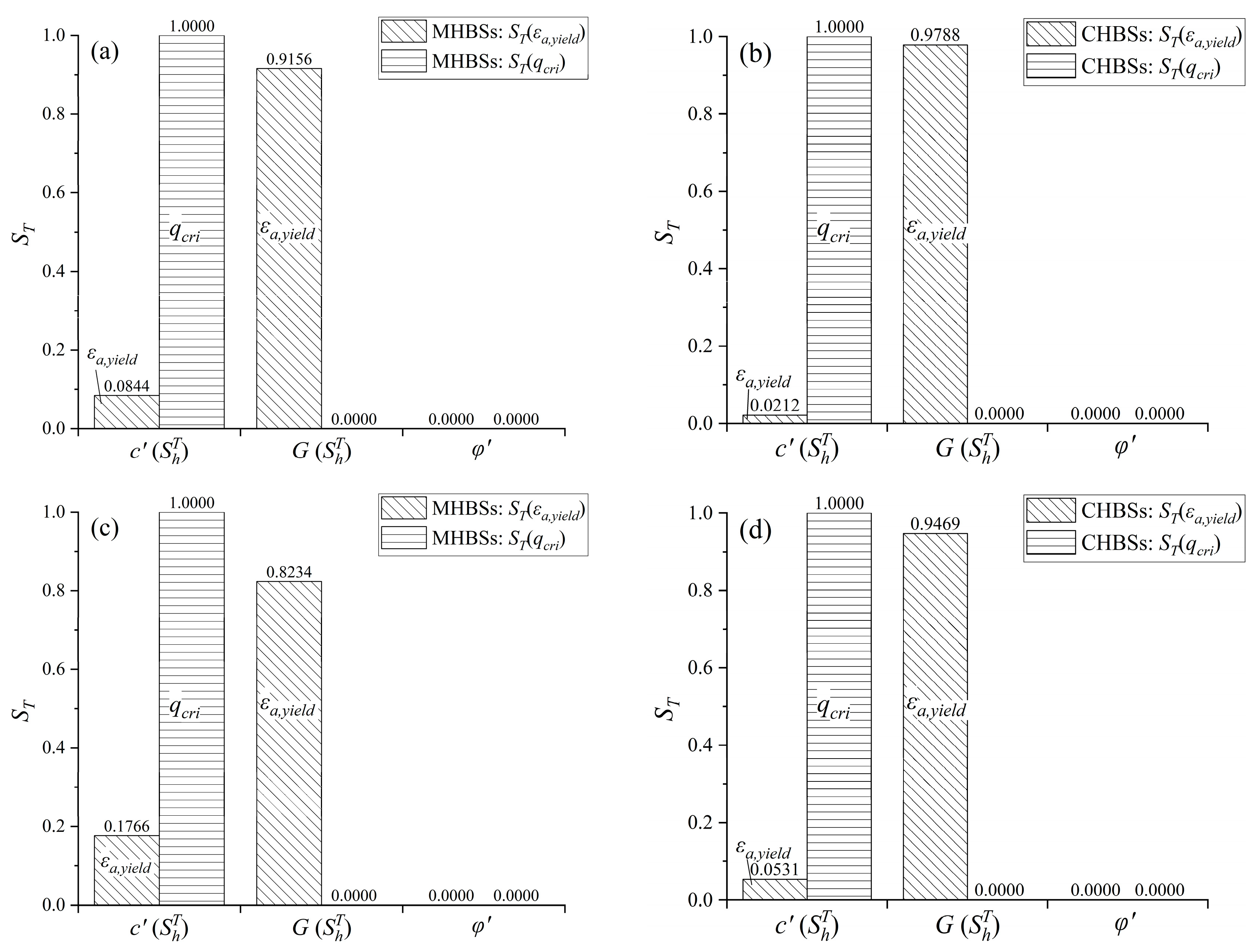
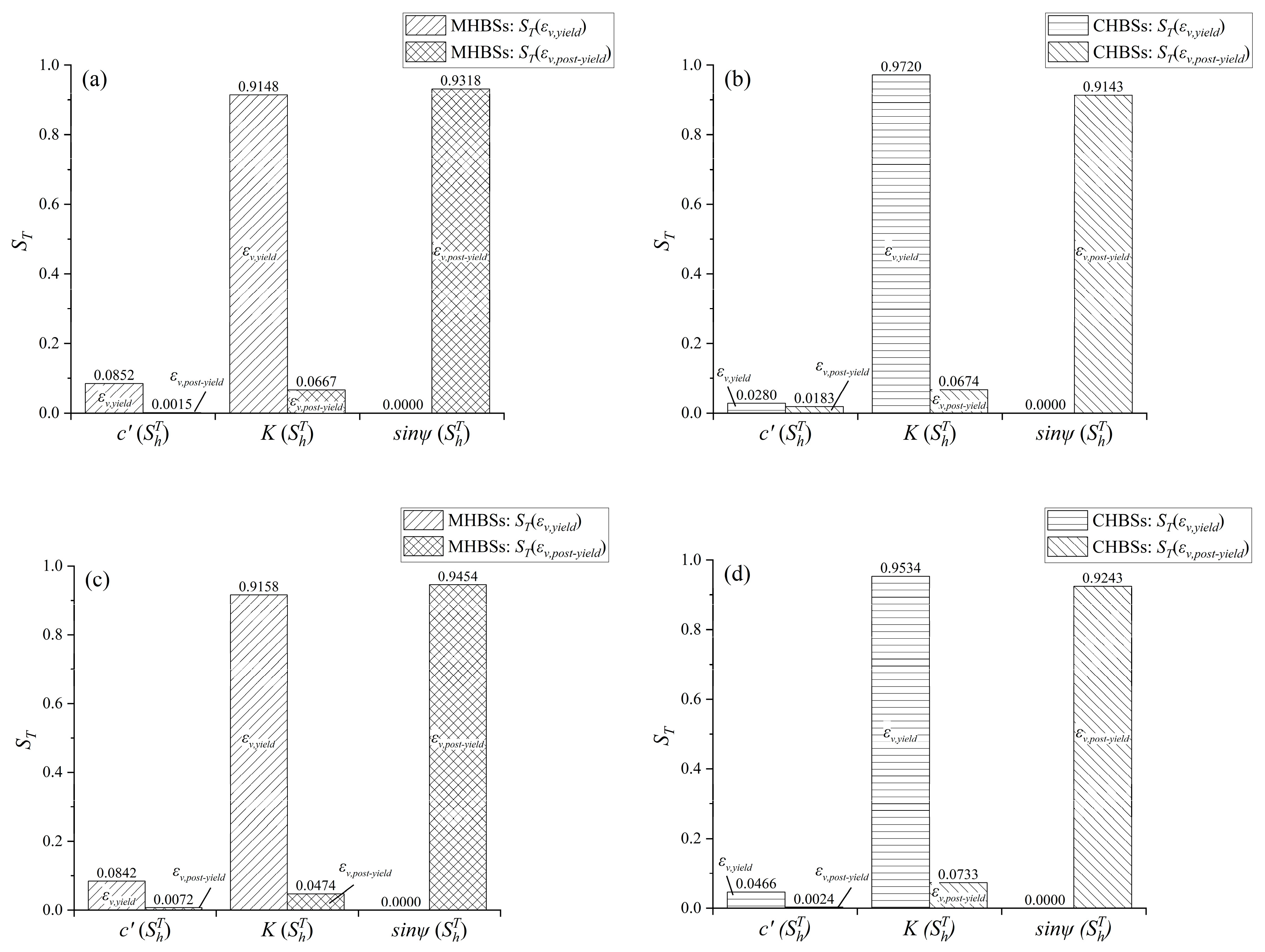
Basic Model Type	Reference	Application Type	Feature/Description	Source of Test Data	Achievement
Mohr–Coulomb model	Klar, Soga, and Ng [30]	Artificial methane hydrate-bearing sediments	c, E, and $\psi$ considered the influence of $S_h$. $\upsilon$ and $\varphi$ were assumed to be independent of $S_h$.	Ebinuma et al. [41]	The modified M-C model could describe the stress–strain behavior and the volumetric dilation with few parameters.
	Pinkert and Grozic [31]	Artificial methane hydrate-bearing sediments	c, E, and $\psi$ considered the influence of $S_h$ and ${\sigma }_3^{\prime }$. $\varphi$ was considered as a function of the plastic shear strain.	Miyazaki, Masui, Sakamoto, Aoki, Tenma, and Yamaguchi [19]

summarizes the application of the M-C model for GHBS simulations. Klar et al. (2010) [30] modified the dilatancy angle, cohesion, and elastic modulus in the M-C framework as a function of the hydrate saturation. To predict the strain-softening behavior and volumetric change of the MHBSs, both the hydrate saturation and effective confining pressure were introduced to the new expressions for the M-C yield criterion by Pinkert et al. (2014) [31]. However, these studies predominantly focused on MHBSs, and there is a noticeable gap in the investigation of the geomechanical response of CHBSs within the same constitutive model framework. This oversight is significant, as CHBSs exhibit unique characteristics, such as different hydrate morphologies and bonding mechanisms, which influence their stress–strain and volumetric responses. Given the increasing development of gas hydrate projects, there is a pressing need for models that can reasonably simulate the behaviors of various GHBS types.

To address these gaps, this study adopted the M-C model and modified it to simulate the stress–strain relationship and volumetric responses of MHBSs and CHBSs to incorporate the effects of different hydrate morphologies and effective confining pressures.

3. A Modified Mohr–Coulomb Model for Gas-Hydrate-Bearing Sediments

Miyazaki et al. (2016) [20] and Luo et al. (2020) [24] proposed that the differences in geomechanical behavior between MHBSs and CHBSs could be attributed to the microstructure of the hydrates and their interaction with the sediment particles. According to the hydrate crystal growth pattern in pore spaces of sediment, the hydrate morphology can be classified into three major types: “Cementing” type, “Load-bearing” type, and “Pore-filling” type [27,42,43,44]. A schematic diagram of the three hydrate morphology types is shown in Figure 4. In the cementing-type morphology, gas hydrates act as bonds connecting soil particles, which increases the sediment strength and stiffness and leads to larger significant volumetric dilatancy. In the load-bearing-type morphology, the growth of gas hydrate mainly occurs at the surface between the soil particles, providing extra support to the sediment skeleton [25,45]. As for the pore-filling-type morphology, the hydrate floats in the pore with no contact between soil particles and provides minimal geomechanical enhancement [33]. It is important to highlight that hydrate formation occurs within different pore spaces of the sediment, leading to the coexistence of these three hydrate forms in GHBSs [46]. Therefore, the total hydrate saturation $S_h^T$ is divided into three portions: $S_h^{cem}$ for the “Cementing” type, $S_h^{LB}$ for the “Load-bearing” type, and $S_h^{PF}$ for the “Pore-filling” type, and can be expressed as in Equation (1) below [47]:

$$S_h^T=S_h^{Cem}+S_h^{LB}+S_h^{PF}=\alpha S_h^T+\beta S_h^T+\gamma S_h^T$$

(1)

where $\alpha , \beta , \mathrm{and} \gamma$ are the hydrate morphology ratios, which are defined as the fractions of the cementing-type hydrate saturation, load-bearing-type hydrate saturation, and pore-filling-type hydrate saturation in the total hydrate saturation, respectively. The summation of $\alpha , \beta , \mathrm{and} \gamma$ equals to one.

From the tests shown in Figure 1 and Figure 2, the effective confining pressure and content of hydrate in GHBSs could improve the shear modulus G, effective cohesion $c^{\prime }$, and dilatancy angle $\psi$ [15,16,20]. The general geomechanical parameters of hydrate-bearing sediments can be considered in terms of both the host sand and hydrate enhancement as follows [31]:

$$G=G_h+G_s$$

(2)

$$c^{\prime }=c_h^{\prime }+c_s^{\prime }$$

(3)

$$sin\psi =sin{\psi }_h+sin{\psi }_s$$

(4)

where the $G_s$, $c_s^{\prime }$, and $sin{\psi }_s$ are the parameters obtained from the tests of a hydrate-free sample, while the $c_s^{\prime }$ is zero for the host sand sample. $G_h$, $c_h^{\prime }$, and ${\psi }_h$ are the hydrate enhancements for the shear modulus, effective cohesion, and dilatancy angle, which are further considered as a function of the hydrate morphology and effective confining pressure ${\sigma }_3^{\prime }$ as follows:

$$G_h=\left(C_G^{Cem}{S}_h^{Cem}+C_G^{LB}{S}_h^{LB}+C_G^{PF}{S}_h^{PF}\right){\sigma }_3^{\prime }=\left(C_G^{Cem}\alpha +C_G^{LB}\beta +C_G^{PF}\gamma \right){\sigma }_3^{\prime }{S}_h^T$$

(5)

$$c^{\prime }=\left(C_{c\prime }^{Cem}{S}_h^{Cem}+C_{c\prime }^{LB}{S}_h^{LB}+C_{c\prime }^{PF}{S}_h^{PF}\right){\sigma }_3^{\prime }=\left(C_{c\prime }^{Cem}\alpha +C_{c\prime }^{LB}\beta +C_{c\prime }^{PF}\gamma \right){\sigma }_3^{\prime }{S}_h^T$$

(6)

$$sin{\psi }_h=\left(C_{{\psi }_h}^{Cem}{S}_{CEM}^H+C_{{\psi }_h}^{LB}{S}_{LB}^H+C_{{\psi }_h}^{PF}{S}_{PF}^H\right){\sigma }_3^{\prime }=\left(C_{\psi }^{cem}\alpha +C_{\psi }^{LB}\beta +C_{\psi }^{PF}\gamma \right){\sigma }_3^{\prime }{S}_h^T$$

(7)

where $C_G^{Cem}$, $C_G^{LB}$, and $C_G^{PF}$ are the parameters for the degree of contribution in $G_h$ with respect to the cementing-type hydrate, load-bearing-type hydrate, and pore-filling-type hydrate, respectively; $C_{c\prime }^{Cem}$, $C_{c\prime }^{LB}$, and $C_{c\prime }^{PF}$ are the degrees of contribution in $c^{\prime }$ with respect to the cementing-type hydrate, load-bearing-type hydrate, and pore-filling-type hydrate, respectively; $C_{{\psi }_h}^{Cem}$, $C_{{\psi }_h}^{LB}$, and $C_{{\psi }_h}^{PF}$ are the degrees of contribution in $sin{\psi }_h$ with respect to the cementing-type hydrate, load-bearing-type hydrate, and pore-filling type hydrate, respectively. These parameters were further determined from fitting experimental data.

Incorporating the hydrate- and confining-pressure-related $c^{\prime }$ (Equation (6)) into the M-C model, the modified yielding function can be obtained as follows:

$$f=q-{\displaystyle \frac{6sin{\phi }^{\prime }}{3-sin{\phi }^{\prime }}}{p}^{\prime }-{\displaystyle \frac{6c^{\prime }\left(S_h^T,{\sigma }_3^{\prime }\right)cos{\phi }^{\prime }}{3-sin{\phi }^{\prime }}}$$

(8)

where q is the deviatoric stress, $p^{\prime }$ is the average effective principal stress, and ${\phi }^{\prime }$ is the drained internal friction angle. Note here that ${\phi }^{\prime }$ is generally assumed to be independent of the hydrate saturation or confining pressure [1,15,48].

Considering the hydrate- and confining-pressure-related $\psi$ (Equations (4) and (7)), the plastic potential function g (in unassociated flow rule) can be expressed as follows:

$$g=q-{\displaystyle \frac{6sin\psi \left(S_h^T,{\sigma }_3^{\prime }\right)}{3-sin\psi \left(S_h^T,{\sigma }_3^{\prime }\right)}}{p}^{\prime }$$

(9)

The modified Mohr–Coulomb model was then applied to simulate the drained triaxial compression tests for MHBSs and CHBSs by both Hyodo et al. (2013, 2014) [14,15] and Miyazaki et al. (2010, 2016) [18,20].

In the modified M-C model base simulation of laboratory testing, the least squares method was used to obtain the model parameters, as shown in

Table 2. Hydrate morphology ratios for MHBSs and CHBSs.

Type	Reference	Effective Confining Pressure/MPa	Cementing Type/α	Load-Bearing Type/β	Drainage Condition
MHBSs	Miyazaki et al. (2010) [18]	1.0	0.7	0.3	Drained
	Hyodo et al. (2013) [14]	1.0
	Hyodo et al. (2013) [14]	5.0
CHBSs	Miyazaki et al. (2016) [20]	1.0	0.2	0.8	Drained
	Hyodo et al. (2014) [15]	1.0
	Hyodo et al. (2014) [15]	5.0

and

Table 3. Hydrate material fitting parameters for proposed modified constitutive model.

Type	Effective Confining Pressure/MPa	Reference	Hydrate Parameters
			${\mathit{G}}_{\mathit{h}}$/MPa		$\mathit{c}\prime$/MPa		$\mathit{s}\mathit{i}\mathit{n}{\mathit{\psi }}_{\mathit{h}}$
			${\mathit{C}}_{\mathit{G}}^{\mathit{C}\mathit{e}\mathit{m}}$	${\mathit{C}}_{\mathit{G}}^{\mathit{L}\mathit{B}}$	${\mathit{C}}_{\mathit{c}\prime }^{\mathit{C}\mathit{e}\mathit{m}}$	${\mathit{C}}_{\mathit{c}\prime }^{\mathit{L}\mathit{B}}$	${\mathit{C}}_{{\mathit{\psi }}_{\mathit{h}}}^{\mathit{C}\mathit{e}\mathit{m}}$	${\mathit{C}}_{{\mathit{\psi }}_{\mathit{h}}}^{\mathit{L}\mathit{B}}$
MHBSs	1.0	Miyazaki et al. (2010) [18]	430	110	1.0	0.4	0.3	0.2
CHBSs	1.0	Miyazaki et al. (2016) [20]
MHBSs	1.0	Hyodo et al. (2013) [14]	127	14	0.26	0.13	0.1	0.08
CHBSs	1.0	Hyodo et al. (2014) [15]
MHBSs	5.0	Hyodo et al. (2013) [14]
CHBSs	5.0	Hyodo et al. (2014) [15]

. Table 2 lists the hydrate morphology ratios for the MHBS and CHBS samples in Hyodo et al. (2013, 2014) [14,15] and Miyazaki et al. (2010, 2016) [18,20]. These data were derived from laboratory tests using the partially water-saturated (PWS) sampling method under effective confining pressures of 1 MPa and 5 MPa. Note that considering the pore-filling-type hydrate often transforms to a load-bearing-type hydrate when in shear [45], and hence could have little effect on the geomechanical enhancement in high-pressure conditions; thus, $\gamma$ was set to 0 for simplification in this study. From Table 2, the cementing type ($\alpha$) was the larger hydrate proportion in the MHBSs, while the load-bearing type ($\beta$) was larger in the CHBSs, which is in good agreement with the microscopic studies of gas hydrate by Zhang et al. (2020) [49] and Sloan et al. (2007) [26]. Their studies found that MHBSs had a higher occupancy in their small cages compared with CHBSs, which may contribute to the more cementing morphology observed in the MHBSs. Table 3 presents the enhancement of degree parameters for each morphology type in the MHBSs and CHBSs. The degree of the contribution parameter results was consistent with the observation [16,50,51] that cementing-type hydrate contributes more to the strength and dilation than loading-bearing-type hydrate.

Figure 5, Figure 6 and Figure 7 compare the modified M-C model simulations with laboratory drained triaxial compression tests on the MHBSs and CHBSs. The test data were sourced from Miyazaki et al. (2010, 2016) [18,20] (Figure 5) and Hyodo et al. (2013, 2014) [14,15] (Figure 6 and Figure 7) under different effective confining pressures and hydrate saturations. The trends of q-${\epsilon }_a$ and ${\epsilon }_v$-${\epsilon }_a$ with increasing hydrate saturation and confining pressure in the numerical simulation generally matched with the laboratory results for both the MHBSs and CHBSs. These results highlight the model’s reliability at capturing the geomechanical properties of both the MHBSs and CHBSs, like critical strength, stiffness, and volumetric change, under different conditions. Despite the challenges of the M-C model in simulating strain-softening, the model proposed in this study represents a significant step forward in modeling both MHBSs and CHBSs within a unified framework. This advancement sets a solid foundation for future studies aiming to predict the geomechanical responses of different GHBS reservoirs.

4. Global Sensitivity Analysis (GSA) for Gas-Hydrate-Bearing Sediments Parameters

This section further describes the global sensitivity analysis to evaluate the influence of hydrate-incorporated constitutive model parameters (i.e., effective cohesion $c^{\prime }$, shear modulus G, bulk modulus K, internal friction ${\phi }^{\prime }$, and dilatancy angle $\psi$) on the geomechanical responses of MHBSs and CHBSs.

4.1. Sobol’s Method of Global Sensitivity Analysis

Sobol (1993) [52] proposed a global sensitivity analysis method called Sobol’s method that involves decomposing the variance to obtain the Sobol’s sensitivity indexes. This method decomposes the output variances of the model or system into fractions that are regarded as the sum of the variances of the input parameters in increasing dimensionality. The sensitivity indexes of Sobol’s method are used to indicate the contribution of each input factor to the changes in the output, or the contribution from interaction with different factors in the model f(x). A schematic diagram of Sobol’s method is shown in Figure 8. The model parameters serve as input factors to calculate their respective contributions to geomechanical responses.

The input model parameters G, K, ${\phi }^{\prime }$, $\psi$, and $c^{\prime }$ are independently and uniformly distributed within an increasing dimensions space $T^d=(x|0\le x_i\le 1;i=1,\dots ,d)$, where i represents different model parameters. The first-order sensitivity index can be written as below:

$$S_i={\displaystyle \frac{D_i}{D}}$$

(10)

where $S_i$ is a measure for the contribution of the variances of the model parameter $x_i$ in the total variance of model parameters, which indicates the primary impacts of $x_i$ to the total output variance; $D_i$ is the variance of the input model parameter $x_i$; and D is the total variance of the model parameters. Similarly, $S_{ij}$ (i $\ne$ j) is defined as the second-order sensitivity index, which is used to calculate the contribution from the interaction between $x_i$ and $x_j$:

$$S_{ij}={\displaystyle \frac{D_{ij\left(i\ne j\right)}}{D}}$$

(11)

where $D_{ij}$ is the common variance of the input model parameters $x_i$ and $x_j$. Finally, the total-order sensitivity index of $x_i$ is defined as the sum of its various-order sensitivity indices:

$$S_{Ti}=S_i+S_{ij\left(i\ne j\right)}+\dots +S_{1\dots i\dots s}$$

(12)

The $S_{Ti}$ measures all the contributions to the model output of the input model parameter $x_i$. Due to the fact that the interaction effect between $x_i$ and $x_{\mathrm{j}}$ is counted in both $S_{Ti}$ and $S_{T\mathrm{j}}$, the total-order sensitivity index of all the input model parameters is equal to 1:

$${\displaystyle \sum }_{\mathrm{i}=1}^{\mathrm{d}}{S}_{\mathrm{T}i}=1$$

(13)

In practical applications, since the exact solution of Sobol’s method is difficult to obtain, the Monte Carlo-approach-based integration is used to solve the integral due to its simplicity and reliability. The total variance of the input model parameters, D, and the variance of the input model parameter $x_i$, $D_i$, can be expressed relatively easily by using the Monte Carlo approach:

$$f_0={\displaystyle \int }{f}^2\left(x\right)dx\approx {\displaystyle \frac{1}{N}}{\displaystyle \sum }_{k=1}^N{f}^2\left(x_k\right)$$

(14)

$$D={\displaystyle \int }{f}^2\left(x\right)dx-f_0^2\approx {\displaystyle \frac{1}{N}}{\displaystyle \sum }_{k=1}^N{f}^2\left(x_k\right)-f_0^2$$

(15)

$$D_i=D-{\displaystyle \frac{1}{2}}{[f\left(x\right)-f\left(x_i,x_{-i}^{\prime }\right)]}^{2}dxd{x}_{-i}^{\prime }\approx D-{\displaystyle \frac{1}{2N}}{\displaystyle \sum }_{k=1}^N{[f\left(x_k\right)-f\left(x_{ik}-x_{-ik}^{\prime }\right)]}^2$$

(16)

where N is the number of iterative calculations, which depends on the size of the input set, $x_{-i}^{\prime }$ is the parameter combination complementary to $x_i$, and $f_0$ is the constant term in the analysis of the variance of f(x) [53].

Sobol’s method requires a substantial amount of sampling data points to be generated for the constitutive model parameters, covering its value range. First of all, the boundary of the parameter space should be determined. Given the limited experimental data in the database, the boundary is determined by considering the 95% confidence interval within the normal distribution of the original data. When generating samples, it is assumed that all the parameters are statistically independent, and the sampling points have an equal distribution throughout the sampling space.

4.2. Results of Global Sensitivity Analysis

The analysis of the total-order sensitivity index $S_{\mathrm{T}}$ for the soil parameters G, K, ${\phi }^{\prime }$, $\psi$, and $c^{\prime }$ revealed distinct geomechanical behaviors in the MHBSs and CHBSs. For the q−${\epsilon }_a$response, the total-order sensitivity index $S_{\mathrm{T}}$ of the soil parameters c’, G, and ${\phi }^{\prime }$ to the critical strength ($q_{\mathrm{cri}}$) and the yielding axial strain (${\epsilon }_{a,yield}$) are presented in Figure 9. These values correspond to the tests conducted by Miyazaki et al. (2010) [18] (Figure 9a), Miyazaki et al. (2016) [20] (Figure 9b), Hyodo et al. (2013) [14] (Figure 9c), and Hyodo et al. (2014) [15] (Figure 9d).

As illustrated in Figure 9, the $S_{\mathrm{T}}\left({\epsilon }_{a,yield}\right)$ of G was significantly larger than that of c’, with a mean value of approximately 0.92, indicating that G played a primary role in influencing the variation of the yield axial strain. A comparison between the MHBSs and CHBSs showed that the $S_{\mathrm{T}}\left({\epsilon }_{a,yield}\right)$ of G was greater in the CHBSs, particularly in Figure 9c,d, where its value for the CHBSs was 1.15 times higher than that for the MHBSs. Conversely, the $S_{\mathrm{T}}\left({\epsilon }_{a,yield}\right)$ of c’ was higher in the MHBSs. These findings suggest that ${\epsilon }_{a,yield}$ in the CHBSs was predominantly influenced by G, while c’ had a more significant effect on ${\epsilon }_{a,yield}$ in the MHBSs. Furthermore, the $S_{\mathrm{T}}\left(q_{\mathrm{cri}}\right)$ of c’ was equal to 1, demonstrating that $q_{\mathrm{cri}}$ was exclusively governed by effective cohesion (c′). This result reflects the assumption that the internal friction angle (${\phi }^{\prime }$) was unaffected by hydrate variations.

In the ${\epsilon }_v$−${\epsilon }_a$ response, the yield volumetric strain (${\epsilon }_{v,yield}$) and the change in volumetric strain after yielding (${\epsilon }_{v,post-yield}$) characterized the volume change. Figure 10 presents the total-order sensitivity index $S_{\mathrm{T}}$ of the parameters c’, K, and sin$\psi$ with respect to ${\epsilon }_{v,yield}$ and ${\epsilon }_{v,post-yield}$. $S_{\mathrm{T}}$ in Figure 10 corresponds to the same tests as in Figure 9.

For ${\epsilon }_{v,yield}$, the $S_{\mathrm{T}}\left({\epsilon }_{v,yield}\right)$ of K exceeded 0.9, over 10 times the value of c’ for both the MHBSs and CHBSs, highlighting the dominant role of K in controlling ${\epsilon }_{v,yield}$. Additionally, the $S_{\mathrm{T}}\left({\epsilon }_{v,yield}\right)$ of sin$\psi$ was zero because sin$\psi$ does not contribute during the elastic stage of the ${\epsilon }_v$−${\epsilon }_a$ response. Notably, the $S_{\mathrm{T}}\left({\epsilon }_{v,yield}\right)$ of K in the CHBSs was, on average, 0.05 larger and approximately 5% greater. In contrast, the $S_{\mathrm{T}}\left({\epsilon }_{v,yield}\right)$ of c’ exhibited larger values in the MHBSs, where its impact was comparable with its role in controlling ${\epsilon }_{a,yield}$. After the volumetric strain yielding, ${\epsilon }_{v,post-yield}$ was primarily governed by sin$\psi$. For both the MHBSs and CHBSs, the $S_{\mathrm{T}}\left({\epsilon }_{v,post-yield}\right)$ of sin$\psi$ exceeded 0.90, whereas the combined $S_{\mathrm{T}}\left({\epsilon }_{v,post-yield}\right)$ values of c’ and K remained below 0.1. The difference in the $S_{\mathrm{T}}\left({\epsilon }_{v,post-yield}\right)$ of sin$\psi$ between the MHBSs and CHBSs was negligible, with sin$\psi$ being approximately 0.02 higher in the MHBSs.

The sensitivity analyses underscored the distinct roles of soil parameters in shaping the geomechanical responses of the MHBSs and CHBSs. These findings enhanced the application of constitutive models and deepened the understanding of the geomechanical behavior of different GHBSs.

5. Conclusions

This paper presents a modified elastic–plastic Mohr–Coulomb (M-C) model for gas hydrate-bearing sediments (GHBSs). Specifically, the model is applied to methane hydrate-bearing sediments (MHBSs) and carbon dioxide hydrate-bearing sediments (CHBSs) to account for differences in the hydrate morphology and effective confining pressure. It incorporates three main hydrate morphology types: cementing, load-bearing, and pore-filling. Based on these morphology types, the total hydrate saturation was divided into three corresponding types defined by the hydrate morphology ratio. Additionally, the model integrates the effects of the effective confining pressure on the hydrate-related soil parameters. The simulation results effectively reproduced the geomechanical behaviors of the MHBSs and CHBSs observed in the laboratory tests. For the CHBS sample, the simulations indicated that the load-bearing morphology was dominant, with a hydrate morphology ratio of 0.8, compared with 0.3 for the MHBSs. The numerical simulations demonstrated that differences in the geomechanical responses between the MHBSs and CHBSs could be quantitatively attributed to variations in the hydrate morphology.

Additionally, a variance-based global sensitivity analysis, conducted using Sobol’s method, underscored the significance of hydrate-related soil parameters in the modified M-C model for both the MHBSs and CHBSs. The hydrate-related effective cohesion (c’) primarily influenced the critical strength ($q_{\mathrm{cri}}$), while the hydrate-related shear modulus (G) predominantly affected the yield axial strain (${\epsilon }_{a,yield}$). The total-order sensitivity index $S_{\mathrm{T}}$ of G for ${\epsilon }_{a,yield}$ could be up to 1.15 times higher in the CHBSs than in the MHBSs. For the volumetric responses, the hydrate-related bulk modulus (K) strongly influenced the yield volumetric strain (${\epsilon }_{v,yield}$), with $S_{\mathrm{T}}$ values that exceeded 0.9—more than 10 times that of c’. Furthermore, the K in CHBS was, on average, 0.05 larger, representing an approximate 5% increase over that in the MHBSs. In the post-yield stage, the hydrate-related dilatancy angle (sinψ) predominantly governed the post-yield volumetric strain (${\epsilon }_{v,post-yield}$), with $S_{\mathrm{T}}$ values that exceeded 0.90 for both the MHBSs and CHBSs. By contrast, the combined contributions of c’ and K remained below 0.1. The quantitative analysis of the relative importance helped to better understand the role of individual hydrate-related soil parameters and revealed the most relevant variants for the MHBS and CHBS geomechanical behavior.

Despite the inherent limitations of the M-C model itself in describing strain-softening behavior, it provides a valuable framework for advancing the development of a unified constitutive model for GHBSs. Future improvements could focus on incorporating mechanisms to capture the post-peak stress behavior, such as strain localization effects or a softening modulus. Enhancing the model’s predictive capabilities in this area would facilitate its application to real-world scenarios, including ${\mathrm{CO}}_2$-injection-induced methane production from deep sediments.