An Assessment of the Residual Stress of Pipelines Subjected to Localized Large Deformations

Abstract

Subsea pipelines subjected to impacts are prone to generating significant deformations and residual stresses, which could reduce their structural integrity and increase the risk of failure. This paper introduces analytical and numerical frameworks aimed at predicting residual stress behavior induced by subsea pipeline impacts. An empirical formula to regulate residual stress levels in extensively deformed submarine pipelines is derived through a parameter-fitting method. This formula enables the swift and accurate computation of the residual stress magnitudes in such pipelines. Abaqus is employed to simulate the residual stresses in large-deformation submarine pipelines. The results of the finite element analysis are validated through experimental work. A comprehensive database is constructed via the finite element method to fit an empirical formula for residual stresses in large-deformation submarine pipelines. The empirical formula places particular emphasis on the influence of the diameter-to-thickness ratio and dent depth on the residual stresses. It is crucial for pipeline design and maintenance.

1. Introduction

Subsea pipelines are crucial for offshore oil and gas resource exploitation and are integral to offshore hydrocarbon transportation. This serves as the backbone of marine hydrocarbon engineering. During their operational lifespan, these pipelines frequently endure impacts from debris, causing substantial structural deformation and residual stress. This residual stress, a self-balancing pattern, does not affect the static load strength of pipeline steel but triggers premature plastic deformation in the pipeline structure. This, in turn, reduces the pipeline stiffness and stability, diminishing the resistance to fatigue failure and impact toughness. Leakage incidents result in significant economic losses to production activities and environmental contamination, emphasizing the critical attention given to residual stress issues in these pipelines.

It is crucial to categorize residual stress measurement techniques on the basis of their impacts on test samples. These methods fall into three categories: fully destructive, partially destructive, and non-destructive measurements [1,2]. Fully destructive techniques, which release all residual stresses, have historically involved mechanical methods such as cutting and sectioning test samples [3,4,5]. While highly precise, these methods are labor-intensive and operationally complex. Partially destructive methods minimize the damage to test samples while providing accurate and reliable measurements. These methods are commonly employed for the onsite evaluation of structural components [6,7,8], with the blind hole method being a prominent example. Non-destructive measurement techniques encompass various approaches, including magnetic testing, X-ray analysis, and ultrasonic examination. These methods share the characteristic of not damaging test samples. Magnetic testing relies on changes in magnetic permeability due to residual stress and offers a cost-effective approach [9,10,11,12]. Nevertheless, it has limitations, such as a restricted measurement depth and susceptibility to external interferences. X-ray testing relies on the response of a sample to X-ray exposure and is valuable because of its non-destructive and repeatable nature [13,14,15]. However, it is constrained by its limited measurement depth and sensitivity to various interferences. Ultrasonic testing distinguishes the residual stress distributions on the basis of a specimen’s varied response to ultrasonic waves [16,17], yet its effectiveness can be influenced by external factors, potentially affecting the measurement precision. Moreover, Peng et al. [18] developed a portable indentation instrument by solving for the biaxial residual stress components via the energy difference functions of two orthogonal indentations.

The residual stress distribution in pipelines is influenced by their fabrication processes, with variations arising from different techniques. Numerical simulations and experiments are employed to analyze the stress conditions experienced by pipelines during fabrication [19,20,21,22,23]. Forouzan et al. [24] used the finite element method to simulate submerged arc welding in large-diameter spiral pipes and the process of static hydraulic expansion. Furthermore, expanding the pipeline diameter effectively reduced the residual tensile stresses generated during the welding process. Hu [25] combined finite element analysis with experiments to research the stress–strain variations in large circular steel pipes during localized induction heating and bending, both in loading and unloading processes. Analyzing the pipeline stress conditions during manufacturing provides insights into the characteristics of residual stress variations [26,27,28]. These findings can be used to develop theoretical models for the distribution of residual stresses [27,29,30,31]. In addition,

Rissaki et al. [32] developed two artificial neural network (ANN) ensemble models to predict the through-thickness residual stress profiles in the weld center line (WCL). This contributes to the assessment of residual stresses during engineering processes.

The residual stresses in pipelines primarily result from significant deformations induced by pipeline dents in marine engineering. The prevalent research models involve hollow suspended tubular structures with fixed terminations subjected to perpendicular impacts in submarine pipeline dent investigations. These impacts lead to pipeline deformations, characterized by both overall bending and localized dimpling. Various equations have been devised, encompassing empirical, semiempirical, and analytically closed-form formulations, aimed at delineating the relationship between impact forces and the ultimate plastic dent depths [33,34,35,36,37,38,39]. These formulations are fundamentally grounded in the tenets of rigid plastic deformation theory and serve to establish the theoretical connection between the local plastic dent depths within the tubular structures and their resilience against impact forces. The validation of these theories is typically achieved through experimental assays and numerical simulations. Research efforts in the context of impact scenarios can be broadly classified into two categories: sharp-object impacts, as exemplified by investigations carried out by Fumes and Amdahl [37] and Wierzbicki and Suh [38], and planar impacts, which take into account the contact width, akin to the studies conducted by Amdahl [33] and Cho [40]. These investigations employ diverse methods to simulate impacts, including the use of sharp wedge-shaped or rectangular indenters. The relationships between the dent depth and impact force are intrinsically linked to the plastic properties of the pipeline material. Theoretical formulations typically focus on tubular structures with fixed ends and utilize rigid plastic theory to establish the relationship between the post-impact cross-sectional dent depth and impact force. While these studies offer valuable insights into the impact of falling objects on subsea pipelines, they often neglect the consideration of residual stresses within the pipelines after dent formation.

Investigations concerning pipeline dents have achieved considerable breadth, delving into the characterization of residual stress distributions and their attendant features subsequent to dent occurrence, alongside the formulation of relationships linking pipeline dents to the external loading conditions. However, in transitioning these scholarly insights into practical engineering utilization, the intricacies inherent within the compiled data and the resulting lack of directness continue to pose hurdles. A conspicuous void exists in the form of an easily comprehensible equation that intuitively encapsulates the nexus between the peak residual stress within the pipeline matrix and the extent of indentation, thereby serving to streamline engineering applications and decision-making processes. This paper combines finite element simulations with experimental investigations to scrutinize the properties of residual stresses in deeply deformed subsea pipelines. The central emphasis lies in the examination of the correlation between the dent depth and the residual stresses within these pipelines, ultimately culminating in the formulation of a mathematical model for the calculation of residual stresses in the context of profoundly deformed subsea pipelines. In Section 2, a finite element model for residual stresses in highly deformed subsea pipelines is established. In Section 3, model experiments on highly deformed subsea pipelines are presented, and experimental data are used to validate the finite element results. In Section 4, a parameter analysis of the characteristics of highly deformed subsea pipelines is conducted. On the basis of the finite element simulation results, a fitting procedure is applied to develop a residual stress formula. Finally, several conclusions are drawn in Section 5.

2. Experimental Investigation of Residual Stresses in Pipes

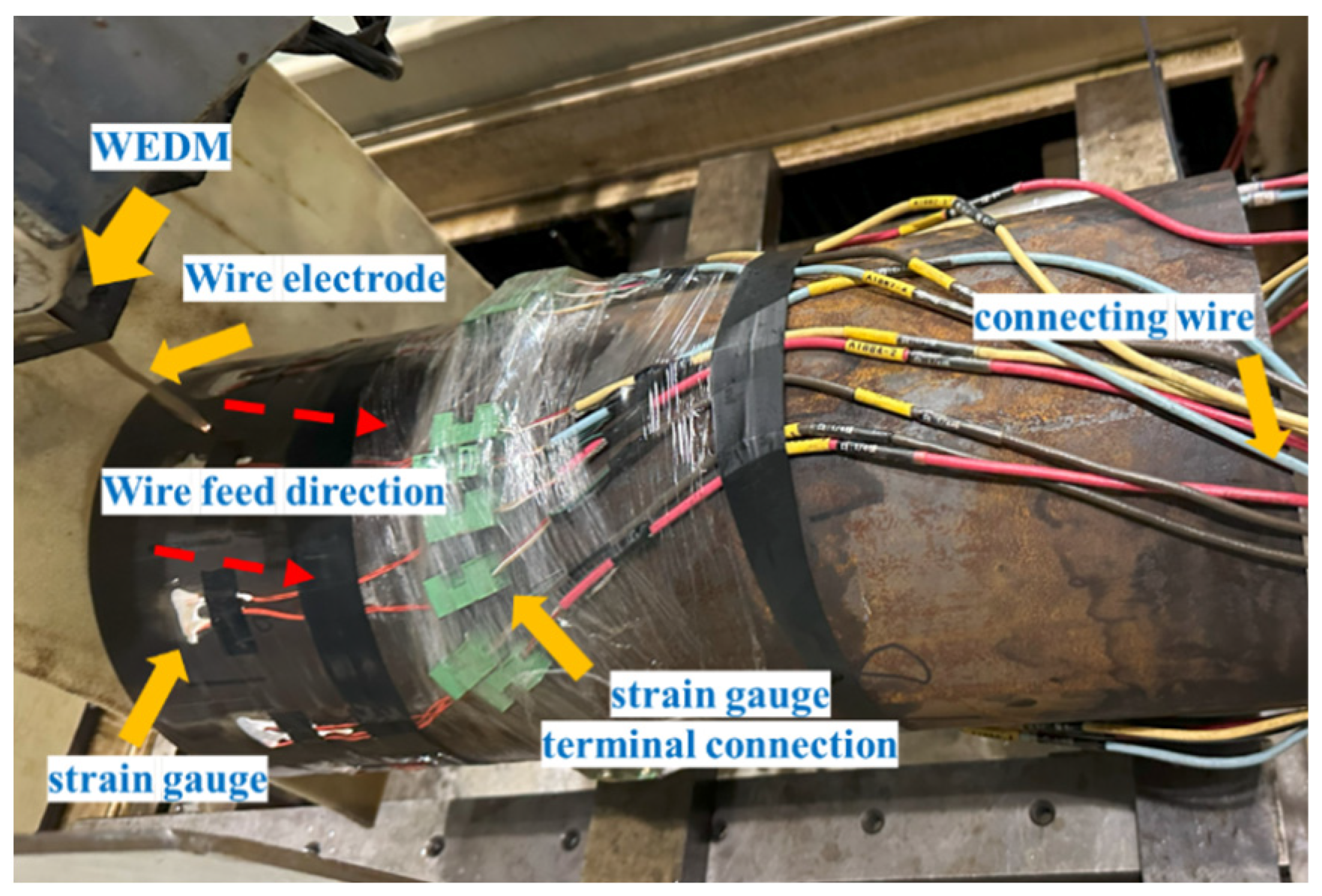
2.1. Model Test

The experiment comprises a pipeline model apparatus, which is responsible for simulating submarine pipelines in marine engineering; a pipeline pressure-loading device, which is used to replicate local dents occurring in actual engineering scenarios; and a measurement and data acquisition system, which is dedicated to quantifying the residual stresses generated after pipeline dents occur.

The X65 pipeline model is chosen to align with engineering practice. The data measurement and acquisition device comprises strain gauges, terminals, and strain-measuring instruments. In the experiment, the midpoint of the pipeline serves as the pressurized section and the cut section for the measurement of residual stress. The measurement points are evenly distributed on both sides of this section along the circumferential direction of the pipeline at a certain distance. The aim is to protect the strain gauges, and the number of measurement points is sufficient to accurately measure stress changes during pipeline deformation and the distribution of residual stress after deformation. Additionally, a redundant number of points is included to guard against the potential failure of individual strain sensors during the pressurization or cutting processes.

The pipeline pressure-loading apparatus, depicted in Figure 1, comprises a large-scale pressure testing machine, a customized fixture, and a specially engineered indenter. The bespoke hammer is mounted onto the indenter. To emulate the subsea pipeline’s dent process, the boundary conditions of the pipeline model are consistent with those of the engineering prototype. The pipeline model is laid flat on the platform of the large-scale pressure-testing machine, and the ends of the pipeline are securely clamped via a custom fixture to prevent rotation during the experiment, simulating the boundary conditions between the subsea pipeline and the seabed. The subsea pipeline model undergoes pressure loading via the pipeline pressure-loading device, with a fixed dent depth set to induce varying degrees of pipeline deformation, thereby simulating the impact deformation experienced by subsea pipelines.

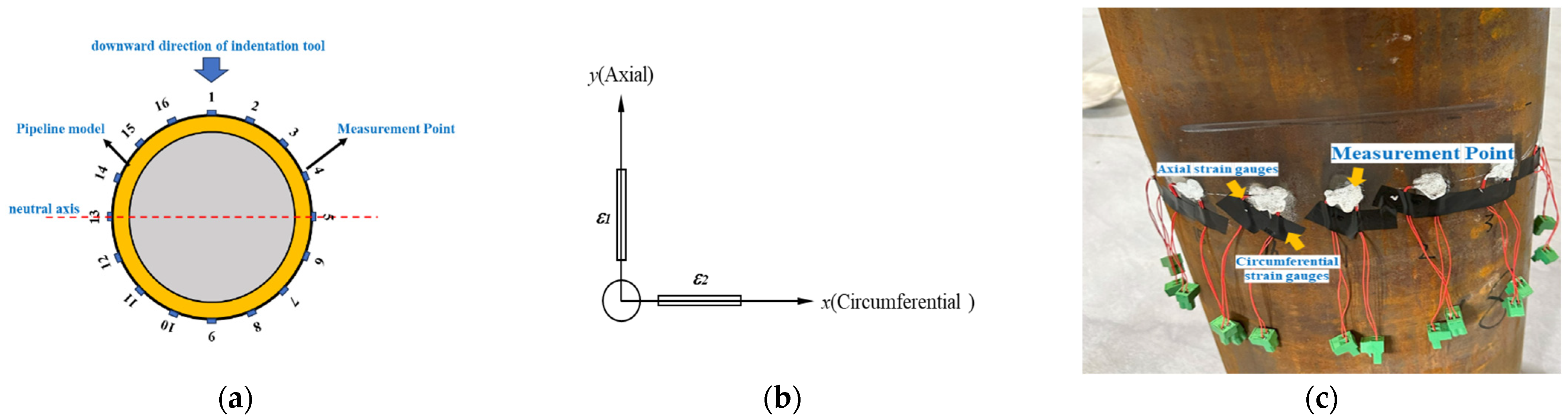
2.2. Residual Stress Measurement

Owing to its ability to release only residual stress, the destructive cutting method is superior in accuracy compared with the partially destructive and non-destructive approaches. After the saw cutting process, the initial residual stress field within the cut section is reinstated to maintain self-equilibrium in the absence of external forces, and self-equilibrium is achieved once more. Hence, the cutting method is chosen for residual stress measurement, as illustrated in Figure 2.

To mitigate temperature effects during the measurement, a wire cutting approach is preferred over saw cutting, employing axial cutting between two points to release the residual stress caused by pipeline deformation. Numerous measurement points, which are evenly distributed on the selected cross-section, are affixed with small strain gauges. These gauges capture the strain during the wire cutting process, allowing for the measurement of the residual stress in the pipeline. The use of the average stress over the strain gauge length enhances the measurement precision, providing an accurate depiction of the residual stress caused by pipeline deformation with a minimal heat-affected zone and a smooth cut section.

2.3. Experimental Conditions

On the basis of the specifications and dimensions of submarine pipelines in practical engineering, three X65 pipeline samples with outer diameters of 168 mm, 219 mm, and 273 mm were selected for experimentation. The pipe models were designed with diameter-to-thickness ratios ranging from 27 to 45, a range commonly used in pipe extrusion tests and finite element numerical simulation tests. The conclusive dimensions of the test pipe models are detailed in

Table 1. Dimensions of the pipeline model.

	D × t (mm)	L (mm)	N
1	Φ168 × 6	1000	3
2	Φ219 × 6	1000	3
3	Φ273 × 6	1000	3

, encompassing outer diameters of 273 mm, 219 mm, and 168 mm, with a wall thickness of 6 mm. To scrutinize the impact of different dent depths on the magnitude and distribution of the residual stresses in the pipeline, various dent depths were applied to pipes with the same size and diameter-to-thickness ratio. The number (N) of each type of pipeline is 3. For ease of description, the non-depersonalization of the pipeline dent depth is employed. This is achieved by taking the absolute value of the dent depth (γ) and dividing it by the pipeline diameter (D). The specific test conditions are detailed in

Table 2. Test conditions.

	D (mm)	t (mm)	n	γ (mm)	γ/D
1	168	6	8	10.08	6%
2	168	6	8	20.16	12%
3	168	6	8	30.24	18%
4	219	6	12	13.14	6%
5	219	6	12	26.28	12%
6	219	6	12	39.42	18%
7	273	6	16	16.38	6%
8	273	6	16	32.76	12%
9	273	6	16	49.14	18%

.

In the experiment, the measurement points for the nine pipeline models were arranged in an equidistant circumferential pattern around the pipeline cross-section. At each measurement point, strain gauges were positioned orthogonally along the axial and circumferential directions of the pipeline. The strains measured by these gauges were used to compute the von Mises stress at each respective point. Given the varying diameters of the pipelines, the number of measurement points differed for each one. The specific number (n) of measurement points for each pipeline is detailed in Table 2. To facilitate subsequent analysis, the measurement point aligned with the axis of the initial contact between the pipeline and the indenter was designated Measurement Point 1. The remaining measurement points were numbered in a clockwise sequence around the pipeline cross-section. The specific layout of the measurement points is shown in Figure 3.

3. Numerical Simulation

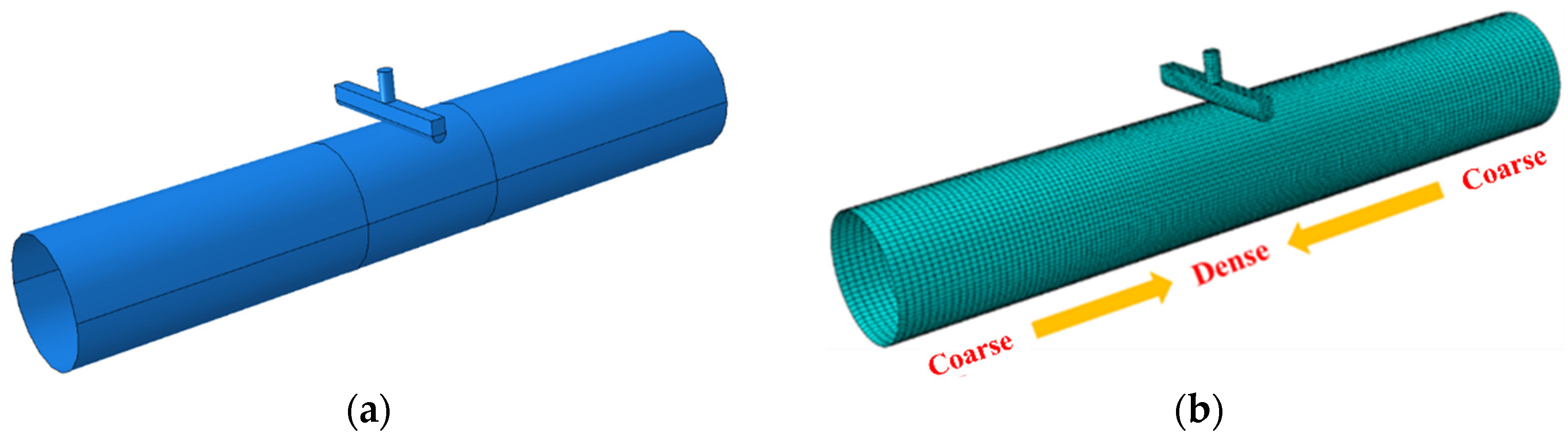
3.1. Finite Element Model

Employing Abaqus/CAE, a detailed finite element model for residual stress resulting from subsea pipeline impacts was created, as depicted in Figure 4. The model consists of a rigid indenter and a subsea pipeline. To meet the needs of the project, the X65 pipeline was selected as the research object. The finite element model for a substantially deformed subsea pipeline is formulated on the basis of the conventional structure of subsea pipelines, comprising a rigid indenter and the pipeline model. The X65 pipeline uses L450 steel, following API standards, with an outer diameter of 168 mm and a 6 mm wall thickness. It is from the China Petroleum Pipeline Bureau, which is located in Lang fang, China. To reduce the impact of the pipeline model’s length on the deformation, the model’s length is ten times larger than the diameter. The specific parameters of the X65 pipeline are presented in

Table 3. Pipe FEM model parameters.

Parameter	Reference Value
Density/kg·m−3	7850
Young’s modulus/GPa	210
Poisson’s ratio	0.3
Yield stress/MPa	550
Reference strain rate of material model/s−1	1

and

Table 4. Yield stress–strain data of the pipeline.

Yield Stress (MPa)	Plastic Strain	Yield Stress (MPa)	Plastic Strain
550	0	711.64	0.13541
582.93	0.00423	727.36	0.15538
611.11	0.0327	739.95	0.17726
633.06	0.05171	754.12	0.20105
670.74	0.07263	762.02	0.22103
683.33	0.09356	776.19	0.24386
699.05	0.11448	794.99	0.2648

.

Owing to the relatively small wall thickness of the subsea pipeline in comparison with its length, S4R shell elements were utilized to construct the finite element model, with the intention of minimizing the computational time. The residual stresses resulting from substantial deformation in subsea pipelines are associated primarily with the dent depth of the pipeline. To investigate the relationship between the residual stress and the pipeline dent, the pipeline was segmented into three zones, with the density incrementally increasing from the outer regions towards the core. This arrangement ensured a homogenous density in the central zone that interfaced with the indenter, thereby enhancing the computational accuracy.

To avoid computational divergence, solid elements were employed to model the indenter. The subsea pipeline experiences localized deformation upon impact, particularly in the vicinity of the contacting object. The C3D8 element calculates the strain at each Gaussian integration point, providing a high level of accuracy. However, the C3D8 element is prone to exhibit hourglass phenomena when simulating large-deformation residual stresses in subsea pipelines. The C3D8R element employs reduced integration techniques to mitigate hourglass effects, making it suitable for handling large deformations and compression problems. Nevertheless, the computational accuracy of the C3D8R element is inferior to that of the C3D8 element. Considering the comprehensive evaluation of the computational accuracy, hourglass control, and computational efficiency, the C3D8I element demonstrates a superior balance. Therefore, the indenter model employs a C3D8I mesh for computation.

3.2. Boundary Conditions

The boundary conditions for the finite element model of subsea pipeline residual stress are illustrated in Figure 5. In marine engineering, submarine pipelines are partially embedded in the seabed, with the lower part in full contact and anchored to the seabed. The pipelines subjected to impacts may experience slight sliding along the seabed. Fixed boundary conditions are imposed at the bottom of the pipeline for computational convenience in the numerical simulation of the residual stresses in the pipeline. Although fixed boundary conditions may produce slightly higher residual stresses than those encountered in actual engineering scenarios, they adhere to the conservative principle typically adopted in engineering design.

The finite element model for subsea pipeline residual stress incorporates a contact simulation between the pipeline and an indenter, mimicking the impact behavior. Given the nature of low-speed collisions in subsea pipeline scenarios, a global general contact method is employed to simulate the normal behavior during contact, utilizing a penalty function to represent the tangential behavior, with a coefficient set at 0.3. Frictional effects are assumed to be isotropic. This modeling approach is designed to accurately capture the intricacies of the pipeline’s response to low-speed impact events, contributing to a nuanced understanding of the residual stress distribution in subsea pipelines.

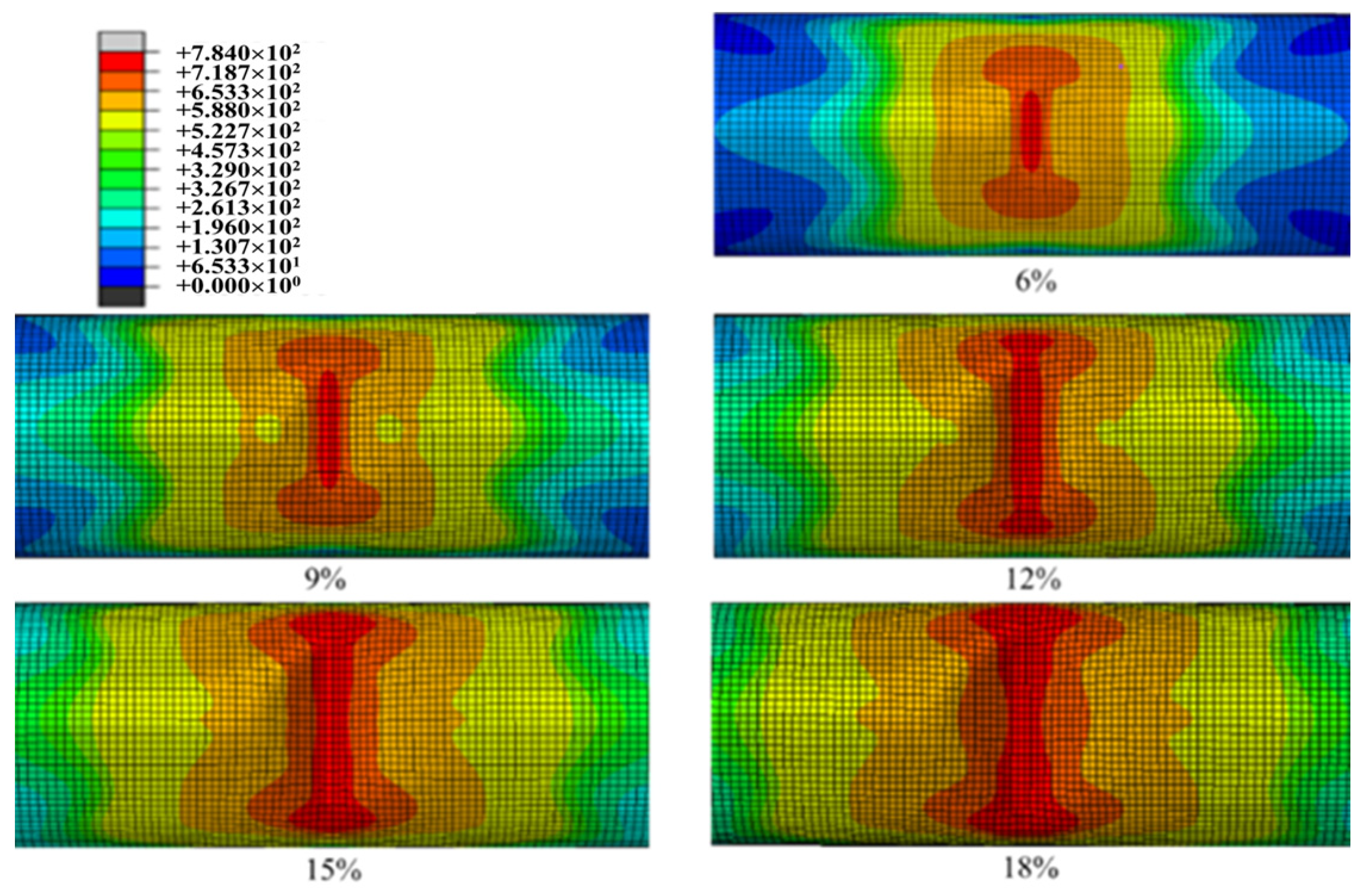
3.3. Residual Stress Simulation

The computational procedure for the determination of the residual stresses in subsea pipelines, as depicted in Figure 6, is intricately linked to the localized indentations resulting from impact incidents. This process unfolds in two distinct phases: loading and unloading. In the absence of residual stresses, the subsea pipeline undergoes elastic deformation, approaching near-zero indentation deformation after unloading. However, in the case of substantial deformation, the pipeline experiences plastic strain, and, after unloading, residual stresses persist as a manifestation of residual deformation. To simulate the aforementioned process, two analysis steps were employed in solving the finite element model, representing the loading and unloading phases. By utilizing a static solution method for computational efficiency, the loading phase involved applying the absolute displacement of the high-stiffness indenter (e.g., 6% diameter, 12% diameter, 18% diameter) to simulate the extent of pipeline deformation. Upon unloading, the distributions of the residual stress and deformation characterize the residual stress and residual deformation of the subsea pipeline.

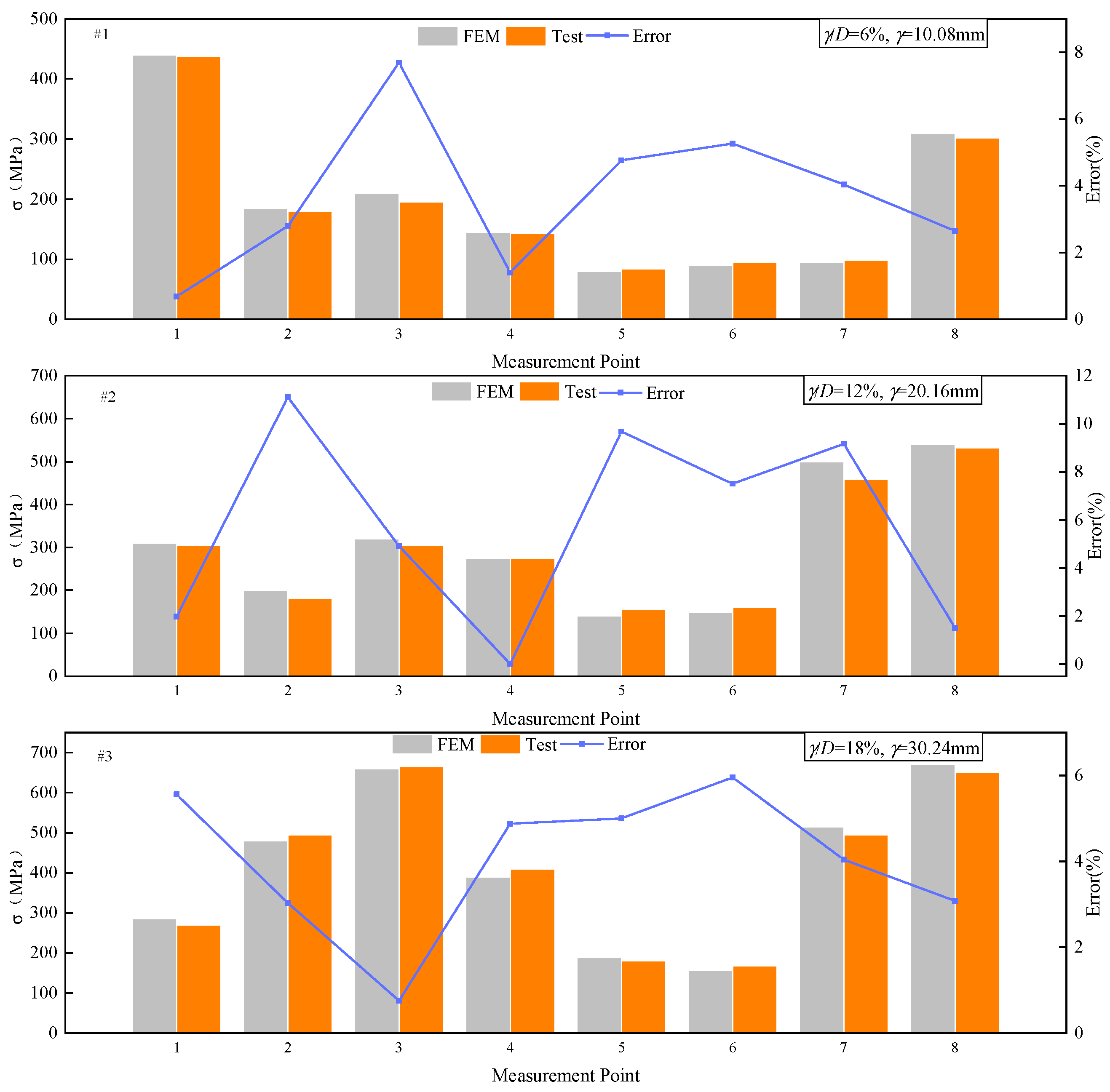
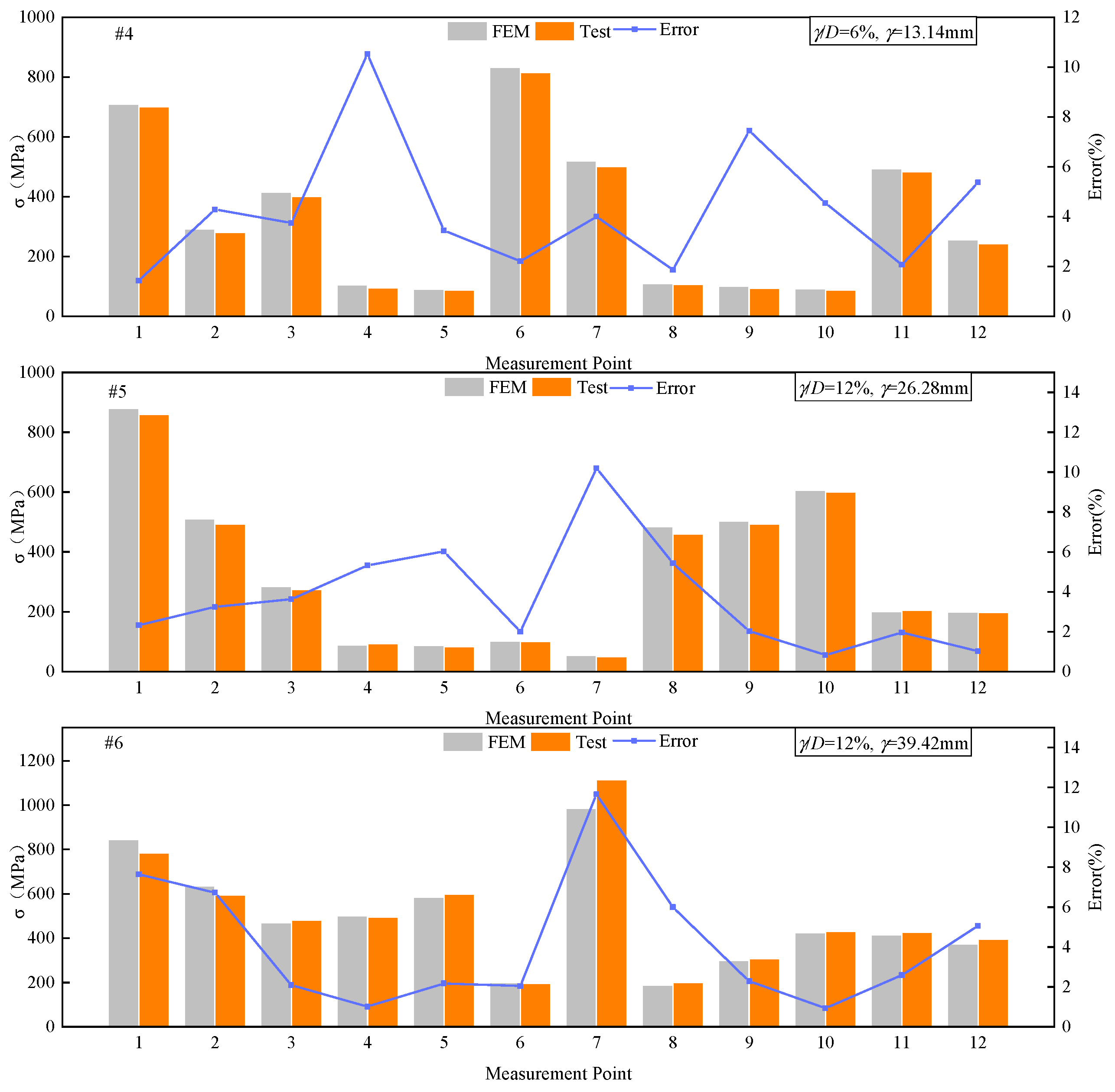
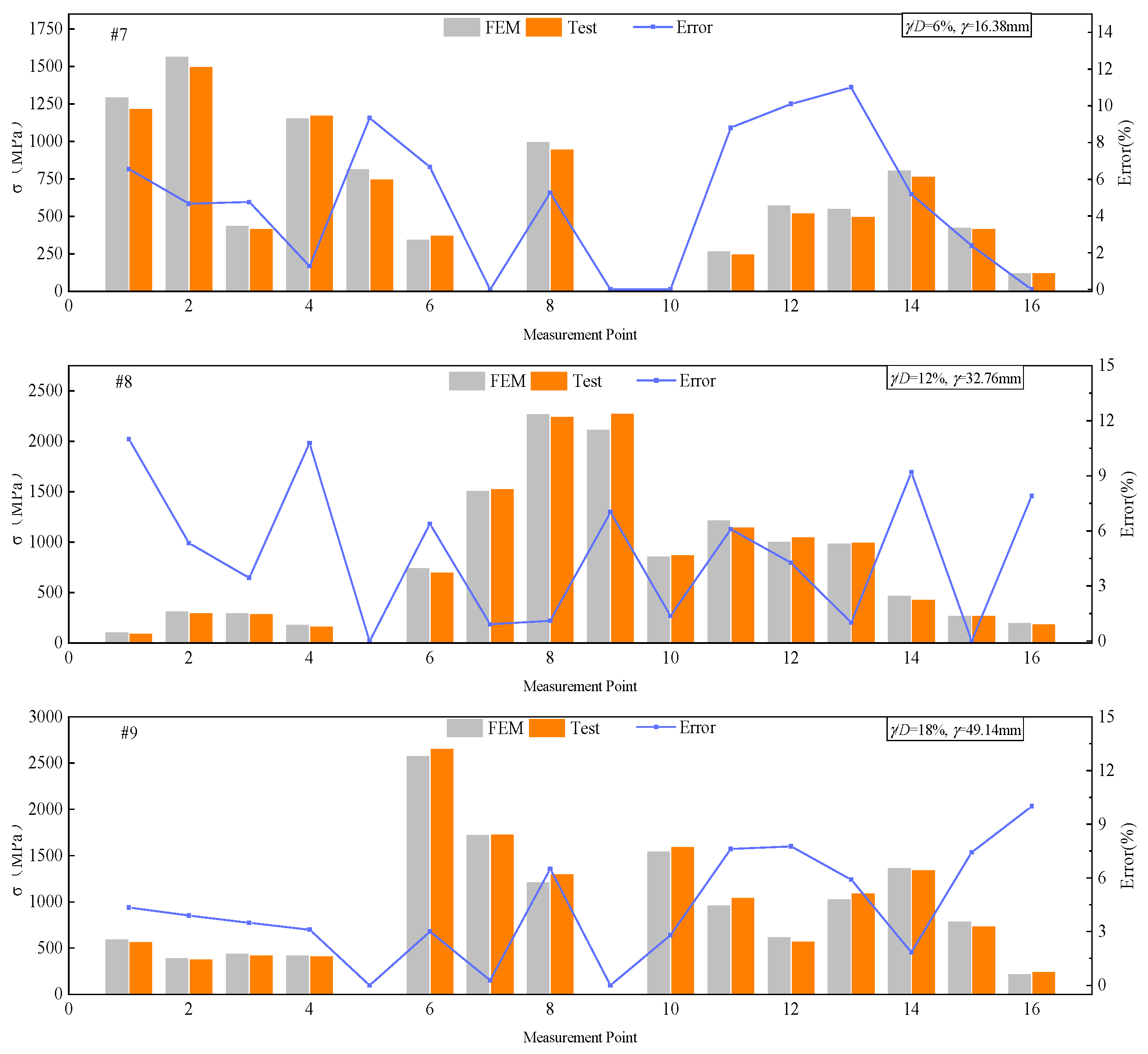
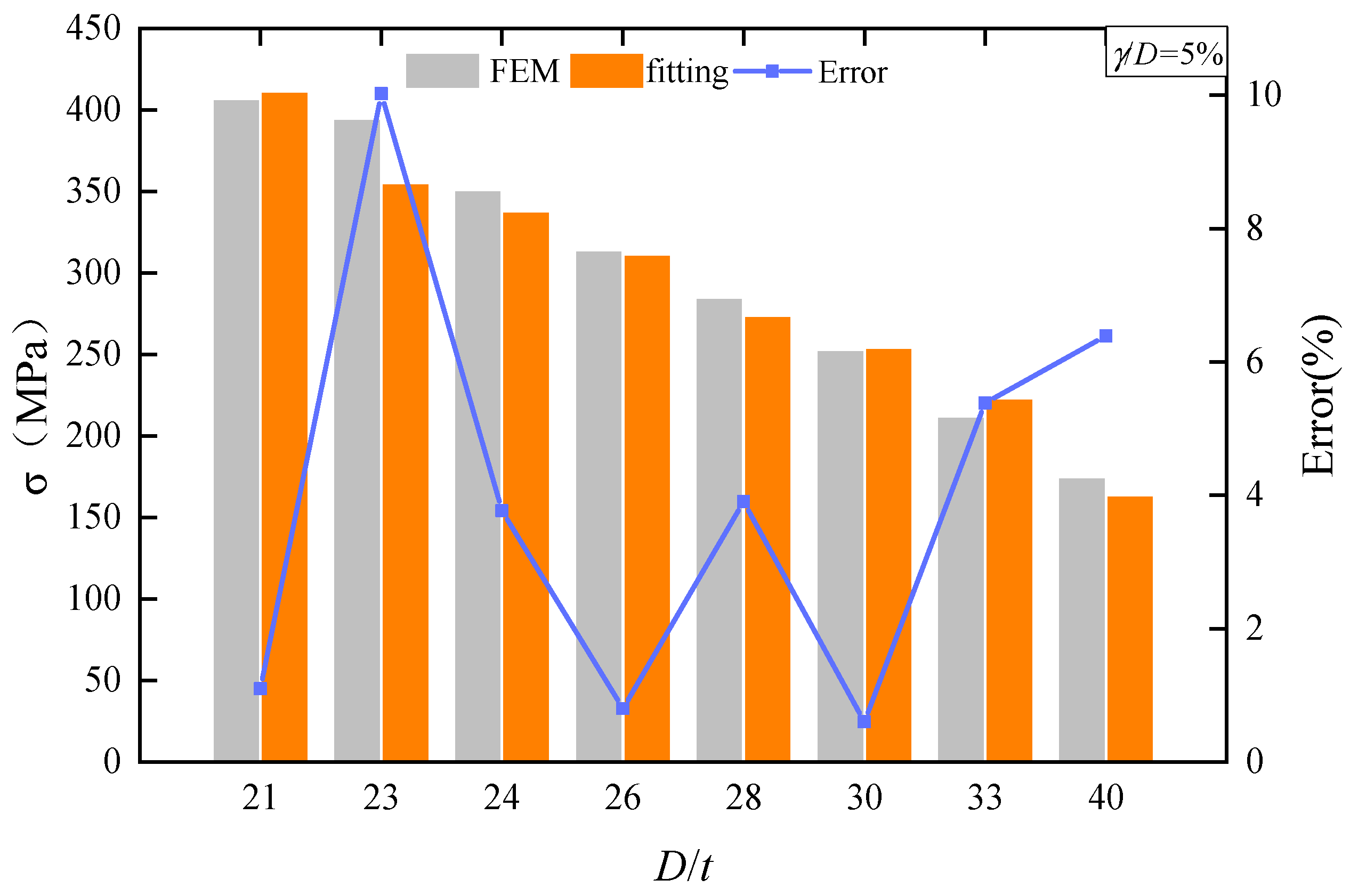
3.4. FEM Verification

To validate the accuracy of the finite element analysis (FEA) results, this study elects to compare the FEA outcomes with experimental data. To ensure the accuracy of the entire verification process, the same data processing methods are employed in the finite element model as were used in the experiments. During the validation, both the circumferential and hoop strains at identical locations, as measured experimentally, are extracted from the finite element model. The von Mises stress is then calculated using the von Mises stress formula, and the results are compared.

Figure 7, Figure 8 and Figure 9 depict a comparative analysis of the experimental and numerical outcomes for the residual stresses in submarine pipelines under various testing conditions. The numerical model is consistent with the experimental observations in terms of the residual stress distribution. Although the numerical predictions slightly surpass the experimental measurements, the disparity is negligible. Furthermore, there is discernible consistency in the circumferential distribution pattern, affirming the robust alignment between the numerical simulations and experimental findings.

Owing to the small dimensions of the strain gauges, positional deviations are inevitable during the attachment process. When these deviations occur within the range of significant changes in residual stress in the pipe, notable measurement errors may result. Furthermore, owing to constraints in the measurement method, a certain distance exists between the measurement section, where the strain gauges are affixed, and the cutting section during the linear cutting process. Consequently, after linear cutting, the residual stress at the strain gauge location is not fully released but attains a new equilibrium. The strain recorded by the gauges reflects the strain released during the transition from the original equilibrium state to the new equilibrium state of residual stress in the circular steel pipe, introducing some measurement errors. Due to the inherent errors present during the experimental process, and the fact that these errors are random, there is the inevitable presence of random errors when using experimental results to validate the outcomes of finite element analysis and empirical formulas. Additionally, when subsea pipelines experience indentation due to loading, the pipeline cross-section undergoes plastic deformation. This implies that irreversible deformation occurs in the axial, circumferential, and thickness directions of the pipeline. Due to limitations in the experimental conditions, it is challenging to measure the deformation in the thickness direction of the pipeline. Therefore, the experiments can only reconstruct the equivalent stress through circumferential and hoop deformations. This stress, in reality, represents the surface stress of the pipeline. During the indentation process, the pipeline surface undergoes significant deformation, distorting the original deformation directions and causing deviations from the axial and hoop directions towards the thickness direction. However, the sensors always measure in directions parallel to the pipeline’s axial and hoop lines, respectively. This leads to random errors.

Figure 7, Figure 8 and Figure 9 reveal the circumferential distribution pattern of residual stress along the pipe. When the diameter-to-thickness ratio is small, the pipe cross-section undergoes significant elastic deformation. Any structural imbalance is localized to the region where the pipe contacts the indenter—specifically, the indented section. At this point, the indented part of the pipe requires a certain degree of internal deformation to maintain the self-equilibrium of the pipe’s cross-sectional structure. The residual stress is most pronounced at the contact point between the pipe and the indenter and gradually diminishes in other regions, showing a decay in residual stress in both directions. The plastic deformation resulting from the pipe impact exhibits discernible attenuation from the impact zone towards the bottom. As the dent depth increases, the bottom position of the pipe experiences buckling deformation due to the compressive effect of the large deformation from above, resulting in buckling deformation at the bottom and sides of the central portion of the pipe. This leads to a relatively gradual decay in the residual stress generated by the pipe impact from the top impact zone towards the bottom, accompanied by a rebound at the bottom.

When the diameter-to-thickness ratio (D/t ratio) is high, pipelines with higher D/t ratios exhibit a greater propensity for and severity of instability at the same burial depth. For shallow burial depths, owing to the loading force exerted by the indenter and the reactive force from the ground (i.e., the test platform) forming a radial force pair, the pipeline undergoes indentation deformation in the contact region with the indenter and buckling deformation at the bottom. As the burial depth increases, the contact area between the pipeline’s bottom and the ground (i.e., the test platform) expands, leading to the extension of the buckling deformation from the bottom towards the pipeline’s mid-axis. This results in the propagation of residual stresses from the bottom towards the mid-axis of the pipeline.

Figure 7, Figure 8 and Figure 9 illustrate the error distribution under various conditions. When the dent depth is small, the pipeline is in the elastic deformation stage, and compression causes deformation at the top and bottom of the pipeline. This renders the deformed top and bottom of the pipeline relatively flat, making the strain direction extracted from the finite element analysis closer to the direction measured in the experiment. Under these conditions, the maximum residual stress occurs at the contact point between the indenter and the pipeline and at the bottom of the pipeline. With sufficient deformation, the magnitudes extracted from the finite element analysis are closer to the experimental values. In contrast, the central region near the neutral axis of the pipeline experiences less deformation, and the influence of deformation in the thickness direction is significant, leading to larger errors.

As the dent depth gradually increases, the plastic deformation at the top and bottom of the pipeline also increases, reducing the proportion of deformation in the thickness direction. This cause the surface stress to be increasingly close to the actual residual stress. Given that the top and bottom of the pipeline experience the highest residual stress and undergo sufficient deformation, the measured errors are smaller; thus, the overall error is reduced. Conversely, in the central region near the neutral axis, increased plastic deformation occurs with deeper dents, causing the deformation direction measured by the sensors to deviate significantly from the true axial and hoop directions. Additionally, the measured residual stress in this area is lower, leading to larger overall errors.

With further increases in the dent depth, the central region near the neutral axis of the pipeline experiences buckling deformation, generating considerable residual deformation and residual stress. At this point, the deformation in the thickness direction is minimal compared to the deformation in other directions, making the surface stress closer to the actual stress, thereby reducing the error.

4. Empirical Formulation for Residual Stresses of Subsea Pipeline

To further analyze the characteristics of the residual stresses in highly deformed subsea pipelines, this paper develops an empirical formula. We established a large dataset based on the finite element analysis and experiments. Through the parametric sensitivity analysis of the pipeline’s residual stresses, we determined the basic framework of the empirical formula. Using the extensive dataset created, we then fitted the coefficients of the empirical formula. This process ultimately led to the determination of an empirical formula for highly deformed subsea pipelines.

4.1. Parameter Analysis of Residual Stress from Subsea Pipeline Impact

The diameter-to-thickness ratio (D/t), which represents the ratio of the outer diameter (D) to the thickness (t) of the pipeline, is a critical parameter influencing the pipeline’s ability to withstand external pressure. To explore the general patterns between the residual stress and various parameters, the non-dimensionalization of the residual stress is carried out. This is achieved by dividing the residual stress (σ) by the yield stress (σ_y). This research investigates the non-dimensionalized residual stress (σ/σ_y) and the relationship between the non-dimensionalized residual stress (σ/σ_y) and the ratio of the diameter to the thickness in relation to the dent depth. To meet the demands of engineering applications, a commonly employed range for the diameter-to-thickness ratio (D/t) is selected, which typically falls within the range of 19–46.

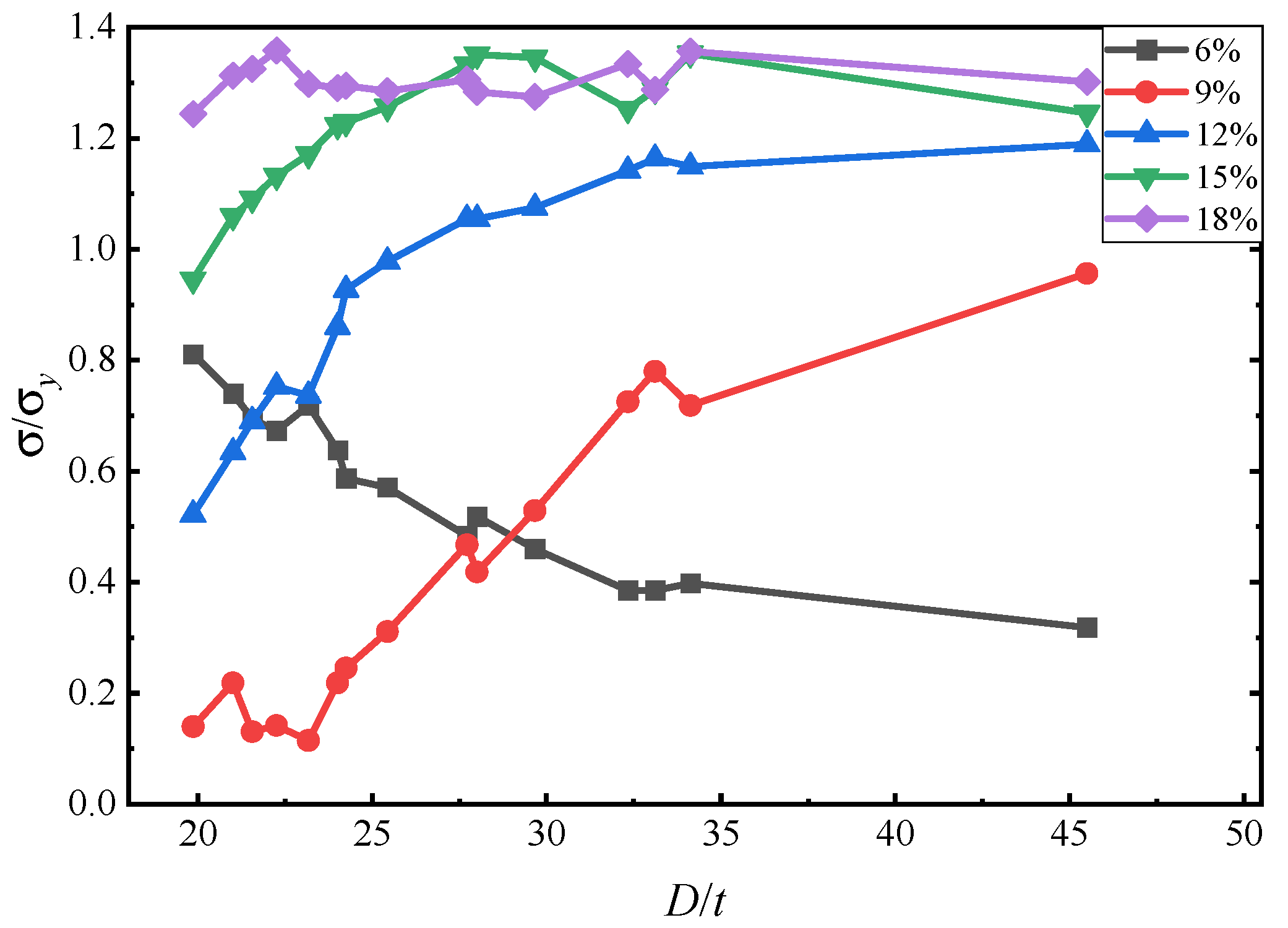
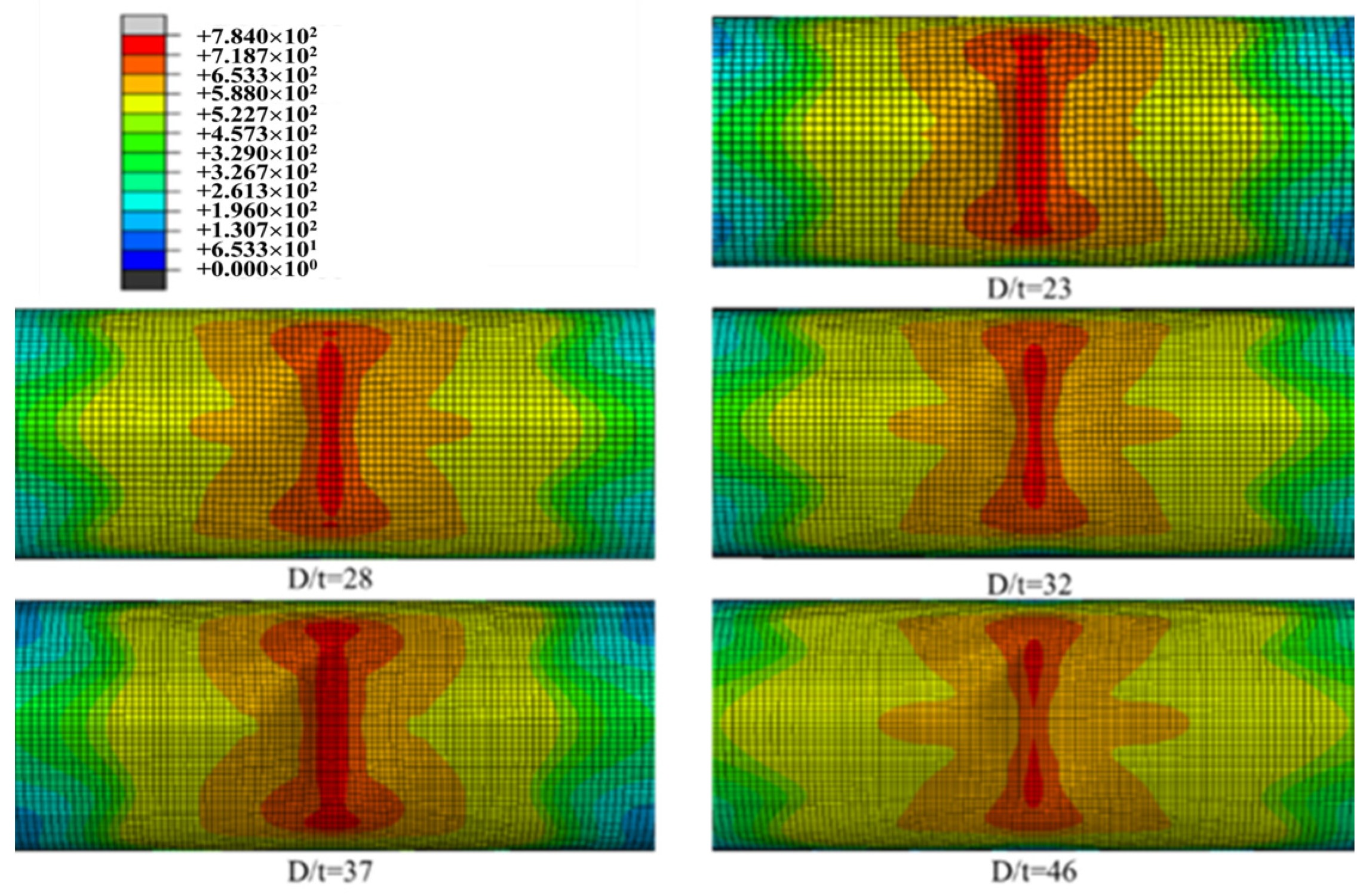
4.1.1. Ratio of Diameter to Wall Thickness

Figure 10 illustrates the relationship between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y) within this specified range. For shallow dent depths, an inverse correlation is observed between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y). As the dent depth (γ/D) increases, there is a gradual transition to a positive correlation between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y). However, when the dent depth (γ/D) is sufficiently large, the residual stress (σ/σ_y) stabilizes around a constant value, resulting in a reduced correlation with the diameter-to-thickness ratio.

The observed phenomenon is due to variations in the degree of buckling instability in the cross-sectional structure of the pipeline at different dent depths. For shallow dent depths (as shown by the black curve in Figure 10), the pipeline’s cross-sectional structure remains stable, and the residual stress (σ/σ_y) primarily arises from the plastic strain-induced deformation of the pipeline material itself. Consequently, there is a negative correlation between the diameter-to-thickness ratio (D/t) and residual stress, as supported by Figure 10. Figure 11 shows the residual stress distributions in pipelines with different diameter-to-thickness ratios at a dent depth (γ/D) of 6%. As the diameter-to-thickness ratio (D/t) increases, the pipeline becomes thinner, leading to a gradual reduction in both the peak and range of the residual stress. This reduction is attributed to the decreasing plastic strain-induced residual deformation, which is especially noticeable at relatively shallow dent depths.

With an increasing dent depth (γ/D), the pipeline stiffness decreases as the diameter-to-thickness ratio (D/t) increases. This is due to the onset of buckling instability in the pipeline’s cross-sectional structure. When the diameter-to-thickness ratio (D/t) is small, the cross-section exhibits stronger resistance to buckling, allowing the pipeline to maintain its original structural configuration with greater stability. Consequently, the critical value for pipeline instability is larger, and the internal deformation required for structural equilibrium is smaller. In general, a larger diameter-to-thickness ratio (D/t) is correlated with higher residual stress and lower remaining strength in the pipeline, making it more prone to damage. As the pipeline undergoes deformation, its cross-section can be viewed as an arch structure. A larger diameter-to-thickness ratio (D/t) increases the flexibility of the pipeline’s cross-section, increasing the susceptibility of the arch structure to instability. Therefore, pipelines with larger diameter-to-thickness ratios experience greater instability at the same dent depth, and the degree of instability tends to gradually increase. This results in larger internal deformations being required to maintain the structural equilibrium, leading to higher residual stress (σ/σ_y). From an energy perspective, at the same dent depth (γ/D), pipelines with larger diameter-to-thickness ratios have less work created by external loads, translating to smaller elastic potential energy. Consequently, the internal deformation energy of the pipeline increases, resulting in greater stored residual stress (σ/σ_y). As the dent depth increases to a certain extent, the cross-section of the pipeline undergoes complete instability. The residual stress (σ/σ_y) in this state is generated by the buckling deformation resulting from structural instability. Consequently, the correlation between the residual stress (σ/σ_y) and the diameter-to-thickness ratio (D/t) weakens and remains within a constant range (as shown by the violet-colored curve in Figure 10).

4.1.2. Dent Depth

Figure 12 shows the relationship between the dent depth (γ/D) and residual stress (σ/σ_y) under different diameter-to-thickness ratios. Pipelines with a small diameter-to-thickness ratio (D/t < 25) exhibit minimal cross-sectional instability when the dent depth is small. The overall structural deformation of the cross-section is limited under these conditions. The deformation caused by external loading is primarily due to the elastic–plastic deformation of the material in the thickness direction of the pipe. The energy imparted by the external load is converted into strain energy associated with the elastic–plastic deformation of the pipe. This leads to significant plastic deformation and residual stress. The cross-sectional structure experiences deformation due to the external load as the dent depth increases further. Given the small D/t ratio, the buckling is limited, and much of the deformation in the cross-sectional structure can rebound. The energy imparted by the external load is dissipated through the elastic deformation of the cross-sectional structure. Reduced energy is converted into the plastic deformation of the material, producing lower residual stresses. Notably, as the diameter-to-thickness ratio of the pipeline increases, under the same dent depth, the pipeline is more prone to buckling instability, resulting in greater residual stress. Therefore, when the diameter-to-thickness ratio is 33, the residual stress in the pipeline gradually increases with the increase in dent depth, without significant recovery. When the diameter-to-thickness ratio further increases, even a small dent depth can cause the pipeline to buckle under pressure, inducing residual stress due to material deformation. Additionally, because of the larger diameter-to-thickness ratio, the pipeline is more sensitive to the dent depth. Consequently, when the dent depth increases further, the structure rapidly buckles and becomes unstable, generating residual stress. This leads to less rebound, with most of the load energy being released through plastic deformation. Thus, when the diameter-to-thickness ratio is 40, within the dent depth range of 6% to 10%, the pipeline is in a transitional phase with no significant change.

However, as the dent depth increases significantly, the cross-sectional structure undergoes substantial buckling deformation, triggering greater residual stresses. Consequently, the residual stress in the pipeline increases with the increasing dent depth. The relationship between the dent depth (γ/D) and residual stress (σ/σ_y) clearly exhibits an overall positive correlation from a fitting perspective. It can be described by a positively correlated curve.

Figure 13 shows the distribution of the residual stress (σ/σ_y) in the pipeline under various dent depths. Figure 12 clearly shows that as the dent depth increases, the region of residual stress (σ/σ_y) distribution gradually expands, accompanied by a progressive increase in the peak values. This means that, under the same diameter-to-thickness ratio (D/t) conditions, a greater dent depth results in a greater load value exceeding the critical buckling threshold. This, in turn, leads to a greater degree of pipeline buckling, with the structure maintaining self-equilibrium and generating significant residual deformation within the pipeline. This substantial internal deformation ultimately contributes to the gradual increase in residual stress (σ/σ_y) within the pipeline.

In the context of the same diameter-to-thickness ratio, increasing the dent depth amplifies the external load on the pipeline. This signifies that, under similar conditions, a greater dent depth corresponds to a higher load threshold that exceeds the critical point for instability. Consequently, the pipeline experiences more pronounced buckling, resulting in substantial self-equilibrium-preserving internal deformations. This, in turn, contributes to the progressive build-up of residual stress (σ/σ_y) within the pipeline. This effect is particularly prominent when the diameter-to-thickness ratio (D/t) is relatively small. In such cases, the elastic deformation of the pipeline’s cross-sectional structure is more substantial. The imbalanced section of the structure is confined to the area where the pipeline contacts the indenter—specifically, the region influenced by the depression. In scenarios with a small diameter-to-thickness ratio, the depressed section of the pipeline must undergo internal deformation to sustain the self-equilibrium of its cross-sectional structure. When the diameter-to-thickness ratio (D/t) is small, the energy resulting from pipeline deformation is dissipated primarily through the elastic deformation of the structure. This leads to diminished residual energy, causing internal deformations to occur mainly in the depressed area to dissipate the remaining energy.

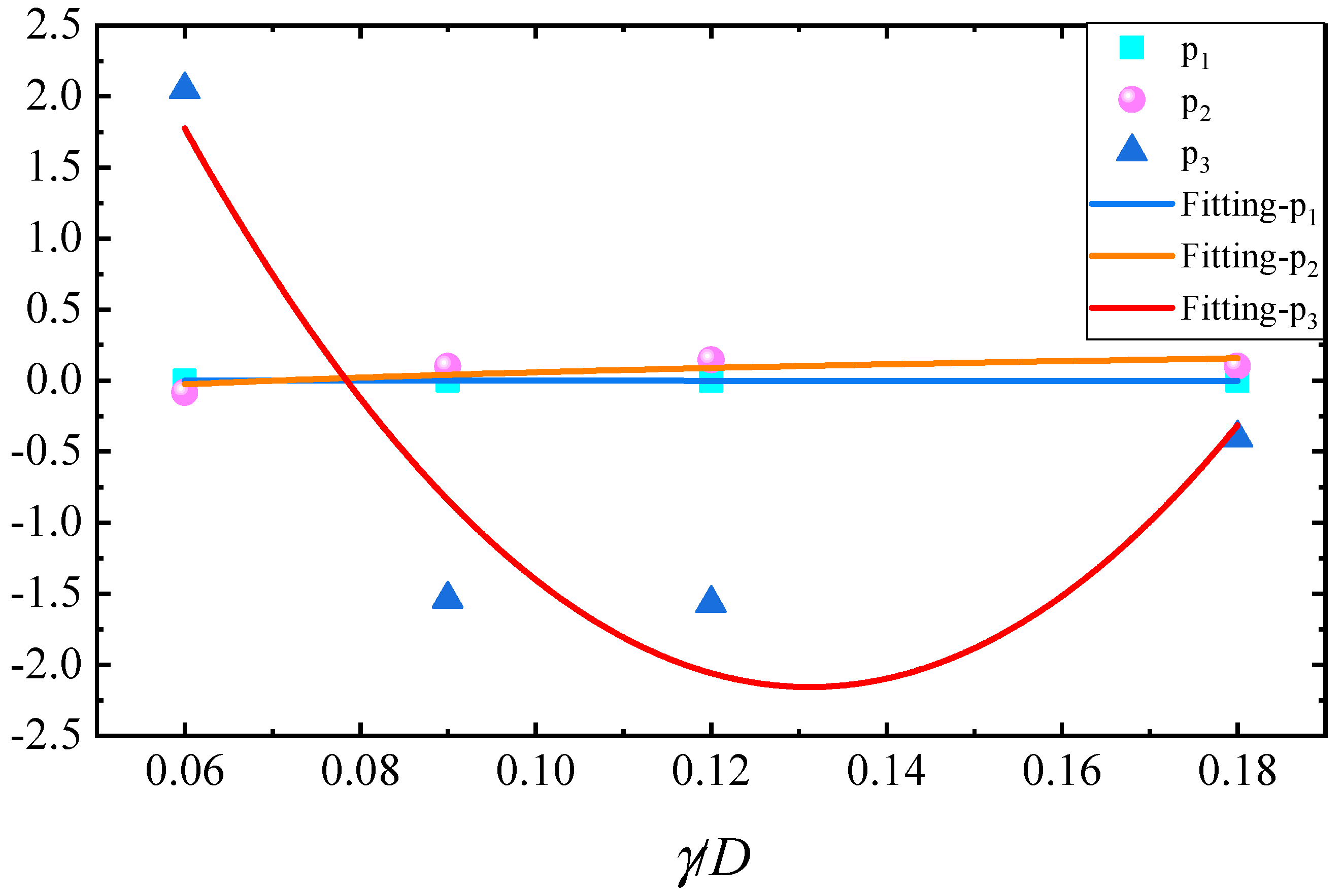
4.2. Empirical Formula Fitting

After non-dimensionalizing and conducting parametric analyses on factors such as the residual stress (σ/σ_y), diameter-to-thickness ratio, and dent depth, overarching patterns among these variables have been elucidated. The impact of the diameter-to-thickness ratio (D/t) on the residual stress (σ/σ_y) is intricate. As illustrated in Figure 10, a complex non-linear relationship exists between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y), which significantly varies across different diameter-to-thickness ratios. When the pipeline’s dent depth is small, the pipeline is in the elastic deformation stage. Under this condition, the residual stress in the pipeline is primarily due to the plastic deformation of the material itself. As the diameter-to-thickness ratio increases, with the same diameter, there is less material, resulting in less plastic deformation. Therefore, the orange curve in Figure 14 presents a negative correlation.

By applying the Levenberg–Marquardt optimization algorithm in the least squares method, a curve fitting process has been employed to model the relationship between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y) on the basis of the finite element simulation results. The fitting equation synthesizes the insights obtained from the parametric analyses of the residual stress (σ/σ_y), with a primary emphasis on swiftly predicting the residual stress (σ/σ_y) in a broader range of diameter-to-thickness ratios for underwater pipelines. Simultaneously, considering the experimental results from this paper and existing relevant research, along with the discrepancies between experimental and finite element simulation data, the fitting equation has been adjusted to increase the accuracy and prioritize safety in predicting the residual stress (σ/σ_y). The ultimately fitted curve is illustrated in Figure 14.

$$\left\{\begin{array}{l}{\displaystyle \frac{\sigma }{{\sigma }_y}}=p_1\ast {({\displaystyle \frac{D}{t}})}^a+p_2\ast {({\displaystyle \frac{D}{t}})}^b+p_3\\ 20<{\displaystyle \frac{D}{t}}<46\\ 0<{\displaystyle \frac{\gamma }{D}}<16\%\end{array}\right.$$

(1)

Figure 14 shows that, within the designated range of diameter-to-thickness ratios (D/t) and dent depths (γ/D), Equation (1) adeptly conforms to individual data points, effectively delineating the correlation between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y) across varying dent depths. The resulting fitted data elucidate numerical values for specific parameters, as detailed in

Table 5. Parameters related to the diameter-to-thickness ratio.

D/t	a	b	P1	P2	P3
19~46	2	1	f (γ/D)	f (γ/D)	f (γ/D)

. Nonetheless, an examination of of Table 5 reveals that parameters p1, p2, and p3 elude determination through fitting, which is attributed to the interdependence of the residual stress (σ/σ_y) not only on the diameter-to-thickness ratio (D/t) but also on the dent depth (γ/D). Furthermore, Figure 15 shows distinct variations in the values of p1, p2, and p3 under diverse dent depths, underscoring their close correlation with the dent depth (γ/D).

Employing the Levenberg–Marquardt optimization algorithm within the framework of the least squares method, coefficients p1, p2, and p3 were iteratively fitted on the basis of the observed trends delineated in Figure 12. A correlation between these coefficients and the dent depth (γ/D) was systematically established. The ultimate results of this fitting procedure are depicted graphically in Figure 15 and formulated in Equation (2). Under the condition of the same dent depth, it can be seen from Equation (1) that p1 and p2 are coefficients closely related to the diameter-to-thickness ratio, while p3 is a coefficient directly associated with the pipeline’s residual stress. When the dent depth changes, p3 represents the effect of the dent depth on the pipeline’s residual stress, whereas p1 and p2 couple the effects of the dent depth and the diameter-to-thickness ratio of the pipeline. Therefore, p3 more intuitively demonstrates the relationship between the dent depth and residual stress. According to Figure 12, it can be observed that as the dent depth increases gradually from 0, the pipeline undergoes a process from elastic deformation to structural buckling. Consequently, there is a recovery phenomenon in the red curve of Figure 15. The fidelity of Equation (2) is evident in accurately capturing each sample point and elucidating the nuanced impact of the dent depth (γ/D) on the residual stress (σ/σ_y). By amalgamating Equation (1) and Equation (2), we derive the definitive formula for the calculation of the residual stress (σ/σy). The computational outcomes, graphically presented in Figure 16, underscore the expeditious and accurate nature and predictive capacity of the formulated equation concerning the magnitude of residual stress (σ/σ_y) in subsea pipelines, being valuable for engineering computational analyses.

When the diameter-to-thickness ratio is large, under the same loading condition, the pipeline experiences a lesser degree of elastic deformation, with a greater proportion of the loading energy being dissipated through elastic deformation. Consequently, under these conditions, the formula exhibits larger discrepancies. Moreover, with a larger diameter-to-thickness ratio, the pipeline cross-section is more susceptible to buckling instability under the same loading condition, leading to greater errors in the predictions.

$$\left\{\begin{array}{l}{p}_1=0.6557\ast {({\displaystyle \frac{\gamma }{D}})}^2-0.1649\ast ({\displaystyle \frac{\gamma }{D}})+0.0085\\ p_2=-90.82\ast {({\displaystyle \frac{\gamma }{D}})}^2-20.039\ast ({\displaystyle \frac{\gamma }{D}})-0.9616\\ p_3=1318\ast {({\displaystyle \frac{\gamma }{D}})}^2-301.5\ast ({\displaystyle \frac{\gamma }{D}})+15.27\end{array}\right.$$

(2)

5. Conclusions

This paper established a finite element model for residual stress (σ/σ_y) in highly deformed subsea pipelines and conducted the experimental validation of the residual stress (σ/σ_y) model. The accuracy of the finite element model was verified through experimental validation. By employing finite element simulation in conjunction with experimentation, a parameter analysis of the residual stress (σ/σ_y) in highly deformed subsea pipelines was conducted. On the basis of the results of the parameter analysis, a formula for the rapid prediction of the residual stress (σ/σ_y) in highly deformed subsea pipelines was derived through fitting. In conclusion, the following findings were obtained.

(1)

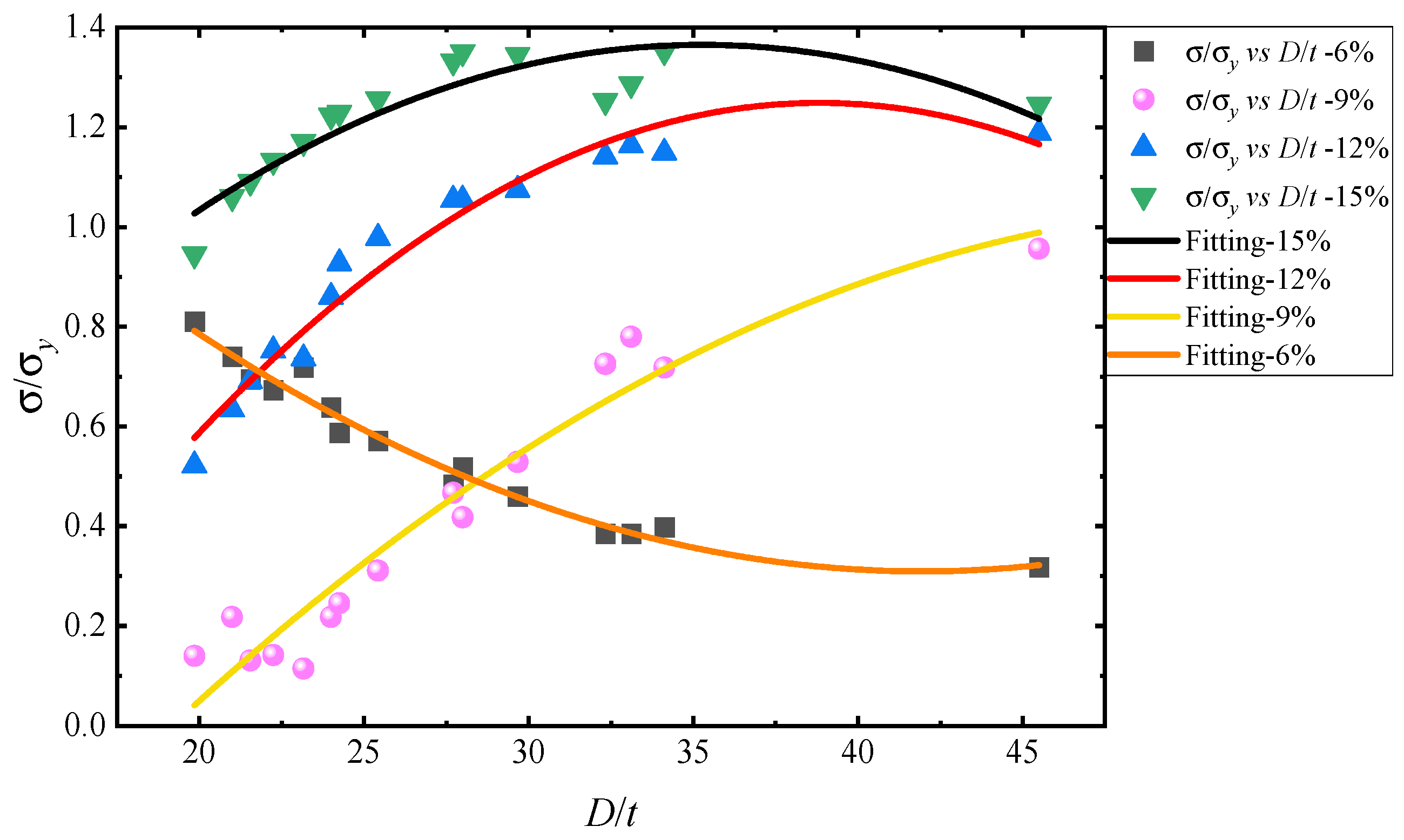
When the pipeline dent depth (γ/D) is small (i.e., less than or equal to 6%), a negative correlation emerges between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y) in subsea pipelines. Larger ratios correspond to smaller stresses, primarily from radial tension–compression in the pipe wall, which induces material plastic strain. In the dent depth (γ/D) range of 6% to 18%, a positive correlation appears between the diameter-to-thickness ratio (D/t) and residual stress (σ/σ_y) in subsea pipelines. Larger ratios are associated with higher stresses, predominantly from residual deformations induced by section buckling in the pipeline. For dent depths (γ/D) exceeding 18%, the residual stress (σ/σ_y) in subsea pipelines becomes independent of the diameter-to-thickness ratio, fluctuating within a constant range of approximately 1.3 times the yield stress. This suggests that, at greater dent depths, the residual stress (σ/σ_y) is not influenced by variations in the diameter-to-thickness ratio (D/t) but remains relatively constant. The observed fluctuations can be attributed to other factors influencing the residual stress (σ/σ_y).

(2)

When the dent depth (γ/D) is less than 18%, a positive correlation is observed between the residual stress (σ/σ_y) in subsea pipelines and the dent depth (γ/D). The greater the dent depth (γ/D) is, the more pronounced the buckling instability in subsea pipelines, resulting in elevated residual stress (σ/σ_y). Reduced dent depths correspond to minimized structural deformation, leading to diminished internal deformations necessary to maintain equilibrium and consequently lower residual stress (σ/σ_y). For smaller diameter-to-thickness ratios and dent depths, buckling deformation in the pipeline section is minimized, yielding less residual stress (σ/σ_y) outside the dent region. The maximum residual stress (σ/σ_y) in the pipeline is localized within the dent region. Conversely, with increasing diameter-to-thickness ratios and dent depths, the buckling deformation in the pipeline section expands, leading to greater residual stresses outside the dent region. The maximum residual stress (σ/σ_y) in the pipeline propagates from the center of the dent towards both sides, ultimately achieving peak values on either side of the dent region.

(3)

The derived formula in this paper adeptly characterizes the physical relationship between the residual stress (σ/σ_y) in subsea pipelines and both the diameter-to-thickness ratio (D/t) and dent depth. This method is capable of swiftly forecasting the residual stress (σ/σ_y) in highly deformed subsea pipelines.

This paper analyzes the residual stress in highly deformed subsea pipelines and formulates a computational equation for the rapid prediction of the residual stress. However, the analysis only considers the influence of two parameters, namely the diameter-to-thickness ratio and dent depth. This research does not encompass the analysis and fitting of material-related parameters for the pipelines. Furthermore, the characteristics of the residual stress under the conditions of large diameter-to-thickness ratios and significant dent depths remain unexplored. Future research works should focus on analyzing the impact of the material parameters on the pipeline behavior and investigating the residual stress characteristics under the conditions of large diameter-to-thickness ratios and substantial dent depths.