Optimization of LED Array Spatial Coverage Characteristics in Underwater Wireless Optical Communication
Abstract
1. Introduction
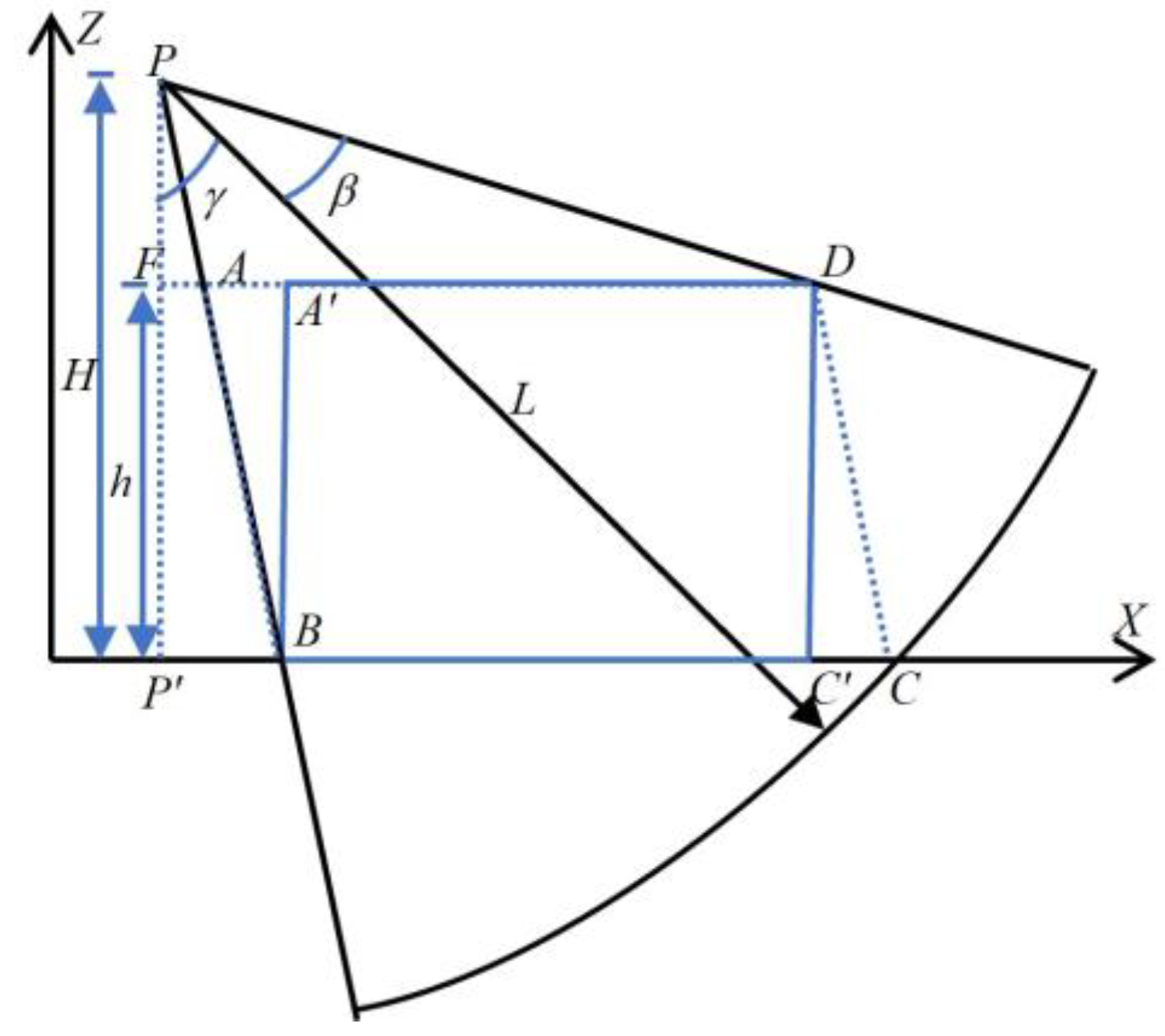
2. System Model
3. System Parameters Optimization
3.1. Optimal Pitch Angle
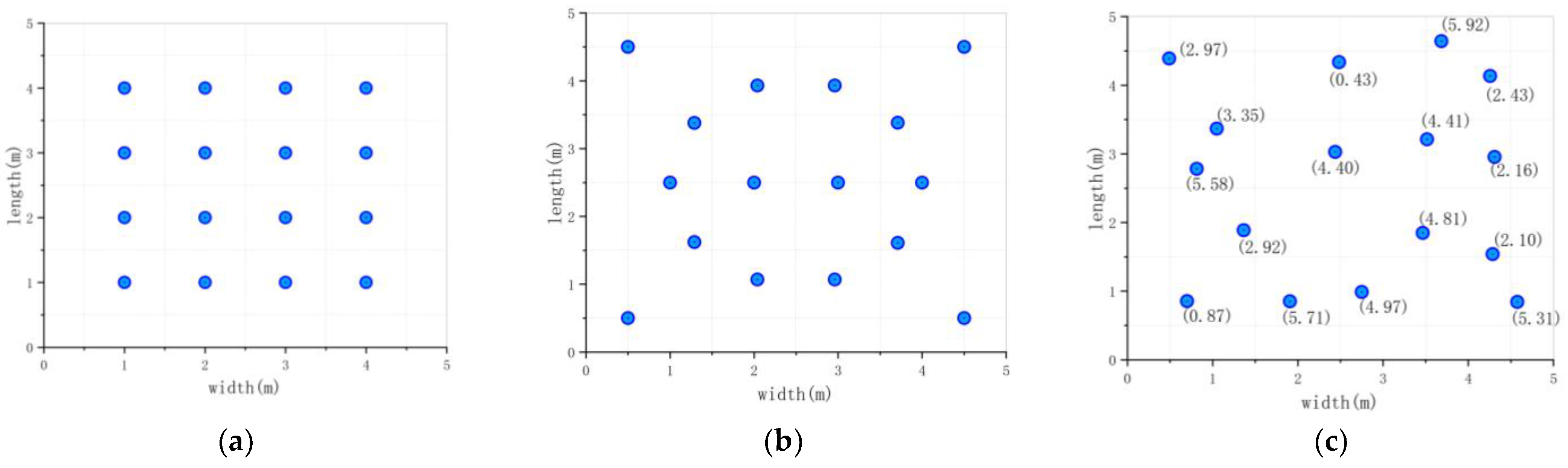
3.2. Improved PSO Algorithm
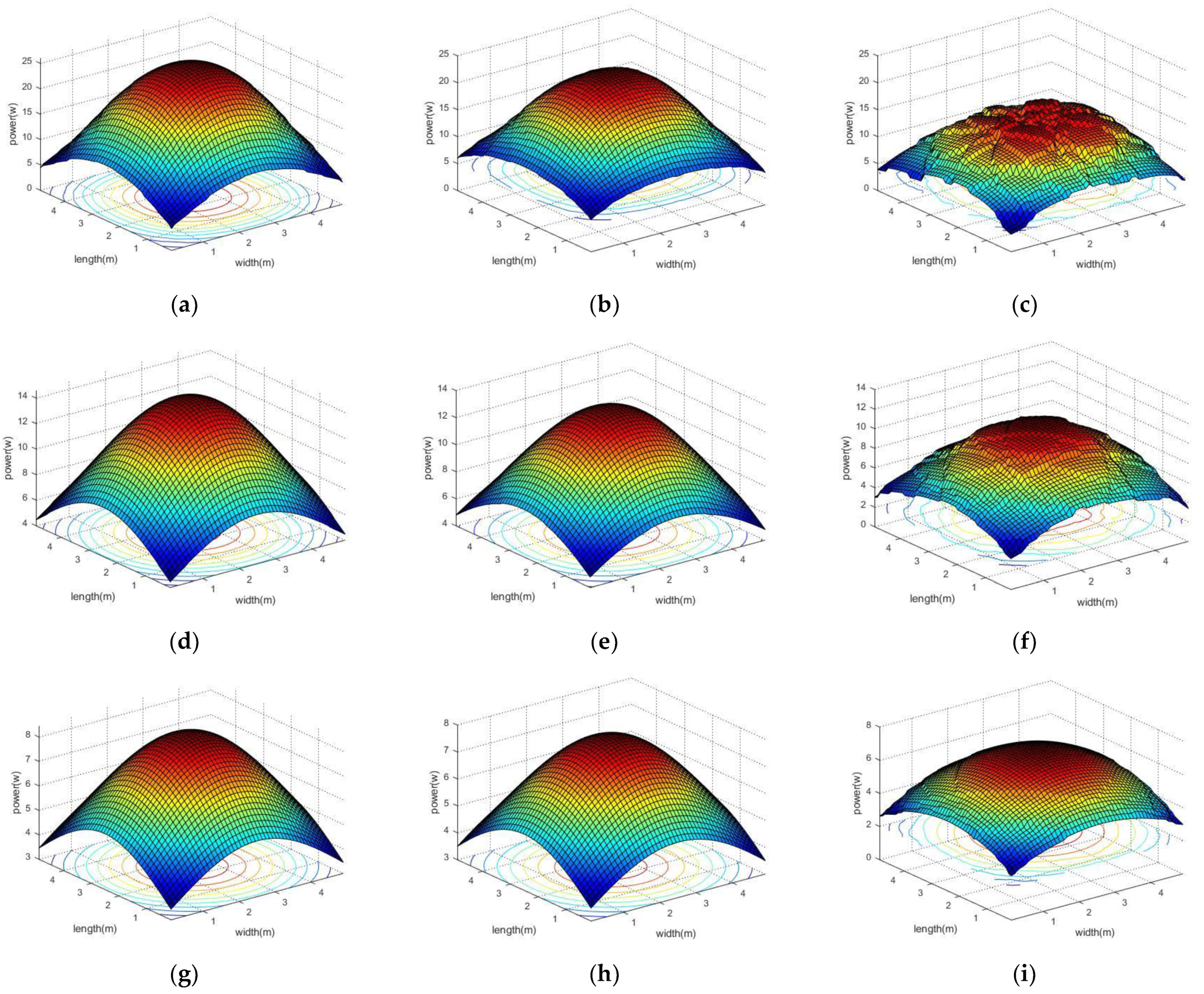
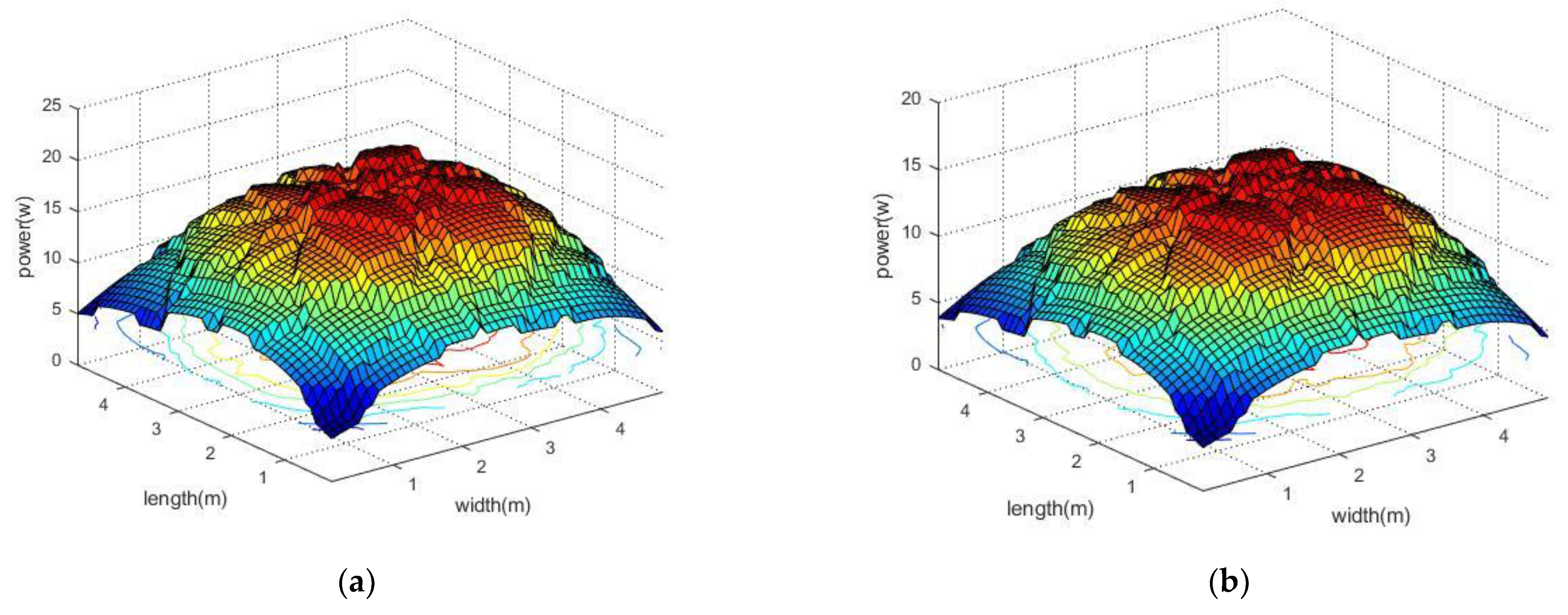
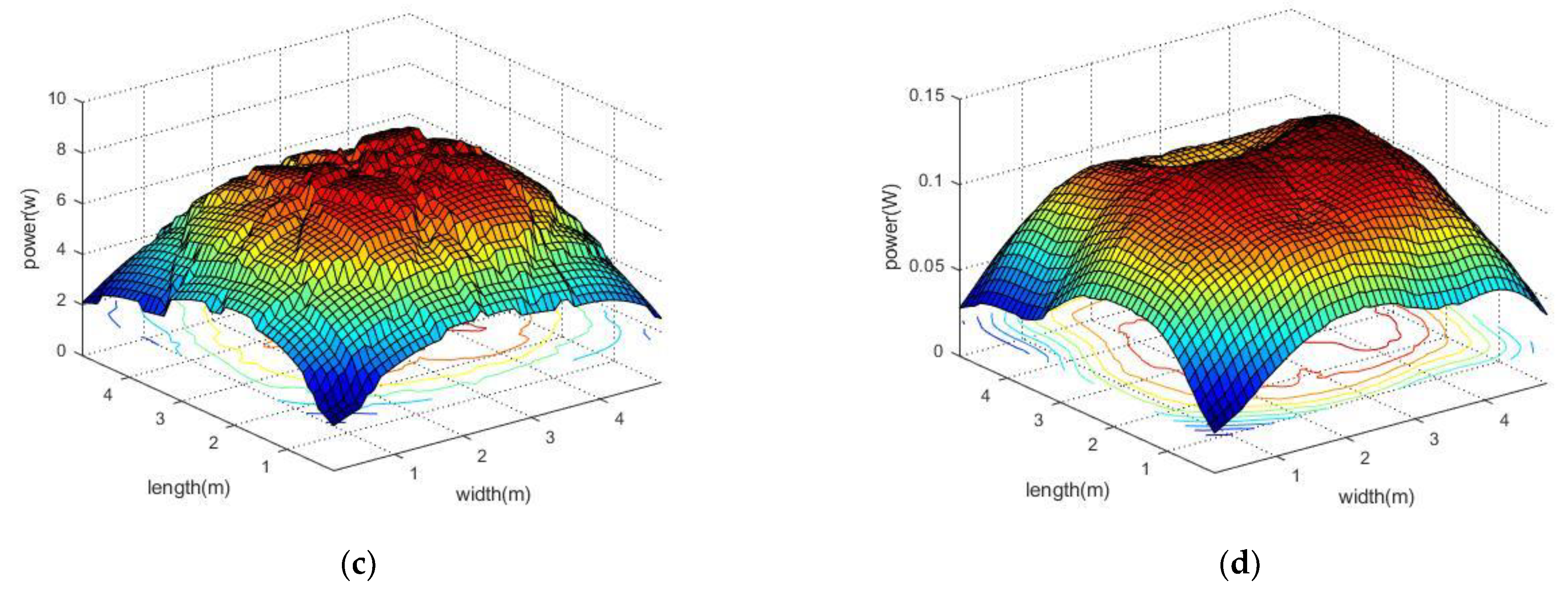
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ali, M.F.; Jayakody, N.K.D.; Chursin, Y.; Affes, S.; Dmitry, S. Recent advances and future directions on underwater wireless communications. Arch. Comput. Methods Eng. 2020, 27, 1379–1412. [Google Scholar] [CrossRef]
- Alexakis, C.; Tsabaris, C. Design of an interactive cellular system for the remote operation of ocean sensors: A pilot study integrating radioactivity sensors. J. Mar. Sci. Eng. 2021, 9, 910. [Google Scholar] [CrossRef]
- Liu, A.; Zhang, R.; Lin, B.; Yin, H. Multi-degree-of-freedom for underwater optical wireless communication with improved transmission performance. J. Mar. Sci. Eng. 2023, 11, 48. [Google Scholar] [CrossRef]
- Connor, J.; Champion, B.; Joordens, M.A. Current algorithms, communication methods and designs for underwater swarm robotics: A review. IEEE Sens. J. 2020, 21, 153–169. [Google Scholar] [CrossRef]
- Wang, C.-N.; Yang, F.-C.; Vo, N.T.M.; Nguyen, V.T.T. Wireless communications for data security: Efficiency assessment of cybersecurity industry—A promising application for UAVs. Drones 2022, 6, 363. [Google Scholar] [CrossRef]
- Zhu, S.; Chen, X.; Liu, X.; Zhang, G.; Tian, P. Recent progress in and perspectives of underwater wireless optical communication. Prog. Quantum Electron. 2020, 73, 100274. [Google Scholar] [CrossRef]
- Li, S.; Qu, W.; Liu, C.; Qiu, T.; Zhao, Z. Survey on high reliability wireless communication for underwater sensor networks. J. Netw. Comput. Appl. 2019, 148, 102446. [Google Scholar] [CrossRef]
- Duntley, S.Q. Light in the sea. J. Opt. Soc. Am. 1963, 53, 214–234. [Google Scholar] [CrossRef]
- Gilbert, G.D.; Stoner, T.R.; Jernigan, J.L. Underwater experiments on the polarization, coherence and scattering properties of a Pulsed Blue-Green Laser. In Underwater Photo Optics I; SPIE: Bellingham, WA, USA, 1966; Volume 7, pp. 8–14. [Google Scholar]
- Zeng, Z.; Fu, S.; Zhang, H.; Dong, Y.; Cheng, J. A survey of underwater optical wireless communications. IEEE Commun. Surv. Tutor. 2017, 19, 204–238. [Google Scholar] [CrossRef]
- Ding, J.; Huang, Z.; Ji, Y. Evolutionary algorithm based power coverage optimization for visible light communications. IEEE Commun. Lett. 2012, 16, 439–441. [Google Scholar] [CrossRef]
- Liu, H.; Lin, Z.; Xu, Y.; Chen, Y.; Pu, X. Coverage uniformity with improved genetic simulated annealing algorithm for indoor Visible Light Communications. Opt. Commun. 2019, 439, 156–163. [Google Scholar] [CrossRef]
- Varma, G.P.; Sushma, R.; Sharma, V.; Kumar, A.; Sharma, G.V.V. Power allocation for uniform illumination with stochastic LED arrays. Opt. Express 2017, 25, 8659–8669. [Google Scholar] [CrossRef] [PubMed]
- Varma, G.P. Optimum power allocation for uniform illuminance in indoor visible light communication. Opt. Express 2018, 26, 8679–8689. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Wang, X.; Chen, Y.; Kong, D.; Xia, P. Optimization lighting layout based on gene density improved genetic algorithm for indoor visible light communications. Opt. Commun. 2017, 390, 76–81. [Google Scholar] [CrossRef]
- Wang, J.; Xu, A.; Ju, J.; Guo, L. Optimization lighting layout of indoor visible light communication system based on improved artificial fish swarm algorithm. J. Opt. 2020, 22, 035701. [Google Scholar]
- Chen, Q.; Zhang, T.; Zheng, W. Optimization of LED layout to improve uniformity of illumination and SNR for indoor visible light communication. In Proceedings of the 2018 28th International Telecommunication Networks and Applications Conference (ITNAC), Sydney, Australia, 21–23 November 2018; pp. 1–3. [Google Scholar]
- Huang, L.; Wang, P.; Wang, J.; Chi, S.; Niu, S.; Nan, X.; Che, H. Optimized design of the light source for an indoor visible light communication system based on an improved bat algorithm. Appl. Opt. 2020, 59, 10638–10644. [Google Scholar] [CrossRef]
- Wei, Z.; Hu, H.; Huang, H. Optimization of location, power allocation and orientation for lighting lamps in a visible light communication system using the firefly algorithm. Opt. Express 2021, 29, 8796–8808. [Google Scholar] [CrossRef]
- Johnson, L.; Green, R.; Leeson, M. A survey of channel models for underwater optical wireless communication. In Proceedings of the 2013 2nd International Workshop on Optical Wireless Communications (IWOW), Newcastle Upon Tyne, UK, 21 October 2013; pp. 1–5. [Google Scholar]
- Uddin, M.S.; Cha, J.S.; Kim, J.Y.; Jang, Y.M. Mitigation technique for receiver performance variation of multi-color channels in visible light communication. Sensors 2011, 11, 6131–6144. [Google Scholar] [CrossRef]
- Kong, M.; Wang, J.; Chen, Y.; Ali, T.; Sarwar, R.; Qiu, Y.; Wang, S.; Han, J.; Xu, J. Security weaknesses of underwater wireless optical communication. Opt. Express 2017, 25, 21509–21518. [Google Scholar] [CrossRef]
- Kaushal, H.; Kaddoum, G. Underwater optical wireless communication. IEEE Access 2016, 4, 1518–1547. [Google Scholar] [CrossRef]
- Gabriel, C.; Khalighi, M.; Bourennane, S.; Léon, P.; Rigaud, V. Monte-Carlo-based channel characterization for underwater optical communication systems. J. Opt. Commun. Netw. 2013, 5, 1–12. [Google Scholar] [CrossRef]
- Engelbrecht, A.P. Computational Intelligence: An Introduction; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Shi, Y.; Eberhart, R.C. Empirical study of particle swarm optimization. In Proceedings of the Congress on Evolutionary Computation, CEC ‘99, Washington, DC, USA, 6–9 July 1999; pp. 1945–1950. [Google Scholar]





| Types of Seawater | a(λ) (m−1) | b(λ) (m−1) | c(λ) (m−1) |
|---|---|---|---|
| pure seawater | 0.041 | 0.003 | 0.044 |
| clear seawater | 0.114 | 0.037 | 0.151 |
| coastal seawater | 0.179 | 0.219 | 0.398 |
| turbid harbor seawater | 0.366 | 1.824 | 2.190 |
| Parameters | Symbol | Value |
|---|---|---|
| size of the model space (m) | L × W × H | 5 × 5 × 5 |
| area of the detector (cm2) | A | 1 |
| LED half-power angle (°) | φ1/2 | 60 |
| quantity of LED arrays | N | 16 |
| single lamp transmitting power (W) | 0.452 | |
| number of lamps in a single LED | 7 × 7 | |
| receiver field of view (°) | ψ | 60 |
| internal reflective index of concentrator | n | 1.5 |
| optical filter gain | Ts | 1 |
| Step | Symbol |
|---|---|
| Step 1 | The required parameters for iteration (number of particles n, range of solution space, the maximum number of iterations Niter, flight speed vi, learning factor ci and inertia factor ω, etc.) are set, and the fitness functions for different seawater characteristics are constructed. |
| Step 2 | The parameter ai = Qid(xid, yid, θid) for each particle, where i takes the values 1, 2, 3, ..., n, calculates the value of the fitness function for each particle, and initializes the number of iterations niter to 1. |
| Step 3 | Comparison of fitness function values based on particle parameters ai and initialization of historical best particles. |
| Step 4 | Updates are made for each particle according to the flight speed, and the values of the fitness function are compared for the new particle parameters ainew after the particles have moved. Updating of the particle parameters when f(ainew) > f(ai). |
| Step 5 | After updating all particles, the best particle in the current particle population is selected. If the current best particle result is better than the historical best particle, update the historical best particle. |
| Step 6 | If niter ≤ Niter, return to step 3, and niter will be self-added by 1. Otherwise, the iterative process of the algorithm is stopped, and the parameter information of the best particle in history after Niter iterations is output. |
| Parameters | Symbol | Value |
|---|---|---|
| particle node size | N | 15 |
| maximum number of iterations | Niter | 4000 |
| learning factor | c1/c2 | 2 |
| flight speed | vi | [−2, 2] |
| inertia factor | ω | [0.4, 2] |
| particle size | n | 16 |
| particle position boundary | L/W | [0, 5] |
| particle angle boundary | θd | [0, 2π] |
| LED Array Layout | Power Variance (dBm) | Average Power (dBm) | Power Range (dBm) |
|---|---|---|---|
| rectangular layout | 2.78 | 16.16 | (4.36, 20.03) |
| hybrid layout | 1.89 | 15.03 | (5.97, 23.10) |
| PSO-optimized layout | 1.44 | 10.82 | (3.29, 15.61) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, A.; Yuan, Y.; Yin, H.; Zhao, H.; Fu, X. Optimization of LED Array Spatial Coverage Characteristics in Underwater Wireless Optical Communication. J. Mar. Sci. Eng. 2023, 11, 253. https://doi.org/10.3390/jmse11020253
Liu A, Yuan Y, Yin H, Zhao H, Fu X. Optimization of LED Array Spatial Coverage Characteristics in Underwater Wireless Optical Communication. Journal of Marine Science and Engineering. 2023; 11(2):253. https://doi.org/10.3390/jmse11020253
Chicago/Turabian StyleLiu, Anliang, Yingming Yuan, Hongxi Yin, Haobo Zhao, and Xianping Fu. 2023. "Optimization of LED Array Spatial Coverage Characteristics in Underwater Wireless Optical Communication" Journal of Marine Science and Engineering 11, no. 2: 253. https://doi.org/10.3390/jmse11020253
APA StyleLiu, A., Yuan, Y., Yin, H., Zhao, H., & Fu, X. (2023). Optimization of LED Array Spatial Coverage Characteristics in Underwater Wireless Optical Communication. Journal of Marine Science and Engineering, 11(2), 253. https://doi.org/10.3390/jmse11020253






