Risk Level Assessment of Typhoon Hazard Based on Loss Utility

Abstract

In the context of climate change with frequent natural disasters, disaster risk assessment can provide great help for related risk decision-making. Based on the theory of loss expectation, this paper presents a quantitative method to assess typhoon disaster risk. Among them, the probability of typhoon occurrence is calculated by fitting the optimal structure function of the sample to the joint distribution of wave height, water increment and wind speed. Then, the loss expectation is expressed as the product of typhoon occurrence probability and loss utility, which is used to quantify the loss result of a typhoon disaster. Using the loss utility theory, the risk grade chart is drawn with the direct economic loss rate and the proportion of the affected population as indicators. The results show that the absolute loss value considering the loss utility is slightly higher than the loss value of the quantitative algorithm by 2% to 25%, indicating that the new model reflects the social group’s aversion to typhoon disaster risk. As can be seen from the risk level zoning map, the highest combined risk level typhoons are Prapiroon 0606 and Chanthu 1003, with a risk level of Category 5. The typhoon comprehensive risk level before 2011 was ≥3, and the typhoon comprehensive risk level from 2012 to 2015 was ≤3. The evaluation model has certain feasibility and practicability, and the results can provide a basis and reference for typhoon risk assessment and decision-making.

1. Introduction

In the context of climate change, which is mainly characterized by global warming, extreme weather and climate disasters have increased significantly and their impact on socio-economic development has become increasingly severe [1,2,3]. Among the extreme weather and climate events, disaster losses caused by tropical cyclones are particularly severe [4,5,6]. For example, the “720” Henan rainstorm event in 2021 was an extreme rainfall event formed under the “push” of Typhoon In-fa. Information from the Central Weather Bureau shows that from 20:00 on the 17th to 20:00 on the 20th, the cumulative rainfall in Zhengzhou is almost equal to the average annual precipitation in Zhengzhou. In addition, the maximum one-hour rainfall has exceeded the extreme value record of the observatory data. This heavy rainfall event caused heavy casualties and widespread social impact. Therefore, professional research for typhoon disaster damage as well as risk level assessment is very necessary for coping with such disasters.

Loss risk can be measured by the expected degree of loss caused by a particular disaster and is a function of the hazard factors and the vulnerability of the disaster-affected body [7]. In the evaluation of typhoon risk losses, the analysis and evaluation standards are often measured according to the size of such average losses. The theoretical methods of risk analysis and evaluation, based on the traditional probability method, have gradually emerged into fuzzy mathematics [8,9], Analytic Hierarchy Process [10], the entropy weight method [11], and many other new methods, and have been greatly developed in practical applications. For example, Chen et al. [12] and Yin et al. [13] multiplied the hazard factor index and the vulnerability index to obtain the typhoon disaster risk index of each study area and drew a risk level map accordingly. Although the assessment of typhoon risk losses has gradually formed a preliminary framework and system, most of them are semi-quantitative and semi-qualitative research results, and there are still relatively few quantitative assessments involving specific losses. However, carrying out refined quantitative typhoon risk assessment is a prerequisite for forward-looking disaster management. Huang [14], Liu et al. [15], and Xu et al. [16] used the information diffusion method to improve typhoon risk estimation and quantify typhoon disaster loss expectations. However, the calculation method of the risk level (R = P × C) is still based on a quantitative algorithm, which cannot reflect the change in the subjective willingness of the social group to the size of the loss. For example, for low-probability, large-loss typhoon disasters and high-probability, small-loss typhoon disasters, the expected value of risk loss calculated by them may be very close, but the degree of aversion of social groups is obviously different. Therefore, it is necessary to explore a quantitative evaluation method that is different from quantitative algorithms.

A certain type of utility value is a subjective representation of the characteristics or state of a certain type of utility. In 1738, Bernoulli first proposed the cardinal utility theory: according to the different personal circumstances of decision makers, the utility felt by increasing the same amount of wealth value is different, and the utility value can be used to express this investment risk attitude. A certain type of utility value is a subjective representation of the characteristics or states of a certain type of utility. In 1738, Bernoulli first proposed the cardinal utility theory: according to the different personal circumstances of decision makers, the utility of increasing the same amount of wealth value is different, and the utility value can be used to express this investment risk attitude. This theory is then widely used in uncertain decision-making in the fields of venture capital [17,18], insurance actuarial [19,20,21], marketing and pricing [14,22,23]. Wu Cisheng et al. [24] (1996) proposed the expected utility decision method of accident loss risk and used the utility function curve to quantitatively analyze the loss of major industrial accidents, which is helpful for enterprises to choose appropriate risk management methods. In the comprehensive safety assessment of ships, Li et al. [25] used the loss utility function to define the loss utility of ship accident risk, and replaced the classical risk formula with the expected risk-utility form, so that the aversion of social groups to risk loss can be considered. The utility theory is introduced in the analysis process of such accident risks, and the evaluation results of loss risk take into account the influence of people’s subjective risk attitude. However, unlike investment behavior, its utility function reflects people’s dissatisfaction and disgust with accidents or dangerous events. In fact, people often show aversion and dissatisfaction with the losses caused by typhoon disasters, and the degree of aversion and dissatisfaction will increase with the degree of risk loss [26]. In contrast, most recent models for risk assessment of typhoons only calculate loss expectations based on quantitative algorithms. There are relatively few studies on risk assessment criteria that take into account the risk attitudes (dissatisfaction and willingness to avoid risk events) of the affected population. Therefore, it is a meaningful preliminary exploration to apply the loss utility function to the quantitative typhoon risk assessment model.

Based on the above analysis, this paper proposes a quantitative assessment method of typhoon disaster risk considering loss utility. The quantitative risk assessment model is based on the loss expectation theory and uses the loss expectation value (expressed by the product of typhoon occurrence probability and loss utility) to measure the risk level of typhoon disasters. The probability of typhoon occurrence is calculated according to the three-dimensional joint distribution function established by the intensity of typhoon disaster-causing factors, and the loss utility is calculated based on the inverse function of the exponential utility function (referred to as the loss utility function in this paper). At the same time, the risk level graph of relative loss rate and typhoon probability is given according to the loss utility function. The remainder of the paper is structured as follows: Section 2 introduces the study area, the data used, and the methodology. Section 3 is the detailed calculation process of typhoon disaster risk analysis and grade evaluation considering the utility. Finally, conclusions are offered in Section 4.

2. Materials and Methods

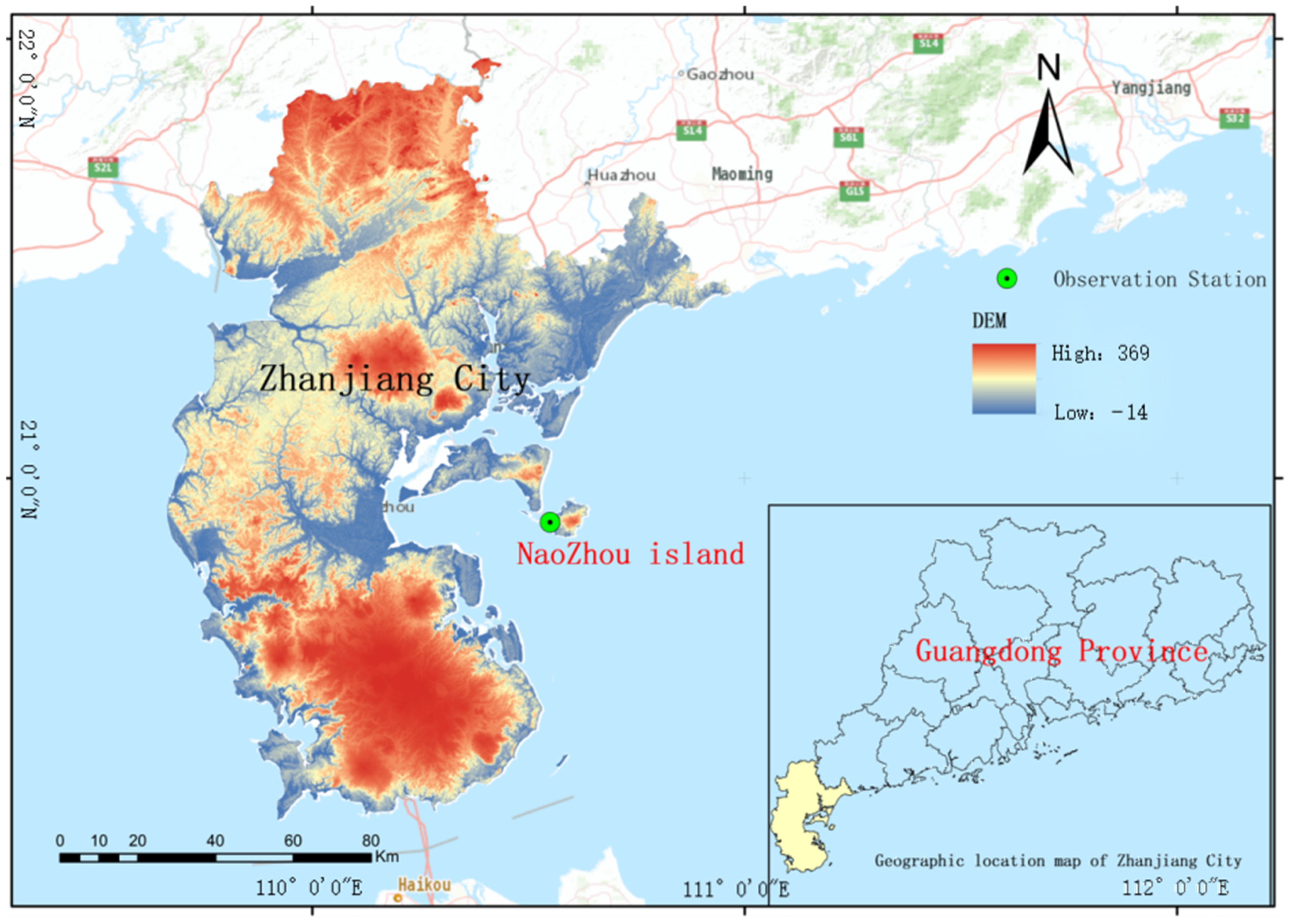
2.1. Study Area

Based on the expected utility theory, the functional relationship between the intensity of disaster-causing factors and the vulnerability of hazard-affected bodies is established, and the loss utility is introduced into the vulnerability analysis of hazard-affected bodies. The expected value of loss utility is used to measure the risk loss caused by possible typhoon disasters, and the typhoon risk level is divided based on the deterministic loss with the same degree of aversion as relative loss aversion. In the new model, it can not only quantify the degree of typhoon risk loss with the loss expectation of loss utility but also reflect the risk attitude of the affected population to typhoon losses.

The degree of dissatisfaction of social groups arising from risky losses can be measured in terms of utility, while in the field of typhoon disaster risk assessment, the main consideration is the degree of dissatisfaction as well as aversion of the affected population due to losses caused by typhoon disasters.

Definition 1.

If n typhoons occur in the study area within a certain time scale, and there are m key indicators for typhoon risk loss assessment identification, let their corresponding loss values be lij (i = 1,2, …, n; j = 1,2, …, m), then the first i typhoon has an aversion utility of μ(lj) (j = 1,2, …, m).

The loss utility function is a mathematical measure of the loss utility. It has the following properties: the loss utility μ increases with the increase in risk loss l, and the risk loss utility increases with the increase in risk loss. That is to say, the first derivative and the second derivative of the loss utility function μ with respect to l are greater than zero. The curve of the loss utility function is shown in Figure 1 below.

There are three common types of utility functions in general: exponential, logarithmic and binomial [27,28]. In view of the research results of Peng et al. [29] in coal mine safety risk assessment, Gao et al. [30] in-flight safety evaluation and Jiang and Liu [31] in the evaluation of dike raising scheme, we determine the expression form of the loss utility function as the following:

$$\mu (l)=-\frac{1}{\alpha }\ln (1-\alpha l)$$

(1)

where α > 0 and it is a constant, termed as the risk aversion parameter, which indicates the aversion of the affected group to typhoon risk losses. The larger it is, the higher the degree of aversion to risk losses.

It is reasonable to choose formula (1) as a concrete expression for the loss utility function:

i.

μ(l)′ > 0 and μ(l)″ > 0 conform to the property of loss of utility (Figure 1);

ii.

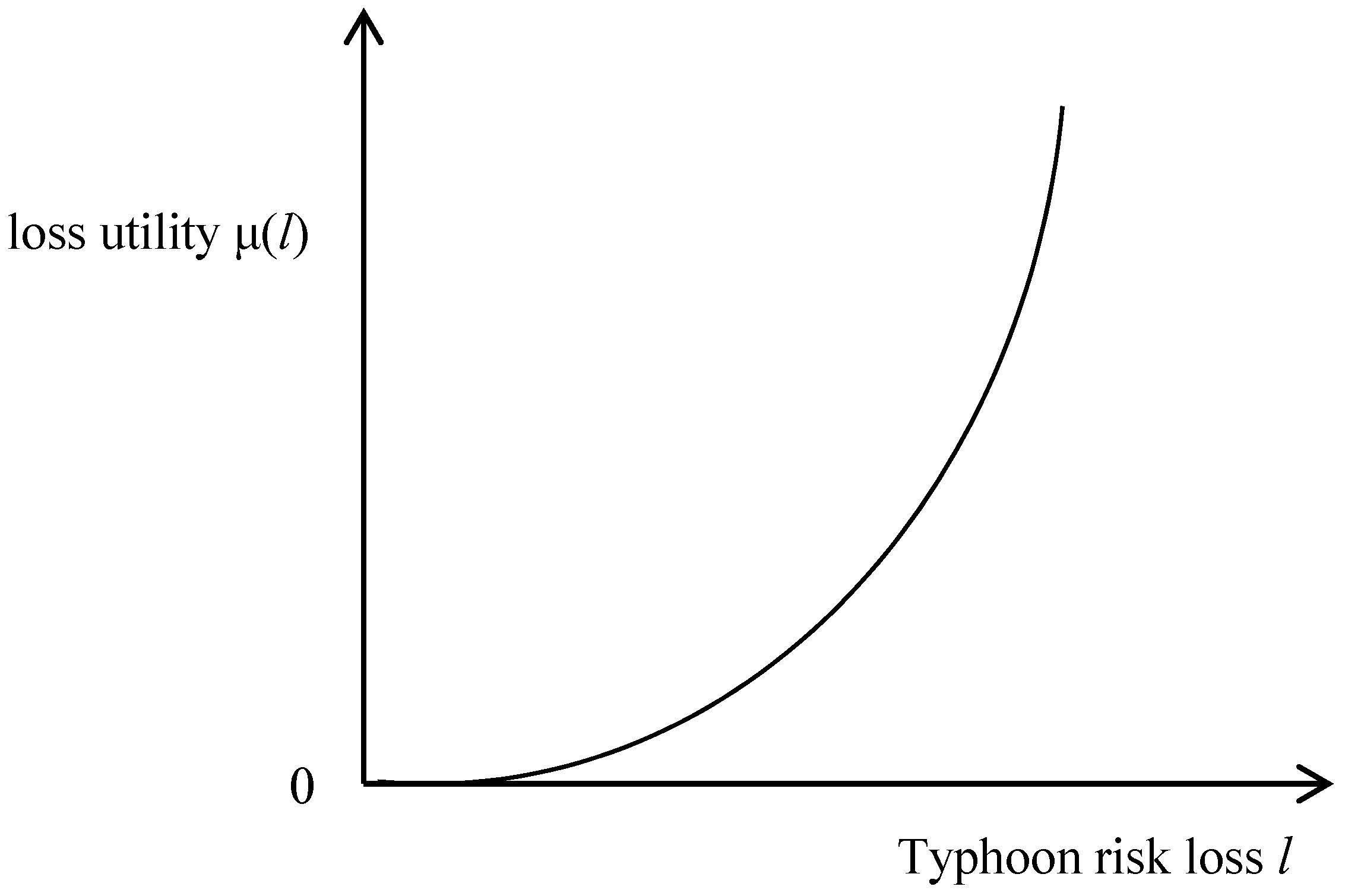
When α = 0, $\underset{\alpha \to 0}{\mathit{lim}}\mu \left(l\right)=-\underset{\alpha \to 0}{\mathit{lim}}\frac{ln\left(1-\alpha l\right)}{\alpha }=-\underset{\alpha \to 0}{\mathit{lim}}\frac{-l}{\left(1-\alpha l\right)}=l$.

That is, when the risk aversion attitude is not considered, the loss utility value is equal to the loss value. It can also be interpreted as “risk neutral” as a special case of loss of utility when the utility coefficient α = 0.

The risk aversion parameter in Equation is unknown, and it is generally necessary to determine its value by the “deciding optimal through questioning method”, “curve fitting method” and “utility consistency method”, etc. [32]. However, due to the difficulty of collecting some disaster relief information, the value of the risk aversion parameter in this paper is taken from the literature, which uses the lower limit of the number of fatalities from different levels of water traffic accidents as the number of accident fatalities l and the amount of fine is used to represent the aversion of the social group $\mathsf{\mu }\left(\mathrm{l}\right)$, with the fit was performed to obtain $\mathrm{a}$ ≈ 0.019 [33].

Definition 2.

If p_i represents the probability of Typhoon i, l_ij (j = 1,2, … m) represents the loss amount of item j caused by typhoon i (such as direct economic loss, casualties, farmland affected area, etc.); U_ij represents the loss expectation of item j caused by the impact of typhoon i; then, according to the expected utility theory of von Neumann and Morgenstern, there are:

$$U_{ij}=p_i\times \mu \left(l_{ij}\right),i=1,\dots ,m,j=\mathrm{1,2},\dots ,m$$

(2)

In the evaluation and analysis of typhoon risk level, U_ij can be used to measure the risk level of the typhoon disaster. The larger U_ij is, the higher the risk level is; otherwise, the smaller the risk level is.

Since μ(lij) = lij when α = 0, then formula (2) degenerates into the classical quantitative algorithm of loss expectation:

$${ U}_{ij}=p_i\times l_{ij},i=1,\dots ,m,j=\mathrm{1,2},\dots ,m$$

(3)

That is, Formula (3) is a special case of Formula (2).

Formula (2) Explanation of the application of the function form used in typhoon risk analysis: Starting from the risk facts, typhoon disasters often cause discontentment and aversion in the affected people, and this dissatisfaction will increase with the aggravation of the degree of loss, and the increasing speed will be faster and faster. Mathematically speaking: For the function describing this dissatisfaction, the first and second derivatives are greater than 0. In this paper, the function describing this phenomenon is taken as the inverse function of the utility function (called loss utility function in this paper), and the specific expression is shown in formula (1): $\mu (l)=-\frac{1}{\alpha }\ln (1-\alpha l)$, it can be found that μ(l)′ > 0, μ(l)″ > 0, so it can be considered that the loss utility function is suitable for typhoon disaster risk analysis. In addition, μ(lij) = lij can be understood as a “risk neutral (α = 0)” case. In other words, in the traditional loss expectation formula, the amount of loss is directly substituted into the calculation, and the risk attitude of the affected body is not considered.

Regarding the probability of typhoon occurrence, it is difficult to give a direct probability value because of their random nature. If the probability of a typhoon is calculated based on the frequency of typhoons, such a probability value only characterizes the likelihood of typhoons occurring during the year and does not reflect the intensity of typhoons. In reality, when a typhoon crosses the harbor, the gale force winds not only act directly on the harbor buildings but can also bring huge waves, posing a threat to the structural safety of the harbor structures. Secondly, the violent atmospheric disturbances caused by the approaching typhoon can bring heavy rainfall and trigger secondary flooding. In addition, typhoons moving into shallow offshore waters can also trigger storm surges if they meet with astronomically high tides, causing abnormal sea level rise and triggering the risk of seawater spillover [31,34,35,36]. Thus, it shows that the hazard of typhoons mainly comes from high winds, huge waves, heavy rainfall and storm surges, which can be characterized by three causative factors, namely wind speed, wave height and water increase. The magnitude of the disaster-causing factors can directly reflect the magnitude of damage when a disaster is likely to occur. Therefore, in this paper, the probability of typhoon occurrence is characterized by the probability of wind speed, wave height and water increase.

Since the Copula functional model is not restricted by the form of marginal distribution, it is able to organically combine different degrees of correlation and correlation patterns among random variables while building a joint structure [37,38]. Therefore, this paper adopts the Copula function for the probability of typhoon occurrence in structure function construction.

If the continuous random variables x,y,z represent the extreme wave height, water increase, and wind speed in the study area under the influence of a typhoon, respectively, assume that they obey marginal distributions of Q(x), G(y) and H(z). The joint probability distribution of the occurrence of each risk factor is denoted as F(x,y,z), According to Sklar’s theorem [11], there exists a unique Copula function C such that:

$$F\left(x,y,z\right)=C\left(Q\left(x\right),G\left(y\right),H\left(z\right)\right)$$

(4)

Common three-dimensional asymmetric Copula functions mainly have the following three types (Q(x), G(y) and H(z) are abbreviated as u, v, w to make the formula more concise):

(1)

Gumbel Copula function:

$$C\left(u,v,w\right)=exp\left\{-{\left[{\left({\left(-\ln u\right)}^{{\theta }_2}+{\left(-\ln v\right)}^{{\theta }_2}\right)}^{{\theta }_1/{\theta }_2}+{\left(-\ln w\right)}^{{\theta }_1}\right]}^{1/{\theta }_1}\right\},{\theta }_1\le {\theta }_2,{\theta }_1,{\theta }_2\in \left(1,\infty \right)$$

(2)

Frank Copula function:

$$C\left(u,v,w\right)=-\frac{1}{{\theta }_1}ln\left\{1-{\left(1-e^{-{\theta }_1}\right)}^{-1}\left[1-{\left(1-{\left(1-e^{-{\theta }_2}\right)}^{-1}\cdot \left(1-e^{-u{\theta }_2}\right)\cdot \left(1-e^{-v{\theta }_2}\right)\right)}^{{\theta }_1/{\theta }_2}\right]\left(1-e^{-w{\theta }_2}\right)\right\},{\theta }_1\le {\theta }_2$$

M6 Copula function: It is qualitatively a combination of two two-dimensional Gumbel copulas with different parameters, so it is also called Asymmetric Gumbel nested copula, with M6₁ Copula, M6₂ Copula, and M6₃ Copula. The expressions of its function structure are as follows:

$$\begin{gathered} C\left(u,v,w\right)=exp\left\{-{\left[{\left({\left(-\ln u\right)}^{{\theta }_{11}}+{\left(-\ln v\right)}^{{\theta }_{11}}\right)}^{{\theta }_{21}/{\theta }_{11}}+{\left(-\ln w\right)}^{{\theta }_{21}}\right]}^{1/{\theta }_{21}}\right\},{\theta }_{11},{\theta }_{21}\in \left(1,\infty \right) \\ C\left(u,v,w\right)=exp\left\{-{\left[{\left({\left(-\ln u\right)}^{{\theta }_{12}}+{\left(-\ln v\right)}^{{\theta }_{12}}\right)}^{{\theta }_{22}/{\theta }_{12}}+{\left(-\ln w\right)}^{{\theta }_{22}}\right]}^{1/{\theta }_{22}}\right\},{\theta }_{12},{\theta }_{22}\in \left(1,\infty \right) \\ C\left(u,v,w\right)=exp\left\{-{\left[{\left({\left(-\ln u\right)}^{{\theta }_{13}}+{\left(-\ln v\right)}^{{\theta }_{13}}\right)}^{{\theta }_{23}/{\theta }_{13}}+{\left(-\ln w\right)}^{{\theta }_{23}}\right]}^{1/{\theta }_{23}}\right\},{\theta }_{13},{\theta }_{23}\in \left(1,\infty \right) \end{gathered}$$

where, u, v and w are edge distribution functions, respectively; θ₁, θ₂, θ₁₁, θ₁₂, θ₁₃, θ₂₁, θ₂₂, and θ₂₃ are all parameters of the Copula function and can be estimated by maximum likelihood method.

If the M6₂ Copula function is used in this paper to construct a three-dimensional joint probability distribution, and if these three variables obey the Gumbell distribution, Pearson distribution and Pearson distribution, respectively, then

$$\begin{gathered} F\left(x,y,z\right)=\mathit{exp}(-\{[(-\mathit{ln}Q\left(x\right){)}^{{\theta }_{12}}+(-\mathit{ln}H\left(z\right){)}^{{\theta }_{12}}{]}^{\frac{{\theta }_{22}}{{\theta }_{12}}} \\ +(-\mathit{ln}G\left(y\right){)}^{{\theta }_{22}}{\}}^{\frac{1}{{\theta }_{22}}}) \\ =\mathit{exp}(-\{[(-\mathit{lnexp}\left\{-\mathit{exp}\left[-a\left(x-b\right)\right]\right\}{)}^{{\theta }_{12}} \\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}+(-\mathit{ln}{\int }_{a_{02}}^z\frac{{{\beta }_2}^{{\alpha }_2}}{\Gamma \left({\alpha }_2\right)}(z-a_{02}{)}^{{\alpha }_2-1}{e}^{-{\beta }_2\left(z-a_{02}\right)}dz{)}^{{\theta }_{12}}{]}^{\frac{{\theta }_{22}}{{\theta }_{12}}} \\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}+(-\mathit{ln}{\int }_{a_{01}}^y\frac{{{\beta }_1}^{{\alpha }_1}}{\Gamma ({\alpha }_1)}(y-a_{01}{)}^{{\alpha }_1-1}{e}^{-{\beta }_1(y-a_{01})}dy{)}^{{\theta }_{22}}{\}}^{\frac{1}{{\theta }_{22}}}) \end{gathered}$$

(5)

where, b and a represent the reciprocal of position coefficient and scale coefficient of the Gumbell distribution, respectively; Γ(α₁) and Γ(α₂) are gamma functions of α1 and α₂, respectively; α₁, β₁, α₂, β₂ are the shape scale parameters of the Pearson-III distribution, and their values are all greater than 0. a₀₁ and a₀₂ are positional unknown parameters of Pearson’s three-type distribution.

Direct economic losses and casualties are chosen as indicators of the vulnerability of the disaster-bearing population, such as L₁ and L₂. Let the vulnerability loss to a study area due to the combined effect of the contributing factors be L_j (x,y,z), then the expected loss due to the typhoon in the study area Uj can be expressed as

$$U_j=F\left(x,y,z\right)\times {\mu (L}_j\left(x,y,z\right)) j=\mathrm{1,2}$$

(6)

where L_j (x,y,z) is the vulnerability function characterizing the risk-bearing body. It can be calculated by identifying the constituent sample from the hazard intensity-loss data recorded in the disaster. Because it is calculated based on direct economic losses or affected populations, it is the absolute loss generated by the occurrence of a typhoon in a given study area. If the value of U_Rj represents the aversion utility of the absolute losses caused by the occurrence of a particular typhoon, then

$${{ U}_{Rj}=\mu (L}_j\left(x,y,z\right)) j=1.2$$

(7)

Absolute loss aversion reflects the degree of aversion caused by a certain absolute loss to the affected population in a fixed study area. The larger the value of absolute loss aversion, the higher the degree of aversion, and vice versa. For typhoon hazards, it is applied to measure the amount of typhoon risk for a particular city.

Since the same loss amount poses different risk sizes for cities of different scales. At this point, it is obviously not reasonable to compare the amount of typhoon risk losses in two different regions by comparing their loss utilities of absolute loss. In contrast, relative loss aversion utility needs to be introduced. The relative loss effect is the calculation of utility for relative losses, which requires calculating the proportion of losses in total assets and then calculating their utility. If the gross product of a study region is denoted as GrossV, the relative loss aversion effect for a typhoon disaster is set to U′_Rj, then we can have

$${U_{Rj}^{\prime }=\mu (L}_j\left(x,y,z\right)/GrossV) j=1.2$$

(8)

In the previous part, we used L(x,y,z) to characterize the potential loss scenario for the hazard-bearing region under some combination of extreme wave height, water increase, and wind speed occurring simultaneously, which is a stochastic loss. For decision-making on typhoon risk, compared to stochastic losses L(x,y,z) we are more interested in the magnitude of the fixed loss l. Theoretically, the dissatisfaction that can be brought about by the losses faced by the disaster-bearing body is the same as the dissatisfaction that can be brought about by the direct losses l. Therefore, we can solve the Equation (6) to get l. At this point, l denotes the definitive loss value with the same degree of aversion as the absolute loss expectation for each typhoon hazard. Similarly, l′ can be inversely calculated according to formula (8). l′ represents the definitive loss value with the same degree of aversion as the total expected relative loss for each typhoon hazard. Something should be noted as follows:

i.

Since Equation (8) is calculated under the condition of relative loss, it is independent of the city scale. It means that the same value brings the same degree of aversion for different cities. l′ clearly has a range of [0,1].

ii.

The transformation of Equations (6) and (8) actually converts the random loss into a fixed loss. For instance, l′ = 30%, then it can be said that the typhoon risk faced by the city is equal to 30% of the total output loss of the city in the sense of relative loss aversion.

When mapping typhoon risk levels map, risk partitioning can be performed by drawing expected loss aversion contours. The area between the abscissa and the ordinate and the first contour is marked as a first-level risk area, and the area between the first contour and the second contour is marked as a second-level risk area so that other level areas can be similarly marked outward. The higher the number, the higher the risk level in the region. Finally, the risk level of each typhoon is judged by its fallout point (F, L′).

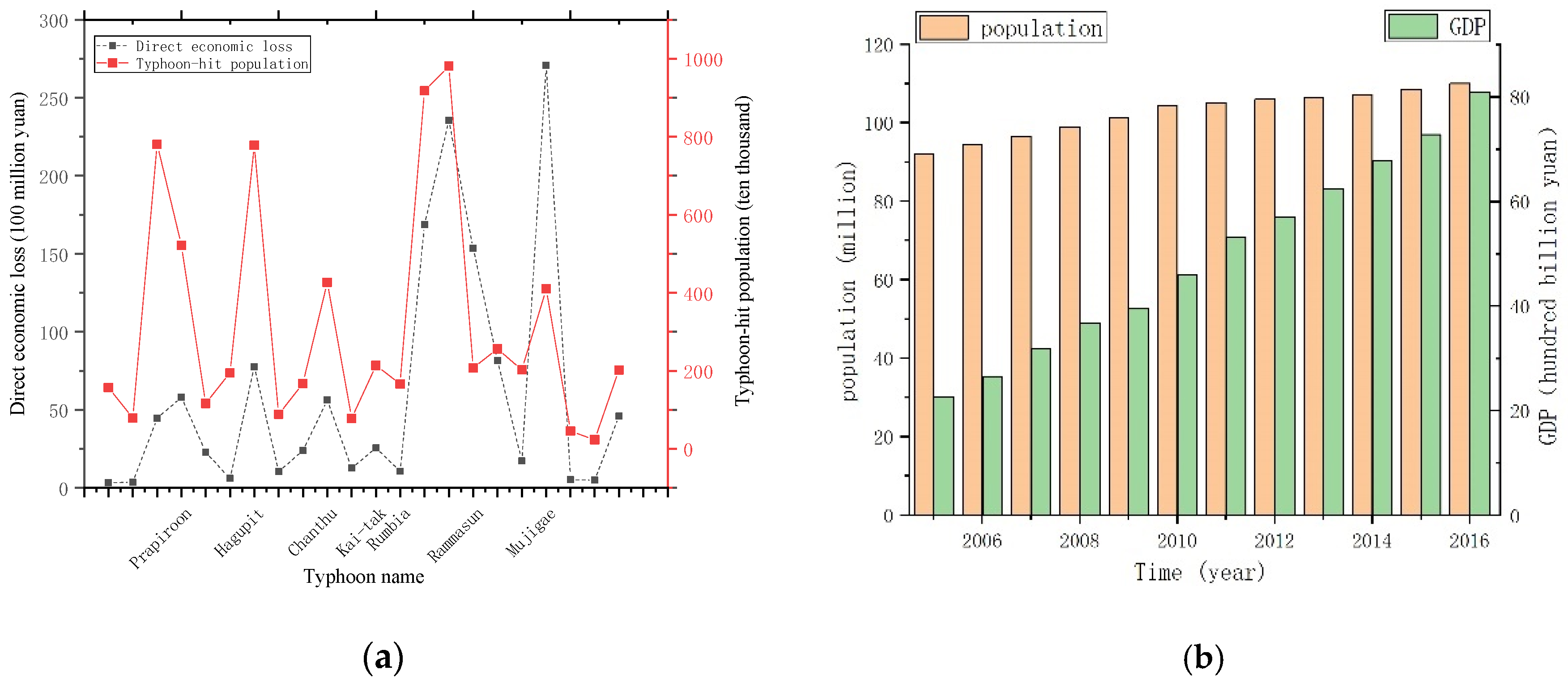
2.2. Data Sources

The study area selected for this paper is Naozhou Island. Naozhou Island is located between 20° and 21°N latitude, about 40 km southeast of Zhanjiang City, Guangdong Province. It is located in the western waters of Guangdong Province and is one of the most severely affected areas by typhoons in China. Its topographic map is shown in Figure 2.

The data used in this paper are mainly typhoon data in the study area (wind speed in the typhoon center, wind wave data during the typhoon), typhoon disaster data and basic situation data of the study area.

Typhoon data included 22 records of typhoon extreme wind speed (in the study area), extreme water increase and extreme wave height from 1990 to 2016 (missing 2004 and 2007). The data are from the Naozhou Oceanographic Observatory and the Naozhou Island Typhoon statistical data set.

The basic data of the study area includes typhoon disaster data, regional population and GDP. The typhoon disaster data collected this time came from the China Marine Disaster Bulletin and the Guangdong Marine Disaster Bulletin, including 22 typhoon disaster data that occurred in Guangdong Province from 2005 to 2016 (as shown in Figure 3a). The data on the permanent population (Gross V₁) and GDP (Gross V₂) of Guangdong Province for 2005–2016 are from the website of the National Bureau of Statistics (as shown in Figure 3b). Considering the availability of data and in order to ensure the accuracy of calculation, this paper selects seven typhoons landing within 100 km of Sal Sal Island from 2005 to 2016 (the typhoon names are marked on the horizontal coordinate in Figure 3a) as samples to calculate the typhoon loss expectation.

3. Engineering Calculation Example

3.1. Model Calculation

3.1.1. Probability of Typhoon Occurrence

The annual extreme wind speed and its accompanying wave height and water increase values were extracted from the collected original data as samples, and then the Kolmogorov-Smirnov test was used to conduct statistical tests on them. The specific calculation results are shown in

Table 1. Statistics of K-S test results for each sample data.

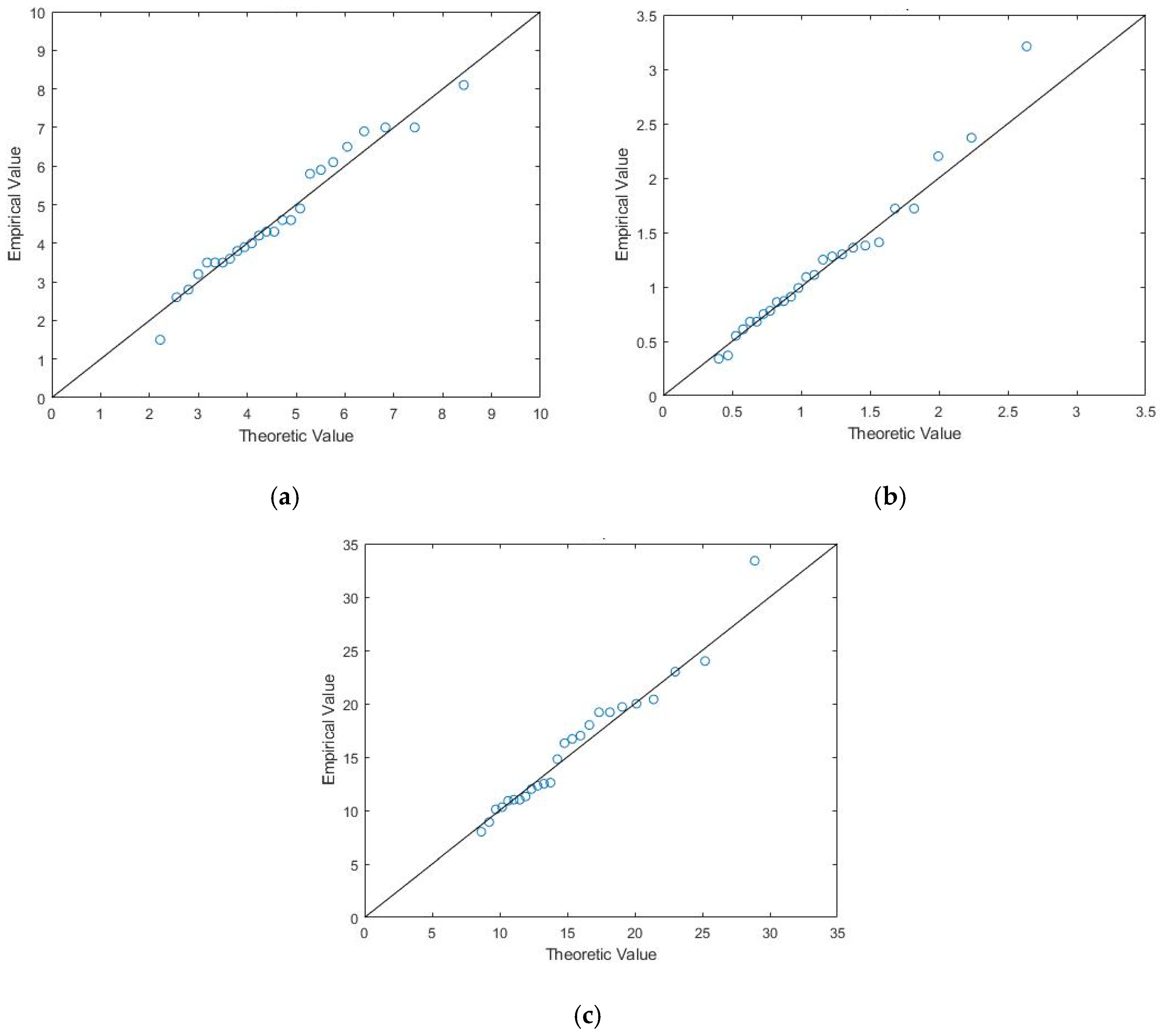
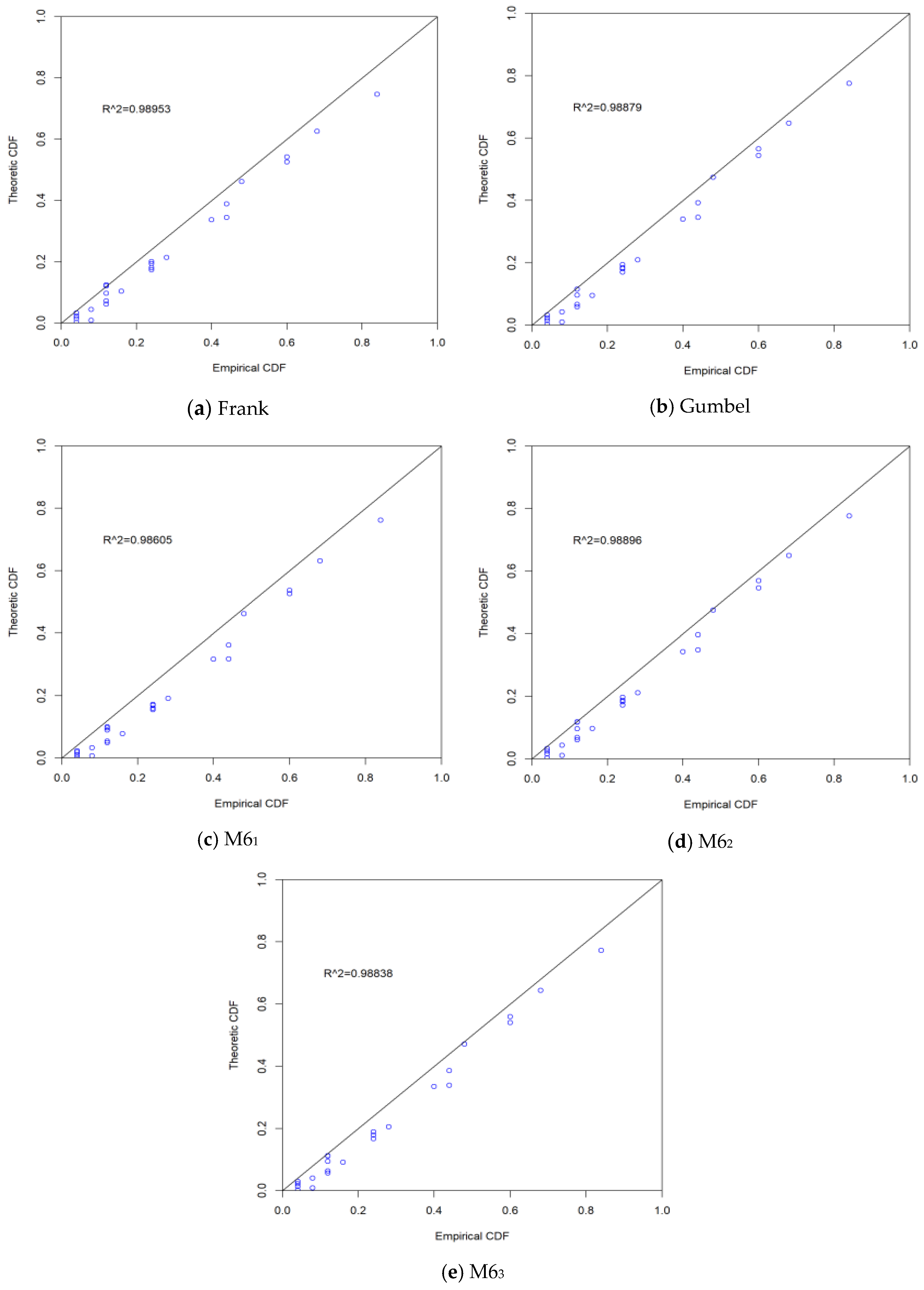
Distributions	Wave Height (m)	Water Increment (m)	Wind Speed (m/s)
Gamma distribution	0.11364 (0.9511)	0.10621 (0.9405)	0.17019 (0.4639)
Gumbel distribution	0.11006 (0.9225)	0.097616 (0.9908)	0.15869 (0.5548)
Pearson-III distribution	0.11716 (0.8825)	0.093219 (0.9816)	0.11475 (0.8970)

below. The values in parentheses are p-values, representing the lowest level of significance difference in the sample.

From the calculated results in Table 1, we can clearly see that all sample data passed the hypothesis test. Moreover, the best distribution of fitting sample data of wave height, water increase and wind speed are the Gumbel distribution, Pearson-Ⅲ distribution and Pearson-Ⅲ distribution, respectively. To further verify the fit of the sample data, Quantile-Quantile (The following will be referred to as Q-Q) plots were plotted for the wave height, wind speed, and water increase samples to test the similarity of the data distributions. The Q-Q diagram is shown in Figure 4, it can be seen from the figure that the sample points are roughly close to the diagonal, and more than 60% of the points fall on the diagonal. So, we can consider the sample data to be approximately obeying the above-preferred distribution types.

Therefore, the optimal marginal distributions selected for this calculation of the wave height, water increase, and wind speed sample series are the Gumbel distribution, the Pearson-III distribution, and the Pearson-III distribution, respectively.

The next step is to construct the 3D joint distribution model. First of all, the Frank Copula function, M6 Copula function and Gumbel Copula function were chosen to fit the 3D sample series of wave height-water increase-wind speed. Then, the maximum likelihood method is used to estimate the parameters. Finally, Root Mean Square Error (abbreviated as RMSE) values and Akaike information criterion (abbreviated as AIC) values of the goodness-of-fit evaluation index are calculated. The results are shown in

Table 2. Parameter estimates and goodness-of-fit tests for Copula function.

Copula Function	Frank	Gumbel	M61	M62	M63
Estimate	2.67	1.415	(1.836, 1.272)	(1.211, 1.433)	(1.399, 1.382)
AIC	−180.258	−184.665	−177.824	−185.179	−183.523
RMSE	0.0261	0.0239	0.0274	0.0237	0.0245

below.

As can be seen from Table 2, the M6₂ Copula function computes the smallest AIC and RMSE values among the selected combinatorial functions. In view of this, it can be concluded that the M6₂ Copula function has the best-fitting effect on the three-dimensional sample sequence.

On this basis, we also plotted Q-Q plots for each group of samples. And the graphs were used to test the fitting effect of different Copula function types for the measured data. The results, as shown in Figure 5, present that the scattering of the sample points tends to be near the diagonal. It means that the fitting is good. From the observed and theoretical values, the M62 Copula function has the best overall fit, which is higher than the other Copula functions.

Therefore, the M6₂ Copula function is the optimal choice for this study to establish the three-dimensional joint distribution of wave height, water increase and wind speed for calculating the probability of typhoon occurrence.

At this point, the specific expression of each edge distribution can be substituted into the M6₂ Copula function to construct the three-dimensional joint distribution of wave height, water increase and wind speed, as shown in Formula (4).

Subsequently, the wave height can be selected separately X = 1.876 m, 4.221 m, 6.565 m; with water increase Y = 0.743 m, 1.673 m, 2.602 m; and wind speed Z = 7.735 m/s, 17.403 m/s, 27.072 m/s as the conditions and plot a four-dimensional slice of the joint distribution of the three variables, as shown in Figure 6.

By substituting the maximum wind speed of the seven typhoons landing on Naozhou Island and their accompanying wave height and water increase data into the three-dimensional joint distribution model established above, the probability of occurrence of each intensity typhoon can be obtained as in

Table 3. Probability of typhoons making landfall within 100 km of Naozhou Island from 2005 to 2016.

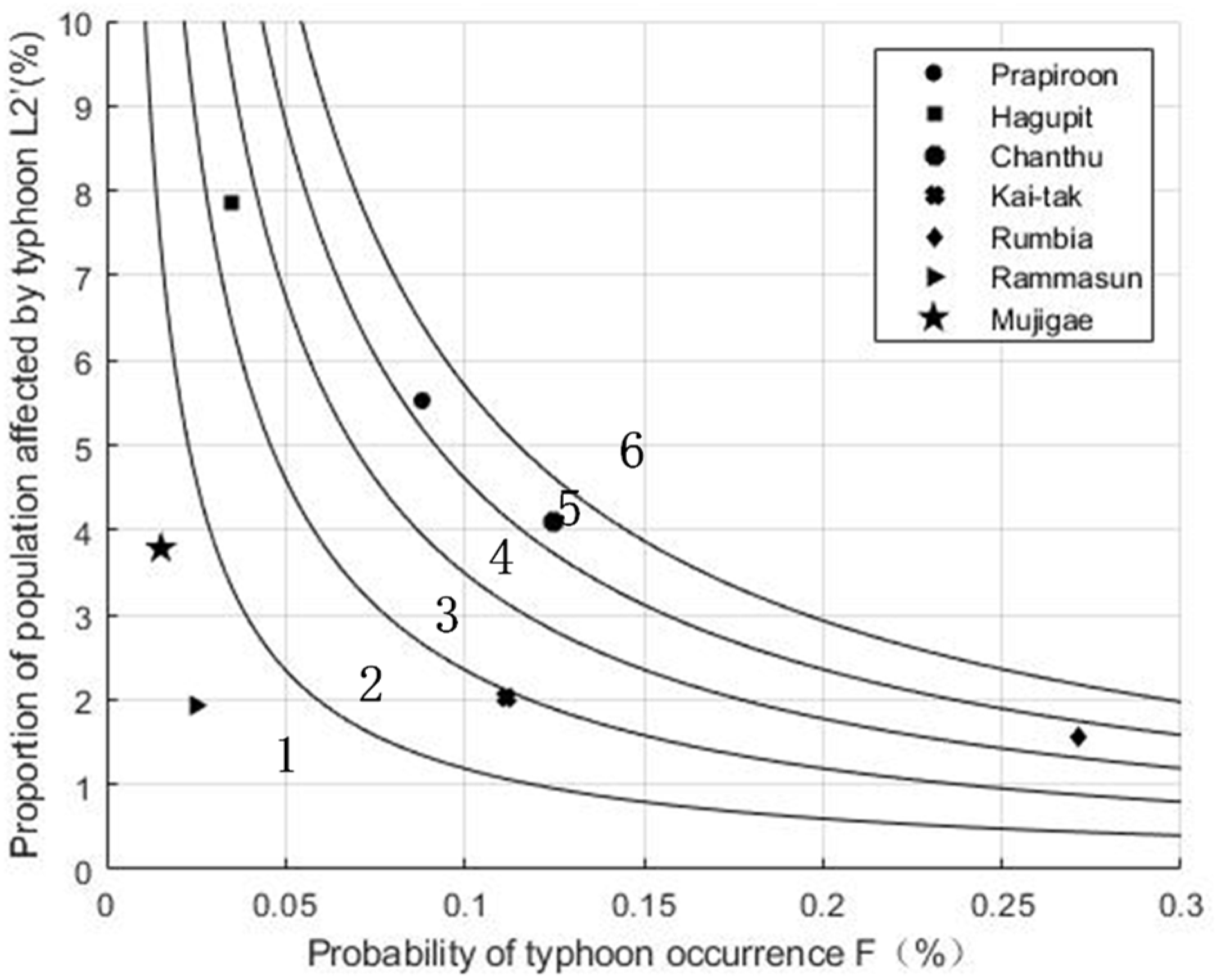
Number	Name	Landing Time	Level	Typhoon Occurrence Probability F
0606	Prapiroon	8.1	12	0.0881
0814	Hagupit	9.24	15	0.0347
1003	Chanthu	7.22	12	0.1248
1213	Kai-tak	8.17	13	0.1116
1306	Rumbia	7.2	11	0.2710
1409	Rammasun	7.18	15	0.0249
1522	Mujigae	10.4	15	0.0152

.

Landfall time is the landfall time of the typhoon, recorded in the form of month/day; The classification is the wind speed classification of extreme wind speed, and the classification standard is carried out according to the national standard of Tropical Cyclone Classification [39].

3.1.2. Absolute Loss Aversion and Fixed Absolute Losses

The International Strategy for Disaster Reduction (ISDR) has proposed a conceptual formula for natural disaster risk. That is: the possibility of damage (loss expectation) caused by natural disasters can be expressed as the product of the hazard of the causal factor and the vulnerability of the disaster-bearing body. The calculation results based on the quantitative algorithm (i.e., R = F × L) are now used as the loss expectation of typhoon disaster risk. At the same time, the results are compared with the loss expectation calculated based on the loss utility theory. Results are statistically shown in

Table 4. Loss expectation statistics for the two evaluation methods.

		(a)	(b)	(c)	(d)	(e)	(f)
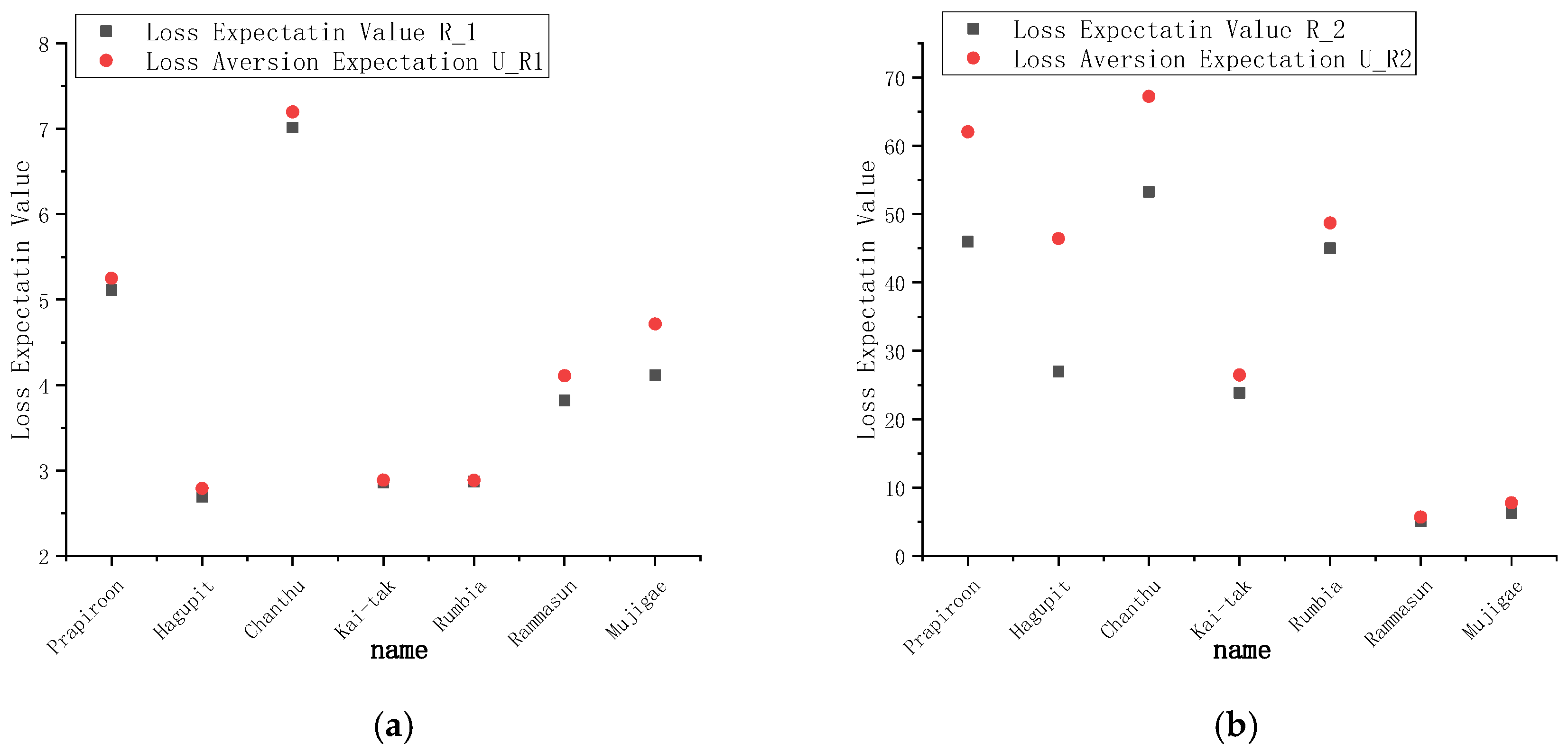
Number	Name	R1	R2	UR1	UR2	l1	l2
0606	Prapiroon	5.112	45.959	5.251	62.056	5.238	60.355
0814	Hagupit	2.693	26.993	2.791	46.434	2.788	45.477
1003	Chanthu	7.014	53.277	7.197	67.232	7.174	65.239
1213	Kai-tak	2.857	23.849	2.890	26.487	2.887	26.173
1306	Rumbia	2.873	44.986	2.886	48.724	2.883	47.671
1409	Rammasun	3.820	5.162	4.110	5.713	4.103	5.698
1522	Mujigae	4.115	6.241	4.716	7.791	4.706	7.764

. In the table, R₁, and R₂ indicate the values of loss expectation calculated based on direct economic loss and affected population indicators, respectively. U_R1 and U_R2 represent the aversion of expectation values of absolute loss calculated based on the two evaluation indicators of direct economic loss and affected population; l₁ and l₂ denote the fixed losses at an equal aversion utility, respectively.

Comparing the calculation results of columns (a)–(d) in Table 4, it can be found that the absolute loss value considering the loss utility is slightly higher than the expected loss value. Taking Typhoon 1003 ‘Chandu’ as an example, the average population affected by this typhoon is 53.277 (in the unit of 10,000 people). The average direct economic loss is 7.014 (in the unit of 100 million dollars). The fixed affected population calculated by the utility function theory is 65.239 (in the unit of 10,000 people) and 7.174 (in the unit of 100 million dollars). Further calculation shows that the calculation results of the two indicators based on the loss expectation aversion model are 22.45% and 2.29% higher than the original model. It indicates that the loss expectation model with the introduction of the loss utility function reflects the avoidance of high risk by the social groups.

The scatter plot of Figure 7 also intuitively shows that the absolute loss assessment considering the loss utility is higher than the loss expectation calculated by the quantity-based method. In addition, it can be seen from Figure 7a that the higher the wind volume in the typhoon center, the greater the loss expectation difference calculated by the two methods. This shows that with the increase in risk, the degree of dissatisfaction and disgust of social groups will also increase. However, there is an anomaly in the figure (Typhoon Hagupit number 0814), which may be due to the difference between the typhoon landing area in urban or rural areas, day or night.In addition, for Figure 7b, from 2006 to 2015, the difference in loss expectation calculated by the two methods becomes gradually smaller over time. This indicates, to some extent, that with the progress of societal awareness, social groups have improved their ability to avoid, prevent and respond to high typhoon risks and so on.

3.1.3. Ranking of Typhoon Risks

In order to achieve comparability of risk magnitude of typhoon disaster occurrence among different regions, relative loss aversion indicators will be introduced by model calculation in the following to realize the transformation of random loss to fixed loss and develop a unified typhoon risk metric to facilitate subsequent risk classification. First, it is necessary to introduce the relative loss of direct economic loss and affected population, respectively, expressed by the direct economic loss rate L₁′ and the proportion of the affected population L₂′, where L₁′ = L₁/GrossV₁, and L₂′ = L₂/GrossV₂. The relative loss aversion effect formula as well as the relative loss aversion conversion formula are then calculated and the results are presented in

Table 5. Table of expected relative loss aversions and fixed relative losses with equal aversions for seven typhoons.

		(a)	(b)	(c)	(d)	(e)	(f)
Name	Level	L1′ (%)	L2′ (%)	UR1′	UR2′	l1′ (%)	l2′ (%)
Prapiroon	12	0.2183	5.5250	0.0192	0.4872	0.0193	0.5117
Hagupit	15	0.2109	7.8628	0.0073	0.2732	0.0073	0.2947
Chanthu	12	0.1221	4.0887	0.0152	0.5105	0.0153	0.5285
Kai-tak	13	0.0449	2.0172	0.0050	0.2252	0.0050	0.2290
Rumbia	11	0.0170	1.5596	0.0046	0.4227	0.0046	0.4273
Rammasun	15	0.2262	1.9330	0.0056	0.0481	0.0056	0.0490
Mujigae	15	0.3718	3.7847	0.0057	0.0576	0.0057	0.0597

. UR1′ and UR2′ represent the aversion utility values of relative loss calculated based on the two evaluation indicators of direct economic loss and affected population, respectively. The l1′ and l2′ are their respective fixed relative losses corresponding to the same degree of aversion.

From the data in column (a) of Table 5, the highest rate of direct economic loss among the seven typhoons is Mujigae with 0.3718%, and the lowest is Rumbia with 0.0170%. From the data in column (b) of Table 5: the highest percentage of the affected population is Hagupit with 7.8628%, and the lowest is Rumbia with 1.5596%. From the data in columns (c) to (f), the two typhoons with higher indicator values are Chanthu and Prapiroon, both of which have a wind force of 12. In terms of absolute random losses, the L₁ and L₂ caused by these two typhoons are similar, but in terms of typhoon occurrence probability, the occurrence probability of Chanthu is slightly bigger than that of Prapiroon (from the extreme value data, the wave height and wind speed data of Chanthu after the landing of Naozhou region are slightly higher than those of Prapiroon). Therefore, the loss expectation aversion model calculates that the relative loss aversion value and fixed relative loss of Chanthu are relatively high. The relative loss aversion values of these two typhoons are also much higher than those of the subsequent high-ranking typhoons, which may be related to the increased awareness of disaster prevention, the improved ability to avoid high-risk events, and the improved accuracy of typhoon warnings and forecasting systems.

In typhoon risk assessment, we can use the relative damage rate caused by typhoon hazards L′ and the probability F of its occurrence to classify the levels. As can be seen from Table 5, the highest direct economic loss of typhoons is 0.3718% and the lowest is 0.017%, which is on the interval of [0,0.5%]. The highest proportion of the affected population is 7.8628% and the lowest is 1.5596%, which is on the interval of [0,10%]. The direct economic loss rate of typhoon disaster solved under the relative loss aversion sense l1′ is on the interval of [0,0.02%], and the percentage of losses of the affected population l₂′ is on the interval [0,0.6%]. Therefore, we can divide the l₁′ and l₂′ intervals into several sections to represent different risk levels, and establish a typhoon risk level classification method according to their values. As shown in Figure 8 and Figure 9:

Taking Figure 8 as an example, the horizontal and vertical values of each point distance in the figure are derived from the typhoon occurrence probability F in Table 3 and the typhoon direct economic loss rate L₁′ in Table 5. Then, the fixed direct economic loss rate l₁′ of typhoon disasters solved in the sense of relative loss aversion is divided into five segments in the range of [0,0.02%]: [0,0.004%,0.008%,0.012%,0.016%,0.02%]. According to the conversion of the degree of equal disgust, the above l₁′ values (l₁′ takes 0.004%, 0.008%, 0.012%, 0.016%, 0.02% ) are brought into the formula (8), that is, U (l₁′) = μ (L₁′) (j = 1 in formula 8, that is, each typhoon disaster is calculated separately), and each l₁′ is worth a set of (F, L₁′) values. The values of each group (F, L₁′) are traced, and the curves in the graph are obtained. Each curve is an isoline of expected loss aversion. Finally, according to the expected loss aversion value of each typhoon disaster in different aversion intervals, the risk level is divided. Figure 9 is the same.

In Figure 8 and Figure 9, the dotted line is the coordinate grid line, and the risk level of the typhoon area is represented by numbers. The greater the number value, the greater the risk, and the maximum level is 6. It can be seen from Figure 8 that when the typhoon risk classification in Naozhou area is based on the direct economic loss rate, Typhoon Hagupit No. 0814, Typhoon Kai-tak No. 1213, Typhoon Rumbia No. 1306, Typhoon Rammasun No.1409 and Typhoon Mujigae No. 1522 are all Level 2 risks, Typhoon Chanthu No. 1003 is Level 4 risk, and Typhoon Prapiroon No. 0606 is Level 5 risk. According to Figure 9, based on the proportion of typhoon-affected population, the typhoon risk level is divided. Both Rammasun and Mujigae have only a level 1 risk, Kai-tak is a level 2 risk, Hagupit is a level 3 risk, Rumbia is a level 4 risk, and Prapiroon and Chanthu are both a level 5 risk.

Through the comparative analysis between the two figures, it is found that the remaining typhoons have a risk rating difference of no more than one grade based on two assessment indicators, except for typhoon No. 1306 Rumbia. The reasons for this result may be related to the time (day or night) and location (rural or urban) of the typhoon.

Table 6. Classification of risk levels for 7 typhoons.

Number	Name	Classification
		L1′	L2′	(L1′+ L2′)/2
0606	Prapiroon	Level 5	Level 5	Level 5
0814	Hagupit	Level 2	Level 3	Level 3
1003	Chanthu	Level 4	Level 5	Level 5
1213	Kai-tak	Level 2	Level 2	Level 2
1306	Rumbia	Level 2	Level 4	Level 3
1409	Rammasun	Level 2	Level 1	Level 2
1522	Mujigae	Level 2	Level 1	Level 2

provides the combined risk level for seven typhoons. The combined risk level is calculated in terms of (L₁′ + L₂′)/2. If (L₁′ + L₂′)/2 is not an integer, it is rounded off.

For the typhoons that landed in the study area from 2006 to 2015, the highest comprehensive risk levels were Prapiroon (No. 0606) and Chanthu (No. 1003), with a risk level of 5. Prapiroon has a risk level of 5, both based on the direct economic loss rate and the assessment of the affected population. In addition, the typhoon comprehensive risk level before 2011 was ≥3, and the typhoon comprehensive risk level from 2012 to 2015 was ≤3.

3.2. Discussion

The results of the classical quantitative algorithm and the model presented in this paper are compared in the following sections. First, partition the risk events. In this paper, a typhoon with a probability higher than 0.09576 is regarded as a typhoon with a higher probability of occurrence. High loss typhoon refers to a typhoon whose loss is higher than the average level (3.82%). As can be seen from the results of Figure 10a, it can be seen that the probability of typhoon Hagupit and Prapiroon not only is lower than 0.09576, but also the loss is lower than the average level of 3.82%, which belongs to the high loss-low probability type (hereinafter referred to as H_L-L_P type). Typhoon Kai-tak and Rumbia, on the contrary, belong to low loss and high probability (L_L-H_P) risk events. According to the classification results in Figure 10b, Hagupit, Prapiroon, Rammasun and Mujigae belong to the H_L-L_P type of risk events. Chanthu, Kai-tak and Rumbia are L_L-H_P risk events. According to the classification results of the two charts, typhoon disasters of H_L-L_P type are Hagupit and Prapiroon. The L_L-H_P typhoons are Kai-tak and Rumbia.

The calculation results of each typhoon disaster based on the two evaluation models are summarized in

Table 7. Comparison of calculation results of two loss expectation models.

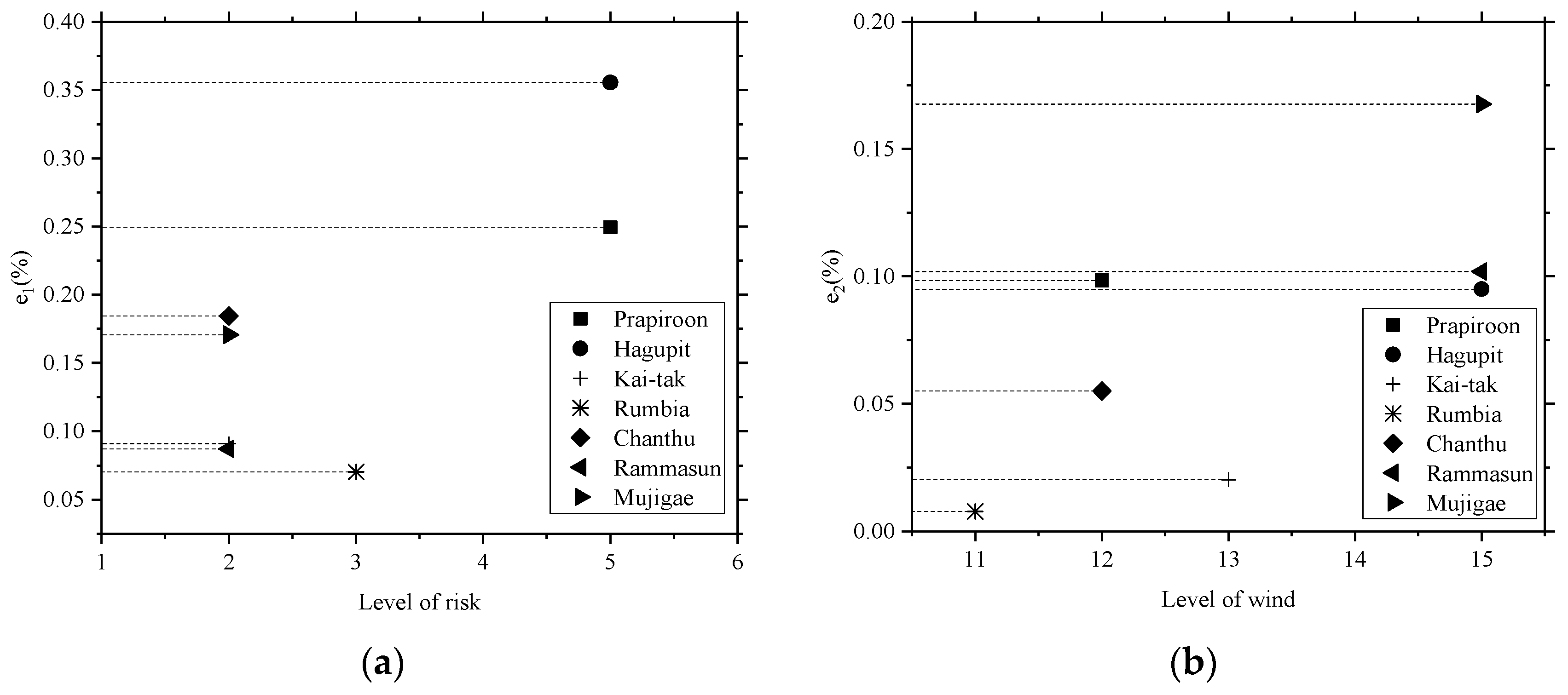
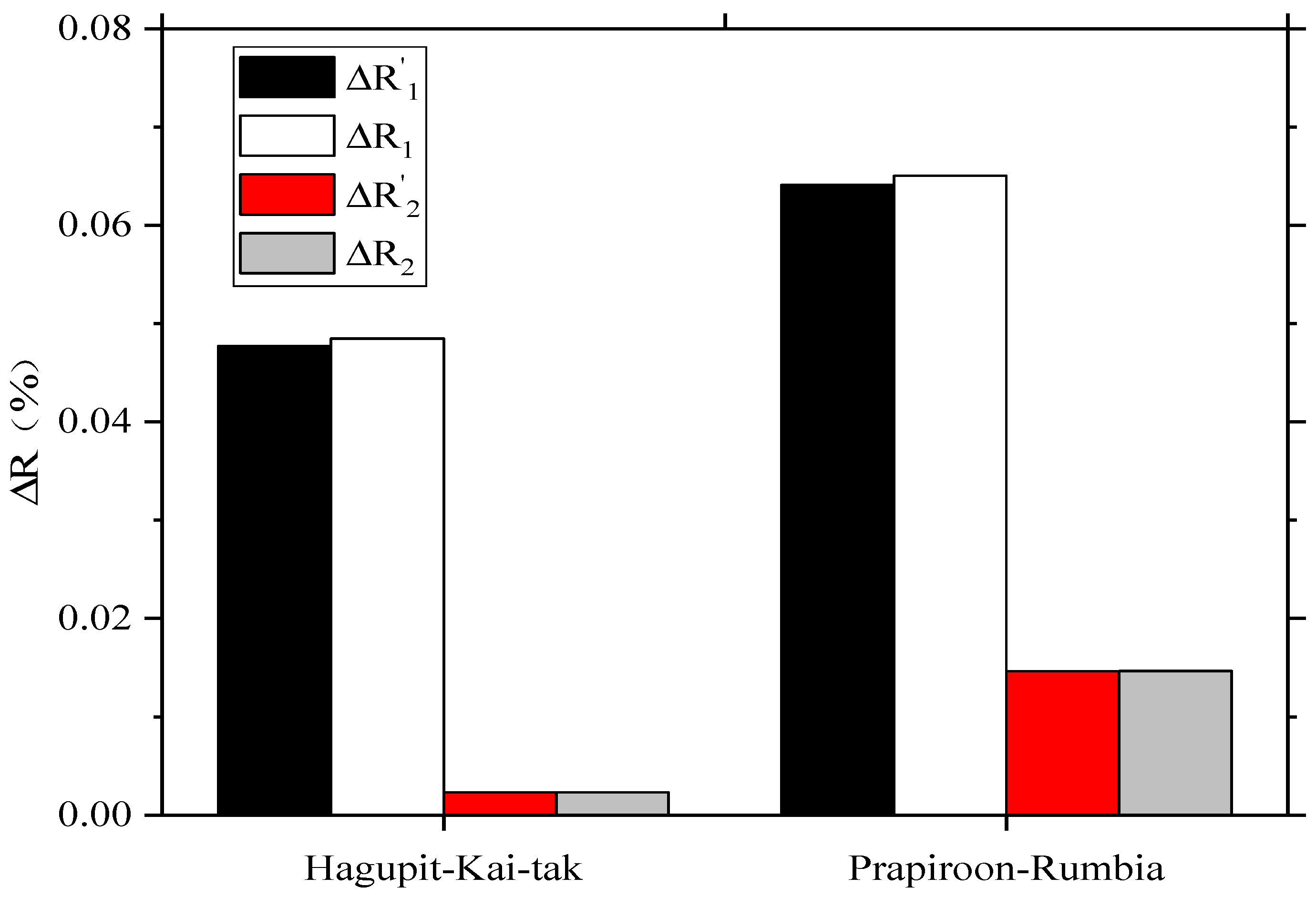
		(a)	(b)	(c)	(d)	(e)	(f)	Rank of Risk	Rank of Wind
	Name	R′1 (%)	R1 (%)	e1 (%)	R′2 (%)	R2 (%)	e2 (%)
HL-LP	Prapiroon	0.4868	0.4880	0.2495	0.019232	0.019234	0.0984	5	15
	Hagupit	0.2728	0.2738	0.3555	0.007318	0.007319	0.0950	5	12
LL-HP	Kai-tak	0.2251	0.2253	0.0909	0.005011	0.005011	0.0202	2	12
	Rumbia	0.4227	0.4229	0.0702	0.004607	0.004607	0.0077	3	15
	Chanthu	0.5103	0.5112	0.1844	0.015238	0.015239	0.0550	2	13
	Rammasun	0.0481	0.0482	0.0871	0.005632	0.005633	0.1019	2	15
	Mujigae	0.0575	0.0576	0.1707	0.005651	0.005652	0.1677	2	11

. In Table 7, (a) and (d) are listed as expected loss rates based on the quantity algorithm (R′₁ = P × L₁, R′₂ = P × L₂), and (b) and (e) are listed as expected loss rates considering utility. In addition, e₁ and e₂ represent the ratio of the difference between the loss expectation of considering utility and the loss expectation of classical quantitative algorithms (e₁ = (R₁ − P × L₁)/P × L₁, e₂ = (R₂ − P × L₂)/P × L₂). The subscript of the variable based on the disaster population rate is 1, and the subscript based on the direct economic loss rate is 2.

As can be seen from Table 7, R_i (I = 1,2) is slightly higher than R′_i (I = 1,2), mainly because the classical loss expectation formula does not consider the risk attitude (i.e., the case of utility coefficient α = 0), and the loss utility function reflects the aversion and dissatisfaction of the disaster-stricken groups to typhoon disasters. Further comparative analysis found that this high situation is not linear, but has a certain relationship with the risk level and typhoon wind speed level. As can be seen from Figure 11, the interpolation ratio e₁, which takes the affected population rate as an indicator, increases roughly with the increase in the typhoon’s comprehensive risk level. The interpolation ratio e₂, which takes the direct economic loss rate as the index, basically increases with the increase in typhoon wind speed level. In addition, e₁ > e₂ indicates to a certain extent that the risk assessment model newly constructed in this paper can better reflect the disaster-affected people’s dissatisfaction with typhoon disaster in the risk assessment model by taking the disaster-affected population as the index.

The differences between the two assessment methods in calculating the risk expectations of “H_L-L_P” and “L_L-H_P” typhoons are as follows. Taking the calculation results of the disaster population rate index in Table 7 as an example, the calculation results of Hagupit and Prapiroon of the “H_L-L_P” type are similar to those of Kai-tak and Rumbia of the “L_L-H_P” type, respectively (see Figure 12). The $\Delta R_1$ and $\Delta R_1^{\prime }$ between Hagupit of the “H_L-L_P” type and Kai-tak of the “L_L-H_P” type are both less than 0.05%, and $\Delta R_2$ and $\Delta R_2^{\prime }$ are both less than 0.01%. The $\Delta R_1$ and $\Delta R_1^{\prime }$ between Prapiroon of the “H_L-L_P” type and Rumbia of the “L_L-H_P” type are both less than 0.08% and $\Delta R_2$ and $\Delta R_2^{\prime }$ are both less than 0.02%.

It is difficult to distinguish the grades of risk based on loss expectations. By using the loss utility function to delineate the typhoon risk levels, the risk levels of Hagupit, Prapiroon, Kai-tak and Rumbia can be determined as levels 5, 5, 2 and 3, which changes the situation that it is difficult to distinguish the two types of typhoon risks of “H_L-L_P” and “L_L-H_P” in traditional calculation.

The typhoon disaster risk assessment model based on the theory of loss utility function can overcome the disadvantages of the traditional loss expectation model based on the quantitative algorithm, which cannot distinguish the risk of high loss, low probability disaster and low loss, high probability disaster. The quantitative measurement and classification of typhoon disaster risk can be carried out through the change of social group’s subjective willingness to disaster risk, which can improve the scientificity and rationality of typhoon disaster risk assessment to a certain extent.

4. Conclusions

Under the background of relatively little research on the quantitative assessment of typhoon disaster risk, this paper introduces utility theory and puts forward a quantitative assessment method of risk, which is scientific and reasonable to some extent. The application of utility theory in this field is a relatively new attempt. From the quantitative evaluation results of the case analysis, the expected loss based on the loss utility function is slightly higher than that of the quantitative algorithm. In addition, the classification map based on the logarithmic loss-utility function also provides a new perspective for risk level assessment. Overall, the quantitative assessment method proposed in this paper has certain feasibility and practicability, and is expected to provide a meaningful reference for typhoon risk assessment and risk decision-making.

In the risk analysis of typhoon disasters, this paper discusses the measurement and classification of typhoon disaster risk when direct economic loss and affected population are taken as loss indicators. Future research may consider collecting more detailed typhoon disaster loss data and conducting typhoon disaster risk assessment from multiple indicators such as house collapse, affected population, casualties and affected area of farmland, so as to obtain more comprehensive and comprehensive assessment results.