Depth-Resolved Modelling of Intra-Swash Morphodynamics Induced by Solitary Waves
Abstract
:1. Introduction
2. Methodology
2.1. Model Description
2.1.1. Numerical Model
2.1.2. Initial and Boundary Conditions
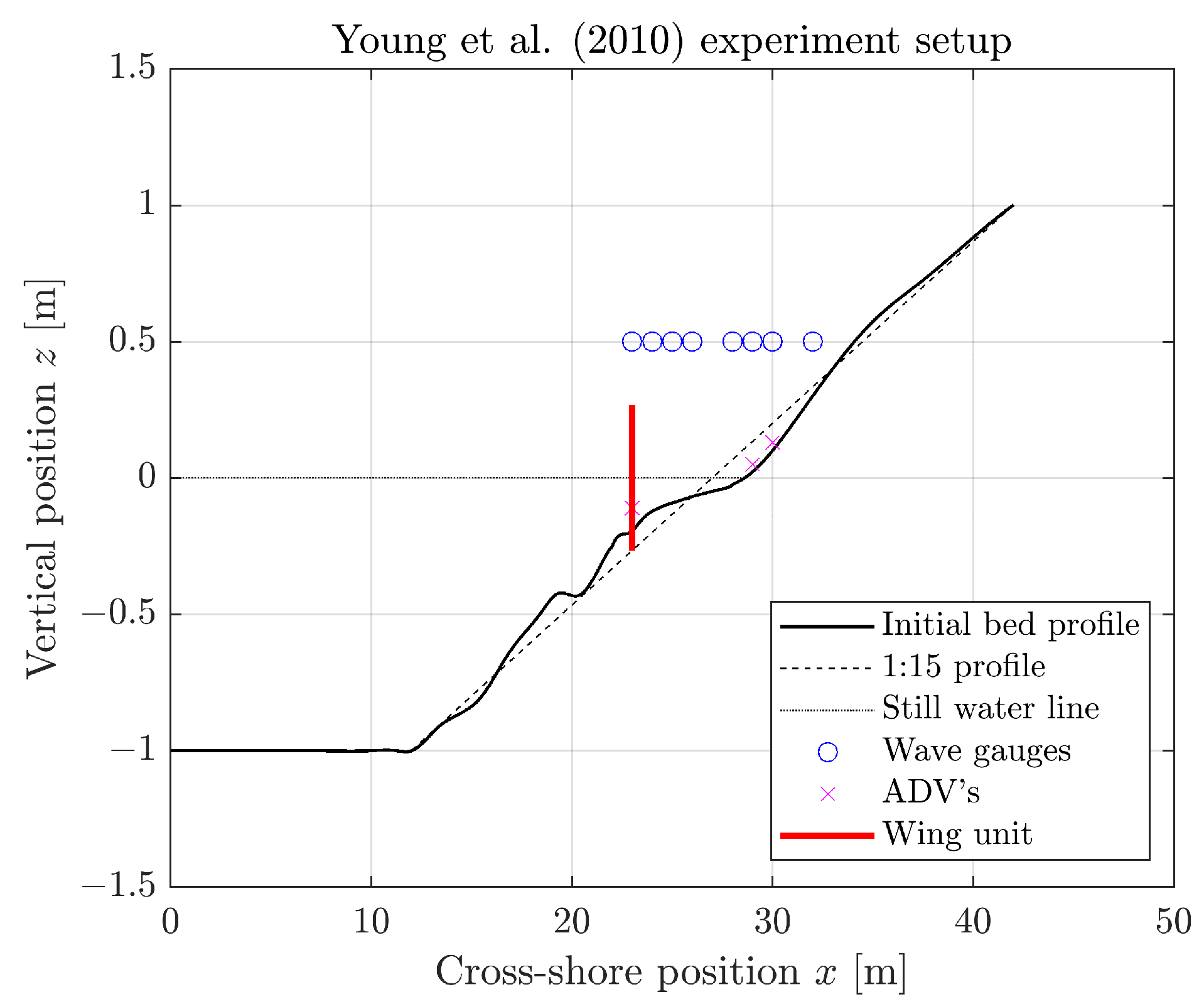
2.1.3. Geometry and Mesh
2.2. Comparison with Measurements
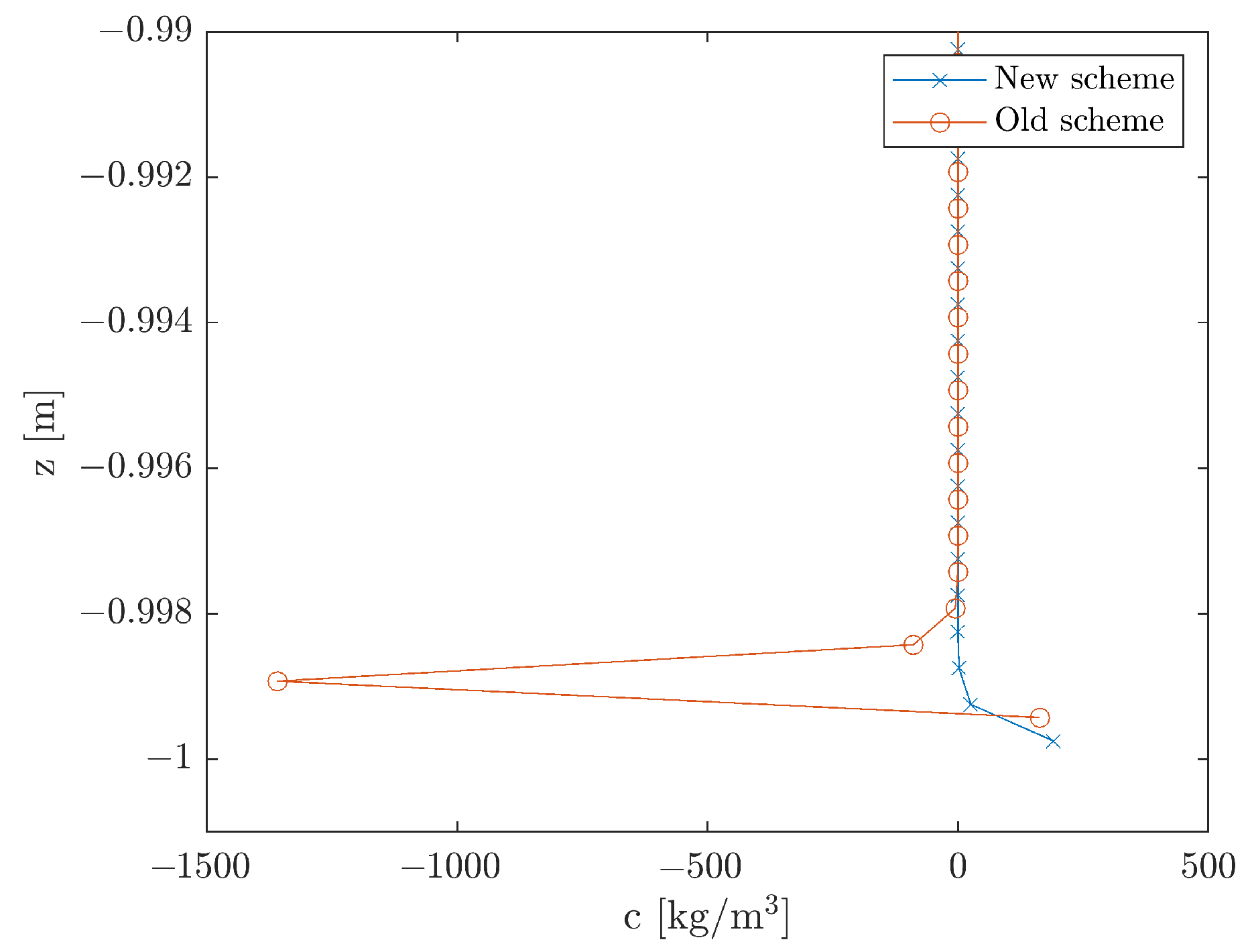
3. New Boundary Divergence Scheme
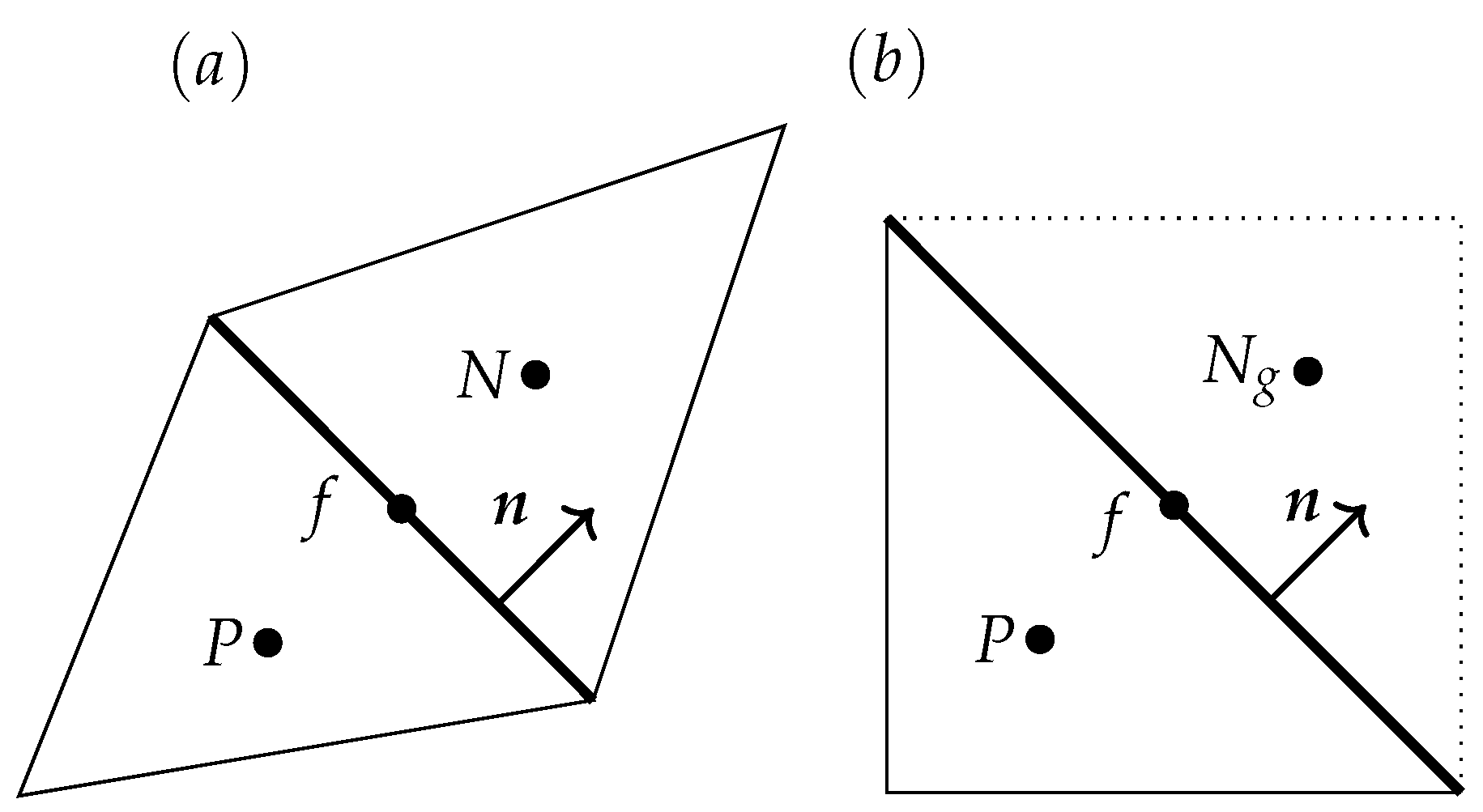
3.1. Discretisation of Divergence Operator
3.2. Treatment of Boundary Faces
3.3. 1D Example
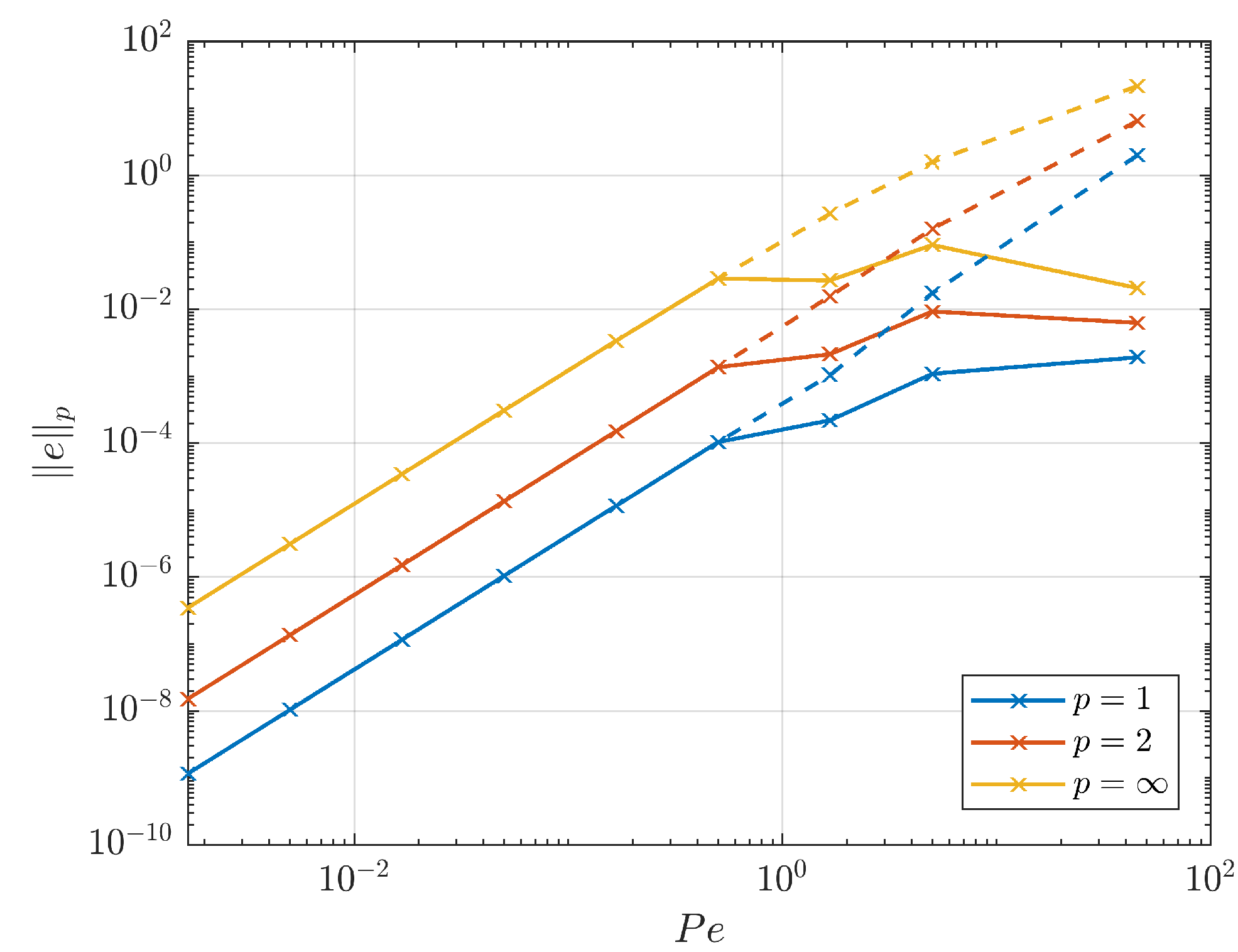
3.4. 2D Validation
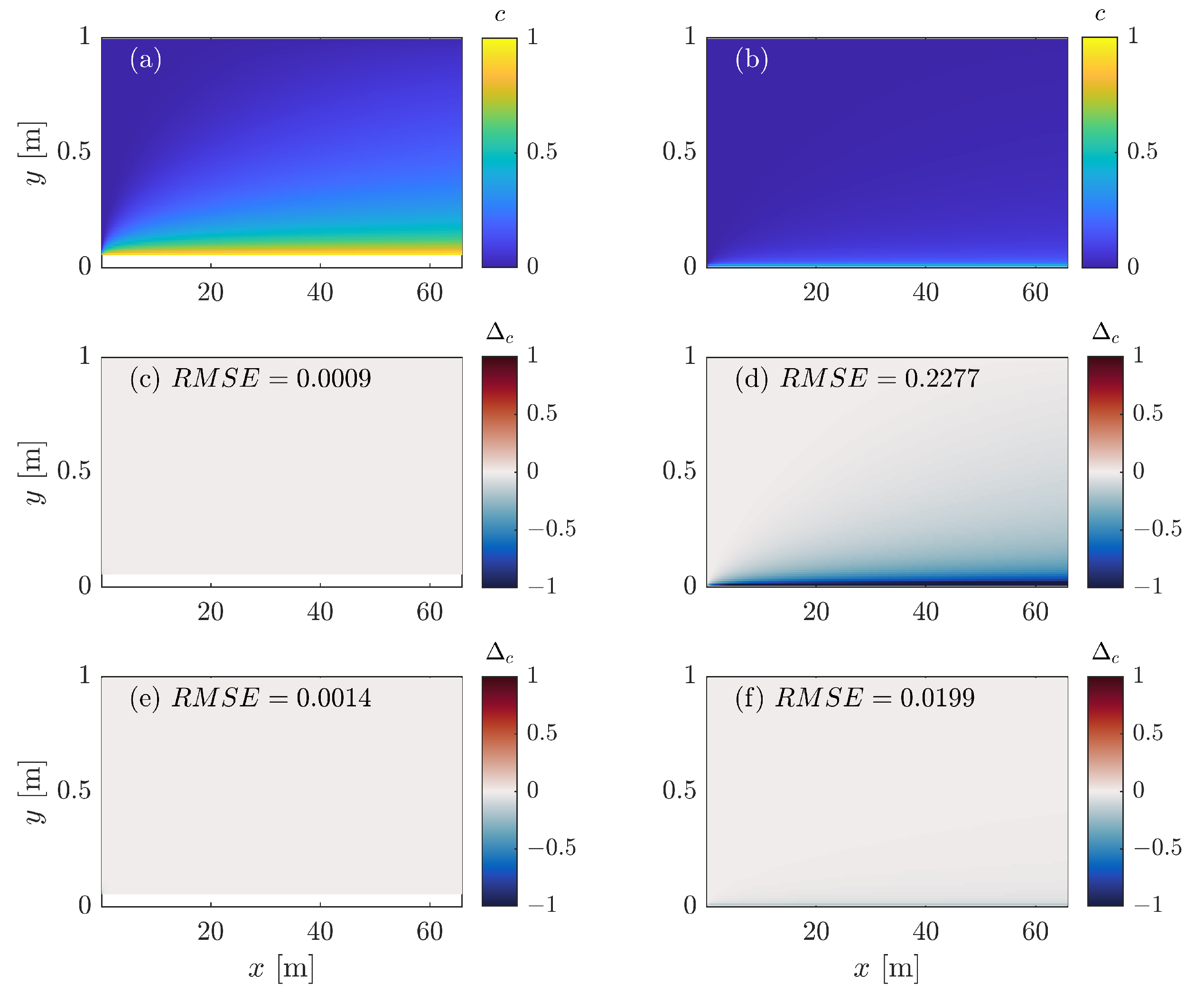
3.5. Application to the Morphodynamic Model
4. Model Performance
4.1. Hydrodynamics
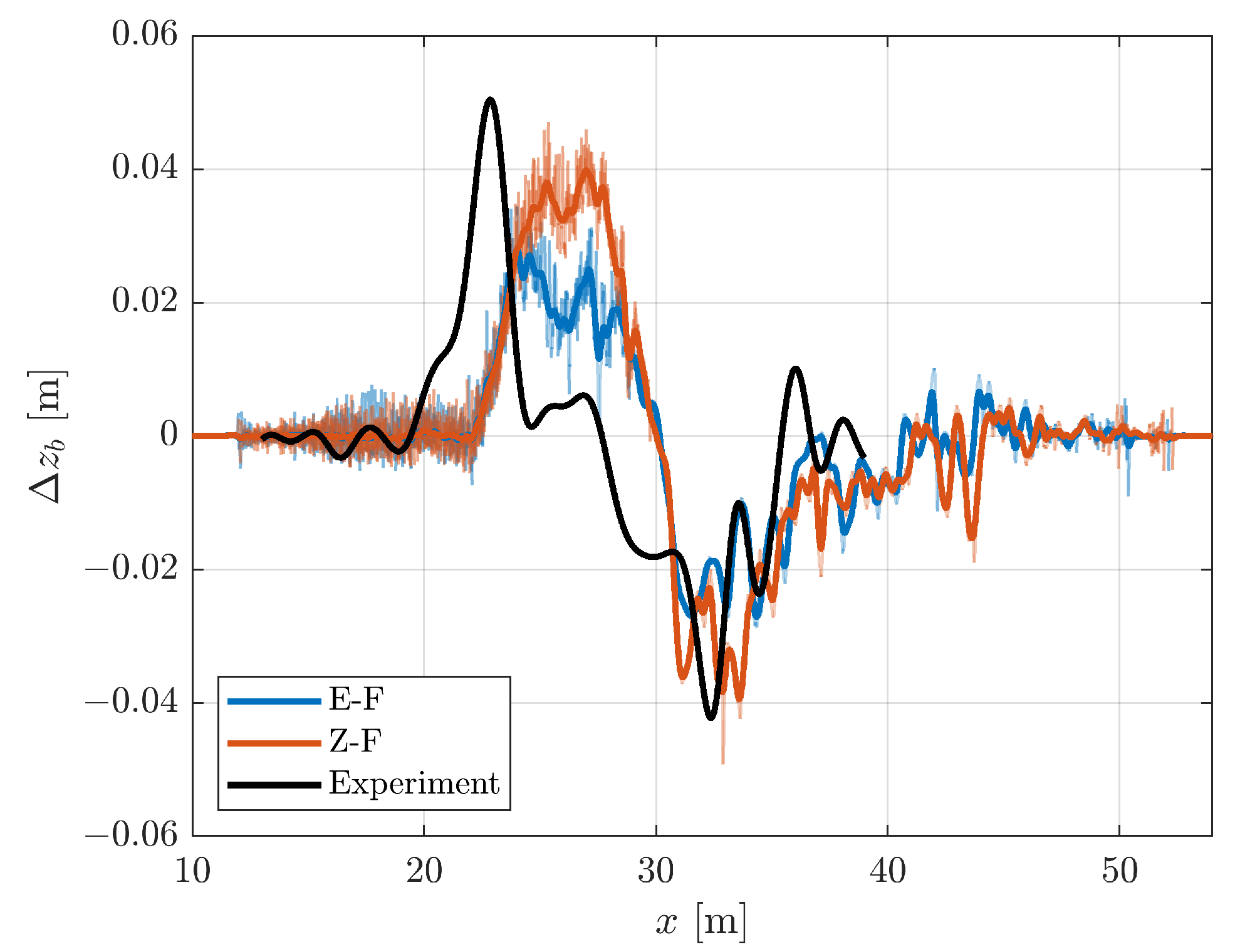
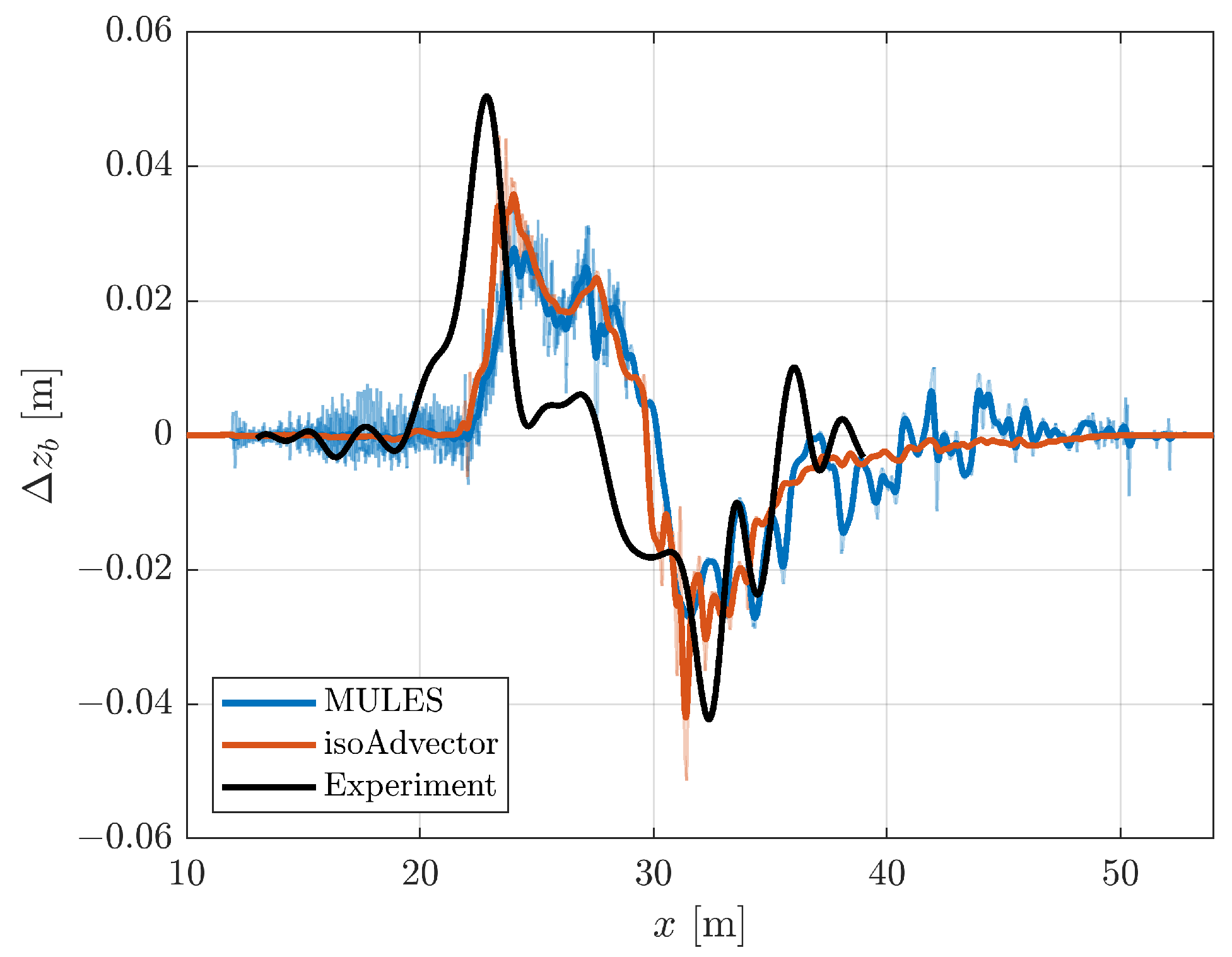
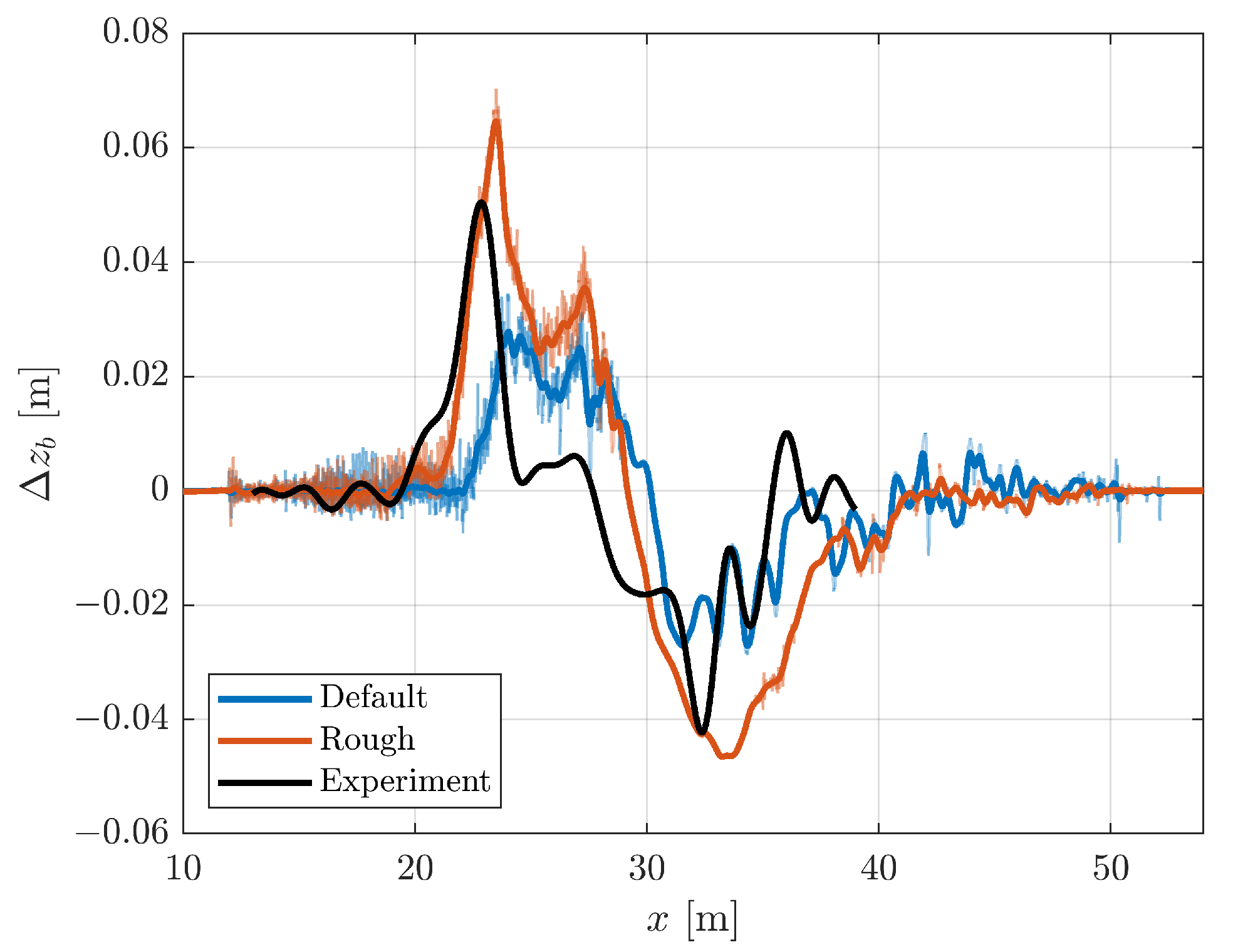
4.2. Suspended Sediment and Morphodynamics
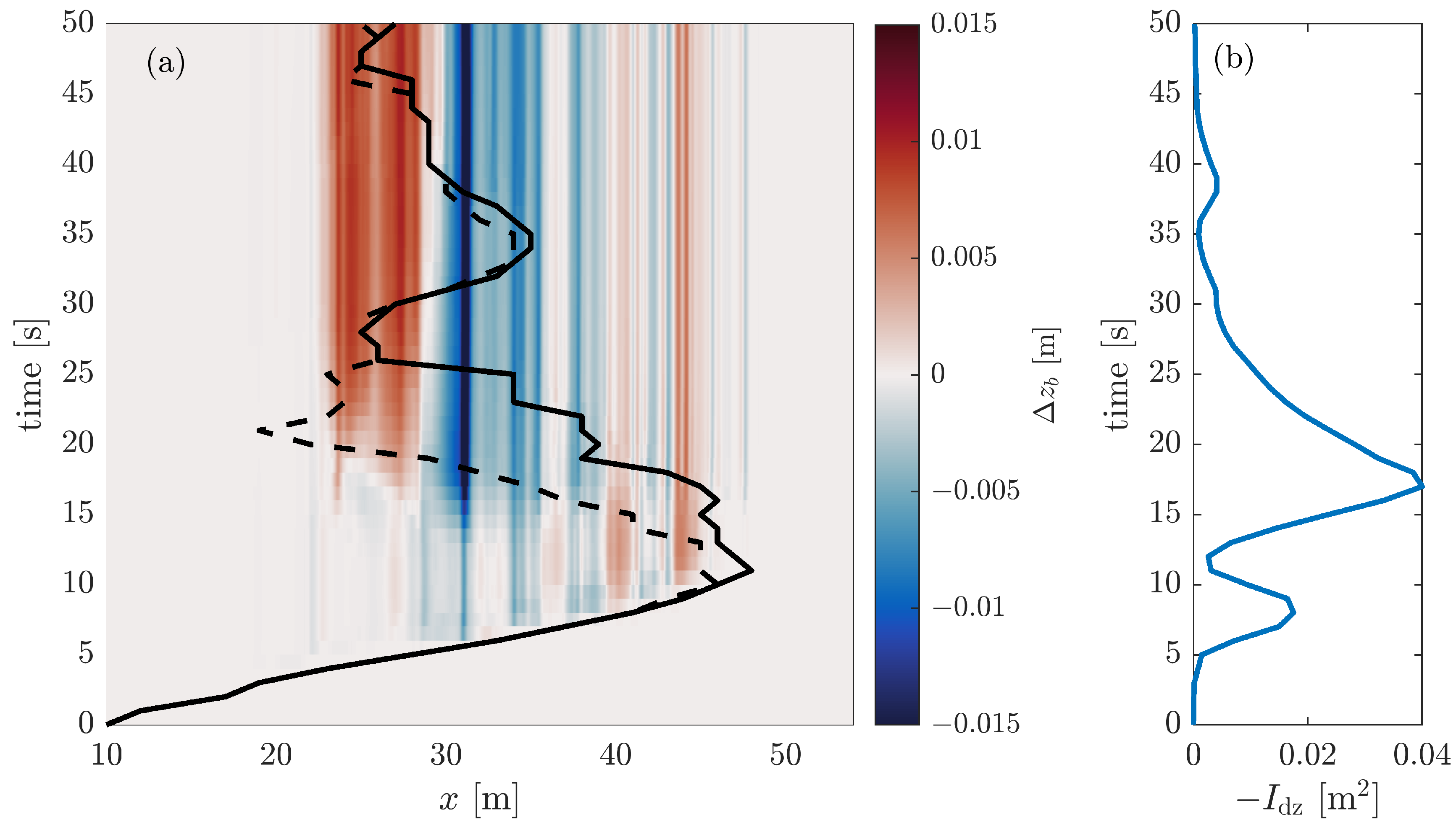
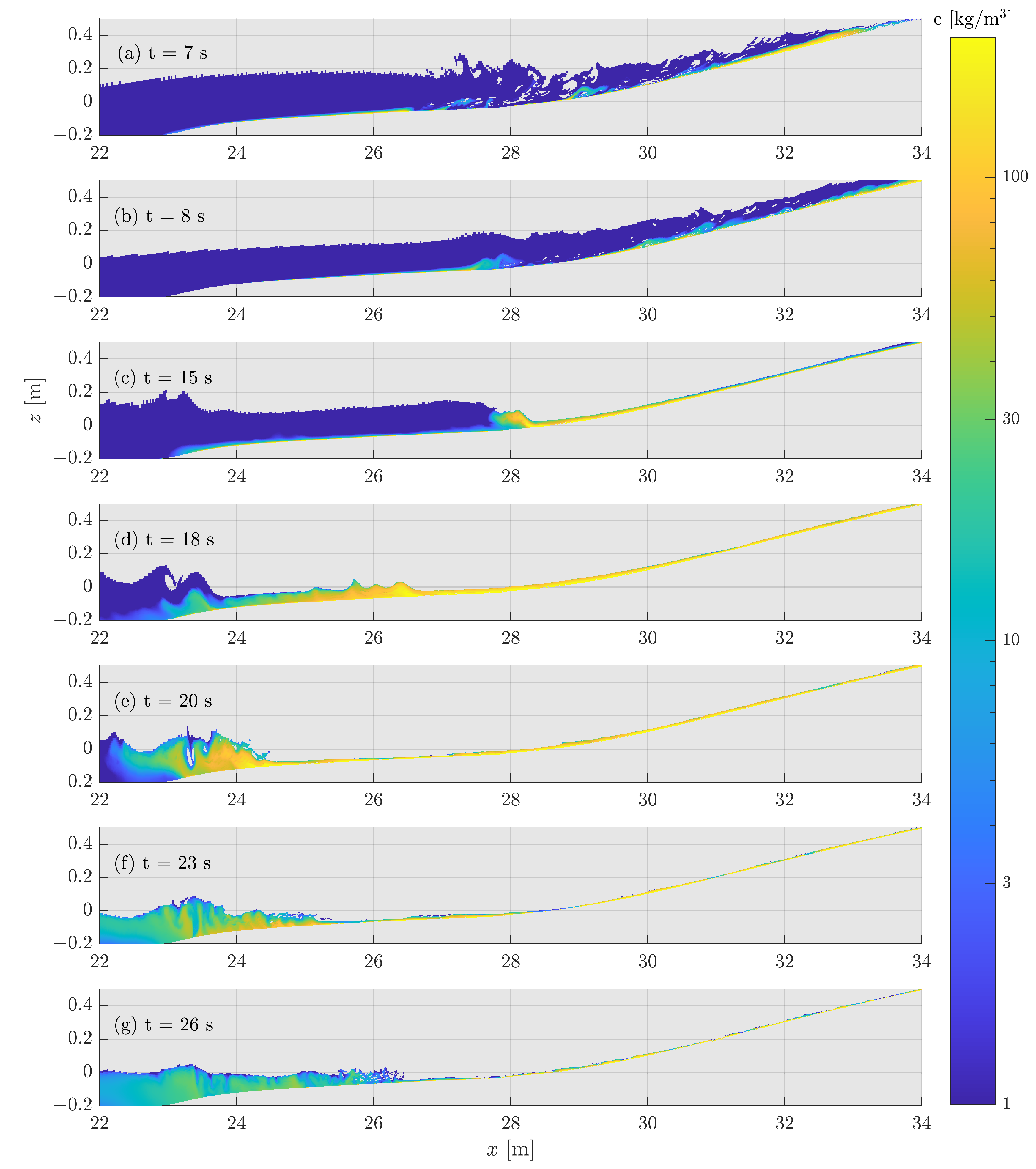
5. Intra-Swash Sediment Dynamics
6. Discussion
6.1. VoF and Reference Concentration Models
6.2. Runup and Location of Deposition
6.3. Sediment Transport and Morphodynamics
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Model Equations
References
- Blenkinsopp, C.E.; Turner, I.L.; Masselink, G.; Russell, P.E. Swash zone sediment fluxes: Field observations. Coast. Eng. 2011, 58, 28–44. [Google Scholar] [CrossRef]
- van der Zanden, J.; Alsina, J.M.; Cáceres, I.; Buijsrogge, R.H.; Ribberink, J.S. Bed level motions and sheet flow processes in the swash zone: Observations with a new conductivity-based concentration measuring technique (CCM+). Coast. Eng. 2015, 105, 47–65. [Google Scholar] [CrossRef] [Green Version]
- Masselink, G.; Evans, D.; Hughes, M.G.; Russell, P. Suspended sediment transport in the swash zone of a dissipative beach. Mar. Geol. 2005, 216, 169–189. [Google Scholar] [CrossRef]
- Puleo, J.A.; Torres-Freyermuth, A. The second international workshop on swash-zone processes. Coast. Eng. 2016, 115, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Zhu, F.; Dodd, N. The morphodynamics of a swash event on an erodible beach. J. Fluid Mech. 2015, 762, 1–29. [Google Scholar] [CrossRef] [Green Version]
- McCall, R.T.; Masselink, G.; Poate, T.G.; Roelvink, J.A.; Almeida, L.P. Modelling the morphodynamics of gravel beaches during storms with XBeach-G. Coast. Eng. 2015, 103, 52–66. [Google Scholar] [CrossRef]
- Incelli, G.; Dodd, N.; Blenkinsopp, C.E.; Zhu, F.; Briganti, R. Morphodynamical modelling of field-scale swash events. Coast. Eng. 2016, 115, 42–57. [Google Scholar] [CrossRef] [Green Version]
- Briganti, R.; Dodd, N.; Incelli, G.; Kikkert, G. Numerical modelling of the flow and bed evolution of a single bore-driven swash event on a coarse sand beach. Coast. Eng. 2018, 142, 62–76. [Google Scholar] [CrossRef]
- Ruffini, G.; Briganti, R.; Alsina, J.M.; Brocchini, M.; Dodd, N.; McCall, R. Numerical Modeling of Flow and Bed Evolution of Bichromatic Wave Groups on an Intermediate Beach Using Nonhydrostatic XBeach. J. Waterw. Port Coast. Ocean Eng. 2020, 146, 04019034. [Google Scholar] [CrossRef]
- Mancini, G.; Briganti, R.; McCall, R.; Dodd, N.; Zhu, F. Numerical modelling of intra-wave sediment transport on sandy beaches using a non-hydrostatic, wave-resolving model. Ocean Dyn. 2021, 71, 1–20. [Google Scholar] [CrossRef]
- Reniers, A.J.H.M.; Gallagher, E.L.; MacMahan, J.H.; Brown, J.A.; van Rooijen, A.A.; van Thiel de Vries, J.S.M.; van Prooijen, B.C. Observations and modeling of steep-beach grain-size variability. J. Geophys. Res. Ocean. 2013, 118, 577–591. [Google Scholar] [CrossRef] [Green Version]
- Torres-Freyermuth, A.; Puleo, J.A.; Pokrajac, D. Modeling swash-zone hydrodynamics and shear stresses on planar slopes using Reynolds-Averaged Navier-Stokes equations. J. Geophys. Res. Ocean. 2013, 118, 1019–1033. [Google Scholar] [CrossRef] [Green Version]
- Higuera, P.; Liu, P.L.; Lin, C.; Wong, W.Y.; Kao, M.J. Laboratory-scale swash flows generated by a non-breaking solitary wave on a steep slope. J. Fluid Mech. 2018, 847, 186–227. [Google Scholar] [CrossRef]
- Mory, M.; Abadie, S.; Mauriet, S.; Lubin, P. Run-up flow of a collapsing bore over a beach. Eur. J. Mech. B/Fluids 2011, 30, 565–576. [Google Scholar] [CrossRef]
- Pintado-Patiño, J.C.; Torres-Freyermuth, A.; Puleo, J.A.; Pokrajac, D. On the role of infiltration and exfiltration in swash zone boundary layer dynamics. J. Geophys. Res. Ocean. 2015, 120, 6329–6350. [Google Scholar] [CrossRef] [Green Version]
- Desombre, J.; Morichon, D.; Mory, M. RANS v 2-f simulation of a swash event: Detailed flow structure. Coast. Eng. 2013, 71, 1–12. [Google Scholar] [CrossRef]
- Zhou, Z.; Hsu, T.J.; Cox, D.; Liu, X. Large-eddy simulation of wave-breaking induced turbulent coherent structures and suspended sediment transport on a barred beach. J. Geophys. Res. Ocean. 2017, 122, 207–235. [Google Scholar] [CrossRef]
- Kim, Y.; Zhou, Z.; Hsu, T.J.; Puleo, J.A. Large eddy simulation of dam-break-driven swash on a rough-planar beach. J. Geophys. Res. Ocean. 2017, 122, 1274–1296. [Google Scholar] [CrossRef]
- Bakhtyar, R.; Ghaheri, A.; Yeganeh-Bakhtiary, A.; Barry, D.A. Process-based model for nearshore hydrodynamics, sediment transport and morphological evolution in the surf and swash zones. Appl. Ocean Res. 2009, 31, 44–56. [Google Scholar] [CrossRef]
- Bakhtyar, R.; Brovelli, A.; Barry, D.A.; Li, L. Wave-induced water table fluctuations, sediment transport and beach profile change: Modeling and comparison with large-scale laboratory experiments. Coast. Eng. 2011, 58, 103–118. [Google Scholar] [CrossRef]
- Jacobsen, N.G.; Fredsoe, J.; Jensen, J.H. Formation and development of a breaker bar under regular waves. Part 1: Model description and hydrodynamics. Coast. Eng. 2014, 88, 182–193. [Google Scholar] [CrossRef]
- Jacobsen, N.G.; Fredsoe, J. Formation and development of a breaker bar under regular waves. Part 2: Sediment transport and morphology. Coast. Eng. 2014, 88, 55–68. [Google Scholar] [CrossRef]
- Jacobsen, N.G.; Fredsoe, J. Cross-Shore Redistribution of Nourished Sand near a Breaker Bar. J. Waterw. Port Coast. Ocean Eng. 2014, 140, 125–134. [Google Scholar] [CrossRef]
- Larsen, B.E.; Fuhrman, D.R.; Baykal, C.; Sumer, B.M. Tsunami-induced scour around monopile foundations. Coast. Eng. 2017, 129, 36–49. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Ong, M.C.; Fuhrman, D.R.; Larsen, B.E. Numerical investigation of wave-plus-current induced scour beneath two submarine pipelines in tandem. Coast. Eng. 2020, 156, 103619. [Google Scholar] [CrossRef]
- Fernandez-Mora, A.; Ribberink, J.S.; Van der Zanden, J.; Van der Werf, J.J.; Jacobsen, N.G. RANS-VOF modeling of hydrodynamics and sand transport under full-scale non-breaking and breaking waves. Coast. Eng. 2017, 1, 29. [Google Scholar] [CrossRef] [Green Version]
- Bakhtyar, R.; Barry, D.A.; Yeganeh-Bakhtiary, A.; Li, L.; Parlange, J.Y.; Sander, G.C. Numerical simulation of two-phase flow for sediment transport in the inner-surf and swash zones. Adv. Water Resour. 2010, 33, 277–290. [Google Scholar] [CrossRef]
- Li, J.; Qi, M.; Fuhrman, D.R. Numerical modeling of flow and morphology induced by a solitary wave on a sloping beach. Appl. Ocean Res. 2019, 82, 259–273. [Google Scholar] [CrossRef]
- Sumer, B.M.; Sen, M.B.; Karagali, I.; Ceren, B.; Fredsøe, J.; Sottile, M.; Zilioli, L.; Fuhrman, D.R. Flow and sediment transport induced by a plunging solitary wave. J. Geophys. Res. Ocean. 2011, 116, 1–15. [Google Scholar] [CrossRef] [Green Version]
- García-Maribona, J.; Lara, J.; Maza, M.; Losada, I. An efficient RANS numerical model for cross-shore beach processes under erosive conditions. Coast. Eng. 2021, 170, 103975. [Google Scholar] [CrossRef]
- Lara, J.L.; Ruju, A.; Losada, I.J. Reynolds averaged NavierStokes modelling of long waves induced by a transient wave group on a beach. Proc. R. Soc. Math. Phys. Eng. Sci. 2011, 467, 1215–1242. [Google Scholar] [CrossRef] [Green Version]
- Jacobsen, N.G. A Full Hydro- and Morphodynamic Description of Breaker Bar Development. Ph.D. Thesis, DTU Mechanical Engineering, Lyngby, Denmark, 2011; p. 200. [Google Scholar]
- Young, Y.L.; Xiao, H.; Maddux, T. Hydro- and morpho-dynamic modeling of breaking solitary waves over a fine sand beach. Part I: Experimental study. Mar. Geol. 2010, 269, 107–118. [Google Scholar] [CrossRef]
- Wilcox, D.C. Formulation of the k-w Turbulence Model Revisited. AIAA J. 2008, 46, 2823–2838. [Google Scholar] [CrossRef] [Green Version]
- Larsen, B.E.; Fuhrman, D.R. On the over-production of turbulence beneath surface waves in Reynolds-averaged Navier-Stokes models. J. Fluid Mech. 2018, 853, 419–460. [Google Scholar] [CrossRef] [Green Version]
- Jacobsen, N.G.; Fuhrman, D.R.; Fredsøe, J. A wave generation toolbox for the open-source CFD library: OpenFoam®. Int. J. Numer. Methods Fluids 2012, 70, 1073–1088. [Google Scholar] [CrossRef]
- Engelund, F.; Fredsoe, J. A sediment transport model for straight alluvial channels. Hydrol. Res. 1976, 7, 293–306. [Google Scholar] [CrossRef] [Green Version]
- Roulund, A.; Sumer, B.M.; Fredsøe, J.; Michelsen, J. Numerical and experimental investigation of flow and scour around a circular pile. J. Fluid Mech. 2005, 534, 351–401. [Google Scholar] [CrossRef]
- Zyserman, J.A.; Fredsøe, J. Data Analysis of Bed Concentration of Suspended Sediment. J. Hydraul. Eng. 1994, 120, 1021–1042. [Google Scholar] [CrossRef]
- Fredsøe, J.; Deigaard, R. Mechanics of Coastal Sediment Transport. In Advanced Series on Ocean Engineering; World Scientific: Singapore, 1992; Volume 3. [Google Scholar] [CrossRef]
- Jacobsen, N.G. Mass conservation in computational morphodynamics: Uniform sediment and infinite availability. Int. J. Numer. Methods Fluids 2015, 78, 233–256. [Google Scholar] [CrossRef]
- Deshpande, S.S.; Anumolu, L.; Trujillo, M.F. Evaluating the performance of the two-phase flow solver interFoam. Comput. Sci. Discov. 2012, 5, 14016. [Google Scholar] [CrossRef]
- Roenby, J.; Bredmose, H.; Jasak, H. A computational method for sharp interface advection. R. Soc. Open Sci. 2016, 3, 160405. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fuhrman, D.R.; Dixen, M.; Jacobsen, N.G. Physically-consistent wall boundary conditions for the k-turbulence model. J. Hydraul. Res. 2010, 48, 793–800. [Google Scholar] [CrossRef]
- Cebeci, T.; Chang, K.C. Calculation of incompressible rough-wall boundary-layer flows. AIAA J. 1978, 16, 740–746. [Google Scholar] [CrossRef]
- Chappelear, J.E. Shallow-water waves. J. Geophys. Res. (1896–1977) 1962, 67, 4693–4704. [Google Scholar] [CrossRef]
- Bosboom, J.; Mol, M.; Reniers, A.J.; Stive, M.J.; de Valk, C.F. Optimal sediment transport for morphodynamic model validation. Coast. Eng. 2020, 158, 103662. [Google Scholar] [CrossRef]
- Ferziger, J.H.; Perić, M. Finite Volume Methods. In Computational Methods for Fluid Dynamics; Springer: Berlin/Heidelberg, Germany, 2002; pp. 63–69. [Google Scholar] [CrossRef]
- Darwish, M.S.; Moukalled, F. TVD schemes for unstructured grids. Int. J. Heat Mass Transf. 2003, 46, 599–611. [Google Scholar] [CrossRef]
- Hjelmfelt, A.T.; Lenau, C.W. Nonequilibrium Transport of Suspended Sediment. J. Hydraul. Div. 1970, 96, 1567–1586. [Google Scholar] [CrossRef]
- Fredsøe, J.; Sumer, B.; Kozakiewicz, A.; Chua, L.H.; Deigaard, R. Effect of externally generated turbulence on wave boundary layer. Coast. Eng. 2003, 49, 155–183. [Google Scholar] [CrossRef]
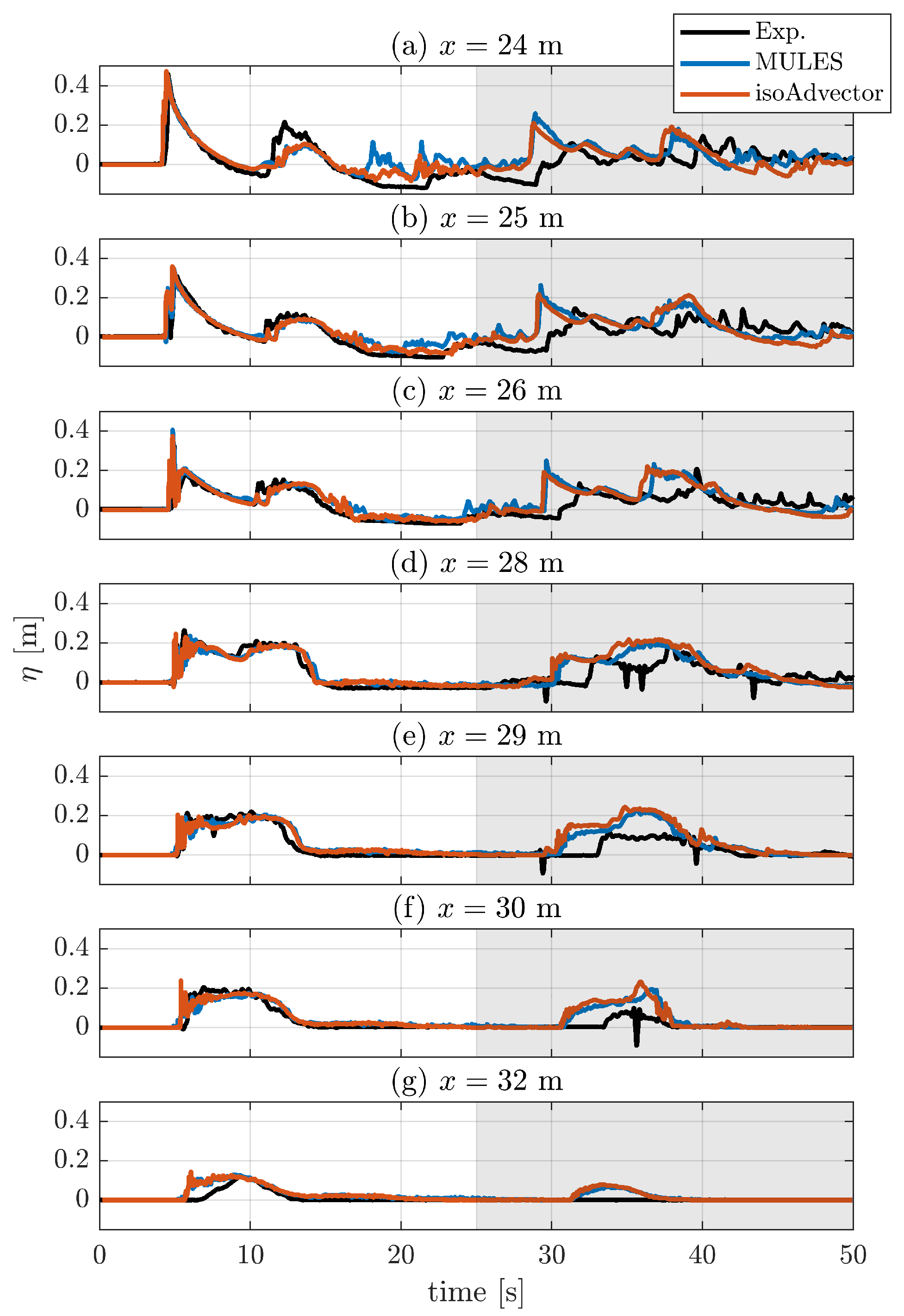
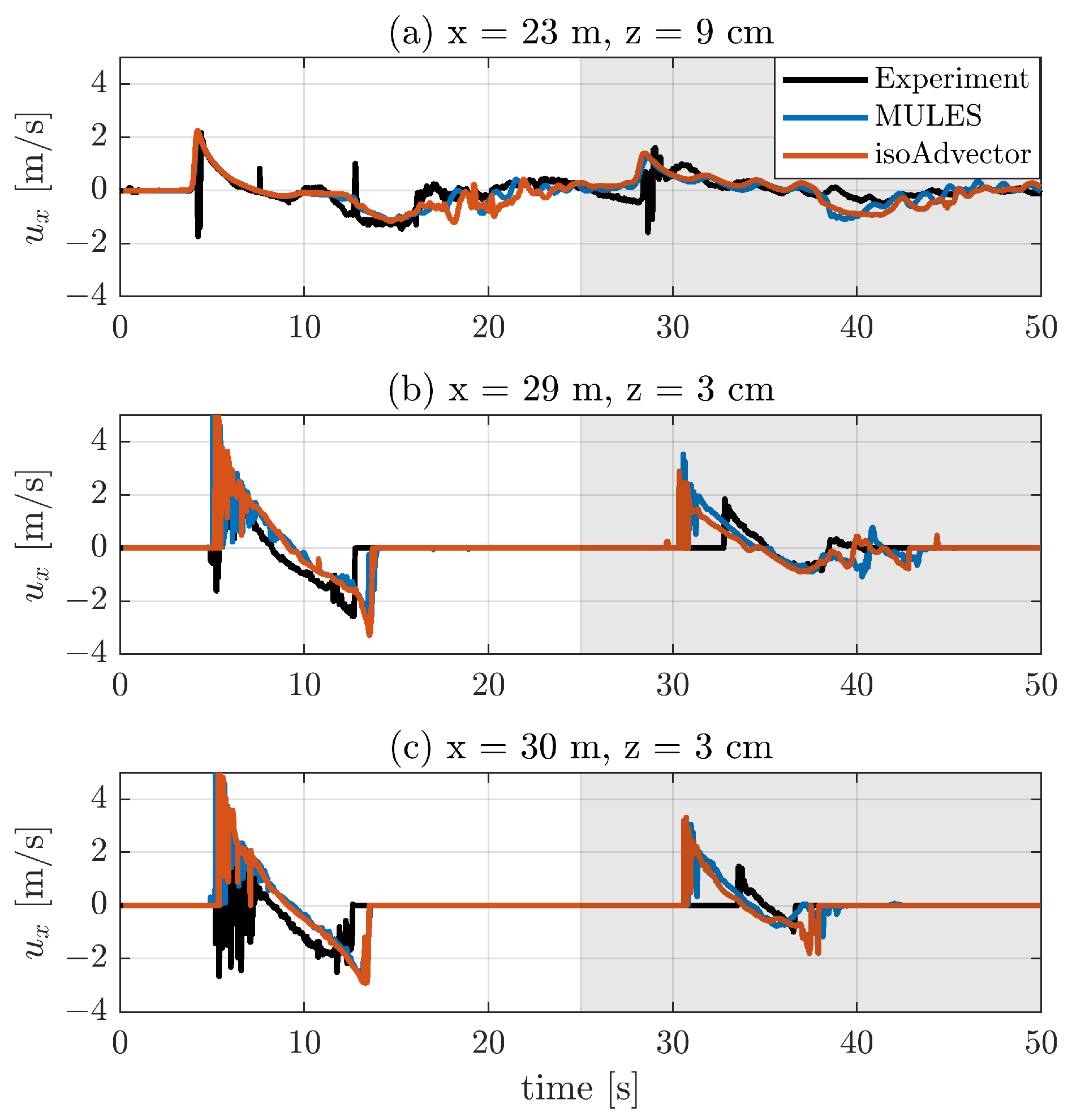
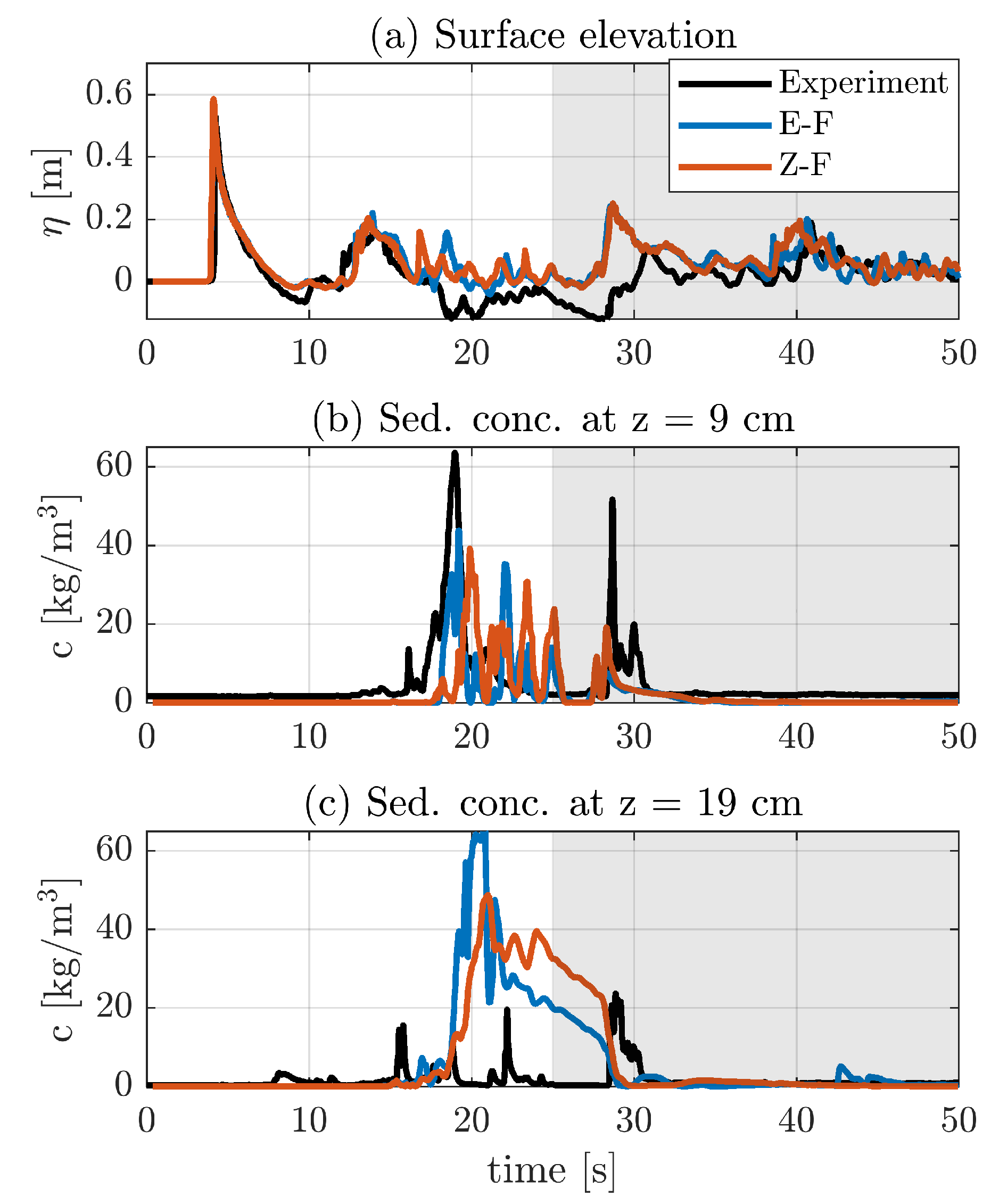

| Surface Elevation | Velocity | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| x [m] | 24 | 25 | 26 | 28 | 29 | 30 | 32 | 23 | 29 | 30 |
| MULES | 0.168 | 0.185 | 0.165 | 0.174 | 0.203 | 0.191 | 0.207 | 0.168 | 0.247 | 0.215 |
| MULES-25 | 0.152 | 0.124 | 0.106 | 0.102 | 0.126 | 0.114 | 0.222 | 0.151 | 0.280 | 0.264 |
| isoAdvector | 0.150 | 0.189 | 0.169 | 0.198 | 0.251 | 0.216 | 0.231 | 0.190 | 0.252 | 0.238 |
| isoAdvector-25 | 0.116 | 0.106 | 0.099 | 0.089 | 0.122 | 0.124 | 0.237 | 0.178 | 0.300 | 0.292 |
| [m] | [m] | |
|---|---|---|
| E-F | 0.104 | 0.458 |
| Z-F | 0.144 | 0.464 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kranenborg, J.W.M.; Campmans, G.H.P.; Jacobsen, N.G.; van der Werf, J.J.; Reniers, A.J.H.M.; Hulscher, S.J.M.H. Depth-Resolved Modelling of Intra-Swash Morphodynamics Induced by Solitary Waves. J. Mar. Sci. Eng. 2022, 10, 1175. https://doi.org/10.3390/jmse10091175
Kranenborg JWM, Campmans GHP, Jacobsen NG, van der Werf JJ, Reniers AJHM, Hulscher SJMH. Depth-Resolved Modelling of Intra-Swash Morphodynamics Induced by Solitary Waves. Journal of Marine Science and Engineering. 2022; 10(9):1175. https://doi.org/10.3390/jmse10091175
Chicago/Turabian StyleKranenborg, Joost W. M., Geert H. P. Campmans, Niels G. Jacobsen, Jebbe J. van der Werf, Ad J. H. M. Reniers, and Suzanne J. M. H. Hulscher. 2022. "Depth-Resolved Modelling of Intra-Swash Morphodynamics Induced by Solitary Waves" Journal of Marine Science and Engineering 10, no. 9: 1175. https://doi.org/10.3390/jmse10091175
APA StyleKranenborg, J. W. M., Campmans, G. H. P., Jacobsen, N. G., van der Werf, J. J., Reniers, A. J. H. M., & Hulscher, S. J. M. H. (2022). Depth-Resolved Modelling of Intra-Swash Morphodynamics Induced by Solitary Waves. Journal of Marine Science and Engineering, 10(9), 1175. https://doi.org/10.3390/jmse10091175









