Experimental ice studies starting in the 1960s focused on ice melting in conditions of free convection absent of any forced shear, oscillatory, or turbulent flows. Convection is flow driven by a temperature, and subsequently density, gradient; convective flows transfer heat as a result. In the free convection experiments we explore below, the temperature and resulting density differences between the ice and adjacent ambient water promote free convection. While there are no mechanically forced flows in these scenarios, unstable density stratifications generated by thermal gradients drive currents, circulation cells, and turbulence.
2.1. Horizontal Ice Sheets
Horizontally-oriented ice exists in the polar regions as sea ice, as undercuts or ungrounded extensions of glaciers, or bottoms of icebergs. Sea ice is of particular interest in studying polar melting. The less sea ice that exists in the oceans of the polar regions, the more the albedo effect is lessened, and the more solar radiation is absorbed into the exposed dark ocean water. The result is a feedback loop in which reduction of sea ice causes water temperatures to rise. This subsequently leads to less sea ice solidification during the fall and winter months, when the sea ice would typically recharge itself, and thus faster melting of existing sea ice in the summer.
Townsend [
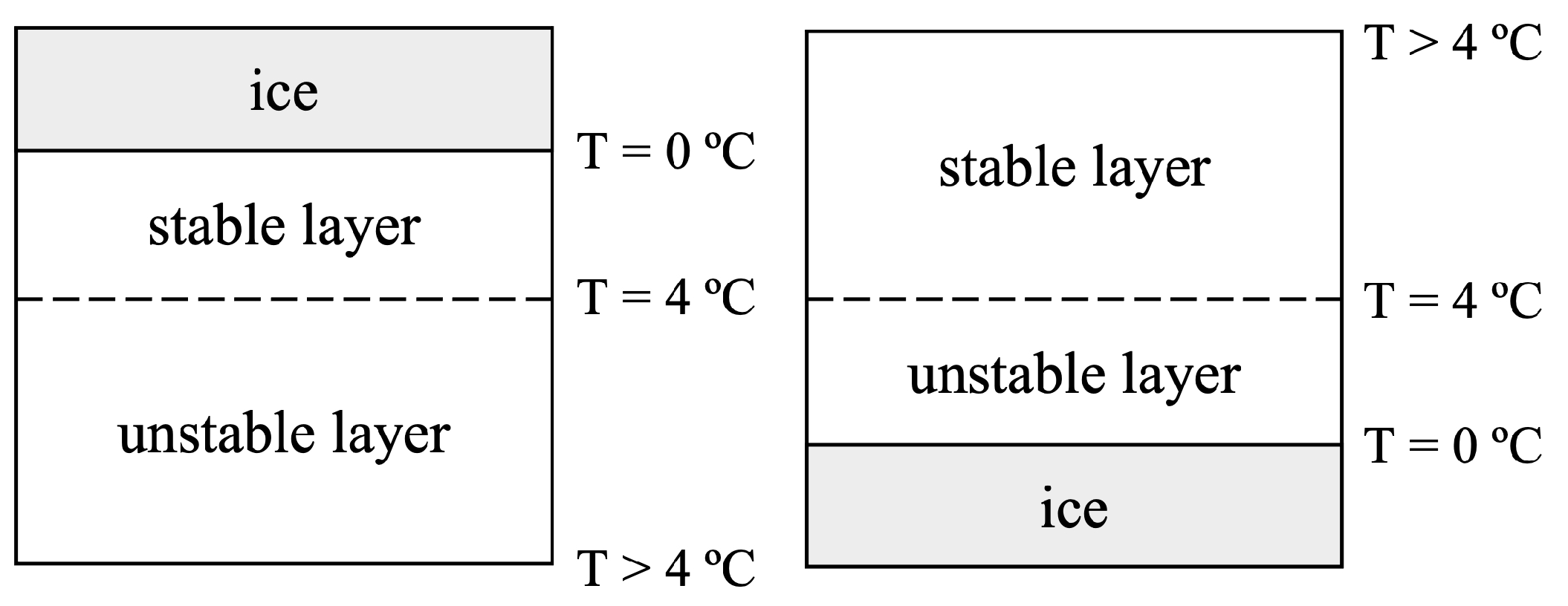
78] studied the effect of convective motion in an underlying unstable layer of fluid on a stable, stratified layer above in a small facility with a 30 cm by 30 cm square base filled to 15 cm with distilled water. The upper stable layer of water was maintained at 25 °C using an overhanging heated plate while the bottom of the tank was chilled to produce a thin layer of ice (
Figure 2). Observations of dye streaks and suspended particles showed the water above the ice to be stable initially. As the ice melted and approximately 0 °C meltwater rose from the bottom ice sheet, overturning occurred in a thin layer above the ice, and rising buoyant columns were visible. The buoyant columns rose to a stable stratification region of 3.2 °C before spreading horizontally at this depth. While this work lacked direct applications to glacier melt, they provided one of the first fundamental studies of ice melting.
Myrup et al. [
79] used an ice cube (1 ft. sides and cooled by a copper plate from below) overlain by fresh water with the intent of better understanding natural convective systems. Three regions were observed: a boundary layer, a convective layer, and an upper layer where convective currents did not penetrate. The cold buoyant plumes in the convective layer rose until they reached a neutrally buoyant layer, at which point they spread horizontally in a wavy motion, suggesting the presence of gravity waves. By analyzing thermocouple temperature data, it was determined the upper inversion layer was cooled by conduction, heat was carried by convection in the convection layer and by conduction to the boundary layer, and this heat was then converted to latent heat as the ice melted.
Adrian [
80] performed similar fundamental experiments to [
78,
79] of turbulent, statistically steady convection over a horizontal ice surface submerged in water and beneath a stably stratified layer in a 33.3 cm by 33.3 cm square base tank. Dural upper and lower plates maintained the water temperature with the top plate set to either 23.3, 25.6, or 33.6 °C, resulting in the convection layer thickness varying slightly based on temperature. The lower plate was kept near 0 °C. An LDV measured vertical velocity of the fluid flow, while thermistors embedded in the ice recorded the temperature. Observations revealed convection in a horizontal layer at a constant depth and a stratified, stable layer where internal gravity waves propagated.
Seki et al. [
76] performed experiments that melted horizontal ice overlain by fresh water that was at an initial temperature of 0 °C. A copper plate heated between 1 and 15 °C was located above the horizontal water layer to investigate free convective heat transfer. The apparatus measured 150 mm by 150 mm with a height of 25 mm. They noted the TMD of water played a significant role in the development of free convection and heat transfer in the water layer for
T≤ 8 °C.
The following works were setup such that the water was either beneath the ice for broad planes of ice, or surrounded ice cylinders for complementary experiments, as compared to the previous studies with only bottom-mounted horizontal ice sheets. While ice cylinders have a simplified, symmetrical shape that would not be found in nature, results of flow patterns around the ice can be applied to scenarios with various natural ice geometries, such as icebergs and sea ice. In laboratory experiments, ref. [
81] studied the free convection of water under a horizontal slab of ice to understand melting that would occur at the underside of an iceberg or sea ice. Experiments were performed in a slightly larger tank than in prior studies, with a 45 cm by 45 cm square base and water depth of 75 cm. The ice was located at the upper surface of water with a salinity of 37.6‰ using a NaCl solution, differing from the previous two studies that used fresh water. The far-field (i.e., ambient) temperature at the bottom of the tank was maintained constant at approximately 0 °C. Ref. [
81] identified three regions of heat transfer: a diffusive boundary layer at the ice-water interface where the temperature increase was linear and salinity nearly approximated the far-field value, an unstable boundary layer of double-diffusive convection below the diffusive boundary layer, and a thermal convection layer in the far-field. Double-diffusive convection occurs when two different density gradients result in different rates of diffusion and a finger-like pattern of density develops. Double-diffusive convection drives mixing of salinity and heat flux at the ice-water boundary [
82,
83]. A one-dimensional model of ice melting in conditions of purely diffusive heat transfer found the ice to melt approximately twice as fast in the laboratory convective case.
Gebhart et al. [
84] conducted experiments of horizontal ice slab melting from below in water to understand the melting of circular sea ice. The ambient water conditions ranged from temperatures of −1.75 to 3 °C with a salinity of 35‰. Observations of passive seeding particles dispersed in the water showed the flow below the ice to move radially inward toward the center of the ice across the entire temperature range. An outward moving horizontal layer of flow was observed directly below the inflow layer, which moved deeper with increasing ambient water temperature. Ref. [
84] noted that the buoyancy force has two diffusive components, thermal and saline, which depend on the local temperature and salinity. Lastly, the local melt rate for all temperatures was found to be higher at the edges of the ice sheet as compared to the center.
To understand the melting of glaciers, ref. [
85] investigated the melting of ice in 35‰ (by weight) saline water at ambient water temperatures ranging from 1.8 to 25.0 °C. The geometry of the ice used in the experiments consisted of horizontal ice cylinders and ice plates inclined at angles from 0 to 75 degrees to the horizontal plane. Visualizations were recorded of melting occurring above and below the inclined ice plates and around the horizontal cylinder. Ref. [
85] determined the melting heat transfer coefficient from the local melting velocity via analysis of photographs of the melting ice. Results showed the melt rate to increase monotonically with the ambient water temperature for both ice geometries, whereas the melt rate was nearly independent of the angle of inclination for the ice plate.
Fukusako et al. [
86] investigated the melting of a horizontally-oriented ice cylinder in ambient water of constant salinity of 35‰, while varying temperature from 2.8 to 20.3 °C in initially quiescent conditions. Visualizations of aluminum powder seeding particles illuminated by a He-Ne laser showed laminar bidirectional flow at the lower region of the ice and upward turbulent flow in the upper region, consistent with prior findings. The velocity at which the ice melted was found to increase linearly as the ambient water temperature increased. Ref. [
87] investigated the free convection melting of a horizontal ice cylinder varying ambient water salinity (5 to 35‰) and temperature (1.8 to 24.0 °C). Visualization of flow patterns around the ice was accomplished by seeding the water with aluminum powder particles and illuminating the particles with a He-Ne laser sheet. PIV, which was concurrently under development and could potentially have been used in this configuration to obtain quantitative velocity measurements from the visualizations, was not used. Three regimes of flow were observed: the density inversion of the saline water where the flow around the cylinder was upward, a lower laminar and unidirectional portion, and a turbulent upper region.
Motivated to understand the melting of glaciers in sea water, ref. [
88] melted horizontal ice plates into a calcium chloride aqueous solution from above. The experiments were described as ice plates melting spontaneously and the temperature decreasing at the melting front despite no initial difference between the ice and water with the temperature for both at the beginning of the experiment set at −5 °C. Flow visualizations revealed natural convection at the melting front, which was dominated by the concentration gradient in this region. A two-dimensional numerical model was used to calculate the theoretical melting, which was comparable to the experiments with a difference of less than 30%.
In direct numerical simulations (DNS) and laboratory studies, ref. [
89] investigated the effect of turbulent convection on melt rates beneath a horizontal ice layer. The experimental facility was a Plexiglas tank, insulated with a layer of Styrofoam and filled with tap water. An ice block (made from distilled water) was positioned at the water surface. Scaling laws were determined to understand the relationship between the melt rate and the far-field temperature. Results revealed the melt rate increased two orders of magnitude with a far-field temperature of 4 to 8 °C. The results also showed a stably stratified diffusive layer that insulated the ice from the warmer, turbulent layer when the far-field temperature was below 8 °C. The stratification of this layer was not present when the far-field temperature was above 8 °C. The flow structures and melt rate results from the DNS were found to agree with the laboratory experiments.
Yen and Galea [
90,
91] performed unique experiments of fixed vertical ice cylinders in a cylindrical apparatus with heating from above. The top surface of the ice was melted, which subsequently resulted in a deepening horizontal layer of water at the top of the ice over time. These experiments aimed to determine when the mode of heat transfer to the ice changed from conduction to convection in the water layer. A cold plate was located at the bottom of the tank, while a warm plate was located at the top. The upper warm plate was varied in temperature from 4.06 to 39.90 °C and the initial ice temperature was varied from −6.5 to −14 °C. The initial ice temperature did not significantly affect the onset of convection. The time at which convection began could be determined from when the inflection point occurred in the data curve of water-ice interface position to time, or at the time when the temperature profile of the water layer, measured by thermistors, diverged from a linear distribution.
Wagman and Catania [
92] performed a study to understand how subglacial hydrology affects the motion of ice in Antarctic ice streams. The ice streams were modelled using a silicone polymer that substituted as ice to simplify the experimental design. The surrogate ice was located over a thin water layer in the experiments. This model allowed the basal sliding of ice flow to be studied in a simplified setup with surrogate ice. Results showed the stability of the ice-stream is influenced by changes in basal lubrication.
Stepanova and Chaplina [
93] investigated the rotation of ice disks placed on a solid surface and on water at a temperature of 20 °C. The ice was dyed for flow visualization. The threshold depth of water for the rotation to occur was 3 cm. While some key details of the experimental setup such as water salinity were not provided, the ice rotation was reported to be slower in saltwater compared to in fresh water. For the water surface experiments, the ice mass loss was found to be exponential with time. Ice rotation was found to be caused by vortex flow underneath the ice. The rotation velocity increased with the water temperature in the reservoir. Rotation did not occur at water temperatures of 5 °C and below due to effects of the TMD of water.
A few other papers also considered ice melting via laboratory experiments, but the applications and temperature ranges were not focused on ice melting in natural scenarios. For example, ref. [
94] investigated natural convection due to proximity to the TMD above ice overlain by a recalculating reservoir with water of a temperature of 70 °C. Accompanying numerical simulations closely matched the experimental results. Ref. [
95] performed experiments to understand how compositionally-driven melting occurs in magma chambers, specifically to explore how ice and wax melted under or over a hot aqueous solution of ethanol. The ice geometries considered included an ice roof, ice floor, and sloping ice floor. Ref. [
96] dissolved both an ice roof and floor in an isopropanol solution to test a scaling analysis. The far-field solution concentrations and temperatures were 22 to 36.5% and approximately −6.2 °C, respectively, for the ice roof experiments. For the ice floor experiments, the ice was grown to a thickness of 3 cm. The far-field concentration and temperature ranged from 16.5 to 23.2% and −14 to 6 °C, respectively. The intended application was to quantify the dissolution that occurs in magma chambers. The measured dissolving velocities were consistent with their scaling analysis.
2.2. Vertical and Tilted Ice Walls
If the ambient water adjacent to a vertically-oriented ice wall is sufficiently warm and/or saline, a meltwater plume will be generated along the melting ice. This relatively buoyant, freshwater melt plume will rise vertically along the ice, entraining surrounding ambient water as turbulence develops due to shear between the upward flow and ambient water. The entrainment will potentially carry warm and/or salty water toward the ice face, subsequently promoting continued melting along the ice face.
Bendell and Gebhart [
62] investigated the affect of the TMD on the flow direction and heat transfer in experiments melting a vertically-oriented ice wall in distilled water. The far-field temperature,
, in the tank was varied from 2.2 °C to 25.2 °C. In doing so, an inversion temperature was observed where the flow switched from upward to downward, due to proximity to the TMD. When
5.6 °C, upflow was observed, and when
5.5 °C, there was an observed downflow, with some overlap existing between 5.5 to 5.6 °C. This reversal occurred at the minimum Nusselt number when
= 5.6 °C. Ref. [
97] performed similar experiments in distilled water, varying the ambient water temperature from 2 to 7 °C. Three regimes of flow, dependent on the water temperature, were observed: upward flow below 4.7 °C, downward flow above 7 °C, and oscillatory flow from 4.7 to 7 °C.
A set of three other experiments also looked at the effect of the TMD on flow direction and temperature regime that produced dual flows. Ref. [
98] melted a vertical slab of ice in deionized water with temperatures ranging from 3.9 to 8.4 °C. The experiments revealed predominantly upflow at 4.40 °C, changing to downflow at 5.40 °C, and bidirectional flow from 4.05 to 5.90 °C. Ref. [
64] later looked at temperature and velocity profiles at a vertical ice face melting in fresh water using the same velocity measurement system as [
97]. Results also showed an upward flow at lower temperatures, downward flow at higher temperatures, and dual flow from 4 to 6 °C. Observations provided more insight in the dual flow: upwards near the ice wall and downwards away from the ice wall at 4 °C; vice versa at 5 °C; and the disappearance of the dual flow above 6 °C. The dual flow in these experiments was important, as outer warmer ambient water was able to be entrained into the plume and subsequently brought in contact with the ice face to enhance melting. The system of [
64] was modeled as two-dimensional, steady-state laminar flow, and the temperature and velocity profiles were comparable to the experimental findings. The minimum Nusselt number was found to occur at a temperature approximately equal to 5.6 °C, similar to previous studies [
62,
98].
Gebhart and Wang [
99] investigated the same three regimes (i.e., dual, up, and down flow) with a vertical ice cylinder in deionized water. Using an optical laser visualization method, they were able to define more types of flows at specific temperature ranges: upflow below 4 °C, upflow with buoyancy flow reversal from 4 to 4.2 °C, downflow in the outer region and circulations from 4.2 to 4.8 °C, upflow near the ice and downflow in the outer region from 4.8 to 5.3 °C, dual flow and convection from 5.3 to 5.5 °C, and complete downflow at the boundary region above 5.5 °C. Moreover, using a vertical cylinder provided the insight that more melting occurred on the vertical ice walls as compared to along the ice bottom. Ref. [
66] studied the melting of a vertically-oriented ice cylinder in water temperatures ranging from 2 to 10 °C, making observations of the ice morphology. Related to the TMD of water, the three morphology profiles of the ice observed were inverted pinnacles at 4 °C due to constant upward boundary layer flow, upward pinnacles at 7 °C from a downward boundary layer flow, and scallops between temperatures of 5 to 7 °C from the formation of recirculating vortices. Ref. [
66] used a phase-field model [
100,
101], suitable for moving boundary problems in free convection, to further understand the shape dynamics observed in their experiments.
Scanlon and Stickland [
63] melted a vertical ice cylinder in a cylindrical apparatus using PIV to visualize natural convection during melting. Their laboratory data were compared with a numerical model. Velocities from the numerical model were found to agree with PIV measurements that found the velocity of the water near the ice to be 3 to 5 mm/s. While the study did not provide additional details regarding the flow phenomena or TMD effects, the work highlighted the potential of numerical models and PIV to capture both qualitative and qualitative results associated with melting.
While the previously-discussed papers investigated vertical ice melting in fresh water, a number of papers starting in the 1980s performed similar experiments in saline water. Ref. [
77], motivated by the melting of icebergs, melted vertical ice in a water tank with uniform salinity and then with a salinity gradient in a second set of experiments with water temperatures of 20 °C and 8.0 to 26.0 °C, respectively, to quantify where the meltwater from the ice was deposited. The flow dynamics were different in saline water as compared to fresh water because of the location at which the meltwater was deposited due to density differences between the two liquids. In these experiments, the thin layer of rising cold meltwater at the ice-water boundary mixed with water outside of this boundary layer and flowed downward until stopping where the far-field conditions were equal in density. The meltwater was deposited at this level and subsequently moved outwards horizontally. A similar result was found by [
102], where the water had a vertical salinity gradient of 10% m
and the temperature varied from 1.8 to 19.3 °C. In a separate experiment, ref. [
102] studied how a turbulent layer flowing upward in a lower unstratified region would affect the formation of horizontal layers in an upper stratified region. The lower region had a uniform density, while the region above was stratified linearly. The horizontal layers again formed at a neutrally buoyant level, but they were located further away from the ice where the turbulent, convective plume propagated up into the stratified region.
In experiments designed to explore the formation of boundary layers along vertical walls, ref. [
103] conducted tests in water adjacent to an ice wall. Conditions of ambient water temperatures below 20 °C and salinity from 30 to 35‰ resulted in laminar bidirectional flow (
Figure 3) located at the bottom of the ice wall and turbulent upward flow above, along the rest of the ice surface. For temperatures greater than 20 °C at the same salinity range, laminar bidirectional flow was observed near the top of the ice, while a downward turbulent flow was observed beneath. The study consisted of numerical simulations that included an eddy diffusivity dependence on the density difference between the ice-water interface and the far-field. The laboratory data were then used to evaluate this dependence, finding the magnitude of the eddy diffusivity to be of the same order as the molecular viscosity where mass injection at the interface and opposing buoyancy forces must be included in the flow model to be accurate.
Carey and Gebhart [
104] investigated buoyancy-driven flow at the interface of a vertical ice sheet in 10‰ saline water while varying ambient water temperatures,
, from 1 to 15 °C. At
= 1 °C, an upward laminar flow formed at the ice boundary. At
= 2 °C, the flow at the boundary was still laminar and traveled upwards, but a weak downflow outside of the boundary layer was also observed. At
= 2.5 °C, a stronger downflow existed, but was overall still laminar and bidirectional. At
= 5 °C, there was upward and turbulent flow near the top of the ice, while towards the bottom, the flow was bidirectional and laminar. At
= 10 °C, the flow behaved similarly to observations at
= 5 °C for the top portion of the ice, but the bottom was fully downward flow. For the last test,
= 15 °C, the flow pattern had changed significantly to mostly turbulent downflow at the ice surface, with a small region of upflow at the top. Although PIV had not yet been formally developed, ref. [
104] was able to measure the lengths of particle streaks from each photograph to obtain velocity estimates [
105].
Sammakia and Gebhart [
106] used ambient water salinity ranging from 14 to 35‰ and water temperatures of −1.75 to 17 °C in experiments melting vertical ice. Velocity profiles were obtained from time exposure photographs. Observations showed the flow to be laminar at all salinity levels when the ambient water temperature was low. This laminar flow direction was upward or bidirectional for lower and higher salinity levels, respectively. For intermediate water temperatures, the flow was bidirectional at the lower region of the ice and, by contrast, upward, turbulent flow for top portion of the ice. At higher ambient water temperatures, there was a split in the flow, with a downward turbulent flow at the lower portion of the ice and an upward layer at the top region due to salinity and thermal gradient buoyancy forces, which are either in opposition or stacked depending on the salinity and temperature levels. A buoyancy force will be upward when ice melts in saline water, but thermal buoyancy can be more variable because of the maximum density of water being at approximately 4 °C. The results presented were similar to those of [
103].
Johnson and Mollendorf [
107] performed experiments with a vertical slab of ice in approximately 35‰ salinity water, varying the ambient water temperature from −1.08 to 20.76 °C. From experiments, it was observed that the salinity gradient determined the final flow direction of the meltwater plume. Laminar upward flow that developed into turbulent flow was observed at the ice-water boundary. The length of the laminar region decreased with increasing ambient water temperature. The thickness of the laminar boundary layer increased with downward distance from the laminar to turbulent transition region, indicating a bidirectional flow similar to findings from [
103]. This behavior was indicative of bidirectional flow. Furthermore, the melting rate decreased monotonically with a decrease in the ambient water temperature. Lastly, the interface salinity increased close to the far-field salinity with decreasing ambient water temperature.
Eijpen et al. [
108] investigated the waterline and subaqueous ice-melt rates of vertical ice in ambient water in which the temperature was varied from 0 to 10 °C, while the salinity was varied between 0, 17.5, and 35‰. The melt rates were observed to be slightly faster in fresh water as compared to saltwater, and the different water and temperature combinations resulted in varying ice-front geometries. Observations found notches to form quickly on the ice above 4 °C and even faster in saline water. Lastly, the temperature-driven density differences were found to drive convection and be a significant melting process at the ice face.
Kerr and McConnochie [
70] dissolved and melted sheets of vertical ice via turbulent convection into saltwater with temperatures ranging from 0.3 to 5.4 °C and salinity from 34.4 to 36.0‰ using NaCl. A meltwater plume formed along the face of the ice. Laminar flow was observed in the plume at a height of 10 to 20 cm from the base of the ice. At a height of 20 cm, the flow transitioned from laminar to turbulent. The thickness and velocity of the turbulent upflow increased with height. The theoretical model developed in the study most accurately predicted dissolution velocity of the vertical ice surface for conditions of water temperatures up to approximately 5 to 6 °C, the temperature range at which turbulent dissolution transitioned to turbulent melting. Moreover, the dissolution velocity had an approximately 4/3 power law dependence on the difference between the water temperature and its freezing point. By contrast, refs. [
107,
109] both used vertical ice sheets that were 20 to 30 cm high; these heights were only high enough to observe the development of mostly laminar compositional convection.
McConnochie and Kerr [
71] subsequently investigated the dissolution of a vertical ice face in cold, saline water with a varying far-field salinity gradient, which was established using a double-bucket method [
110]. They confirmed the salinity gradients of the water via a conductivity-temperature probe (CTP). The experimental apparatus, described in [
70], was located in a 4 °C cold room. Thermistors were used to measure the temperature at the ice-water interface, while ablation velocity was determined from photographs of the interface region over time. The plume velocity was determined from shadowgraph particle tracking velocimetry (shadowgraph PTV). Double-diffusive layers were observed near the plume (similar to [
77,
102]), indicating significant detrainment from the plume according to [
71]. The salinity stratification of the far-field water was found to reduce the ablation velocity, plume velocity (i.e., 1/3 power law effect), and interface temperature. The ablation velocity of the ice also decreased with height. Ref. [
71] noted that plume buoyancy will decrease or increase in a homogeneous or stratified fluid, respectively. Ref. [
71] defined a stratification parameter,
S, to determine in which scenarios ambient stratification should be considered. The authors noted when
, past homogeneous stratification models would not be accurate at specific heights along the vertical ice wall. Stratification parameters were said to range from 20 to 130 for Antarctica and Greenland ice shelves, indicating ambient stratification (in addition to other factors including far-field temperature and salinity) would affect melting rates in these regions. Last, ref. [
71] noted how the presence of a salinity gradient may result in detrainment of water from the plume to the far-field, affecting buoyancy and velocity, among other plume properties, and thus heat and salt transfer to the ice.
Motivated by floating ice shelves, ref. [
73] studied dissolution of a sloping ice wall in cold saline water via turbulent compositional convection. The wall was sloped at angles of 0 (control case) and 20–39.5 degrees to the horizontal. The scaling analysis of [
70] for the dissolution of a vertical ice wall was the basis of the model used for the case presented here that used sloping ice walls. The model they used had no free parameters and no dependence on height. Interfacial temperature and interfacial composition were found to be independent of slope, and the dissolution velocity was proportional to
, where
is the angle of the sloping surface relative to the vertical. The model results were within an experimental uncertainty of 10% for both ablation velocity and interface temperatures. The ablation velocity was predicted as a function of ocean temperature and basal slope. The model was applied to the underside of a Greenland glacier and was successful in predicting an ablation rate similar to that observed by [
111].
In experiments presented by [
112], a vertical ice wall was melted in an ammonium chloride-water solution in both numerical and laboratory studies. The facility was a square cavity. Observations showed the development of temperature and concentration gradients in initially homogeneous liquid caused by the melting process. The system also showed double-diffusive convection processes resulting in stable concentration stratification above a convective layer. The double-diffusive convection processes were found to cause significant fluctuations in local melt rates, temperature, and concentration at the ice water interface. Further experiments in [
113] involved melting a vertical ice layer in an ammonium chloride-water solution. The facility was a rectangular tank with differentially heated side walls. Again, a double-diffusive region was observed, similar to [
112], that can result in significant mixing and subsequent enhancement of local melt rates.
Bénard et al. [
114] performed melting experiments of a vertical ice face in a sodium carbonate aqueous solution to study thermohaline convection. Ref. [
115] similarly melted vertical ice in a sodium carbonate aqueous solution in an effort to distinguish dissolution effects (i.e., ice loss due to the sodium carbonate solution) relative to melting from thermal gradients (i.e., differential heating and cooling across the experimental facility). Local interfacial temperatures and front velocities were measured to understand fluid motion and local heat transfer that developed into recirculating cells.
Sugawara et al. [
116] conducted experiments with a 100 mm vertical ice face to investigate melting in flow conditions of free convection while varying ambient water temperature in an initially homogeneous calcium chloride (20‰) aqueous solution. Results showed that spontaneous melting occurred even when there was no initial temperature difference between the ice face and ambient water. The aspect ratio of the size of the liquid volume, defined as height divided by width, had no influence on the melting rate at the beginning of the experiment. However, a large aspect ratio was found to decrease melting as the experiment progressed. Visualizations revealed an upflow at the boundary layer as the ice melted and a downward thermal convection with a counter-clockwise circulation outside of the boundary layer.
A few other ice melting experiments were identified in the literature that had applications or water properties outside of the range of seawater characteristics. Ref. [
117] melted freely floating ice in an isothermally heated horizontal cylinder. Flow visualizations revealed how the TMD affected flow regimes around the ice and the interface shape of the ice. The temperatures at the top and bottom of the cylinder wall were varied from 2.5 to 17.5 °C and 2.9 to 19.3 °C, respectively, and the melting rates were observed to increase with wall temperature. Ref. [
118] melted ice in a cylindrical enclosure heated from the bottom at 100 °C via conduction. A cooling rate sensor was developed to measure the phase change velocity and to correlate the melting time to melt thickness, mass of melt, volume of melt, and energy of melt values.