Explosion Characteristics of Hydrogen Gas in Varying Ship Ventilation Tunnel Geometries: An Experimental Study
Abstract
:1. Introduction
2. Hydrogen Explosion Test in Tunnels
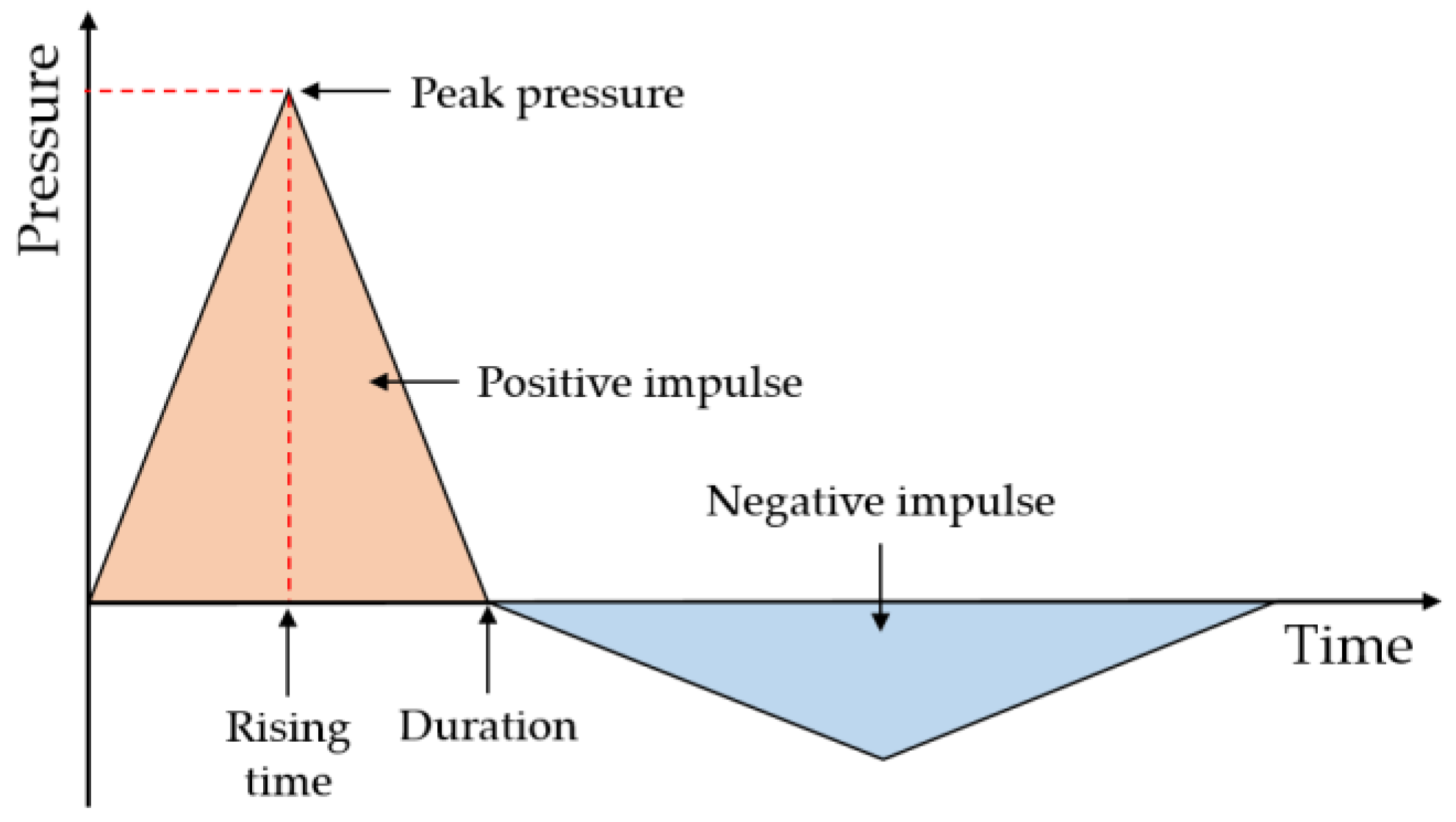
2.1. General Gas Explosion Load Characteristics
2.2. Method of Explosion Test and Apparatus
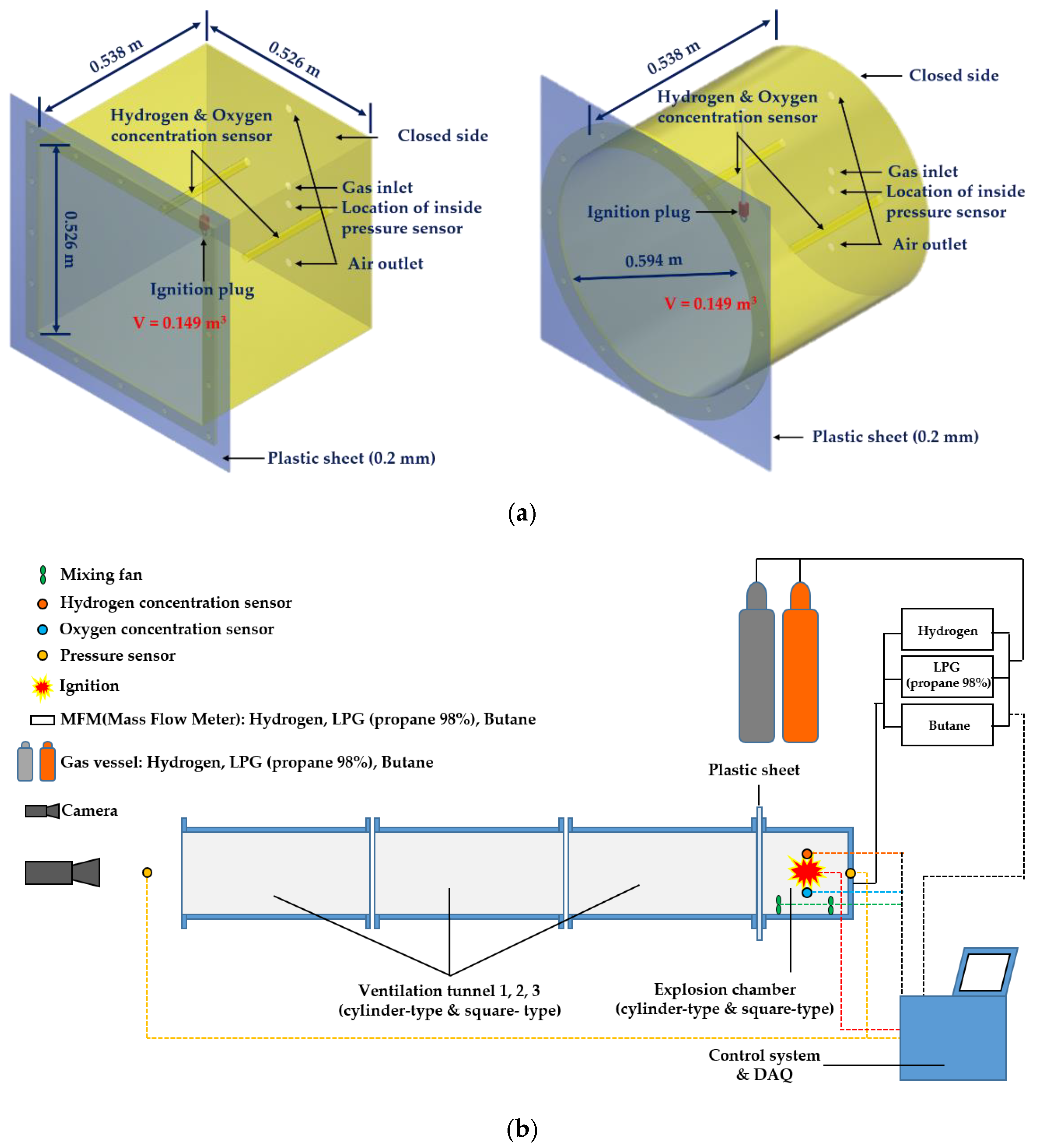
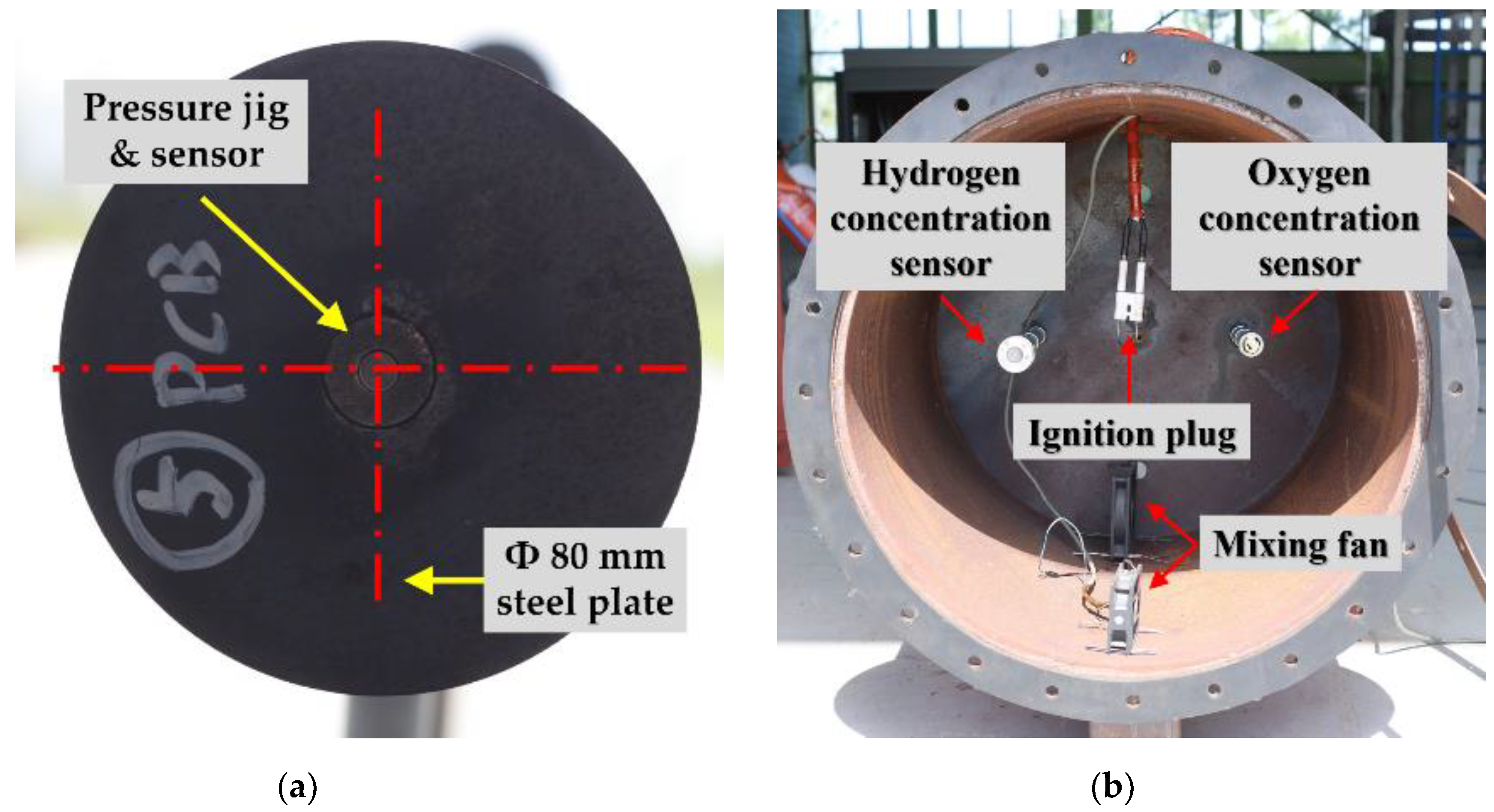
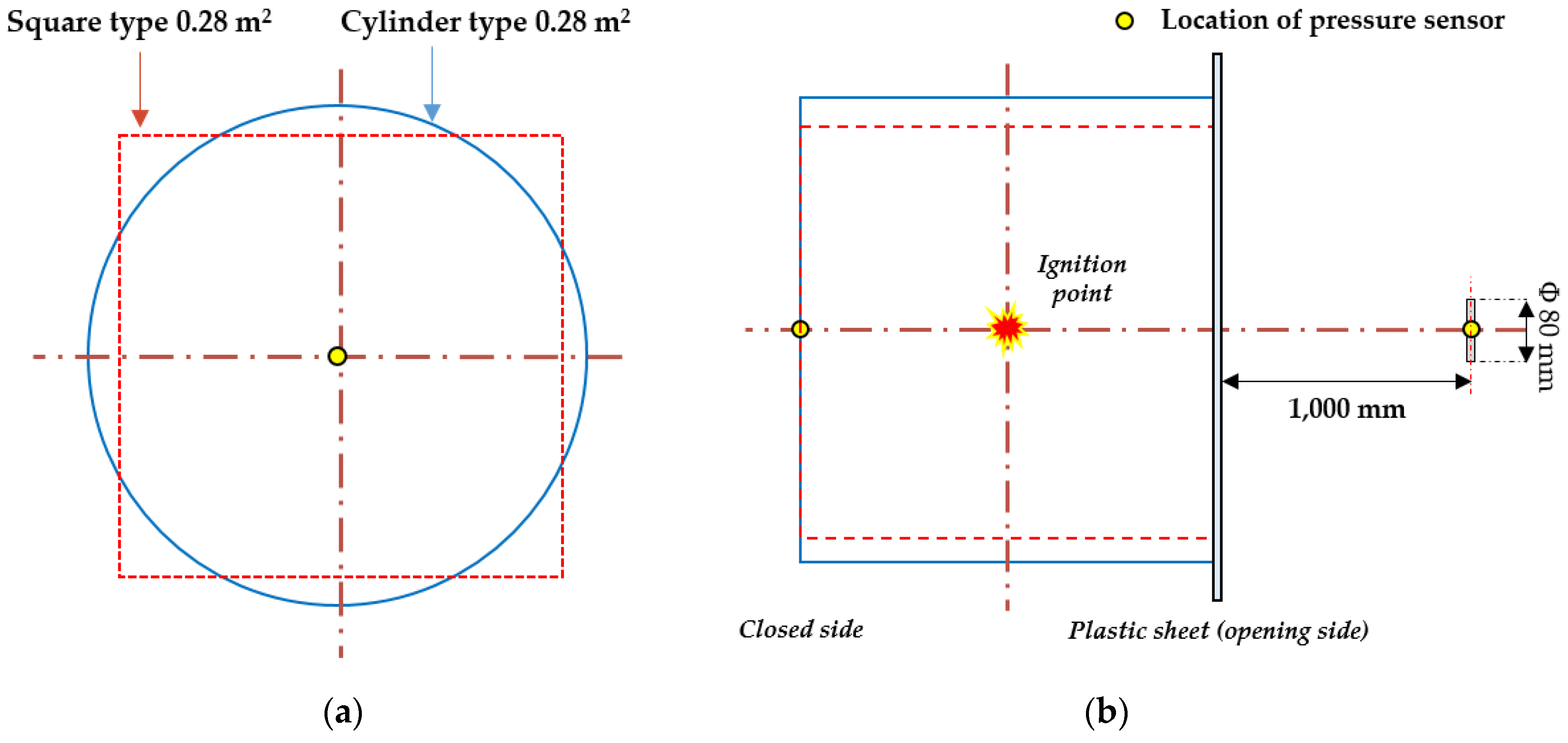
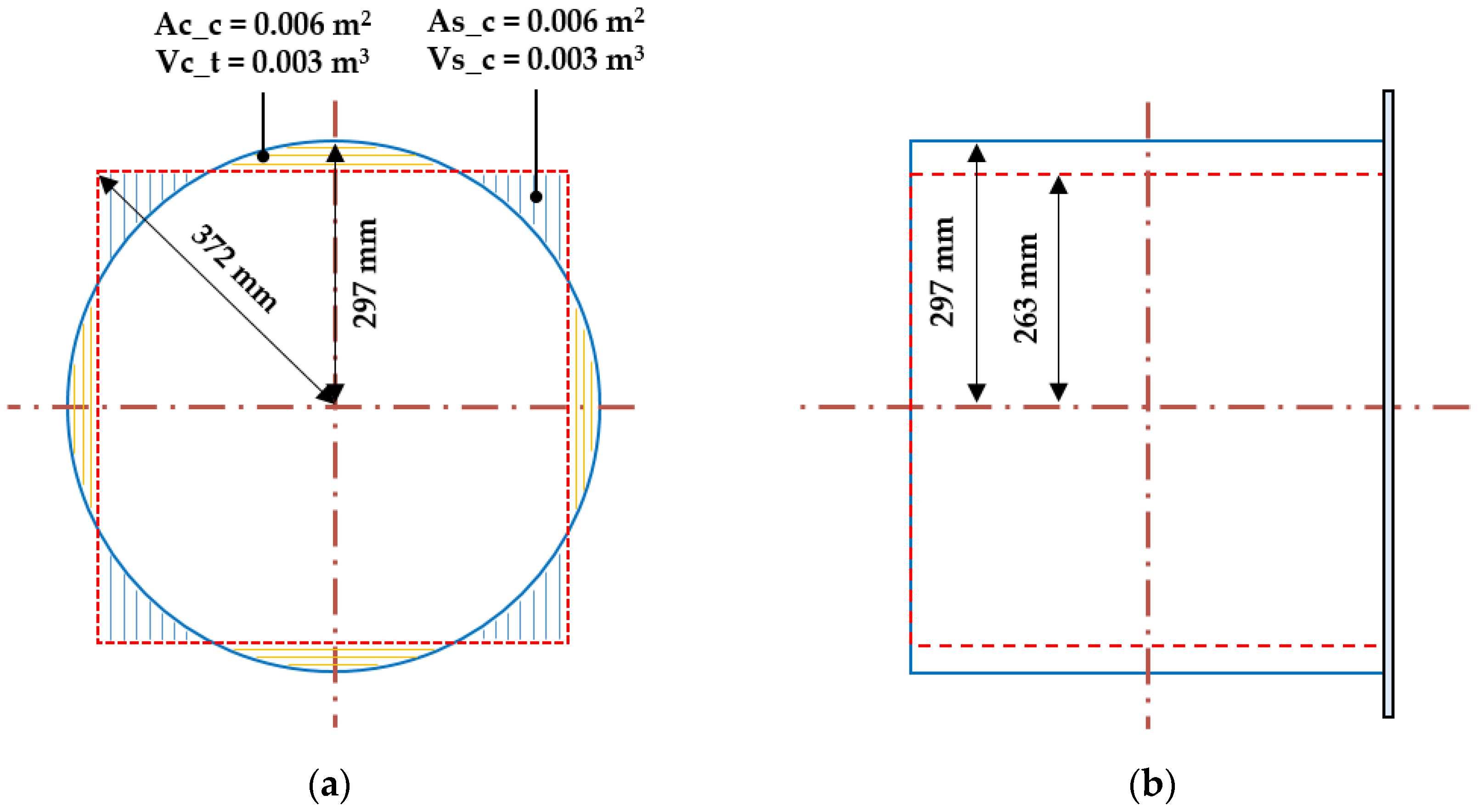
2.2.1. Target Explosion Chamber and Tunnel
2.2.2. Explosion Test
3. Results and Discussion
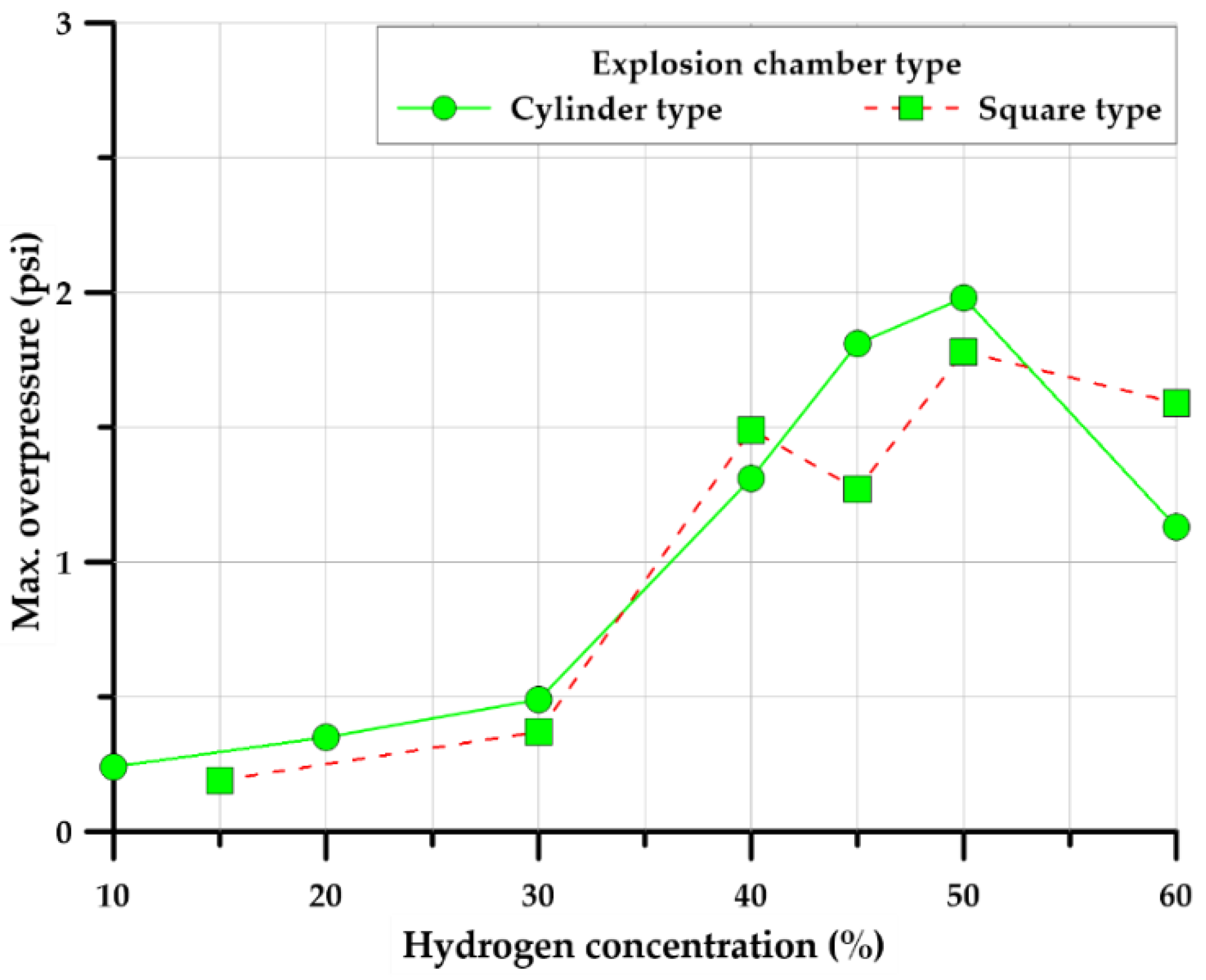
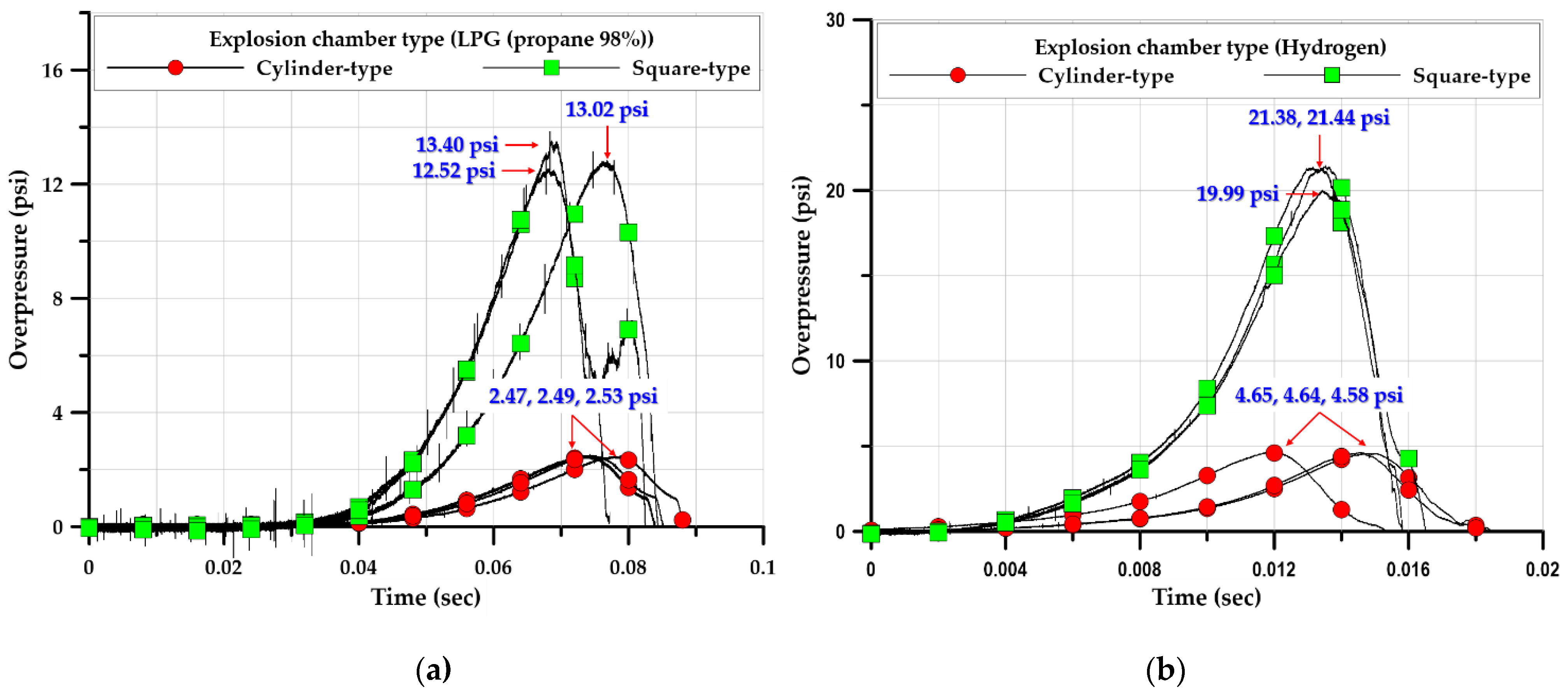
3.1. Explosion Test of Cylinder-Type Chamber
3.2. Explosion Test of Square-Type Chamber
3.3. Discussion
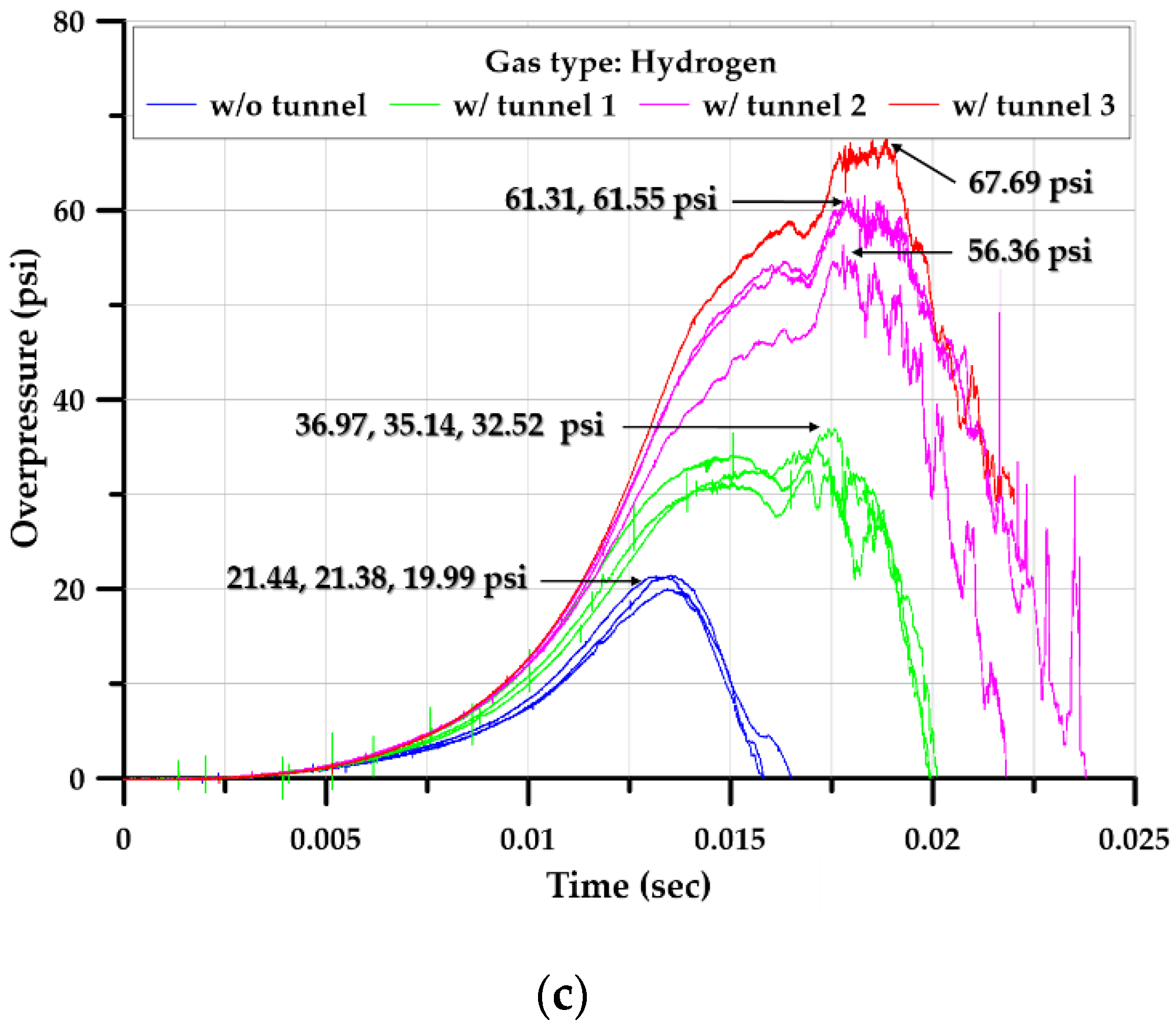
3.3.1. Overpressure
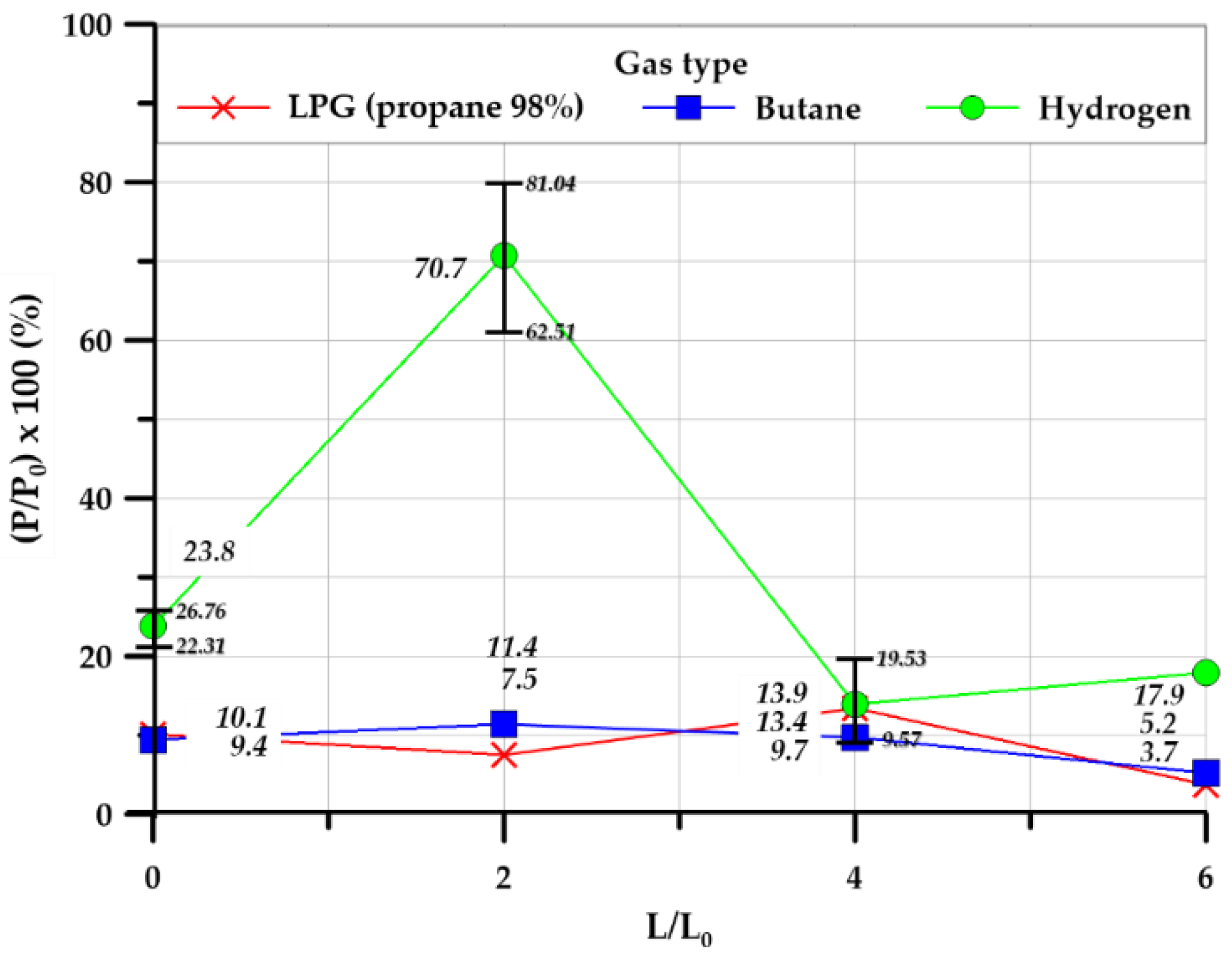
3.3.2. Explosion Chamber Type
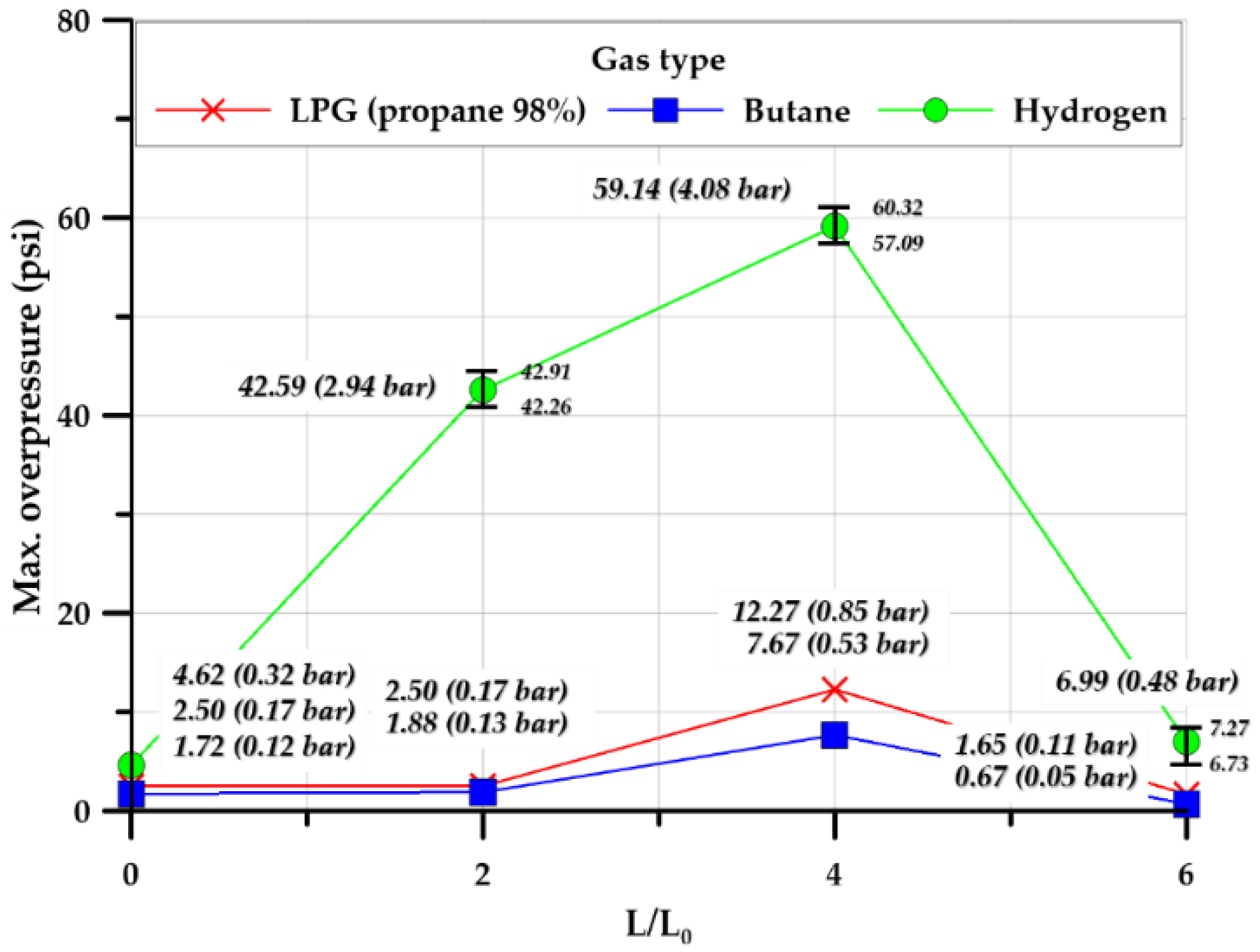
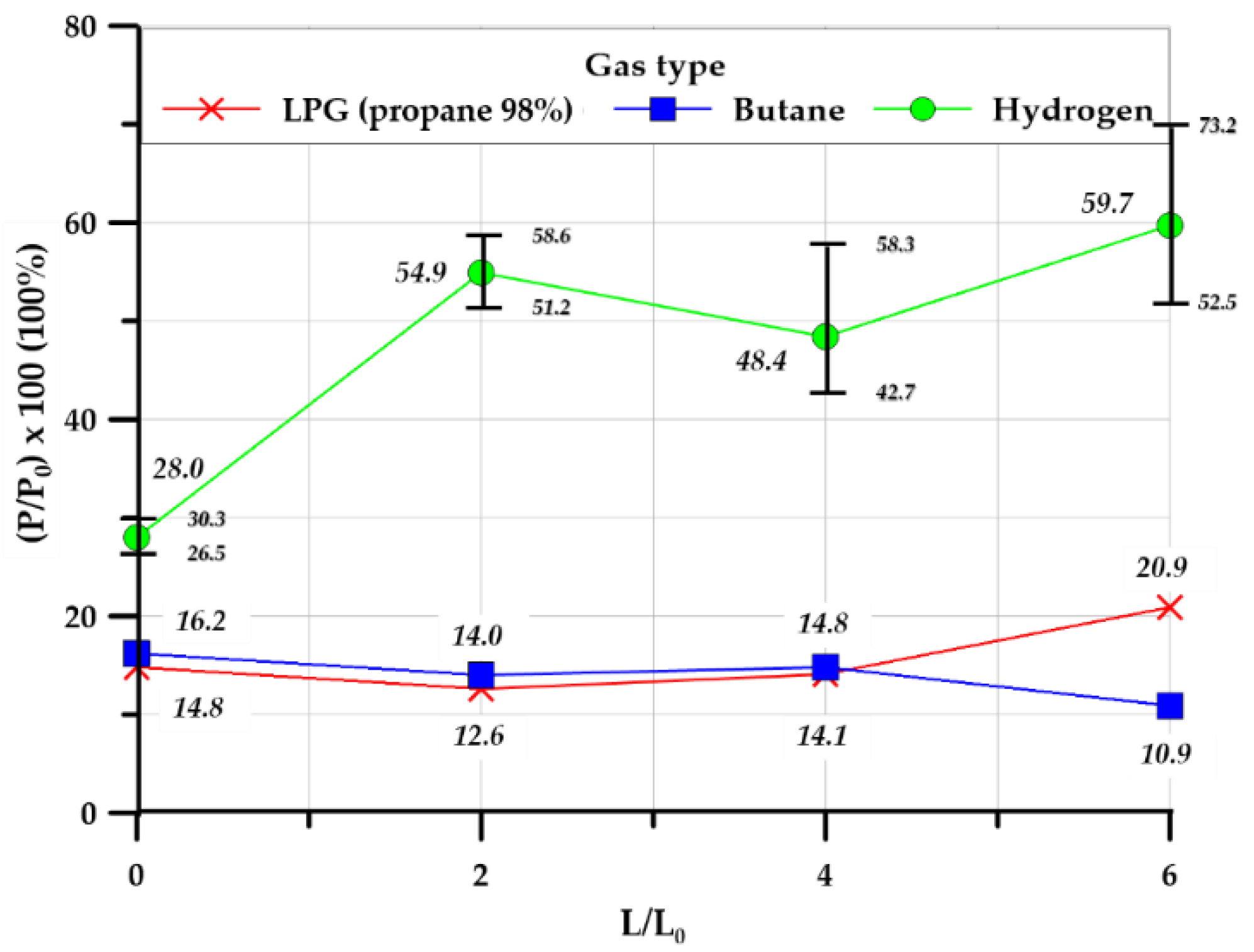
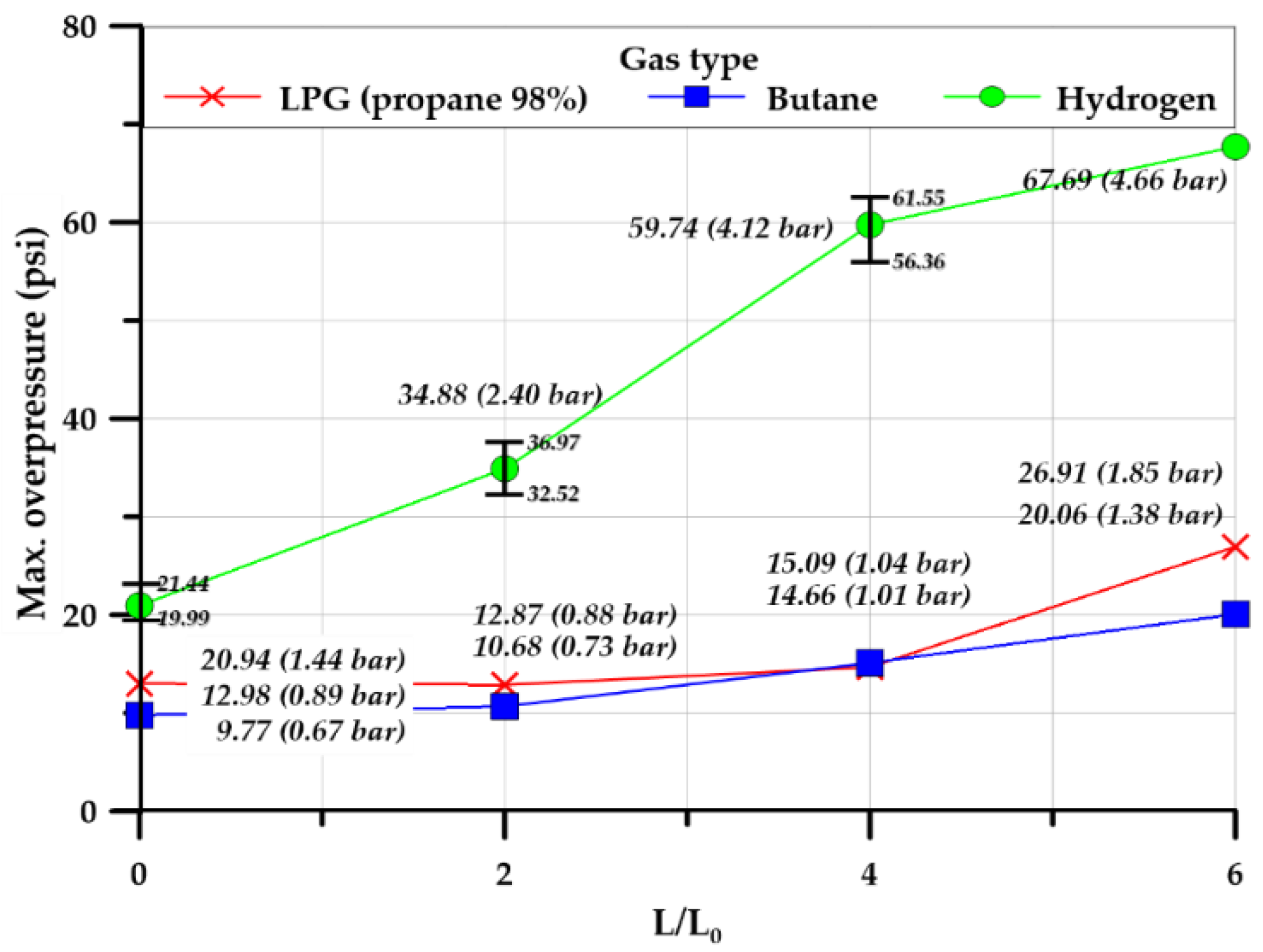
3.3.3. Effect of Tunnel Length
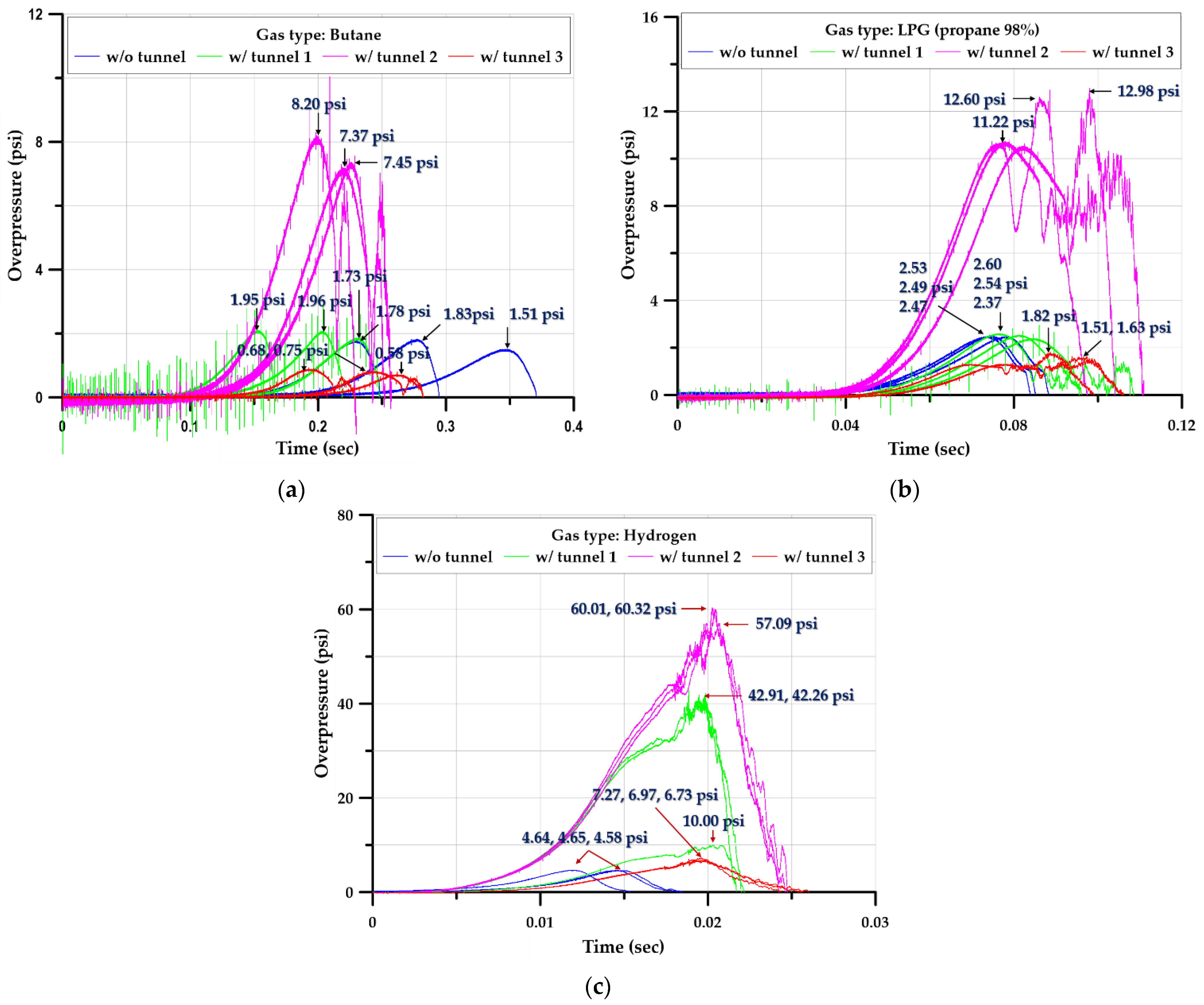
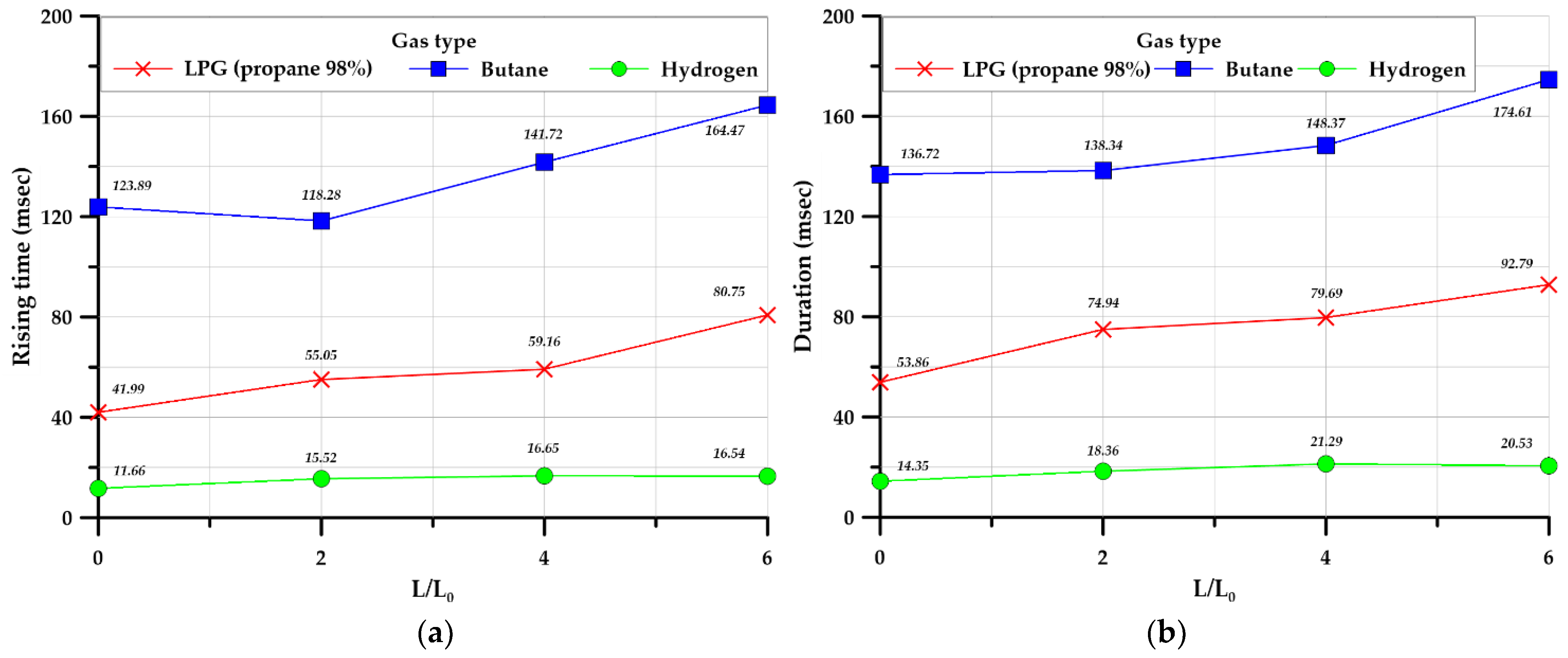
3.3.4. Rising and Duration Times
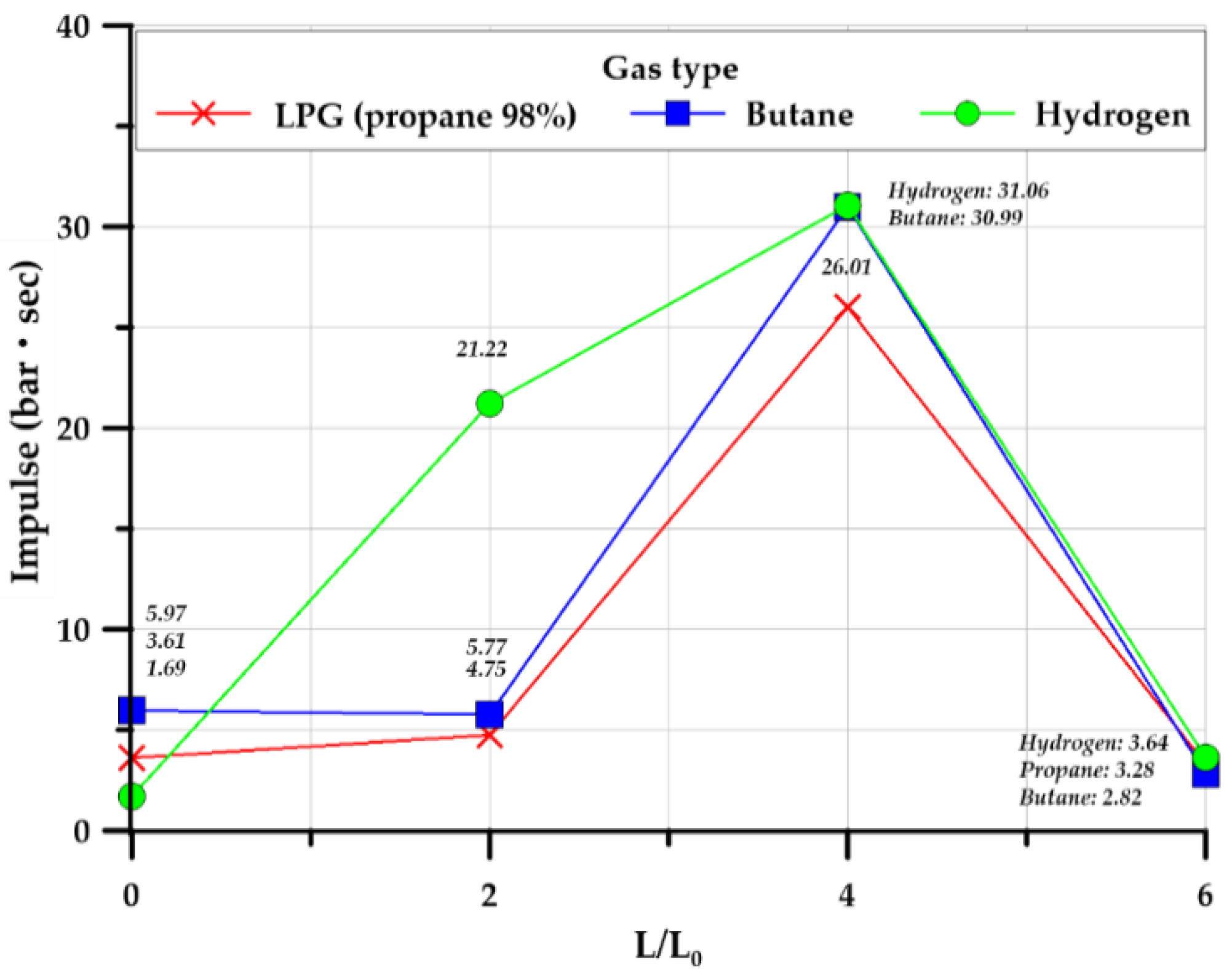
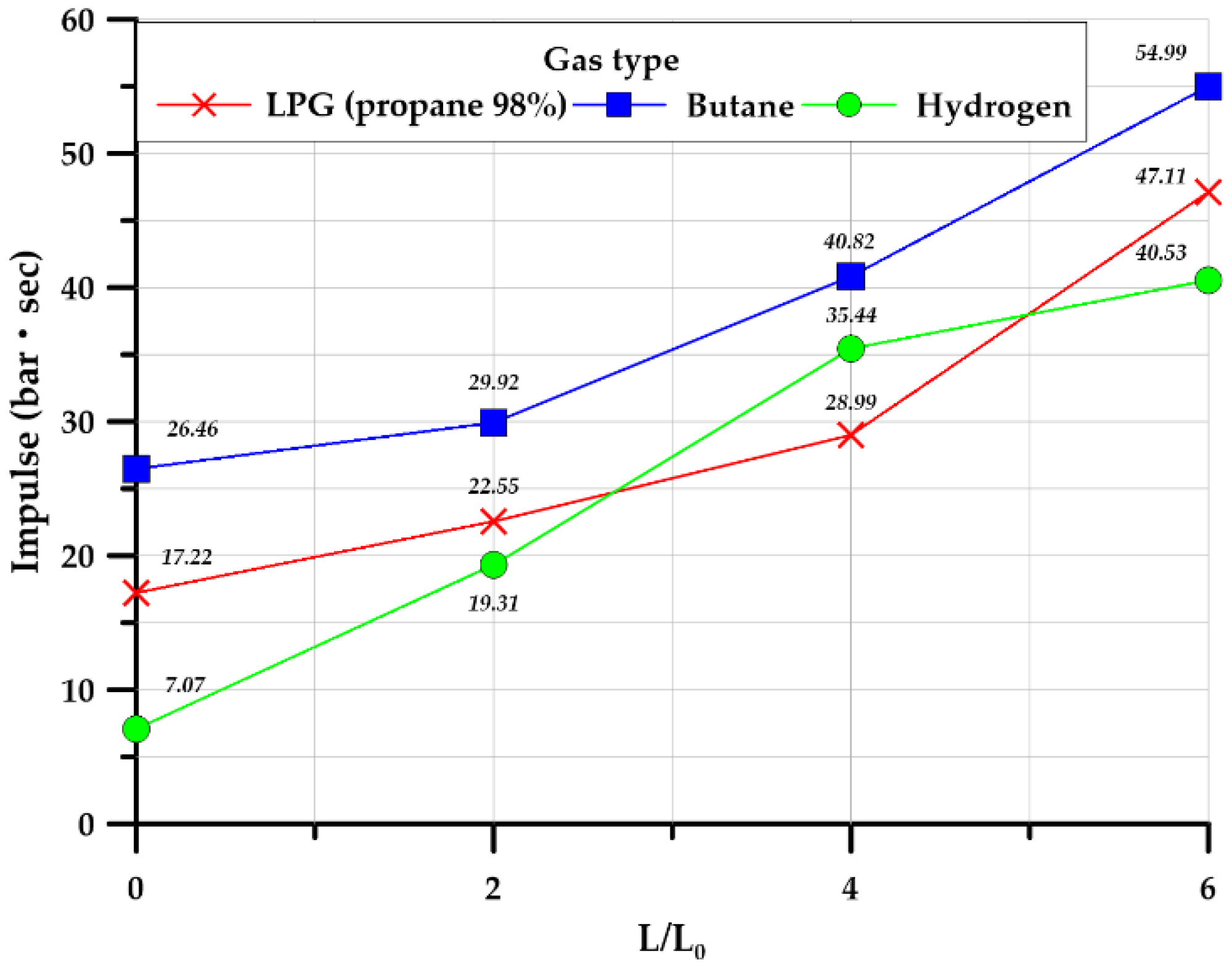
3.3.5. Impulse
4. Conclusions and Remarks
- (1)
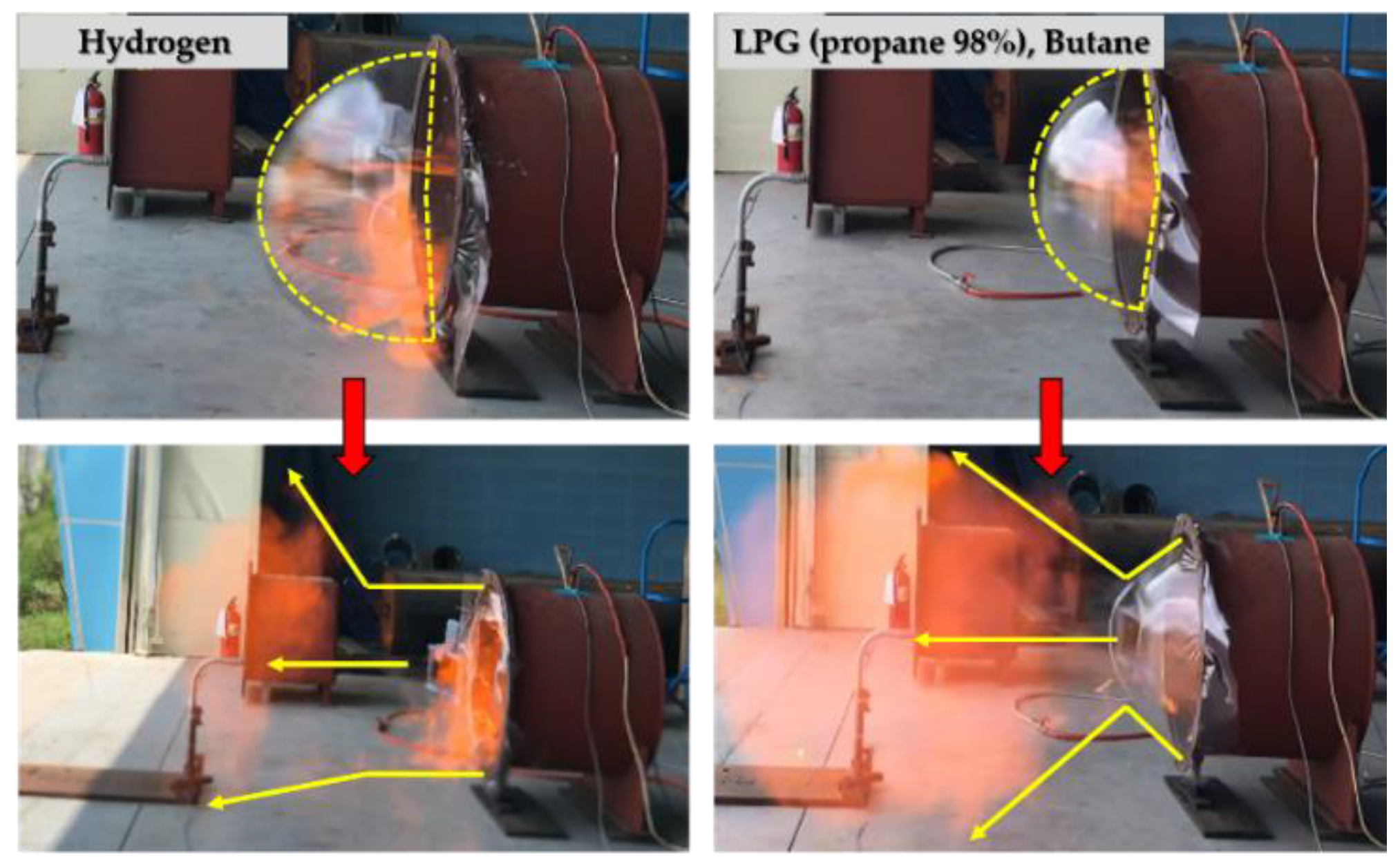
- The rupture shape varied depending on the type of combustible gas. This yielded different results from general experimental techniques because of the variable area on which the pressure was applied.
- (2)
- Hydrogen produced a shorter rising time and duration than the hydrocarbon-based gases considered; however, the explosive overpressure was higher than that of the hydrocarbon-based gases.
- (3)
- According to the conventional view, in a tunnel in which the explosion overpressure can move following an explosion in the explosion chamber, the explosion overpressure tends to increase as the tunnel length–explosion chamber length ratio increases. However, in the case of the cylindrical shape in this study, the pressure inside the explosion chamber was highest at a 4.0 length ratio, but the lowest explosion overpressure was observed at a length ratio of 6.0. For the square-type explosion chamber, as the length ratio increased, the internal explosion overpressure tended to increase. Butane, which had a relatively long duration, showed the highest impact. For a tunnel structure in which shock waves and flames can propagate after an explosion-induced structural rupture, the maximum overpressure and impact of the explosion increase, and the rising time and duration tend to be maintained or decreased as a function of explosion safety based on the design parameters of tunnel geometry.
- (4)
- To utilize hydrogen as a fuel for ships, it is necessary to consider a safety design based on hydrogen explosion load profiles to prevent structural damage, loss of property, and threat to human life. These experimental results are especially useful for developing new hydrogen fuel ship codes and design guidance against the existing IGF and IGC.
- (5)
- This study aimed to confirm the characteristics of internal and external pressures during explosions in cylindrical and square exhaust vents. The results can serve as a reference for structural designs of validation tunnels and surrounding ship structures considering the explosion characteristics of exhaust ports. The study also aimed to establish an experimental database to guide the estimation of the length of validation tunnels through analysis of the effects of tunnel length on the pressure characteristics at the end of the tunnels. Using CFD and experimental investigations, future research should investigate the explosion-proof characteristics of the fire-extinguishing area to develop measures to reduce explosion-induced damage.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wähner, A.; Gramse, G.; Langer, T.; Beyer, B. Determination of the minimum ignition energy on the basis of a statistical approach. J. Loss Prev. Process Ind. 2013, 26, 1655–1660. [Google Scholar] [CrossRef]
- Le, H.; Liu, Y.; Mannan, M.S. Lower flammability limits of hydrogen and light hydrocarbons at sub-atmospheric pressures. Ind. Eng. Chem. Res. 2013, 52, 1372–1378. [Google Scholar] [CrossRef]
- Kuznetsov, M.; Kobelt, S.; Grune, J.; Jordan, T. Flammability limits and laminar flame speed of hydrogen-air mixtures at subatmospheric pressures. Int. J. Hydrogen Energy 2012, 37, 17580–17588. [Google Scholar] [CrossRef]
- Piqueras, C.M.; García-Serna, J.; Cocero, M.J. Estimation of lower flammability limits in high-pressure systems. Application to the direct synthesis of hydrogen peroxide using supercritical and near-critical CO2 and air as diluents. J. Supercrit. Fluids 2011, 56, 33–40. [Google Scholar] [CrossRef]
- Zlochower, I.A.; Green, G.M. The limiting oxygen concentration and flammability limits of gases and gas mixtures. J. Loss Prev. Process Ind. 2009, 22, 499–505. [Google Scholar] [CrossRef]
- Wierzba, I.; Kilchyk, V. Flammability limits of hydrogen carbon monoxide mixtures at moderately elevated temperatures. Int. J. Hydrogen Energy 2001, 26, 639–643. [Google Scholar] [CrossRef]
- Shrestha, S.O.B.; Wierzba, I.; Karim, G.A. Prediction of the extent of diluents concentrations in flammability limited gaseous fuel-diluent mixtures in air. Appl. Therm. Eng. 2009, 29, 2574–2578. [Google Scholar] [CrossRef]
- Li, G.; Wu, J.; Wang, S.; Bai, J.; Wu, D.; Qi, S. Effects of gas concentration and obstacle location on overpressure and flame propagation characteristics of hydrocarbon fuel-air explosion in a semi-confined pipe. Fuel 2021, 285, 119268. [Google Scholar] [CrossRef]
- Bauwens, C.R.; Chaffee, J.; Dorofeev, S.B. Vented explosion overpressures from combustion of hydrogen and hydrocarbon mixtures. Int. J. Hydrogen Energy 2011, 36, 2329–2336. [Google Scholar] [CrossRef]
- Mukhim, E.D.; Abbasi, T.; Tauseef, S.M.; Abbasi, S.A. A method for the estimation of overpressure generated by open air hydrogen explosions. J. Loss Prev. Process Ind. 2018, 52, 99–107. [Google Scholar] [CrossRef]
- Bao, Q.; Fang, Q.; Zhang, Y.; Chen, L.; Yang, S.; Li, Z. Effects of gas concentration and venting pressure on overpressure transients during vented explosion of methane–air mixtures. Fuel 2016, 175, 40–48. [Google Scholar] [CrossRef]
- Li, P.; Huang, P.; Liu, Z.; Du, B.; Li, M. Experimental study on vented explosion overpressure of methane/air mixtures in manhole. J. Hazard. Mater. 2019, 374, 349–355. [Google Scholar] [CrossRef] [PubMed]
- Cao, Y.; Wang, Y.; Song, X.; Xing, H.; Li, B.; Xie, L. External overpressure of vented hydrogen-air explosion in the tube. Int. J. Hydrogen Energy 2019, 44, 2343–32350. [Google Scholar] [CrossRef]
- Zhu, Y.; Wang, D.; Shao, Z.; Zhu, X.; Xu, C.; Zhang, Y. Investigation on the overpressure of methane-air mixture gas explosions in straight large-scale tunnels. Process Saf. Environ. Prot. 2020, 135, 101–112. [Google Scholar] [CrossRef]
- Liu, C.; Liao, Y.; Yang, W.; Yin, Y.; Wang, Y.; Cui, G.; Li, Y. Estimation of explosion overpressure associated with background leakage in natural gas pipelines. J. Nat. Gas Sci. Eng. 2021, 89, 103883. [Google Scholar] [CrossRef]
- Meng, Q.; Wu, C.; Li, J.; Wu, P.; Xu, S.; Wang, Z. A study of pressure characteristics of methane explosion in a 20 m buried tunnel and influence on structural behaviour of concrete elements. Eng. Fail. Anal. 2021, 122, 105273. [Google Scholar] [CrossRef]
- Li, Z.; Chen, L.; Yan, H.; Fang, Q.; Zhang, Y.; Xiang, H.; Liu, Y.; Wang, S. Gas explosions of methane-air mixtures in a large-scale tube. Fuel 2021, 285, 119239. [Google Scholar] [CrossRef]
- Wang, C.; Guo, J.; Zhang, K.; Du, S.; Chen, H.; Yang, F. Experiments on duct-vented explosion of hydrogen–methane–air mixtures: Effects of equivalence ratio. Fuel 2022, 308, 122060. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, Q.; Li, M.; Yuan, M.; Wu, D.; Qian, X. Experimental study on explosion characteristics of DME-blended LPG mixtures in a closed vessel. Fuel 2019, 248, 232–240. [Google Scholar] [CrossRef]
- Lee, T.S.; Sung, J.Y.; Park, D.J. Experimental investigations on the deflagration explosion characteristics of different DME–LPG mixtures. Fire Saf. J. 2012, 49, 62–66. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, D. Comparison of the explosion characteristics of hydrogen, propane, and methane clouds at the stoichiometric concentrations. Int. J. Hydrogen Energy 2017, 42, 14794–14808. [Google Scholar] [CrossRef]
- Li, D.; Zhang, Q.; Ma, Q.; Shen, S. Comparison of explosion characteristics between hydrogen/air and methane/air at the stoichiometric concentrations. Int. J. Hydrogen Energy 2015, 40, 8761–8768. [Google Scholar] [CrossRef]
- Wang, X.; Wang, C.; Fan, X.; Guo, F.; Zhang, Z. Effects of hydrogen concentration and film thickness on the vented explosion in a small obstructed rectangular container. Int. J. Hydrogen Energy 2019, 44, 22752–22759. [Google Scholar] [CrossRef]
- Sato, Y.; Iwabuchi, H.; Groethe, M.; Merilo, E.; Chiba, S. Experiments on hydrogen deflagration. J. Power Sources 2006, 159, 144–148. [Google Scholar] [CrossRef]
- Witkowski, A.; Rusin, A.; Majkut, M.; Stolecka, K. Analysis of compression and transport of the methane/hydrogen mixture in existing natural gas pipelines. Int. J. Press. Vessels Piping 2018, 166, 24–34. [Google Scholar] [CrossRef]
- Eckhoff, R.K. Explosion Hazard in the Process Industries, 1st ed.; Gulf Publishing Company: Houston, TX, USA, 2005; p. 27. [Google Scholar]
- Sochet, I. Blast effects of external explosions. In Eighth International Symposium on Hazards, Prevention, and Mitigation of Industrial Explosions; HAL Open Science: Yokohama, Japan, 2010; p. hal-00629253. [Google Scholar]
- PCB PIEZOTRONICS. Available online: https://www.pcb.com/ (accessed on 3 February 2022).
- Czujko, J. Design of Offshore Facilities to Resist Gas Explosion Hazard, 1st ed.; CorrOcean ASA: Sandvika, Norway, 2001. [Google Scholar]








| Manufacturing Company | PCB PIEZOTRONICS |
|---|---|
| Model | 113B27 (High frequency ICP® pressure sensor) |
| Measurement Range (for ±5 V output) | 100 psi |
| Useful Over range (for ±10 V output) | 200 psi |
| Sensitivity (±15%) | 50 mV/psi |
| Maximum Pressure | 1 kpsi |
| Resolution | 1 mpsi |
| Resonant Frequency | ≥500 kHz |
| Rise Time | ≤1.0 sec. |
| Low Frequency Response (−5%) | 0.5 Hz |
| Non-Linearity | ≤1.0% FS |
| Gas Type | Explosion Chamber Type (Cylinder and Square) | L/L0 (Length Ratio) | V (m3) | Concentration (%) |
|---|---|---|---|---|
| LPG (propane 98%) | Only chamber | 0 | 0.15 | 4.2–4.5 |
| Chamber + Tunnel 1 | 2 | 0.45 | ||
| Chamber + Tunnel 2 | 4 | 0.75 | ||
| Chamber + Tunnel 3 | 6 | 1.05 | ||
| Butane | Only chamber | 0 | 0.15 | 4.5–5.0 |
| Chamber + Tunnel 1 | 2 | 0.45 | ||
| Chamber + Tunnel 2 | 4 | 0.75 | ||
| Chamber + Tunnel 3 | 6 | 1.05 | ||
| Hydrogen | Only chamber | 0 | 0.15 | 45.0–50.0 |
| Chamber + Tunnel 1 | 2 | 0.45 | ||
| Chamber + Tunnel 2 | 4 | 0.75 | ||
| Chamber + Tunnel 3 | 6 | 1.05 |
| Gas Type | Area (cm2) | Average Area (cm2) |
|---|---|---|
| Butane | 234 | 302 |
| 384 | ||
| 288 | ||
| LPG (propane 98%) | 607 | 663 |
| 759 | ||
| 622 | ||
| Hydrogen | 1073 | 1108 |
| 1274 | ||
| 977 |
| Gas Type | Area (cm2) | Average Area (cm2) |
|---|---|---|
| Butane | 531 | 498 |
| 481 | ||
| 481 | ||
| LPG (propane 98%) | 458 | 587 |
| 535 | ||
| 768 | ||
| Hydrogen | 896 | 1062 |
| 1092 | ||
| 1196 |
| Cylinder-Type | Length Ratio | ||||||||||||
| 0 | 2 | 4 | 6 | ||||||||||
| LPG | C4H10 | H2 | LPG | C4H10 | H2 | LPG | C4H10 | H2 | LPG | C4H10 | H2 | ||
| Average overpressure (psi) | Inside | 2.50 | 1.72 | 4.62 | 2.50 | 1.88 | 42.58 | 12.27 | 7.67 | 59.14 | 1.65 | 0.67 | 6.99 |
| Outside | 0.37 | 0.28 | 1.29 | 1.57 | 0.26 | 23.41 | 1.73 | 1.14 | 28.62 | 0.35 | 0.07 | 4.17 | |
| Pressure ratio (%) | 14.82 | 16.28 | 28.05 | 12.65 | 14.01 | 54.97 | 14.13 | 14.86 | 48.39 | 20.97 | 10.95 | 59.75 | |
| Rising time (inside) (msec) | 50.99 | 134.47 | 13.15 | 67.78 | 109.37 | 16.49 | 58.64 | 148.61 | 17.62 | 47.44 | 229.86 | 14.11 | |
| Duration (inside) (msec) | 61.41 | 154.46 | 14.71 | 87.11 | 124.94 | 18.99 | 74.01 | 180.22 | 21.70 | 56.72 | 264.05 | 20.09 | |
| Impulse (inside) (bar·sec) | 3.61 | 5.97 | 1.69 | 4.75 | 5.77 | 21.22 | 26.01 | 30.99 | 31.06 | 3.28 | 2.82 | 3.65 | |
| Square-Type | Length Ratio | ||||||||||||
| 0 | 2 | 4 | 6 | ||||||||||
| LPG | C4H10 | H2 | LPG | C4H10 | H2 | LPG | C4H10 | H2 | LPG | C4H10 | H2 | ||
| Maximum overpressure (psi) | Inside | 12.98 | 9.77 | 20.94 | 12.88 | 10.68 | 34.88 | 14.66 | 15.09 | 59.74 | 26.91 | 20.06 | 67.69 |
| Outside | 1.31 | 0.92 | 4.98 | 0.97 | 1.22 | 24.67 | 1.96 | 1.46 | 8.34 | 1.02 | 1.05 | 11.65 | |
| Pressure ratio (%) | 10.11 | 9.41 | 23.78 | 7.51 | 11.39 | 70.74 | 13.39 | 9.67 | 13.96 | 3.78 | 5.25 | 17.21 | |
| Rising time (inside) (msec) | 41.99 | 123.89 | 11.66 | 55.05 | 118.28 | 15.52 | 59.16 | 141.72 | 16.65 | 80.75 | 164.47 | 16.54 | |
| Duration (inside) (msec) | 53.86 | 136.72 | 14.35 | 74.94 | 138.34 | 18.35 | 79.69 | 148.37 | 21.29 | 92.76 | 174.61 | 20.53 | |
| Impulse (inside) (bar·sec) | 17.22 | 26.46 | 7.07 | 22.55 | 29.92 | 19.31 | 28.99 | 40.82 | 35.44 | 47.11 | 54.99 | 40.57 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, S.W.; Kim, J.H.; Seo, J.K. Explosion Characteristics of Hydrogen Gas in Varying Ship Ventilation Tunnel Geometries: An Experimental Study. J. Mar. Sci. Eng. 2022, 10, 532. https://doi.org/10.3390/jmse10040532
Park SW, Kim JH, Seo JK. Explosion Characteristics of Hydrogen Gas in Varying Ship Ventilation Tunnel Geometries: An Experimental Study. Journal of Marine Science and Engineering. 2022; 10(4):532. https://doi.org/10.3390/jmse10040532
Chicago/Turabian StylePark, Soung Woo, Jeong Hwan Kim, and Jung Kwan Seo. 2022. "Explosion Characteristics of Hydrogen Gas in Varying Ship Ventilation Tunnel Geometries: An Experimental Study" Journal of Marine Science and Engineering 10, no. 4: 532. https://doi.org/10.3390/jmse10040532
APA StylePark, S. W., Kim, J. H., & Seo, J. K. (2022). Explosion Characteristics of Hydrogen Gas in Varying Ship Ventilation Tunnel Geometries: An Experimental Study. Journal of Marine Science and Engineering, 10(4), 532. https://doi.org/10.3390/jmse10040532







