Relationship of the Quanta-to-Energy Ratio of Photosynthetically Active Radiation with Chlorophyll-a in Case I Seawater
Abstract
1. Introduction
2. Materials and Methods
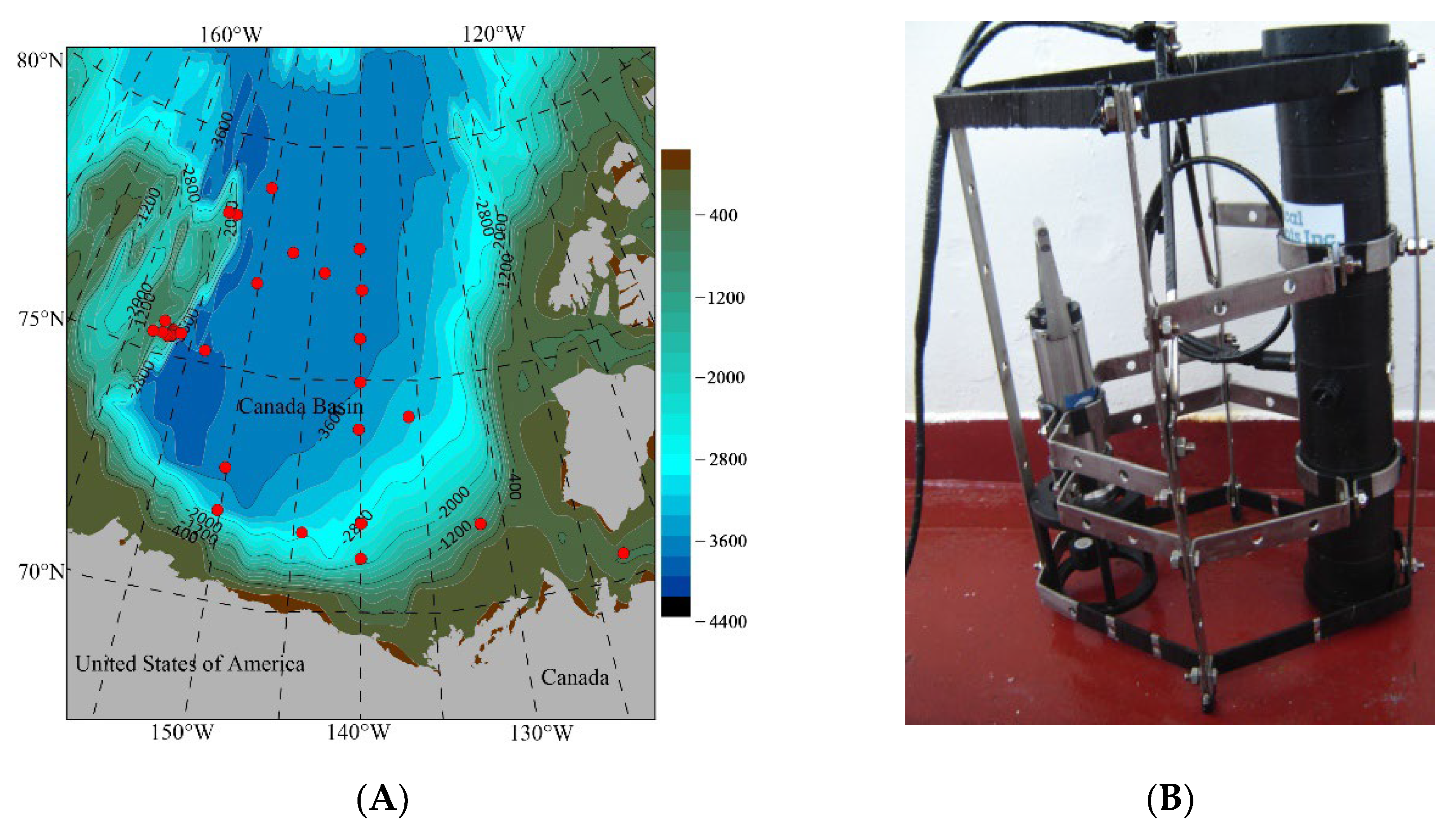
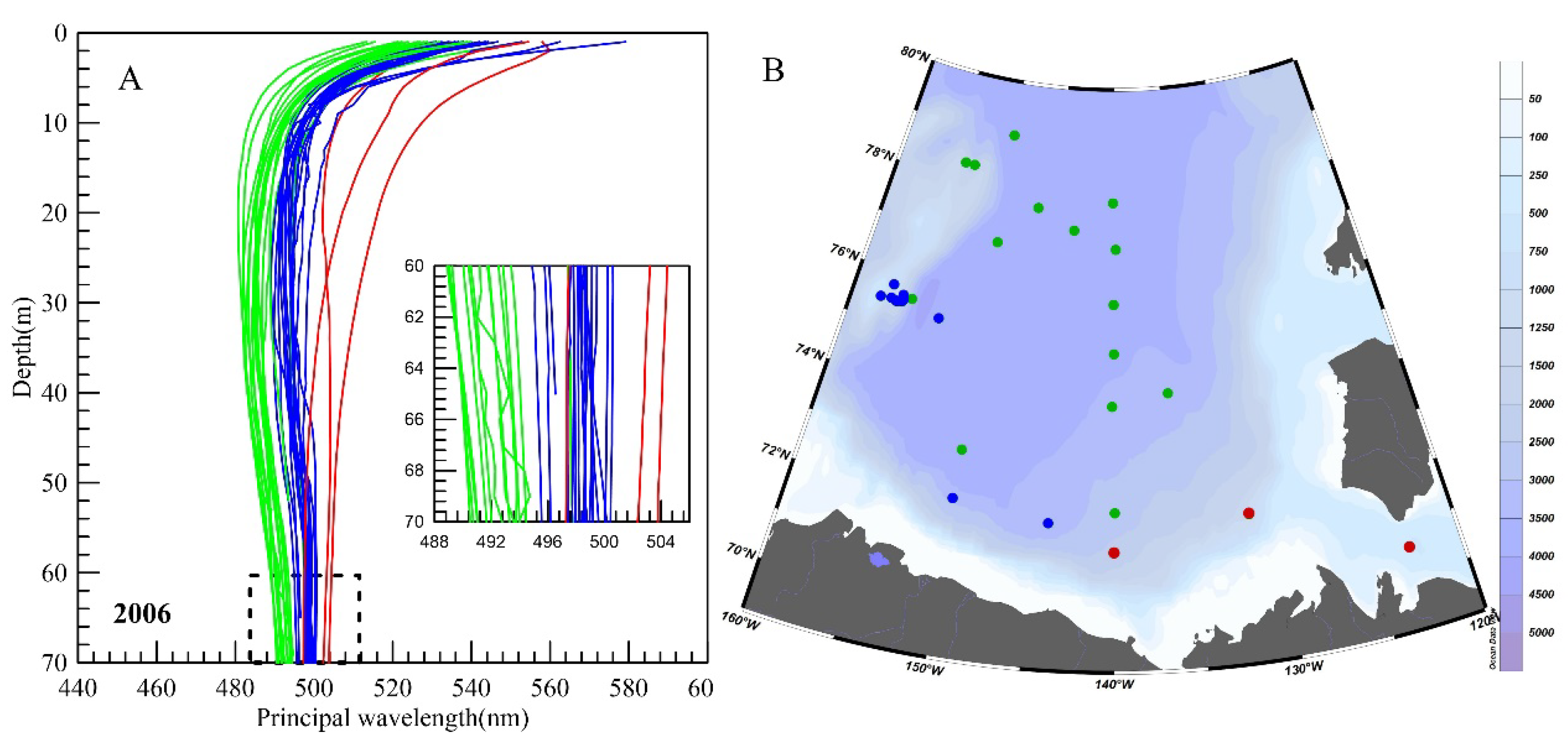
2.1. Materials
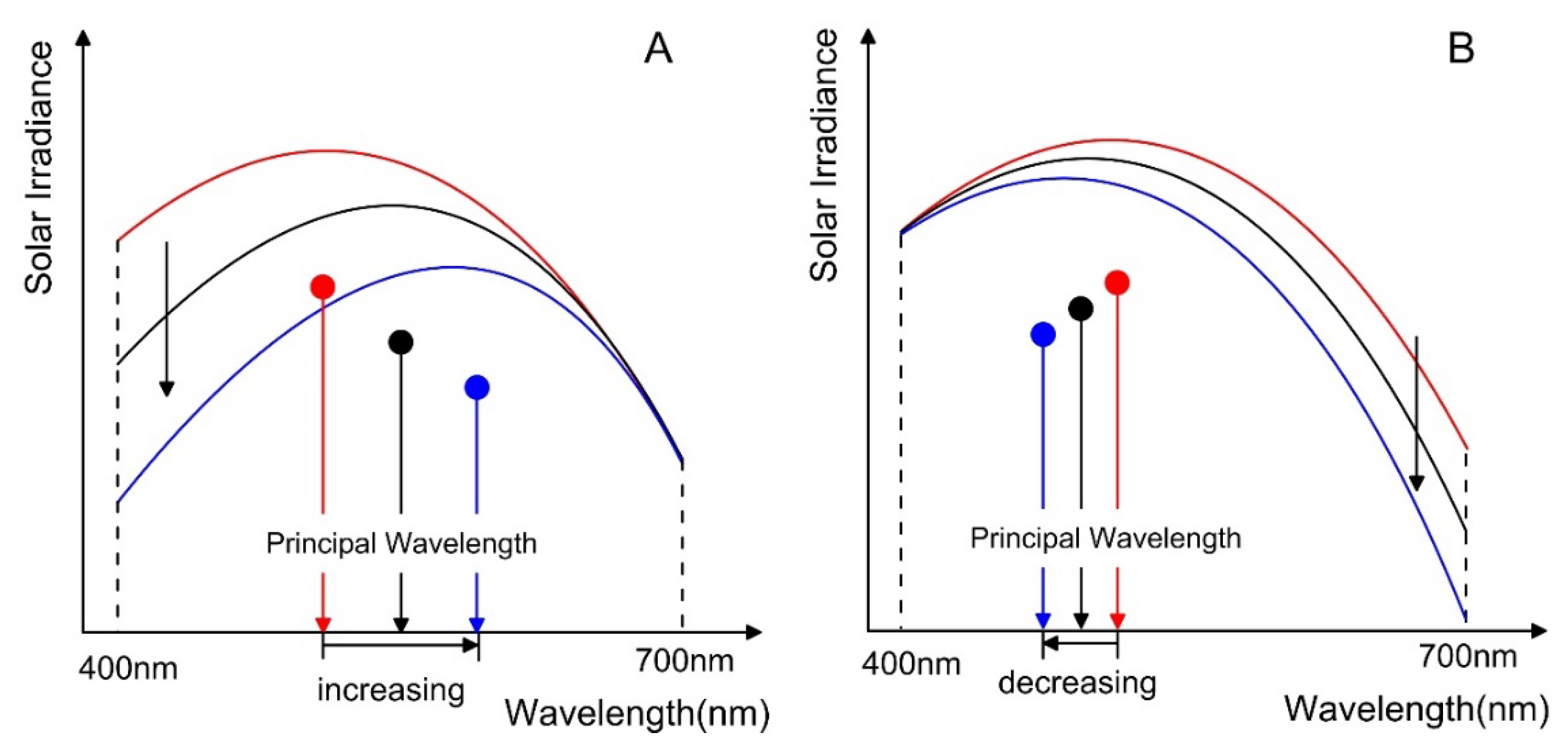
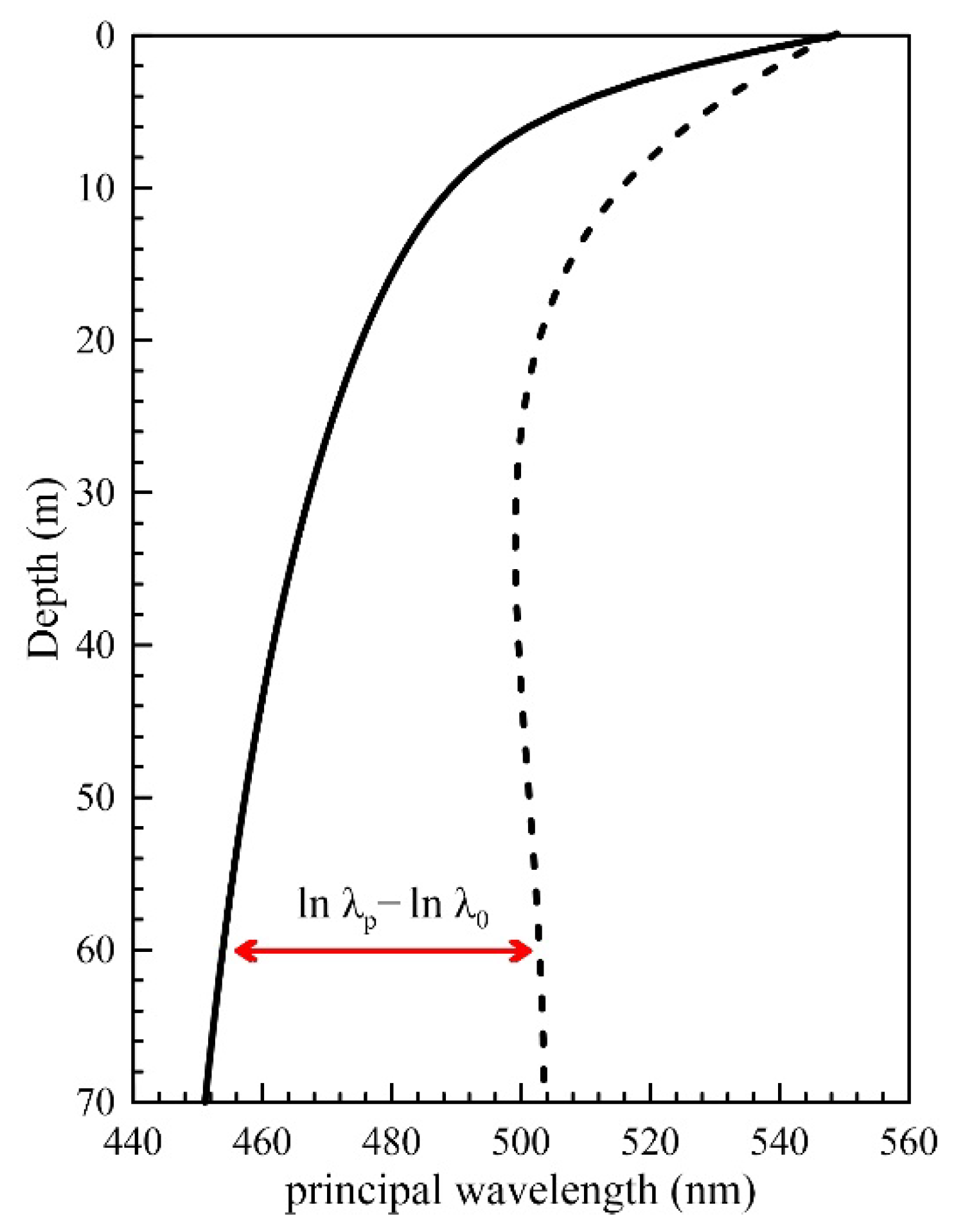
2.2. Principal Wavelength
3. Theoretical Analysis of
3.1. Exponential Function of
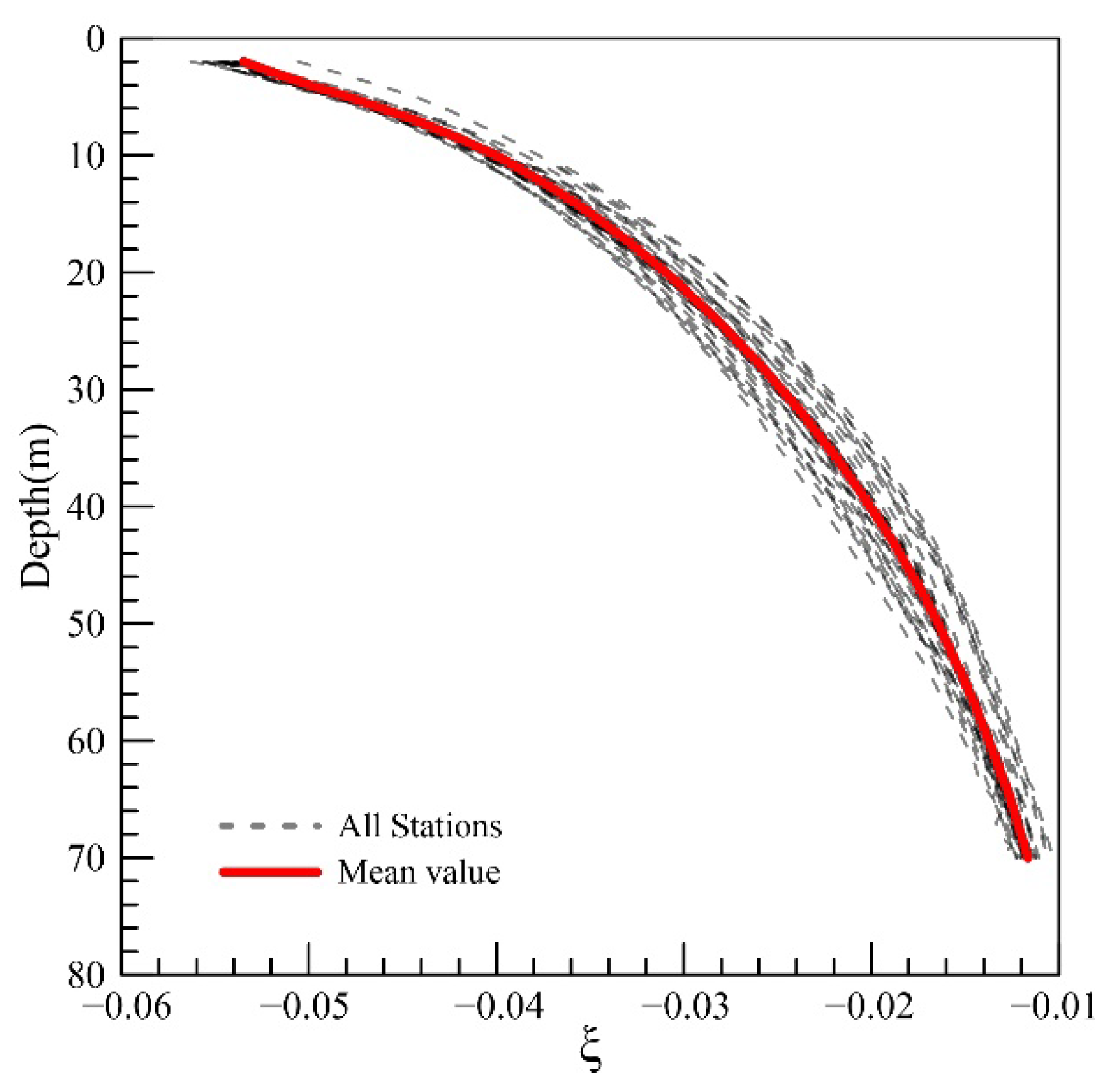
3.2. The Deviation of the
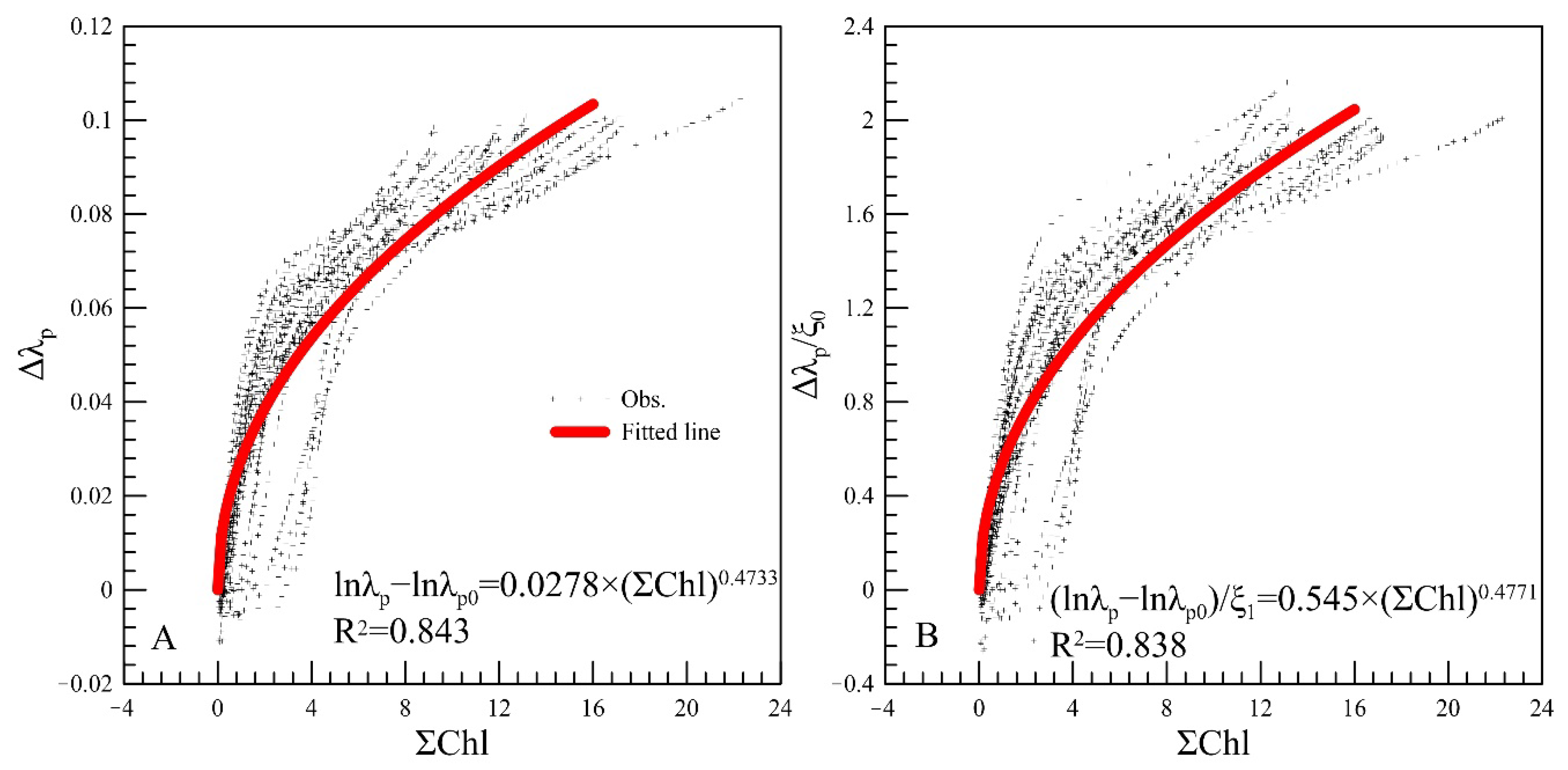
3.3. The Semi-Empirical Formula between and Chlorophyll-a Concentration
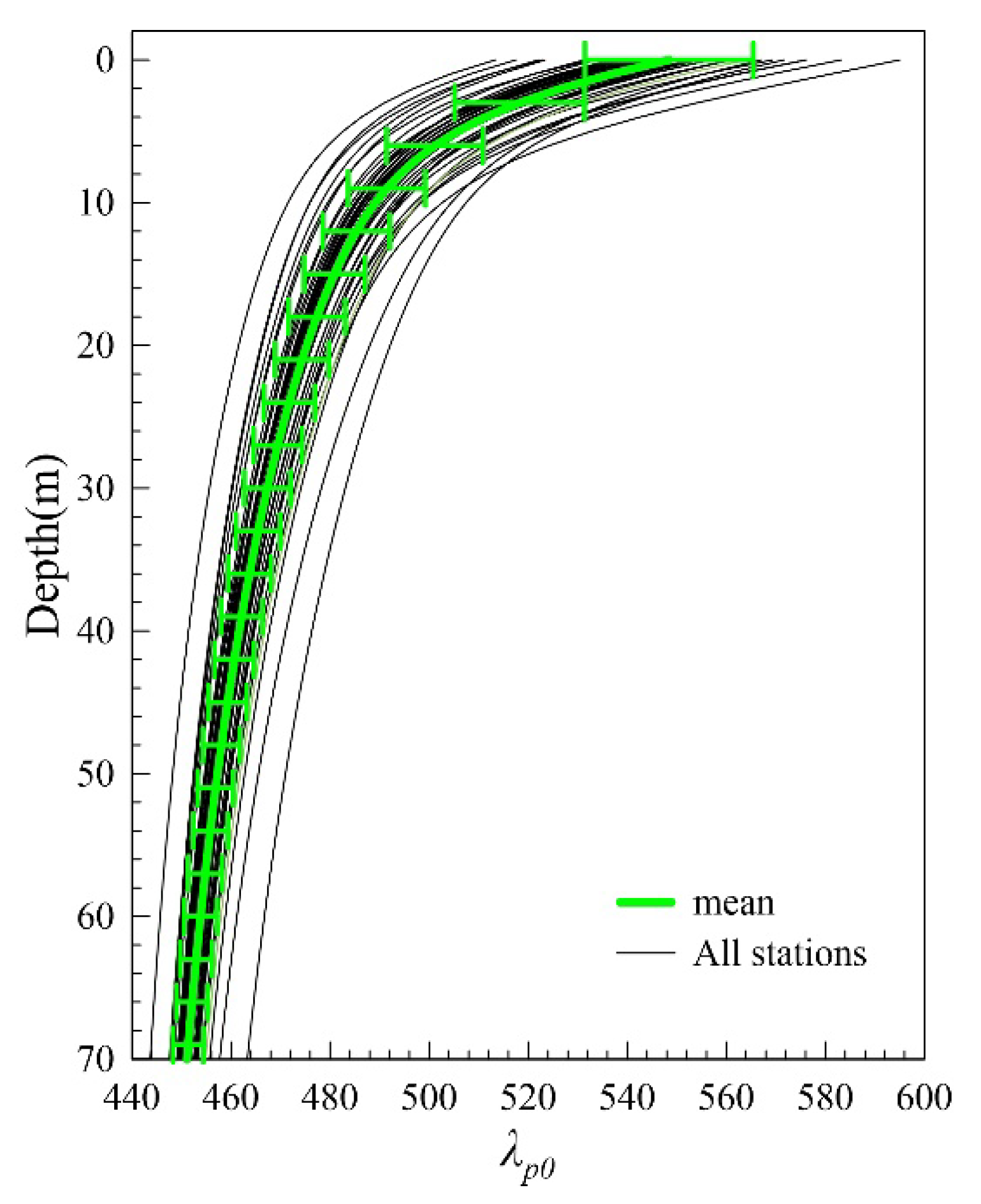
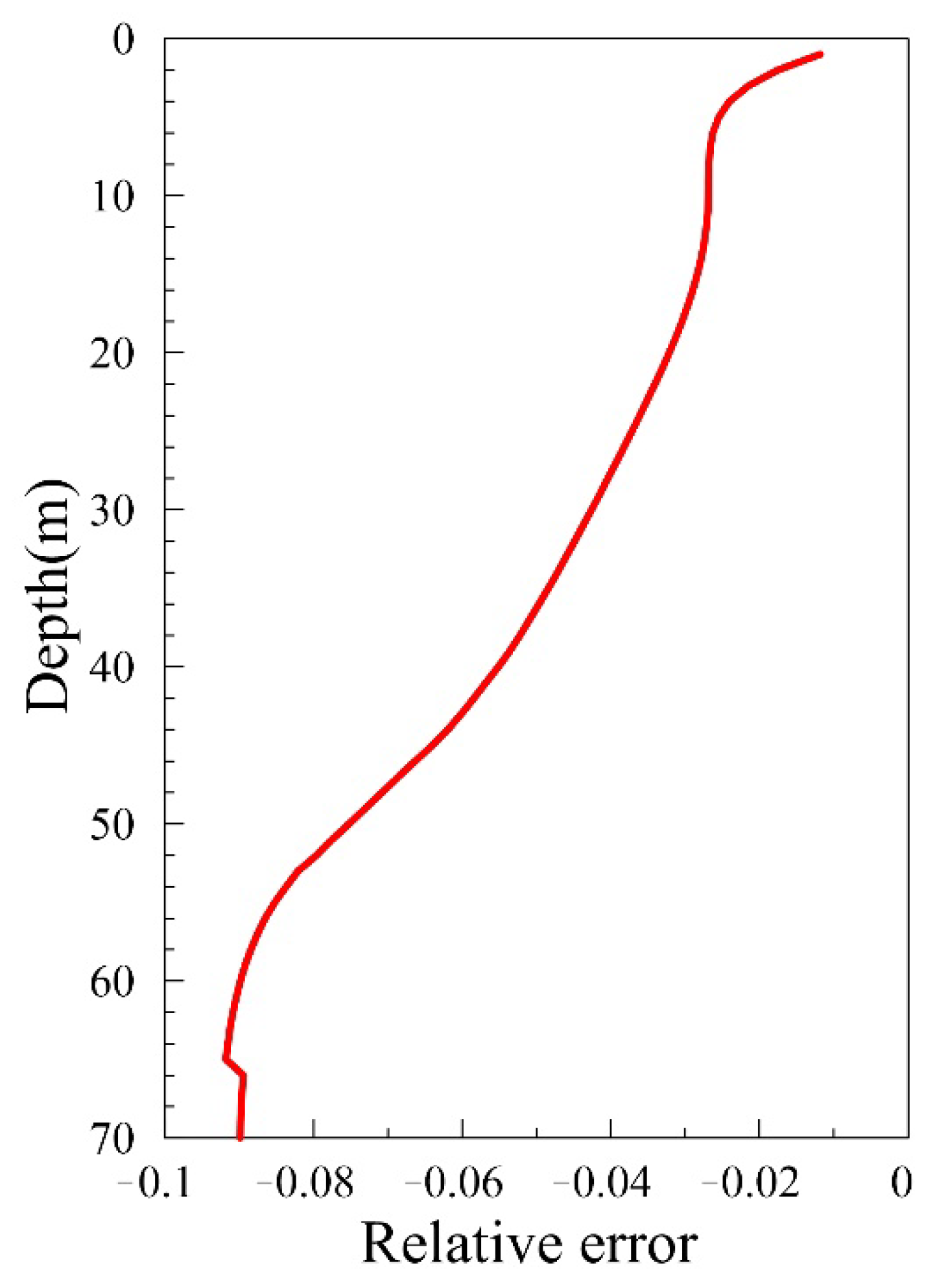
3.4. The Ideal Profile of for Pure Seawater
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhao, J.; Wang, W.; Cooper, L. Calculation of Photosynthetically Available Radiation Using Multispectral Data in the Arctic. Chin. J. Polar Res. 2010, 22, 91–103. [Google Scholar] [CrossRef]
- Alados, I.; Foyo-Moreno, I.; Alados-Arboledas, L. Photosynthetically Active Radiation: Measurements and Modelling. Agric. For. Meteorol. 1996, 78, 121–131. [Google Scholar] [CrossRef]
- Frouin, R.; Lingner, D.W.; Gautier, C.; Baker, K.S.; Smith, R.C. A Simple Analytical Formula to Compute Clear Sky Total and Photosynthetically Available Solar Irradiance at the Ocean Surface. J. Geophys. Res. Ocean. 1989, 94, 9731–9742. [Google Scholar] [CrossRef]
- Emmanuel, D.; Phillipe, D.; Malik, C. Radiative Transfer Code: Application to the Calculation of PAR. J. Earth Syst. Sci. 2000, 109, 407–413. [Google Scholar] [CrossRef]
- Akitsu, T.K.; Nasahara, K.N.; Ijima, O.; Hirose, Y.; Ide, R.; Takagi, K.; Kume, A. The Variability and Seasonality in the Ratio of Photosynthetically Active Radiation to Solar Radiation: A Simple Empirical Model of the Ratio. Int. J. Appl. Earth Obs. Geoinf. 2022, 108, 102724. [Google Scholar] [CrossRef]
- Zhou, H.; Yue, X.; Lei, Y.; Zhang, T.; Tian, C.; Ma, Y.; Cao, Y. Responses of Gross Primary Productivity to Diffuse Radiation at Global FLUXNET Sites. Atmos. Environ. 2021, 244, 117905. [Google Scholar] [CrossRef]
- Huang, G.; Li, X.; Lu, N.; Wang, X.; He, T. A General Parameterization Scheme for the Estimation of Incident Photosynthetically Active Radiation under Cloudy Skies. IEEE Trans. Geosci. Remote Sens. 2020, 58, 6255–6265. [Google Scholar] [CrossRef]
- Mercado, L.M.; Bellouin, N.; Sitch, S.; Boucher, O.; Huntingford, C.; Wild, M.; Cox, P.M. Impact of Changes in Diffuse Radiation on the Global Land Carbon Sink. Nature 2009, 458, 1014–1017. [Google Scholar] [CrossRef]
- Ren, X.; He, H.; Zhang, L.; Yu, G. Global Radiation, Photosynthetically Active Radiation, and the Diffuse Component Dataset of China, 1981–2010. Earth Syst. Sci. Data 2018, 10, 1217–1226. [Google Scholar] [CrossRef]
- Alados, I.; Olmo, F.J.; Foyo-Moreno, I.; Alados-Arboledas, L. Estimation of Photosynthetically Active Radiation under Cloudy Conditions. Agric. For. Meteorol. 2000, 102, 39–50. [Google Scholar] [CrossRef]
- Sasai, T.; Ichii, K.; Yamaguchi, Y.; Nemani, R. Simulating Terrestrial Carbon Fluxes Using the New Biosphere Model “Biosphere Model Integrating Eco-Physiological and Mechanistic Approaches Using Satellite Data” (BEAMS). J. Geophys. Res. Biogeosci. 2005, 110. [Google Scholar] [CrossRef]
- Zhao, M.; Running, S.W.; Nemani, R.R. Sensitivity of Moderate Resolution Imaging Spectroradiometer (MODIS) Terrestrial Primary Production to the Accuracy of Meteorological Reanalyses. J. Geophys. Res. Biogeosci. 2006, 111. [Google Scholar] [CrossRef]
- Akitsu, T.; Kume, A.; Hirose, Y.; Ijima, O.; Nasahara, K.N. On the Stability of Radiometric Ratios of Photosynthetically Active Radiation to Global Solar Radiation in Tsukuba, Japan. Agric. For. Meteorol. 2015, 209–210, 59–68. [Google Scholar] [CrossRef]
- González, J.A.; Calbó, J. Modelled and Measured Ratio of PAR to Global Radiation under Cloudless Skies. Agric. For. Meteorol. 2002, 110, 319–325. [Google Scholar] [CrossRef]
- Moon, P. Proposed Standard Solar-Radiation Curves for Engineering Use. J. Franklin Inst. 1940, 230, 583–617. [Google Scholar] [CrossRef]
- Mizoguchi, Y.; Yasuda, Y.; Ohtani, Y.; Watanabe, T.; Kominami, Y.; Yamanoi, K. A Practical Model to Estimate Photosynthetically Active Radiation Using General Meteorological Elements in a Temperate Humid Area and Comparison among Models. Theor. Appl. Climatol. 2014, 115, 583–589. [Google Scholar] [CrossRef]
- Foyo-Moreno, I.; Alados, I.; Alados-Arboledas, L. A New Conventional Regression Model to Estimate Hourly Photosynthetic Photon Flux Density under All Sky Conditions. Int. J. Climatol. 2017, 37, 1067–1075. [Google Scholar] [CrossRef]
- Aguiar, L.J.G.; Fischer, G.R.; Ladle, R.J.; Malhado, A.C.M.; Justino, F.B.; Aguiar, R.G.; da Costa, J.M.N. Modeling the Photosynthetically Active Radiation in South West Amazonia under All Sky Conditions. Theor. Appl. Climatol. 2012, 108, 631–640. [Google Scholar] [CrossRef]
- Meek, D.W.; Hatfield, J.L.; Howell, T.A.; Idso, S.B.; Reginato, R.J. Generalized Relationship between Photosynthetically Active Radiation and Solar Radiation. Agron. J. 1984, 76, 939–945. [Google Scholar] [CrossRef]
- Ge, S.; Smith, R.G.; Jacovides, C.P.; Kramer, M.G.; Carruthers, R.I. Dynamics of Photosynthetic Photon Flux Density (PPFD) and Estimates in Coastal Northern California. Theor. Appl. Climatol. 2011, 105, 107–118. [Google Scholar] [CrossRef]
- Udo, S.O.; Aro, T.O. Global PAR Related to Global Solar Radiation for Central Nigeria. Agric. For. Meteorol. 1999, 97, 21–31. [Google Scholar] [CrossRef]
- Li, R.; Zhao, L.; Ding, Y.; Wang, S.; Ji, G.; Xiao, Y.; Liu, G.; Sun, L. Monthly Ratios of PAR to Global Solar Radiation Measured at Northern Tibetan Plateau, China. Sol. Energy 2010, 84, 964–973. [Google Scholar] [CrossRef]
- McCree, K.J. Test of current definitions of photosynthetically active radiation against leaf photosynthesis data. Agric. Meteorol. 1972, 10, 443–453. [Google Scholar] [CrossRef]
- Morel, A.; Smith, R.C. Relation between Total Quanta and Total Energy for Aquatic Photosynthesis. Limnol. Oceanogr. 1974, 19, 591–600. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, W.; Kang, S.H.; Yang, E.J.; Kim, T.W. Optical Properties in Waters around the Mendeleev Ridge Related to the Physical Features of Water Masses. Deep. Sea Res. Part II Top. Stud. Oceanogr. 2015, 120, 43–51. [Google Scholar] [CrossRef]
- Wang, W.; Zhao, J. Variation of Diffuse Attenuation Coefficient of Downwelling Irradiance in the Arctic Ocean. Acta Oceanol. Sin. 2014, 33, 53–62. [Google Scholar] [CrossRef]
- Chang, G.C.; Dickey, T.D. Coastal Ocean Optical Influences on Solar Transmission and Radiant Heating Rate. J. Geophys. Res. Ocean. 2004, 109, C01020. [Google Scholar] [CrossRef]
- Reinart, A.; Arst, H.; Blanco-Sequeiros, A.; Herlevi, A. Relation between Underwater Irradiance and Quantum Irradiance in Dependence on Water Transparency at Different Depths in the Water Bodies. J. Geophys. Res. Ocean. 1998, 103, 7749–7752. [Google Scholar] [CrossRef]
- Lavoie, D.; Denman, K.; Michel, C. Modeling Ice Algal Growth and Decline in a Seasonally Ice-Covered Region of the Arctic (Resolute Passage, Canadian Archipelago). J. Geophys. Res. Ocean. 2005, 110, 1–17. [Google Scholar] [CrossRef]
- Dye, D.G. Spectral Composition and Quanta-to-Energy Ratio of Diffuse Photosynthetically Active Radiation under Diverse Cloud Conditions. J. Geophys. Res. Atmos. 2004, 109, 1–12. [Google Scholar] [CrossRef]
- Lee, Z.P.; Du, K.P.; Arnone, R. A Model for the Diffuse Attenuation Coefficient of Downwelling Irradiance. J. Geophys. Res. C Ocean. 2005, 110, 1–10. [Google Scholar] [CrossRef]
- Lee, Z.P.; Darecki, M.; Carder, K.L.; Davis, C.O.; Stramski, D.; Rhea, W.J. Diffuse Attenuation Coefficient of Downwelling Irradiance: An Evaluation of Remote Sensing Methods. J. Geophys. Res. C Ocean. 2005, 110, 1–9. [Google Scholar] [CrossRef]
- Morel, A. Are the Empirical Relationships Describing the Bio-Optical Properties of Case 1 Waters Consistent and Internally Compatible? J. Geophys. Res. Ocean. 2009, 114, 1–15. [Google Scholar] [CrossRef]
- Austin, R.W.; Petzold, T.J. Spectral Dependence of the Diffuse Attenuation Coefficient of Light in Ocean Waters. Ocean. Optics. VII 1984, 489, 1689–1699. [Google Scholar] [CrossRef]
- Babin, M.; Arrigo, K.; Bélanger, S.; Forget, M.-H.; Frouin, R.; Hill, V.; Hirawake, T.; Matsuoka, A.; Mitchell, B.G.; Reynolds, R.A. Ocean Colour Remote Sensing in Polar Seas; International Ocean Colour Coordinating Group: Dartmouth, NS, Canada, 2015. [Google Scholar]
- Cota, G.F.; Harrison, W.G.; Platt, T.; Sathyendranath, S.; Stuart, V. Bio-Optical Properties of the Labrador Sea. J. Geophys. Res. Ocean. 2003, 108. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Zheng, J.; Jing, C.; Zhao, J. Relationship of the Quanta-to-Energy Ratio of Photosynthetically Active Radiation with Chlorophyll-a in Case I Seawater. J. Mar. Sci. Eng. 2022, 10, 2005. https://doi.org/10.3390/jmse10122005
Wang W, Zheng J, Jing C, Zhao J. Relationship of the Quanta-to-Energy Ratio of Photosynthetically Active Radiation with Chlorophyll-a in Case I Seawater. Journal of Marine Science and Engineering. 2022; 10(12):2005. https://doi.org/10.3390/jmse10122005
Chicago/Turabian StyleWang, Weibo, Jianhua Zheng, Chunsheng Jing, and Jinping Zhao. 2022. "Relationship of the Quanta-to-Energy Ratio of Photosynthetically Active Radiation with Chlorophyll-a in Case I Seawater" Journal of Marine Science and Engineering 10, no. 12: 2005. https://doi.org/10.3390/jmse10122005
APA StyleWang, W., Zheng, J., Jing, C., & Zhao, J. (2022). Relationship of the Quanta-to-Energy Ratio of Photosynthetically Active Radiation with Chlorophyll-a in Case I Seawater. Journal of Marine Science and Engineering, 10(12), 2005. https://doi.org/10.3390/jmse10122005






