Dynamic Responses of a Multilayered Transversely Isotropic Poroelastic Seabed Subjected to Ocean Waves and Currents
Abstract
1. Introduction
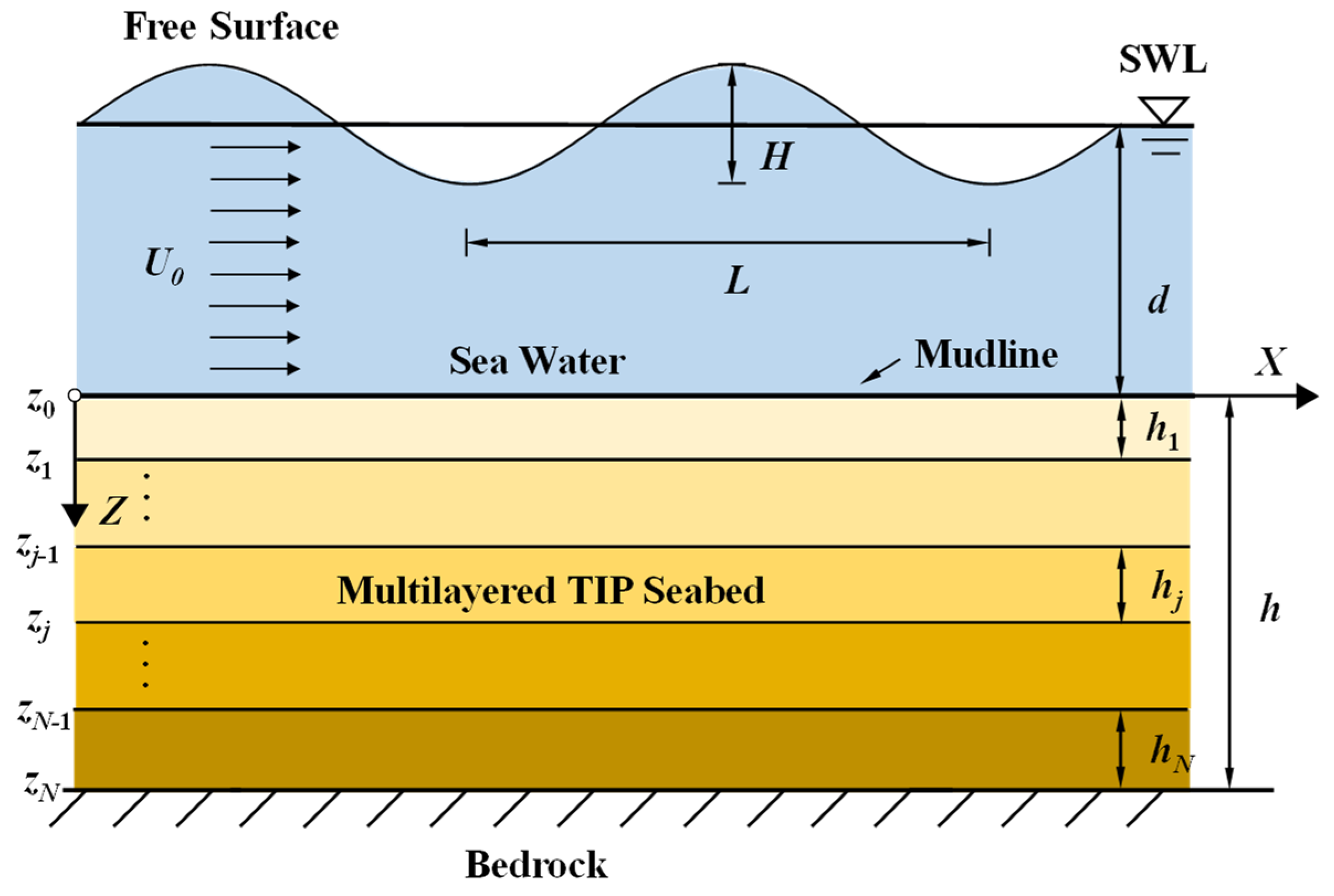
2. Seawater-Seabed-Bedrock System
2.1. Governing Equations
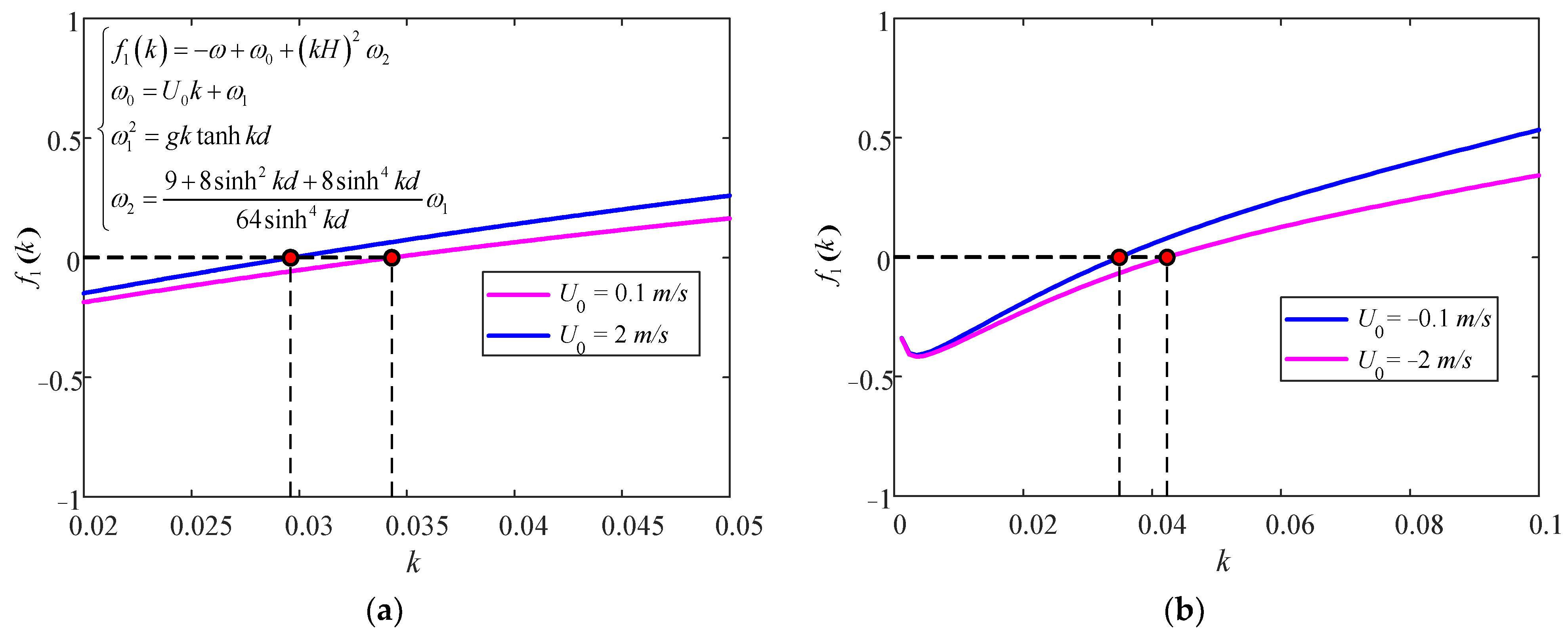
2.2. Wave-Current Interaction
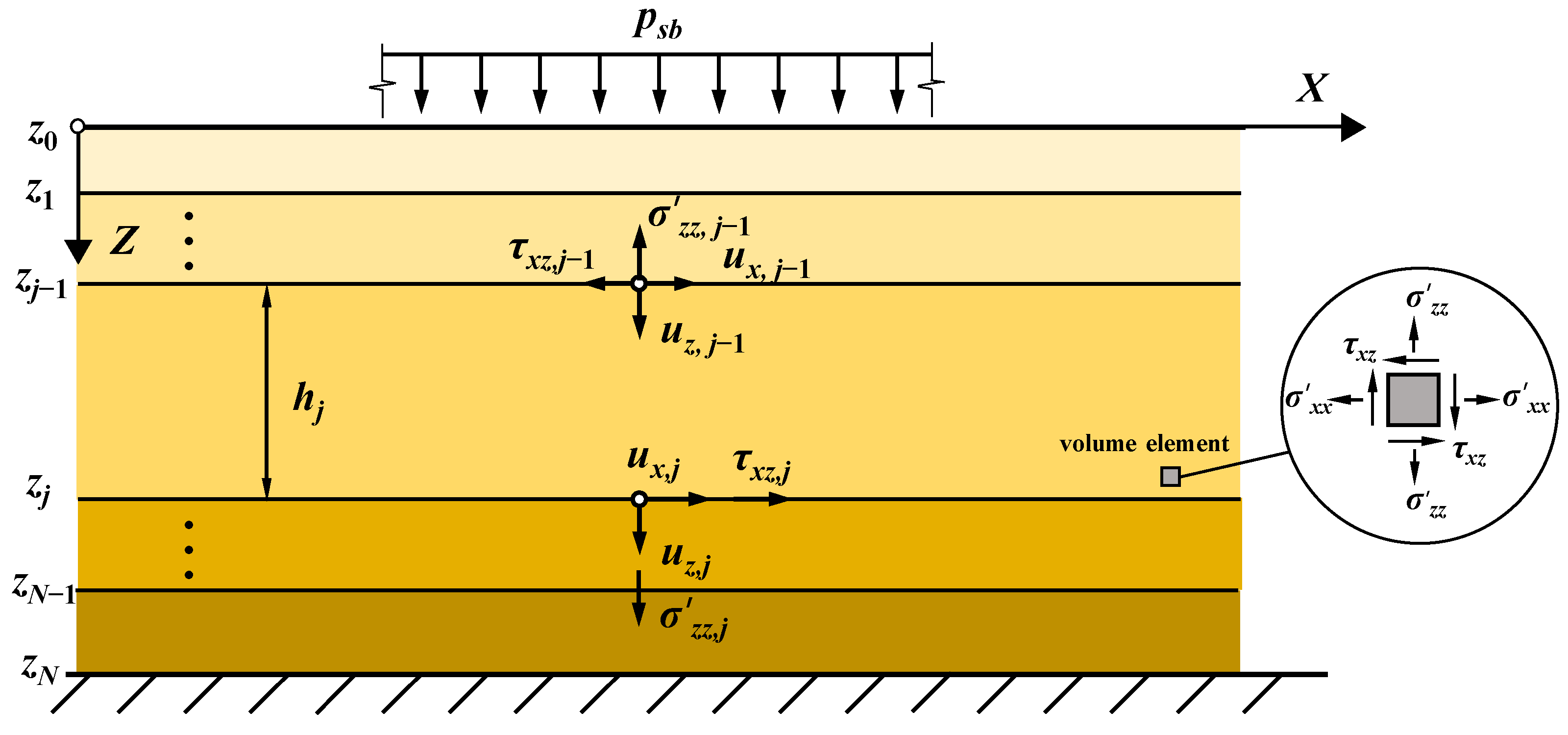
2.3. Boundary Conditions
3. Semi-Analytical Solution
3.1. General Solution to a TIP Layer
3.2. Dynamic Stiffness Matrix of TIP Multilayers
4. Verification of the Semi-Analytical Solution Based on Isotropic Layers
5. Semi-Analytical Solution Applied to TIP Layers and Parametric Study
5.1. Single-Layered Seabed
5.2. Multilayered Seabed
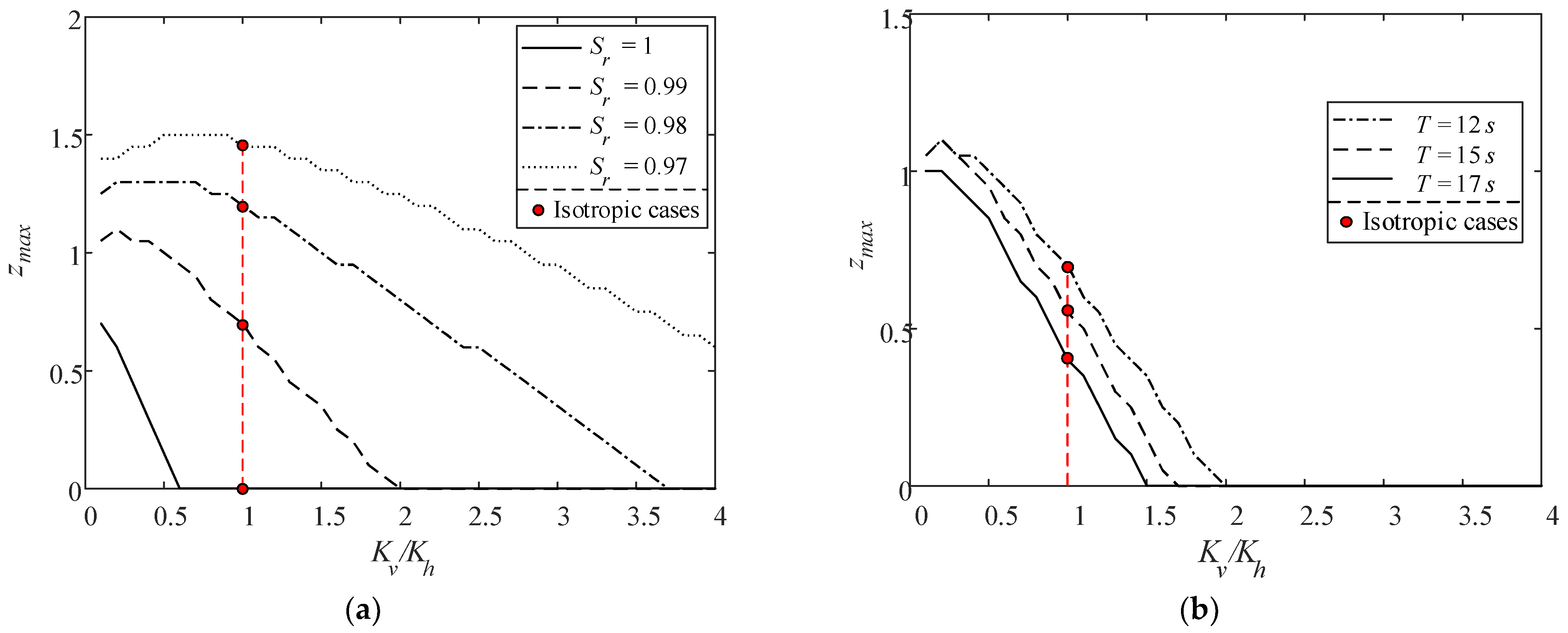
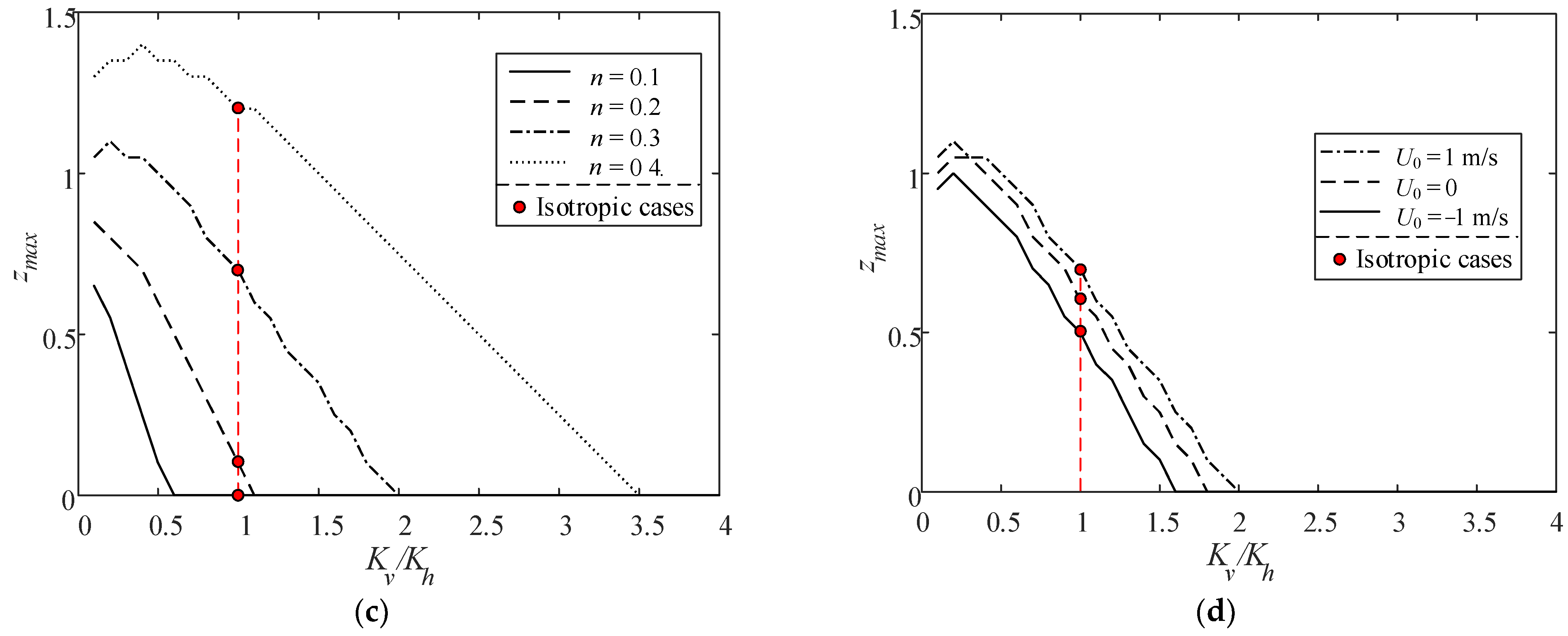
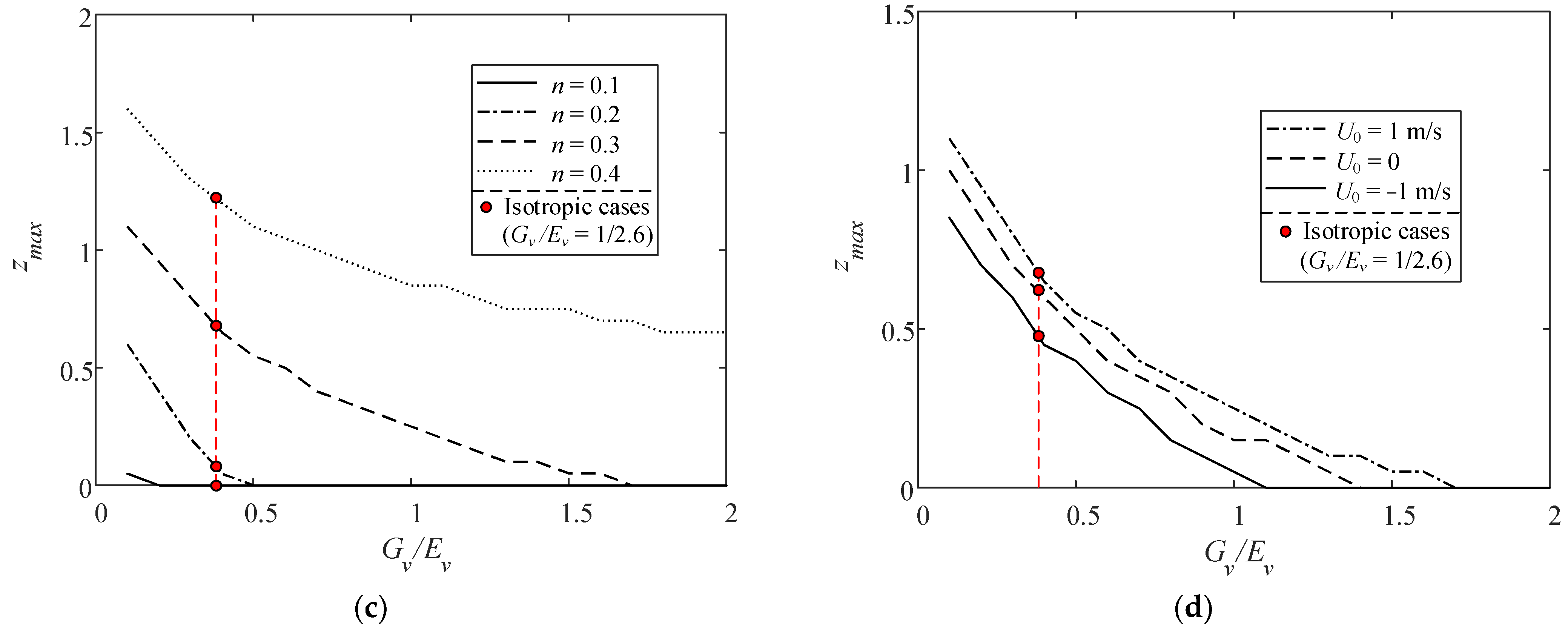
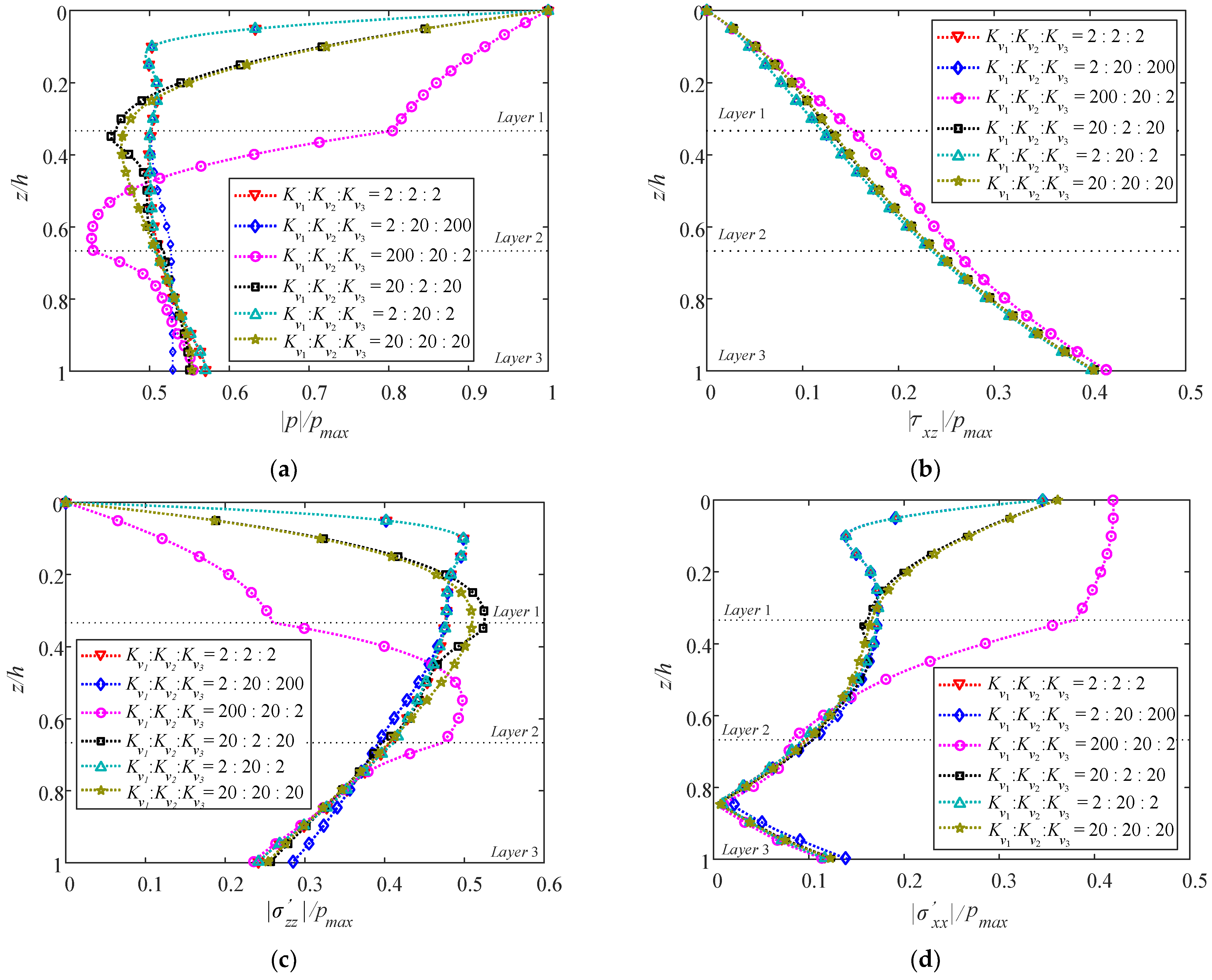
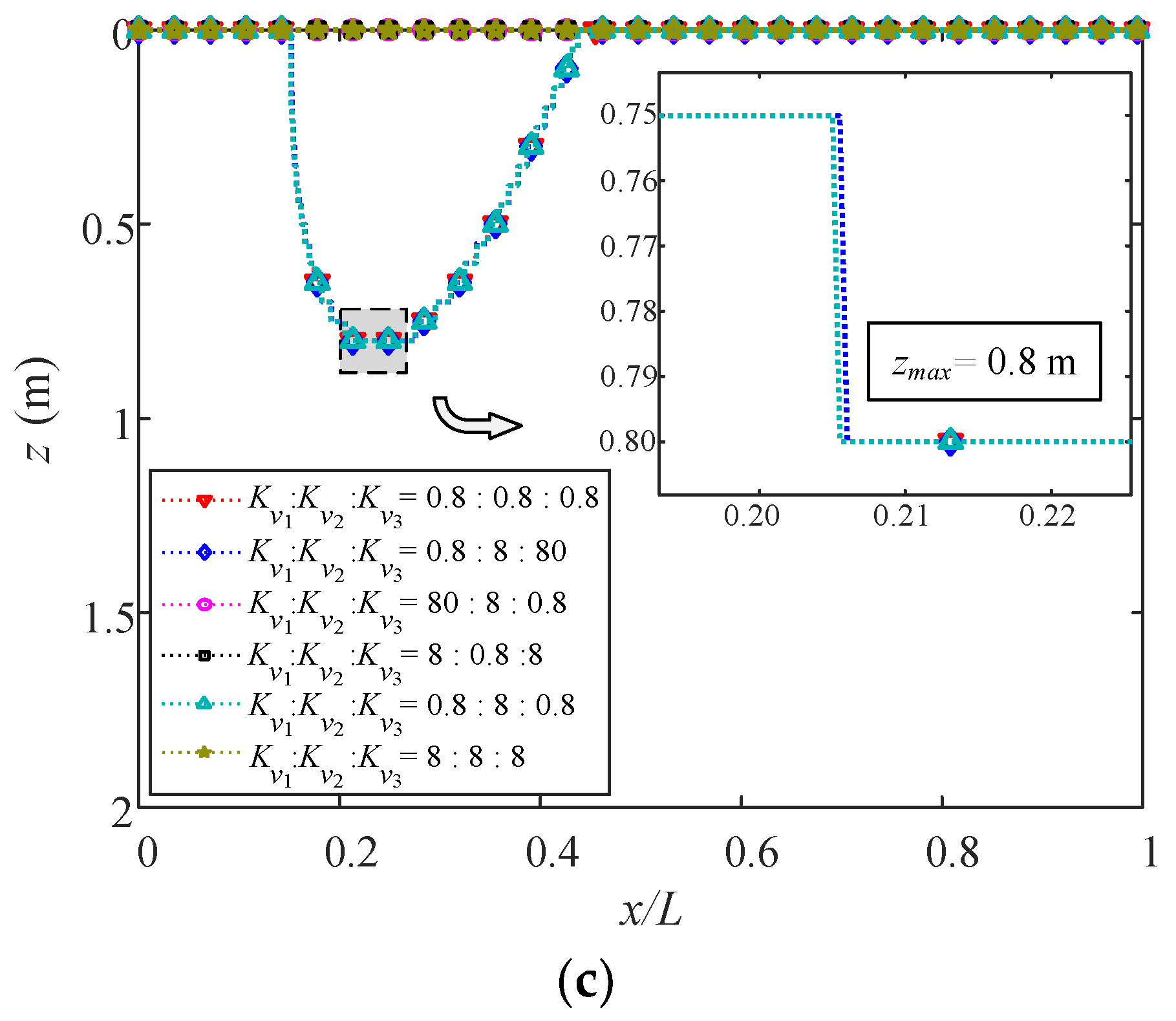
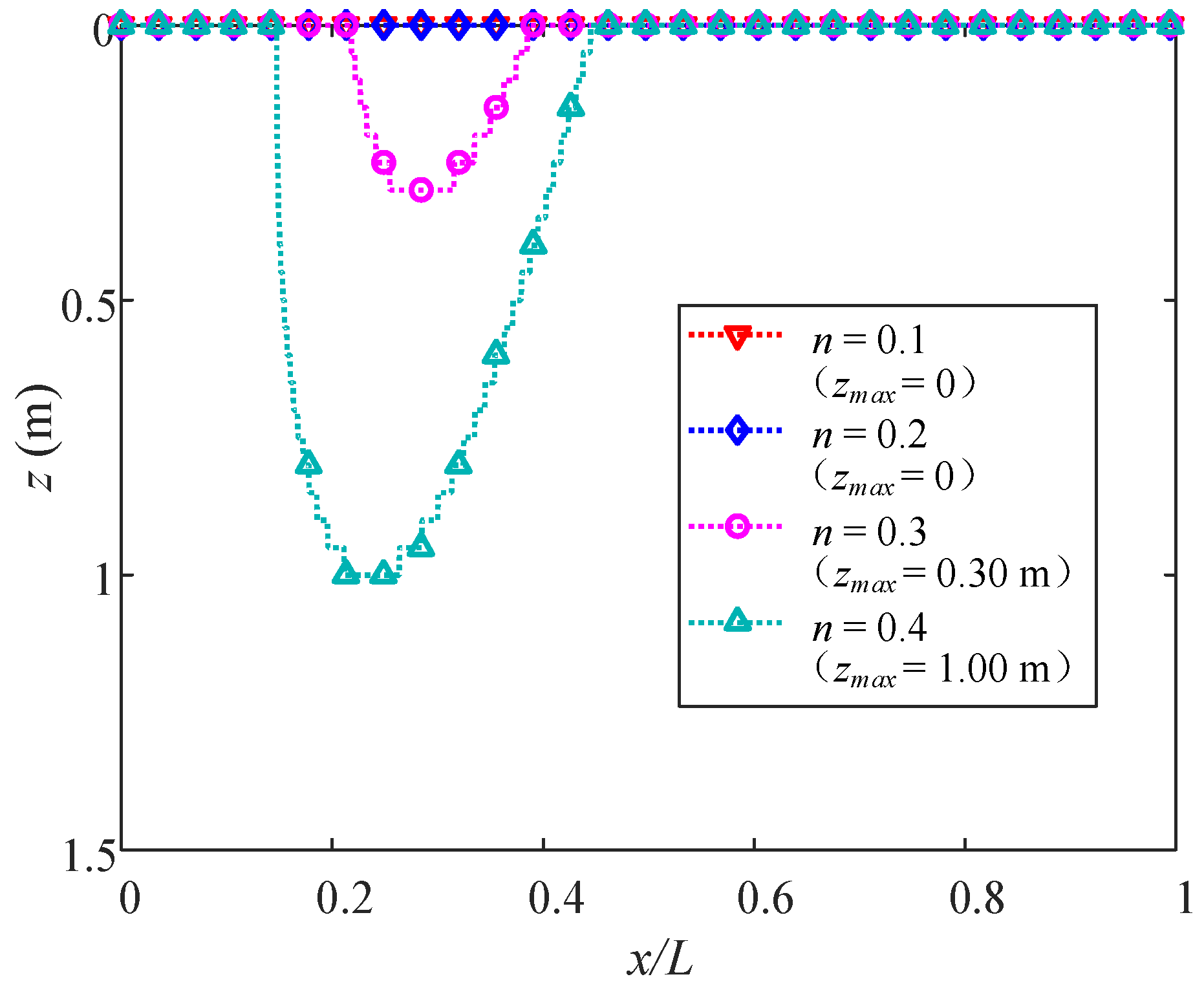
5.2.1. Effects of Permeability Coefficient
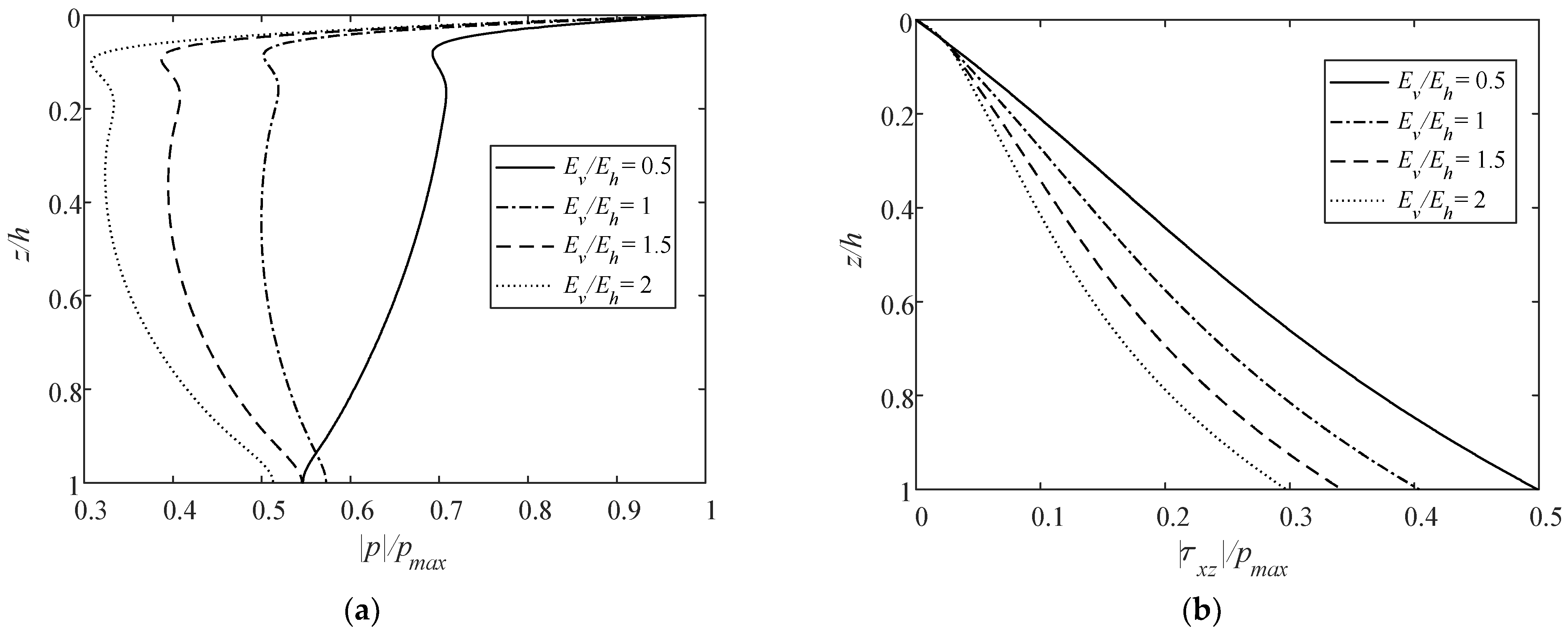
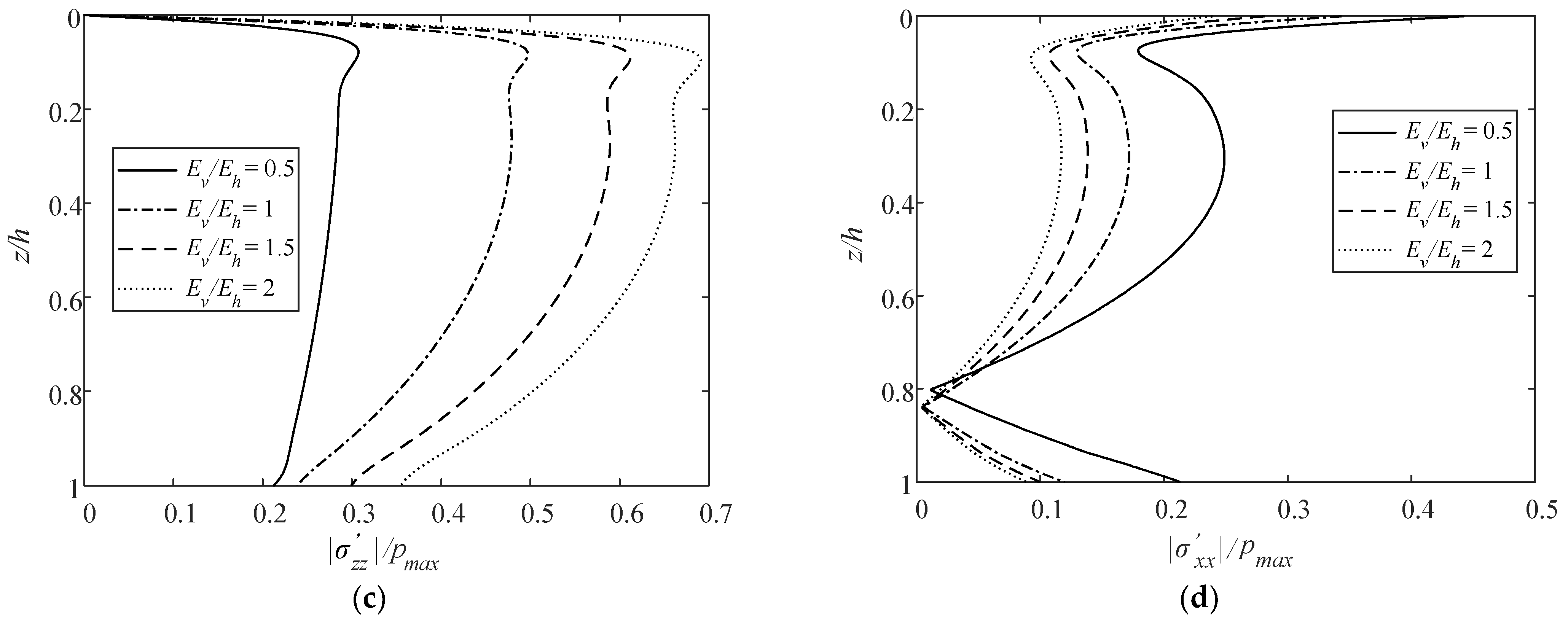
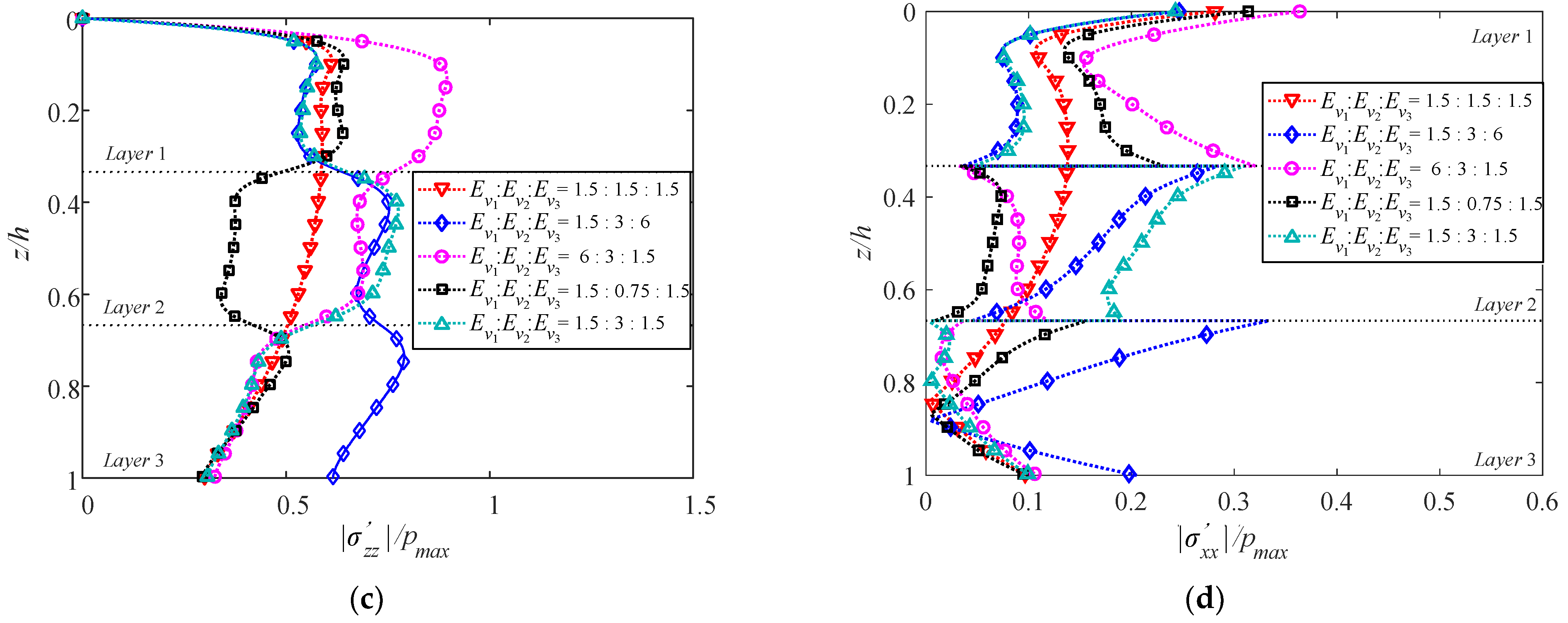
5.2.2. Effects of Young’s Modulus
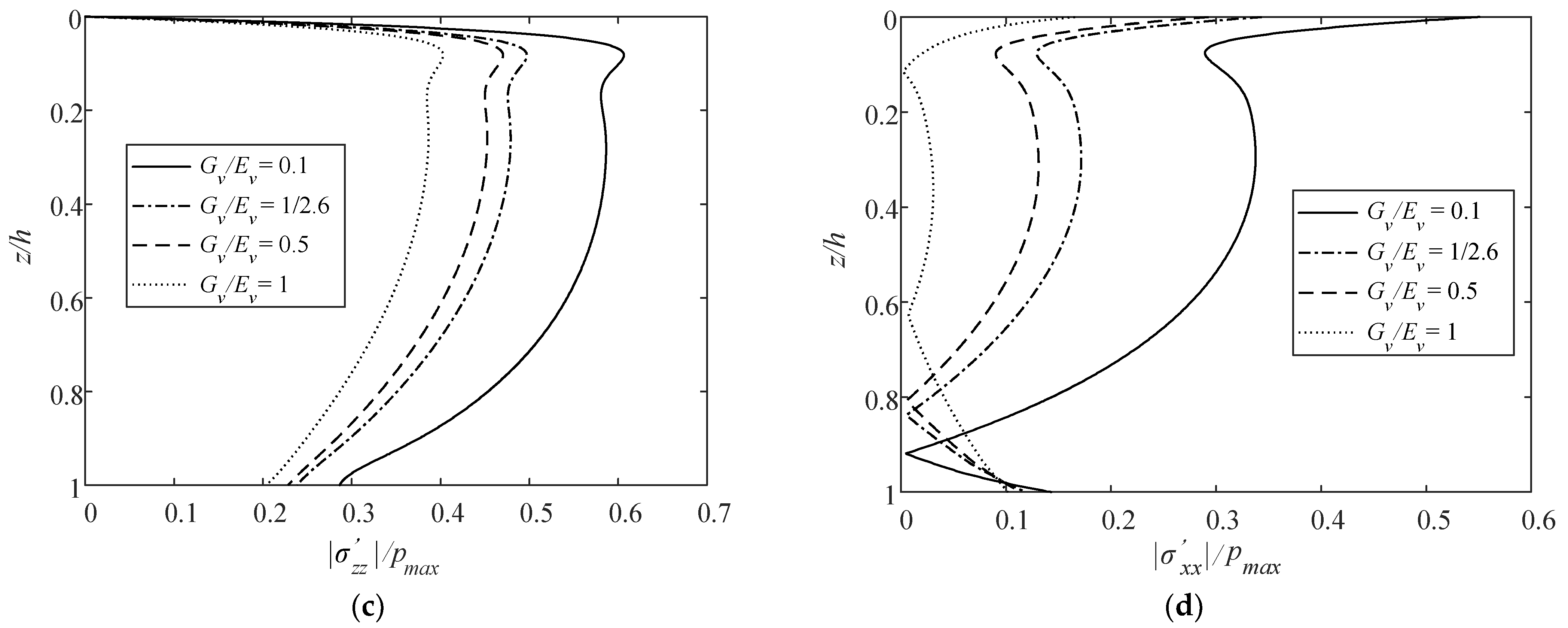
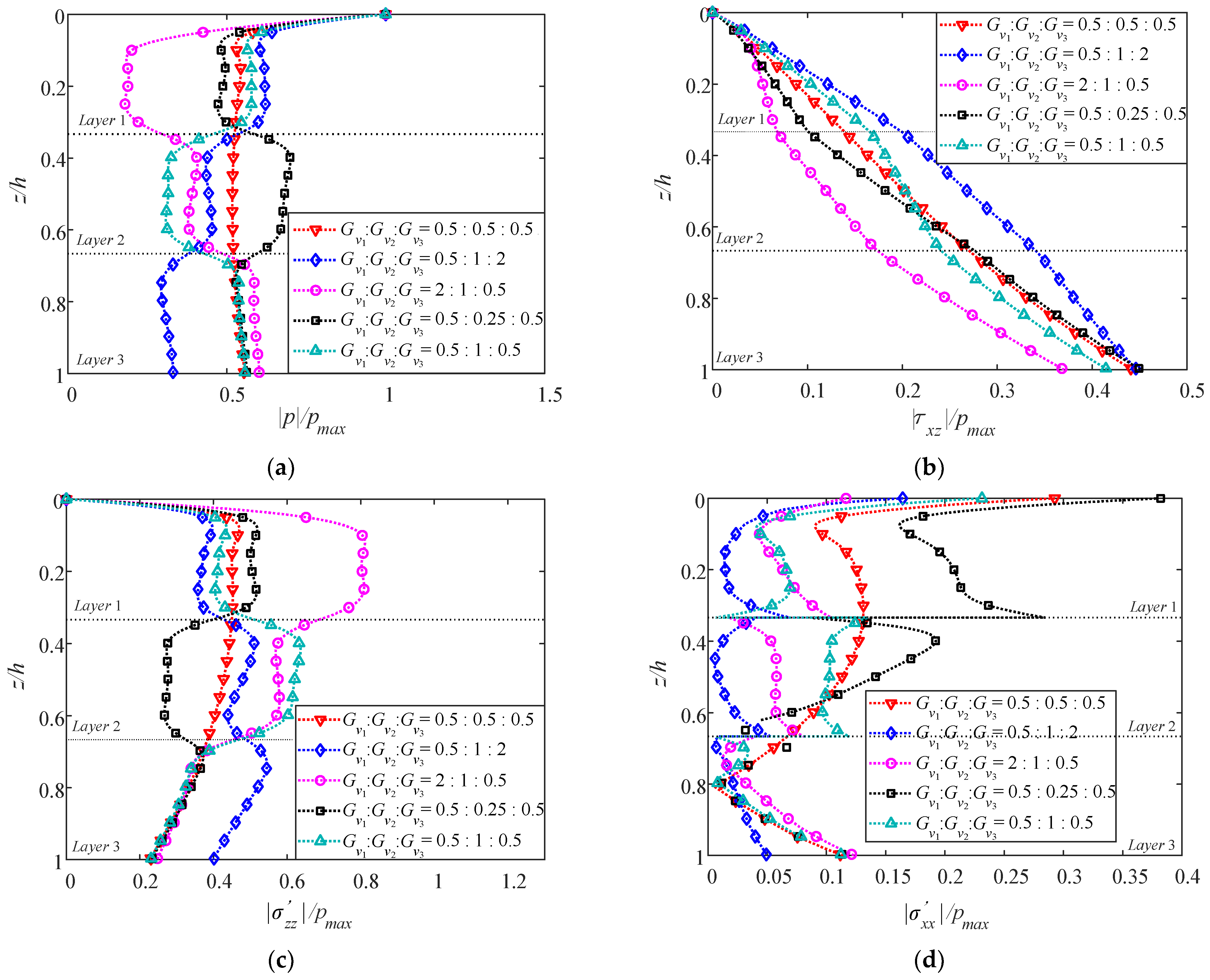
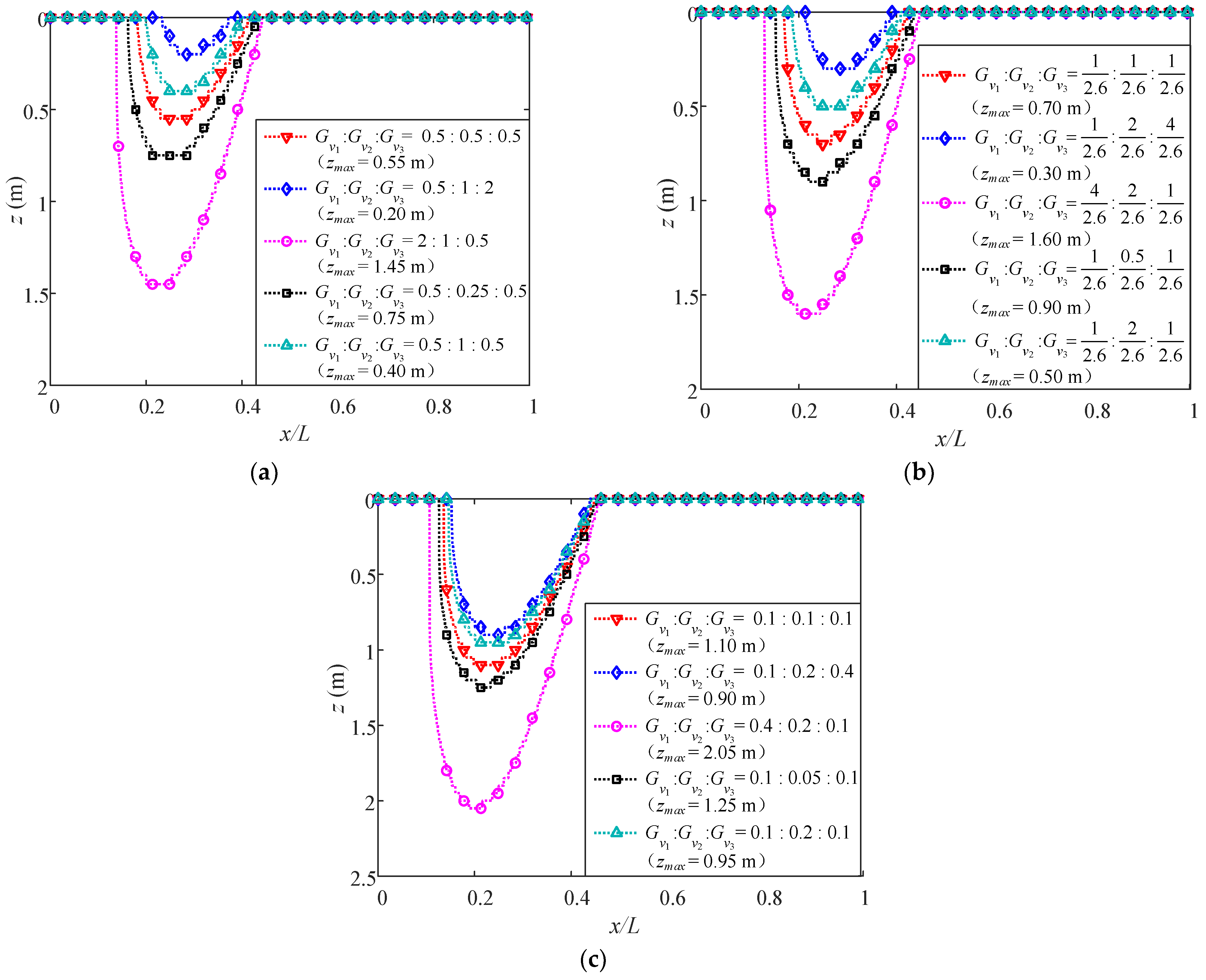
5.2.3. Effects of Shear Modulus
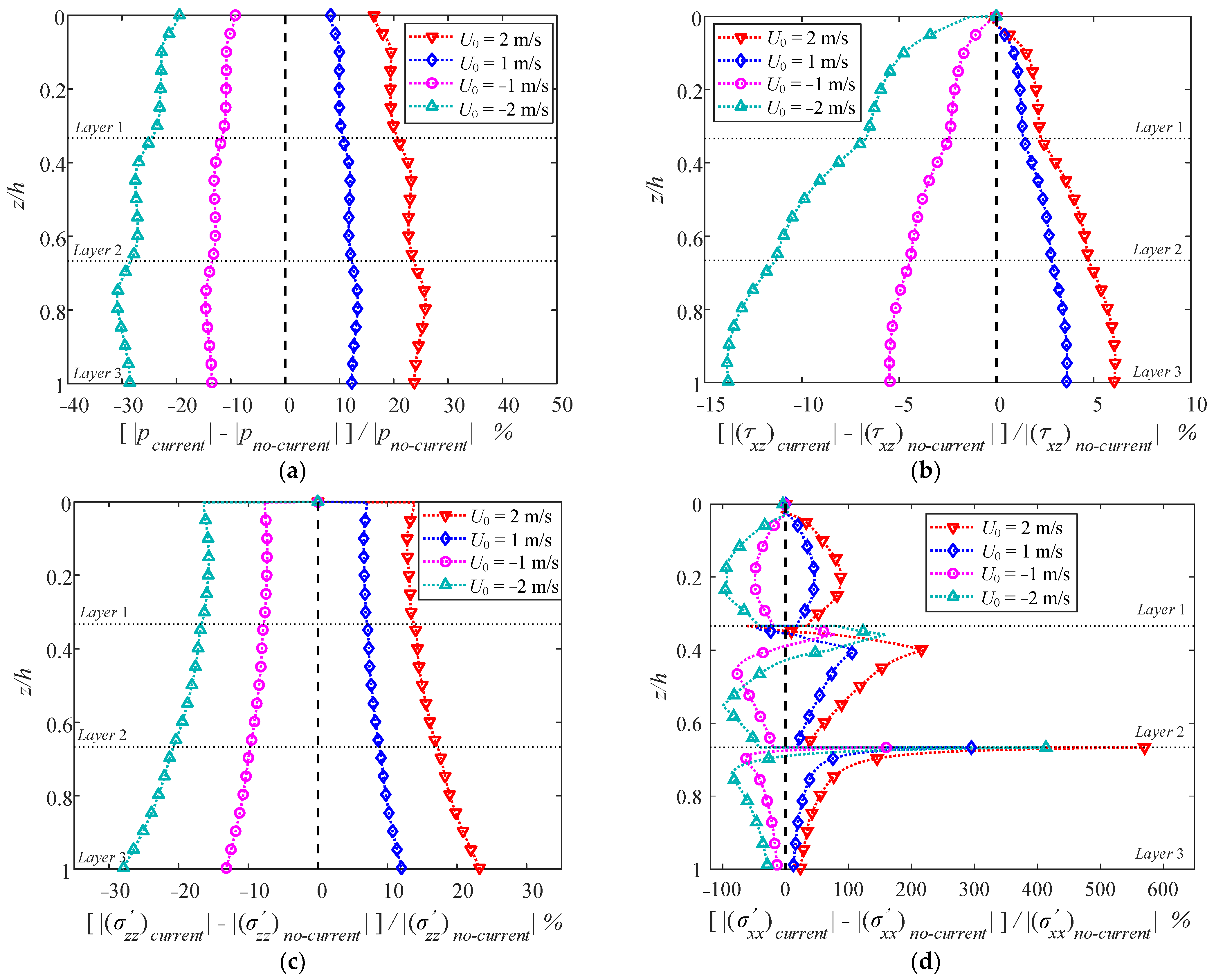
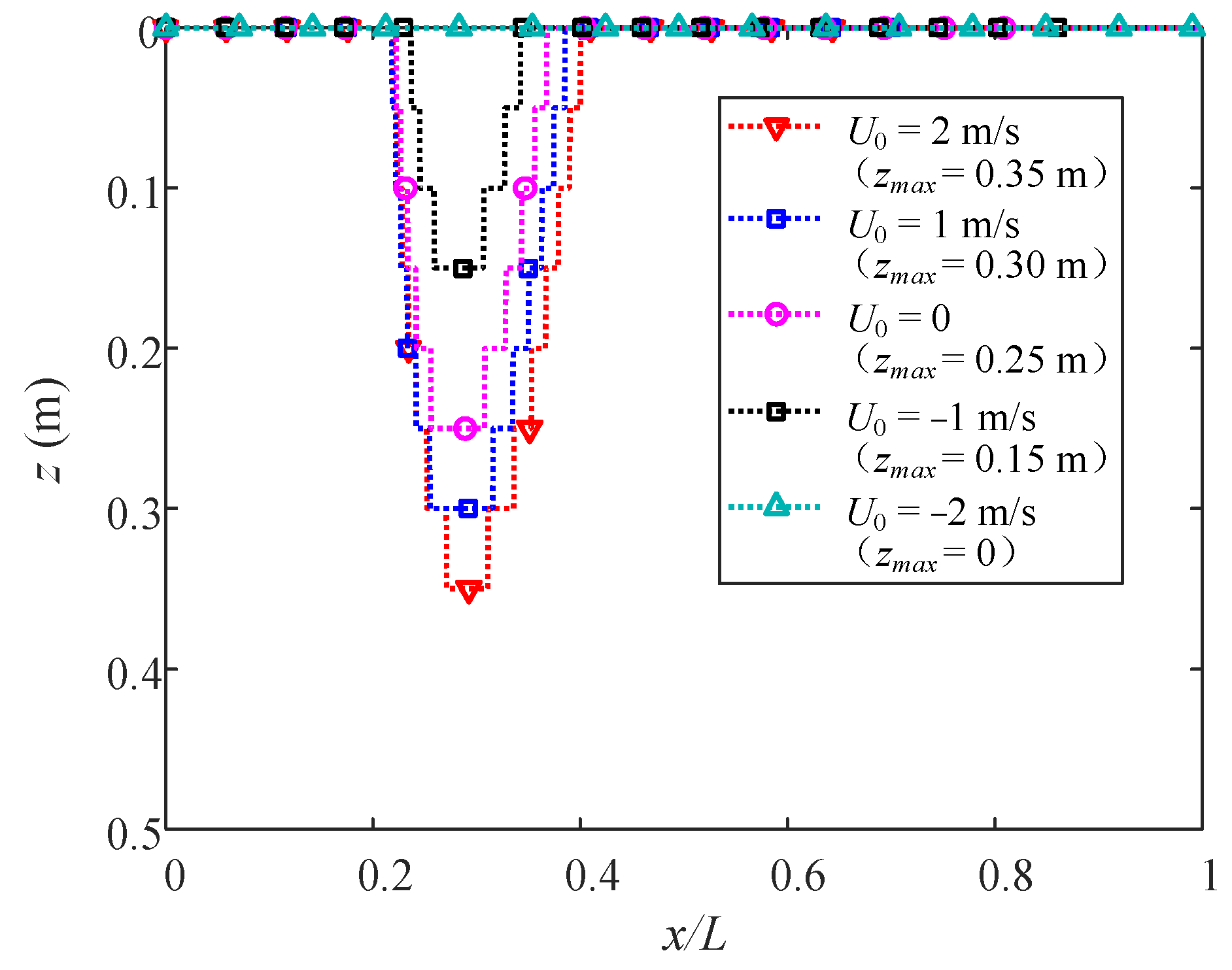
5.2.4. Effects of Current
6. Conclusions
- (1)
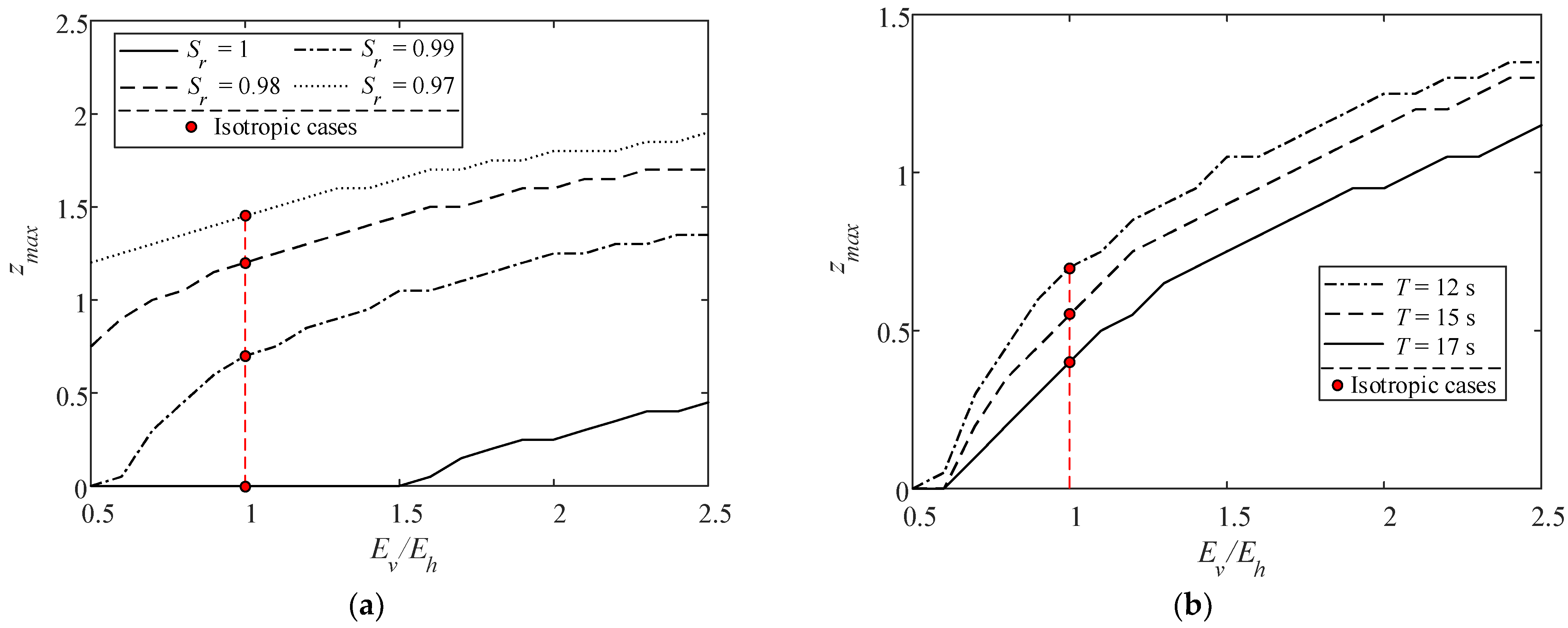
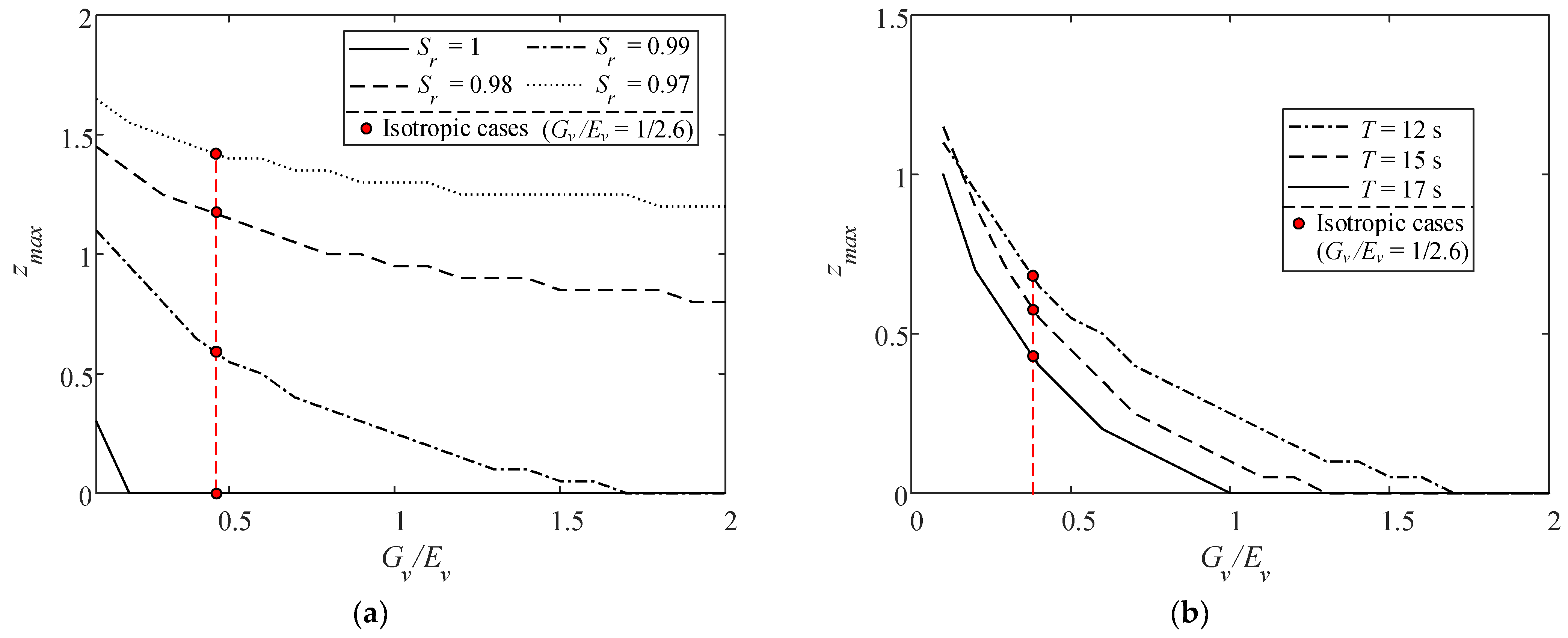
- For both the single-layered and the multilayered TIP seabed, the dynamic responses are more sensitive to the anisotropic ratios of Ev/Eh and Gv/Ev than to Kv/Kh. The variations of Ev/Eh and Gv/Ev dramatically alter all responses of |p|, |τxz|, || and ||, but the variations of Kv/Kh have a limited influence on these responses, especially |τxz|.
- (2)
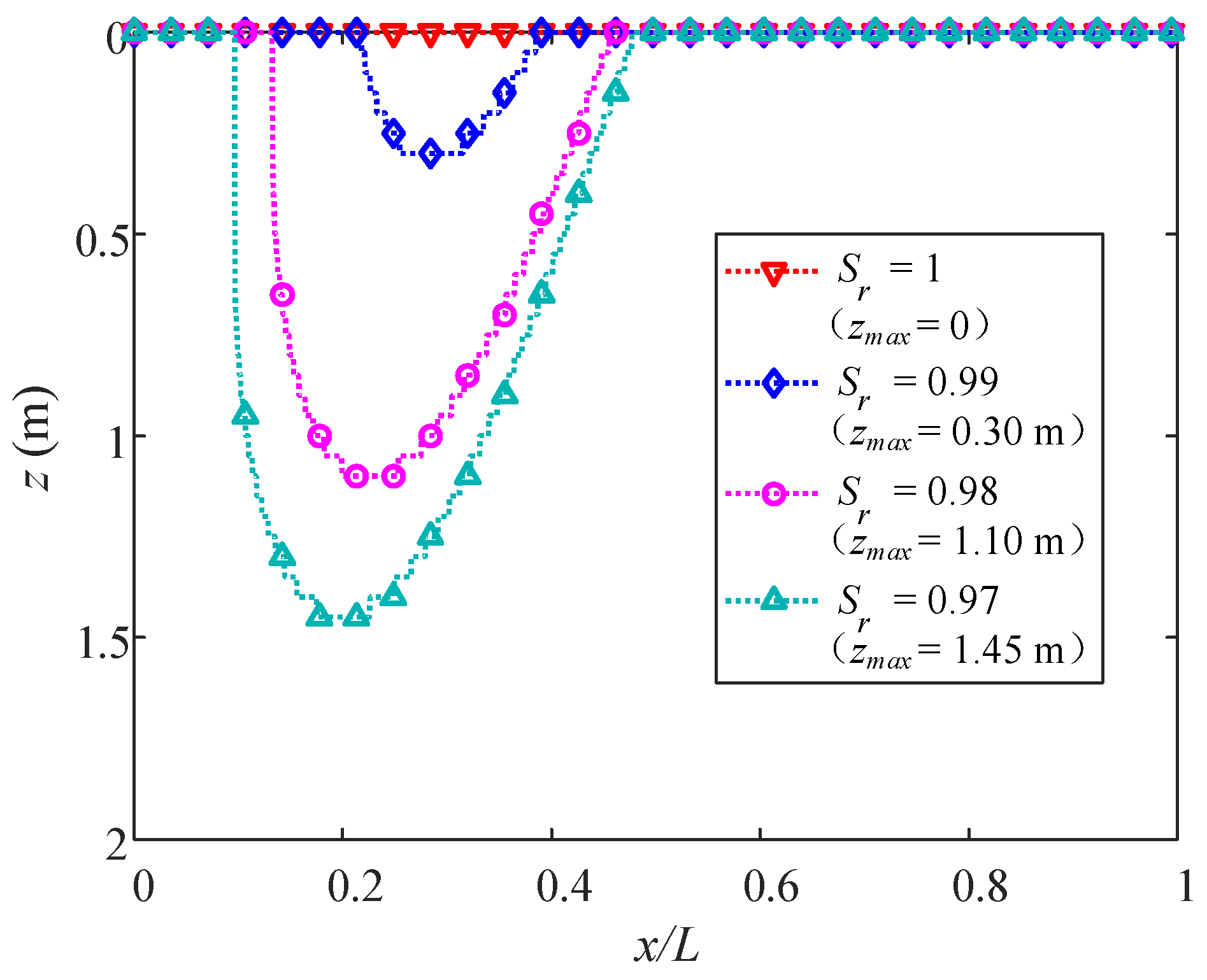
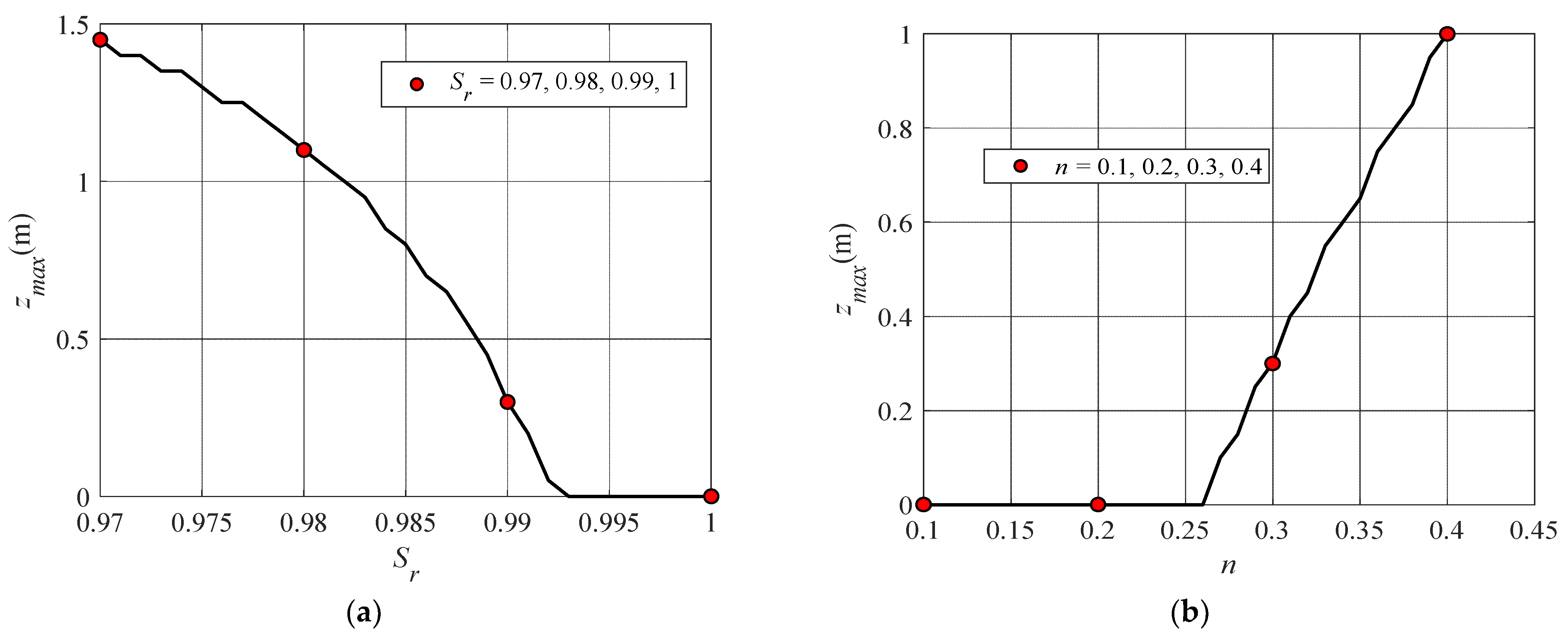
- For both the single-layered and the multilayered TIP seabed, a lower degree of quasi-saturation rate (i.e., 0.97), a higher porosity, a shorter wave period, and a following current result in a larger maximum liquefaction depth.
- (3)
- For a single-layered TIP seabed, the maximum liquefaction depths increase with decreasing Kv/Kh, Gv/Ev, and increasing Ev/Eh when Kh, Ev, and Eh are fixed, respectively, for these three ratios. Moreover, the conclusion drawn by a previous work [35] that transient liquefaction does not occur in fully saturated TIP sediments is valid, but only for an infinite thickness of the seabed, or for finite thickness with linear waves. Nonetheless, liquefaction does occur in a fully saturated and finite thickness TIP seabed under nonlinear waves.
- (4)
- For a multilayered TIP seabed, the anisotropic characteristics of the top layer dominate all dynamic responses and the maximum liquefaction depth. The contribution of stratified soil to dynamic responses and the maximum liquefaction depth gradually decays towards the bedrock. Regardless of the permeability level of the interlayer, its influence on the dynamic responses of the multilayered TIP seabed is rather limited.
- (5)
- A following current in waves aggravates the liquefaction while an opposing current suppresses the liquefaction. When a following and an opposing current are of the same magnitude, the latter results in a more notable variation on the maximum liquefaction depth than the former does. The influence of current becomes greater along with the depth, as compared to a scenario in the absence of a current.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
Appendix D
Appendix E
Appendix F
Appendix G
References
- Christian, J.T.; Taylor, P.K.; Yen, J.K.C.; Erali, D.R. Large diameter underwater pipeline for nuclear power plant designed against soil liquefaction. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 5 May 1974; pp. 597–606. [Google Scholar]
- Damgaard, J.S.; Sumer, B.M.; Teh, T.C.; Palmer, A.C.; Foray, P.; Osorio, D. Guidelines for Pipeline On-Bottom Stability on Liquefied Noncohesive Seabeds. J. Waterw. Port Coast. Ocean Eng. 2006, 132, 300–309. [Google Scholar] [CrossRef]
- Miyamoto, T.; Yoshinaga, S.; Soga, F.; Shimizu, K.; Kawamata, R.; Sato, M. Seismic Prospecting Method Applied to the De-tection of Offshore Breakwater Units Settling in the Seabed. Coast. Eng. J. 1989, 32, 103–112. [Google Scholar] [CrossRef]
- Padgett, J.; Desroches, R.; Nielson, B.; Yashinsky, M.; Kwon, O.S.; Burdette, N.; Tavera, E. Bridge Damage and Repair Costs from Hurricane Katrina. J. Bridge Eng. 2008, 13, 6–14. [Google Scholar] [CrossRef]
- Zen, K.; Umehara, Y.; Finn, W.D.L. A case study of the wave-induced liquefaction of sand layers under damaged breakwater. In Proceedings of the 3rd Canadian Conference on Marine Geotechnical Engineering, St. John’s, NL, Canada, 11–13 June 1986; pp. 505–520. [Google Scholar]
- Lundgren, H.; Lindhardt, J.H.C.; Romhild, C.J. Stability of break waters on poros foundation. In Proceedings of the 12th In-ternational Conference on Soil Mechanics and Foundation Engineering, Rio de Janeiro, Brazil, 13–18 August 1989; pp. 451–454. [Google Scholar]
- Ulker, M.B.C.; Rahman, M.S.; Guddati, M.N. Breaking wave-induced response and instability of seabed around caisson breakwater. Int. J. Numer. Anal. Methods Geomech. 2012, 36, 362–390. [Google Scholar] [CrossRef]
- Biot, M.A. General Theory of Three-Dimensional Consolidation. J. Appl. Phys. 1941, 12, 155–164. [Google Scholar] [CrossRef]
- Biot, M.A. Theory of propagation of elastic waves in a fluid-saturated porous solid. I. Low-frequency range. Int. J. Acoust. Vibr. 1956, 28, 168–178. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Chang, C.T.; Bettess, P. Drained, undrained, consolidating and dynamic behaviour assumptions in soils. Géotechnique 1980, 30, 385–395. [Google Scholar] [CrossRef]
- Yamamoto, T.; Koning, H.L.; Sellmeijer, H.; Hijum, E.V. On the response of a poro-elastic bed to water waves. J. Fluid Mech. 1978, 87, 193–206. [Google Scholar] [CrossRef]
- Madsen, O.S. Wave-induced pore-pressures and effective stresses in a porous bed. Geotechnique 1978, 28, 377–393. [Google Scholar] [CrossRef]
- Seymour, B.R.; Jeng, D.S.; Hsu, J.R.C. Transient soil response in a porous seabed with variable permeability. Ocean Eng. 1996, 23, 27–46. [Google Scholar] [CrossRef]
- Yamamoto, T. Wave-induced pore pressures and effective stresses in inhomogeneous seabed foundations. Ocean Eng. 1981, 8, 1–16. [Google Scholar] [CrossRef]
- Rahman, M.S.; El-Zahaby, K.; Booker, J. A semi-analytical method for the wave-induced seabed response. Int. J. Numer. Anal. Methods Geomech. 1994, 18, 213–236. [Google Scholar] [CrossRef]
- Liu, H.; Jeng, D.S. A semi-analytical solution for random wave-induced soil response and seabed liquefaction in marine sed-iments. Ocean Eng. 2007, 34, 1211–1224. [Google Scholar] [CrossRef]
- Cheng, A.H.D.; Liu, P.L.F. Seepage force on a pipeline buried in a poroelastic seabed under wave loadings. Appl. Ocean Res. 1986, 8, 22–32. [Google Scholar] [CrossRef]
- Ulker, M.B.C.; Rahman, M.S.; Guddati, M.N. Wave-induced dynamic response and instability of seabed around caisson breakwater. Ocean Eng. 2010, 37, 1522–1545. [Google Scholar] [CrossRef]
- Ulker, M.B.C.; Rahman, M.S.; Jeng, D.S. Wave-induced response of seabed: Various formulations and their applicability. Appl. Ocean Res. 2009, 31, 12–24. [Google Scholar] [CrossRef]
- Chen, W.Y.; Jeng, D.S.; Chen, W.; Chen, G.X.; Zhao, H.Y. Seismic-induced dynamic responses in a poro-elastic seabed: Solutions of different formulations. Soil Dyn. Earthq. Eng. 2020, 131, 106021. [Google Scholar] [CrossRef]
- Jeng, D.S.; Rahman, M.S.; Lee, T.L. Effects of Inertia Forces on Wave-Induced Seabed Response. In Proceedings of the 19th International Offshore and Polar Engineering Conference, Brest, France, 30 May–4 June 1999. [Google Scholar]
- Jeng, D.S.; Rahman, M.S. Effective stresses in a porous seabed of finite thickness: Inertia effects. Can. Geotech. J. 2000, 37, 1383–1392. [Google Scholar] [CrossRef][Green Version]
- Jeng, D.S.; Lee, T.L. Dynamic response of porous seabed to ocean waves. Comput. Geotech. 2001, 28, 99–128. [Google Scholar] [CrossRef]
- Gatmiri, B. Simplified finite element analysis of wave-induced effective stresses and pore pressures in permeable sea beds. Geotechnique 1990, 40, 15–30. [Google Scholar] [CrossRef]
- Thomas, S.D. A finite element model for the analysis of wave induced stresses, displacements and pore pressures in an un-saturated seabed II: Model verification. Comput. Geotech. 1995, 17, 107–132. [Google Scholar] [CrossRef]
- Schumacher, T.; Higgins, C.; Bradner, C.; Cox, D.; Yim, S.C. Large-scale Wave Flume Experiments on Highway Bridge Su-perstructures Exposed to Hurricane Wave Forces. In Proceedings of the 6th National Seismic Conference on Bridges and Highways, Charleston, WV, USA, 27–30 July 2008. [Google Scholar]
- Onorato, M.; Osborne, A.R.; Serio, M.; Cavaleri, L.; Brandini, C.; Stansberg, C.T. Extreme waves, modulational instability and second order theory: Wave flume experiments on irregular waves. Eur. J. Mech. 2006, 25, 586–601. [Google Scholar] [CrossRef]
- Kirca, V.S.O.; Sumer, B.M.; Fredsoe, J. Influence of Clay Content on Wave-Induced Liquefaction. J. Waterw. Port Coast. Ocean Eng. 2014, 140, 04014024. [Google Scholar] [CrossRef]
- Tzang, S.Y. Unfluidized soil responses of a silty seabed to monochromatic waves. Coast. Eng. 1998, 35, 283–301. [Google Scholar] [CrossRef]
- Ye, J.H.; Jeng, D.S. Response of Porous Seabed to Nature Loadings: Waves and Currents. J. Eng. Mech. 2012, 138, 601–613. [Google Scholar] [CrossRef]
- Zhang, Y.; Jeng, D.S.; Gao, F.P.; Zhang, J.S. An analytical solution for response of a porous seabed to combined wave and current loading. Ocean Eng. 2013, 57, 240–247. [Google Scholar] [CrossRef]
- Liu, B.; Jeng, D.S.; Zhang, J.S. Dynamic Response in a Porous Seabed of Finite Depth to Combined Wave and Current Loadings. J. Coast. Res. 2014, 30, 765–776. [Google Scholar]
- Cheng, H.D. Material coefficients of anisotropic poroelasticity. Int. J. Rock Mech. 1997, 34, 199–205. [Google Scholar] [CrossRef]
- Jeng, D.S. Effect of cross-anisotropic behaviour on wave-induced seabed response. Géotechnique 1998, 48, 555–561. [Google Scholar] [CrossRef]
- Jeng, D.S. Soil Response in Cross-Anisotropic Seabed due to Standing Waves. J. Rock Mech. Geotech. 1997, 123, 9–19. [Google Scholar] [CrossRef]
- Zhang, Z.; Pan, E. Time-harmonic response of transversely isotropic and layered poroelastic half-spaces under general buried loads. Appl. Math. Model. 2020, 80, 426–453. [Google Scholar] [CrossRef]
- Ye, Z.; Ai, Z.Y. Dynamic analysis of multilayered unsaturated poroelastic media subjected to a vertical time-harmonic load. Appl. Math. Model. 2021, 90, 394–412. [Google Scholar] [CrossRef]
- Li, X.; Zhang, Z.; Pan, E. Wave-induced dynamic response in a transversely isotropic and multilayered poroelastic seabed. Soil Dyn. Earthq. Eng. 2020, 139, 106365. [Google Scholar] [CrossRef]
- Sparks, A.D.W. Theoretical considerations of stress equations for partly saturated soils. In Proceedings of the 3rd Regular Conference for Africa on Soil Mechanics and Foundation Engineering, Salisbury, UK, 2–9 June 1963; pp. 215–218. [Google Scholar]
- Laing, B. Consolidation of Compacted and Unsaturated Clays. Géotechnique 1965, 15, 267–286. [Google Scholar]
- Chang, C.S.; Duncan, J.M. Consolidation analysis for partly saturated clay by using an elastic-plastic effective stress-strain model. Int. J. Numer. Anal. Methods Geomech. 1983, 7, 39–55. [Google Scholar] [CrossRef]
- Biot, M.A. Mechanics of Deformation and Acoustic Propagation in Porous Media. J. Appl. Phys. 1962, 33, 1482–1498. [Google Scholar] [CrossRef]
- Verruijt, A. Elastic Storage of Aquifers. In Flow through Porous Media; Wiest, R.J.M.D., Ed.; Academic Press: New York, NY, USA, 1969; pp. 331–376. [Google Scholar]
- Esrig, M.I.; Kirby, R.C. Implications of gas content for predicting the stability of submarine slopes. Mar. Geotechnol. 1977, 2, 81–100. [Google Scholar] [CrossRef]
- Hsu, H.C.; Chen, Y.Y.; Hsu, J.R.C.; Tseng, W.J. Nonlinear water waves on uniform current in Lagrangian coordinates. J. Nonlinear Math. Phys. 2009, 16, 47–61. [Google Scholar] [CrossRef]
- Liu, H.; Pan, E. Time-harmonic loading over transversely isotropic and layered elastic half-spaces with imperfect interfaces. Soil Dyn. Earthq. Eng. 2018, 107, 35–47. [Google Scholar] [CrossRef]
- Feng, S.; Ding, X.; Zheng, Q.; Chen, Z. Extended stiffness matrix method for horizontal vibration of a rigid disk embedded in stratified soils. Appl. Math. Model. 2020, 77, 663–689. [Google Scholar] [CrossRef]
- Qi, W.G.; Li, C.F.; Jeng, D.S.; Gao, F.-P.; Liang, Z.D. Combined wave-current induced excess pore-pressure in a sandy seabed: Flume observations and comparisons with theoretical models. Coast. Eng. 2019, 147, 89–98. [Google Scholar] [CrossRef]
- Olabarrieta, M.; Medina, R.; Castanedo, S. Effects of wave-current interaction on the current profile. Coast. Eng. 2010, 57, 643–655. [Google Scholar] [CrossRef]
- Le Méhauté, B. An Introduction to Hydrodynamics and Water Waves; Springer: Berlin/Heidelberg, Germany, 1976; p. 205. ISBN 978-3-642-85569-6. [Google Scholar]
- Zen, K.; Yamazaki, H. Mechanism of wave-induced liquefaction and densification in seabed. Soils Found. 1990, 30, 90–104. [Google Scholar] [CrossRef]
- Rahman, M.S. Wave-induced instability of seabed: Mechanism and conditions. Mar. Geotechnol. 1991, 10, 277–299. [Google Scholar] [CrossRef]
- Terzaghi, K.; Peck, R.B.; Mesri, G. Soil Mechanics in Engineering Practice, 3rd ed.; John Wiley & Sons, Inc.: New York, NY, USA, 1996; ISBN 978-0-471-08658-1. [Google Scholar]
- Tsai, C.P. Wave-induced liquefaction potential in a porous seabed in front of a breakwater. Ocean Eng. 1995, 22, 1–18. [Google Scholar] [CrossRef]
- Zheng, X.Y.; Li, H.B.; Rong, W.D.; Li, W. Joint earthquake and wave action on the monopile wind turbine foundation: An experimental study. Mar. Struct. 2015, 44, 125–141. [Google Scholar] [CrossRef]
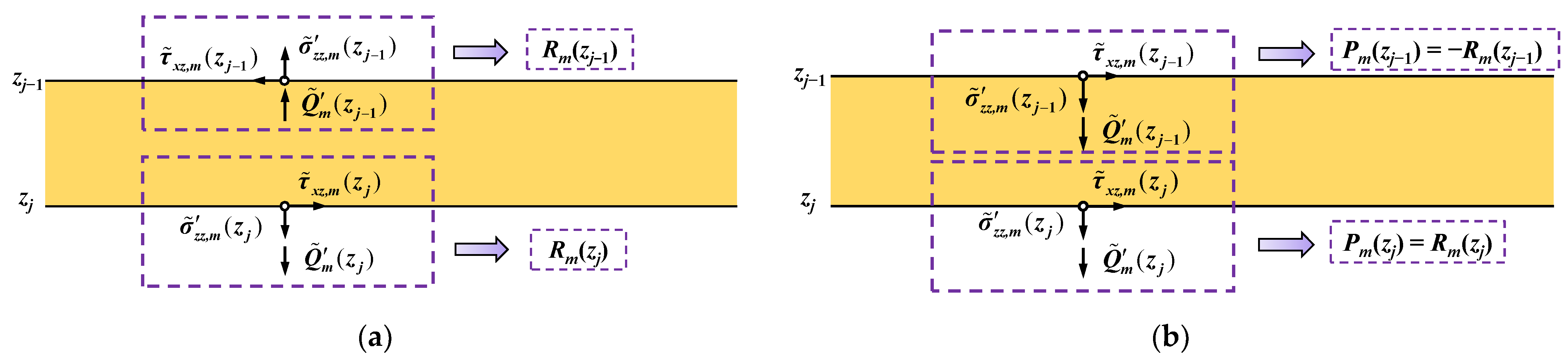


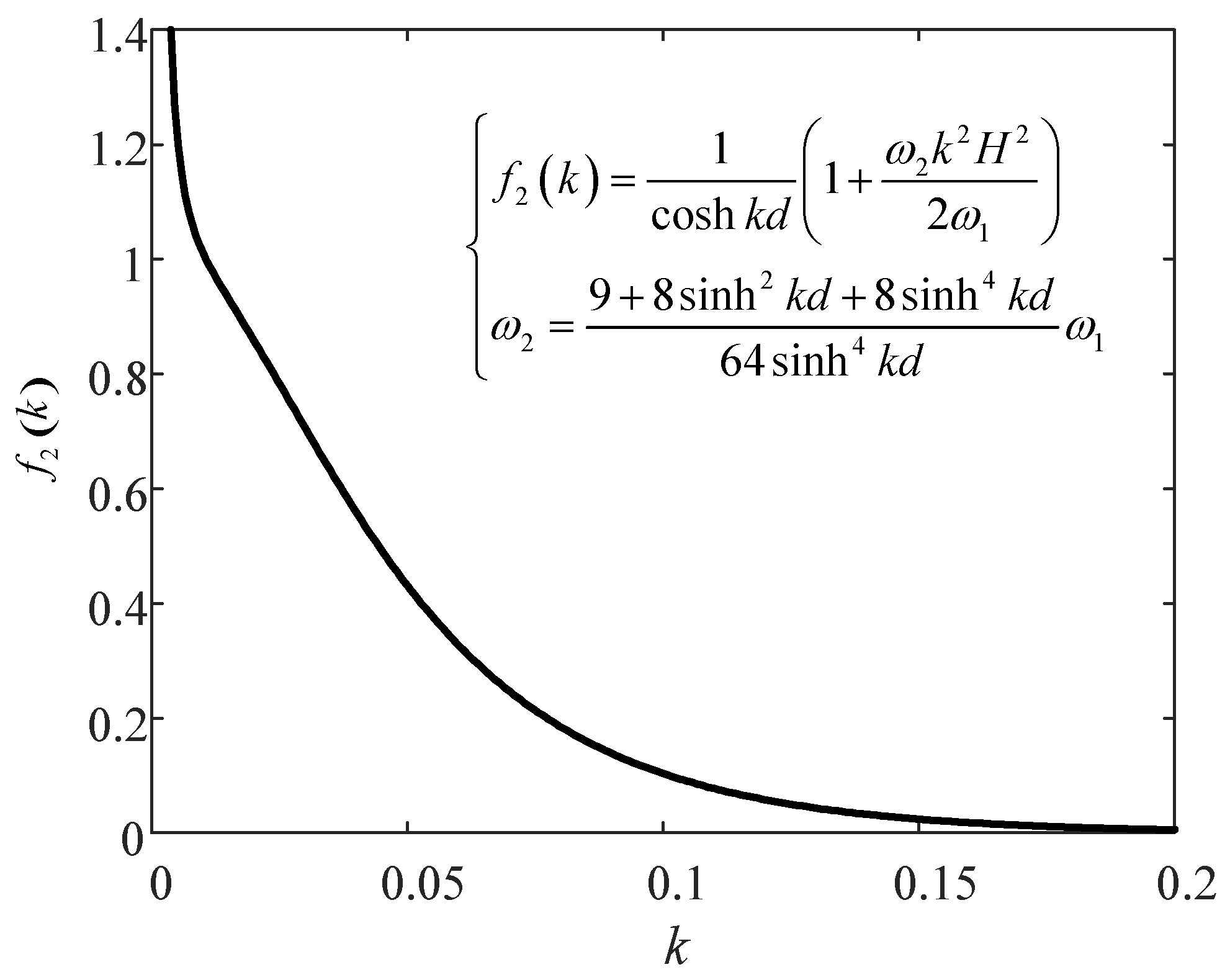
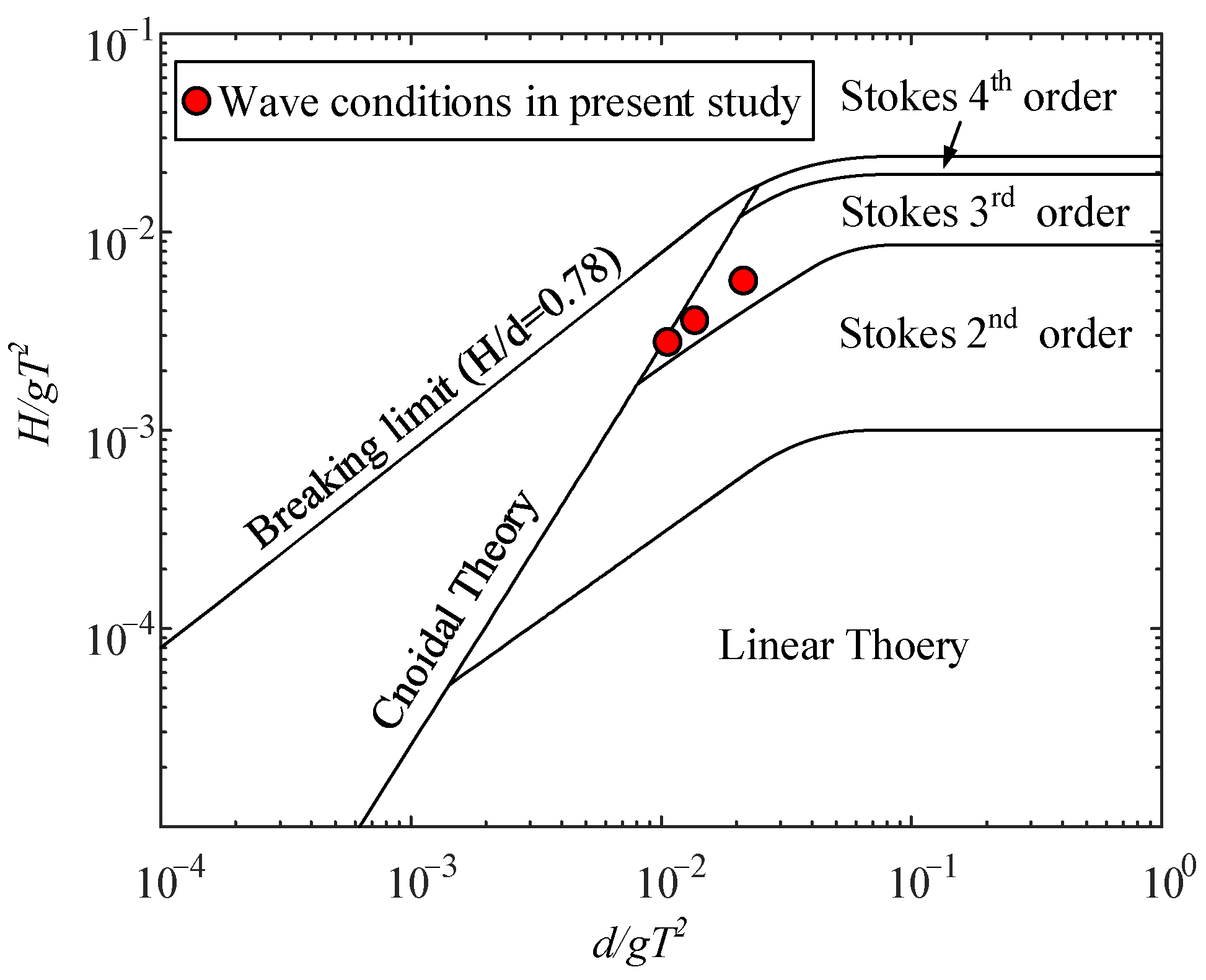
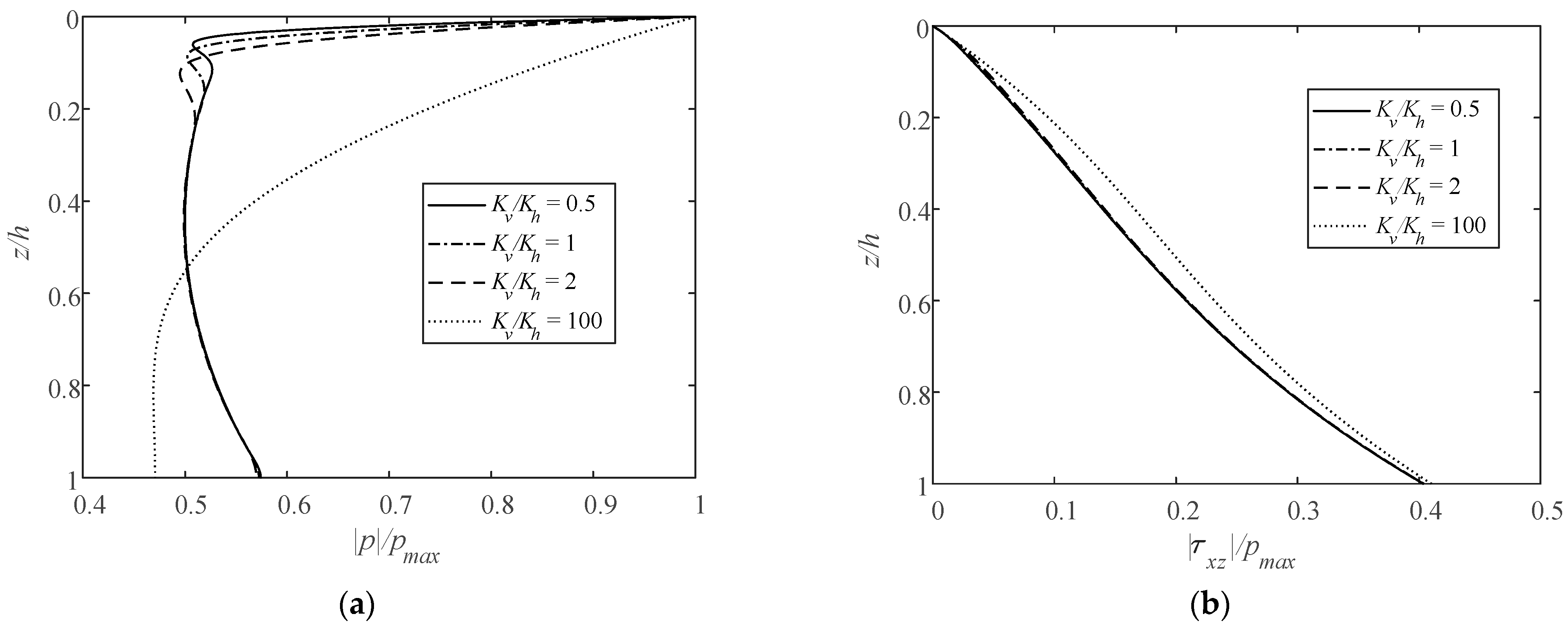
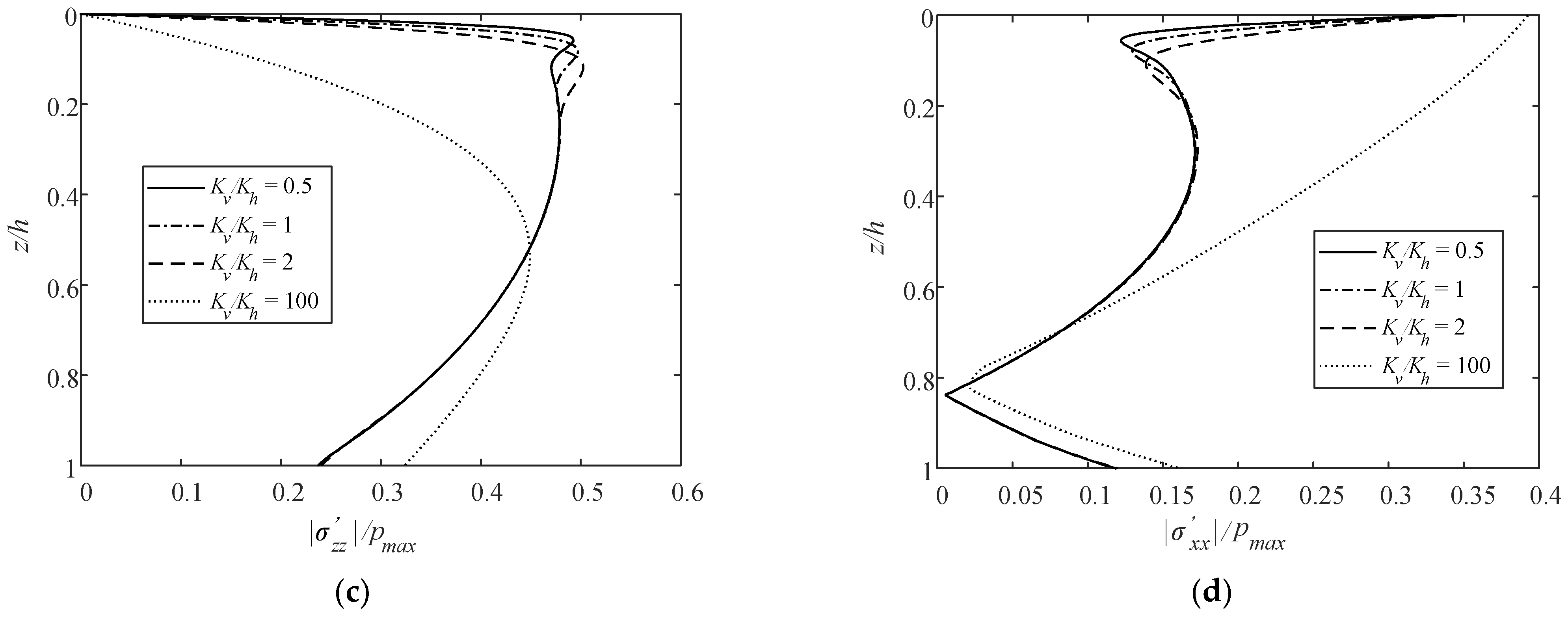






| Parameters | Value | |
|---|---|---|
| Wave conditions | Wave period, T | 12.5 s |
| Water depth, d | 20 m | |
| Wavelength, L | 159.95 m | |
| Wave height, H | 12 m | |
| Soil properties | Thickness of seabed, h | 25 m |
| Density of soil skeleton, ρs | 2650 kg/m3 | |
| Density of pore fluid, ρf | 1000 kg/m3 | |
| Bulk modulus of soil skeleton, Ks | 3.6 × 1010 Pa | |
| True bulk modulus of elasticity of water, Kw | 2 × 109 Pa | |
| Permeability coefficient, K | 1 × 10−4 m/s | |
| Shear modulus, G | 1 × 107 Pa | |
| Poisson’s ratio, ν | 0.3 | |
| Degree of saturation, Sr | 1.0 | |
| Porosity, n | 0.3 |
| Parameters | Value | |
|---|---|---|
| Wave conditions | Water depth, d | 0.5 m |
| Wave height, H | 9.5 × 10−2 m | |
| Soil properties | Thickness of seabed, h | 0.5 m |
| Density of soil skeleton, ρs | 2682 kg/m3 | |
| Density of pore fluid, ρf | 1000 kg/m3 | |
| True bulk modulus of elasticity of water, Kw | 2 × 109 Pa | |
| Permeability coefficient, K | 1.88 × 10−4 m/s | |
| Shear modulus, G | 1 × 107 Pa | |
| Poisson’s ratio, ν | 0.3 | |
| Degree of saturation, Sr | 0.995 | |
| Void ratio, e | 0.771 |
| Parameters | Value | |
|---|---|---|
| Wave and current conditions | Water depth, d | 30 m |
| Wave height, H | 8 m | |
| Wave period, T | 12~17 s († Typical value: 12 s) | |
| Current velocity, U0 | −2 m/s~2 m/s († Typical value: 1 m/s) | |
| Soil properties | Thickness of seabed, h1 = h2 = h3 | 10 m |
| Density of soil skeleton, ρs | 2650 kg/m3 | |
| Density of pore fluid, ρf | 1000 kg/m3 | |
| Bulk modulus of soil skeleton, Ks | 3.6 × 1010 Pa | |
| True bulk modulus of elasticity of water, Kw | 2 × 109 Pa | |
| Poisson’s ratio, νh = νv | 0.3 | |
| Permeability coefficient, Kh, Kv | 5 × 10−5~2 × 10−2 m/s († Typical value: 1 × 10−4 m/s) | |
| Shear modulus, Gv | (6.5~52) × 106 Pa († Typical value: 1 × 107 Pa) | |
| Young’s modulus, Eh, Ev | (13~156) × 106 Pa († Typical value: 2.6 × 107 Pa) | |
| Degree of saturation *, Sr | 0.96~1 († Typical value: 0.99) | |
| Porosity, n | 0.1~0.4 († Typical value: 0.3) |
| Case | H (m) | T (s) | d (m) | H/gT2 | d/gT2 |
|---|---|---|---|---|---|
| 1 | 8 | 12 | 30 | 0.0057 | 0.0213 |
| 2 | 8 | 15 | 30 | 0.0036 | 0.0136 |
| 3 | 8 | 17 | 30 | 0.0028 | 0.0106 |
| Case | Wave Conditions | Soil Properties | Thickness | Liquefaction | |||||
|---|---|---|---|---|---|---|---|---|---|
| Table 6 | Table 3 | Table 6 | Table 3 | Infinite | Finite | ||||
| Linear Model | Nonlinear Model | Linear Model | Nonlinear Model | ||||||
| 1 | × | × | × | No | |||||
| 2 | × | × | × | Yes | |||||
| 3 | × | × | × | No | |||||
| 4 | × | × | × | No | |||||
| 5 | × | × | × | No | |||||
| 6 | × | × | × | Yes | |||||
| 7 | × | × | × | No | |||||
| 8 | × | × | × | No | |||||
| 9 | × | × | × | No | |||||
| 10 | × | × | × | Yes | |||||
| 11 | × | × | × | No | |||||
| 12 | × | × | × | No | |||||
| 13 | × | × | × | No | |||||
| 14 | × | × | × | Yes | |||||
| 15 | × | × | × | No | |||||
| 16 | × | × | × | No | |||||
| Parameters | Value | |
|---|---|---|
| Wave and current conditions | d | 20 m |
| H | 12 m | |
| T | 12.5 s | |
| L | 159.95 m | |
| Soil properties | ρs | 2000 kg/m3 |
| ρf | 1000 kg/m3 | |
| νh = νv | 0.3 | |
| Kh = Kv | 1 × 10−4 m/s | |
| Ev | 1 × 107 Pa | |
| Gv | 0.6 × 107 Pa | |
| n | 0.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Zhang, Q.; Zheng, X.Y.; Lei, Y. Dynamic Responses of a Multilayered Transversely Isotropic Poroelastic Seabed Subjected to Ocean Waves and Currents. J. Mar. Sci. Eng. 2022, 10, 73. https://doi.org/10.3390/jmse10010073
Chen X, Zhang Q, Zheng XY, Lei Y. Dynamic Responses of a Multilayered Transversely Isotropic Poroelastic Seabed Subjected to Ocean Waves and Currents. Journal of Marine Science and Engineering. 2022; 10(1):73. https://doi.org/10.3390/jmse10010073
Chicago/Turabian StyleChen, Xi, Qi Zhang, Xiang Yuan Zheng, and Yu Lei. 2022. "Dynamic Responses of a Multilayered Transversely Isotropic Poroelastic Seabed Subjected to Ocean Waves and Currents" Journal of Marine Science and Engineering 10, no. 1: 73. https://doi.org/10.3390/jmse10010073
APA StyleChen, X., Zhang, Q., Zheng, X. Y., & Lei, Y. (2022). Dynamic Responses of a Multilayered Transversely Isotropic Poroelastic Seabed Subjected to Ocean Waves and Currents. Journal of Marine Science and Engineering, 10(1), 73. https://doi.org/10.3390/jmse10010073






