Biomimetic Structural Design for Reducing the Adhesion Between Wet Rice Leaves and Metal Surfaces
Abstract
1. Introduction
2. Materials and Methods
2.1. Basic Characters of Y-Liangyou Rice Plant
2.2. Design of the Adhesion-Reducing Surface Structure of Cleaning Device
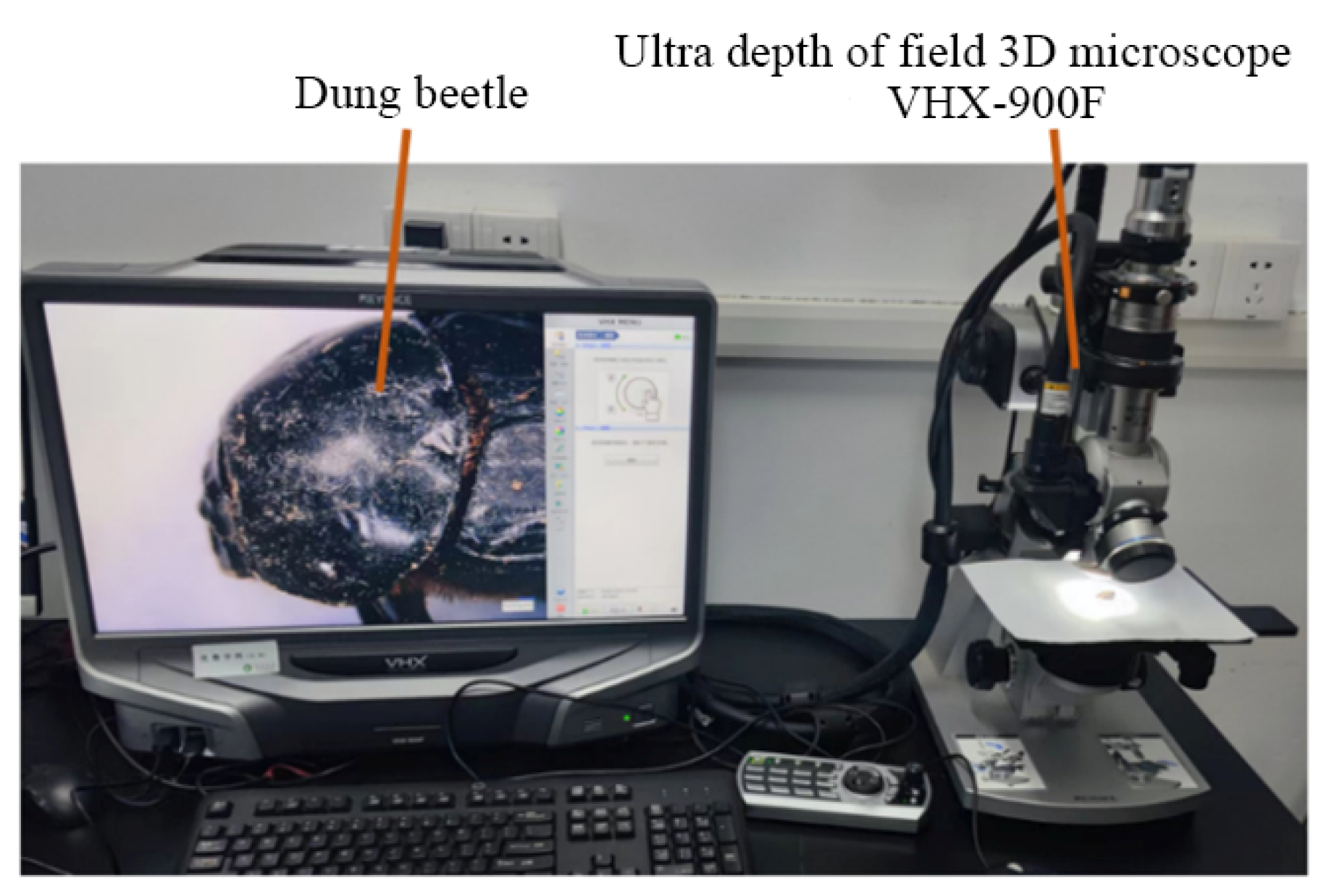
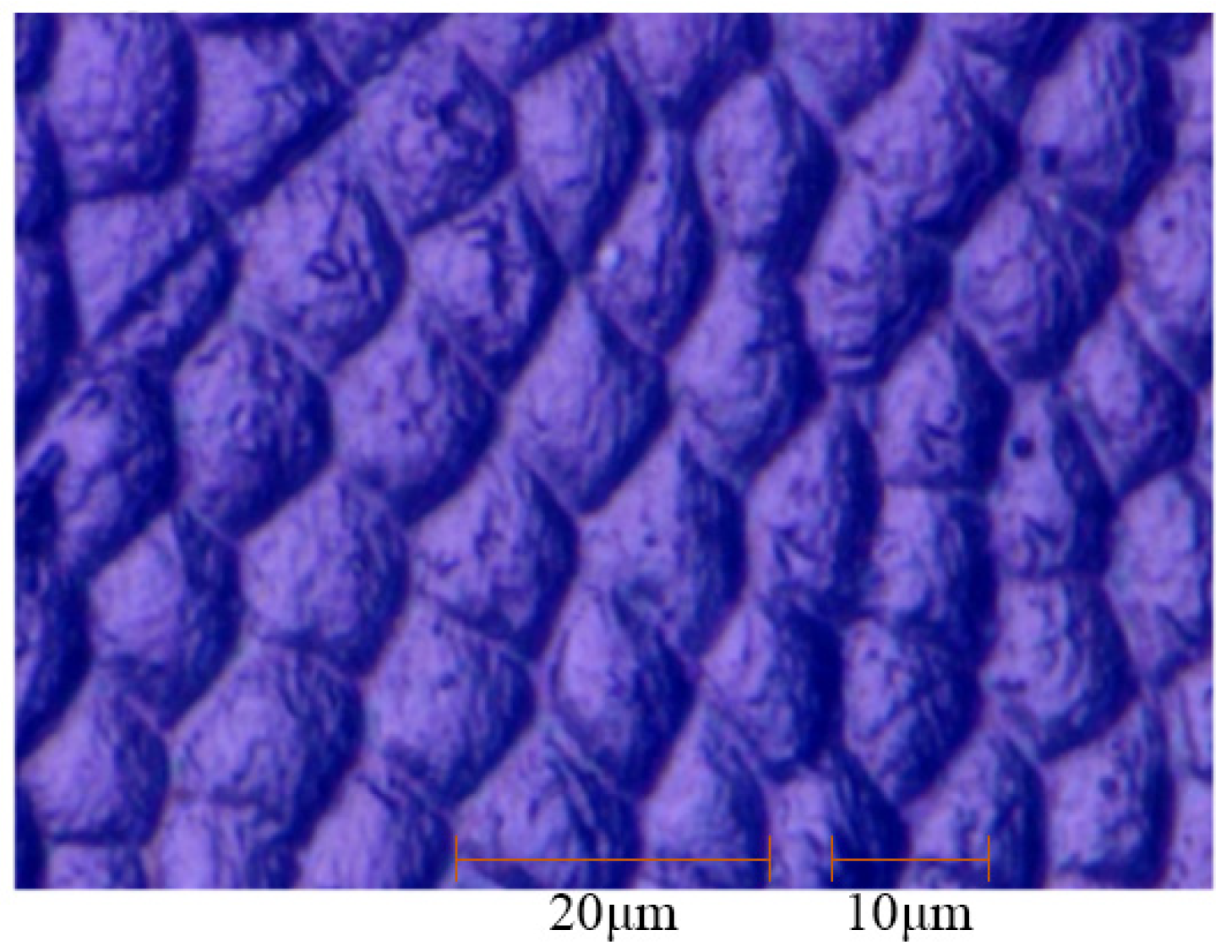
2.2.1. Extraction of the Surface Morphology of Dung Beetle
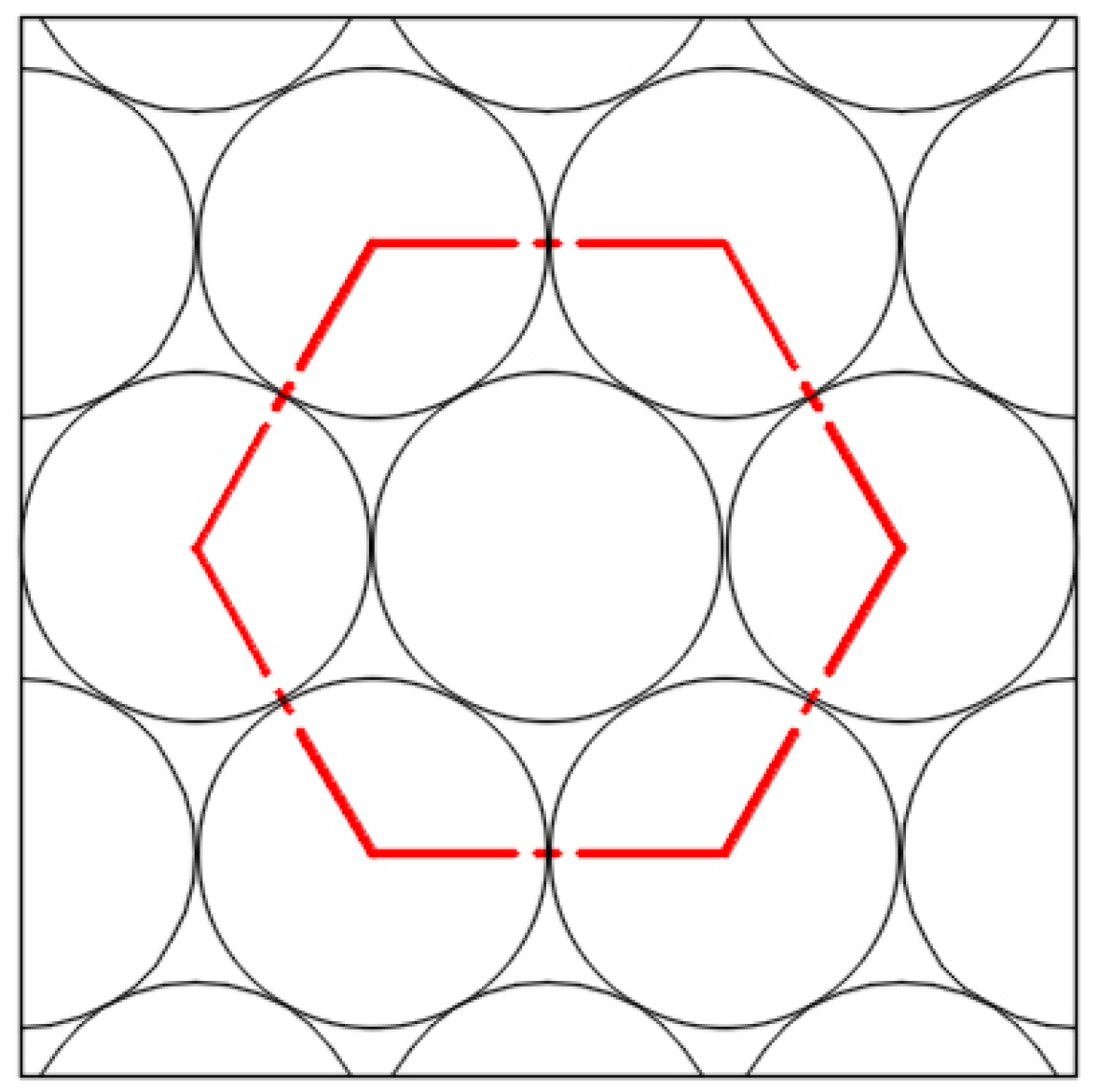
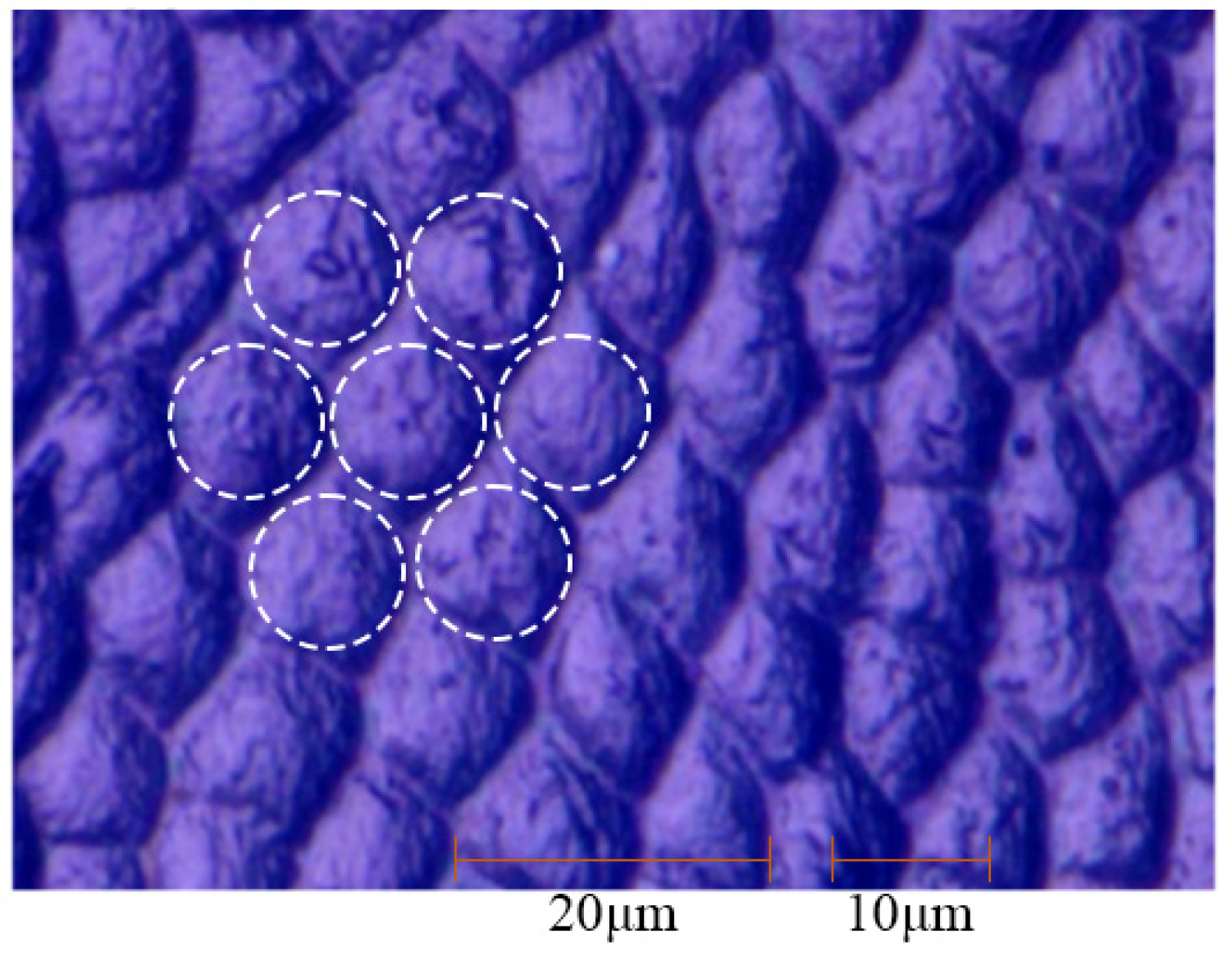
2.2.2. Biomimetic Surface Structure Design
2.2.3. Convex Hull Structure Arrangement Design
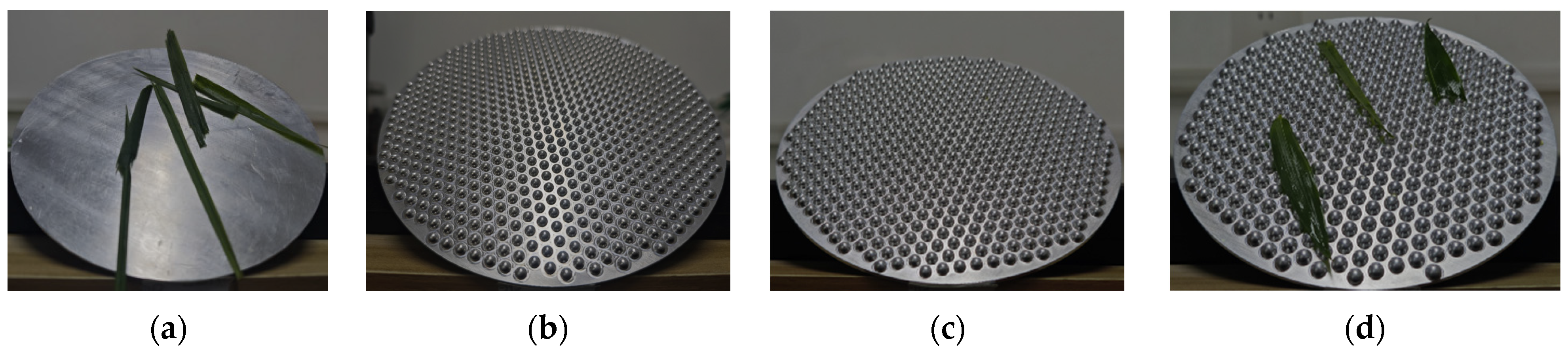
2.2.4. Bionic Convex Hull Metal Templates
2.3. The Establishment of the Mathematical Model
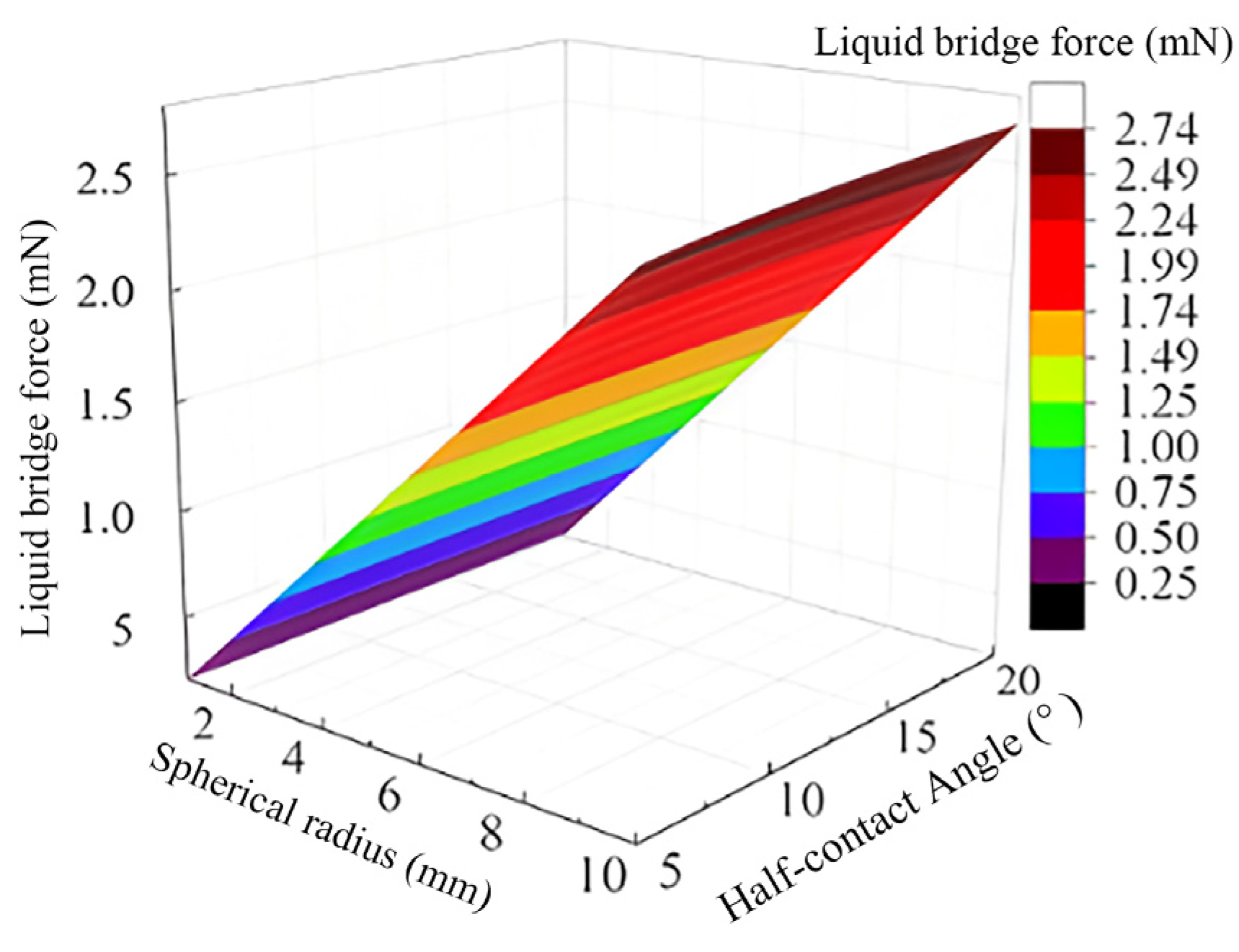
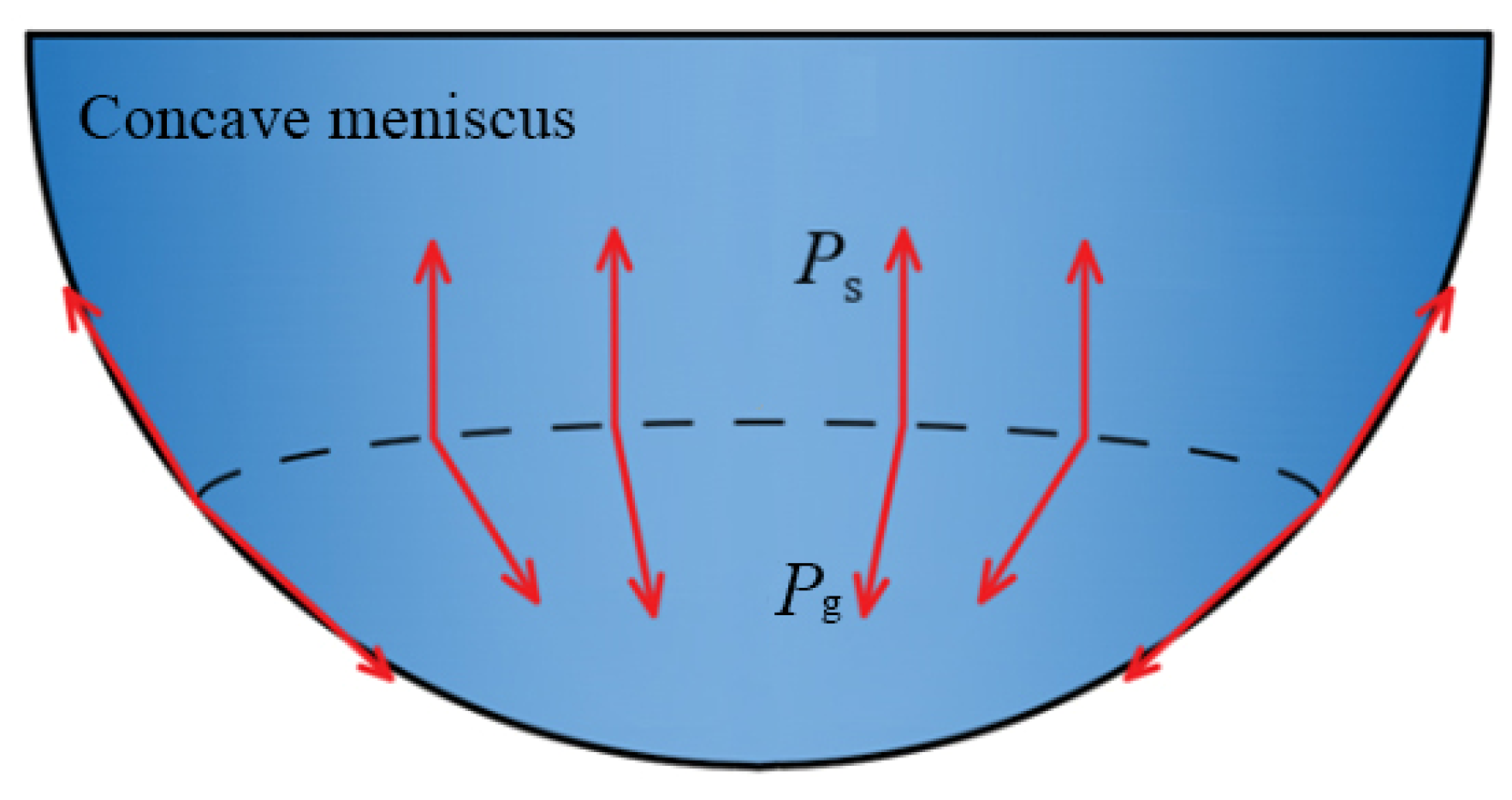
2.3.1. Establishment of the Liquid Bridge Model Between Sphere and Plane
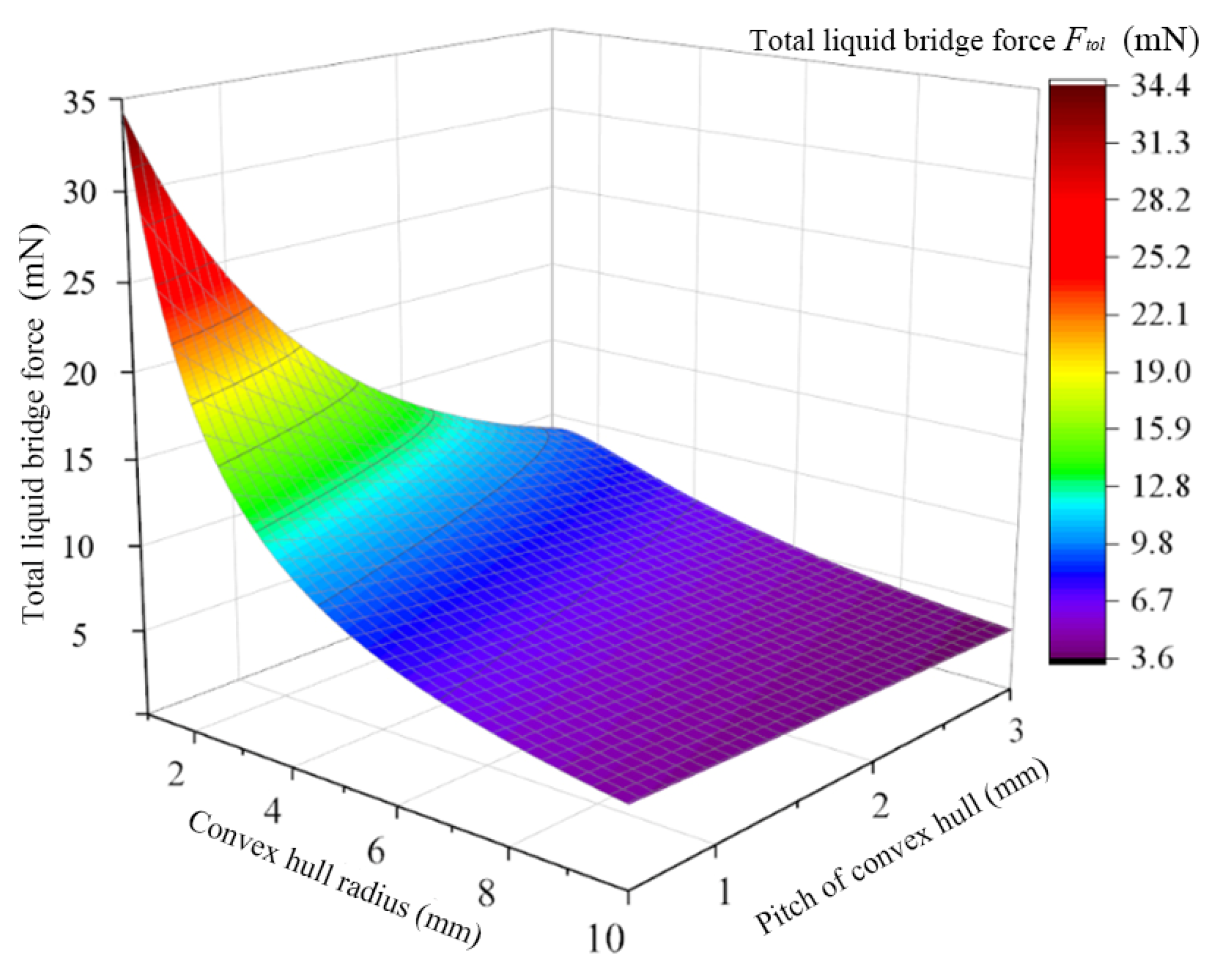
2.3.2. Establishment of a Functional Model of the Relationship Between the Total Liquid Bridge Force and the Radius and Spacing of Convex Hull
2.4. Experiment Specific Methods and Processes
2.4.1. Preparation of Wet Rice Leaves
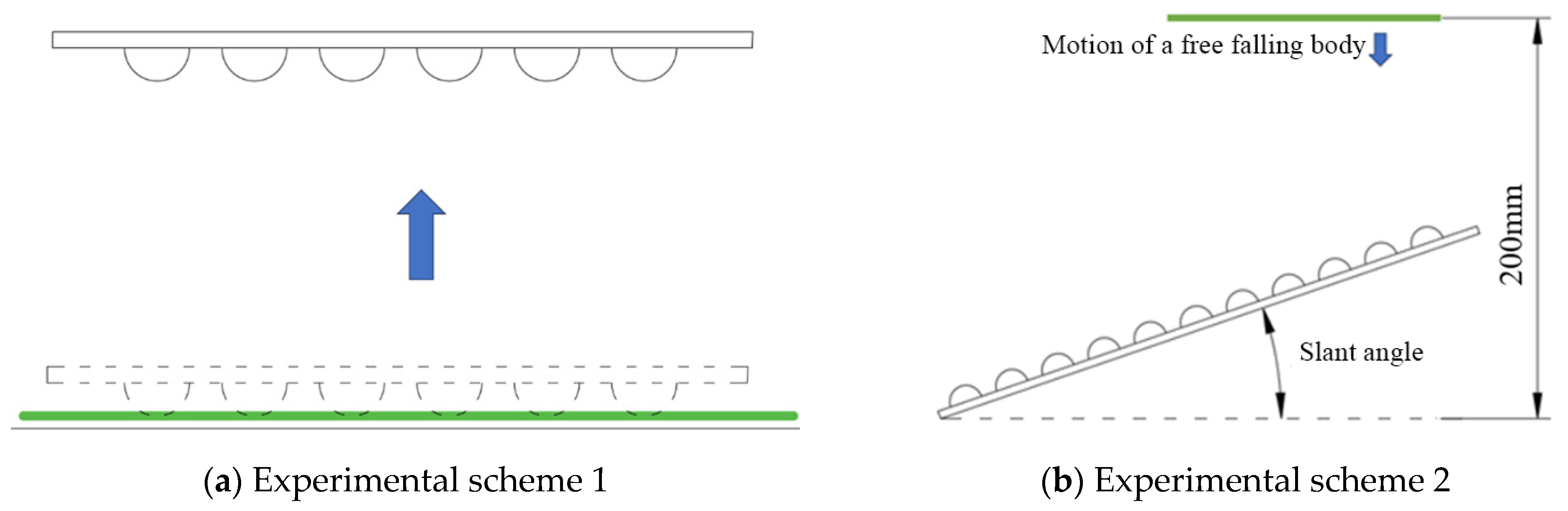
2.4.2. Design of Controlled Experiments
3. Discussion
3.1. Experimental Result
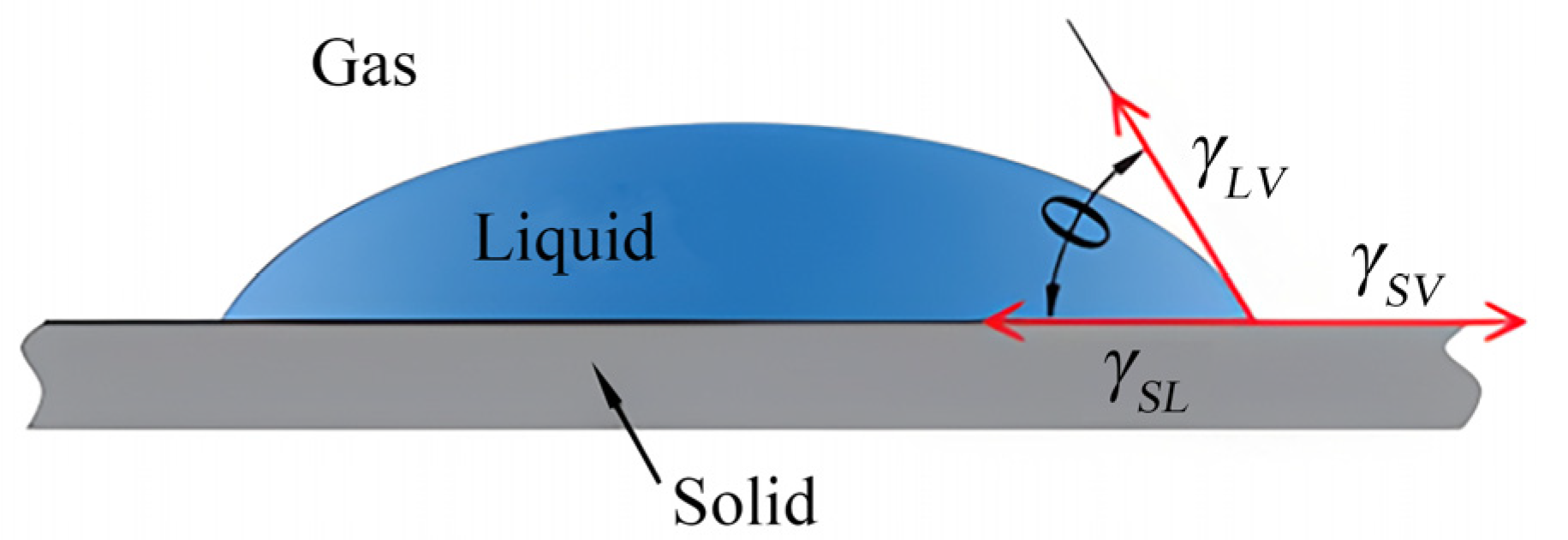
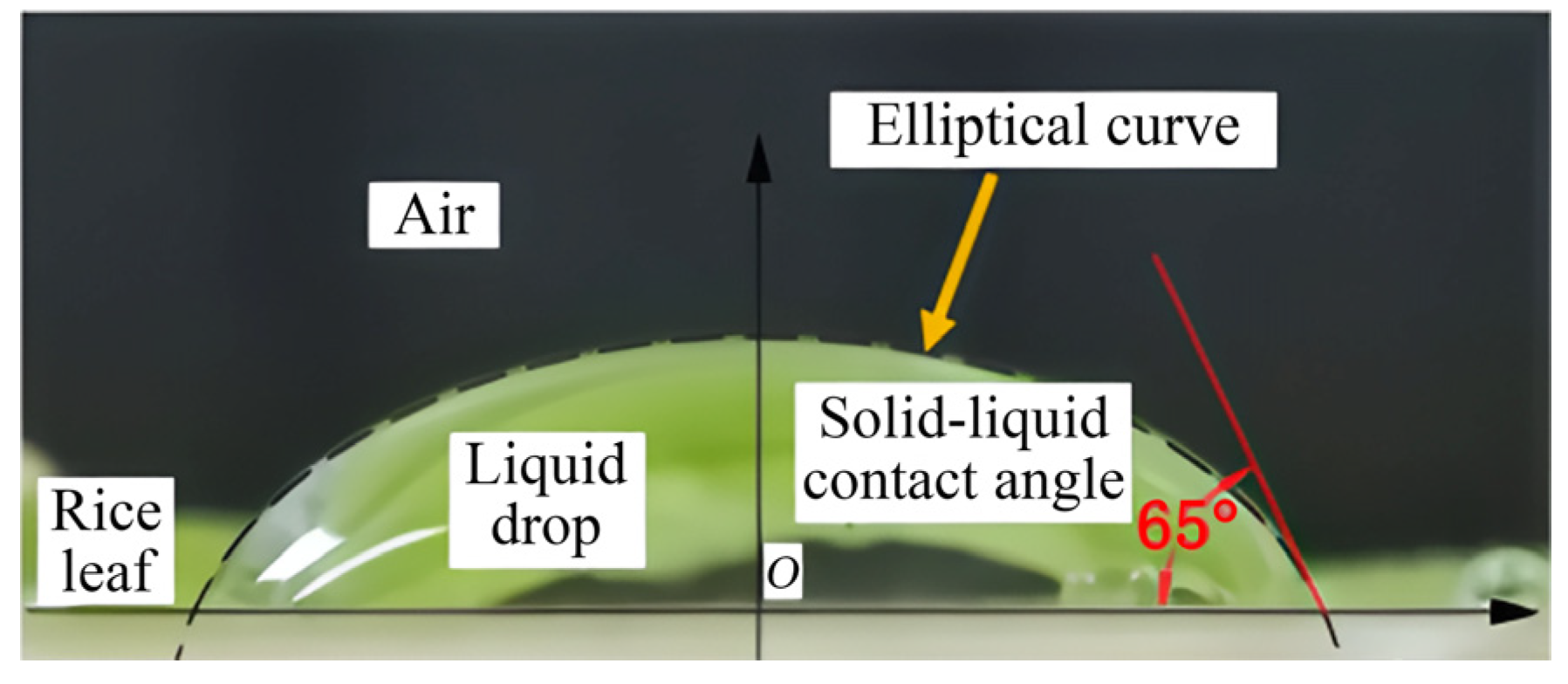
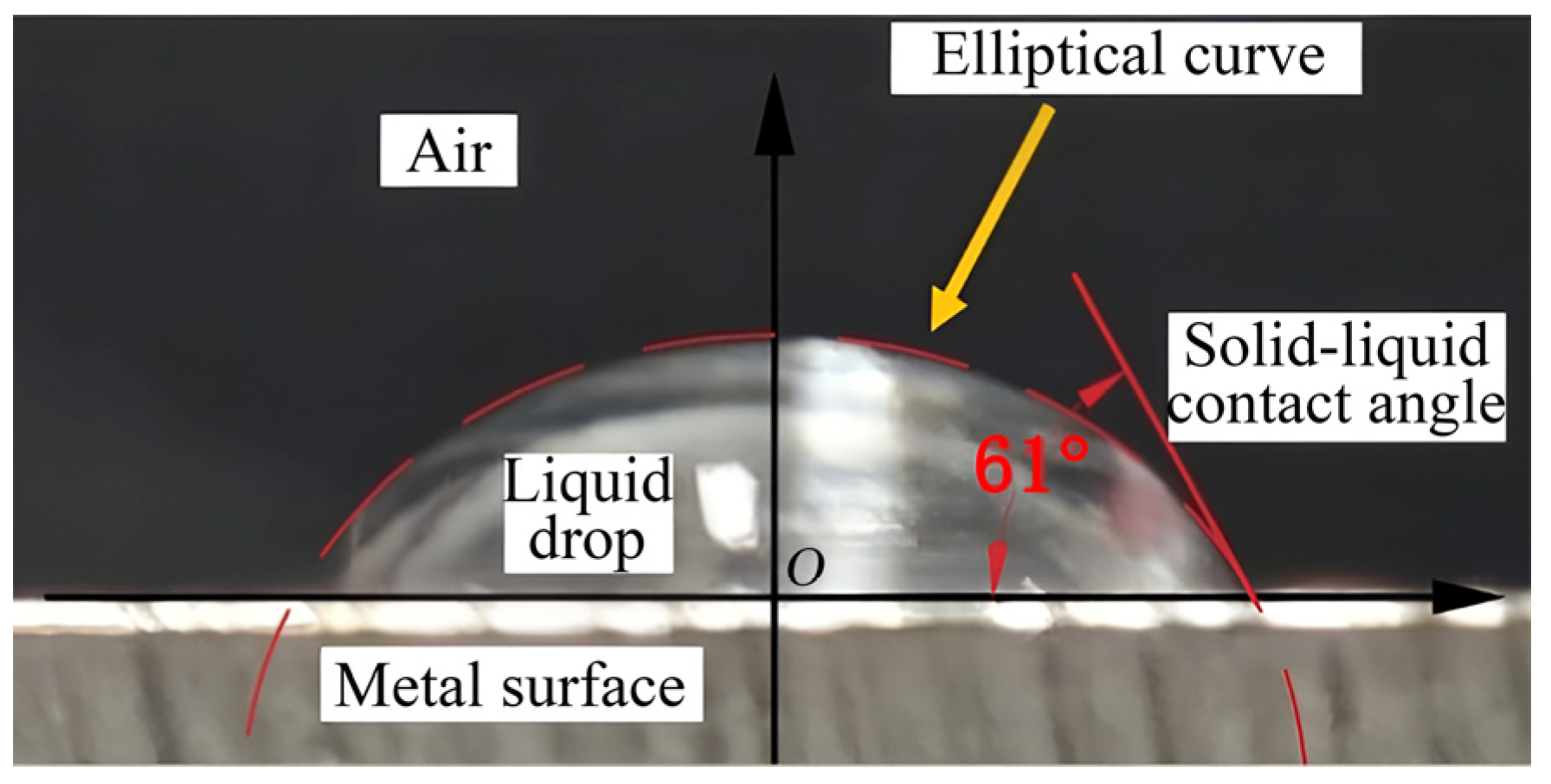
3.2. Surface Wetting Ability Analysis of Rice Leaves and Metal Plane
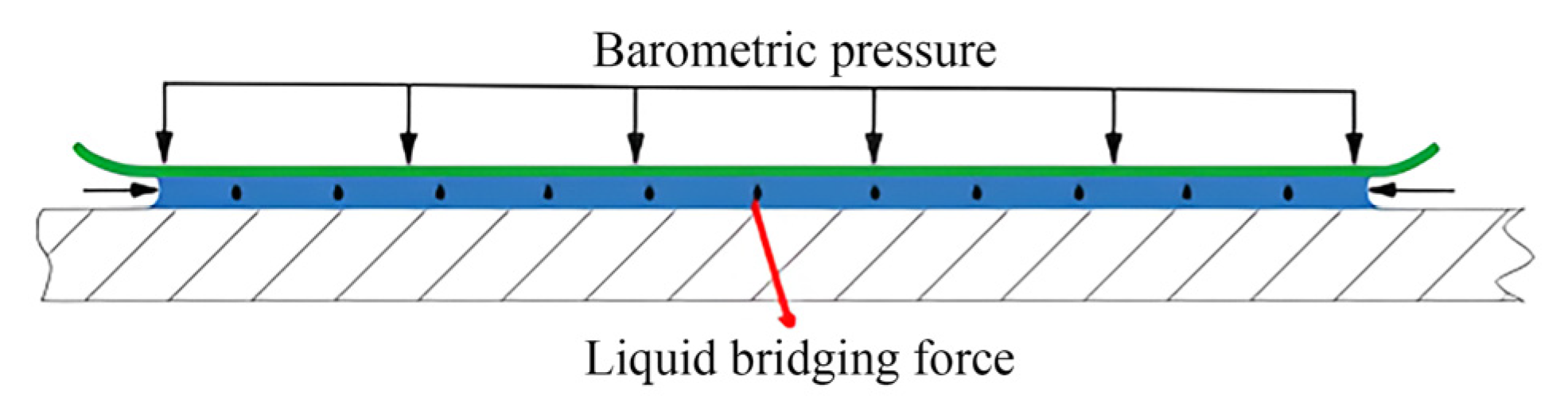
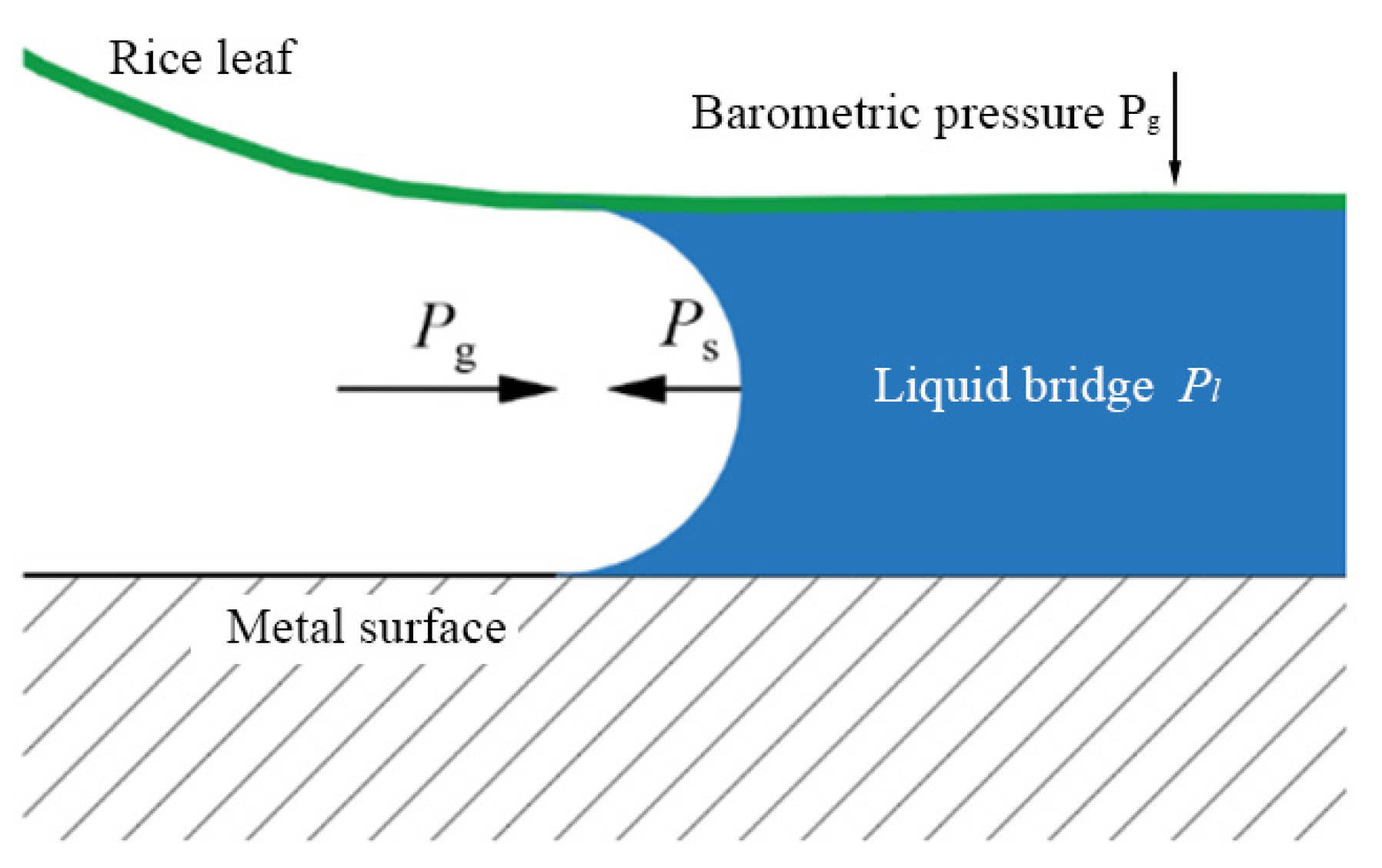
3.3. Mechanical Analysis Between Wet Rice Leaf and Metal Wall
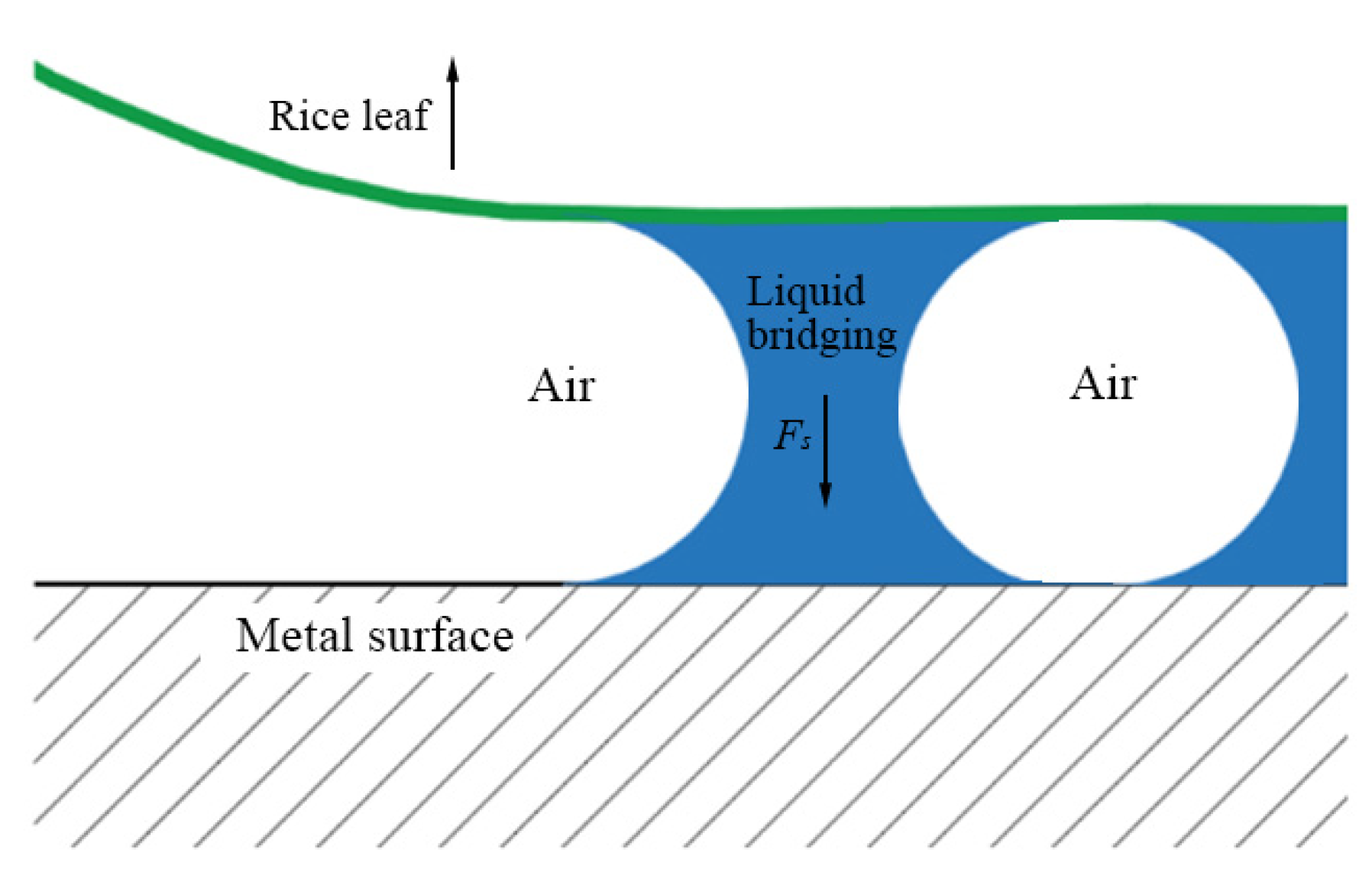
3.4. Analysis of Adhesion Reduction in the Dung Beetle Surface Convex Envelope Structure
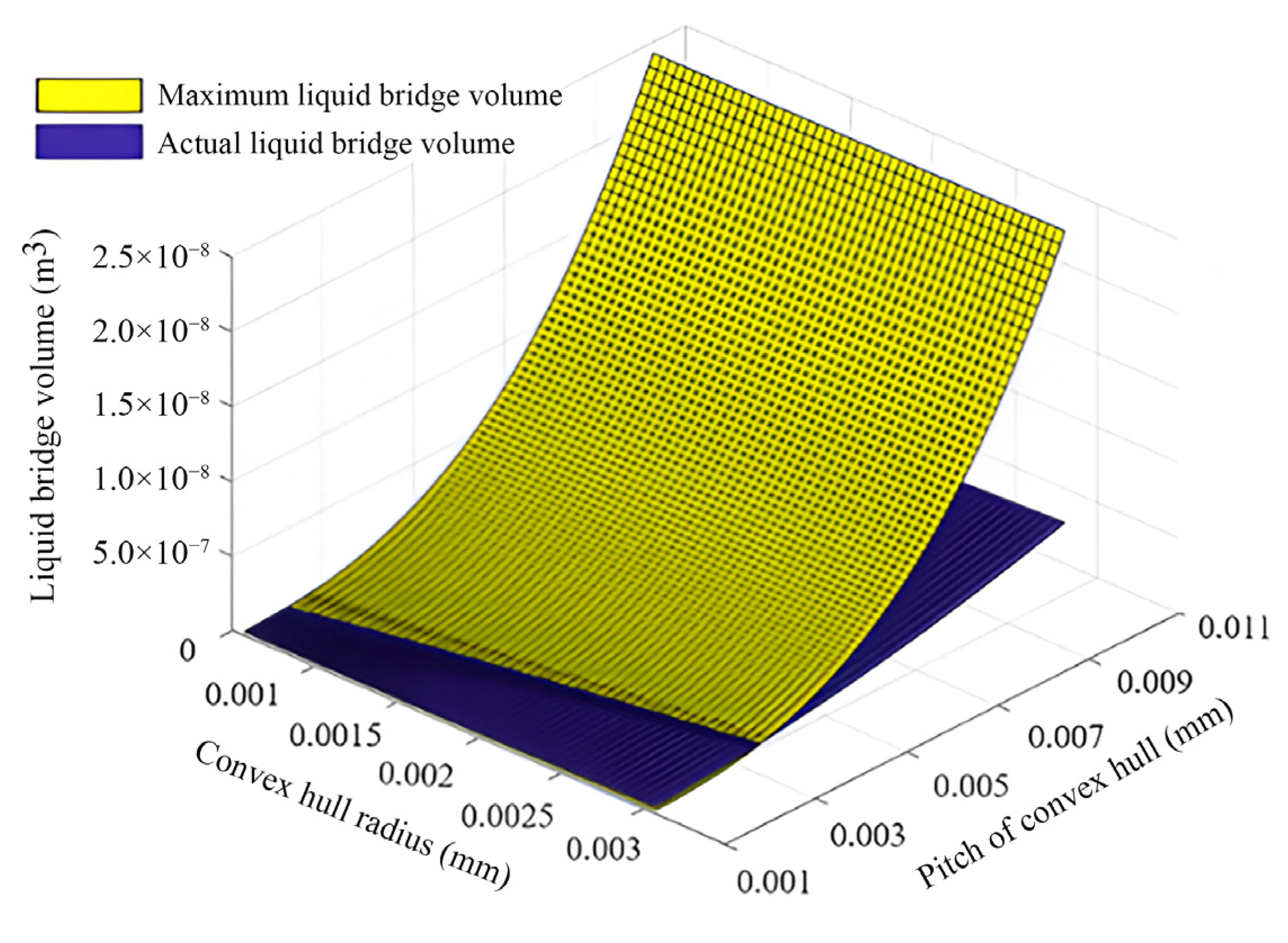
3.5. Analysis of Structural the Parameters of Bionic Convex Hull
4. Conclusions
- (1)
- The results show that the adhesion between the wet leaf and the wall is the largest when the additional pressure is present. When there is a gap between the convex hulls, that is, when there is an air layer between the wet leaves and the wall surface of the cleaning device, the adhesion effect would be reduced.
- (2)
- From the contact experiment results, it can be concluded that the convex hull surface structure can effectively reduce the static adhesion between the leaf and the wall. And the impact experiment results show that, compared with the plane surface, the adsorption capacity of the wet leaf on the convex hull surface is less, the shedding rate is higher, and the adhesion reduction effect is better.
- (3)
- A metal wall with a convex hull structure is proposed by the bionics method. The simulation results show that the minimum liquid bridge force exists when the convex hull radius and the convex hull spacing are 2.47 mm and 1.38 mm, respectively. The contact experiment results show that the static adhesion between the convex hull surface and the wet leaf is worse than that of the plane surface, and the adhesion reduction effect is better.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| β | Number of convex hull per unit area |
| rs | Radius of convex hull (m) |
| ds | Pitch of convex hull (m) |
| ∆p | Liquid bridge gas–liquid pressure difference (Pa) |
| γ | Liquid surface energy coefficient (0.0728 J at 20 °C) |
| ρ1 | Radius of liquid surface arc (m) |
| ρ2 | Distance from liquid level to center of rotation (m) |
| R | Spherical radius (m) |
| φ | Half-contact angle (°) |
| h | The distance from the sphere to the plane (m) |
| θ | Contact angle (°) |
| a | The length of line segment O2G (m) |
| V | Liquid bridge volume (m3) |
| Flg | Static liquid bridge force (N) |
| Vt | Volume of liquid attached to leaf surface (m3) |
| δL | Liquid mass per unit area of the leaf (kg) |
| Al | The area of one side surface of the leaf (m2) |
| ρl | The density of liquid (kg/m3) |
| nc | Number of convex hull in leaf contact |
| Ftol | Liquid bridge force between leaf and wall (N) |
| γSV | Solid–gas surface free energy (J) |
| γSL | Solid–liquid surface free energy (J) |
| γLV | Liquid–gas surface free energy (J) |
| Pl | The average pressure inside the liquid bridge (Pa) |
| Pg | Standard atmospheric pressure (Pa) |
| Ps | Additional pressure (Pa) |
| Fs | Liquid bridge resistance (N) |
| Al | Contact area between rice leaf and wall (m2) |
| Vs | The average volume of the liquid bridge between the individual convex hull and the leaf (m3) |
References
- Zhang, T.; Li, Y.M.; Xu, L.Z.; Liu, Y.B.; Ji, K.Z.; Jiang, S. Experimental Study on Fluidization Behaviors of Wet Rice Threshed Materials with Hot Airflow. Agriculture 2022, 12, 601. [Google Scholar] [CrossRef]
- Zhong, Y.; Sun, J.; Yao, K.; Cheng, J.; Du, X. Detection of rice (with husk) moisture content based on hyperspectral imaging technology combined with MSLPP–ESMA–SVR model. J. Food Saf. 2024, 44, e13112. [Google Scholar] [CrossRef]
- Liang, Z.; Li, Y.; De Baerdemaeker, J.; Xu, L.; Saeys, W. Development and testing of a multi-duct cleaning device for tangential-longitudinal flow rice combine harvesters. Biosyst. Eng. 2019, 182, 95–106. [Google Scholar] [CrossRef]
- Xu, L.; Chai, X.; Gao, Z.; Li, Y.; Wang, Y. Experimental study on driver seat vibration characteristics of crawler-type combine harvester. Int. J. Agric. Biol. Eng. 2019, 12, 90–97. [Google Scholar] [CrossRef]
- Ding, B.C.; Liang, Z.W.; Qi, Y.Q.; Ye, Z.K.; Zhou, J.H. Improving Cleaning Performance of Rice Combine Harvesters by DEM-CFD Coupling Technology. Agriculture 2022, 12, 1457. [Google Scholar] [CrossRef]
- Iqbal, B.; Alabbosh, K.F.; Jalal, A.; Suboktagin, S.; Elboughdiri, N. Sustainable food systems transformation in the face of climate change: Strategies, challenges, and policy implications. Food Sci. Biotechnol. 2024, 34, 871–883. [Google Scholar] [CrossRef]
- Ji, K.; Li, Y.; Liu, Y.; Yu, Z.; Cheng, J. Vibration signal extraction and analysis of combine harvester based on low-pass filter-eemd combination. Eng. Agrícola 2025, 44, e20240006. [Google Scholar] [CrossRef]
- Li, Y.; Xu, L.; Lv, L.; Shi, Y.; Yu, X. Study on Modeling Method of a Multi-Parameter Control System for Threshing and Cleaning Devices in the Grain Combine Harvester. Agriculture 2022, 12, 1483. [Google Scholar] [CrossRef]
- Zhang, T.; Li, Y.; You, G. Experimental Study on the Cleaning Performance of Hot Air Flow Cleaning Device. Agriculture 2023, 13, 1828. [Google Scholar] [CrossRef]
- Wang, L.; Chai, X.; Huang, J.; Hu, J.; Cui, Z. Efficient and Low-Loss Cleaning Method for Non-Uniform Distribution of Threshed Materials Based on Multi-Wing Curved Combination Air Screen in Computational Fluid Dynamics/Discrete Element Method Simulations. Agriculture 2024, 14, 895. [Google Scholar] [CrossRef]
- Liu, W.; Zeng, S.; Chen, X. Vortex Cleaning Device for Rice Harvester: Design and Bench Test. Agriculture 2024, 14, 866. [Google Scholar] [CrossRef]
- Cai, J.; Ma, J.; Zhang, Z.; Chen, X.; Liu, D.; Liang, C. Fragmentation of wet agglomerates after normal impact with a flat surface: Experimental study and DEM simulation. Chem. Eng. Sci. 2025, 307, 121339. [Google Scholar] [CrossRef]
- Liu, Y.; Li, Y.; Chen, L.; Zhang, T.; Liang, Z.; Huang, M.; Su, Z. Study on Performance of Concentric Threshing Device with Multi-Threshing Gaps for Rice Combines. Agriculture 2021, 11, 1000. [Google Scholar] [CrossRef]
- Hussain, S.; Jianjun, H.; Yong, C.; Ali, A.; Song, H.; Zheng, D.; Farid, M.U.; Ghafoor, A.; Ahmed, M. CFD study of self-cleaning system of multi-stage tangential roller threshing unit for precise buckwheat breeding. Heliyon 2024, 10, e27180. [Google Scholar] [CrossRef]
- Fu, J.; Zhang, M.; Cheng, C.; Zhao, H.; Ren, L. Mechanism study of the effect of a surface liquid film on the collision adhesion behaviour of rice stalks. Biosyst. Eng. 2025, 251, 61–72. [Google Scholar] [CrossRef]
- Cheng, C.; Fu, J.; Chen, Z.; Ren, L. Effect of vibration parameters of vibrating screen for harvester on adhesion characteristics of threshed mixtures with different moistures. Trans. Chin. Soc. Agric. Eng. Trans. CSAE 2019, 35, 29–36. [Google Scholar] [CrossRef]
- De Bisschop, F.R.E.; Rigole, W.J.L. A physical model for liquid capillary bridges between adsorptive solid spheres: The nodoid of plateau. J. Colloid Interface Sci. 1982, 88, 117–128. [Google Scholar] [CrossRef]
- Lian, G.; Thornton, C.; Adams, M.J. A Theoretical Study of the Liquid Bridge Forces between Two Rigid Spherical Bodies. J. Colloid Interface Sci. 1993, 161, 138–147. [Google Scholar] [CrossRef]
- Pitois, O.; Moucheront, P.; Chateau, X. Liquid Bridge between Two Moving Spheres: An Experimental Study of Viscosity Effects. J. Colloid Interface Sci. 2000, 231, 26–31. [Google Scholar] [CrossRef]
- Pitois, O.; Moucheront, P.; Chateau, X. Rupture energy of a pendular liquid bridge. Eur. Phys. J. B-Condens. Matter Complex Syst. 2001, 23, 79–86. [Google Scholar] [CrossRef]
- Darabi, P.; Li, T.; Pougatch, K.; Salcudean, M.; Grecov, D. Modeling the evolution and rupture of stretching pendular liquid bridges. Chem. Eng. Sci. 2010, 65, 4472–4483. [Google Scholar] [CrossRef]
- Soulié, F.; Cherblanc, F.; El Youssoufi, M.S.; Saix, C. Influence of liquid bridges on the mechanical behaviour of polydisperse granular materials. Int. J. Numer. Anal. Methods Geomech. 2006, 30, 213–228. [Google Scholar] [CrossRef]
- Rossetti, D.; Pepin, X.; Simons, S.J.R. Rupture energy and wetting behavior of pendular liquid bridges in relation to the spherical agglomeration process. J. Colloid Interface Sci. 2003, 261, 161–169. [Google Scholar] [CrossRef] [PubMed]
- Lu, N.; Lechman, J.; Miller, K.T. Experimental Verification of Capillary Force and Water Retention between Uneven-Sized Spheres. J. Eng. Mech. 2008, 134, 385–395. [Google Scholar] [CrossRef]
- Lievano, D.; Velankar, S.; McCarthy, J.J. The rupture force of liquid bridges in two and three particle systems. Powder Technol. 2017, 313, 18–26. [Google Scholar] [CrossRef]
- Wang, J.-P.; Gallo, E.; François, B.; Gabrieli, F.; Lambert, P. Capillary force and rupture of funicular liquid bridges between three spherical bodies. Powder Technol. 2017, 305, 89–98. [Google Scholar] [CrossRef]
- Pu, C.; Liu, F.; Zhang, Z.; Cheng, J.; Zhao, W. Experimental study on liquid force and profile between two moving sphere particles under different liquid content condition. J. Hydraul. Eng. 2020, 51, 81–91. [Google Scholar]
- Liu, J.-L.; Li, G.-S.; Nie, Z.-X. Morphology and Liquid Bridge Force of an Axisymmetric Liquid Bridge. J. Xihua Univ. Nat. Sci. Ed. 2010, 29, 1–5. [Google Scholar]
- Wang, X.W.; Yu, Y. Study of Gravitation Effect on Rupture Distance of Liquid Bridge between Two Flat Substrates. J. Exp. Mech. 2012, 27, 70–76. [Google Scholar]
- He, Y.; Wu, J.; Xiao, S.; Fang, H.; Zheng, Q. Investigating the Wettability of Rapeseed Leaves. Appl. Eng. Agric. 2021, 37, 399–409. [Google Scholar] [CrossRef]
- Pang, D.; Cong, H.; Li, X.; Li, H.; Gao, X. Liquid-bridge Flow between Two Slender Plates: Formation and Fluid Mechanics. Chem. Eng. Res. Des. 2021, 170, 304–313. [Google Scholar] [CrossRef]
- Yang, L.; Chen, Z.Y.; Xiao, H.P. Calculation of volume at both ends of liquid bridge between two parallel plates. Coll. Phys. 2021, 40, 12–14. [Google Scholar] [CrossRef]
- Wang, X.; Zhou, H.; Tong, J. The simplification and analytical verification of the static liquid bridge force model for particle adhesion in inferior seedling substrates. Surf. Interfaces 2024, 53, 104994. [Google Scholar] [CrossRef]
- Ma, J.; Liu, K.; Dong, X.; Chen, C.; Qiu, B.; Zhang, S. Effects of Leaf Surface Roughness and Contact Angle on In Vivo Measurement of Droplet Retention. Agronomy 2022, 12, 2228. [Google Scholar] [CrossRef]
- Zhao, J.; Yu, G.; Jiang, G. Fabrication of a Bionic Superhydrophobic Surface with Photothermal and Electrothermal Performance for All-Weather Anti-Icing. Langmuir ACS J. Surf. Colloids 2025, 41, 7580–7591. [Google Scholar] [CrossRef]
- Neinhuis, C.; Barthlott, W. Characterization and Distribution of Water-repellent, Self-cleaning Plant Surfaces. Ann. Bot. 1997, 79, 667–677. [Google Scholar] [CrossRef]
- Lin, N.; Liu, X.Y. Correlation between hierarchical structure of crystal networks and macroscopic performance of mesoscopic soft materials and engineering principles. Chem. Soc. Rev. 2015, 44, 7881–7915. [Google Scholar] [CrossRef]
- Yang, J.; Liu, G.; Zhang, K.; Li, P.; Yan, H.; Yan, Y.; Zheng, Y.; Zhao, Z.; Zhang, L.; Liu, X.; et al. Sunflower-Inspired Superhydrophobic Surface with Composite Structured Microcone Array for Anisotropy Liquid/Ice Manipulation (Small 52/2024). Small 2024, 20, 2470390. [Google Scholar] [CrossRef]
- Li, J.; Deng, J.; Zhou, C.; Yang, J.; Shin, S.; Binks, B.P.; Cho, N.J. Biomimetic Superhydrophobic Surfaces by Nanoarchitectonics with Natural Sunflower Pollen (Small 7/2025). Small 2025, 21, 2570048. [Google Scholar] [CrossRef]
- Jing, Z.; Ding, J.; Zhang, T.; Yang, D.; Qiu, F.; Chen, Q.; Xu, J. Flexible, versatility and superhydrophobic biomass carbon aerogels derived from corn bracts for efficient oil/water separation. Food Bioprod. Process. 2019, 115, 134–142. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, X.; Shi, J.; Liu, L.; Zhang, X.; Zou, X.; Xiao, J.; Zhai, X.; Zhang, D.; Li, Y.; et al. A visual bi-layer indicator based on roselle anthocyanins with high hydrophobic property for monitoring griskin freshness. Food Chem. 2021, 355, 129573. [Google Scholar] [CrossRef] [PubMed]
- Yue, P.; Zhang, M.; Zhao, T.; Liu, P.; Peng, F.; Yang, L. Eco-friendly epoxidized Eucommia ulmoides gum based composite coating with enhanced super-hydrophobicity and corrosion resistance properties. Ind. Crops Prod. 2024, 214, 118523. [Google Scholar] [CrossRef]
- Sharifi, N.; Pugh, M.; Moreau, C.; Dolatabadi, A. Developing hydrophobic and superhydrophobic TiO2 coatings by plasma spraying. Surf. Coat. Technol. 2016, 289, 29–36. [Google Scholar] [CrossRef]
- Yang, Y.; Li, X.; Zheng, X.; Chen, Z.; Zhou, Q.; Chen, Y. 3D-Printed Biomimetic Super-Hydrophobic Structure for Microdroplet Manipulation and Oil/Water Separation. Adv. Mater. 2018, 30, 1704912. [Google Scholar] [CrossRef]
- Guan, C.; Fu, J.; Cui, Z.; Wang, S.; Gao, Q.; Yang, Y. Evaluation of the tribological and anti-adhesive properties of different materials coated rotary tillage blades. Soil Tillage Res. 2021, 209, 104933. [Google Scholar] [CrossRef]
- Xie, Z.; Tian, Y.; Zhong, F.; Xiang, G.; Li, S.; Yuan, Q. Enhancing bond properties between epoxy resins and cementitious materials through entropy-driven hydrophobic interaction. Compos. Part B Eng. 2025, 296, 112251. [Google Scholar] [CrossRef]
- Feng, S.; Delannoy, J.; Malod, A.; Zheng, H.; Quéré, D.; Wang, Z. Tip-induced flipping of droplets on Janus pillars: From local reconfiguration to global transport. Sci. Adv. 2020, 6, eabb4540. [Google Scholar] [CrossRef]
- Barthlott, W.; Schimmel, T.; Wiersch, S.; Koch, K.; Brede, M.; Barczewski, M.; Walheim, S.; Weis, A.M.; Kaltenmaier, A.; Leder, A.; et al. The Salvinia Paradox: Superhydrophobic Surfaces with Hydrophilic Pins for Air Retention Under Water. Adv. Mater. 2010, 22, 2325–2328. [Google Scholar] [CrossRef]
- Vuckovac, M.; Latikka, M.; Liu, K.; Huhtamäki, T.; Ras, R.H.A. Uncertainties in contact angle goniometry. Soft Matter 2019, 15, 7089–7096. [Google Scholar] [CrossRef]
- Yuan, L.; Tang, Z.; Liu, S.; Wang, T.; Ding, Z. Design for Copying Grouser and Bionic Convex Hull Patterns on Track Surfaces of Crawler Combine Harvesters. Agriculture 2024, 14, 1079. [Google Scholar] [CrossRef]
- Zhan, Y.; Pang, Z.; Tan, G. Optically/thermally dual-responsive shape memory superhydrophobic surfaces with advanced multi-functionalities. Compos. Part A Appl. Sci. Manuf. 2025, 192, 108812. [Google Scholar] [CrossRef]
- Hotta, K.; Takeda, K.; Iinoya, K. The capillary binding force of a liquid bridge. Powder Technol. 1974, 10, 231–242. [Google Scholar] [CrossRef]
- Willett, C.; Adams, M.; Johnson, S.; Seville, J.P.K. Capillary Bridges between Two Spherical Bodies. Langmuir 2000, 16, 9396–9405. [Google Scholar] [CrossRef]
- Mikami, T.; Kamiya, H.; Horio, M. Numerical simulation of cohesive powder behavior in a fluidized bed. Chem. Eng. Sci. 1998, 53, 1927–1940. [Google Scholar] [CrossRef]
- Bian, X.; Huang, H.; Chen, L. Formation of special liquid bridges between a single plate and parallel plates. AIP Adv. 2019, 9, 095018. [Google Scholar] [CrossRef]
- He, Z.T.; Wu, C.M.; Yu, J.j.; Li, Y.R. Dynamic analysis of adsorbate behavior in nanopores: Liquid bridge formation, cavitation, and contact angle evaluation via molecular dynamics simulations. J. Mol. Liq. 2024, 414, 126146. [Google Scholar] [CrossRef]
- Jeyanthi, S.; Venkatakrishnaiah, R.; Raju, K.V.B. Multilayer geocell-reinforced soils using mayfly optimisation predicts circular foundation load settlement. Int. J. Hydromechatron. 2024, 7, 31–48. [Google Scholar] [CrossRef]
- Cunegatto, E.H.T.; Zinani, F.S.F.; Rigo, S.J. Multi-objective optimisation of micromixer design using genetic algorithms and multi-criteria decision-making algorithms. Int. J. Hydromechatron. 2024, 7, 224–249. [Google Scholar] [CrossRef]






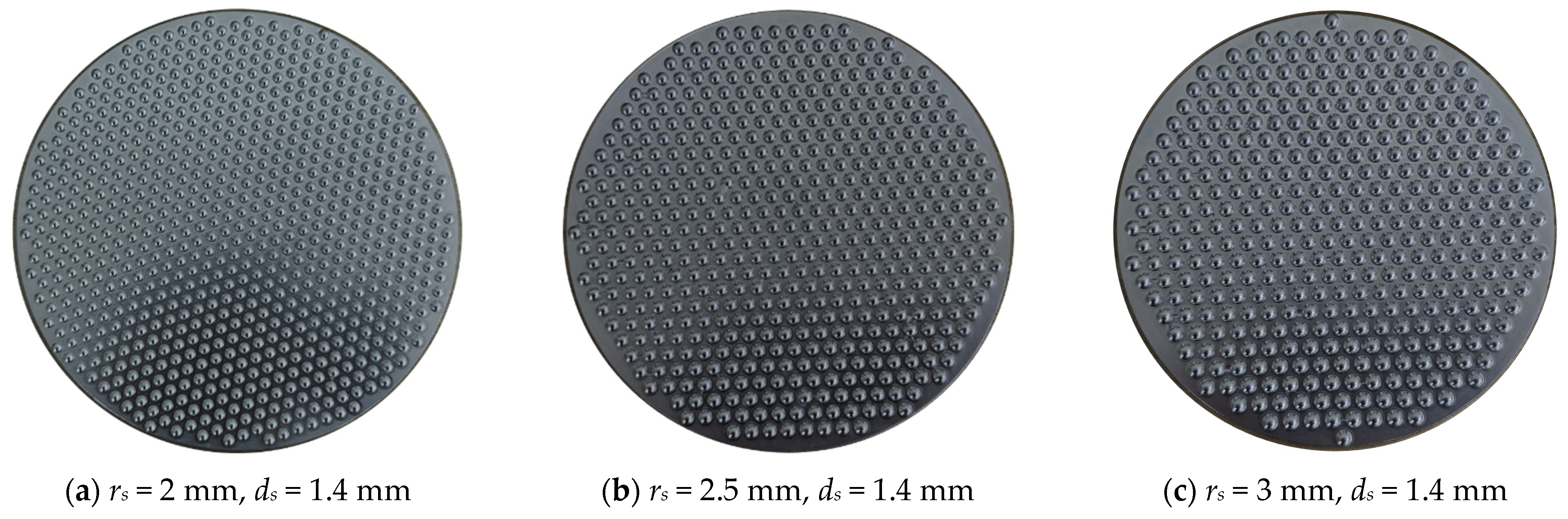
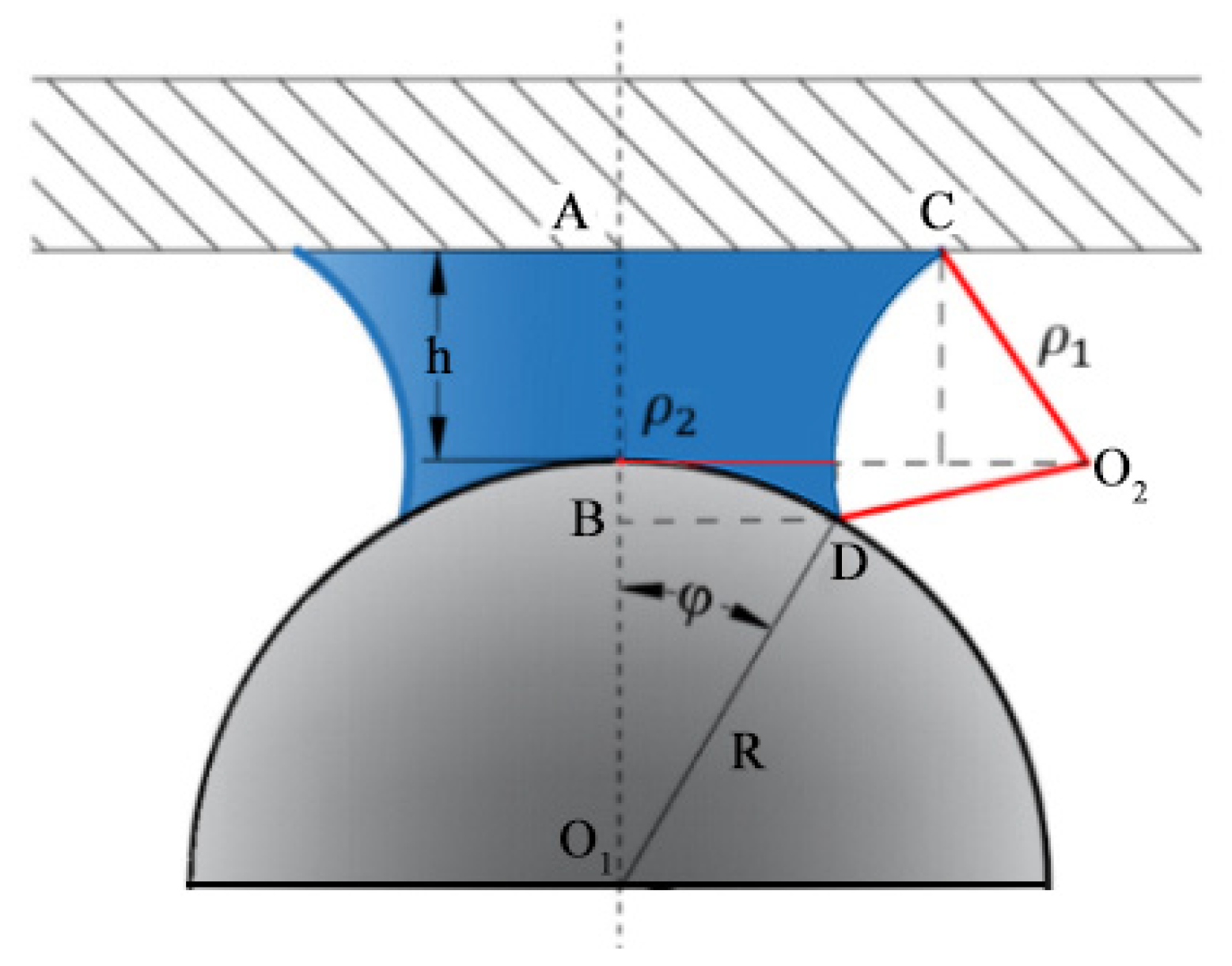
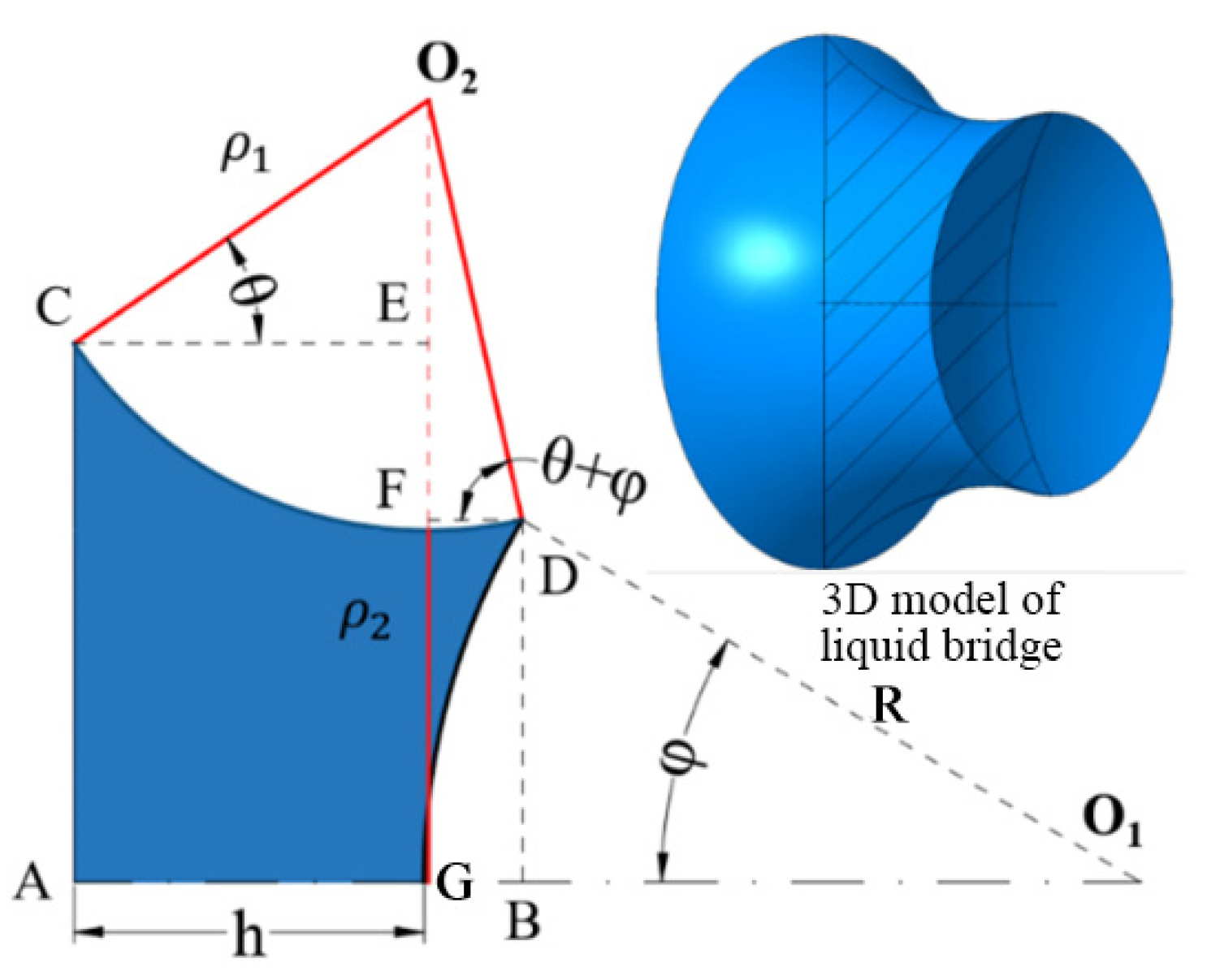
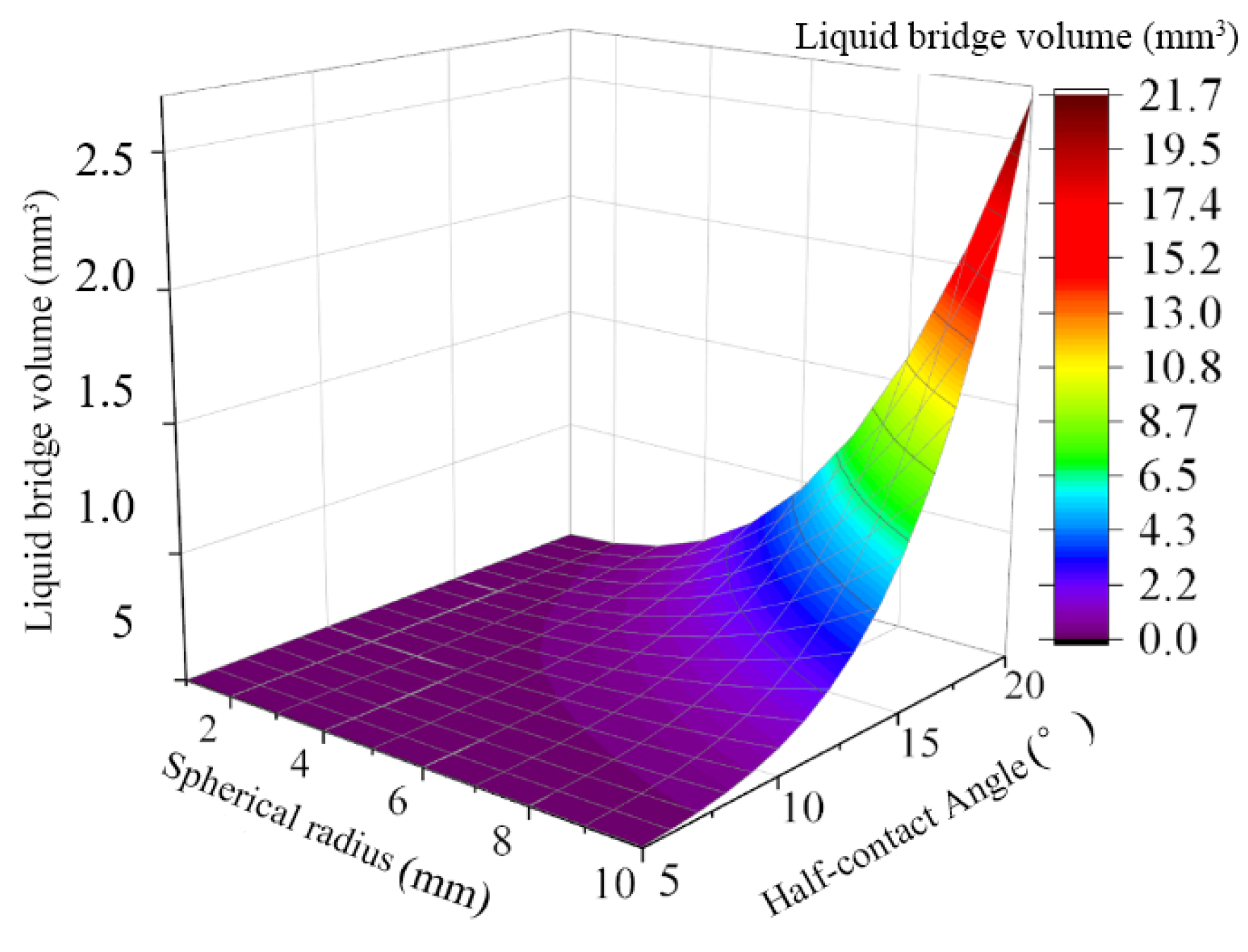




| Varieties | Country of Origin/Time | Leaf Mass per Area (g·m−2) | Leaf Thickness (mm) | Leaf Density (mg·mm−3) |
|---|---|---|---|---|
| Y-Liangyou No. 1 | China/2006 | 50.7 ± 0.9 | 0.33 ± 0.09 | 0.155 ± 0.003 |
| Y-Liangyou No. 2 | China/2011 | 49.4 ± 0.6 | 0.33 ± 0.03 | 0.151 ± 0.002 |
| Group 1 (g) | Group 2 (g) | Group 3 (g) | Group 4 (g) | Group 5 (g) | Average (g) | ||
|---|---|---|---|---|---|---|---|
| Leaves | Unevaporated | 18.4 | 22.7 | 18.8 | 17.4 | 21.2 | 19.7 |
| After evaporation | 14.9 | 18.4 | 15.2 | 14.1 | 17.4 | 16 | |
| Mass change value | 3.5 | 4.3 | 3.6 | 3.3 | 3.8 | 3.7 |
| Time | 1 (g) | 2 (g) | 3 (g) | 4 (g) | 5 (g) | Average (g) | Sample Standard Deviation |
|---|---|---|---|---|---|---|---|
| Plane | 1.38 | 0.98 | 1.27 | 1.08 | 1.24 | 1.19 | 0.142 |
| Convex hull surface rs = 2 mm | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| Convex hull surface rs = 2.5 mm | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| Convex hull surface rs = 3 mm | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| Inclination Angle | Time | 1 (g) | 2 (g) | 3 (g) | 4 (g) | 5 (g) | Average (g) | Sample Standard Deviation |
|---|---|---|---|---|---|---|---|---|
| 20° | Plane | 2.12 | 2.23 | 1.98 | 2.17 | 1.83 | 2.066 | 0.144 |
| Convex hull surface rs = 2 mm | 0.31 | 0.43 | 0.51 | 0.36 | 0.47 | 0.416 | 0.073 | |
| Convex hull surface rs = 2.5 mm | 0.63 | 0.46 | 0.55 | 0.42 | 0.45 | 0.502 | 0.077 | |
| Convex hull surface rs = 3 mm | 0.52 | 0.49 | 0.61 | 0.46 | 0.42 | 0.500 | 0.064 | |
| 45° | Plane | 0.28 | 0.35 | 0.24 | 0.24 | 0.39 | 0.300 | 0.060 |
| Convex hull surface rs = 2 mm | 0 | 0 | 0 | 0.08 | 0.05 | 0.026 | 0.033 | |
| Convex hull surface rs = 2.5 mm | 0 | 0 | 0.04 | 0 | 0 | 0.008 | 0.016 | |
| Convex hull surface rs = 3 mm | 0.23 | 0.21 | 0.18 | 0.14 | 0.15 | 0.182 | 0.034 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qian, P.; He, Q.; Tang, Z.; Gu, T. Biomimetic Structural Design for Reducing the Adhesion Between Wet Rice Leaves and Metal Surfaces. Agriculture 2025, 15, 921. https://doi.org/10.3390/agriculture15090921
Qian P, He Q, Tang Z, Gu T. Biomimetic Structural Design for Reducing the Adhesion Between Wet Rice Leaves and Metal Surfaces. Agriculture. 2025; 15(9):921. https://doi.org/10.3390/agriculture15090921
Chicago/Turabian StyleQian, Pengfei, Qi He, Zhong Tang, and Tingwei Gu. 2025. "Biomimetic Structural Design for Reducing the Adhesion Between Wet Rice Leaves and Metal Surfaces" Agriculture 15, no. 9: 921. https://doi.org/10.3390/agriculture15090921
APA StyleQian, P., He, Q., Tang, Z., & Gu, T. (2025). Biomimetic Structural Design for Reducing the Adhesion Between Wet Rice Leaves and Metal Surfaces. Agriculture, 15(9), 921. https://doi.org/10.3390/agriculture15090921








