Finite Element Simulations and Experimental Analysis for Efficient Mugwort Harvesting
Abstract
1. Introduction
2. Materials and Methods
2.1. Three-Dimensional Engineering Modelling of the Branch, Stem, and Leaf of Mugwort
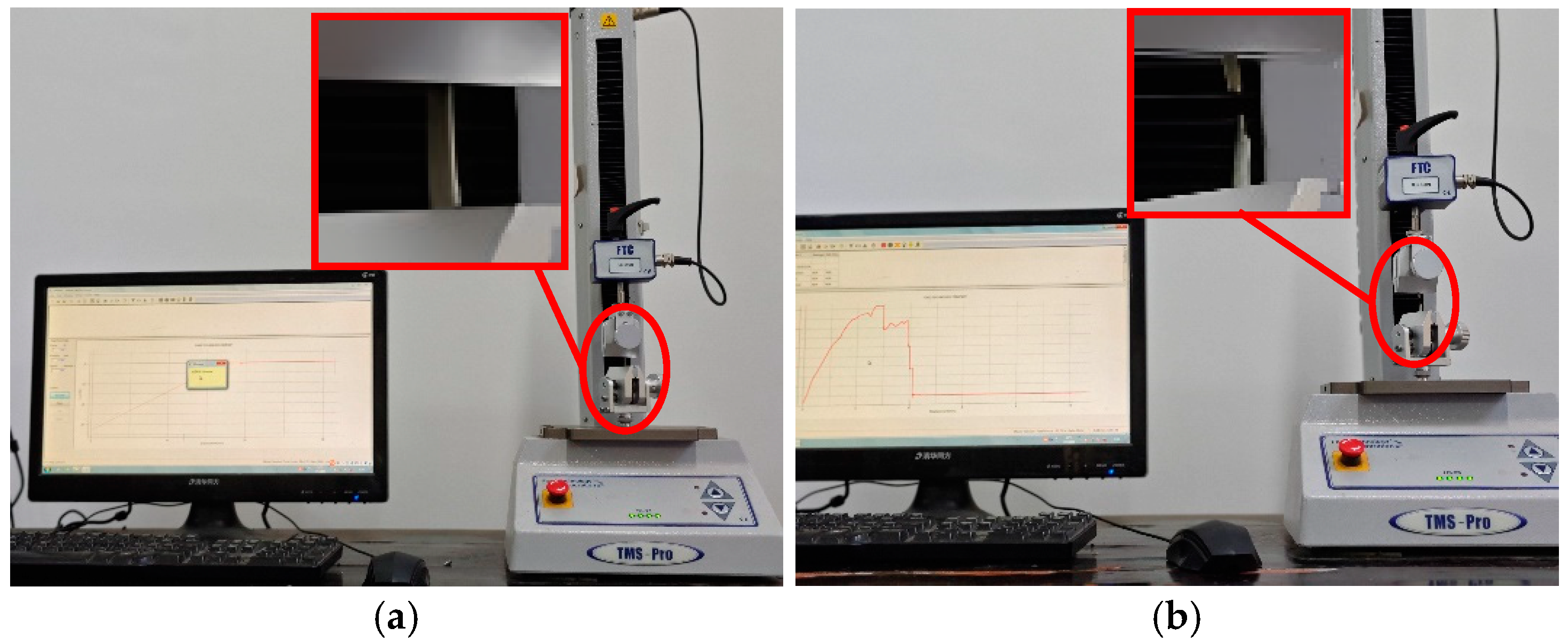
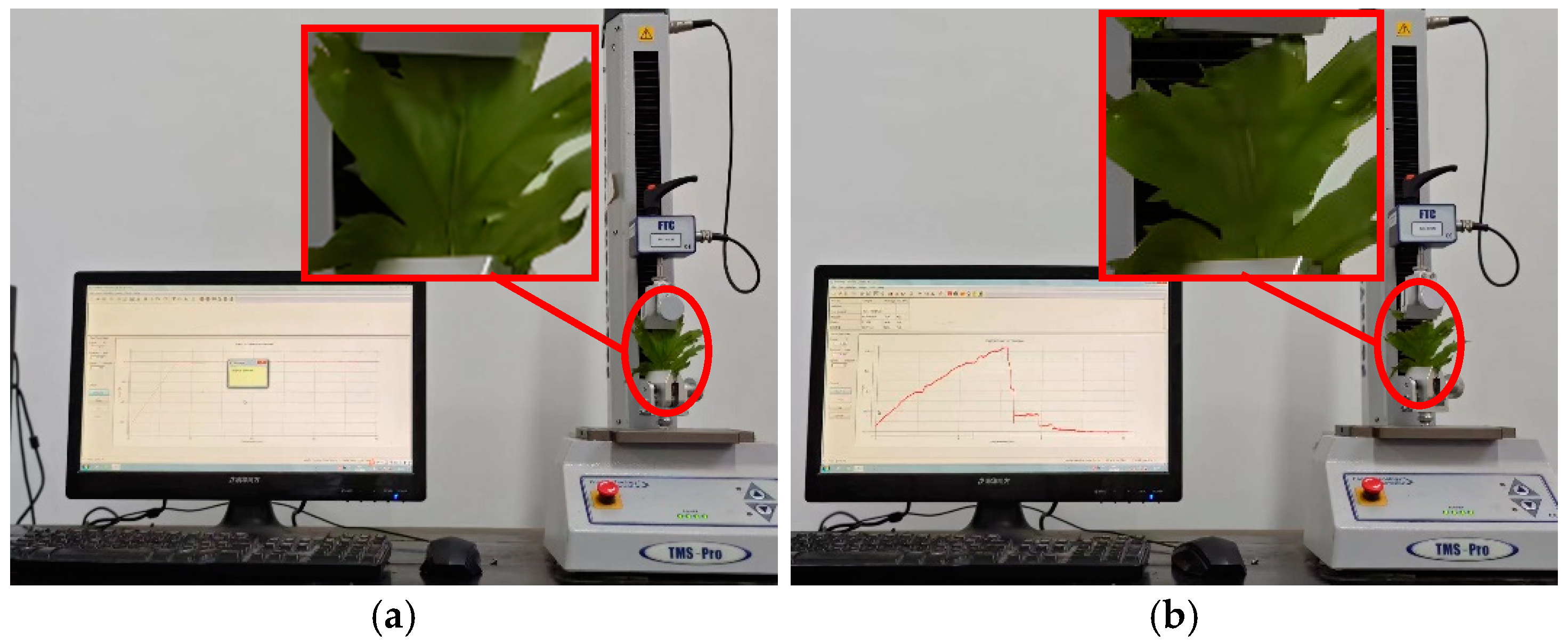
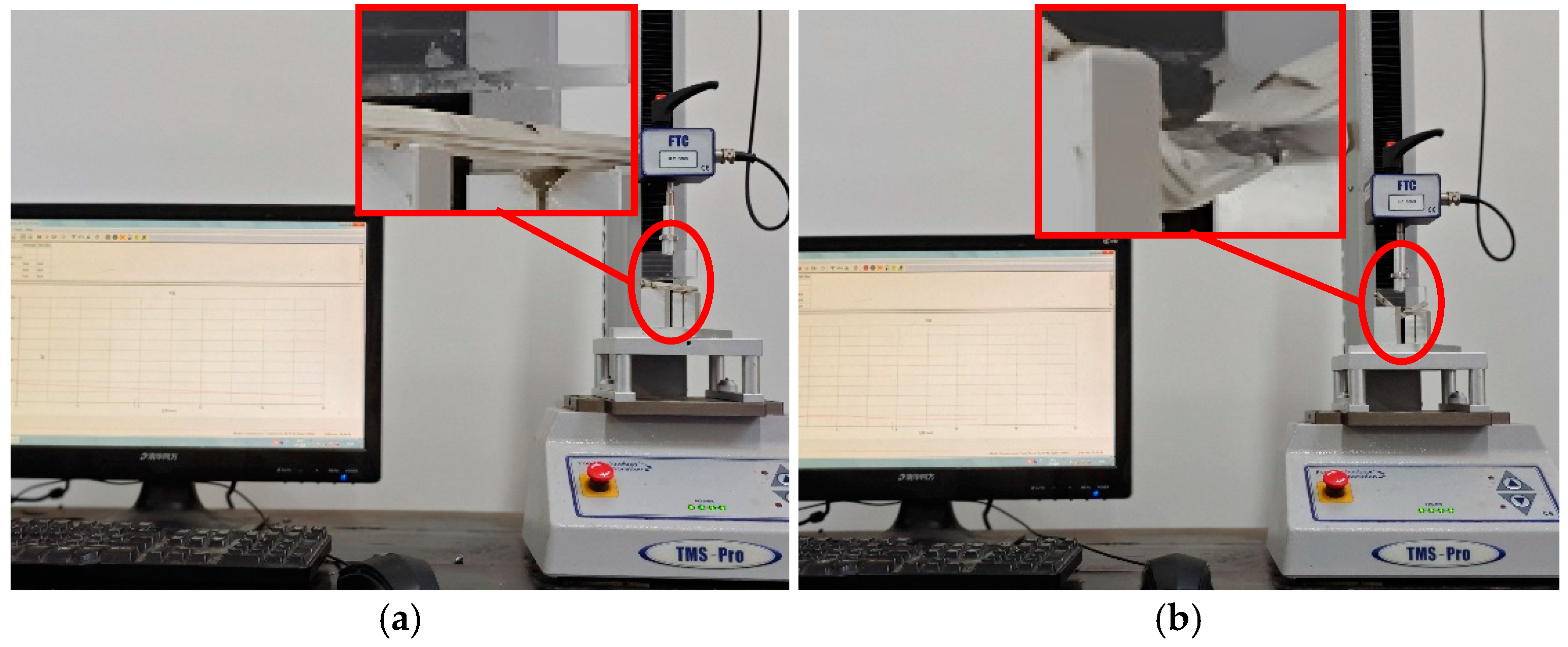
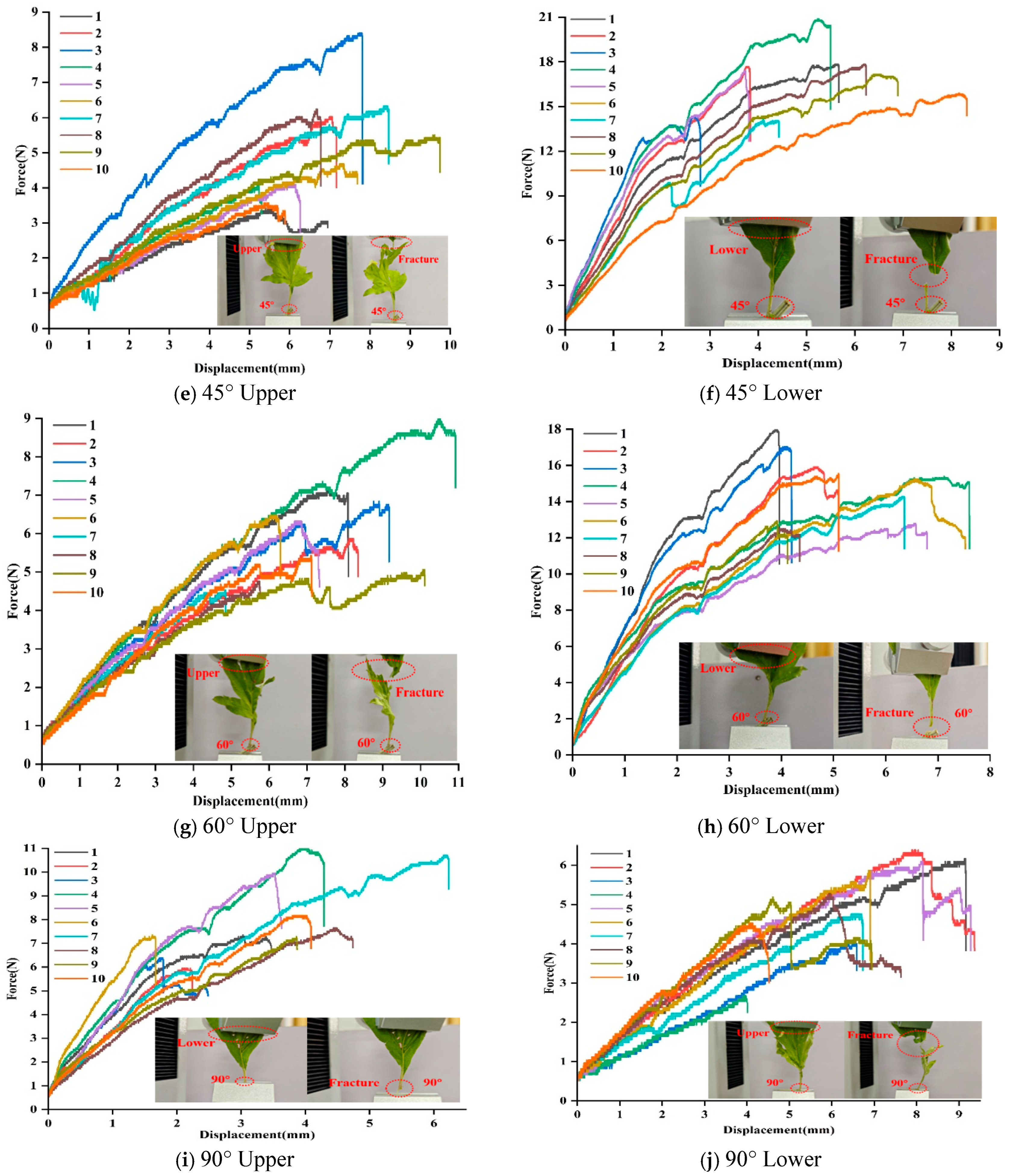
2.2. Physical Tests on Branch, Stem, and Leaf of Mugwort
2.3. Mechanical Modelling of Branch, Stem, and Leaf of Mugwort
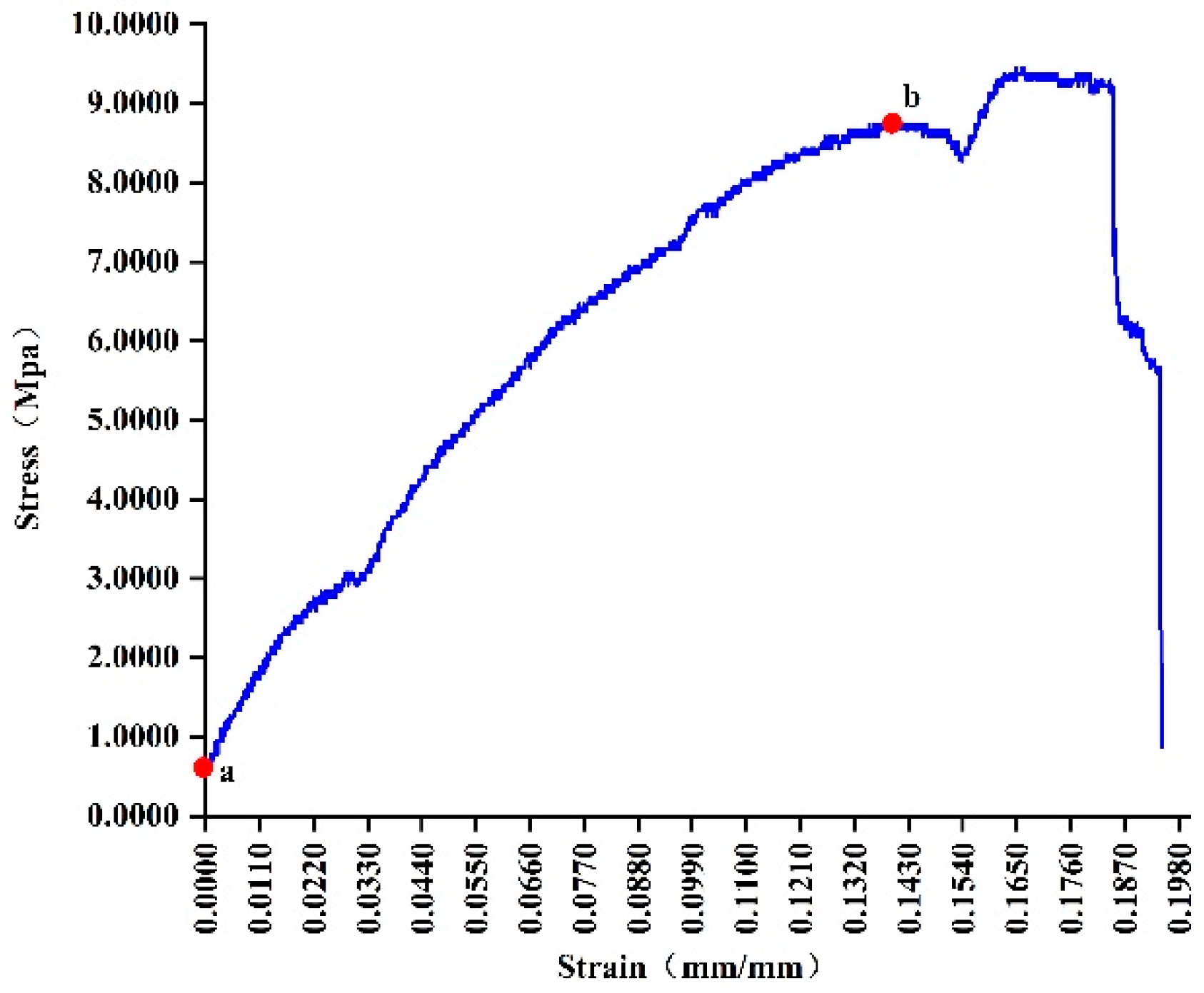
2.3.1. Elastic Moduli
2.3.2. Densities
2.3.3. Poisson Ratio
2.4. Harvesting Simulation Based on the Finite Element Method
3. Results and Discussion
3.1. Harvesting Simulation Analysis
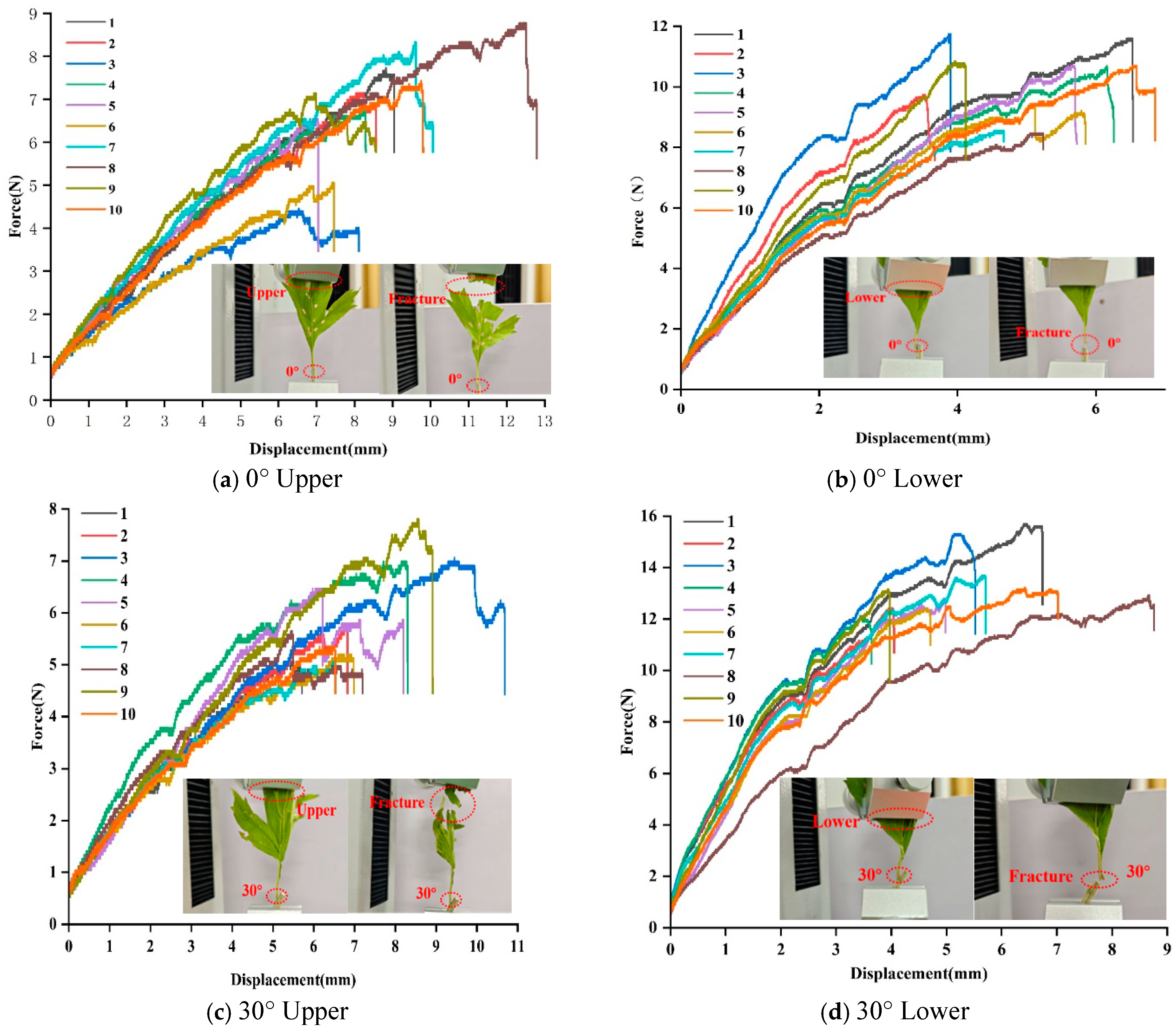
3.2. Field Test Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Anwar, F.; Ahmad, N.; Alkharfy, K.M.; Gilani, A.-u.-H. Mugwort (Artemisia vulgaris) Oils. In Essential Oils in Food Preservation, Flavor and Safety; Academic Press: Cambridge, MA, USA, 2016. [Google Scholar] [CrossRef]
- Kim, W.-S.; Choi, W.J.; Lee, S.; Kim, W.J.; Lee, D.C.; Sohn, U.D.; Shin, H.-S.; Kim, W. Anti-inflammatory, Antioxidant and Antimicrobial Effects of Artemisinin Extracts from Artemisia annua L. Korean J. Physiol. Pharmacol. 2015, 19, 21–27. [Google Scholar] [CrossRef] [PubMed]
- Pandey, A.K.; Singh, P. The Genus Artemisia: A 2012–2017 Literature Review on Chemical Composition, Antimicrobial, Insecticidal and Antioxidant Activities of Essential Oils. Medicines 2017, 4, 68. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, A.; Gangopadhyay, D.; Chowdhury, T. Economical and Environmental Importance of Mulberry: A Review. Int. J. Plant Environ. 2017, 3, 67–72. [Google Scholar] [CrossRef]
- Szopa, A.; Pajor, J.; Klin, P.; Rzepiela, A.; Elansary, H.; Al-Mana, F.; Mattar, M.; Ekiert, H. Artemisia absinthium L.—Importance in the History of Medicine, the Latest Advances in Phytochemistry and Therapeutical, Cosmetological and Culinary Uses. Plants 2020, 9, 1063. [Google Scholar] [CrossRef] [PubMed]
- Yurchyshyn, O. Search for biologically actuve substances with antimicrobial and antibiotic potentiating properties amoung members of the genus artemisia againts the main pathogens of infectious skin lesions. Art Med. 2021, 5, 84–89. [Google Scholar] [CrossRef]
- Jin, S.; Wang, Y.; Liu, T.; Ning, X.; Liang, R.; Hu, K.; Cao, J. Preparation and Application of Volatilized Wormwood Essence Derived Naturally into Green Insecticide. Molecules 2024, 29, 2877. [Google Scholar] [CrossRef] [PubMed]
- Guyer, D. Mechanical Harvesting. In Advanced Automation for Tree Fruit Orchards and Vineyards; Springer: Cham, Switzerland, 2023. [Google Scholar] [CrossRef]
- Pargi Sanjay, J.; Gupta, P.; Balas, P.R.; Bambhaniya, V.U. Comparison between Manual Harvesting and Mechanical Harvesting. J. Sci. Res. Rep. 2024, 30, 917–934. [Google Scholar] [CrossRef]
- Wang, D. Structural Design and Experimental Analysis of High Efficiency Mugwort Defoliation Machine. Master’s Thesis, Hubei University of Technology, Wuhan, China, 2020. [Google Scholar] [CrossRef]
- Dong, W.T. Design and Experimental Study on the Defoliation Machine of Mugwort. Master’s Thesis, Huazhong Agricultural University, Wuhan, China, 2022. [Google Scholar] [CrossRef]
- Sun, S. Experimental Study on the Influencing Factors of Defoliation Quality of Mugwort Defoliation Machine; Hubei University of Technology: Wuhan, China, 2018; CNKI:CDMD:2.1018.957170. [Google Scholar]
- Hou, J.; Yu, Y.; Tang, Z.; Zhang, L.; Jin, J.; Wang, W. Optimization and experiment on mechanical vibration harvesting process parameters of mulberry. INMATEH Agric. Eng. 2024, 72, 557–568. [Google Scholar] [CrossRef]
- Wang, W.; Lu, H.; Zhang, S.; Yang, Z. Damage caused by multiple impacts of litchi fruits during vibration harvesting. Comput. Electron. Agric. 2019, 162, 732–738. [Google Scholar] [CrossRef]
- Gilman, E. Branch-to-Stem Diameter Ratio Affects Strength of Attachment. J. Arboric. 2003, 29, 291–294. [Google Scholar] [CrossRef]
- Kane, B.; Farrell, R.; Zedaker, S.; Loferski, J.; Smith, D. Failure Mode and Prediction of the Strength of Branch Attachments. Arboric. Urban For. 2008, 34, 308–316. [Google Scholar] [CrossRef]
- Li, J.; Karkee, M.; Zhang, Q.; Xiao, K.; Feng, T. Characterizing apple picking patterns for robotic harvesting. Comput. Electron. Agric. 2016, 127, 633–640. [Google Scholar] [CrossRef]
- Davidson, J.; Silwal, A.; Karkee, M.; Mo, C.; Zhang, Q. Hand-Picking Dynamic Analysis for Undersensed Robotic Apple Harvesting. Trans. ASABE 2016, 59, 745–758. [Google Scholar] [CrossRef]
- Zhao, J.; Tsuchikawa, S.; Ma, T.; Hu, G.; Yun, C.; Wang, Z.; Chen, Q.; Gao, Z.; Chen, J. Modal Analysis and Experiment of a Lycium barbarum L. Shrub for Efficient Vibration Harvesting of Fruit. Agriculture 2021, 11, 519. [Google Scholar] [CrossRef]
- Zhao, J.; Ma, T.; Inagaki, T.; Chen, Q.; Gao, Z.; Sun, L.; Cai, H.; Chen, C.; Li, C.; Zhang, S.; et al. Finite Element Method Simulations and Experiments of Detachments of Lycium barbarum L. Forests 2021, 12, 699. [Google Scholar] [CrossRef]
- Yun, C.; Zhao, J.; Chen, Q.; Chen, J. Simulation for Fitting the Bending Shape of Fruit Branches of Lycium barbarum Based on the Finite Element Method. Horticulturae 2021, 7, 434. [Google Scholar] [CrossRef]
- Zhao, J.; Sugirbay, A.; Chen, Y.; Zhang, S.; Liu, F.; Bu, L.; Yun, C.; Wang, Z.; Chen, J. FEM explicit dynamics simulation and NIR hyperspectral reflectance imaging for determination of impact bruises of Lycium barbarum L. Postharvest Biol. Technol. 2019, 155, 102–110. [Google Scholar] [CrossRef]
- Celik, H.K. Determination of bruise susceptibility of pears (Ankara variety) to impact load by means of FEM-based explicit dynamics simulation. Postharvest Biol. Technol. 2017, 128, 83–97. [Google Scholar] [CrossRef]
- Nie, H.-B. Determination of elastic modulus of cemented carbides by three-point bend tests. Fenmo Yejin Cailiao Kexue Yu Gongcheng/Mater. Sci. Eng. Powder Metall. 2010, 15, 606–610. [Google Scholar]
- Silberschmidt, V. Comprehensive Mechanics of Materials; Elsevier: Amsterdam, The Netherlands, 2024. [Google Scholar]
- Qing, C.R. Research on Angular Resistance and Plant Structure of Rapeseed Carob and Plucking Mechanism for Low-Loss Mechanised Harvesting; Jiangsu University: Zhenjiang, China, 2021. [Google Scholar]
- The Poisson’s Ratio. Available online: https://baike.baidu.com/item/%E6%B3%8A%E6%9D%BE%E6%AF%94/5920115?fr=aladdin (accessed on 25 May 2021).
- Wang, Y. Abaqus Analysis User’s Guide: Materials Volume, 1st ed.; China Machine Press: Beijing, China, 2018; pp. 27–28. [Google Scholar]
- Bu, L.; Hu, G.; Chen, C.; Sugirbay, A.; Chen, J. Experimental and simulation analysis of optimum picking patterns for robotic apple harvesting. Sci. Hortic. 2019, 261, 108937. [Google Scholar] [CrossRef]

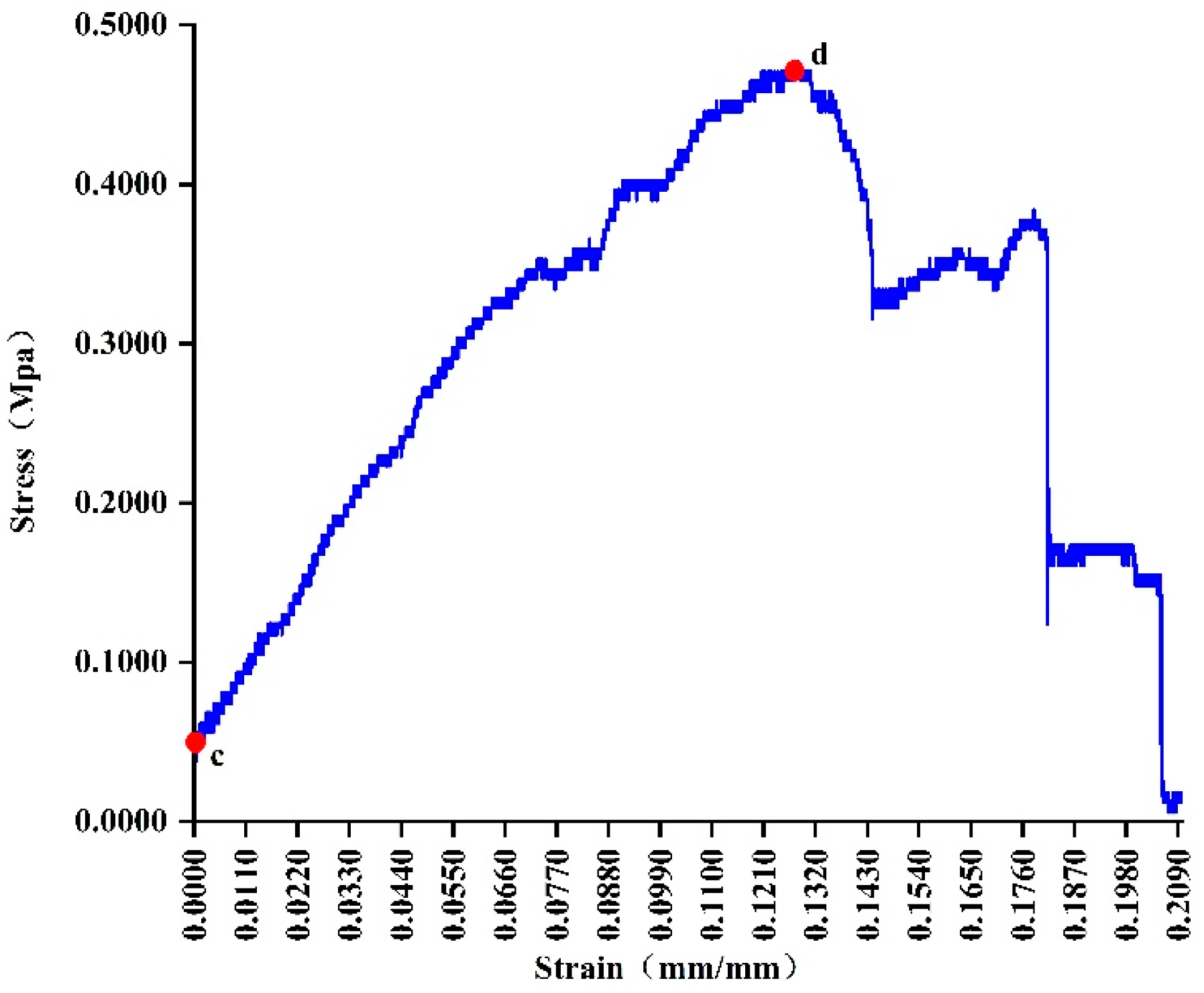
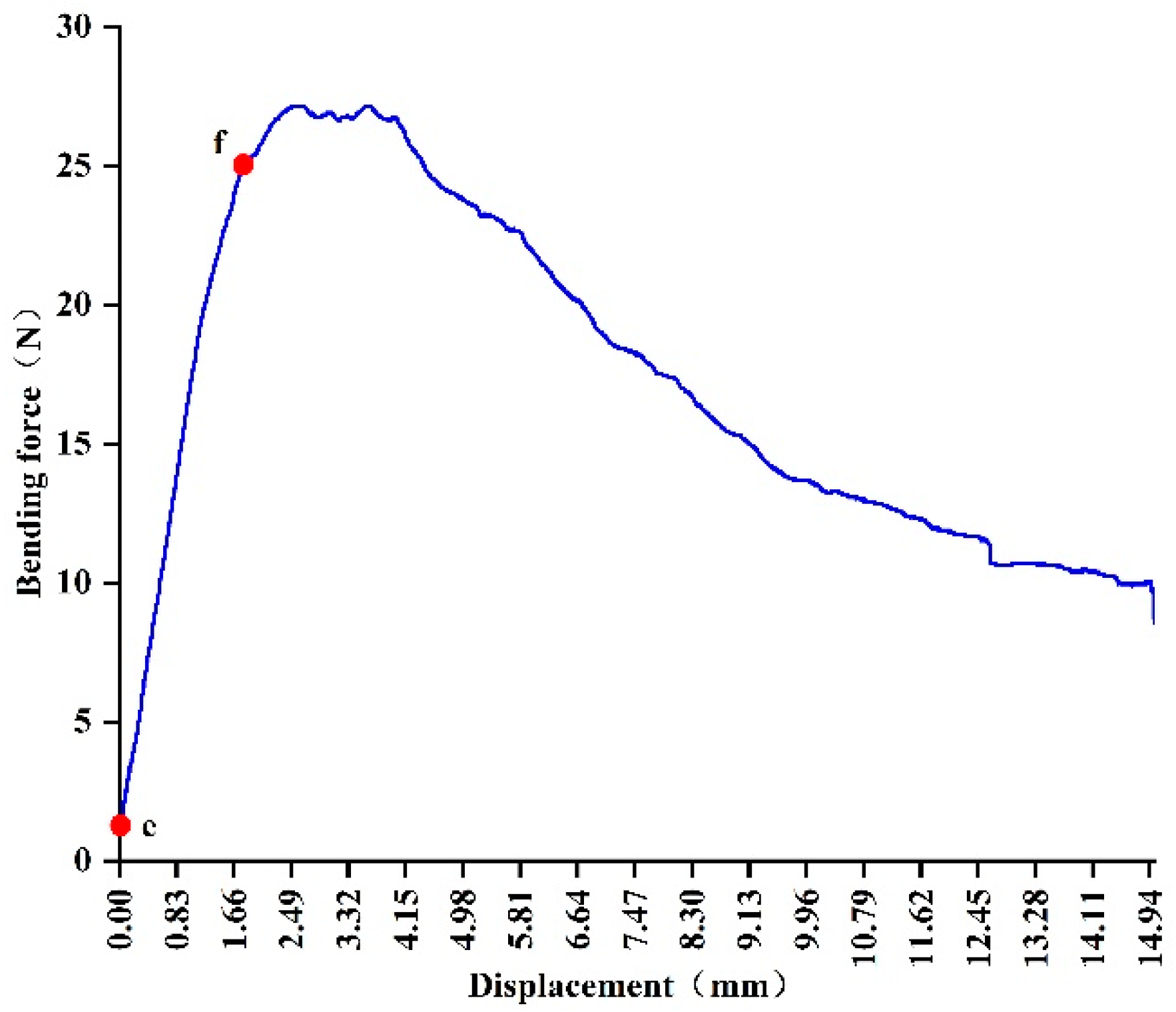
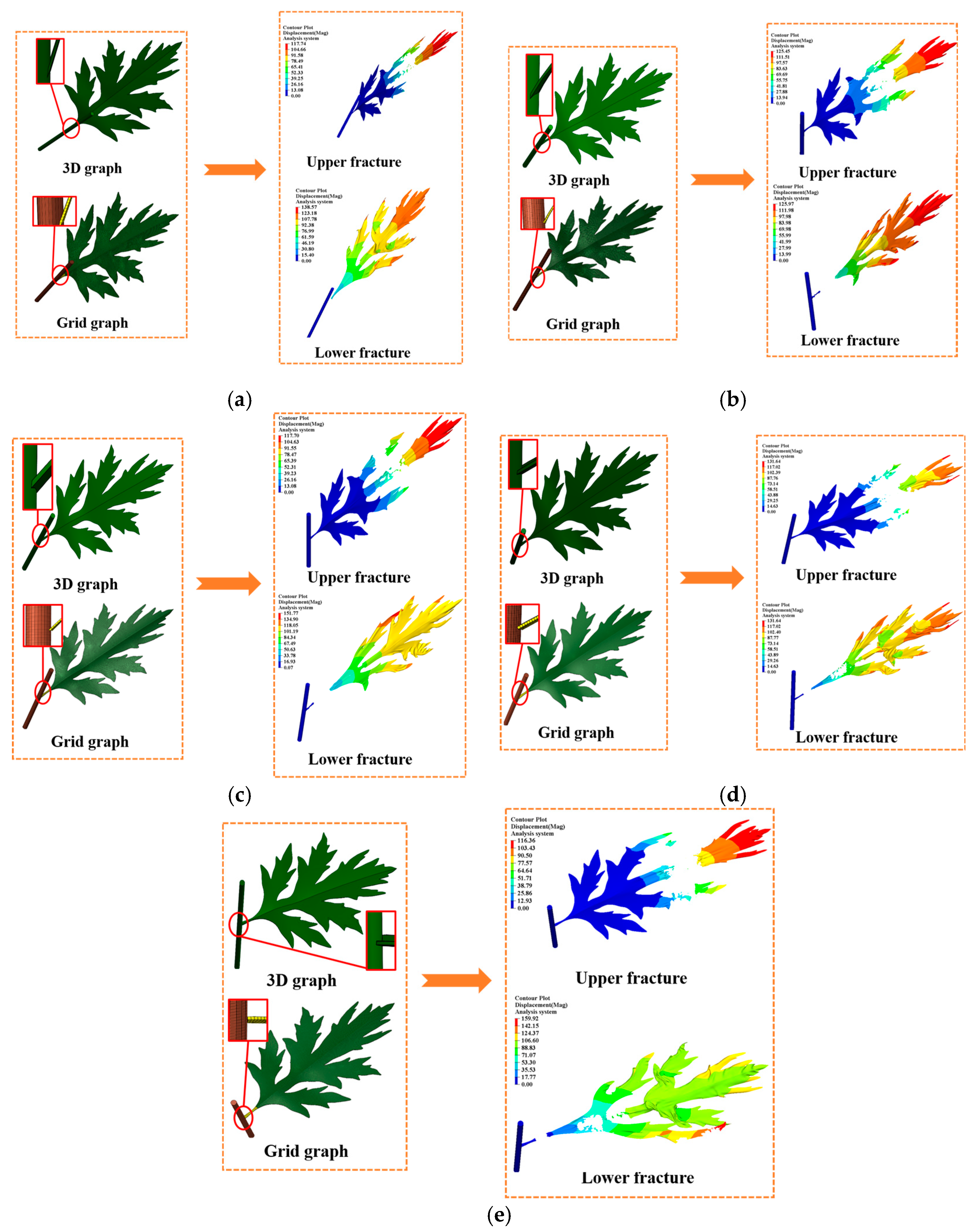

| Item | Elastic Modulus (MPa) | Density (kg/m3) | Poisson Ratio |
|---|---|---|---|
| The mugwort branch | 1.6766 × 103 | 926.25 | 0.3 |
| The mugwort stem | 5.7787 × 101 | 722.16 | 0.38 |
| The mugwort leaf | 3.3486 | 647.50 | 0.4 |
| Item | Maximum Value (N) | Minimum Value (N) | Mean Value (N) | Standard Deviation (N) |
|---|---|---|---|---|
| 0° upper | 8.77 | 4.46 | 6.94 | 1.27 |
| 0° lower | 11.75 | 8.47 | 10.25 | 1.10 |
| 30° upper | 7.81 | 5.06 | 6.04 | 0.93 |
| 30° lower | 15.69 | 12.12 | 13.37 | 1.15 |
| 45° upper | 8.4 | 3.42 | 5.23 | 1.48 |
| 45° lower | 20.89 | 14.12 | 16.73 | 2.03 |
| 60° upper | 8.99 | 4.53 | 6.14 | 1.26 |
| 60° lower | 17.99 | 12.49 | 14.96 | 1.74 |
| 90° upper | 6.39 | 2.68 | 5.10 | 1.09 |
| 90° lower | 11.08 | 5.95 | 8.19 | 1.69 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Zhang, D.; Hu, X.; Lu, R. Finite Element Simulations and Experimental Analysis for Efficient Mugwort Harvesting. Agriculture 2024, 14, 1875. https://doi.org/10.3390/agriculture14111875
Wang S, Zhang D, Hu X, Lu R. Finite Element Simulations and Experimental Analysis for Efficient Mugwort Harvesting. Agriculture. 2024; 14(11):1875. https://doi.org/10.3390/agriculture14111875
Chicago/Turabian StyleWang, Siqi, Daode Zhang, Xinyu Hu, and Rui Lu. 2024. "Finite Element Simulations and Experimental Analysis for Efficient Mugwort Harvesting" Agriculture 14, no. 11: 1875. https://doi.org/10.3390/agriculture14111875
APA StyleWang, S., Zhang, D., Hu, X., & Lu, R. (2024). Finite Element Simulations and Experimental Analysis for Efficient Mugwort Harvesting. Agriculture, 14(11), 1875. https://doi.org/10.3390/agriculture14111875





