The Influence of the Periodontal Breakdown over the Amount of Orthodontic Force Reaching the Dental Pulp and NVB During Orthodontic Movements—A Biomechanical Finite Element Analysis
Abstract
1. Introduction
2. Materials and Methods
3. Results
4. Discussion
5. Conclusions
- 1.
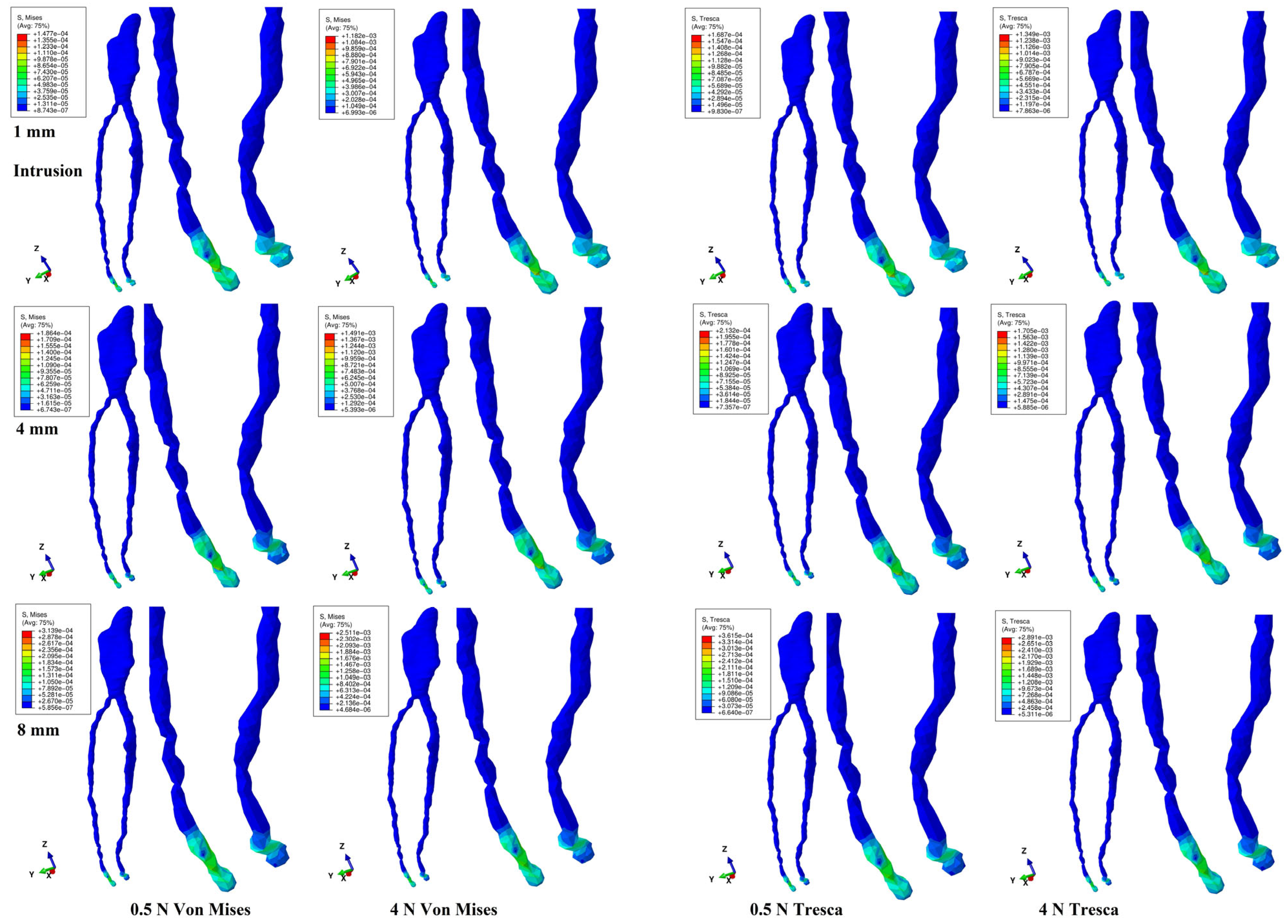
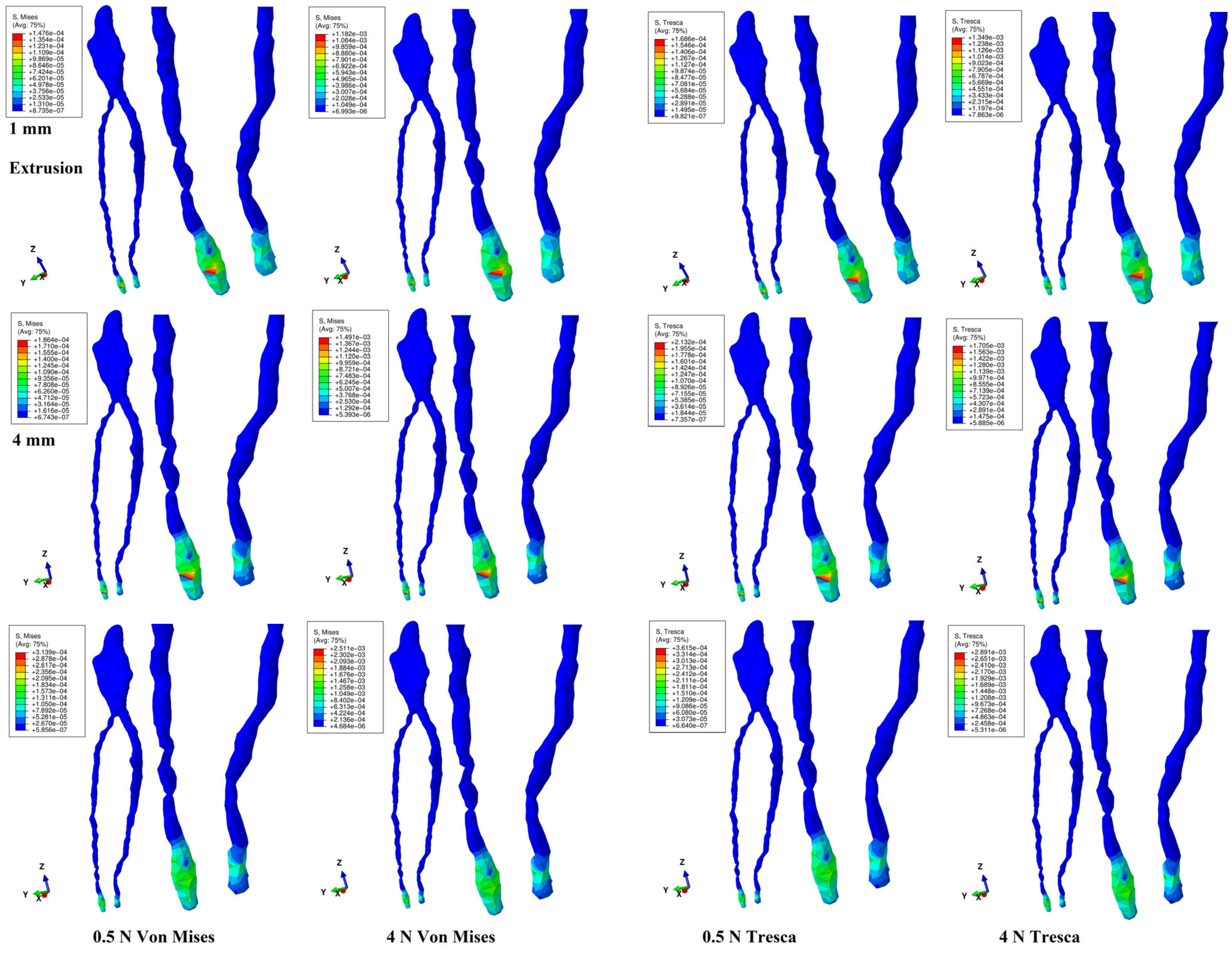
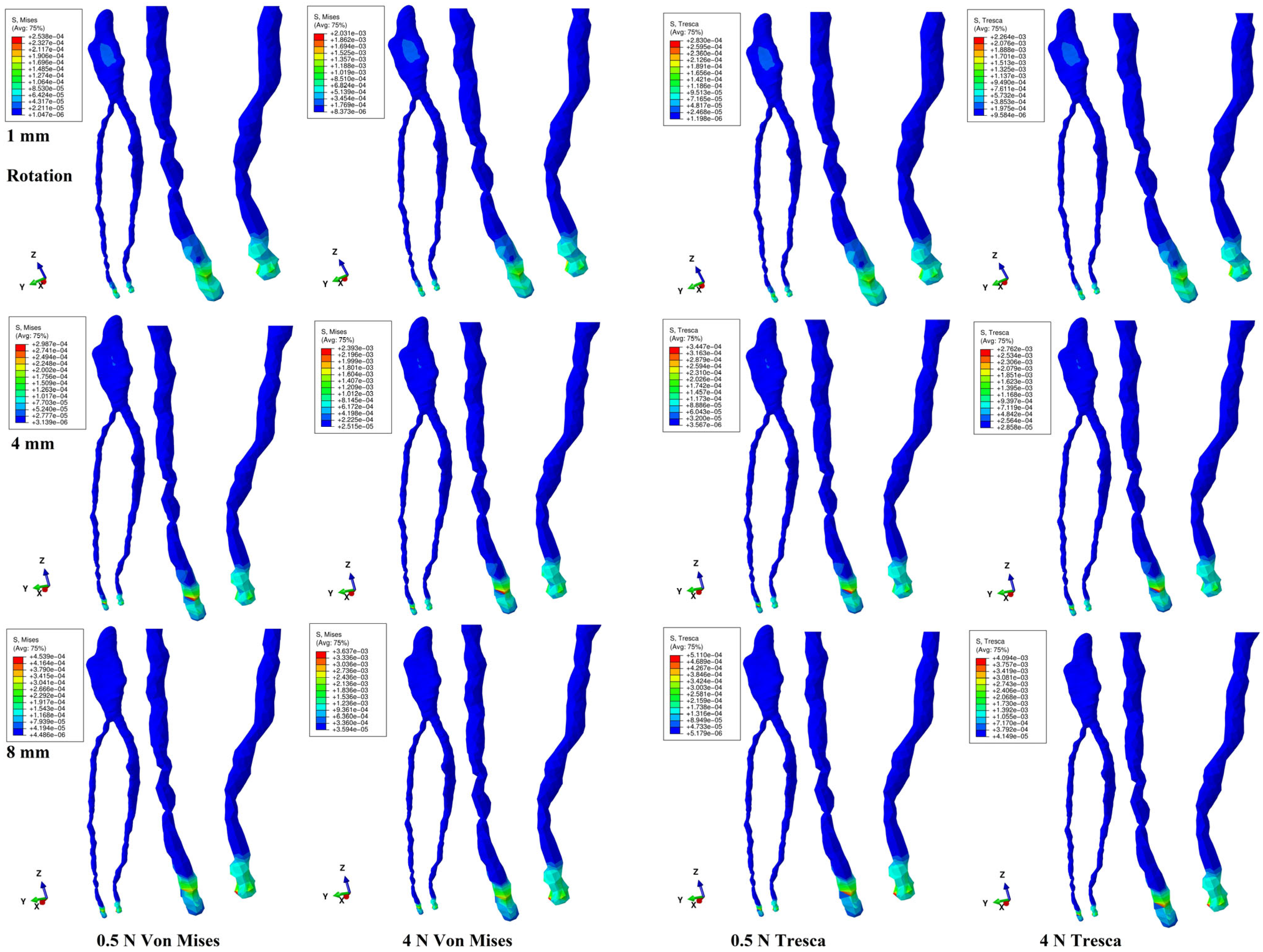
- During the gradual horizontal periodontal breakdown simulation, both methods displayed a similar color-coded stress distribution in the NVB and pulp for the two orthodontic loads and five movements.
- 2.
- Quantitatively, for 0.5 and 4 N force, during the 1–8 mm bone loss simulation, the NVB stress was 5.7–10.7 times higher than the pulpal stress but lower than the local physiological maximum hydrostatic pressure, with no tissular ischemic risks.
- 3.
- A gradual tissular stress increase (doubling and tripling at 8 mm loss when compared with 1 mm) was seen, strictly correlated with the bone loss level for both forces and five movements.
- 4.
- For 1 mm bone loss, only 2–3% of the applied force manifested at the NVB level (0.27–0.5% for pulp), while for 8 mm loss, the received stress was 4–10% for the NVB (0.6–0.9% for pulp) when compared to the applied force.
- 5.
- When the NVB stress was assessed, the tissular absorption–dissipation ability of the tooth (i.e., enamel, dentine, and stainless-steel bracket) was around 97% for 1 mm bone loss and around 90–93% for 8 mm loss, with a similar pattern for both forces.
- 6.
- When the pulpal stress was assessed, an absorption–dissipation rate of around 99% in the tooth (i.e., enamel, dentine, and bracket) was seen for the 1–8 mm periodontal breakdown, similarly for both forces.
- 7.
- Only translation displayed pulpal stress (coronal and radicular) during the periodontal breakdown for both loads and methods, while all other movements displayed only NVB stress (most visible during intrusion and extrusion).
- 8.
- Despite the reduced amount of stress reaching the NVB and dental pulp, the orthodontic treatments for cases of periodontal loss should be considered with care, especially for the teeth displaying various signs of previous dental treatments and occlusal trauma.
6. Practical Implications
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ricucci, D.; Siqueira, J.F., Jr.; Rôças, I.N. Pulp Response to Periodontal Disease: Novel Observations Help Clarify the Processes of Tissue Breakdown and Infection. J. Endod. 2021, 47, 740–754. [Google Scholar] [CrossRef] [PubMed]
- Minch, L.E.; Sarul, M.; Nowak, R.; Kawala, B.; Antoszewska-Smith, J. Orthodontic intrusion of periodontally-compromised maxillary incisors: 3-Dimensional finite element method analysis. Adv. Clin. Exp. Med. 2017, 26, 829–833. [Google Scholar] [CrossRef] [PubMed]
- França, C.M.; Riggers, R.; Muschler, J.L.; Widbiller, M.; Lococo, P.M.; Diogenes, A.; Bertassoni, L.E. 3D-Imaging of Whole Neuronal and Vascular Networks of the Human Dental Pulp via CLARITY and Light Sheet Microscopy. Sci. Rep. 2019, 9, 10860. [Google Scholar] [CrossRef]
- Wu, J.; Liu, Y.; Wang, D.; Zhang, J.; Dong, X.; Jiang, X.; Xu, X. Investigation of effective intrusion and extrusion force for maxillary canine using finite element analysis. Comput. Methods Biomech. Biomed. Eng. 2019, 22, 1294–1302. [Google Scholar] [CrossRef]
- Wu, J.; Liu, Y.; Li, B.; Wang, D.; Dong, X.; Sun, Q.; Chen, G. Numerical simulation of optimal range of rotational moment for the mandibular lateral incisor, canine and first premolar based on biomechanical responses of periodontal ligaments: A case study. Clin. Oral Investig. 2021, 25, 1569–1577. [Google Scholar] [CrossRef]
- Hohmann, A.; Wolfram, U.; Geiger, M.; Boryor, A.; Kober, C.; Sander, C.; Sander, F.G. Correspondences of hydrostatic pressure in periodontal ligament with regions of root resorption: A clinical and a finite element study of the same human teeth. Comput. Methods Programs Biomed. 2009, 93, 155–161. [Google Scholar] [CrossRef]
- Hohmann, A.; Wolfram, U.; Geiger, M.; Boryor, A.; Sander, C.; Faltin, R.; Faltin, K.; Sander, F.G. Periodontal ligament hydrostatic pressure with areas of root resorption after application of a continuous torque moment. Angle Orthod. 2007, 77, 653–659. [Google Scholar] [CrossRef]
- Moga, R.-A.; Olteanu, C.D.; Delean, A.G. Investigating the Ability of the Tooth and Surrounding Support Tissues to Absorb and Dissipate Orthodontic Loads during Periodontal Breakdown—Finite Elements Analysis. Appl. Sci. 2024, 14, 1041. [Google Scholar] [CrossRef]
- Moga, R.A.; Olteanu, C.D.; Daniel, B.M.; Buru, S.M. Finite Elements Analysis of Tooth-A Comparative Analysis of Multiple Failure Criteria. Int. J. Environ. Res. Public Health 2023, 20, 4133. [Google Scholar] [CrossRef]
- Proffit, W.R.; Fields, H.W., Jr.; Sarver, D.M.; Ackerman, J.L. Contemporary Orthodontics, 5th ed.; Elsevier: St. Louis, MO, USA, 2012. [Google Scholar]
- Moga, R.-A.; Olteanu, C.D.; Delean, A.G. The Amount of Orthodontic Force Reaching the Dental Pulp and Neuro-Vascular Bundle During Orthodontic Movements in the Intact Periodontium. Medicina 2024, 60, 2045. [Google Scholar] [CrossRef]
- Weissheimer, T.; Silva, E.; Pinto, K.P.; Só, G.B.; Rosa, R.A.; Só, M.V.R. Do orthodontic tooth movements induce pulp necrosis? A systematic review. Int. Endod. J. 2021, 54, 1246–1262. [Google Scholar] [CrossRef] [PubMed]
- Yamaguchi, M.; Fukasawa, S. Is Inflammation a Friend or Foe for Orthodontic Treatment? Inflammation in Orthodontically Induced Inflammatory Root Resorption and Accelerating Tooth Movement. Int. J. Mol. Sci. 2021, 22, 2388. [Google Scholar] [CrossRef] [PubMed]
- Vitali, F.C.; Cardoso, I.V.; Mello, F.W.; Flores-Mir, C.; Andrada, A.C.; Dutra-Horstmann, K.L.; Duque, T.M. Effect of orthodontic force on dental pulp histomorphology and tissue factor expression. Angle Orthod. 2021, 91, 830–842. [Google Scholar] [CrossRef]
- Bauss, O.; Rohling, J.; Meyer, K.; Kiliaridis, S. Pulp vitality in teeth suffering trauma during orthodontic therapy. Angle Orthod. 2009, 79, 166–171. [Google Scholar] [CrossRef]
- Bauss, O.; Rohling, J.; Rahman, A.; Kiliaridis, S. The effect of pulp obliteration on pulpal vitality of orthodontically intruded traumatized teeth. J. Endod. 2008, 34, 417–420. [Google Scholar] [CrossRef]
- Bauss, O.; Röhling, J.; Sadat-Khonsari, R.; Kiliaridis, S. Influence of orthodontic intrusion on pulpal vitality of previously traumatized maxillary permanent incisors. Am. J. Orthod. Dentofac. Orthop. 2008, 134, 12–17. [Google Scholar] [CrossRef]
- Bauss, O.; Schäfer, W.; Sadat-Khonsari, R.; Knösel, M. Influence of orthodontic extrusion on pulpal vitality of traumatized maxillary incisors. J. Endod. 2010, 36, 203–207. [Google Scholar] [CrossRef]
- Cărămizaru, M.; Pleşea, I.E.; Dragomir, L.P.; Popescu, M.R.; Uscatu, C.D.; Şerbănescu, M.S.; Alexandru, D.O.; Comănescu, T.M. Quantitative assessment of morphological changes of dental pulp components of teeth affected by occlusal trauma. Rom. J. Morphol. Embryol. Rev. Roum. Morphol. Embryol. 2018, 59, 729–740. [Google Scholar]
- Rusu Olaru, A.; Popescu, M.R.; Pleşea, I.E.; Şerbănescu, M.S.; Pleşea, R.M.; Cojocaru, M.O.; Coculescu, E.C. Abrasion and dental pulp morphological changes in occlusal dysfunction. Rom. J. Morphol. Embryol. Rev. Roum. Morphol. Embryol. 2024, 65, 279–290. [Google Scholar] [CrossRef]
- Strobl, H.; Haas, M.; Norer, B.; Gerhard, S.; Emshoff, R. Evaluation of pulpal blood flow after tooth splinting of luxated permanent maxillary incisors. Dent. Traumatol. 2004, 20, 36–41. [Google Scholar] [CrossRef]
- Cox, C.F.; Hafez, A.A. Biocomposition and reaction of pulp tissues to restorative treatments. Dent. Clin. N. Am. 2001, 45, 31–48. [Google Scholar] [CrossRef] [PubMed]
- Murray, P.E.; Hafez, A.A.; Windsor, L.J.; Smith, A.J.; Cox, C.F. Comparison of pulp responses following restoration of exposed and non-exposed cavities. J. Dent. 2002, 30, 213–222. [Google Scholar] [CrossRef] [PubMed]
- Kitasako, Y.; Ikeda, M.; Tagami, J. Pulpal responses to bacterial contamination following dentin bridging beneath hard-setting calcium hydroxide and self-etching adhesive resin system. Dent. Traumatol. 2008, 24, 201–206. [Google Scholar] [CrossRef]
- Kitasako, Y.; Murray, P.E.; Tagami, J.; Smith, A.J. Histomorphometric analysis of dentinal bridge formation and pulpal inflammation. Quintessence Int. 2002, 33, 600–608. [Google Scholar]
- Cardenas-Duque, L.M.; Yoshida, M.; Goto, G. Pulpal response to different pulp capping methods after pulp exposure by air abrasion. J. Clin. Pediatr. Dent. 2002, 26, 269–273. [Google Scholar] [CrossRef]
- Farughi, A.; Rouhani, A.; Shahmohammadi, R.; Jafarzadeh, H. Clinical comparison of sensitivity and specificity between sensibility and vitality tests in determining the pulp vitality of mandibular premolars. Aust. Endod. J. 2021, 47, 474–479. [Google Scholar] [CrossRef]
- Balevi, B. Cold pulp testing is the simplest and most accurate of all dental pulp sensibility tests. Evid.-Based Dent. 2019, 20, 22–23. [Google Scholar] [CrossRef]
- Emshoff, R.; Emshoff, I.; Moschen, I.; Strobl, H. Diagnostic characteristics of pulpal blood flow levels associated with adverse outcomes of luxated permanent maxillary incisors. Dent. Traumatol. 2004, 20, 270–275. [Google Scholar] [CrossRef]
- Patro, S.; Meto, A.; Mohanty, A.; Chopra, V.; Miglani, S.; Das, A.; Luke, A.M.; Hadi, D.A.; Meto, A.; Fiorillo, L.; et al. Diagnostic Accuracy of Pulp Vitality Tests and Pulp Sensibility Tests for Assessing Pulpal Health in Permanent Teeth: A Systematic Review and Meta-Analysis. Int. J. Environ. Res. Public Health 2022, 19, 9599. [Google Scholar] [CrossRef]
- Murray, P.E.; Hafez, A.A.; Smith, A.J.; Cox, C.F. Identification of hierarchical factors to guide clinical decision making for successful long-term pulp capping. Quintessence Int. 2003, 34, 61–70. [Google Scholar]
- Moga, R.A.; Olteanu, C.D.; Buru, S.M.; Botez, M.D.; Delean, A.G. Finite Elements Analysis of Biomechanical Behavior of the Bracket in a Gradual Horizontal Periodontal Breakdown—A Comparative Analysis of Multiple Failure Criteria. Appl. Sci. 2023, 13, 9480. [Google Scholar] [CrossRef]
- Moga, R.A.; Delean, A.G.; Buru, S.M.; Botez, M.D.; Olteanu, C.D. Orthodontic Internal Resorption Assessment in Periodontal Breakdown-A Finite Elements Analysis (Part II). Healthcare 2023, 11, 2622. [Google Scholar] [CrossRef] [PubMed]
- Moga, R.-A.; Olteanu, C.D.; Delean, A.G. The Importance of Boundary Conditions and Failure Criterion in Finite Element Analysis Accuracy—A Comparative Assessment of Periodontal Ligament Biomechanical Behavior. Appl. Sci. 2024, 14, 3370. [Google Scholar] [CrossRef]
- Javed, F.; Al-Kheraif, A.A.; Romanos, E.B.; Romanos, G.E. Influence of orthodontic forces on human dental pulp: A systematic review. Arch. Oral Biol. 2015, 60, 347–356. [Google Scholar] [CrossRef]
- Mainkar, A.; Kim, S.G. Diagnostic Accuracy of 5 Dental Pulp Tests: A Systematic Review and Meta-analysis. J. Endod. 2018, 44, 694–702. [Google Scholar] [CrossRef]
- Perez-Gonzalez, A.; Iserte-Vilar, J.L.; Gonzalez-Lluch, C. Interpreting finite element results for brittle materials in endodontic restorations. Biomed. Eng. Online 2011, 10, 44. [Google Scholar] [CrossRef]
- Toms, S.R.; Eberhardt, A.W. A nonlinear finite element analysis of the periodontal ligament under orthodontic tooth loading. Am. J. Orthod. Dentofac. Orthop. 2003, 123, 657–665. [Google Scholar] [CrossRef]
- Hemanth, M.; Deoli, S.; Raghuveer, H.P.; Rani, M.S.; Hegde, C.; Vedavathi, B. Stress Induced in the Periodontal Ligament under Orthodontic Loading (Part I): A Finite Element Method Study Using Linear Analysis. J. Int. Oral Health JIOH 2015, 7, 129–133. [Google Scholar]
- Hemanth, M.; Deoli, S.; Raghuveer, H.P.; Rani, M.S.; Hegde, C.; Vedavathi, B. Stress Induced in Periodontal Ligament under Orthodontic Loading (Part II): A Comparison of Linear Versus Non-Linear Fem Study. J. Int. Oral Health JIOH 2015, 7, 114–118. [Google Scholar]
- Geramy, A. Initial stress produced in the periodontal membrane by orthodontic loads in the presence of varying loss of alveolar bone: A three-dimensional finite element analysis. Eur. J. Orthod. 2002, 24, 21–33. [Google Scholar] [CrossRef]
- Geramy, A.; Faghihi, S. Secondary trauma from occlusion: Three-dimensional analysis using the finite element method. Quintessence Int. 2004, 35, 835–843. [Google Scholar] [PubMed]
- Shaw, A.M.; Sameshima, G.T.; Vu, H.V. Mechanical stress generated by orthodontic forces on apical root cementum: A finite element model. Orthod. Craniofacial Res. 2004, 7, 98–107. [Google Scholar] [CrossRef] [PubMed]
- Gupta, M.; Madhok, K.; Kulshrestha, R.; Chain, S.; Kaur, H.; Yadav, A. Determination of stress distribution on periodontal ligament and alveolar bone by various tooth movements—A 3D FEM study. J. Oral Biol. Craniofacial Res. 2020, 10, 758–763. [Google Scholar] [CrossRef] [PubMed]
- Merdji, A.; Mootanah, R.; Bachir Bouiadjra, B.A.; Benaissa, A.; Aminallah, L.; Chikh, E.B.O.; Mukdadi, S. Stress analysis in single molar tooth. Mater. Sci. Eng. C Mater. Biol. Appl. 2013, 33, 691–698. [Google Scholar] [CrossRef]
- Roscoe, M.G.; Cattaneo, P.M.; Dalstra, M.; Ugarte, O.M.; Meira, J.B.C. Orthodontically induced root resorption: A critical analysis of finite element studies’ input and output. Am. J. Orthod. Dentofac. Orthop. 2021, 159, 779–789. [Google Scholar] [CrossRef]
- Wu, J.L.; Liu, Y.F.; Peng, W.; Dong, H.Y.; Zhang, J.X. A biomechanical case study on the optimal orthodontic force on the maxillary canine tooth based on finite element analysis. J. Zhejiang Univ. Sci. B 2018, 7, 535–546. [Google Scholar] [CrossRef]
- Xing, J.; Zhang, G.; Sun, M.; Pan, H.; Zhang, C.; Liu, Y.; Li, K.; He, Z.; Zhang, K.; Wang, J.; et al. Clinical insights into tooth extraction via torsion method: A biomechanical analysis of the tooth-periodontal ligament complex. Front. Bioeng. Biotechnol. 2024, 12, 1479751. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhu, J.; Yin, D.; Liu, Y. Improved stomatognathic model for highly realistic finite element analysis of temporomandibular joint biomechanics. J. Mech. Behav. Biomed. Mater. 2024, 160, 106780. [Google Scholar] [CrossRef]
- Zhu, Y.; Zheng, F.; Gong, Y.; Zhu, J.; Yin, D.; Liu, Y. Effect of occlusal contact on TMJ loading during occlusion: An in silico study. Comput. Biol. Med. 2024, 178, 108725. [Google Scholar] [CrossRef]
- Zheng, F.; Gong, Y.; Zhu, Y.; Yin, D.; Liu, Y. Three-dimensional theoretical model for effectively describing the effect of craniomaxillofacial structural factors on loading situation in the temporomandibular joint. J. Mech. Behav. Biomed. Mater. 2024, 151, 106371. [Google Scholar] [CrossRef]
- Zhang, X.; Li, M.Q.; Guo, J.; Yang, H.W.; Yu, J.; Li, G.J. An analysis of the optimal intrusion force of the maxillary central incisor with root horizontal resorption using the finite element method and curve fitting. Comput. Methods Biomech. Biomed. Eng. 2022, 25, 1471–1486. [Google Scholar] [CrossRef] [PubMed]
- Hussein Mahmood Ghuloom, K.; Mascarenhas, R.; Parveen, S.; Husain, A. Finite element analysis of orthodontically induced stress in the periodontal ligament of the maxillary first molar with simulated bone loss. J. Comput. Methods Sci. Eng. 2017, 17, 243–252. [Google Scholar] [CrossRef]
- Shetty, B.; Fazal, I.; Khan, S.F. FEA analysis of Normofunctional forces on periodontal elements in different angulations. Bioinformation 2022, 18, 245–250. [Google Scholar] [CrossRef] [PubMed]
- Dederichs, M.; Joedecke, P.; Weber, C.T.; Guentsch, A. Functional Load Capacity of Teeth with Reduced Periodontal Support: A Finite Element Analysis. Bioengineering 2023, 10, 1330. [Google Scholar] [CrossRef]
- Agrawal, R.; Narang, S.; Ahmed, H.; Prasad, S.; Reddy, S.; Aila, S. Influence of Occlusal Bite Forces on Teeth with Altered Periodontal Support: A Three-Dimensional Finite Element Stress Analysis. J. Pharm. Bioallied Sci. 2021, 13 (Suppl. S1), S688–S691. [Google Scholar] [CrossRef]
- Shi, H.; Kang, F.F.; Liu, Q. Stress induced on permanent mandible first molar and space maintainer under normal masticatory forces: A finite element study. PeerJ 2024, 12, e17456. [Google Scholar] [CrossRef]
- Lyu, X.; Cao, X.; Chen, L.; Liu, Y.; Li, H.; Hu, C.; Tan, J. Accumulated biomechanical effects of mandibular molar mesialization using clear aligners with auxiliary devices: An iterative finite element analysis. Prog. Orthod. 2023, 24, 13. [Google Scholar] [CrossRef]
- Ubuzima, P.; Nshimiyimana, E.; Mukeshimana, C.; Mazimpaka, P.; Mugabo, E.; Mbyayingabo, D.; Mohamed, A.S.; Habumugisha, J. Exploring biological mechanisms in orthodontic tooth movement: Bridging the gap between basic research experiments and clinical applications—A comprehensive review. Ann. Anat. Anat. Anz. 2024, 255, 152286. [Google Scholar] [CrossRef]
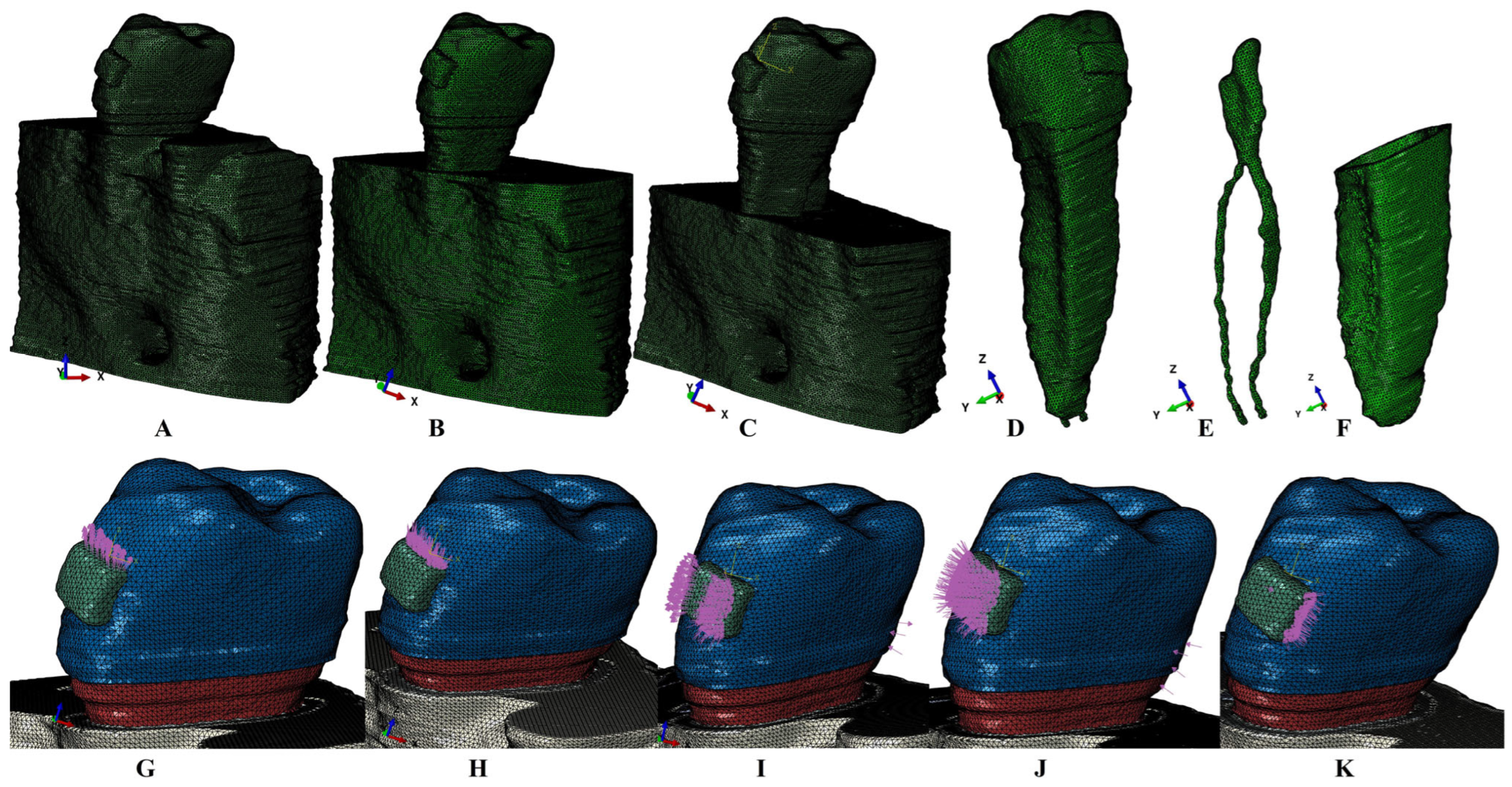


| Materials | Young’s Modulus, E (GPa) | Poisson Ratio, ʋ | Refs. |
|---|---|---|---|
| Enamel | 80 | 0.33 | [8,9,11,32,33,34] |
| Dentin/cementum | 18.6 | 0.31 | [8,9,11,32,33,34] |
| Pulp and NVB | 0.0021 | 0.45 | [8,9,11,32,33,34] |
| PDL | 0.0667 | 0.49 | [8,9,11,32,33,34] |
| Cortical bone | 14.5 | 0.323 | [8,9,11,32,33,34] |
| Trabecular bone | 1.37 | 0.3 | [8,9,11,32,33,34] |
| Stainless steel bracket (Cr-Co) | 218 | 0.33 | [8,9,11,32,33,34] |
| Resorption (mm) | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | ||
|---|---|---|---|---|---|---|---|---|---|---|
| Intrusion | Tresca | NVB | 1.12 | 1.31 | 1.51 | 1.70 | 2.00 | 2.29 | 2.59 | 2.88 |
| 4 N/40 KPa | c | 0.11 | 0.12 | 0.13 | 0.15 | 0.17 | 0.20 | 0.22 | 0.25 | |
| VM | NVB | 0.94 | 1.12 | 1.30 | 1.49 | 1.88 | 2.06 | 2.18 | 2.50 | |
| c | 0.10 | 0.11 | 0.12 | 0.13 | 0.15 | 0.17 | 0.19 | 0.21 | ||
| Tresca | NVB | 0.14 | 0.16 | 0.19 | 0.21 | 0.25 | 0.29 | 0.32 | 0.36 | |
| 0.5 N/5 KPa | c | 0.01 | 0.02 | 0.02 | 0.02 | 0.02 | 0.02 | 0.03 | 0.03 | |
| VM | NVB | 0.12 | 0.14 | 0.16 | 0.19 | 0.24 | 0.26 | 0.27 | 0.33 | |
| c | 0.01 | 0.01 | 0.01 | 0.02 | 0.02 | 0.02 | 0.02 | 0.03 | ||
| Extrusion | Tresca | NVB | 1.12 | 1.31 | 1.51 | 1.70 | 2.00 | 2.29 | 2.59 | 2.88 |
| 4 N/40 KPa | c | 0.11 | 0.12 | 0.13 | 0.15 | 0.17 | 0.20 | 0.22 | 0.25 | |
| VM | NVB | 0.94 | 1.12 | 1.30 | 1.49 | 1.74 | 2.06 | 2.18 | 2.50 | |
| c | 0.10 | 0.11 | 0.12 | 0.13 | 0.15 | 0.17 | 0.19 | 0.21 | ||
| Tresca | NVB | 0.14 | 0.16 | 0.19 | 0.21 | 0.25 | 0.29 | 0.32 | 0.36 | |
| 0.5 N/5 KPa | c | 0.01 | 0.02 | 0.02 | 0.02 | 0.02 | 0.02 | 0.03 | 0.03 | |
| VM | NVB | 0.12 | 0.14 | 0.16 | 0.19 | 0.22 | 0.26 | 0.27 | 0.31 | |
| c | 0.01 | 0.01 | 0.01 | 0.02 | 0.02 | 0.02 | 0.02 | 0.03 | ||
| Translation | Tresca | NVB | 0.85 | 0.95 | 1.06 | 1.17 | 1.30 | 1.44 | 1.58 | 1.72 |
| 4 N/40 KPa | c | 0.15 | 0.16 | 0.18 | 0.20 | 0.21 | 0.22 | 0.23 | 0.24 | |
| VM | NVB | 0.74 | 0.83 | 0.92 | 1.01 | 1.14 | 1.28 | 1.42 | 1.56 | |
| c | 0.18 | 0.18 | 0.19 | 0.20 | 0.20 | 0.20 | 0.21 | 0.21 | ||
| Tresca | NVB | 0.11 | 0.12 | 0.13 | 0.15 | 0.16 | 0.18 | 0.20 | 0.21 | |
| 0.5 N/5 KPa | c | 0.02 | 0.02 | 0.02 | 0.03 | 0.03 | 0.03 | 0.03 | 0.03 | |
| VM | NVB | 0.09 | 0.10 | 0.11 | 0.13 | 0.14 | 0.16 | 0.18 | 0.19 | |
| c | 0.02 | 0.02 | 0.02 | 0.02 | 0.03 | 0.03 | 0.03 | 0.03 | ||
| Rotation | Tresca | NVB | 1.20 | 1.61 | 2.01 | 2.75 | 3.08 | 3.42 | 3.75 | 4.08 |
| 4 N/40 KPa | c | 0.21 | 0.23 | 0.24 | 0.26 | 0.29 | 0.32 | 0.35 | 0.38 | |
| VM | NVB | 1.29 | 1.58 | 1.87 | 2.17 | 2.31 | 2.45 | 2.60 | 2.75 | |
| c | 0.19 | 0.20 | 0.21 | 0.22 | 0.26 | 0.29 | 0.33 | 0.36 | ||
| Tresca | NVB | 0.15 | 0.20 | 0.25 | 0.34 | 0.39 | 0.43 | 0.47 | 0.51 | |
| 0.5 N/5 KPa | c | 0.03 | 0.03 | 0.03 | 0.03 | 0.04 | 0.04 | 0.04 | 0.05 | |
| VM | NVB | 0.16 | 0.20 | 0.23 | 0.27 | 0.29 | 0.31 | 0.32 | 0.34 | |
| c | 0.02 | 0.02 | 0.03 | 0.03 | 0.03 | 0.04 | 0.04 | 0.04 | ||
| Tipping | Tresca | NVB | 1.21 | 1.45 | 1.68 | 1.91 | 2.18 | 2.45 | 2.72 | 2.98 |
| 4 N/40 KPa | c | 0.17 | 0.18 | 0.19 | 0.19 | 0.21 | 0.23 | 0.24 | 0.26 | |
| VM | NVB | 1.05 | 1.25 | 1.46 | 1.66 | 1.90 | 2.15 | 2.39 | 2.63 | |
| c | 0.15 | 0.15 | 0.15 | 0.15 | 0.17 | 0.19 | 0.21 | 0.23 | ||
| Tresca | NVB | 0.15 | 0.18 | 0.21 | 0.24 | 0.27 | 0.31 | 0.34 | 0.37 | |
| 0.5 N/5 KPa | c | 0.02 | 0.02 | 0.02 | 0.02 | 0.03 | 0.03 | 0.03 | 0.03 | |
| VM | NVB | 0.13 | 0.16 | 0.18 | 0.21 | 0.24 | 0.27 | 0.30 | 0.33 | |
| c | 0.02 | 0.02 | 0.02 | 0.02 | 0.02 | 0.02 | 0.03 | 0.03 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moga, R.-A.; Olteanu, C.D.; Delean, A.G. The Influence of the Periodontal Breakdown over the Amount of Orthodontic Force Reaching the Dental Pulp and NVB During Orthodontic Movements—A Biomechanical Finite Element Analysis. J. Clin. Med. 2025, 14, 2094. https://doi.org/10.3390/jcm14062094
Moga R-A, Olteanu CD, Delean AG. The Influence of the Periodontal Breakdown over the Amount of Orthodontic Force Reaching the Dental Pulp and NVB During Orthodontic Movements—A Biomechanical Finite Element Analysis. Journal of Clinical Medicine. 2025; 14(6):2094. https://doi.org/10.3390/jcm14062094
Chicago/Turabian StyleMoga, Radu-Andrei, Cristian Doru Olteanu, and Ada Gabriela Delean. 2025. "The Influence of the Periodontal Breakdown over the Amount of Orthodontic Force Reaching the Dental Pulp and NVB During Orthodontic Movements—A Biomechanical Finite Element Analysis" Journal of Clinical Medicine 14, no. 6: 2094. https://doi.org/10.3390/jcm14062094
APA StyleMoga, R.-A., Olteanu, C. D., & Delean, A. G. (2025). The Influence of the Periodontal Breakdown over the Amount of Orthodontic Force Reaching the Dental Pulp and NVB During Orthodontic Movements—A Biomechanical Finite Element Analysis. Journal of Clinical Medicine, 14(6), 2094. https://doi.org/10.3390/jcm14062094







