From “Black Box” to a Real Description of Overall Mass Transport through Membrane and Boundary Layers
Abstract
:1. Introduction
2. Theory
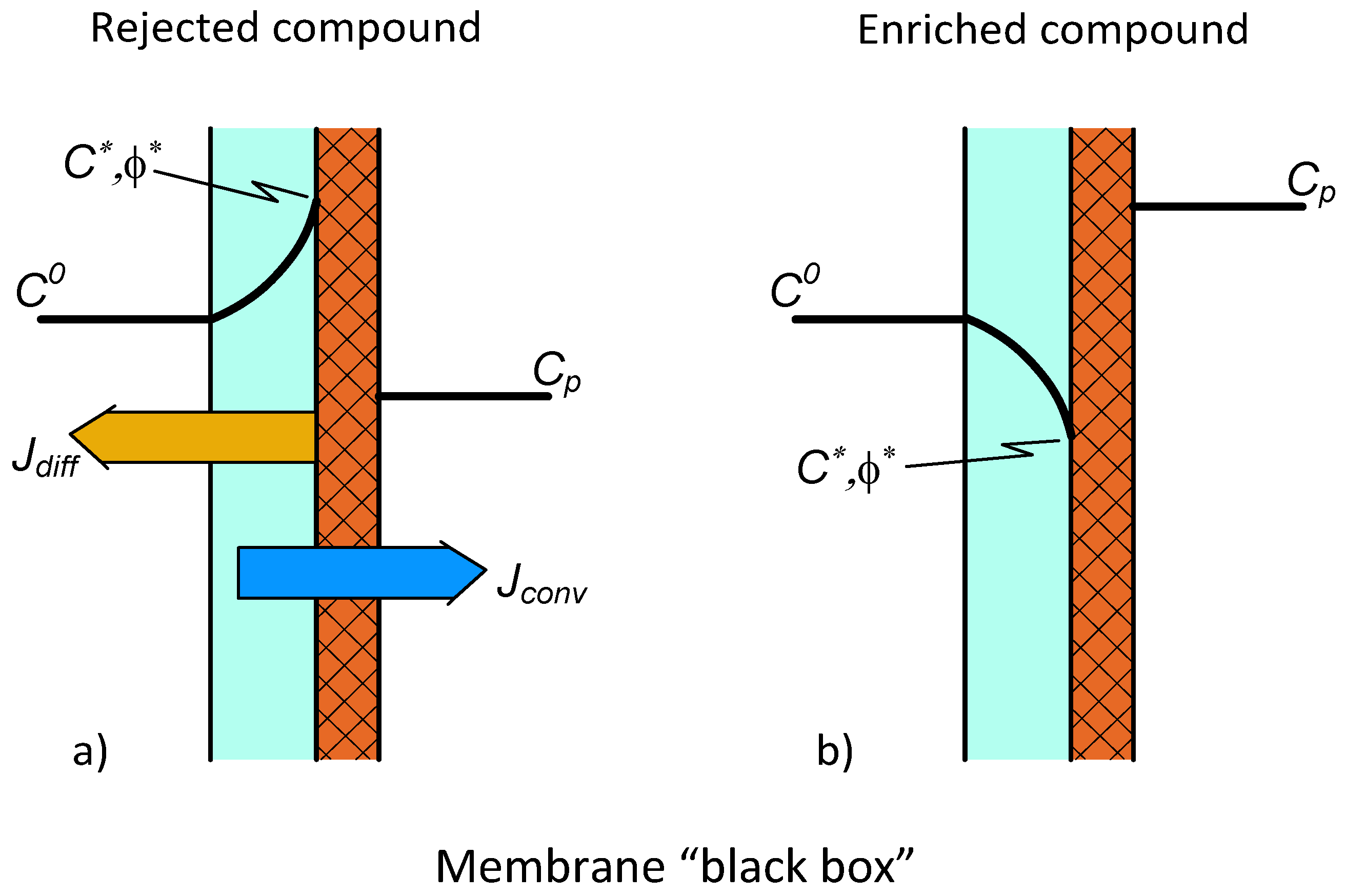
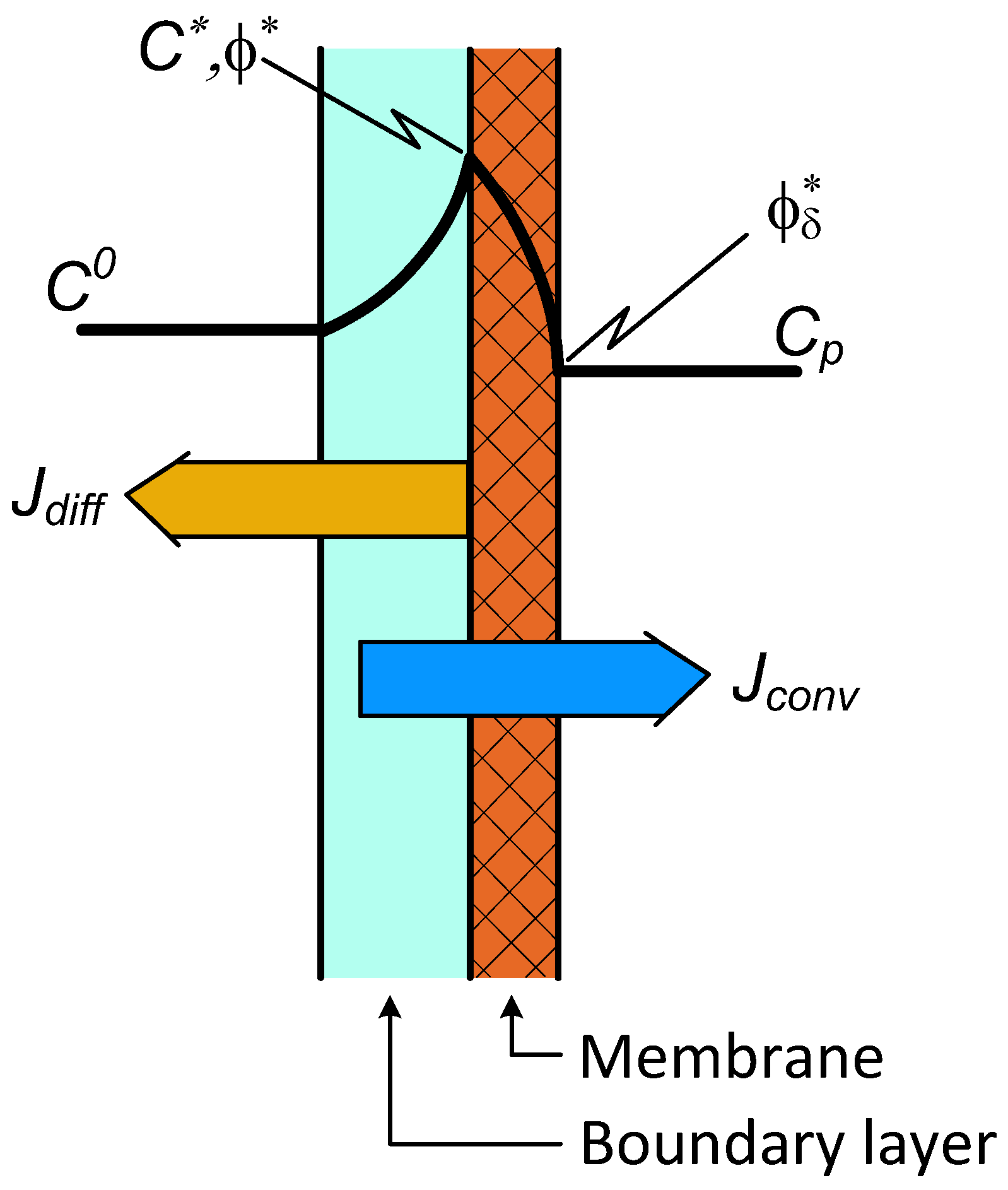
2.1. “Black Box” Model
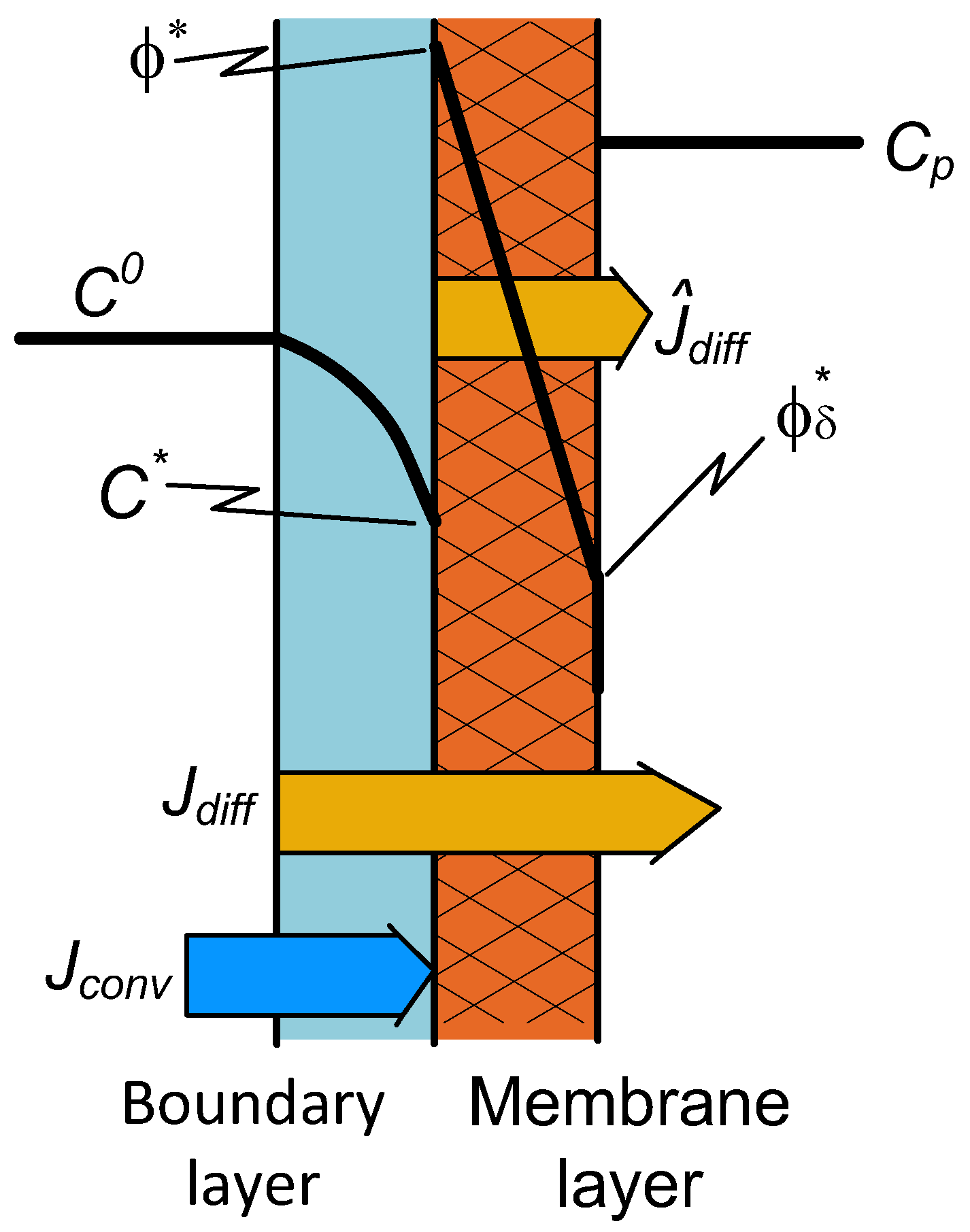
2.2. Solute Transport Using the Solution-Diffusion Model for the Membrane Layer
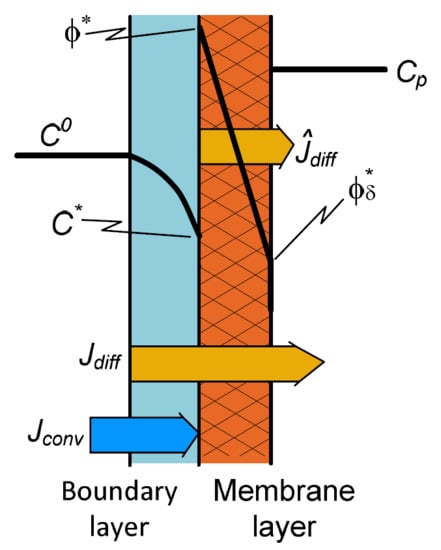
2.3. Two-Layer Transport with Diffusion Plus Convection for Both the Transport Layers
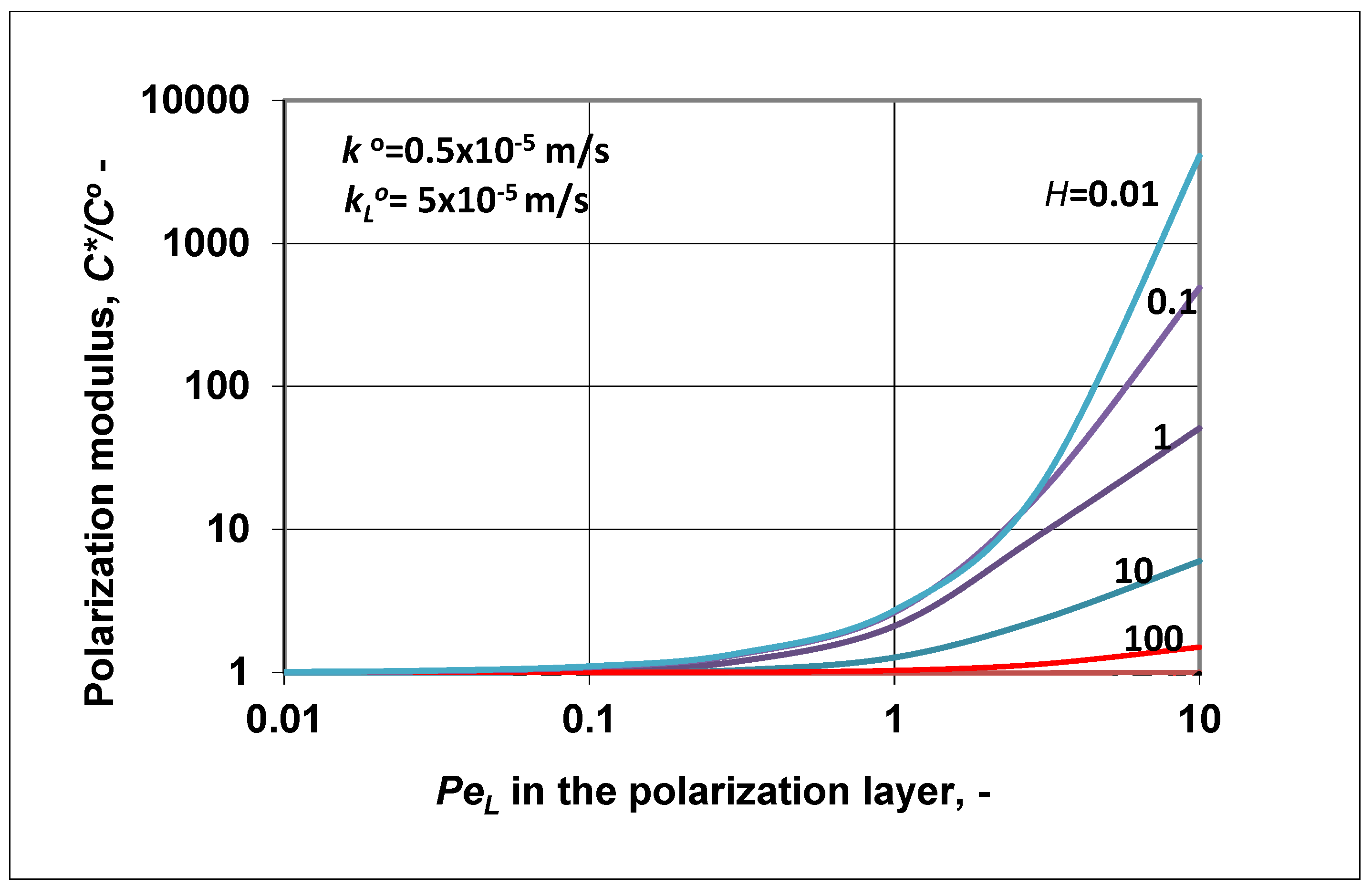
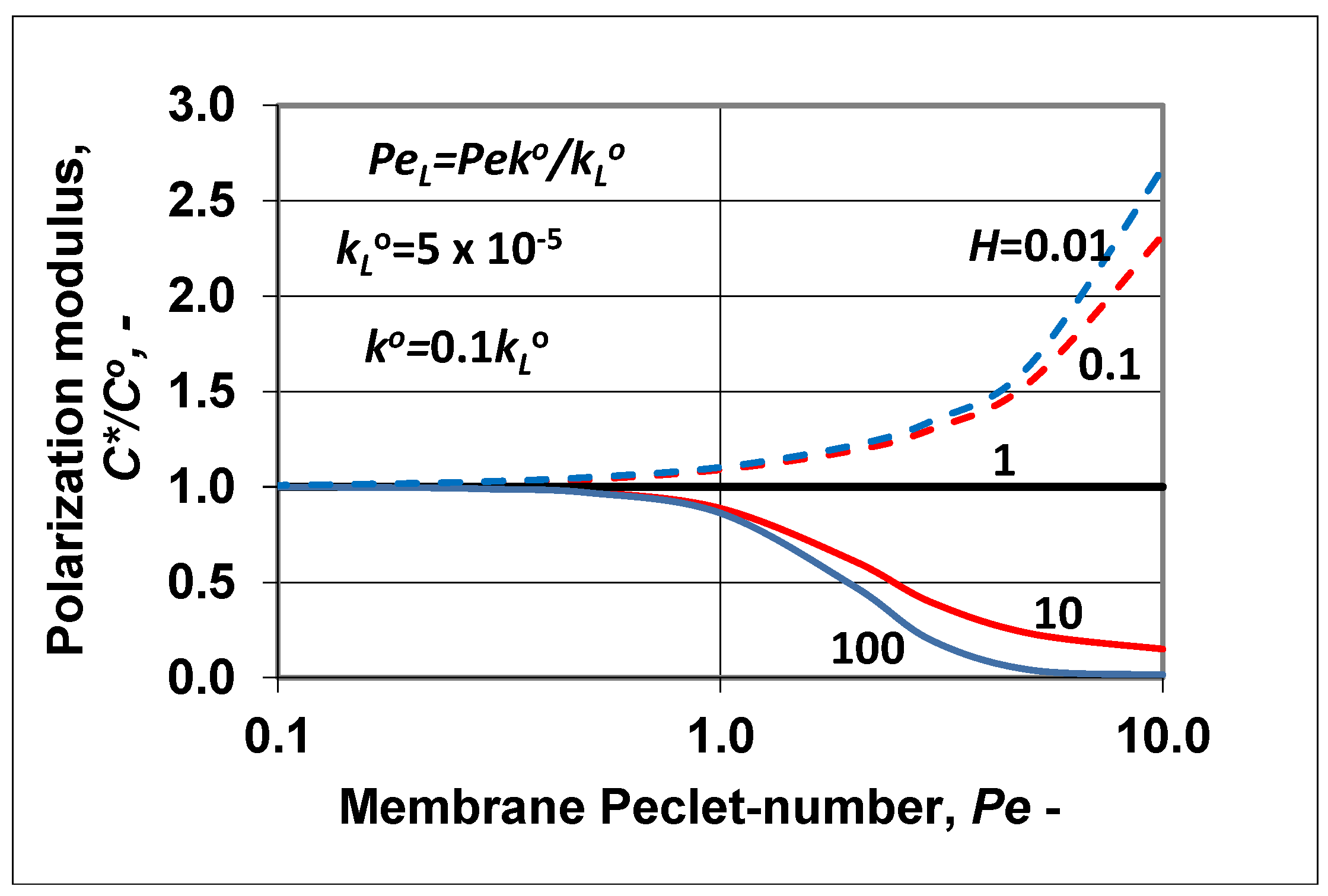
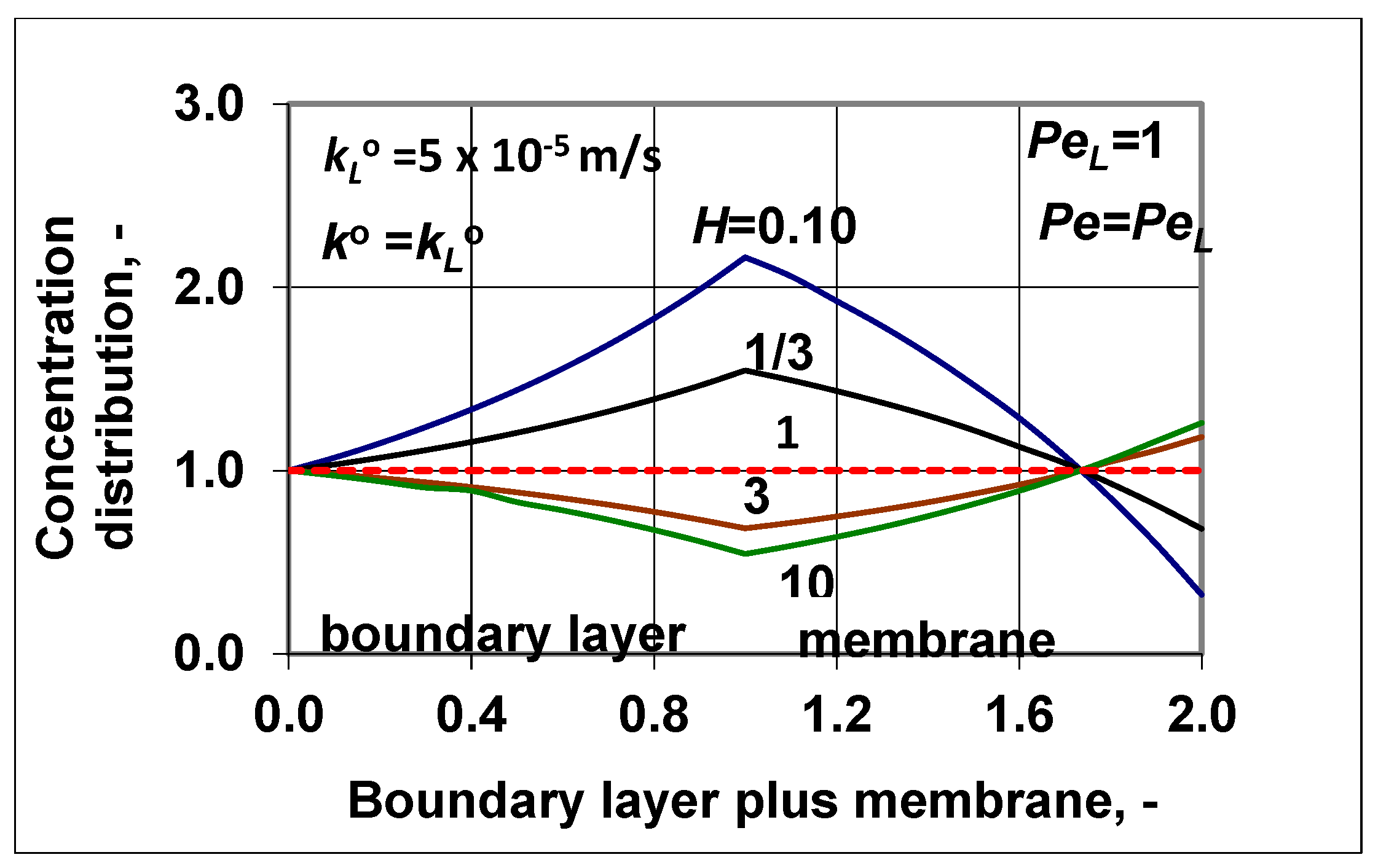
3. Results and Discussion
3.1. Transport with Dense Membranes
3.2. Transport with Convection in a Porous Membrane Layer
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| C | concentration in the fluid phase, kg/m3 |
| D | diffusion coefficient, m2/s |
| E | enhancement, - |
| intrinsic enhancement, - | |
| H | solubility coefficient, kg/kg |
| Jo | mass transfer rate, kg/m2s |
| membrane diffusive mass transfer coefficient, m/s | |
| diffusion coefficient in the feed fluid phase, m/s | |
| Pe | Peclet number (Equation (3)) |
| y | local coordinate, m |
| β | mass transfer coefficient in presence of convective velocity, m/s |
| ϕ | solute concentration in the membrane, kg/kg |
| Superscript: | |
| * | interface |
| inlet | |
| Subscript: | |
| L | fluid phase |
| p | outlet |
References
- Mulder, M. Basic Principle of Membrane Technology, 2nd ed.; Kluwer Academic Publisher: Dordrecht, The Netherlands, 1996; pp. 210–278. ISBN 978-0-7923-4248-9. [Google Scholar]
- Scott, K.; Hughes, H. Industrial Membrane Separation Technology; Springer-Science+Business Media: Dordrecht, The Netherlands, 1996; pp. 67–112. ISBN 978-94-010-4274-1. [Google Scholar]
- Wijmans, J.G.; Baker, R.W. The Solution-Diffusion Model: A Limited Approach in Membrane Permeation. In Material Science of Membranes for Gas and Vapor Separation; Yampolskii, Y., Pinnau, I., Freeman, B.D., Eds.; Wiley: Chichester, UK, 2006; pp. 139–188. ISBN 0-470-85345-X. [Google Scholar]
- Lonsdale, H.K.; Merten, L.; Riley, R.L. Transport properties of cellulose acetate osmotic membranes. Appl. Polym. Sci. 1965, 9, 1341–1362. [Google Scholar] [CrossRef]
- Kimura, S.; Sourirajan, S. Analysis of data in reverse osmosis with porous cellulose acetate membrane used. AIChE J. 1967, 13, 497–503. [Google Scholar] [CrossRef]
- Kedem, O.; Katchalsky, A. Thermodynamic analysis of the permeability of biological membranes to non-electrolytes. Biochim. Biophys. Acta 1958, 27, 229–246. [Google Scholar] [CrossRef]
- Kedem, O.; Spiegler, K. Thermodynamics of hyperfiltration (reverse osmosis): Criteria for efficient membranes. Desalination 1966, 1, 311–326. [Google Scholar] [CrossRef]
- Soltanieh, M.; Gill, W.N. Review of reverse osmosis membranes and transport models. Chem. Eng. Commun. 1981, 12, 279–363. [Google Scholar] [CrossRef]
- Bitter, J.G. Transport Mechanism in Membrane Separation Processes; Plenum Press: New York, NY, USA, 1991; ISBN-13: 978-0306438493; ISBN-10: 0306438496. [Google Scholar]
- Baker, R.W. Membrane Technology and Application, 2nd ed.; Wiley: Chichester, UK, 2004; pp. 1–558. ISBN 978-0-47-085445-7. [Google Scholar]
- Nagy, E. Basic Equation of Mass Transport through a Membrane Layer; Elsevier: Amsterdam, The Netherlands, 2018; pp. 201–204. ISBN 978-0-12-813722-2. [Google Scholar]
- Neel, J. Pervaporation. In Membrane Separations Technology, Principles and Applications; Nobles, R.D., Stern, S.A., Eds.; Elsevier: Amsterdam, The Netherlands; pp. 143–204. ISBN 0-444-81633-X.
- Pervaporation. Membrane Technology and Application, 3rd ed.; Baker, R.W., Ed.; Wiley: Chichester, UK, 2012; pp. 379–416. ISBN 978-0-470-74372-0. [Google Scholar]
- Baker, R.W.; Wijmans, J.G.; Athayde, A.L.; Daniels, L.I.; Ly, J.H.; Le, M. The effect of concentration polarization on the separation of volatile organic compounds from water by pervaporation. J. Membr. Sci. 1997, 137, 159–172. [Google Scholar] [CrossRef]
- Sherwood, T.K.; Brian, P.L.T.; Fisher, R.E. Desalination by reverse osmosis. I & E.C. Fundam. 1967, 6, 2–12. [Google Scholar] [CrossRef]
- Bhattacharyya, D.; Williams, E. Reverse osmosis. In Membrane Handbook; Ho, W., Sinkar, K., Eds.; Van Notstrand Reinhold: New York, NY, USA, 1992; pp. 265–355. [Google Scholar]
- Bowen, W.R.; Welfoot, J.S. Modeling of performance of membrane nanofiltration—Critical assessment and model development. Chem. Eng. Sci. 2002, 57, 1121–1137. [Google Scholar] [CrossRef]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena; Wiley: New York, NY, USA, 1960. [Google Scholar]
- Kelsey, L.J.; Pillarela, M.R.; Zydney, A.L. Theoretical analysis of convective flow profiles in a hollow fiber membrane bioreactor. Chem. Eng. Sci. 1990, 45, 3211–3220. [Google Scholar] [CrossRef]
- Lee, K.L.; Baker, R.W.; Lonsdale, H.K. Membrane for power generation by pressure retarded osmosis. J. Membr. Sci. 1981, 8, 141–171. [Google Scholar] [CrossRef]
- McCutcheon, J.R.; Elimelech, M. Modeling water flux in forward osmosis: Implication for improved membrane design. AIChE J. 2007, 1. [Google Scholar] [CrossRef]
- Bui, N.N.; Arena, J.T.; McCutcheon, J.R. Proper accounting of mass transfer resistances in forward osmosis improving the accuracy of model predictions of structural parameters. J. Membr. Sci. 2015, 492, 289–302. [Google Scholar] [CrossRef]
- Nagy, E.; Kulcsár, E. Mass transport through biocatalytic membrane reactors. Desalination 2009, 245, 422–436. [Google Scholar] [CrossRef]
- Nagy, E. Mass transport through a convection flow catalytic membrane layer with dispersed nanometer-sized catalyst. Ind. Eng. Chem Res. 2010, 49, 1057–1062. [Google Scholar] [CrossRef]
- Nagy, E. Diffusive plus convective mass transport through catalytic membrane layer with dispersed nanometer-sized catalyst. Int. J. Comp. Mat. 2012, 2, 79–91. [Google Scholar] [CrossRef]
- Gu, Y.; Bacchin, P.; Favier, I.; Gin, D.; Lahitte, J.-F.; Noble, R.D.; Gómez, M.; Remigy, J.-C. Catalytic membrane reactor for Suzuki-Miyaura C-C cross-coupling: Explanation for its high efficiency via modeling. AIChE J. 2017, 63, 698–701. [Google Scholar] [CrossRef]
- Nagy, E. Coupled effect of the membrane properties and concentration polarization in pervaporation: Unified mass transport model. Sep. Purif. Technol. 2010, 73, 194–201. [Google Scholar] [CrossRef]
- Nagy, E.; Kulcsár, E.; Nagy, A. Membrane mass transport by nanofiltration: Coupled effect of the polarization and membrane layers. J. Membr. Sci. 2011, 368, 215–222. [Google Scholar] [CrossRef]
- Nagy, E. Nanofiltration of uncharged solutes: Effect of the polarization and membrane layers on separation. Desal. Water. Treat. 2011, 34, 70–74. [Google Scholar] [CrossRef]
- Achilli, A.; Cath, T.H.; Childress, A.E. Power generation with pressure retarded osmosis: An experimental and theoretical investigations. J. Membr. Sci. 2009, 343, 42–52. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nagy, E.; Vitai, M. From “Black Box” to a Real Description of Overall Mass Transport through Membrane and Boundary Layers. Membranes 2019, 9, 18. https://doi.org/10.3390/membranes9020018
Nagy E, Vitai M. From “Black Box” to a Real Description of Overall Mass Transport through Membrane and Boundary Layers. Membranes. 2019; 9(2):18. https://doi.org/10.3390/membranes9020018
Chicago/Turabian StyleNagy, Endre, and Márta Vitai. 2019. "From “Black Box” to a Real Description of Overall Mass Transport through Membrane and Boundary Layers" Membranes 9, no. 2: 18. https://doi.org/10.3390/membranes9020018
APA StyleNagy, E., & Vitai, M. (2019). From “Black Box” to a Real Description of Overall Mass Transport through Membrane and Boundary Layers. Membranes, 9(2), 18. https://doi.org/10.3390/membranes9020018