Significance of Brownian Motion for Nanoparticle and Virus Capture in Nanocellulose-Based Filter Paper
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation of Filter Paper
2.3. Thickness Evaluation
2.4. Filtration of Gold Nanoparticles
2.5. Filtration of ΦX174 Bacteriophages
2.6. Theoretical Modeling of Hydrodynamic Velocity and Brownian Motion
3. Results and Discussion
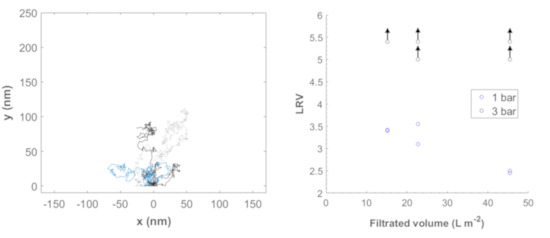
3.1. Theoretical Modeling of Hydrodynamic Velocity and Brownian Motion
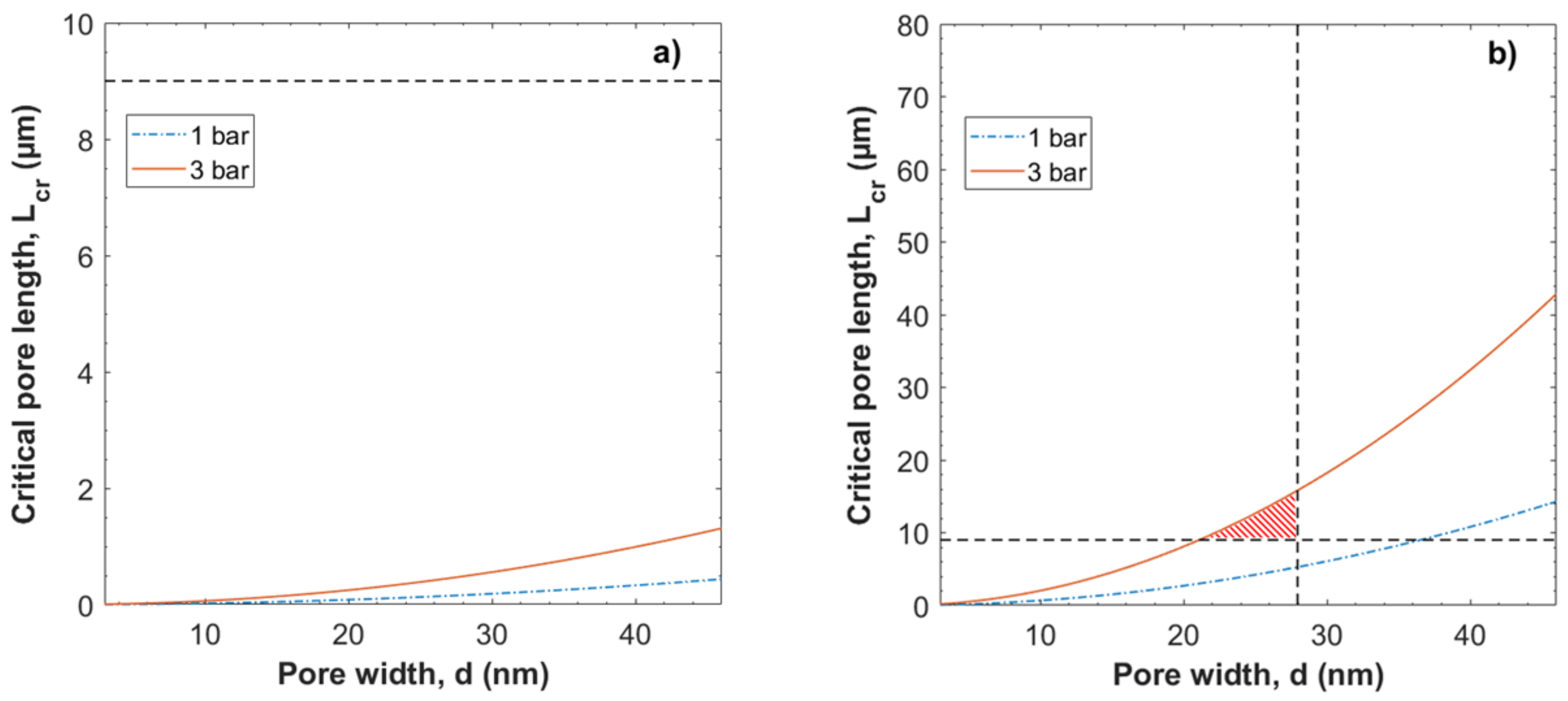
3.2. Evaluation of the Péclet Number and Critical Flow Velocity for Particle Constraint
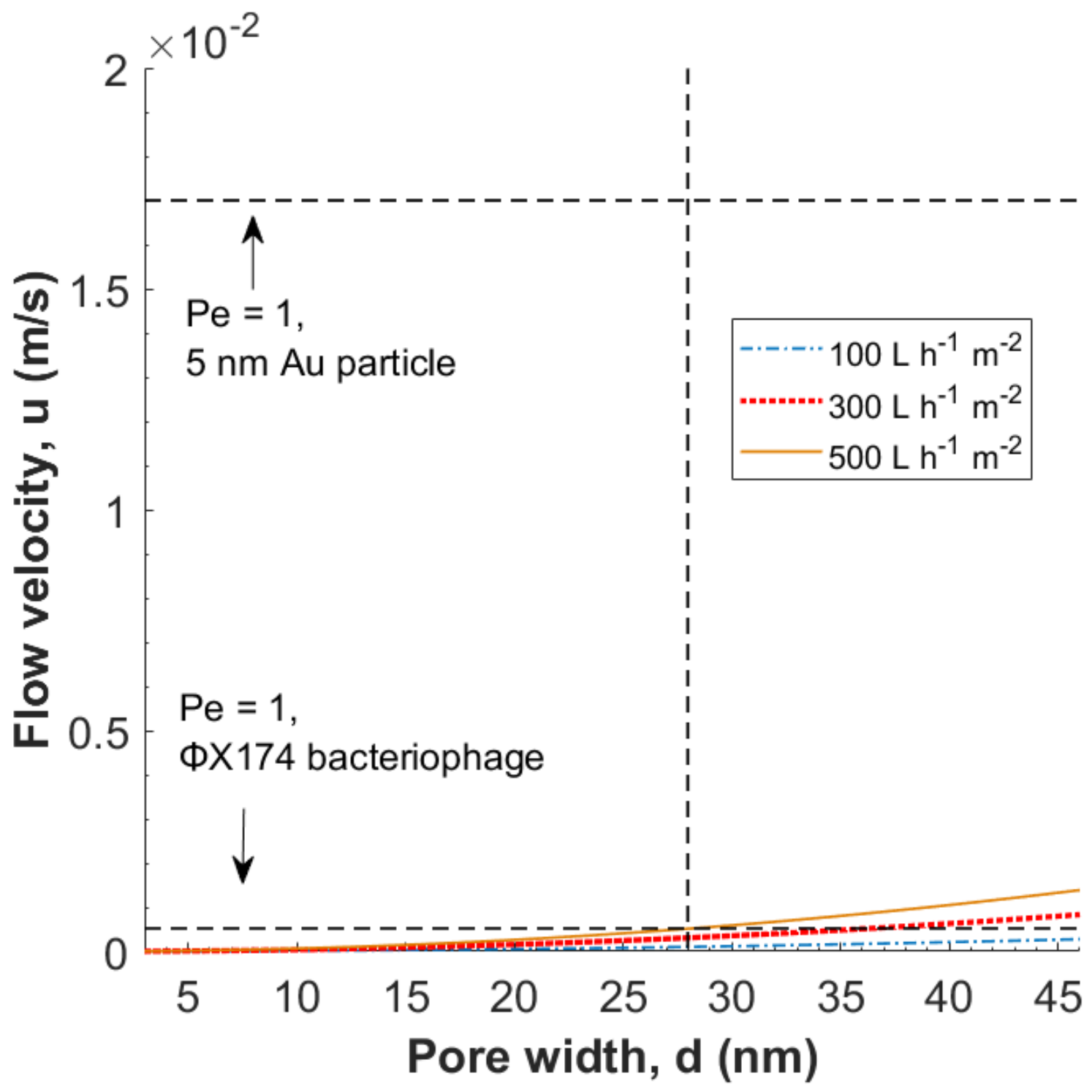
3.3. Modeling of Local Flow Velocities
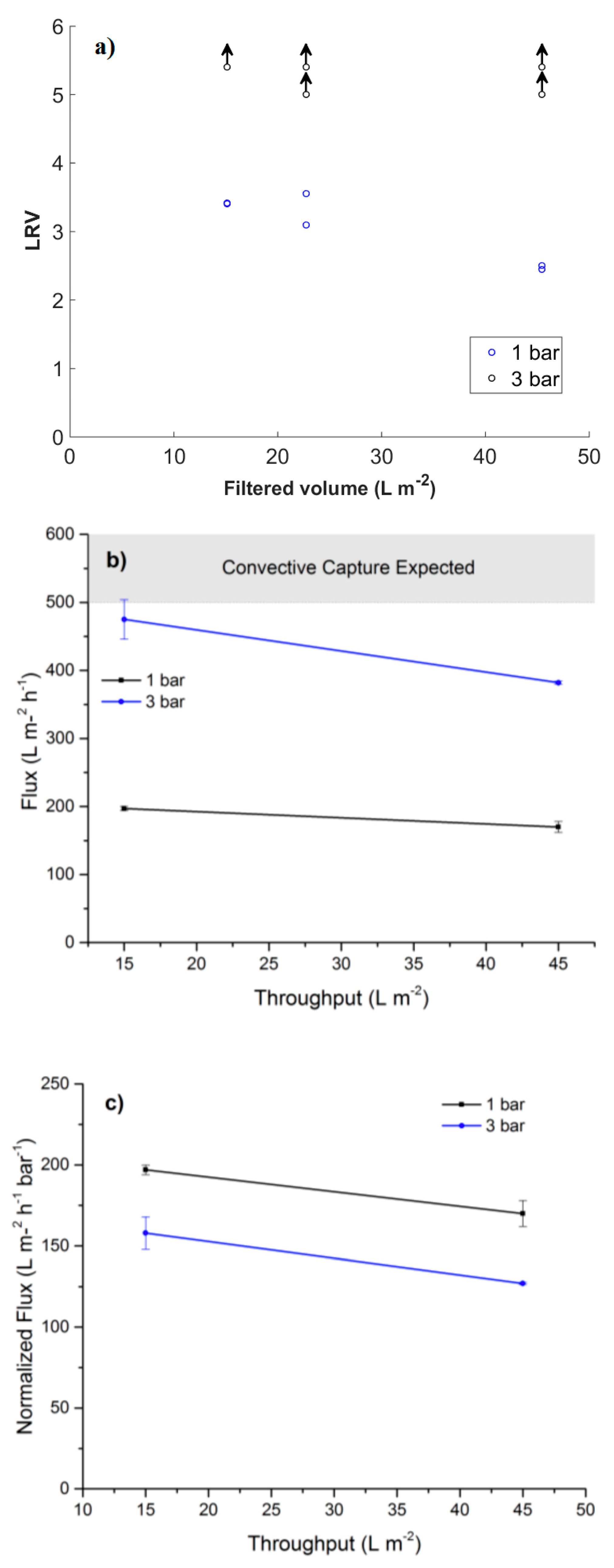
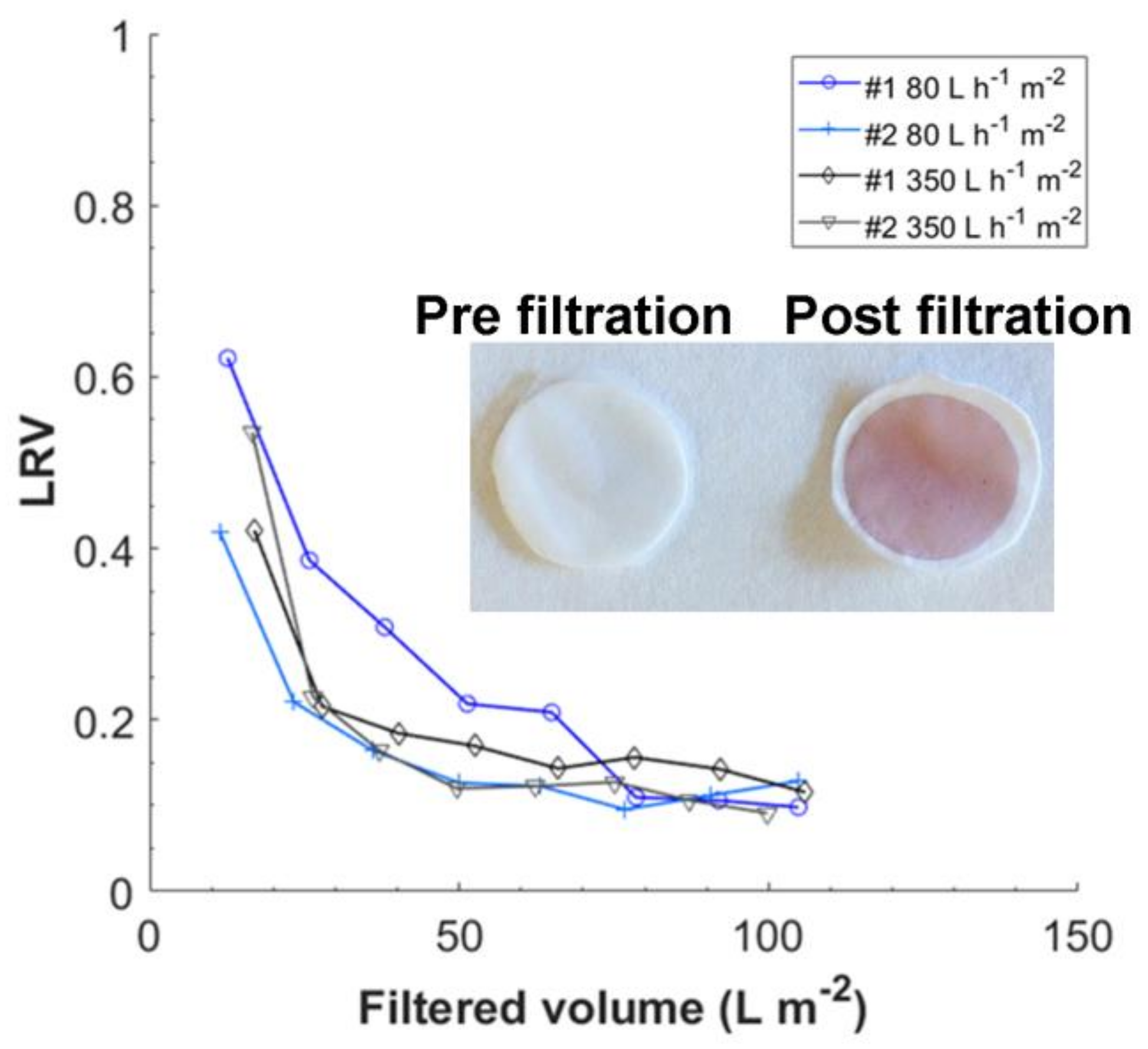
3.4. Filtration of ΦX174 Bacteriophages
3.5. Filtration of Nanoparticles
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Remington, K.M. Fundamental strategies for viral clearance. Available online: https://bioprocessintl.com/wp-content/uploads/2015/05/13-5-Remington-Secured1.pdf (accessed on 5 October 2018).
- Asper, M. Virus breakthrough after pressure release during virus retentive filtration. In PDA; Barcelona: Barcelona, Spain, 2011. [Google Scholar]
- Woods, M.A.; Zydney, A.L. Effects of a pressure release on virus retention with the ultipor dv20 membrane. Biotechnol. Bioeng. 2014, 111, 545–551. [Google Scholar] [CrossRef] [PubMed]
- Dishari, S.K.; Venkiteshwaran, A.; Zydney, A.L. Probing effects of pressure release on virus capture during virus filtration using confocal microscopy. Biotechnol. Bioeng. 2015, 112, 2115–2122. [Google Scholar] [CrossRef] [PubMed]
- Chen, D. Viral clearance using traditional, well-understood unit operations (session I): Virus-retentive filtration. PDA J. Pharm. Sci. Technol. 2014, 68, 38–50. [Google Scholar] [CrossRef] [PubMed]
- Batchelor, G.K. An Introduction to Fluid Dynamics; Cambridge University Press: Cambridge, UK, 1967; p. 180. [Google Scholar]
- Levy, D.J. Some Physical Properties of Enzymes. J. Infect. Dis. 1905, 2, 1–48. [Google Scholar] [CrossRef]
- Liu, S.; Carroll, M.; Iverson, R.; Valera, C.; Vennari, J.; Turco, K.; Piper, R.; Kiss, R.; Lutz, H. Development and qualification of a novel virus removal filter for cell culture applications. Biotechnol. Progr. 2000, 16, 425–434. [Google Scholar] [CrossRef] [PubMed]
- Trilisky, E.I.; Lenhoff, A.M. Flow-dependent entrapment of large bioparticles in porous process media. Biotechnol. Bioeng. 2009, 104, 127–133. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, A.; Hongo-Hirasaki, T.; Uchi, Y.; Hayashida, H.; Nagoya, F. Effect of hydrodynamic forces on virus removal capability of planova™ filters. AIChE J. 2014, 60, 2286–2297. [Google Scholar] [CrossRef]
- Lemons, D.S.; Gythiel, A. Paul langevin’s 1908 paper “on the theory of brownian motion” [“sur la théorie du mouvement brownien,” c. R. Acad. Sci. (Paris) 146, 530–533 (1908)]. Am. J. Phys. 1997, 65, 1079–1081. [Google Scholar] [CrossRef]
- Nelson, E. Dynamical Theories of Brownian Motion. Math. Notes 1967, 131, 2381–2396. [Google Scholar] [CrossRef]
- Atkins, P.; de Paula, J. Atkins’ Physical Chemistry; Oxford University Press: Oxford, UK, 2010; p. 773. [Google Scholar]
- Stokes, G.G. On the Effect of the Internal Friction of Fluids on the Motion of Pendulums. Trans. Cambridge Philos. Soc. 1851, 9, 8. [Google Scholar]
- Odar, F.; Hamilton, W.S. Forces on a Sphere Accelerating in a Viscous Fluid. J. Fluid Mech. 1964, 18, 302–314. [Google Scholar] [CrossRef]
- Odar, F. Verification of the Proposed Equation for Calculation of the Forces on a Sphere Accelerating in a Viscous Fluid. J. Fluid Mech. 1966, 25, 591–592. [Google Scholar] [CrossRef]
- Satoh, A. Introduction to Molecular-Microsimulation of Colloidal Dispersions; Elsevier: Amsterdam, The Netherlands, 2003; pp. 127–132. [Google Scholar]
- Milne-Thomson, L.M. Theoretical Hydrodynamics; Macmillan: London, UK, 1968. [Google Scholar]
- Gustafsson, S.; Lordat, P.; Hanrieder, T.; Asper, M.; Schaefer, O.; Mihranyan, A. Mille-feuille paper: A novel type of filter architecture for advanced virus separation applications. Mater. Horiz. 2016, 3, 320–327. [Google Scholar] [CrossRef]
- Gustafsson, S.; Mihranyan, A. Strategies for tailoring the pore-size distribution of virus retention filter papers. ACS Appl. Mater. Interfaces 2016, 8, 13759–13767. [Google Scholar] [CrossRef] [PubMed]
- Quellmalz, A.; Mihranyan, A. Citric acid cross-linked nanocellulose-based paper for size-exclusion nanofiltration. ACS Biomater. Sci. Eng. 2015, 1, 271–276. [Google Scholar] [CrossRef]
- Metreveli, G.; Wågberg, L.; Emmoth, E.; Belak, S.; Strømme, M.; Mihranyan, A. A size-exclusion nanocellulose filter paper for virus removal. Adv. Healthc. Mater. 2014, 3, 1546–1550. [Google Scholar] [CrossRef] [PubMed]
- Asper, M.; Hanrieder, T.; Quellmalz, A.; Mihranyan, A. Removal of xenotropic murine leukemia virus by nanocellulose based filter paper. Biologicals 2015, 43, 452–456. [Google Scholar] [CrossRef] [PubMed]
- Gefroh, E.; Dehghani, H.; McClure, M.; Connell-Crowley, L.; Vedantham, G. Use of mmv as a single worst-case model virus in viral filter validation studies. PDA J. Pharm. Sci. Technol. 2014, 68, 297–311. [Google Scholar] [CrossRef] [PubMed]
- Gustafsson, S.; Manukyan, L.; Mihranyan, A. Protein–nanocellulose interactions in paper filters for advanced separation applications. Langmuir 2017, 33, 4729–4736. [Google Scholar] [CrossRef] [PubMed]
- Mihranyan, A. Cellulose from cladophorales green algae: From environmental problem to high-tech composite materials. J. Appl. Polym. Sci. 2011, 119, 2449–2460. [Google Scholar] [CrossRef]
- Sinsheimer, R.L. Bacteriophage φX174; King, R.C., Ed.; Springer US: Boston, MA, USA, 1974; pp. 323–325. [Google Scholar]
- Kleinstreuer, C. Microfluidics and Nanofluidics: Theory and Selected Applications; John Wiley & Sons: Hoboken, NJ, USA, 2014; p. 205. [Google Scholar]
- Rott, N. Note on the History of the Reynolds Number. Annu. Rev. Fluid Mech. 1990, 22, 1–12. [Google Scholar] [CrossRef]
- Barrett, E.P.; Joyner, L.G.; Halenda, P.P. The determination of pore volume and area distributions in porous substances. I. Computations from nitrogen isotherms. J. Am. Chem. Soc. 1951, 73, 373–380. [Google Scholar] [CrossRef]




© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gustafsson, O.; Gustafsson, S.; Manukyan, L.; Mihranyan, A. Significance of Brownian Motion for Nanoparticle and Virus Capture in Nanocellulose-Based Filter Paper. Membranes 2018, 8, 90. https://doi.org/10.3390/membranes8040090
Gustafsson O, Gustafsson S, Manukyan L, Mihranyan A. Significance of Brownian Motion for Nanoparticle and Virus Capture in Nanocellulose-Based Filter Paper. Membranes. 2018; 8(4):90. https://doi.org/10.3390/membranes8040090
Chicago/Turabian StyleGustafsson, Olof, Simon Gustafsson, Levon Manukyan, and Albert Mihranyan. 2018. "Significance of Brownian Motion for Nanoparticle and Virus Capture in Nanocellulose-Based Filter Paper" Membranes 8, no. 4: 90. https://doi.org/10.3390/membranes8040090
APA StyleGustafsson, O., Gustafsson, S., Manukyan, L., & Mihranyan, A. (2018). Significance of Brownian Motion for Nanoparticle and Virus Capture in Nanocellulose-Based Filter Paper. Membranes, 8(4), 90. https://doi.org/10.3390/membranes8040090