Effect of DS Concentration on the PRO Performance Using a 5-Inch Scale Cellulose Triacetate-Based Hollow Fiber Membrane Module
Abstract
:1. Introduction
2. Experimental
2.1. Chemicals
2.2. HF Membrane Module
2.3. CTA HF Module Performance
2.3.1. PRO/RO Hybrid System
2.3.2. RO Experiments
2.3.3. PRO Performance Evaluation
2.3.4. Module Performance Comparison between SW and HF Configuration
3. Results and Discussion
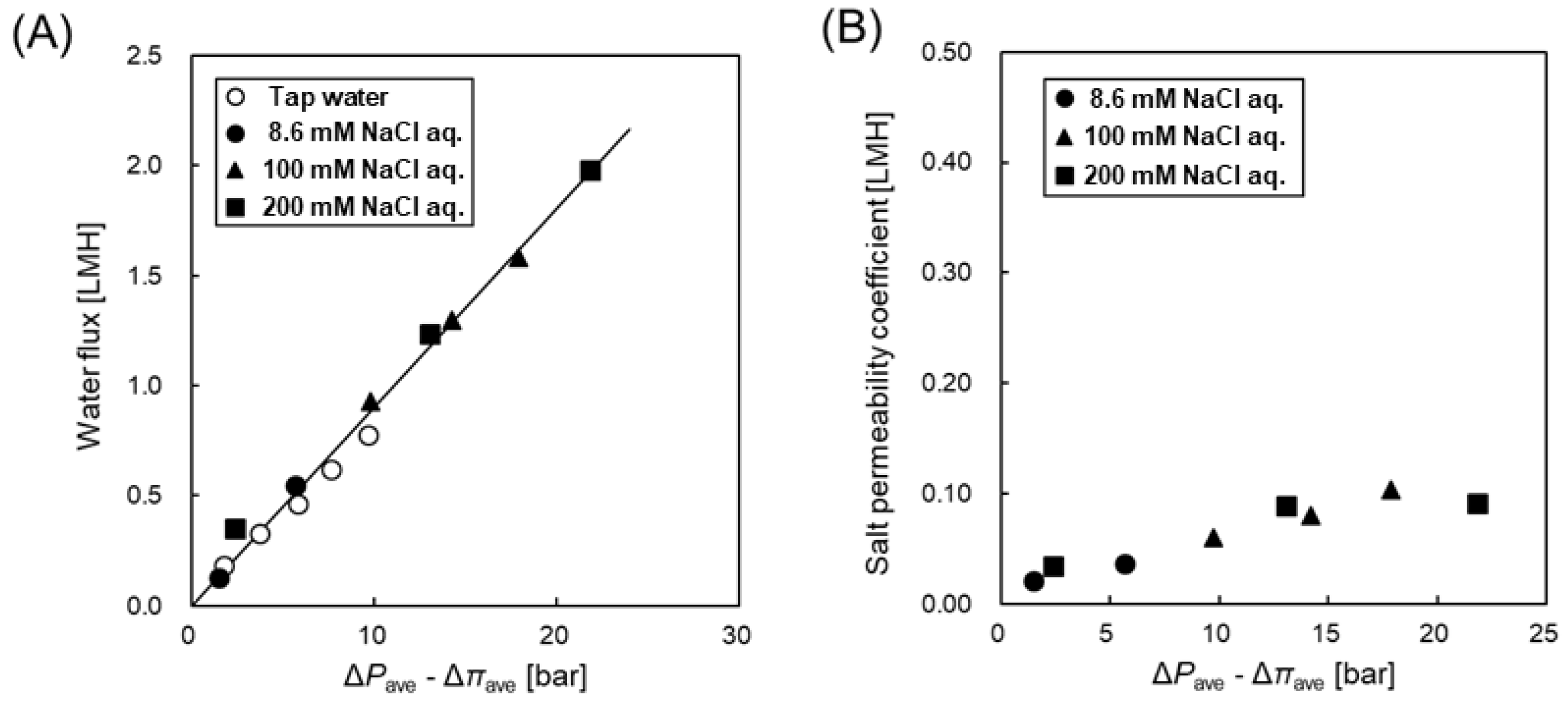
3.1. Water and Salt Permeability in the RO Experiment
3.2. PRO Performance
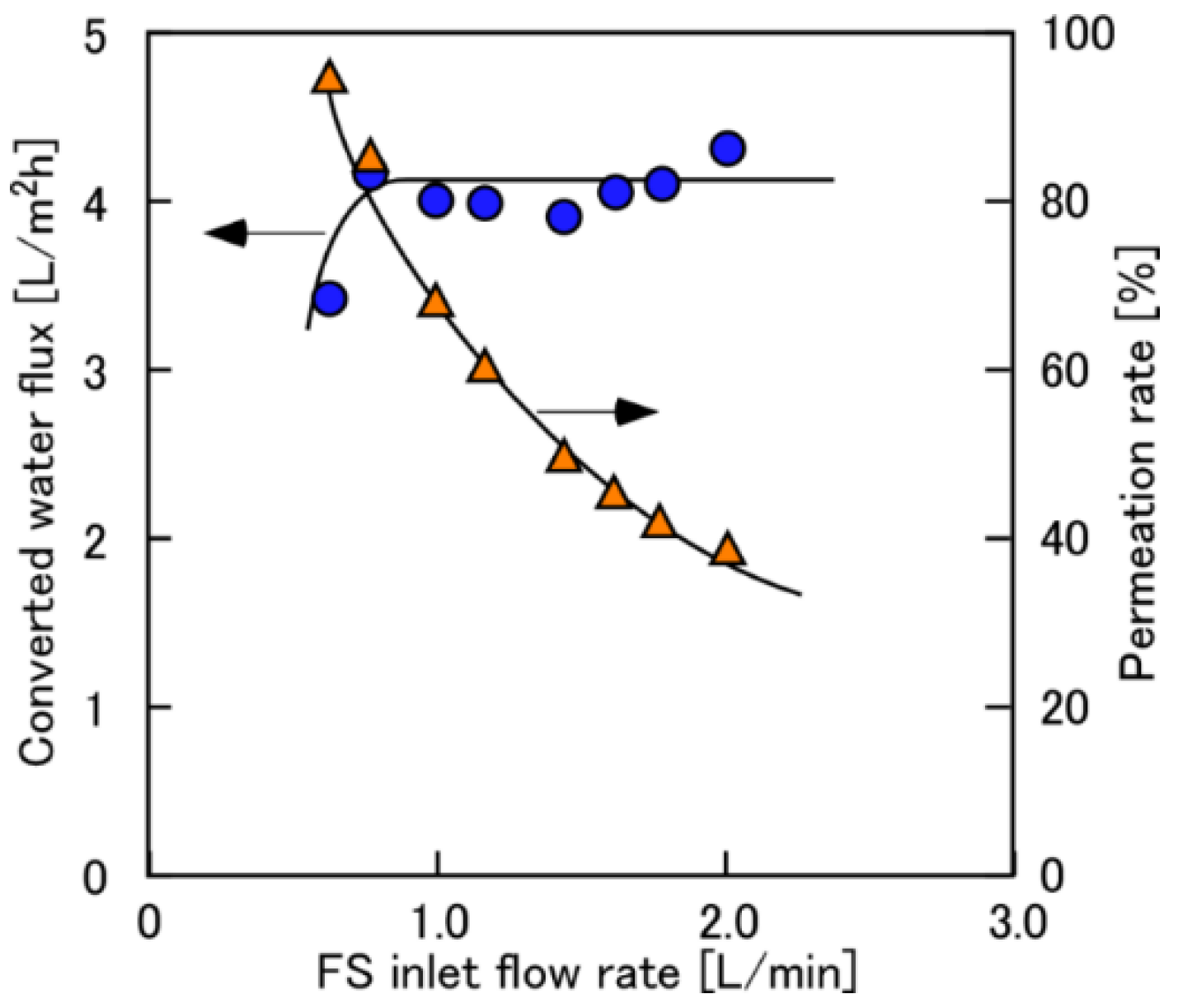
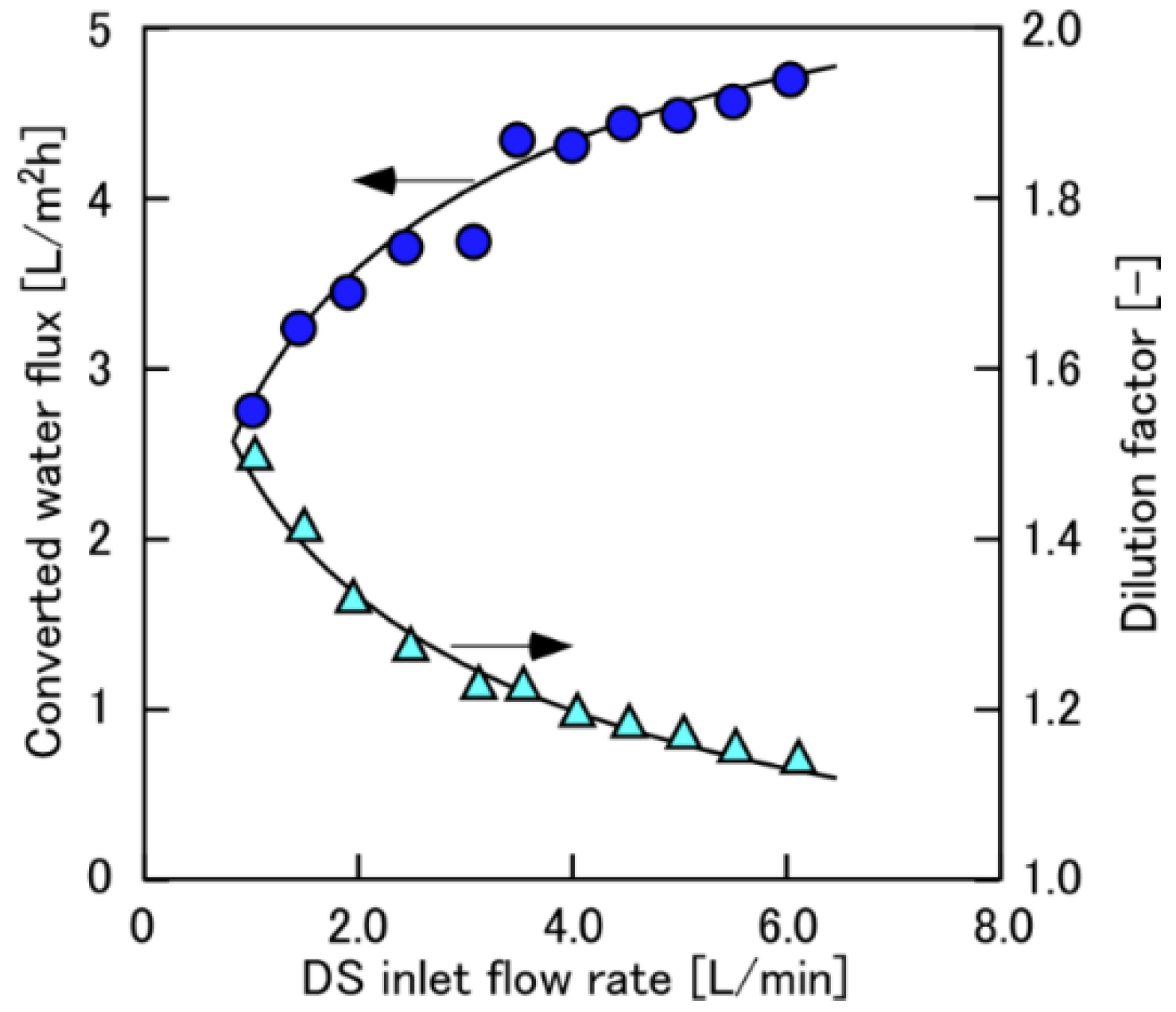
3.2.1. Effects of QFS,in and QDS,in on Water Flux
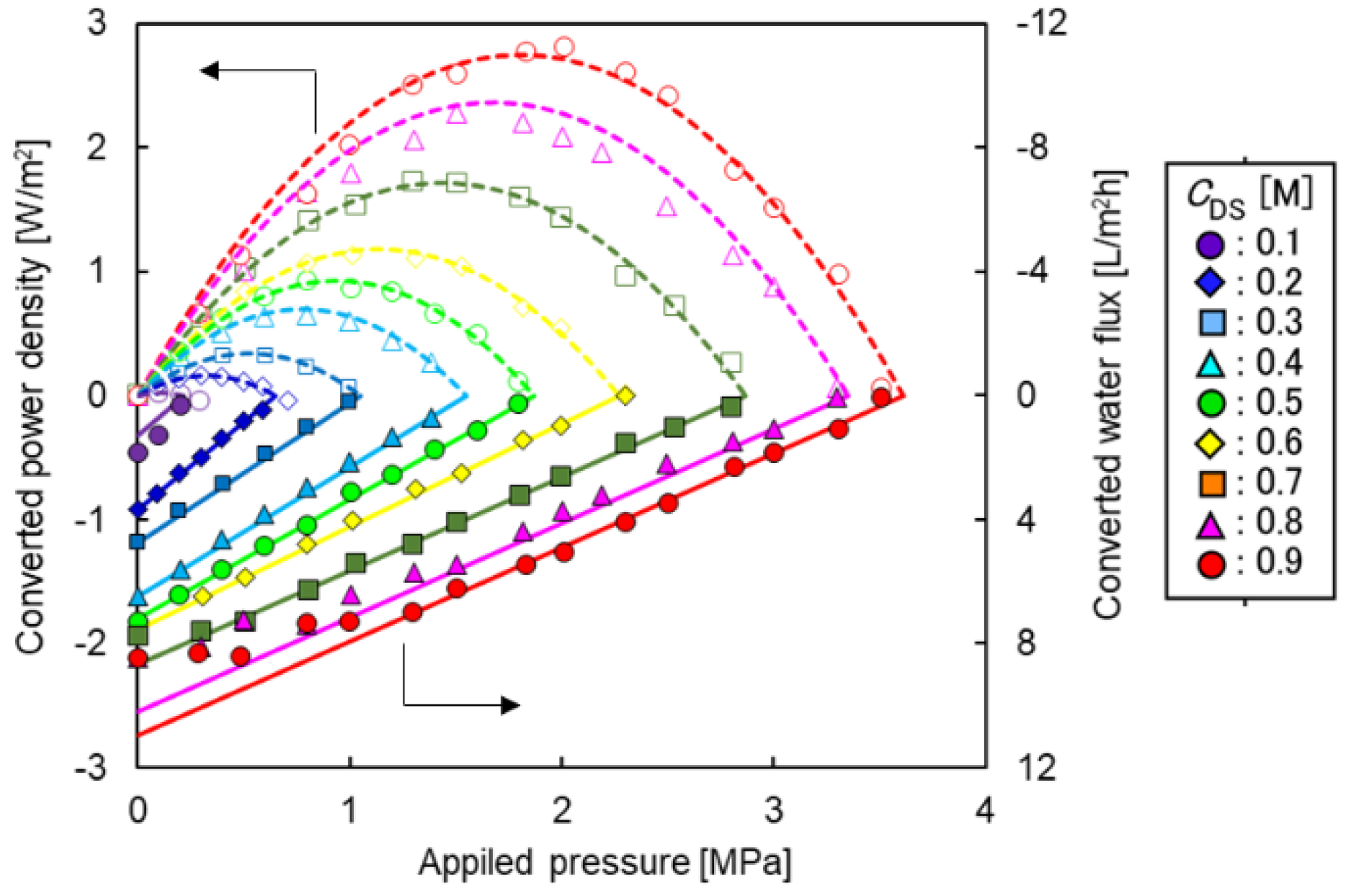
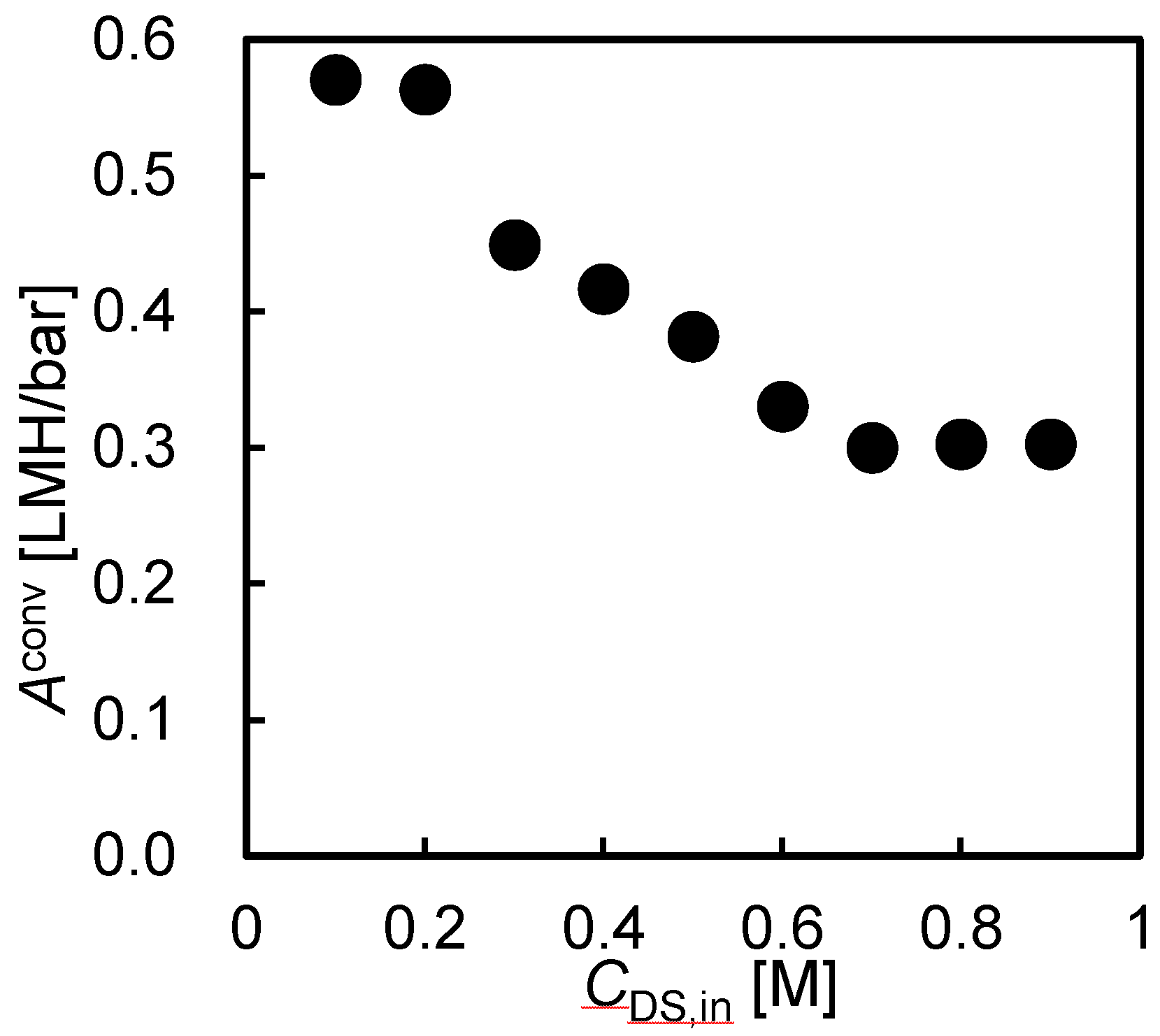
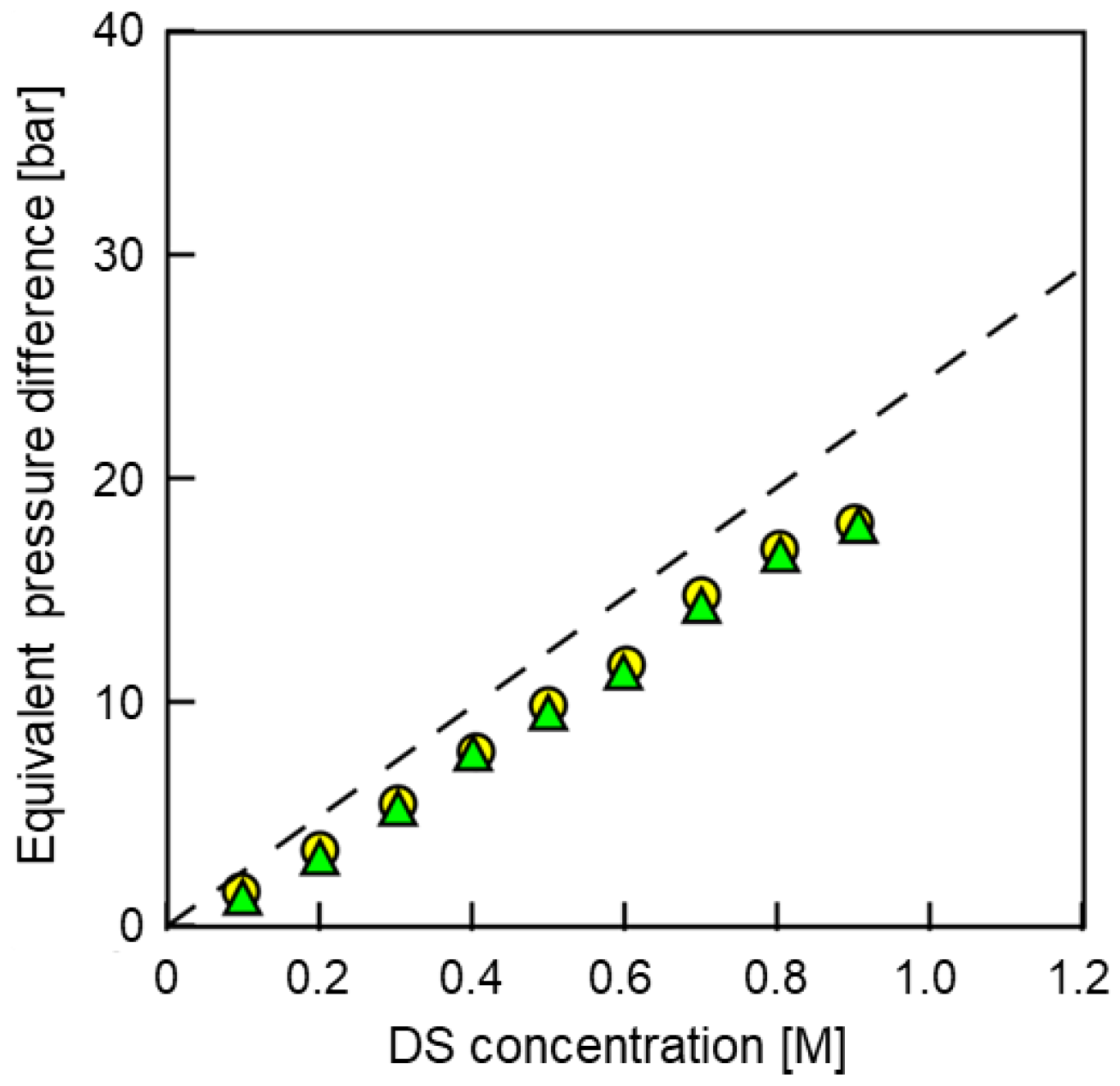
3.2.2. Effects of Applied Hydraulic Pressure and DS Concentration
3.2.3. Deviations from the Theory
3.3. Module Performance Comparison in PRO with Different Configurations
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- McGinnis, R.L.; Elimelech, M. Global challenges in energy and water supply: The promise of engineered osmosis. Environ. Sci. Technol. 2008, 42, 8625–8629. [Google Scholar] [CrossRef] [PubMed]
- Yip, N.Y.; Elimelech, M. Thermodynamic and Energy Efficiency Analysis of Power Generation from Natural Salinity Gradients by Pressure Retarded Osmosis. Environ. Sci. Technol. 2012, 46, 5230–5239. [Google Scholar] [CrossRef] [PubMed]
- Długołecki, P.; Nymeijer, K.; Metz, S.; Wessling, M. Current status of ion exchange membranes for power generation from salinity gradients. J. Membr. Sci. 2008, 319, 214–222. [Google Scholar] [CrossRef]
- Post, J.W.; Hamelers, H.V.M.; Buisman, C.J.N. Energy Recovery from Controlled Mixing Salt and Fresh Water with a Reverse Electrodialysis System. Environ. Sci. Technol. 2008, 42, 5785–5790. [Google Scholar] [CrossRef] [PubMed]
- Post, J.W.; Veerman, J.; Hamelers, H.V.M.; Euverink, G.J.W.; Metzb, S.J.; Nymeijer, K.; Buisman, C.J.N. Salinity-gradient power: Evaluation of pressure-retarded osmosis and reverse electrodialysis. J. Membr. Sci. 2007, 288, 218–230. [Google Scholar] [CrossRef]
- Klaysom, C.; Cath, T.Y.; Depuydt, T.; Vankelecom, I.F. Forward and pressure retarded osmosis: Potential solutions for global challenges in energy and water supply. Chem. Soc. Rev. 2013, 42, 6959–6989. [Google Scholar] [CrossRef] [PubMed]
- Loeb, S.; Norman, R.S. Osmotic power plants. Science 1975, 189, 654–655. [Google Scholar] [CrossRef] [PubMed]
- Loeb, S. Production of energy from concentrated brines by pressure-retarded osmosis. I. Preliminary technical and economic correlations. J. Membr. Sci. 1976, 1, 49–63. [Google Scholar] [CrossRef]
- Thorsen, T.; Holt, T. The potential for power production from salinity gradients by pressure retarded osmosis. J. Membr. Sci. 2009, 335, 103–110. [Google Scholar] [CrossRef]
- Achilli, A.; Childress, A.E. Pressure retarded osmosis: From the vision of Sidney Loeb to the first prototype installation—Review. Desalination 2010, 261, 205–211. [Google Scholar] [CrossRef]
- Patel, S. Norway inaugurates osmotic power plant. Power. 2 January 2010, p. 154. Available online: http://www.powermag.com/norway-inaugurates-osmotic-power-plant/ (accessed on 19 April 2018).
- Skilhagen, S.E.; Dugstad, J.E.; Aaberg, R.J. Osmotic power—Power production based on the osmotic pressure difference between waters with varying salt gradients. Desalination 2008, 220, 476–482. [Google Scholar] [CrossRef]
- Gerstandt, K.; Peinemann, K.V.; Skilhagen, S.E.; Thorsen, T.; Holt, T. Membrane processes in energy supply for an osmotic power plant. Desalination 2008, 224, 64–70. [Google Scholar] [CrossRef]
- Yip, N.Y.; Tiraferri, A.; Phillip, W.A.; Schiffman, J.D.; Hoover, L.A.; Kim, Y.C.; Elimelech, M. Thin-film composite pressure retarded osmosis membranes for sustainable power generation from salinity gradients. Environ. Sci. Technol. 2011, 45, 4360–4369. [Google Scholar] [CrossRef] [PubMed]
- Yip, N.Y.; Elimelech, M. Performance limiting effects in power generation from salinity gradients by pressure retarded osmosis. Environ. Sci. Technol. 2011, 45, 10273–10282. [Google Scholar] [CrossRef] [PubMed]
- Achilli, A.; Cath, T.Y.; Childress, A.E. Power generation with pressure retarded osmosis: An experimental and theoretical investigation. J. Membr. Sci. 2009, 343, 42–52. [Google Scholar] [CrossRef]
- She, Q.; Jin, X.; Tang, C.Y. Osmotic power production from salinity gradient resource by pressure retarded osmosis: Effects of operating conditions and reverse solute diffusion. J. Membr. Sci. 2012, 401–402, 262–273. [Google Scholar] [CrossRef]
- Kim, Y.C.; Elimelech, M. Adverse impact of feed channel spacers on the performance of pressure retarded osmosis. Environ. Sci. Technol. 2012, 46, 4673–4681. [Google Scholar] [CrossRef] [PubMed]
- She, Q.; Wonga, Y.W.; Zhao, S.; Tang, C.Y. Organic fouling in pressure retarded osmosis: Experiments, mechanisms and implications. J. Membr. Sci. 2013, 428, 181–189. [Google Scholar] [CrossRef]
- Kim, Y.C.; Elimelech, M. Potential of osmotic power generation by pressure retarded osmosis using seawater as feed solution: Analysis and experiments. J. Membr. Sci. 2013, 429, 330–337. [Google Scholar] [CrossRef]
- Zhang, S.; Fu, F.; Chung, T.S. Substrate modifications and alcohol treatment on thin film composite membranes for osmotic power. Chem. Eng. Sci. 2013, 87, 40–50. [Google Scholar] [CrossRef]
- Alsvik, I.L.; Hagg, M.B. Preparation of thin film composite membranes with polyamide film on hydrophilic supports. J. Membr. Sci. 2013, 428, 225–231. [Google Scholar] [CrossRef]
- Hoover, L.A.; Schiffman, J.D.; Elimelech, M. Nanofibers in thin-film composite membrane support layers: Enabling expanded application of forward and pressure retarded osmosis. Desalination 2013, 308, 73–81. [Google Scholar] [CrossRef]
- Loeb, S.; van Hessen, F.; Shahaf, D. Production of energy from concentrated brines by pressure-retarded osmosis. II. Experimental results and projected energy cost. J. Membr. Sci. 1976, 1, 249–269. [Google Scholar] [CrossRef]
- Chou, S.; Wang, R.; Shi, L.; She, Q.; Tang, C.Y.; Fane, A.G. Thin-flim composite hollow fiber membranes for pressure retarded osmosis (PRO) process with high power density. J. Membr. Sci. 2012, 389, 25–33. [Google Scholar] [CrossRef]
- Sivertsen, E.; Holt, T.; Thelin, W.; Brekke, G. Modelling mass transport in hollow fibre membranes used for pressure retarded osmosis. J. Membr. Sci. 2012, 417–418, 69–79. [Google Scholar] [CrossRef]
- Helfer, F.; Lemckert, C.; Anissimov, Y.G. Osmotic power with pressure retarded osmosis: Theory, performance and trends—A review. J. Membr. Sci. 2014, 453, 337–358. [Google Scholar] [CrossRef]
- Xu, Y.; Peng, X.; Tang, C.Y.; Fu, Q.S.; Nie, S. Effect of draw solution concentration and operating conditions on forward osmosis and pressure retarded osmosis performance in a spiral wound module. J. Membr. Sci. 2010, 348, 298–309. [Google Scholar] [CrossRef]
- Kim, Y.C.; Kim, Y.; Oh, D.; Lee, K.H. Experimental investigation of a spiral-wound pressure-retarded osmosis membrane module for osmotic power generation. Environ. Sci. Technol. 2013, 47, 2966–2973. [Google Scholar] [CrossRef] [PubMed]
- Shibuya, M.; Yasukawa, M.; Goda, S.; Sakurai, H.; Takahashi, T.; Higa, M.; Matsuyama, H. Experimental and theoretical study of a forward osmosis hollow fiber membrane module with a cross-wound configuration. J. Membr. Sci. 2016, 504, 10–19. [Google Scholar] [CrossRef]
- Higa, M.; Shigefuji, D.; Shibuya, M.; Izumikawa, S.; Ikebe, Y.; Yasukawa, M.; Endo, N.; Tanioka, A. Experimental study of a hollow fiber membrane module in pressure-retarded osmosis: Module performance comparison with volumetric-based power outputs. Desalination 2017, 420, 45–53. [Google Scholar] [CrossRef]
- Shibuya, M.; Yasukawa, M.; Takahashi, T.; Miyoshi, T.; Higa, M.; Matsuyama, H. Effects of operating conditions and membrane structures on the performance of hollow fiber forward osmosis membranes in pressure assisted osmosis. Desalination 2015, 365, 381–388. [Google Scholar] [CrossRef]
- Han, G.; Zhang, S.; Li, X.; Widjojo, N.; Chung, T.-S. Thin film composite forward osmosis membranes based on polydopamine modified polysulfone substrates with enhancements in both water flux and salt rejection. Chem. Eng. Sci. 2012, 80, 219–231. [Google Scholar] [CrossRef]
- Lee, K.L.; Baker, R.W.; Lonsdale, H.K. Membranes for power generation by pressure-retarded osmosis. J. Membr. Sci. 1981, 8, 141–171. [Google Scholar] [CrossRef]
- Yasukawa, M.; Mishima, S.; Shibuya, M.; Saeki, D.; Takahashi, T.; Miyoshi, T.; Matsuyama, H. Preparation of a forward osmosis membrane using a highly porous polyketone as a novel support. J. Membr. Sci. 2015, 487, 51–59. [Google Scholar] [CrossRef]
- Yasukawa, M.; Tanaka, Y.; Takahashi, T.; Shibuya, M.; Mishima, S.; Matsuyama, H. Effect of molecular weight of draw solute on water permeation in forward osmosis process. Ind. Eng. Chem. Res. 2015, 54, 8239–8246. [Google Scholar] [CrossRef]


| CTA-HF Module A | |
|---|---|
| Material | Cellulose triacetate |
| Configuration | Radial flow with crossly wounded HF config. |
| Number of ports | 4 |
| Module (Element) diameter | 176 (136) mm |
| Module (Element) length | 825 (683) mm |
| Inner diameter of hollow fiber | 60 µm |
| Outer diameter of hollow fiber | 160 µm |
| Number of hollow fibers | 214,000 |
| Membrane area | 72 m2 |
| Water permeability A | 0.09 LMH/bar |
| Salt permeability B | 0.029 LMH |
| Module | Type | Diameter | Length | Mod. vol., Vmod | Sm | Sm/Vmod | CDS | T | ΔP at Wmax | Wmaxarea | Wconvmaxarea | Wmaxvol | Ref | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| (inch) | (mm) | (inch) | (mm) | (m3) | (m2) | (1/m) | (M) | (°C) | (bar) | (W/m2) | (W/m2) | (kW/m3) | |||
| Hydrowell® | SW | 2.5 * | 63.5 * | 11.8 | 300 | 9.50 × 10−4 * | 0.94 | 989 * | 0.50 | 22–24 | 4.5 | 0.40 | - | 0.40 * | [28] |
| SW PRO | SW | 7.9 | 200 | 39.4 | 1000 | 3.14 × 10−2 | 29 | 923 | 0.52 | 25 | 9.8 | 0.81 | - | 0.75 | [29] |
| CTA HF (A) | HF | 5.4 | 136 | 25.1 | 638 | 9.27× 10-3 | 72 | 7769 | 0.10 | 25–27 | 1.1 | 0.00 | 0.02 | 0.02 | this study |
| 0.20 | 25–27 | 3.3 | 0.03 | 0.17 | 0.20 | this study | |||||||||
| 0.30 | 25–27 | 5.3 | 0.05 | 0.32 | 0.38 | this study | |||||||||
| 0.40 | 25–27 | 7.9 | 0.10 | 0.65 | 0.78 | this study | |||||||||
| 0.50 | 25–27 | 9.4 | 0.14 | 0.93 | 1.09 | [31] | |||||||||
| 0.60 | 25–27 | 11.4 | 0.17 | 1.13 | 1.36 | this study | |||||||||
| 0.70 | 25–27 | 14.4 | 0.27 | 1.73 | 2.08 | this study | |||||||||
| 0.80 | 25–27 | 16.8 | 0.35 | 2.28 | 2.74 | this study | |||||||||
| 0.90 | 25–27 | 18.0 | 0.44 | 2.82 | 3.39 | this study | |||||||||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yasukawa, M.; Shigefuji, D.; Shibuya, M.; Ikebe, Y.; Horie, R.; Higa, M. Effect of DS Concentration on the PRO Performance Using a 5-Inch Scale Cellulose Triacetate-Based Hollow Fiber Membrane Module. Membranes 2018, 8, 22. https://doi.org/10.3390/membranes8020022
Yasukawa M, Shigefuji D, Shibuya M, Ikebe Y, Horie R, Higa M. Effect of DS Concentration on the PRO Performance Using a 5-Inch Scale Cellulose Triacetate-Based Hollow Fiber Membrane Module. Membranes. 2018; 8(2):22. https://doi.org/10.3390/membranes8020022
Chicago/Turabian StyleYasukawa, Masahiro, Daisuke Shigefuji, Masafumi Shibuya, Yuki Ikebe, Ryuto Horie, and Mitsuru Higa. 2018. "Effect of DS Concentration on the PRO Performance Using a 5-Inch Scale Cellulose Triacetate-Based Hollow Fiber Membrane Module" Membranes 8, no. 2: 22. https://doi.org/10.3390/membranes8020022
APA StyleYasukawa, M., Shigefuji, D., Shibuya, M., Ikebe, Y., Horie, R., & Higa, M. (2018). Effect of DS Concentration on the PRO Performance Using a 5-Inch Scale Cellulose Triacetate-Based Hollow Fiber Membrane Module. Membranes, 8(2), 22. https://doi.org/10.3390/membranes8020022






