Breath-Figure Self-Assembly, a Versatile Method of Manufacturing Membranes and Porous Structures: Physical, Chemical and Technological Aspects
Abstract
:1. Introduction
2. Impact of the Polymer Architecture and Physical Parameters of the Process of Breath-Figure Self-Assembly
3. Processes Used for Breath-Figure Self-Assembly
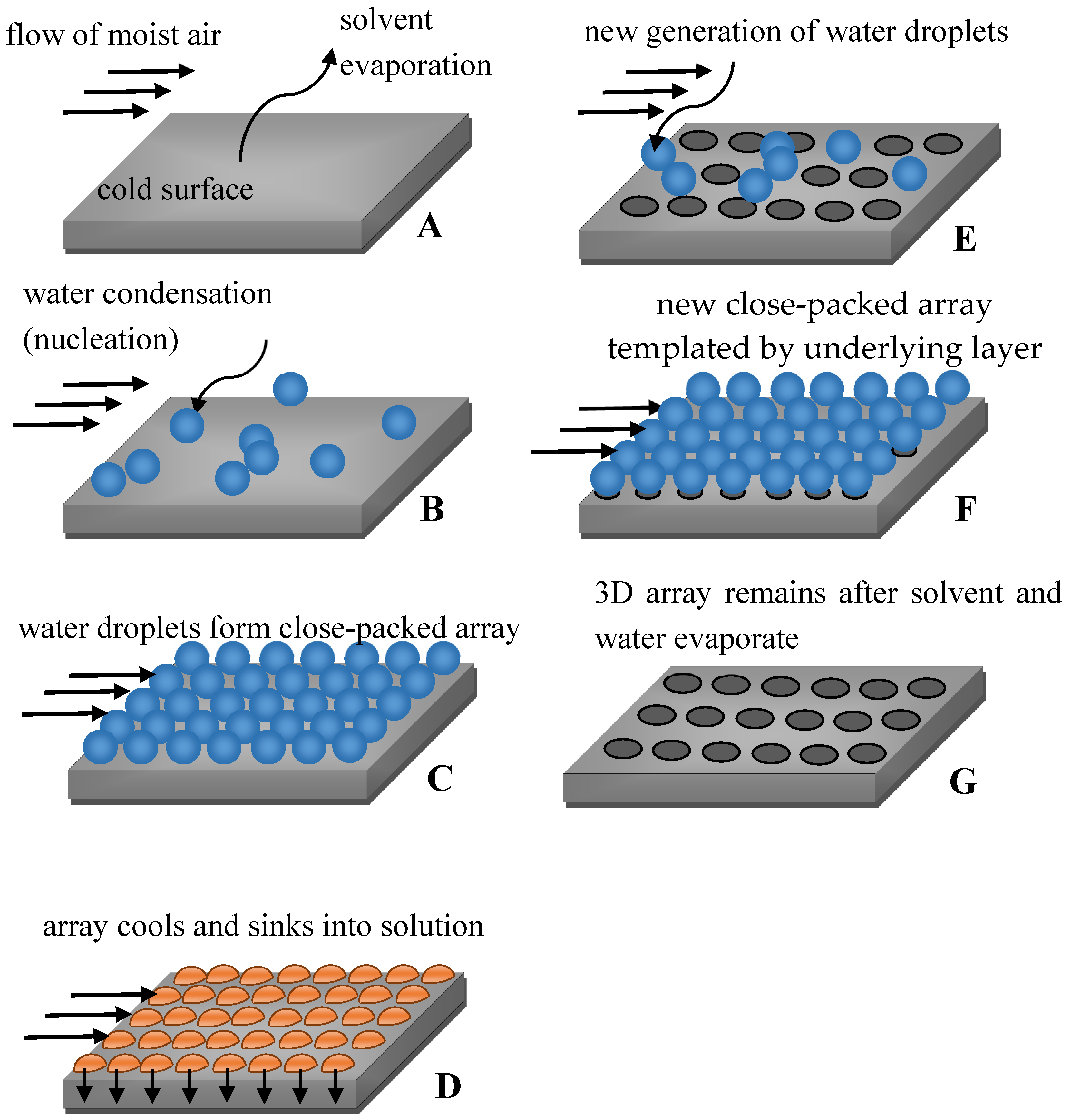
4. Main Stages of Breath-Figure Self-Assembly
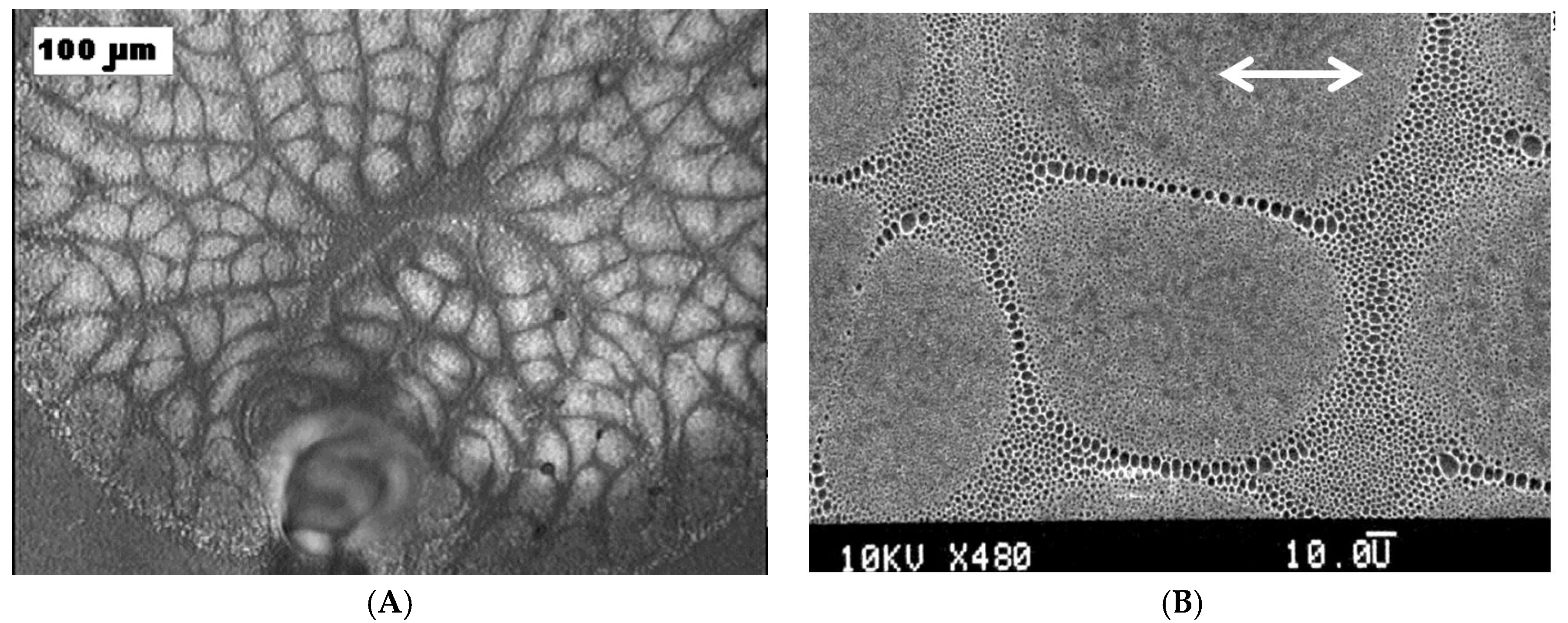
5. Multi-Scale Patterning Observed under “Breath-Figure Self-Assembly”
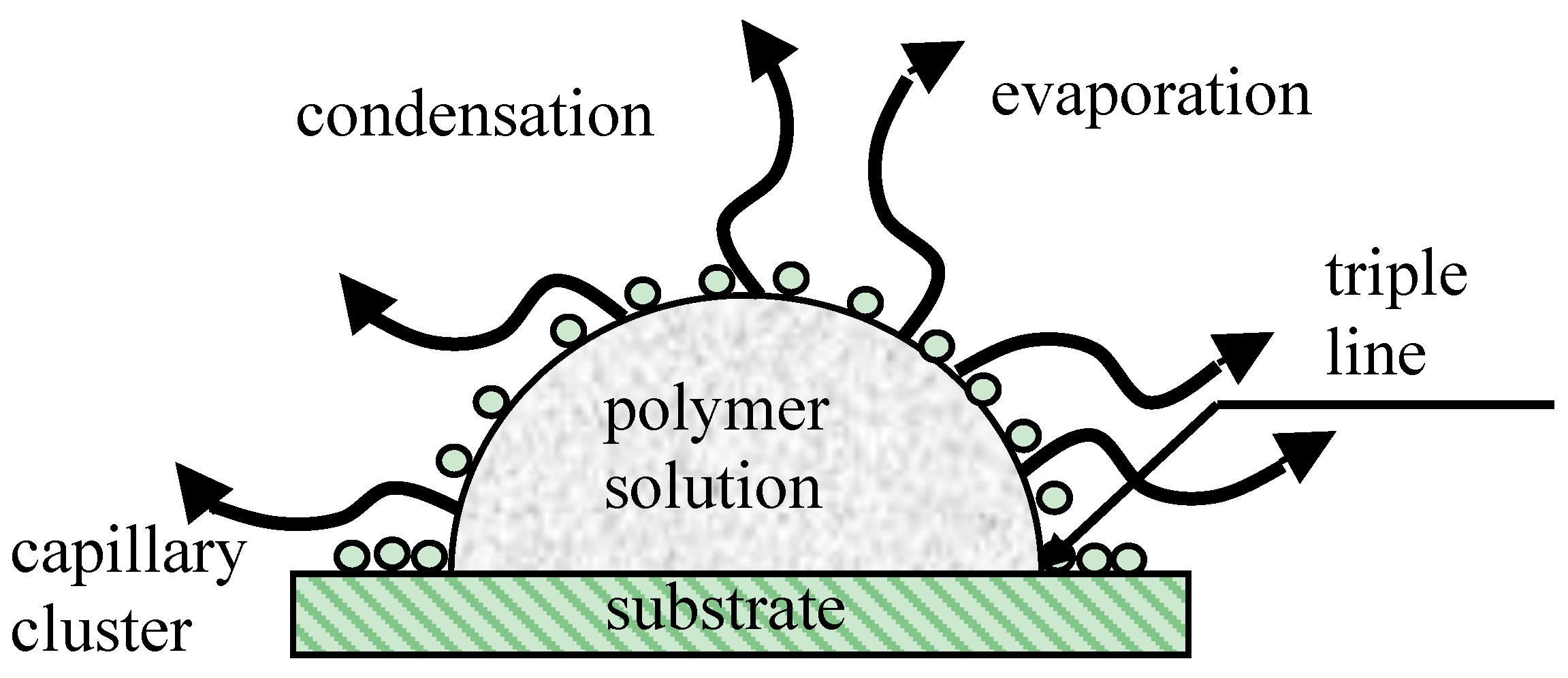
6. Main Physical Processes Involved in Breath-Figure Self-Assembly and the Hierarchy of Their Temporal Scales
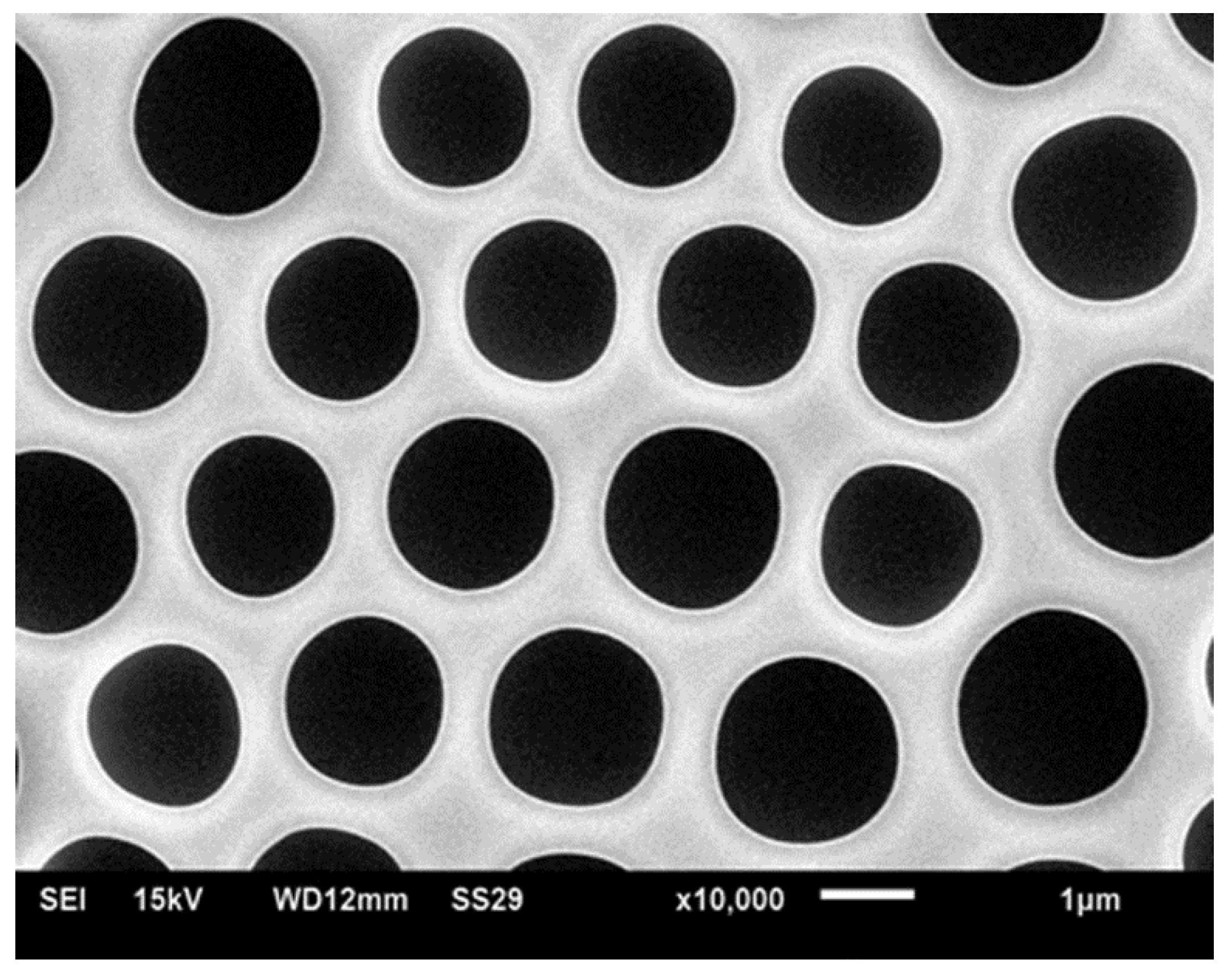
7. Characterization of Patterns Obtained with Breath-Figure Self-Assembly
7.1. Characterization of the Ordering of Patterns
7.2. Surface Characterization of Patterns Obtained with Breath-Figure Self-Assembly
8. Novel Applications of Breath-Figure Self-Assembly
9. Breath-Figure Self-Assembly and Manufacturing of Membranes
10. Conclusions
Acknowledgments
Conflicts of Interest
References
- Gugliuzza, A.; Aceto, M.C.; Macedonio, F.; Drioli, E. Water droplets as template for next generation self-assembled poly-(etheretherketone) with Cardo membranes. J. Phys. Chem. B 2008, 112, 10483–10496. [Google Scholar] [CrossRef] [PubMed]
- Ulbricht, M. Advanced functional polymer membranes. Polymer 2006, 47, 2217–2262. [Google Scholar] [CrossRef]
- Schechter, A.; Savinell, R.F. Imidazole and 1-methyl imidazole in phosphoric acid doped polybenzimidazole, electrolyte for fuel cells. Solid State Ionics 2002, 147, 181–187. [Google Scholar] [CrossRef]
- Gugliuzza, A.; Perrotta, M.L.; Drioli, E. Controlled bulk properties of composite polymeric solutions for extensive structural order of honeycomb polysulfone membranes. Membranes 2016, 6, 27. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.-S.; Swager, T.M. Fluorescent porous polymer films as TNT chemosensors: Electronic and structural effects. J. Am. Chem. Soc. 1998, 120, 11864–11873. [Google Scholar] [CrossRef]
- Li, Y.T.; Cunin, F.; Link, J.R.; Gao, T.; Betts, R.T.; Reiver, S.H.; Chin, V. Polymer replicas of photonic porous silicon for sensing and drug delivery applications. Science 2003, 299, 2045–2047. [Google Scholar] [CrossRef] [PubMed]
- Rezwan, R.; Chen, Q.Z.; Blaker, J.J.; Boccaccini, A.R. Biodegradable and bioactive porous polymer/inorganic composite scaffolds for bone tissue engineering. Biomaterials 2006, 27, 3413–3431. [Google Scholar] [CrossRef] [PubMed]
- Lin, A.S.P.; Barrows, T.H.; Cartmell, S.H.; Guldberg, R.E. Microarchitectural and mechanical characterization of oriented porous polymer scaffolds. Biomaterials 2003, 24, 481–489. [Google Scholar] [CrossRef]
- Li, H.; Zhao, X.; Wu, P.; Zhang, S.; Geng, B. Facile preparation of superhydrophobic and superoleophilic porous polymer membranes for oil/water separation from a polyarylester polydimethylsiloxane block copolymer. J. Mater. Sci. 2016, 51, 3211–3218. [Google Scholar] [CrossRef]
- Cheng, B.; Lib, Z.; Li, Q.; Ju, J.; Kang, W.; Naebe, M. Development of smart poly(vinylidene fluoride)-graft-poly(acrylic acid) tree-like nanofiber membrane for pH-responsive oil/water separation. J. Membr. Sci. 2017, 534, 1–8. [Google Scholar] [CrossRef]
- Bormashenko, E.; Balter, S.; Bormashenko, Y.; Aurbach, D. Honeycomb structures obtained with breath figures self-assembly allow water/oil separation. Colloids Surf. A 2012, 415, 394–398. [Google Scholar] [CrossRef]
- Liu, C.; Yang, J.; Tang, Y.; Yin, L.; Tang, H.; Li, C. Versatile fabrication of the magnetic polymer-based graphene foam and applications for oil–water separation. Colloids Surf. A 2015, 468, 10–16. [Google Scholar] [CrossRef]
- Wan, L.-S.; Li, J.W.; Ke, B.-B.; Xu, Z.-K. Ordered microporous membranes templated by breath figures for size-selective separation. J. Am. Chem. Soc. 2012, 134, 95–98. [Google Scholar] [CrossRef] [PubMed]
- Muñoz-Bonilla, A.; Fernández-García, M.; Hernández, J.R. Towards hierarchically ordered functional porous polymeric surfaces prepared by the breath figures approach. Prog. Polym. Sci. 2014, 39, 510–554. [Google Scholar]
- Bunz, U.H.F. Breath figures as a dynamic templating method for polymers and nanomaterials. Adv. Mater. 2006, 18, 973–989. [Google Scholar] [CrossRef]
- Zhang, A.; Bai, H.; Li, L. Breath Figure: A Nature-inspired preparation method for ordered porous films. Chem. Rev. 2015, 115, 9801–9868. [Google Scholar] [CrossRef] [PubMed]
- Aitken, J. Breath figures. Nature 1911, 86, 516–617. [Google Scholar] [CrossRef]
- Rayleigh, L. Breath figures. Nature 1911, 86, 416–417. [Google Scholar] [CrossRef]
- Rayleigh, L. Breath figures. Nature 1912, 90, 436–438. [Google Scholar] [CrossRef]
- Briscoe, B.; Galvin, K. The effect of surface fog on the transmittance of light. J. Sol. Energy 1991, 46, 191–197. [Google Scholar] [CrossRef]
- Beysens, D. The formation of dew. Atmos. Res. 1995, 39, 215–237. [Google Scholar] [CrossRef]
- Beysens, D.; Knobler, C.M. Growth of breath figures. Phys. Rev. Lett. 1986, 57, 1433–1436. [Google Scholar] [CrossRef] [PubMed]
- Marcos-Martin, M.; Beysens, D.; Bouchaud, J.P.; Godrèche, C.; Yekutieli, I. Self-diffusion and ‘visited’ surface in the droplet condensation problem (breath figures). Phys. A 1995, 214, 396–412. [Google Scholar] [CrossRef]
- Knobler, C.M.; Beysens, D. Growth of breath figures on fluid surfaces. Europhys. Lett. 1988, 6, 707. [Google Scholar] [CrossRef]
- Steyer, A.; Guenoun, P.; Beysens, D.; Knobler, C.M. Two-dimensional ordering during droplet growth on a liquid surface. Phys. Rev. B 1990, 42, 1086–1089. [Google Scholar] [CrossRef]
- Widawski, G.; Rawiso, B.; Francois, B. Self-organized honeycomb morphology of star-polymer polystyrene films. Nature 1994, 369, 387–389. [Google Scholar] [CrossRef]
- Francois, B.; Pitois, O.; Francois, J. Polymer films with a self- organized honeycomb morphology. Adv. Mater. 1995, 7, 1041–1044. [Google Scholar] [CrossRef]
- Pitois, O.; Francois, B. Formation of ordered micro-porous membranes. Eur. Phys. J. B 1999, 8, 225–231. [Google Scholar] [CrossRef]
- Pitois, O.; François, B. Crystallization of condensation droplets on a liquid surface. Colloid Polym. Sci. 1999, 277, 574–578. [Google Scholar] [CrossRef]
- François, B.; Ederlé, Y.; Mathis, C. Honeycomb membranes made from C60(PS)6. Synth. Met. 1999, 103, 2362–2363. [Google Scholar] [CrossRef]
- Adamson, A.W.; Gast, A.P. Physical Chemistry of Surfaces, 6th ed.; Wiley-Interscience Publishers: New York, NY, USA, 1990. [Google Scholar]
- Erbil, H.Y. Surface Chemistry of Solid and Liquid Interfaces; Blackwell: Oxford, UK, 2006. [Google Scholar]
- De Gennes, P.-G.; Brochard-Wyart, F.; Quéré, D. Capillarity and Wetting Phenomena; Springer: Berlin, Germany, 2003. [Google Scholar]
- Bormashenko, E. Wetting of Real Surfaces; De Gruyter: Berlin, Germany, 2013. [Google Scholar]
- Srinivasarao, M.; Collings, D.; Philips, A.; Patel, S. Three-dimensionally ordered array of air bubbles in a polymer film. Science 2001, 292, 79–83. [Google Scholar] [CrossRef] [PubMed]
- Song, L.; Bly, R.K.; Wilson, J.N.; Bakbak, S.; Park, J.O.; Srinivasarao, N.; Bunz, U.H.F. Facile microstructuring of organic semiconducting polymers by the breath figure method: Hexagonally ordered bubble arrays in rigid rod-polymers. Adv. Mater. 2004, 16, 115–118. [Google Scholar] [CrossRef]
- Bolognesi, A.; Mercogliano, C.; Yunus, S.; Civardi, M.; Comoretto, D.; Turturro, A. Self-organization of polystyrenes into ordered microstructured films and their replication by soft lithography. Langmuir 2005, 21, 3480–3485. [Google Scholar] [CrossRef] [PubMed]
- Zhu, L.-W.; Ou, Y.; Wan, L.-S.; Xu, Z.K. Polystyrenes with hydrophilic end groups: Synthesis, characterization, and effects on the self-assembly of breath figure arrays. J. Phys. Chem. B 2014, 118, 845–854. [Google Scholar] [CrossRef] [PubMed]
- Amirkhani, M.; Berger, N.; Abdelmohsen, M.; Zocholl, F.; Gonçalves, M.R.; Marti, O. The effect of different stabilizers on the formation of self-assembled porous film via the breath-figure technique. J. Polym. Sci. B 2011, 49, 1430–1436. [Google Scholar] [CrossRef]
- Peng, J.; Han, Y.; Yang, Y.; Li, B. The influencing factors on the macroporous formation in polymer films by water droplet templating. Polymer 2004, 45, 447–452. [Google Scholar] [CrossRef]
- Ferrari, E.; Fabbri, P.; Pilati, F. Solvent and substrate contributions to the formation of breath figure patterns in polystyrene films. Langmuir 2011, 27, 1874–1881. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.; Gao, C.; Yan, D. Honeycomb-patterned photoluminescent films fabricated by self-assembly of hyperbranched polymers. Angew. Chem. 2007, 46, 4128–4131. [Google Scholar] [CrossRef] [PubMed]
- Jenekhe, S.A.; Chen, X.L. Self-Assembly of ordered microporous materials from rod-coil block copolymers. Science 1999, 283, 372–375. [Google Scholar] [CrossRef] [PubMed]
- Matsuyama, H.; Ohga, K.; Maki, T.; Teramoto, M. The Effect of polymer molecular weight on the structure of a honeycomb patterned thin film prepared by solvent evaporation. J. Chem. Eng. Jpn. 2004, 37, 588–591. [Google Scholar] [CrossRef]
- Xu, Y.; Zhu, B.; Xu, Yo. A study on formation of regular honeycomb pattern in polysulfone film. Polymer 2005, 46, 713–717. [Google Scholar] [CrossRef]
- Bormashenko, E.; Pogreb, R.; Stanevsky, O.; Bormashenko, Y.; Gendelman, O. Formation of honeycomb patterns in evaporated polymer solutions: Influence of the molecular weight. Mater. Lett. 2005, 59, 3553–3557. [Google Scholar] [CrossRef]
- Li, Z.; Ma, X.; Kong, Q.; Zang, D.; Guan, X.; Ren, X. Static and dynamic hydrophobic properties of honeycomb structured films via breath figure method. J. Phys. Chem. C 2016, 120, 18659–18664. [Google Scholar] [CrossRef]
- Govor, L.V.; Bashmakov, I.A.; Kiebooms, R.; Dykonov, V.; Parisi, J. Self-organized networks based on conjugated polymers. Adv. Mater. 2001, 13, 588–591. [Google Scholar] [CrossRef]
- Deepak, V.D.; Asha, S.K. Random and AB diblock copolymers of tricyclodecanemethanol urethane methacrylate with styrene: Synthesis and morphology characterization. J. Polym. Sci. A 2008, 46, 1278–1288. [Google Scholar] [CrossRef]
- Erdogan, B.; Song, L.; Wilson, J.N.; Park, J.O.; Srinivasarao, M.; Bunz, U.H.F. Permanent bubble arrays from a cross-linked poly(para-phenyleneethynylene): Picoliter holes without microfabrication. J. Am. Chem. Soc. 2004, 126, 3678–3679. [Google Scholar] [CrossRef] [PubMed]
- Karikari, A.S.; Williams, A.R.; Heisey, C.L.; Rawlett, A.M.; Lon, T.E. Porous thin films based on photo-cross-linked star-shaped Poly(d,l-lactide)s. Langmuir 2006, 22, 9687–9693. [Google Scholar] [CrossRef] [PubMed]
- Zhu, L.-W.; Yang, W.; Wan, L.-S.; Xu, Z.-K. Synthesis of core cross-linked star polystyrene with functional end groups and self-assemblies templated by breath figures. Polym. Chem. 2014, 5, 5175–5182. [Google Scholar] [CrossRef]
- Yabu, H.; Tanaka, M.; Ijiro, K.; Shimomura, M. Preparation of honeycomb-patterned polyimide films by self-organization. Langmuir 2003, 19, 6297–6300. [Google Scholar] [CrossRef]
- Bormashenko, E.; Schechter, A.; Stanevsky, O.; Stein, T.; Balter, S.; Musin, A.; Bormashenko, Y.; Pogreb, R.; Barkay, Z.; Aurbach, D. Free-standing, thermostable, micrometer-scale honeycomb polymer films and their properties. Macromol. Mater. Eng. 2008, 293, 872–877. [Google Scholar] [CrossRef]
- Bormashenko, E.; Balter, S.; Malkin, A.; Aurbach, D. Polysulfone membranes demonstrating asymmetric diode-like water permeability and their applications. Macromol. Mater. Eng. 2014, 299, 27–30. [Google Scholar] [CrossRef]
- Gong, J.; Xu, B.; Tao, X. Breath figure micromolding approach for regulating the microstructures of polymeric films for triboelectric nanogenerators. ACS Appl. Mater. Interfaces 2017, 9, 4988–4997. [Google Scholar] [CrossRef] [PubMed]
- Sharma, V.; Song, L.; Jones, R.L.; Barrow, M.S.; Williams, P.R.; Srinivasarao, M. Effect of solvent choice on breath-figure-templated assembly of “holey” polymer films. Europhys. Lett. 2010, 91, 38001. [Google Scholar] [CrossRef]
- Bormashenko, E.; Pogreb, R.; Stanevsky, O.; Bormashenko, Y.; Tamir, S.; Cohen, R.; Nunberg, M.; Gaisin, V.-Z.; Gorelik, M.; Gendelman, O. Mesoscopic and submicroscopic patterning in thin polymer films: Impact of the solvent. Mater. Lett. 2005, 59, 2461–2464. [Google Scholar] [CrossRef]
- Battenbo, H.; Cobley, R.J.; Wilks, S.P. A quantitative study of the formation of breath figure templated polymer materials. Soft Matter 2011, 7, 10864–10873. [Google Scholar] [CrossRef]
- Karthaus, O.; Maruyama, N.; Cieren, X.; Shimomura, M.; Hasegawa, H.; Hashimoto, T. Water-assisted formation of micrometer-size honeycomb patterns of polymers. Langmuir 2000, 16, 6071–6076. [Google Scholar] [CrossRef]
- Bormashenko, E.; Balter, S.; Aurbach, D. On the Nature of the breath figures self-assembly in evaporated polymer solutions: Revisiting physical factors governing the patterning. Macromol. Chem. Phys. 2012, 213, 1742–1747. [Google Scholar] [CrossRef]
- Bormashenko, E.; Malkin, A.; Musin, A.; Bormashenko, Y.; Whyman, G.; Litvak, N.; Barkay, Z.; Machavariani, V. Mesoscopic patterning in evaporated polymer solutions: Poly(ethylene glycol) and room-temperature-vulcanized polyorganosilanes/-siloxanes promote formation of honeycomb structures. Macromol. Chem. Phys. 2008, 209, 567–576. [Google Scholar] [CrossRef]
- Bormashenko, E. Correct values of Rayleigh and Marangoni numbers for liquid layers deposited on thin substrates. Ind. Eng. Chem. Res. 2008, 47, 1726–1728. [Google Scholar] [CrossRef]
- Hernández-Guerrero, M.; Stenzel, M.H. Honeycomb structured polymer films via breath figures. Polym. Chem. 2012, 3, 563–577. [Google Scholar] [CrossRef]
- Nurmawati, B.M.H.; Vetrichelvan, M.; Valiyaveettil, S. Morphological investigations of self-assembled films from a pyridine -incorporated poly (p-phenylene). J. Porous Mater. 2006, 13, 315–317. [Google Scholar]
- Maruyama, N.; Koito, T.; Nishida, J.; Sawadaishi, T.; Cieren, X.; Ijiro, K.; Karthaus, O.; Shimomura, M. Mesoscopic patterns of molecular aggregates on solid substrates. Thin Solid Films 1998, 327, 854–856. [Google Scholar] [CrossRef]
- Stenzel, M.H.; Barner-Kowollik, C.; Davis, T.P. Formation of honeycomb-structured, porous films via breath figures with different polymer architectures. J. Polym. Sci. A 2006, 44, 2363–2375. [Google Scholar] [CrossRef]
- Kabuto, T.; Hashimoto, Y.; Karthaus, O. Thermally stable and solvent resistant mesoporous honeycomb films from a crosslinkable polymer. Adv. Funct. Mater. 2007, 17, 3569–3573. [Google Scholar] [CrossRef]
- Sun, W.; Ji, J.; Shen, J. Rings of nanoparticle-decorated honeycomb-structured polymeric film: The Combination of Pickering emulsions and capillary flow in the breath figures method. Langmuir 2008, 24, 11338–11341. [Google Scholar] [CrossRef] [PubMed]
- Lakshmi, V.; Raju, A.; Resmi, V.G.; Pancrecious, J.K.; Rajan, T.P.D.; Pavithran, C. Amino-functionalized breath-figure cavities in polystyrene-alumina hybrid films: Effect of particle concentration and dispersion. Phys. Chem. Chem. Phys. 2016, 18, 7367–7373. [Google Scholar]
- Wang, L.-P.; Yin, K.-Y.; Li, G.; Liu, Q.; Deng, A.-X.; Ma, H.-Y. Rhodamine B-loaded star polystyrenes and their luminescent honeycomb-patterned porous films. React. Funct. Polym. 2016, 99, 59–64. [Google Scholar] [CrossRef]
- Madej, W.; Budkowski, A.; Raczkowska, J.; Rysz, J. Breath figures in polymer and polymer blend films spin-coated in dry and humid ambience. Langmuir 2008, 24, 3517–3524. [Google Scholar] [CrossRef] [PubMed]
- Park, M.S.; Kim, J.K. Breath figure patterns prepared by spin coating in a dry environment. Langmuir 2004, 20, 5347–5352. [Google Scholar] [CrossRef] [PubMed]
- Park, M.S.; Kim, J.K. Broad-band antireflection coating at near-Infrared wavelengths by a breath figure. Langmuir 2005, 21, 11404–11408. [Google Scholar] [CrossRef] [PubMed]
- Park, M.S.; Joo, W.; Kim, J.K. Porous structures of polymer films prepared by spin coating with mixed solvents under humid condition. Langmuir 2006, 22, 4594–4598. [Google Scholar] [CrossRef] [PubMed]
- Muñoz-Bonilla, A.; Ibarboure, E.; Papon, E.; Rodriguez-Hernandez, J. Self-organized hierarchical structures in polymer surfaces: Self-assembled nanostructures within breath figures. Langmuir 2009, 25, 6493–6499. [Google Scholar] [CrossRef] [PubMed]
- Arora, J.S.; Cremaldi, J.C.; Hollerana, M.K.; Ponnusamya, T.; Sunkaraa, B.; He, J.; Pesika, N.S.; John, V.T. Hierarchical patterning of hydrogels by replica molding of impregnated breath figures leads to superoleophobicity. Nanoscale 2016, 8, 18446–18453. [Google Scholar] [CrossRef] [PubMed]
- Galeotti, F.; Trespidi, F.; Pasini, M. Breath figure-assisted fabrication of nanostructured coating on silicon surface and evaluation of its antireflection power. J. Nanomater. 2016, 2016, 1–8. [Google Scholar] [CrossRef]
- Yabu, H.; Shimomura, M. Single-step fabrication of transparent superhydrophobic porous polymer films. Chem. Mater. 2005, 17, 5231–5234. [Google Scholar] [CrossRef]
- Yang, H.; Jiang, P. Self-cleaning diffractive macroporous films by doctor blade coating. Langmuir 2010, 26, 12598–12604. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.; Jiang, P. Large-scale colloidal self-assembly by doctor blade coating. Langmuir 2010, 26, 13173–13182. [Google Scholar] [CrossRef] [PubMed]
- Mansouri, J.; Yapit, E.; Chen, V. Polysulfone filtration membranes with isoporous structures prepared by a combination of dip-coating and breath figure approach. J. Membr. Sci. 2013, 444, 237–251. [Google Scholar] [CrossRef]
- Van-Tien Bui, V.-T.; Thuy, L.T.; Tran, Q.C.; Nguyen, V.-T.; Dao, V.-D.; Choi, J.S.; Choi, H.-S. Ordered honeycomb biocompatible polymer films via a one-step solution-immersion phase separation used as a scaffold for cell cultures. Chem. Eng. J. 2017, 320, 561–569. [Google Scholar]
- Bera, S.; Pal, M.; Sarkar, S.; Jana, S. Hierarchically structured macro with nested mesoporous zinc Indium Oxide conducting film. ACS Appl. Mater. Interfaces 2017, 9, 4420–4424. [Google Scholar] [CrossRef] [PubMed]
- Schmelzer, J.W.P.; Schmelzer, J., Jr. Reconciling Gibbs and van der Waals: A new approach to nucleation theory. J. Chem. Phys. 2000, 112, 3820–3831. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Course of Theoretical Physics Vol 5: Statistical Physics, 2nd ed.; Pergamon Press: Oxford, UK, 1969. [Google Scholar]
- Saunders, A.T.; Dickson, J.L.; Shah, P.S.; Lee, M.Y.; Lim, R.T.; Johnston, K.P.; Korgel, B.A. Breath figure templated self-assembly of porous diblock copolymer films. Phys. Rev. E 2006, 73, 031608. [Google Scholar] [CrossRef] [PubMed]
- Adamson, A.W.; Gast, A.P. Physical Chemistry of Surfaces, 6th ed.; Wiley: New York, NY, USA, 1997. [Google Scholar]
- Lothe, J.; Pound, G.M. Reconsiderations of nucleation theory. J. Chem. Phys. 1962, 36, 2080–2085. [Google Scholar] [CrossRef]
- Binder, R.; Stauffer, D. Statistical theory of nucleation, condensation and coagulation. Adv. Phys. 1976, 25, 343–396. [Google Scholar] [CrossRef]
- Zeldovich, Y.B. On the theory of formation of new phase. Cavitation. J. Exp. Theor. Phys. USSR 1942, 12, 525–538. (In Russian) [Google Scholar]
- Sigsbee, R.A. Vapor to condensed-phase heterogeneous nucleation. In Nucleation; Zettlemoyer, A.C., Ed.; Marcel Decker: New York, NY, USA, 1969; pp. 151–224. [Google Scholar]
- Böker, A.; Lin, Y.; Chiapperini, K.; Horowitz, R.; Thompson, N.; Carreon, V.; Xu, T.; Abetz, C.; Skaff, H.; Dinsmore, A.D.; et al. Hierarchical nanoparticle assemblies formed by decorating breath figures. Nat. Mater. 2004, 3, 302–306. [Google Scholar]
- Escalé, P.; Rubatat, L.; Billon, L.; Save, M. Recent advances in honeycomb-structured porous polymer films prepared via breath figures. Eur. Polym. J. 2012, 48, 1001–1025. [Google Scholar]
- Bormashenko, E.; Pogreb, R.; Musin, A.; Stanevsky, O.; Bormashenko, Y.; Whyman, G.; Gendelman, O.; Barkay, Z. Self-assembly in evaporated polymer solutions: Influence of the solution concentration. J. Colloid Interface Sci. 2006, 297, 534–540. [Google Scholar] [CrossRef] [PubMed]
- Bormashenko, E.; Pogreb, R.; Stanevsky, O.; Bormashenko, Y.; Stein, T.; Gengelman, O. Mesoscopic patterning in evaporated polymer solutions: New experimental data and physical Mechanisms. Langmuir 2005, 21, 9604–9609. [Google Scholar] [CrossRef] [PubMed]
- Bormashenko, E.; Whyman, G.; Pogreb, R.; Stanevsky, O.; Hakham-Itzhaq, M.; Gendelman, O. Self-assembly in evaporated polymer solutions: Patterning on two scales. Isr. J. Chem. 2007, 47, 319–328. [Google Scholar] [CrossRef]
- Nie1, Z.; Kumacheva, E. Patterning surfaces with functional polymers. Nat. Mater. 2008, 7, 277–290. [Google Scholar] [CrossRef] [PubMed]
- Tokuhisa, H.; Tsukamoto, S.; Morita, S.; Ise, S.; Tomita, M.; Shirakawa, N. Fabrication of micro-textured surfaces for a high hydrophobicity by evaporative patterning using screen mesh templates. Appl. Surf. Sci. 2017, 400, 64–70. [Google Scholar] [CrossRef]
- Pototsky, A.; Bestehorn, M.; Merkt, D.; Thiele, U. Morphology changes in the evolution of liquid two-layer films. J. Chem. Phys. 2005, 122, 224711. [Google Scholar] [CrossRef] [PubMed]
- Pototsky, A.; Bestehorn, M.; Merkt, D.; Thiele, U. Alternative pathways of dewetting for a thin liquid two-layer film. Phys. Rev. E 2004, 70, 025201(R). [Google Scholar] [CrossRef] [PubMed]
- Müller-Buschbaum, P.; Bauer, E.; Wunnicke, O.; Stamm, M. The control of thin film morphology by the interplay of dewetting, phase separation and microphase separation. J. Phys. Condens. Matter 2005, 17, S363–S386. [Google Scholar] [CrossRef]
- Colinet, P.; Legros, J.C.; Velarde, M.G. Nonlinear Dynamics of Surface-Tension-Driven Instabilities; Wiley: Berlin, Germany, 2001. [Google Scholar]
- Nepomnyashchy, A.A.; Velarde, M.G.; Colinet, P. Interfacial Phenomena and Convection; Chapman & Hall/CRC: Boca Raton, FL, USA, 2002. [Google Scholar]
- Reichenbach, J.; Linde, H. Linear perturbation analysis of surface-tension-driven convection at a plane interface (Marangoni instability). J. Colloid Interface Sci. 1981, 84, 433–443. [Google Scholar] [CrossRef]
- Linde, H.; Velarde, M.G.; Wierschem, A.; Waldhelm, W.; Loeschke, K.; Rednikov, A.Y. Interfacial wave motions due to Marangoni instability. J. Colloid Interface Sci. 1997, 188, 16–26. [Google Scholar] [CrossRef]
- Oron, A.; Nepomnyashchy, A.A. Long-wavelength thermocapillary instability with the Soret effect. Phys. Rev. E 2004, 69, 016313. [Google Scholar] [CrossRef] [PubMed]
- Regnier, V.C.; Dauby, P.C.; Lebon, G. Linear and nonlinear Rayleigh-Bénard-Marangoni instability with surface deformations. Phys. Fluids 2000, 12, 2787–2799. [Google Scholar] [CrossRef]
- Zhang, N.; Chao, D.F. Mechanisms of convection instability in thin liquid layers induced by evaporation. Int. Commun. Heat Mass Transf. 1999, 26, 1069–1080. [Google Scholar] [CrossRef]
- Münch, A.; Please, C.P.; Wagner, B. Spin coating of an evaporating polymer solution. Phys. Fluids 2011, 23, 102101. [Google Scholar] [CrossRef]
- Mitov, Z.; Kumacheva, E. Convection-induced patterns in phase-separating polymeric fluids. Phys. Rev. Lett. 1998, 81, 3427. [Google Scholar] [CrossRef]
- Minařík, A.; Smolka, P.; Minařík, M.; Mráček, A.; Rajnohová, E.; Minaříková, M.; Gřundělová, L.; Foglarová, M.; Velebný, V. A special instrument for the defined modification of polymer properties in solutions and polymer layers. Measurement 2017, 97, 218–225. [Google Scholar] [CrossRef]
- Wrzecionko, E.; Minařík, A.; Smolka, P.; Minařík, M.; Humpolíček, P.; Rejmontová, P.; Mráček, A.; Minaříková, M.; Gřundělová, L. Variations of polymer porous surface structures via the time-sequenced dosing of mixed solvents. ACS Appl. Mater. Interfaces 2017, 9, 6472–6481. [Google Scholar] [CrossRef] [PubMed]
- Fowler, P.D.; Ruscher, C.; McGraw, J.D.; Forrest, J.A.; Dalnoki-Veress, K. Controlling Marangoni-induced instabilities in spin-cast polymer films: How to prepare uniform films. Eur. Phys. J. E 2016, 39, 90. [Google Scholar] [CrossRef] [PubMed]
- Bormashenko, E.; Balter, S.; Pogreb, R.; Bormashenko, Y.; Gendelman, O.; Aurbach, D. On the mechanism of patterning in rapidly evaporated polymer solutions: Is temperature-gradient-driven Marangoni instability responsible for the large-scale patterning? J. Colloid Interface Sci. 2010, 343, 602–607. [Google Scholar] [CrossRef] [PubMed]
- Grigoriev, R. Control of evaporatively driven instabilities of thin liquid films. Phys. Fluids 2002, 14, 1895. [Google Scholar] [CrossRef]
- De Gennes, P.G. Instabilities during the evaporation of a film: Non-glassy polymer + volatile solvent. Eur. Phys. J. E 2001, 6, 421–424. [Google Scholar] [CrossRef]
- De Gennes, P.G. Solvent evaporation of spin cast films: “Crust” effects. Eur. Phys. J. E 2002, 7, 31–34. [Google Scholar] [CrossRef]
- Bormashenko, E. Surface instabilities and patterning at liquid/vapor interfaces: Exemplifications of the “hairy ball theorem”. Colloid Interface Sci. Commun. 2015, 5, 5–7. [Google Scholar] [CrossRef]
- Eisenberg, M.; Guy, R. A proof of the hairy ball theorem. Am. Math. Mon. 1979, 86, 571–574. [Google Scholar] [CrossRef]
- Bormashenko, E.; Aurbach, D.; Whyman, G.; Stein, T.; Bormashenko, Y.; Pogreb, R. On the role of the Plateau borders in the pattern formation occurring in thin evaporated polymer layers. Colloid Surf. A 2008, 312, 245–248. [Google Scholar] [CrossRef]
- Park, S.H.; Xia, Y. Macroporous membranes with highly ordered and three-dimensionally interconnected spherical pores. Adv. Mater. 1998, 10, 1045–1048. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, C. Micropatterning of proteins on 3D porous polymer film fabricated by using the breath-figure method. Adv. Mater. 2007, 19, 913–916. [Google Scholar] [CrossRef]
- Dong, R.; Yan, J.; Ma, H.; Fang, Y.; Hao, J. Dimensional architecture of Ferrocenyl-based oligomer honeycomb-patterned Films: From monolayer to multilayer. Langmuir 2011, 20, 9052–9056. [Google Scholar] [CrossRef] [PubMed]
- Kralchevsky, P.A.; Danov, K.D.; Denkov, N.D. Chemical physics of colloid systems and interfaces. In Surface and Colloidal Chemistry; Birdi, K.S., Ed.; CRC Press: Boca Raton, FL, USA, 2003; Chapter 5. [Google Scholar]
- Kralchevsky, P.A.; Nagayama, K. Capillary forces between colloidal particles. Langmuir 1994, 10, 23–36. [Google Scholar] [CrossRef]
- Kralchevsky, P.A.; Nagayama, K. Capillary interactions between particles bound to interfaces, liquid films and biomembranes. Adv. Colloid Interface Sci. 2000, 85, 145–192. [Google Scholar] [CrossRef]
- Kralchevsky, P.A.; Paunov, V.N.; Ivanov, I.B.; Nagayama, K. Capillary meniscus interactions between colloidal particles attached to a liquid-fluid interface. J. Colloid Interface Sci. 1992, 151, 79–94. [Google Scholar] [CrossRef]
- Bragg, L.; Nye, J.F. A dynamical model of a crystal structure. Proc. R. Soc. Lond. A 1947, 190, 474–481. [Google Scholar] [CrossRef]
- Lomer, W.M. The forces between floating bubbles and a quantitative study of the Bragg “Bubble Model” of a crystal. Math. Proc. Camb. Philos. Soc. 1949, 45, 660–673. [Google Scholar] [CrossRef]
- Peiranski, P. Two-Dimensional interfacial colloidal crystals. Phys. Rev. Lett. 1980, 45, 569–573. [Google Scholar] [CrossRef]
- Dong, R.; Ma, H.; Yan, J.; Fang, Y.; Hao, J. Tunable morphology of 2D honeycomb-patterned films and the hydrophobicity of a Ferrocenyl-based oligomer. Chem. Eur. J. 2011, 17, 7674–7684. [Google Scholar] [CrossRef] [PubMed]
- Dou, Y.; Jin, M.; Zhou, G.; Shui, L. Breath figure method for construction of honeycomb films. Membranes 2015, 5, 399–424. [Google Scholar] [CrossRef] [PubMed]
- Tadmor, R. Line energy and the relation between advancing, receding and Young contact angles. Langmuir 2004, 20, 7659–7664. [Google Scholar] [CrossRef] [PubMed]
- Xia, Y.; Gates, B.; Yin, Y.; Sun, Y. Self-Assembly of Monodispersed Spherical Colloids into Complex Structures. In Surface and Colloid Chemistry, 2nd ed.; Birdy, K.S., Ed.; CRC press: Boca Raton, FL, USA, 2003; pp. 555–579. [Google Scholar]
- Yin, Y.; Xia, Y. Self-assembly of monodispersed colloidal spheres into complex aggregates with well-defined sized, shapes and structures. Adv. Mater. 2001, 13, 267. [Google Scholar] [CrossRef]
- De León, A.S.; Malhotra, S.; Molina, M.; Haag, R.; Calderón, M.; Rodríguez-Hernández, J.; Muñoz-Bonilla, A. Dendritic amphiphiles as additives for honeycomb-like patterned surfaces by breath figures: Role of the molecular characteristics on the pore morphology. J. Colloid Interface Sci. 2015, 440, 263–271. [Google Scholar] [CrossRef] [PubMed]
- Wu, C.-H.; Ting, W.-H.; Lai, Y.-W.; Dai, S.A.; Su, W.-C.; Tung, S.-H.; Jeng, R.J. Tailored honeycomb-like polymeric films based on amphiphilic poly(urea/malonamide) dendrons. RSC Adv. 2016, 6, 91981–91990. [Google Scholar] [CrossRef]
- Fedorets, A.A. On the mechanism of non-coalescence in a drop cluster. JETP Lett. 2005, 81, 437–441. [Google Scholar] [CrossRef]
- Fedorets, A.A.; Dombrovsky, L.A.; Smirnov, A.M. The use of infrared self-emission measurements to retrieve surface temperature of levitating water droplets. Infrared Phys. Technol. 2015, 69, 238–243. [Google Scholar] [CrossRef]
- Fedorets, A.A.; Frenkel, M.; Shulzinger, E.; Dombrovsky, L.A.; Bormashenko, E.; Nosonovsky, M. Self-assembled levitating clusters of water droplets: Pattern formation and stability. Sci. Rep. 2017, 7, 1888. [Google Scholar] [CrossRef] [PubMed]
- Eggers, J.; Lister, J.R.; Stone, H.A. Coalescence of liquid drops. J. Fluid Mech. 1999, 401, 293–310. [Google Scholar] [CrossRef]
- Aarts, D.G.A.L.; Lekkerkerker, H.N.W.; Guo, H.; Wegdam, G.H.; Bonn, D. Hydrodynamics of droplet coalescence. Phys. Rev. Lett. 2005, 95, 164503. [Google Scholar] [CrossRef] [PubMed]
- Karpitschka, S.; Riegler, H. Sharp transition between coalescence and non-coalescence of sessile drops. J. Fluid Mech. 2014, 743, R1. [Google Scholar] [CrossRef]
- Karpitschka, S.; Riegler, H. Quantitative experimental study on the transition between fast and delayed coalescence of sessile droplets with different but completely miscible liquid. Langmuir 2010, 26, 11823–11829. [Google Scholar] [CrossRef] [PubMed]
- Karpitschka, S.; Riegler, H. Noncoalescence of sessile drops from different but miscible liquids: Hydrodynamic analysis of the twin drop contour as a self-stabilizing traveling wave. Phys. Rev. Lett. 2012, 109, 066103. [Google Scholar] [CrossRef] [PubMed]
- Dell’Aversana, P.; Banavar, J.R.; Koplik, J. Suppression of coalescence by shear and temperature gradients. Phys. Fluids 1996, 8, 15–28. [Google Scholar] [CrossRef]
- Neitzel, G.P.; Dell’Aversana, P. Noncoalescence and nonwetting behavior of liquids. Annu. Rev. Fluid Mech. 2002, 34, 267–289. [Google Scholar] [CrossRef]
- Deegan, R.D.; Bakajin, O.; Dupont, T.F.; Huber, G.; Nagel, S.R.; Witten, T.A. Capillary flow as the cause of ring stains from dried liquid drops. Nature 1997, 389, 827–829. [Google Scholar] [CrossRef]
- Deegan, R.D.; Bakajin, O.; Dupont, T.F.; Huber, G.; Nagel, S.R.; Witten, T.A. Contact line deposits in an evaporating drop. Phys. Rev. E 2000, 62, 756–765. [Google Scholar] [CrossRef]
- Hu, H.; Larson, R.G. Marangoni effect reverses coffee-ring depositions. J. Phys. Chem. B 2006, 110, 7090–7094. [Google Scholar] [CrossRef] [PubMed]
- Hu, H.; Larson, R.G. Analysis of the Effects of Marangoni stresses on the microflow in an evaporating sessile droplet. Langmuir 2005, 21, 3972–3980. [Google Scholar] [CrossRef] [PubMed]
- Ma, H.; Hao, J. Evaporation-induced ordered honeycomb structures of gold nanoparticles at the air/water interface. Chem. Eur. J. 2010, 16, 655–660. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Peng, J.; Huang, W.H.; Wu, Y.; Fu, J.; Cong, Y.; Xue, L.J.; Han, Y.C. Ordered honeycomb-structured gold nanoparticle films with changeable pore morphology: From circle to ellipse. Langmuir 2005, 21, 2017–2021. [Google Scholar] [CrossRef] [PubMed]
- Saito, Y.; Shimomura, M.; Yabu, H. Breath figures of nanoscale bricks: A universal method for creating hierarchic porous materials from inorganic nanoparticles stabilized with mussel-inspired copolymers. Macromol. Rapid Commun. 2014, 35, 1763–1769. [Google Scholar] [CrossRef] [PubMed]
- Saunders, A.E.; Shah, P.S.; Sigman, M.B.; Hanrath, T.; Hwang, H.S.; Lim, K.T.; Johnston, K.P.; Korgel, B.A. Inverse opal nanocrystal superlattice films. Nano Lett. 2004, 4, 1943–1948. [Google Scholar] [CrossRef]
- Escalé, P.; Save, M.; Billon, L.; Ruokolainen, J.; Rubatat, L. When block copolymer self-assembly in hierarchically ordered honeycomb films depicts the breath figure process. Soft Matter 2016, 12, 790–797. [Google Scholar] [CrossRef] [PubMed]
- Roe, B.P. Probability and Statistics in Experimental Physics; Springer: New York, NY, USA, 2001. [Google Scholar]
- Kumar, V.S.; Kumaran, V. Voronoi cell volume distribution and configurational entropy of hard-spheres. J. Chem. Phys. 2005, 123, 114501. [Google Scholar] [CrossRef] [PubMed]
- Barthélemy, M. Spatial networks. Phys. Rep. 2011, 499, 1–101. [Google Scholar] [CrossRef]
- Limaye, A.V.; Narhe, R.D.; Dhote, A.M.; Ogale, S.B. Evidence for convective effects in breath figure formation on volatile fluid surfaces. Phys. Rev. Lett. 1996, 76, 3762–3765. [Google Scholar] [CrossRef] [PubMed]
- Bormashenko, E.; Musin, A.; Whyman, G.; Barkay, Z.; Zinigrad, M. Revisiting the fine structure of the triple line. Langmuir 2013, 29, 14163–14167. [Google Scholar] [CrossRef] [PubMed]
- Yamazaki, H.; Ito, K.; Yabu, H.; Shimomura, M. Formation and control of line defects caused by tectonics of water droplet arrays during self-organized honeycomb-patterned polymer film formation. Soft Matter 2014, 10, 2741–2747. [Google Scholar] [CrossRef] [PubMed]
- Marmur, A. A guide to the equilibrium contact angles maze. In Contact Angle Wettability and Adhesion; Mittal, K.L., Ed.; Brill/VSP: Leiden, The Netherlands, 2009; Volume 6, pp. 3–18. [Google Scholar]
- Zhou, Y.; Huang, J.; Sun, W.; Ju, Y.; Yang, P.; Ding, L.; Chen, Z.-R.; Kornfield, J.A. Fabrication of active surfaces with metastable microgel layers formed during breath figure templating. ACS Appl. Mater. Interfaces 2017, 9, 4177–4183. [Google Scholar] [CrossRef] [PubMed]
- Male, U.; Shina, B.K.; Huh, D.S. Coupling of breath figure method with interfacial polymerization: Bottom-surface functionalized honeycomb-patterned porous films. Polymer 2017, 119, 206–211. [Google Scholar] [CrossRef]
- Bormashenko, E.; Bormashenko, Y.; Pogreb, R.; Stanevsky, O. Micrometrically scaled textured metallic hydrophobic interfaces validate the Cassie-Baxter wetting hypothesis. J. Colloid Interface Sci. 2006, 302, 308–311. [Google Scholar] [CrossRef] [PubMed]
- Yabu, H.; Takebayashi, M.; Tanaka, M.; Shimomura, M. Superhydrophobic and lipophobic Properties of self-organized honeycomb and pincushion structures. Langmuir 2005, 21, 3235–3237. [Google Scholar] [CrossRef] [PubMed]
- Cassie, A.B.D.; Baxter, S. Wettability of porous surfaces. Trans. Faraday Soc. 1944, 40, 546–551. [Google Scholar] [CrossRef]
- Cassie, A.B.D. Contact angles. Discuss. Faraday Soc. 1948, 3, 11–16. [Google Scholar] [CrossRef]
- Nosonovsky, N.; Bhushan, B. Superhydrophobic surfaces and emerging applications: Non-adhesion, energy, green engineering. Curr. Opin. Colloid Interface Sci. 2009, 14, 270–280. [Google Scholar] [CrossRef]
- Nosonovsky, N.; Bhushan, B. Biologically inspired surfaces: Broadening the scope of roughness. Adv. Funct. Mater. 2008, 18, 843–855. [Google Scholar] [CrossRef]
- Whyman, G.; Bormashenko, E. How to make the Cassie wetting state stable? Langmuir 2011, 27, 871–8176. [Google Scholar] [CrossRef] [PubMed]
- Arinstein, A.; Burman, M.; Gendelman, O.; Zussman, E. Effect of supramolecular structure on polymer nanofibre elasticity. Nat. Nanotechnol. 2007, 2, 59–62. [Google Scholar] [CrossRef] [PubMed]
- Martínez-Campos, E.; Elzein, T.; Bejjani, A.; García-Granda, M.J.; Santos-Coquillat, A.; Ramos, V.; Muñoz-Bonilla, A.; Rodríguez-Hernández, J. Toward cell selective surfaces: Cell adhesion and proliferation on breath figures with antifouling surface chemistry. ACS Appl. Mater. Interfaces 2016, 8, 6344–6353. [Google Scholar] [CrossRef] [PubMed]
- Kawano, T.; Sato, M.; Yabu, H.; Shimomura, M. Honeycomb-shaped surface topography induces differentiation of human mesenchymal stem cells (hMSCs): Uniform porous polymer scaffolds prepared by the breath figure technique. Biomater. Sci. 2014, 2, 52–56. [Google Scholar] [CrossRef] [PubMed]
- Gong, J.; Xu, B.; Tao, X.; Li, L. Binary breath figures for straightforward and controllable self-assembly of microspherical caps. Phys. Chem. Chem. Phys. 2016, 18, 13629–13637. [Google Scholar] [CrossRef] [PubMed]
- Abbaspour, M.; Pourabbas, B.; Azimi, M. Solid-state supercapacitor based on breath figured polymethyl methacrylate deposited by graphene: The effect of electrode surface. J. Mater. Sci. Mater. Electron. 2017. [Google Scholar] [CrossRef]
- Bormashenko, E.; Pogreb, R.; Balter, S.; Aurbach, D. Electrically controlled membranes exploiting Cassie-Wenzel wetting transitions. Sci. Rep. 2013, 3, 3028. [Google Scholar] [CrossRef] [PubMed]
- Saito, Y.; Yabu, H. Bio-inspired low frictional surfaces having micro-dimple arrays prepared with honeycomb patterned porous films as wet etching masks. Langmuir 2015, 31, 959–963. [Google Scholar] [CrossRef] [PubMed]
- Kamei, J.; Abe, H.; Yabu, H. Biomimetic bubble-repellent tubes: Microdimple arrays enhance repellency of bubbles inside of tubes. Langmuir 2017, 33, 585–590. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.-M.; Lai, J.-H. Recent advances in preparation and morphology control of polymeric membranes formed by nonsolvent induced phase separation. Curr. Opin. Chem. Eng. 2013, 2, 229–237. [Google Scholar] [CrossRef]
- Cong, H.L.; Wang, J.K.; Yu, B.; Tang, J. Preparation of a highly permeable ordered porous microfiltration membrane of brominated poly(phenylene oxide) on an ice substrate by the breath figure method. Soft Matter 2012, 8, 8835–8839. [Google Scholar] [CrossRef]
- Yu, B.; Cong, H.; Li, Z.; Yuan, H.; Peng, Q.; Chi, .; Yang, Sh.; Yang, R.; Wickramasinghe, S.R.; Tang, J. Polymer Sci. B. Fabrication of highly ordered porous membranes of cellulose triacetate on ice substrates using breath figure method. J. Polym. Sci. B 2015, 53, 552–558. [Google Scholar] [CrossRef]
- Sakatani, Y.; Boissière, C.; Grosso, D.; Nicole, L.; Soler-Illia, G.J.A.A.; Sanchez, C. Coupling nanobuilding block and breath figures approaches for the designed construction of hierarchically templated porous materials and membranes. Chem. Mater. 2008, 20, 1049–1056. [Google Scholar] [CrossRef]
- Gugliuzza, A.; Pingitore, V.; Drioli, E. Relationships between structure and electrical sensing of breathable membranes. Mater. Today Proc. 2016, 3, 308–312. [Google Scholar] [CrossRef]
- Tripathi, B.K.; Pande, P. Breath figure templating for fabrication of polysulfone microporous membranes with highly ordered monodispersed porosity. J. Membr. Sci. 2014, 471, 201–210. [Google Scholar] [CrossRef]
- Yuan, H.; Yu, B.; Cong, H.; Peng, Q.; Yang, Z.; Luo, Y.; Chi, M. A highly permeable brominated poly(phenylene oxide) (BPPO) microfiltration membrane with binary porous structures was fabricated by combination of the breath figure and colloidal crystal template methods. J. Colloid Interface Sci. 2016, 461, 232–238. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Zhang, N.; Ni, D.; Sun, K. Preparation of honeycomb porous solid oxide fuel cell cathodes by breath figures method. Int. J. Hydrog. Energy 2011, 36, 7641–7648. [Google Scholar] [CrossRef]
- Li, J.; Zhang, N.; Ni, D.; Sun, K. Preparation of honeycomb porous La0.6Sr0.4Co0.2Fe0.8O3-δGd0.2Ce0.8O2-δ composite cathodes by breath figures method for solid oxide fuel cells. Appl. Surf. Sci. 2011, 258, 50–57. [Google Scholar]
© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bormashenko, E. Breath-Figure Self-Assembly, a Versatile Method of Manufacturing Membranes and Porous Structures: Physical, Chemical and Technological Aspects. Membranes 2017, 7, 45. https://doi.org/10.3390/membranes7030045
Bormashenko E. Breath-Figure Self-Assembly, a Versatile Method of Manufacturing Membranes and Porous Structures: Physical, Chemical and Technological Aspects. Membranes. 2017; 7(3):45. https://doi.org/10.3390/membranes7030045
Chicago/Turabian StyleBormashenko, Edward. 2017. "Breath-Figure Self-Assembly, a Versatile Method of Manufacturing Membranes and Porous Structures: Physical, Chemical and Technological Aspects" Membranes 7, no. 3: 45. https://doi.org/10.3390/membranes7030045
APA StyleBormashenko, E. (2017). Breath-Figure Self-Assembly, a Versatile Method of Manufacturing Membranes and Porous Structures: Physical, Chemical and Technological Aspects. Membranes, 7(3), 45. https://doi.org/10.3390/membranes7030045





