Inertial Memory Effects in Molecular Transport Across Nanoporous Membranes
Abstract
1. Introduction
2. Background
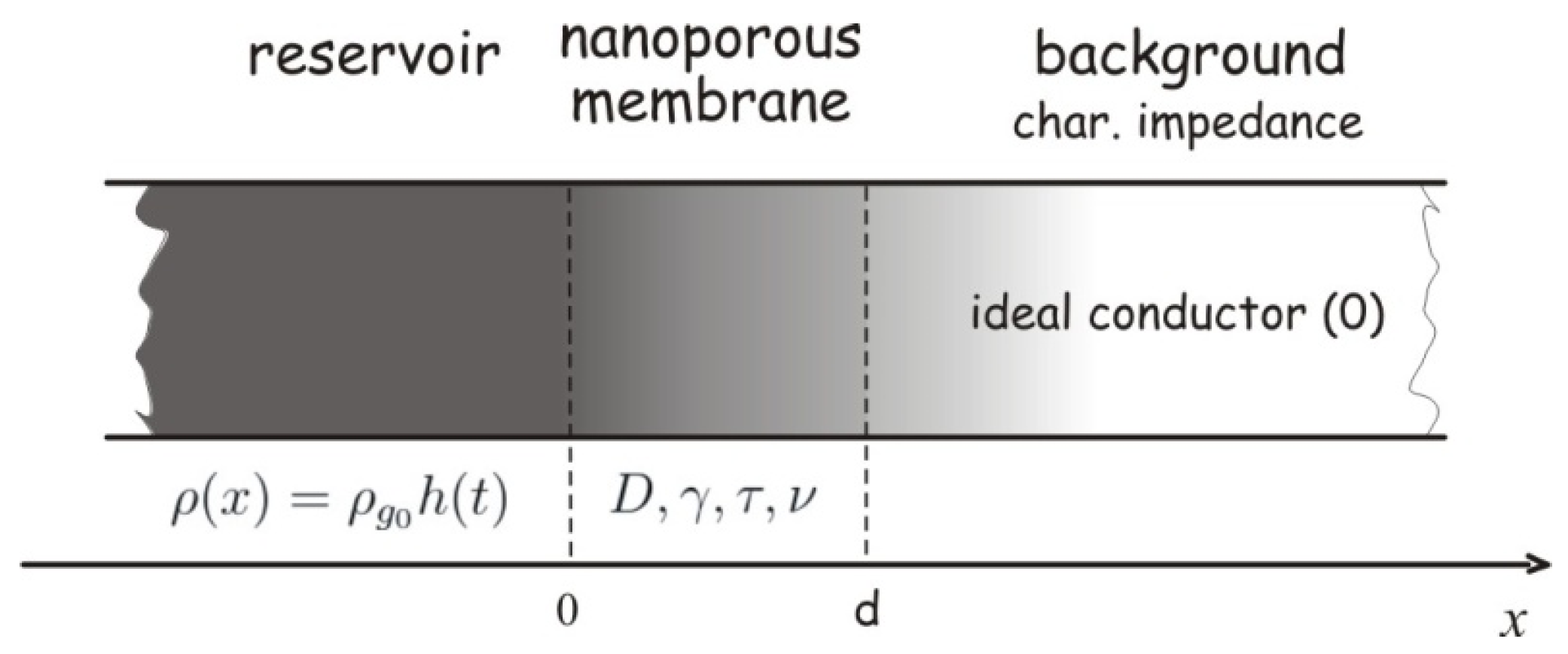
3. Delayed Flux and Inertial Fading Memory Paradigm: Derivation of the Causal Models of Particle Transport
4. Particle Transport Across the Porous Membrane-Spectral Functions
5. Analyses and Discussion
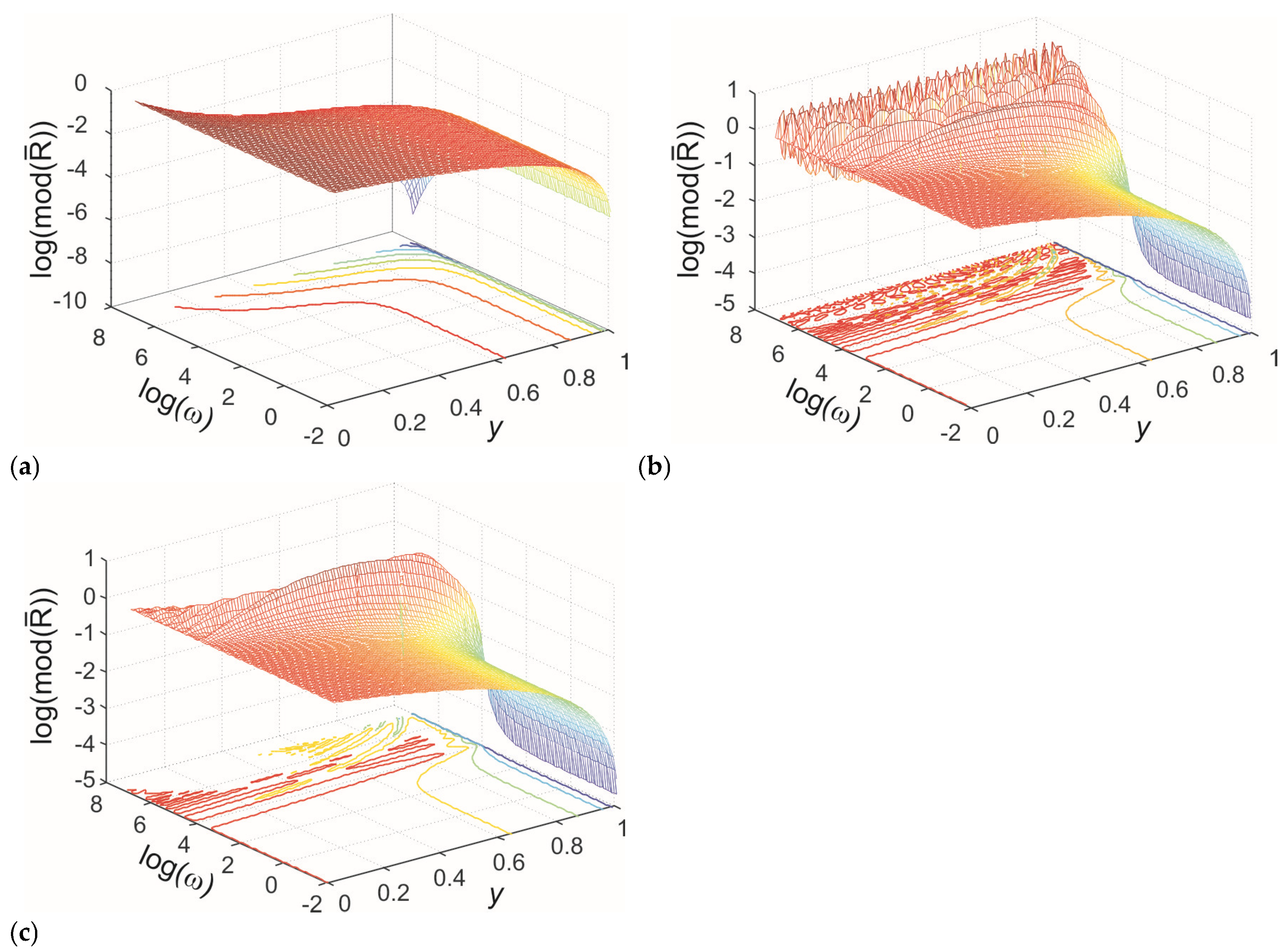
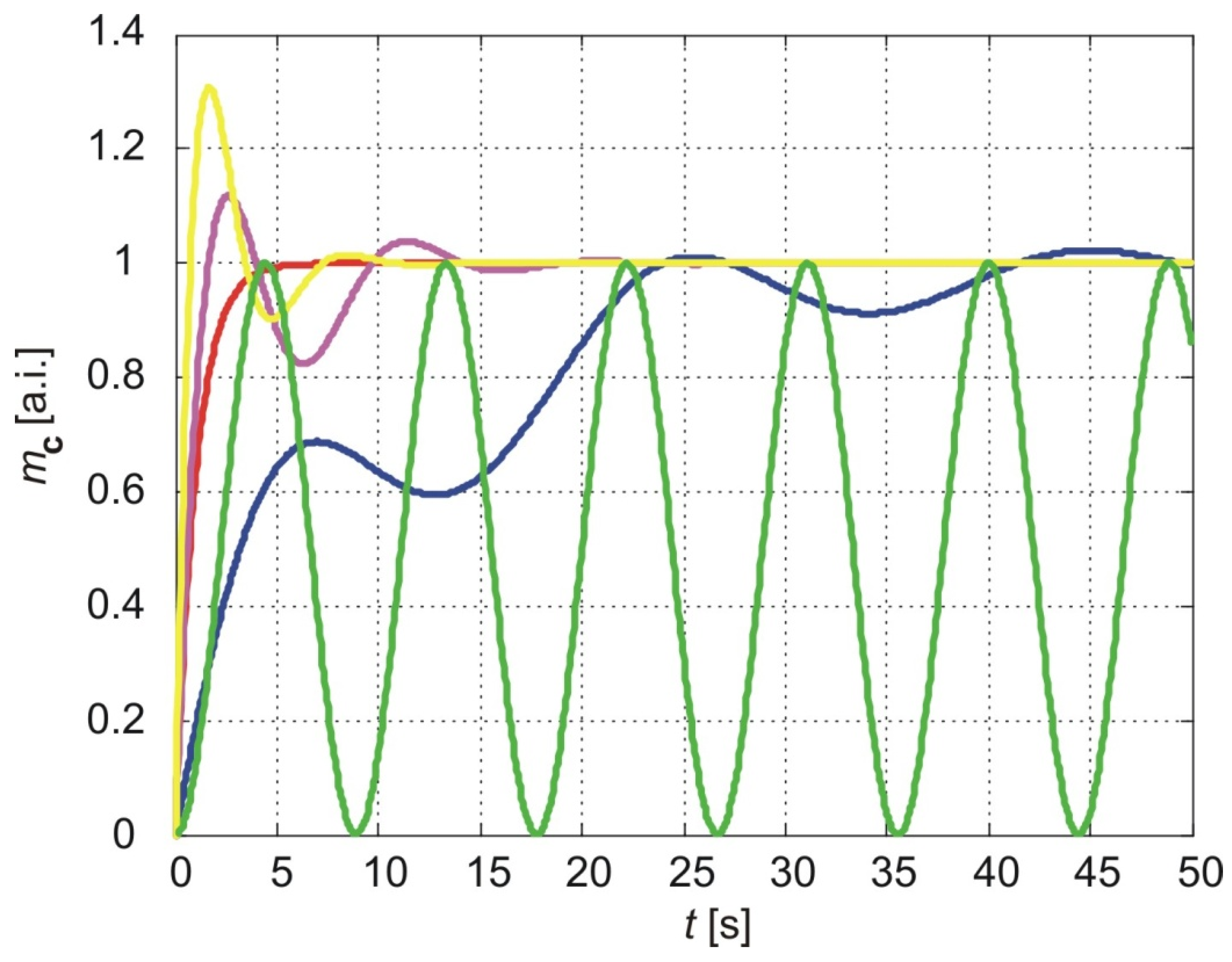
5.1. Inertial Memory Effect on Spectral Function of the Profile of Concentration and Back-Side Flux
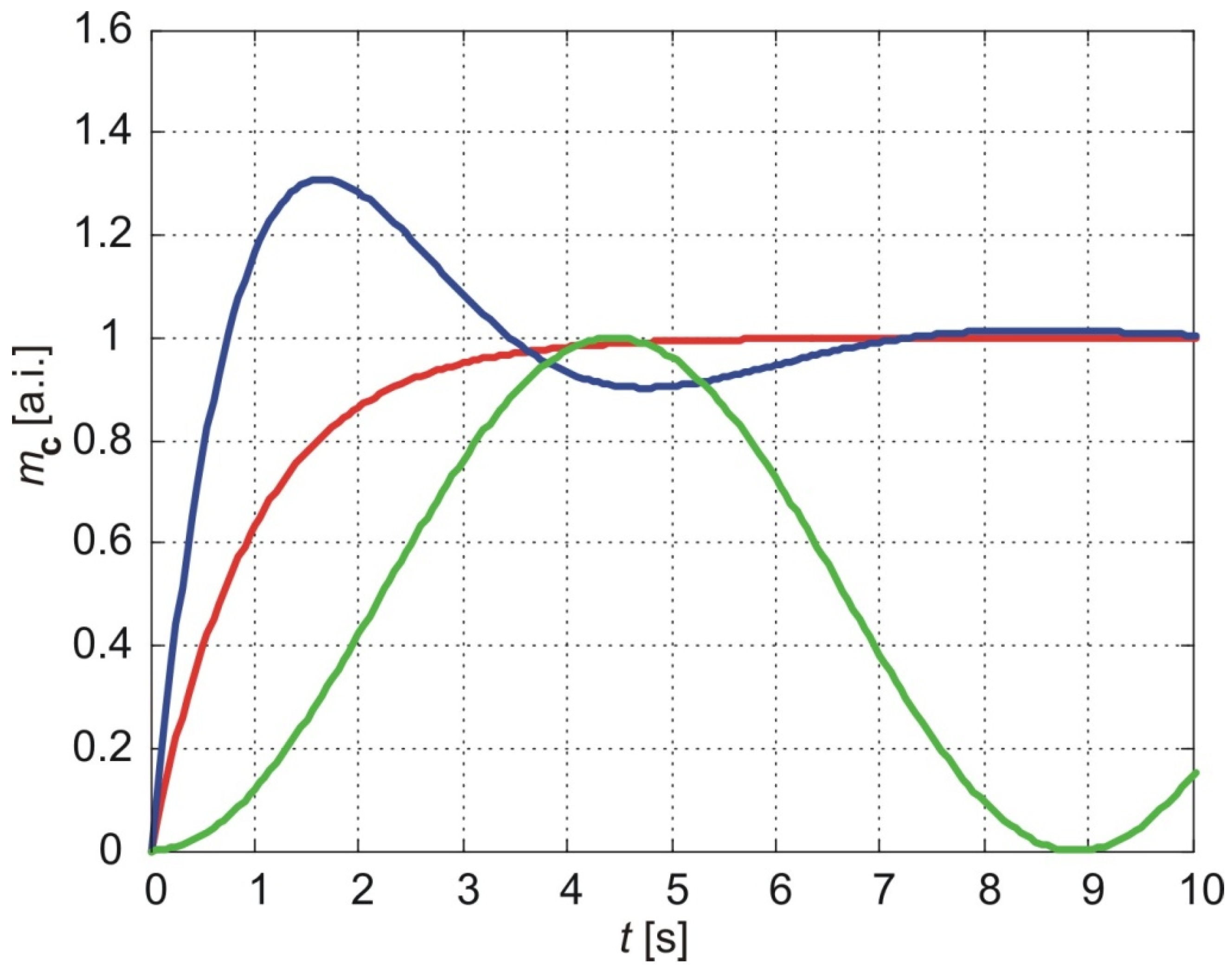
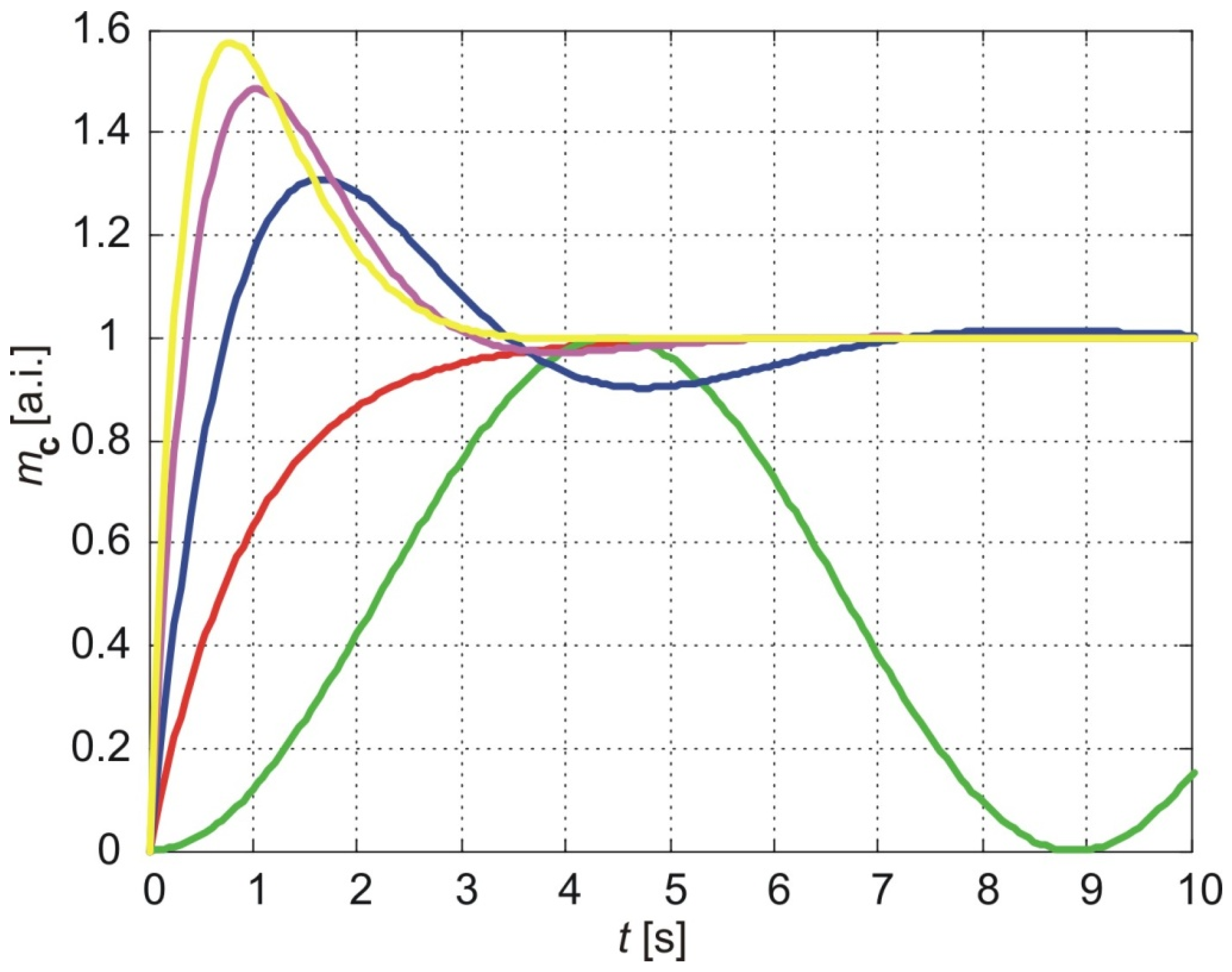
5.2. The Influence of Inertial Memory to the Evolution of Cumulative Amounts of Particles Delivered from Thin Nanoporous Membranes
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Fractional Differ-Integrals—Definitions
Appendix B
References
- Polarz, S.; Smarsly, B. Nanoporous materials. J. Nanosci. Nanotechnol. 2002, 2, 581–612. [Google Scholar] [CrossRef] [PubMed]
- Broom, D.P.; Thomas, K.M. Gas adsorption by nanoporous materials: Future applications and experimental challenges. MRS Bull. 2013, 38, 412–421. [Google Scholar] [CrossRef]
- Ross, D. Hydrogen storage: The major technological barrier to the development of hydrogen fuel cell cars. Vacuum 2006, 80, 1084–1089. [Google Scholar] [CrossRef]
- Azevedo, C.; Tavernier, B.; Vignes, J.L.; Cenedese, P.; Dubot, P. Design of Nanoporous Alumina Structure and Surface Properties for Dental Composite. Key Eng. Mater. 2008, 361–363, 809–812. [Google Scholar] [CrossRef]
- Gultepe, E.; Nagesha, D.; Sridhar, S.; Amiji, M. Nanoporous inorganic membranes or coatings for sustained drug delivery in implantable devices. Adv. Drug Deliv. Rev. 2010, 62, 305–315. [Google Scholar] [CrossRef]
- Kaerger, J.; Ruthven, D.M. Diffusion in nanoporous materials: Fundamental principles, insights and challenges. New J. Chem. 2016, 40, 4027–4048. [Google Scholar] [CrossRef]
- Wu, H.; Wang, D.; Schwartz, D.K. Connecting Hindered Transport in Porous Media across Length Scales: From Single-Pore to Macroscopic. J. Phys. Chem. Lett. 2020, 11, 8825–8831. [Google Scholar] [CrossRef]
- Isaiev, M.; Tutashkonko, S.; Jean, V.; Termentzidis, K.; Nychyporuk, T.; Andrusenko, D.; Marty, O.; Burbelo, R.M.; Lacroix, D.; Lysenko, V. Thermal conductivity of meso-porous germanium. App. Phys. Lett. 2014, 105, 031912. [Google Scholar] [CrossRef]
- Fernandes, M.; Simon, L.; Loney, N.W. Mathematical modeling of transdermal drug delivery systems: Analysis and applications. J. Membr. Sci. 2005, 256, 184–192. [Google Scholar] [CrossRef]
- Cesarone, F.; Caputo, M.; Cametti, C. Memory formalism in the passive diffusion across, highly heterogeneous systems. J. Membr. Sci. 2005, 250, 79–84. [Google Scholar] [CrossRef]
- Caputo, M.; Cametti, C.; Ruggero, V. Time and spatial concentration profile inside a membrane by means of a memory formalism. Phys. A 2008, 387, 2010–2018. [Google Scholar] [CrossRef]
- Metzler, R.; Rajyaguru, A.; Berkowitz, B. Modelling anomalous diffusion in semi-infinite disordered systems and porous media. New J. Phys. 2022, 24, 123004. [Google Scholar] [CrossRef]
- Koh, Y.R.; Shirazi-HD, M.; Vermeersch, B.; Mohammed, A.M.S.; Shao, J.; Pernot, G.; Bahk, J.-H.; Manfra, M.J.; Shakouri, A. Quasi-ballistic thermal transport in Al0.1Ga0.9N thin film semiconductors. Appl. Phys. Lett. 2016, 109, 243107. [Google Scholar] [CrossRef]
- Korabel, N.; Klages, R.; Chechkin, A.V.; Sokolov, I.M.; Gonchar, V.Y. Fractal properties of anomalous diffusion in intermittent maps. Phys. Rev. E 2007, 75, 036213. [Google Scholar] [CrossRef]
- Chen, K.; Wang, B.; Granick, S. Memoryless self-reinforcing directionality in endosomal active transport within living cells. Nat. Mater. 2015, 14, 589. [Google Scholar] [CrossRef] [PubMed]
- Gupta, S.; De Mel, J.U.; Perera, R.M.; Zolnierczuk, P.; Bleuel, M.; Faraone, A.; Schneider, G.J. Dynamics of phospholipid membranes beyond thermal undulations. J. Phys. Chem. Lett. 2018, 9, 2956–2960. [Google Scholar] [CrossRef] [PubMed]
- Hofling, F.; Franosch, T. Anomalous transport in the crowded world of biological cells. Rep. Prog. Phys. 2013, 76, 046602. [Google Scholar] [CrossRef]
- Crank, J. The Mathematics of Diffusion; Oxford University Press: Oxford, UK, 1970. [Google Scholar]
- Carslaw, H.S.; Jaeger, J.C. Conduction of Heat in Solids; Oxford University Press: Oxford, UK, 1959; ISBN 0 19 8533 68 3. [Google Scholar]
- Scher, H.; Montroll, E.W. Anomalous transit-time dispersion in amorphous solids. Phys. Rev. B 1975, 12, 2455. [Google Scholar] [CrossRef]
- Wu, H.; Schwartz, D.K. Nanoparticle Tracking to Probe Transport in Porous Media. Acc. Chem. Res. 2020, 53, 2130–2139. [Google Scholar] [CrossRef] [PubMed]
- Simon, F.; Weiss, L.E.; van Teeffelen, S. A guide to single-particle tracking. Nat. Rev. Methods Primers 2024, 4, 66. [Google Scholar] [CrossRef]
- Zagato, E.; Forier, K.; Martens, T.; Neyts, K.; Demeester, J.; De Smedt, S.; Remaut, K.; Braeckmans, K. Single-particle tracking for studying nanomaterial dynamics: Applications and fundamentals in drug delivery. Nanomedicine 2014, 9, 913–927. [Google Scholar] [CrossRef]
- Peshkov, V. Second sound in helium II. J. Phys. 1944, 8, 381–389. [Google Scholar]
- Narayanamurti, V.; Dynes, R.C. Observation of second sound in bismuth. Phys. Rev. Lett. 1972, 28, 1461–1465. [Google Scholar] [CrossRef]
- Mitra, K.; Kumar, S.; Vedevarz, A.; Moallemi, M.K. Experimental Evidence of Hyperbolic Heat Conduction in Processed Meat. J. Heat Transf. 1995, 117, 568–573. [Google Scholar] [CrossRef]
- Kirsanov, Y.A.; Kirsanov, A.Y.; Yudakhin, A.E. Measurement of thermal relaxation and temperature damping time in a solid. High Temp. 2017, 55, 114–119. [Google Scholar] [CrossRef]
- Ding, Z.; Chen, K.; Song, B.; Shin, J.; Maznev, A.A.; Nelson, K.A.; Chen, G. Observation of second sound in graphite over 200K. Nat. Commun. 2022, 13, 285. [Google Scholar] [CrossRef] [PubMed]
- Jeong, J.; Li, X.; Lee, S.; Shi, L.; Wang, Y. Transient hydrodynamic lattice cooling by picosecond laser irradiation of graphite. Phys. Rev. Lett. 2021, 127, 085901. [Google Scholar] [CrossRef] [PubMed]
- Scher, H.; Shlesinger, M.F.; Bendler, J.T. Time-Scale Invariance in Transport and Relaxation. Phys. Today 1991, 44, 26. [Google Scholar] [CrossRef]
- Hilfer, R. Fractional Diffusion Based on Riemann-Liouville Fractional Derivatives. J. Phys. Chem. B 2000, 104, 3914–3917. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The Random Walk’s Guide to Anomalous Diffusion: A Fractional Dynamics Approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Metzler, R.; Jeon, J.-H.; Cherstvy, A.G.; Barkai, E. Anomalous Diffusion Models and Their Properties: Nonstationarity, Non-ergodicity, and Ageing at the Centenary of Single Particle Tracking. Phys. Chem. Chem. Phys. 2014, 16, 24128. [Google Scholar] [CrossRef]
- Metzler, R.; Jeon, J.-H.; Cherstvy, A.G. Non-Brownian Diffusion in Lipid Membranes: Experiments and Simulations. Biochim. Biophys. Acta Biomembr. 2016, 1858, 2451. [Google Scholar] [CrossRef]
- Nørregaard, K.; Metzler, R.; Ritter, C.M.; Berg-Sørensen, K.; Oddershede, L.B. Manipulation and Motion of Organelles and Single Molecules in Living Cells. Chem. Rev. 2017, 117, 4342. [Google Scholar] [CrossRef]
- Galovic, S.; Djordjevic, A.I.; Kovacevic, B.Z.; Djordjevic, K.L.; Chevizovich, D. Influence of Local Thermodynamic Non-Equilibrium to Photothermally Induced Acoustic Response of Complex Systems. Fractal Fract. 2024, 8, 399. [Google Scholar] [CrossRef]
- Djordjevic, K.L.; Milicevic, D.; Galovic, S.P.; Suljovrujic, E.; Jacimovski, S.K.; Furundzic, D.; Popovic, M. Photothermal Response of Polymeric Materials Including Complex Heat Capacity. Int. J. Thermophys. 2022, 43, 68. [Google Scholar] [CrossRef]
- Metzler, R.; Nonnenmacher, T.F. Fractional diffusion: Exact representations of spectral functions. J. Phys. A Math. Gen. 1997, 30, 1089–1093. [Google Scholar] [CrossRef]
- Joseph, D.D.; Preciozi, L. Heat wave. Rev. Mod. Phys. 1989, 61, 41–73. [Google Scholar] [CrossRef]
- Čukić, M.; Galovic, S. Mathematical modeling of anomalous diffusive behavior in transdermal drug-delivery including time-delayed flux concept. Chaos Solitons Fractals 2023, 172, 113584. [Google Scholar] [CrossRef]
- Ozisik, M.N.; Tzou, D.Y. On the wave theory of heat conduction. ASME J. Heat Transf. 1994, 116, 526–535. [Google Scholar] [CrossRef]
- Novikov, I.A. Resonant generation of harmonic thermal waves in media with memory. J. Eng. Phys Thermophys. 1992, 62, 367–372. [Google Scholar] [CrossRef]
- Novikov, I.A. Harmonic thermal waves in materials with thermal memory. J. Appl. Phys. 1997, 81, 1067–1072. [Google Scholar] [CrossRef]
- Galovic, S.; Kostoski, D. Photothermal wave propagation in media with thermal memory. J. Appl. Phys. 2003, 93, 3063–3071. [Google Scholar] [CrossRef]
- Zhukovsky, K. Operational Approach and Solutions of Hyperbolic Heat Conduction Equations. Axioms 2016, 5, 28. [Google Scholar] [CrossRef]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F.; Rogosin, S.V. Mittag-Leffler Functions, Related Topics and Applications; Springer: Berlin/Heidelberg, Germany, 2014; ISBN 978-3-662-43930-2. [Google Scholar] [CrossRef]
- Inglezakis, V.J. The concept of “capacity” in zeolite ion-exchange systems. J. Colloid Interface Sci. 2005, 281, 68–79. [Google Scholar] [CrossRef]
- Athinarayanan, B.; Jeong, D.-Y.; Kang, J.-H.; Koo, B.-H. Fabrication of nanoporous aluminum-oxide composite membranes. J. Korean Phys. Soc. 2015, 67, 1970–1976. [Google Scholar] [CrossRef]
- Cattaneo, C. Sur une forme de l’equation de la chaleur eliminant le paradowe d’une propagation instantanee. Comptes Rendus Acad. Sci. 1958, 247, 431. [Google Scholar]
- Vernotte, P. Sur quelques complications possible dans les phenomenes de conduction de la chaleur. Comptes Rendus Acad. Sci. 1961, 252, 2190. [Google Scholar]
- Herrera, L. Causal Heat Conduction Contravening the Fading Memory Paradigm. Entropy 2019, 21, 950. [Google Scholar] [CrossRef]
- Sobolev, S.L. Local non-equilibrium transport models. Phys. Uspekhi 1997, 40, 1043–1053. [Google Scholar] [CrossRef]
- Green, A.E.; Rivlin, R.S. The mechanics of non–linear materials with memory (Part III). Arch. Ration. Mech. Anal. 1959, 4, 387–404. [Google Scholar] [CrossRef]
- Green, A.E.; Rivlin, R.S. The mechanics of non–linear materials with memory (Part I). Arch. Ration. Mech. Anal. 1957, 1, 1–21. [Google Scholar] [CrossRef]
- Coleman, B.D.; Noll, W. Foundations of linear viscoelasticity. Rev. Mod. Phys. 1961, 33, 239–249. [Google Scholar] [CrossRef]
- Coleman, B.D.; Mizel, V.J. On the general theory of fading memory. Arch. Ration. Mech. Anal. 1968, 29, 18–31. [Google Scholar] [CrossRef]
- Saut, J.C.; Joseph, D.D. Fading memory. Arch. Ration. Mech. Anal. 1983, 81, 53–95. [Google Scholar] [CrossRef]
- Oldham, K.B.; Spanier, J. The Fractional Calculus; Academic: New York, NY, USA, 1974. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Tateishi, A.; Ribeiro, H.V.; Lenzi, E.K. The role of the fractional time-derivative operators in anomalous diffusion. Front. Phys. 2017, 5, 1–5. [Google Scholar] [CrossRef]
- Kiryakova, V.S. Generalized Fractional Calculus and Applications; Longman Scientific and Technical: Harlow, UK, 1994. [Google Scholar]
- Lenzi, E.K.; Somer, A.; Zola, R.S.; da Silva, L.R.; Lenzi, M.K. A Generalized Diffusion Equation: Solutions and Anomalous Diffusion. Fluids 2023, 8, 34. [Google Scholar] [CrossRef]
- Somer, A.; Popovic, M.; da Cruz, G.; Novatski, A.; Lenzi, E.; Galovic, S. Anomalous thermal diffusion in two-layer system: The temperature profile and photoacoustic signal for rear light incidence. Int. J. Therm. Sci. 2022, 179, 107661. [Google Scholar] [CrossRef]
- Dzielinski, A.; Sierociuk, D.; Sarwas, G. Some applications of fractional order calculus. Bull. Pol. Acad. Sci. Tech. Sci. 2010, 58, 583–592. [Google Scholar] [CrossRef]
- Somer, A.; Galovic, S.; Lenzi, E.; Novatski, A.; Djordjevic, K. Temperature profile and thermal piston component of photoacoustic response calculated by the fractional dual-phase-lag heat conduction theory. Int. J. Heat Mass Transf. 2023, 203, 123801. [Google Scholar] [CrossRef]
- Chen, Y.Q.; Petras, I.; Vinagre, B.M. A List of Laplace and Inverse Laplace Transforms Related to Fractional Order Calculus. Available online: https://ivopetras.tripod.com/foc_laplace.pdf (accessed on 30 November 2001).
- Anissimov, Y.; Watkinson, A. Modelling skin penetration using the Laplace transform technique. Ski. Pharmacol. Physiol. 2013, 26, 286–294. [Google Scholar] [CrossRef]
- Anissimov, Y.G.; Jepps, O.G.; Dancik, Y.; Roberts, M.S. Mathematical and farmacokinetics modelling of epidermal and dermo transport processes. Adv. Drug Deliv. Rev. 2013, 65, 169–190. [Google Scholar] [CrossRef] [PubMed]
- Naegel, A.; Heisig, M.; Wittum, G. Detailed modelling of skin penetration: An overview. Adv. Drug Deliv. Rev. 2013, 65, 191–207. [Google Scholar] [CrossRef] [PubMed]
- Galovic, S.P.; Djordjevic, K.L.; Nesic, M.V.; Popovic, M.N.; Markushev, D.K.; Todorovic, D.M. Time-domain minimum-volume cell photoacoustic of thin semiconductor layer. I. Theory. J. App. Phys. 2023, 133, 245701. [Google Scholar] [CrossRef]
- Lynn, P.A. The Laplace Transform and the Z-Transform. In Electronic Signals and Systems; Macmillan Education: London, UK, 1986; pp. 225–272. ISBN 978-0-333-39164-8. [Google Scholar] [CrossRef]
- Roberts, G.E.; Kaufman, H. Table of Laplace Transform; W.B. Saunders Company: Philadelphia, PA, USA; London, UK, 1966. [Google Scholar]
- Baumann, G. Sinc Based Inverse Laplace Transforms, Mittag-Leffler Functions and Their Approximation for Fractional Calculus. Fractal Fract. 2021, 5, 43. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Galovic, S.; Čukić, M.; Chevizovich, D. Inertial Memory Effects in Molecular Transport Across Nanoporous Membranes. Membranes 2025, 15, 11. https://doi.org/10.3390/membranes15010011
Galovic S, Čukić M, Chevizovich D. Inertial Memory Effects in Molecular Transport Across Nanoporous Membranes. Membranes. 2025; 15(1):11. https://doi.org/10.3390/membranes15010011
Chicago/Turabian StyleGalovic, Slobodanka, Milena Čukić, and Dalibor Chevizovich. 2025. "Inertial Memory Effects in Molecular Transport Across Nanoporous Membranes" Membranes 15, no. 1: 11. https://doi.org/10.3390/membranes15010011
APA StyleGalovic, S., Čukić, M., & Chevizovich, D. (2025). Inertial Memory Effects in Molecular Transport Across Nanoporous Membranes. Membranes, 15(1), 11. https://doi.org/10.3390/membranes15010011







