3D-CFD Modeling of Hollow-Fiber Membrane Contactor for CO2 Absorption Using MEA Solution
Abstract
1. Introduction
2. Mathematical Model Approach
- Isothermal conditions for the fluids;
- The membranes are operating in non-wet condition;
- The CO2 solubility in aqueous MEA solution is calculated with Henry’s law;
- Fully developed laminar flow for both fluid phases (i.e., gas and liquid).
2.1. Continuity Equation
2.2. Navier–Stokes Equations—Tubes and Shell
2.3. Species Transport—Tubes
2.4. Species Transport—Membranes
2.5. Species Transport—Shell
3. Results and Discussion
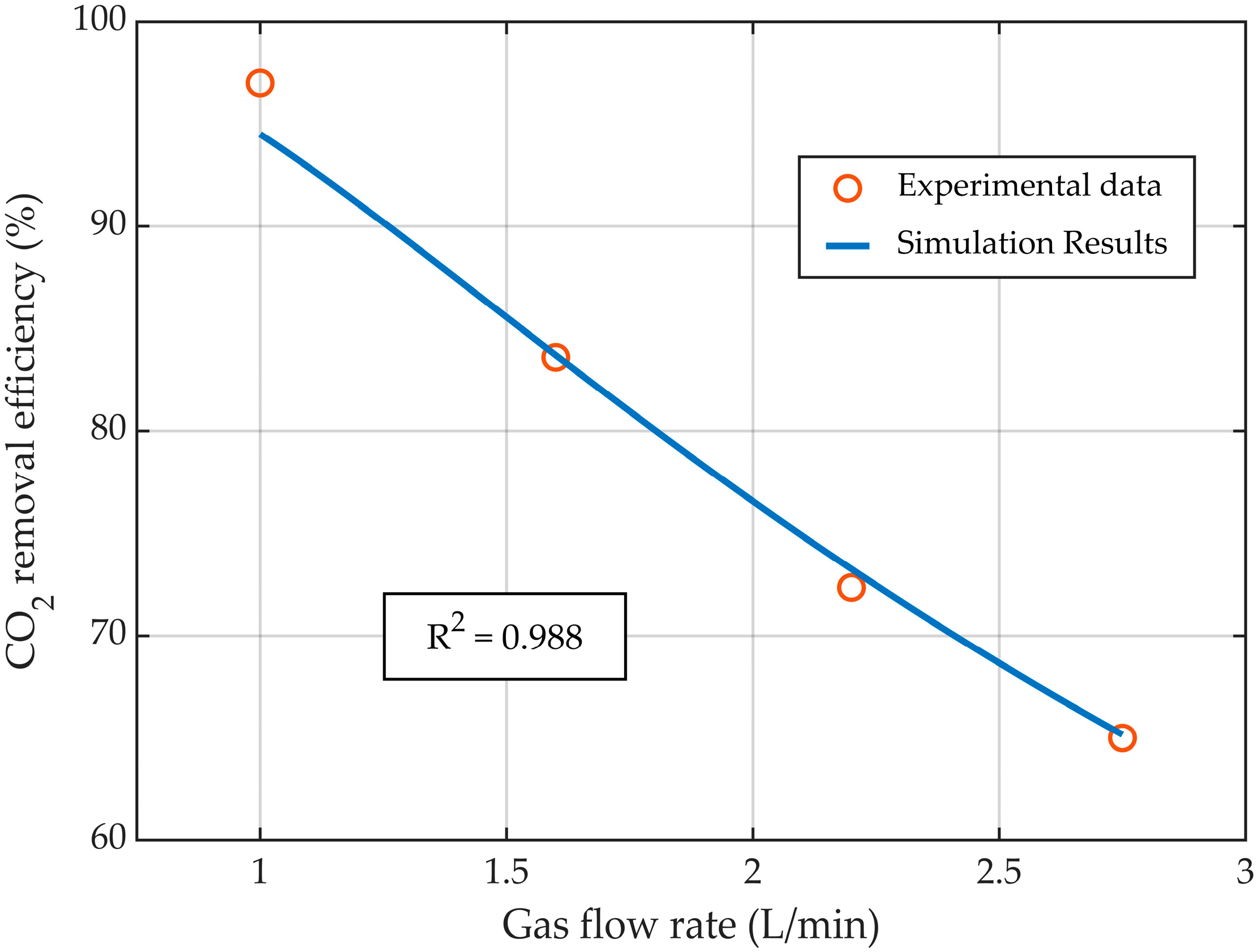
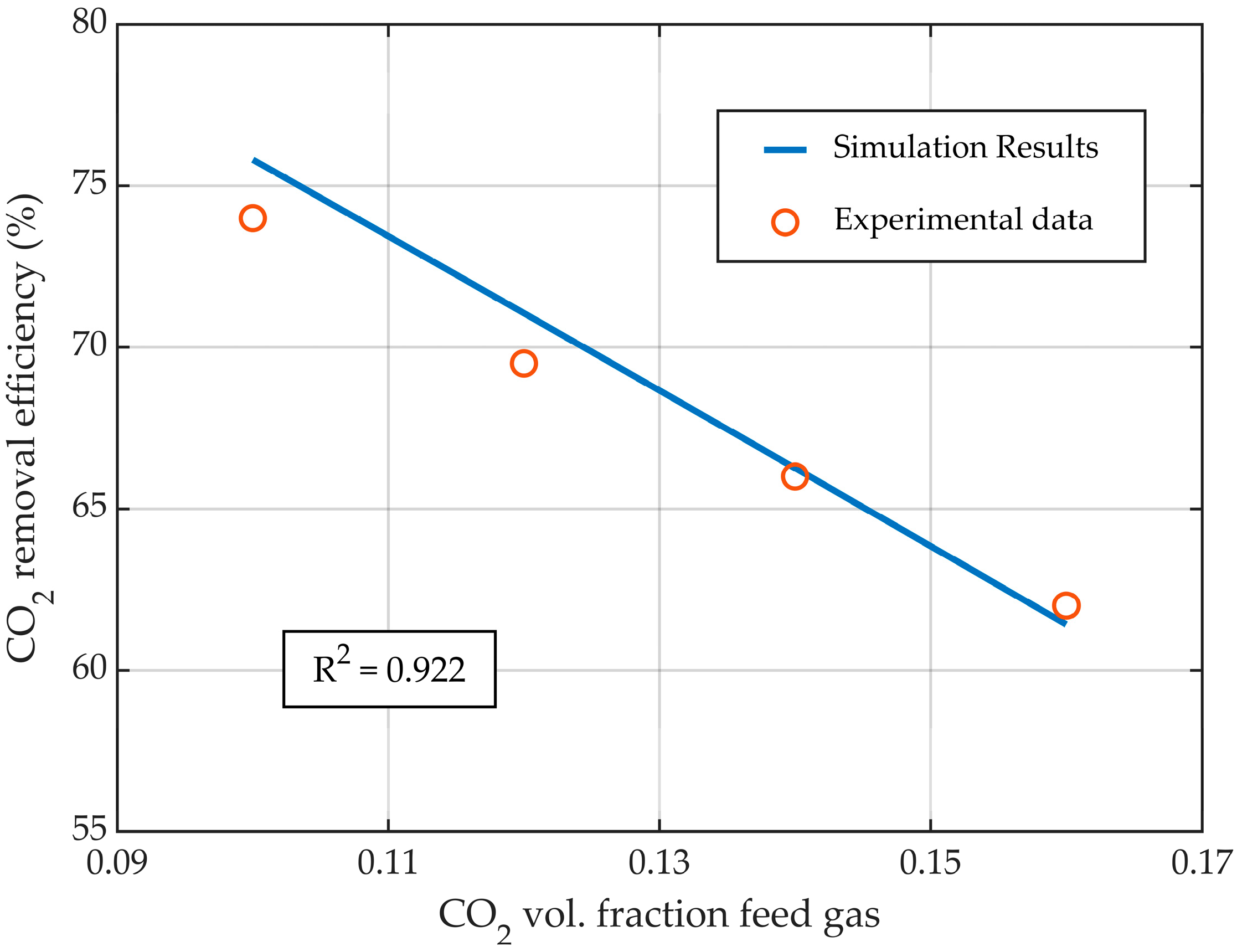
3.1. Model Validation
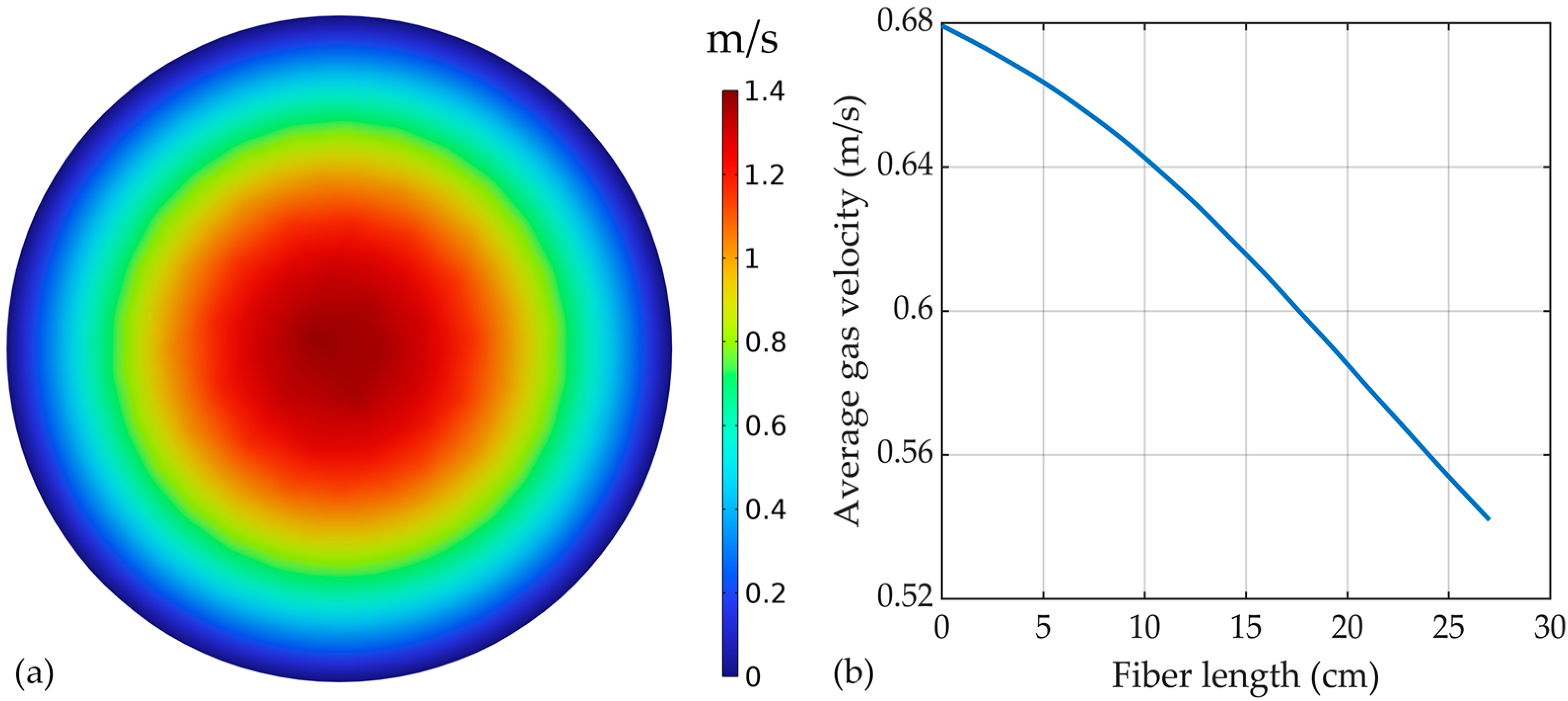
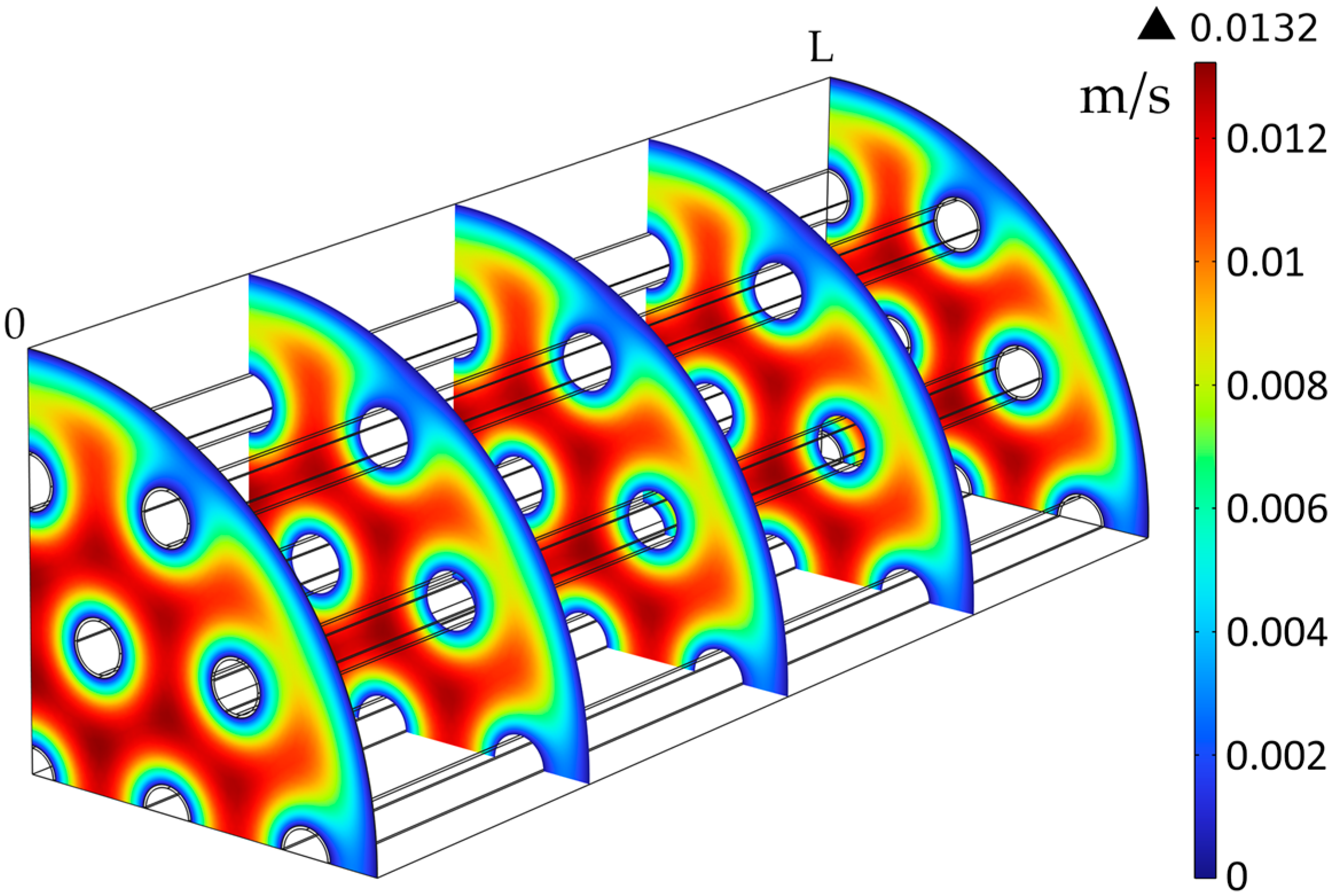
3.2. Velocity Profiles
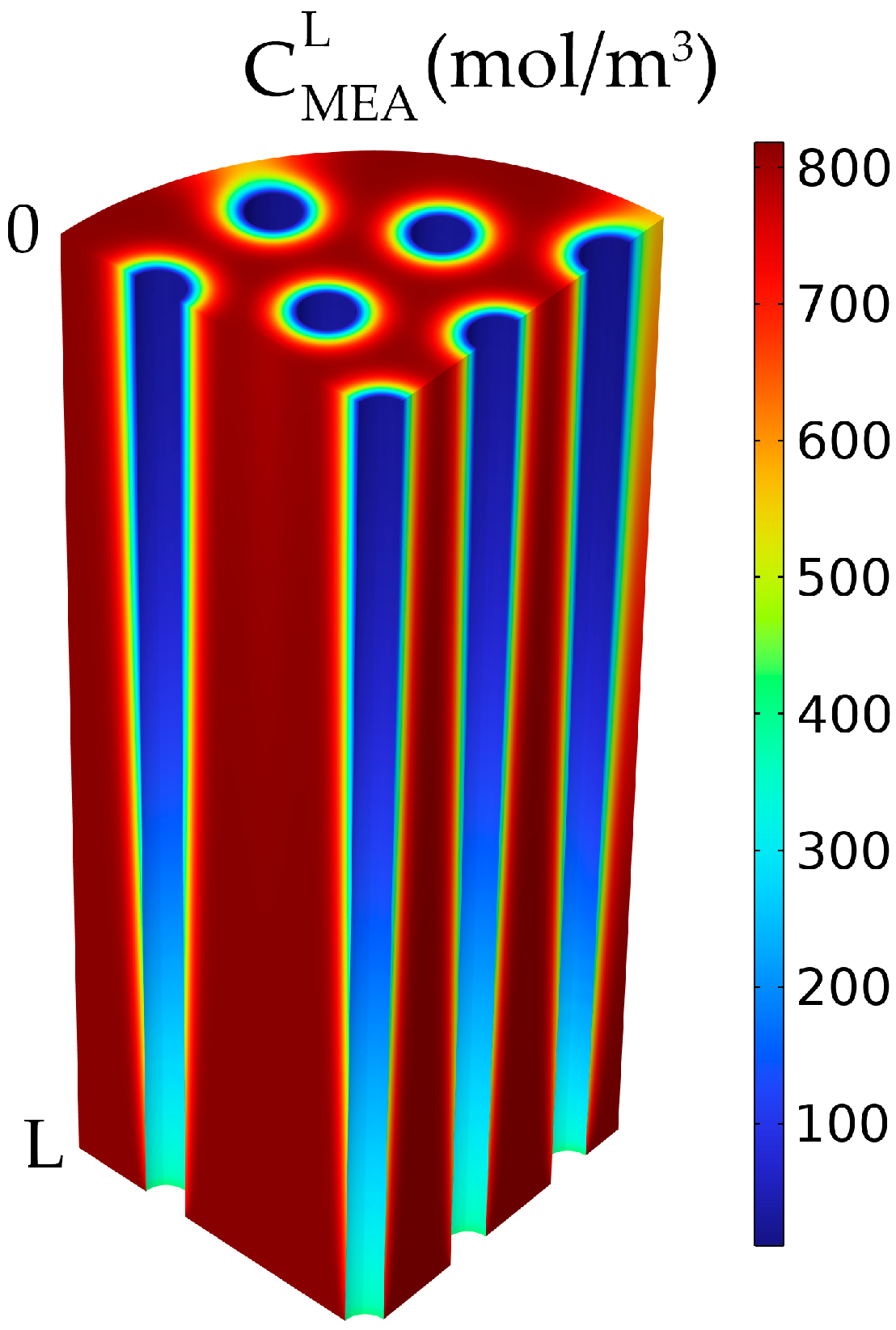
3.3. Concentration Profiles
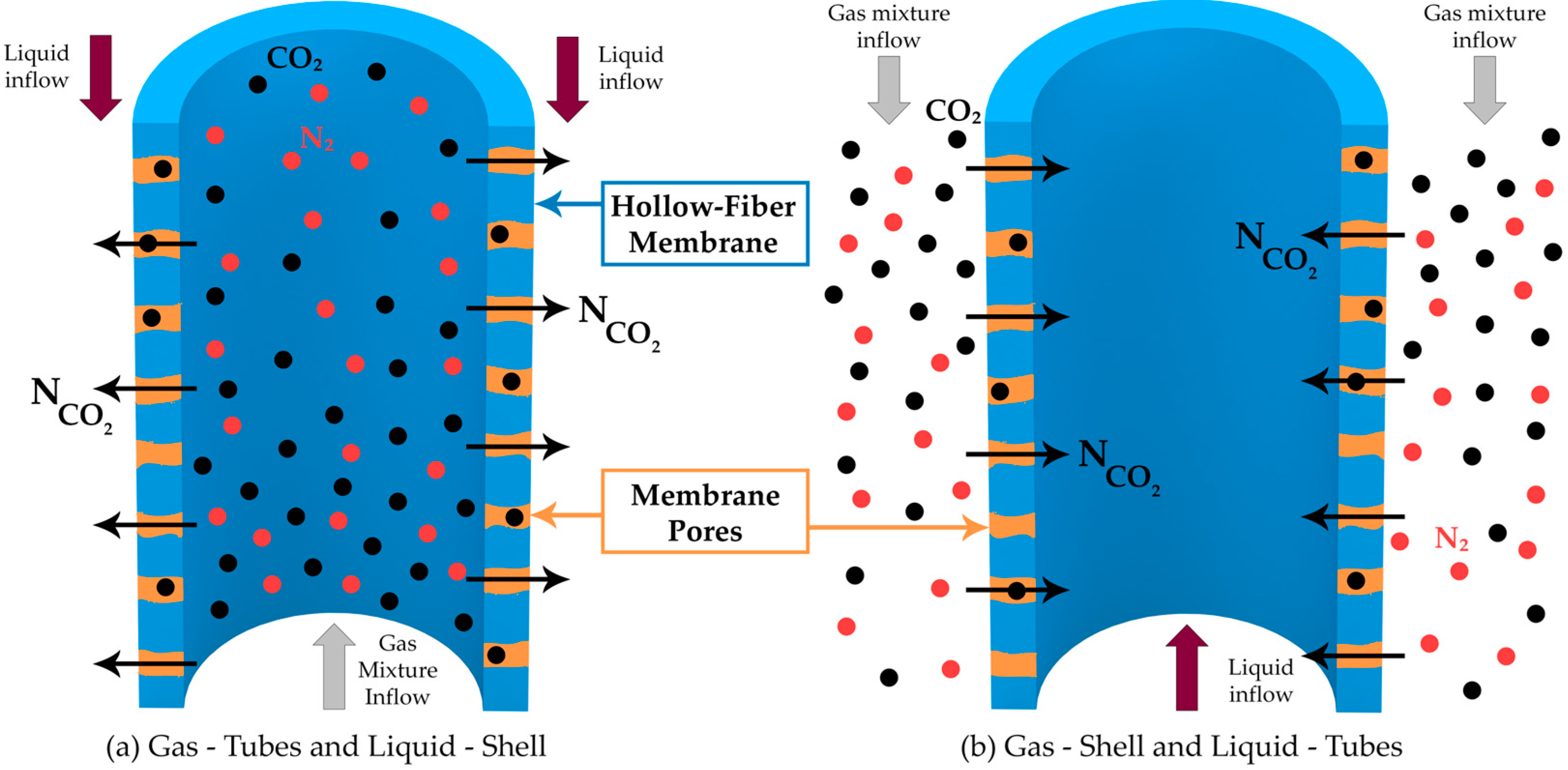
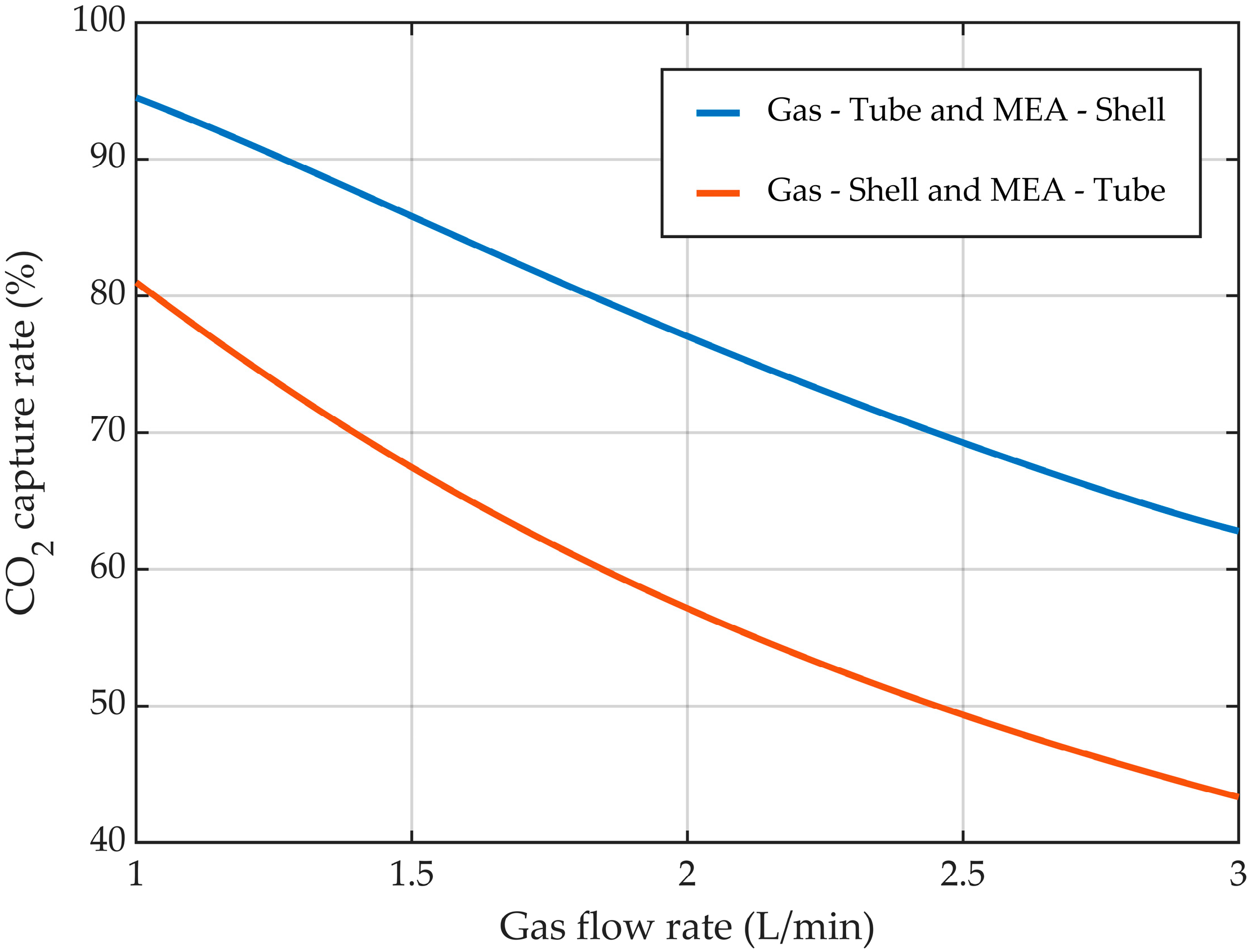
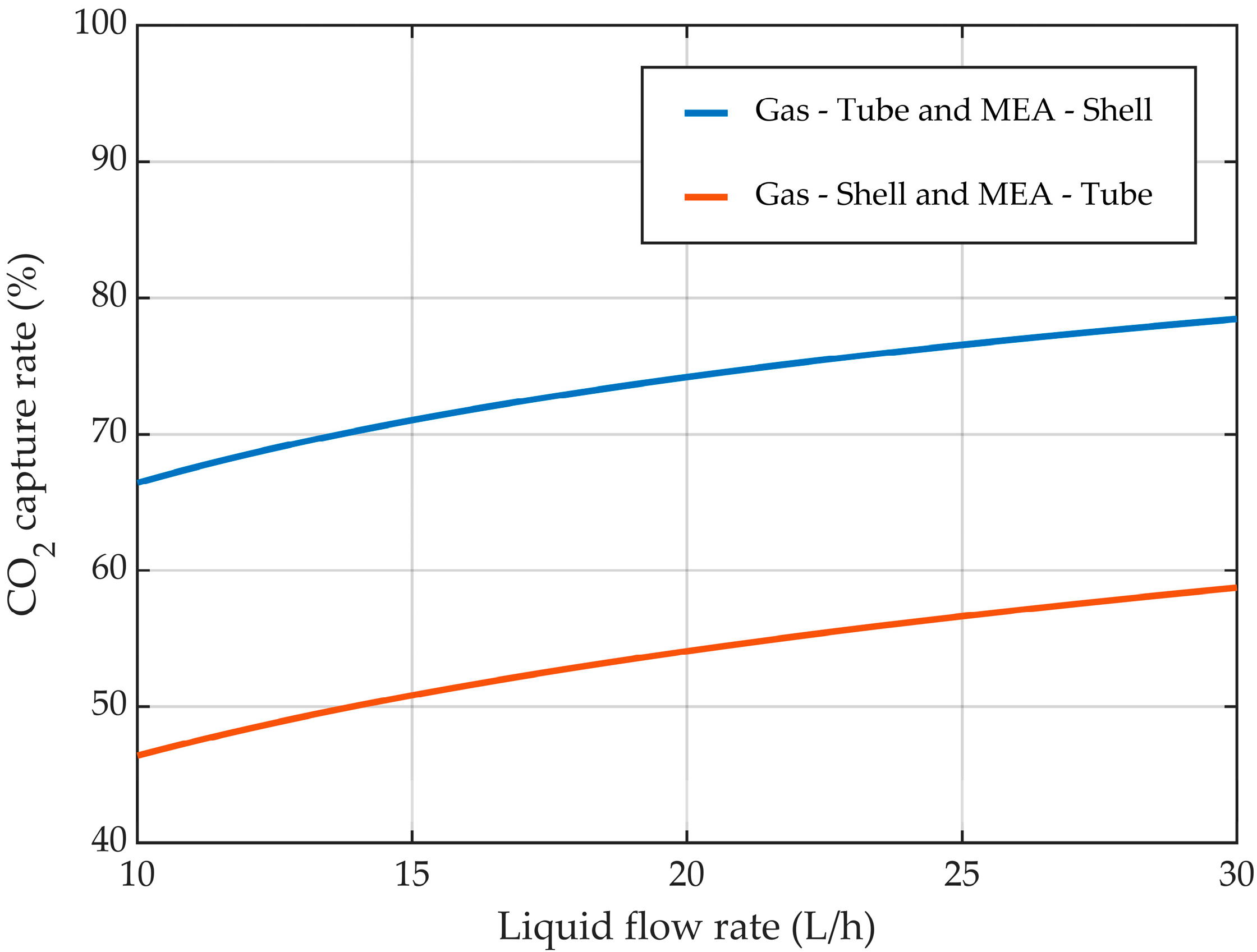
3.4. Tubes vs. Shell
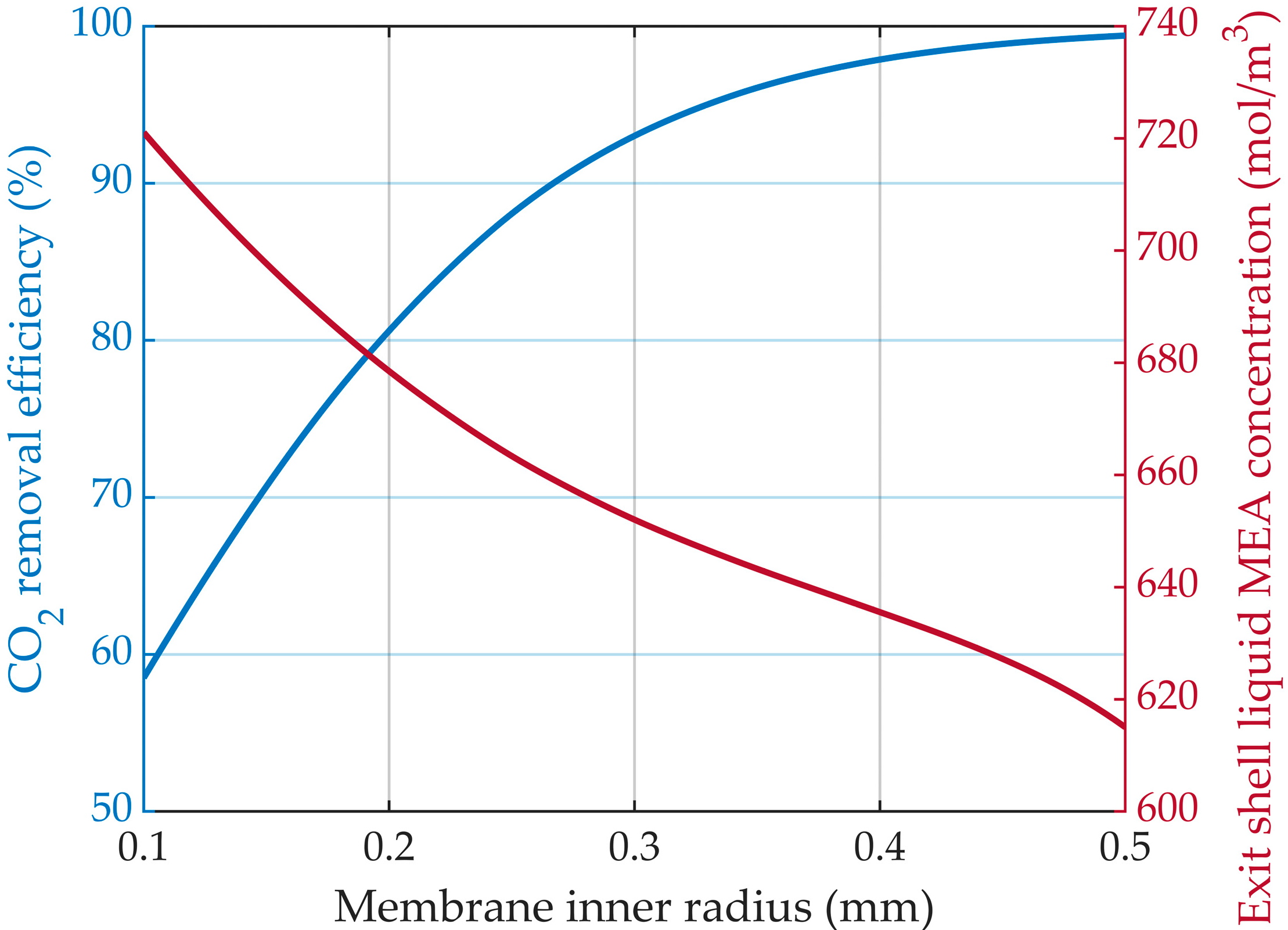
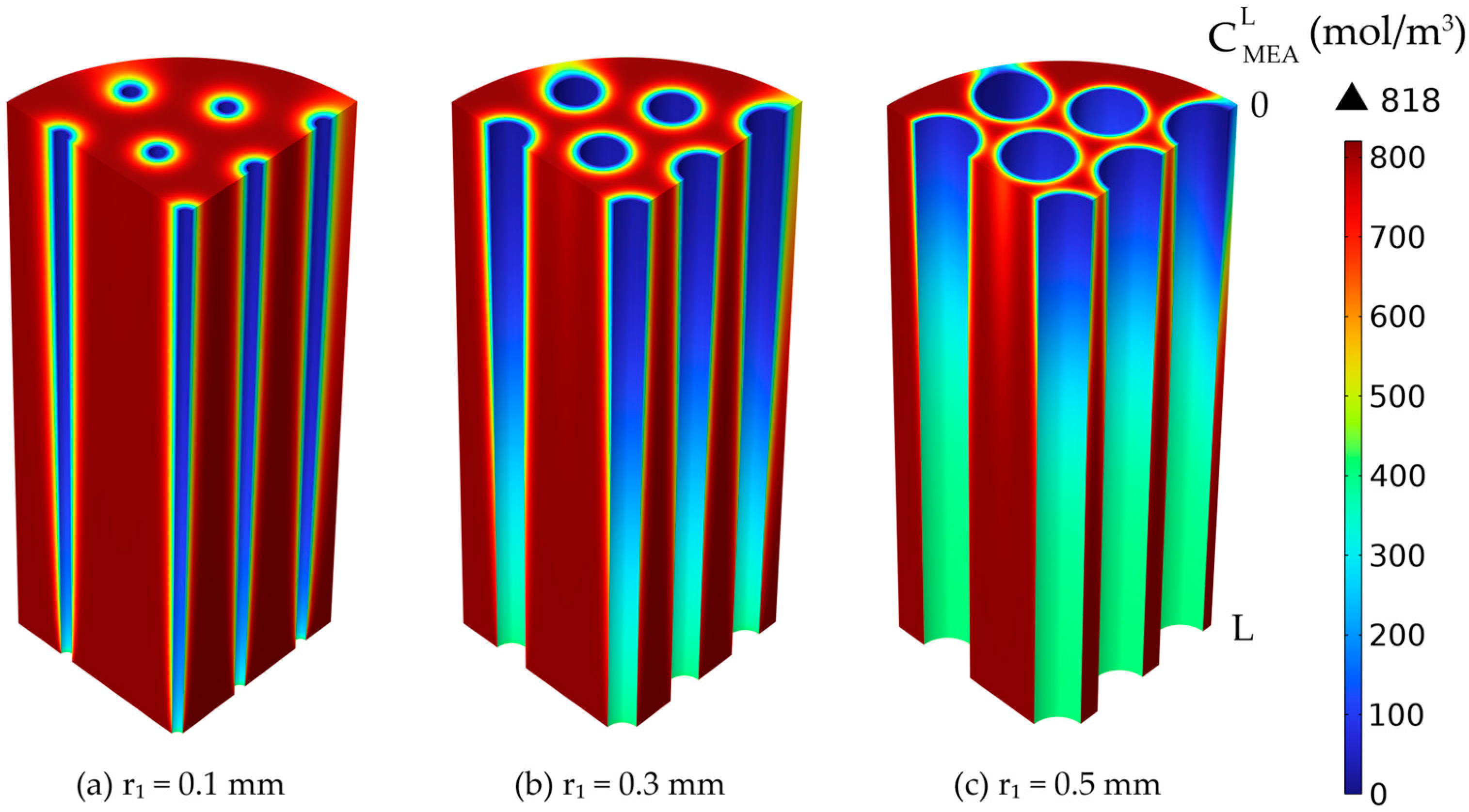
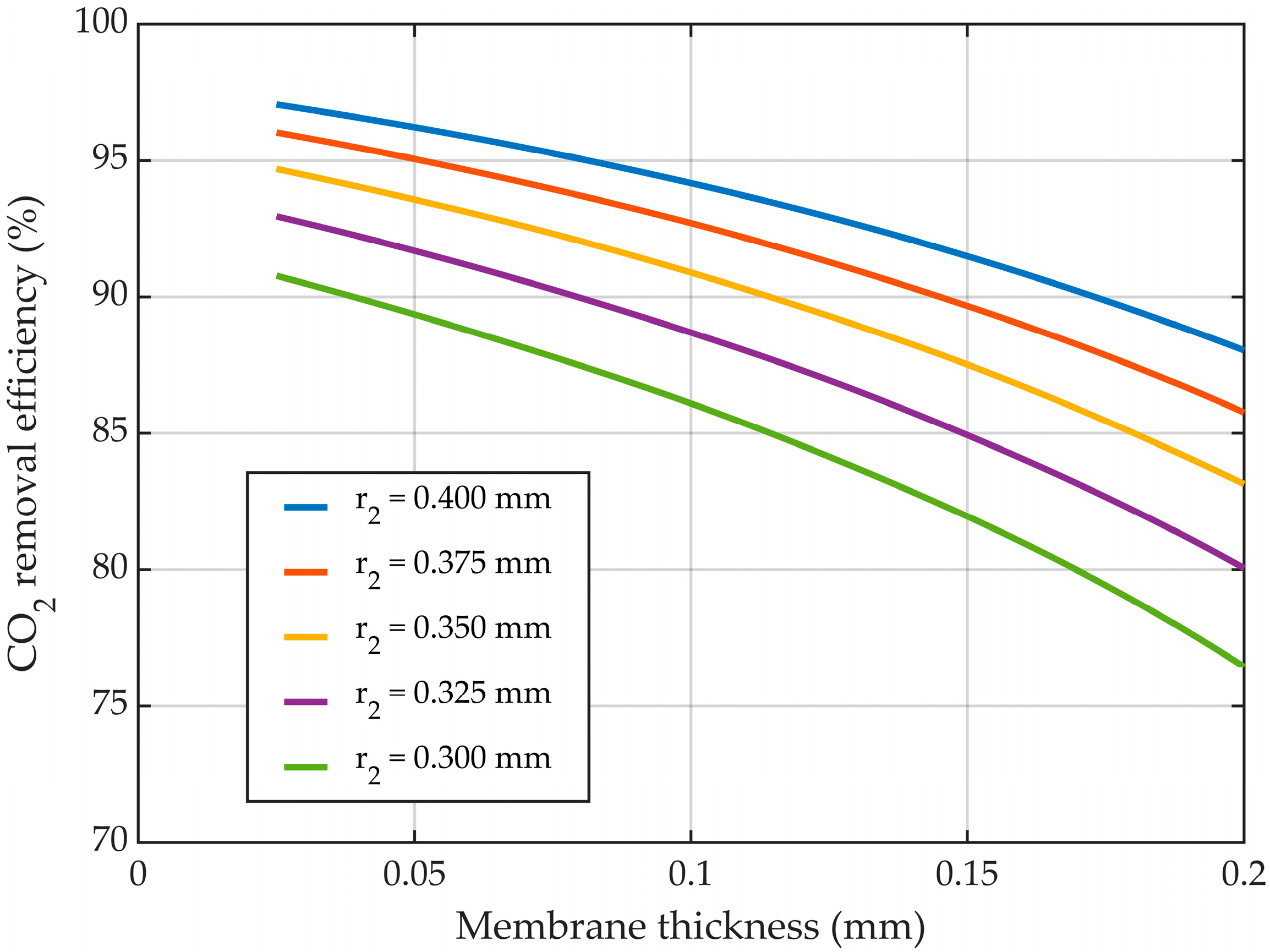
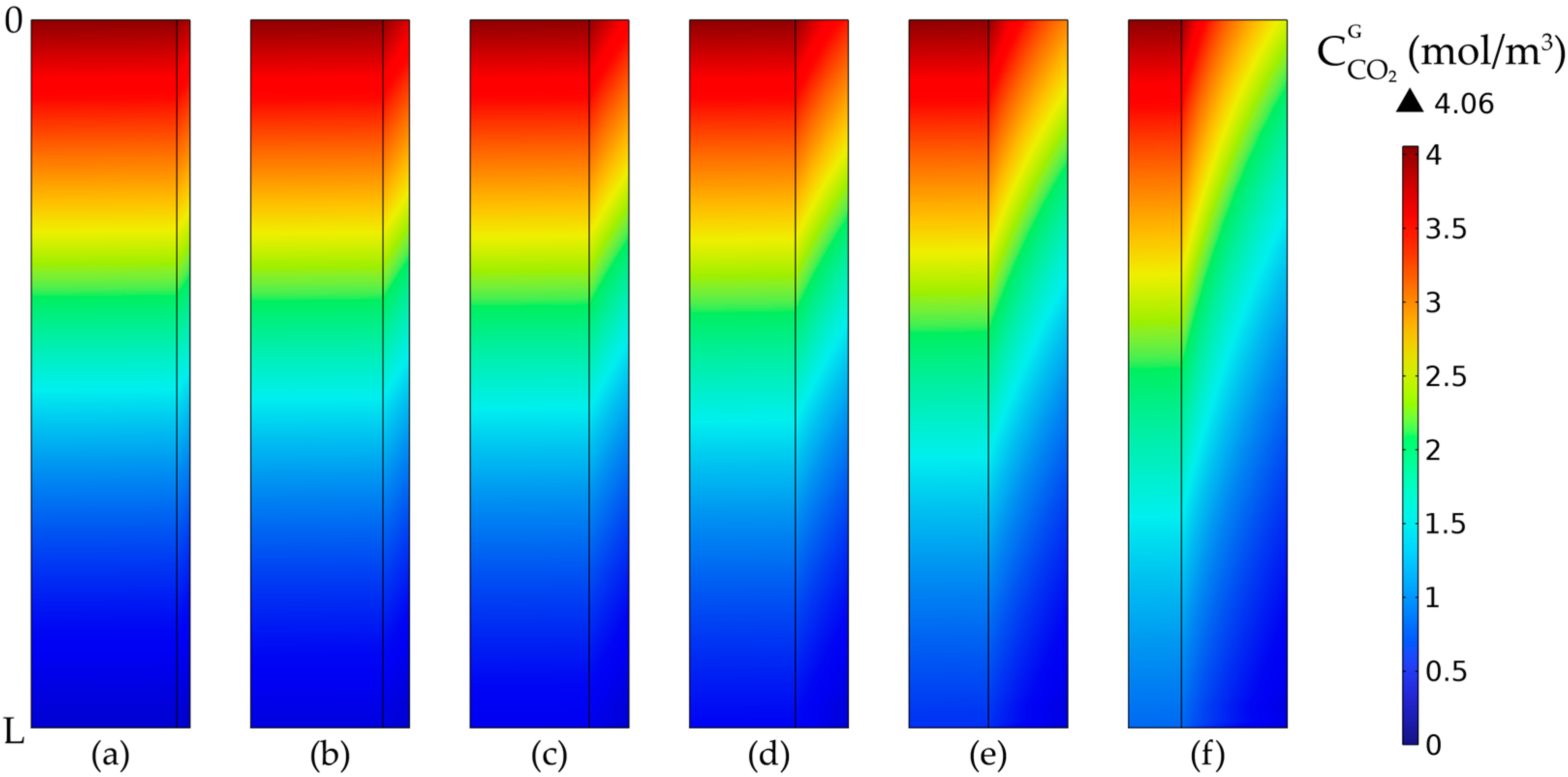
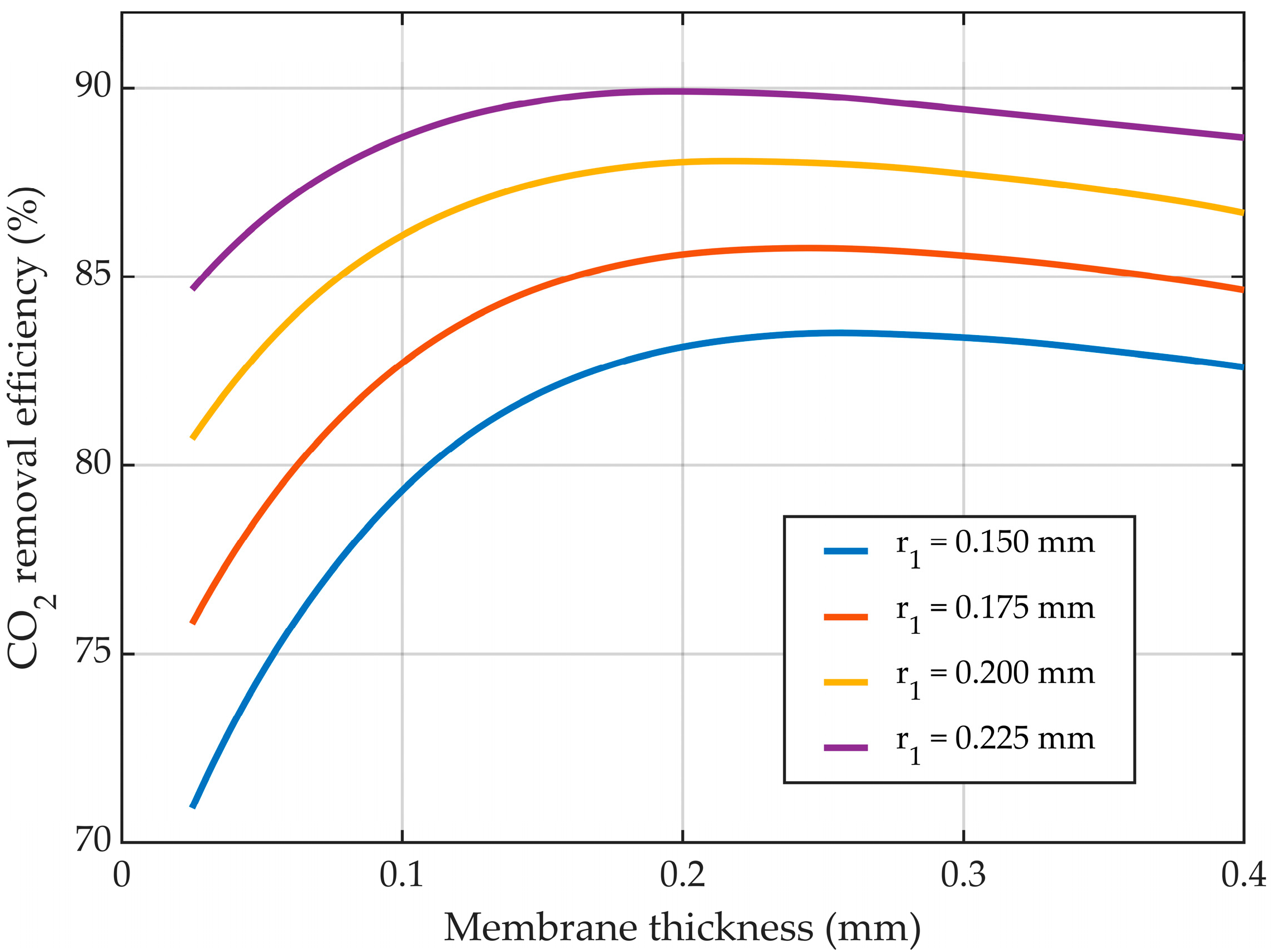
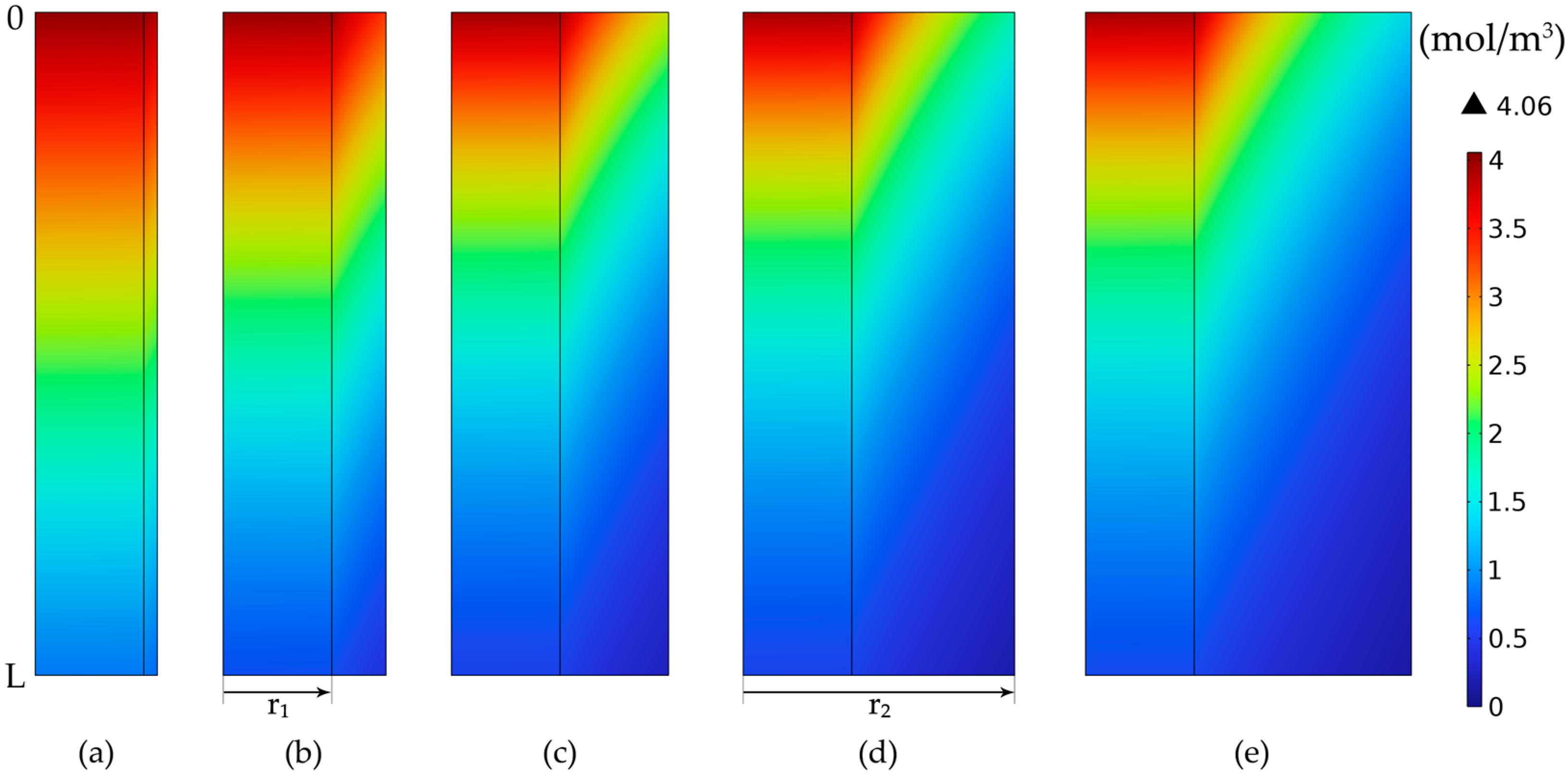
3.5. Membrane Dimensions
3.5.1. Constant Membrane Thickness
3.5.2. Variable Membrane Thickness—r2 Constant
3.5.3. Variable Membrane Thickness—r1 Constant
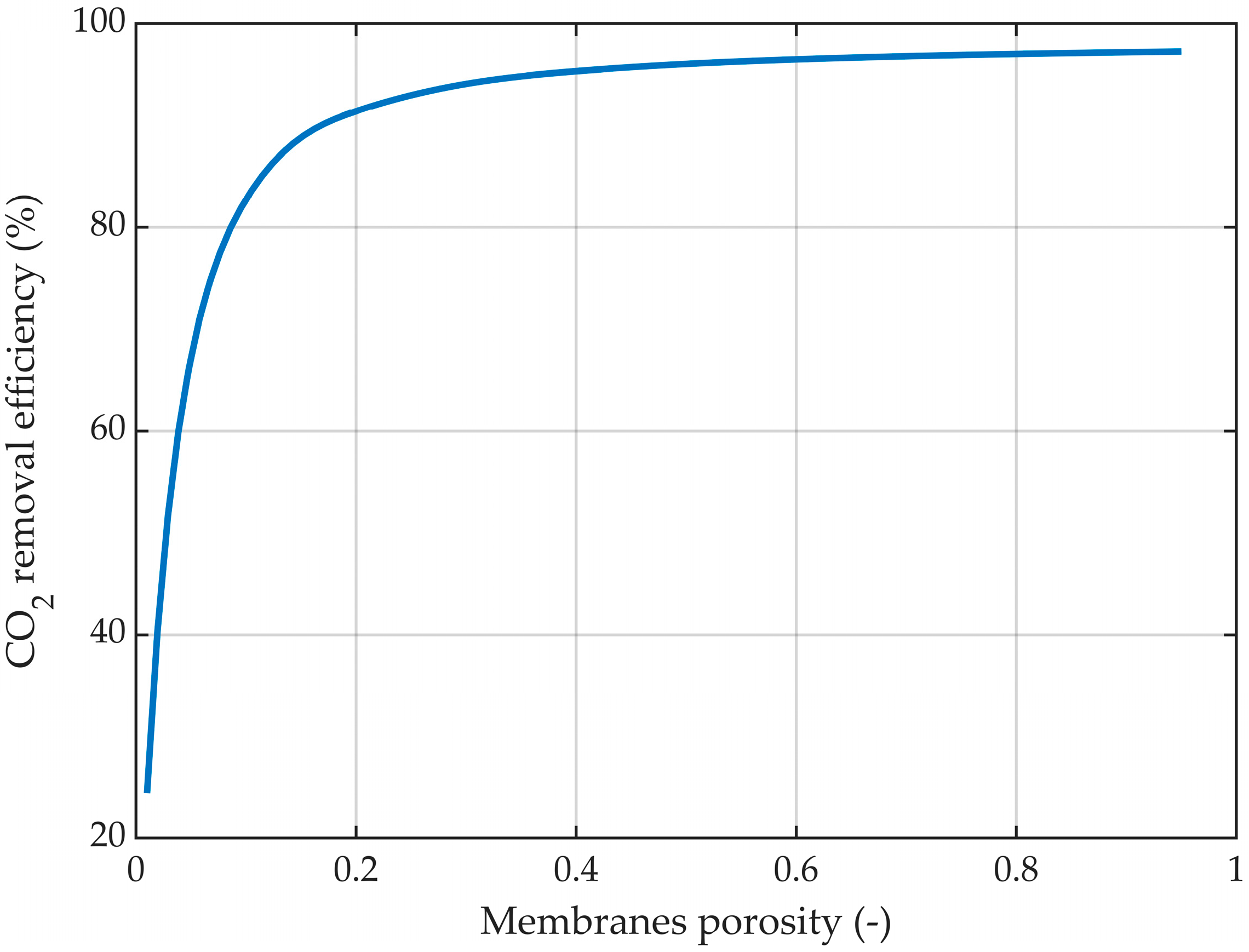
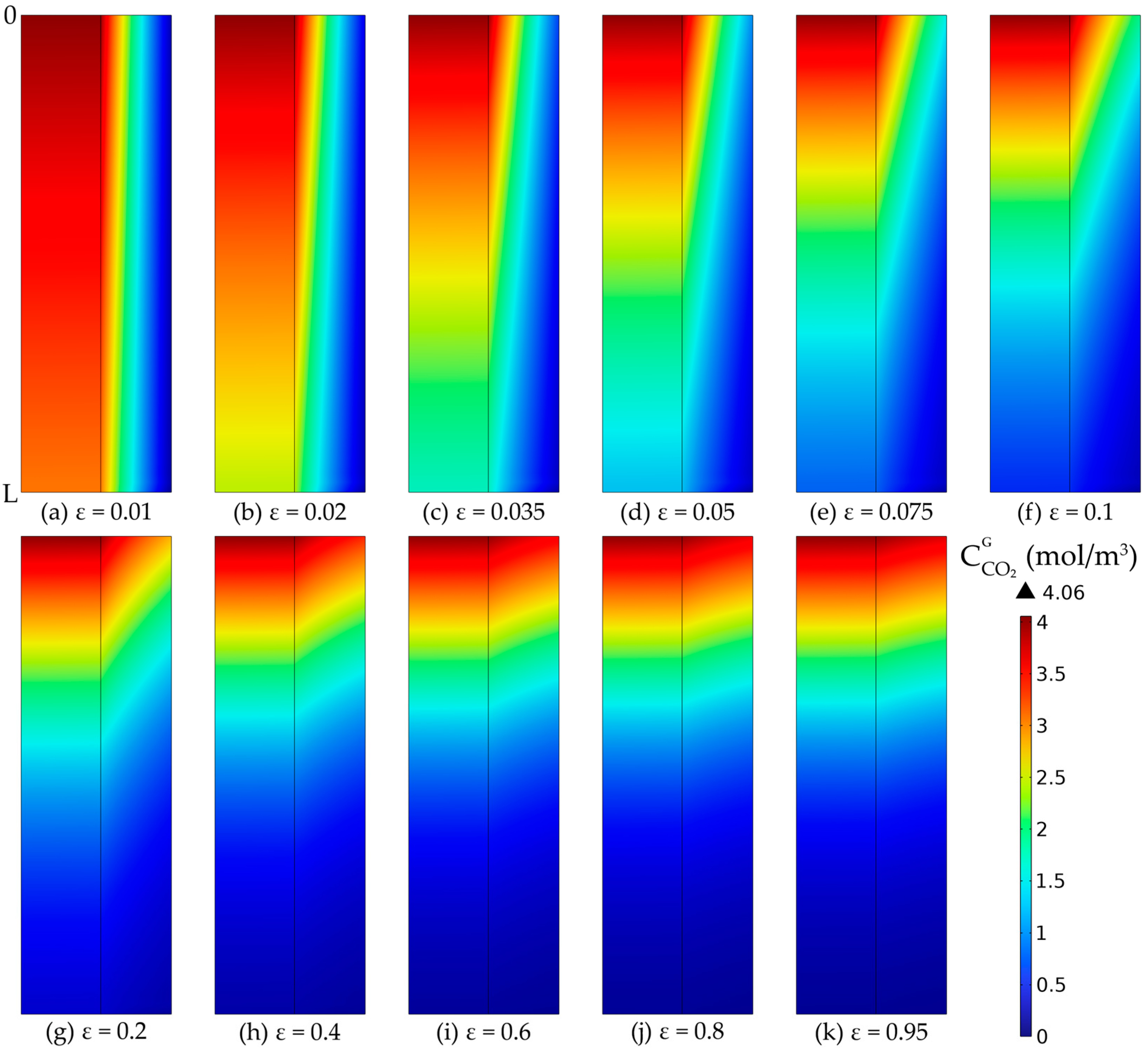
3.6. Membrane Porosity
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| c | concentration of species (mol/m3) |
| d1 | membrane inside diameter (m) |
| dh | hydraulic diameter (m) |
| dlm | logarithmic diameter (m) |
| diffusion coefficient of species j in phase i and membrane (m2/s) | |
| E | enhancement factor (-) |
| Henry’s coefficient of CO2 in the liquid MEA solution (-) | |
| CO2 mass transfer flux from gas to liquid (mol/m2 s) | |
| partial CO2 mass transfer coefficient in the i phase and through membrane pores (m/s) | |
| global CO2 mass transfer coefficient (m/s) | |
| L | membranes length (m) |
| n | number of membranes (-) |
| P | pressure (bar) |
| Q | volumetric flow rate (m3/s) |
| r1 | membrane inside radius (m) |
| r2 | membrane outside radius (m) |
| rmod | module inner radius (m) |
| rp | average membranes pores radius (m) |
| reaction rate (mol/m3 s) | |
| Re | Reynolds number (-) |
| Sc | Schmidt number (-) |
| Sh | Sherwood number (-) |
| T | temperature (K) |
| u | superficial fluid velocity (m/s) |
| Superscripts/subscripts | |
| i | gas (g) and liquid (L) phase |
| j | system components |
| Greek letters | |
| membrane thickness (m) | |
| membrane porosity (-) | |
| dynamic viscosity (Pa s) | |
| fluid density (kg/m3) | |
| membrane tortuosity (-) | |
References
- Nocito, F.; Dibenedetto, A. Atmospheric CO2 Mitigation Technologies: Carbon Capture Utilization and Storage. Curr. Opin. Green Sustain. Chem. 2020, 21, 34–43. [Google Scholar] [CrossRef]
- Eskandari, M.; Khaksar, S.A.N.; Keshavarz, P. CO2 Absorption Using Benzylamine as Absorbent and Promoter in a Hollow Fiber Membrane Contactor: A Numerical Study. J. CO2 Util. 2022, 66, 102287. [Google Scholar] [CrossRef]
- Storrs, K.; Lyhne, I.; Drustrup, R. A Comprehensive Framework for Feasibility of CCUS Deployment: A Meta-Review of Literature on Factors Impacting CCUS Deployment. Int. J. Greenh. Gas Control 2023, 125, 103878. [Google Scholar] [CrossRef]
- Gkotsis, P.; Peleka, E.; Zouboulis, A. Membrane-Based Technologies for Post-Combustion CO2 Capture from Flue Gases: Recent Progress in Commonly Employed Membrane Materials. Membranes 2023, 13, 898. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, L.; Ren, T.; Gao, P.; Sang, H.; Yang, X.; Li, Z.; Wang, Z.; Li, S.; Li, G.K. Carbon Capture from Humid Gases Using Alkaline-Promoted Polypyrrole by a Vacuum Swing Adsorption Process. Sep. Purif. Technol. 2023, 323, 124399. [Google Scholar] [CrossRef]
- Entesari, N.; Divsalar, A.; Tsotsis, T.T. Modeling and Simulation of a Reactive Separation System for Carbon Capture and Utilization in Biogas Streams. Chem. Eng. Process.-Process Intensif. 2020, 156, 108093. [Google Scholar] [CrossRef]
- Vadillo, J.M.; Díaz-Sainz, G.; Gómez-Coma, L.; Garea, A.; Irabien, A. Chemical and Physical Ionic Liquids in CO2 Capture System Using Membrane Vacuum Regeneration. Membranes 2022, 12, 785. [Google Scholar] [CrossRef]
- Fu, L.; Ren, Z.; Si, W.; Ma, Q.; Huang, W.; Liao, K.; Huang, Z.; Wang, Y.; Li, J.; Xu, P. Research Progress on CO2 Capture and Utilization Technology. J. CO2 Util. 2022, 66, 102260. [Google Scholar] [CrossRef]
- Ding, H.; Zhang, Y.; Dong, Y.; Wen, C.; Yang, Y. High-Pressure Supersonic Carbon Dioxide (CO2) Separation Benefiting Carbon Capture, Utilisation and Storage (CCUS) Technology. Appl. Energy 2023, 339, 120975. [Google Scholar] [CrossRef]
- Gabelman, A.; Hwang, S.-T. Hollow Fiber Membrane Contactors. J. Membr. Sci. 1999, 159, 61–106. [Google Scholar] [CrossRef]
- Mansourizadeh, A.; Ismail, A.F. Hollow Fiber Gas–Liquid Membrane Contactors for Acid Gas Capture: A Review. J. Hazard. Mater. 2009, 171, 38–53. [Google Scholar] [CrossRef]
- Xia, J.; Zhang, Z.; Wang, L.; Wang, F.; Miao, H.; Zhang, H.; Xia, L.; Yuan, J. Performance Evaluation and Optimization of Hollow Fiber Membrane Contactors for Carbon Dioxide Absorption: A Comparative Study of Ammonia, Ethanolamine, and Diethanolamine Solvents. J. Environ. Chem. Eng. 2023, 11, 111354. [Google Scholar] [CrossRef]
- Cesari, L.; Castel, C.; Favre, E. Membrane Contactors for Intensified Gas-Liquid Absorption Processes with Physical Solvents: A Critical Parametric Study. J. Membr. Sci. 2021, 635, 119377. [Google Scholar] [CrossRef]
- Taghvaie Nakhjiri, A.; Heydarinasab, A.; Bakhtiari, O.; Mohammadi, T. Numerical Simulation of CO2/H2S Simultaneous Removal from Natural Gas Using Potassium Carbonate Aqueous Solution in Hollow Fiber Membrane Contactor. J. Environ. Chem. Eng. 2020, 8, 104130. [Google Scholar] [CrossRef]
- Rivero, J.R.; Panagakos, G.; Lieber, A.; Hornbostel, K. Hollow Fiber Membrane Contactors for Post-Combustion Carbon Capture: A Review of Modeling Approaches. Membranes 2020, 10, 382. [Google Scholar] [CrossRef]
- Mavroudi, M.; Kaldis, S.P.; Sakellaropoulos, G.P. A Study of Mass Transfer Resistance in Membrane Gas–Liquid Contacting Processes. J. Membr. Sci. 2006, 272, 103–115. [Google Scholar] [CrossRef]
- Kumar, P.S.; Hogendoorn, J.A.; Feron, P.H.M.; Versteeg, G.F. Approximate Solution to Predict the Enhancement Factor for the Reactive Absorption of a Gas in a Liquid Flowing through a Microporous Membrane Hollow Fiber. J. Membr. Sci. 2003, 213, 231–245. [Google Scholar] [CrossRef]
- Rode, S.; Nguyen, P.T.; Roizard, D.; Bounaceur, R.; Castel, C.; Favre, E. Evaluating the Intensification Potential of Membrane Contactors for Gas Absorption in a Chemical Solvent: A Generic One-Dimensional Methodology and Its Application to CO2 Absorption in Monoethanolamine. J. Membr. Sci. 2012, 389, 1–16. [Google Scholar] [CrossRef]
- Saeed, M.; Deng, L. Post-Combustion CO2 Membrane Absorption Promoted by Mimic Enzyme. J. Membr. Sci. 2016, 499, 36–46. [Google Scholar] [CrossRef]
- Khaisri, S.; deMontigny, D.; Tontiwachwuthikul, P.; Jiraratananon, R. A Mathematical Model for Gas Absorption Membrane Contactors That Studies the Effect of Partially Wetted Membranes. J. Membr. Sci. 2010, 347, 228–239. [Google Scholar] [CrossRef]
- Chu, Y.; Lindbråthen, A.; Lei, L.; He, X.; Hillestad, M. Mathematical Modeling and Process Parametric Study of CO2 Removal from Natural Gas by Hollow Fiber Membranes. Chem. Eng. Res. Des. 2019, 148, 45–55. [Google Scholar] [CrossRef]
- Boributh, S.; Rongwong, W.; Assabumrungrat, S.; Laosiripojana, N.; Jiraratananon, R. Mathematical Modeling and Cascade Design of Hollow Fiber Membrane Contactor for CO2 Absorption by Monoethanolamine. J. Membr. Sci. 2012, 401–402, 175–189. [Google Scholar] [CrossRef]
- Bozonc, A.-C.; Cormos, A.-M.; Dragan, S.; Dinca, C.; Cormos, C.-C. Dynamic Modeling of CO2 Absorption Process Using Hollow-Fiber Membrane Contactor in MEA Solution. Energies 2022, 15, 7241. [Google Scholar] [CrossRef]
- Al-Marzouqi, M.H.; El-Naas, M.H.; Marzouk, S.A.M.; Al-Zarooni, M.A.; Abdullatif, N.; Faiz, R. Modeling of CO2 Absorption in Membrane Contactors. Sep. Purif. Technol. 2008, 59, 286–293. [Google Scholar] [CrossRef]
- Hosseinzadeh, A.; Hosseinzadeh, M.; Vatani, A.; Mohammadi, T. Mathematical Modeling for the Simultaneous Absorption of CO2 and SO2 Using MEA in Hollow Fiber Membrane Contactors. Chem. Eng. Process. Process Intensif. 2017, 111, 35–45. [Google Scholar] [CrossRef]
- Shirazian, S.; Pishnamazi, M.; Rezakazemi, M.; Nouri, A.; Jafari, M.; Noroozi, S.; Marjani, A. Implementation of the Finite Element Method for Simulation of Mass Transfer in Membrane Contactors. Chem. Eng. Technol. 2012, 35, 1077–1084. [Google Scholar] [CrossRef]
- Faiz, R.; Al-Marzouqi, M. CO2 Removal from Natural Gas at High Pressure Using Membrane Contactors: Model Validation and Membrane Parametric Studies. J. Membr. Sci. 2010, 365, 232–241. [Google Scholar] [CrossRef]
- Goyal, N.; Suman, S.; Gupta, S.K. Mathematical Modeling of CO2 Separation from Gaseous-Mixture Using a Hollow-Fiber Membrane Module: Physical Mechanism and Influence of Partial-Wetting. J. Membr. Sci. 2015, 474, 64–82. [Google Scholar] [CrossRef]
- Rezakazemi, M.; Niazi, Z.; Mirfendereski, M.; Shirazian, S.; Mohammadi, T.; Pak, A. CFD Simulation of Natural Gas Sweetening in a Gas–Liquid Hollow-Fiber Membrane Contactor. Chem. Eng. J. 2011, 168, 1217–1226. [Google Scholar] [CrossRef]
- Shirazian, S.; Moghadassi, A.; Moradi, S. Numerical Simulation of Mass Transfer in Gas–Liquid Hollow Fiber Membrane Contactors for Laminar Flow Conditions. Simul. Model. Pract. Theory 2009, 17, 708–718. [Google Scholar] [CrossRef]
- Nakhjiri, A.T.; Heydarinasab, A.; Bakhtiari, O.; Mohammadi, T. Experimental Investigation and Mathematical Modeling of CO2 Sequestration from CO2/CH4 Gaseous Mixture Using MEA and TEA Aqueous Absorbents through Polypropylene Hollow Fiber Membrane Contactor. J. Membr. Sci. 2018, 565, 1–13. [Google Scholar] [CrossRef]
- Vadillo, J.M.; Gómez-Coma, L.; Garea, A.; Irabien, A. CO2 Desorption Performance from Imidazolium Ionic Liquids by Membrane Vacuum Regeneration Technology. Membranes 2020, 10, 234. [Google Scholar] [CrossRef] [PubMed]
- Pozzobon, V.; Perré, P. Mass Transfer in Hollow Fiber Membrane Contactor: Computational Fluid Dynamics Determination of the Shell Side Resistance. Sep. Purif. Technol. 2020, 241, 116674. [Google Scholar] [CrossRef]
- Cai, J.J.; Hawboldt, K.; Abdi, M.A. Analysis of the Effect of Module Design on Gas Absorption in Cross Flow Hollow Membrane Contactors via Computational Fluid Dynamics (CFD) Analysis. J. Membr. Sci. 2016, 520, 415–424. [Google Scholar] [CrossRef]
- Boucif, N.; Corriou, J.P.; Roizard, D.; Favre, E. Carbon Dioxide Absorption by Monoethanolamine in Hollow Fiber Membrane Contactors: A Parametric Investigation. AIChE J. 2012, 58, 2843–2855. [Google Scholar] [CrossRef]
- Yang, M.-C.; Cussler, E.L. Designing Hollow-Fiber Contactors. AIChE J. 1986, 32, 1910–1916. [Google Scholar] [CrossRef]
- Ghobadi, J.; Ramirez, D.; Jerman, R.; Crane, M.; Khoramfar, S. CO2 Separation Performance of Different Diameter Polytetrafluoroethylene Hollow Fiber Membranes Using Gas-Liquid Membrane Contacting System. J. Membr. Sci. 2018, 549, 75–83. [Google Scholar] [CrossRef]

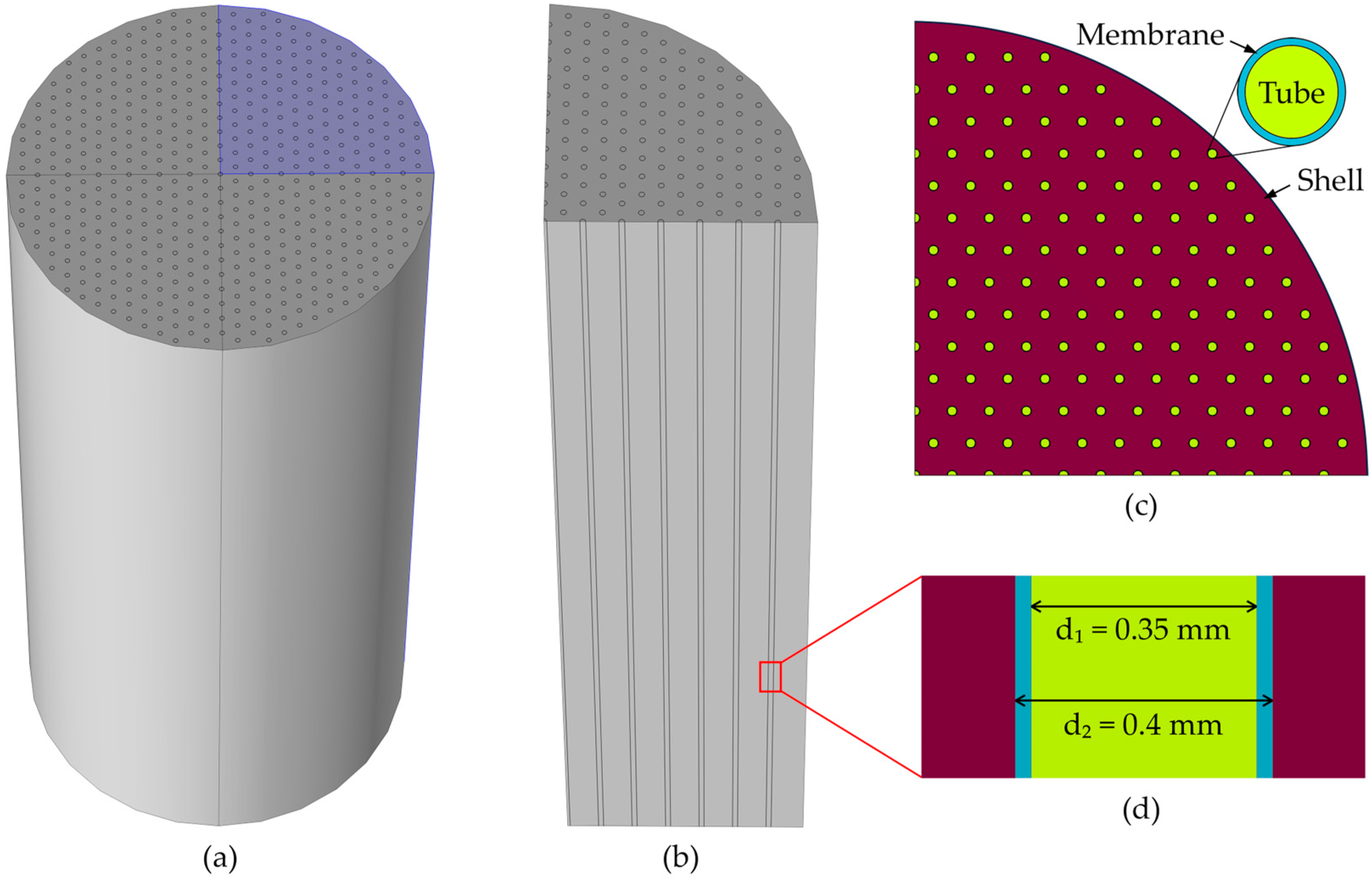
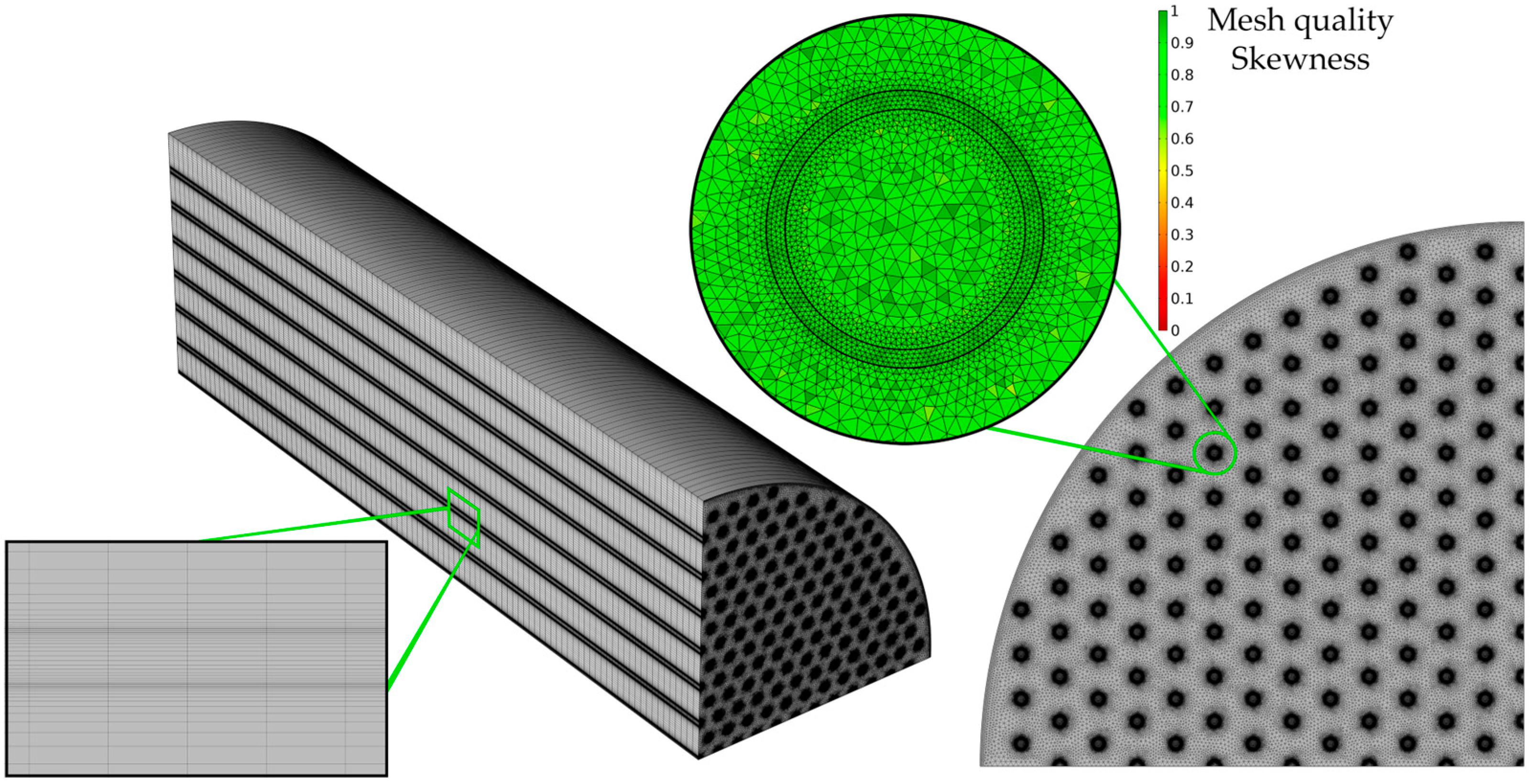


| Parameters | Value | Unit |
|---|---|---|
| Membrane inner radius (r1) | 0.175 | mm |
| Membrane outer radius (r2) | 0.2 | mm |
| Number of membranes (n) | 510 | - |
| Module inner radius (rmod) | 1.75 | cm |
| Membrane length (L) | 27 | cm |
| Membrane porosity () | 0.17 | - |
| Membrane tortuosity () | 19.7 | - |
| Average pores radius (rp) | 0.05 | μm |
| Parameters | Value | Unit |
|---|---|---|
| Gas flow rate (QG) | 1–3 | L/min |
| Liquid flow rate (QL) | 10–30 | L/h |
| CO2 gas concentration () | 10 | vol% |
| MEA liquid concentration (CMEA) | 5 | wt% |
| Temperature (T) | 298 | K |
| Pressure (P) | 1 | bar |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bozonc, A.-C.; Sandu, V.-C.; Cormos, C.-C.; Cormos, A.-M. 3D-CFD Modeling of Hollow-Fiber Membrane Contactor for CO2 Absorption Using MEA Solution. Membranes 2024, 14, 86. https://doi.org/10.3390/membranes14040086
Bozonc A-C, Sandu V-C, Cormos C-C, Cormos A-M. 3D-CFD Modeling of Hollow-Fiber Membrane Contactor for CO2 Absorption Using MEA Solution. Membranes. 2024; 14(4):86. https://doi.org/10.3390/membranes14040086
Chicago/Turabian StyleBozonc, Alexandru-Constantin, Vlad-Cristian Sandu, Calin-Cristian Cormos, and Ana-Maria Cormos. 2024. "3D-CFD Modeling of Hollow-Fiber Membrane Contactor for CO2 Absorption Using MEA Solution" Membranes 14, no. 4: 86. https://doi.org/10.3390/membranes14040086
APA StyleBozonc, A.-C., Sandu, V.-C., Cormos, C.-C., & Cormos, A.-M. (2024). 3D-CFD Modeling of Hollow-Fiber Membrane Contactor for CO2 Absorption Using MEA Solution. Membranes, 14(4), 86. https://doi.org/10.3390/membranes14040086








