Competition between Hydration Shell and Ordered Water Chain Induces Thickness-Dependent Desalination Performance in Carbon Nanotube Membrane
Abstract
1. Introduction
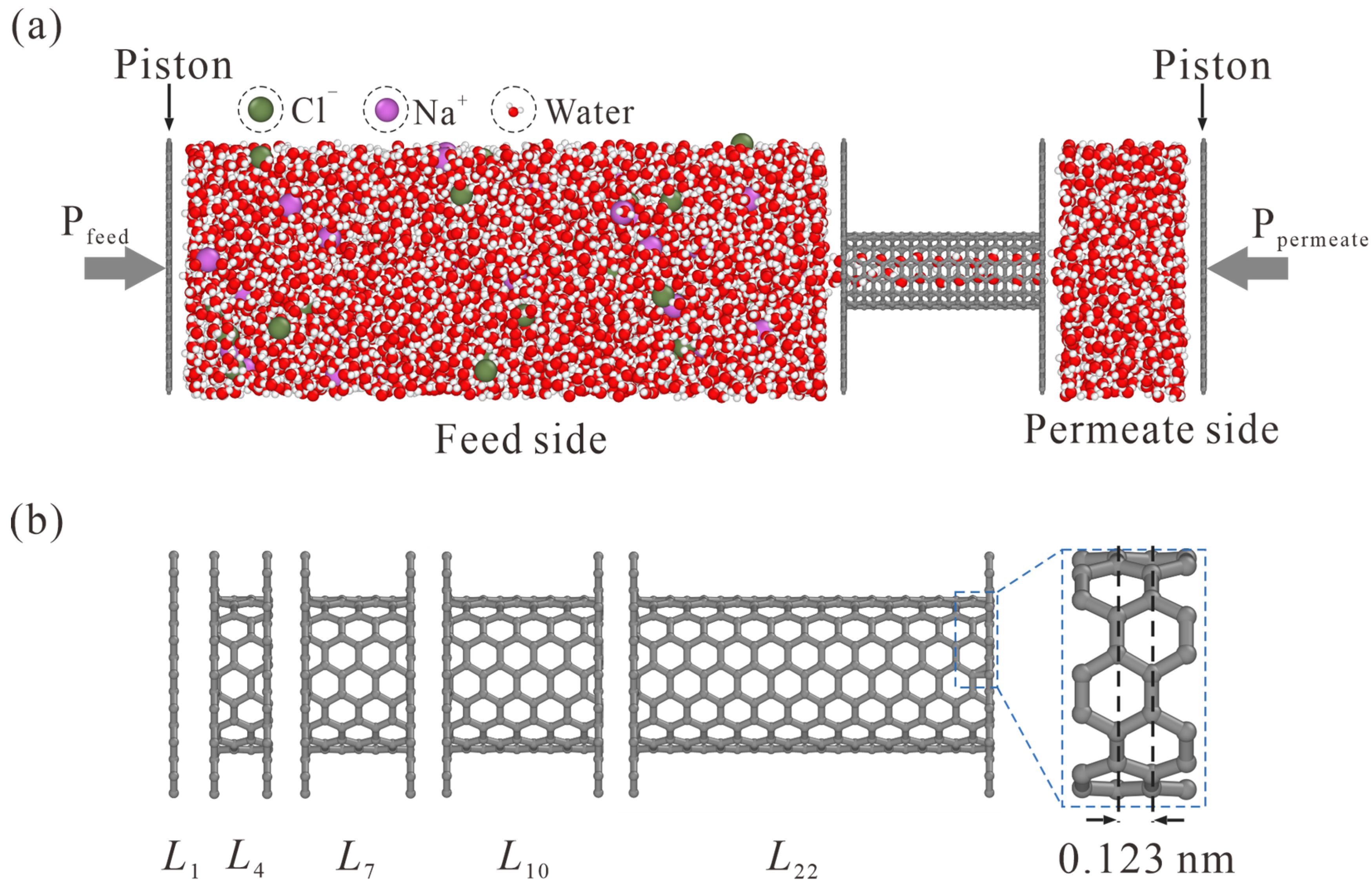
2. Simulation Details
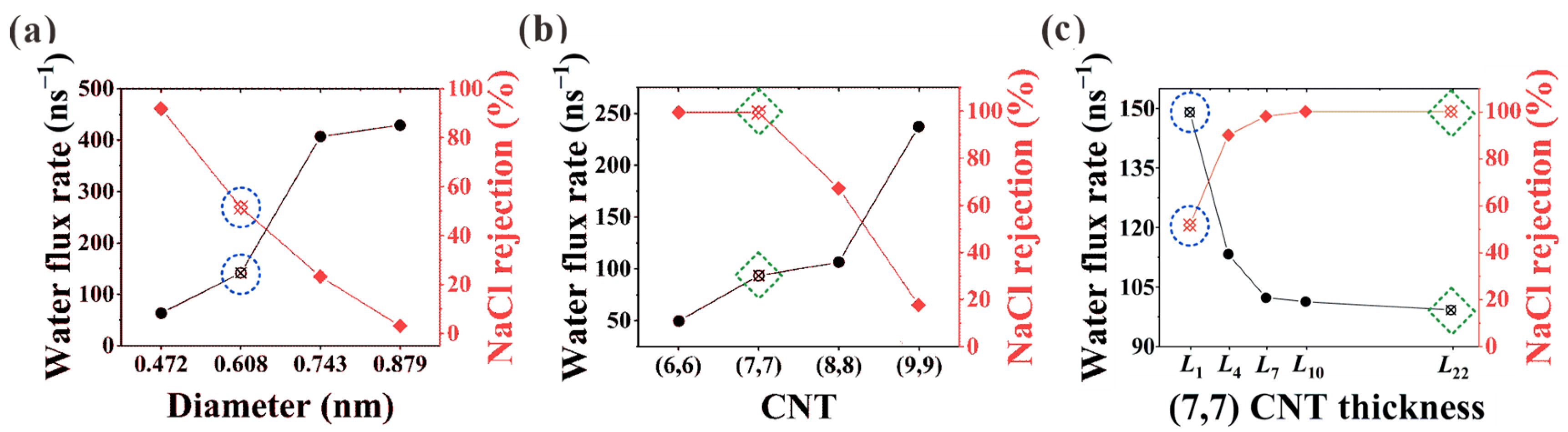
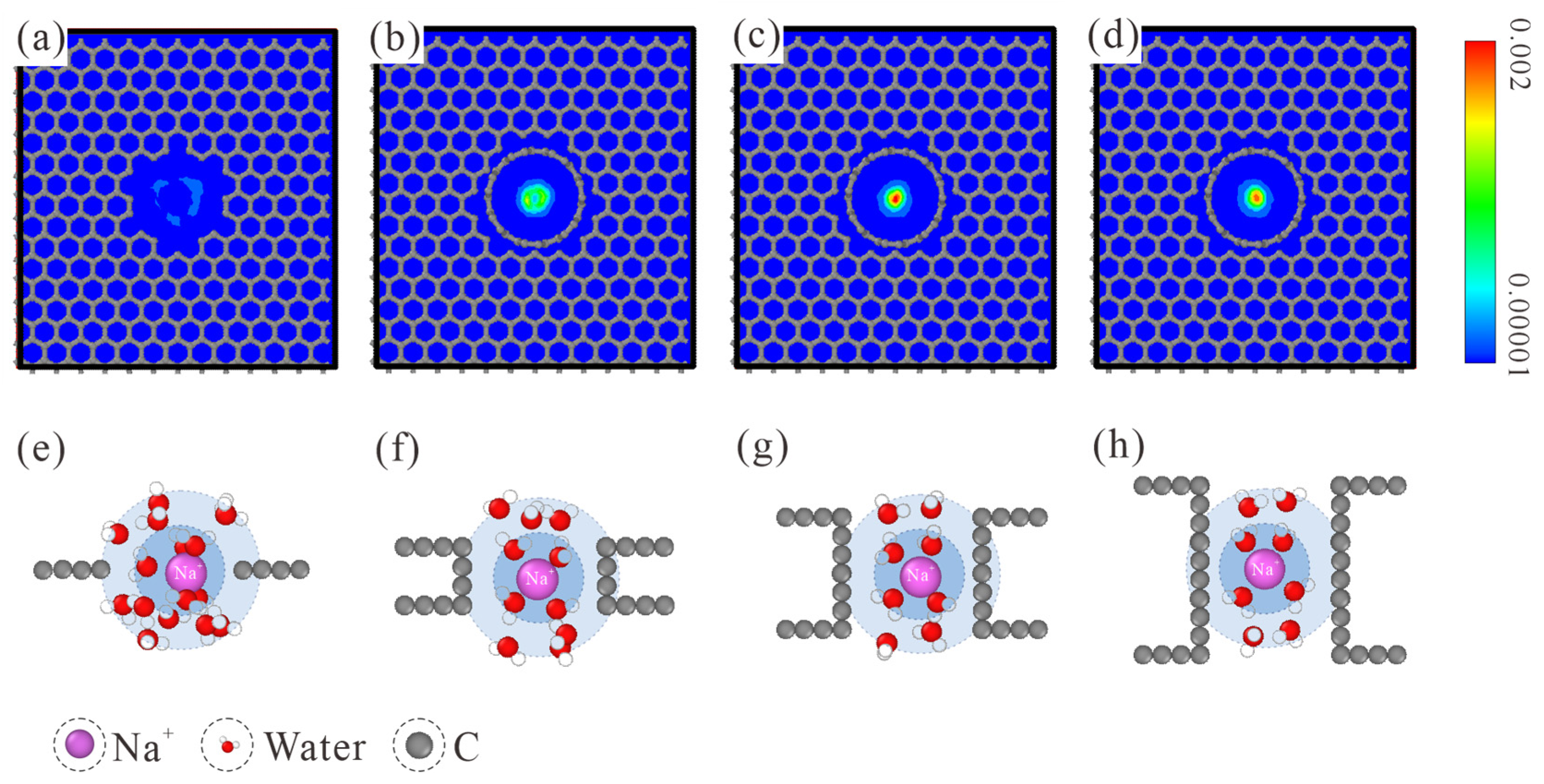
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Raza, A.; Hassan, J.Z.; Mahmood, A.; Nabgan, W.; Ikram, M. Recent Advances in Membrane-Enabled Water Desalination by 2D Frameworks: Graphene and Beyond. Desalination 2022, 531, 115684. [Google Scholar] [CrossRef]
- Werber, J.R.; Osuji, C.O.; Elimelech, M. Materials for Next-Generation Desalination and Water Purification Membranes. Nat. Rev. Mater. 2016, 1, 16018. [Google Scholar] [CrossRef]
- Elimelech, M.; Phillip, W.A. The Future of Seawater Desalination: Energy, Technology, and the Environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef] [PubMed]
- Geise, G.M.; Park, H.B.; Sagle, A.C.; Freeman, B.D.; McGrath, J.E. Water Permeability and Water/Salt Selectivity Tradeoff in Polymers for Desalination. J. Membr. Sci. 2011, 369, 130–138. [Google Scholar] [CrossRef]
- Ghaffour, N.; Missimer, T.M.; Amy, G.L. Technical Review and Evaluation of the Economics of Water Desalination: Current and Future Challenges for Better Water Supply Sustainability. Desalination 2013, 309, 197–207. [Google Scholar] [CrossRef]
- Lim, Y.J.; Goh, K.; Kurihara, M.; Wang, R. Seawater Desalination by Reverse Osmosis: Current Development and Future Challenges in Membrane Fabrication—A Review. J. Membr. Sci. 2021, 629, 119292. [Google Scholar] [CrossRef]
- Boretti, A.; Al-Zubaidy, S.; Vaclavikova, M.; Al-Abri, M.; Castelletto, S.; Mikhalovsky, S. Outlook for Graphene-Based Desalination Membranes. npj Clean Water 2018, 1, 5. [Google Scholar] [CrossRef]
- Liu, G.; Jin, W.; Xu, N. Graphene-Based Membranes. Chem. Soc. Rev. 2015, 44, 5016–5030. [Google Scholar] [CrossRef]
- Foller, T.; Wang, H.; Joshi, R. Rise of 2D Materials-Based Membranes for Desalination. Desalination 2022, 536, 115851. [Google Scholar] [CrossRef]
- Cohen-Tanugi, D.; Grossman, J.C. Water Desalination across Nanoporous Graphene. Nano Lett. 2012, 12, 3602–3608. [Google Scholar] [CrossRef]
- Suk, M.E.; Aluru, N.R. Water Transport through Ultrathin Graphene. J. Phys. Chem. Lett. 2010, 1, 1590–1594. [Google Scholar] [CrossRef]
- Li, Y.; Yu, Y.; Qian, J.; Wu, H.; Wang, F. Anomalous Ion Transport through Angstrom-Scale Pores: Effect of Hydration Shell Exchange on Ion Mobility. Appl. Surf. Sci. 2021, 560, 150022. [Google Scholar] [CrossRef]
- Hummer, G.; Rasaiah, J.C.; Noworyta, J.P. Water Conduction through the Hydrophobic Channel of a Carbon Nanotube. Nature 2001, 414, 188–190. [Google Scholar] [CrossRef]
- Corry, B. Designing Carbon Nanotube Membranes for Efficient Water Desalination. J. Phys. Chem. B 2008, 112, 1427–1434. [Google Scholar] [CrossRef] [PubMed]
- Corry, B. Water and Ion Transport through Functionalised Carbon Nanotubes: Implications for Desalination Technology. Energy Environ. Sci. 2011, 4, 751. [Google Scholar] [CrossRef]
- Borg, M.K.; Lockerby, D.A.; Ritos, K.; Reese, J.M. Multiscale Simulation of Water Flow through Laboratory-Scale Nanotube Membranes. J. Membr. Sci. 2018, 567, 115–126. [Google Scholar] [CrossRef]
- Nair, R.R.; Wu, H.A.; Jayaram, P.N.; Grigorieva, I.V.; Geim, A.K. Unimpeded Permeation of Water Through Helium-Leak–Tight Graphene-Based Membranes. Science 2012, 335, 442–444. [Google Scholar] [CrossRef]
- Joshi, R.K.; Carbone, P.; Wang, F.C.; Kravets, V.G.; Su, Y.; Grigorieva, I.V.; Wu, H.A.; Geim, A.K.; Nair, R.R. Precise and Ultrafast Molecular Sieving Through Graphene Oxide Membranes. Science 2014, 343, 752–754. [Google Scholar] [CrossRef]
- Esfandiar, A.; Radha, B.; Wang, F.C.; Yang, Q.; Hu, S.; Garaj, S.; Nair, R.R.; Geim, A.K.; Gopinadhan, K. Size Effect in Ion Transport through Angstrom-Scale Slits. Science 2017, 358, 511–513. [Google Scholar] [CrossRef]
- Zhou, K.; Xu, Z. Renormalization of Ionic Solvation Shells in Nanochannels. ACS Appl. Mater. Interfaces 2018, 10, 27801–27809. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, S.; Mi, B.; Wang, J.; Ding, J. Surface Slip on Rotating Graphene Membrane Enables the Temporal Selectivity That Breaks the Permeability-Selectivity Trade-Off. Sci. Adv. 2020, 6, eaba9471. [Google Scholar] [CrossRef]
- Zhang, X.; Wei, M.; Xu, F.; Wang, Y. Thickness-Dependent Ion Rejection in Nanopores. J. Membr. Sci. 2020, 601, 117899. [Google Scholar] [CrossRef]
- Gong, X.; Li, J.; Xu, K.; Wang, J.; Yang, H. A Controllable Molecular Sieve for Na+ and K+ Ions. J. Am. Chem. Soc. 2010, 132, 1873–1877. [Google Scholar] [CrossRef]
- Shao, Q.; Huang, L.; Zhou, J.; Lu, L.; Zhang, L.; Lu, X.; Jiang, S.; Gubbins, K.E.; Shen, W. Molecular Simulation Study of Temperature Effect on Ionic Hydration in Carbon Nanotubes. Phys. Chem. Chem. Phys. 2008, 10, 1896–1906. [Google Scholar] [CrossRef] [PubMed]
- Xue, M.; Qiu, H.; Shen, C.; Zhang, Z.; Guo, W. Ion Hydration under Nanoscale Confinement: Dimensionality and Scale Effects. J. Phys. Chem. Lett. 2022, 13, 4815–4822. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.; Grigera, J.R.; Straatsma, T.P. The Missing Term in Effective Pair Potentials. J. Phys. Chem. 1987, 91, 6269–6271. [Google Scholar] [CrossRef]
- Shao, Q.; Zhou, J.; Lu, L.; Lu, X.; Zhu, Y.; Jiang, S. Anomalous Hydration Shell Order of Na+ and K+ inside Carbon Nanotubes. Nano Lett. 2009, 9, 989–994. [Google Scholar] [CrossRef]
- Hockney, R.W.; Eastwood, J.W. Computer Simulation Using Particles; CRC Press: Boca Raton, FL, USA, 2021. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Zhang, X.; Wei, M.; Xu, F.; Wang, Y. Pressure-Dependent Ion Rejection in Nanopores. J. Phys. Chem. C 2020, 124, 20498–20505. [Google Scholar] [CrossRef]
- Zhu, F.; Tajkhorshid, E.; Schulten, K. Pressure-Induced Water Transport in Membrane Channels Studied by Molecular Dynamics. Biophys. J. 2002, 83, 154–160. [Google Scholar] [CrossRef]
- Wang, L.; Wu, H.; Wang, F. Water Desalination Using Nano Screw Pumps with a Considerable Processing Rate. RSC Adv. 2017, 7, 20360–20368. [Google Scholar] [CrossRef]
- Zhou, K.; Qian, C.; Liu, Y. Quantifying the Structure of Water and Hydrated Monovalent Ions by Density Functional Theory-Based Molecular Dynamics. J. Phys. Chem. B 2022, 126, 10471–10480. [Google Scholar] [CrossRef] [PubMed]
- Galib, M.; Baer, M.D.; Skinner, L.B.; Mundy, C.J.; Huthwelker, T.; Schenter, G.K.; Benmore, C.J.; Govind, N.; Fulton, J.L. Revisiting the Hydration Structure of Aqueous Na+. J. Chem. Phys. 2017, 146, 084504. [Google Scholar] [CrossRef] [PubMed]
- White, J.A.; Schwegler, E.; Galli, G.; Gygi, F. The Solvation of Na+ in Water: First-Principles Simulations. J. Chem. Phys. 2000, 113, 4668–4673. [Google Scholar] [CrossRef]
- He, Z.; Zhou, J.; Lu, X.; Corry, B. Ice-like Water Structure in Carbon Nanotube (8,8) Induces Cationic Hydration Enhancement. J. Phys. Chem. C 2013, 117, 11412–11420. [Google Scholar] [CrossRef]
- Thomas, M.; Corry, B. A Computational Assessment of the Permeability and Salt Rejection of Carbon Nanotube Membranes and Their Application to Water Desalination. Phil. Trans. R. Soc. A 2016, 374, 20150020. [Google Scholar] [CrossRef] [PubMed]

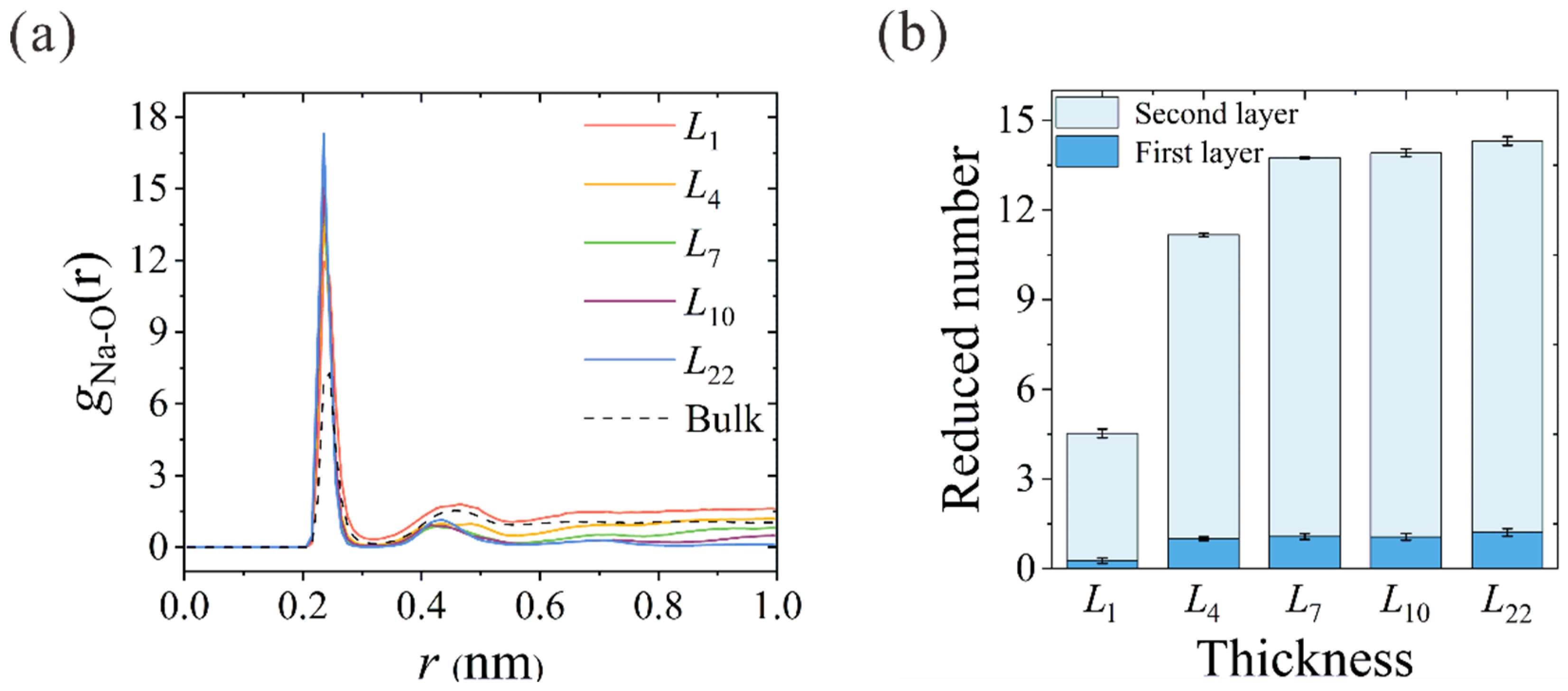

| Bulk | L1 | L4 | L7 | L10 | L22 | |
|---|---|---|---|---|---|---|
| Nc1 | 5.6 | 5.2 | 4.5 | 4.4 | 4.4 | 4.3 |
| Nc2 | 17.1 | 12.9 | 7.0 | 4.4 | 4.3 | 4.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Wang, L.; Xia, J.; Wang, R.; Tang, C.; Wang, C. Competition between Hydration Shell and Ordered Water Chain Induces Thickness-Dependent Desalination Performance in Carbon Nanotube Membrane. Membranes 2023, 13, 525. https://doi.org/10.3390/membranes13050525
Liu S, Wang L, Xia J, Wang R, Tang C, Wang C. Competition between Hydration Shell and Ordered Water Chain Induces Thickness-Dependent Desalination Performance in Carbon Nanotube Membrane. Membranes. 2023; 13(5):525. https://doi.org/10.3390/membranes13050525
Chicago/Turabian StyleLiu, Siyi, Liya Wang, Jun Xia, Ruijie Wang, Chun Tang, and Chengyuan Wang. 2023. "Competition between Hydration Shell and Ordered Water Chain Induces Thickness-Dependent Desalination Performance in Carbon Nanotube Membrane" Membranes 13, no. 5: 525. https://doi.org/10.3390/membranes13050525
APA StyleLiu, S., Wang, L., Xia, J., Wang, R., Tang, C., & Wang, C. (2023). Competition between Hydration Shell and Ordered Water Chain Induces Thickness-Dependent Desalination Performance in Carbon Nanotube Membrane. Membranes, 13(5), 525. https://doi.org/10.3390/membranes13050525






