Interplay between Forced Convection and Electroconvection during the Overlimiting Ion Transport through Anion-Exchange Membranes: A Fourier Transform Analysis of Membrane Voltage Drops
Abstract
1. Introduction
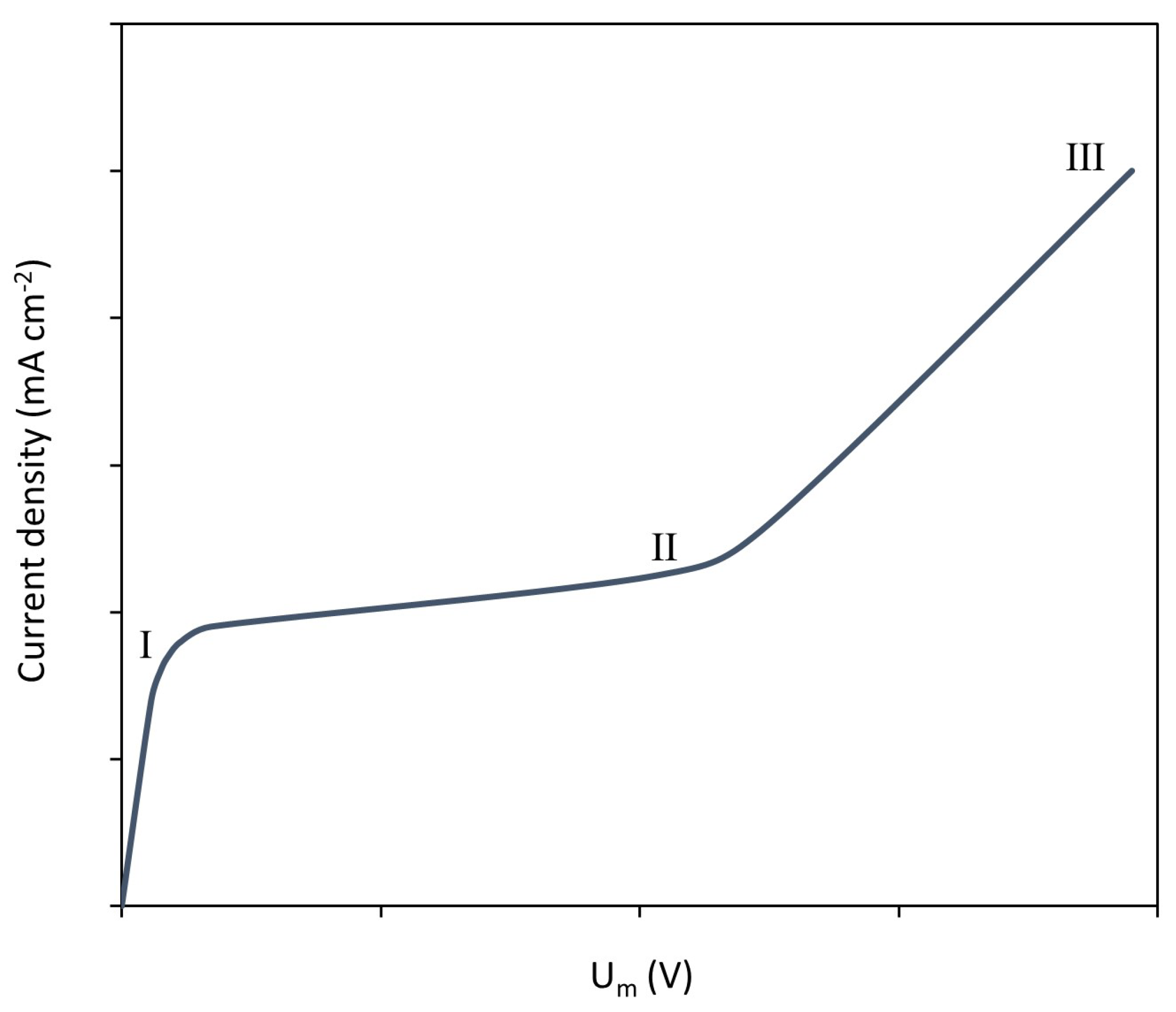
- Region I (quasi-ohmic): the current density increases linearly with the voltage until is reached. In this region, ion flux is proportional to the applied electric field.
- Region II (plateau): in this region, an increase in the applied voltage implies a subtle or almost absent increase in current density. The scarcity of ions near the diluted membrane surface is the main cause for larger membrane resistances.
- Region III (overlimiting): if the applied voltage is further increased, transport phenomena other than diffusion and migration arise, and current densities above can be registered.
2. Materials and Methods
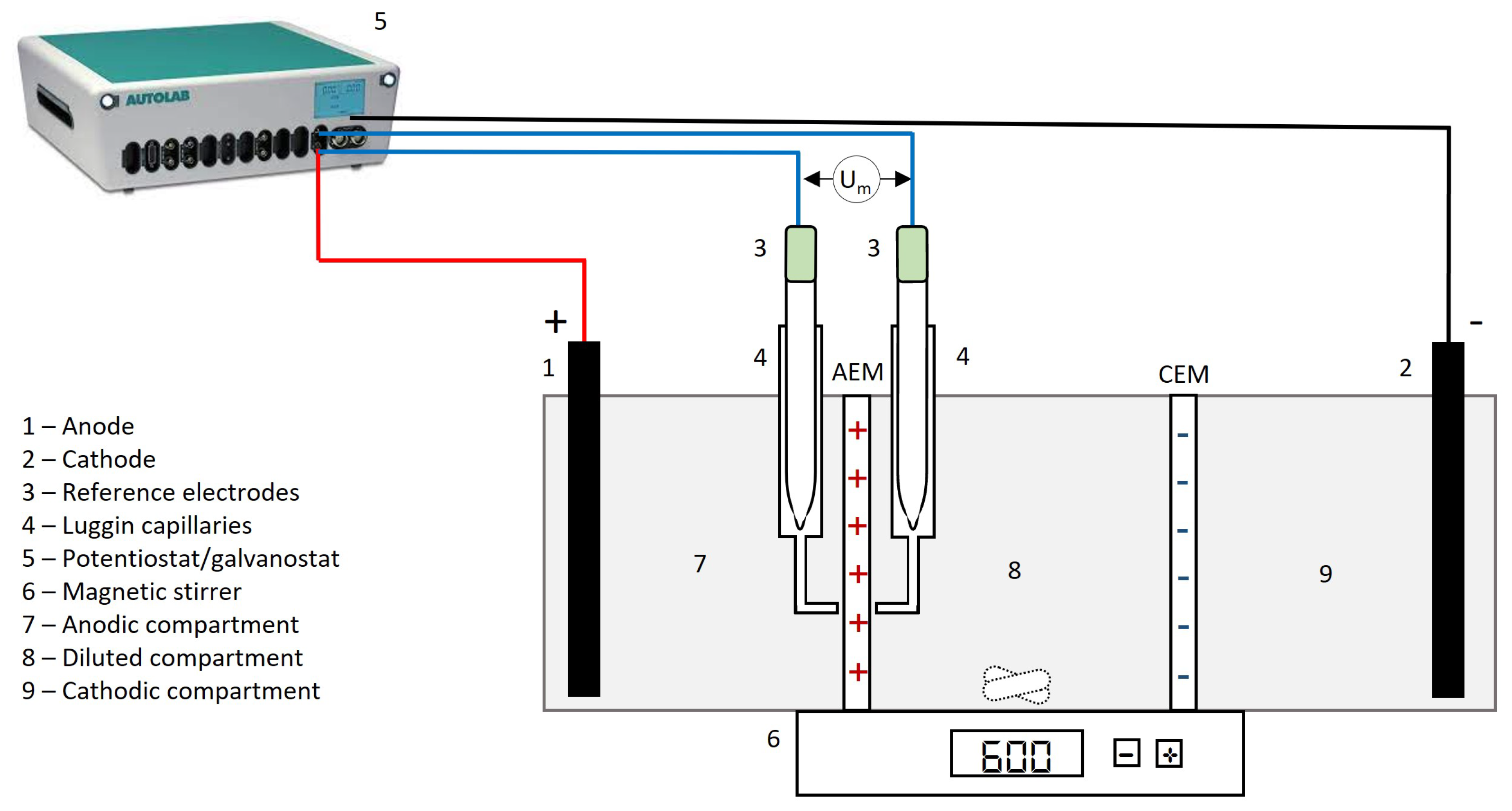
2.1. Membranes and Electrodialysis Cell
2.2. Electrochemical Characterization of the Membrane-Electrolyte Systems
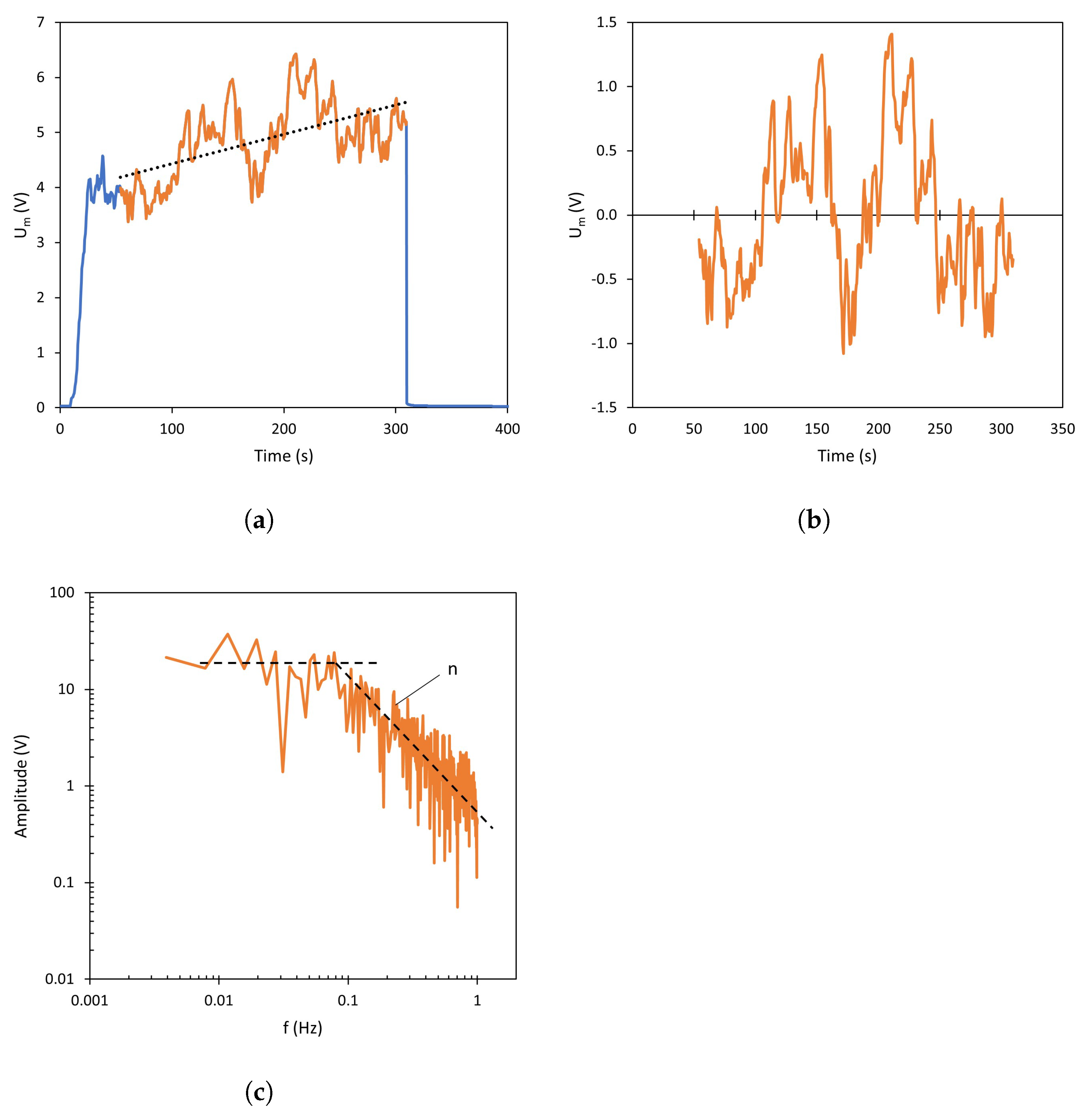
2.3. Treatment of Chronopotentiometric Data and Fourier Transform Analysis
3. Results and Discussion
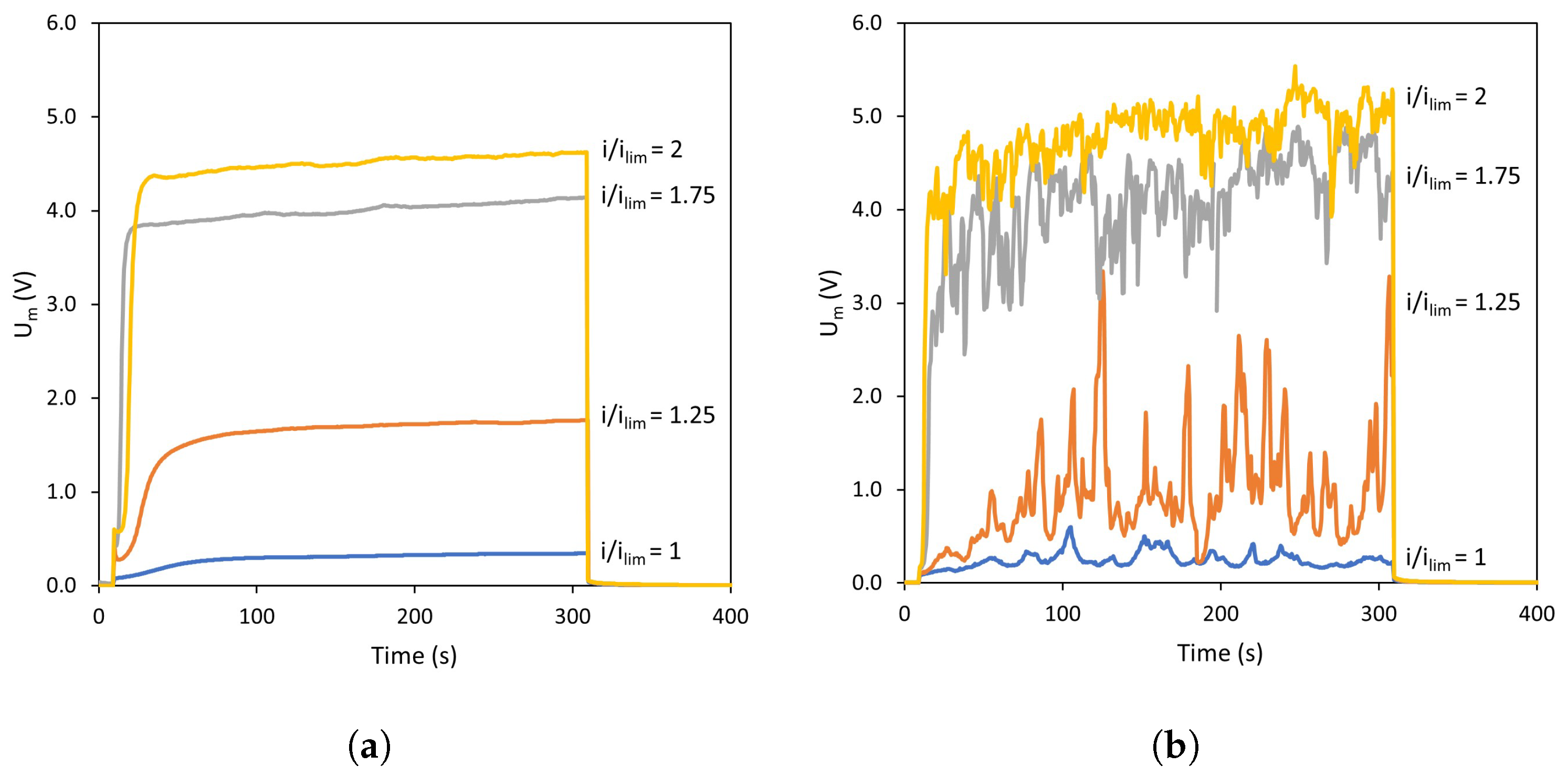
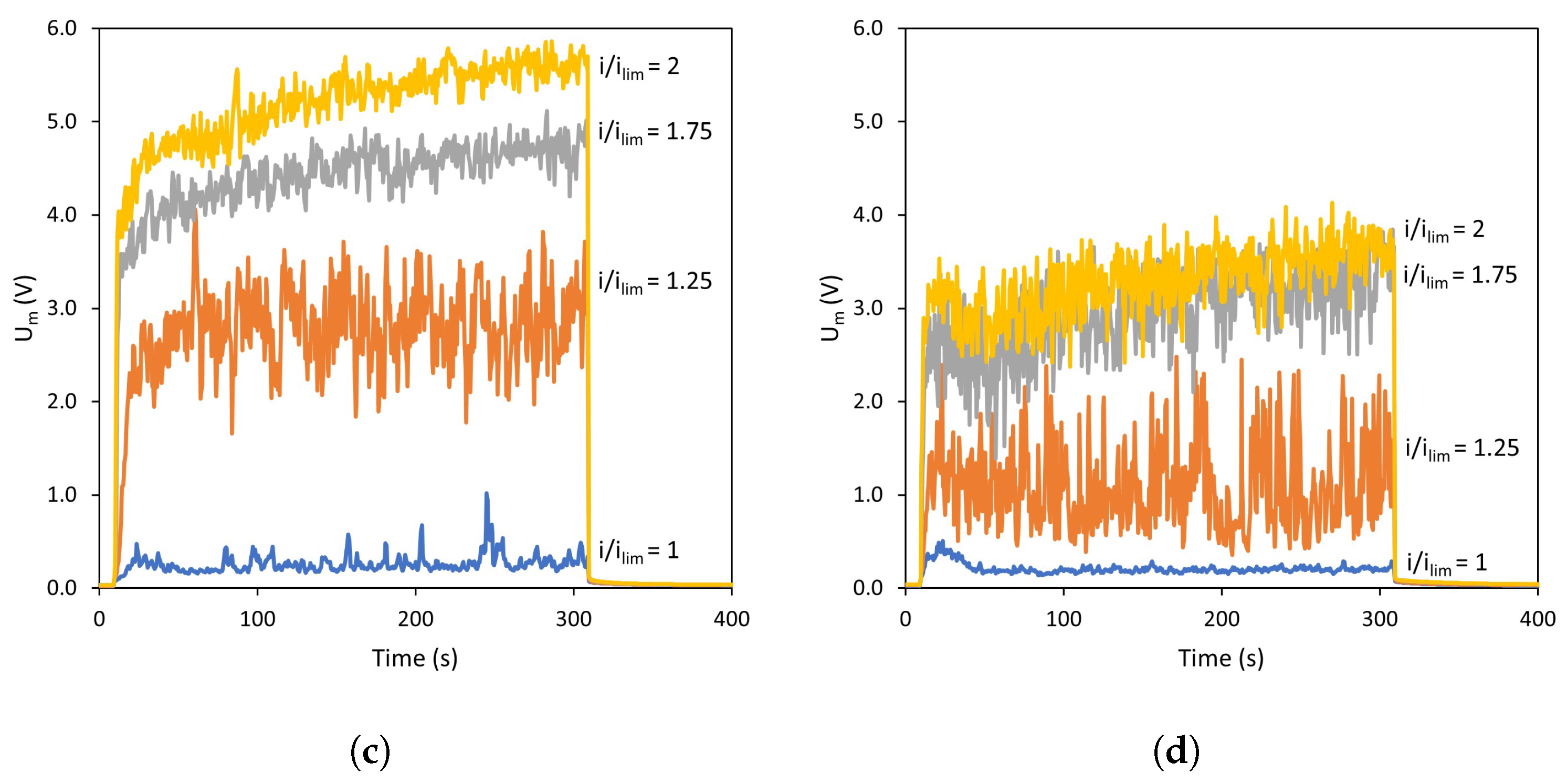
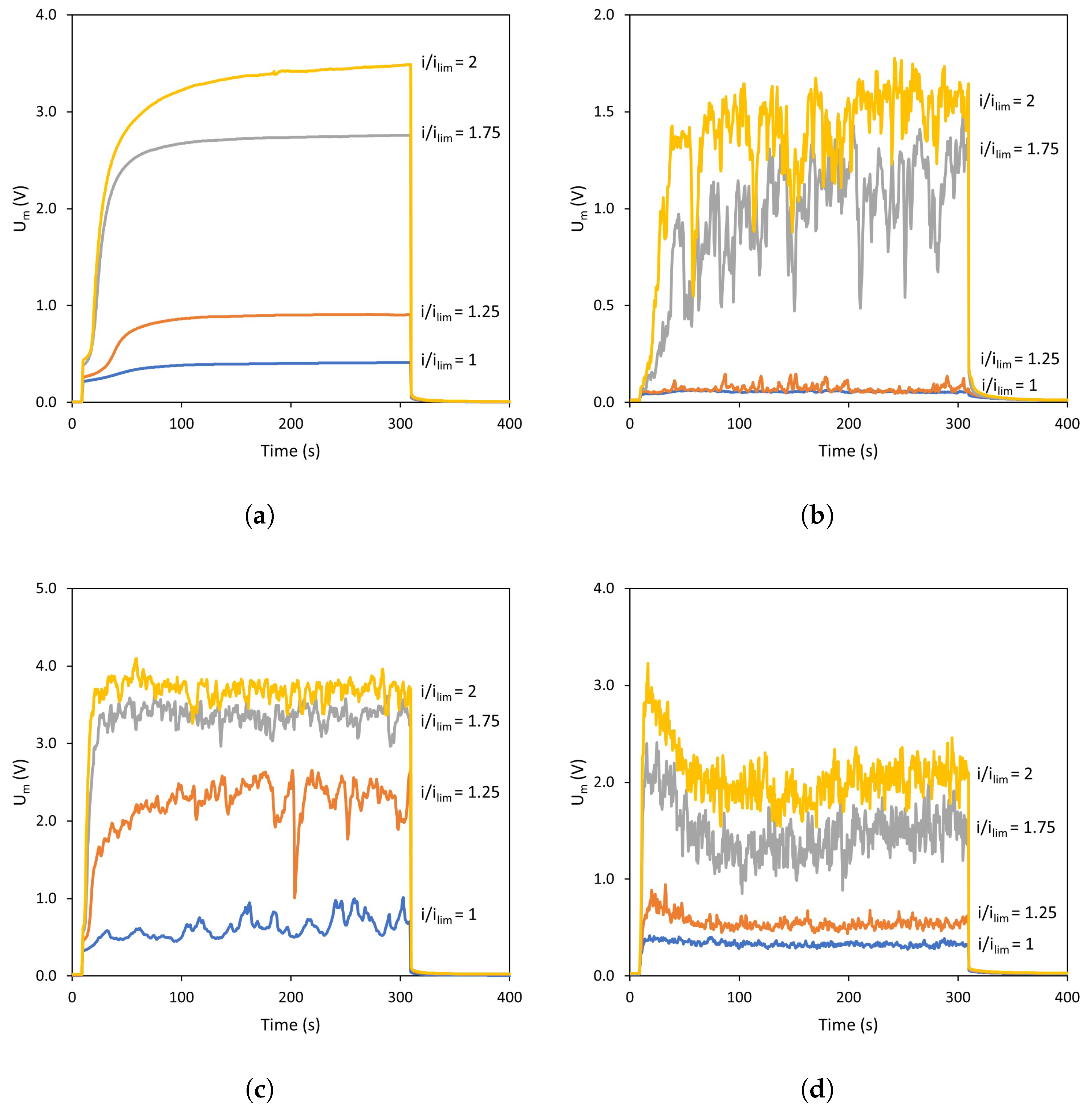
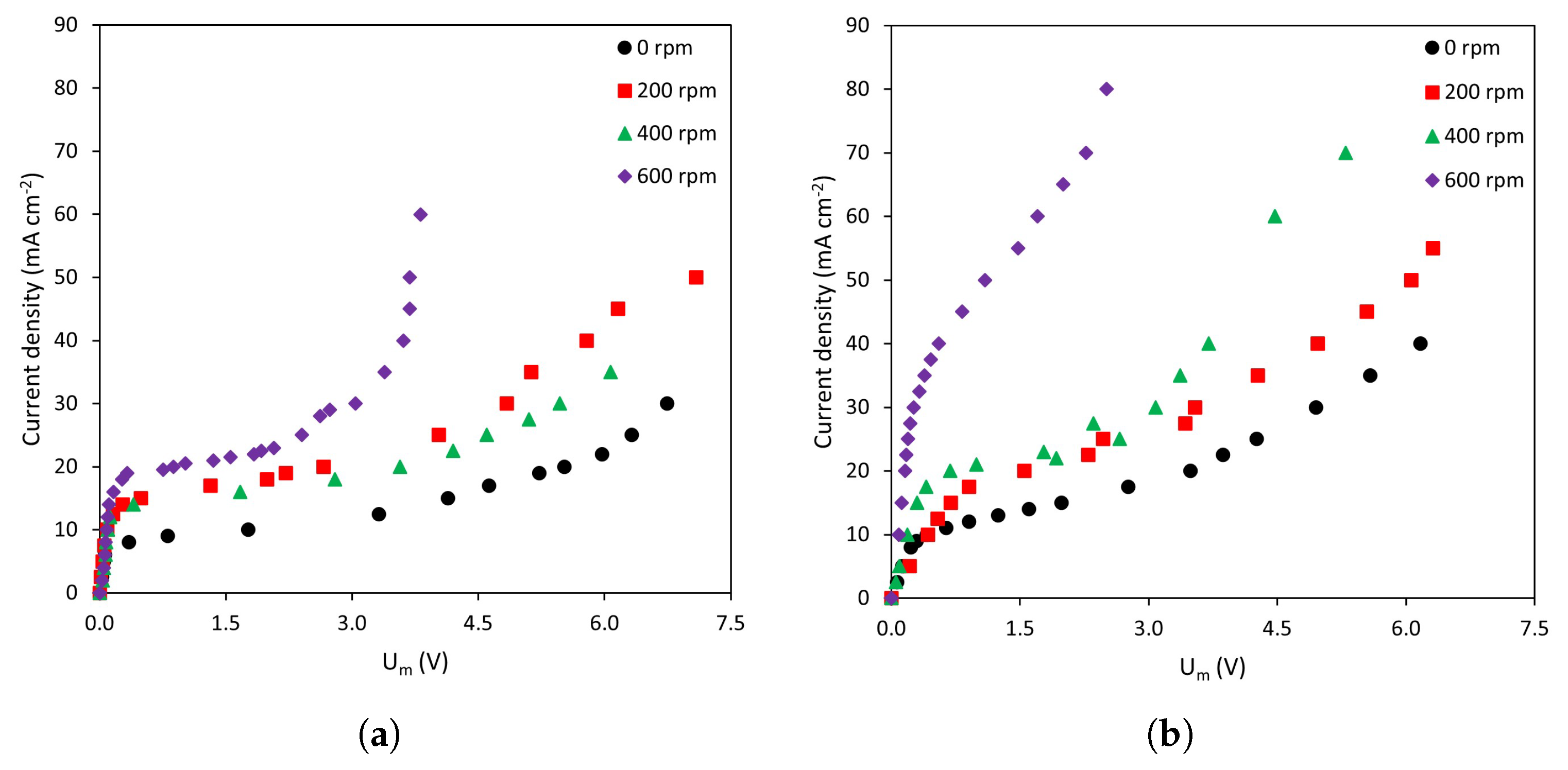
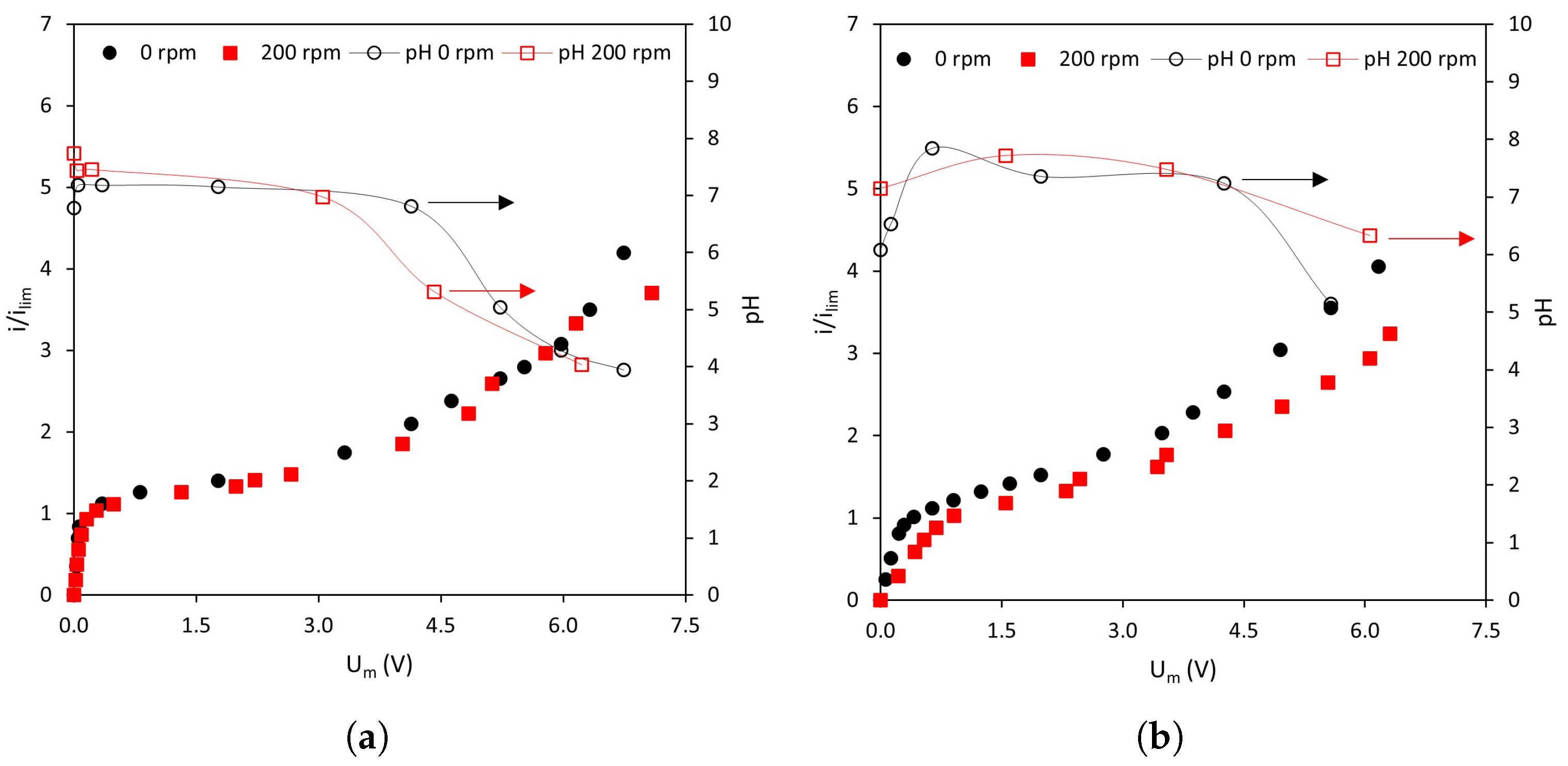
3.1. Effect of Forced Convection, Applied Current Density and Membrane Type on the Current–Voltage Characteristics: and
3.2. Amplitude of Membrane Voltage Oscillations
3.3. FT Analysis of Membrane Voltage Signals
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ED | Electrodialysis |
| FT | Fourier Transform |
| FFT | Fast Fourier Transform |
Appendix A
References
- Gurreri, L.; Tamburini, A.; Cipollina, A.; Micale, G. Electrodialysis applications in wastewater treatment for environmental protection and resources recovery: A systematic review on progress and perspectives. Membranes 2020, 10, 146. [Google Scholar] [CrossRef] [PubMed]
- Martí-Calatayud, M.C.; Buzzi, D.C.; García-Gabaldón, M.; Bernardes, A.M.; Tenório, J.A.S.; Pérez-Herranz, V. Ion transport through homogeneous and heterogeneous ion-exchange membranes in single salt and multicomponent electrolyte solutions. J. Membr. Sci. 2014, 466, 45–57. [Google Scholar] [CrossRef]
- Luiz, A.; Spencer, E.; McClure, D.D.; Coster, H.G.; Barton, G.W.; Kavanagh, J.M. Membrane selection for the desalination of bio-refinery effluents using electrodialysis. Desalination 2018, 428, 1–11. [Google Scholar] [CrossRef]
- Mandal, P.; Mondal, R.; Goel, P.; Bhuvanesh, E.; Chatterjee, U.; Chattopadhyay, S. Selective recovery of carboxylic acid through PVDF blended anion exchange membranes using electrodialysis. Sep. Purif. Technol. 2022, 292, 121069. [Google Scholar] [CrossRef]
- Roghmans, F.; Evdochenko, E.; Martí-Calatayud, M.C.; Garthe, M.; Tiwari, R.; Walther, A.; Wessling, M. On the permselectivity of cation-exchange membranes bearing an ion selective coating. J. Membr. Sci. 2020, 600, 117854. [Google Scholar] [CrossRef]
- Martí-Calatayud, M.C.; Sancho-Cirer Poczatek, M.; Pérez-Herranz, V. Trade-off between operating time and energy consumption in pulsed electric field electrodialysis: A comprehensive simulation study. Membranes 2021, 11, 43. [Google Scholar] [CrossRef]
- Moya, A.A.; Belashova, E.; Sistat, P. Numerical simulation of linear sweep and large amplitude ac voltammetries of ion-exchange membrane systems. J. Membr. Sci. 2015, 474, 215–223. [Google Scholar] [CrossRef]
- Uzdenova, A.; Kovalenko, A.; Urtenov, M.; Nikonenko, V. 1D mathematical modelling of non-stationary ion transfer in the diffusion layer adjacent to an ion-exchange membrane in galvanostatic mode. Membranes 2018, 8, 84. [Google Scholar] [CrossRef]
- Kovalenko, A.; Wessling, M.; Nikonenko, V.; Mareev, S.; Moroz, I.; Evdochenko, E.; Urtenov, M.K. Space-charge breakdown phenomenon and spatio-temporal ion concentration and fluid flow patterns in overlimiting current electrodialysis. J. Membr. Sci. 2021, 636, 119583. [Google Scholar] [CrossRef]
- Barros, K.S.; Martí-Calatayud, M.C.; Scarazzato, T.; Bernardes, A.M.; Espinosa, D.C.R.; Pérez-Herranz, V. Investigation of ion-exchange membranes by means of chronopotentiometry: A comprehensive review on this highly informative and multipurpose technique. Adv. Colloid Interface Sci. 2021, 293, 102439. [Google Scholar] [CrossRef]
- Balster, J.; Yildirim, M.; Stamatialis, D.; Ibanez, R.; Lammertink, R.; Jordan, V.; Wessling, M. Morphology and microtopology of cation-exchange polymers and the origin of the overlimiting current. J. Phys. Chem. B 2007, 111, 2152–2165. [Google Scholar] [CrossRef] [PubMed]
- Akberova, E.M.; Vasil’eva, V.I.; Zabolotsky, V.I.; Novak, L. Effect of the sulfocation-exchanger dispersity on the surface morphology, microrelief of heterogeneous membranes and development of electroconvection in intense current modes. J. Membr. Sci. 2018, 566, 317–328. [Google Scholar] [CrossRef]
- Gil, V.; Porozhnyy, M.; Rybalkina, O.; Butylskii, D.; Pismenskaya, N. The development of electroconvection at the surface of a heterogeneous cation-exchange membrane modified with perfluorosulfonic acid polymer film containing titanium oxide. Membranes 2020, 10, 125. [Google Scholar] [CrossRef]
- Butylskii, D.; Moroz, I.; Tsygurina, K.; Mareev, S. Effect of surface inhomogeneity of ion-exchange membranes on the mass transfer efficiency in pulsed electric field modes. Membranes 2020, 10, 40. [Google Scholar] [CrossRef]
- Choi, J.H.; Lee, H.J.; Moon, S.H. Effects of electrolytes on the transport phenomena in a cation-exchange membrane. J. Colloid Interface Sci. 2001, 238, 188–195. [Google Scholar] [CrossRef] [PubMed]
- Martí-Calatayud, M.C.; García-Gabaldón, M.; Pérez-Herranz, V. Study of the effects of the applied current regime and the concentration of chromic acid on the transport of Ni2+ ions through Nafion 117 membranes. J. Membr. Sci. 2012, 392–393, 137–149. [Google Scholar] [CrossRef]
- Uzdenova, A. 2D mathematical modelling of overlimiting transfer enhanced by electroconvection in flow-through electrodialysis membrane cells in galvanodynamic mode. Membranes 2019, 9, 39. [Google Scholar] [CrossRef] [PubMed]
- Seo, M.; Kim, W.; Lee, H.; Kim, S.J. Non-negligible effects of reinforcing structures inside ion exchange membrane on stabilization of electroconvective vortices. Desalination 2022, 538, 115902. [Google Scholar] [CrossRef]
- Liang, Y.; Fimbres Weihs, G.; Fletcher, D. CFD study of the effect of unsteady slip velocity waveform on shear stress in membrane systems. Chem. Eng. Sci. 2018, 192, 16–24. [Google Scholar] [CrossRef]
- Magnico, P. Electro-kinetic instability in a laminar boundary layer next to an ion exchange membrane. Int. J. Mol. Sci. 2019, 20, 2393. [Google Scholar] [CrossRef]
- Urtenov, M.K.; Uzdenova, A.M.; Kovalenko, A.V.; Nikonenko, V.V.; Pismenskaya, N.D.; Vasil’eva, V.I.; Sistat, P.; Pourcelly, G. Basic mathematical model of overlimiting transfer enhanced by electroconvection in flow-through electrodialysis membrane cells. J. Membr. Sci. 2013, 447, 190–202. [Google Scholar] [CrossRef]
- Kwak, R.; Pham, V.S.; Han, J. Sheltering the perturbed vortical layer of electroconvection under shear flow. J. Fluid Mech. 2017, 813, 799–823. [Google Scholar] [CrossRef]
- Martí-Calatayud, M.C.; García-Gabaldón, M.; Pérez-Herranz, V.; Ortega, E. Determination of transport properties of Ni(II) through a Nafion cation-exchange membrane in chromic acid solutions. J. Membr. Sci. 2011, 379, 449–458. [Google Scholar] [CrossRef]
- Martí-Calatayud, M.C.; García-Gabaldón, M.; Pérez-Herranz, V.; Sales, S.; Mestre, S. Synthesis and electrochemical behavior of ceramic cation-exchange membranes based on zirconium phosphate. Ceram. Int. 2013, 39, 4045–4054. [Google Scholar] [CrossRef]
- Krol, J.J.; Wessling, M.; Strathmann, H. Chronopotentiometry and overlimiting ion transport through monopolar ion exchange membranes. J. Membr. Sci. 1999, 162, 155–164. [Google Scholar] [CrossRef]
- Korzhova, E.; Pismenskaya, N.; Lopatin, D.; Baranov, O.; Dammak, L.; Nikonenko, V. Effect of surface hydrophobization on chronopotentiometric behavior of an AMX anion-exchange membrane at overlimiting currents. J. Membr. Sci. 2016, 500, 161–170. [Google Scholar] [CrossRef]
- Akberova, E.M.; Vasil’eva, V.I. Effect of the resin content in cation-exchange membranes on development of electroconvection. Electrochem. Commun. 2020, 111, 106659. [Google Scholar] [CrossRef]
- Pismenskaya, N.D.; Pokhidnia, E.V.; Pourcelly, G.; Nikonenko, V.V. Can the electrochemical performance of heterogeneous ion-exchange membranes be better than that of homogeneous membranes? J. Membr. Sci. 2018, 566, 54–68. [Google Scholar] [CrossRef]
- Choi, J.H.; Kim, S.H.; Moon, S.H. Heterogeneity of ion-exchange membranes: The effects of membrane heterogeneity on transport properties. J. Colloid Interface Sci. 2001, 241, 120–126. [Google Scholar] [CrossRef]
- Zabolotsky, V.I.; Novak, L.; Kovalenko, A.V.; Nikonenko, V.V.; Urtenov, M.H.; Lebedev, K.A.; However, A.Y. Electroconvection in systems with heterogeneous ion-exchange membranes. Pet. Chem. 2017, 57, 779–789. [Google Scholar] [CrossRef]
- Gil, V.V.; Porozhnyy, M.V.; Rybalkina, O.A.; Sabbatovskiy, K.G.; Nikonenko, V.V. Modification of a heterogeneous cation-exchange membrane by Ti-Si based particles to enhance electroconvection and mitigate scaling during electrodialysis. Electrochim. Acta 2021, 391, 138913. [Google Scholar] [CrossRef]
- Roghmans, F.; Evdochenko, E.; Stockmeier, F.; Schneider, S.; Smailji, A.; Tiwari, R.; Mikosch, A.; Karatay, E.; Kühne, A.; Walther, A.; et al. 2D patterned ion-exchange membranes induce electroconvection. Adv. Mater. Interfaces 2019, 6, 1801309. [Google Scholar] [CrossRef]
- Nikonenko, V.V.; Pismenskaya, N.D.; Belova, E.I.; Sistat, P.; Huguet, P.; Pourcelly, G.; Larchet, C. Intensive current transfer in membrane systems: Modelling, mechanisms and application in electrodialysis. Adv. Colloid Interface Sci. 2010, 160, 101–123. [Google Scholar] [CrossRef]
- Butylskii, D.Y.; Troitskiy, V.A.; Ponomar, M.A.; Moroz, I.A.; Sabbatovskiy, K.G.; Sharafan, M.V. Efficient anion-exchange membranes with anti-scaling properties obtained by surface modification of commercial membranes using a polyquaternium-22. Membranes 2022, 12, 1065. [Google Scholar] [CrossRef]
- Rubinstein, S.M.; Manukyan, G.; Staicu, A.; Rubinstein, I.; Zaltzman, B.; Lammertink, R.G.; Mugele, F.; Wessling, M. Direct observation of a nonequilibrium electro-osmotic instability. Phys. Rev. Lett. 2008, 101, 236101. [Google Scholar] [CrossRef] [PubMed]
- Kwak, R.; Pham, V.S.; Lim, K.M.; Han, J. Shear flow of an electrically charged fluid by ion concentration polarization: Scaling laws for electroconvective vortices. Phys. Rev. Lett. 2013, 110, 114501. [Google Scholar] [CrossRef] [PubMed]
- Li, G.; Townsend, A.; Archer, L.A.; Koch, D.L. Suppression of electroconvective and morphological instabilities by an imposed cross flow of the electrolyte. Phys. Rev. Fluids 2021, 6, 33701. [Google Scholar] [CrossRef]
- Lide, D.R. (Ed.) CRC Handbook of Chemistry and Physics, 85th ed.; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Rybalkina, O.A.; Solonchenko, K.V.; Butylskii, D.Y.; Nikonenko, V.V.; Pismenskaya, N.D. Effect of the parameters of pulsed electric fields on the average current density in the electrodialysis desalination of a phosphate-containing solution. Membr. Membr. Technol. 2022, 4, 385–397. [Google Scholar] [CrossRef]
- Gil, V.V.; Andreeva, M.A.; Pismenskaya, N.D.; Nikonenko, V.V.; Larchet, C.; Dammak, L. Effect of counterion hydration numbers on the development of electroconvection at the surface of heterogeneous cation- exchange membrane modified with an MF-4SK film. Pet. Chem. 2016, 56, 440–449. [Google Scholar] [CrossRef]
- Budnikov, E.Y.; Maksimychev, A.V.; Kolyubin, A.V.; Timashev, S.F. Space and time correlations in a dissipative structure emerging in an electrochemical system with a cation-exchange membrane. Russ. J. Electrochem. 2001, 37, 95–103. [Google Scholar] [CrossRef]
- Liu, W.; Zhou, Y.; Shi, P. Critical selection of shear sheltering in electroconvective flow from chaotic to steady state. J. Fluid Mech. 2022, 946, A3. [Google Scholar] [CrossRef]


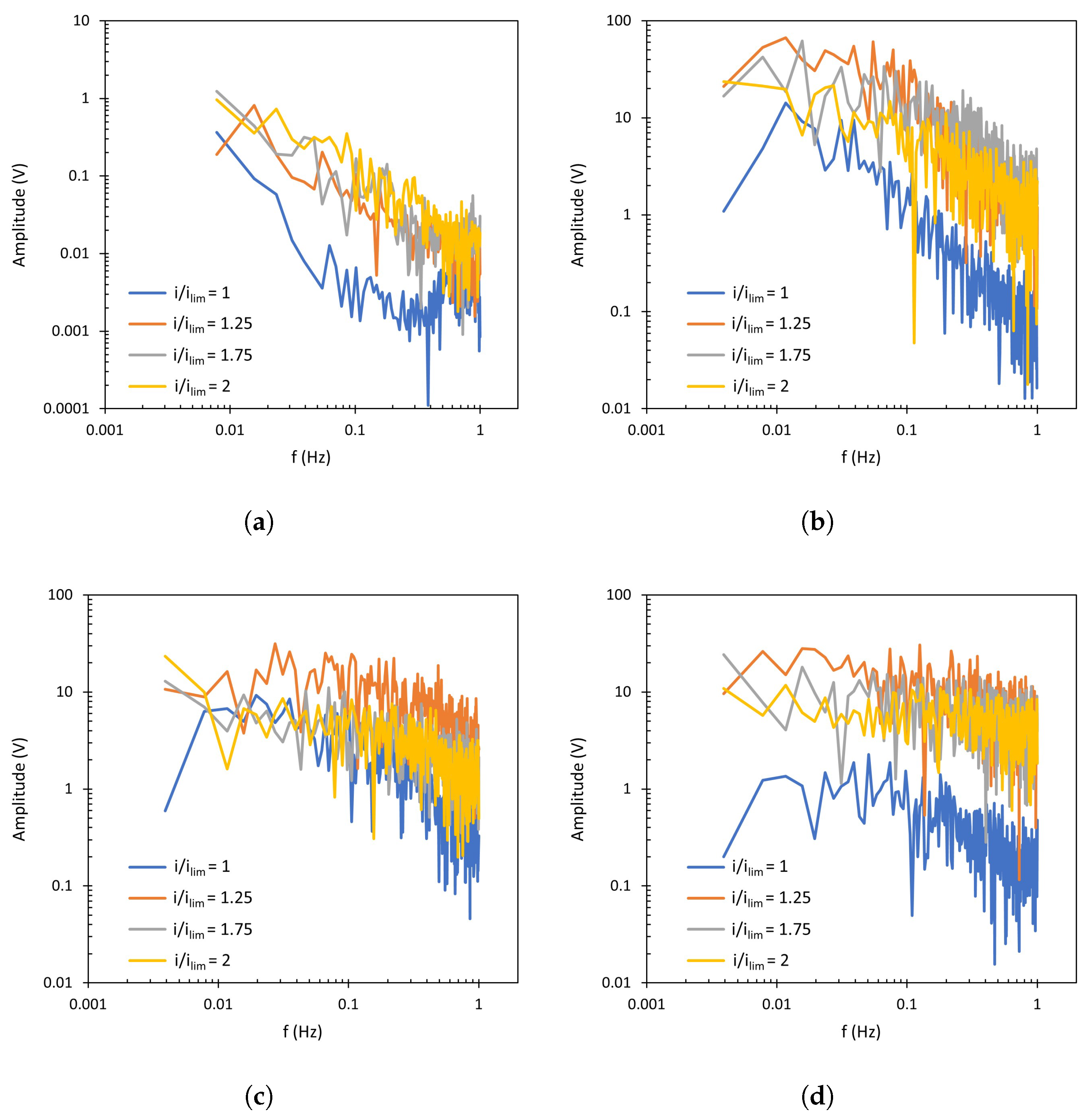
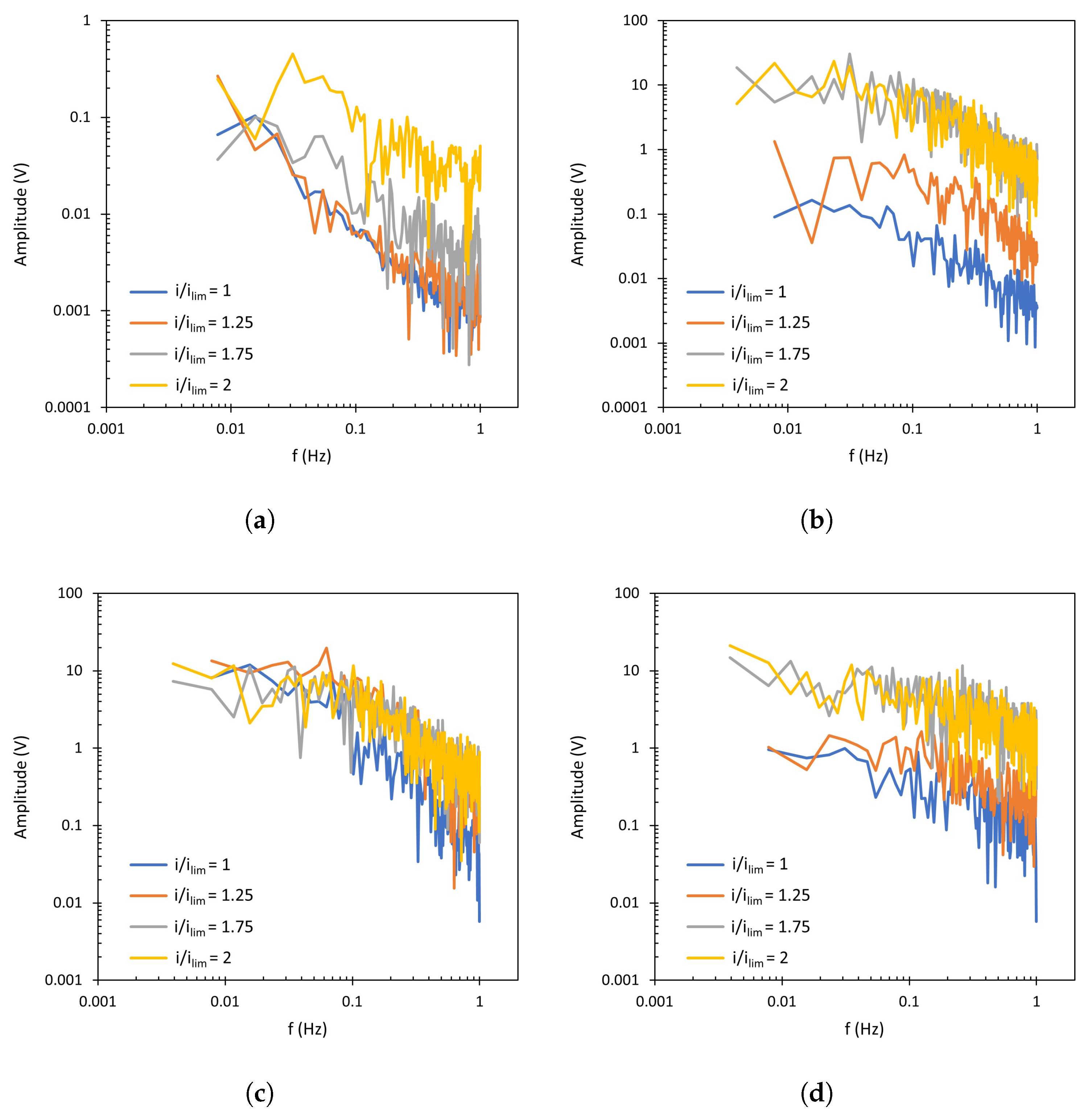
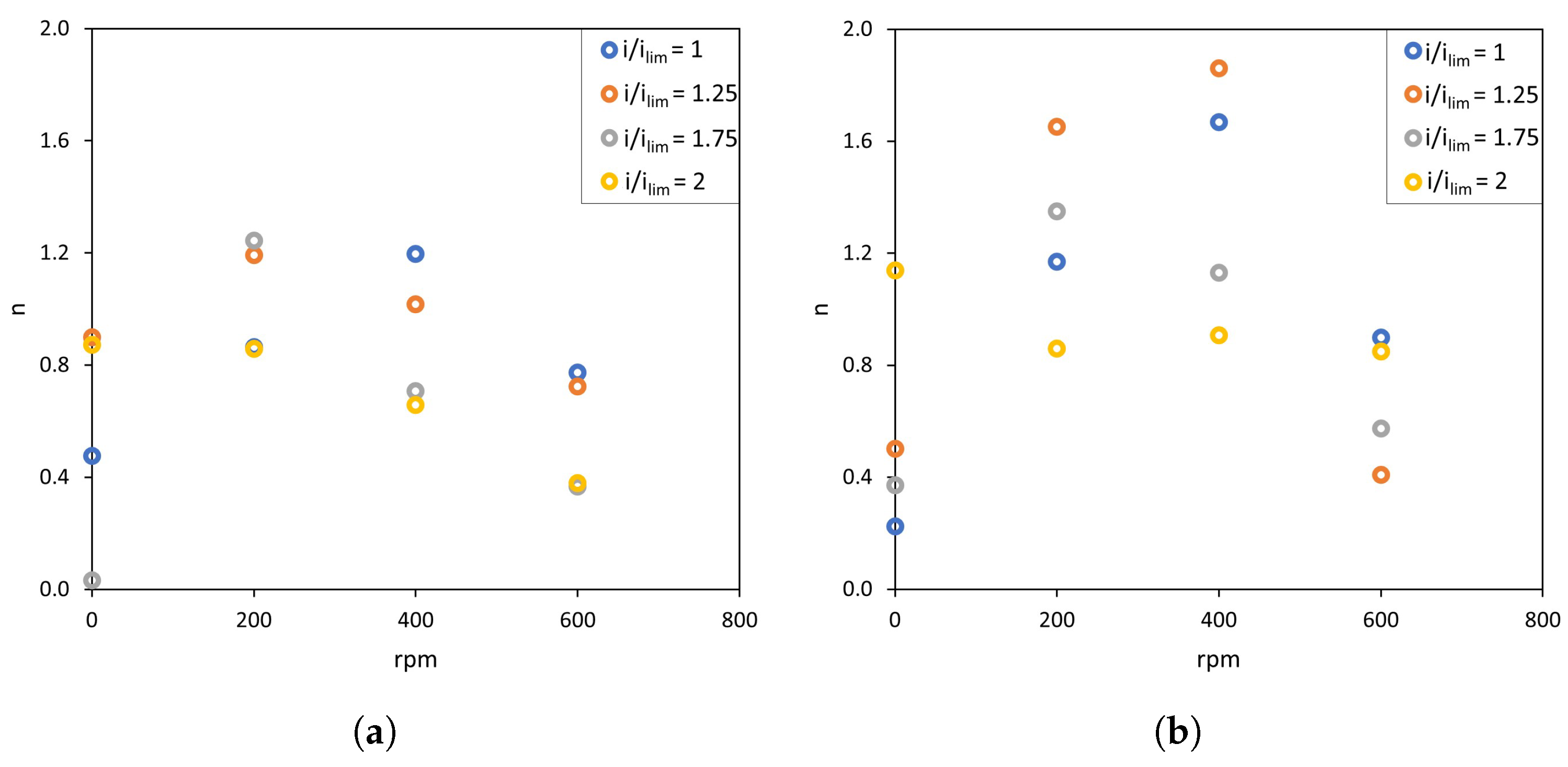
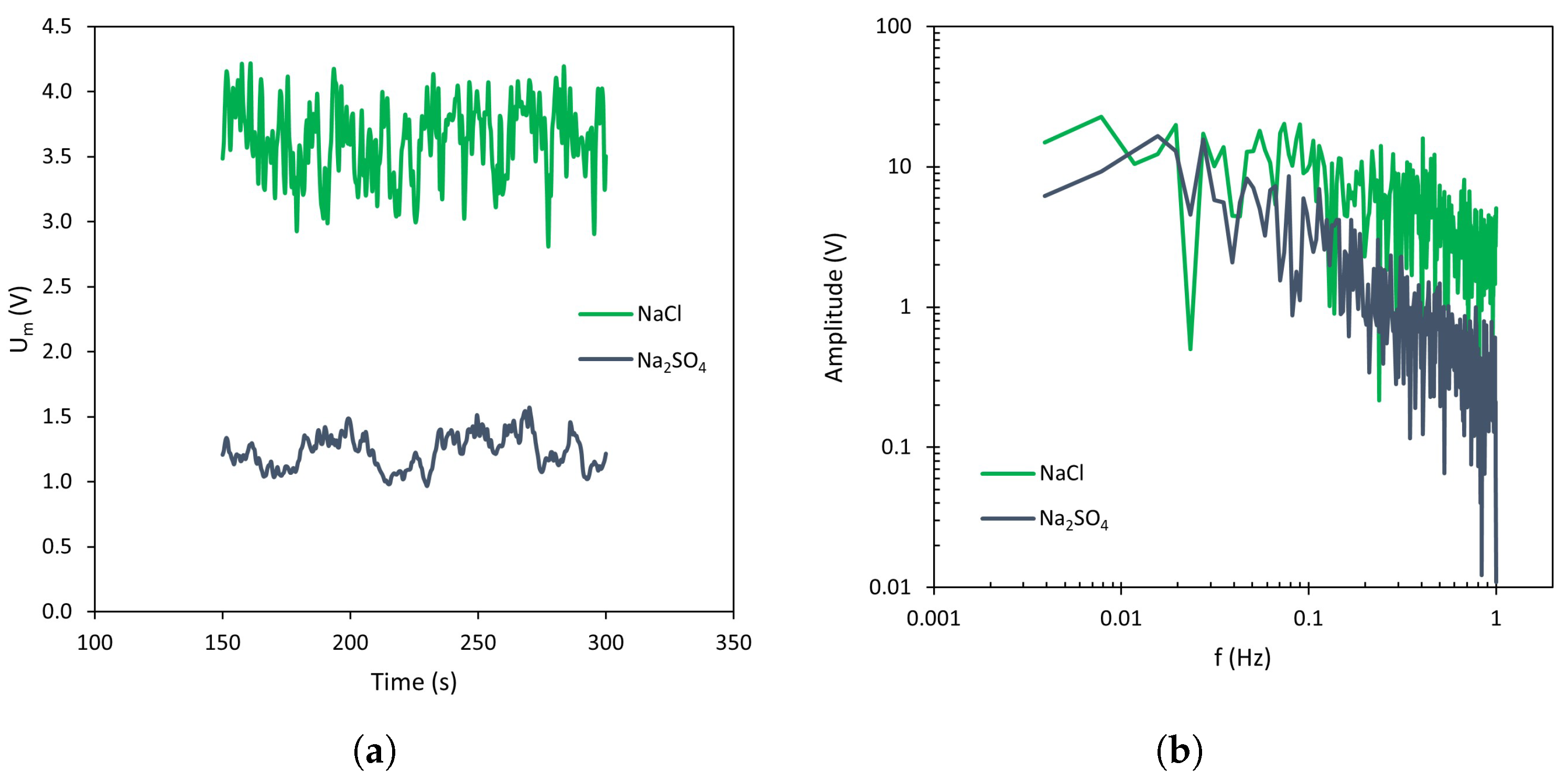

| Membrane | Electrolyte | Stirring Rate | Reynolds Number |
|---|---|---|---|
| AMV-N | NaCl | 0 rpm | 0 |
| AMV-N | NaCl | 200 rpm | 1498 |
| AMV-N | NaCl | 400 rpm | 2995 |
| AMV-N | NaCl | 600 rpm | 4493 |
| HC-A | NaCl | 0 rpm | 0 |
| HC-A | NaCl | 200 rpm | 1498 |
| HC-A | NaCl | 400 rpm | 2995 |
| HC-A | NaCl | 600 rpm | 4493 |
| Membrane | Electrolyte | Stirring Rate | Reynolds Number |
|---|---|---|---|
| AMV-N | NaCl | 400 rpm | 2995 |
| AMV-N | NaSO | 400 rpm | 2971 |
| HC-A | NaCl | 400 rpm | 2995 |
| HC-A | NaSO | 400 rpm | 2971 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hernández-Pérez, L.; Martí-Calatayud, M.C.; Montañés, M.T.; Pérez-Herranz, V. Interplay between Forced Convection and Electroconvection during the Overlimiting Ion Transport through Anion-Exchange Membranes: A Fourier Transform Analysis of Membrane Voltage Drops. Membranes 2023, 13, 363. https://doi.org/10.3390/membranes13030363
Hernández-Pérez L, Martí-Calatayud MC, Montañés MT, Pérez-Herranz V. Interplay between Forced Convection and Electroconvection during the Overlimiting Ion Transport through Anion-Exchange Membranes: A Fourier Transform Analysis of Membrane Voltage Drops. Membranes. 2023; 13(3):363. https://doi.org/10.3390/membranes13030363
Chicago/Turabian StyleHernández-Pérez, Lorena, Manuel César Martí-Calatayud, Maria Teresa Montañés, and Valentín Pérez-Herranz. 2023. "Interplay between Forced Convection and Electroconvection during the Overlimiting Ion Transport through Anion-Exchange Membranes: A Fourier Transform Analysis of Membrane Voltage Drops" Membranes 13, no. 3: 363. https://doi.org/10.3390/membranes13030363
APA StyleHernández-Pérez, L., Martí-Calatayud, M. C., Montañés, M. T., & Pérez-Herranz, V. (2023). Interplay between Forced Convection and Electroconvection during the Overlimiting Ion Transport through Anion-Exchange Membranes: A Fourier Transform Analysis of Membrane Voltage Drops. Membranes, 13(3), 363. https://doi.org/10.3390/membranes13030363








